Lidar-Based Morphometry of Conical Hills in Temperate Karst Areas in Slovenia
Abstract
1. Introduction
2. Materials and Methods
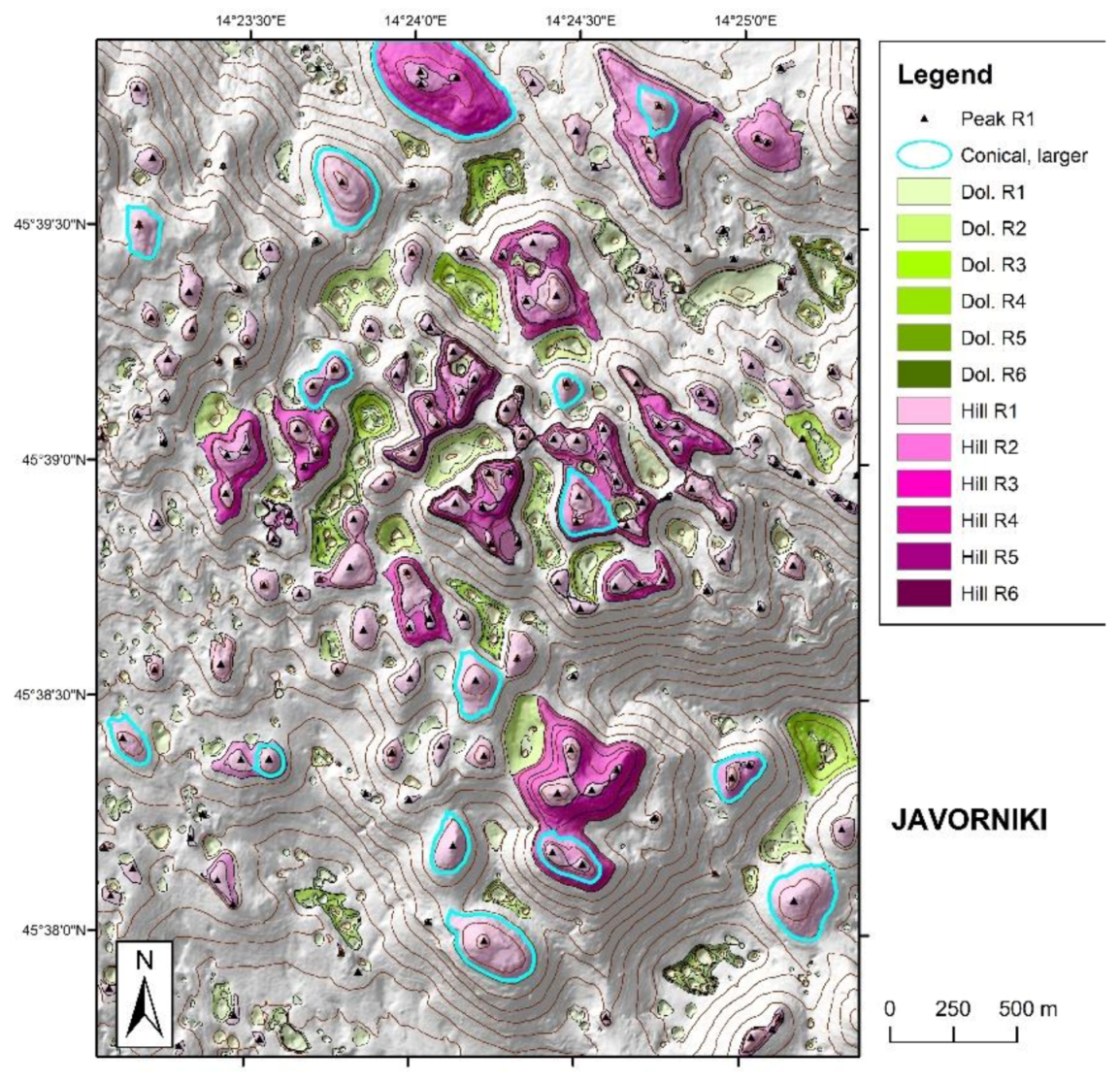
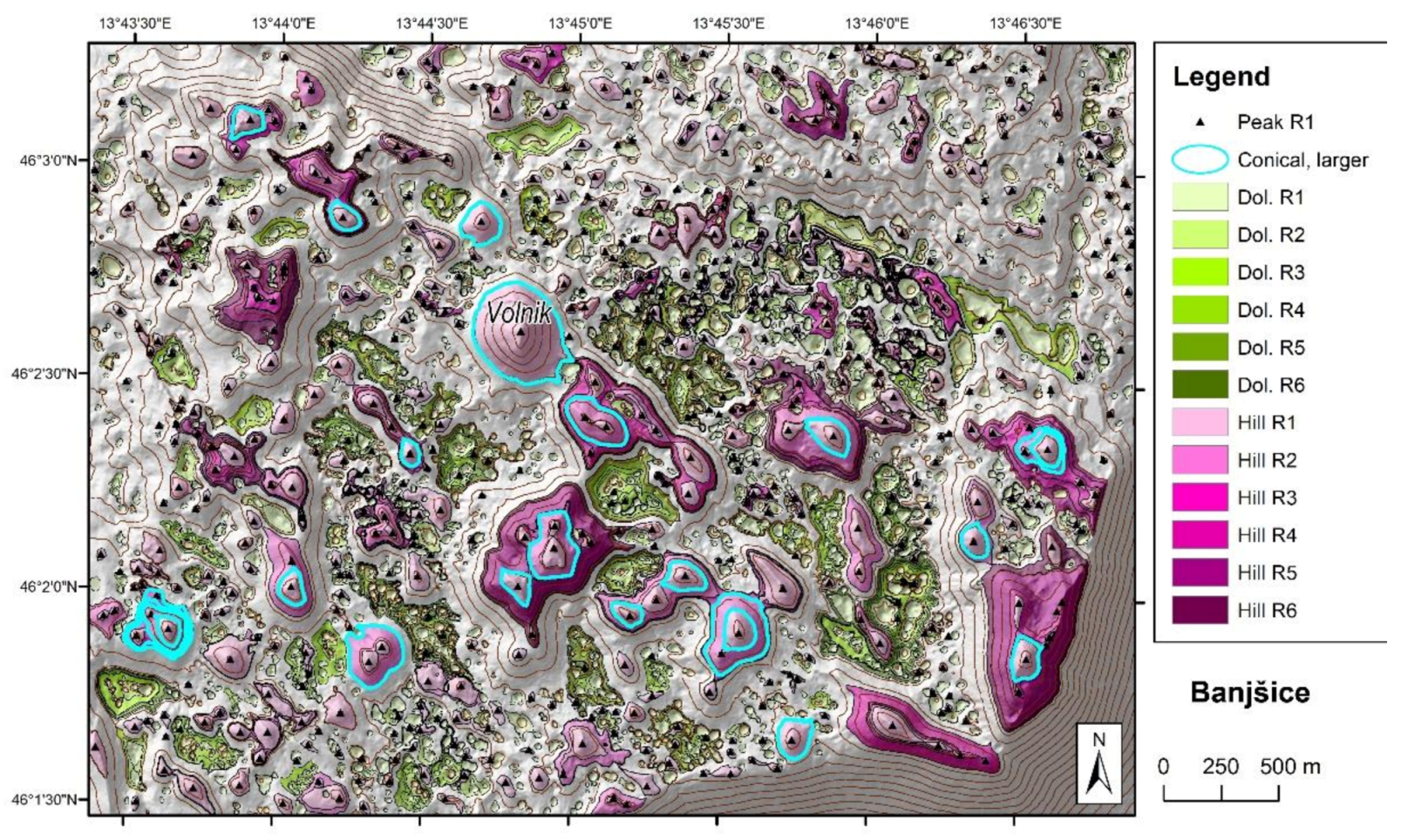
2.1. Study Areas
2.2. Base Data
2.3. Method
3. Results
3.1. Density and Area Ratio
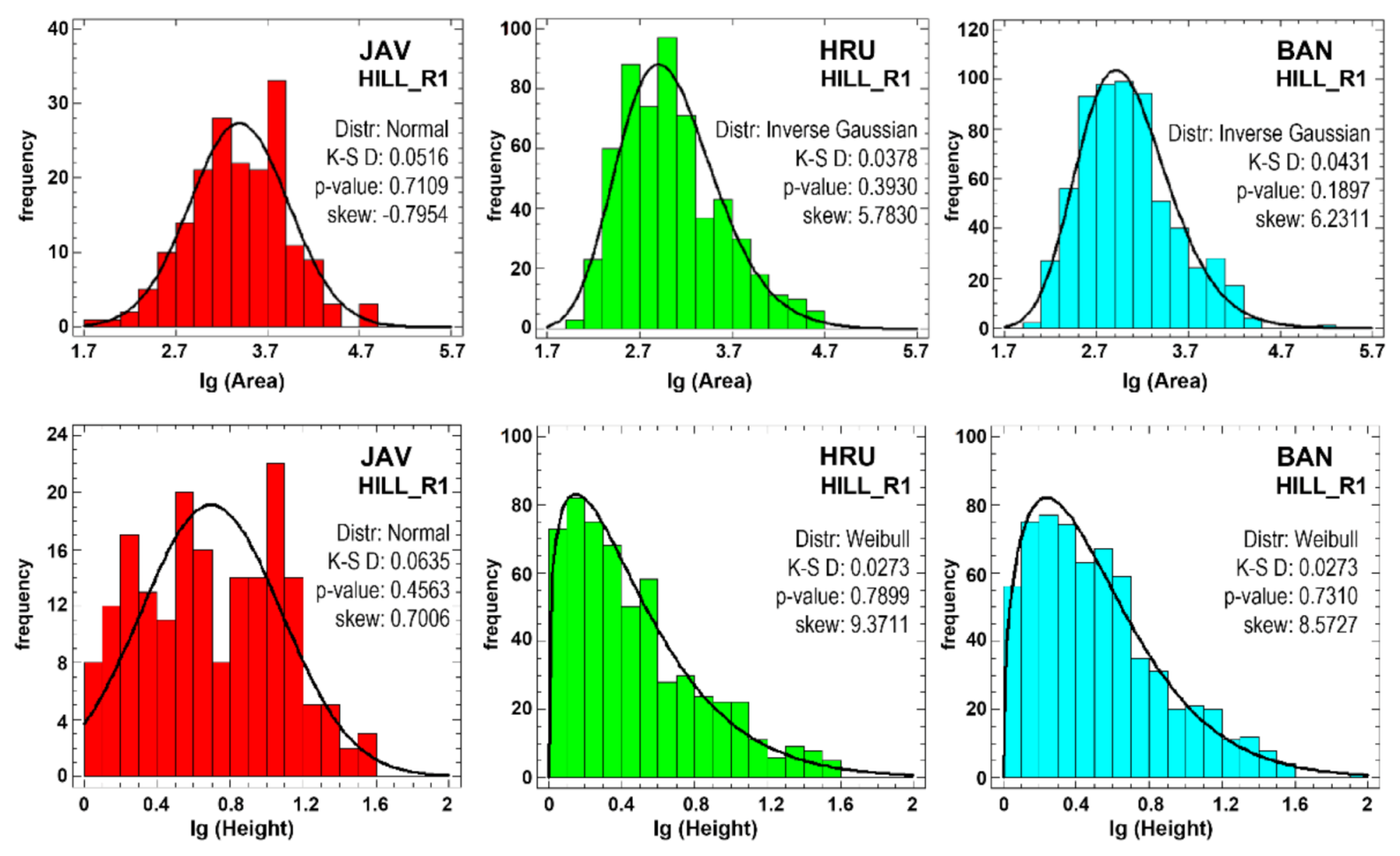
3.2. Size Parameters
3.3. Dimensionless Parameters
3.4. Peaks and Sinks in Higher Rank Forms
4. Discussion
- Theoretically, the two methods should result the same set of forms, i.e., they should, in principle, delineate the nested dolines and hills in the same way. The outlines of the forms are linked to a given elevation level by each method, so neither method solves the problem of complex delineation mentioned in the Introduction. Naturally, in further morphometric calculations, there are certain differences between the methods;
- In practice, the contour interval determines the accuracy of the delineation in case of the vector-based method. If contour lines are calculated based on a high-resolution DEM, then high accuracy can also be achieved with the vector method;
- With GIS software containing hydrological tools, the algorithm used in this article can be relatively easily implemented (although its application can be significantly accelerated by programming). The contour-based method (to our knowledge) requires the development of several stand-alone GIS tools but, once developed, they may be more efficient than the raster-based algorithm. In the absence of detailed information and analysis, this is so far only a hypothesis.
- addition of more morphometric indicators to the analyses that were not included in this paper (e.g., hill orientation, symmetry conditions, etc.);
- comparison of different study areas. One may even think of comparing karstic and non-karstic areas; but primarily the comparison of residual hills occurring in karst areas with different climates, different geological and topographic conditions using the same methodology can be an important goal.
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ford, D.; Williams, P.D. Karst Hydrogeology and Geomorphology; John Wiley & Sons: Chichester, West Sussex, UK, 2007. [Google Scholar]
- Day, M. Morphology and distribution of residual limestone hill in the karst of northern Puerto Rico. Bull. Geol. Soc. Am. 1978, 89, 426–432. [Google Scholar] [CrossRef]
- Ford, D. Jovan Cvijić and the founding of karst geomorphology. Environ. Geol. 2007, 51, 675–684. [Google Scholar] [CrossRef]
- Williams, P.W. Illustrating morphometric analysis of karst with examples from New Guinea. Z. Für Geomorphol. 1971, 15, 40–61. [Google Scholar]
- Troester, J.W.; White, E.L.; White, W.B. A comparison of sinkhole depth frequency distributions in temperate and tropic karst regions. In Proceedings of the 1st Multidisciplinary Conference on Sinkholes, Orlando, FL, USA, 15–17 October 1984; pp. 65–73. [Google Scholar]
- Kemmerly, P.R. Spatial analysis of a karst depression population: Clues to genesis. GSA Bull. 1982, 93, 1078–1086. [Google Scholar] [CrossRef]
- Kemmerly, P.R. Exploring a contagion model for karst-terrane evolution. GSA Bull. 1986, 97, 619–625. [Google Scholar] [CrossRef]
- Faivre, S.; Reiffsteck, P. from doline distribution to tectonics movements example of the Velebit mountain range, Croatia. Acta Carsologica 2002, 31, 139–154. [Google Scholar] [CrossRef]
- Pahernik, M. Spatial density of dolines in the Croatian territory. Hrvat. Geogr. Glas. 2012, 74, 5–26. [Google Scholar] [CrossRef]
- Telbisz, T. Új megközelítések a töbör-morfológiában az Aggteleki-karszt példáján (New perspectives in doline-morphometry—Aggtelek karst as an example). Földr. Közl. 2001, 125, 95–108. [Google Scholar]
- Gao, Y.; Alexander, E.C.; Barnes, R.J. Karst database implementation in Minnesota: Analysis of sinkhole distribution. Environ. Geol. 2005, 47, 1083–1098. [Google Scholar] [CrossRef]
- Pardo-Igúzquiza, E.; Dowd, P.A.; Telbisz, T. On the size-distribution of solution dolines in carbonate karst: Lognormal or power model? Geomorphology 2020, 351, 106972. [Google Scholar] [CrossRef]
- Balázs, D. Relief types of tropical karst areas. In Proceedings of the International Geographical Union European Regional Conference Symposium on Karst-Morphogenesis, Budapest, Hungary, 5–9 August 1971; pp. 16–32. [Google Scholar]
- Huang, W.; Deng, C.J.; Day, M. Differentiating tower karst (fenglin) and cockpit karst (fengcong) using DEM contour, slope, and centroid. Environ. Earth Sci. 2014, 72, 407–416. [Google Scholar] [CrossRef]
- Yang, X.; Tang, G.; Meng, X.; Xiong, L. Classification of karst fenglin and fengcong landform units based on spatial relations of terrain feature points from DEMs. Remote Sens. 2019, 11, 1950. [Google Scholar] [CrossRef]
- Liang, F.; Xu, B. Discrimination of tower-, cockpit-, and non-karst landforms in Guilin, Southern China, based on morphometric characteristics. Geomorphology 2014, 204, 42–48. [Google Scholar] [CrossRef]
- Lyew-Ayee, P.; Viles, H.A.; Tucker, G.E. The use of GIS-based digital morphometric techniques in the study of cockpit karst. Earth Surf. Process. Landf. 2007, 32, 165–179. [Google Scholar] [CrossRef]
- Day, M. Doline morphology and development in Barbados. Ann. Assoc. Am. Geogr. 1983, 73, 206–219. [Google Scholar] [CrossRef]
- Székely, B.; Telbisz, T.; Koma, Z.; Kelemen, K.; Szmorad, F.; Deák, M.; Látos, T.; Standovár, T. Geomorphometric correlations of vegetation cover properties and topographic karst features based on high-resolution LiDAR DTM of Aggtelek Karst, NE Hungary. In Proceedings of the EGU General Assembly Conference Abstracts, Vienna, Austria, 12–17 April 2015; Volume 17. [Google Scholar]
- De Carvalho, O.A.; Guimarães, R.F.; Montgomery, D.R.; Gillespie, A.R.; Trancoso Gomes, R.A.; De Souza Martins, É.; Silva, N.C. Karst depression detection using ASTER, ALOS/PRISM and SRTM-derived digital elevation models in the Bambuí group, Brazil. Remote Sens. 2014, 6, 330–351. [Google Scholar] [CrossRef]
- Orndorff, R.C.; Weary, D.J.; Lagueux, K.M. Geographic information systems analysis of geologic controls on the distribution on dolines in the Ozarks of South-Central Missouri, USA. Acta Carsologica 2000, 29, 161–175. [Google Scholar] [CrossRef]
- Denizman, C.A.N. Morphometric and spatial distribution parameters of karstic depressions, Lower Suwannee River Basin, Florida. J. Cave Karst Stud. 2003, 65, 29–35. [Google Scholar]
- Angel, J.C.; Nelson, D.O.; Panno, S.V. Comparison of a new GIS-based technique and a manual method for determining sinkhole density: An example from Illinois’ sinkhole plain. J. Cave Karst Stud. 2004, 66, 9–17. [Google Scholar]
- Telbisz, T.; Mari, L.; Ćalić, J. Doline morphometry of Mt. Miroč using GIS methods. Glas. Srp. Geogr. Drustva 2007, 87, 21–30. [Google Scholar] [CrossRef]
- Čeru, T.; Šegina, E.; Gosar, A. Geomorphological dating of pleistocene conglomerates in central Slovenia based on spatial analyses of dolines using LiDAR and ground penetrating radar. Remote Sens. 2017, 9, 1213. [Google Scholar] [CrossRef]
- Hofierka, J.; Gallay, M.; Bandura, P.; Šašak, J. Identification of karst sinkholes in a forested karst landscape using airborne laser scanning data and water flow analysis. Geomorphology 2018, 308, 265–277. [Google Scholar] [CrossRef]
- Šegina, E.; Benac, Č.; Rubinić, J.; Knez, M. Morphometric Analyses of dolines—The problem of delineation and calculation of basic parameters. Acta Carsologica 2018, 47. [Google Scholar] [CrossRef]
- Obu, J.; Podobnikar, T. Algoritem za prepoznavanje kraških kotanj na podlagi digitalnega modela reliefa (Algorithm for karst depression recognition using digital terrain model). Geod. Vestn. 2013, 57, 260–270. [Google Scholar] [CrossRef]
- Bauer, C. Analysis of dolines using multiple methods applied to airborne laser scanning data. Geomorphology 2015, 250, 78–88. [Google Scholar] [CrossRef]
- Kobal, M.; Bertoncelj, I.; Pirotti, F.; Dakskobler, I.; Kutnar, L. Using Lidar data to analyse sinkhole characteristics relevant for understory vegetation under forest cover—Case study of a high karst area in the dinaric mountains. PLoS ONE 2015, 10, e0122070. [Google Scholar] [CrossRef]
- Telbisz, T.; Látos, T.; Deák, M.; Székely, B.; Koma, Z.; Standovár, T. The advantage of lidar digital terrain models in doline morphometry compared to topographic map based datasets—Aggtelek karst (Hungary) as an example. Acta Carsologica 2016, 45, 5–18. [Google Scholar] [CrossRef]
- Öztürk, M.Z.; Şimşek, M.; Şener, M.F.; Utlu, M. GIS based analysis of doline density on Taurus Mountains, Turkey. Environ. Earth Sci. 2018, 77, 536. [Google Scholar] [CrossRef]
- Wu, Q.; Deng, C.; Chen, Z. Automated delineation of karst sinkholes from LiDAR-derived digital elevation models. Geomorphology 2016, 266, 1–10. [Google Scholar] [CrossRef]
- Stepišnik, U.; Ilc Klun, M.; Repe, B. Assessment of educational potential of geodiversity on example of Cerknica Polje, Slovenia. Dela 2017, 47, 5–39. [Google Scholar] [CrossRef][Green Version]
- Mihevc, A.; Prelovšek, M.; Zupan Hajna, N. (Eds.) Introduction to the Dinaric Karst; Karst Research Institute at ZRC SAZU: Postojna, Slovenia, 2010. [Google Scholar]
- Žebre, M.; Stepišnik, U. Glaciokarst geomorphology of the northern Dinaric Alps: Snežnik (Slovenia) and Gorski Kotar (Croatia). J. Maps 2016, 12, 873–881. [Google Scholar] [CrossRef]
- Bočić, N.; Pahernik, M.; Bognar, A. Geomorphological characteristics of the Slunj karst plateau. Hrvat. Geogr. Glas. 2010, 72, 24–25. [Google Scholar] [CrossRef]
- Gutiérrez, F.; Gutiérrez, M. Karst landforms. In Landforms of the Earth: An Illustrated Guide; Gutiérrez, F., Gutiérrez, M., Eds.; Springer International Publishing: Cham, Switzerland, 2016; pp. 59–101. ISBN 978-3-319-26947-4. [Google Scholar]
- Cucchi, F.; Finocchiaro, F. Karst landforms in Friuli Venezia Giulia: From Alpine to coastal karst. In Landscapes and Landforms of Italy; Soldati, M., Marchetti, M., Eds.; World Geomorphological Landscapes; Springer International Publishing: Cham, Switzerland, 2017; pp. 147–156. ISBN 978-3-319-26194-2. [Google Scholar]
- Jakál, J. Porovnávacia analýza krasových planín Západných Karpát (Comparative analysis of karst plateaux in the Western Carpathians). Geogr. Cas. 2001, 53, 3–21. [Google Scholar]
- Şener, M.; Öztürk, M. Relict drainage effects on distribution and morphometry of karst depressions: A casestudy from Central Taurus (Turkey). J. Cave Karst Stud. 2019, 81, 33–43. [Google Scholar] [CrossRef]
- Gunn, J. (Ed.) Encyclopedia of Caves and Karst Science; Taylor & Francis Books: Oxfordshire, UK, 2004. [Google Scholar]
- Šikić, D.; Pleničar, M.; Šparica, M. Osnovna Geološka Karta SFRJ, List Ilirska Bistrica, 1:100,000; Savezni Geološki Zavod: Belgrade, Serbia, 1972. [Google Scholar]
- Buser, S.; Grad, K.; Pleničar, M. Osnovna Geološka Karta SFRJ, List Postojna, 1:100,000; Savezni Geološki Zavod: Belgrade, Serbia, 1967. [Google Scholar]
- Buser, S. Osnovna Geološka Karta SFRJ, List Tolmin in Videm, 1:100,000; Savezni Geološki Zavod: Belgrade, Serbia, 1987. [Google Scholar]
- Lidar GIS Viewer, Slovenia. Available online: http://gis.arso.gov.si/evode/profile.aspx?id=atlas_voda_Lidar@Arso&culture=en-US (accessed on 28 May 2021).
- Verbovšek, T.; Gabor, L. Morphometric properties of dolines in Matarsko Podolje, SW Slovenia. Environ. Earth Sci. 2019, 78, 396. [Google Scholar] [CrossRef]
- Telbisz, T.; Mari, L.; Szabó, L. Geomorphological characteristics of the Italian Side of Canin Massif (Julian Alps) using digital terrain analysis and field observations. Acta Carsologica 2011, 40, 255–266. [Google Scholar] [CrossRef]
- Faivre, S.; Pahernik, M. Structural influences on the spatial distribution of dolines, Island of Brac, Croatia. Z. Für Geomorphol. 2007, 487–503. [Google Scholar] [CrossRef]
- Plan, L.; Decker, K. Quantitative karst morphology of the Hochschwab plateau, Eastern Alps, Austria. Z. Für Geomorphol. Suppl. Issues 2006, 147, 29–54. [Google Scholar]
- Bondesan, A.; Meneghel, M.; Sauro, U. Morphometric analysis of dolines. Int. J. Speleol. 1992, 21, 1–55. [Google Scholar] [CrossRef]
- Davis, J.C. Statistics and Data Analysis in Geology, 3rd ed.; JohnWiley & Sons: New York, NY, USA, 2002; ISBN 978-0-471-17275-8. [Google Scholar]



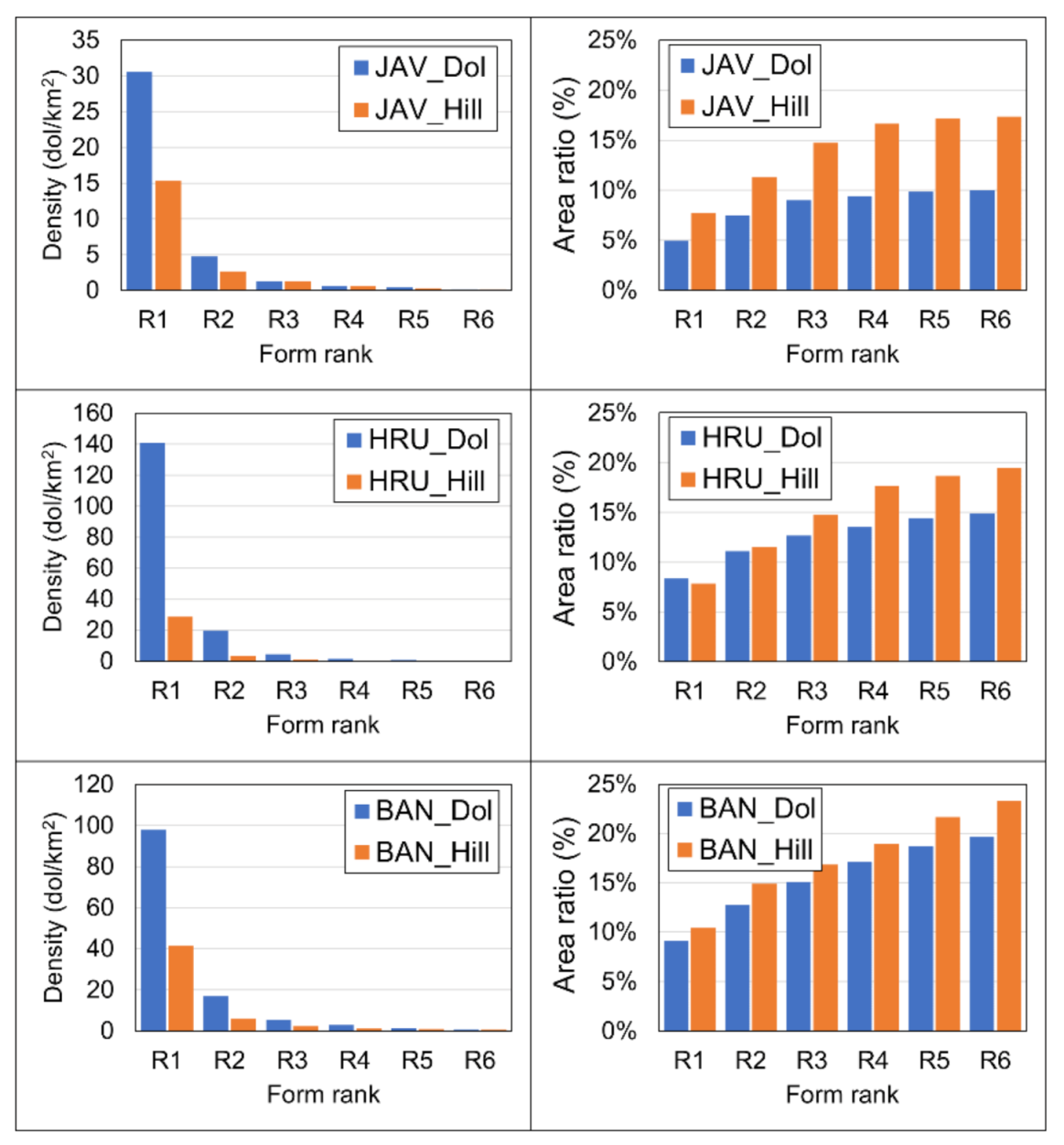
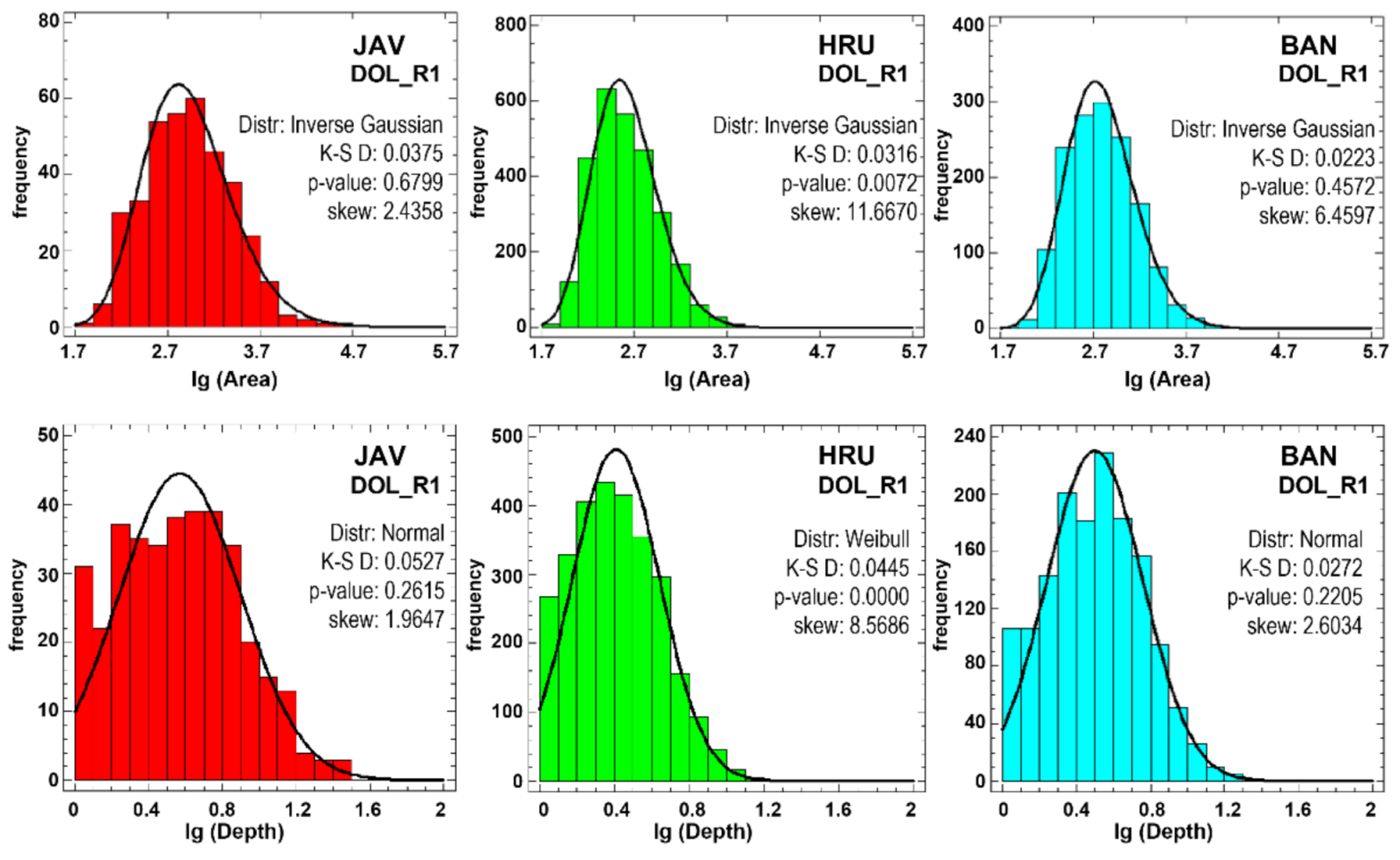


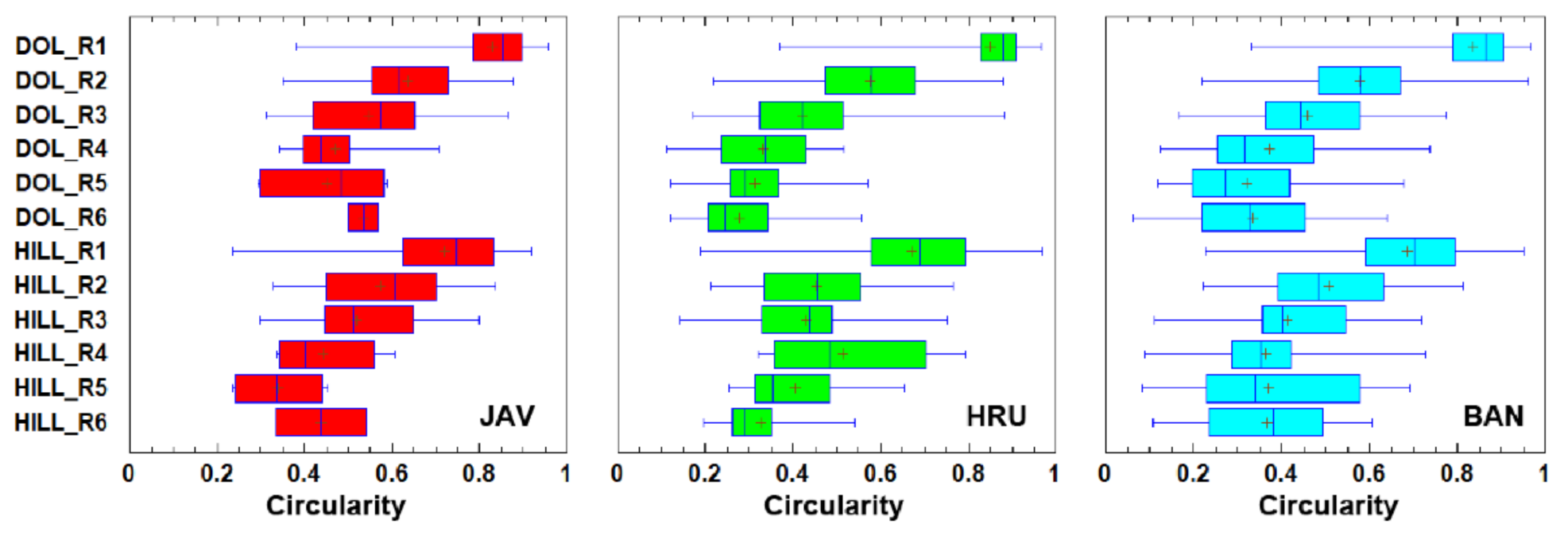

| Site | Form Rank | Count | Area Ratio | Density (km-2) | Planform Area (m2) | Vertical Range (m) | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| Min | Median | Max | Min | Median | Max | |||||
| JAVORNIKI | DOL_R1 | 367 | 5% | 30.6 | 71 | 809 | 41,404 | 1.0 | 3.7 | 30.8 |
| DOL_R2 | 57 | 7% | 4.8 | 452 | 5744 | 53,397 | 1.9 | 9.4 | 35.6 | |
| DOL_R3 | 15 | 9% | 1.3 | 8279 | 22,067 | 57,585 | 9.5 | 16.3 | 37.4 | |
| DOL_R4 | 7 | 9% | 0.6 | 19,846 | 27,239 | 29,484 | 12.4 | 19.2 | 24.6 | |
| DOL_R5 | 5 | 10% | 0.4 | 26,226 | 35,155 | 82,404 | 12.9 | 24.9 | 29.0 | |
| DOL_R6 | 2 | 10% | 0.2 | 35,005 | 40,979 | 46,954 | 17.1 | 22.5 | 27.9 | |
| HILL_R1 | 184 | 8% | 15.3 | 62 | 2574 | 60,898 | 1.0 | 4.7 | 39.8 | |
| HILL_R2 | 32 | 11% | 2.7 | 3628 | 13,751 | 122,984 | 4.3 | 14.9 | 50.6 | |
| HILL_R3 | 15 | 15% | 1.3 | 13,834 | 40,884 | 143,134 | 6.0 | 25.2 | 53.4 | |
| HILL_R4 | 7 | 17% | 0.6 | 49,233 | 72,179 | 212,276 | 20.7 | 29.8 | 51.8 | |
| HILL_R5 | 4 | 17% | 0.3 | 71,553 | 88,116 | 159,686 | 23.7 | 27.1 | 36.1 | |
| HILL_R6 | 2 | 17% | 0.2 | 86,341 | 93,424 | 100,506 | 27.6 | 28.8 | 30.0 | |
| HRUŠICA | DOL_R1 | 2817 | 8% | 140.9 | 51 | 373 | 15,198 | 1.0 | 2.5 | 20.1 |
| DOL_R2 | 395 | 11% | 19.8 | 270 | 2206 | 17,733 | 1.4 | 5.5 | 21.1 | |
| DOL_R3 | 88 | 13% | 4.4 | 1542 | 8195 | 34,172 | 4.2 | 9.4 | 19.2 | |
| DOL_R4 | 31 | 14% | 1.6 | 7713 | 20,755 | 61,738 | 7.0 | 14.0 | 21.1 | |
| DOL_R5 | 15 | 14% | 0.8 | 22,770 | 44,549 | 103,544 | 9.3 | 19.5 | 23.5 | |
| DOL_R6 | 10 | 15% | 0.5 | 29,734 | 64,627 | 128,604 | 10.5 | 20.5 | 25.5 | |
| HILL_R1 | 572 | 8% | 28.6 | 42 | 975 | 36,511 | 1.0 | 2.4 | 39.0 | |
| HILL_R2 | 68 | 12% | 3.4 | 301 | 7158 | 153,884 | 1.7 | 8.7 | 66.3 | |
| HILL_R3 | 20 | 15% | 1.0 | 3636 | 45,364 | 261,077 | 7.0 | 30.9 | 76.6 | |
| HILL_R4 | 11 | 18% | 0.6 | 22,279 | 161,160 | 451,903 | 10.2 | 50.4 | 104.4 | |
| HILL_R5 | 9 | 19% | 0.5 | 50,956 | 192,972 | 493,279 | 23.8 | 52.7 | 108.6 | |
| HILL_R6 | 5 | 20% | 0.3 | 109,180 | 266,729 | 329,912 | 44.9 | 57.2 | 77.4 | |
| BANJŠICE | DOL_R1 | 1494 | 9% | 97.9 | 53 | 590 | 17,982 | 1.0 | 3.2 | 26.2 |
| DOL_R2 | 259 | 13% | 17.0 | 318 | 3242 | 37,062 | 1.9 | 6.8 | 26.3 | |
| DOL_R3 | 81 | 15% | 5.3 | 2427 | 10,474 | 48,129 | 3.9 | 11.7 | 22.1 | |
| DOL_R4 | 43 | 17% | 2.8 | 6726 | 22,887 | 88,279 | 6.2 | 14.8 | 24.4 | |
| DOL_R5 | 21 | 19% | 1.4 | 16,322 | 46,773 | 135,356 | 12.8 | 18.1 | 29.5 | |
| DOL_R6 | 10 | 20% | 0.7 | 35,556 | 66,867 | 248,300 | 16.7 | 22.6 | 31.5 | |
| HILL_R1 | 634 | 10% | 41.5 | 87 | 950 | 133,426 | 1.0 | 2.9 | 98.2 | |
| HILL_R2 | 90 | 15% | 5.9 | 219 | 5854 | 76,199 | 1.5 | 8.2 | 54.4 | |
| HILL_R3 | 35 | 17% | 2.3 | 2918 | 23,432 | 104,132 | 4.2 | 18.8 | 57.3 | |
| HILL_R4 | 17 | 19% | 1.1 | 12,423 | 55,430 | 204,890 | 10.4 | 37.2 | 63.1 | |
| HILL_R5 | 14 | 22% | 0.9 | 34,892 | 68,219 | 214,706 | 11.7 | 45.3 | 93.3 | |
| HILL_R6 | 9 | 23% | 0.6 | 65,984 | 104,777 | 327,234 | 17.3 | 55.9 | 104.2 | |
| Site | Form Rank | Area ~ H | Circularity | H / D | |||||
|---|---|---|---|---|---|---|---|---|---|
| r2 | exponent | Min | Median | Max | Min | Median | Max | ||
| JAVORNIKI | DOL_R1 | 0.73 | 0.60 | 0.38 | 0.85 | 0.96 | 0.04 | 0.12 | 0.75 |
| DOL_R2 | 0.68 | 0.52 | 0.35 | 0.61 | 0.88 | 0.04 | 0.11 | 0.34 | |
| DOL_R3 | 0.59 | 0.51 | 0.31 | 0.57 | 0.86 | 0.07 | 0.11 | 0.16 | |
| DOL_R4 | 0.31 | 1.05 | 0.34 | 0.44 | 0.71 | 0.07 | 0.10 | 0.13 | |
| DOL_R5 | 0.58 | 0.62 | 0.29 | 0.48 | 0.59 | 0.06 | 0.09 | 0.12 | |
| DOL_R6 | 0.50 | 0.53 | 0.57 | 0.08 | 0.10 | 0.11 | |||
| HILL_R1 | 0.79 | 0.64 | 0.24 | 0.75 | 0.92 | 0.03 | 0.09 | 0.25 | |
| HILL_R2 | 0.72 | 0.58 | 0.33 | 0.61 | 0.84 | 0.05 | 0.11 | 0.18 | |
| HILL_R3 | 0.84 | 0.71 | 0.30 | 0.51 | 0.80 | 0.04 | 0.10 | 0.13 | |
| HILL_R4 | 0.97 | 0.59 | 0.34 | 0.40 | 0.61 | 0.08 | 0.09 | 0.10 | |
| HILL_R5 | 0.83 | 0.49 | 0.24 | 0.34 | 0.45 | 0.07 | 0.08 | 0.09 | |
| HILL_R6 | 0.33 | 0.44 | 0.54 | 0.08 | 0.08 | 0.08 | |||
| HRUŠICA | DOL_R1 | 0.71 | 0.55 | 0.37 | 0.88 | 0.97 | 0.03 | 0.11 | 0.77 |
| DOL_R2 | 0.67 | 0.51 | 0.22 | 0.58 | 0.88 | 0.05 | 0.10 | 0.48 | |
| DOL_R3 | 0.56 | 0.42 | 0.17 | 0.42 | 0.88 | 0.04 | 0.09 | 0.15 | |
| DOL_R4 | 0.24 | 0.34 | 0.11 | 0.34 | 0.52 | 0.04 | 0.08 | 0.13 | |
| DOL_R5 | 0.25 | 0.29 | 0.12 | 0.29 | 0.57 | 0.04 | 0.07 | 0.11 | |
| DOL_R6 | 0.22 | 0.25 | 0.12 | 0.24 | 0.56 | 0.04 | 0.07 | 0.09 | |
| HILL_R1 | 0.81 | 0.60 | 0.19 | 0.69 | 0.97 | 0.03 | 0.08 | 0.22 | |
| HILL_R2 | 0.88 | 0.69 | 0.21 | 0.46 | 0.77 | 0.04 | 0.10 | 0.18 | |
| HILL_R3 | 0.86 | 0.66 | 0.14 | 0.44 | 0.75 | 0.05 | 0.11 | 0.15 | |
| HILL_R4 | 0.87 | 0.67 | 0.32 | 0.48 | 0.79 | 0.06 | 0.14 | 0.15 | |
| HILL_R5 | 0.86 | 0.61 | 0.25 | 0.35 | 0.65 | 0.08 | 0.13 | 0.15 | |
| HILL_R6 | 0.58 | 0.33 | 0.20 | 0.29 | 0.54 | 0.10 | 0.11 | 0.15 | |
| BANJŠICE | DOL_R1 | 0.71 | 0.58 | 0.33 | 0.87 | 0.97 | 0.02 | 0.12 | 0.43 |
| DOL_R2 | 0.64 | 0.49 | 0.22 | 0.58 | 0.96 | 0.04 | 0.11 | 0.27 | |
| DOL_R3 | 0.46 | 0.38 | 0.17 | 0.44 | 0.77 | 0.05 | 0.10 | 0.19 | |
| DOL_R4 | 0.32 | 0.31 | 0.13 | 0.32 | 0.74 | 0.05 | 0.08 | 0.17 | |
| DOL_R5 | 0.15 | 0.16 | 0.12 | 0.27 | 0.68 | 0.05 | 0.07 | 0.13 | |
| DOL_R6 | 0.02 | 0.05 | 0.06 | 0.33 | 0.64 | 0.04 | 0.07 | 0.13 | |
| HILL_R1 | 0.85 | 0.65 | 0.23 | 0.70 | 0.95 | 0.03 | 0.09 | 0.24 | |
| HILL_R2 | 0.89 | 0.70 | 0.22 | 0.48 | 0.81 | 0.05 | 0.10 | 0.23 | |
| HILL_R3 | 0.87 | 0.76 | 0.11 | 0.40 | 0.72 | 0.06 | 0.10 | 0.22 | |
| HILL_R4 | 0.82 | 0.73 | 0.09 | 0.35 | 0.73 | 0.06 | 0.12 | 0.20 | |
| HILL_R5 | 0.72 | 0.86 | 0.08 | 0.34 | 0.69 | 0.06 | 0.11 | 0.20 | |
| HILL_R6 | 0.67 | 0.84 | 0.11 | 0.38 | 0.61 | 0.06 | 0.15 | 0.19 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Telbisz, T. Lidar-Based Morphometry of Conical Hills in Temperate Karst Areas in Slovenia. Remote Sens. 2021, 13, 2668. https://doi.org/10.3390/rs13142668
Telbisz T. Lidar-Based Morphometry of Conical Hills in Temperate Karst Areas in Slovenia. Remote Sensing. 2021; 13(14):2668. https://doi.org/10.3390/rs13142668
Chicago/Turabian StyleTelbisz, Tamás. 2021. "Lidar-Based Morphometry of Conical Hills in Temperate Karst Areas in Slovenia" Remote Sensing 13, no. 14: 2668. https://doi.org/10.3390/rs13142668
APA StyleTelbisz, T. (2021). Lidar-Based Morphometry of Conical Hills in Temperate Karst Areas in Slovenia. Remote Sensing, 13(14), 2668. https://doi.org/10.3390/rs13142668







