Azimuth Resolution Improvement and Target Parameters Inversion for Distributed Shipborne High Frequency Hybrid Sky-Surface Wave Radar
Abstract
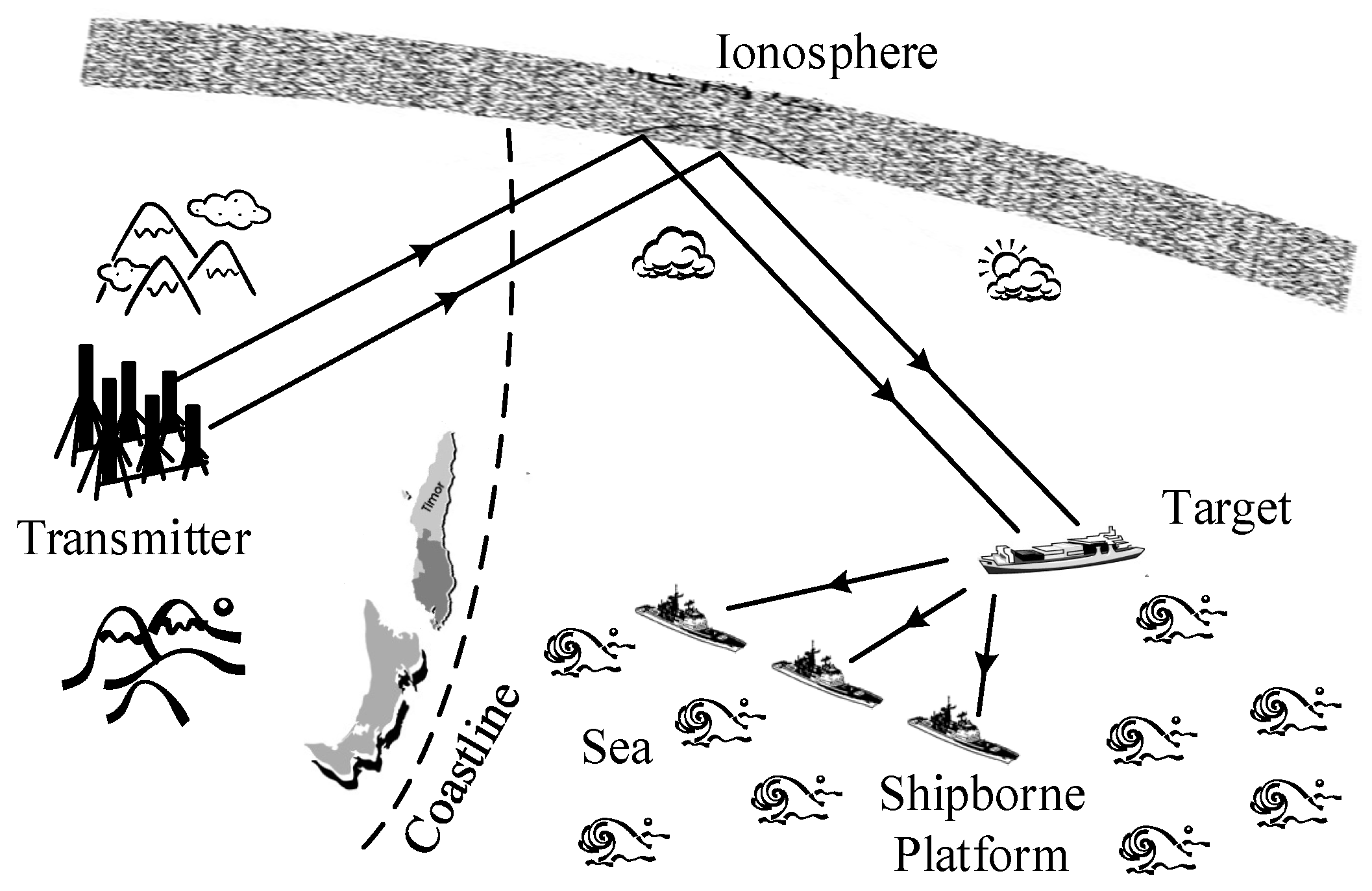
:1. Introduction
2. Azimuth Resolution Improvement of the Moving Target via Spectral Synthesis
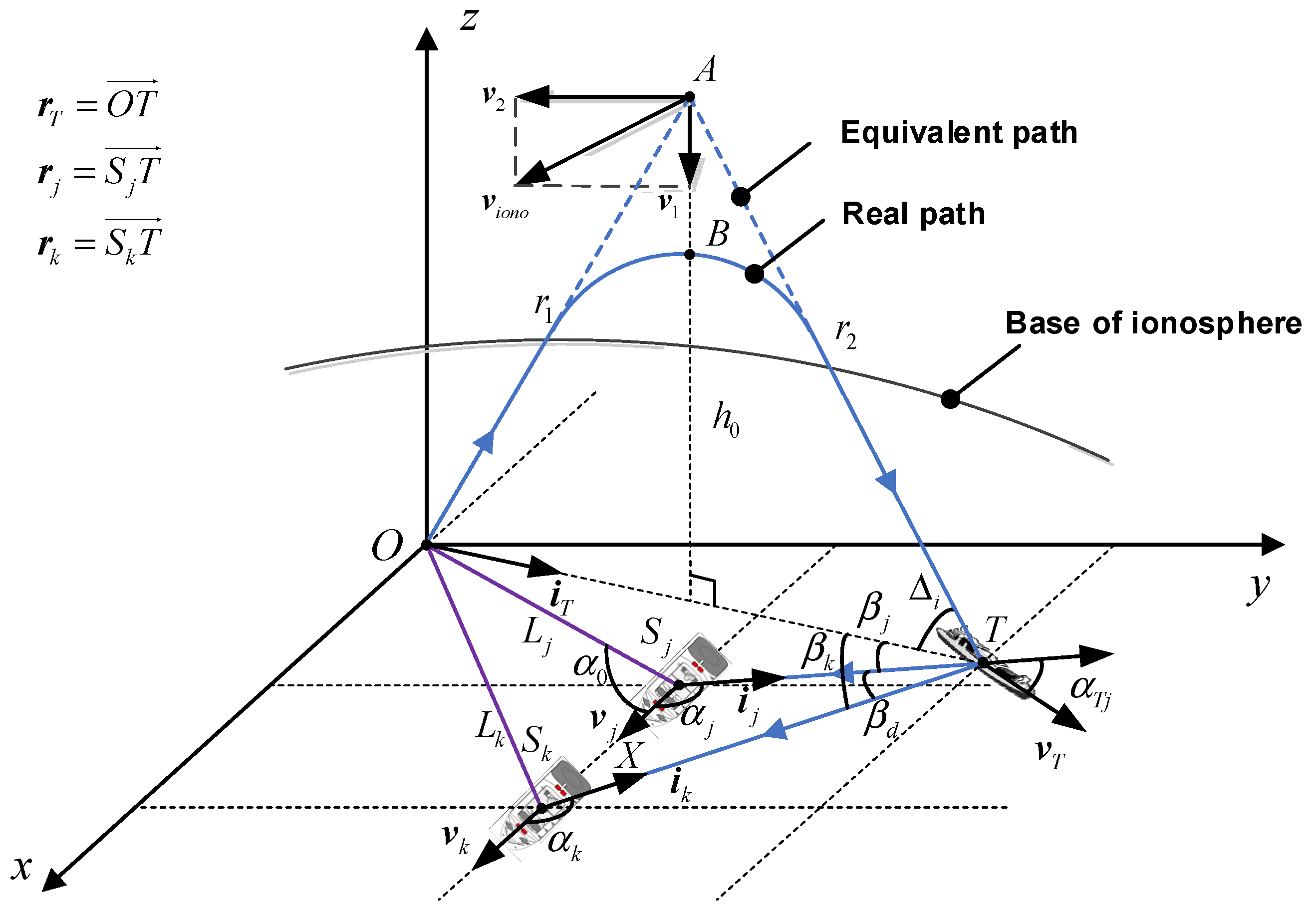
2.1. Signal Model
- : half the length of the sky-wave path, which is equal to ;
- : position vector from O to T, where is the magnitude of ;
- : virtual reflection height;
- : velocity vector of the target T (with magnitude );
- : velocity vector of the shipborne platform (with magnitude );
- : velocity vector of the ionospheric reflector, which can be decomposed into the vertical component and the horizontal component .
- : position vector from to T, where is the magnitude of , is the unit vector in the direction of ;
- : clockwise rotation angle from to , i.e., bistatic angle;
- : clockwise rotation angle from to ;
- : ground distance between the transmitter O and the receiver , i.e., baseline length;
- : clockwise rotation angle from to the position vector from to O;
- : clockwise rotation angle from to ,which denotes the target direction;
- : clockwise rotation angle from to ;
- : grazing angle.
2.2. Improvement of the Azimuth Resolution
- (1)
- The aperture time to obtain the maximum synthetic aperture length.
- (2)
- The inverse relation between the azimuth resolution and the azimuth bandwidth.
- (3)
- The azimuth resolution improvement principle.
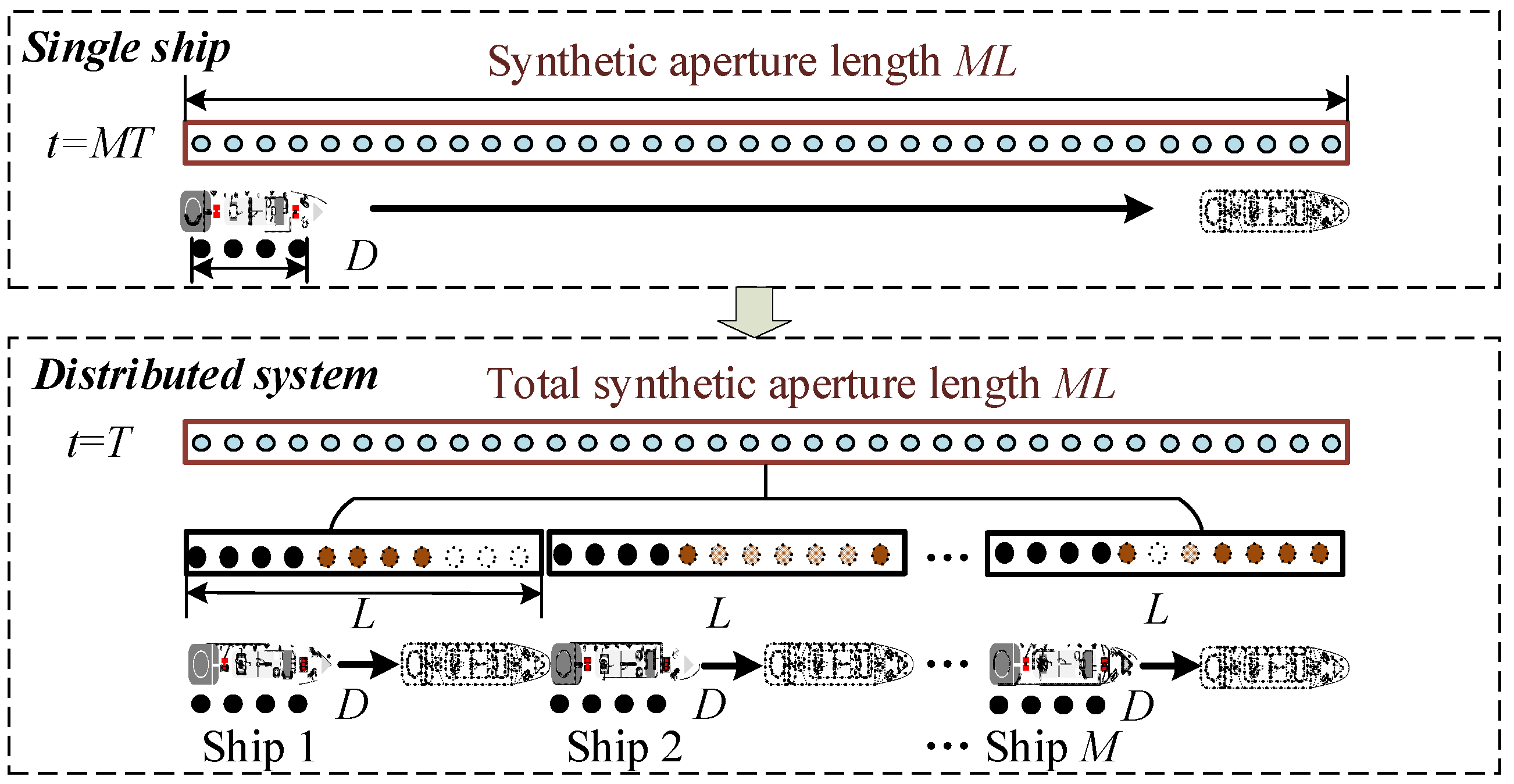
3. Azimuth Resolution Improvement Algorithm for the Distributed Shipborne HFHSSWR
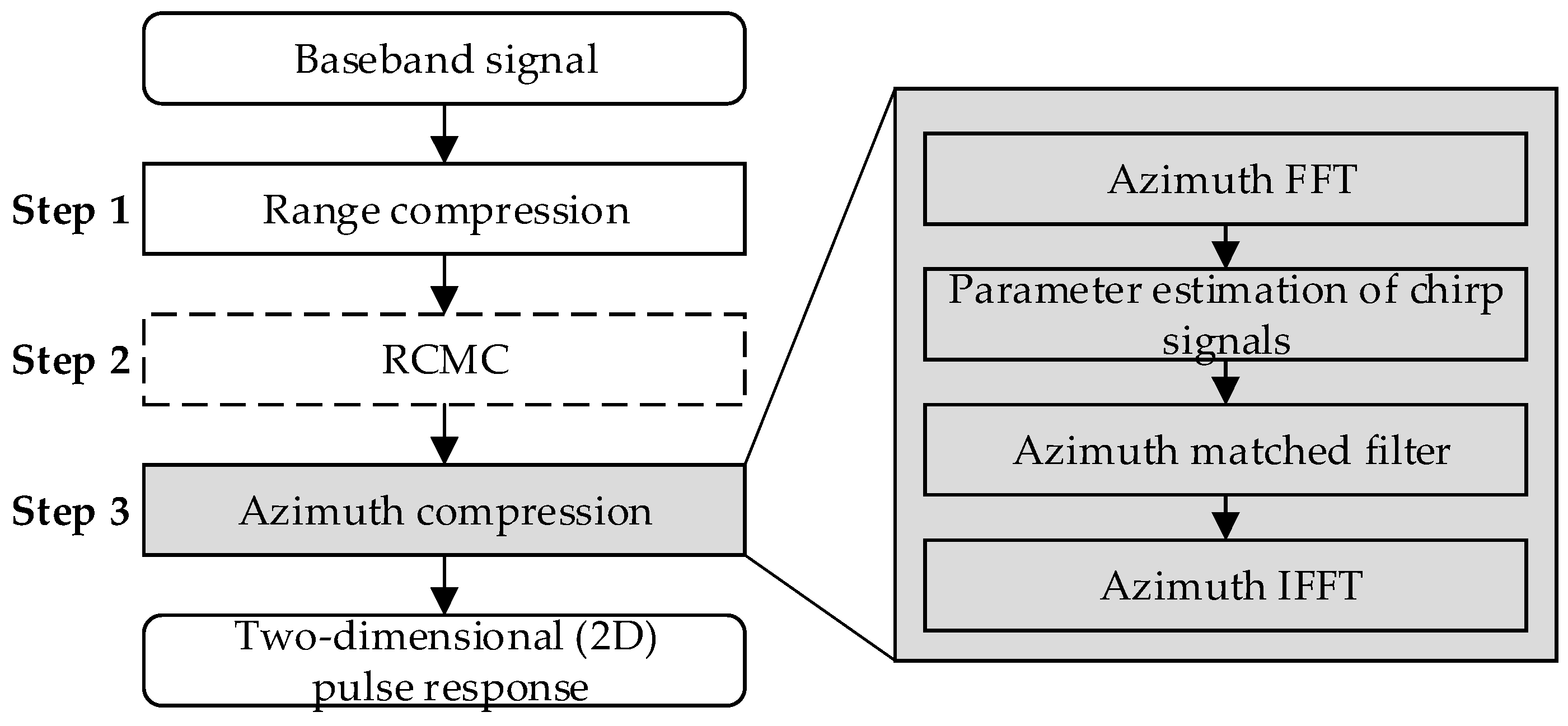
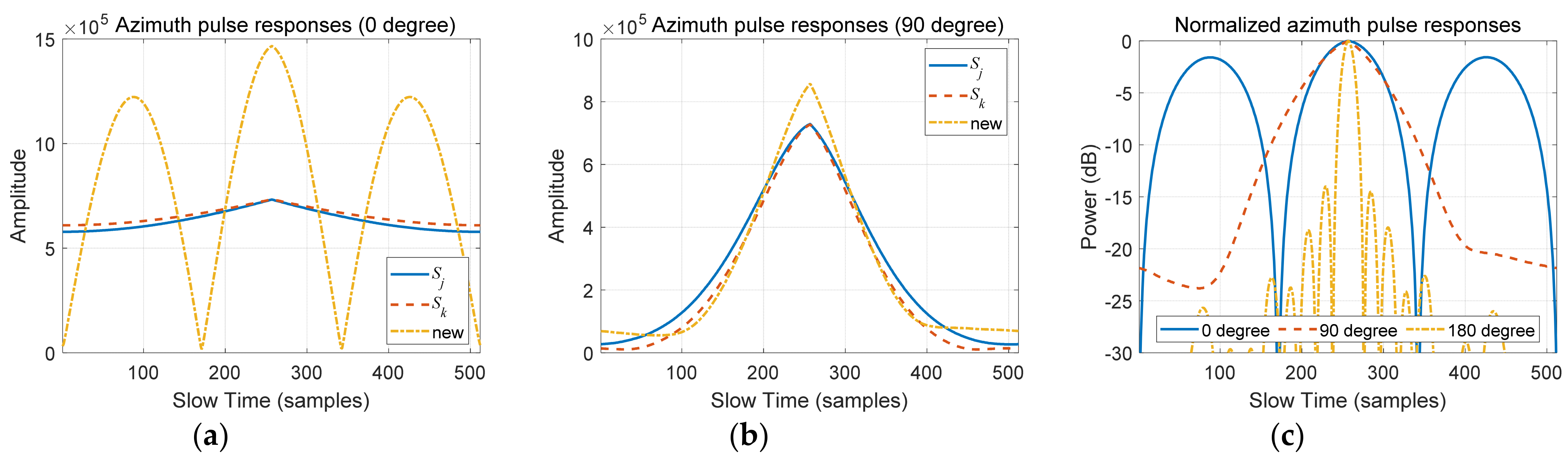
3.1. Focused Processing of the Moving Target Echo for a Single Shipborne HFHSSWR
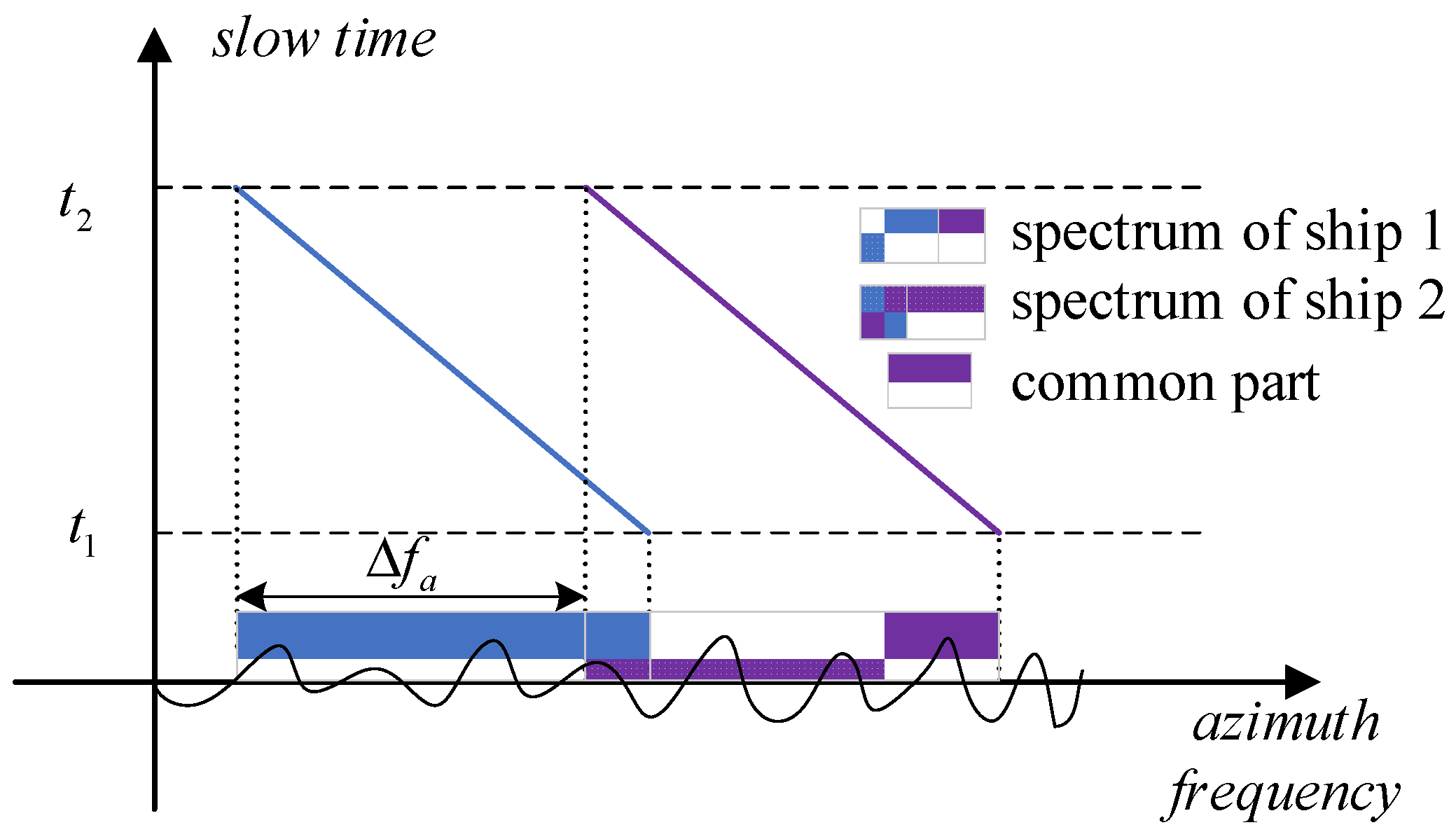
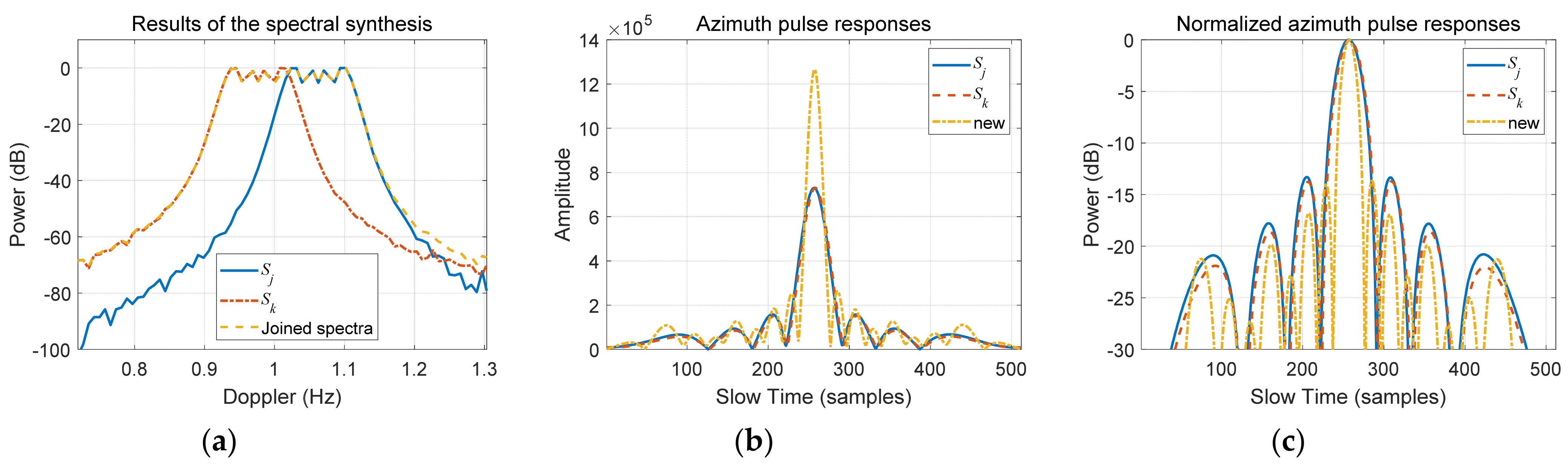
3.2. Distributed Synthetic Aperture Processing
4. Target Velocity Vector and Azimuth Parameters Inversion
4.1. Target Parameters Inversion Method
4.1.1. Target Parameters Inversion Based on the Echo Signals from Two Platforms
4.1.2. Target Parameters Inversion Based on the Echo Signals from Three Platforms
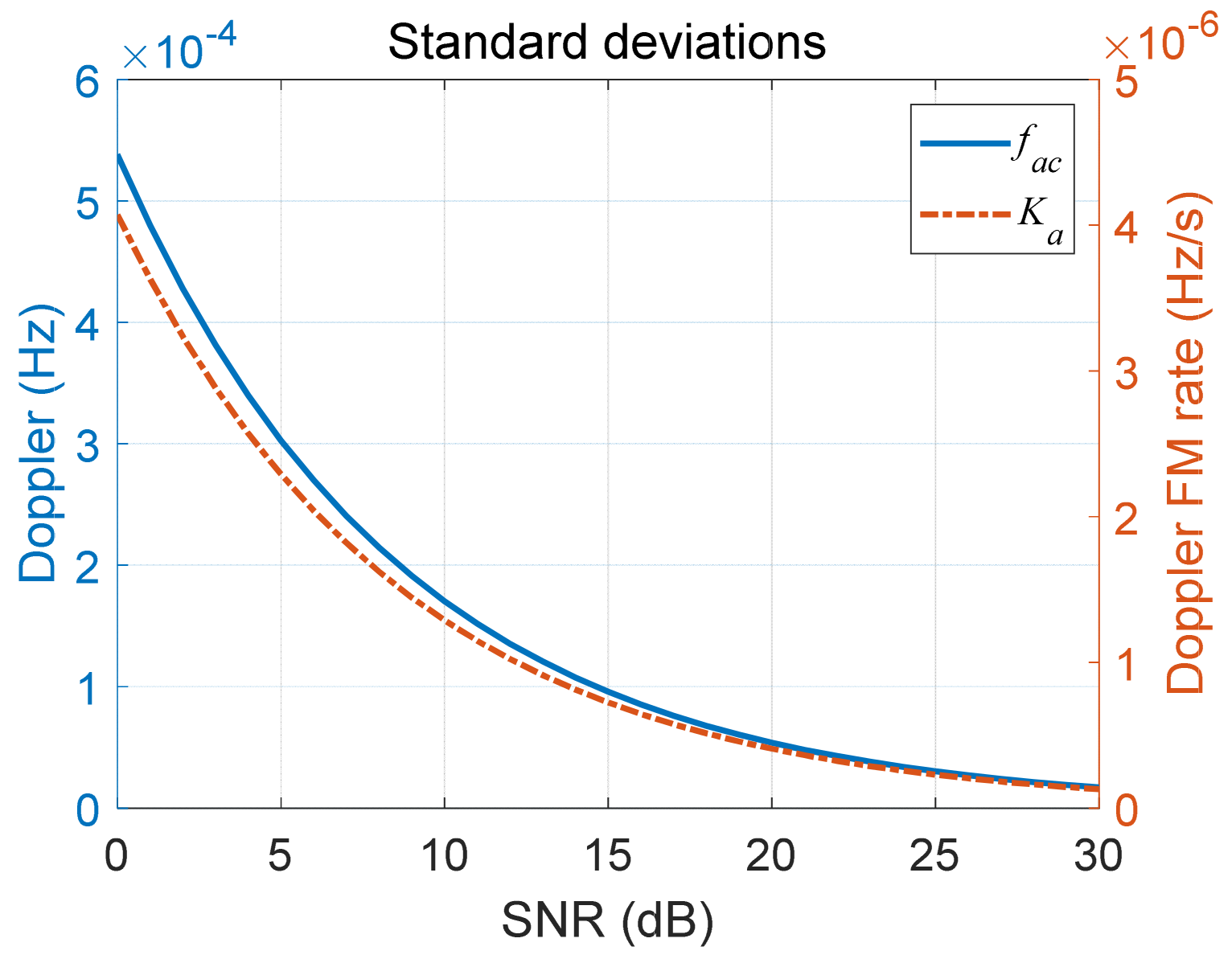
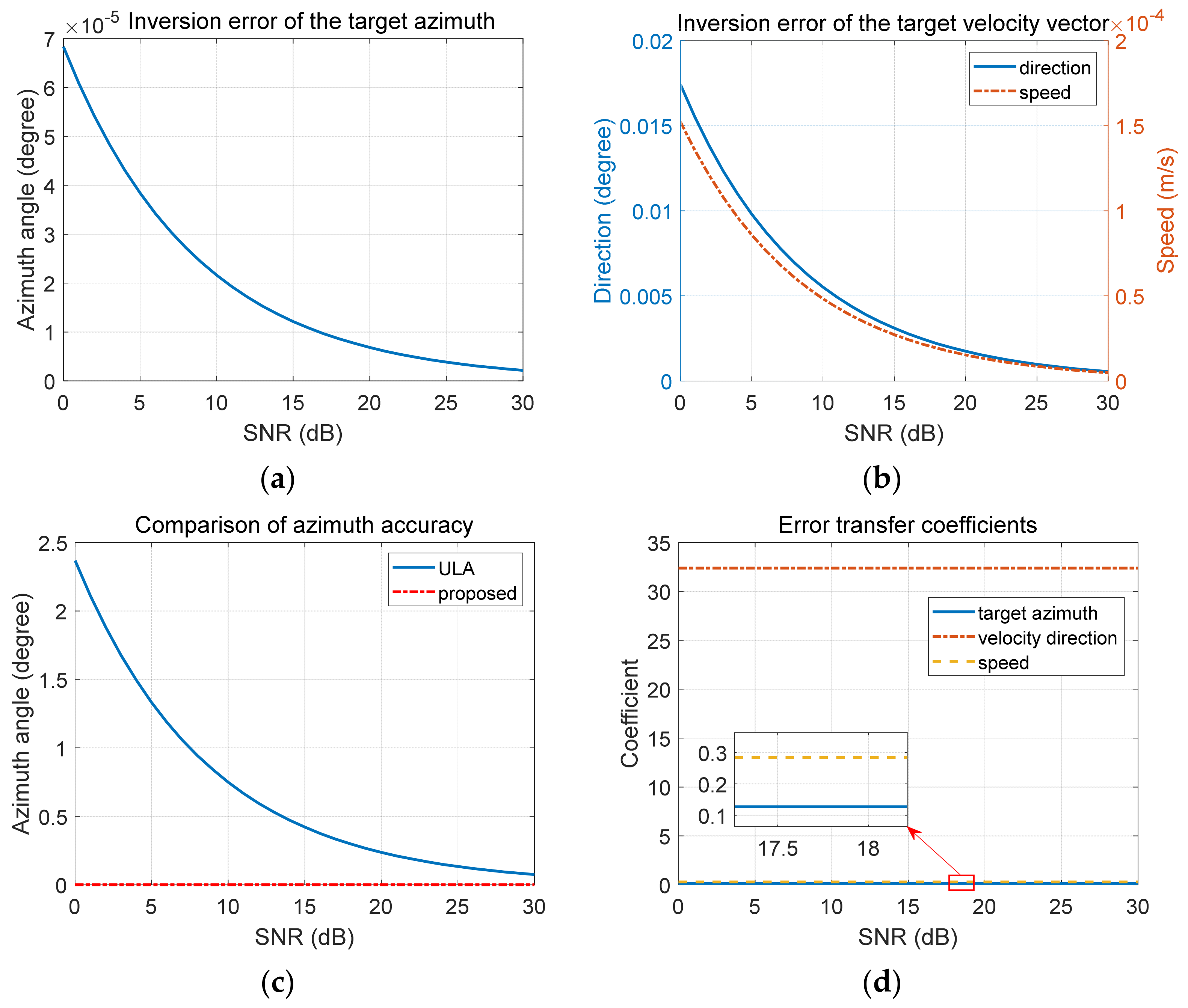
4.2. Analysis on the Azimuth Inversion Accuracy
5. Simulation Experiments
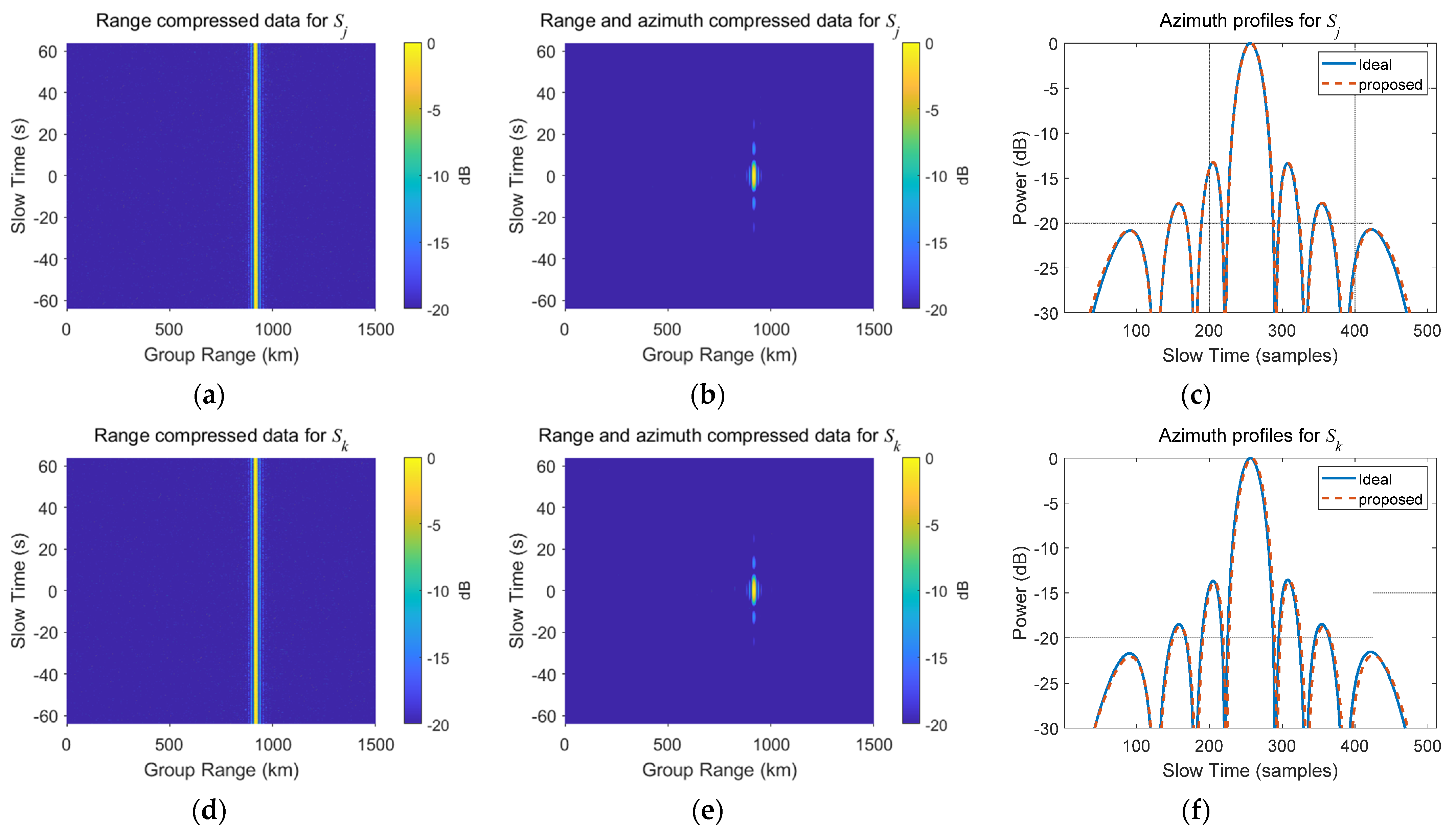
5.1. Distributed Synthetic Aperture Simulation
5.2. Parameter Inversion Results
5.2.1. Target Parameters Inversion Results for Two Ships
5.2.2. Target Parameters Inversion Results for Three Ships
6. Discussion
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Appendix A
Appendix B
References
- Zhou, X.; Wei, Y.; Liu, Y. Broadened Sea Clutter Suppression Method for Shipborne HF Hybrid Sky-Surface Wave Radar. In Proceedings of the 2020 IEEE Radar Conference (RadarConf20), Florence, Italy, 21–25 September 2020. [Google Scholar]
- Zhu, Y.; Wei, Y.; Yu, L. Ionospheric Decontamination for HF Hybrid Sky-Surface Wave Radar on a Shipborne Platform. IEEE Geosci. Remote Sens. Lett. 2017, 14, 2162–2166. [Google Scholar] [CrossRef]
- Wei, Y.; Zhu, Y. Simulation Study of First-Order Sea Clutter Doppler Spectra for Shipborne High Frequency Radar via Hybrid Sky-Surface Wave Propagation. J. Appl. Remote Sens. 2017, 11, 014001. [Google Scholar] [CrossRef]
- Anderson, S. Bistatic and Stereoscopic Configurations for HF Radar. Remote Sens. 2020, 12, 689. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Q.; Yue, X.; Zhang, L.; Wu, X.; Wang, L. Correction of Ionospheric Distortion on HF Hybrid Sky-Surface Wave Radar Calibrated by Direct Wave. Radio Sci. 2019, 54, 380–396. [Google Scholar] [CrossRef]
- Ding, M.; Wei, Y.; Yu, L.; Tong, P. Adaptive Suppression of Main-Lobe Spread Doppler Clutter with High Directivity for HFSSWR Using Oblique Projection. Electron. Lett. 2019, 55, 1245–1247. [Google Scholar] [CrossRef]
- Yang, L.-Q.; Fan, J.-M.; Guo, L.-X.; Liu, W.; Li, X.; Feng, J.; Lou, P.; Lu, Z.-X. Simulation Analysis and Experimental Study on the Echo Characteristics of High-Frequency Hybrid Sky–Surface Wave Propagation Mode. IEEE Trans. Antennas Propag. 2018, 66, 4821–4831. [Google Scholar] [CrossRef]
- Wei, Y.; Tong, P.; Xu, R.; Yu, L. Experimental Analysis of a HF Hybrid Sky-Surface Wave Radar. IEEE Aerosp. Electron. Syst. Mag. 2018, 33, 32–40. [Google Scholar] [CrossRef]
- Li, M.; Zhang, L.; Wu, X.; Yue, X.; Emery, W.J.; Yi, X.; Liu, J.; Yang, G. Ocean Surface Current Extraction Scheme With High-Frequency Distributed Hybrid Sky-Surface Wave Radar System. IEEE Trans. Geosci. Remote Sens. 2018, 56, 4678–4690. [Google Scholar] [CrossRef]
- Ji, Y.; Zhang, J.; Chu, X.; Wang, Y.; Yang, L. Ocean Surface Current Measurement with High-Frequency Hybrid Sky–Surface Wave Radar. Remote Sens. Lett. 2017, 8, 617–626. [Google Scholar] [CrossRef]
- Cui, X.; Gong, W.; Ye, X.; Pan, L.; Chen, Y. Application of Wuhan Ionospheric Oblique Backscattering Sounding System (WIOBSS) for Sea-State Detection. IEEE Geosci. Remote Sens. Lett. 2016, 13, 389–393. [Google Scholar] [CrossRef]
- Chen, S.; Huang, W.; Gill, E.W. First-Order Bistatic High-Frequency Radar Power for Mixed-Path Ionosphere-Ocean Propagation. IEEE Geosci. Remote Sens. Lett. 2016, 13, 1940–1944. [Google Scholar] [CrossRef]
- Chen, S.; Gill, E.W.; Huang, W. A First-Order HF Radar Cross-Section Model for Mixed-Path Ionosphere–Ocean Propagation With an FMCW Source. IEEE J. Ocean. Eng. 2016, 41, 982–992. [Google Scholar] [CrossRef]
- Walsh, J.; Gill, E.W.; Huang, W.; Chen, S. On the Development of a High-Frequency Radar Cross SectionModel for Mixed Path Ionosphere–Ocean Propagation. IEEE Trans. Antennas Propag. 2015, 63, 2655–2664. [Google Scholar] [CrossRef]
- Zhao, Z.; Wan, X.; Zhang, D.; Cheng, F. An Experimental Study of HF Passive Bistatic Radar Via Hybrid Sky-Surface Wave Mode. IEEE Trans. Antennas Propag. 2013, 61, 415–424. [Google Scholar] [CrossRef]
- Riddolls, R.J. Limits on the Detection of Low-Doppler Targets by a High Frequency Hybrid Sky-Surface Wave Radar System. In Proceedings of the 2008 IEEE Radar Conference, Rome, Italy, 26–30 May 2008. [Google Scholar]
- Melyanovcky, P.A.; Turgenev, I.S. Bistatic HF Radar for Oceanography Applications with the Use of Both Ground and Space Waves. Telecommun. Radio Eng. 1997, 51, 73–80. [Google Scholar] [CrossRef]
- Martino, G.D.; Iodice, A. Maritime Surveillance with Synthetic Aperture Radar; Institution of Engineering and Technology: London, UK, 2020; ISBN 978-1-78561-602-0. [Google Scholar]
- Cumming, I.G.; Wong, F.H. Digital Processing of Synthetic Aperture Radar Data: Algorithms and Implementation; Artech House: Boston, MA, USA, 2005; ISBN 978-1-58053-058-3. [Google Scholar]
- Carrer, L.; Zancanella, F.; Bruzzone, L. Mars HF Surface Imaging by Processing SHARAD Off-Nadir Echoes. In Proceedings of the EPSC-DPS Joint Meeting 2019, Geneva, Switzerland, 15–20 September 2019; Volume 13. [Google Scholar]
- Kobayashi, T.; Ono, T. SAR/InSAR Observation by an HF Sounder. J. Geophys. Res. Planets 2007, 112, E03S90. [Google Scholar] [CrossRef] [Green Version]
- Chen, J.; Li, Z.; Li, C.; Zhou, Y. Signal Processing Algorithm of Spaceborne SIMO HF-SAR for Three-Dimensional Topside Ionosphere Exploration. In Proceedings of the 2009 5th International Conference on Wireless Communications, Networking and Mobile Computing, Beijing, China, 24–26 September 2009. [Google Scholar]
- Li, L.; Li, F. Ionosphere Tomography Based on Spaceborne SAR. Adv. Space Res. 2008, 42, 1187–1193. [Google Scholar] [CrossRef]
- Ding, F.; Zhao, C.; Chen, Z.; Li, J. Sea Echoes for Airborne HF/VHF Radar: Mathematical Model and Simulation. Remote Sens. 2020, 12, 3755. [Google Scholar] [CrossRef]
- Voronovich, A.; Zavorotny, V. An Evaluation of the HF/VHF Synthetic Aperture Radar System for Ocean Wave Spectra Measurement. In Proceedings of the 2017 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Fort Worth, TX, USA, 23–28 July 2017; pp. 1530–1533. [Google Scholar]
- Peng, H.; Xue, W.; Zhu, S.; Yu, G. An Improved Model of HF-SAR for Estimating Surface Current Velocity. In Proceedings of the 2007 1st Asian and Pacific Conference on Synthetic Aperture Radar, Huangshan, China, 5–9 November 2007; pp. 760–765. [Google Scholar]
- Xue, W.; Zhang, M.; Tang, J.; Han, S. Surface Current Extraction by Onboard High Frequency SAR. In Proceedings of the TENCON 2006–2006 IEEE Region 10 Conference, Hong Kong, China, 14–16 November 2006. [Google Scholar]
- Nithirochananont, U.; Antoniou, M.; Cherniakov, M. Passive Coherent Multistatic SAR Using Spaceborne Illuminators. IET Radar Sonar Navig. 2020, 14, 628–636. [Google Scholar] [CrossRef]
- Wojaczek, P.; Cristallini, D. Optimal Trajectories for Range Resolution Improvement in Multi-PCL SAR. AEU-Int. J. Electron. Commun. 2017, 73, 173–182. [Google Scholar] [CrossRef]
- Xu, H.; Wang, J.; Yuan, J.; Wang, R.; Shan, X. Range-Resolution Improvement for Spaceborne/Airborne Bistatic Synthetic Aperture Radar Using Stepped-Frequency Chirp Trains. IET Signal Process. 2015, 9, 377–386. [Google Scholar] [CrossRef]
- Zhao, B.; Liu, M.; Lu, X.; Li, C.; Wang, P. High Resolution Imaging on Distributed SAR Using Joint Space-Time-Frequency Method. In Proceedings of the 2014 12th International Conference on Signal Processing (ICSP), Hangzhou, China, 19–23 October 2014; pp. 330–335. [Google Scholar]
- Cristallini, D.; Pastina, D.; Lombardo, P. Exploiting MIMO SAR Potentialities With Efficient Cross-Track Constellation Configurations for Improved Range Resolution. IEEE Trans. Geosci. Remote Sens. 2011, 49, 38–52. [Google Scholar] [CrossRef]
- Guillaso, S.; Reigber, A.; Ferro-Famil, L.; Pottier, E. Range Resolution Improvement of Airborne SAR Images. IEEE Geosci. Remote Sens. Lett. 2006, 3, 135–139. [Google Scholar] [CrossRef]
- Marechal, R.; Amiot, T.; Attia, S.; Aguttes, J.-P.; Souyris, J.-C. Distributed SAR for Performance Improvement. In Proceedings of the 2005 IEEE International Geoscience and Remote Sensing Symposium, IGARSS ’05, Seoul, Korea, 29 July 2005; Volume 2, pp. 1030–1033. [Google Scholar]
- Prati, C.; Rocca, F. Improving Slant-Range Resolution with Multiple SAR Surveys. IEEE Trans. Aerosp. Electron. Syst. 1993, 29, 135–143. [Google Scholar] [CrossRef]
- Richards, M.A. Fundamentals of Radar Signal Processing, 2nd ed.; McGraw-Hill Education: New York, NY, USA, 2014; ISBN 978-0-07-179832-7. [Google Scholar]
- Fabrizio, G.A. High Frequency Over-The-Horizon Radar: Fundamental Principles, Signal Processing, and Practical Applications; McGraw-Hill Education: New York, NY, USA, 2013; ISBN 978-0-07-162127-4. [Google Scholar]
- Harris, T.J.; Cervera, M.A.; Pederick, L.H.; Quinn, A.D. Separation of O/X Polarization Modes on Oblique Ionospheric Soundings. Radio Sci. 2017, 52, 1522–1533. [Google Scholar] [CrossRef] [Green Version]
- Gething, P.J.D. Relation between Oblique and Ground Path-Lengths in Ionospheric Propagation over a Curved Earth. Nature 1962, 193, 260–261. [Google Scholar] [CrossRef]
- Breit, G.; Tuve, M.A. A Test of the Existence of the Conducting Layer. Phys. Rev. 1926, 28, 554–575. [Google Scholar] [CrossRef]
- Ding, M.; Tong, P.; Wei, Y.; Yu, L. Multiple Phase Screen Modeling of HF Wave Field Scintillations Caused by the Irregularities in Inhomogeneous Media. Radio Sci. 2021, 56, e2020RS007239. [Google Scholar] [CrossRef]
- Zernov, N.; Gherm, V.; Zaalov, N. High-Frequency EM Wave Field Propagation in the Disturbed Ionosphere: Review of Recent Research at St. Petersburg State University. In Proceedings of the 2019 Russian Open Conference on Radio Wave Propagation (RWP), Kazan, Russia, 1–6 July 2019; Volume 1, pp. 25–30. [Google Scholar]
- Chen, S.; Gill, E.W.; Huang, W. A High-Frequency Surface Wave Radar Ionospheric Clutter Model for Mixed-Path Propagation with the Second-Order Sea Scattering. IEEE Trans. Antennas Propag. 2016, 64, 5373–5381. [Google Scholar] [CrossRef]
- Ravan, M.; Adve, R.S. Modeling the Received Signal for the Canadian Over-the-Horizon-Radar. In Proceedings of the 2013 IEEE Radar Conference (RadarCon13), Ottawa, ON, Canada, 29 April–3 May 2013; pp. 1–6. [Google Scholar]
- Ravan, M.; Riddolls, R.J.; Adve, R.S. Ionospheric and Auroral Clutter Models for HF Surface Wave and Over-the-Horizon Radar Systems. Radio Sci. 2012, 47, RS3010. [Google Scholar] [CrossRef]
- Yao, G.; Xie, J.; Huang, W. HF Radar Ocean Surface Cross Section for the Case of Floating Platform Incorporating a Six-DOF Oscillation Motion Model. IEEE J. Ocean. Eng. 2021, 46, 156–171. [Google Scholar] [CrossRef]
- Ma, Y.; Huang, W.; Gill, E.W. High-Frequency Radar Ocean Surface Cross Section Incorporating a Dual-Frequency Platform Motion Model. IEEE J. Ocean. Eng. 2018, 43, 195–204. [Google Scholar] [CrossRef]
- Walsh, J.; Huang, W.; Gill, E.W. The Second-Order High Frequency Radar Ocean Surface Cross Section for an Antenna on a Floating Platform. IEEE Trans. Antennas Propag. 2012, 60, 4804–4813. [Google Scholar] [CrossRef]
- Walsh, J.; Huang, W.; Gill, E.W. The First-Order High Frequency Radar Ocean Surface Cross Section for an Antenna on a Floating Platform. IEEE Trans. Antennas Propag. 2010, 58, 2994–3003. [Google Scholar] [CrossRef]
- Barbarossa, S. Analysis of Multicomponent LFM Signals by a Combined Wigner-Hough Transform. IEEE Trans. Signal Process. 1995, 43, 1511–1515. [Google Scholar] [CrossRef]
- Barbarossa, S.; Zanalda, A. A Combined Wigner-Ville and Hough Transform for Cross-Terms Suppression and Optimal Detection and Parameter Estimation. In Proceedings of the ICASSP-92: 1992 IEEE International Conference on Acoustics, Speech, and Signal Processing, San Francisco, CA, USA, 23–26 March 1992. [Google Scholar]
- Serbes, A. On the Estimation of LFM Signal Parameters: Analytical Formulation. IEEE Trans. Aerosp. Electron. Syst. 2018, 54, 848–860. [Google Scholar] [CrossRef]
- Farrance, I.; Frenkel, R. Uncertainty of Measurement: A Review of the Rules for Calculating Uncertainty Components through Functional Relationships. Clin. Biochem. Rev. 2012, 33, 49–75. [Google Scholar]
- Ristic, B.; Boashash, B. Comments on “The Cramer-Rao Lower Bounds for Signals with Constant Amplitude and Polynomial Phase. IEEE Trans. Signal Process. 1998, 46, 1708–1709. [Google Scholar] [CrossRef]
- Peleg, S.; Porat, B. The Cramer-Rao Lower Bound for Signals with Constant Amplitude and Polynomial Phase. IEEE Trans. Signal Process. 1991, 39, 749–752. [Google Scholar] [CrossRef]
- Nocedal, J.; Wright, S. Numerical Optimization, 2nd ed.; Springer: New York, NY, USA, 2006; ISBN 978-0-387-30303-1. [Google Scholar]
- Zhu, Y.; Wei, Y.; Tong, P. Wavefront Correction of Ionospherically Propagated HF RadioWaves Using Covariance Matching Techniques. Radioengineering 2017, 26, 330–336. [Google Scholar] [CrossRef]



| Types | Parameters |
|---|---|
| Known quantities | , , , ,, , , , |
| Unknown quantities | , , |
| Functions of unknown quantities | , , , , , , , , , |
| Parameters | Values |
|---|---|
| Carrier frequency | 20 MHz |
| Bandwidth | 30 kHz |
| Sampling rate in range | 200 kHz |
| Pulse width | 0.01024 s |
| Pulse repeat frequency (PRF) | 4 Hz |
| Coherent Integration Time (CIT) | 128 s |
| Antenna aperture D | 50 m |
| Input SNR | 0 dB |
| Virtual height | 205 km |
| Vertical plasma motion velocity | −10 m/s |
| Grazing angle | 28.6 degree |
| Target speed | 15 m/s |
| Target velocity direction (North by East) | 188 degree |
| Distance between and | 1920 m |
| Parameters | ||
|---|---|---|
| Baseline length | 700 km | - |
| Direction of the baseline (North by East) | 68.7 degree | - |
| Direction of the vector (North by East) | 97.5 degree | - |
| Distance from the target to the receiver | 60 km | - |
| Speed of the receiving ship | 15 m/s | 15 m/s |
| Velocity direction (North by East) | 8 degree | 6.7 degree |
| Parameters | |||
|---|---|---|---|
| theoretical value (Hz) | 1.0551 | 0.9678 | |
| estimated value (Hz) | 1.0549 | 0.9674 | |
| absolute error (Hz) | 1.3631 × 10−4 | 4.4751 × 10−4 | |
| relative error | 0.01% | 0.05% | |
| theoretical value (Hz/s) | −9.7017 × 10−4 | −9.5998 × 10−4 | |
| estimated value (Hz/s) | −9.5998 × 10−4 | −9.6866 × 10−4 | |
| absolute error (Hz/s) | 1.0190 × 10−5 | 8.6807 × 10−6 | |
| relative error | 1.05% | 0.90% | |
| PSLR (dB) | ISLR (dB) | IRW (Cells) | |
|---|---|---|---|
| −13.30 | −9.24 | 29.53 | |
| −13.71 | −9.84 | 28.94 | |
| Distributed system | −13.65 | −8.94 | 17.10 |
| Target Azimuth (Degree) | Target Velocity Direction (Degree) | Target Speed (m/s) | |
|---|---|---|---|
| 45 | 1.7053 × 10−13 | 1.1369 × 10−13 | 8.8818 × 10−15 |
| −45 | 1.7621 × 10−12 | 2.5580 × 10−13 | 8.5265 × 10−14 |
| Input SNR (dB) | Target Azimuth (Degree) | Target Velocity Direction (Degree) | Target Speed (m/s) |
|---|---|---|---|
| 0 | 3.6801 × 10−2 | 6.8546 × 10−2 | 6.4599 × 10−2 |
| 30 | 1.1624 × 10−3 | 2.1594 × 10−3 | 2.0414 × 10−3 |
| Input SNR (dB) | Target Azimuth (Degree) | Target Velocity Direction (Degree) | Target Speed (m/s) |
|---|---|---|---|
| 0 | 6.8355 × 10−5 | 1.7437 × 10−2 | 1.5230 × 10−4 |
| 30 | 2.1616 × 10−6 | 5.5139 × 10−4 | 4.8376 × 10−6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, M.; Tong, P.; Wei, Y.; Yu, L. Azimuth Resolution Improvement and Target Parameters Inversion for Distributed Shipborne High Frequency Hybrid Sky-Surface Wave Radar. Remote Sens. 2021, 13, 2471. https://doi.org/10.3390/rs13132471
Ding M, Tong P, Wei Y, Yu L. Azimuth Resolution Improvement and Target Parameters Inversion for Distributed Shipborne High Frequency Hybrid Sky-Surface Wave Radar. Remote Sensing. 2021; 13(13):2471. https://doi.org/10.3390/rs13132471
Chicago/Turabian StyleDing, Mingkai, Peng Tong, Yinsheng Wei, and Lei Yu. 2021. "Azimuth Resolution Improvement and Target Parameters Inversion for Distributed Shipborne High Frequency Hybrid Sky-Surface Wave Radar" Remote Sensing 13, no. 13: 2471. https://doi.org/10.3390/rs13132471
APA StyleDing, M., Tong, P., Wei, Y., & Yu, L. (2021). Azimuth Resolution Improvement and Target Parameters Inversion for Distributed Shipborne High Frequency Hybrid Sky-Surface Wave Radar. Remote Sensing, 13(13), 2471. https://doi.org/10.3390/rs13132471






