Estimation of the Lateral Dynamic Displacement of High-Rise Buildings under Wind Load Based on Fusion of a Remote Sensing Vibrometer and an Inclinometer
Abstract
:1. Introduction
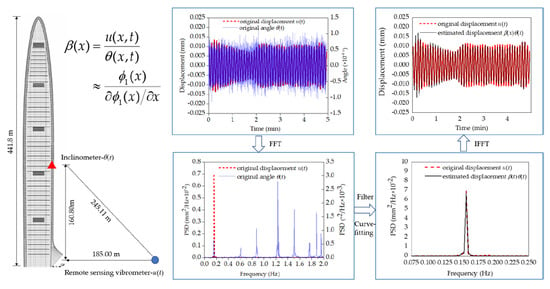
2. Theoretical Derivation
3. Dynamic Displacement Estimation by Fitting Coefficient β(x)
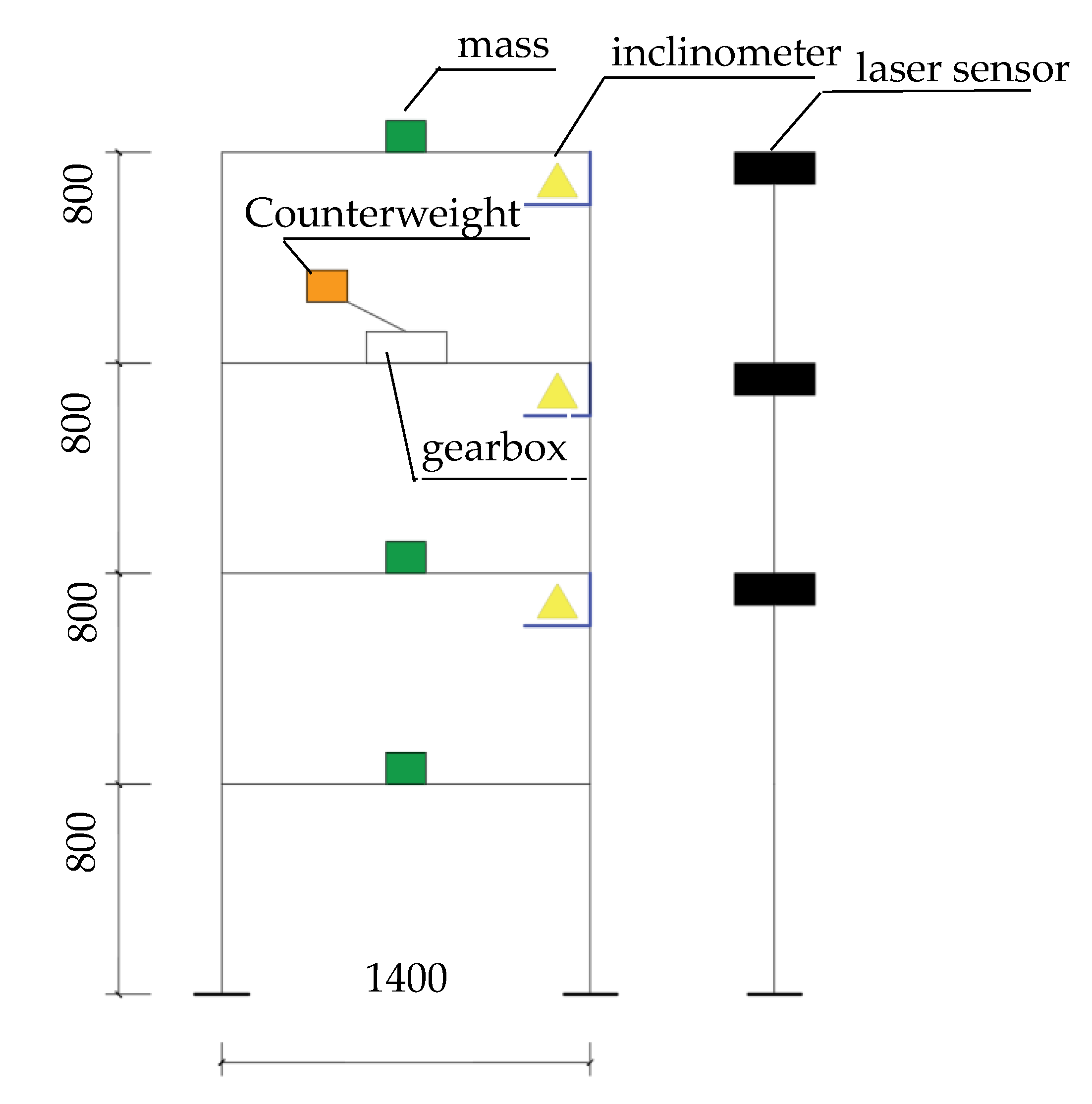
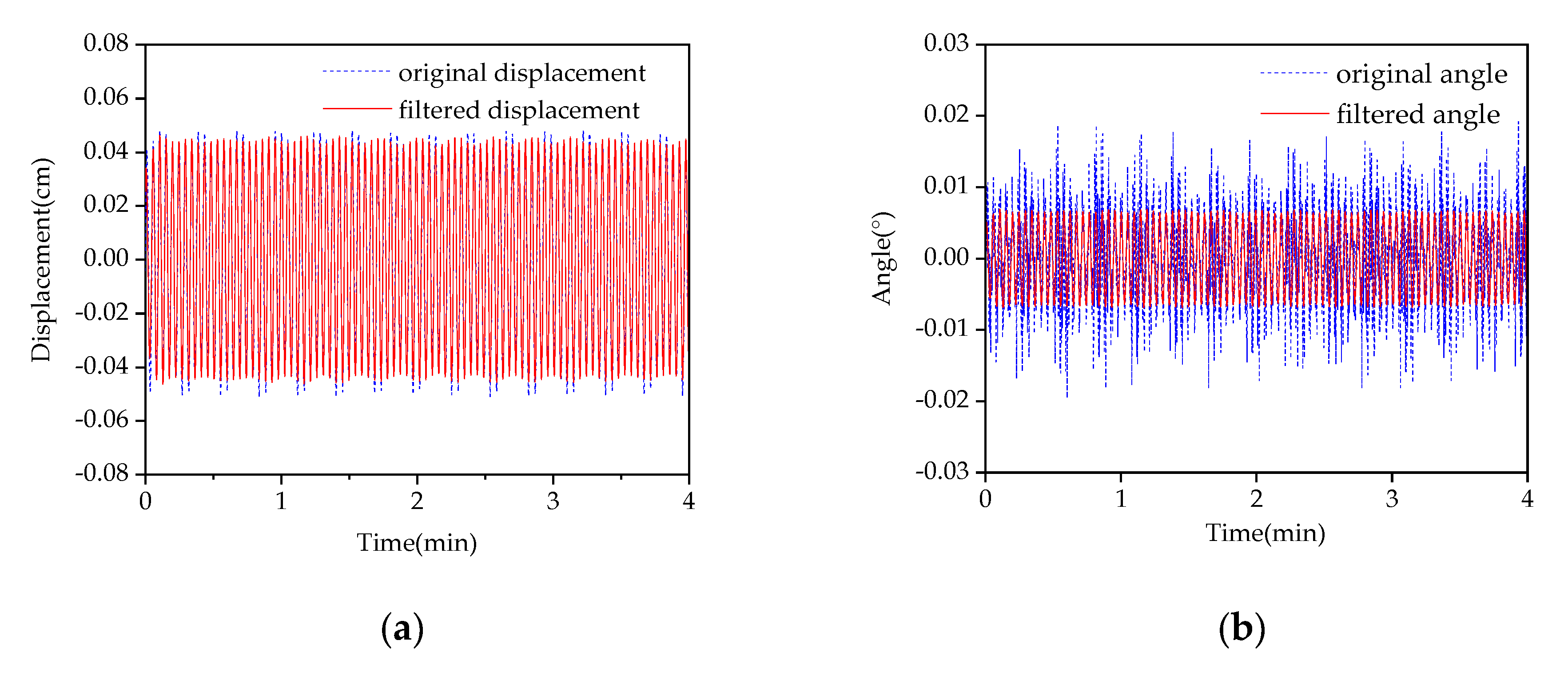
3.1. Laboratoty Dynamic Experiment
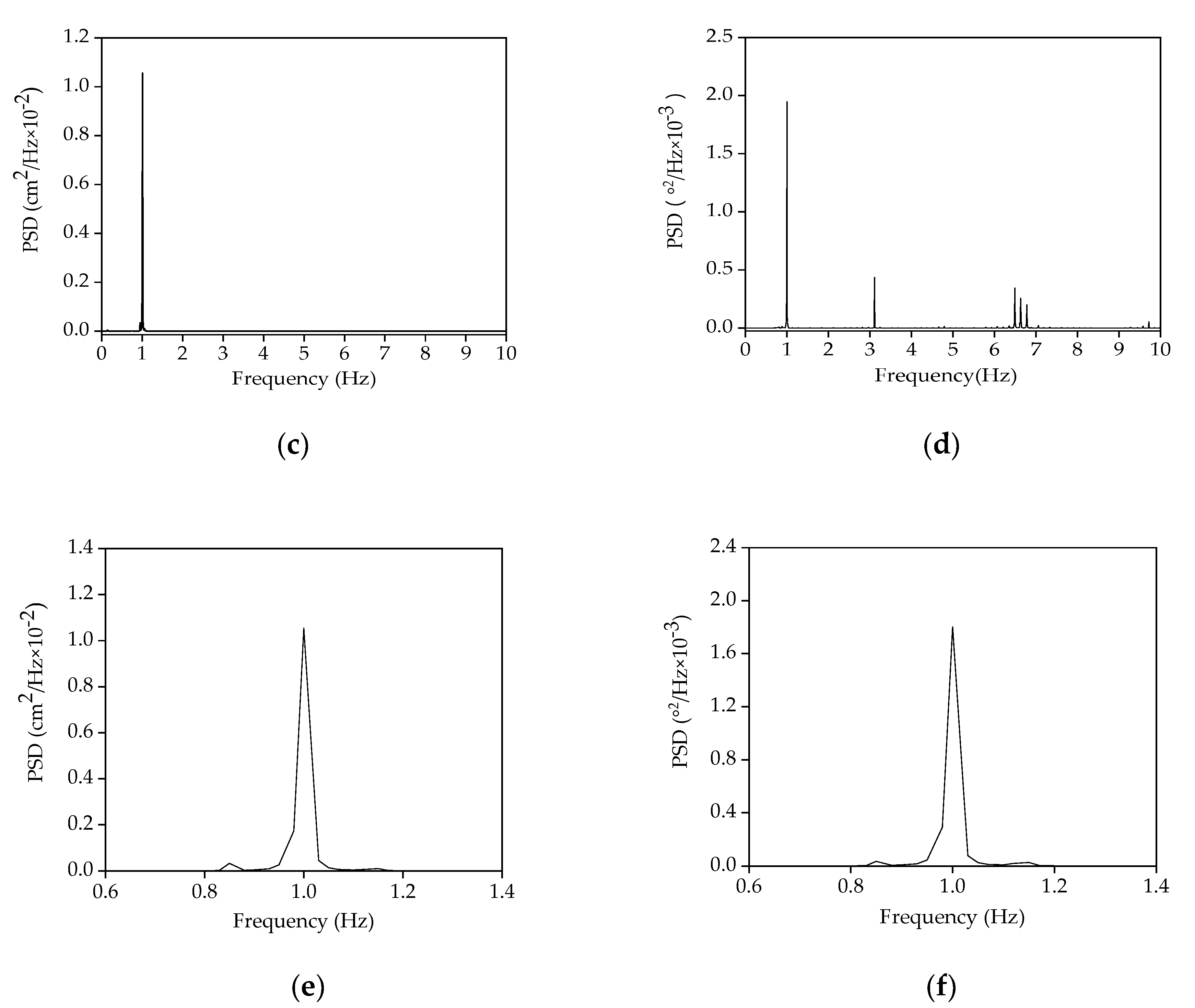
3.2. Fitting Coefficient β(x) and the Dynamic Displacement Estimation
4. Dynamic Displacement Estimation of High-Rise Buildings
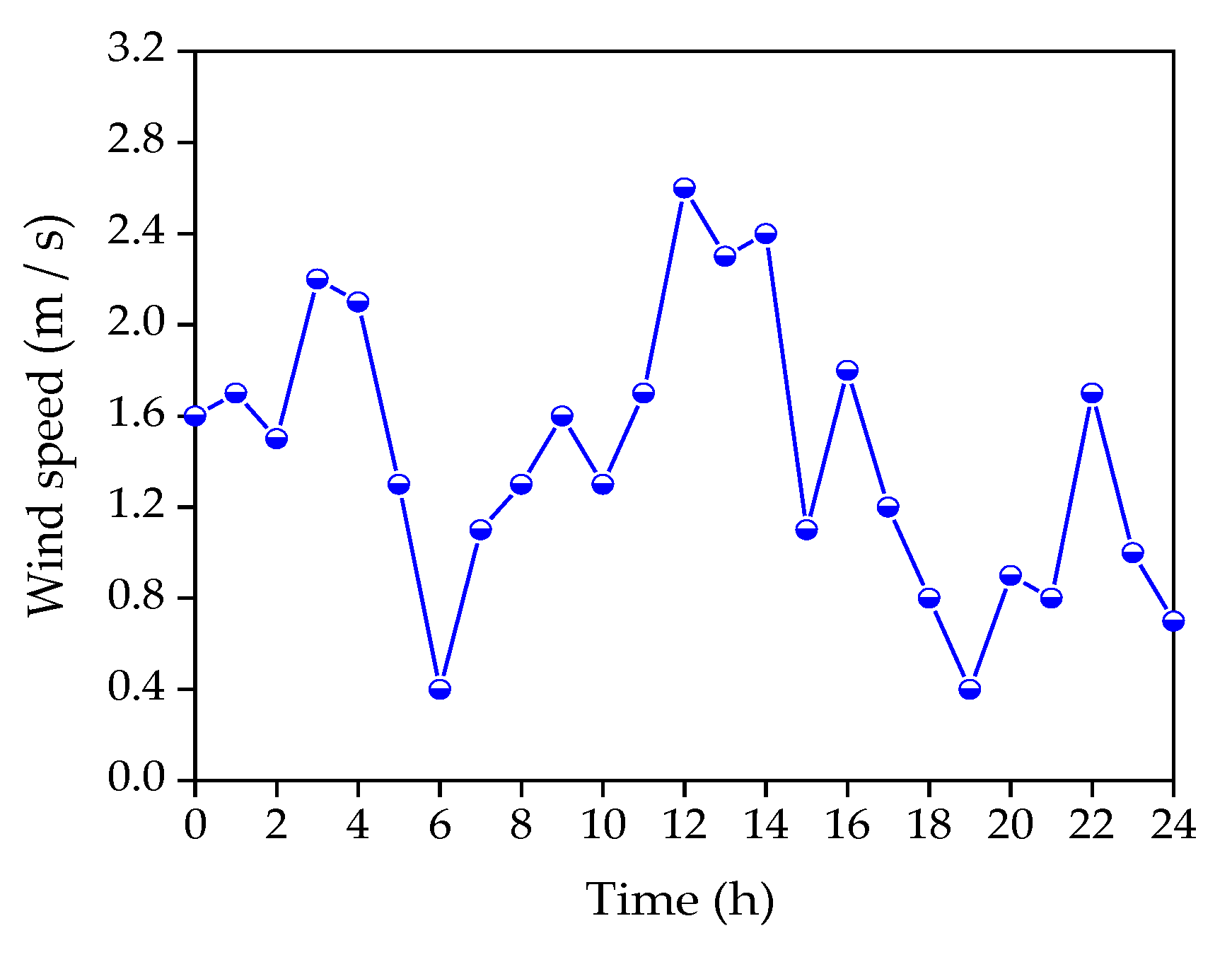
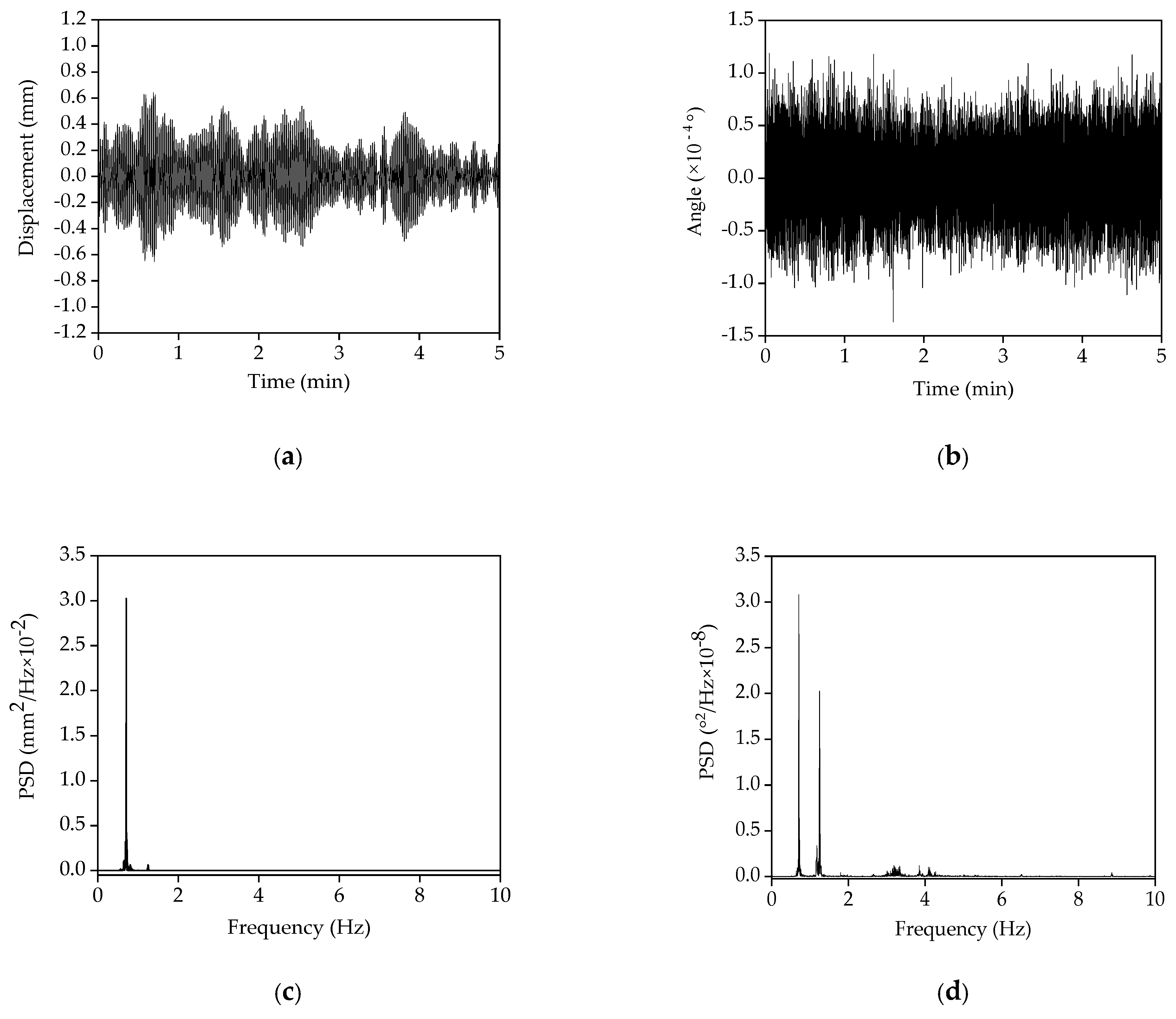
4.1. Dynamic Displacement Estimation a Shear Wall High-Rise Building
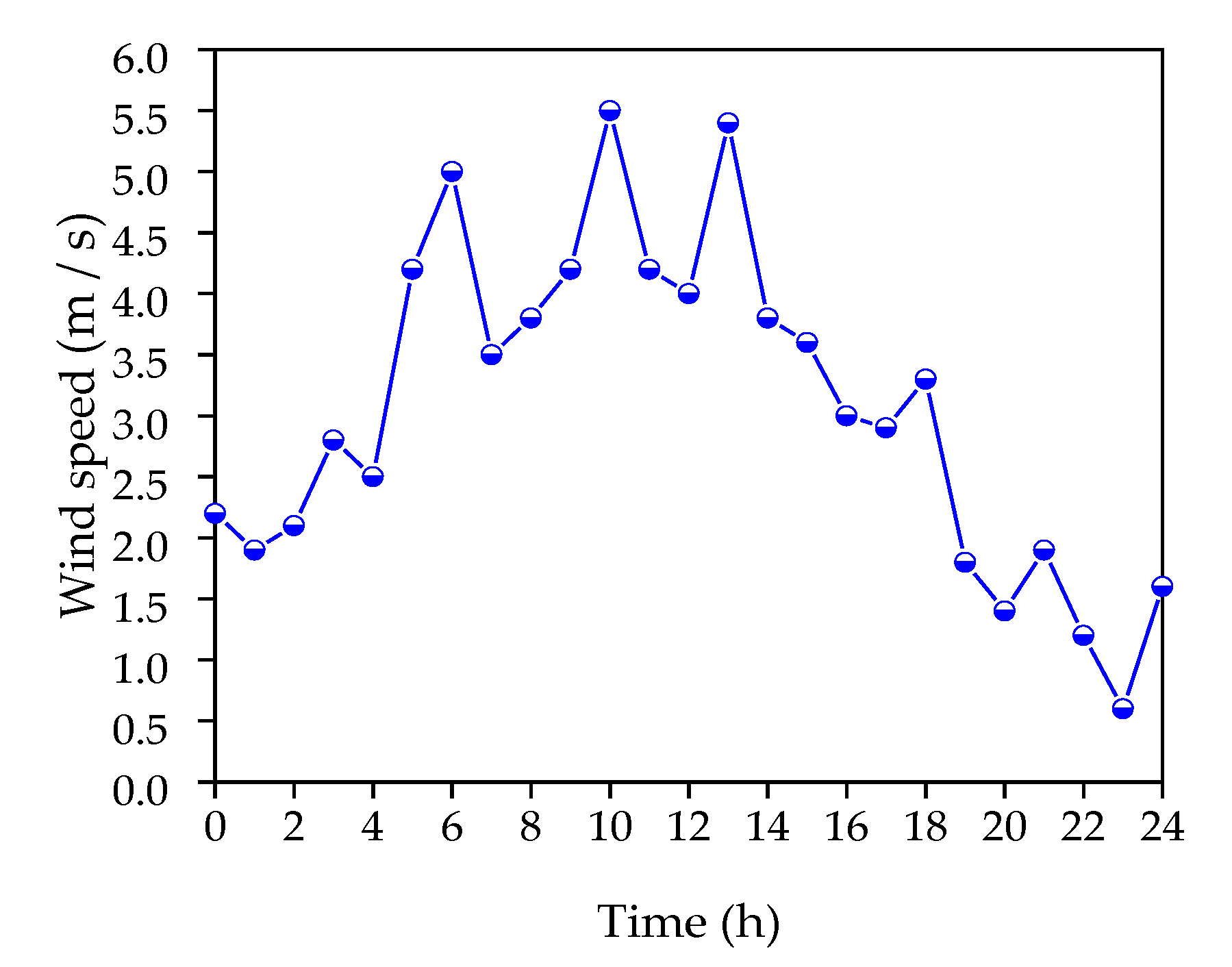
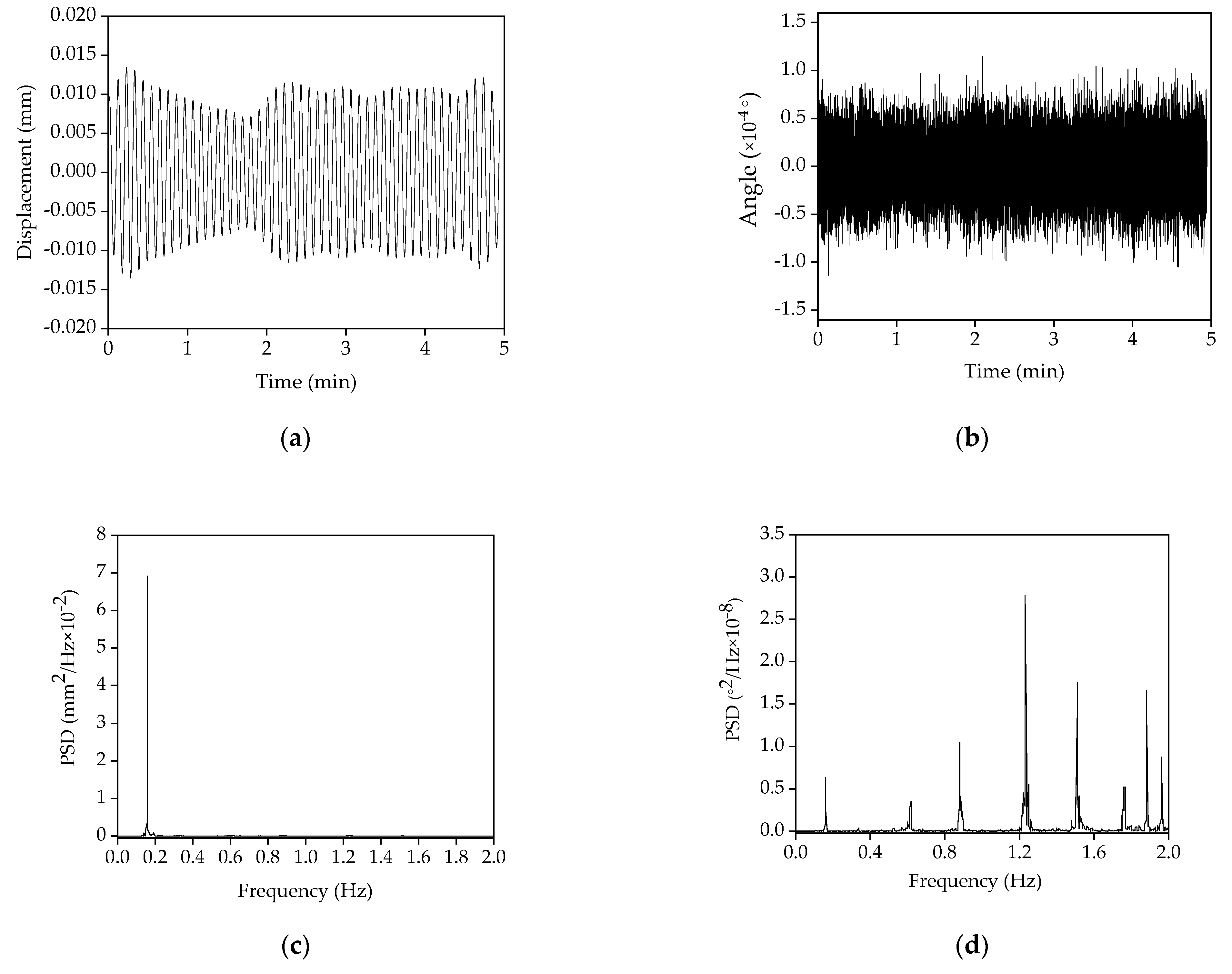
4.2. Dynamic Displacement Estimation of the Kingkey 100 Skyscraper
5. Conclusions and Future Works
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Au, S.K.; Zhang, F.L. Top field observations on modal properties of two super tall buildings under strong wind. J. Wind. Eng. Ind. Aerodyn. 2012, 101, 12–23. [Google Scholar] [CrossRef]
- Zhang, F.L.; Xiong, H.B.; Shi, W.X.; Ou, X. Structural health monitoring of a super tall building during different stages using a bayesian approach. Struct. Control Health Monit. 2016, 10, 102–114. [Google Scholar]
- Yi, J.; Zhang, J.W.; Li, Q.S. Dynamic characteristics and wind-Induced responses of a super-tall building during typhoons. J. Wind. Eng. Ind. Aerodyn. 2013, 121, 116–130. [Google Scholar] [CrossRef]
- Ye, X.W.; Jin, T.; Yun, C.B. A review on deep learning-based structural health monitoring of civil infrastructures. Smart Struct. Syst. 2019, 5, 567–586. [Google Scholar]
- Wu, L.; Casciati, F. Local Positioning systems versus structural monitoring: A review. Struct. Control Health Monit. 2014, 21, 1209–1221. [Google Scholar] [CrossRef]
- Park, J.; Sim, S.; Jung, H.; Spencer, B.F. Development of a wireless displacement measurement system using acceleration responses. Sensors 2013, 13, 8377–8392. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gomez, J.A.; Ozdagli, A.I.; Moreu, F. Reference-free dynamic displacements of railroad bridges using low-cost sensors. J. Intell. Mater. Syst. Struct. 2019, 5, 567–586. [Google Scholar] [CrossRef]
- Zheng, W.; Dan, D.; Cheng, W.; Xia, Y. Real-time dynamic displacement monitoring with double integration of acceleration based on recursive least squares method. Measurement 2019, 141, 460–471. [Google Scholar] [CrossRef]
- Xiong, H.B.; Cao, J.X.; Zhang, F.L. Inclinometer-based method to monitor displacement of high-rise buildings. Struct. Monit. Maint. 2018, 5, 111–127. [Google Scholar]
- Xia, Y.; Zhang, P.; Ni, Y.; Zhu, H. Deformation monitoring of a super-tall structure using real-time strain data. Eng. Struct. 2014, 67, 29–38. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.; Zhang, J.; Duan, W. Deflection distribution estimation of tied-arch bridges using long-gauge strain measurements. Struct. Control Health Monit. 2018, 25, e2119. [Google Scholar] [CrossRef]
- Yi, T.; Li, H.; Gu, M. Recent research and applications of GPS-based monitoring technology for high-rise structures. Struct. Control Health Monit. 2013, 20, 649–670. [Google Scholar] [CrossRef]
- Im, S.B.; Hurlebaus, S.; Kang, Y.J. Summary review of GPS technology for structural health monitoring. J. Struct. Eng. 2013, 139, 1653–1664. [Google Scholar] [CrossRef]
- Wang, G.; Ding, Y.; Feng, D. Evaluation of the wind-resistant performance of long-span cable-stayed bridge using the monitoring correlation between the static cross wind and its displacement response. Shock Vib. 2018, 2018, 1–10. [Google Scholar] [CrossRef]
- Quesada-Olmo, N.; Jimenez-Martinez, M.J.; Farjas-Abadia, M. Real-time high-rise building monitoring system using global navigation satellite system technology. Measurement 2018, 123, 115–124. [Google Scholar] [CrossRef]
- Zhang, Q.; Ma, C.; Meng, X. Galileo augmenting GPS single-frequency single-epoch precise positioning with baseline constrain for bridge dynamic monitoring. Remote Sens. 2019, 11, 438. [Google Scholar] [CrossRef] [Green Version]
- Yu, J.; Meng, X.; Yan, B. Global Navigation Satellite System-based positioning technology for structural health monitoring: A review. Struct. Control Health Monit. 2019, 27, e2467. [Google Scholar] [CrossRef] [Green Version]
- Feng, D.; Feng, M.; Ozer, E. A vision-based sensor for noncontact structural displacement measurement. Sensors 2015, 15, 16557–16575. [Google Scholar] [CrossRef]
- Xu, Y.; Brownjohn, J.; Kong, D. A non-contact vision-based system for multipoint displacement monitoring in a cable-stayed footbridge. Struct. Control Health Monit. 2018, 25, e2155. [Google Scholar] [CrossRef] [Green Version]
- Ni, Y.Q.; Wang, Y.W.; Liao, W.Y. A vision-based system for long-distance remote monitoring of dynamic displacement: Experimental verification on a supertall structure. Smart Struct. Syst. 2019, 24, 769–781. [Google Scholar]
- Luo, L.; Feng, M.Q.; Wu, Z.Y. Robust vision sensor for multi-point displacement monitoring of bridges in the field. Eng. Struct. 2018, 163, 255–266. [Google Scholar] [CrossRef]
- Li, C.; Chen, W.; Liu, G.A. Noncontact FMCW radar sensor for displacement measurement in structural health monitoring. Sensors 2015, 15, 7412–7433. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hu, J.; Guo, J.; Xu, Y. Differential ground-based radar interferometry for slope and civil structures monitoring: Two case studies of landslide and bridge. Remote Sens. 2019, 11, 2887. [Google Scholar] [CrossRef] [Green Version]
- Luzi, G.; Crosetto, M.; Fernández, E. Radar interferometry for monitoring the vibration characteristics of buildings and civil structures: Recent case studies in Spain. Sensors 2017, 17, 669. [Google Scholar] [CrossRef] [Green Version]
- Casciati, F.; Wu, L.J. Local positioning accuracy of laser sensors for structural health monitoring. Struct. Control Health Monit. 2013, 20, 728–739. [Google Scholar] [CrossRef]
- Garg, P.; Moreu, F.; Ozdagli, A. Noncontact dynamic displacement measurement of structures using a moving laser doppler vibrometer. J. Bridge Eng. 2019, 24, 04019089. [Google Scholar] [CrossRef]
- Prochniewicz, D.; Szpunar, R.; Walo, J. A new study of describing the reliability of GNSS Network RTK positioning with the use of quality indicators. Meas. Sci. Technol. 2017, 28, 15012. [Google Scholar] [CrossRef]
- Koo, G.; Kim, K.; Chung, J.Y. Development of a high precision displacement measurement system by fusing a low cost RTK-GPS sensor and a force feedback accelerometer for infrastructure monitoring. Sensors 2017, 17, 2745. [Google Scholar] [CrossRef] [Green Version]
- Koo, G.; Kim, K.; Chung, J.Y. Structural displacement estimation through multi-rate fusion of accelerometer and RTK-GPS displacement and velocity measurements. Measurement 2018, 130, 223–235. [Google Scholar]
- Kerle, N.; Janssen, L.L.F.; Hurneman, G.C.A. Principles of Remote Sensing; The International Institute for Geo-Information Science and Earth Observation (TC): Overijssel, The Netherlands, 2004. [Google Scholar]
- Lillesand, T.M.; Kiefer, R.W.; Chipman, J.W. Remote Sensing and Image Interpretation, 7th ed.; John Wiley Sons, Inc.: Hoboken, NJ, USA, 2015. [Google Scholar]
- Smyth, A.; Wu, M. Multi-rate Kalman filtering for the data fusion of displacement and acceleration response measurements in dynamic system monitoring. Mech. Syst. Signal Process. 2007, 21, 706–723. [Google Scholar] [CrossRef]
- Xu, Y.; Brownjohn, J.M.W.; Hester, D. Long-span bridges: Enhanced data fusion of GPS displacement and deck accelerations. Eng. Struct. 2017, 147, 639–651. [Google Scholar] [CrossRef] [Green Version]
- Xu, Y.; Brownjohn, J.M.W.; Huseynov, F. Accurate deformation monitoring on bridge structures using a cost-effective sensing system combined with a camera and accelerometers: Case study. J. Bridge Eng. 2019, 24, 05018014. [Google Scholar] [CrossRef] [Green Version]
- Castagnetti, C.; Bassoli, E.; Vincenzi, L. Dynamic assessment of masonry towers based on terrestrial radar interferometer and accelerometers. Sensors 2019, 19, 1319. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kim, J.; Kim, K.; Sohn, H. Autonomous dynamic displacement estimation from data fusion of acceleration and intermittent displacement measurements. Mech. Syst. Signal Process. 2014, 42, 194–205. [Google Scholar] [CrossRef]
- Park, J.W.; Sim, S.H.; Jung, H.J. Wireless displacement sensing system for bridges using multi-sensor fusion. Smart Mater. Struct. 2014, 23, 45022. [Google Scholar] [CrossRef]
- Ozdagli, A.I.; Gomez, J.A.; Moreu, F. Real-time reference-free displacement of railroad bridges during train-crossing events. J. Bridge Eng. 2017, 22, 04017073. [Google Scholar] [CrossRef]
- Davenport, A.G. The treatment of wind loading on tall buildings. In Proceedings of the Symposium on Tall Buildings, Southampton, UK, 13–15 April 1966; pp. 3–44. [Google Scholar]
- Davenport, A.G. The response of six building shapes to turbulent wind. Philos. Trans. R. Soc. A: Math. Phys. Eng. Sci. 1971, 269, 385–394. [Google Scholar]
- Holmes, J.D. Wind Loading of Structures, 2nd ed.; Taylor and Francis: Didcot, UK, 2007. [Google Scholar]
- Clough, R.W.; Penzien, J. Dynamics of Structures, 3rd ed.; Computers Structures, Inc.: Berkeley, CA, USA, 1995. [Google Scholar]
- Hu, W.H.; Xu, Z.M.; Bian, Y.-H.; Tang, D.-H.; Lu, W.; Teng, J.; Han, X.H. Investigation on dynamic property of a full-scale super high-rise building based on distributed synchronous acquisition. Eng. Struct. Submitted.









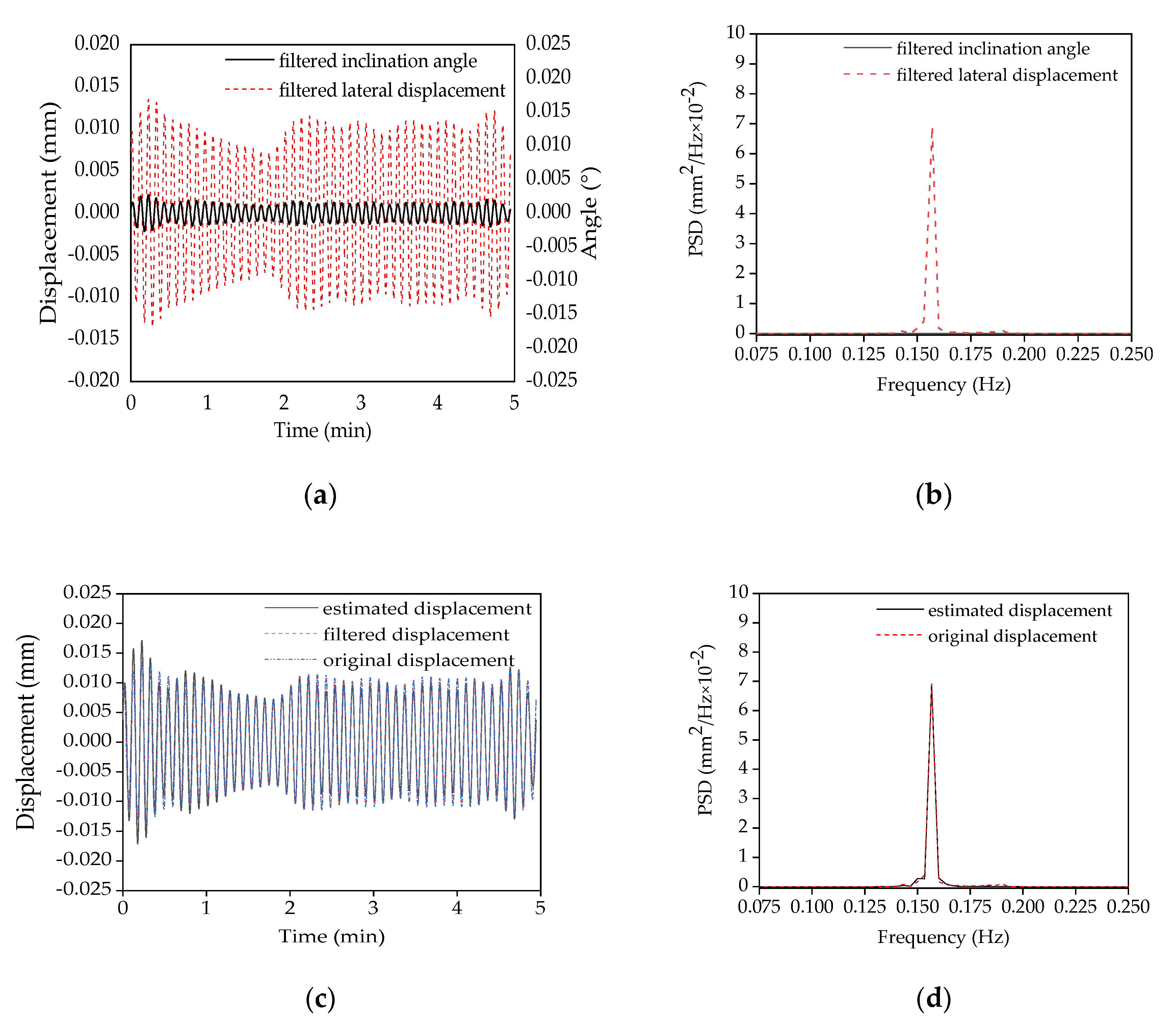
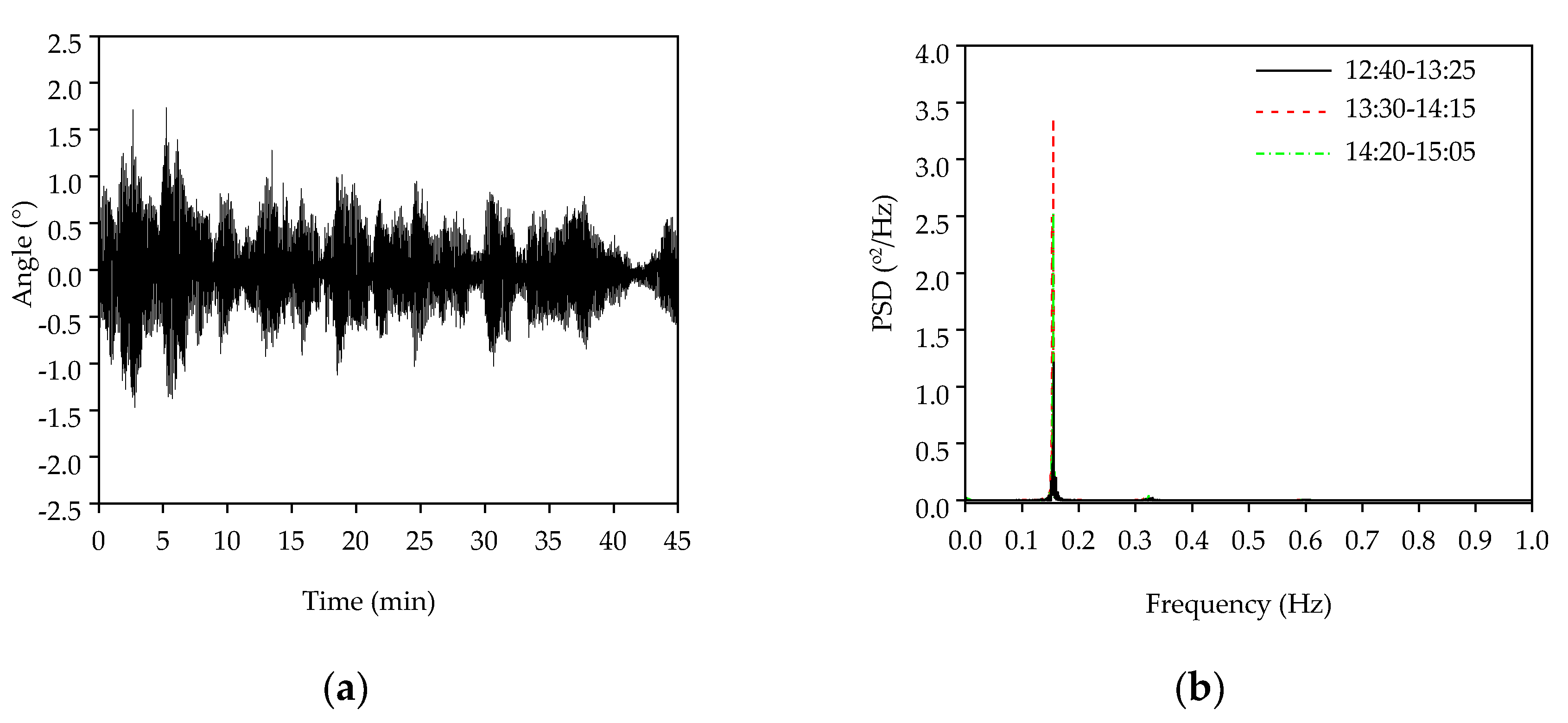
| Mode Order | Frequency (Hz) | ||
|---|---|---|---|
| FE Model | Displacement | Inclination Angle | |
| 1 | 1.04 | 0.97 | 0.98 |
| 2 | 3.82 | 3.52 | 3.52 |
| 3 | 7.63 | 6.90 | 7.01 |
| External Excitation | Mode Order | |||||||
|---|---|---|---|---|---|---|---|---|
| 1st | 2nd | 3rd | The Rest | |||||
| Displacement | Angle | Displacement | Angle | Displacement | Angle | Displacement | Angle | |
| Random vibration | 96.8 | 85.4 | 2.5 | 7.2 | 0.6 | 5.7 | 0.1 | 1.7 |
| 2 rad/s | 96.4 | 72.5 | 2.9 | 12.3 | 0.6 | 9.6 | 0.1 | 5.6 |
| 5 rad/s | 96.2 | 78.5 | 3.1 | 8.8 | 0.6 | 6.8 | 0.1 | 5.9 |
| 8 rad/s | 95.1 | 75.4 | 3.1 | 9.5 | 1.5 | 8.9 | 0.3 | 6.1 |
| External Excitation | Original Displacement | Coefficient β(x) | Estimated Displacement by Multiplying β(x) with the Inclination Angle | Difference (%) |
|---|---|---|---|---|
| Random | 4.89 | 23.75 | 4.66 | 4.7 |
| 2 rad/s | 0.039 | 24.54 | 0.038 | 2.5 |
| 5 rad/s | 0.050 | 24.12 | 0.052 | 3.8 |
| 8 rad/s | 0.376 | 23.51 | 0.377 | 0.2 |
| Story | External Excitation | Coefficient β(x) | Mean Value | FE Model |
|---|---|---|---|---|
| 2 | Random | 23.14 | 23.08 | 24.09 |
| 2 rad/s | 23.55 | |||
| 5 rad/s | 22.62 | |||
| 8 rad/s | 23.03 | |||
| 3 | Random | 23.86 | 23.34 | 24.16 |
| 2 rad/s | 23.15 | |||
| 5 rad/s | 23.18 | |||
| 8 rad/s | 23.16 | |||
| 4 | Random | 23.75 | 23.98 | 24.27 |
| 2 rad/s | 24.54 | |||
| 5 rad/s | 24.12 | |||
| 8 rad/s | 23.51 |
| Mode Order | Frequency (Hz) | |||
|---|---|---|---|---|
| FE Model | Modal Identification | |||
| Displacement | Inclination Angle | Acceleration | ||
| 1 | 0.72 | 0.70 | 0.70 | 0.69 |
| 2 | 3.38 | 3.16 | 3.25 | 3.15 |
| 3 | 7.19 | 6.74 | 6.76 | 6.87 |
| 4 | 11.14 | 11.19 | 10.69 | 11.12 |
| Experiment Number | Mode Order | |||||||
|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | The Rest | |||||
| Displacement | Angle | Displacement | Angle | Displacement | Angle | Displacement | Angle | |
| 1 | 96.9 | 85.4 | 1.8 | 11.0 | 1.1 | 3.4 | 0.2 | 0.2 |
| 2 | 95.7 | 81.1 | 2.3 | 12.3 | 1.9 | 6.5 | 0.1 | 0.1 |
| 3 | 95.5 | 78.6 | 2.9 | 13.2 | 1.4 | 8.9 | 0.1 | 0.3 |
| 4 | 97.1 | 79.1 | 1.5 | 12.2 | 1.3 | 8.6 | 0.1 | 0.1 |
| 5 | 96.5 | 84.0 | 1.8 | 11.9 | 1.3 | 4.0 | 0.4 | 0.1 |
| 6 | 95.4 | 76.4 | 2.4 | 14.2 | 1.9 | 9.2 | 0.3 | 0.2 |
| Floor | Method | Displacement (mm) | |||||
|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | ||
| 14 | Original displacement | 0.302 | 0.261 | 0.315 | 0.265 | 0.688 | 0.421 |
| Coefficient β(x) and inclination angle | 0.300 | 0.260 | 0.303 | 0.232 | 0.693 | 0.413 | |
| Difference (%) | 0.6 | 0.4 | 3.8 | 2.5 | 0.7 | 1.9 | |
| 23 | Original displacement | 0.530 | 0.646 | 0.499 | 0.774 | 1.614 | 0.939 |
| Coefficient β(x) and Inclination angle | 0.536 | 0.636 | 0.495 | 0.758 | 1.600 | 0.934 | |
| Difference (%) | 1.1 | 1.5 | 0.8 | 2.1 | 0.8 | 0.5 | |
| Floor | Experimental Group | Fitted β(x) Value | Mean | FE Model |
|---|---|---|---|---|
| 14 | 1 | 482.88 | 483.70 | 500.24 |
| 2 | 487.84 | |||
| 3 | 481.44 | |||
| 4 | 486.40 | |||
| 5 | 479.16 | |||
| 6 | 481.22 | |||
| 23 | 1 | 493.92 | 488.85 | 510.05 |
| 2 | 490.42 | |||
| 3 | 489.12 | |||
| 4 | 484.64 | |||
| 5 | 489.92 | |||
| 6 | 488.16 |
| Mode Order | Frequency (Hz) | ||
|---|---|---|---|
| Displacement | Inclination Angle | Acceleration | |
| 1 | 0.159 | 0.160 | 0.155 |
| 2 | 0.583 | 0.608 | 0.596 |
| 3 | 0.881 | 0.889 | 0.865 |
| 4 | 1.235 | 1.239 | 1.204 |
| 5 | 1.755 | 1.751 | 1.731 |
| Experiment Number | Mode Order | |||||
|---|---|---|---|---|---|---|
| 1 | 2 | The Rest | ||||
| Displacement | Angle | Displacement | Angle | Displacement | Angle | |
| 1 | 96.1 | 20.3 | 2.8 | 9.5 | 1.1 | 70.2 |
| 2 | 96.8 | 23.9 | 2.3 | 10.1 | 2.0 | 66.0 |
| 3 | 95.5 | 26.6 | 2.9 | 4.7 | 1.5 | 67.7 |
| Floor | Method | Displacement (mm) | ||
|---|---|---|---|---|
| 1 | 2 | 3 | ||
| 18F | Original lateral displacement | 0.0143 | 0.0081 | 0.0079 |
| Coefficient β(x) and Inclination angle | 0.0141 | 0.0077 | 0.0082 | |
| Difference (%) | 1.4 | 4.9 | 3.8 | |
| 37F | Original lateral displacement | 0.0261 | 0.0360 | 0.0310 |
| Coefficient β(x) and Inclination angle | 0.0253 | 0.0365 | 0.0324 | |
| Difference (%) | 3.1 | 1.4 | 4.5 | |
| Floor | Fitted β(x) Value | Mean |
|---|---|---|
| 37F | 576.9 | 575.1 |
| 578.5 | ||
| 569.8 | ||
| 18F | 589.4 | 581.7 |
| 578.3 | ||
| 577.5 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, W.-H.; Xu, Z.-M.; Liu, M.-Y.; Tang, D.-H.; Lu, W.; Li, Z.-H.; Teng, J.; Han, X.-H.; Said, S.; Rohrmann, R.G. Estimation of the Lateral Dynamic Displacement of High-Rise Buildings under Wind Load Based on Fusion of a Remote Sensing Vibrometer and an Inclinometer. Remote Sens. 2020, 12, 1120. https://doi.org/10.3390/rs12071120
Hu W-H, Xu Z-M, Liu M-Y, Tang D-H, Lu W, Li Z-H, Teng J, Han X-H, Said S, Rohrmann RG. Estimation of the Lateral Dynamic Displacement of High-Rise Buildings under Wind Load Based on Fusion of a Remote Sensing Vibrometer and an Inclinometer. Remote Sensing. 2020; 12(7):1120. https://doi.org/10.3390/rs12071120
Chicago/Turabian StyleHu, Wei-Hua, Zeng-Mao Xu, Ming-Yue Liu, De-Hui Tang, Wei Lu, Zuo-Hua Li, Jun Teng, Xiao-Hui Han, Samir Said, and Rolf. G. Rohrmann. 2020. "Estimation of the Lateral Dynamic Displacement of High-Rise Buildings under Wind Load Based on Fusion of a Remote Sensing Vibrometer and an Inclinometer" Remote Sensing 12, no. 7: 1120. https://doi.org/10.3390/rs12071120
APA StyleHu, W.-H., Xu, Z.-M., Liu, M.-Y., Tang, D.-H., Lu, W., Li, Z.-H., Teng, J., Han, X.-H., Said, S., & Rohrmann, R. G. (2020). Estimation of the Lateral Dynamic Displacement of High-Rise Buildings under Wind Load Based on Fusion of a Remote Sensing Vibrometer and an Inclinometer. Remote Sensing, 12(7), 1120. https://doi.org/10.3390/rs12071120