Improved Zenith Tropospheric Delay Modeling Using the Piecewise Model of Atmospheric Refractivity
Abstract
1. Introduction
2. Data and Methodology for Calculating ZTD
2.1. ERA5 Reanalysis Data
2.2. Radiosonde Data
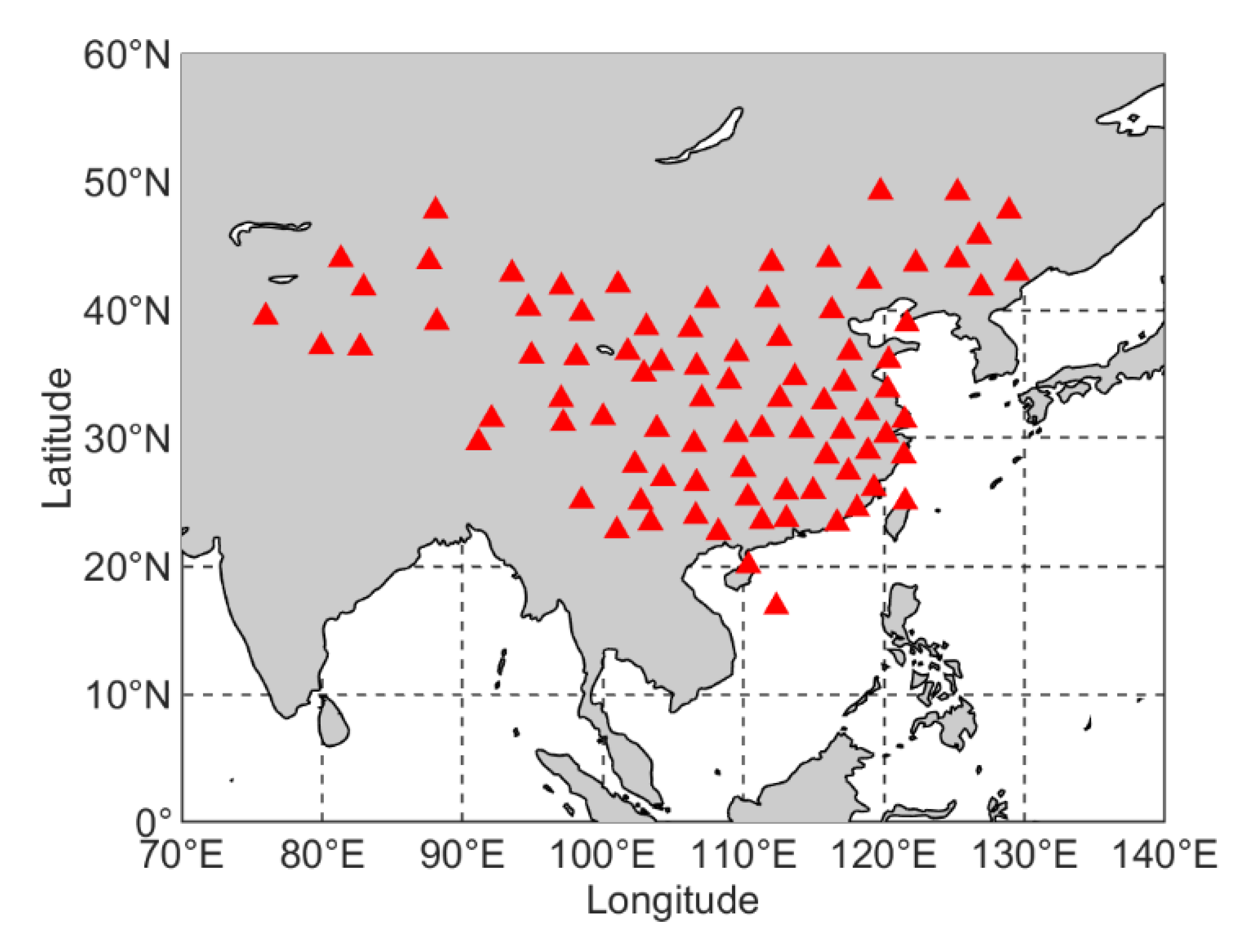
2.3. CMONOC Data
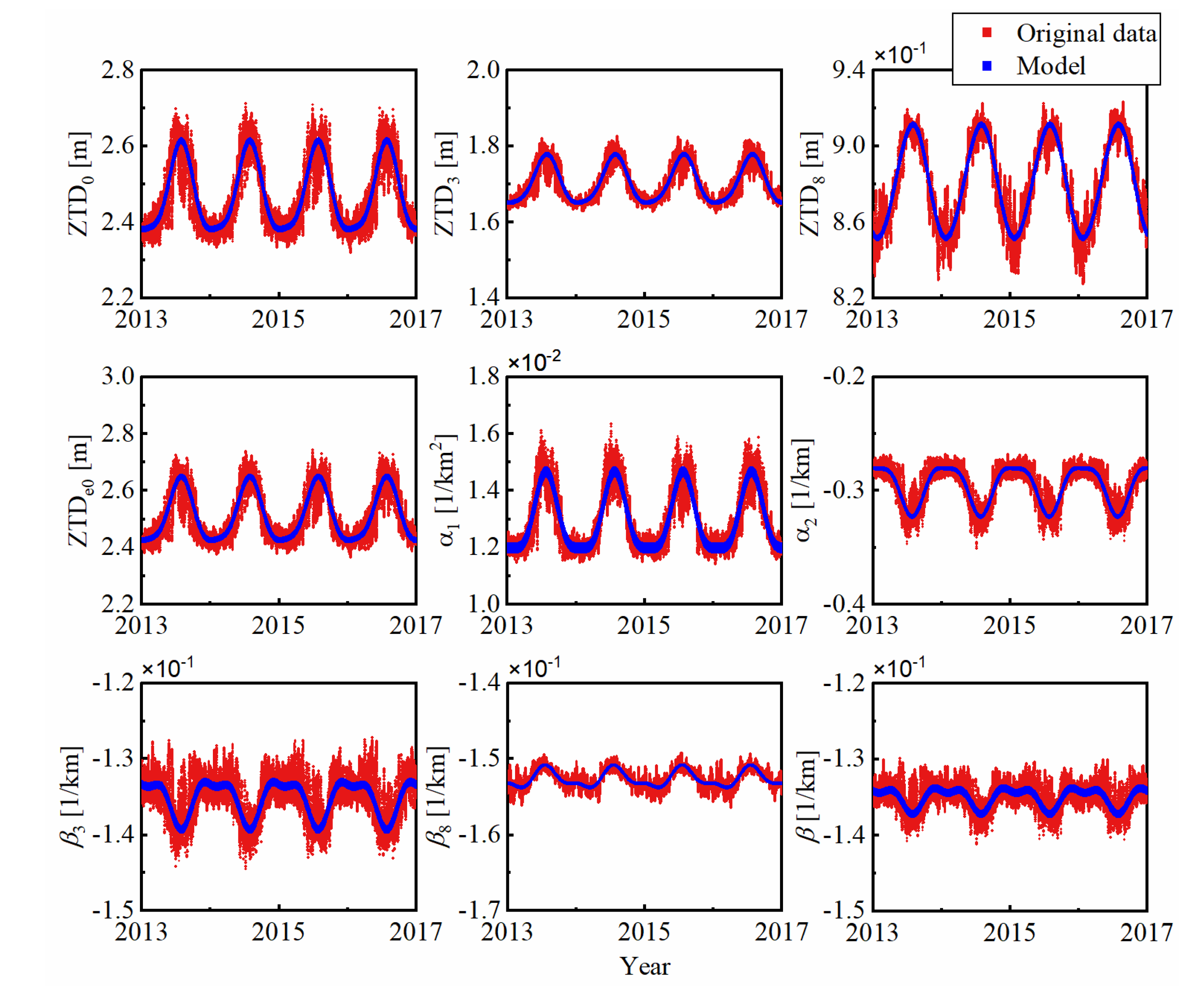
3. RGZTD Model Establishment
4. Model Validation
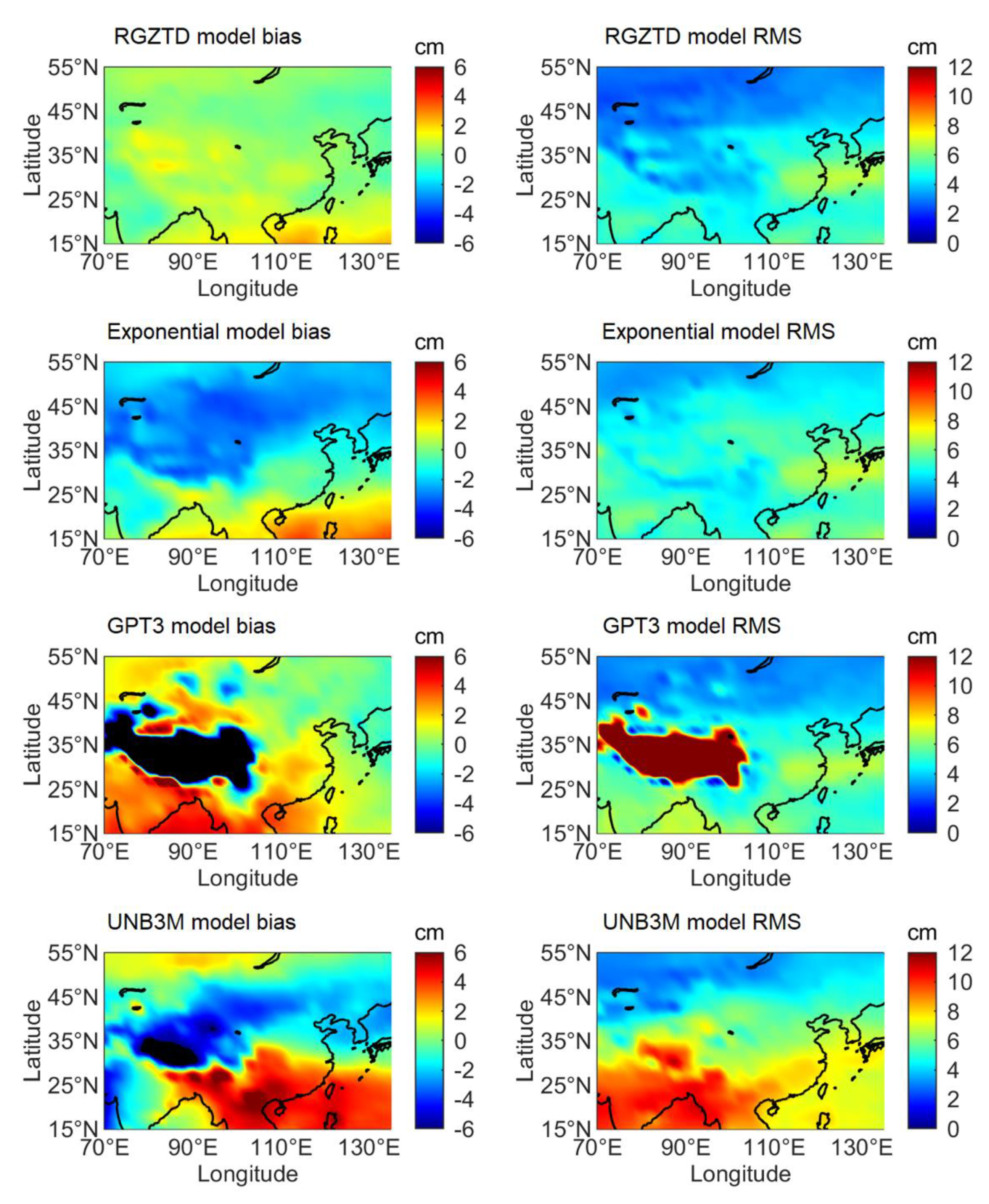
4.1. Accuracy Analysis Based on ERA5 ZTD Data
4.2. Accuracy Analysis Based on Radiosonde ZTD Data
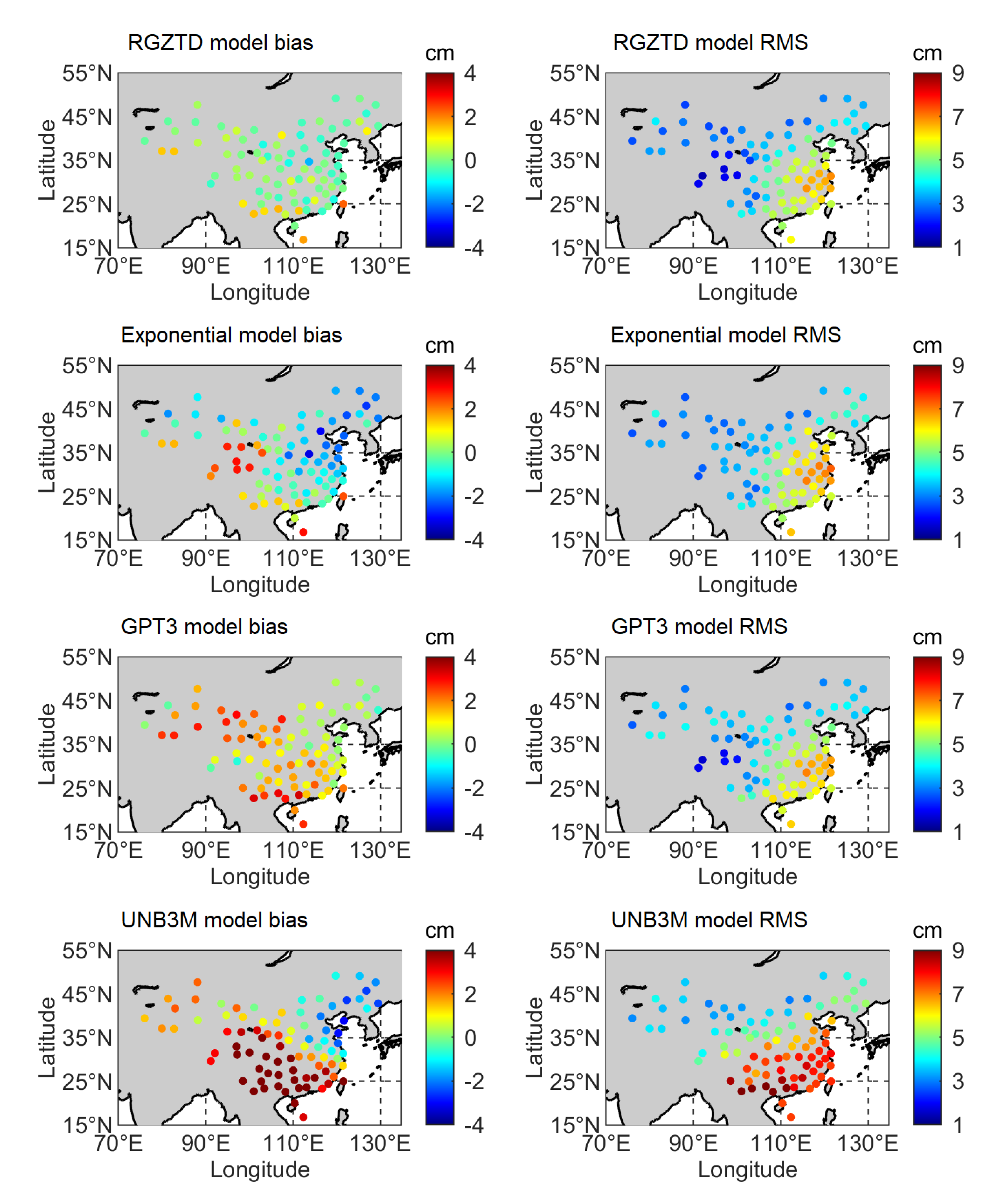
4.3. Accuracy Analysis Based on CMONOC Tropospheric Products
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Penna, N.; Dodson, A.; Chen, W. Assessment of EGNOS tropospheric correction model. J. Navig. 2001, 54, 37–55. [Google Scholar] [CrossRef]
- Zheng, F.; Lou, Y.; Gu, S.; Gong, X.; Shi, C. Modeling tropospheric wet delays with national GNSS reference network in China for BeiDou precise point positioning. J. Geod. 2018, 92, 545–560. [Google Scholar] [CrossRef]
- Black, H.D. An easily implemented algorithm for the tropospheric range correction. J. Geophys. Res. Solid Earth 1978, 83, 1825–1828. [Google Scholar] [CrossRef]
- Hopfield, H.S. Two-quartic tropospheric refractivity profile for correcting satellite data. J. Geophys. Res. (1896–1977) 1969, 74, 4487–4499. [Google Scholar] [CrossRef]
- Saastamoinen, J. Atmospheric correction for troposphere and stratosphere in radio ranging of satellites. In Proceedings of the 3rd Int Symp on the Use of Artificial Satellites for Geodesy, Washington, DC, USA, 15–17 April 1972. [Google Scholar]
- Collins, J.P.; Langley, R.B. A Tropospheric Delay Model for the User of the Wide Area Augmentation System; Department of Geodesy and Geomatics Engineering, University of New Brunswick: Fredericton, NB, Canada, 1997. [Google Scholar]
- Collins, P.; Langley, R.; LaMance, J. Limiting factors in tropospheric propagation delay error modelling for GPS airborne navigation. In Proceedings of the 52nd ION Annual Meeting, Cambridge, MA, USA, 19–21 June 1996; Volume 3. [Google Scholar]
- Ueno, M.; Hoshinoo, K.; Matsunaga, K.; Kawai, M.; Nakao, H.; Langley, R.B.; Bisnath, S.B. Assessment of atmospheric delay correction models for the Japanese MSAS. In Proceedings of the 14th International Technical Meeting of the Satellite Division of The Institute of Navigation (ION GPS 2001), Salt Lake City, UT, USA, 11–14 September 2001; pp. 2341–2350. [Google Scholar]
- Li, W.; Yuan, Y.; Ou, J.; He, Y. IGGtrop_SH and IGGtrop_rH: Two Improved Empirical Tropospheric Delay Models Based on Vertical Reduction Functions. IEEE Trans. Geosci. Remote Sens. 2018, 56, 5276–5288. [Google Scholar] [CrossRef]
- Li, W.; Yuan, Y.; Ou, J.; Li, H.; Li, Z. A new global zenith tropospheric delay model IGGtrop for GNSS applications. Chin. Sci. Bull. 2012, 57, 2132–2139. [Google Scholar] [CrossRef]
- Yao, Y.; Hu, Y.; Yu, C.; Zhang, B.; Guo, J. An improved global zenith tropospheric delay model GZTD2 considering diurnal variations. Nonlinear Process. Geophys. 2016, 23, 127–136. [Google Scholar] [CrossRef]
- Yao, Y.-B.; He, C.-Y.; Zhang, B.; Xu, C.-Q. A new global zenith tropospheric delay model GZTD. Chin. J. Geophys. Chin. Ed. 2013, 56, 2218–2227. [Google Scholar] [CrossRef]
- Schueler, T. The TropGrid2 standard tropospheric correction model. Gps Solut. 2014, 18, 123–131. [Google Scholar] [CrossRef]
- Dousa, J.; Elias, M. An improved model for calculating tropospheric wet delay. Geophys. Res. Lett. 2014, 41, 4389–4397. [Google Scholar] [CrossRef]
- Sun, J.; Wu, Z.; Yin, Z.; Ma, B. A simplified GNSS tropospheric delay model based on the nonlinear hypothesis. Gps Solut. 2017, 21, 1735–1745. [Google Scholar] [CrossRef]
- Chen, P.; Ma, Y.; Liu, H.; Zheng, N. A New Global Tropospheric Delay Model Considering the Spatiotemporal Variation Characteristics of ZTD With Altitude Coefficient. Earth Space Sci. 2020, 7. [Google Scholar] [CrossRef]
- Li, L.; Xu, Y.; Yan, L.; Wang, S.; Liu, G.; Liu, F. A Regional NWP Tropospheric Delay Inversion Method Based on a General Regression Neural Network Model. Sensors 2020, 20, 3167. [Google Scholar] [CrossRef] [PubMed]
- Charoenphon, C.; Satirapod, C. Improving the accuracy of real-time precipitable water vapour using country-wide meteorological model with precise point positioning in Thailand. J. Spat. Sci. 2020. [Google Scholar] [CrossRef]
- Chen, J.; Wang, J.; Wang, A.; Ding, J.; Zhang, Y. SHAtropE-A Regional Gridded ZTD Model for China and the Surrounding Areas. Remote Sens. 2020, 12, 165. [Google Scholar] [CrossRef]
- Boehm, J.; Moeller, G.; Schindelegger, M.; Pain, G.; Weber, R. Development of an improved empirical model for slant delays in the troposphere (GPT2w). GPS Solut. 2015, 19, 433–441. [Google Scholar] [CrossRef]
- Landskron, D.; Boehm, J. VMF3/GPT3: Refined discrete and empirical troposphere mapping functions. J. Geod. 2018, 92, 349–360. [Google Scholar] [CrossRef]
- Albergel, C.; Dutra, E.; Munier, S.; Calvet, J.-C.; Munoz-Sabater, J.; de Rosnay, P.; Balsamo, G. ERA-5 and ERA-Interim driven ISBA land surface model simulations: Which one performs better? Hydrol. Earth Syst. Sci. 2018, 22, 3515–3532. [Google Scholar] [CrossRef]
- Hersbach, H.; Dee, D. ERA5 Reanalysis Is in Production. Available online: https://www.ecmwf.int/en/newsletter/147/news/era5-reanalysis-production (accessed on 17 October 2020).
- Ge, S. GPS Radio Occultation and the Role of Atmospheric Pressure on Spaceborne Gravity Estimation over Antarctica. Ph.D. Thesis, The Ohio State University, Columbus, OH, USA, 2006. [Google Scholar]
- Salamon, S.J.; Hansen, H.J.; Abbott, D. Modelling radio refractive index in the atmospheric surface layer. In Electronics Letters; Institution of Engineering and Technology: London, UK, 2015; Volume 51, pp. 1119–1121. [Google Scholar]
- Bean, B.R.; Thayer, G.D. Models of the Atmospheric Radio Refractive Index. Proc. IRE 1959, 47, 740–755. [Google Scholar] [CrossRef]
- Askne, J.; Nordius, H. Estimation of tropospheric delay for microwaves from surface weather data. Radio Sci. 1987, 22, 379–386. [Google Scholar] [CrossRef]
- Huang, L.; Jiang, W.-P.; Liu, L.; Chen, H.; Ye, S. A new global grid model for the determination of atmospheric weighted mean temperature in GPS precipitable water vapor. J. Geod. 2018. [Google Scholar] [CrossRef]



| Mean of Bias | Mean of RMS | |
|---|---|---|
| RGZTD model | 0.38 | 4.18 |
| Exponential model | −1.24 | 4.83 |
| GPT3 model | −1.52 | 7.11 |
| UNB3M model | 0.04 | 6.39 |
| Mean of Bias | Mean of RMS | |
|---|---|---|
| RGZTD model | 0.07 | 4.19 |
| Exponential model | −0.32 | 4.51 |
| GPT3 model | 1.30 | 4.46 |
| UNB3M model | 1.89 | 6.02 |
| Mean of Bias | Mean of RMS | |
|---|---|---|
| RGZTD model | −0.81 | 3.76 |
| Exponential model | −0.97 | 4.01 |
| GPT3 model | 0.42 | 3.74 |
| UNB3M model | 1.31 | 5.42 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, L.; Gao, J.; Zhu, D.; Zheng, N.; Li, Z. Improved Zenith Tropospheric Delay Modeling Using the Piecewise Model of Atmospheric Refractivity. Remote Sens. 2020, 12, 3876. https://doi.org/10.3390/rs12233876
Yang L, Gao J, Zhu D, Zheng N, Li Z. Improved Zenith Tropospheric Delay Modeling Using the Piecewise Model of Atmospheric Refractivity. Remote Sensing. 2020; 12(23):3876. https://doi.org/10.3390/rs12233876
Chicago/Turabian StyleYang, Liu, Jingxiang Gao, Dantong Zhu, Nanshan Zheng, and Zengke Li. 2020. "Improved Zenith Tropospheric Delay Modeling Using the Piecewise Model of Atmospheric Refractivity" Remote Sensing 12, no. 23: 3876. https://doi.org/10.3390/rs12233876
APA StyleYang, L., Gao, J., Zhu, D., Zheng, N., & Li, Z. (2020). Improved Zenith Tropospheric Delay Modeling Using the Piecewise Model of Atmospheric Refractivity. Remote Sensing, 12(23), 3876. https://doi.org/10.3390/rs12233876







