Strain Pattern and Kinematics of the Canary Islands from GNSS Time Series Analysis
Abstract
1. Introduction
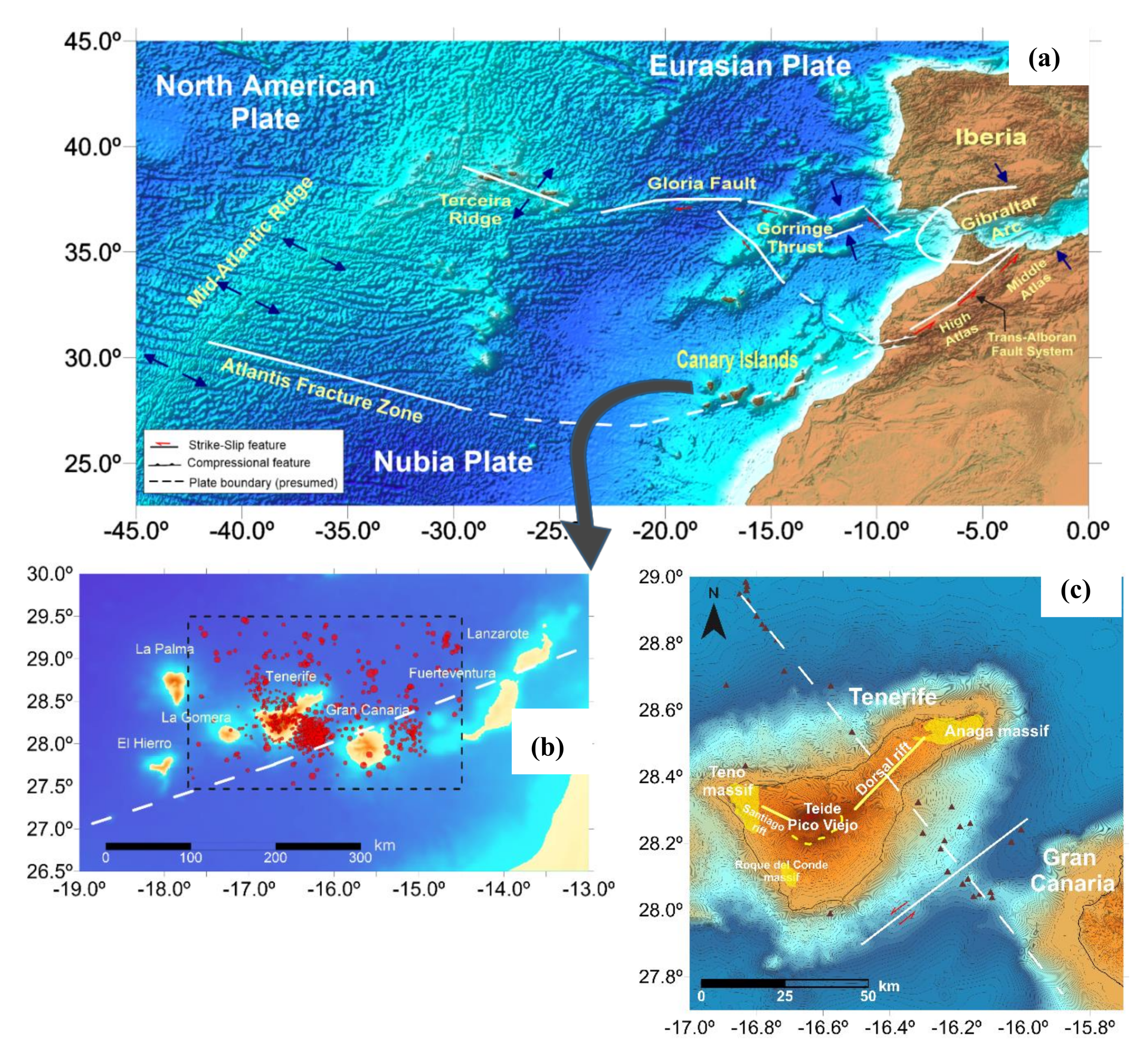
2. Geodynamic and Tectonic Context
3. Analysis of GNSS Data
- (1)
- Residuals-Stacking-Filtering: for each daily solution (d) and GNSS site (s), the residual (r) [observed (O)-predicted value (C)] is computed:
- (2)
- Computation of the common mode by means of stacking, by averaging the residuals from all the sites (S) in the network:Equation (2) represents the common-mode bias, which, in principle, should improve with the number of sites.
- (3)
- Filtering: for each site, the common-mode bias is subtracted from the observed position, resulting in a filtered position:
- (1)
- As the study area is small, the sphericity of Earth is neglected, and the Earth’s surface is approximated by a flat surface model. Hence, plane-strain deformation remains in the horizontal plane.
- (2)
- Considering the principles of mechanics, we assume that the deformation of networks reflects the real movements of Earth’s crust and that the area between geodetic points could be considered as a continuous medium in which the strain is homogeneously distributed across the triangular area between the three GNSS sites.
- (3)
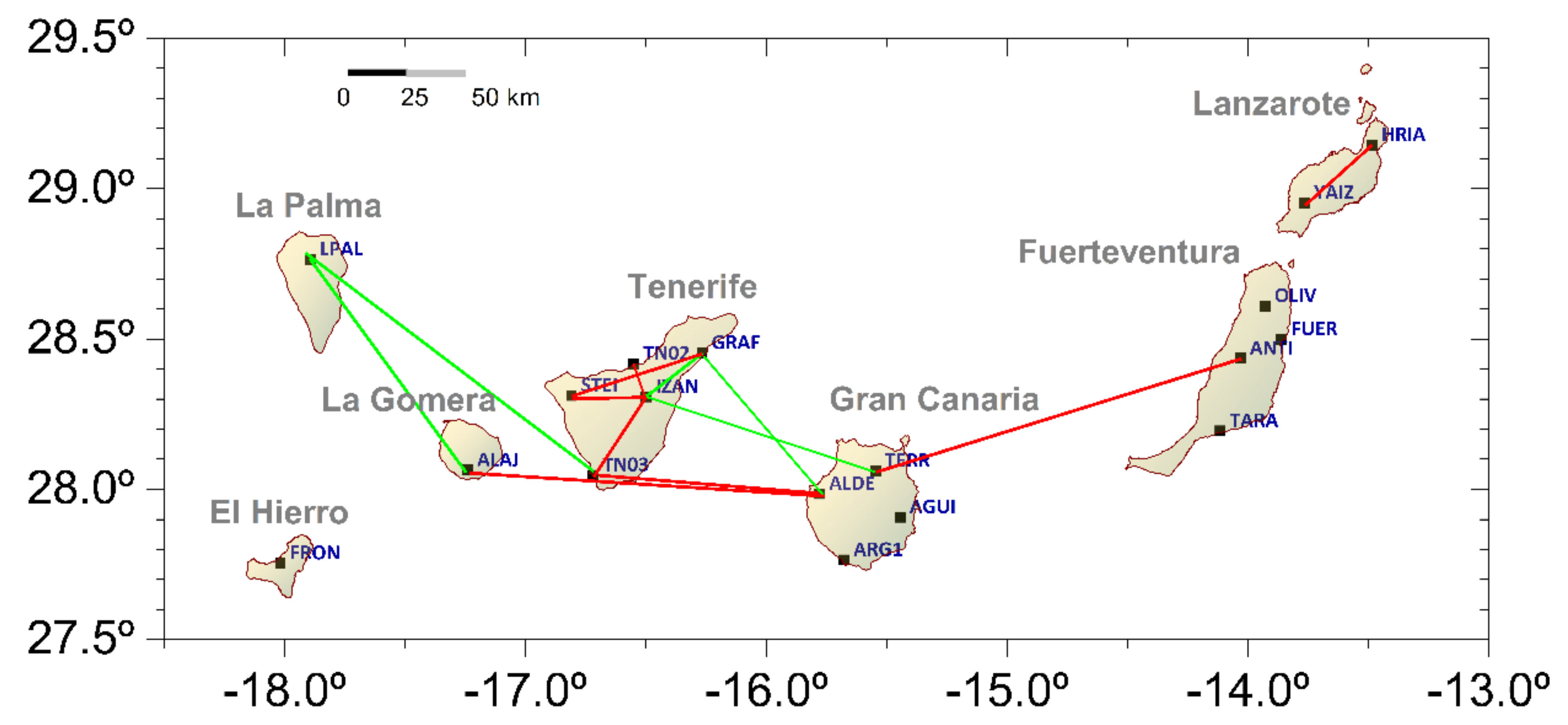
- Vertical velocities do not significantly affect the physical interpretation of the strain. Even if the latter assumption above is naive in areas displaying significant uplift or subsidence, in our case, stations with negligible vertical displacement (<3 mm/year) have been selected; hence, we are confident that 2D strain can provide useful insights into ground deformation patterns. The parameters of the strain were calculated from displacements () estimated by a constant velocity for the whole study period (6.7 years between 2011 and 2017). The velocities measured at the three GNSS sites are the result of the three components of crustal velocity: translation, rotation and distortion. For each of the GNSS sites, we know the horizontal coordinates of the initial site location (xo, yo) as well as the east–west and north–south instantaneous velocities (Vx, Vy). We solve for the “3-site triangle strain” problem, which is a well-known perfectly constrained problem [43] (p. 157). The principal strain axes are found by computing the eigenvectors of the 2-D strain tensor . The eigenvectors are unit vectors in the directions of the principal strain axes. The eigenvalues of are the principal extensions in the principal directions. The larger of the two eigenvalues are the greater principal extension, ε1, and the length of the semi-major axis of the horizontal strain ellipse is equal to the greater principal strain, S1 = ε1 + 1. The semi-minor axis is S2 = ε2 + 1, where ε2 is the lesser principal extension. For each triangle, we compute the strain in terms of elongations (strain unit) and resulting dilatation (mean strain), the latter of which is referred to as the triangle centroid. The main strain parameters for each triangle are listed in Table 2. Included in the Supplementary Materials is Figure S1, which illustrates the geometric configuration of each triangle.
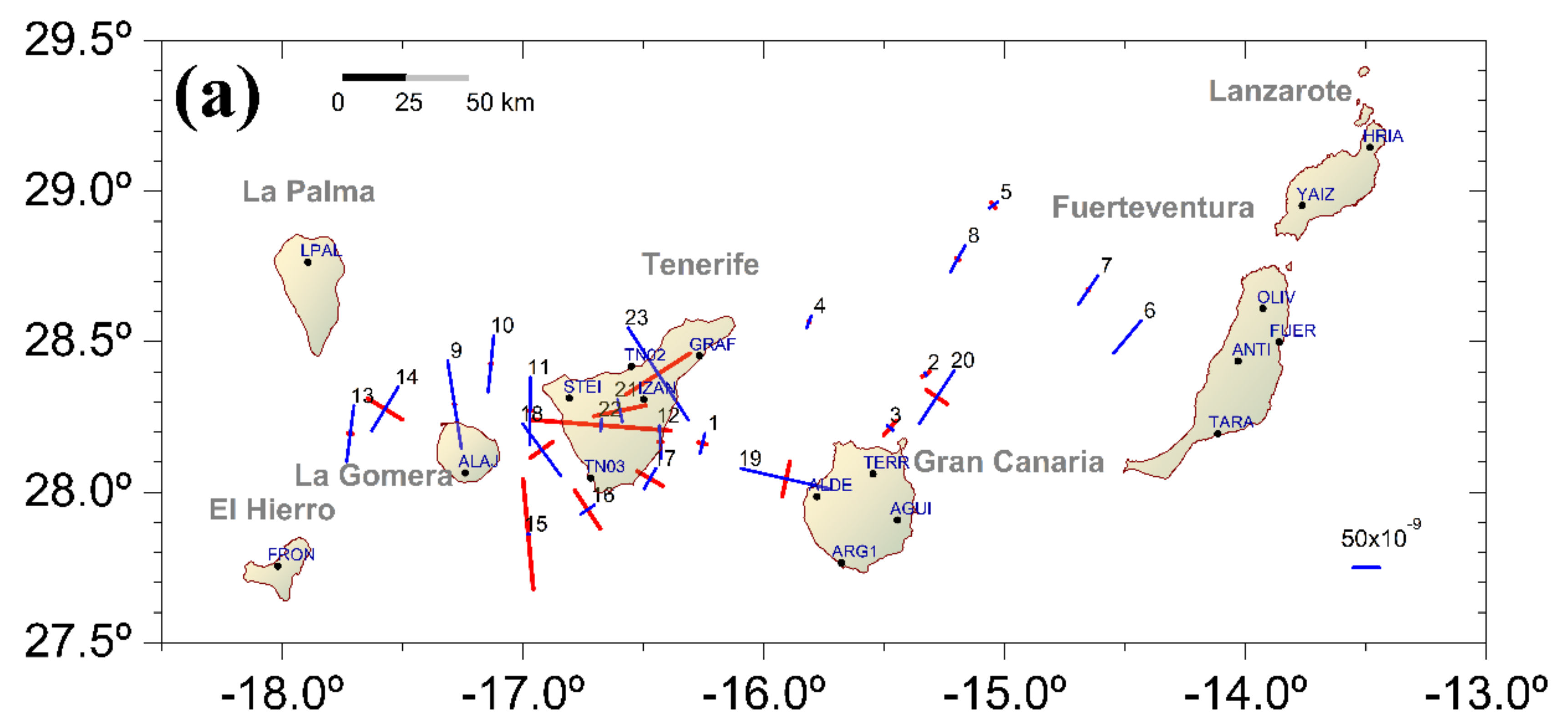
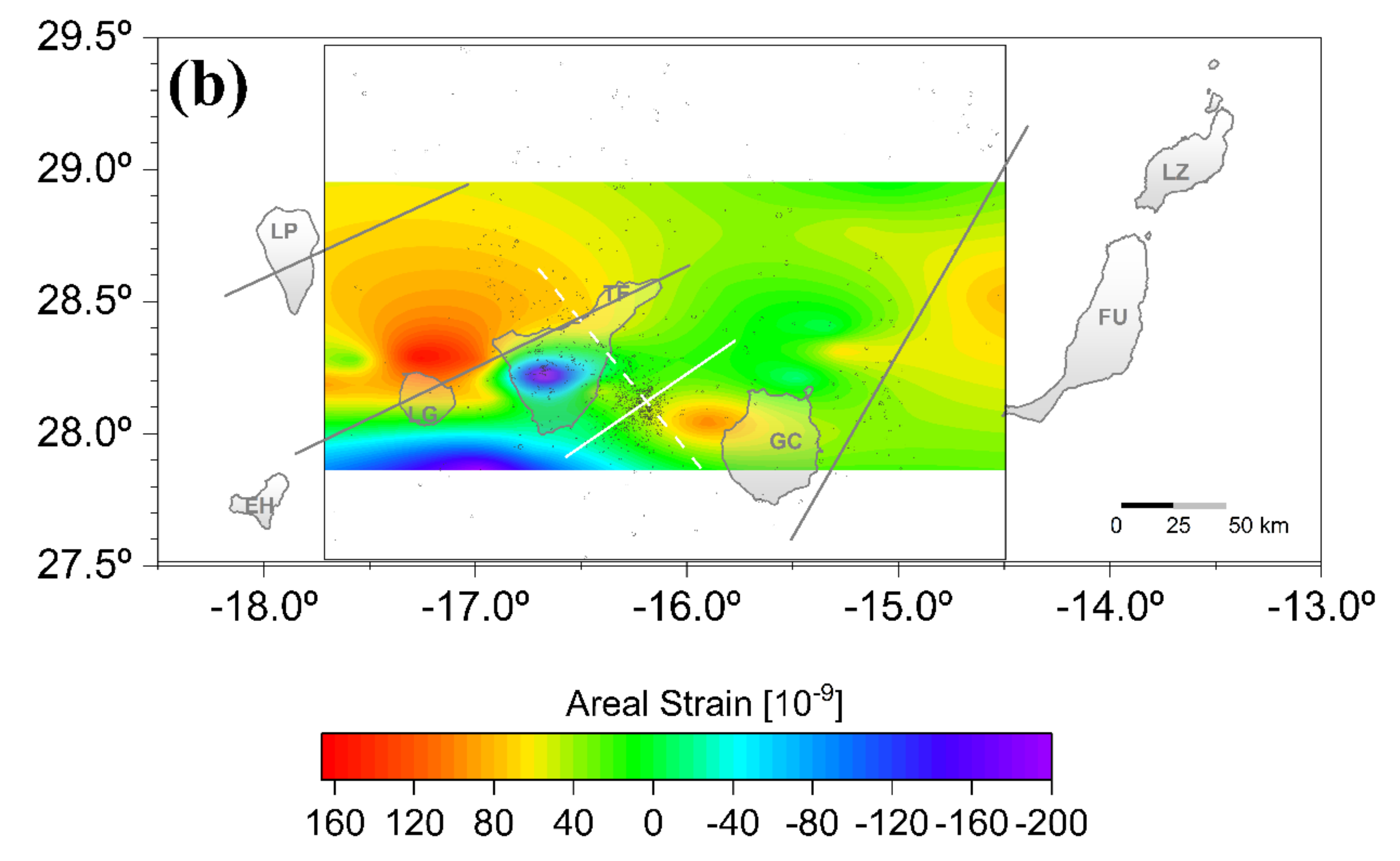
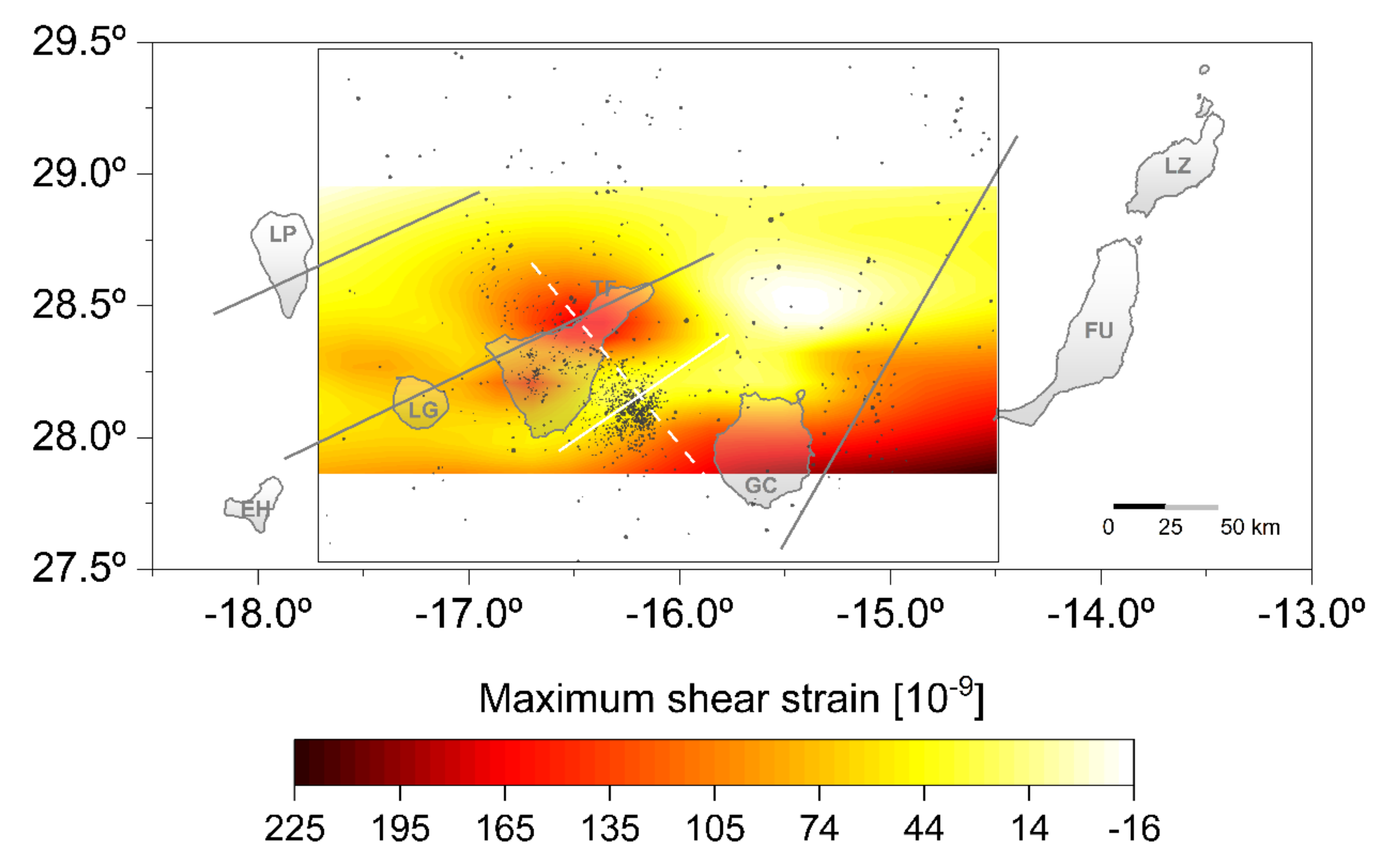
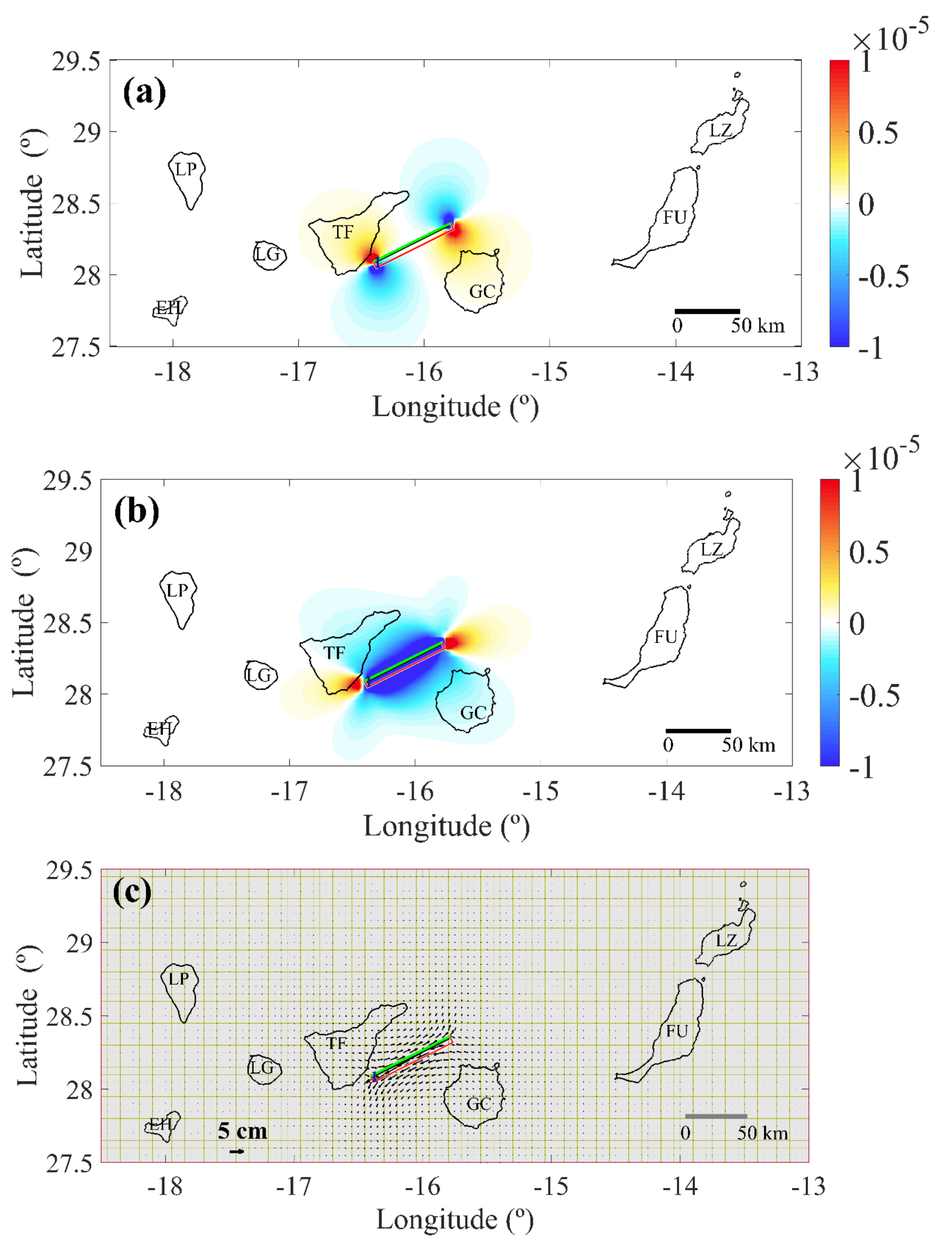
4. Results
5. Discussion
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Chousianitis, K.; Ganas, A.; Evangelidis, C.P. Strain and rotation rate patterns of mainland Greece from continuous GPS data and comparison between seismic and geodetic moment release. J. Geophys. Res. Solid Earth 2015, 120, 3909–3931. [Google Scholar] [CrossRef]
- Savage, J.C. Euler-Vector Clustering of GPS Velocities Defines Microplate Geometry in Southwest Japan. J. Geophys. Res. Solid Earth 2018, 123, 1954–1968. [Google Scholar] [CrossRef]
- Tzanis, A.; Chailas, S.; Sakkas, V.; Lagios, E. Tectonic deformation in the Santorini volcanic complex (Greece) as inferred by joint analysis of gravity, magnetotelluric and DGPS observations. Geophys. J. Int. 2019, 220, 461–489. [Google Scholar] [CrossRef]
- Haines, A.J.; Wallace, L.M. New Zealand-Wide Geodetic Strain Rates Using a Physics-Based Approach. Geophys. Res. Lett. 2020, 47, e2019GL084606. [Google Scholar] [CrossRef]
- Bastos, L.; Bos, M.S.; Fernandes, R.M. Deformation and Tectonics: Contribution of GPS Measurements to Plate Tectonics—Overview and Recent Developments. In Sciences of Geodesy-I; Xu, G., Ed.; Springer: Berlin/Heidelberg, Germany, 2010; pp. 155–184. [Google Scholar] [CrossRef]
- Blewitt, G.; Hammond, W.C.; Kreemer, C. Harnessing the GPS Data Explosion for Interdisciplinary Science. Eos 2018, 99, 99. [Google Scholar] [CrossRef]
- Larson, K.M. Unanticipated Uses of the Global Positioning System. Annu. Rev. Earth Planet. Sci. 2019, 47, 19–40. [Google Scholar] [CrossRef]
- Wdowinski, S.; Bock, Y.; Zhang, J.; Fang, P.; Genrich, J. Southern California permanent GPS geodetic array: Spatial filtering of daily positions for estimating coseismic and postseismic displacements induced by the 1992 Landers earthquake. J. Geophys. Res. Space Phys. 1997, 102, 18057–18070. [Google Scholar] [CrossRef]
- Martin, A.; Sevilla, M.; Zurutuza, J. Crustal deformation study in the Canary Archipelago by the analysis of GPS observations. J. Appl. Geodesy 2014, 8, 129–140. [Google Scholar] [CrossRef]
- López, C.; García-Cañada, L.; Martí, J.; Cerdeña, I.D. Early signs of geodynamic activity before the 2011–2012 El Hierro eruption. J. Geodyn. 2017, 104, 1–14. [Google Scholar] [CrossRef][Green Version]
- Fernández, J.; Yu, T.-T.; Rodríguez-Velasco, G.; González-Matesanz, J.; Romero, R.; Rodríguez, G.; Quiros, R.; Dalda, A.; Aparicio, A.; Blanco, M. New geodetic monitoring system in the volcanic island of Tenerife, Canaries, Spain. Combination of InSAR and GPS techniques. J. Volcanol. Geotherm. Res. 2003, 124, 241–253. [Google Scholar] [CrossRef]
- Berrocoso, M.; Carmona, J.; Fernandez-Ros, A.; Perez-Pena, A.; Ortiz, R.; García, A. Kinematic model for Tenerife Island (Canary Islands, Spain): Geodynamic interpretation in the Nubian plate context. J. Afr. Earth Sci. 2010, 58, 721–733. [Google Scholar] [CrossRef]
- García, A.; Fernández-Ros, A.; Berrocoso, M.; Marrero, J.M.; Prates, G.; De La Cruz-Reyna, S.; Ortiz, R. Magma displacements under insular volcanic fields, applications to eruption forecasting: El Hierro, Canary Islands, 2011–2013. Geophys. J. Int. 2014, 197, 322–334. [Google Scholar] [CrossRef]
- Riccardi, U.; Arnoso, J.; Benavent, M.; Velez, E.; Tammaro, U.; Montesinos, F.G. Exploring deformation scenarios in Timanfaya volcanic area (Lanzarote, Canary Islands) from GNSS and ground based geodetic observations. J. Volcanol. Geotherm. Res. 2018, 357, 14–24. [Google Scholar] [CrossRef]
- Barbero, I.; Torrecillas, C.; Prates, G.; Páez, R.; Garate, J.; García, A.; Berrocoso, M. Assessment of ground deformation following Tenerife’s 2004 volcanic unrest (Canary Islands). J. Geodyn. 2018, 121, 1–8. [Google Scholar] [CrossRef]
- Alzola, A.S.; Martí, J.; García-Yeguas, A.; Gil, A.J. Subsidence and current strain patterns on Tenerife Island (Canary Archipelago, Spain) derived from continuous GNSS time series (2008–2015). J. Volcanol. Geotherm. Res. 2016, 327, 240–248. [Google Scholar] [CrossRef]
- Ancochea, E.; Brändle, J.; Cubas, C.; Hernan, F.; Huertas, M. Volcanic complexes in the eastern ridge of the Canary Islands: the Miocene activity of the island of Fuerteventura. J. Volcanol. Geotherm. Res. 1996, 70, 183–204. [Google Scholar] [CrossRef]
- Carracedo, J.C.; Pérez Torrado, F.J.; Ancochea, E.; Meco, J.; Hernán, F.; Cubas, C.R.; Casillas, R.; Rodríguez Badiola, E.; Ahijado, A. Cenozoic volcanism II: The Canary Islands. In The Geology of Spain; Gibbons, W., Moreno, T., Eds.; Geological Society London: London, UK, 2002; p. 632. [Google Scholar]
- Watts, A.B.; Peirce, C.; Collier, J.S.; Dalwood, R.; Canales, J.P.; Henstock, T.J. A seismic study of lithospheric flexure in the vicinity of Tenerife, Canary Islands. Earth Planet. Sci. Lett. 1997, 146, 431–447. [Google Scholar] [CrossRef]
- Schmincke, H.U. Volcanism; Springer: Berlin/Heidelberg, Germany, 2004; p. 324. [Google Scholar] [CrossRef]
- Llanes, P. Estructura de la Litosfera en el Entorno de las Islas Canarias a Partir del Análisis Gravimétrico e Isostático: Implicaciones Geodinámicas. Ph.D. Thesis, Univ. Complutense of Madrid, Madrid, Spain, 2006; p. 195. [Google Scholar]
- Troll, V.R.; Carracedo, J.C. The Geology of the Canary Islands; Elsevier BV: Amsterdam, The Netherlands, 2016; p. 636. [Google Scholar]
- Carracedo, J.C. Growth, structure, instability and collapse of Canarian volcanoes and comparisons with Hawaiian volcanoes. J. Volcanol. Geotherm. Res. 1999, 94, 1–19. [Google Scholar] [CrossRef]
- Mantovani, E.; Viti, M.; Babbucci, D.; Albarello, D. Nubia-Eurasia kinematics: An alternative interpretation from Mediterranean and North Atlantic evidence. Ann Geophys. 2007, 50, 341–366. [Google Scholar] [CrossRef]
- Ruiz, C.; García-Cacho, L.; Arana, V.; Luque, A.; Felpeto, A. Submarine volcanism surrounding Tenerife, Canary Islands: Implications for tectonic controls, and oceanic shield forming processes. J. Volcanol. Geotherm. Res. 2000, 103, 105–119. [Google Scholar] [CrossRef]
- Anguita, F.; Hernan, F. The Canary Islands origin: A unifying model. J. Volcanol. Geotherm. Res. 2000, 103, 1–26. [Google Scholar] [CrossRef]
- Anguita, F.; Hernan, F. A propagating fracture model versus a hot spot origin for the Canary islands. Earth Planet. Sci. Lett. 1975, 27, 11–19. [Google Scholar] [CrossRef]
- Bosshard, E.; Macfarlane, D.J. Crustal structure of the western Canary Islands from seismic refraction and gravity data. J. Geophys. Res. Space Phys. 1970, 75, 4901–4918. [Google Scholar] [CrossRef]
- Zhao, D.; Lei, J.; Inoue, T.; Yamada, A.; Gao, S.S. Deep structure and origin of the Baikal rift zone. Earth Planet. Sci. Lett. 2006, 243, 681–691. [Google Scholar] [CrossRef]
- Fourel, L.; Milelli, L.; Jaupart, C.; Limare, A. Generation of continental rifts, basins, and swells by lithosphere instabilities. J. Geophys. Res. Solid Earth 2013, 118, 3080–3100. [Google Scholar] [CrossRef]
- Duggen, S.; Hoernle, K.; Hauff, F.; Kluegel, A.; Bouabdellah, M.; Thirlwall, M.F. Flow of Canary mantle Plume material through a subcontinental lithospheric corridor beneath Africa to the Mediterranean: REPLY. Geology 2010, 38, e203. [Google Scholar] [CrossRef]
- Geyer, A.; Marti, J.; Villaseñor, A. First-order estimate of the Canary Islands plate-scale stress field: Implications for volcanic hazard assessment. Tectonophysics 2016, 679, 125–139. [Google Scholar] [CrossRef]
- Mezcua, J.; Buforn, E.; Udias, A.; Rueda, J. Seismotectonics of the Canary Islands. Tectonophysics 1992, 208, 447–452. [Google Scholar] [CrossRef]
- Del Fresno, C.; Cerdeña, I.D.; Cesca, S.; Buforn, E. The 8 October 2011 Earthquake at El Hierro (Mw 4.0): Focal Mechanisms of the Mainshock and Its Foreshocks. Bull. Seism. Soc. Am. 2014, 105, 330–340. [Google Scholar] [CrossRef]
- Telesca, L.; Lovallo, M.; López, C.; Molist, J.M. Multiparametric statistical investigation of seismicity occurred at El Hierro (Canary Islands) from 2011 to 2014. Tectonophysics 2016, 672, 121–128. [Google Scholar] [CrossRef]
- Jiménez-Munt, I.; Fernández, M.; Torne, M.; Bird, P.; Torne, M. The transition from linear to diffuse plate boundary in the Azores–Gibraltar region: Results from a thin-sheet model. Earth Planet. Sci. Lett. 2001, 192, 175–189. [Google Scholar] [CrossRef]
- Jiménez-Munt, I.; Negredo, A.M. Neotectonic modelling of the western part of the Africa–Eurasia plate boundary: From the Mid-Atlantic ridge to Algeria. Earth Planet. Sci. Lett. 2003, 205, 257–271. [Google Scholar] [CrossRef]
- Blanco-Montenegro, I.; Nicolosi, I.; Pignatelli, A.; García, A.; Chiappini, M. New evidence about the structure and growth of ocean island volcanoes from aeromagnetic data: The case of Tenerife, Canary Islands. J. Geophys. Res. Space Phys. 2011, 116. [Google Scholar] [CrossRef]
- Blanco-Montenegro, I.; Montesinos, F.G.; Arnoso, J. Aeromagnetic anomalies reveal the link between magmatism and tectonics during the early formation of the Canary Islands. Sci. Rep. 2018, 8, 42. [Google Scholar] [CrossRef]
- Blanco-Montenegro, I.; Montesinos, F.G.; Nicolosi, I.; Arnoso, J.; Chiappini, M. Three-Dimensional Magnetic Models of La Gomera (Canary Islands): Insights Into the Early Evolution of an Ocean Island Volcano. Geochem. Geophys. Geosystems 2020, 21, 2019gc008787. [Google Scholar] [CrossRef]
- Kreemer, C.; Blewitt, G.; Klein, E.C. A geodetic plate motion and Global Strain Rate Model. Geochem. Geophys. Geosyst. 2014, 15, 3849–3889. [Google Scholar] [CrossRef]
- Moritz, H. Advanced Least-Square Methods. Reports of the Department of Geodetic Science; Report 175; Ohio State University: Columbus, OH, USA, 1972; p. 129. [Google Scholar]
- Allmendinger, R.W.; Cardozo, N.; Fisher, D.M. Structural Geology Algorithms; Cambridge University Press (CUP): Cambridge, UK, 2011. [Google Scholar]
- Shen, Z.-K.; Jackson, D.D.; Ge, B.X. Crustal deformation across and beyond the Los Angeles basin from geodetic measurements. J. Geophys. Res. Space Phys. 1996, 101, 27957–27980. [Google Scholar] [CrossRef]
- Wu, Y.; Jiang, Z.-S.; Yang, G.; Wei, W.; Liu, X. Comparison of GPS strain rate computing methods and their reliability. Geophys. J. Int. 2011, 185, 703–717. [Google Scholar] [CrossRef]
- Szafarczyk, A.; Ulmaniec, M.; Borowiec, W. An attempt to apply tensor calculus to evaluate the deformation condition of vertical upper embankment zones for a landfill located in a mining area, based on satellite measurement results. Rep. Geod. 2007, 82, 317–326. [Google Scholar]
- Tammaro, U.; DeMartino, P.; Obrizzo, F.; Brandi, G.; D’Alessandro, A.; Dolce, M.; Malaspina, S.; Serio, C.; Pingue, F. Somma Vesuvius volcano: Ground deformations from CGPS observations (2001–2012). Ann. Geophys. 2013, 56, S0456. [Google Scholar] [CrossRef]
- Araszkiewicz, A. Reference Frame Realization Impact on Network Deformation—Geodynamic Research in Tectonic Stable Areas. In Proceedings of the 14th SGEM GeoConference on Informatics, Geoinformatics and Remote Sensing, Albena, Bulgaria, 17–26 June 2014; STEF92 Technology Ltd.: Sofia, Bulgaria, 2014; Volume 2, pp. 427–434. [Google Scholar]
- Segall, P. Earthquake and Volcano Deformation; Walter de Gruyter GmbH: Berlin, Germany, 2010; p. 432. [Google Scholar]
- Luzum, B.; Petit, G. The IERS Conventions (2010): Reference systems and new models. Proc. Int. Astron. Union 2012, 10, 227–228. [Google Scholar] [CrossRef]
- Lyard, F.; Lefevre, F.; Letellier, T.; Francis, O. Modelling the global ocean tides: Modern insights from FES2004. Ocean. Dyn. 2006, 56, 394–415. [Google Scholar] [CrossRef]
- Altamimi, Z.; Rebischung, P.; Métivier, L.; Collilieux, X. ITRF2014: A new release of the International Terrestrial Reference Frame modeling nonlinear station motions. J. Geophys. Res. Solid Earth 2016, 121, 6109–6131. [Google Scholar] [CrossRef]
- Jaeger, J.C.; Cook, N.G.W.; Zimmerman, R.W. Fundamentals of Rock Mechanics, 4th ed.; Blackwell Publishing Ltd.: Hoboken, NJ, USA, 2007; ISBN 13: 978-0-632-05759-7. [Google Scholar]
- DeMets, C.; Gordon, R.G.; Vogt, P. Location of the Africa-Australia-India Triple Junction and Motion between the Australian and Indian Plates: Results from an Aeromagnetic Investigation of the Central Indian and Carlsberg Ridges. Geophys. J. Int. 1994, 119, 893–930. [Google Scholar] [CrossRef]
- González, P.J.; Fernández, J. Error estimation in multitemporal InSAR deformation time series, with application to Lanzarote, Canary Islands. J. Geophys. Res. Space Phys. 2011, 116. [Google Scholar] [CrossRef]
- Ancochea, E.; Fuster, J.; Ibarrola, E.; Cendrero, A.; Coello, J.; Hernan, F.; Cantagrel, J.M.; Jamond, C. Volcanic evolution of the island of Tenerife (Canary Islands) in the light of new K-Ar data. J. Volcanol. Geotherm. Res. 1990, 44, 231–249. [Google Scholar] [CrossRef]
- Fernández, J.; Tizzani, P.; Manzo, M.; Borgia, A.; González, P.J.; Martí, J.; Pepe, A.; Camacho, A.G.; Casu, F.; Berardino, P.; et al. Gravity-driven deformation of Tenerife measured by InSAR time series analysis. Geophys. Res. Lett. 2009, 36, 04306. [Google Scholar] [CrossRef]
- De Vallejo, L.I.G.; García-Mayordomo, J.; Insua-Arévalo, J.M. Probabilistic Seismic-Hazard Assessment of the Canary Islands. Bull. Seism. Soc. Am. 2006, 96, 2040–2049. [Google Scholar] [CrossRef]
- García, A.; Ortiz, R.; Marrero, J.M.; Sánchez, N.; Vila, J.; Correig, A.M.; Marcià, R.; Sleeman, R.; Tárraga, M. Monitoring the reawakening of Canary Islands’ Teide Volcano. Eos 2006, 87, 61. [Google Scholar] [CrossRef]
- Gottsmann, J.; Wooller, L.; Marti, J.; Fernandez, J.; Camacho, A.G.; González, P.J.; García, A.; Rymer, H. New evidence for the reawakening of Teide volcano. Geophys. Res. Lett. 2006, 33, 20311. [Google Scholar] [CrossRef]
- Fernández, C.; De La Nuez, J.; Casillas, R.; García-Navarro, E. Stress fields associated with the growth of a large shield volcano (La Palma, Canary Islands). Tectonics 2002, 21, 13-1–13-18. [Google Scholar] [CrossRef]
- Toda, S.; Stein, R.S.; Richards-Dinger, K.; Bozkurt, S.B. Forecasting the evolution of seismicity in southern California: Animations built on earthquake stress transfer. J. Geophys. Res. Space Phys. 2005, 110. [Google Scholar] [CrossRef]
- Lin, J.; Stein, R.S. Stress triggering in thrust and subduction earthquakes and stress interaction between the southern San Andreas and nearby thrust and strike-slip faults. J. Geophys. Res. Space Phys. 2004, 109. [Google Scholar] [CrossRef]
- Okada, Y. Internal deformation due to shear and tensile faults in a half-space. Bull. Seismol. Soc. Am. 1992, 82, 1018–1040. [Google Scholar]
- Hearn, E.H. What can GPS data tell us about the dynamics of post-seismic deformation? Geophys. J. Int. 2003, 155, 753–777. [Google Scholar] [CrossRef]
- Ablay, G.J.; Palmer, M.R.; Carroll, M.; Marti, J.; Sparks, R.S.J. Basanite-Phonolite Lineages of the Teide-Pico Viejo Volcanic Complex, Tenerife, Canary Islands. J. Pet. 1998, 39, 905–936. [Google Scholar] [CrossRef]
- Ancochea, E.; Huertas, M.; Cantagrel, J.; Coello, J.; Fúster, J.; Arnaud, N.; Ibarrola, E. Evolution of the Cañadas edifice and its implications for the origin of the Cañadas Caldera (Tenerife, Canary Islands). J. Volcanol. Geotherm. Res. 1999, 88, 177–199. [Google Scholar] [CrossRef]
- Carracedo, J.C.; Badiola, E.R.; Guillou, H.; Paterne, M.; Scaillet, S.; Perez-Torrado, F.J.; Paris, R.; Fra-Paleo, U.; Hansen, A. Eruptive and structural history of Teide Volcano and rift zones of Tenerife, Canary Islands. GSA Bull. 2007, 119, 1027–1051. [Google Scholar] [CrossRef]
- Marti, J.; Geyer, A.; Andújar, J.; Teixidó, F.; Costa, F. Assessing the potential for future explosive activity from Teide–Pico Viejo stratovolcanoes (Tenerife, Canary Islands). J. Volcanol. Geotherm. Res. 2008, 178, 529–542. [Google Scholar] [CrossRef]
- Almendros, J.; Ibáñez, J.; Carmona, E.; Zandomeneghi, D. Array analyses of volcanic earthquakes and tremor recorded at Las Cañadas caldera (Tenerife Island, Spain) during the 2004 seismic activation of Teide volcano. J. Volcanol. Geotherm. Res. 2007, 160, 285–299. [Google Scholar] [CrossRef]
- Marti, J.; Ortiz, R.; Gottsmann, J.; García, A.; De La Cruz-Reyna, S. Characterising unrest during the reawakening of the central volcanic complex on Tenerife, Canary Islands, 2004–2005, and implications for assessing hazards and risk mitigation. J. Volcanol. Geotherm. Res. 2009, 182, 23–33. [Google Scholar] [CrossRef]
- Cerdeña, I.D.; Del Fresno, C.; Cantavella, J.V.; Felpeto, A.; Lozano, L.; Medina, L.C.; Torres, P.A.; Luengo-Oroz, N.; Solares, J.M.M.; Blanco, M.; et al. Comment on “Geochemical evidences of seismo-volcanic unrests at the NW rift-zone of Tenerife, Canary Islands, inferred from diffuse CO2 emission” by Hernández P. A., Padilla G., Barrancos J., Melián G., Padrón E., Asensio-Ramos M., Rodríguez F., Pérez N. M., Alonso M., and Calvo D. [Bull Volcanol (2017) 79:30]. Bull. Volcanol. 2017, 80, 1–4. [Google Scholar] [CrossRef]
- Gottsmann, J.; Camacho, A.G.; Marti, J.; Wooller, L.; Fernandez, J.; García, A.; Rymer, H. Shallow structure beneath the Central Volcanic Complex of Tenerife from new gravity data: Implications for its evolution and recent reactivation. Phys. Earth Planet. Inter. 2008, 168, 212–230. [Google Scholar] [CrossRef]
- Aparicio, S.S.-M.; Martí, J.; Montesinos, F.; Gómez, A.B.; De Pablo, J.P.; Fernández, P.V.; García-Maroto, M.C. Gravimetric study of the shallow basaltic plumbing system of Tenerife, Canary Islands. Phys. Earth Planet. Inter. 2019, 297, 106319. [Google Scholar] [CrossRef]
- Garcia-Yeguas, A.; Koulakov, I.; Ibáñez, J.; Rietbrock, A. High resolution 3D P wave velocity structure beneath Tenerife Island (Canary Islands, Spain) based on tomographic inversion of active-source data. J. Geophys. Res. Space Phys. 2012, 117, 09309. [Google Scholar] [CrossRef]
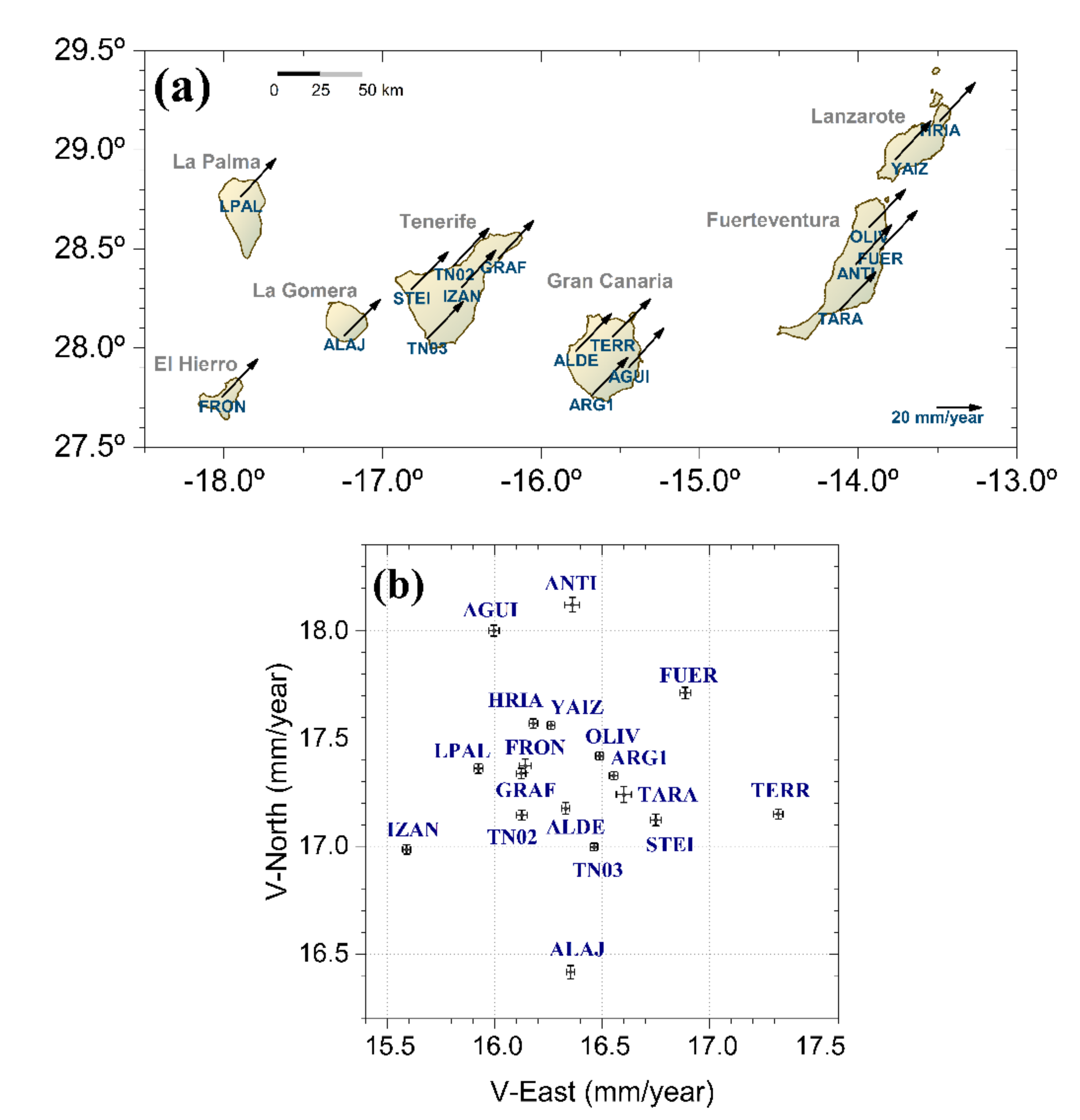
| Station | Island | Longitude (°) | Latitude (°) | Height (m) | Vx | SDVx | Vy | SDVy |
|---|---|---|---|---|---|---|---|---|
| FRON | El Hierro | −18.011 | 27.754 | 308.2 | 16.1 | 0.03 | 17.4 | 0.03 |
| LPAL | La Palma | −17.894 | 28.764 | 2199.2 | 16.0 | 0.02 | 17.9 | 0.03 |
| ALAJ | La Gomera | −17.241 | 28.064 | 899.1 | 16.6 | 0.02 | 16.9 | 0.03 |
| TN02 | Tenerife | −16.551 | 28.418 | 54.5 | 16.1 | 0.03 | 17.2 | 0.02 |
| TN03 | Tenerife | −16.719 | 28.047 | 58.5 | 16.5 | 0.02 | 17.0 | 0.02 |
| IZAN | Tenerife | −16.500 | 28.308 | 2417.5 | 16.0 | 0.02 | 17.0 | 0.02 |
| STEI | Tenerife | −16.816 | 28.298 | 988.6 | 16.8 | 0.02 | 17.1 | 0.03 |
| GRAF | Tenerife | −16.268 | 28.454 | 138.5 | 16.1 | 0.02 | 17.3 | 0.02 |
| ALDE | Gran Canaria | −15.780 | 27.985 | 122.4 | 16.3 | 0.02 | 17.2 | 0.03 |
| ARG1 | Gran Canaria | −15.681 | 27.761 | 67.8 | 16.5 | 0.02 | 17.4 | 0.02 |
| AGUI | Gran Canaria | −15.446 | 27.904 | 329.1 | 16.0 | 0.02 | 18.0 | 0.03 |
| TERR | Gran Canaria | −15.548 | 28.060 | 648.8 | 17.3 | 0.02 | 17.2 | 0.02 |
| OLIV | Fuerteventura | −13.928 | 28.610 | 275.0 | 16.6 | 0.02 | 17.3 | 0.02 |
| FUER | Fuerteventura | −13.860 | 28.499 | 76.8 | 16.9 | 0.03 | 17.7 | 0.03 |
| ANTI | Fuerteventura | −14.014 | 28.423 | 316.0 | 16.4 | 0.03 | 18.1 | 0.03 |
| TARA | Fuerteventura | −14.115 | 28.194 | 60.0 | 16.6 | 0.04 | 17.2 | 0.04 |
| HRIA | Lanzarote | −13.485 | 29.145 | 319.8 | 16.2 | 0.02 | 17.6 | 0.02 |
| YAIZ | Lanzarote | −13.766 | 28.952 | 233.5 | 16.3 | 0.02 | 17.7 | 0.02 |
| Triangles | Baseline | Elongation/Shortening | ε1 | θ1 (°) | ε2 | θ2 (°) | Dilatation | |
|---|---|---|---|---|---|---|---|---|
| 1 | ALDE-GRAF-TN03 | ALDE-GRAF | 24.92 | 37.49 | −13.0 | −15.54 | 77.0 | 21.96 |
| ALDE-TN03 | −10.53 | |||||||
| GRAF-TN03 | −0.79 | |||||||
| 2 | ALDE-HRIA-TN03 | ALDE-HRIA | 1.89 | 6.46 | 32.7 | −15.27 | −57.3 | −8.81 |
| ALDE-TN03 | −10.53 | |||||||
| HRIA-TN03 | −1.15 | |||||||
| 3 | ALDE-OLIV-TN03 | ALDE-OLIV | 9.28 | 15.21 | 48.8 | −34.46 | −41.2 | −19.24 |
| ALDE-TN03 | −10.53 | |||||||
| OLIV-TN03 | 3.92 | |||||||
| 4 | ALDE-LPAL-YAIZ | ALDE-LPAL | 13.60 | 23.31 | −21.8 | 3.66 | 68.2 | 26.97 |
| ALDE-YAIZ | 3.94 | |||||||
| LPAL-YAIZ | 5.80 | |||||||
| 5 | HRIA-LPAL-YAIZ | HRIA-LPAL | 4.27 | 19.01 | −53.0 | −13.48 | 37.0 | 5.53 |
| HRIA-YAIZ | −11.54 | |||||||
| LPAL-YAIZ | 5.80 | |||||||
| 6 | ALDE-OLIV-YAIZ | ALDE-OLIV | 9.28 | 78.95 | −40.2 | 0.77 | 49.8 | 79.72 |
| ALDE-YAIZ | 3.94 | |||||||
| OLIV-YAIZ | 17.42 | |||||||
| 7 | GRAF-OLIV-YAIZ | GRAF-OLIV | 12.64 | 63.56 | −33.7 | −3.57 | 56.3 | 59.99 |
| GRAF-YAIZ | 5.06 | |||||||
| OLIV-YAIZ | 17.42 | |||||||
| 8 | LPAL-OLIV-YAIZ | LPAL-OLIV | 10.97 | 55.97 | −29.3 | −6.90 | 60.7 | 49.08 |
| LPAL-YAIZ | 5.80 | |||||||
| OLIV-YAIZ | 17.42 | |||||||
| 9 | LPAL-TN03-ALAJ | LPAL-TN03 | 31.34 | 162.36 | 8.9 | −2.02 | −81.1 | 160.34 |
| LPAL-ALAJ | 73.84 | |||||||
| TN03-ALAJ | −0.28 | |||||||
| 10 | GRAF-LPAL-ALAJ | GRAF-LPAL | 8.36 | 104.85 | −5.9 | −3.19 | 84.1 | 101.65 |
| GRAF-ALAJ | 6.70 | |||||||
| LPAL-ALAJ | 73.84 | |||||||
| 11 | ALDE-LPAL-ALAJ | ALDE-LPAL | 13.60 | 122.68 | −0.7 | −8.35 | 89.3 | 114.33 |
| ALDE-ALAJ | −7.41 | |||||||
| LPAL-ALAJ | 73.84 | |||||||
| 12 | ALDE-GRAF-ALAJ | ALDE-GRAF | 24.92 | 59.53 | 3.8 | −7.41 | −86.2 | 52.12 |
| ALDE-ALAJ | −7.41 | |||||||
| GRAF-ALAJ | 6.70 | |||||||
| 13 | LPAL-ALAJ-FRON | LPAL-ALAJ | 73.84 | 103.20 | −7.5 | −8.77 | 82.5 | 94.43 |
| LPAL-FRON | 2.44 | |||||||
| ALAJ-FRON | −4.59 | |||||||
| 14 | LPAL-FRON-STEI | LPAL-FRON | 2.44 | 94.00 | −31.3 | −73.39 | 58.7 | 20.61 |
| LPAL-STEI | 48.44 | |||||||
| FRON-STEI | −8.96 | |||||||
| 15 | ALAJ-FRON-ARG1 | ALAJ-FRON | −4.59 | −4.41 | −84.6 | −201.55 | 5.4 | −205.96 |
| ALAJ-ARG1 | −7.88 | |||||||
| FRON-ARG1 | −6.43 | |||||||
| 16 | FRON-IZAN-ARG1 | FRON-IZAN | 7.53 | 30.32 | −56.0 | −84.65 | 34.0 | −54.33 |
| FRON-ARG1 | −6.43 | |||||||
| IZAN-ARG1 | 29.90 | |||||||
| 17 | ALAJ-IZAN-ARG1 | ALAJ-IZAN | −50.71 | 43.75 | −30.4 | −54.23 | 59.6 | −10.48 |
| ALAJ-ARG1 | −7.88 | |||||||
| IZAN-ARG1 | 29.90 | |||||||
| 18 | TN03-ALAJ-STEI | TN03-ALAJ | −0.28 | 116.91 | 36.1 | −50.02 | −53.9 | 66.89 |
| TN03-STEI | 6.92 | |||||||
| ALAJ-STEI | 91.42 | |||||||
| 19 | IZAN-ARG1-TERR | IZAN-ARG1 | 29.90 | 169.18 | 77.1 | −65.34 | −12.9 | 103.84 |
| IZAN-TERR | 111.30 | |||||||
| ARG1-TERR | 10.95 | |||||||
| 20 | GRAF-TERR-ANTI | GRAF-TERR | 89.95 | 117.47 | −33.6 | −45.58 | 56.4 | 71.89 |
| GRAF-ANTI | 6.88 | |||||||
| TERR-ANTI | −29.10 | |||||||
| 21 | STEI-TN03-GRAF | STEI-TN03 | 6.92 | 40.92 | 11.7 | −96.23 | −78.3 | −55.31 |
| STEI-GRAF | −64.43 | |||||||
| TN03-GRAF | −0.79 | |||||||
| 22 | STEI-TN03-IZAN | STEI-TN03 | 6.92 | 22.20 | −4.6 | −253.76 | 85.4 | −231.56 |
| STEI-IZAN | −252.90 | |||||||
| TN03-IZAN | −100.90 | |||||||
| 23 | TN02-IZAN-GRAF | IZAN-GRAF | 153.00 | 202.06 | 33.0 | −138.42 | −57.0 | 63.64 |
| IZAN-TN02 | −23.81 | |||||||
| GRAF-TN02 | 4.87 | |||||||
| Baseline | Largest Elongation | Baseline | Largest Shortening |
|---|---|---|---|
| LPAL-ALAJ | 73.84 | ALDE-TN03 | −10.53 |
| LPAL-TN03 | 31.34 | TN03-IZAN | −100.90 |
| ALDE-GRAF | 24.92 | STEI-GRAF | −64.43 |
| IZAN-TERR | 111.30 | STEI-IZAN | −252.90 |
| IZAN-GRAF | 153.00 | IZAN-TN02 | −23.81 |
| TERR-ANTI | −29.10 | ||
| HRIA-YAIZ | −11.54 |
| Triangle | Largest Dilatation | Triangle | Largest Contraction | ||
|---|---|---|---|---|---|
| 9 | LPAL-TN03-ALAJ | 160.34 | 3 | ALDE-OLIV-TN03 | −19.24 |
| 10 | GRAF-LPAL-ALAJ | 101.65 | 21 | STEI-TN03-GRAF | −55.31 |
| 11 | ALDE-LPAL-ALAJ | 114.33 | 22 | STEI-TN03-IZAN | −231.56 |
| Parameter | |
|---|---|
| Poisson’s ratio | 0.25 |
| Young modulus | 80.0 GPa |
| Friction coefficient | 0.4 |
| Fault length | 15.0 km |
| Calculation depth | 4.0 km |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arnoso, J.; Riccardi, U.; Benavent, M.; Tammaro, U.; Montesinos, F.G.; Blanco-Montenegro, I.; Vélez, E. Strain Pattern and Kinematics of the Canary Islands from GNSS Time Series Analysis. Remote Sens. 2020, 12, 3297. https://doi.org/10.3390/rs12203297
Arnoso J, Riccardi U, Benavent M, Tammaro U, Montesinos FG, Blanco-Montenegro I, Vélez E. Strain Pattern and Kinematics of the Canary Islands from GNSS Time Series Analysis. Remote Sensing. 2020; 12(20):3297. https://doi.org/10.3390/rs12203297
Chicago/Turabian StyleArnoso, Jose, Umberto Riccardi, Maite Benavent, Umberto Tammaro, Fuensanta G. Montesinos, Isabel Blanco-Montenegro, and Emilio Vélez. 2020. "Strain Pattern and Kinematics of the Canary Islands from GNSS Time Series Analysis" Remote Sensing 12, no. 20: 3297. https://doi.org/10.3390/rs12203297
APA StyleArnoso, J., Riccardi, U., Benavent, M., Tammaro, U., Montesinos, F. G., Blanco-Montenegro, I., & Vélez, E. (2020). Strain Pattern and Kinematics of the Canary Islands from GNSS Time Series Analysis. Remote Sensing, 12(20), 3297. https://doi.org/10.3390/rs12203297