The Impact of Different Ocean Tide Loading Models on GNSS Estimated Zenith Tropospheric Delay Using Precise Point Positioning Technique
Abstract
1. Introduction
2. Data and Methodologies
2.1. GNSS and Radiosonde Data
2.2. OTL Models
2.3. ZTD Calculation with Radiosonde
2.4. ZTD Calculation with GNSS
3. Results
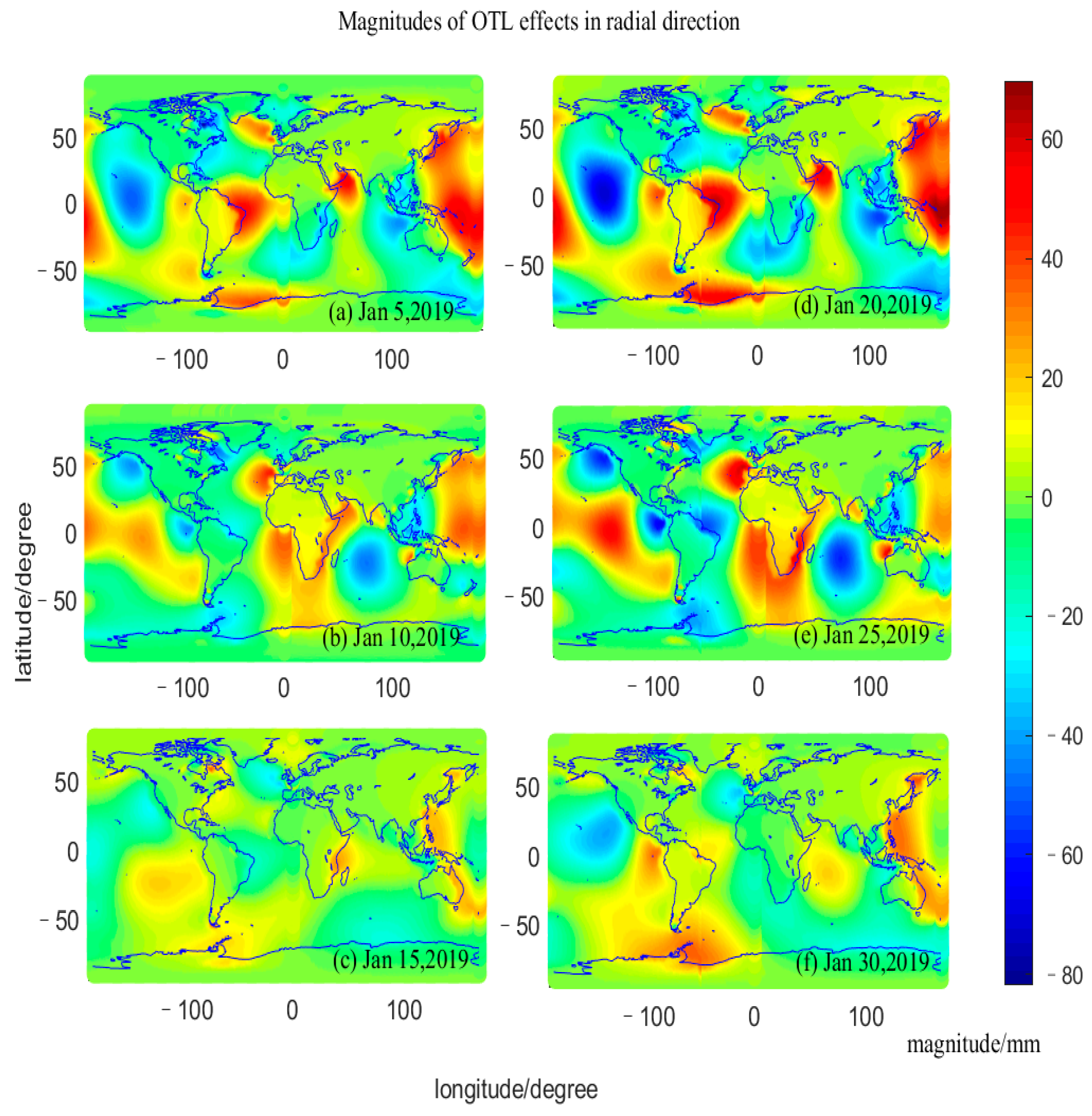
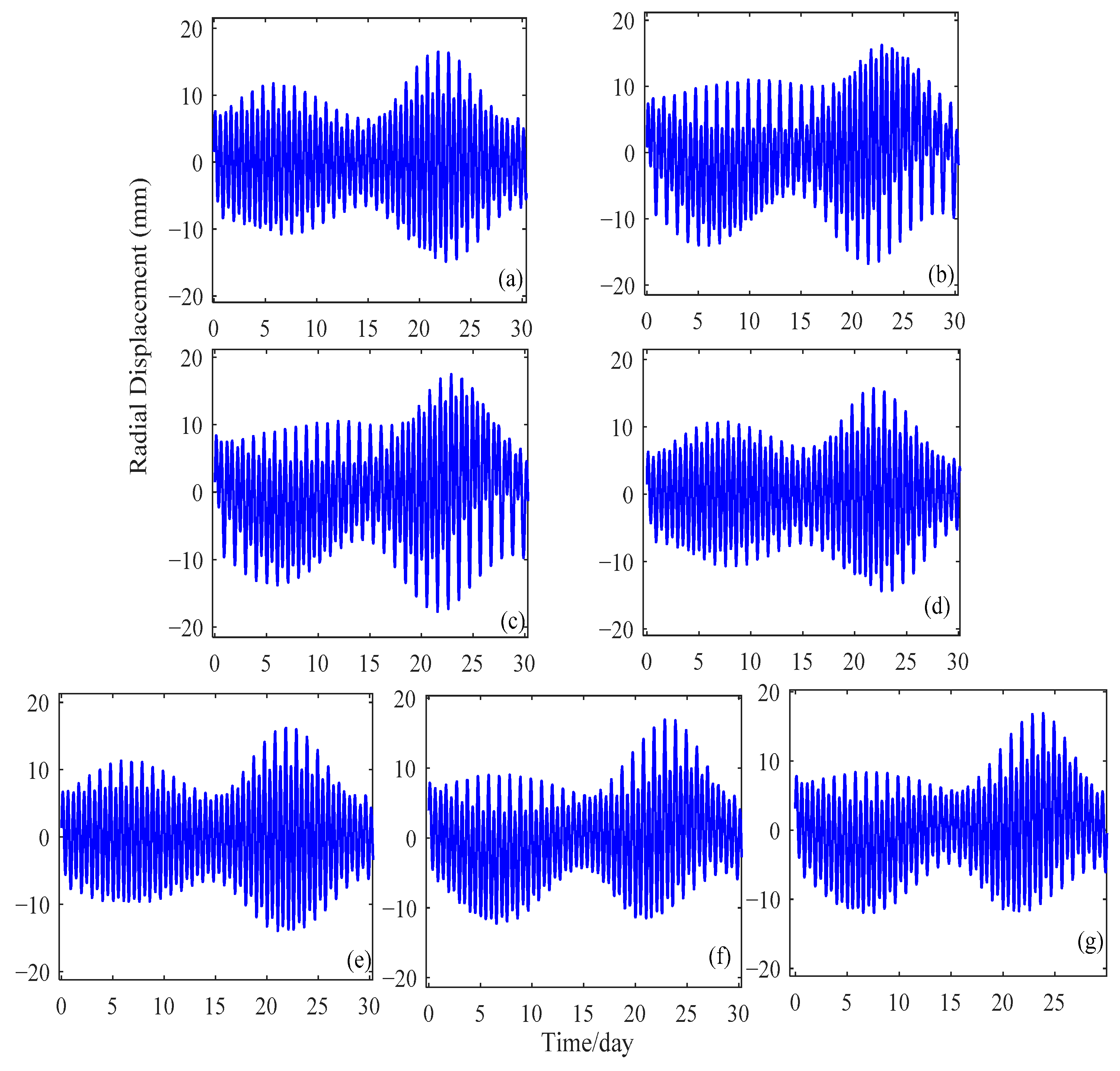
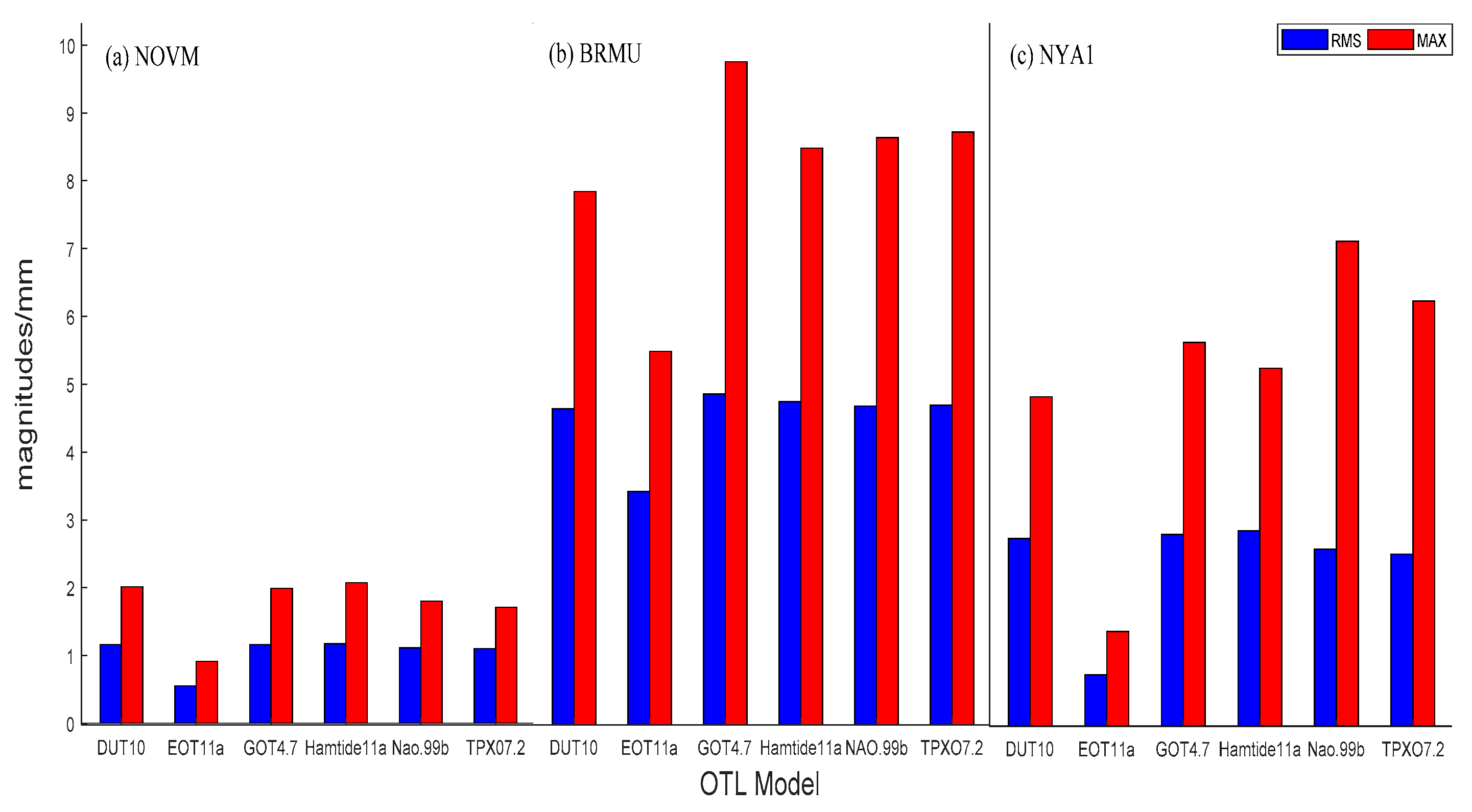
3.1. Temporal and Spatial Characteristics of the OTL Models
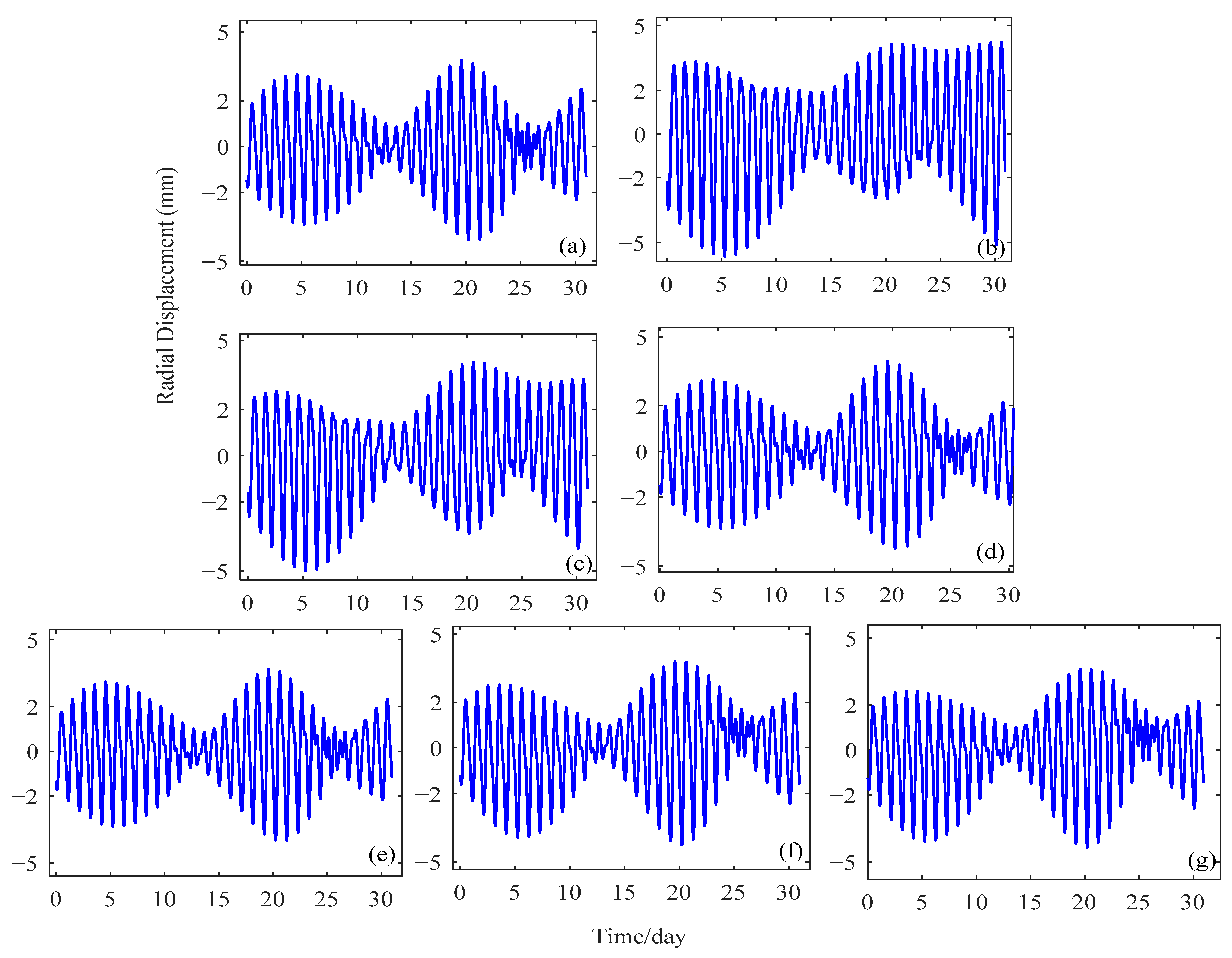
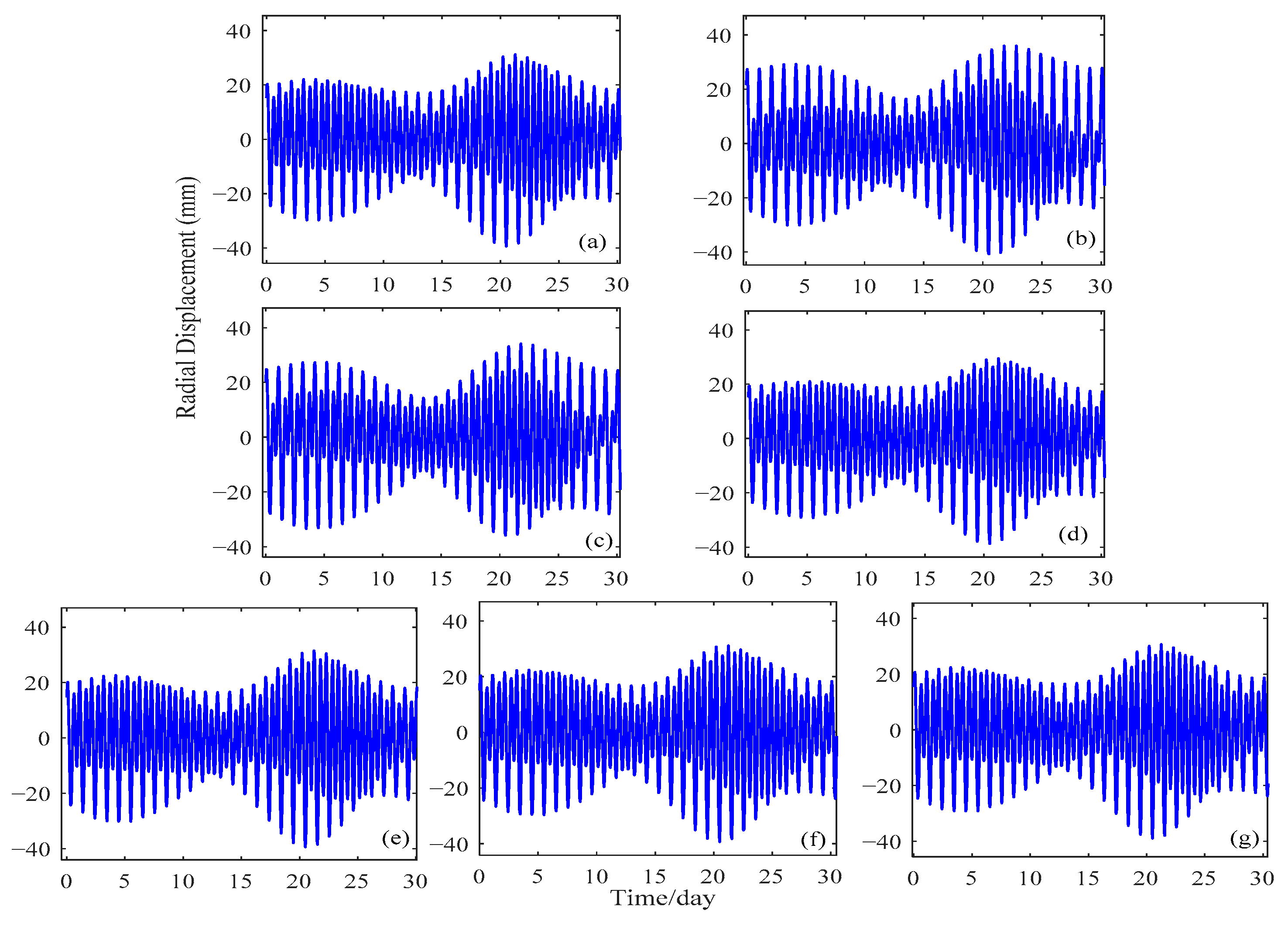
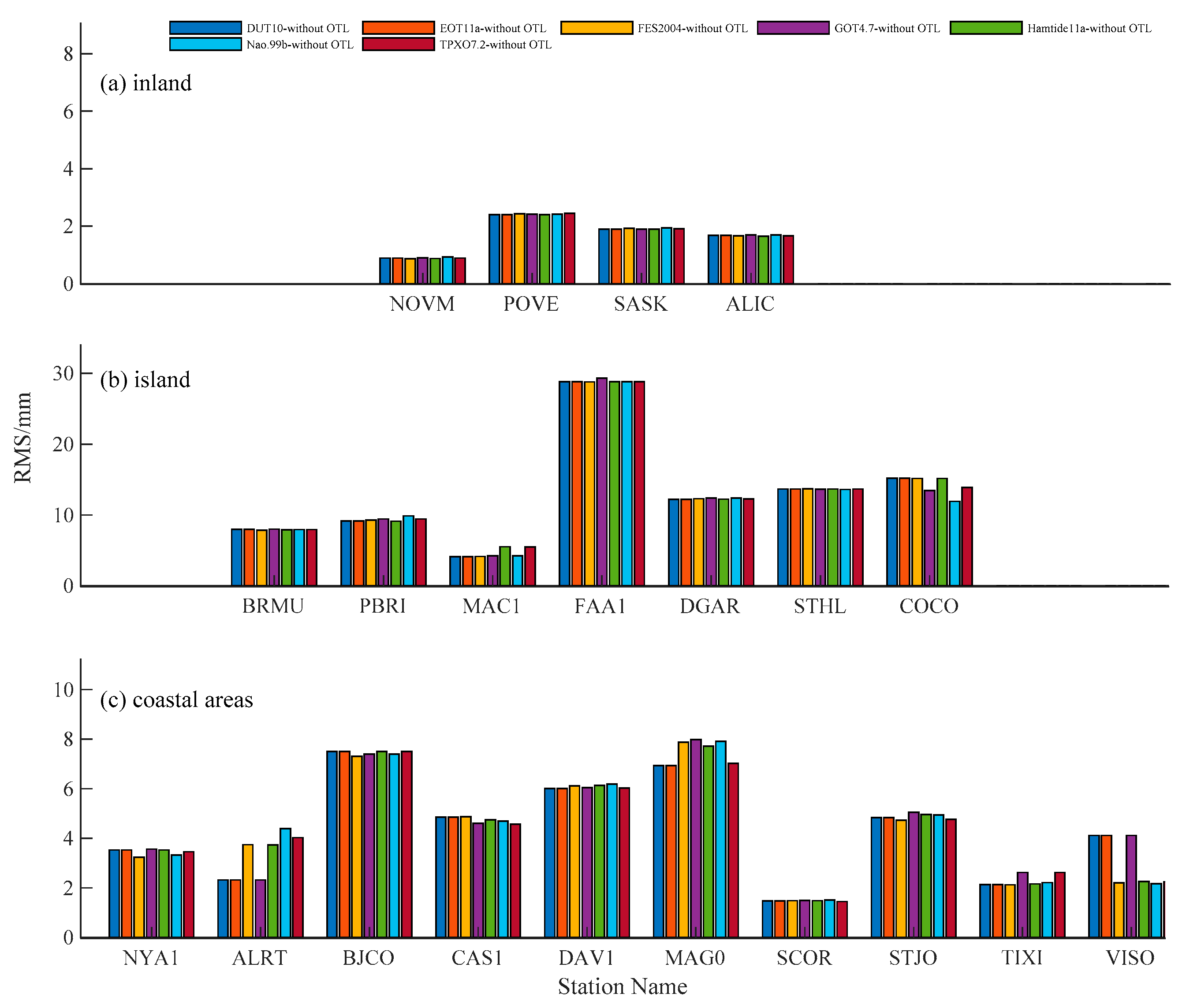
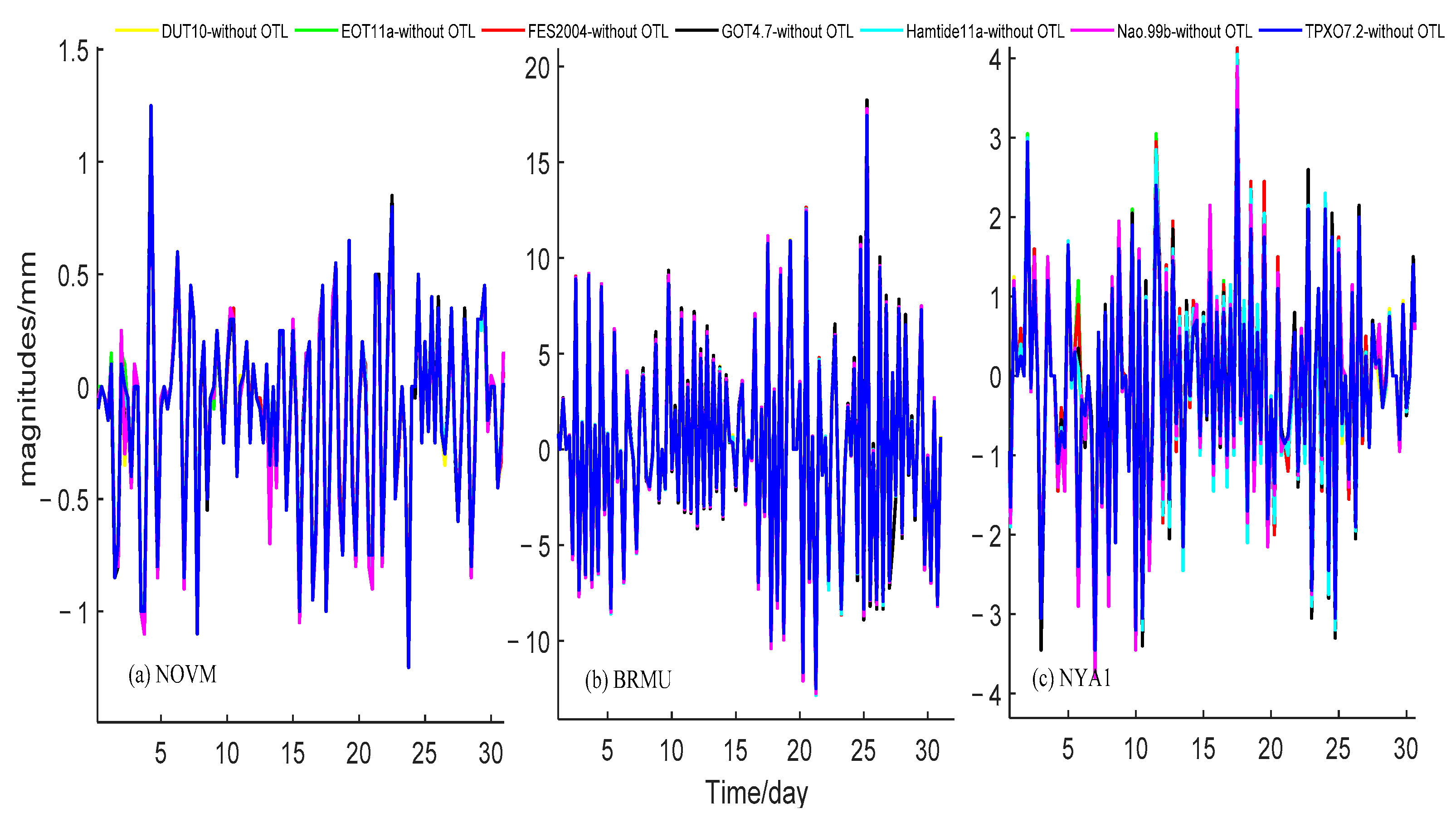
3.2. GNSS-Derived ZTD
3.3. Radiosonde
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Li, X.; Dick, G.; Ge, M.; Heise, S.; Wickert, J.; Bender, M. Real-time GPS sensing of atmospheric water vapor: Precise point positioning with orbit, clock, and phase delay corrections. Geophys. Res. Lett. 2014, 41, 10. [Google Scholar] [CrossRef]
- Singh, D.; Ghosh, J.K.; Kashyap, D. Precipitable water vapor estimation in India from GPS-derived zenith delays using radiosonde data. Meteorol. Atmos. Phys. 2014, 123, 3–4. [Google Scholar] [CrossRef]
- Wang, H.; Wei, M.; Li, G.; Zhou, S.; Zeng, Q. Analysis of precipitable water vapor from GPS measurements in Chengdu region: Distribution and evolution characteristics in autumn. Adv. Space Res. 2013, 52, 4. [Google Scholar] [CrossRef]
- Means, J.D.; Cayan, D. Precipitable Water from GPS Zenith Delays Using North American Regional Reanalysis Meteorology. J. Atmos. Ocean. Technol. 2013, 30, 3. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, K.; Wu, S.; Fan, S.; Cheng, Y. Water vapor-weighted mean temperature and its impact on the determination of precipitable water vapor and its linear trend. J. Geophys. Res. Atmos. 2016, 121, 833–852. [Google Scholar] [CrossRef]
- Dragert, H.; James, T.S.; Lambert, A. Ocean loading corrections for continuous GPS: A case study at the Canadian coastal site Holberg. Geophys. Res. Lett. 2000, 27, 14. [Google Scholar] [CrossRef]
- Luo, X. GPS Stochastic Modelling: Signal Quality Measures and ARMA Processes; Springer: New York, NY, USA, 2013; p. 78. [Google Scholar]
- Dach, R.; Dietrich, R. Influence of the ocean loading effect on GPS derived precipitable water vapor. Geophys. Res. Lett. 2000, 27, 18. [Google Scholar] [CrossRef]
- De Haan, S. National/Regional Operational Procedures of GPS Water Vapour Networks and Agreed International Procedures; KNMI: De Bilt, The Netherlands, 2006; p. 20. [Google Scholar]
- Kim, T.H.; Shibuya, K.; Doi, K.; Aoyama, Y.; Hayakawa, H. Validation of global ocean tide models using the superconducting gravimeter data at Syowa Station, Antarctica, and in situ tide gauge and bottom-pressure observations. Polar Sci. 2010, 5, 1. [Google Scholar] [CrossRef]
- Padman, L.; Erofeeva, S.Y.; Fricker, H.A. Improving Antarctic tide models by assimilation of ICESat laser altimetry over ice shelves. Geophys. Res. Lett. 2008, 35, 22. [Google Scholar] [CrossRef]
- Ray, R.A. Global ocean tide model from Topex/Poseidon altimetry: GOT 99.2. NASA Tech Memo. 1999, 58, 209478. [Google Scholar]
- Egbert, G.D.; Ray, R.D. Deviation of Long-Period Tides from Equilibrium: Kinematics and Geostrophy. J. Phys. Oceanogr. 2003, 33, 822–839. [Google Scholar] [CrossRef][Green Version]
- Lei, J.; Li, F.; Zhang, S.; Ke, H.; Zhang, Q.; Li, W. Accuracy Assessment of Recent Global Ocean Tide Models around Antarctica. ISPRS Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2017, 42, 1521. [Google Scholar] [CrossRef]
- Lyard, F.; Lefevre, F.; Letellier, T.; Francis, O. Modelling the global ocean tides: Modern insights from FES2004. Ocean. Dyn. 2006, 56, 5–6. [Google Scholar] [CrossRef]
- Urschl, C.; Dach, R.; Hugentobler, U.; Schaer, S.; Beutler, G. Validating ocean tide loading models using GPS. J. Geod. 2005, 78, 10. [Google Scholar] [CrossRef]
- Jin, S.; Wu, Y.; Heinkelmann, R.; Park, J. Diurnal and semidiurnal atmospheric tides observed by co-located GPS and VLBI measurements. J. Atmos. Sol.-Terr. Phys. 2008, 70, 1366–1372. [Google Scholar] [CrossRef]
- Gokhan, G.; Jin, S. Evaluation of ocean tide loading effects on GPS-Estimated precipitable water vapour in Turkey. Geod. Geodyn. 2016, 7, 1. [Google Scholar] [CrossRef]
- Gurbuz, G.; Jin, S.; Mekik, C. Effects of ocean tide models on GNSS-estimated ZTD and PWV in Turkey. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2015, 40, 255. [Google Scholar] [CrossRef]
- Yuan, Y.; Zhang, K.; Rohm, W.; Choy, S.; Norman, R.; Wang, C.S. Real-Time retrieval of precipitable water vapor from GPS precise point positioning. Geophys. Res. Atmos. 2014, 119, 10044–10057. [Google Scholar] [CrossRef]
- Villiger, A.; Dach, R. International GNSS Service Technical Report 2018 (IGS Annual Report). IGS Cent. Bur. Univ. Bern. 2019. [Google Scholar] [CrossRef]
- Cheng, Y.; Andersen, O.B. Improvement in global ocean tide model in shallow water regions. In OST-ST Meeting on Altimetry for Oceans and Hydrology; Technical University of Denmark: Lyngby, Denmark, 2010; Poster, SV.1-68 45. [Google Scholar]
- Savcenko, R.; Bosch, W. EOT11a—Empirical Ocean Tide Model From Multi-Mission Satellite Altimetry; Report 89; Deutsches Geodätisches Forschungsinstitut: München, Germany, 2012. [Google Scholar]
- Le Provost, C.; Genco, M.; Lyard, F.; Vincent, P.; Canceil, P. Spectroscopy of the world ocean tides from a finite element hydrodynamic model. J. Geophys. Res. Ocean. . 1994, 99, 24777–24797. [Google Scholar] [CrossRef]
- Le Provost, C.; Lyard, F.; Molines, J.; Genco, M.; Rabilloud, F. A hydrodynamic ocean tide model improved by assimilating a satellite altimeter-derived data set. J. Geophys. Res. Ocean. 1998, 103, 5513–5529. [Google Scholar] [CrossRef]
- Lefèvre, F.; Lyard, F.; Provost, C.L. FES98: A new global tide finite element solution independent of altimetry. Geophys. Res. Lett. 2000, 27, 17. [Google Scholar] [CrossRef]
- Lefevre, F.; Lyard, F.; Provost, C.L.; Schrama, E.J. FES99: A Global Tide Finite Element Solution Assimilating Tide Gauge and Altimetric Information. J. Atmos. Ocean. Technol. 2002, 19, 1345–1356. [Google Scholar] [CrossRef]
- Taguchi, E.; Stammer, D.; Zahel, W. Inferring deep ocean tidal energy dissipation from the global high-resolution data-assimilative HAMTIDE model. J. Geophys. Res. 2014, 119, 4573–4592. [Google Scholar] [CrossRef]
- Matsumoto, K.; Takanezawa, T.; Ooe, M. Ocean Tide Models Developed by Assimilating TOPEX/POSEIDON Altimeter Data into Hydrodynamical Model: A Global Model and a Regional Model around Japan. J. Oceanogr. 2000, 56, 5. [Google Scholar] [CrossRef]
- Egbert, G.D.; Bennett, A.F.; Foreman, M.G.G. TOPEX/POSEIDON tides estimated using a global inverse model. J. Geophys. Res. Ocean. 1994, 99, 24821–24852. [Google Scholar] [CrossRef]
- Egbert, G.D.; Erofeeva, S.Y. Efficient Inverse Modeling of Barotropic Ocean Tides. J. Atmos. Ocean. Technol. 2002, 19, 183–204. [Google Scholar] [CrossRef]
- Zhang, Q.; Ye, J.; Zhang, S.; Han, F. Precipitable water vapor retrieval and analysis by multiple data sources: Ground-based GNSS, radio occultation, radiosonde, microwave satellite, and NWP reanalysis data. J. Sens. 2018, 2018, 1–13. [Google Scholar] [CrossRef]
- Liang, H.; Cao, Y.; Wan, X.; Xu, Z.; Wang, H.; Hu, H. Meteorological applications of precipitable water vapor measurements retrieved by the national GNSS network of China. Geod. Geodyn. 2015, 6, 135–142. [Google Scholar] [CrossRef]
- Grejnerbrzezinska, D.A. GPS-PWV estimation and validation with radiosonde data and numerical weather prediction model in Antarctica. Gps Solut. 2013, 17, 29–39. [Google Scholar]
- Zhao, Q.; Yao, Y.; Yao, W.; Zhang, S. GNSS-derived PWV and comparison with radiosonde and ECMWF ERAInterim data over mainland China. J. Atmos. Solar Terr. Phys. 2019, 182, 85–92. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, K.; Wu, S.; Li, Z.; Cheng, Y.; Li, L.; Yuan, H. The correlation between GNSS-derived precipitable water vapor and sea surface temperature and its responses to El Niño–Southern Oscillation. Remote Sens. Environ. 2018, 216, 1–12. [Google Scholar] [CrossRef]
- Durre, I.; Vose, R.S.; Wuertz, D.B. Overview of the integrated global radiosonde archive. J. Clim. 2006, 19, 53–68. [Google Scholar] [CrossRef]
- Haase, J.S.; Vedel, H.; Ge, M.; Calais, E. GPS zenith tropospheric delay (ZTD) variability in the Mediterranean. Pergamon 2001, 26, 6–8. [Google Scholar] [CrossRef]
- Lambeck, K. Geophysical Geodesy: The Slow Deformations of the Earth; Oxford University Press: Oxford, UK, 1988; p. 710. [Google Scholar]
- Shum, C.K.; Woodworth, P.L.; Andersen, O.B.; Egbert, G.D.; Francis, O.; King, C.; Klosko, S.M.; Le Provost, C.; Li, X.; Molines, J.M.; et al. Accuracy assesment of recent ocean tide models. Geophys. Res. Lett. 1997, 102, 25173–25194. [Google Scholar] [CrossRef]
- Melachroinos, S.A.; Biancale, R.; Llubes, M.; Perosanz, F.; Lyard, F.; Vergnolle, M.; Bouin, M.-N.; Masson, F.; Nicolas, J.; Morel, L. Ocean tide loading (OTL) displacements from global and local grids: comparisons to GPS estimates over the shelf of Brittany, France. J. Geod. 2008, 82, 357–371. [Google Scholar] [CrossRef]
- Farrell, W. Deformation of the Earth by surface loads. Rev. Geophys. 1972, 10, 761–797. [Google Scholar] [CrossRef]
- Agnew, D.C. SPOTL: Some Programs for Ocean-Tide Loading. Scripps Institution of Oceanography. SIO Ref. Ser. 1996, 96, 35. [Google Scholar]
- Francis, O.; Mazzega, P. Global charts of ocean tide loading effects. J. Geophys. Res. Ocean. 1990, 95, 11411–11424. [Google Scholar] [CrossRef]
- Seidelmann, P.K. Explanatory Supplement to the Astronomical Almanac; University Science Books: Herndon, VA, USA, 1992. [Google Scholar]
- Takasu, T.; Yasuda, A. Development of the low-cost RTK-GPS receiver with an open source program package RTKLIB. In Proceedings of the International symposium on GPS/GNSS, Jeju, Korea, 4–6 November 2009; pp. 4–6. [Google Scholar]
- Niell, A. Global mapping functions for the atmosphere delay at radio wavelengths. J. Geophys. Res. Solid Earth 1996, 101, 3227–3246. [Google Scholar] [CrossRef]
- Böhm, J.; Niell, A.; Tregoning, P.; Schuh, H. Global Mapping Function (GMF): A new empirical mapping function based on numerical weather model data. Geophys. Res. Lett. 2006, 33, 7. [Google Scholar] [CrossRef]
- Qiu, C.; Wang, X.; Li, Z.; Zhang, S.; Li, H.; Zhang, J.; Yuan, H. The Performance of Different Mapping Functions and Gradient Models in the Determination of Slant Tropospheric Delay. Remote Sens. 2020, 12, 130. [Google Scholar] [CrossRef]
- MacMillan, D. Atmospheric gradients from very long baseline interferometry observations. Geophys. Res. Lett. 1995, 22, 1041–1044. [Google Scholar] [CrossRef]
- Li, X.; Ge, M.; Dai, X.; Ren, X.; Fritsche, M.; Wickert, J.; Schuh, H. Accuracy and reliability of multi-GNSS real-time precise positioning: GPS, GLONASS, BeiDou, and Galileo. J. Geod. 2015, 89, 607–635. [Google Scholar] [CrossRef]
- Mohammed, J.; Moore, T.; Hill, C.; Bingley, R.M. Alternative Strategy for Estimating Zenith Tropospheric Delay from Precise Point Positioning. In Proceedings of the 2020 IEEE/ION Position, Location and Navigation Symposium (PLANS), Portland, OR, USA, 20–23 April 2020; pp. 247–258. [Google Scholar]
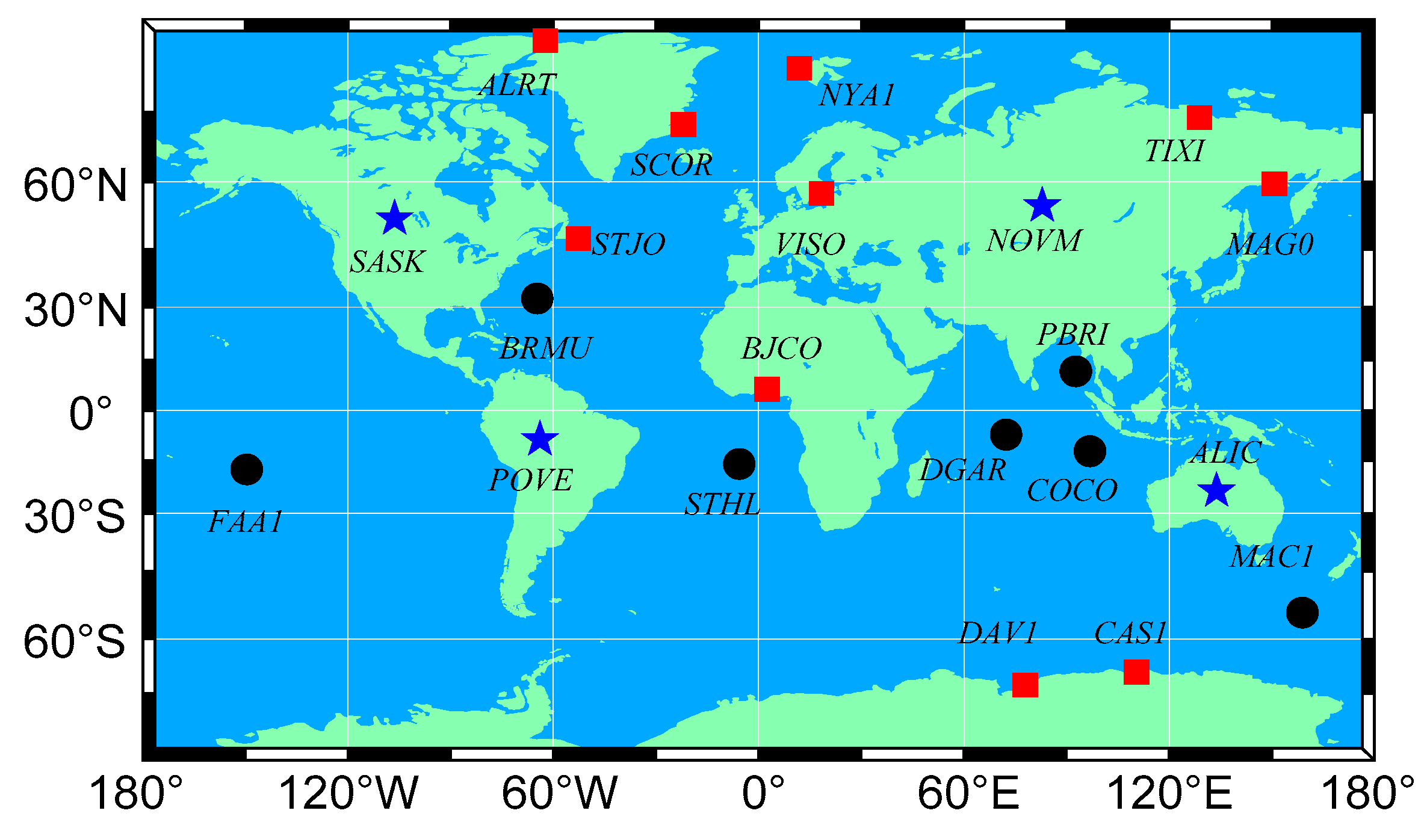


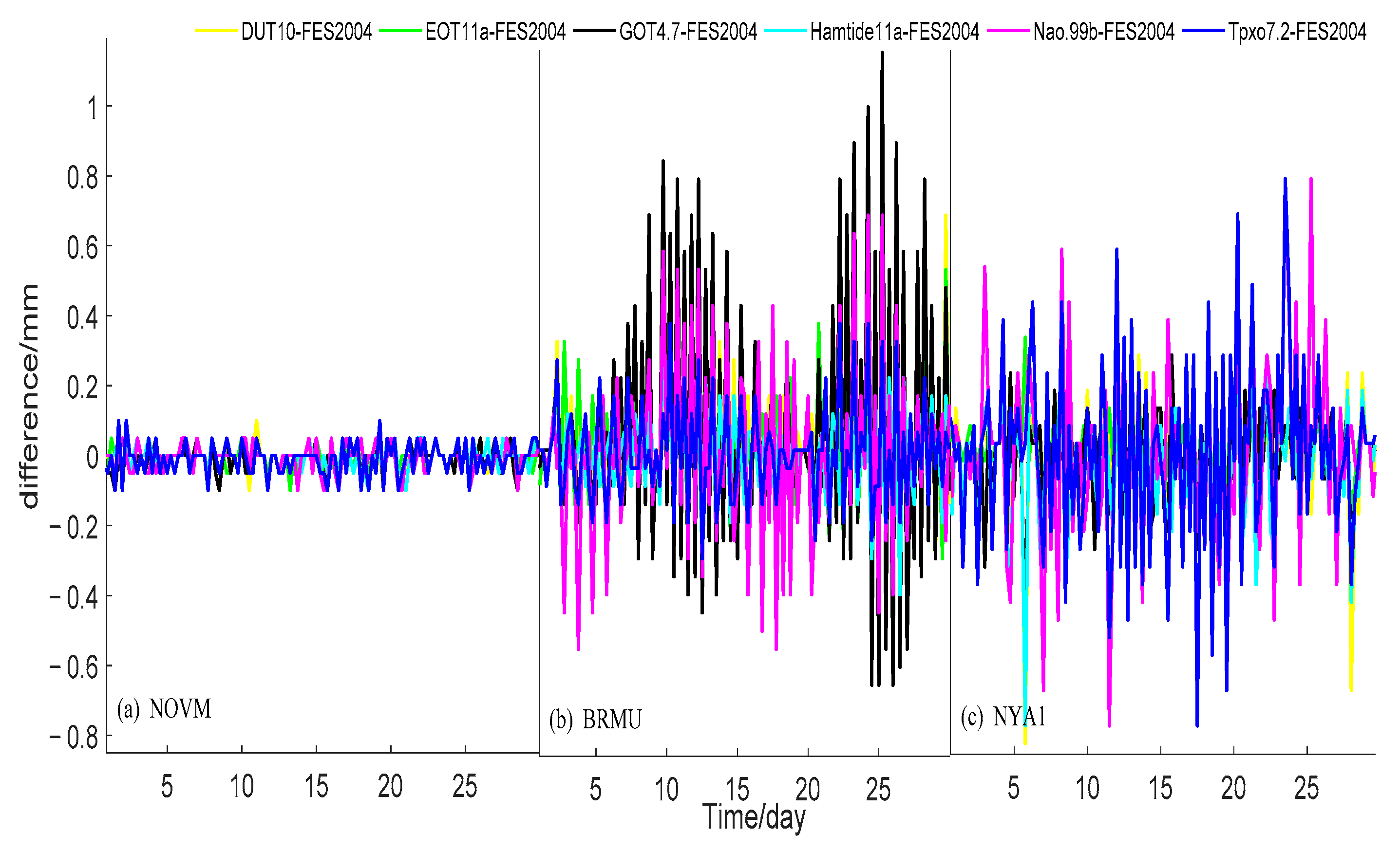
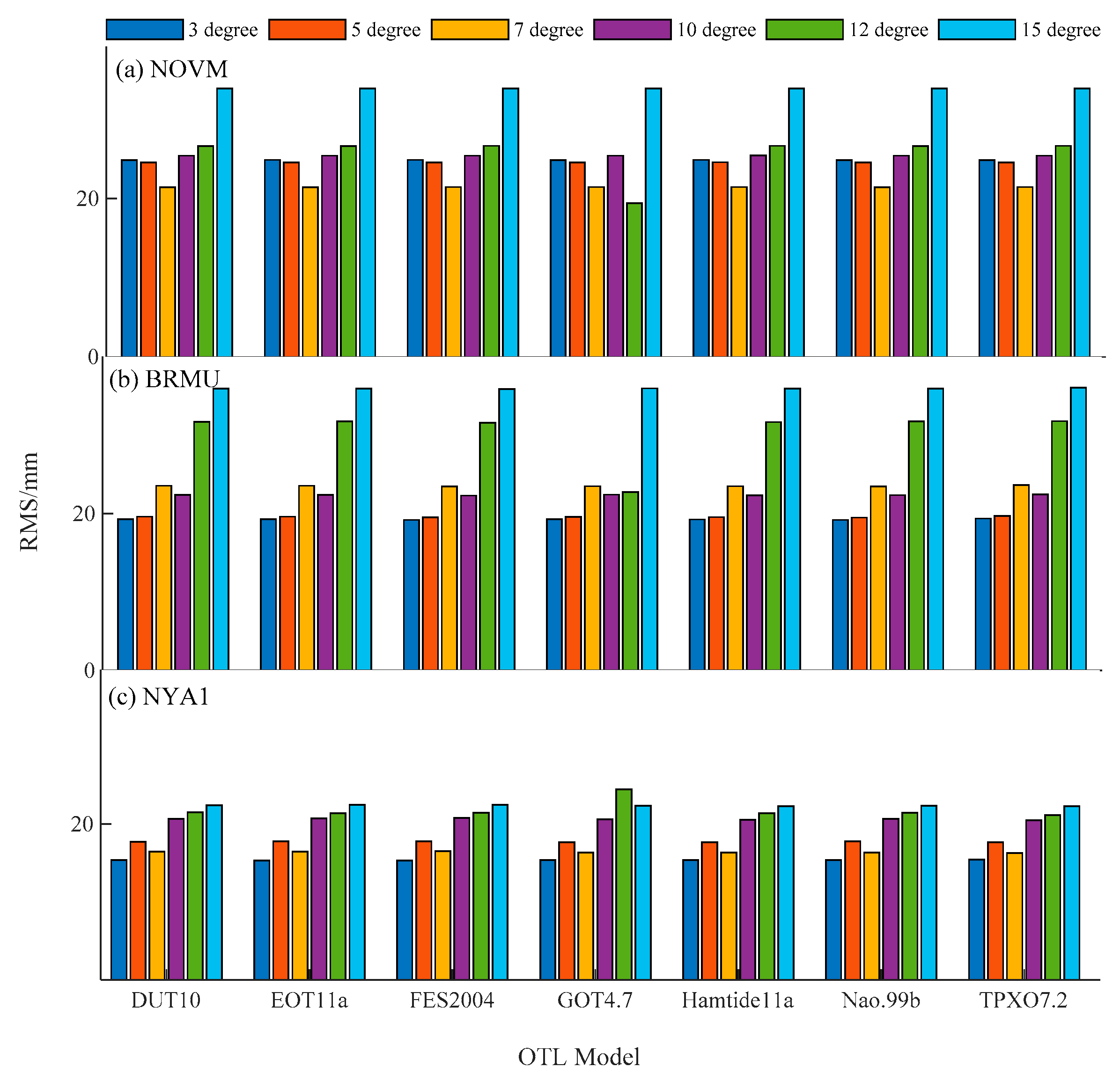
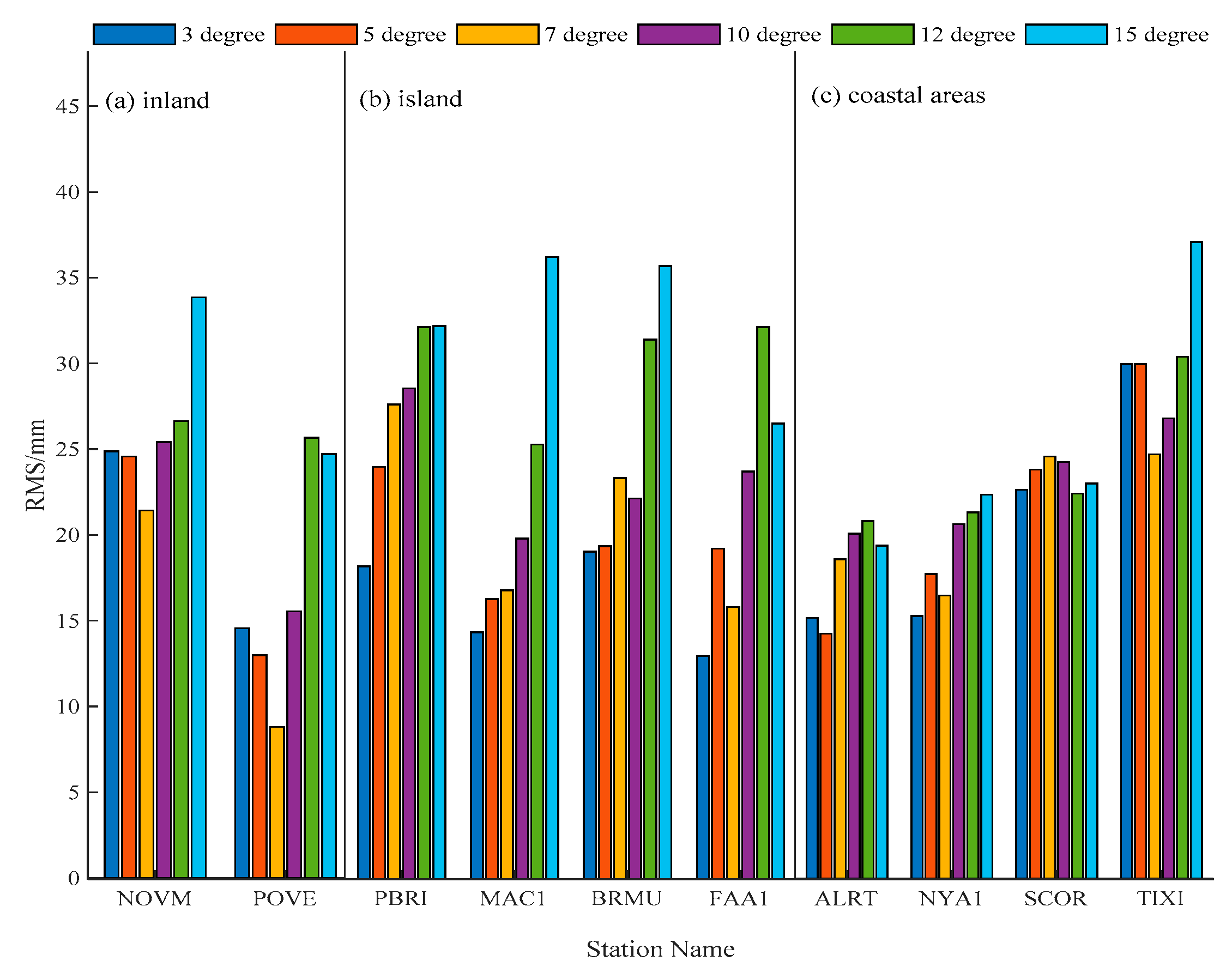
| GNSS Site | Radiosonde Site | Distance(km) | Position | GNSS Height(m) | Radiosonde Height (m) |
|---|---|---|---|---|---|
| ALRT | 71082 | 0.652 | coastal | 78.123 | 77.39 |
| NYA1 | 01004 | 1.746 | coastal | 84.237 | 67.80 |
| SCOR | 04339 | 0.589 | coastal | 128.5 | 126.22 |
| TIXI | 21824 | 0.762 | coastal | 47.067 | 0.83 |
| PBRI | 43333 | 3.682 | island | −22.583 | 16.72 |
| MAC1 | 94998 | 0.919 | island | −6.797 | −12.79 |
| BRMU | 78016 | 1.531 | island | −11.626 | 1.99 |
| FAA1 | 91938 | 0.844 | island | 12.052 | 4.82 |
| POVE | 82824 | 1.111 | inland | 119.562 | 114.09 |
| NOVM | 29634 | 0.608 | inland | 150.139 | 106.69 |
| Model | Resolution | Tide Model Type | Coverage |
|---|---|---|---|
| GOT4.7 | 0.5° × 0.5° | H + T/P + ERS | Global |
| NAO. 99 b | 0.5° × 0.5° | H + T/P | Global |
| DUT10 | 0.125° × 0.125° | H + T/P + Jason − ½ + ERS + T/G | Global |
| EOT11a | 0.125° × 0.125° | H + T/P + Jason − ½ + ERS + Envisat | Global |
| FES2004 | 0.125° × 0.125° | H + T/P + T/G + ERS | Global |
| Hamtide.2011a | 0.125° × 0.125° | H + T/P + Jason − 1/2 | Global |
| TPXO7.2 | 0.25° × 0.25° | H + T/P + T/G + ERS | Global |
| Item | Strategies |
|---|---|
| Frequencies | GPS L1 and L2 dual-frequency |
| Filter Type | Smoother combined solution with forward and backward filter solutions |
| Elevation cutoff angle | 10° |
| Ocean Tides | DUT10, EOT11a, FES2004, GOT4.7, Hamtide11a, Nao.99b, and TPXO7.2 |
| Satellite Ephemeris, Clock and Earth Rotation Parameters | IGS final products |
| Ionosphere | Ionosphere-free linear combination with dual-frequency |
| Troposphere | Estimate ZTD and horizontal gradient parameters |
| Mapping function | NMF |
| Receiver phase center | PCO and PCV values from igs14.atx |
| Satellite phase center | PCO and PCV values from igs14.atx |
| Phase-windup effect | Model corrected |
| ZTD temporal resolution | 30 s |
| Gradient parameters temporal resolution | 30 s |
| Integer Ambiguity Resolution | Continuously static integer ambiguities are estimated |
| Station | DUT10 | EOT11a | FES2004 | GOT4.7 | Hamtide11a | Nao. 99b | TPXO7.2 | Without OTL | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Rms | Bias | Rms | Bias | Rms | Bias | Rms | Bias | Rms | Bias | Rms | Bias | Rms | Bias | Rms | Bias | |
| ALRT | 20.18 | 12.75 | 20.19 | 12.78 | 20.19 | 12.77 | 20.20 | 12.83 | 20.19 | 12.78 | 13.39 | 8.51 | 20.21 | 12.84 | 20.32 | 12.93 |
| BRMU | 19.67 | −10.93 | 19.64 | −10.91 | 19.59 | −10.78 | 19.59 | −11.00 | 19.60 | −10.90 | 19.54 | −10.86 | 19.72 | −11.08 | 25.68 | −15.48 |
| FAA1 | 23.85 | −11.94 | 23.86 | −11.94 | 23.88 | −11.96 | 23.75 | −11.73 | 23.83 | −11.85 | 23.83 | −11.84 | 23.85 | −11.90 | 25.00 | −12.65 |
| MAC1 | 19.99 | −16.08 | 19.95 | −16.00 | 19.96 | −16.02 | 19.91 | −15.99 | 19.87 | −15.91 | 19.86 | −15.90 | 19.98 | −16.04 | 21.79 | −17.33 |
| NOVM | 22.66 | 16.81 | 22.67 | 16.82 | 22.69 | 16.85 | 22.65 | 16.81 | 22.68 | 16.83 | 22.63 | 16.80 | 22.65 | 16.80 | 23.32 | 17.32 |
| NYA1 | 20.67 | 14.28 | 20.73 | 14.34 | 20.75 | 14.38 | 20.58 | 14.22 | 20.54 | 14.14 | 20.64 | 14.28 | 20.51 | 14.13 | 20.86 | 14.23 |
| PBRI | 28.68 | −24.66 | 28.86 | −24.88 | 28.75 | −24.68 | 28.80 | −24.78 | 28.69 | −24.62 | 28.78 | −24.70 | 28.97 | −24.88 | 32.56 | −29.32 |
| SCOR | 27.10 | 26.48 | 27.05 | 26.43 | 27.05 | 26.44 | 26.96 | 26.34 | 26.99 | 26.36 | 27.11 | 26.50 | 26.94 | 26.31 | 28.07 | 27.53 |
| TIXI | 26.90 | 20.67 | 26.93 | 20.67 | 26.94 | 20.69 | 26.95 | 20.71 | 26.91 | 20.66 | 26.87 | 20.64 | 26.89 | 20.64 | 28.06 | 22.02 |
| POVE | 15.56 | −9.57 | 15.57 | −9.59 | 15.56 | −9.58 | 15.59 | −9.61 | 15.55 | −9.54 | 15.57 | −9.58 | 15.56 | −9.57 | 15.62 | −8.66 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Wang, X.; Li, Z.; Li, S.; Qiu, C.; Li, H.; Zhang, S.; Li, L. The Impact of Different Ocean Tide Loading Models on GNSS Estimated Zenith Tropospheric Delay Using Precise Point Positioning Technique. Remote Sens. 2020, 12, 3080. https://doi.org/10.3390/rs12183080
Zhang J, Wang X, Li Z, Li S, Qiu C, Li H, Zhang S, Li L. The Impact of Different Ocean Tide Loading Models on GNSS Estimated Zenith Tropospheric Delay Using Precise Point Positioning Technique. Remote Sensing. 2020; 12(18):3080. https://doi.org/10.3390/rs12183080
Chicago/Turabian StyleZhang, Jinglei, Xiaoming Wang, Zishen Li, Shuhui Li, Cong Qiu, Haobo Li, Shaotian Zhang, and Li Li. 2020. "The Impact of Different Ocean Tide Loading Models on GNSS Estimated Zenith Tropospheric Delay Using Precise Point Positioning Technique" Remote Sensing 12, no. 18: 3080. https://doi.org/10.3390/rs12183080
APA StyleZhang, J., Wang, X., Li, Z., Li, S., Qiu, C., Li, H., Zhang, S., & Li, L. (2020). The Impact of Different Ocean Tide Loading Models on GNSS Estimated Zenith Tropospheric Delay Using Precise Point Positioning Technique. Remote Sensing, 12(18), 3080. https://doi.org/10.3390/rs12183080






