Hazard Assessment of Debris Flows in the Reservoir Region of Wudongde Hydropower Station in China
Abstract
:1. Introduction
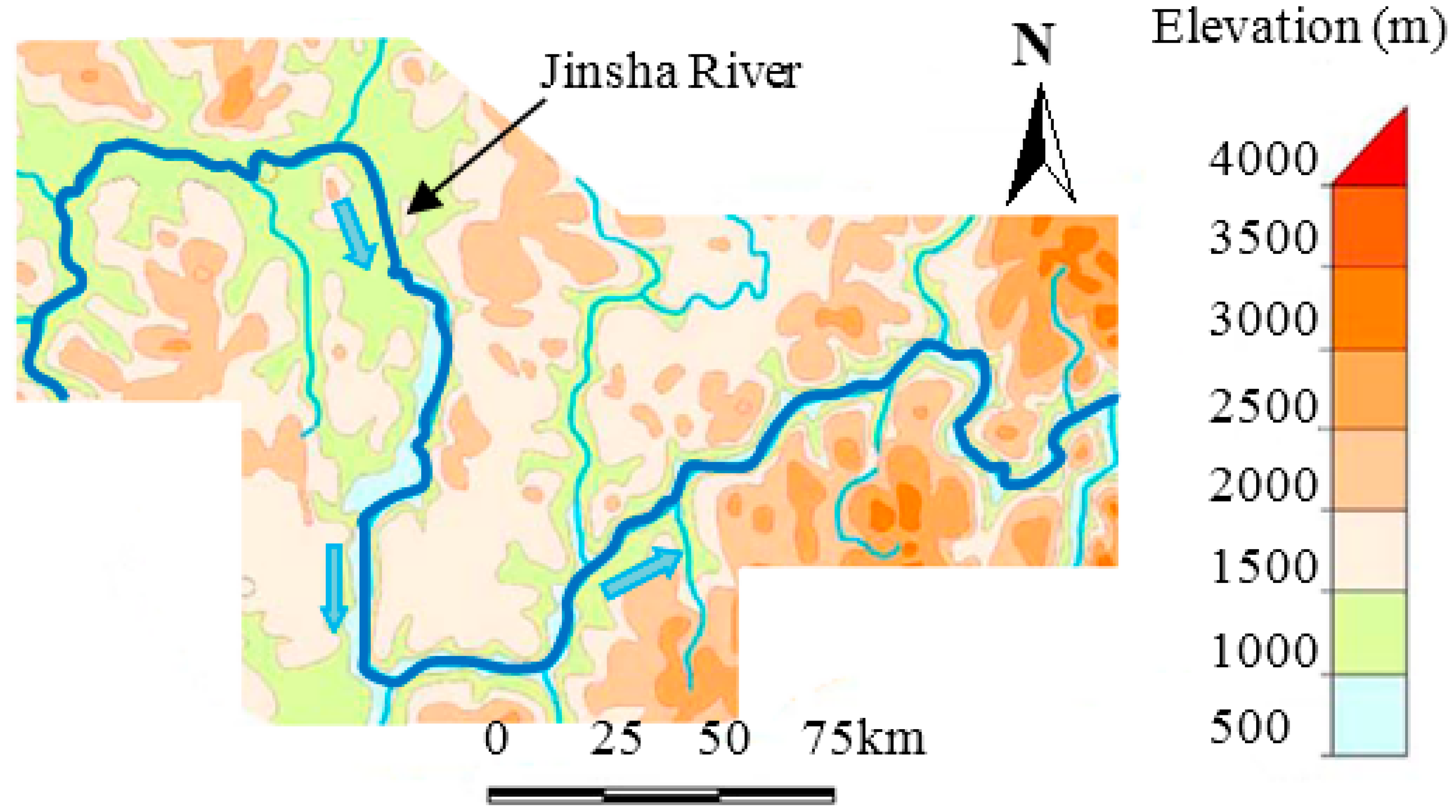
2. Study Area

3. Data Acquisition
| Category | Influencing Factors |
|---|---|
| Topography | Basin area, main channel length, maximum elevation difference, average slope of material source region, average gradient of the main channel, ravine density, main channel sinuosity |
| Geology | Loose material volume, active main channel proportion |
| Hydrology | Maximum daily rainfall, Average annual rainfall |
| Vegetation | Vegetation coverage, the normalized difference vegetation index |
| Human activity | Reclaim wasteland index, population density |
4. Methodology
4.1. Interpretation of Satellite Images
4.1.1. SPOT5 Remote Sensing Images
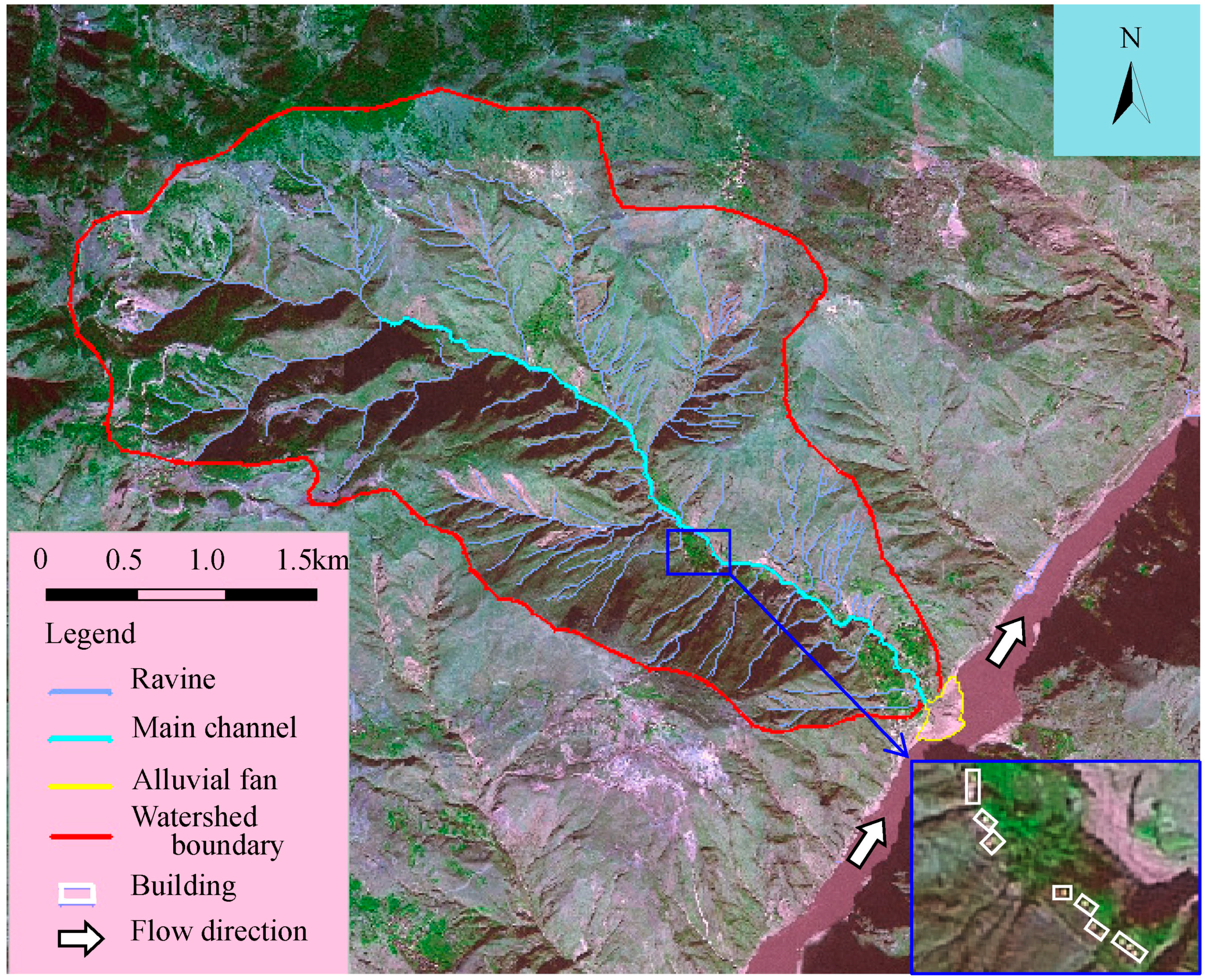
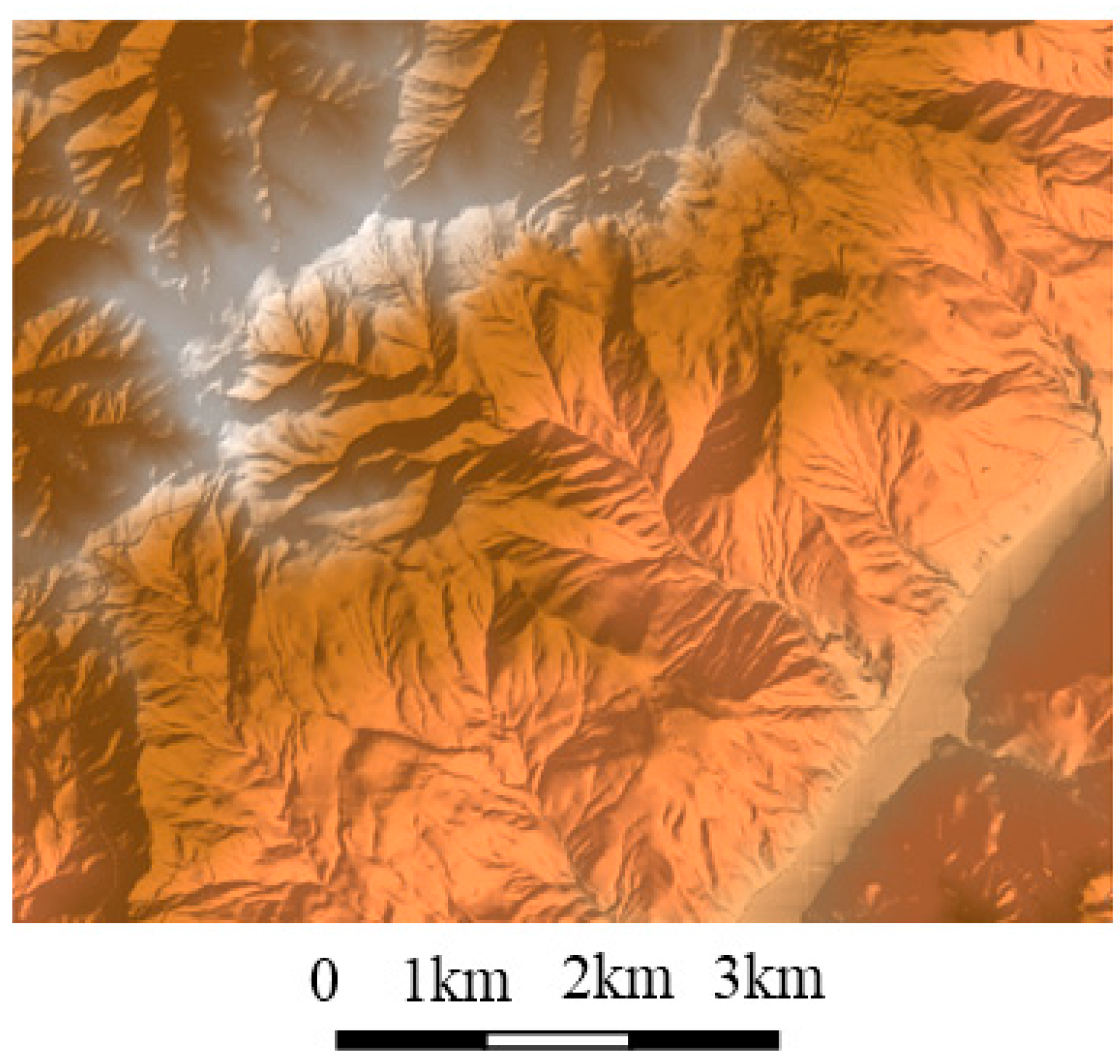
4.1.2. Digital Elevation Model (DEM)

4.2. Influencing Factors
4.3. Analytic Hierarchy Process
| Intensity of Weight | Definition | Explanation |
|---|---|---|
| 1 | Equal importance | Two factors contribute equally to objectives |
| 3 | Weak/moderate importance of one over another | Experience and judgment slightly favored one factor over another |
| 5 | Essential or strong importance | Experience and judgment strongly favor one factor over another |
| 7 | Very strong or demonstrated importance | One factor is favored very strongly over another; its dominance demonstrated in practice |
| 9 | Absolute importance | Evidence favoring one factor over another is of the highest possible order of affirmation |
| 2,4,6,8 | Intermediate values between the two adjacent scale values | Used to represent compromise between the priorities listed above |
| Reciprocals of above non-zero numbers | If factor i has one of the above non-zero numbers assigned to it when compared to factor j, then factor j has the reciprocal value when compared with factor i |
| Order | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
|---|---|---|---|---|---|---|---|---|
| RI | 0.58 | 0.90 | 1.12 | 1.24 | 1.32 | 1.41 | 1.45 | 1.49 |
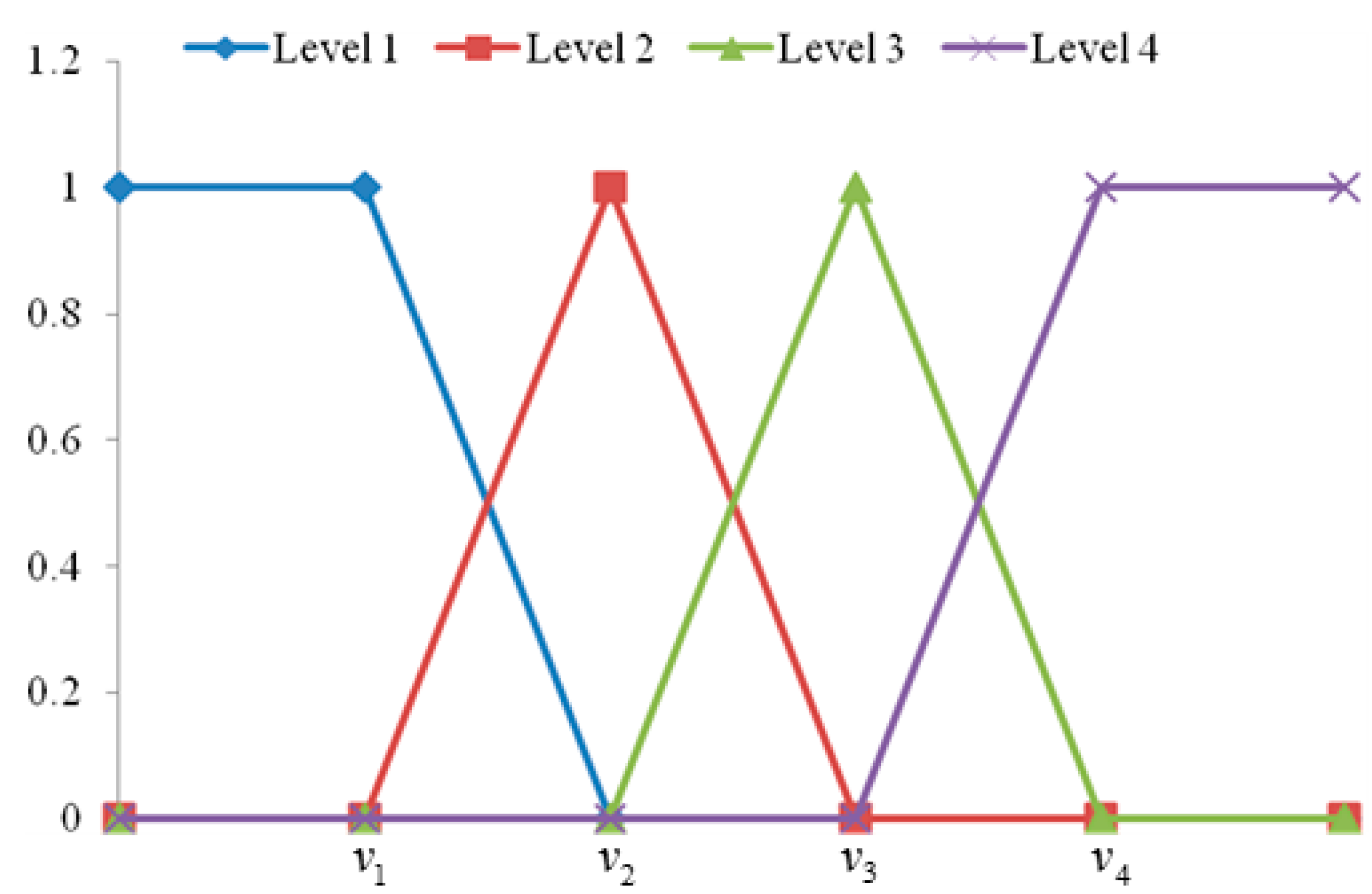
4.4. Fuzzy Synthetic Evaluation
5. Results
| Gully | M (×104 m3) | F (Numbers/100 Years) | S1 (km2) | S2 (km) | S3 (km) | S4 (km−1) | S5 | S6 | S7 (mm) | S8 (Person/km2) |
|---|---|---|---|---|---|---|---|---|---|---|
| Xiabaitan | 7.04 | 54 | 3.1 | 3.08 | 1.26 | 5.51 | 1.19 | 0.22 | 110 | 40 |
| Shangbaitan | 5.7 | 74 | 0.91 | 1.87 | 0.67 | 10.29 | 1.08 | 0.59 | 110 | 110 |
| Zhugongdi | 6.89 | 23 | 6.5 | 4.98 | 1.34 | 6.24 | 1.15 | 0.5 | 110 | 50 |
| Yindi | 23.6 | 35 | 60.5 | 20.17 | 2.25 | 5.08 | 1.23 | 0.24 | 110 | 20 |
| Canyu | 25.73 | 23 | 256 | 29.63 | 1.48 | 2.26 | 1.47 | 0.26 | 110 | 4 |
| Xiushui | 3.9 | 5 | 8.58 | 2.2 | 1.67 | 6.9 | 1.2 | 0.35 | 110 | 5 |
| Menggu | 31.4 | 102 | 37.1 | 10.52 | 1.74 | 6.73 | 1.13 | 0.46 | 110 | 10 |
| Jiache | 68.7 | 599 | 15.6 | 5.07 | 1.33 | 7.4 | 1.22 | 0.56 | 110 | 6 |
| Fujia | 12.9 | 66 | 8.62 | 5.16 | 1.53 | 6.34 | 1.26 | 0.44 | 110 | 10 |
| Aiba | 35.2 | 323 | 6.66 | 5.09 | 1.43 | 8.43 | 1.19 | 0.87 | 110 | 40 |
| Zhuza | 31.2 | 11 | 152.6 | 26.3 | 1.3 | 4.32 | 1.7 | 0.08 | 110 | 4 |
| Heizhe | 15.035 | 2 | 51.7 | 13.9 | 1.31 | 5.12 | 1.15 | 0.12 | 110 | 9 |
| Yanshuijing | 18.5 | 5 | 48.58 | 14.43 | 1.35 | 9.25 | 1.22 | 0.29 | 110 | 2 |
| Nuozhacun | 77.9 | 577 | 32.61 | 10.5 | 1.15 | 4.96 | 1.17 | 0.62 | 110 | 30 |
| Lalakuang | 22.97 | 120 | 17.88 | 7.14 | 1.41 | 5.52 | 1.08 | 0.72 | 110 | 5 |
| Zhangmu | 5.54 | 47 | 4.62 | 5.39 | 0.73 | 9.7 | 1.42 | 0.6 | 110 | 10 |
| Hepiao | 5.1 | 20 | 9.1 | 6.83 | 1.08 | 9.9 | 1.32 | 0.29 | 110 | 20 |
| Hongmenchang | 30.5 | 37 | 46.9 | 12.9 | 1.92 | 6.6 | 1.29 | 0.54 | 110 | 20 |
| Tianfang | 23 | 171 | 13.1 | 5.6 | 1.06 | 9.3 | 1.17 | 0.61 | 110 | 30 |
| Zhili | 46.5 | 19 | 120.6 | 15.8 | 1.61 | 6.3 | 1.28 | 0.45 | 110 | 12 |
| Yajiede | 6.86 | 6 | 22.3 | 9.3 | 1.61 | 4.7 | 1.31 | 0.18 | 110 | 11 |
| Pingdicun | 11.5 | 110 | 24.2 | 9.9 | 1.47 | 5.9 | 1.14 | 0.73 | 110 | 30 |
| Fangshanguo | 47.9 | 27 | 98 | 20.2 | 1.39 | 4.63 | 1.38 | 0.67 | 110 | 30 |
| Daqian | 13.5 | 74 | 18.9 | 5.1 | 0.59 | 10.95 | 1.11 | 0.744 | 110 | 15 |
| Fapa | 16.41 | 12 | 24.1 | 13.12 | 1.43 | 5.22 | 1.26 | 0.47 | 110 | 30 |
| Daqing | 7.22 | 6 | 31.8 | 7.32 | 0.64 | 6.02 | 1.1 | 0.49 | 110 | 10 |
| Factor | Classification Level Threshold | Factor | Classification Level Threshold | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Slight | Moderate | Severe | High | Slight | Moderate | Severe | High | ||
| M (×104 m3) | 1 | 10 | 100 | 200 | S4 (km−1) | 5 | 10 | 20 | 50 |
| F (numbers/100 years) | 10 | 50 | 100 | 200 | S5 | 1.1 | 1.25 | 1.4 | 1.6 |
| S1 (km2) | 0.5 | 10 | 35 | 50 | S6 | 0.1 | 0.3 | 0.6 | 1 |
| S2 (km) | 1 | 5 | 10 | 20 | S7 (mm) | 25 | 50 | 100 | 200 |
| S3 (km) | 0.2 | 0.5 | 1 | 2 | S8 (person/km2) | 50 | 150 | 250 | 350 |
| Debris-Flow Catchment | The Relative Importance Set | Assessment Level | Extension Theory | Grey Relation | |||
|---|---|---|---|---|---|---|---|
| Slight | Moderate | Severe | High | ||||
| Xiabaitan | 0.385 | 0.512 | 0.086 | 0.017 | Moderate | Moderate | Moderate |
| Shangbaitan | 0.360 | 0.437 | 0.200 | 0.003 | Moderate | Moderate | Moderate |
| Zhugongdi | 0.423 | 0.467 | 0.089 | 0.022 | Moderate | Moderate | Moderate |
| Yindi | 0.241 | 0.391 | 0.061 | 0.307 | Moderate | Moderate | Moderate |
| Canyu | 0.306 | 0.305 | 0.106 | 0.283 | Slight | Slight | Slight |
| Xiushui | 0.579 | 0.330 | 0.050 | 0.041 | Slight | Slight | Slight |
| Menggu | 0.108 | 0.244 | 0.572 | 0.077 | Severe | Severe | Severe |
| Jiache | 0.084 | 0.365 | 0.291 | 0.260 | Moderate | Moderate | Moderate |
| Fujia | 0.129 | 0.679 | 0.159 | 0.033 | Moderate | Moderate | Moderate |
| Aiba | 0.121 | 0.448 | 0.138 | 0.292 | Moderate | Moderate | Moderate |
| Zhuza | 0.412 | 0.185 | 0.121 | 0.282 | Slight | Moderate | Slight |
| Heizhe | 0.418 | 0.237 | 0.127 | 0.219 | Slight | Slight | Slight |
| Yanshuijing | 0.281 | 0.364 | 0.145 | 0.209 | Moderate | Moderate | Moderate |
| Nuozhacun | 0.144 | 0.081 | 0.518 | 0.256 | Severe | Severe | Moderate |
| Lalakuang | 0.139 | 0.377 | 0.400 | 0.084 | Severe | Severe | Severe |
| Zhangmu | 0.259 | 0.627 | 0.110 | 0.004 | Moderate | Moderate | Moderate |
| Hepiao | 0.349 | 0.530 | 0.114 | 0.007 | Moderate | Moderate | Moderate |
| Hongmenchang | 0.179 | 0.399 | 0.211 | 0.211 | Moderate | Moderate | Moderate |
| Tianfang | 0.044 | 0.529 | 0.251 | 0.177 | Moderate | Moderate | Moderate |
| Zhili | 0.290 | 0.259 | 0.201 | 0.251 | Slight | Slight | Slight |
| Yajiede | 0.482 | 0.276 | 0.205 | 0.037 | Slight | Slight | Slight |
| Pingdicun | 0.126 | 0.333 | 0.474 | 0.066 | Severe | Severe | Severe |
| Fangshanguo | 0.271 | 0.244 | 0.205 | 0.280 | High | High | High |
| Daqian | 0.034 | 0.692 | 0.257 | 0.017 | Moderate | Moderate | Moderate |
| Fapa | 0.359 | 0.341 | 0.247 | 0.052 | Slight | Severe | Severe |
| Daqing | 0.440 | 0.309 | 0.249 | 0.003 | Slight | Slight | Slight |
6. Discussion
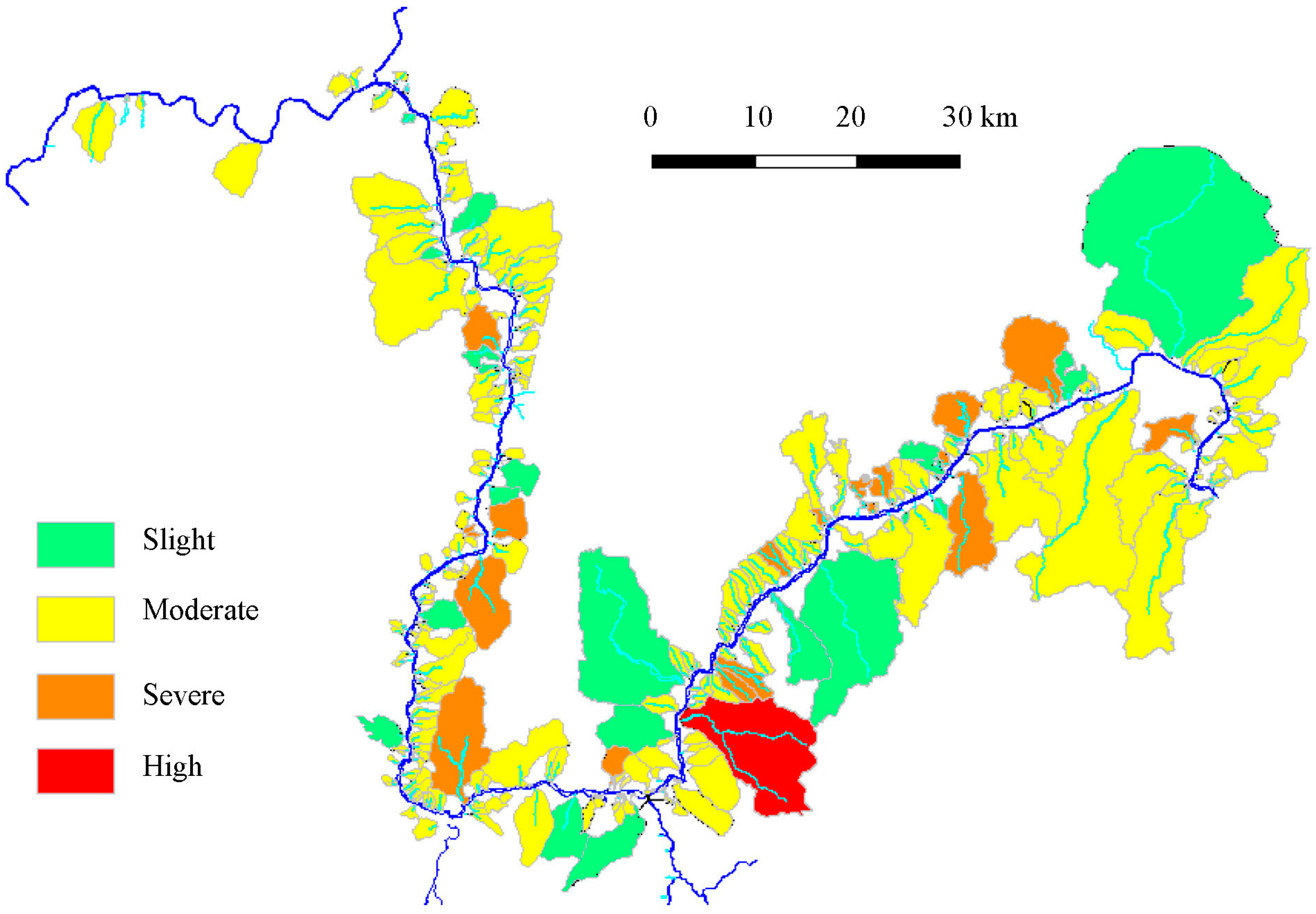
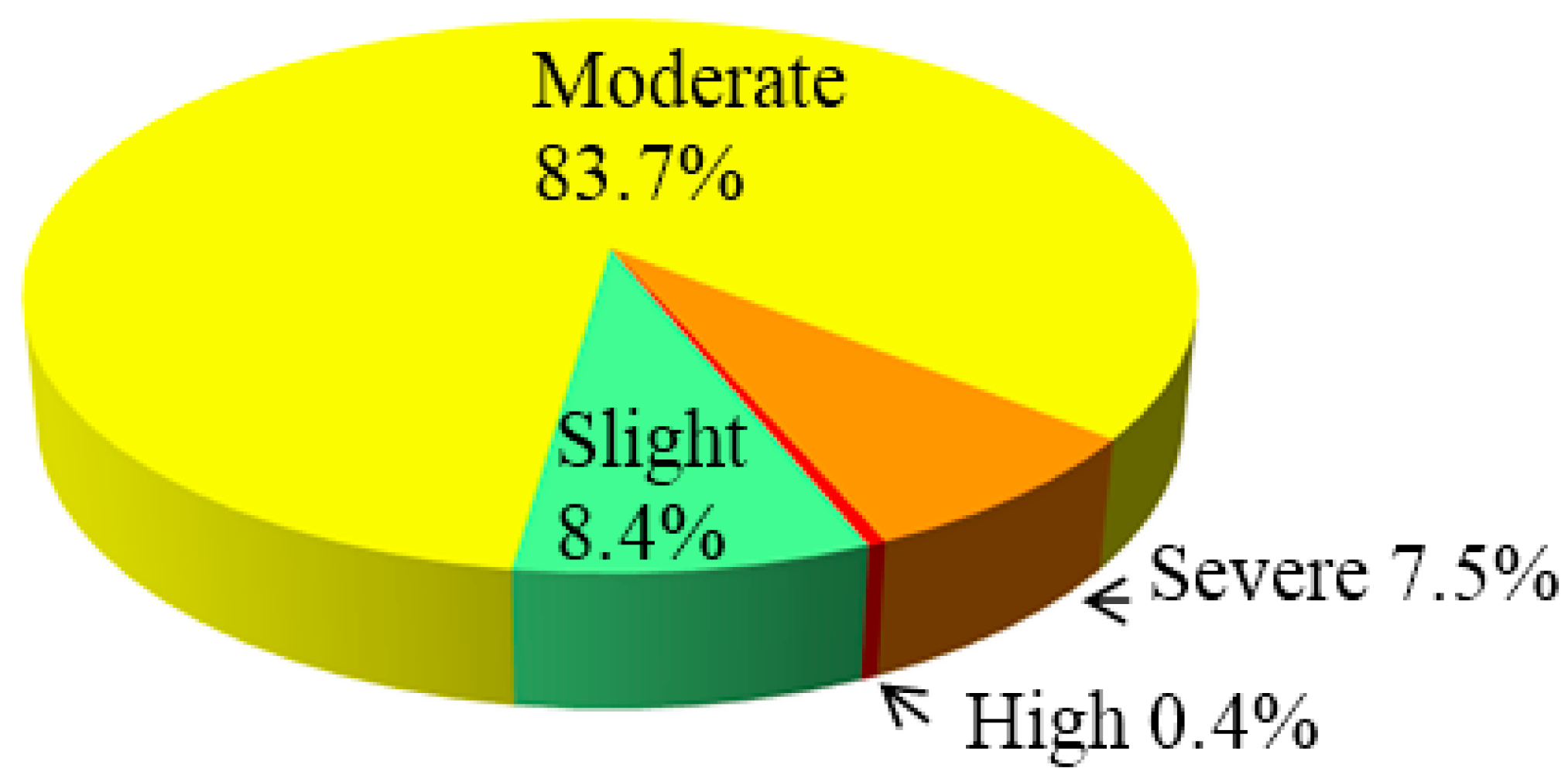
7. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Swanson, F.J.; Kratz, T.K.; Caine, N.; Woodmansee, R.G. Landform effects on ecosystem patterns and processes. BioScience 1988, 38, 92–98. [Google Scholar] [CrossRef]
- Swanson, F.J.; Johnson, S.L.; Gregory, S.V.; Acker, S.A. Flood disturbance in a forested mountain landscape. BioScience 1998, 48, 681–689. [Google Scholar] [CrossRef]
- Hürlimann, M.; Copons, R.; Altimir, J. Detailed debris flow hazard assessment in Andorra, A multidisciplinary approach. Geomorphology 2006, 78, 359–372. [Google Scholar] [CrossRef]
- Tang, C.; Vanasch, T.W.J.; Chang, M.; Chen, G.Q.; Zhao, X.H.; Huang, X.C. Catastrophic debris flows on 13 August 2010 in the Qingping area, southwestern China: The combined effects of a strong earthquake and subsequent rainstorms. Geomorphology 2012, 139–140, 559–576. [Google Scholar] [CrossRef]
- Cheng, Z.L.; Zhu, P.Y.; Liu, L.J. Study on the relationship between the debris flow and the rainfall factor. Nat. Hazards 1998, 7, 118–120. (In Chinese) [Google Scholar]
- Cui, P.; Liu, S.J.; Tan, W.P. Progress of debris flow forecast in China. J. Nat. Disasters 2000, 9, 10–15. [Google Scholar]
- Iverson, R.M. Physics of debris flows. Rev. Geophys. 1997, 35, 245–296. [Google Scholar] [CrossRef]
- Chiou, I.J.; Chen, C.H.; Liu, W.L.; Huang, S.M.; Chang, Y.M. Methodology of disaster risk assessment for debris flows in a river basin. Stoch. Environ. Res. Risk Assess. 2015, 29, 775–792. [Google Scholar] [CrossRef]
- Liang, W.J.; Zhuang, D.F.; Jiang, D.; Pan, J.J.; Ren, H.Y. Assessment of debris flow hazards using a Bayesian Network. Geomorphology 2012, 171, 94–100. [Google Scholar] [CrossRef]
- Liu, G.X.; Dai, E.F.; Ge, Q.S.; Wu, W.X.; Xu, X.C. A similarity-based quantitative model for assessing regional debris-flow hazard. Nat. Hazards 2013, 69, 295–310. [Google Scholar] [CrossRef]
- Eidsvig, U.M.K.; Papathoma-Köhle, M.; Du, J.; Glade, T.; Vangelsten, B.V. Quantification of uncertainty in debris flow vulnerability assessment. Eng. Geol. 2014, 181, 15–26. [Google Scholar] [CrossRef]
- Liu, X.L.; Tang, C. Debris-Flow Risk Evaluation; Science Press: Beijing, China, 1995. [Google Scholar]
- Waythomas, C.F. Formation and failure of volcanic debris dam in the Chakachatna River valley associated with eruptions of the spur volcanic complex, Alaska. Geomorphology 2001, 39, 111–129. [Google Scholar] [CrossRef]
- Petley, D. Global patterns of loss of life from landslides. Geology 2012, 40, 927–930. [Google Scholar] [CrossRef]
- Liu, X.; Yue, Z.Q.; Tham, L.G.; Lee, C.F. Empirical assessment of debris flow risk on a regional scale in Yunnan province, Southwestern China. Environ. Manag. 2002, 30, 249–264. [Google Scholar] [CrossRef] [PubMed]
- Jordan, P.; Covert, S.A. Debris Flows and Floods Following the 2003 Wildfires in Southern British Columbia. Eviron. Eng. Geosci. 2009, 15, 217–234. [Google Scholar] [CrossRef]
- Lin, P.S.; Lin, J.Y.; Hung, J.C.; Yang, M.D. Assessing debris-flow hazard in a watershed in Taiwan. Eng. Geol. 2002, 66, 295–313. [Google Scholar] [CrossRef]
- Liu, X.L.; Lei, J.Z. A method for assessing regional debris flow risk: An application in Zhaotong of Yunnan province (SW China). Geomorphology 2003, 52, 181–191. [Google Scholar] [CrossRef]
- Chang, T.C.; Chao, R.J. Application of back-propagation networks in debris flow prediction. Eng. Geol. 2006, 85, 270–280. [Google Scholar] [CrossRef]
- Chang, T.C. Risk degree of debris flow applying neural networks. Nat. Hazards 2007, 42, 209–224. [Google Scholar] [CrossRef]
- Procter, J.N.; Cronin, S.J.; Zernack, A.V.; Lube, G.; Stewart, R.B.; Nemeth, K.; Keys, H. Debris flow evolution and the activation of an explosive hydrothermal system; Te Maari, Tongariro, New Zealand. J. Volcanol. Geotherm. Res. 2014, 286, 303–316. [Google Scholar] [CrossRef]
- Di, B.F.; Chen, N.S.; Cui, P.; Li, Z.L.; He, Y.P.; Gao, Y.C. GIS-based risk analysis of debris flow: An application in Sichuan, southwest China. Int. J. Sediment. Res. 2008, 23, 138–148. [Google Scholar] [CrossRef]
- Saaty, T.L. Modeling unstructured decision problems-the theory of analytical hierarchies. Math. Comput. Simul. 1977, 20, 147–158. [Google Scholar] [CrossRef]
- Saaty, T.L. The Analytic Hierarchy Process: Planning, Priority Setting Resource Allocation; McGraw-Hill: New York, NY, USA, 1980. [Google Scholar]
- Mi, C.Q.; Zhang, X.D.; Li, S.M.; Yang, J.Y.; Zhu, D.H.; Yang, Y. Assessment of environment lodging stress for maize using fuzzy synthetic evaluation. Math. Comput. Model. 2011, 54, 1053–1060. [Google Scholar] [CrossRef]
- Wang, J.H.; Lu, X.G.; Jiang, M. Fuzzy Synthetic Evaluation of Wetland Soil Quality Degradation: A Case Study on the Sanjiang Plain Northeast China. Pedosphere 2009, 19, 756–764. [Google Scholar] [CrossRef]
- Duan, Z.F.; Pang, Z.H.; Wang, X.Y. Sustainability evaluation of limestone geothermal reservoirs with extended production histories in Beijing and Tianjin, China. Geothermics 2011, 40, 125–135. [Google Scholar] [CrossRef]
- Lu, X.W.; Loretta, Y.L.; Lei, K.; Wang, L.J.; Zhai, Y.X.; Zhai, M. Water quality assessment of Wei River, China using fuzzy synthetic evaluation. Environ. Earth Sci. 2010, 60, 1693–1699. [Google Scholar] [CrossRef]
- Kuang, Y.H.; Xu, L.R.; Liu, B.C.; Yao, J.C. A new method for choosing zonation indicators of mudflow danger degrees based on the rough set theory. J. Geomech. 2006, 12, 236–241. [Google Scholar]
- Liu, T.; Zhang, H.J.; Wu, J.D.; Hou, X.F.; Zheng, G.Q.; Ye, Z.H. Application of analytic hierarchy process in debris flow risk degree assessment—A case study of Miyun County, Beijing City. Bull. Soil Water Conserv. 2008, 28, 6–10. [Google Scholar]
- Li, C.K.; Goldstein, R.M. Studies of multibaseline spaceborne interferometric synthetic aperture radars. IEEE Trans. Geosci. Remote Sens. 1990, 28, 88–97. [Google Scholar] [CrossRef]
- Antrop, M.; Eetvelde, V.V. Changing patterns in the urbanized countryside of Western Europe. Landsc. Ecol. 2004, 15, 257–270. [Google Scholar] [CrossRef]
- Thomlinsson, J.R.; Rivera, L.Y. Suburban growth in Luquillo, Puerto Rico: Some consequences of development on natural and semi-natural systems. Landsc. Urban. Plan. 2006, 49, 19–23. [Google Scholar] [CrossRef]
- Wan, S.; Lei, T.C.; Huang, P.C.; Chou, T.Y. The knowledge rules of debris flow event: A case study for investigation Chen Yu Lan River, Taiwan. Eng. Geol. 2008, 98, 102–114. [Google Scholar] [CrossRef]
- Wan, S.; Lei, T.C. A knowledge-based decision support system to analyze the debris-flow problems at Chen-Yu-Lan River, Taiwan. Knowl. Based Syst. 2009, 22, 580–588. [Google Scholar] [CrossRef]
- Chen, J.; Chen, Y.H.; He, C.Y.; Shi, P.J. Sub-pixel model for vegetation fraction estimation based on land cover classification. J. Remote Sens. 2001, 5, 416–422. [Google Scholar]
- Dan, S.M.; Xu, H.X.; Dan, B.; He, F.; Shi, C.C.; Ren, G.Y. Monitoring and evaluation of grassland desertification in litang county using multi-temporal remote sensing images. In Proceedings of the 14th Youth Conference on Communication, Dalian, China, 24–26 July 2009; pp. 209–213.
- Hungr, O.; Morgan, G.C.; Kellerhals, R. Quantitative analysis of debris torrent hazards for design of remedial measures. Canad. Geotech. J. 1984, 21, 663–677. [Google Scholar] [CrossRef]
- Ning, N.; Shu, H.P.; Liu, D.F.; Ma, J.Z. Hazard assessment of debris flow based on the entropy weight method and fuzzy evaluation method. J. Lanzhou Univ. Nat. Sci. 2014, 3, 369–375. [Google Scholar]
- Luo, Z.; Yang, S. Comparative study on several scales in AHP. Syst. Eng. Theor. Pract. 2004, 9, 51–60. [Google Scholar]
- Crawford, G.; Williams, C. A note on the analysis of subjective judgment matrices. J. Math. Psychol. 1985, 29, 387–405. [Google Scholar] [CrossRef]
- Sudhir, D.; Bupinder, S.; Shalini, G. Analysis of groundwater quality using fuzzy synthetic evaluation. J. Hazard. Mater. 2007, 147, 938–946. [Google Scholar] [CrossRef] [PubMed]
- Guleda, O.E.; Ibrahim, D.; Halil, H. Assessment of urban air quality in Istanbul using fuzzy synthetic evaluation. Atmos. Environ. 2004, 38, 3809–3815. [Google Scholar] [CrossRef]
- Chang, N.B.; Chen, H.W.; Ning, S.K. Identification of river water quality using the Fuzzy synthetic evaluation approach. J. Environ. Manag. 2001, 63, 293–305. [Google Scholar] [CrossRef] [PubMed]
- Zhang, W.; Chen, J.P.; Wang, Q. Susceptibility analysis of large-scale debris flows based on combination weighting and extension methods. Nat. Hazards 2013, 66, 1073–1100. [Google Scholar] [CrossRef]
- Niu, C.C.; Wang, Q.; Chen, J.P.; Wang, K.; Zhang, W.; Zhou, F.J. Debris-flow hazard assessment based on stepwise discriminant analysis and extension theory. Q. J. Eng. Geol. Hydrogeol. 2014, 47, 211–222. [Google Scholar] [CrossRef]
- Zhang, W.; Li, H.Z.; Chen, J.P.; Zhang, C.; Xu, L.M.; Sang, W.F. Comprehensive hazard assessment and protection of debris flows along Jinsha River close to the Wudongde dam site in China. Nat. Hazards 2011, 58, 459–477. [Google Scholar] [CrossRef]
- Zhao, B.; Xu, W.Y.; Liang, G.L.; Meng, Y.D. Stability evaluation model for high rock slope based on element extension theory. Bull. Eng. Geol. Environ. 2015, 74, 301–314. [Google Scholar] [CrossRef]
- Kuang, H.B.; Kilgour, D.M.; Hipel, K.W. Grey-based PROMETHEE II with application to evaluation of source water protection strategies. Inf. Sci. 2015, 294, 376–389. [Google Scholar] [CrossRef]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niu, C.; Wang, Q.; Chen, J.; Zhang, W.; Xu, L.; Wang, K. Hazard Assessment of Debris Flows in the Reservoir Region of Wudongde Hydropower Station in China. Sustainability 2015, 7, 15099-15118. https://doi.org/10.3390/su71115099
Niu C, Wang Q, Chen J, Zhang W, Xu L, Wang K. Hazard Assessment of Debris Flows in the Reservoir Region of Wudongde Hydropower Station in China. Sustainability. 2015; 7(11):15099-15118. https://doi.org/10.3390/su71115099
Chicago/Turabian StyleNiu, Cencen, Qing Wang, Jianping Chen, Wen Zhang, Liming Xu, and Ke Wang. 2015. "Hazard Assessment of Debris Flows in the Reservoir Region of Wudongde Hydropower Station in China" Sustainability 7, no. 11: 15099-15118. https://doi.org/10.3390/su71115099
APA StyleNiu, C., Wang, Q., Chen, J., Zhang, W., Xu, L., & Wang, K. (2015). Hazard Assessment of Debris Flows in the Reservoir Region of Wudongde Hydropower Station in China. Sustainability, 7(11), 15099-15118. https://doi.org/10.3390/su71115099







