Influence of the Thermal Inertia in the European Simplified Procedures for the Assessment of Buildings’ Energy Performance
Abstract
:1. Introduction


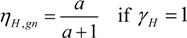
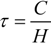

2. Modeling
| Material | Thermal Conductivity [W/m K] | Specific Heat Capacity [kJ/kg K] | Mass Density [kg/m3] |
|---|---|---|---|
| Heavy Concrete | 1.700 | 0.84 | 2200 |
| XPS | 0.034 | 1.45 | 33.5 |
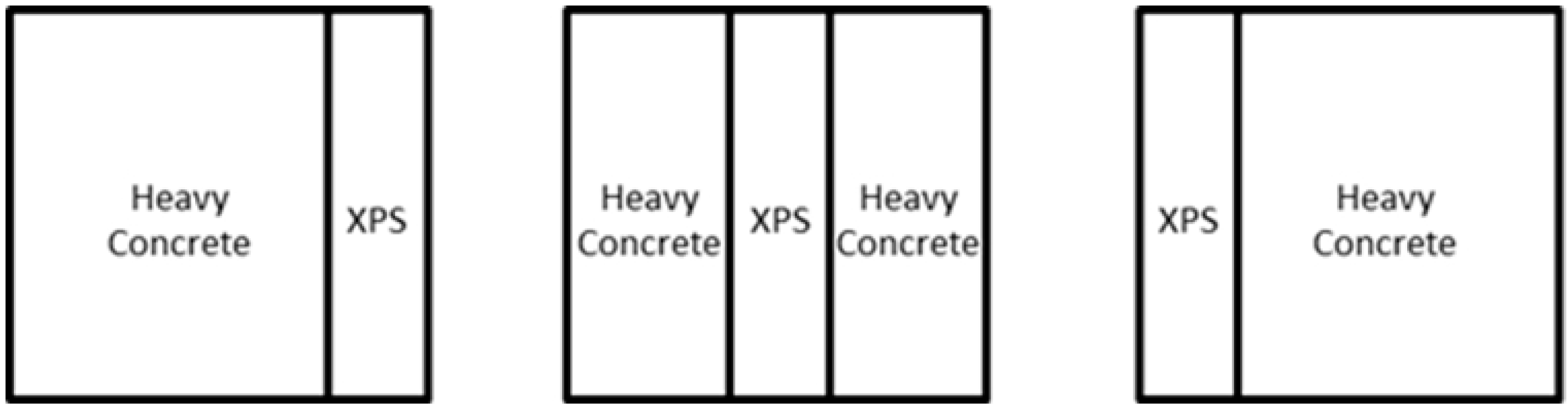
| − | Wall 2 | Wall 3 | |||
|---|---|---|---|---|---|
| Thickness [m] | Thickness [m] | Thickness [m] | |||
| Ext | − | Ext | − | Ext | − |
| Heavy Concrete | 0.2 | Heavy Concrete | 0.1 | XPS | 0.02 |
| XPS | 0.02 | XPS | 0.02 | Heavy Concrete | 0.2 |
| Heavy Concrete | 0.1 | ||||
| Int | − | Int | − | Int | − |
| U-Value [W/m2 K] | 1.139 | U-Value [W/m2 K] | 1.139 | U-Value [W/m2 K] | 1.139 |
| Solar Absorbance | 0.6 | Solar Absorbance | 0.6 | Solar Absorbance | 0.6 |
3. Results and Discussion
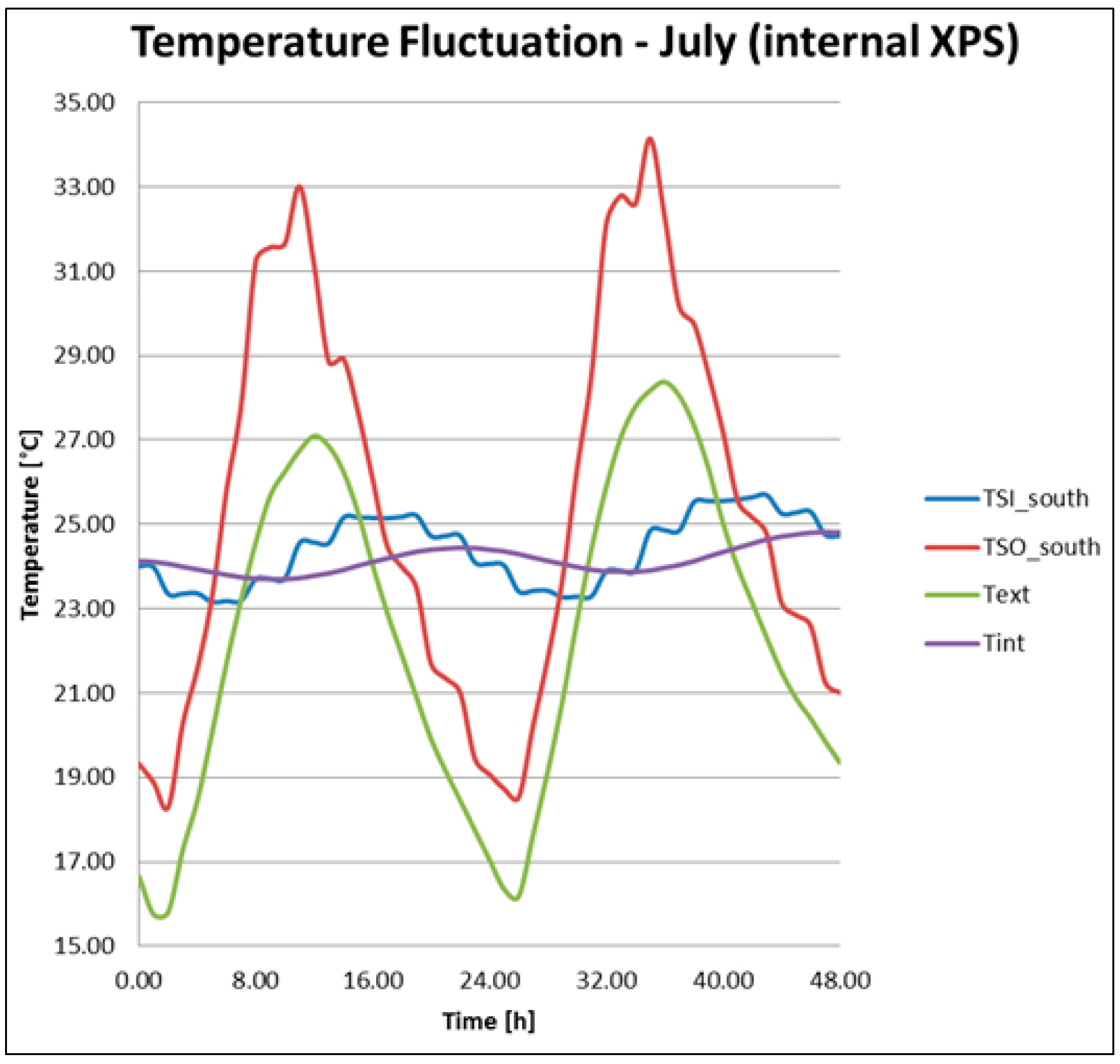
| Wall 1 | Maximum External Surface Temperature [°C] | Maximum Internal Surface Temperature [°C] | Surface Temperature Variation (Ext-Int) [°C] | Thermal Lag [h] |
| 33.01 | 25.21 | 7.80 | 7 |

| Wall 2 | Maximum External Surface Temperature [°C] | Maximum Internal Surface Temperature [°C] | Surface Temperature Variation (Ext-Int) [°C] | Thermal Lag [h] |
| 34.78 | 24.89 | 9.90 | 6 |
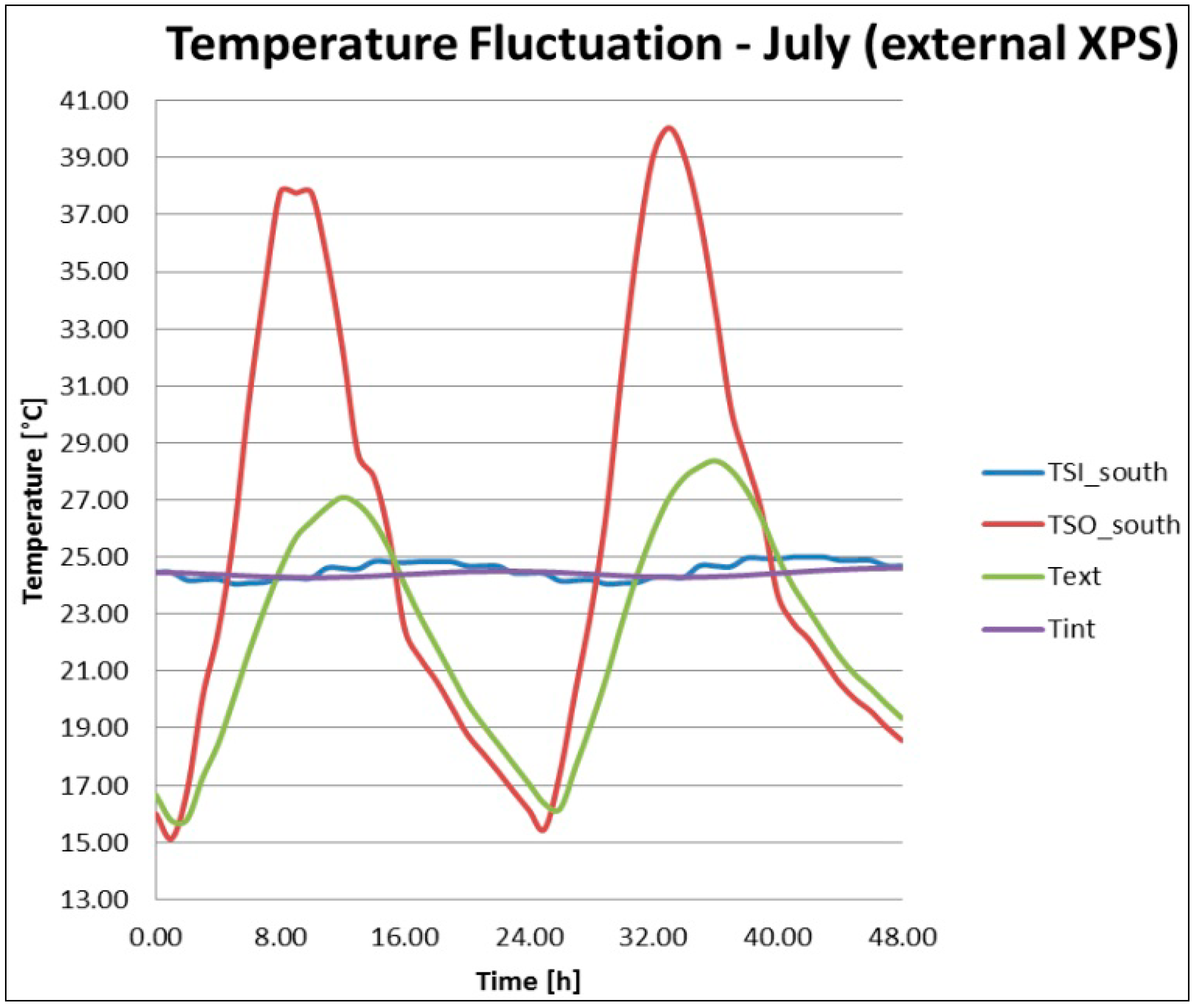
| Wall 3 | Maximum External Surface Temperature [°C] | Maximum Internal Surface Temperature [°C] | Surface Temperature Variation (Ext-Int) [°C] | Thermal Lag [h] |
| 37.81 | 24.85 | 12.96 | 9 |
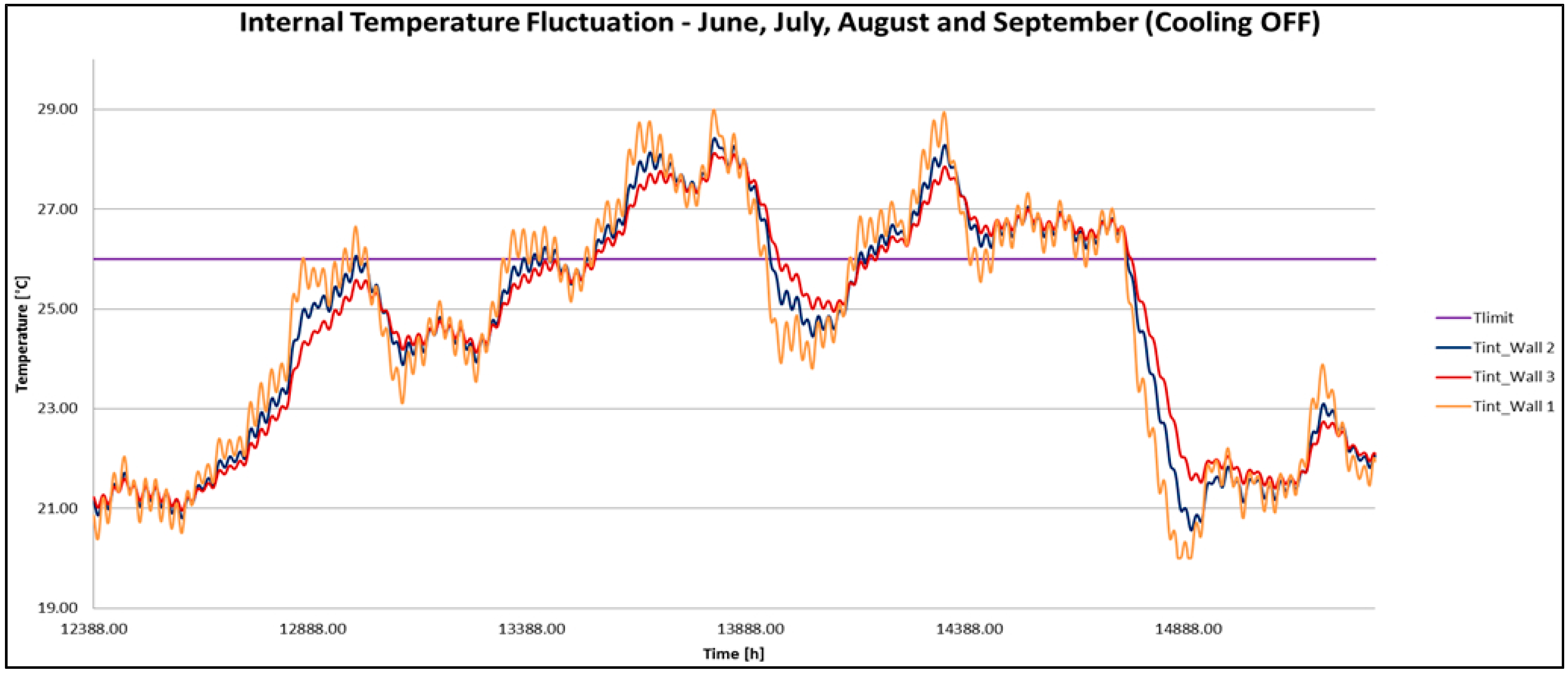
| UNI EN ISO 13786 | Thermal Lag [h] | Attenuation Factor |
|---|---|---|
| Wall 1 | 5.7 | 0.451 |
| Wall 2 | 7.2 | 0.418 |
| Wall 3 | 6.7 | 0.266 |
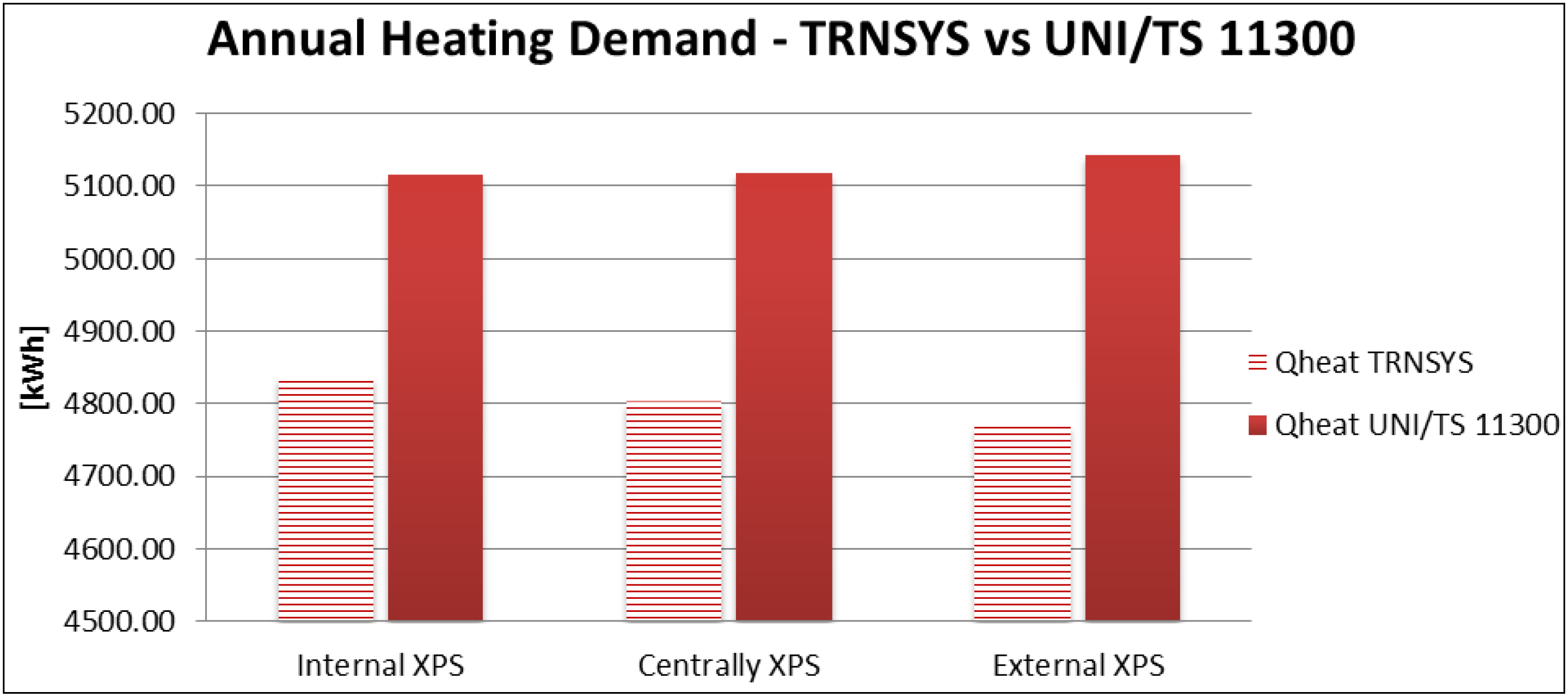
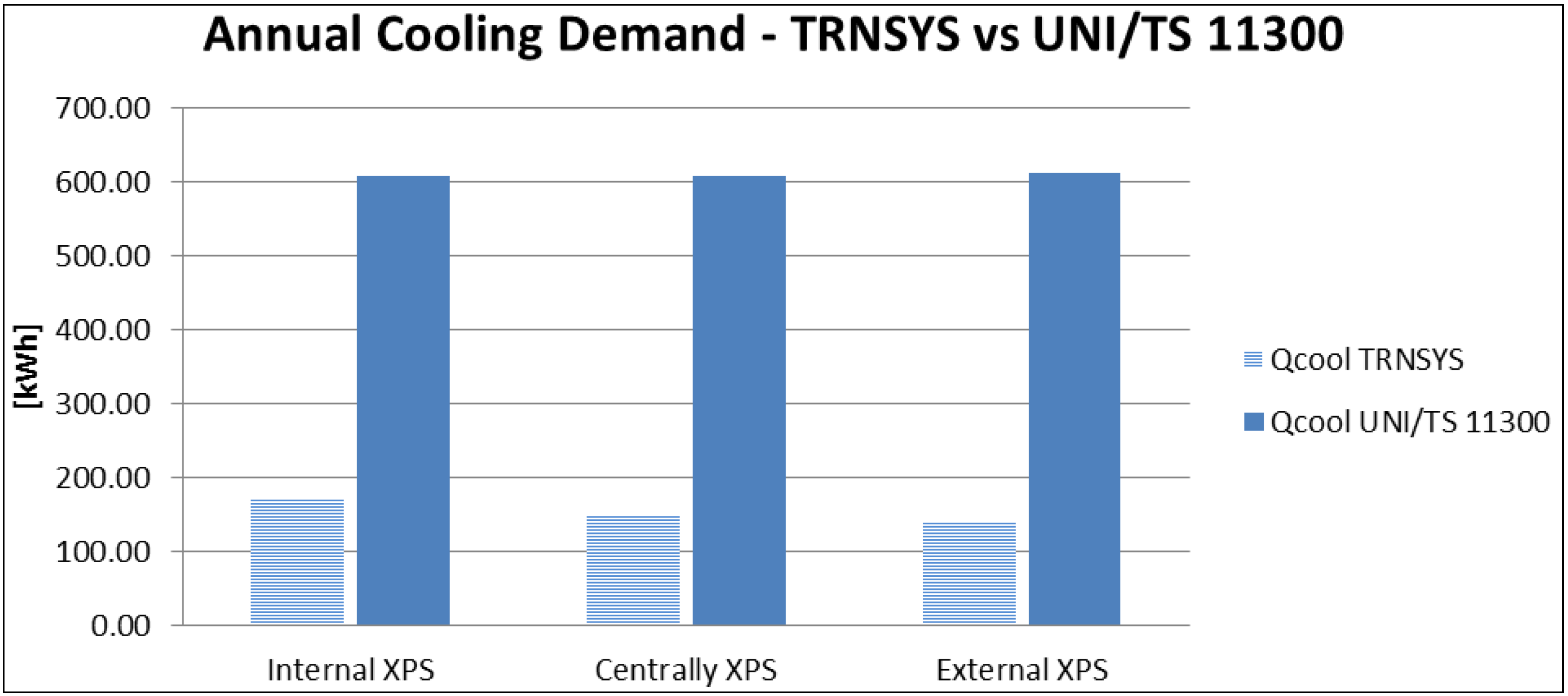
| Wall type | QH,nd TRNSYS [kWh] | QH,nd UNI TS 11300 [kWh] | Heating Percentage Difference [%] | QC,nd TRNSYS [kWh] | QC,nd UNI TS 11300 [kWh] | Cooling Percentage Difference [%] |
|---|---|---|---|---|---|---|
| Wall 1 | 4837.63 | 5115.19 | −5.43 | 170.49 | 607.78 | −71.95 |
| Wall 2 | 4802.31 | 5117.70 | −6.16 | 148.22 | 608.43 | −77.09 |
| Wall 3 | 4771.66 | 5142.67 | −7.21 | 140.02 | 611.71 | −77.11 |
4. Conclusions
Author Contributions
Nomenclature
| QH,nd | Energy demand for heating [Wh] |
| QC,nd | Energy demand for cooling [Wh] |
| QH,tr | Heat dissipation through opaque and transparent surfaces [Wh] |
| QH,ve | Ventilation losses [Wh] |
| Qint | Internal gains [Wh] |
| Qsol | Solar gains [Wh] |
| ηH,gn | Utilization factor of thermal contributions |
| ηC,Is | Utilization factor of thermal dispersion |
| C | Internal heat capacity [J/K] |
| H | Total heat loss coefficient [W/K] |
| τ | Time constant [h] |
| γH | Total gains and total heat dissipation ratio |
| QH,hr | Total heat dissipation [Wh] |
| Qgn | Total gains [Wh] |
| a | Numerical parameter |
| XPS | Extruded polystyrene |
| U-Value | Thermal transmittance value [W/m2K] |
Conflicts of Interest
References and Notes
- European Parliament. European Directive 2002/91/CE on the energy performance of buildings. Available online: http://eur-lex.europa.eu/legal-content/EN/TXT/?uri=CELEX:32002L0091 (accessed on 5 June 2014).
- Gregory, K.; Moghtaderi, B.; Sugo, H.; Page, A. Effect of thermal mass on the thermal performance of various Australian residential construction systems. Energy Build. 2008, 40, 459–465. [Google Scholar] [CrossRef]
- Collet, F.; Serres, L.; Miriel, J.; Bart, M. Study of thermal behavior of clay wall facing south. Build. Environ. 2006, 41, 307–315. [Google Scholar] [CrossRef]
- Bojic, M.; Yik, F.; Sat, P. Influence of thermal insulation position in building envelope on the space cooling of high-rise residential building in Hong Kong. Energy Build. 2001, 33, 569–581. [Google Scholar] [CrossRef]
- Aste, N.; Angelotti, A.; Buzzetti, M. The influence of the external walls thermal inertia on the energy performance of well insulated buildings. Energy Build. 2009, 41, 1181–1187. [Google Scholar] [CrossRef]
- Bond, D.E.M.; Clark, W.W.; Kimber, M. Configuring wall layers for improved insulation performance. Appl. Energy 2013, 112, 235–245. [Google Scholar] [CrossRef]
- Kossecka, E.; Kosny, J. Influence of insulation configuration on heating and cooling loads in a continuously used building. Energy Build. 2002, 34, 321–331. [Google Scholar] [CrossRef]
- De LietoVollaro, R.; Evangelisti, L.; Carnielo, E.; Basilicata, C.; Battista, G.; Gori, P.; Guattari, C.; Fanchiotti, A. An Integrated Approach for an Historical Buildings Energy Analysis in a Smart Cities Perspective. Energy Procedia 2014, 45, 372–378. [Google Scholar] [CrossRef]
- Ferrari, S.; Baldinazzo, M. Assessment of the energy performance of buildings: From simplifiedprocedures to dynamicanalysis. In Proceedings of the AICARR Conference, Certificazione energetica: Normative e modelli di calcolo per il sistema edificio-impianto posti a confronto.
- UNI TS 11300-1, Determination of the buildingsenergydemand for the air-conditioning in summer and winter. (In Italian: Determinazione del fabbisogno di energia termica dell’edificio per la climatizzazione estiva ed invernale.)
- Asdrubali, F.; Bonaut, M.; Battisti, M.; Venegas, M. Comparative study of energy regulations for buildings in Italy and Spain. Energy Build. 2008, 40, 1805–1815. [Google Scholar] [CrossRef]
- UNI EN ISO 13790, Energy performance of buildings—Calculation of energy use for heating and cooling.
- UNI EN ISO 13786, Thermal performance of building components—Dynamic thermal characteristics—Calculation methods.
- Transient System Simulation Tool. Available online: http://sel.me.wisc.edu/trnsys (accessed on 5 June 2014).
- Mitalas, G.P. Transfer function method of calculating cooling loads, heat extraction and space temperature. ASHRAE J. 1972, 14, 54–56. [Google Scholar]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Evangelisti, L.; Battista, G.; Guattari, C.; Basilicata, C.; De Lieto Vollaro, R. Influence of the Thermal Inertia in the European Simplified Procedures for the Assessment of Buildings’ Energy Performance. Sustainability 2014, 6, 4514-4524. https://doi.org/10.3390/su6074514
Evangelisti L, Battista G, Guattari C, Basilicata C, De Lieto Vollaro R. Influence of the Thermal Inertia in the European Simplified Procedures for the Assessment of Buildings’ Energy Performance. Sustainability. 2014; 6(7):4514-4524. https://doi.org/10.3390/su6074514
Chicago/Turabian StyleEvangelisti, Luca, Gabriele Battista, Claudia Guattari, Carmine Basilicata, and Roberto De Lieto Vollaro. 2014. "Influence of the Thermal Inertia in the European Simplified Procedures for the Assessment of Buildings’ Energy Performance" Sustainability 6, no. 7: 4514-4524. https://doi.org/10.3390/su6074514
APA StyleEvangelisti, L., Battista, G., Guattari, C., Basilicata, C., & De Lieto Vollaro, R. (2014). Influence of the Thermal Inertia in the European Simplified Procedures for the Assessment of Buildings’ Energy Performance. Sustainability, 6(7), 4514-4524. https://doi.org/10.3390/su6074514








