4.2. Contribution of Informatization Investment to Economic Growth
First, we analyzed the degree of influence of Japanese regional informatization investment on each unit of regional economic growth from 1980 to 2007. From a preliminary observation of
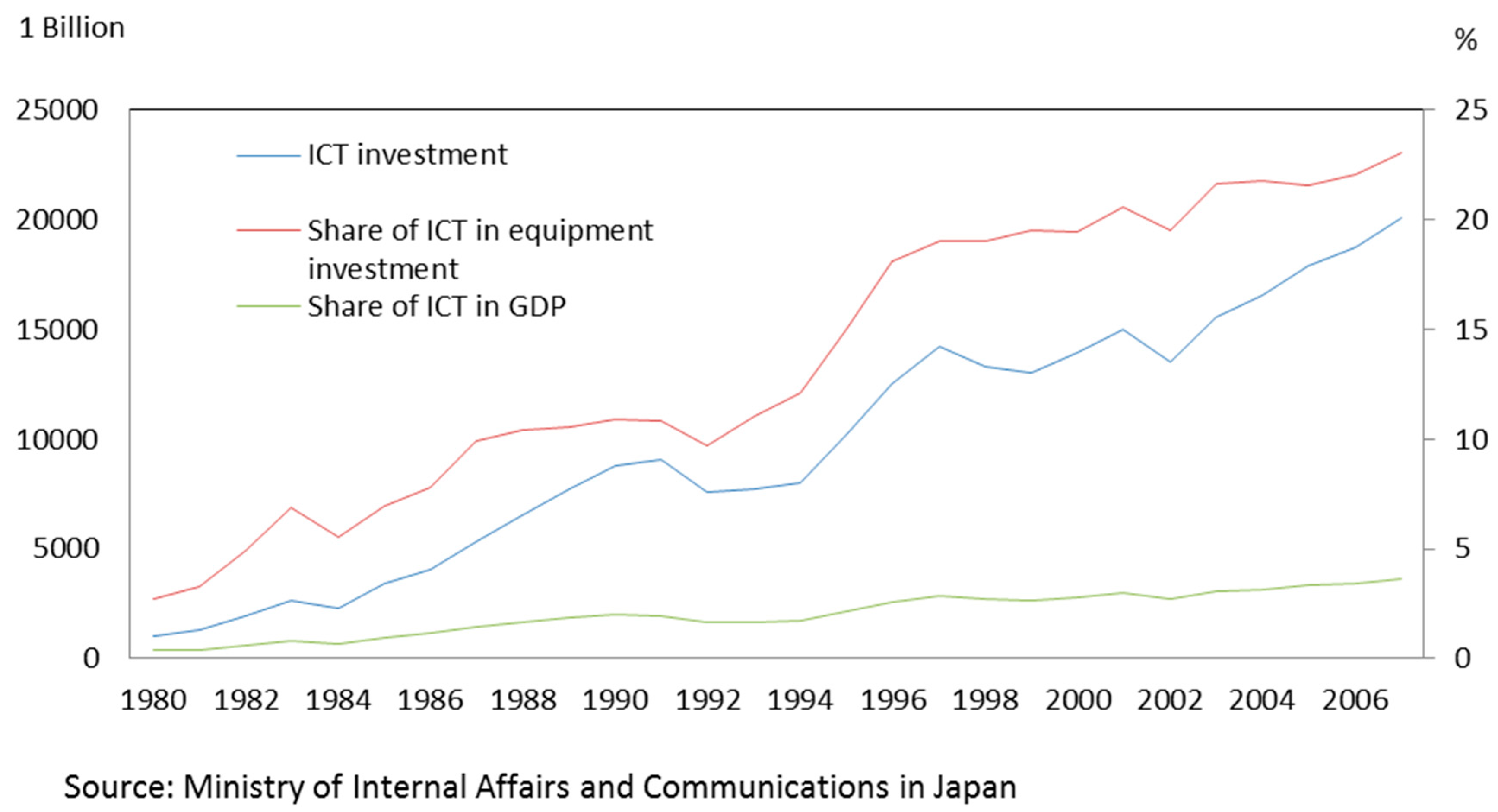
Figure 1, 1997 seems to be the demarcation point of informatization investment. This needs to be further verified and confirmed. The article adopts the collective value of Japanese regional ICT investment data to test Equation (2); the result shows that the test statistics, at F = 6.2969 and LR = 22.8226, are significant; however, the test statistics for the adjacent years (
i.e., 1996 and 1998) are not significant. Therefore, 1997 can be considered a real catastrophic point. We analyze the three periods 1980–2007, 1980–1996, and 1997–2007 separately; for convenience, we use protophase and anaphase to represent the two successive phases, 1980–1996 and 1997–2007, respectively.
For spatial analysis, we should first determine the model to be adopted for estimation. The process could be divided into three steps: First, determine whether to adopt the spatial lag model (SAR), spatial error model (SEM), or a common non-spatial model. Second, determine whether a fixed effect model or random effect model should be adopted; if a fixed effect model is employed, a spatial fixed effect, time fixed effect, or bidirectional fixed effect must be chosen. Third, determine whether the spatial Durbin model is suitable or not [
18,
19,
20]. The model test results for the three periods can be summarized as follows.
First, for the feasibility of the non-spatial model, we compared all test statistics, the Lagrange multiplier (LM) test result, spatial lag, and spatial error test for 1980–2007. The LM spatial lag test statistic is 160.12, and the LM spatial error is 39.29 in the non-spatial model, negating the no-spatial-effect hypothesis. Obviously, a spatial effect does exist. The robust LM test statistic, too, rejects the hypothesis of no spatial effect (robust LM lag: 122.67, robust LM error: 1.84). For 1980–1996, the test statistics are 81.65, 18.37, 65.77, and 2.49 respectively. For 1997–2007, the values are 107.10, 27.16, 84.55, and 4.61, respectively. Both the LM test and the robust LM test for the three periods show that the statistical magnitude of spatial lag is greater than the spatial error. Therefore, we can confirm that the spatial lag model provides a better result than does the spatial error model.
Second, the LM test result for 1980–2007 also shows that the spatial lag in the time effects of the statistical magnitude of LM and robust LM is larger than the spatial error while the spatial lag is lower than spatial error for the fixed effect. For the regional individual + time effects, the spatial lag is larger than the spatial error. The other two periods show similar characteristics. Moreover, the likelihood ratio test result for all three periods negates not only the possibility that the spatial fixed effect is not significant (2287.93, 2022.51, and 1005.92, degree of freedom = 47, p < 0.01) but also the null hypothesis that the time fixed effect is not significant (276.85, 184.52, and 176.02, degree of freedom = 28, p < 0.01), which shows the feasibility of incorporating spatial fixed effects or time fixed effects into the model.
Third, the results of the spatial Durbin model indicate that the random effect model with spatial lag is suitable for the periods of 1980–2007 and 1980–1996 (For 1980–2007, Wald lag = 2.8765, p = 0.5787; Wald error = 8.2522, p = 0.0828; LR lag = 4.0260, p = 0.4025; LR_error = 8.8985, p = 0.0637; Hausman = 12.8183, p = 0.1710. For 1980–1996, Wald lag = 2.3271, p = 0.6758; Wald error = 0.4736, p = 0.9760; LR lag = 1.9933, p = 0.7370; LR_error = 0.4491, p = 0.9783; Hausman = 16.8142, p = 0.0517. According to the Wald test and LR test, the random effect model should be employed for the analysis). However, the bidirectional fixed effect model with spatial lag is suitable for the period 1997–2007.
Table 1 shows the statistical results on the effect of regional informatization investment on economic growth in Japan from 1980 to 2007.
For 1980–2007, the random effect model has a better fit, indicating that the regional and temporal heterogeneity is independent of the explanatory variables in the long term. Therefore, we can consider them as random factors and include them in the model. From the degree of fit perspective, the R2 values for 1980–2007, 1980–1996, and 1997–2007 are 0.9344, 0.9683, and 0.9725, respectively, and the corrected R2 values are 0.6549, 0.5732, and 0.3728, respectively. Here, the difference between R2 and the corrected R2 is that the latter neglects the variance explained by the spatial fixed effect, implying that the fixed effect explains the whole variation. What is more, when taking the spatial lag of the explained variable as explanatory variable it takes R2 to explain, and when taking the spatial lag of the explained variable as no explanatory variable it takes corrected R2 to explain. It can be seen that spatial fixed effect should be incorporated into the model, which has a good fit overall.
Table 1.
Regional informatization and economic growth in Japan: spatial and time-period fixed effects (1980–2007).
Table 1.
Regional informatization and economic growth in Japan: spatial and time-period fixed effects (1980–2007).
| 1980–2007 | 1980–1996 | 1997–2007 |
|---|
| Spatial Random Effects | Spatial Random Effects | Spatial and Time-Period Fixed Effects |
|---|
| W × ln(GDP/L) | 0.1160 | 0.1360 | 0.0216 |
| (3.3661) | (3.0992) | (0.3908) |
| ln(NICT/L) | 0.3689 | 0.3557 | 0.7658 |
| (13.7340) | (10.7594) | (11.4621) |
| ln(ICT/L) | 0.0441 | 0.0438 | −0.1143 |
| (3.6558) | (2.9222) | (−2.1016) |
| ln(R&D) | 0.0063 | 0.0021 | 0.0071 |
| (5.0761) | (1.2910) | (3.0808) |
| ln(Tran) | 0.0232 | 0.0023 | 0.0907 |
| (3.3592) | (0.2918) | (3.3162) |
| W × ln(NICT/L) | 0.1247 | −0.0055 | −0.4220 |
| (2.6262) | (−0.0905) | (−4.1424) |
| W × ln(ICT/L) | −0.0384 | −0.0426 | 0.2800 |
| (−2.2990) | (−2.1397) | (4.0379) |
| W × ln(R&D) | 0.0051 | 0.0070 | −0.0140 |
| (1.9789) | (2.0104) | (−3.1874) |
| W × ln(Tran) | 0.0078 | 0.0119 | 0.4549 |
| (0.5605) | (0.7603) | (7.5783) |
| phi | 0.0929 | 0.0684 | |
| (6.8830) | (6.8698) | |
| σ2 | 0.0003 | 0.0002 | 0.0001 |
| R2 | 0.9344 | 0.9683 | 0.9725 |
| Corrected R2 | 0.6549 | 0.5732 | 0.3728 |
| Log L | 2941.6 | 2101.9 | 1606.5 |
| Wald test spatial lag | 20.1575 (p = 0.001) | 10.02 (p = 0.04) | 90.83 (p = 0.001) |
| Wald test spatial error | 32.3197 (p = 0.001) | 11.45 (p = 0.01) | 87.55 (p = 0.001) |
| LR test spatial lag | | | 90.88 (p = 0.001) |
| LR test spatial error | | | 88.59 (p = 0.001) |
| Direct effect ln(NICT/L) | 0.3755 | 0.3573 | 0.7629 |
| (14.160) | (10.789) | (11.540) |
| Direct effect ln(NICT/L) | 0.3755 | 0.3573 | 0.7629 |
| (14.160) | (10.789) | (11.540) |
| Indirect effect ln(NICT/L) | 0.1845 | 0.0497 | −0.4112 |
| (3.524) | (0.757) | (−4.248) |
| Total effect ln(NICT/L) | 0.5600 | 0.4070 | 0.3517 |
| (9.145) | (5.079) | (4.062) |
| Direct effect ln(ICT/L) | 0.0433 | 0.0423 | −0.1095 |
| (3.750) | (2.943) | (−2.017) |
| Indirect effect ln(ICT/L) | −0.0365 | −0.0405 | 0.2788 |
| (−2.070) | (−1.955) | (4.148) |
| Total effect ln(ICT/L) | 0.0068 | 0.0018 | 0.1693 |
| (0.438) | (0.104) | (3.772) |
| Direct effect ln(R&D) | 0.0064 | 0.0024 | 0.0070 |
| (5.021) | (1.456) | (3.040) |
| Indirect effect ln(R&D) | 0.0064 | 0.0079 | −0.0140 |
| (2.246) | (1.974) | (−3.094) |
| Total effect ln(R&D) | 0.0128 | 0.0103 | −0.0070 |
| (3.812) | (2.178) | (−1.424) |
| Direct effect ln(Tran) | 0.0235 | 0.0026 | 0.0933 |
| (3.407) | (0.321) | (3.457) |
| Indirect effect ln(Tran) | 0.0113 | 0.0134 | 0.4643 |
| (0.726) | (0.767) | (7.542) |
| Total effect ln(Tran) | 0.0348 | 0.0160 | 0.5576 |
| (2.060) | (0.838) | (8.219) |
From the perspective of each explanatory variable coefficient, the coefficients other than W × ln(ICT/L) are significantly positive for 1980–2007. The coefficient of W × ln(Tran) is not significant, while the others are significant. Moreover, the spatial lag of ICT/L is negative, which means that the contribution of ICT to economic growth is mainly derived from its agglomeration effect, rather than the diffusion effect. Quite obviously, ICT has a spillover effect.
Meanwhile, some interesting changes have occurred over the two successive phases. In the protophase, ln(ICT/L) is 0.044 and W × ln(ICT/L) is 0.0070. Both t values are significant. In the anaphase, ln(ICT/L) is –0.114 and W × ln(ICT/L) is 0.28. Both values of t are significant. Therefore, we can conclude that in the protophase, namely, the period before the diffusion of Internet, the effect of ICT investment was mainly felt in the local area while the effect on the perimeter was limited. With the diffusion of Internet and other new ICT technologies, the ICT investment effect is strengthened, and the effect on the perimeter gradually manifests, so that the spillover effect indeed plays a major role.
Now, this raises a question: Is the spatial effect of ICT investment on earth derived from the local area or from the perimeter? To determine the answer, we need to further observe the direct and indirect effects. In the non-spatial model, the ln(NICT/L) estimators of the protophase random effect and the subsequent bi-directional fixed effect are 0.351 and 0.5178, respectively. For the direct effect, the ln(ICT/L) estimators are 0.0857 and 0.081, respectively. However, in the spatial Durbin model, the direct effect estimators are 0.357 and 0.7629 for ln(NICT/L) and 0.0423 and −0.1095 for ln(ICT/L). This means that in the protophase the non-information capital distribution rate in the non-spatial model is underestimated by 1.68%, and the information capital distribution rate is underestimated by 202.6%. In the later stage, the former is underestimated by 30.9%, while the latter is overestimated by 26%. Corresponding to the situation, the labor distribution rates for two periods are 0.5634 and 0.3922 in the non-spatial model and 0.6004 and 0.3466 in the spatial Durbin model; the latter model underestimates the labor distribution rate by 6.16% in the protophase and overestimates it by 13.13% in the later stage. The underestimation or overestimation can be illustrated by the feedback effect, regardless of whether it is direct or indirect.
The difference between the direct effect and the variable coefficient is called feedback effect, the magnitude of which reflects the influential degree of lag effect of the independent variable and induced variable. The feedback effects of ln(ICT/L) during two successive periods are −0.0015 and 0.0048, respectively, which means the ICT investment effect in the protophase is mainly derived from local investment and the influence on the perimeter is almost negligible. However, later ICT investment is influenced distinctively by the perimeter besides being influenced by the local effect.
In the protophase, the direct effect of ln(ICT/L) is 0.0423, and the t value is significant. The indirect effect is −0.0405, but its t value is not significant. In the later stage, the direct and indirect effects are −0.1095 and 0.2788, respectively, and their t values are significant. It can be seen that from 1997 local ICT investment starts to exert significant influence on the economic growth of circumjacent areas. The degree of interaction of the two kinds of effects can be represented by the ratio of the indirect effect to the direct effect. The quotient is 0.48928 in the protophase and 0.71791 in the later stage, which means the indirect effect from the information capital distribution rate is just 48.93% of the direct effect in the protophase, although it rises to 71.79% in the later stage. This implies that a change in the information capital distribution rate, in the protophase, results in a proportionate change in the labor distribution rate of the adjacent and local areas in the ratio of 1:2.04; in the later stage, the reciprocal inter-region influence is almost negligible, the corresponding ratio being 1:1.39, but the reciprocal influence is strengthened significantly. We can conclude that the ln(ICT/L) coefficient changes from 1980–1996 to 1997–2007 because of the interaction effect among the regions. Obviously, the ICT capital input does have a spatial spillover effect.
4.3. Spillover Effect and Network Effect of Informatization
In the foregoing analysis, we explored the contribution of ICT investment to economic growth under the hypothesis of constant returns to scale. The model does not take into account whether or not ICT investment has increasing returns due to the scale effect on the regional economy. However, new ICT investment will strengthen the network connections of other enterprises, producing an external effect that creates an increasing returns-to-scale effect on the regional economy. Moreover, the massive diffusion of the personal computer and the introduction of the Internet since 1995, plus the mobile phone and other network communication equipment, have developed a social network system with the stronger osmosis property, and the relevance and interpenetration of enterprise and other social aspects have been further strengthened. Whether the development of the social network system will strengthen the spillover effect of ICT investment is an important question that needs to be examined as a future direction of this research. In other words, one would naturally like to know what kind of effect social networks and ICT investments will impose on economic growth.
We employ model (4) and its derivative, the spatial Durbin model, to answer the above question. In model (12), the variable ln(ICTit) is used to explore the network externality of ICT investment. In a macroeconomic situation, we expect the variable to be positive, but in the context of a regional economy, the expected variable could be either positive or negative. Combined with the paradoxical geographies of the digital economy, if the variable is positive, it means there is a spillover or spreading effect, and the world is flat theory is verified. If the variable is negative, it means there is no spillover effect, while there could be a convergence effect, and the digital divide is supported. Based on Equation (4), the study has taken advantage of the cross-term of ICT to explore the informatization external effect under the interaction of enterprise ICT investment and the social information network. Thus, Equation (4) can be extended to Equation (19):
In Equation (19), U represents the ubiquitous informatization index. Now, we can extend the model.
The variables in Equations (19) and (20) have the same meaning as in Equations (4) and (12), respectively.
Table 1 shows that informatization investment in Japan increased significantly after 1995 while the Internet and other means of communication diffused on a massive scale around 1997. Meanwhile, the analysis in the above section also shows that 1997 is a demarcation point. Therefore, we employed data for 11 years, from 1997 to 2007, to analyze the external network effect of informatization.
In general, the spatial spillover effect becomes attenuated as the distance increases. In order to test the effect of spatial distance on ICT spatial spillover, we designed first-order (W1), second-order (W2), and third-order (W3) spatial adjacency weights.
Table 2 shows the test results of the Lagrange multiplier and robust Lagrange multiplier approaches. It can be seen that the null hypothesis of the spatial effect on the non-spatial model has not been negated and the spatial lag model is better than the spatial error model. The tests provided significant results for spatial fixed effects, time fixed effects, or bi-directional fixed effects. Besides, LR also shows that the spatial fixed effect (1005.93, degree of freedom = 47,
p < 0.001) and the time fixed effect (176.03, degree of freedom = 11,
p < 0.001) are significant, which means that the bi-directional fixed effects approach is an appropriate choice.
Table 3 provides the estimated results of informatization input spatial spillover from 1997 to 2007. The first row in the table gives the estimated results with first-order weights (
W1). The second row provides the estimated results with second-order weights (
W2). The third row indicates the estimated results with third-order weights (
W3). From the estimated results with first-order weights,
R2 is 0.9744, and the corrected
R2 is 0.4156; therefore, the model fits well. The Wald lag and Wald error test statistics are 128.18 (
p = 0.001) and 118.57 (
p = 0.001), respectively. The LR lag and LR error test statistics are 124.71 (
p = 0.001) and 121.72 (
p = 0.001), respectively. These results mean that the spatial Durbin model with spatial lag is an appropriate choice. The estimated coefficients with first-order weights are significant for all variables except
W ×
ln(K/L), which is negative but not significant(
t = −1.94). Both
ln(ICT) and
W×
ln(ICT) are significant. ICT is negative but not significant, which means local ICT investment will not lead to an external effect or a spatial spillover effect. However, it is too early to draw a conclusion. In the local area,
ln(ICT) is negative, which means that there may be an excessive investment problem. However,
W ×
ln(ICT) is positive, and the coefficient is larger than
ln(ICT), which suggests a significant spillover effect. This can be verified from the total effect (direct effect = 0.1885,
t = 4.466).
At 0.6152, the coefficient value of ln(K/L) is underestimated by 9.0% in the non-spatial bi-directional fixed effect model compared to the spatial Durbin model. This suggests the error of neglecting the spatial effect in the non-spatial model. In the spatial Durbin model of the bi-directional fixed effect, the indirect effect of ln(K/L) is 19.87% of the direct effect. In other words, a change in the capital distribution rate results in a proportionate change in the labor distribution rate of the adjacent and local areas in the ratio of 1:5.03.
Table 2.
Results of the Lagrange multiplier and the robust Lagrange multiplier.
Table 2.
Results of the Lagrange multiplier and the robust Lagrange multiplier.
| OLS Estimation | Spatial Fixed Effects | Time-Period Fixed Effects | Spatial and Time-Period Fixed Effects |
|---|
| ln(KL) | 0.375 | 0.208 | 0.307 | 0.615 |
| (19.839) | (6.623) | (15.14) | (11.48) |
| ln(ICT) | 0.071 | −0.009 | 0.071 | 0.033 |
| (14.09) | (−0.299) | (15.43) | (1.018) |
| ln(R&D) | 0.004 | 0.008 | 0.007 | 0.008 |
| (2.926) | (2.959) | (5.148) | (3.490) |
| ln(Tran) | −0.007 | 0.178 | -0.012 | 0.107 |
| (−1.914) | (10.67) | (-3.449) | (3.891) |
| Intercept | −1.339 | | | |
| (−38.66) | | | |
| R2 | 0.724 | 0.806 | 0.716 | 0.251 |
| Durbin-Watson | 1.871 | 1.497 | 2.029 | 1.730 |
| Log L | 1009.80 | 1473.10 | 1058.20 | 1561.10 |
| LM spatial lag | 107.10 | 27.25 | 46.39 | 2.34 |
| LM spatial error | 27.17 | 31.72 | 5.45 | 3.63 |
| Robust LM spatial lag | 84.55 | 0.00 | 51.88 | 0.38 |
| Robust LM spatial error | 4.62 | 4.48 | 10.95 | 1.68 |
Table 3.
The spatial spillover effect of informatization.
Table 3.
The spatial spillover effect of informatization.
| Spatial and Time-Period Fixed Effects W1 | Spatial and Time-Period Fixed Effects W2 | Spatial and Time-Period Fixed Effects W3 |
|---|
| W × ln(GDP/L) | 0.0219 | 0.2427 | −0.0585 |
| (0.4002) | (3.4463) | (−0.5255) |
| ln(K/L) | 0.6770 | 0.6468 | 0.6720 |
| (13.4205) | (12.2567) | (12.8019) |
| ln(ICT) | −0.3270 | −0.2757 | −0.2097 |
| (−5.3955) | (−5.2624) | (−4.8450) |
| ln(R&D) | 0.0073 | 0.0073 | 0.0047 |
| (3.2597) | (3.1083) | (2.0160) |
| ln(Tran) | 0.0912 | 0.0982 | 0.1049 |
| (3.4579) | (3.5318) | (3.8223) |
| W × ln(K/L) | −0.1782 | −0.1819 | −0.2231 |
| (−1.9370) | (−1.2550) | (−1.1099) |
| W × ln(ICT) | 0.5115 | 0.5248 | 0.6550 |
| (6.9291) | (6.3031) | (6.7266) |
| W × ln(R&D) | −0.0129 | −0.0147 | −0.0290 |
| (−3.0264) | (−1.9917) | (−2.5442) |
| W × ln(Tran) | 0.4593 | 0.2474 | 0.6359 |
| (7.8760) | (2.4894) | (3.9463) |
| σ2 | 0.0001 | 0.0001 | 0.0001 |
| R2 | 0.9744 | 0.9719 | 0.9725 |
| Corrected R2 | 0.4156 | 0.3521 | 0.3707 |
| Log L | 1624.8 | 1598.6 | 1607.0 |
| Wald test spatial lag | 128.18(p=0.000) | 66.413(p=0.001) | 87.41(p=0.000) |
| Wald test spatial error | 118.57(p=0.000) | 49.21(p=0.001) | 73.32(p=0.000) |
| LR test spatial lag | 124.71(p=0.000) | 69.25(p=0.001) | 91.03(p=0.000) |
| LR test spatial error | 121.72(p=0.000) | 59.87(p=0.001) | 90.21(p=0.000) |
| Direct effect ln(K/L) | 0.6761 | 0.6456 | 0.6717 |
| (14.099) | (11.971) | (12.760) |
| Indirect effect ln(K/L) | −0.1676 | −0.0363 | −0.2569 |
| (−1.847) | (−0.207) | (−1.367) |
| Total effect ln(K/L) | 0.5085 | 0.6093 | 0.4147 |
| (4.915) | (3.135) | (2.162) |
| Direct effect ln(ICT) | −0.3230 | −0.2606 | −0.2148 |
| (−5.399) | (−5.177) | (−4.837) |
| Indirect effect ln(ICT) | 0.5115 | 0.5921 | 0.6375 |
| (6.988) | (6.271) | (6.512) |
| Total effect ln(ICT) | 0.1885 | 0.3315 | 0.4228 |
| (4.466) | (4.523) | (5.450) |
| Direct effect ln(R&D) | 0.0072 | 0.0069 | 0.0048 |
| (3.295) | (2.919) | (2.003) |
| Indirect effect ln(R&D) | −0.0128 | −0.0167 | −0.0282 |
| (−3.058) | (−1.721) | (−2.520) |
| Total effect ln(R&D) | −0.0055 | −0.0098 | −0.0234 |
| (−1.200) | (−0.964) | (−1.987) |
| Direct effect ln(Tran) | 0.0943 | 0.1076 | 0.1033 |
| (3.672) | (3.963) | (3.856) |
| Indirect effect ln(Tran) | 0.4674 | 0.3512 | 0.6036 |
| (7.622) | (2.741) | (3.708) |
| Total effect ln(Tran) | 0.5618 | 0.4588 | 0.7069 |
| (8.439) | (3.303) | (4.224) |
In terms of information investment in the non-spatial model, the coefficient of ln(ICT) is 0.033 (t = 0.96), which is an overestimation. In the spatial model, the direct effect is −0.323 (t = −5.399), and the indirect effect is 0.5115 (t = 6.988). These results indicate that ICT affects economic growth through spatial spillover. We arrived at similar conclusionsanalyzing the effect of R&D capital stock (R&D) and transportation infrastructure capital stock (Tran). R&D capital stock and transportation infrastructure capital stock affect economic growth through spatial spillover.
From the range of spatial spillover, the indirect effects of ICT from the three adjacency matrix conditions of W1, W2, and W3 do not decrease with an increase in the spatial scope. However, they tend to increase. We can conclude that the spatial spillover of ICT is not confined to the adjacent area. It is characterized by cross-regional influence on the economic development.
In terms of the above ICT investment analysis, what would happen if informatization gradually permeates the whole society?
Table 4 shows the estimated result of the bi-directional fixed effect with the spatial Durbin model (20) involving the cross-term of ICT and the ubiquitous index, which is the social informatization development standard. In the cross-term,
R2 and the corrected
R2 are 0.9725 and 0.372, respectively, no different from
Table 3. These passed the Wald test and the LR test, which means the spatial Durbin model is an appropriate choice.
In contrast to
Table 3, all variables have the same empirical result as in
Table 4, except
ln(ICT ×
U), which means that the model is stable. Moreover, the estimated coefficient of
ln(ICT ×
U) changes from negative to positive, with the spatial lag being positive. This means that under the influence of social informatization, the external effect and spillover effect of ICT investment are strengthened.
From the analysis of the two above-mentioned models, we can conclude as follows. First, for the 1997–2007 period, the effect of the introduction and diffusion of informatization on Japanese regional economic growth, as well as the effect of the increasing returns to scale, is significant. Second, informatization investment does not necessarily lead to network external effects and the regional spatial spillover effect, and may show spatial convergence for local investment. Third, the extensive introduction and diffusion of informatization has enhanced the effect of informatization investment and strengthened network externality and the spatial spillover effect of investment, spearheading regional economic development as a whole. This implies the effect of increasing returns to scale.
Table 4.
The spatial spillover effect of interaction.
Table 4.
The spatial spillover effect of interaction.
| Spatial and Time-Period Fixed Effects W1 | Spatial and Time-Period Fixed Effects W2 | Spatial and Time-Period Fixed Effects W3 |
|---|
| W × ln(GDP/L) | 0.0303 | 0.1954 | −0.0638 |
| (0.5456) | (2.6482) | (−0.5579) |
| ln(K/L) | 0.7152 | 0.7323 | 0.7311 |
| (13.4275) | (13.0346) | (13.0560) |
| ln(ICT × U) | 0.0372 | 0.0244 | 0.0388 |
| (1.9332) | (1.2315) | (2.1657) |
| ln(R&D) | 0.0078 | 0.0094 | 0.0070 |
| (3.3782) | (3.9636) | (2.8941) |
| ln(Tran) | 0.1056 | 0.1169 | 0.1300 |
| (3.8423) | (4.0572) | (4.5437) |
| W × ln(K/L) | −0.0706 | 0.0627 | 0.0063 |
| (−0.6756) | (0.3695) | (0.0261) |
| W × ln(ICT × U) | 0.0379 | 0.0938 | 0.0972 |
| (1.3909) | (2.3452) | (1.8055) |
| W × ln(R&D) | −0.0138 | −0.0206 | −0.0421 |
| (−3.1066) | (−2.7568) | (−3.6214) |
| W × ln(Tran) | 0.4445 | 0.2310 | 0.2976 |
| (7.4031) | (2.2478) | (1.8457) |
| σ2 | 0.0001 | 0.0001 | 0.0001 |
| R2 | 0.9725 | 0.9702 | 0.9705 |
| Corrected R2 | 0.3720 | 0.3153 | 0.3237 |
| Log L | 1606.5 | 1584.7 | 1588.5 |
| Wald test spatial lag | 61.47 (p = 0.001) | 16.99 (p = 0.001) | 26.44 (p = 0.001) |
| Wald test spatial error | 62.24 (p = 0.001) | 16.14 (p = 0.002) | 25.85 (p = 0.001) |
| LR test spatial lag | 64.84 (p = 0.001) | 18.89 (p = 0.001) | 29.64 (p = 0.001) |
| LR test spatial error | 65.93 (p = 0.001) | 18.58 (p = 0.001) | 30.77 (p = 0.001) |
| Direct effect ln(K/L) | 0.7173 | 0.7371 | 0.7284 |
| (13.046) | (12.938) | (13.570) |
| Indirect effect ln(K/L) | −0.0500 | 0.2452 | −0.0457 |
| (−0.504) | (1.265) | (−0.209) |
| Total effect ln(K/L) | 0.6673 | 0.9824 | 0.6827 |
| (5.792) | (4.535) | (2.974) |
| Direct effect ln(ICT × U) | 0.0365 | 0.0284 | 0.0388 |
| (1.892) | (1.447) | (2.106) |
| Indirect effect ln(ICT × U) | 0.0407 | 0.1175 | 0.0873 |
| (1.494) | (2.626) | (1.713) |
| Total effect ln(ICT × U) | 0.0772 | 0.1458 | 0.1261 |
| (3.338) | (3.568) | (2.746) |
| Direct effect ln(R&D) | 0.0078 | 0.0088 | 0.0071 |
| (3.469) | (3.724) | (3.001) |
| Indirect effect ln(R&D) | −0.0138 | −0.0227 | −0.0403 |
| (−3.006) | (−2.412) | (−3.424) |
| Total effect ln(R&D) | −0.0060 | −0.0139 | −0.0331 |
| (−1.214) | (−1.386) | (−2.678) |
| Direct effect ln(Tran) | 0.1085 | 0.1239 | 0.1276 |
| (4.065) | (4.207) | (4.406) |
| Indirect effect ln(Tran) | 0.4611 | 0.3062 | 0.2732 |
| (7.283) | (2.365) | (1.803) |
| Total effect ln(Tran) | 0.5696 | 0.4301 | 0.4008 |
| (8.326) | (3.052) | (2.508) |










 measures, for region i, the influential effect of the independent variable xir on the dependent variable, which represents the direct effect. For region j,
measures, for region i, the influential effect of the independent variable xir on the dependent variable, which represents the direct effect. For region j,  , i ≠ j, measures the influential effect of the independent variable xjr on the dependent variable, which represents the indirect effect. The diagonal elements in Sr(W) represent the direct effects and the off-diagonal elements the indirect effects.
, i ≠ j, measures the influential effect of the independent variable xjr on the dependent variable, which represents the indirect effect. The diagonal elements in Sr(W) represent the direct effects and the off-diagonal elements the indirect effects.