Optimal Elasto-Plastic Analysis of Prestressed Concrete Beams by Applying Residual Plastic Deformation Limitations
Abstract
1. Introduction
2. The Theory of Limited Residual Plastic Deformation Inside Steel Elements
- = actual stresses.
- and = actual strains and displacements.
- = fictitious stresses that would occur if the material were purely elastic.
- and fictitious elastic strains and displacements corresponding to .
- = actual residual stress distribution.
- = any arbitrary, time-independent self-stress distribution.
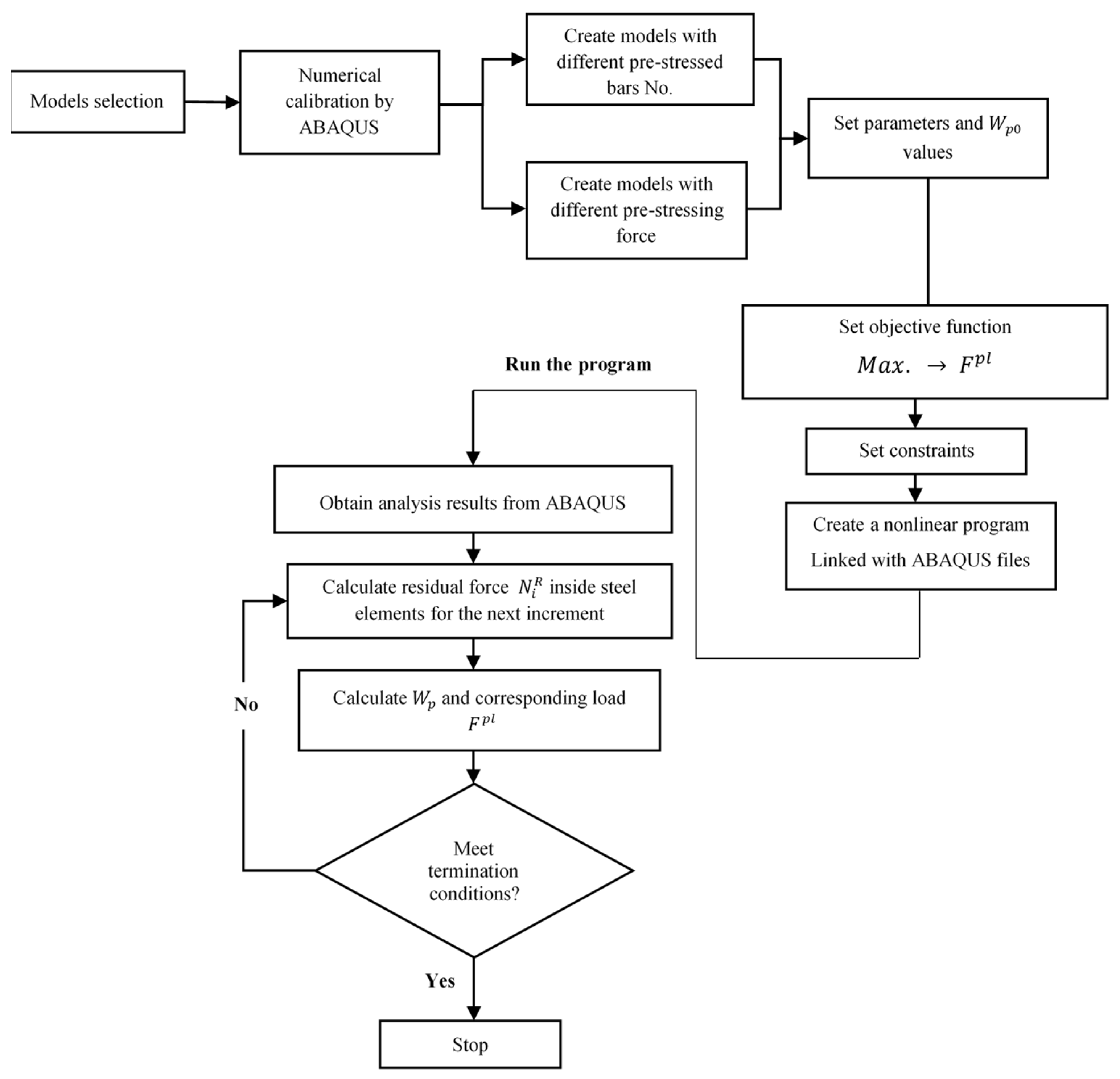
3. Optimization Problem
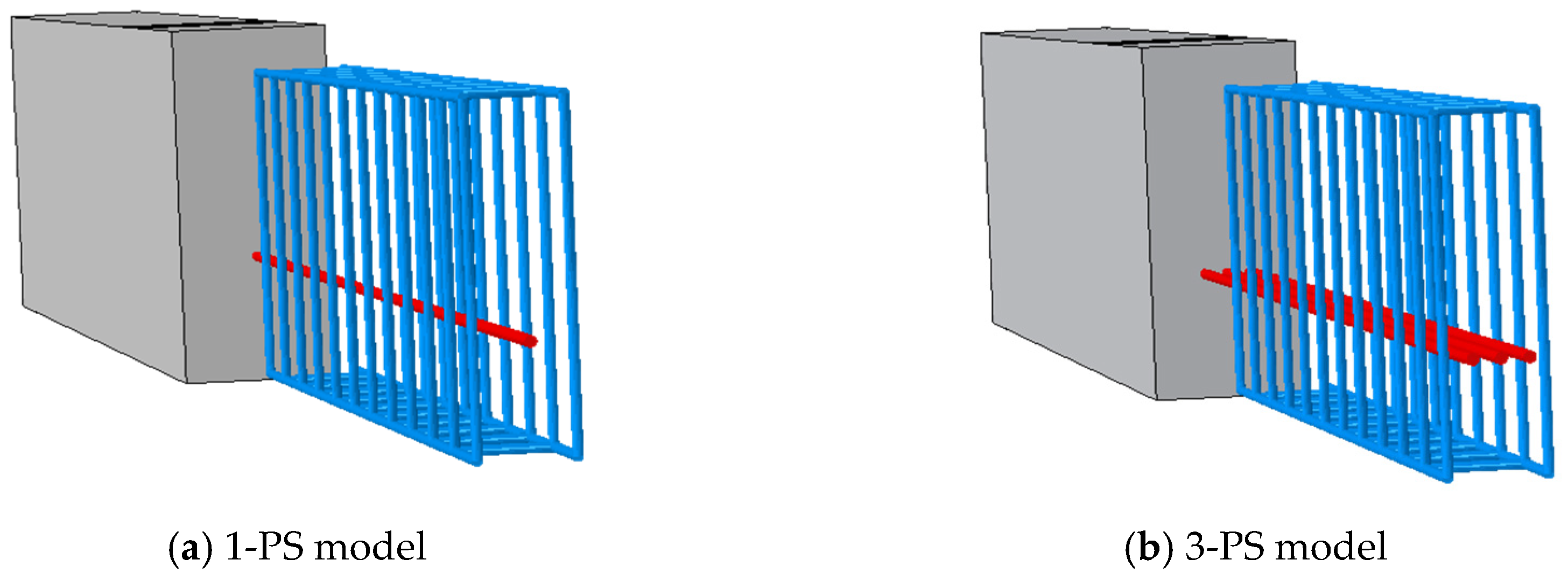
4. Models with Different Prestressing Tendon Numbers
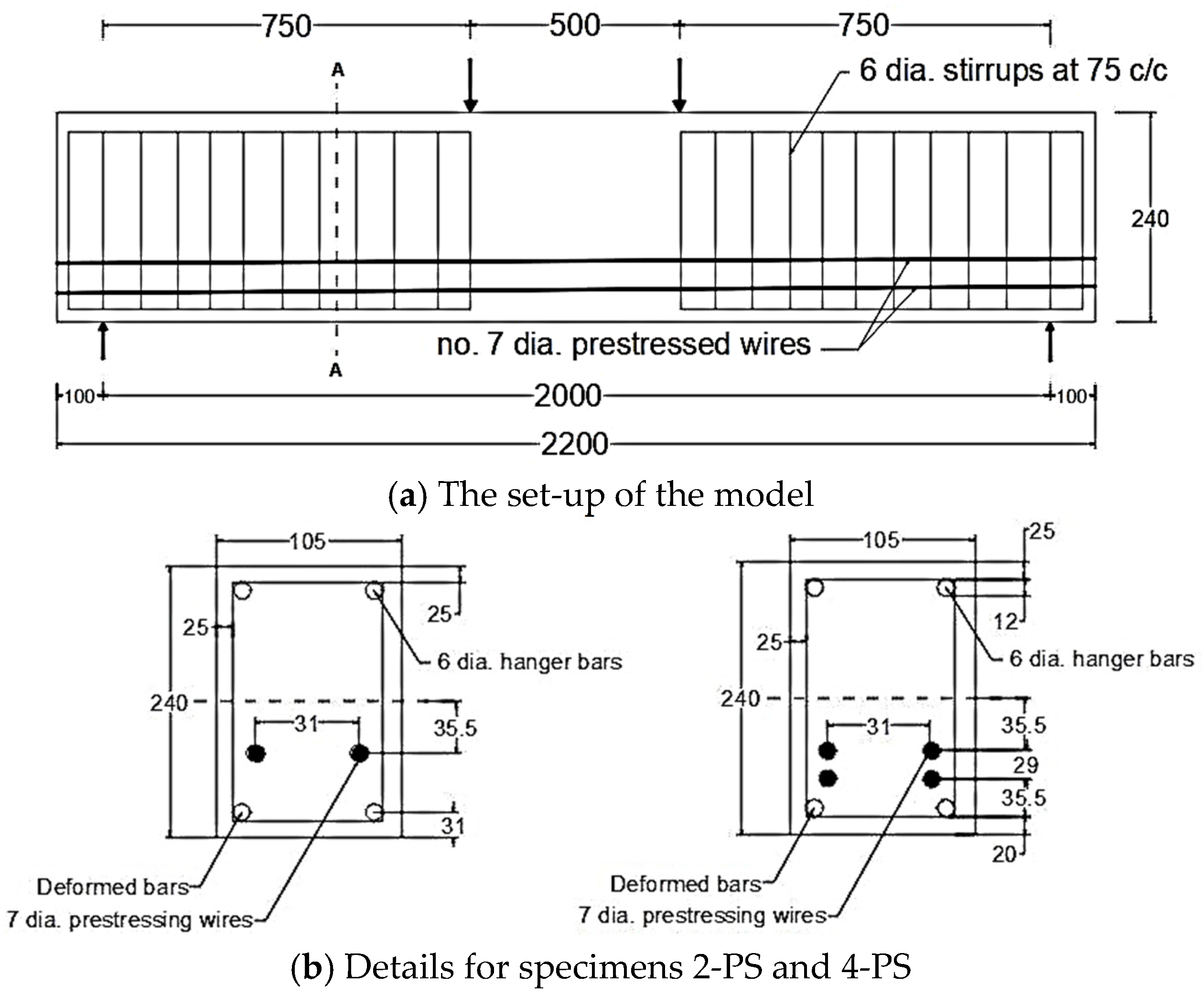
4.1. Numerical Modelling of the Benchmarks
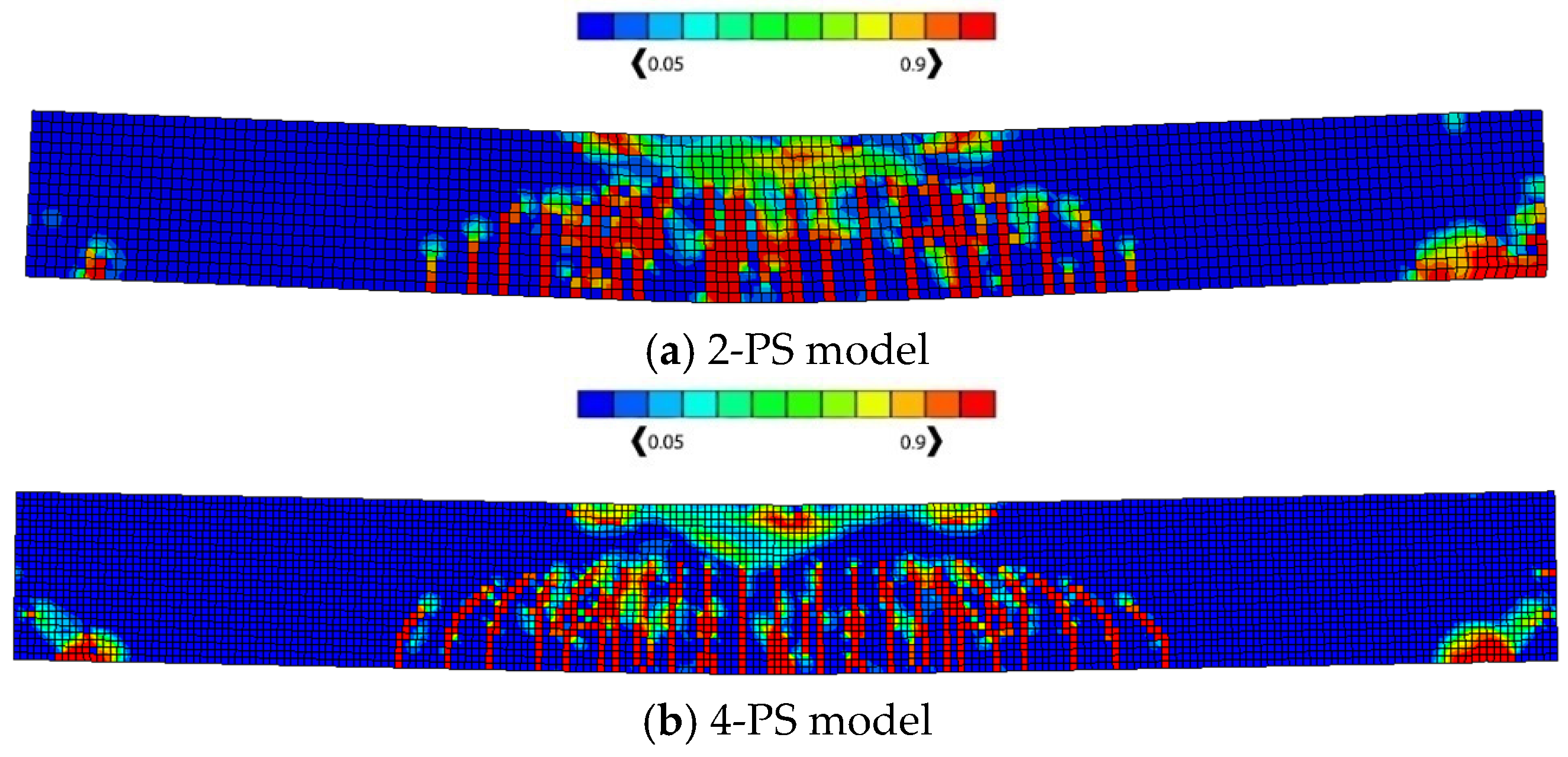
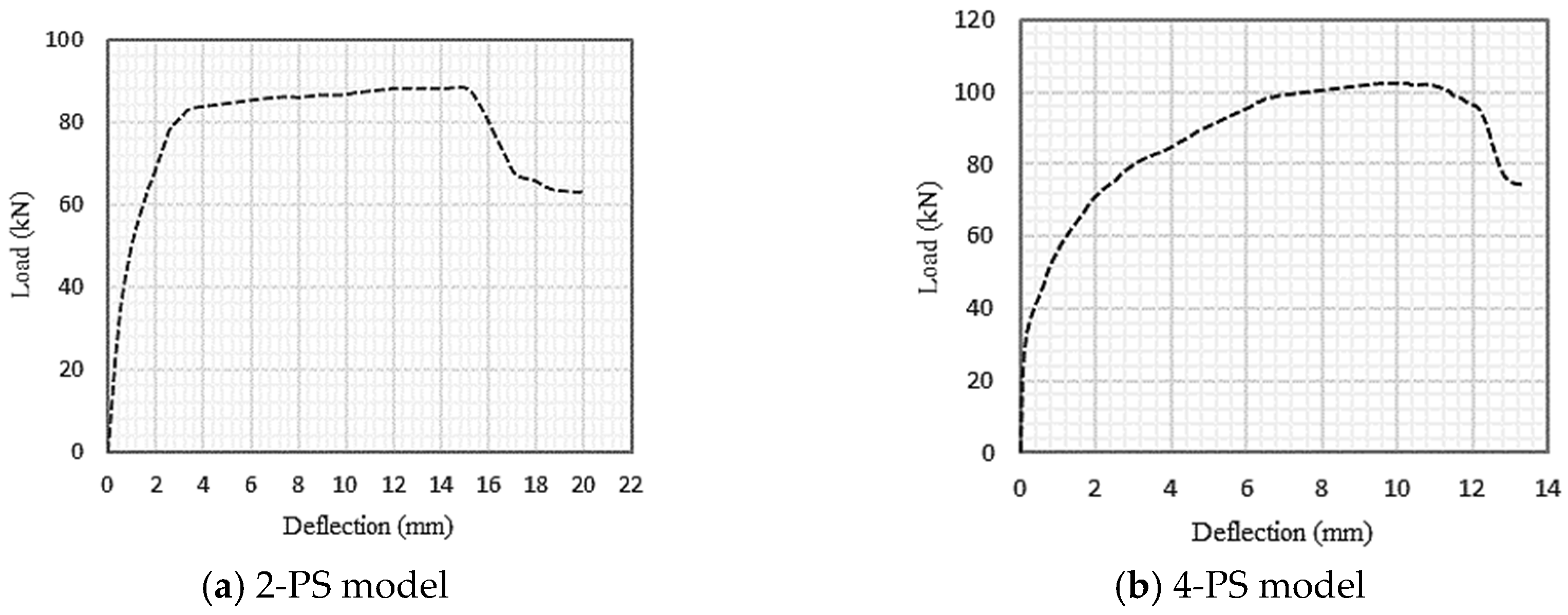
4.2. Results and Discussion of the Application of the Optimization Problem
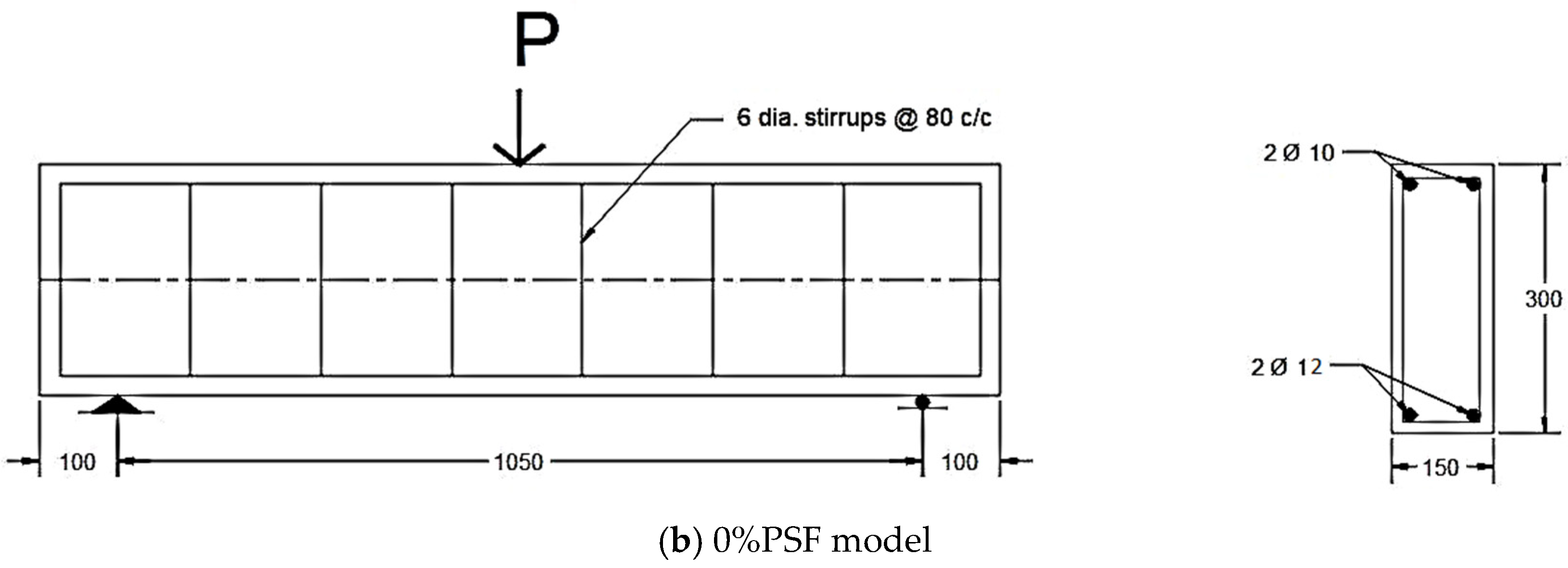
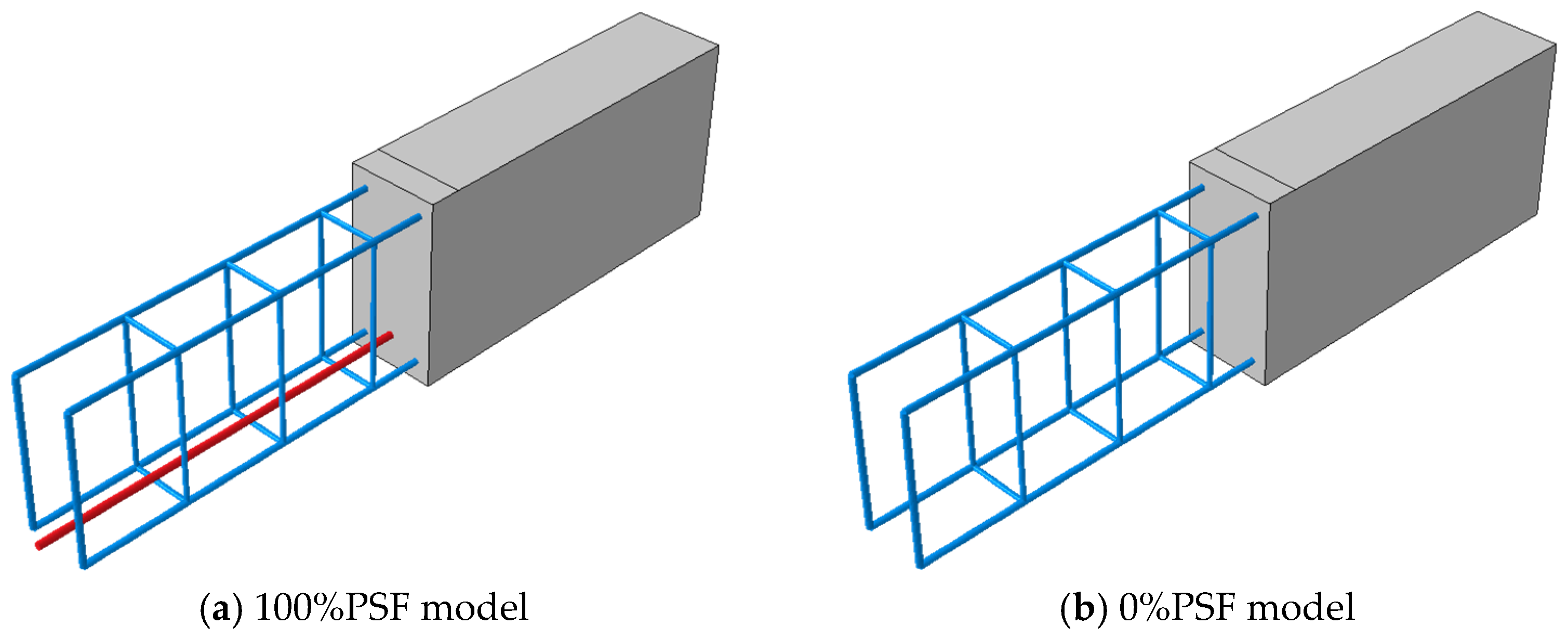
5. Models with Different Prestressing Forces
5.1. Numerical Modelling of the Benchmarks
5.2. Results and Discussion of the Application of the Optimization Problem
6. Conclusions
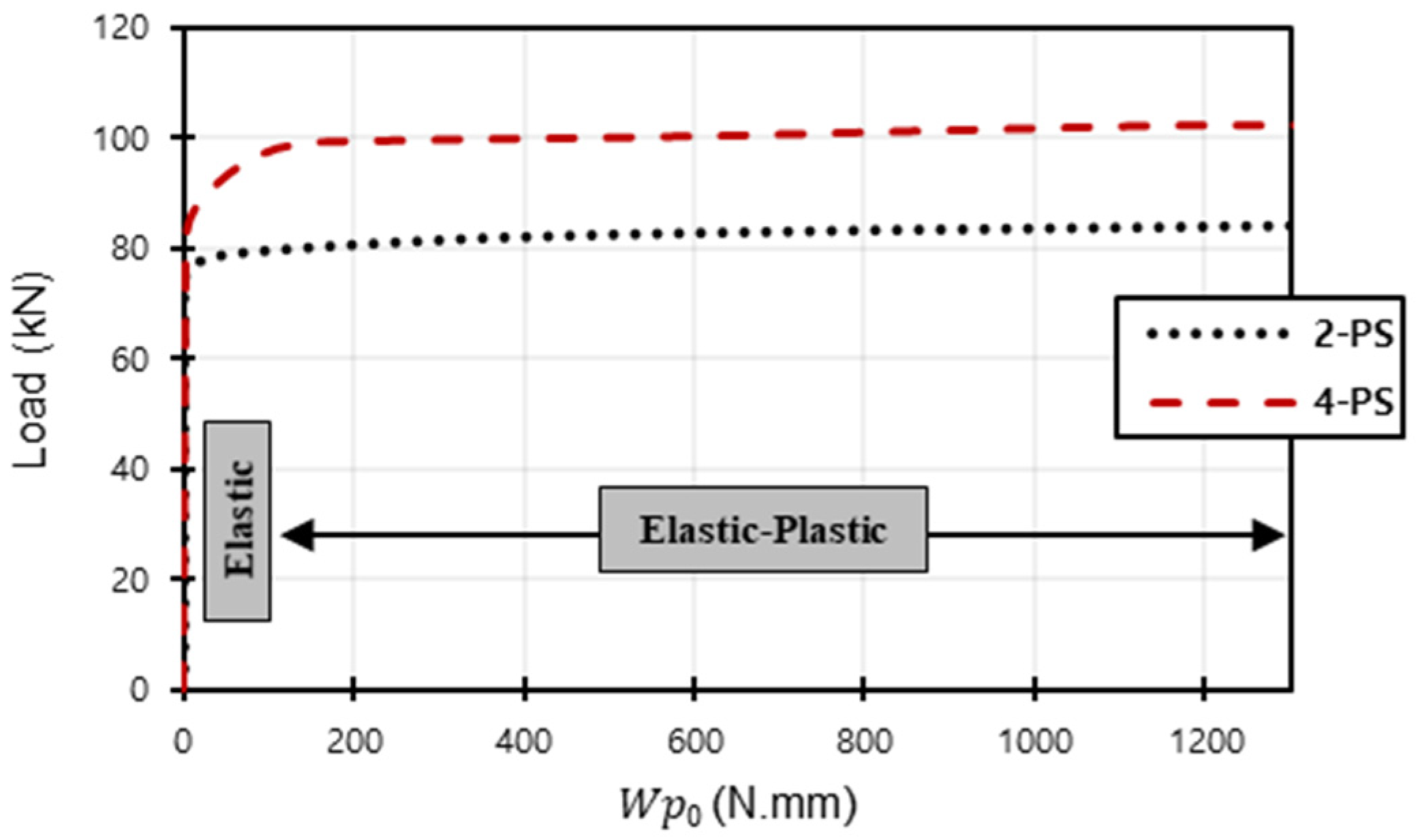
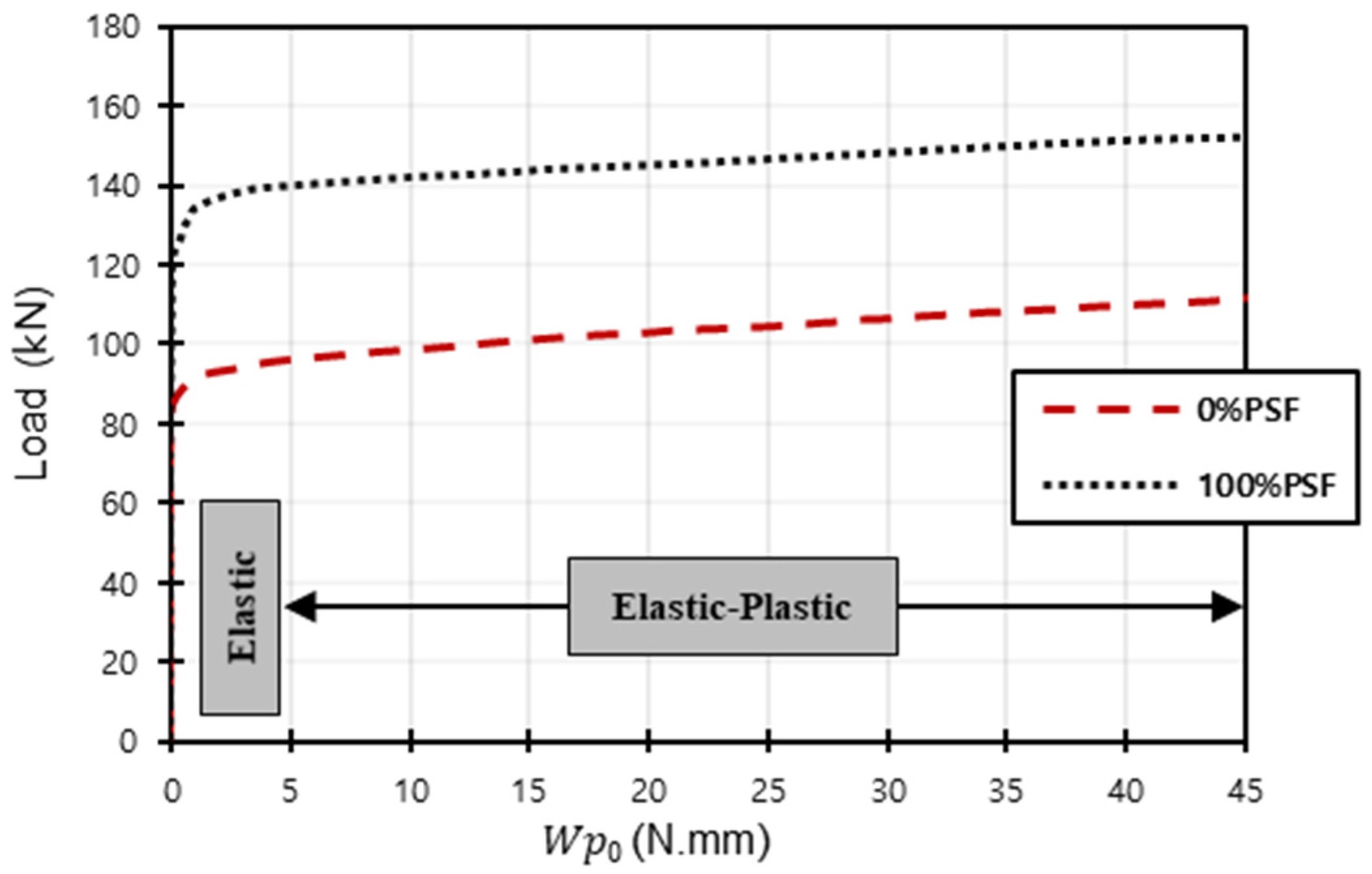
- In most cases, an increase in the value of the allowable amount of energy will increase the corresponding loading values produced.
- The load– curve in the 2-PS model begins to take the direction of the horizontal line with lower loads than its counterpart in the 4-PS model, which can be explained by the fact that the 4-PS model contains more tendons, so the damages caused by internal stresses generated inside them are delayed in formation and accumulate more slowly compared to the other model.
- With a given value of allowed complementary strain energy, the corresponding load in the case of 2-PS will be smaller than in the case of 4-PS, implying that the yielded steel elements accumulate more in the first model, resulting in faster failure under lower loads.
- When the permissible complementary strain energy is almost zero, a reflection of general elastic behaviour is produced within the limits of the initial loads; however, as this value increases, the load– curves begin to take a path that leads to plastic behaviour, passing through the elasto-plastic region.
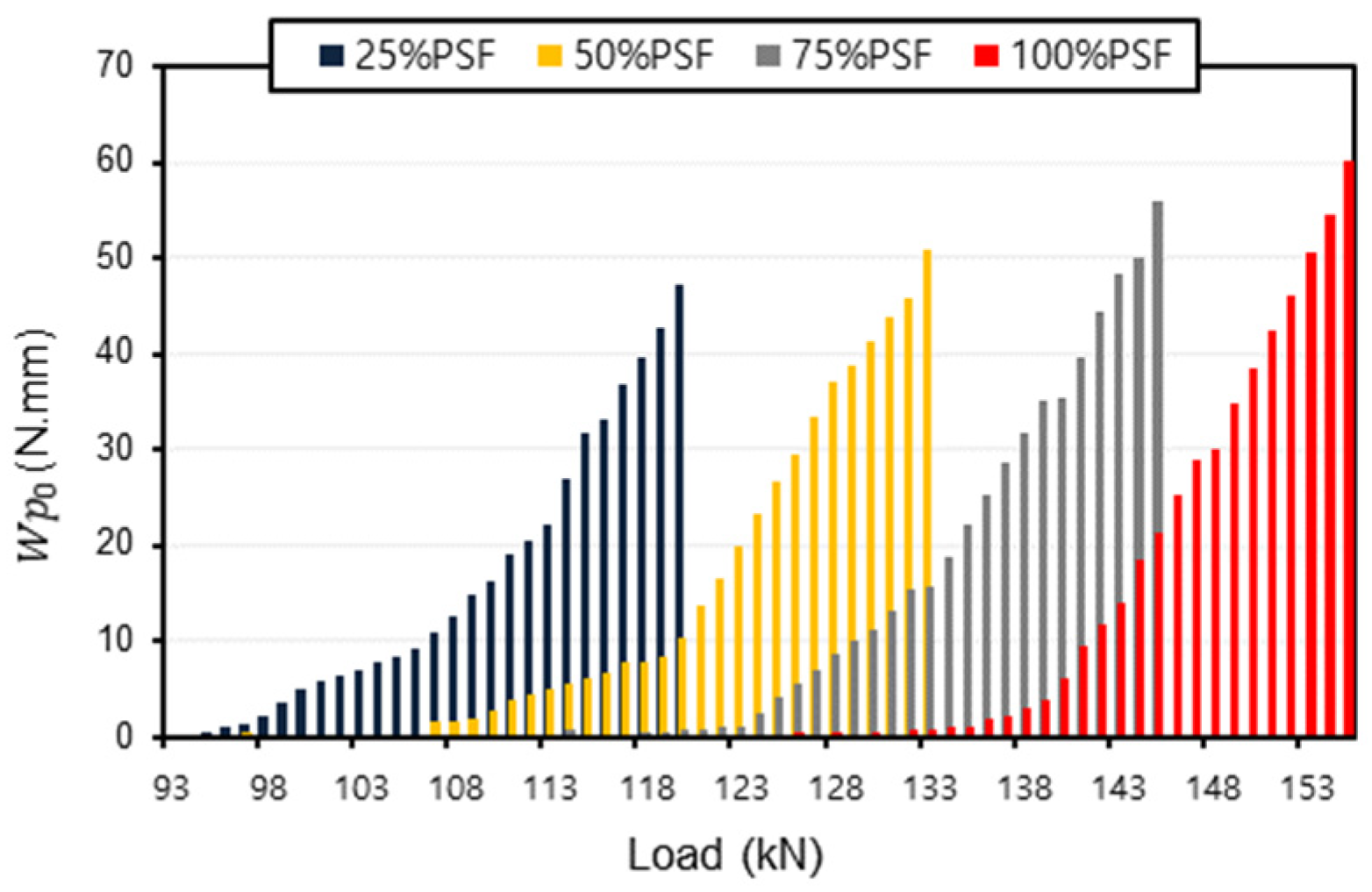
- When the provided allowable complementary strain energy value grows, so does the damage caused by high-stress intensity in steel and tension-damaged areas in concrete.
- Increasing the number of prestressed tendons results in higher load values for lower permissible complementary strain energy. This occurs as a result of the strengthening added by increasing the number of prestressed tendons, where increasing the number of these tendons would increase the required load to cause the yield inside the steel elements.
- The allowable complementary strain energy value produces an increase in the associated load values, indicating that a higher plasticity state has been achieved.
- When compared to a specific allowable complementary strain energy value, the 0%PSF model provides lower load values than the 100%PSF model. This may suggest that the steel used in the 0%PSF model experiences higher stresses under lower loads, which may reflect the general damage behaviour of the beams.
- The use of prestressed tendons in a 100%PSF model strengthened the beam and postponed the initiation of stresses within the steel elements, leading to higher load values and a smaller allowable complementary strain energy value. This is because the process of having yielded steel elements necessitates higher loads.
- Increasing the prestressing force inside the tendon strengthens the models, leading to an increase in the assumed allowable complementary strain energy value, which in turn leads to an increase in the load necessary to cause yield within the steel elements and a corresponding decrease in the corresponding allowable complementary strain energy value, which depends on the number of yielded elements.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, Y.; Fan, Y. Experimental study on flexural behavior of prestressed concrete beams reinforced by CFRP under chloride environment. Adv. Civ. Eng. 2019, 2424518. [Google Scholar] [CrossRef]
- Padmarajaiah, S.K.; Ramaswamy, A. A finite element assessment of flexural strength of prestressed concrete beams with fiber reinforcement. Cem. Concr. Compos. 2002, 24, 229–241. [Google Scholar] [CrossRef]
- Kim, Y.J.; Shi, C.; Green, M.F. Ductility and cracking behavior of prestressed concrete beams strengthened with prestressed CFRP sheets. J. Compos. Constr. 2008, 12, 274–283. [Google Scholar] [CrossRef]
- Badawy, A.H.; Hassan, A.; El-Kady, H.; El-Hafaz, A. The behavior of reinforced and pre-stressed concrete beams under elevated temperature. Int. J. Eng. Res. Afr. 2020, 47, 15–30. [Google Scholar] [CrossRef]
- Bonopera, M.; Chang, K.C.; Chen, C.C.; Sung, Y.C.; Tullini, N. Experimental study on the fundamental frequency of prestressed concrete bridge beams with parabolic unbonded tendons. J. Sound Vib. 2019, 455, 150–160. [Google Scholar] [CrossRef]
- Noble, D.; Nogal, M.; Pakrashi, V. The effect of prestress force magnitude and eccentricity on the natural bending frequencies of uncracked prestressed concrete beams. J. Sound Vib. 2016, 365, 22–44. [Google Scholar] [CrossRef]
- Cohn, M.Z.; Dinovitzer, A.S. Application of structural optimization. J. Struct. Eng. 1994, 120, 617–650. [Google Scholar] [CrossRef]
- Lepš, M.; Šejnoha, M. New approach to optimization of reinforced concrete beams. Comput. Struct. 1994, 81, 1957–1966. [Google Scholar] [CrossRef]
- Coello, C.C.; Hernández, F.S.; Farrera, F.A. Optimal design of reinforced concrete beams using genetic algorithms. Expert Syst. Appl. 1997, 12, 101–108. [Google Scholar] [CrossRef]
- Rahmanian, I.; Lucet, Y.; Tesfamariam, S. Optimal design of reinforced concrete beams: A review. Comput. Concr. 2014, 13, 457–482. [Google Scholar] [CrossRef]
- Chutani, S.; Singh, J. Design optimization of reinforced concrete beams. J. Inst. Eng. India Ser. A 2017, 98, 429–435. [Google Scholar] [CrossRef]
- Gokul, P.; Sabarigirivasan, L. Finite element analysis of RC beams with and without openings. Mater. Today Proc. 2022, 68, 2541–2550. [Google Scholar] [CrossRef]
- Lezgy-Nazargah, M.; Dezhangah, M.; Sepehrinia, M. The effects of different FRP/concrete bond-slip laws on the 3D nonlinear FE modeling of retrofitted RC beams—A sensitivity analysis. Steel Compos. Struct. 2018, 26, 347–360. [Google Scholar] [CrossRef]
- Yeganeh-Salman, A.; Lezgy-Nazargah, M. Evaluating the accuracy of mass scaling method in non-linear quasi-static finite element analysis of RC structures. Struct. Eng. Mech. 2023, 8, 485–500. [Google Scholar] [CrossRef]
- Bendsee, M.P.; Sokolowski, J. Design sensitivity analysis of elastic-plastic analysis problems. Mech. Struct. Mach. 1988, 16, 81–102. [Google Scholar] [CrossRef]
- Newman, J.C., Jr.; Armen, H., Jr. Elastic-plastic analysis of a propagating crack under cyclic loading. AIAA J. 1975, 13, 1017–1023. [Google Scholar] [CrossRef]
- Tin-Loi, F. Optimum shakedown design under residual displacement constraints. Struct. Multidiscip. Optim. 2000, 19, 130–139. [Google Scholar] [CrossRef]
- Atkočiūnas, J.; Merkevičiūtė, D.; Venskus, A. Optimal shakedown design of bar systems: Strength, stiffness and stability constraints. Comput. Struct. 2008, 86, 757–1768. [Google Scholar] [CrossRef]
- Weichert, D.; Maier, G. Inelastic Behaviour of Structures Under Variable Repeated Loads: Direct Analysis Methods; Springer: Vienna, Austria, 2002. [Google Scholar] [CrossRef]
- Levy, N.H.; Einav, I.; Hull, T. Cyclic shakedown of piles subjected to two-dimensional lateral loading. Int. J. Numer. Anal. Methods Geomech. 2009, 33, 1339–1361. [Google Scholar] [CrossRef]
- Kaliszky, S.; Lógó, J. Optimal plastic limit and shake-down design of bar structures with constraints on plastic deformation. Eng. Struct. 1997, 19, 19–27. [Google Scholar] [CrossRef]
- Kaliszky, S.; Lógó, J. Optimal strengthening of elasto-plastic trusses with plastic deformation and stability constraints. Struct. Optim. 1999, 18, 296–299. [Google Scholar] [CrossRef]
- De La Fuente, A.; De Brito, J. Sustainable structural design: A review focused on the optimization of reinforced concrete structures. J. Clean. Prod. 2019, 211, 528–544. [Google Scholar] [CrossRef]
- Ibrahim, A.M.; Al-Khafaji, Z.A.; Al-Qadi, I.L. Optimal design of reinforced concrete structures for sustainable development. Sustain. Cities Soc. 2018, 42, 341–352. [Google Scholar] [CrossRef]
- Rad, M.M.; Ibrahim, S.K.; Logo, J. Limit design of reinforced concrete haunched beams by the control of the residual plastic deformation. Structures 2022, 39, 987–996. [Google Scholar] [CrossRef]
- Simulia, D.S. ABAQUS 2018 User’s Manual. Analysis User’s Guide, Volume IV: Elements; Dassault Systems: Paris, France, 2018. [Google Scholar]
- Rad, M.M.; Lógó, J.; Knabel, J.; Tauzowski, P. Reliability based limit design of steel frames with limited residual strain energy capacity. Proc. Appl. Math. Mech. 2009, 9, 709–710. [Google Scholar] [CrossRef]
- Lógó, J.; Vásárhelyi, A.; Rad, M.M.; Stocki, R. Reliability based limit analysis of steel frames with limited residual strain energy capacity. Proc. Appl. Math. Mech. 2008, 8, 10041–10044. [Google Scholar] [CrossRef]
- Rad, M.M.; Ibrahim, S.K. Optimal plastic analysis and design of pile foundations under reliable conditions. Period. Polytech. Civ. Eng. 2021, 65, 761–767. [Google Scholar] [CrossRef]
- Kaliszky, S.; Lógó, J. Plastic behaviour and stability constraints in the shakedown analysis and optimal design of trusses. Struct. Multidiscip. Optim. 2002, 24, 118–124. [Google Scholar] [CrossRef]
- Lógó, J.; Rad, M.M.; Knabel, J.; Tauzowski, P. Reliability based design of frames with limited residual strain energy capacity. Period. Polytech. Civ. Eng. 2011, 55, 13–20. [Google Scholar] [CrossRef]
- Capurso, M.; Corradi, L.; Maier, G. Bounds on deformations and displacements in shakedown theory. Materiaux et Structures sous Chargement Cyclique. In Proceedings of the Séminaire Organisé par le Laboratoire de Mécanique des Solides, Palaiseau, France, 28–29 September 1978; Association Amicale des Ingénieursanciens Eléves de l’E.N.P.C.: Paris, France, 1978; pp. 231–244. [Google Scholar]
- Capurso, M. A displacement bounding principle in shakedown of structures subjected to cyclic loads. Int. J. Solids Struct. 1974, 10, 77–92. [Google Scholar] [CrossRef]
- Gervytė, A.; Jarmolajeva, E. Analysis and optimization of elastic-plastic framing structures under complex constraints. Eng. Struct. Technol. 2013, 5, 159–166. [Google Scholar] [CrossRef]





| P (kN) | ||
|---|---|---|
 | ||
| 0 | 70 |  |
| 31 | 78 | 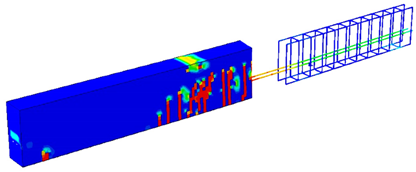 |
| 770 | 83 |  |
| 2788 | 87 |  |
| P (kN) | ||
|---|---|---|
 | ||
| 0 | 80 | 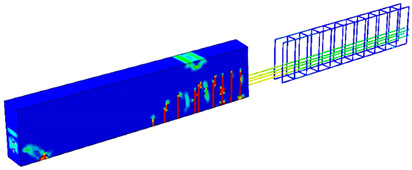 |
| 70 | 95 | 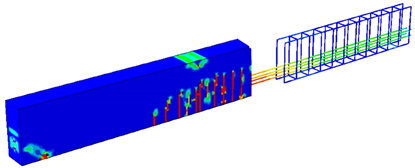 |
| 298 | 98 |  |
| 1300 | 100 |  |
| P (kN) | ||
|---|---|---|
 | ||
| 0 | 70 | 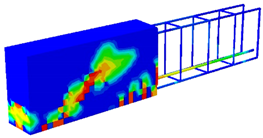 |
| 2 | 125 |  |
| 60 | 155 |  |
| P (kN) | ||
|---|---|---|
 | ||
| 0 | 80 | 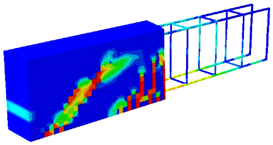 |
| 2.5 | 92 |  |
| 40 | 114 | 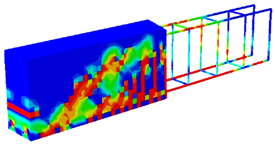 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khaleel Ibrahim, S.; Movahedi Rad, M. Optimal Elasto-Plastic Analysis of Prestressed Concrete Beams by Applying Residual Plastic Deformation Limitations. Sustainability 2023, 15, 5742. https://doi.org/10.3390/su15075742
Khaleel Ibrahim S, Movahedi Rad M. Optimal Elasto-Plastic Analysis of Prestressed Concrete Beams by Applying Residual Plastic Deformation Limitations. Sustainability. 2023; 15(7):5742. https://doi.org/10.3390/su15075742
Chicago/Turabian StyleKhaleel Ibrahim, Sarah, and Majid Movahedi Rad. 2023. "Optimal Elasto-Plastic Analysis of Prestressed Concrete Beams by Applying Residual Plastic Deformation Limitations" Sustainability 15, no. 7: 5742. https://doi.org/10.3390/su15075742
APA StyleKhaleel Ibrahim, S., & Movahedi Rad, M. (2023). Optimal Elasto-Plastic Analysis of Prestressed Concrete Beams by Applying Residual Plastic Deformation Limitations. Sustainability, 15(7), 5742. https://doi.org/10.3390/su15075742







