Innovative Design Method of Jet Grouting Systems for Sustainable Ground Improvements
Abstract
1. Introduction
2. MPS-CAE Method
3. Jet Grouting System
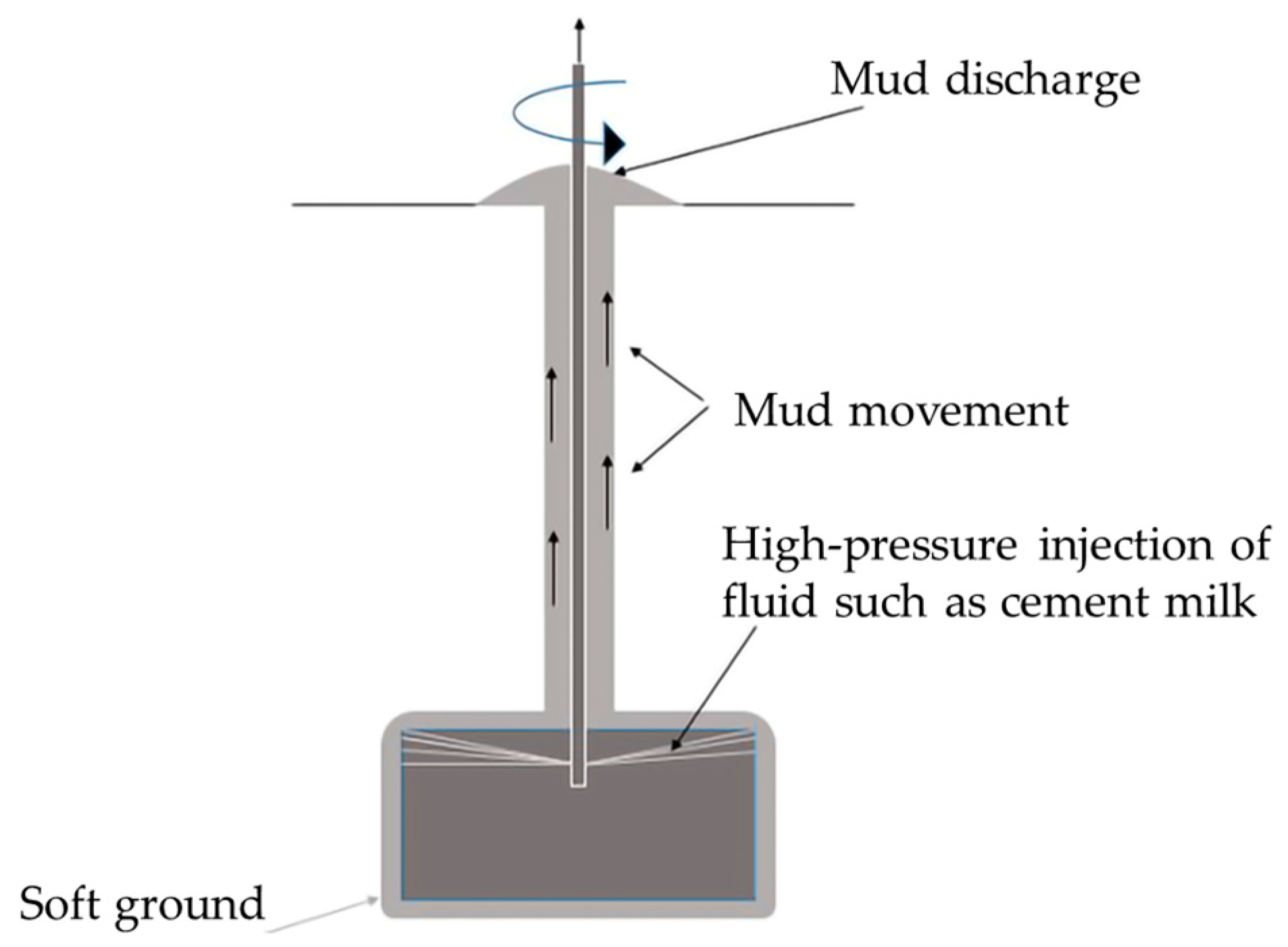
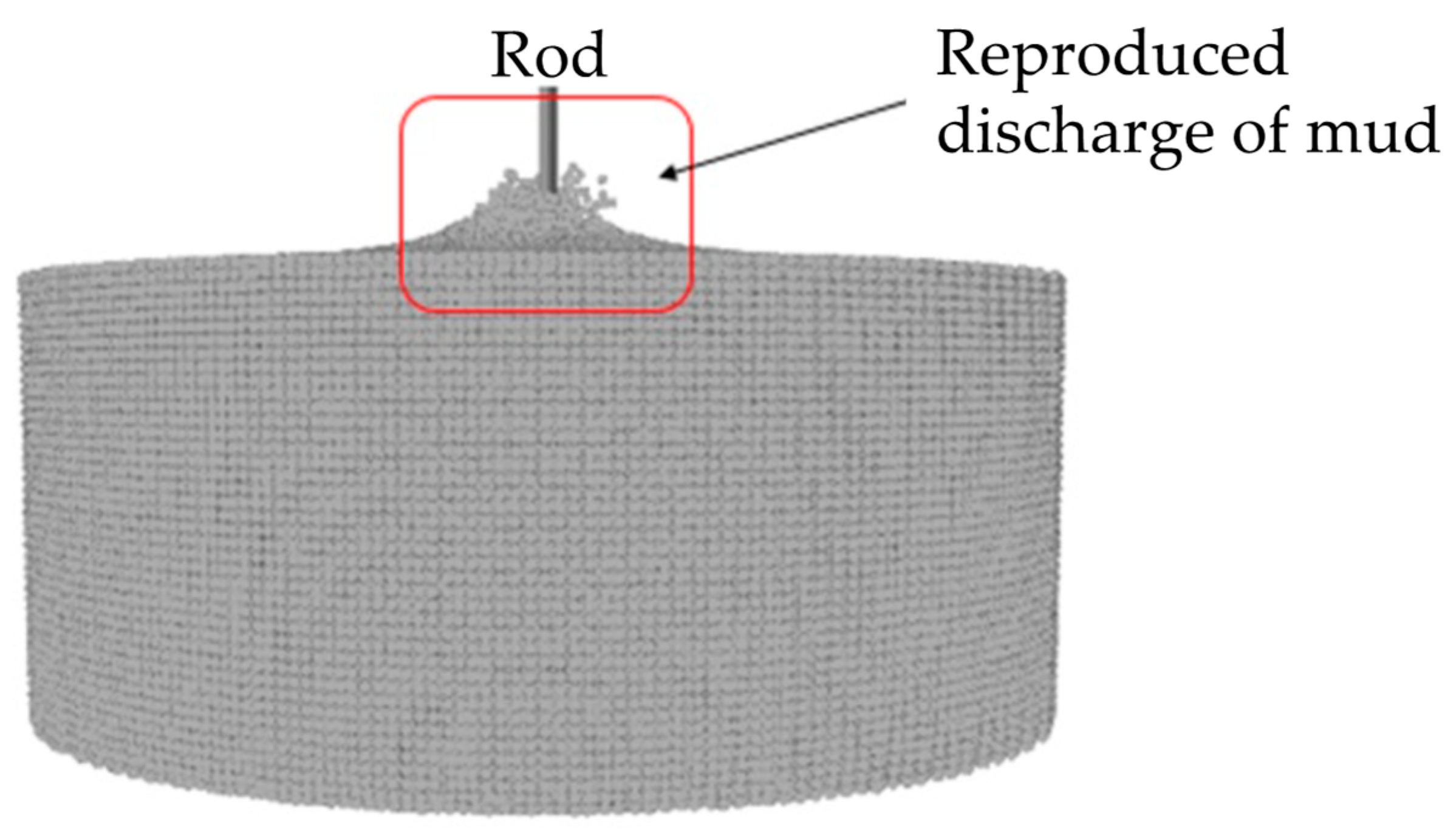
3.1. Mud Drainage by Jet Grouting System
3.2. Quality Control Method and Issues of Improved Body by Jet Grouting Method
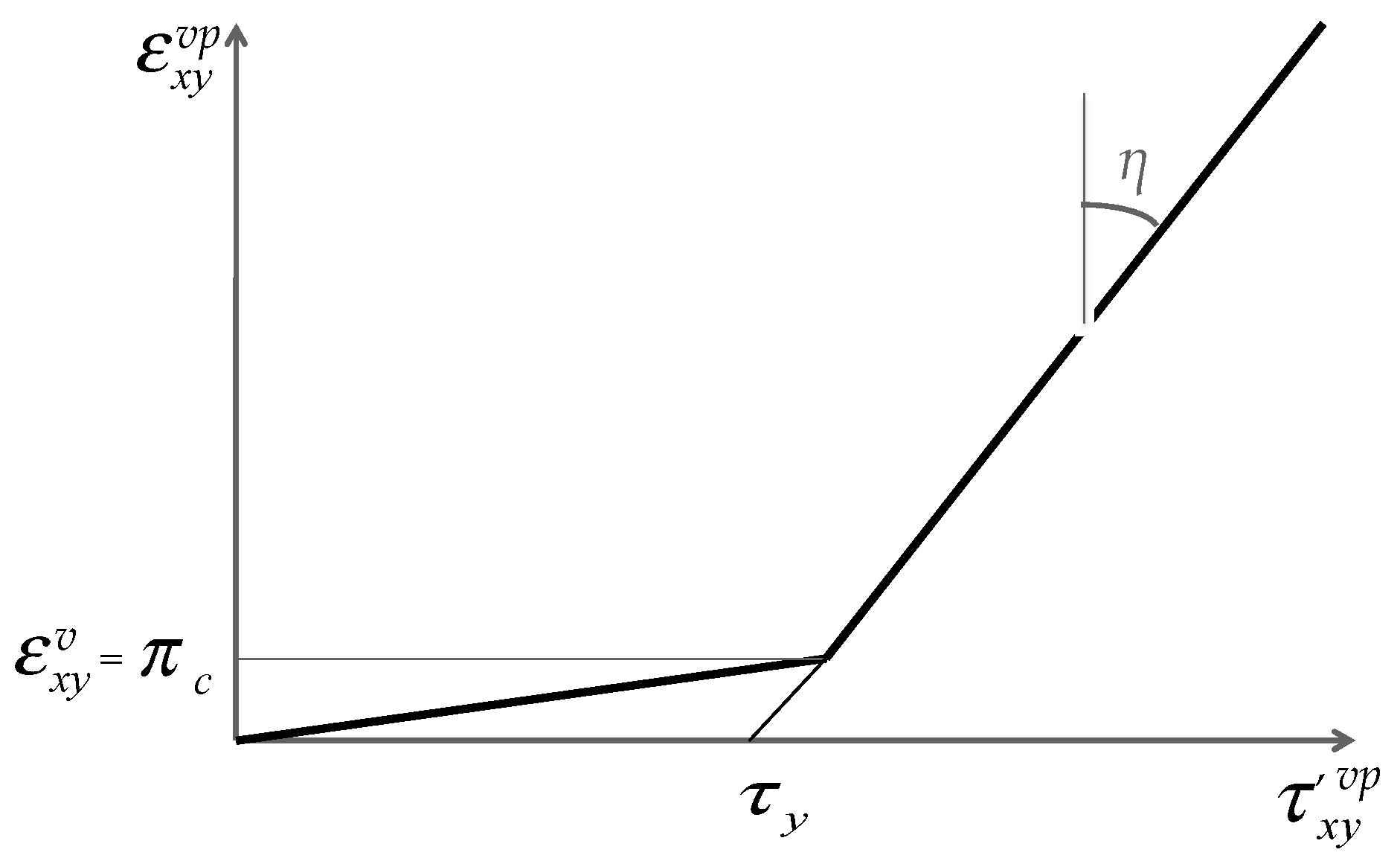
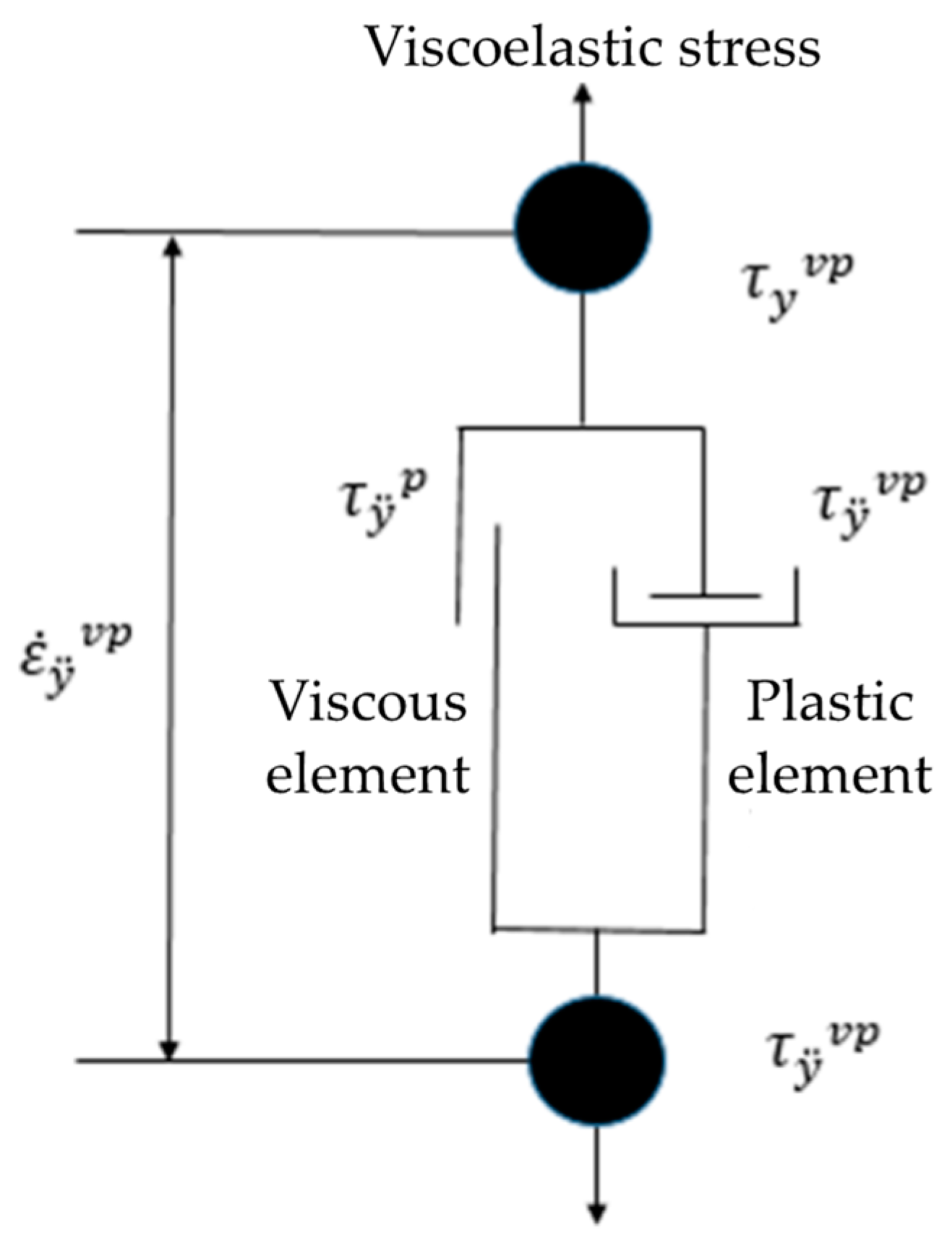
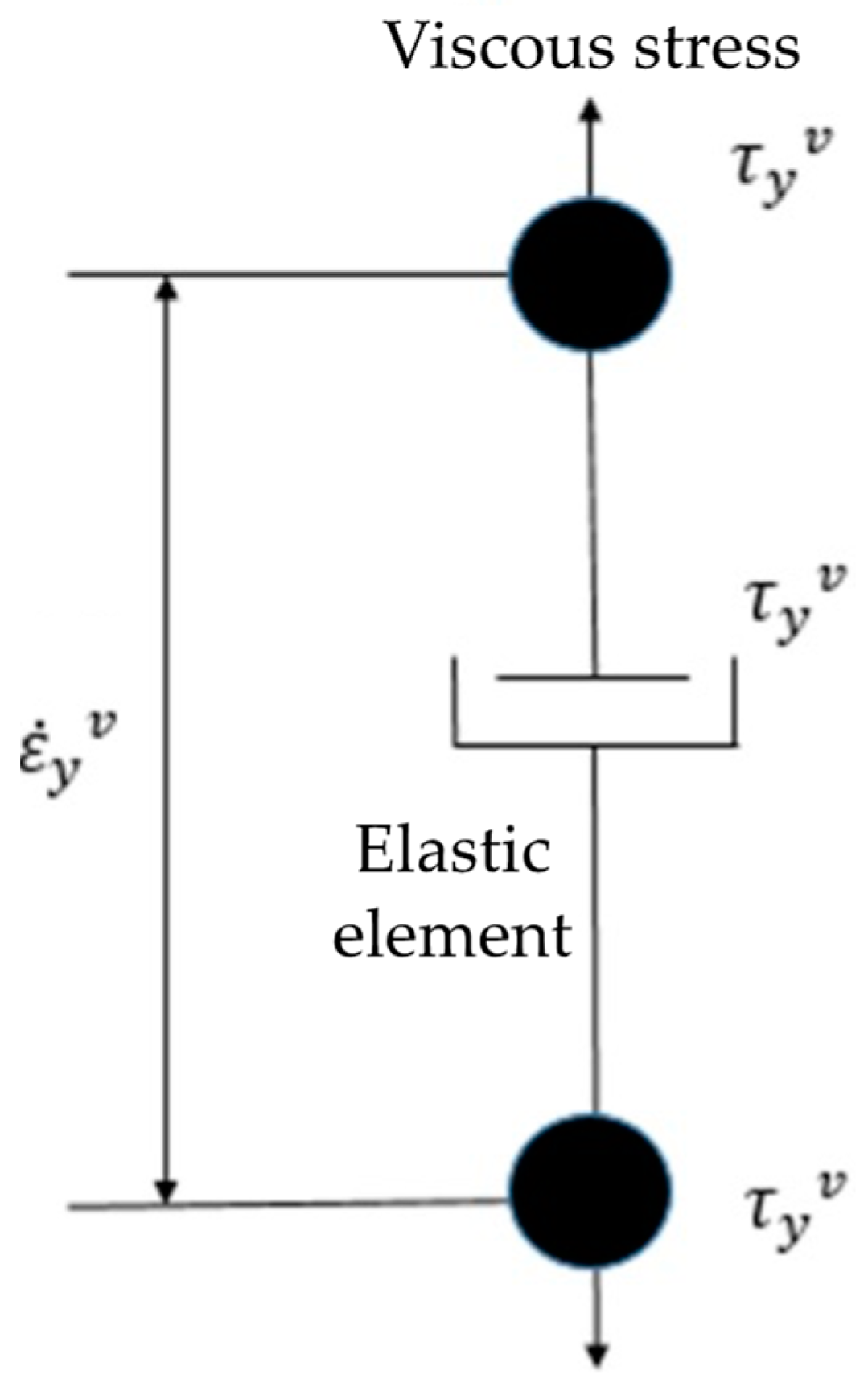
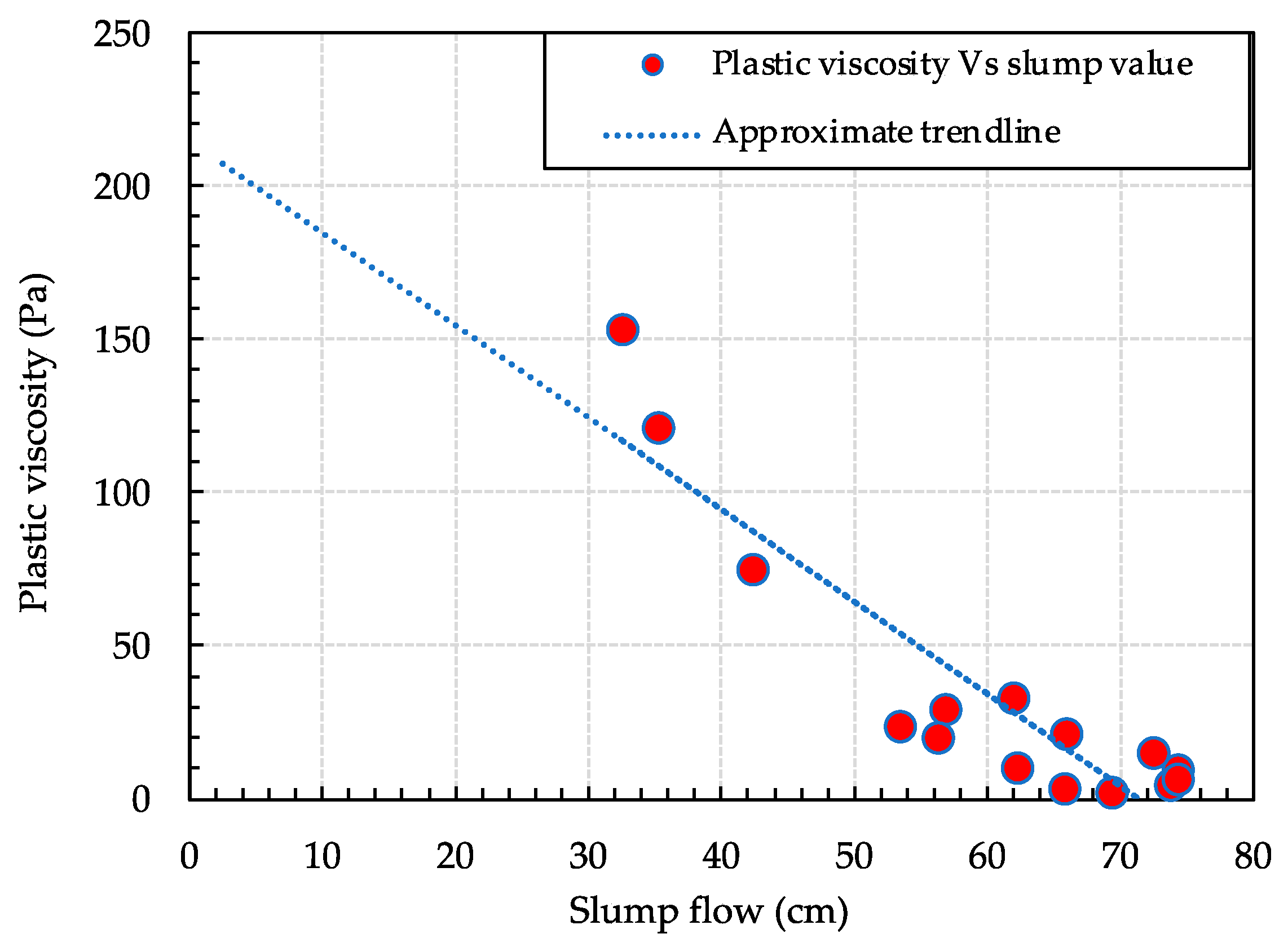
3.3. Application of the Bingham Fluid
4. Target, Conditions, and Material Parameters for Analysis
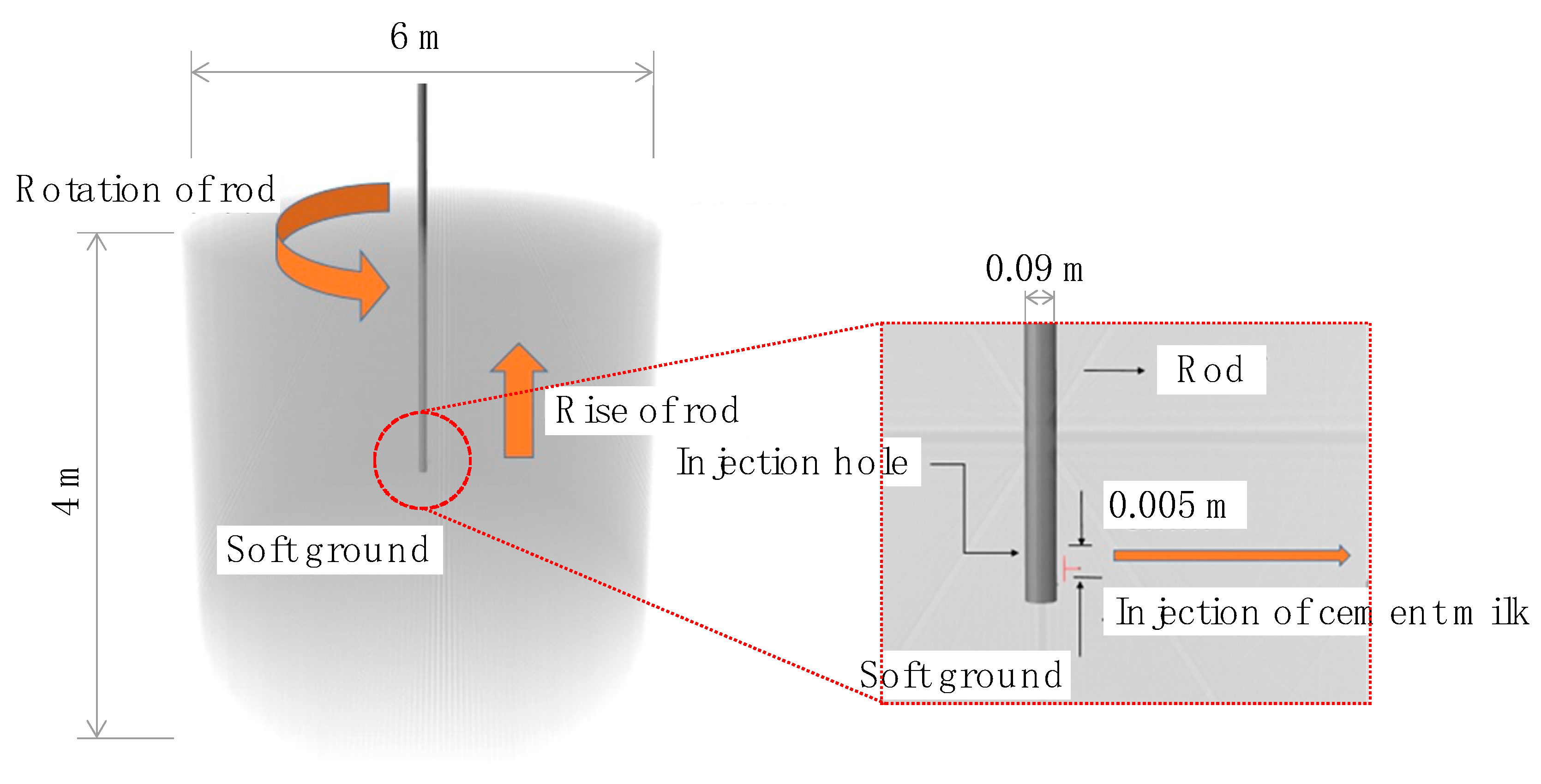
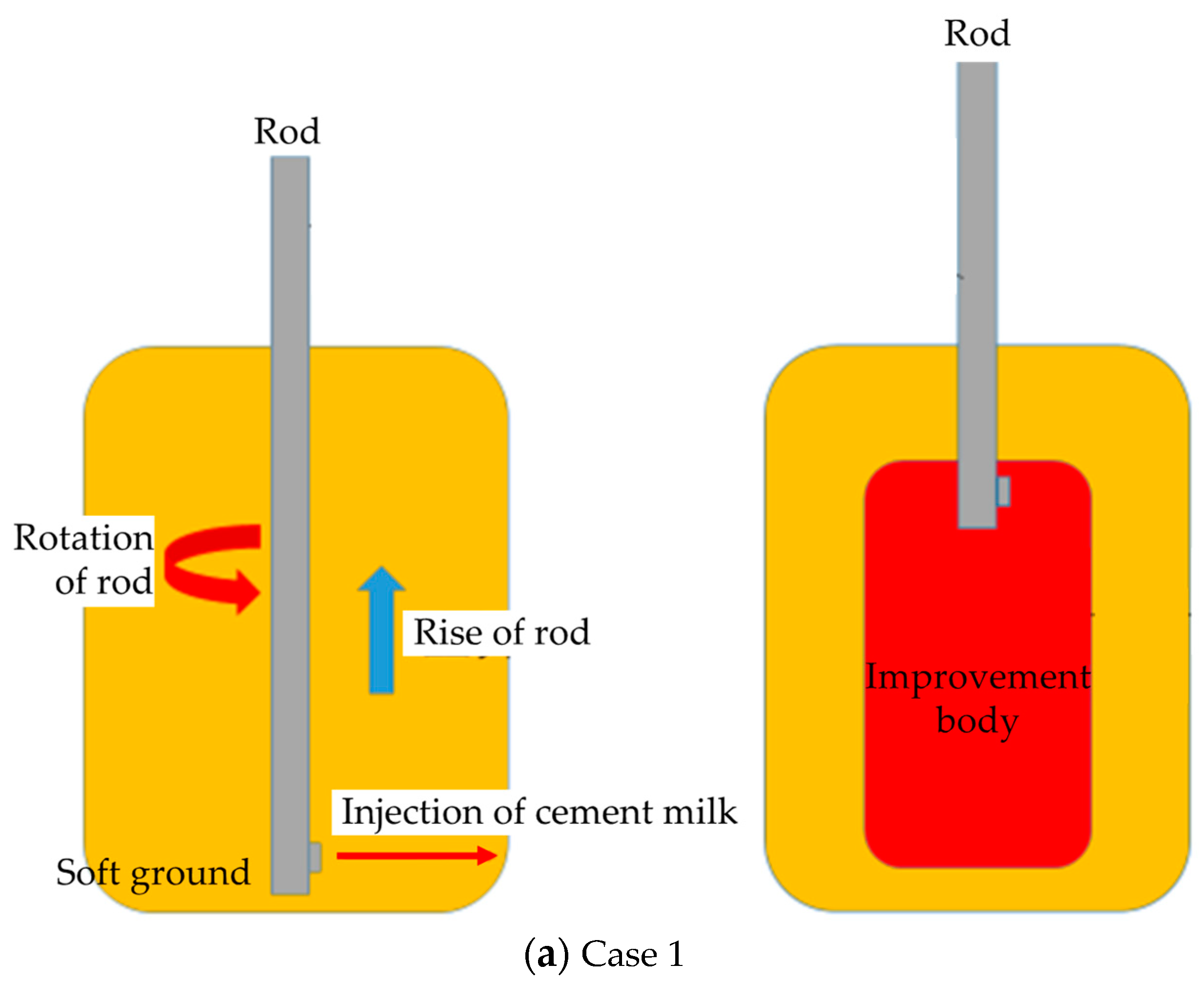
4.1. Analysis Target
4.2. Analysis Conditions
4.3. Material Parameters
5. Results and Discussion
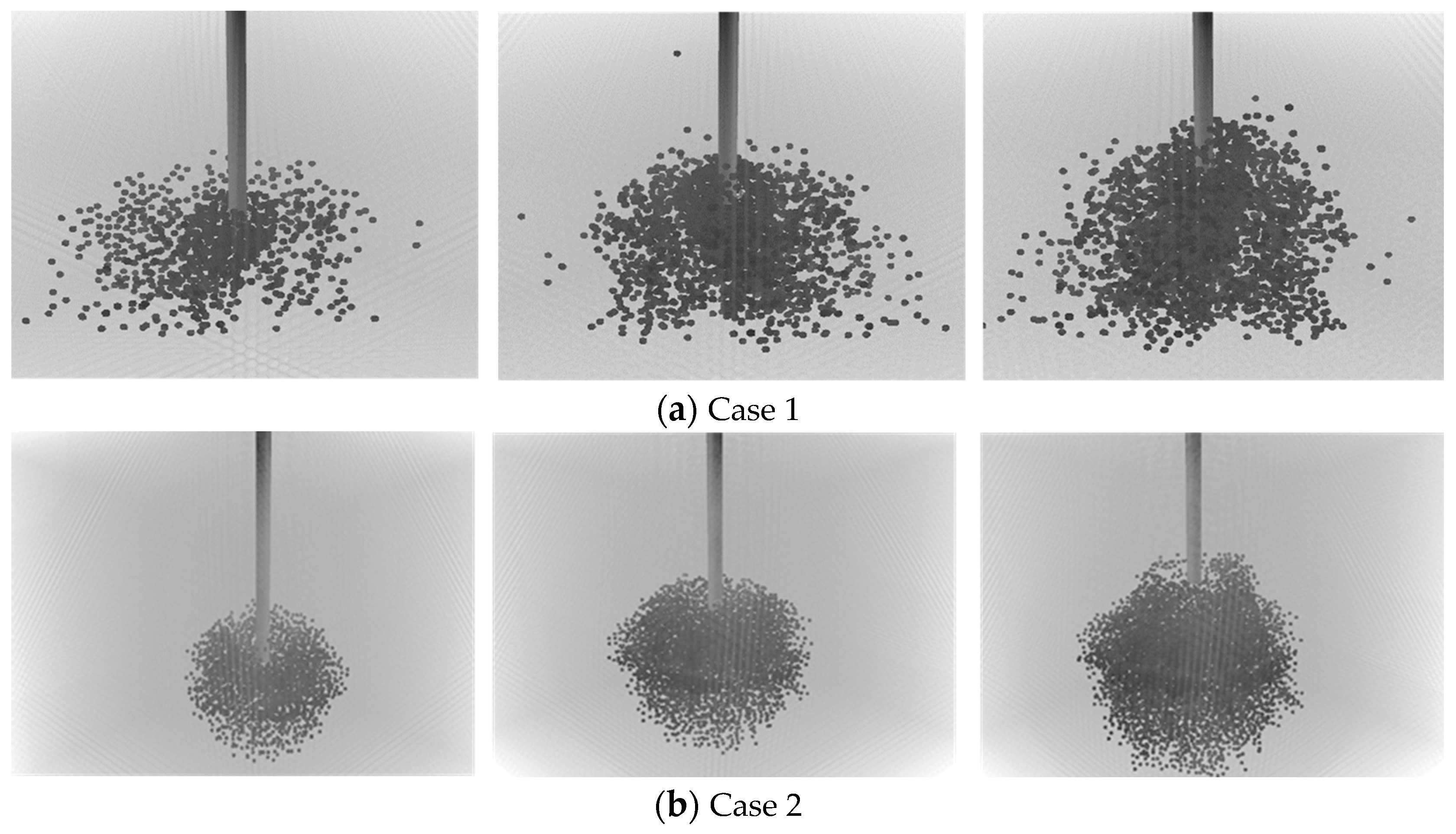
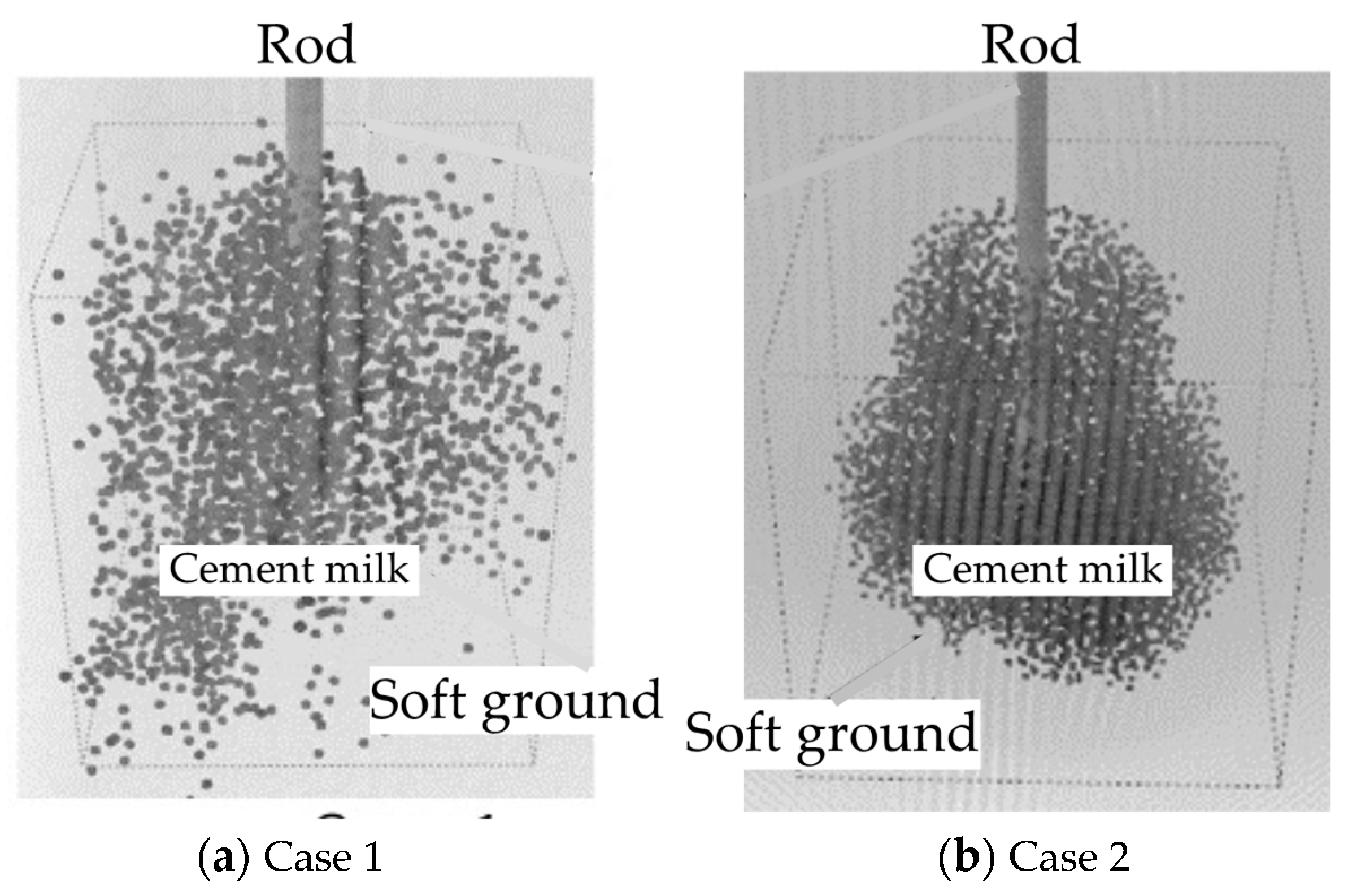
5.1. Reproduction of Improved Body Construction Condition
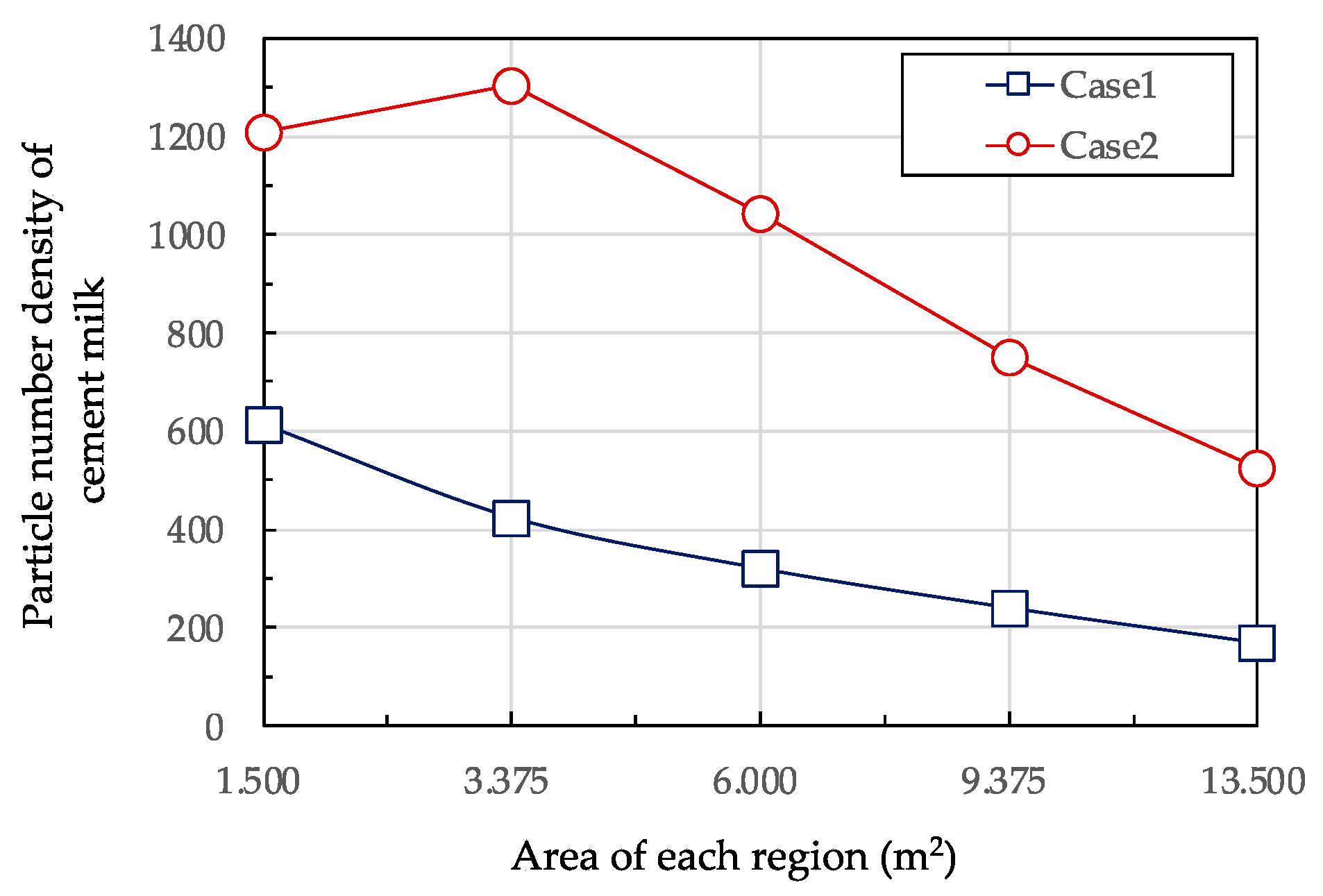
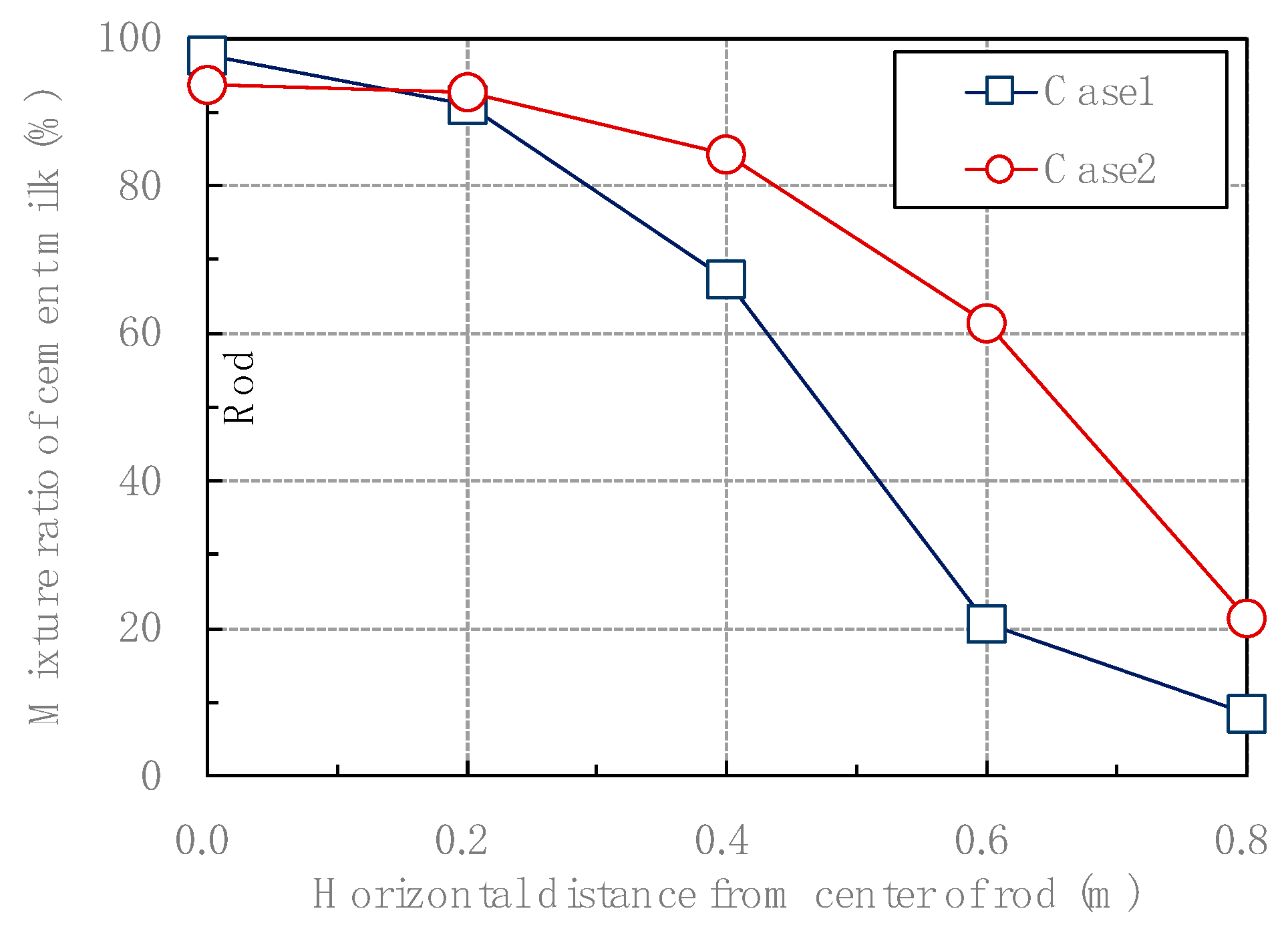
5.2. Comparison of Improved Body Diameters
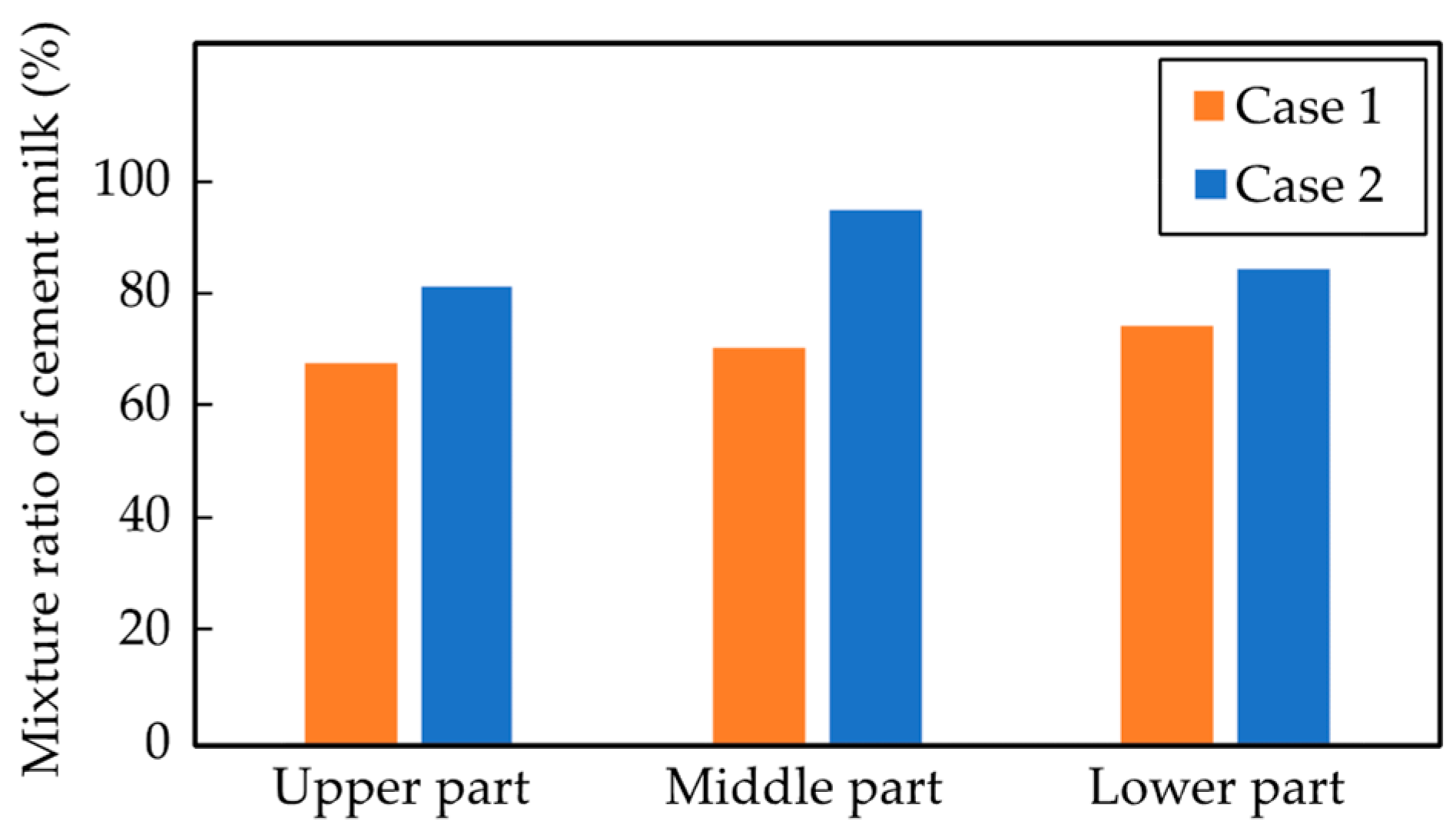
5.3. Comparison of Upper, Middle, and Lower Parts of Improved Body
6. Conclusions
- (1)
- Case 2 of the ascending and descending method showed a higher particle number density, with the maximum density at the middle portion.
- (2)
- Case 2 produced a higher effective diameter, assuming an 80% mixing ratio as the benchmark.
- (3)
- Meanwhile, the mixing ratio throughout the height of the soil body improvement is lower, but uniform in Case 1 compared to Case 2.
- (4)
- The mud discharge was recreated in both cases, but there were no distinct changes in the amount of mud discharge, suggesting the success in the development of a more effective method than the traditional technique.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Covil, C.S.; Skinner, A.E. Jet grouting—A review of some of the operating parameters that form the basis of the jet grouting process. Grouting Ground 1994, 605–629. [Google Scholar] [CrossRef]
- Modoni, G.; Croce, P.; Mongiovì, L. Theoretical modelling of jet grouting. Géotechnique 2006, 56, 335–347. [Google Scholar] [CrossRef]
- Shibazaki, M. State of Practice of Jet Grouting. In Grouting and Ground Treatment; American Society of Civil Engineers: Reston, VA, USA, 2003. [Google Scholar] [CrossRef]
- Burke, G.K. Jet Grouting Systems: Advantages and Disadvantages. In Proceedings of the GeoSupport 2004, Orlando, FL, USA, 29–31 January 2003. [Google Scholar] [CrossRef]
- Choi, R.F.Y. Review of the jet Grouting Method. Bachelor’s Thesis, University of Southern Queensland, Darling Heights, Australia, 2005. [Google Scholar]
- Shimano, A.; Yamazaki, J.; Shinsaka, T. The study about work progress control of jet grouting method. In Proceedings of the 12th National Symposium on Ground Improvement, Kyoto, Japan, 27–28 October 2016; pp. 455–458. [Google Scholar]
- Komaki, T.; Fukada, S.; Sumi, K.; Nakanishi, Y.; Tanaka, N. Demonstration experiment of evolution technology by combination mixing slurry of middle pressure injection total system. In Proceedings of the 53th Japan National Conference on Geotechnical Engineering, Takamatsu, Japan, 25–27 July 2018; pp. 835–836. [Google Scholar]
- Komaki, T.; Fukada, S.; Shimano, A.; Nakanishi, Y. Efficient of Mechanical mixing method with middle pressure injection by High performance fluidizing agent. In Proceedings of the 13th National Symposium on Ground Improvement, Tokyo, Japan, 18–19 October 2018; pp. 375–378. [Google Scholar]
- Inazumi, S.; Komaki, T.; Nakanishi, Y.; Hashida, H.; Suzuki, M. Qualities evaluation on high pressure jet mixing ground improvement methods by distinct element method (DEM). In Proceedings of the 13th National Symposium on Ground Improvement, Tokyo, Japan, 18–19 October 2018; pp. 211–216. [Google Scholar]
- Nakao, K.; Inazumi, S.; Takahashi, T.; Nontananandh, S. Numerical simulation of the liquefaction phenomenon by MPSM-DEM coupled CAES. Sustainability 2022, 14, 7517. [Google Scholar] [CrossRef]
- Inazumi, S.; Shakya, S.; Komaki, T.; Nakanishi, Y. Numerical analysis on performance of middle-pressure jet grouting method for ground improvement. Geosciences 2021, 11, 313. [Google Scholar] [CrossRef]
- Hamri, O.; Léon, J.C.; Giannini, F.; Falcidieno, B. Software environment for CAD/CAE integration. Adv. Eng. Softw. 2010, 41, 1211–1222. [Google Scholar] [CrossRef]
- Nakao, K.; Inazumi, S.; Takaue, T.; Tanaka, S.; Shinoi, T. Evaluation of discharging surplus soils for relative stirred deep mixing methods by MPS-CAE analysis. Sustainability 2022, 14, 58. [Google Scholar] [CrossRef]
- Shakya, S.; Inazumi, S.; Nontananandh, S. Potential of computer-aided engineering in the design of ground improvement technologies. Appl. Sci. 2022, 12, 9675. [Google Scholar] [CrossRef]
- Ni, J.C.; Cheng, W.C. Quality control of double fluid jet grouting below groundwater table: Case history. Soils Found. 2014, 54, 1039–1053. [Google Scholar] [CrossRef]
- Burke, G.; Cacoilo, D.; Chadwick, K. SuperJet Grouting: New Technology for In Situ Soil Improvement. Transp. Res. Rec. J. Transp. Res. Board 2000, 1721, 45–53. [Google Scholar] [CrossRef]
- Croce, P.; Flora, A. Analysis of single-fluid jet grouting. Géotechnique 2000, 50, 739–748. [Google Scholar] [CrossRef]
- Lunardi, P. Ground improvement by means of jet-grouting. Ground Improv. 1997, 1, 65–85. [Google Scholar] [CrossRef]
- Wang, Z.F.; Shen, S.L.; Ho, E.C.; Kim, Y.H. Jet grouting practice: An overview. Geotech. Eng. 2013, 44, 88–96. [Google Scholar]
- Hattori, M. An attempt to apply mathematical foundation of moving particle simulation to the MPS software “Paticleworks”. Proc. Symp. Educ. Appl. Inf. Technol. 2015, 9, 59–62. [Google Scholar]
- Shakibaeini, A.; Jin, Y.C. MPS mesh-free particle method for multiphase flows. Comput. Methods Appl. Mech. Eng. 2012, 229–232, 13–26. [Google Scholar] [CrossRef]
- Tanaka, M.; Cardoso, R.; Bahai, H. Multi-resolution MPS method. J. Comput. Phys. 2018, 359, 106–136. [Google Scholar] [CrossRef]
- Croce, P.; Flora, A.; Modoni, G. Jet Grouting: Technology, Design and Control; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Harada, K.; Ohbayashi, J.; Matsumoto, J.; Kubo, Y.; Akima, T. New ground improvement technologies under restricted conditions in Japan. In Proceedings of the 15th Asian Regional Conference on Soil Mechanics and Geotechnical Engineering, Fukuoka, Japan, 9–13 November 2015; Japanese Geotechnical Society Special Publication: Tokyo, Japan, 2016. [Google Scholar] [CrossRef]
- Njock, P.G.A.; Chen, J.; Modoni, G.; Arulrajah, A.; Kim, Y.H. A review of jet grouting practice and development. Arab. J. Geosci. 2018, 11, 459. [Google Scholar] [CrossRef]
- Wanik, L.; Mascolo, M.C.; Bzówka, J.; Modoni, G.; Shen, J.S.L. Experimental Evidence on the Strength of Soil Treated with Single and Double Fluid Jet Grouting. In Grouting 2017; American Society of Civil Engineers: Reston, VA, USA, 2017. [Google Scholar] [CrossRef]
- Toraldo, C.; Modoni, G.; Ochmański, M.; Croce, P. The characteristic strength of jet-grouted material. Géotechnique 2018, 68, 262–279. [Google Scholar] [CrossRef]
- Modoni, G.; Bzówka, J.; Pieczyrak, J. Experimental investigation and numerical modelling on the axial loading of jet grouting columns. Archit. Civ. Eng. Environ. 2010, 3, 69–78. [Google Scholar]
- Zhu, H.; Martys, N.S.; Ferraris, C.; Kee, D.D. A numerical study of the flow of Bingham-like fluids in two-dimensional vane and cylinder rheometers using a smoothed particle hydrodynamics (SPH) based method. J. Non-Newton. Fluid Mech. 2010, 165, 362–375. [Google Scholar] [CrossRef]
- Fukuzawa, Y.; Tomiyama, H.; Shibata, K.; Koshizuka, S. Flow analysis of highly viscous non-Newtonian fluid by MPS method. Trans. Jpn. Soc. Comput. Eng. Sci. 2014, 2014, 20140007. [Google Scholar] [CrossRef]
- Shakya, S.; Inazumi, S. Soil behavior modeling by mps-cae simulation. Geomate J. 2023, 24, 18–25. [Google Scholar] [CrossRef]
- Güllü, H. A new prediction method to rheological behavior of grout with bottom ash for jet grouting columns. Soils Found. 2017, 57, 384–396. [Google Scholar] [CrossRef]
- Güllü, H.; Cevik, A.; Al-Ezzi, K.M.; Gülsan, M.E. On the rheology of using geopolymer for grouting: A comparative study with cement-based grout included fly ash and cold bonded fly ash. Constr. Build. Mater. 2019, 196, 594–610. [Google Scholar] [CrossRef]
- Tomiyama, J.; Iribe, T.; Sakihara, K.; Iraha, S.; Yamada, Y. Application of MPS method to flow analysis of fresh concrete. J. Struct. Eng. A 2009, 55, 164–171. [Google Scholar] [CrossRef]
- Li, Z.; Ohkubo, T.; Tanigawa, Y. Flow performance of high-fluidity concrete. J. Mater. Civ. Eng. 2004, 16, 588–596. [Google Scholar] [CrossRef]
- Ferraris, C.F.; Brower, L.E. Comparison of Concrete Rheometers: International Tests at MBT (Cleveland OH, USA) in May 2003; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2004; pp. 41–70.


| Case | Rotation Speed (rpm) | Spray Volume (m3/min) | Rod Motion |
|---|---|---|---|
| 1 | 20 | 0.09 | 1.5 m/min upward |
| 2 | 20 | 0.09 | 3 m/min upward |
| 3 m/min downward |
| Density (kg/m3) | Plastic Viscosity (Pa·s) | Yield Value (Pa) | |
|---|---|---|---|
| Cement milk | 1600 | 0.28 | 0 |
| Ground | 2300 | 200 | 100,000 |
| Case | Volume of Area (m3) | 13.5 | 9.375 | 6 | 3.375 | 1.5 |
|---|---|---|---|---|---|---|
| 1 | Number of particles of cement milk | 2265 | 2239 | 1924 | 1434 | 921 |
| Number of ground particles | 8049 | 7276 | 4684 | 2265 | 694 | |
| Total particle numbers | 10,314 | 9515 | 6608 | 3699 | 1615 | |
| Density of number of particles (number/m3) | 168 | 239 | 320 | 424 | 614 | |
| 2 | Number of particles of cement milk | 7114 | 7027 | 6275 | 4401 | 1832 |
| Number of ground particles | 11,936 | 9448 | 3923 | 1011 | 259 | |
| Total particle numbers | 19,048 | 16,475 | 10,198 | 5411 | 2091 | |
| Particle number density (number/m3) | 527 | 750 | 1045 | 1304 | 1211 |
| Case | Apart from Central Axis (m) | 0.0 | 0.2 | 0.4 | 0.6 | 0.8 | |
|---|---|---|---|---|---|---|---|
| 1 | Number of particles | Cement milk | 173 | 163 | 122 | 38 | 15 |
| Ground | 4 | 13 | 59 | 147 | 165 | ||
| Total | 177 | 179 | 181 | 185 | 180 | ||
| Mixture ratio of improved body (%) | 97.7 | 91.1 | 67.4 | 20.5 | 8.3 | ||
| 2 | Number of particles | Cement milk | 193 | 167 | 225 | 169 | 68 |
| Ground | 13 | 13 | 42 | 106 | 250 | ||
| Total | 205 | 180 | 267 | 275 | 318 | ||
| Mixture ratio of improved body (%) | 93.7 | 92.8 | 84.3 | 61.5 | 21.4 | ||
| Case | Portion | Upper | Middle | Lower | |
|---|---|---|---|---|---|
| 1 | Number of particles | Cement milk | 156 | 165 | 167 |
| Ground | 73 | 67 | 56 | ||
| Total | 229 | 232 | 223 | ||
| Density of number of particles (number/m3) | 68 | 71 | 75 | ||
| 2 | Number of particles | Cement milk | 330 | 383 | 342 |
| Ground | 73 | 18 | 61 | ||
| Total | 403 | 401 | 403 | ||
| Density of number of particles (number/m3) | 82 | 96 | 85 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shakya, S.; Inazumi, S.; Chao, K.C.; Wong, R.K.N. Innovative Design Method of Jet Grouting Systems for Sustainable Ground Improvements. Sustainability 2023, 15, 5602. https://doi.org/10.3390/su15065602
Shakya S, Inazumi S, Chao KC, Wong RKN. Innovative Design Method of Jet Grouting Systems for Sustainable Ground Improvements. Sustainability. 2023; 15(6):5602. https://doi.org/10.3390/su15065602
Chicago/Turabian StyleShakya, Sudip, Shinya Inazumi, Kuo Chieh Chao, and Ricky K. N. Wong. 2023. "Innovative Design Method of Jet Grouting Systems for Sustainable Ground Improvements" Sustainability 15, no. 6: 5602. https://doi.org/10.3390/su15065602
APA StyleShakya, S., Inazumi, S., Chao, K. C., & Wong, R. K. N. (2023). Innovative Design Method of Jet Grouting Systems for Sustainable Ground Improvements. Sustainability, 15(6), 5602. https://doi.org/10.3390/su15065602







