Optimization of Timetables on the Bratislava–Žilina–Košice Route in the Period after the End of the COVID-19 Pandemic
Abstract
1. Introduction
2. Materials and Methods
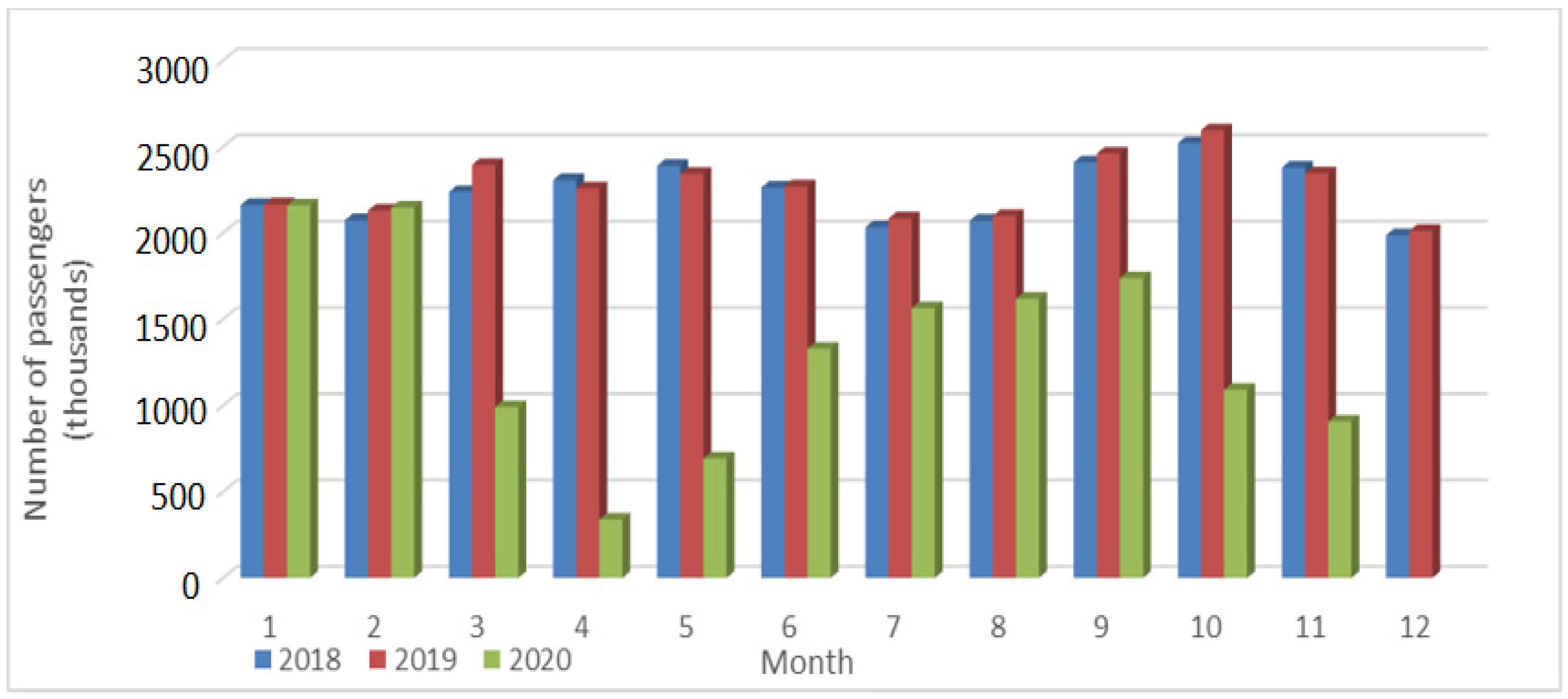
2.1. Passenger Frequencies during the Year 2020
2.2. Scientific Methods
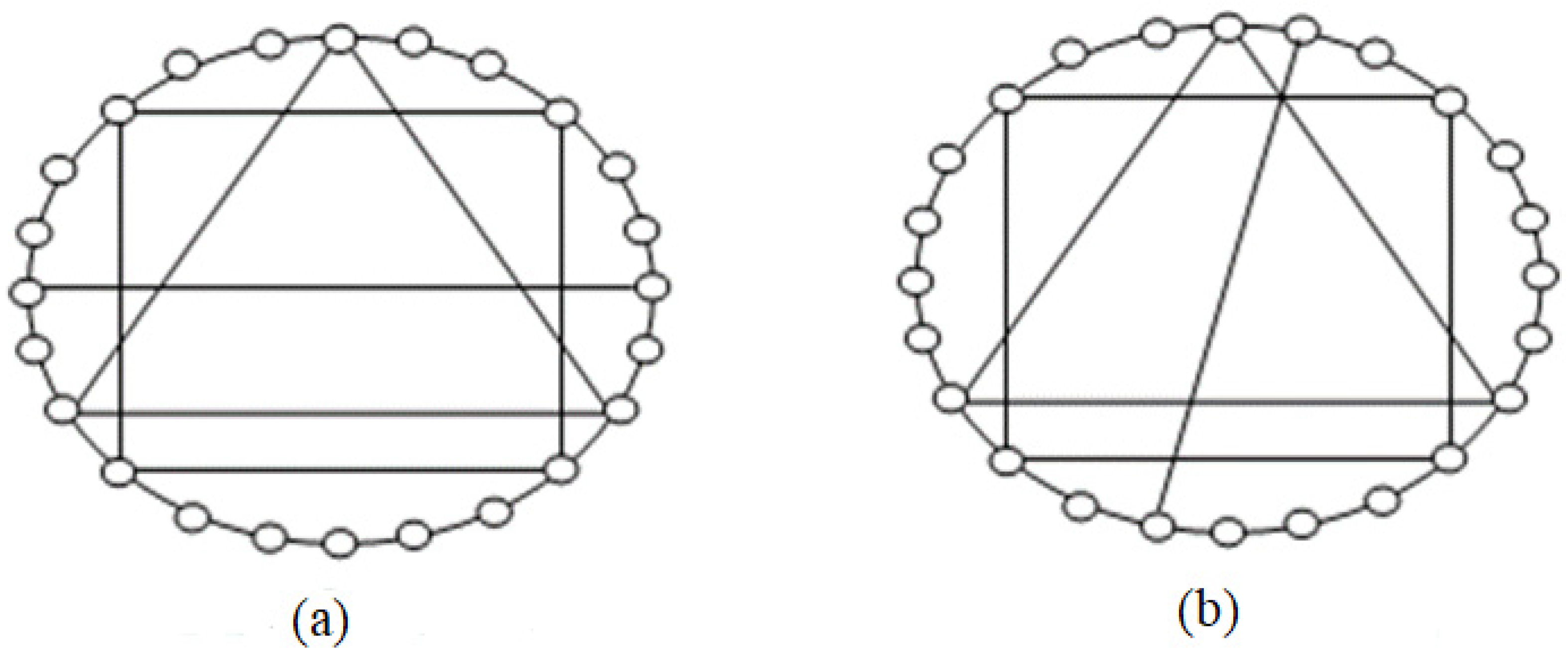
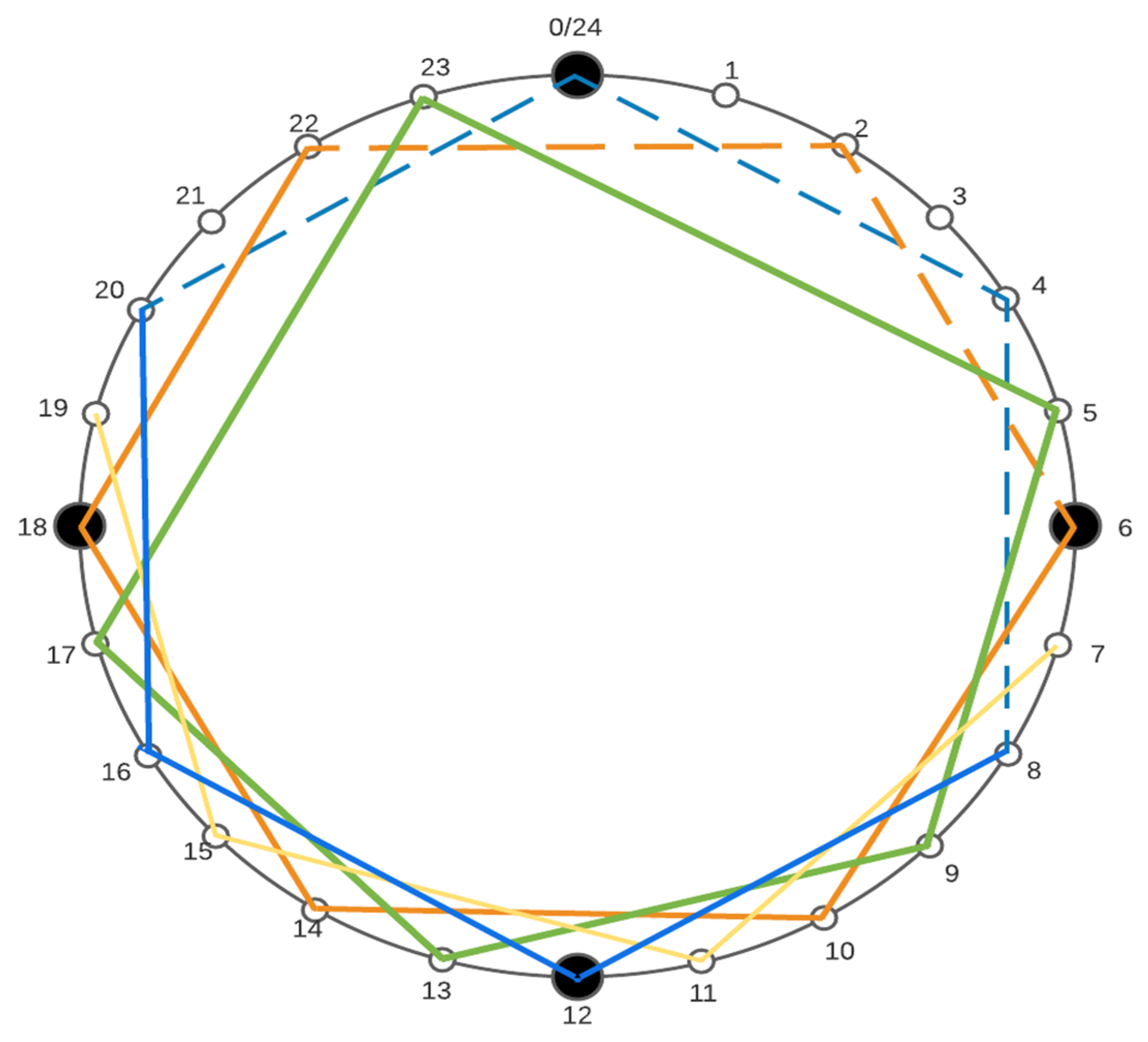
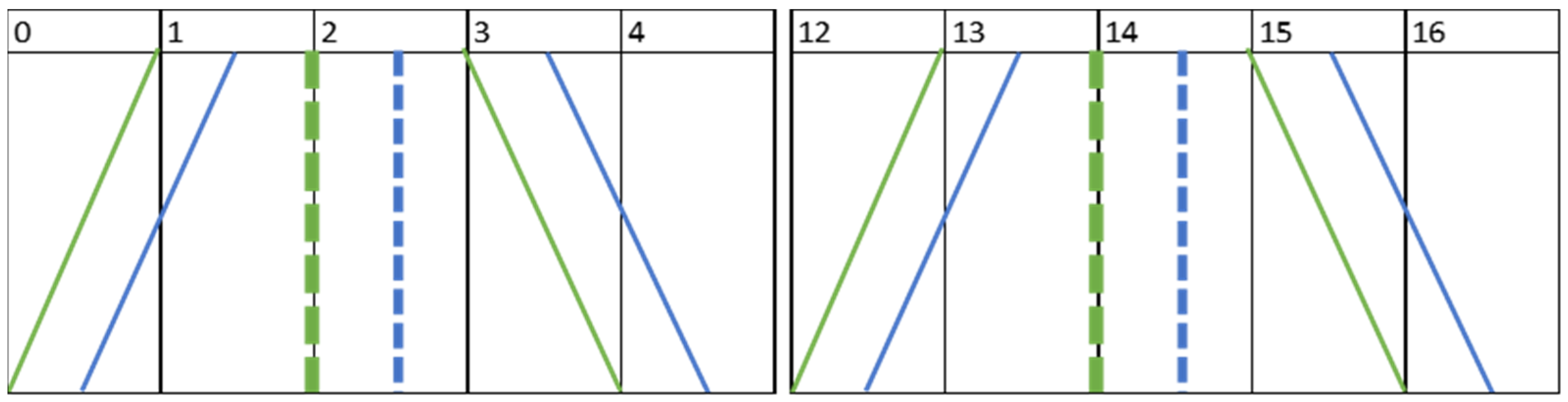
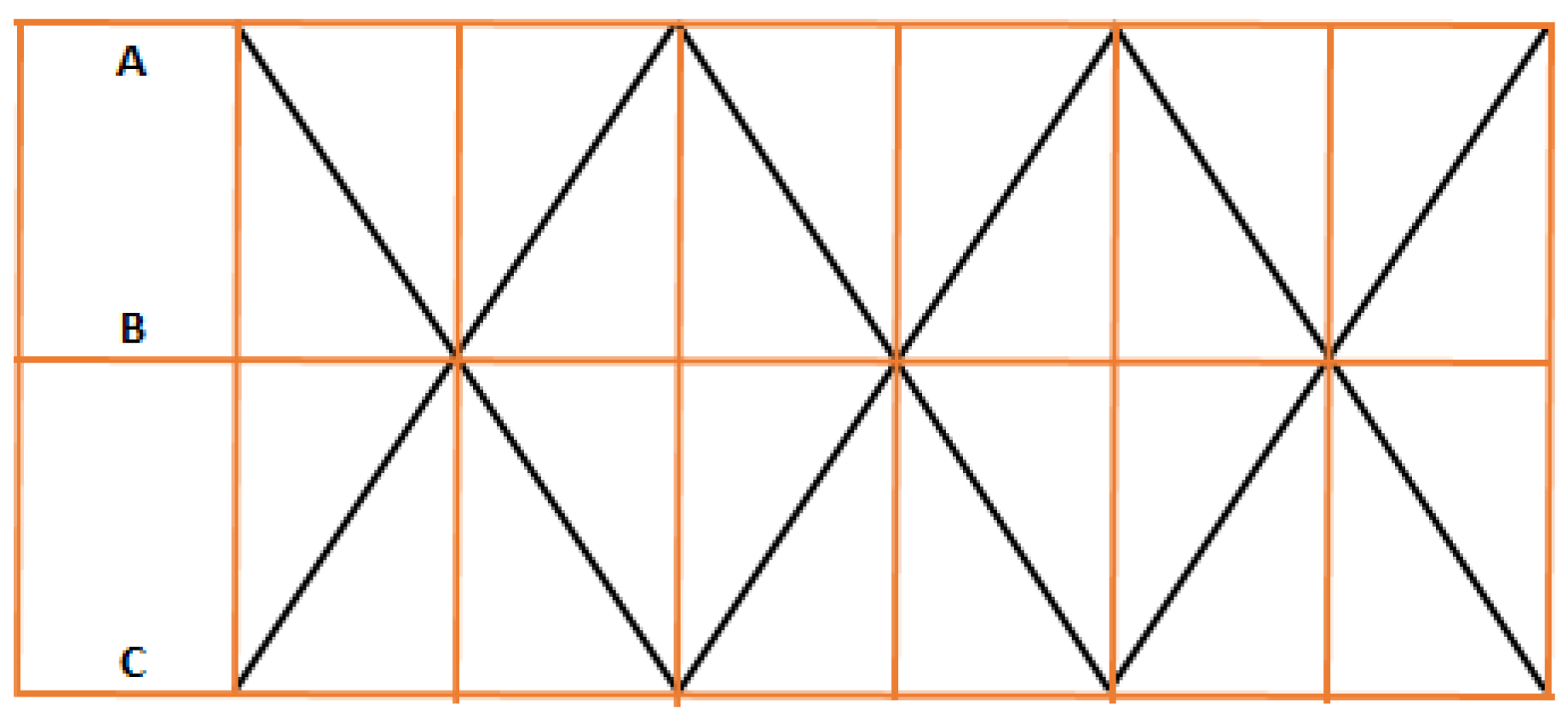
2.2.1. Method of Inscribed n-Angles
- Brown color—Inter City train line on the (Vienna)—Bratislava–Žilina–Košice route,
- Blue color—Express train line on the Bratislava–Žilina–Košice route,
- Green color—Fast train line on the Bratislava–Žilina–Košice route,
- Yellow color—Fast train line on the Bratislava–Žilina–Prešov route.
2.2.2. Additional Methods
- Synthesis method—this method, based on experience or logic, proceeds from the simplest principles to more complex ones by merging and connecting individual parts into a whole. In the case of proposals and outputs of this paper, it is a combination of partial proposals of individual connections and lines on the route representing a complete 24-h systematic timetable;
- Deduction method—it is a scientific method in which specific, special and less general elements and conclusions are derived from general outputs and conclusions. In this case, it is the application of general standards of a transport service to specific draft timetables;
- Brainstorming method—it is known as a creative method used to solve various problems using the generation of progressive ideas and thoughts. The result should be an original and unique solution to a specific problem, which also represents the proposals and outputs listed in the chapter;
- Delphi method—it is an expert, in other words, prognostic method looking for a group solution to a certain problem, based on the opinions, estimates, and solutions of a group of selected experts whose knowledge was also used in the given proposals [29].
2.3. Draft Standards of a Transport Service in National Long-Distance Transport
2.3.1. Fast, High-Quality, All-Day Transport
2.3.2. Symmetrical Time Positions of Individual Connections
2.3.3. Rationalization of Transfer Links to Lower Category Trains
2.3.4. Rationalization of a Band Service in Long-Distance Transport
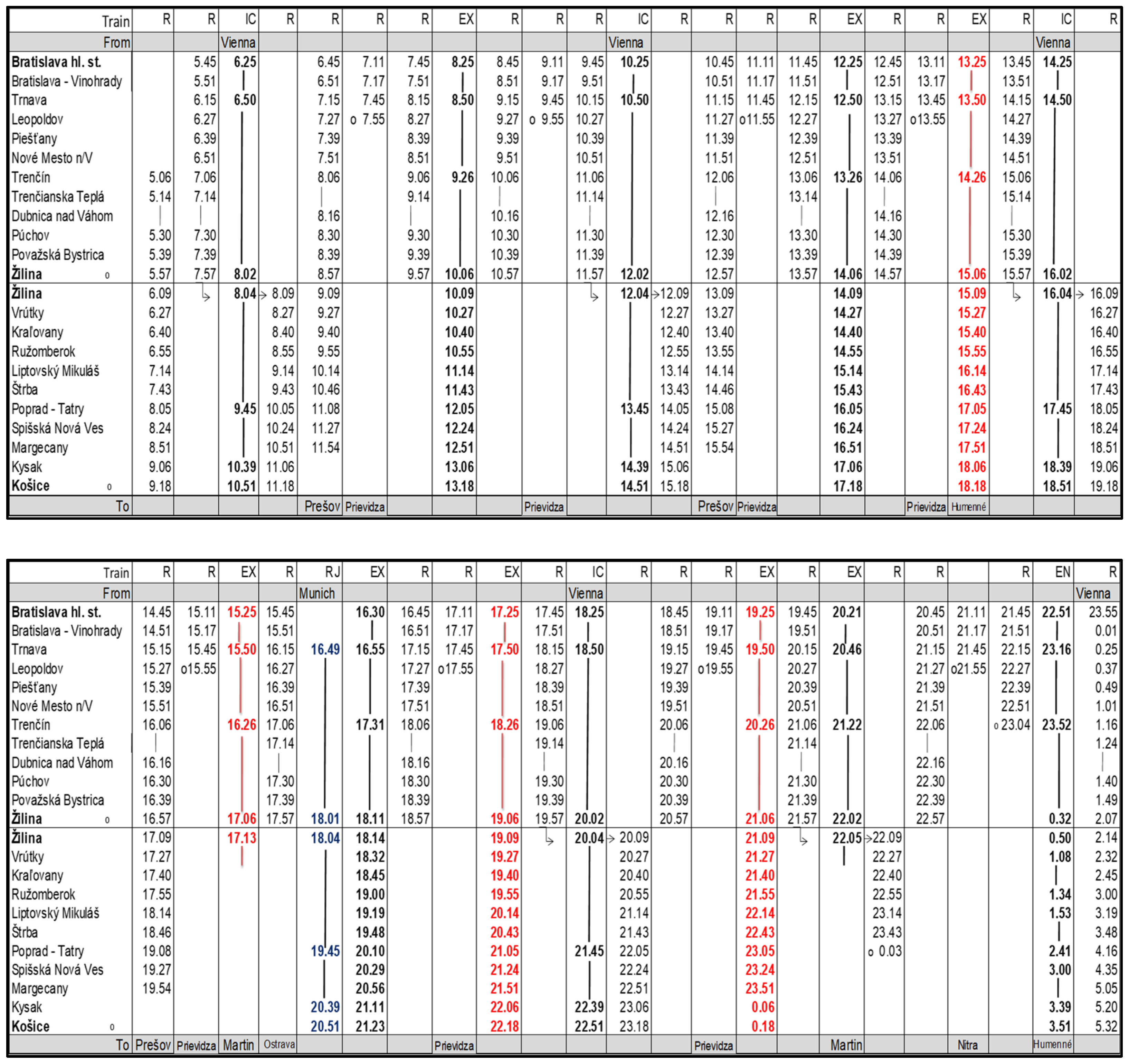
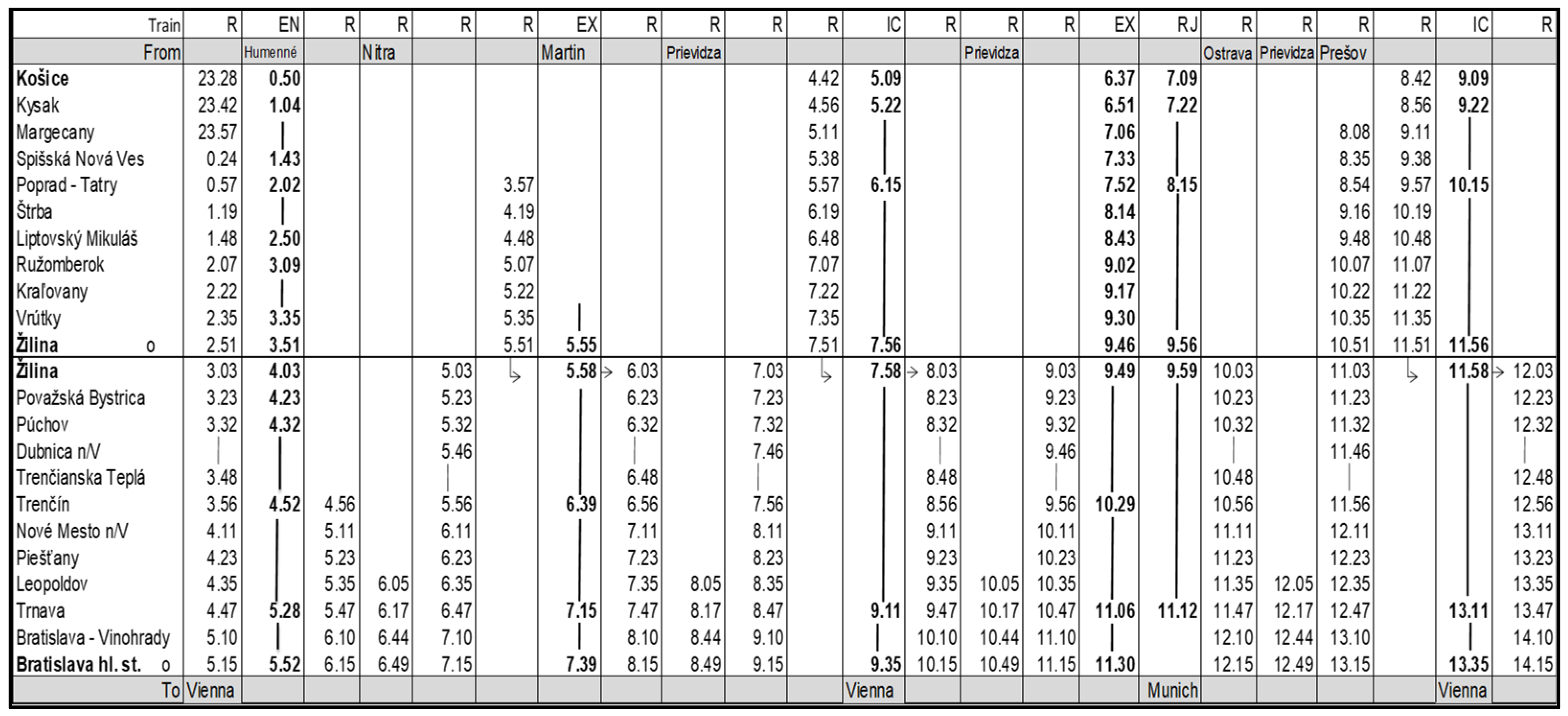
3. Results
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ministry of Transport and Construction of the Slovak Republic. Strategy for the Development of Public Passenger and Non-Motorized Transport in the Slovak Republic until 2020; Ministry of Transport and Construction of the Slovak Republic: Bratislava, Slovakia, 2014; 68p. [Google Scholar]
- Boarnet, M.G.; Haughwout, A.F. Do Highways Matter? Evidence and Policy Implications of Highways’ Influence on Metropolitan Development; University of California Transportation Center: Berkeley, CA, USA, 2020. [Google Scholar]
- Lampa, M.; Smolejová, M. Fleet Optimalization Based on the Monte Carlo Method. Acta Logist. 2020, 7, 17–21. [Google Scholar]
- Mašek, J.; Kendra, M.; Čamaj, J. Model of the Transport Capacity of the Train and Railway Track Based on Used Types of Wagons. In Proceedings of the 20th International Conference, Kaunas, Lithuania, 5–7 October 2016; pp. 584–588. [Google Scholar]
- Buková, B.; Brumerčíková, E.; Kondek, P. Determinants of the EU Transport Market. In Proceedings of the 2016 International Conference on Engineering Science and Management, Zhengzhou, China, 13–14 August 2016; pp. 249–252. [Google Scholar]
- Zheng, M.; Zhou, R.; Liu, S.; Liu, F.; Guo, X. Route Design Model of Multiple Feeder Bus Service Based on Existing Bus Lines. J. Adv. Transp. 2020, 1, 8853872. [Google Scholar] [CrossRef]
- Gašparík, J.; Stopka, O.; Pečený, L. Quality evaluation in regional passenger rail transport. Naše More 2015, 62, 114–118. [Google Scholar] [CrossRef]
- Carteni, A.; D´Acierno, L.; Gallo, M.A. Rational Decision-Making Process with Public Engagement for Designing Public Transport Services: A Real Case Application in Italy. Sustainability 2020, 12, 6303. [Google Scholar] [CrossRef]
- L’upták, V.; Bartuška, L.; Hanzl, J. Assessment of Connection Quality on Transport Networks Applying the Empirical Models in Traffic Planning: A Case Study. In Proceedings of the 22st International Scientific Conference Transport Means, Trakai, Lithuania, 3–5 October 2018. [Google Scholar]
- L’upták, V.; Gašparík, J.; Chovancová, M. Proposal for Evaluating a Connection Quality within Transport Networks. In MATEC Web of Conferences; EDP Sciences: Les Ulis, France, 2017. [Google Scholar] [CrossRef][Green Version]
- Kleprlík, J. Creation of Shift Essentials; University of Pardubice: Pardubice, Czech Republic, 2007; Volume 5, pp. 48–51. [Google Scholar]
- Brumerčíková, E.; Šperka, A. Problems of Access to Services at Railway Stations in Freight Transport in the Slovak Republic. Sustainability 2020, 12, 8018. [Google Scholar] [CrossRef]
- Pellegrini, P.; Marlière, G.; Rodriguez, J. Optimal train routing and scheduling for managing traffic perturbations in complex junctions. Transp. Res. Part B Methodol. 2014, 59, 58–80. [Google Scholar] [CrossRef]
- Sun, Q.; Wang, X.; Ma, F.; Han, Y.; Cheng, Q. Synergetic Effect and Spatial-Temporal Evolution of Railway Transportation in Sustainable Development of Trade: An Empirical Study Based on the Belt and Road. Sustainability 2019, 11, 1721. [Google Scholar] [CrossRef]
- Drabek, M. Irregularities in Czech Integrated Periodic Timetable. In MATEC Web of Conferences; EDP Sciences: Les Ulis, France, 2018; Volume 235, pp. 51–54. [Google Scholar]
- Gašparík, J.; L’upták, V.; Kurekov, P.V.; Meško, P. Methodology for assessing transport connections on the integrated transport network. Commun. Sci. Lett. Univ. Zilina 2017, 19, 61–67. [Google Scholar]
- Kuník, P. The Proposal of the Integrated Cycle Timetable in Slovakia in 2012. Master´s Thesis, University of Pardubice, Pardubice, Czech Republic, 2012. [Google Scholar]
- Kampf, R.; Stopka, O.; Bartuska, L.; Zeman, K. Circulation of vehicles as an important parameter of public transport efficiency. Connections 2015, 5, 6. [Google Scholar]
- Abramović, B.; Šimunec, I. Optimization of Railway Traffic on Varaždin–Golubovec Railway Line. In Proceedings of the International Symposium EURO-ŽEL, Žilina, Slovakia, 5–6 June 2012; Tribun EU Press: Brno, Czech Republic, 2012. [Google Scholar]
- Hansen, I.A.; Pachl, J. Railway Timetabling and Operations: Analysis, Modelling, Optimisation, Simulation, Performance, Evaluation; DVV Media: Hamburg, Germany, 2014. [Google Scholar]
- Mako, P.; Dávid, A.; Böhm, P.; Savu, S. Sustainable Transport in the Danube Region. Sustainability 2021, 13, 6797. [Google Scholar] [CrossRef]
- Lábsky, V. Coordination of Public Transport Backbone Lines by Mathematical Programming Model. Ph.D. Thesis, University of Pardubice, Pardubice, Czech Republic, 2018. [Google Scholar]
- Dočkalíková, I.; Kashi, K. Employees Recruitment: Selecting the Best Candidates by the Utilization of AHP and WSA Method. In Proceedings of the 7th International Days of Statistics and Economics Conference, Prague, Czech Republic, 19–21 September 2013. [Google Scholar]
- Abramović, B.; Nedeliaková, E.; Panák, M. Synergy in Logistics Process for Railway Transport. In Business Logistics in Modern Management; Josip Juraj Strossmayer University of Osijek: Osijek, Croatia, 2017. [Google Scholar]
- Staňo, P. Impact of the COVID-19 Pandemic on Passenger Rail Transport in the Slovak Republic. Master’s Thesis, University of Žilina, Žilina, Slovakia, 2021. [Google Scholar]
- Yaghini, M.; Nikoo, N.; Ahadi, H.R. An integer programming model for analysing impacts of different train types on railway line capacity. Transport 2014, 29, 28–35. [Google Scholar] [CrossRef]
- Tuzar, A. Transport Theory; University of Pardubice: Pardubice, Czech Republic, 1996; 75p, ISBN 8071940399. [Google Scholar]
- Dedík, M. Methodology for Evaluating the potential of Railway Infrastructure for the Provision of Transport Services in Integrated Transport System. Ph.D. Thesis, University of Žilina, Žilina, Slovakia, 2020. [Google Scholar]
- Dorda, M. Part IV. Prognostic Methods in Transport. 2020. Available online: http://homel.vsb.cz/~dor028/Prognozy.pdf (accessed on 14 October 2020).
- Dedík, M.; Čechovič, T.; Gašparík, J.; Majerčák, J. Rationalization of the passenger transport as an important system. Transp. Res. Procedia 2019, 40, 193–200. [Google Scholar] [CrossRef]
- Hrabáček, J. Periodic Transport on Transport Networks and their Optimization. Ph.D. Thesis, University of Pardubice Pardubice, Pardubice, Czech Republic, 2010. [Google Scholar]
- Gašparík, J.; Dedík, M.; Čechovič, L. Estimation of Transport Potential in Regional Rail Passenger Transport by Using the Innovative Mathematical-Statistical Gravity Approach. Sustainability 2020, 12, 3821. [Google Scholar] [CrossRef]
- Gašparík, J.; Zitrický, V. A new approach to estimating the occupation time of the railway infrastructure. Transport 2011, 25, 387–393. [Google Scholar] [CrossRef][Green Version]
- Bulíček, J.; Drdla, P.; Matuška, J. Operational Reliability of a Periodic Railway Line. Transp. Res. Procedia 2021, 53, 106–113. [Google Scholar] [CrossRef]
- Kontaxi, E.; Ricci, S. Techniques and Methodologies for Railway Capacity Analysis: Comparative Studies and Integration Perspectives. In Proceedings of the 3rd International Seminar on Railway Operations Modelling and Analysis, Zurich, Switzerland, 11–13 February 2009. [Google Scholar]
- Nachtigall, P.; Široký, J.; Tischer, E. Assessing the efficiency of increasing the track speed in the line section Rokycany–Plzeň hl. n. Sustainability 2020, 12, 7415. [Google Scholar] [CrossRef]
- Mankowski, C.; Weiland, D.; Abramovic, B. Impact of Railway Investments on Regional Development—Case Study of Pomeranian Metropolitan Railway. Promet-Traffic Transp. 2019, 31, 669–679. [Google Scholar] [CrossRef]
- Černá, A.; Černý, J. Managerial Decision-Making on Transport Systems; University of Pardubice: Pardubice, Czech Republic, 2014; 226p, ISBN 9788073958497. [Google Scholar]





| Indicators Comparison | Current Status | Proposal | Differences |
|---|---|---|---|
| Average travel time (hours) | 5.47 | 4.96 | −0.51 |
| Average travel speed (km/h) | 80.99 | 89.31 | +8.32 |
| Number of connections [-] | 22 | 34 | +12 |
| Average interval between connections (hours) | 1.69 | 1.14 | −0.55 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dedík, M.; Zitrický, V.; Valla, M.; Gašparík, J.; Figlus, T. Optimization of Timetables on the Bratislava–Žilina–Košice Route in the Period after the End of the COVID-19 Pandemic. Sustainability 2022, 14, 5031. https://doi.org/10.3390/su14095031
Dedík M, Zitrický V, Valla M, Gašparík J, Figlus T. Optimization of Timetables on the Bratislava–Žilina–Košice Route in the Period after the End of the COVID-19 Pandemic. Sustainability. 2022; 14(9):5031. https://doi.org/10.3390/su14095031
Chicago/Turabian StyleDedík, Milan, Vladislav Zitrický, Michal Valla, Jozef Gašparík, and Tomasz Figlus. 2022. "Optimization of Timetables on the Bratislava–Žilina–Košice Route in the Period after the End of the COVID-19 Pandemic" Sustainability 14, no. 9: 5031. https://doi.org/10.3390/su14095031
APA StyleDedík, M., Zitrický, V., Valla, M., Gašparík, J., & Figlus, T. (2022). Optimization of Timetables on the Bratislava–Žilina–Košice Route in the Period after the End of the COVID-19 Pandemic. Sustainability, 14(9), 5031. https://doi.org/10.3390/su14095031






