Multi-Objective Energy Optimal Scheduling of Multiple Pulsed Loads in Isolated Power Systems
Abstract
1. Introduction
1.1. Motivation
1.2. Related Works
- (1)
- In different modes, the optimization objectives and constraints of multiple pulsed load energy optimization scheduling in IPS are quite different. When the conditions such as pulsed load running time, running times, available power and real-time control performance are changed, the corresponding energy optimization scheduling method needs to be given to maximize the effectiveness of multi-pulsed loads.
- (2)
- In the IPS operation, a variety of complicated working conditions need to be considered, especially in special scenarios such as accelerated catch-up and rapid evacuation, and the mobility and suppression capabilities of the system need to be considered at the same time. Only in this way can we improve the survivability of ships and achieve multi-objective optimization of systems in special scenarios.
1.3. Main Contribution
1.4. Paper Organization
2. Problem Formulation
2.1. Assumption
2.2. The Typical Operation Model and Senarios of IPS
- A.
- Normal Mode
- B.
- Emergency Mode
- C.
- Suppression Mode
2.3. The Chosen of Optimal Targets
2.4. Mathematical Model
3. Solution Method
3.1. Algorithm for General Situation
3.2. Particular Solution Considering Typical Engineering Scenarios
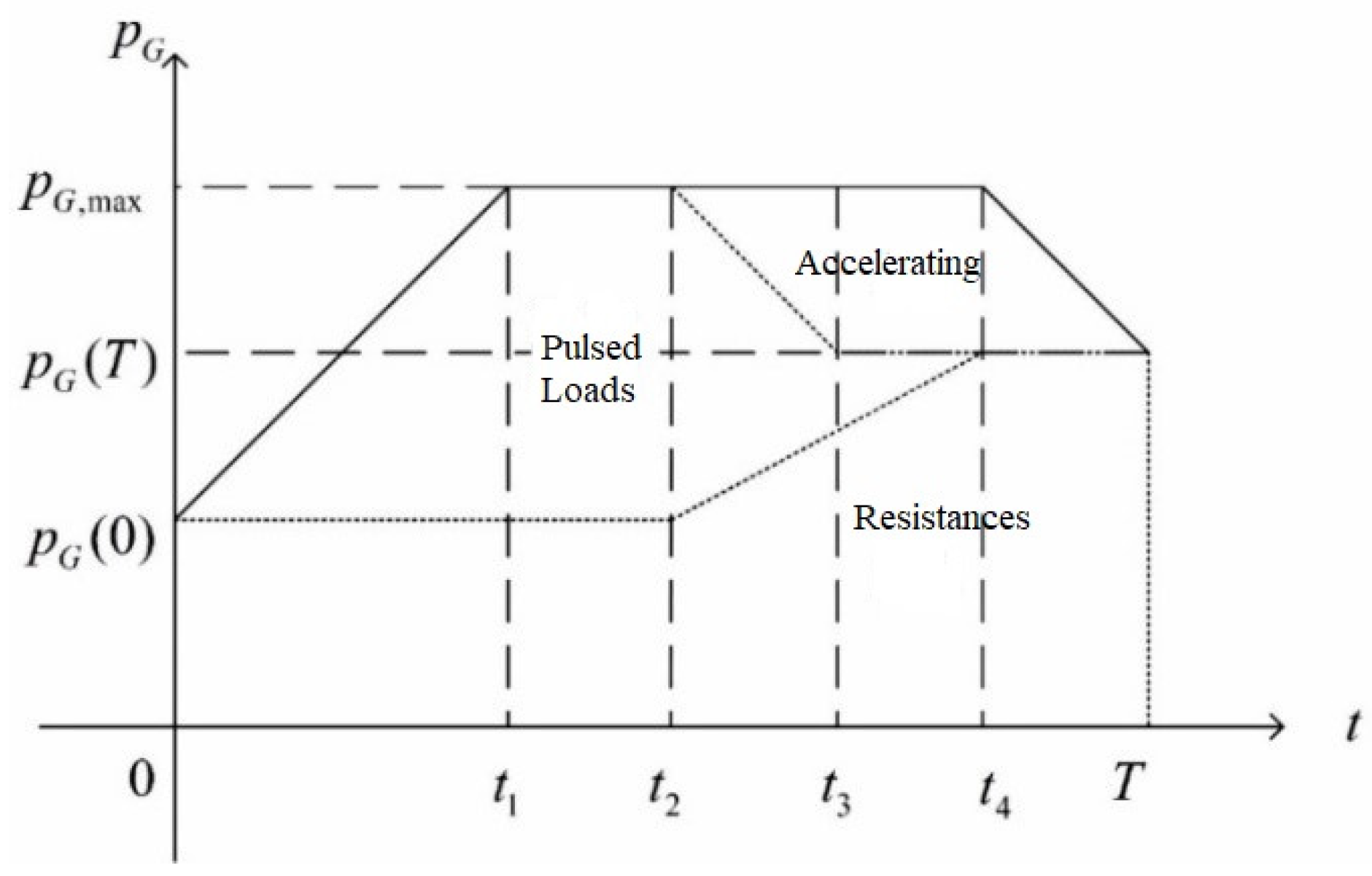
3.2.1. Accelerating the Catch-Up Situation
3.2.2. Accelerating the Evacuation Situation
4. Case Study
4.1. Case Settings
4.2. Results and Analyses
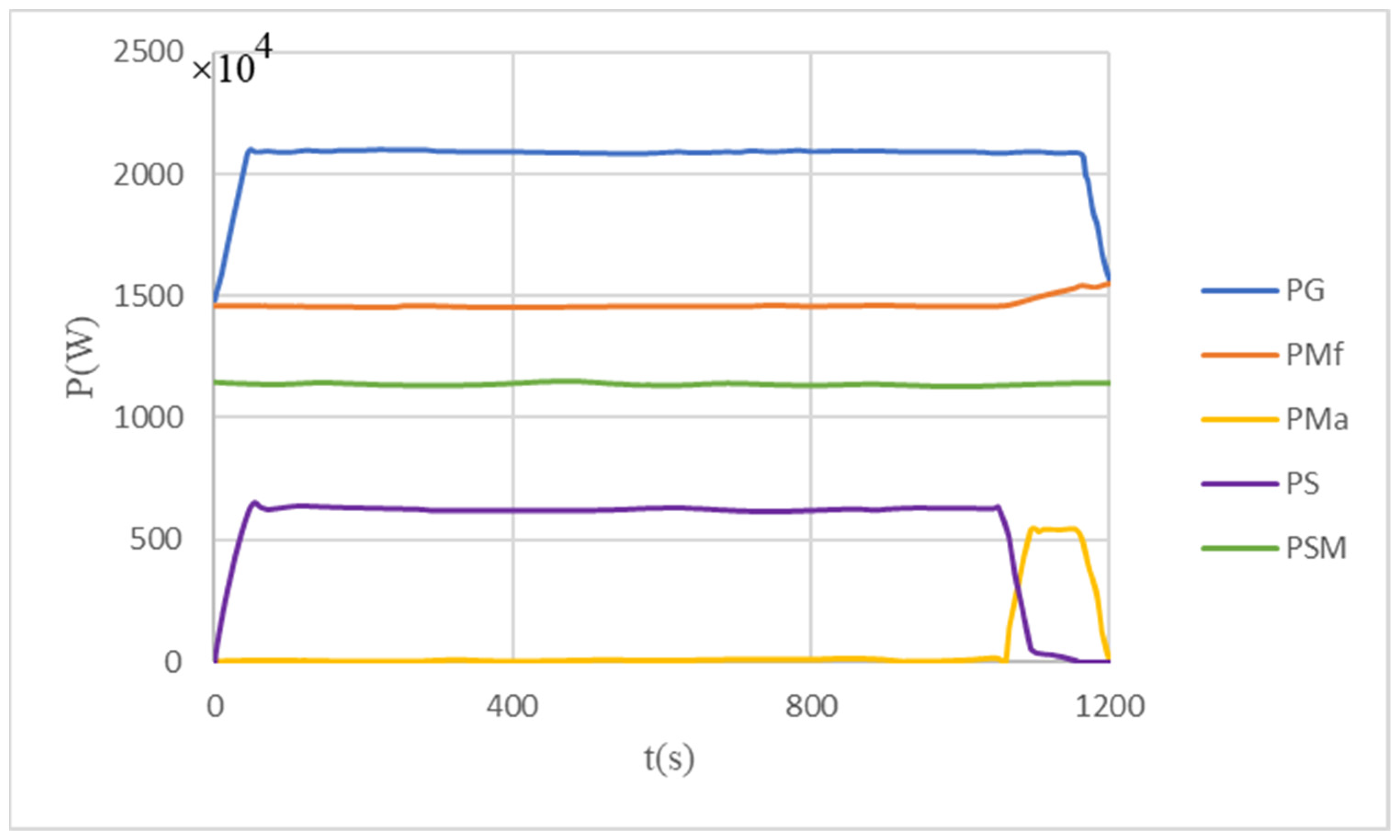
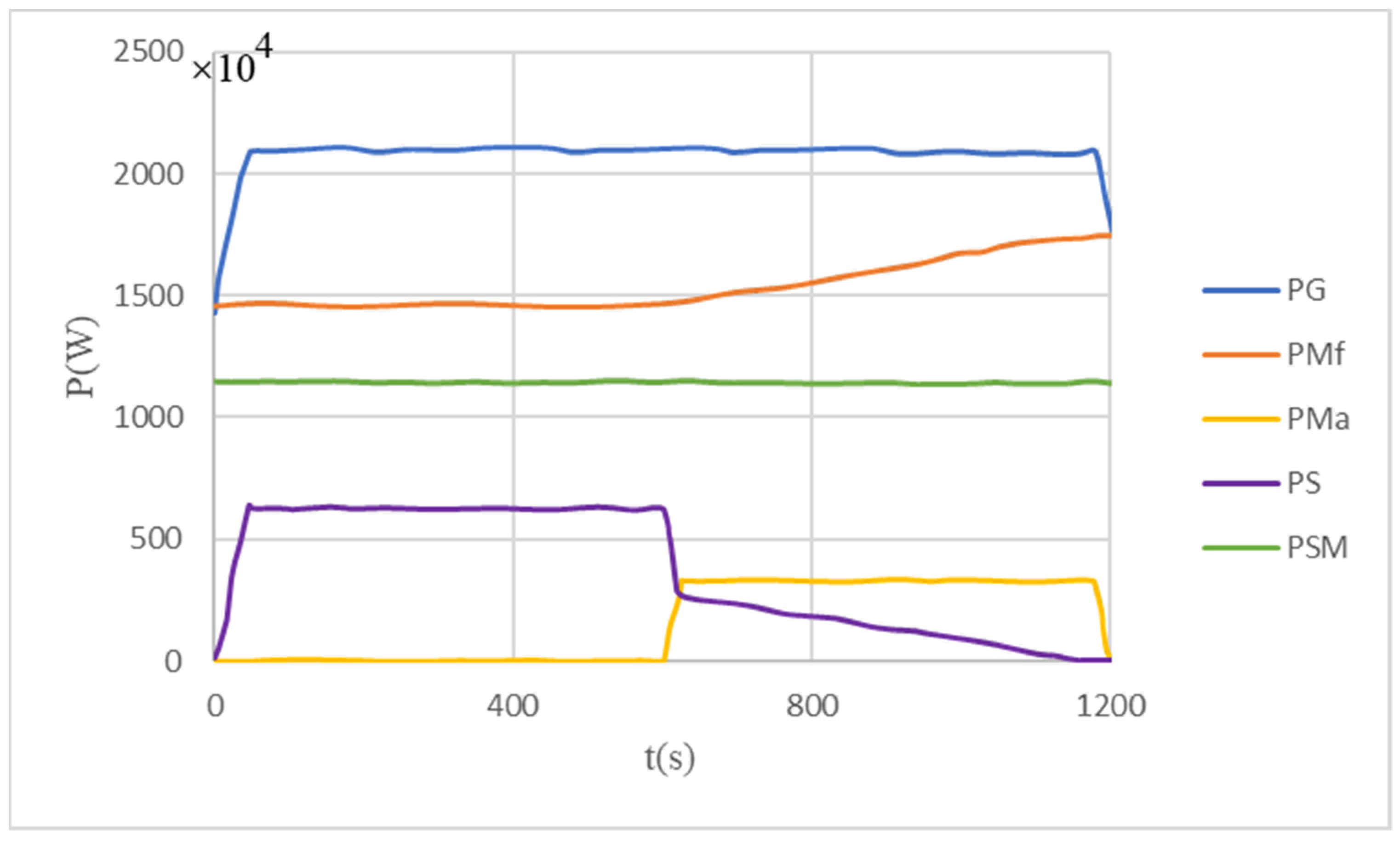
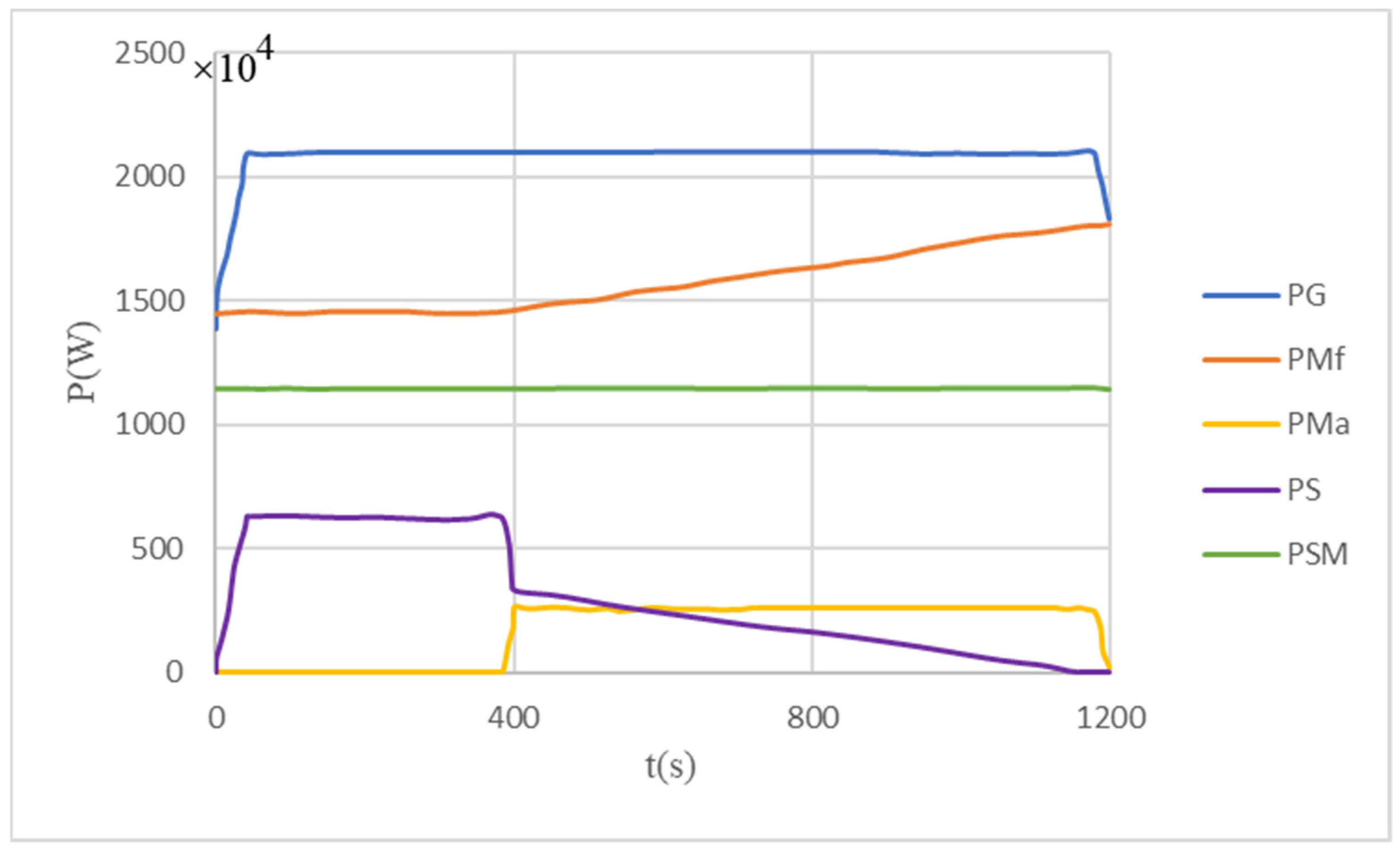
4.2.1. General Situation
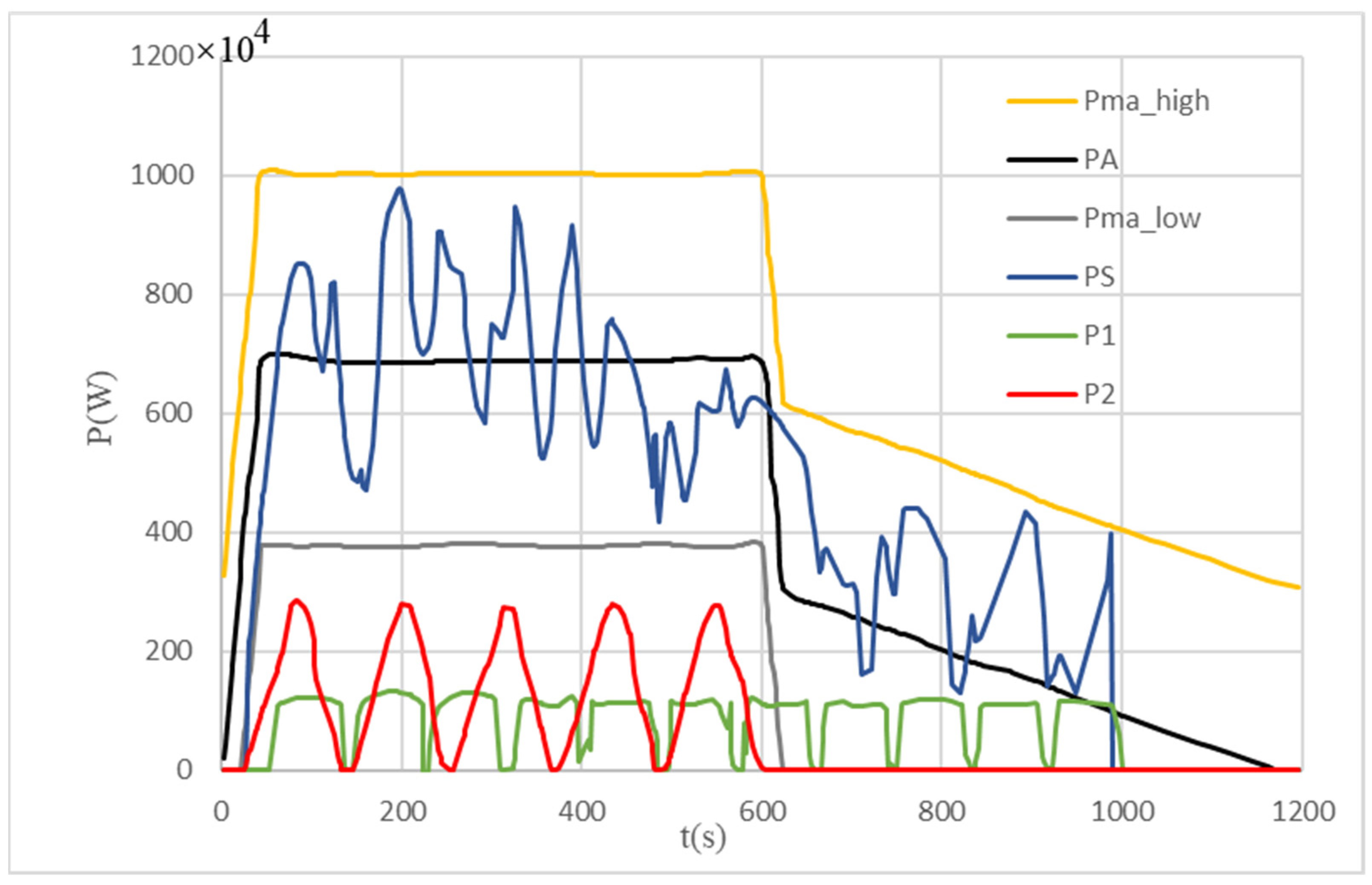
4.2.2. Typical Engineering Scenarios
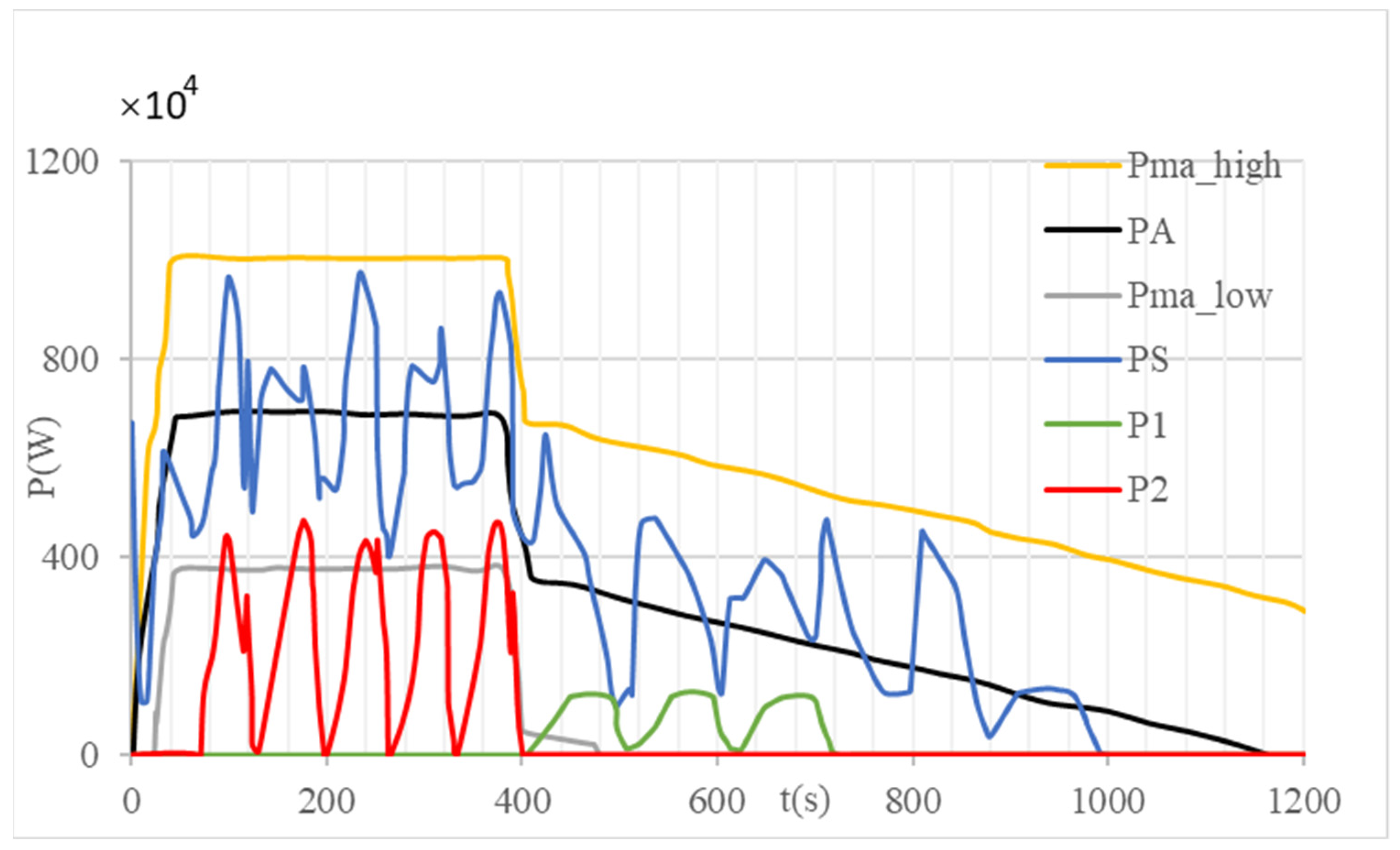
Accelerating the Catch-Up Situation
Accelerating the Evacuation Situation
5. Conclusions and Prospects
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B

References
- Dashkovskiy, S.N.; Rüffer, B.S. Local ISS of large-scale interconnections and estimates for stability regions. Syst. Control. Lett. 2010, 59, 241–247. [Google Scholar] [CrossRef]
- Ibrahim, M.; Jemei, S.; Wimmer, G.; Hissel, D. Nonlinear autoregressive neural network in an energy management strategy for battery/ultra-capacitor hybrid electrical vehicles. Electr. Power Syst. Res. 2016, 136, 262–269. [Google Scholar] [CrossRef]
- Qin, B.; Wang, W.; Li, W.; Li, F.; Ding, T. Multiobjective Energy Management of Multiple Pulsed Loads in Shipboard Integrated Power Systems. IEEE Syst. J. 2022, 1–12, (Early Access Article). [Google Scholar] [CrossRef]
- Tan, L.; Yang, Q.; Im, W.; Liu, W. Adaptive critic design based cooperative control for pulsed power loads accommodation in shipboard power system. IET Gener. Transm. Distrib. 2016, 10, 2739–2747. [Google Scholar] [CrossRef]
- Im, W.S.; Wang, C.; Tan, L.; Liu, W.; Liu, L. Cooperative controls for pulsed power load accommodation in a shipboard power system. IEEE Trans. Power Syst. 2016, 31, 5180–5189. [Google Scholar] [CrossRef]
- Qin, B.; Liu, W.; Li, H.; Ding, T.; Ma, K.; Liu, T. Impact of System Inherent Characteristics on Initial-Stage Short-Circuit Current of MMC-based MTDC Transmission systems. IEEE Trans. Power Syst. 2022, 37, 3913–3922. [Google Scholar] [CrossRef]
- Mohamed, A.; Salehi, V.; Mohammed, O. Real-time energy management algorithm for mitigation of pulse loads in hybrid IPSs. IEEE Trans. Smart Grid 2012, 3, 1911–1922. [Google Scholar] [CrossRef]
- Vafamand, N.; Mardani, M.M.; Khooban, M.H.; Blaabjerg, F.; Boudjadar, J. Pulsed power load effect mitigation in DC shipboard microgrids: A constrained model-predictive approach. IET Power Electron. 2019, 12, 2155–2160. [Google Scholar] [CrossRef]
- Penthia, T.; Panda, A.K.; Patnaik, N.; Mohanty, P.R. Performance of SMES system with non-linear dynamic evolution control approach for pulsed power load compensation. IET Gener. Transmiss. Distrib. 2020, 14, 1872–1881. [Google Scholar] [CrossRef]
- Weiming, M.A. On comprehensive development of electrization and informationization in naval ships. J. Nav. Univ. Eng. 2010, 22, 1–4. [Google Scholar]
- Hein, K.; Xu, Y.; Wilson, G.; Gupta, A.K. Coordinated optimal voyage planning and energy management of all-electric ship with hybrid energy storage system. IEEE Trans. Power Syst 2021, 36, 2355–2365. [Google Scholar] [CrossRef]
- Li, H.; Qin, B.; Jiang, Y.; Zhao, Y.; Shi, W. Data-Driven Optimal Scheduling for Underground Space Based Integrated Hydrogen Energy System. IET Renew. Power Gener. 2022, 16, 2521–2531. [Google Scholar] [CrossRef]
- Feng, X.; Butler-Purry, K.L.; Zourntos, T. A multi-agent system framework for real-time electric load management in MVAC all-electric ship power systems. IEEE Trans. Power Syst. 2015, 30, 1327–1336. [Google Scholar] [CrossRef]
- Elsayed, A.T.; Mohammed, O.A. Distributed flywheel energy storage systems for mitigating the effects of pulsed loads. In Proceedings of the 2014 IEEE PES General Meeting Conference & Exposition, National Harbor, MD, USA, 27–31 July 2014; pp. 1–5. [Google Scholar]
- Li, F.; Chen, Y.; Xie, R.; Shen, C.; Zhang, L.; Qin, B. Optimal Operation Planning for Orchestrating Multiple pulsed loads with Transient Stability Constraints in Isolated Power Systems. IEEE Access 2018, 6, 18685–18693. [Google Scholar] [CrossRef]
- Salehi, V.; Mirafzal, B.; Mohammed, O. Pulse-load effects on ship power system stability. In Proceedings of the IECON 2010-36th Annual Conference on IEEE Industrial Electronics Society, Glendale, CA, USA, 7–10 November 2010; pp. 3353–3358. [Google Scholar]
- Lashway, C.R.; Elsayed, A.T.; Mohammed, O.A. Hybrid energy storage management in ship power systems with multiple pulsed loads. Electr. Power Syst. Res. 2016, 141, 50–62. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, Q.; Gong, W.; Gryning, M.S. H∞ robust current control for DFIG-Based wind turbine subject to grid voltage dis-tortions. IEEE Trans. Sustain. Energy 2017, 8, 816–825. [Google Scholar] [CrossRef]
- Qin, B.; Sun, H.; Ma, J.; Li, W.; Ding, T.; Wang, Z.; Zomaya, A. Robust H∞ control of doubly fed wind generator via state-dependent riccati equation technique. IEEE Trans. Power Syst. 2019, 34, 2390–2400. [Google Scholar] [CrossRef]
- Shahzad, M.; Akram, W.; Arif, M.; Khan, U.; Ullah, B. Optimal siting and sizing of distributed generators by strawberry plant propagation algorithm. Energies 2021, 14, 1744. [Google Scholar] [CrossRef]
- Shahzad, M.; Shafiullah, Q.; Akram, W.; Arif, M.; Ullah, B. Reactive power support in radial distribution network using mine blast algorithm. Elektron. Ir Elektrotechnika 2021, 27, 33–40. [Google Scholar] [CrossRef]
- Shahzad, M.; Qadir, A.; Ullah, N.; Mahmood, Z.; Saad, N.M.; Ali, S.S.A. Optimization of On-Grid Hybrid Renewable Energy System: A Case Study on Azad Jammu and Kashmir. Sustainability 2022, 14, 5757. [Google Scholar] [CrossRef]
- Bilal, M.; Shahzad, M.; Arif, M.; Ullah, B.; Hisham, S.B.; Ali, S.S.A. Annual Cost and Loss Minimization in a Radial Distribution Network by Capacitor Allocation Using PSO. Appl. Sci. 2021, 11, 11840. [Google Scholar] [CrossRef]
- Scarabaggio, P.; Carli, R.; Dotoli, M. Noncooperative Equilibrium Seeking in Distributed Energy Systems Under AC Power Flow Nonlinear Constraints. IEEE Trans. Control. Netw. Syst. 2022, 1–12. [Google Scholar] [CrossRef]
- Yao, M.; Molzahn, D.K.; Mathieu, J.L. An optimal power-flow approach to improve power system voltage stability using demand response, IEEE Trans. Control. Netw. Syst. 2019, 6, 1015–1025. [Google Scholar] [CrossRef]
- Scarabaggio, P.; Carli, R.; Cavone, G.; Dotoli, M. Smart Control Strategies for Primary Frequency Regulation through Electric Vehicles: A Battery Degradation Perspective. Energies 2020, 13, 4586. [Google Scholar] [CrossRef]
- Yan, G.; Liu, D.; Li, J. A cost accounting method of the Li-ion battery energy storage system for frequency regulation considering the effect of life degradation. Prot. Control. Mod. Power Syst. 2018, 3, 4. [Google Scholar] [CrossRef]



| Mode | Working Condition | Target | Variables and Means |
|---|---|---|---|
| Normal Mode | Berthing on shore | Economy | Long time scale: Load forecasting, Unit commitment |
| Accelerating | |||
| Decelerating | Middle time scale: Optimization of generator operating point | ||
| Uniform Velocity | |||
| Berthing at sea | Short time scale: Automatic generation control | ||
| Emergency Mode | Emergency transfer | Safety, self-healing, Maneuverability | Regulating power distribution |
| Comloss | Suppressive ability | Charging capacity | |
| Suppression Mode | Attack | Maneuverability | Charging and discharging power regulation |
| Defense | Suppressive ability | Power distribution |
| No. | (MJ) | (s) | No. | (MJ) | (s) | ||
|---|---|---|---|---|---|---|---|
| 1 | 80 | 5 | 10 | 5 | 145 | 8 | 16 |
| 2 | 160 | 7 | 25 | 6 | 130 | 9 | 20 |
| 3 | 120 | 10 | 18 | 7 | 90 | 6.5 | 8 |
| 4 | 105 | 6 | 13 | 8 | 110 | 11 | 19 |
m/s | t | m/s | MW/(m/s)3 | s | MW | s/m |
|---|---|---|---|---|---|---|
| 8 | 500,000 | 10 | 0.03 | 1200 | 3 | 10 |
MW/s | MW | MW/s | MW/s | MW/s | m/s | m/s |
|---|---|---|---|---|---|---|
| 0.4 | 22 | 0.15 | 0.4 | 0.15 | 8.06 | 8.67 |
m/s | T | m/s | MW/(m/s)3 | S | MW |
|---|---|---|---|---|---|
| 8 | 500,000 | 10 | 0.03 | 1200 | 3 |
MW/s | MW | MW/s | MW/s | MW/s |
|---|---|---|---|---|
| 0.4 | 22 | 0.15 | 0.4 | 0.15 |
m/s | t | MW/(m/s)3 | s | MW | |
|---|---|---|---|---|---|
| 8 | 500,000 | 1800 | 0.03 | 1200 | 3 |
MW/s | MW | MW/s | MW/s | MW/s |
|---|---|---|---|---|
| 0.4 | 22 | 0.15 | 0.4 | 0.15 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, F.; Liu, D.; Qin, B.; Sun, K.; Wang, D.; Liang, H.; Zhang, C.; Tao, T. Multi-Objective Energy Optimal Scheduling of Multiple Pulsed Loads in Isolated Power Systems. Sustainability 2022, 14, 16021. https://doi.org/10.3390/su142316021
Li F, Liu D, Qin B, Sun K, Wang D, Liang H, Zhang C, Tao T. Multi-Objective Energy Optimal Scheduling of Multiple Pulsed Loads in Isolated Power Systems. Sustainability. 2022; 14(23):16021. https://doi.org/10.3390/su142316021
Chicago/Turabian StyleLi, Fan, Dong Liu, Boyu Qin, Ke Sun, Dan Wang, Hanqing Liang, Cheng Zhang, and Taikun Tao. 2022. "Multi-Objective Energy Optimal Scheduling of Multiple Pulsed Loads in Isolated Power Systems" Sustainability 14, no. 23: 16021. https://doi.org/10.3390/su142316021
APA StyleLi, F., Liu, D., Qin, B., Sun, K., Wang, D., Liang, H., Zhang, C., & Tao, T. (2022). Multi-Objective Energy Optimal Scheduling of Multiple Pulsed Loads in Isolated Power Systems. Sustainability, 14(23), 16021. https://doi.org/10.3390/su142316021





