Accounting for Expansive Soil Movement in Geotechnical Design—A State-of-the-Art Review
Abstract
1. Introduction
2. Expansive Soil Behaviour
3. Problems with Expansive Soils
4. Investigations of Expansive Soil Behaviour
4.1. Laboratory Investigations
4.2. Field Investigations
4.3. Numerical Investigations
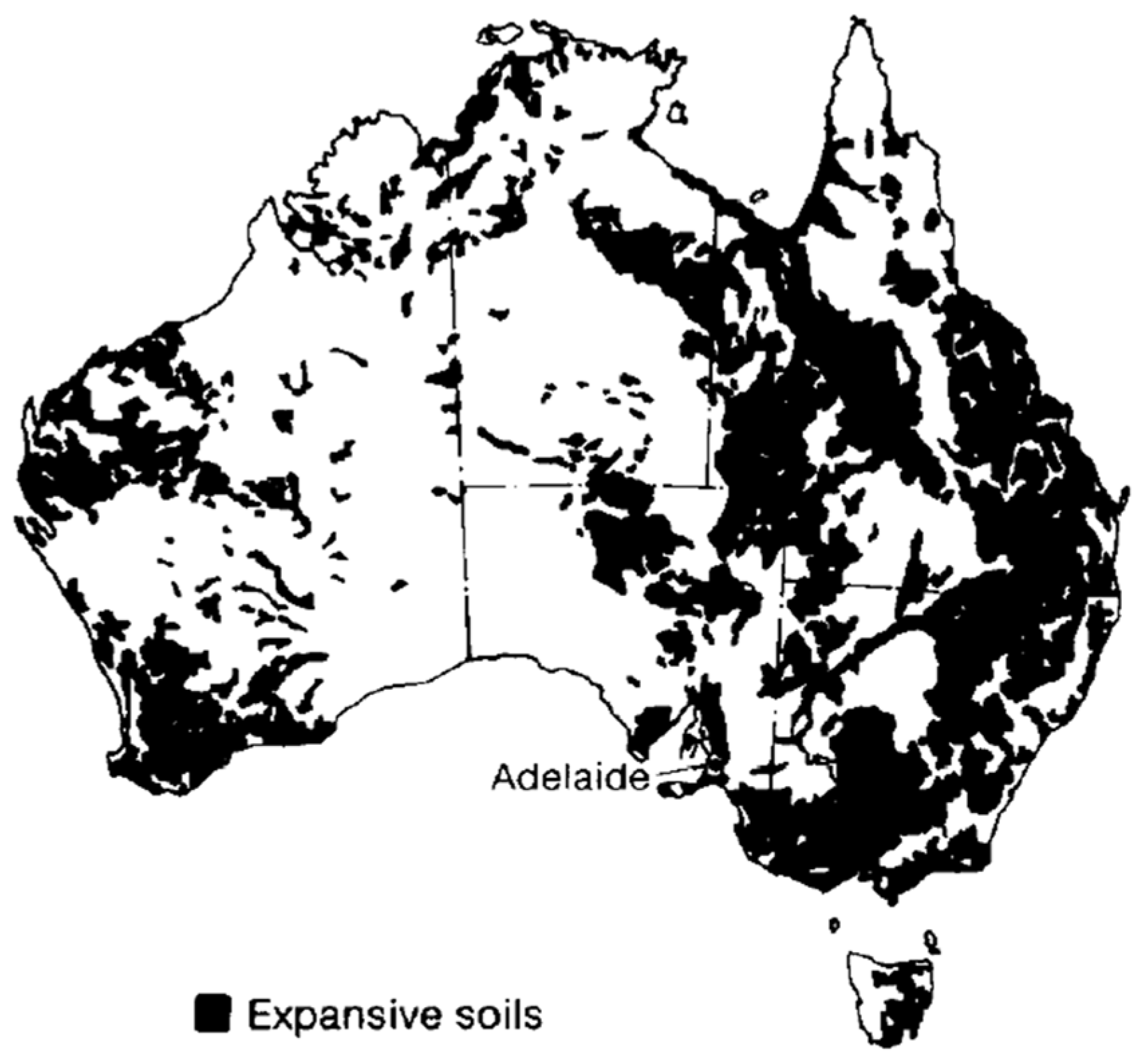
5. Australian Design Practice for Residential Footings on Expansive Soils
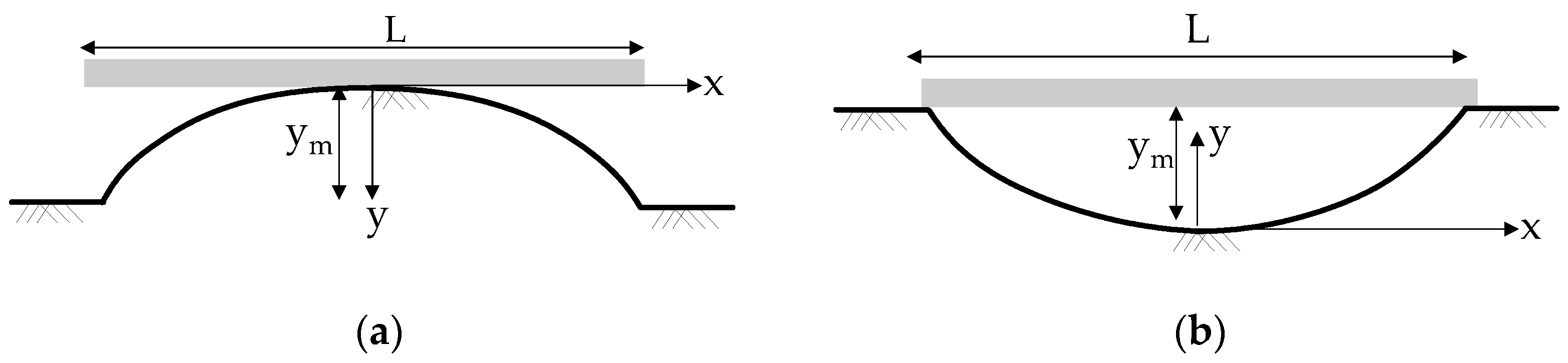
5.1. Characteristic Surface Movement Prediction
5.1.1. Instability Index
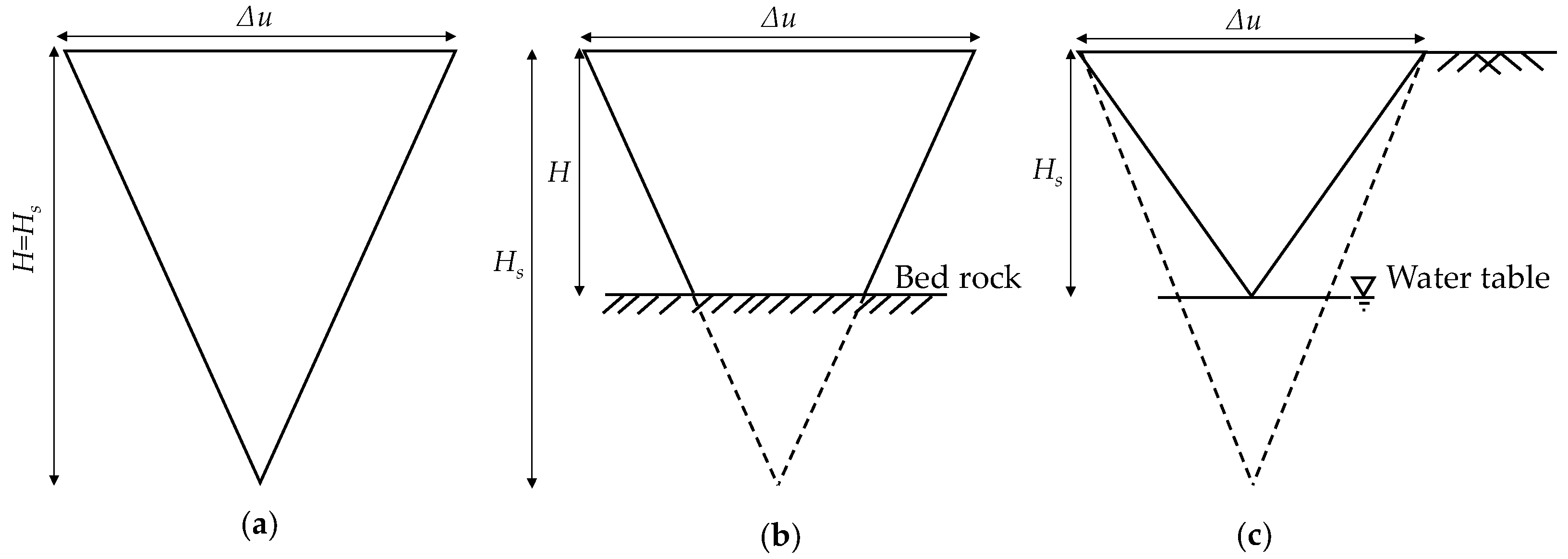
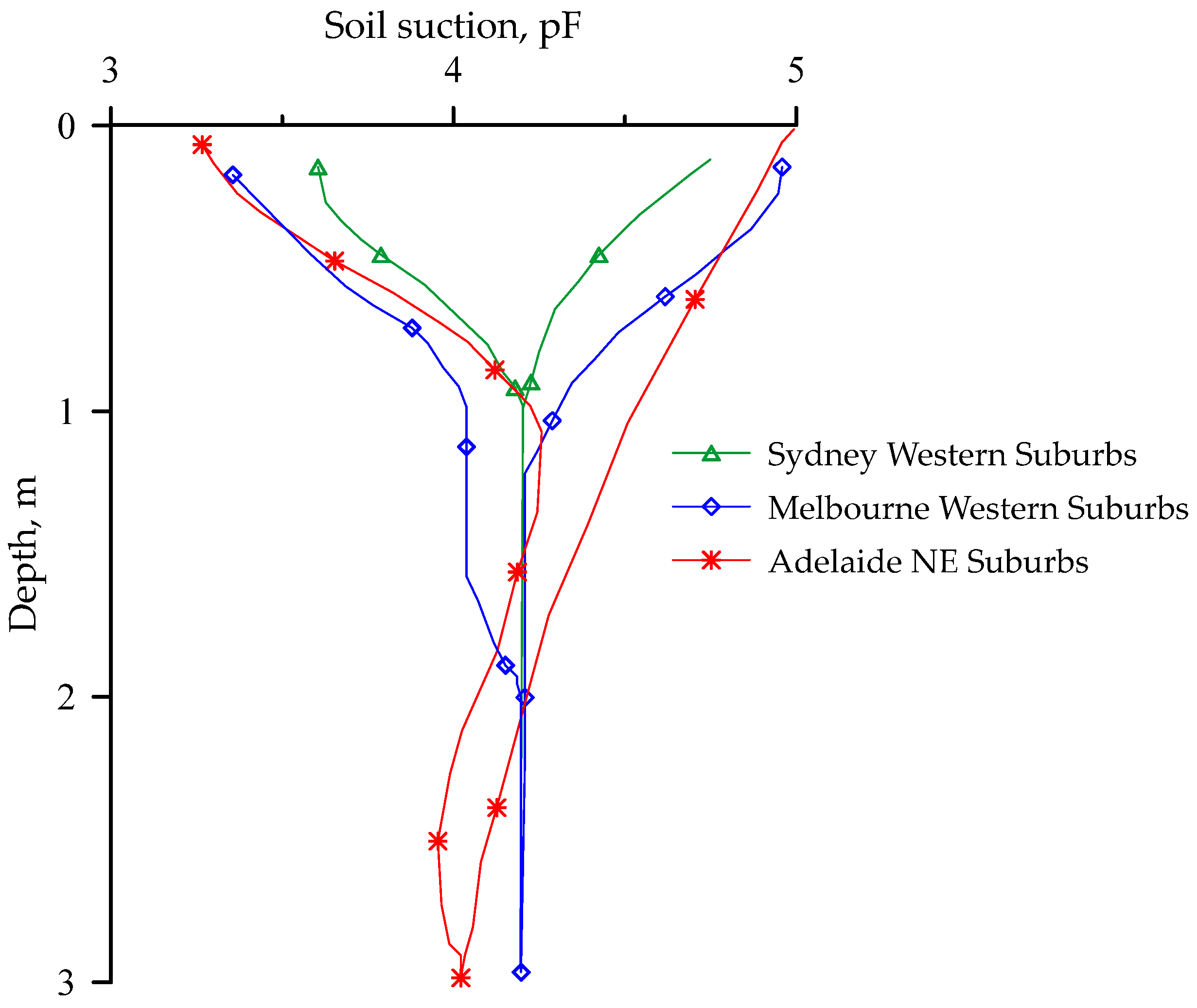
5.1.2. Depth of the Design Soil Suction Change (Hs)
5.1.3. Soil Suction Change at the Ground Surface (Δu)
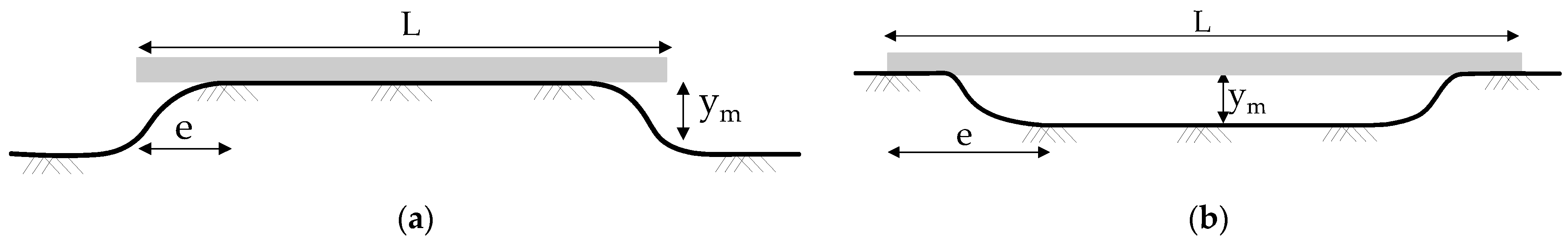
5.1.4. Mound Shape and Soil’s Stiffness
5.2. Effect of Climate on Hs and Correlation with TMI
5.3. Comparison with American Practice on Expressing Expansion Potential
5.4. Summary
6. Conclusions
- The behaviour of expansive soil can be complex. The expansive soil movement can be influenced by several factors, including its mineralogy and hydraulic and atmospheric boundary condition.
- Many different types of structures can be affected. Some of the specific examples found in the literature are lightweight residential buildings, road pavements, underground pipelines, slopes, and other infrastructures.
- It is expected that, due to climate change, the problems due to expansive soils will worsen in many parts of the world and should be given proper consideration for building climate-resilient structures.
- The effect of climate change may not be uniform across a state or other jurisdiction, and the local condition needs to be taken into account (calculation of local TMI and other variables may be needed).
- Laboratory tests commonly used for the quantification of reactivity are simplified and can be time-consuming. The number of field studies is also limited due to the expenses and effort involved.
- Current practice is based on a simplification of a complex process and caution should be exercised when estimating the soil reactivity as a function of conventional soil parameters such as the liquid limit or plasticity index.
- Numerical tools have been used to capture the behaviour of expansive soil and its interactions with different types of structures and can be a useful tool for a better understanding of the process or site-specific designs. They can be categorised into three groups, i.e., (1) modelling of the seepage due to interaction with the atmospheric boundary; (2) modelling of volume change and related ground movement, and (3) modelling of the interaction between the soil and structure constructed on or within the shallow depth of it.
- Advanced numerical methods for unsaturated seepage will require inputs of the SWCC and unsaturated hydraulic conductivity function and can be a complex process.
- Several past studies have attempted modelling of the interaction between soil–atmospheric boundaries and structures. However, several assumptions have been made, including some on the calculation of effective stress, which can have important consequences for the outputs of the numerical model.
- This review recommends further studies to incorporate the effects of the future climate, as geo-structures are designed to achieve a 50- to 100-year service life.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Appendix A
| SWCC | Hydraulic Conductivity Function | Thermal Properties | Climate Data | Vegetation Influence | Boundary Condition | Reference |
|---|---|---|---|---|---|---|
| Developed through the measured soil suction with corresponding moisture content in Vadose/w software | The equation proposed by Fredlund et al. [67] was used to develop this function in the Vadose/w model. Ksat was measured. | The measured thermal conductivity, including the specific heat capacity variation with the moisture content, was taken from another site near the study area [126]. | Weather data collected from the nearest weather station were assigned in the Vadose/w model. | Considered in Vadose/w through LAI, RD, and PML factors. |
| [93] |
| Obtained from Li et al. [94] | Kunsat was formulated based on Forchheimer [127]. Ksat = 1 × 10−7 to 1 × 10−9 (m/s). | - | - | - | A soil column of height:width:length = 11:11:11 m was considered to avoid the impact of boundary conditions. | [85] |
| Suction Change | Hs(m) | Strain | Reference |
|---|---|---|---|
| SWCC from Vadose/w model captures the suction profile | 0.6 to 3 | , α = 0.28 [128] | [93] |
| 1.2 pF | 1.3 to 4 | [85] |
| Stress Equation | Bulk Modulus | Elastic or Shear Modulus | Poisson’s Ratio | Boundary Condition | Reference | |
|---|---|---|---|---|---|---|
[128] | Second-order polynomial equation in the FLAC3D model was developed from lab tests of the soil to generate the soil stiffness (E) and gravimetric moisture relationship. | νsoil = 0.45 | Same as Table A1. | [93] | ||
| Equation (25) Equation (26) | Log bulk modulus, κ, commonly adopted values were 0.01–0.06. | E = tensor of soil elastic constants | νsoil = 0.1 to 0.4 |
| Mechanical behaviour of soil | [85] |
| Elastic modulus of concrete (Ec) = 20–25 GPa | νconcrete = 0.2 | Slab-on-grade model | ||||
References
- Hasan, S.; Patowary, M.Z.; Sazzad, M. Comparison of the behaviour of expansive soil using different admixtures. In Proceedings of the Second International Conference on Research and Innovation in Civil Engineering, Chattogram, Bangladesh, 10–11 January 2020. [Google Scholar]
- Koukouzas, N.; Tyrologou, P.; Koutsovitis, P.; Karapanos, D.; Karkalis, C. Soil stabilization. In Handbook of Fly Ash; Elsevier Inc.: Amsterdam, The Netherlands, 2022; pp. 475–500. [Google Scholar]
- Nelson, J.; Miller, D.J. Expansive Soils: Problems and Practice in Foundation and Pavement Engineering; John Wiley & Sons: Hoboken, NJ, USA, 1992. [Google Scholar]
- Jones, L.D.; Ian, J. Chapter C5—Expansive soils. In Institution of Civil Engineers Manuals Series; Institution of Civil Engineers: London, UK, 2012. [Google Scholar]
- Rosenbalm, D.; Zapata, C.E. Effect of wetting and drying cycles on the behavior of compacted expansive soils. J. Mater. Civ. Eng. 2017, 29, 04016191. [Google Scholar] [CrossRef]
- Considine, M.L. Soils shrink, trees drink, and houses crack. ECOS 1984, 41, 13–15. [Google Scholar]
- Mills, A.; Love, P.E.; Williams, P. Defect Costs in Residential Construction. J. Constr. Eng. Manag. 2009, 135, 12–16. [Google Scholar] [CrossRef]
- Carey, A. Owners Find Homes Are Cracking Under Pressure. Domain. 2011. Available online: https://www.domain.com.au/news/owners-find-homes-are-cracking-under-pressure-20111221-1p5or/ (accessed on 2 July 2022).
- Johanson, S. Thousands of Suburban Home Owners Facing Financial Ruin. Age. 2014. Available online: https://www.theage.com.au/national/victoria/thousands-of-suburban-home-owners-facing-financial-ruin-20140607-39q4z.html (accessed on 2 July 2022).
- Li, J.; Sun, X. Evaluation of changes of the Thornthwaite moisture index in Victoria. Aust. Geomech. J. 2015, 50, 39–49. [Google Scholar]
- ACCSP. Australia’s Changing Climate. Available online: https://www.climatechangeinaustralia.gov.au/media/ccia/2.2/cms_page_media/176/AUSTRALIAS_CHANGING_CLIMATE_1.pdf (accessed on 11 March 2022).
- Karim, M.R.; Hughes, D.; Kelly, R.; Lynch, K. A rational approach for modelling the meteorologically induced pore water pressure in infrastructure slopes. Eur. J. Environ. Civ. Eng. 2020, 24, 2361–2382. [Google Scholar] [CrossRef]
- Karim, M.R.; Hughes, D.; Rahman, M.M. Estimating hydraulic conductivity assisted with numerical analysis for unsaturated soil—A case study. Geotech. Eng. 2021, 52, 12–19. [Google Scholar]
- Karim, M.R.; Rahman, M.M.; Nguyen, H.B.K.; Newsome, P.L.; Cameron, D. TMI soil moisture index for South Australia under current and future climate scenarios. In Proceedings of the 20th International Conference on Soil Mechanics and Geotechnical Engineering, A Geotechnical Discovery Down Under, Sydney, Australia, 1–5 May 2022; Rahman, M.M., Jaksa, M., Eds.; Australian Geomechanics Society: Sydney, Australia, 2022; pp. 2233–2236. [Google Scholar]
- Karim, M.R.; Hughes, D.; Rahman, M.M. Unsaturated Hydraulic Conductivity Estimation—A Case Study Modelling the Soil-Atmospheric Boundary Interaction. Processes 2022, 10, 1306. [Google Scholar] [CrossRef]
- Karim, M.R.; Rahman, M.M.; Nguyen, K.; Cameron, D.; Iqbal, A.; Ahenkorah, I. Changes in Thornthwaite Moisture Index and Reactive Soil Movements under Current and Future Climate Scenarios-A Case Study. Energies 2021, 14, 6760. [Google Scholar] [CrossRef]
- Smethurst, J.; Clarke, D.; Powrie, W. Factors controlling the seasonal variation in soil water content and pore water pressures within a lightly vegetated clay slope. Géotechnique 2012, 62, 429–446. [Google Scholar] [CrossRef]
- Vardon, P.J. Climatic influence on geotechnical infrastructure: A review. Environ. Geotech. 2015, 2, 166–174. [Google Scholar] [CrossRef]
- Alonso, E.; Gens, A.; Delahaye, C. Influence of rainfall on the deformation and stability of a slope in overconsolidated clays: A case study. Hydrogeol. J. 2003, 11, 174–192. [Google Scholar] [CrossRef]
- Karim, R.; Rahman, M.; Hughes, D. Estimation of soil hydraulic conductivity assisted by numerical tools: Two case studies. In Proceedings of the 5th International Conference on Geotechnical and Geophysical Site Characterisation, Gold Coast, Australia, 5–9 September 2016; pp. 491–496. [Google Scholar]
- Lynch, K.; Hughes, D.; Karim, R.; Harley, R.; Bell, A.; Mckinley, J.; Donohue, S.; Bergamo, P. Evolving techniques for characterising and monitoring the stability of infrastructure slopes Le développement de techniques pour la caractérisation et la surveillance de la stabilité des talus. In Proceedings of the 16th European conference on Soil Mechanics and Geotechnical Engineering, Edinburgh, UK, 13–17 September 2015; pp. 1615–1620. [Google Scholar]
- Hughes, D.; Karim, M.R.; Briggs, K.; Glendinning, S.; Toll, D.; Dijkstra, T.; Powrie, W.; Dixon, N. A comparison of numerical modelling techniques to predict the effect of climate on infrastructure slopes. In Proceedings of the Geotechnical Engineering for Infrastructure and Development—XVI European Conference on Soil Mechanics and Geotechnical Engineering, ECSMGE 2015, Edinburgh, UK, 13–17 September 2015; pp. 3663–3668. [Google Scholar]
- Glendinning, S.; Helm, P.R.; Rouainia, M.; Stirling, R.A.; Asquith, J.D.; Hughes, P.N.; Toll, D.G.; Clarke, D.; Powrie, W.; Smethurst, J.; et al. Research-informed design, management and maintenance of infrastructure slopes: Development of a multi-scalar approach. IOP Conf. Ser. Earth Environ. Sci. 2015, 26, 012005. [Google Scholar] [CrossRef]
- Harley, R.; Sivakumar, V.; Hughes, D.; Karim, M.R.; Barbour, S.L. Progressive deformation of glacial till due to viscoplastic straining and pore pressure variation. In Proceedings of the 67th Canadian Geotechnical Conference, Regina, SK, Canada, 28 September–1 October 2014. [Google Scholar]
- Smethurst, J.; Briggs, K.M.; Powrie, W.; Ridley, A.; Butcher, D.J.E. Mechanical and hydrological impacts of tree removal on a clay fill railway embankment. Geotechnique 2015, 65, 869–882. [Google Scholar] [CrossRef]
- Briggs, K.M.; Smethurst, J.A.; Powrie, W.; O’Brien, A.S.; Butcher, D.J.E. Managing the extent of tree removal from railway earthwork slopes. Ecol. Eng. 2013, 61, 690–696. [Google Scholar] [CrossRef]
- Briggs, K.M.; Smethurst, J.A.; Powrie, W.; O’brien, A.S. Wet winter pore pressures in railway embankments. Proc. Inst. Civ. Eng. 2012, 166, 451–465. [Google Scholar] [CrossRef]
- Smethurst, J.; Clarke, D.; Powrie, W. Seasonal changes in pore water pressure in a grass covered cut slope in London clay. Géotechnique 2006, 56, 523–537. [Google Scholar] [CrossRef]
- Rouainia, M.; Davies, O.; O’Brien, T. Numerical modelling of climate effects on slope stability. Proc. Inst. Civ. Eng. Eng. Sustain. 2009, 162, 81–89. [Google Scholar] [CrossRef]
- Karim, M.R.; Lo, S.-C.R. Estimation of the hydraulic conductivity of soils improved with vertical drains. Comput. Geotech. 2015, 63, 299–305. [Google Scholar] [CrossRef]
- Swarna, S.T.; Hossain, K.; Pandya, H.; Mehta, Y.A. Assessing climate change impact on asphalt binder grade selection and its implications. Transp. Res. Rec. 2021, 2675, 786–799. [Google Scholar] [CrossRef]
- Puppala, A.J.; Griffin, J.A.; Hoyos, L.R.; Chomtid, S. Studies on sulfate-resistant cement stabilization methods to address sulfate-induced soil heave. J. Geotech. Geoenvironmental Eng. 2004, 130, 391–402. [Google Scholar] [CrossRef]
- Wang, Y.-X.; Guo, P.-P.; Ren, W.-X.; Yuan, B.-X.; Yuan, H.-P.; Zhao, Y.-L.; Shan, S.-B.; Cao, P. Laboratory investigation on strength characteristics of expansive soil treated with jute fiber reinforcement. Int. J. Geomech. 2017, 17, 04017101. [Google Scholar] [CrossRef]
- Aniculăesi, M.; Stanciu, A.; Lungu, I. Swell-shrink behavior of expansive soils stabilized with eco-cement. In Proceedings of the Geotechnical and Geophysical Site Characterization: 4th International Conference on Site Characterization ISC-4, Pernambuco, Brazil, 18–21 September 2012; pp. 1677–1682. [Google Scholar]
- Chittoori, B.C.; Pathak, A.; Burbank, M.; Islam, M.T. Application of bio-stimulated calcite precipitation to stabilize expansive soils: Field trials. In Proceedings of the Geo-Congress 2020: Biogeotechnics, Minneapolis, MN, USA, 25–28 February 2020; pp. 111–120. [Google Scholar]
- Thyagaraj, T.; Rao, S.M.; Sai Suresh, P.; Salini, U. Laboratory studies on stabilization of an expansive soil by lime precipitation technique. J. Mater. Civ. Eng. 2012, 24, 1067–1075. [Google Scholar] [CrossRef]
- Nagesh, S.; Jagadeesh, H.; Nithin, K. Study on effect of laboratory roller compaction on unconfined compressive strength of lime treated soils. Int. J. Geo-Eng. 2021, 12, 22. [Google Scholar] [CrossRef]
- Rai, P.; Pei, H.; Meng, F.; Ahmad, M. Utilization of marble powder and magnesium phosphate cement for improving the engineering characteristics of soil. Int. J. Geosynth. Ground Eng. 2020, 6, 31. [Google Scholar] [CrossRef]
- Rai, P.; Qiu, W.; Pei, H.; Chen, J.; Ai, X.; Liu, Y.; Ahmad, M. Effect of fly ash and cement on the engineering characteristic of stabilized subgrade soil: An experimental study. Geofluids 2021, 2021, 1368194. [Google Scholar] [CrossRef]
- Puppala, A.J.; Punthutaecha, K.; D’Souza, N. Assessments of Fly Ash and Bottom Ash Stabilization Methods on Soft and Natural Expansive Soils. In Proceedings of the International Conference on Advances in Civil Engineering, Indian Institute of Technology, Kharagpur, India, 3–5 January 2002. [Google Scholar]
- Rogers, J.D.; Olshansky, R.; Rogers, R.B. Damage to foundations from expansive soils. Claims People 1993, 3, 1–4. [Google Scholar]
- Fratta, D.; Aguettant, J.; Roussel-Smith, L. Introduction to Soil Mechanics Laboratory Testing; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Mitchell, J.K.; Soga, K. Fundamentals of Soil Behavior; John Wiley & Sons: New York, NJ, USA, 2005; Volume 3. [Google Scholar]
- Nelson, J.D.; Chao, K.C.; Overton, D.D.; Nelson, E.J. Foundation Engineering for Expansive Soils; John Wiley & Sons: Hoboken, NJ, USA, 2015. [Google Scholar]
- Masia, M.J.; Totoev, Y.Z.; Kleeman, P.W. Modeling expansive soil movements beneath structures. J. Geotech. Geoenvironmental Eng. 2004, 130, 572–579. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhao, F.H.; Shi, W.C.; Li, J. A general discussion of soil-water interaction in expansive soil. Applied Mechanics and Materials 2013, 368–370, 1591–1595. [Google Scholar] [CrossRef]
- Clayton, C.R.; Xu, M.; Whiter, J.T.; Ham, A.; Rust, M. Stresses in cast-iron pipes due to seasonal shrink-swell of clay soils. Proc. Inst. Civ. Eng. Water Manag. 2010, 163, 157–162. [Google Scholar] [CrossRef]
- Pritchard, O.G.; Hallett, S.H.; Farewell, T.S. Soil movement in the UK–Impacts on critical infrastructure. Infrastruct. Transit. Res. Consort. 2013. Available online: https://www.itrc.org.uk/wp-content/PDFs/Soil-movement-impacts-UK-infrastructure.pdf (accessed on 5 April 2022).
- Hughes, P.; Glendinning, S.; Mendes, J.; Parkin, G.; Toll, D.; Gallipoli, D.; Miller, P. Full-scale testing to assess climate effects on embankments. Proc. Inst. Civ. Eng. Eng. Sustain. 2009, 162, 67–79. [Google Scholar] [CrossRef]
- Chao, K.C.; Kang, J.B.; Nelson, J.D. Evaluation of Failure of Embankment Slope Constructed with Expansive Soils. Geotech. Eng. 2018, 49, 140–149. [Google Scholar]
- Postill, H.; Helm, P.R.; Dixon, N.; Glendinning, S.; Smethurst, J.; Rouainia, M.; Briggs, K.M.; El-Hamalawi, A.; Blake, A. Forecasting the long-term deterioration of a cut slope in high-plasticity clay using a numerical model. Eng. Geol. 2021, 280, 105912. [Google Scholar] [CrossRef]
- Agus, S.S.; Schanz, T.; Fredlund, D.G. Measurements of suction versus water content for bentonite–sand mixtures. Can. Geotech. J. 2010, 47, 583–594. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Rahardjo, H. Soil Mechanics for Unsaturated Soils; John Wiley & Sons: Hoboken, NJ, USA, 1993. [Google Scholar]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Xing, A. Equations for the soil-water characteristic curve. Can. Geotech. J. 1994, 31, 521–532. [Google Scholar] [CrossRef]
- Karunarathne, A.N. Investigation of expansive soil for design of light residential footings in Melbourne. Ph.D. Thesis, Swinburne University of Technology, Melbourne, Australia, 2016. [Google Scholar]
- Aubertin, M.; Mbonimpa, M.; Bussière, B.; Chapuis, R. A model to predict the water retention curve from basic geotechnical properties. Can. Geotech. J. 2003, 40, 1104–1122. [Google Scholar] [CrossRef]
- Fredlund, M.D.; Wilson, G.W.; Fredlund, D.G. Use of the grain-size distribution for estimation of the soil-water characteristic curve. Can. Geotech. J. 2002, 39, 1103–1117. [Google Scholar] [CrossRef]
- Saxton, K.E.; Rawls, W.J. Soil water characteristic estimates by texture and organic matter for hydrologic solutions. Soil Sci. Soc. Am. J. 2006, 70, 1569–1578. [Google Scholar] [CrossRef]
- de Gitirana, F.N., Jr.; Fredlund, D.G. Soil-water characteristic curve equation with independent properties. J. Geotech. Geoenvironmental Eng. 2004, 130, 209–212. [Google Scholar] [CrossRef]
- Witczak, M.W.; Zapata, C.E.; Houston, W.N. Models incorporated into the Current Enhanced Integrated Climatic Model: NCHRP 9-23. In Project Findings and Additional Changes after Version 0.7; NCHRP: Tempe, AZ, USA, 2006. [Google Scholar]
- Zapata, C.E. Uncertainty in Soil-Water-Characteristic Curve and Impacts on Unsaturated Shear Strength Predictions. Ph.D. Thesis, Arizona State University, Tempe, AZ, USA, 1999. [Google Scholar]
- Kovács, G. Seepage Hydraulics; Elsevier Science Publishers: Amsterdam, The Netherlands, 1981. [Google Scholar]
- Fredlund, D.G.; Rahardjo, H.; Fredlund, M.D. Unsaturated Soil Mechanics in Engineering Practice; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Bussière, B. Colloquium 2004: Hydrogeotechnical properties of hard rock tailings from metal mines and emerging geoenvironmental disposal approaches. Can. Geotech. J. 2007, 44, 1019–1052. [Google Scholar] [CrossRef]
- Green, R.; Corey, J. Calculation of hydraulic conductivity: A further evaluation of some predictive methods. Soil Sci. Soc. Am. J. 1971, 35, 3–8. [Google Scholar] [CrossRef]
- Fredlund, D.; Xing, A.; Huang, S. Predicting the permeability function for unsaturated soils using the soil-water characteristic curve. Can. Geotech. J. 1994, 31, 533–546. [Google Scholar] [CrossRef]
- Kong, L.-w.; Wang, M.; Guo, A.-g.; Wang, Y. Effect of drying environment on engineering properties of an expansive soil and its microstructure. J. Mt. Sci. 2017, 14, 1194–1201. [Google Scholar] [CrossRef]
- Zhan, T.L.T.; Chen, R.; Ng, C.W.W. Wetting-induced softening behavior of an unsaturated expansive clay. Landslides 2014, 11, 1051–1061. [Google Scholar] [CrossRef]
- Puppala, A.J.; Katha, B.; Hoyos, L.R. Volumetric shrinkage strain measurements in expansive soils using digital imaging technology. Geotech. Test. J. 2004, 27, 547–556. [Google Scholar]
- Puppala, A.J.; Manosuthikij, T.; Chittoori, B.C. Swell and shrinkage strain prediction models for expansive clays. Eng. Geol. 2014, 168, 1–8. [Google Scholar] [CrossRef]
- Sun, K.Q.; Tang, C.S.; Liu, C.L.; Li, H.D.; Wang, P.; Leng, T. Research methods of soil desiccation cracking behavior. Rock Soil Mech. 2017, 38, 11–26. [Google Scholar] [CrossRef]
- Fityus, S.; Smith, D.; Allman, M. Expansive soil test site near Newcastle. J. Geotech. Geoenvironmental Eng. 2004, 130, 686–695. [Google Scholar] [CrossRef]
- Fityus, S.; Walsh, P.; Kleeman, P. The influence of climate as expressed by the Thornthwaite index on the design depth of moisture change of clay soils in the Hunter Valley. In Proceedings of the Conference on geotechnical engineering and engineering geology in the Hunter Valley, Newcastle, Australia, 11–13 July 1998; pp. 251–265. [Google Scholar]
- AS2870; Residential Slabs and Footings. Standards Australia: Sydney, Australia, 2011.
- Li, J.; Guo, L. Field Investigation and Numerical Analysis of Residential Building Damaged by Expansive Soil Movement Caused by Tree Root Drying. J. Perform. Constr. Facil. 2017, 31, D4016003. [Google Scholar] [CrossRef]
- Cheng, Y.Z.; Huang, X.M.; Li, C.; Shen, Z.P. Field and numerical investigation of soil-atmosphere interaction at Nairobi, Kenya. Eur. J. Environ. Civ. Eng. 2017, 21, 1326–1340. [Google Scholar] [CrossRef]
- Mitchell, P.W. The Design of Shallow Footings on Expansive Soil. Ph.D. Thesis, University of Adelaide, Adelaide, Australia, 1984. [Google Scholar]
- Fernandes, M.; Denis, A.; Fabre, R.; Lataste, J.F.; Chretien, M. In situ study of the shrinkage-swelling of a clay soil over several cycles of drought-rewetting. Eng. Geol. 2015, 192, 63–75. [Google Scholar] [CrossRef]
- Blight, G. The vadose zone soil-water balance and transpiration rates of vegetation. Géotechnique 2003, 53, 55–64. [Google Scholar] [CrossRef]
- Azizi, A.; Kumar, A.; Toll, D.G. Coupling cyclic and water retention response of a clayey sand subjected to traffic and environmental cycles. Géotechnique 2021, 1–17. [Google Scholar] [CrossRef]
- Khalili, N.; Khabbaz, M. A unique relationship for χ for the determination of the shear strength of unsaturated soils. Geotechnique 1998, 48, 681–687. [Google Scholar] [CrossRef]
- Mendes, J.; Toll, D. Influence of initial water content on the mechanical behavior of unsaturated sandy clay soil. Int. J. Geomech. 2016, 16, D4016005. [Google Scholar] [CrossRef]
- Toll, D.G.; Md Rahim, M.S.; Karthikeyan, M.; Tsaparas, I. Soil–atmosphere interactions for analysing slopes in tropical soils in Singapore. Environ. Geotech. 2018, 6, 361–372. [Google Scholar] [CrossRef]
- Teodosio, B.; Baduge, K.S.K.; Mendis, P. Simulating reactive soil and substructure interaction using a simplified hydro-mechanical finite element model dependent on soil saturation, suction and moisture-swelling relationship. Comput. Geotech. 2020, 119, 103359. [Google Scholar] [CrossRef]
- Teodosio, B.; Baduge, K.S.K.; Mendis, P. Relationship between reactive soil movement and footing deflection: A coupled hydro-mechanical finite element modelling perspective. Comput. Geotech. 2020, 126, 103720. [Google Scholar] [CrossRef]
- Mitchell, P. A simple method of design of shallow footings on expansive soil. In Proceedings of the 5th International Conference on Expansive Soils, Adelaide, Australia, 21–23 May 1984. [Google Scholar]
- Qi, S.; Vanapalli, S.K. Simulating Hydraulic and Mechanical Responses of Unsaturated Expansive Soil Slope to Rainfall: Case Study. Int. J. Geomech. 2018, 18, 05018002. [Google Scholar] [CrossRef]
- Chan, D.; Rajeev, P.; Kodikara, J.; Gallage, C. Field Performance of In-Service Cast Iron Gas Reticulation Pipe Buried in Reactive Clay. J. Pipeline Syst. Eng. Pract. 2016, 7, 04015025. [Google Scholar] [CrossRef]
- Gallage, C.P.K.; Kodikara, J.; Chan, D. Response of a plastic pipe buried in expansive clay. Proc. Inst. Civ. Eng. Geotech. Eng. 2012, 165, 45–57. [Google Scholar] [CrossRef]
- Weerasinghe, D.; Kodikara, J.; Bui, H. Numerical modelling of swelling/shrinkage behaviour of unsaturated soils for buried pipe stress analysis. In Proceedings of the 6th Asia-Pacific Conference on Unsaturated Soils, Guilin, China, 23–26 October 2015; pp. 615–620. [Google Scholar]
- Zornberg, J.; Roodi, G. Use of geosynthetics to mitigate problems associated with expansive clay subgrades. Geosynth. Int. 2021, 28, 279–302. [Google Scholar] [CrossRef]
- Karunarathne, A.; Fardipour, M.; Gad, E.; Rajeev, P.; Disfani, M.; Sivanerupan, S.; Wilson, J. Modelling of climate induced moisture variations and subsequent ground movements in expansive soils. Geotech. Geol. Eng. 2018, 36, 2455–2477. [Google Scholar] [CrossRef]
- Li, J.; Sun, D.a.; Sheng, D.; Sloan, S.; Fredlund, D. Preliminary study on soil water characteristics of Maryland clay. In Proceedings of the 3rd Asian Conference on Unsaturated Soils, Nanjing, China, 21–23 April 2007; pp. 569–574. [Google Scholar]
- Bishop, A.W. The principle of effective stress. Tek. Ukebl. 1959, 39, 859–863. [Google Scholar]
- Abaqus, V. 6.14, Online Documentation Help, Theory Manual: Dassault Systems. 2016. Available online: http://130.149.89.49:2080/v2016/index.html (accessed on 7 July 2022).
- Rutqvist, J.; Börgesson, L.; Chijimatsu, M.; Kobayashi, A.; Jing, L.; Nguyen, T.; Noorishad, J.; Tsang, C.-F. Thermohydromechanics of partially saturated geological media: Governing equations and formulation of four finite element models. Int. J. Rock Mech. Min. Sci. 2001, 38, 105–127. [Google Scholar] [CrossRef]
- Leao, S. Mapping potential risk for housing damage from ground movement due to climate change. Int. J. Environ. Sci. Dev. 2014, 5, 387. [Google Scholar] [CrossRef]
- Cowan, S.; Gibbons, P. The application of As 2870 for subgrade treatment design of Expansive Clay in Adelaide. In Proceedings of the South Australian Chapter of the Australian Geomechanics Society, Adelaide, Australia, 15 October 2018. [Google Scholar]
- Gibbons, P.; Cowan, S. Subgrade treatment design for expansive soils in Adelaide using Australian standard as 2870–is this the right approach? Aust. Geomech. J 2018, 53, 97–106. [Google Scholar]
- Richards, B.; Peter, P.; Emerson, W. The effects of vegetation on the swelling and shrinking of soils in Australia. Geotechnique 1983, 33, 127–139. [Google Scholar] [CrossRef]
- AS1289.7.1.3; Soil Reactivity Tests-Determination of the Shrinkage Index of a Soil—Core Shrinkage Index. Standards Australia: Sydney, Australia, 1998. Available online: https://store.standards.org.au/product/as-1289-7-1-3-1998 (accessed on 6 April 2022).
- AS1289.7.1.2; Soil Reactivity Tests-Determination of the Shrinkage Index of a Soil—Loaded Shrinkage Index. Standards Australia: Sydney, Australia, 1998. Available online: https://www.techstreet.com/standards/as-1289-7-1-2-1998?product_id=2048396 (accessed on 6 April 2022).
- AS1289.7.1.1; Soil Reactivity Tests-Determination of the Shrinkage Index of a Soil—Shrink-Sewll Index. Standards Australia: Sydney, Australia, 2003. Available online: https://store.standards.org.au/product/as-1289-7-1-1-2003 (accessed on 6 April 2022).
- Mitchell, P.; Avalle, D. A technique to predict expansive soil movements. In Proceedings of the 5th International Conference on Expansive Soils, Adelaide, Australia, 21–23 May 1984; pp. 124–130. [Google Scholar]
- Li, J.; Zhou, A.N. The Australian approach to residential footing design on expansive soils. Appl. Mech. Mater. 2013, 438–439, 593–598. [Google Scholar] [CrossRef]
- Fityus, S.G.; Cameron, D.A.; Walsh, P.F. The shrink swell test. Geotech. Test. J. 2005, 28, 92–101. [Google Scholar]
- Cameron, D. Dealing with reactive clay soils through a national standard. Aust. Geomech. J. 2018, 53, 51–65. [Google Scholar]
- Cameron, D. Tests for reactivity and prediction of ground movement. Aust. Civ. Eng. Trans. 1989, CE31, 121–132. [Google Scholar]
- Zou, J. Assessment of the Reactivity of Expansive Soil in Melbourne Metropolitan Area; RMIT University: Melbourne, Australia, 2015. [Google Scholar]
- Austroads. Guide to Pavement Technology Part 2: Pavement Structural Design; Austroads Ltd.: Sydney, Australia, 2017; Available online: https://austroads.com.au/publications/pavement/agpt02 (accessed on 15 October 2021).
- Mitchell, P.W. Overview of Adelaide Soil and Geology. In Proceedings of the Laboratory Testing of Expansive Soils, South Australian Chapter of the Australian Geomechanics Society, Adelaide, Australia, 23 September 2015. [Google Scholar]
- NHBC. NHBC Standards 2020. Available online: https://nhbc-standards.co.uk/2020/4-foundations/4-2-building-near-trees/4-2-4-the-effects-of-trees-on-shrinkable-soils/ (accessed on 6 April 2022).
- Walsh, P.; Cameron, D. SAA HB28-1997: The design of residential slabs and footings. In Standards Australia; Standards Australia: Sydney, Australia, 1997; Available online: https://standards.org.au/access-standards (accessed on 15 October 2021).
- Walsh, P.F. The Analysis of Stiffened Rafts on Expansive Clays; Division of Building Research, CSIRO: Melbourne, Australia, 1978. [Google Scholar]
- Mitchell, P.W. The Structural Analysis of Footings on Expansive Soil; Kenneth, W.G., Ed.; Smith & Associates: Woodforde, Australia, 1979. [Google Scholar]
- Walsh, P.; Fityus, S.; Kleeman, P. A note on the depth of design suction change for clays in South Western Australia and South Eastern Queensland. Aust. Geomech. 1998, 33, 37–40. [Google Scholar]
- Hu, Y.; Saraceni, P.; Cocks, G.; Zhou, M. TMI Assessment and Climate Zones in Western Australia. Aust. Geomech. J. 2016, 51, 79–89. [Google Scholar]
- Davenport, F. Problem Soils-A West Australian Perspective. Aust. Geomech. 2007, 42, 1–20. [Google Scholar]
- Snethen, D.R.; Johnson, L.D.; Patrick, D.M. An Evaluation of Expedient Methodology for Identification of Potentially Expansive Soils. Report No. FHWA-RE-77-94; Soils and Pavements Laboratory, US Army Engineers Waterways Experiment Station: Vicksburg, MS, USA, 1977. Available online: https://rosap.ntl.bts.gov/view/dot/37844 (accessed on 31 May 2022).
- American Association of State Highway and Transportation Officials (AASHTO). Standard Method of Test for—Determining Expansive Soils, AASHTO Designation: T258-81. 2008. Available online: https://www.scribd.com/document/551825683/AASHTO-T-258-81-Determining-Expansive-Soils-TOAZ-INFO (accessed on 31 May 2022).
- Lytton, R.; Aubeny, C.; Bulut, R. Design Procedure for Pavements on Expansive Soils; Report No. FHWA/TX-05/0-4518-1; Texas Transportation Institute, The Texas A&M University System: College Station, TX, USA, 2005; Volume 1, Available online: https://static.tti.tamu.edu/tti.tamu.edu/documents/0-4518-1-V1.pdf (accessed on 31 May 2022).
- Zornberg, J.G.; Prozzi, J.P.; Gupta, R.; Luo, R.; McCartney, J.; Ferreira, J.; Nogueira, C. Validating Mechanisms in Geosynthetic Reinforced Pavements; Report No. FHWA/TX-08/0-4829-1; Center for Transportation Research, The University of Texas at Austin: Austin, TX, USA, 2008; Available online: https://ctr.utexas.edu/wp-content/uploads/pubs/0_4829_1.pdf (accessed on 31 May 2022).
- Dye, H.B. Moisture Movement through Expansive Soil and Impact on Performance of Residential Structures; Arizona State University: Tempe, AZ, USA, 2008. [Google Scholar]
- Mitchell, P. Climate change effects on expansive soil movements. In Proceedings of the 18th International Conference on Soil Mechanics and Geotechnical Engineering, Paris, France, 2–6 September 2013; pp. 1159–1162. [Google Scholar]
- Rajeev, P.; Chan, D.; Kodikara, J. Ground–atmosphere interaction modelling for long-term prediction of soil moisture and temperature. Can. Geotech. J. 2012, 49, 1059–1073. [Google Scholar] [CrossRef]
- Forchheimer, P. Wasserbewegung durch boden. Z. Ver. Dtsch. Ing. 1901, 45, 1782–1788. [Google Scholar]
- Rajeev, P.; Kodikara, J. Numerical analysis of an experimental pipe buried in swelling soil. Comput. Geotech. 2011, 38, 897–904. [Google Scholar] [CrossRef]
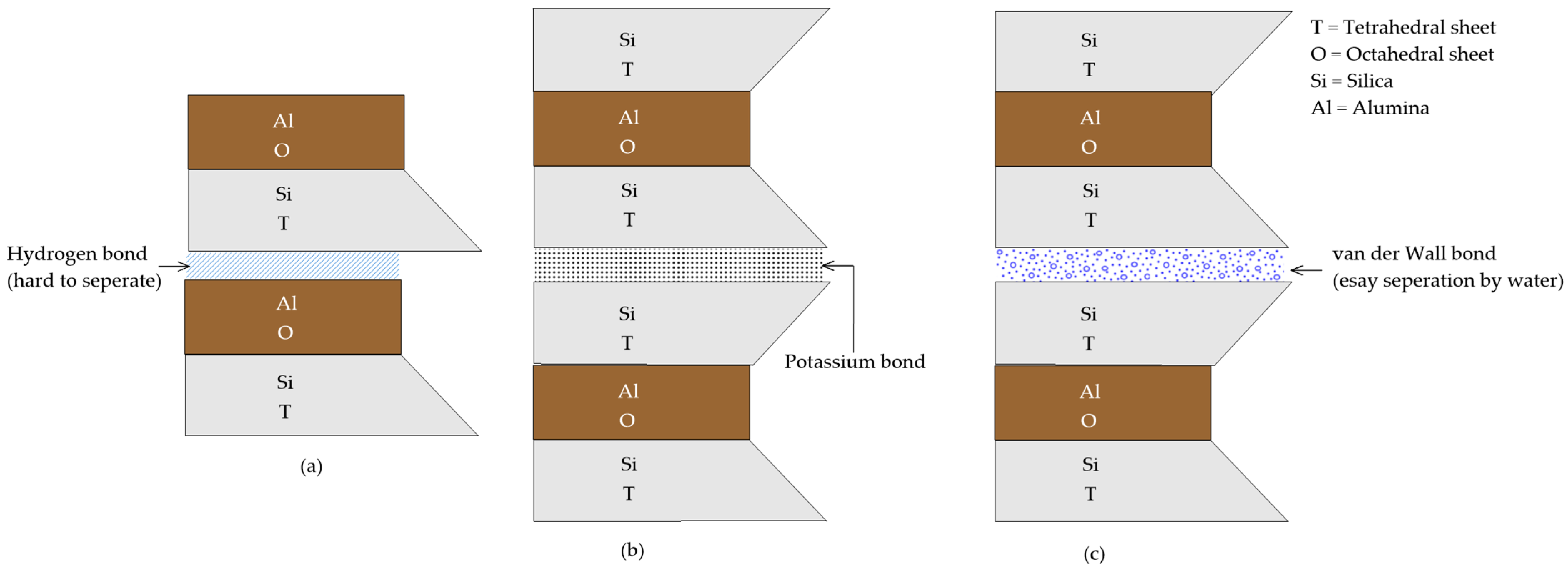

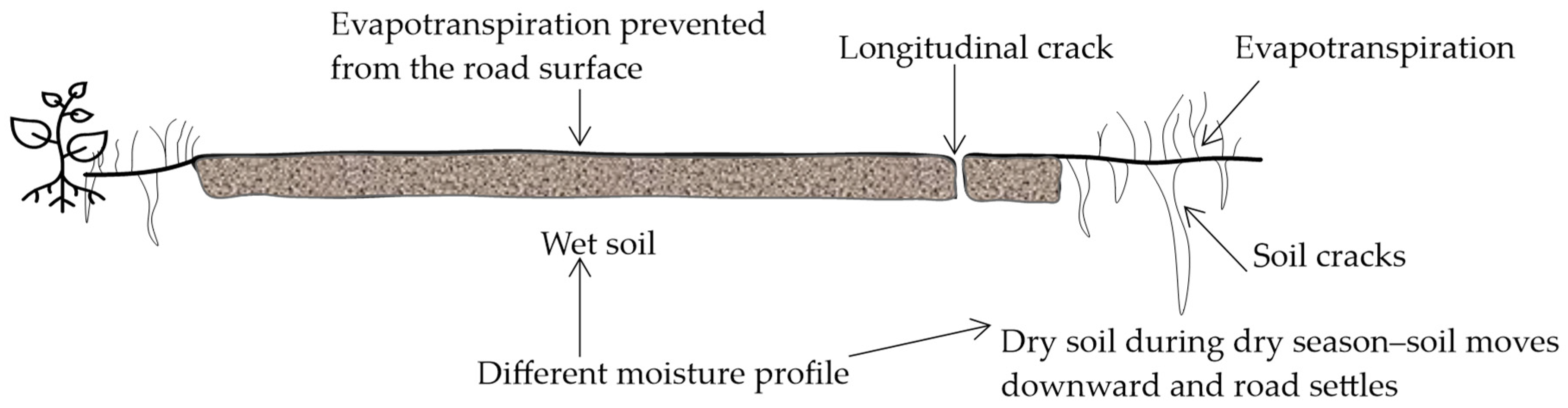
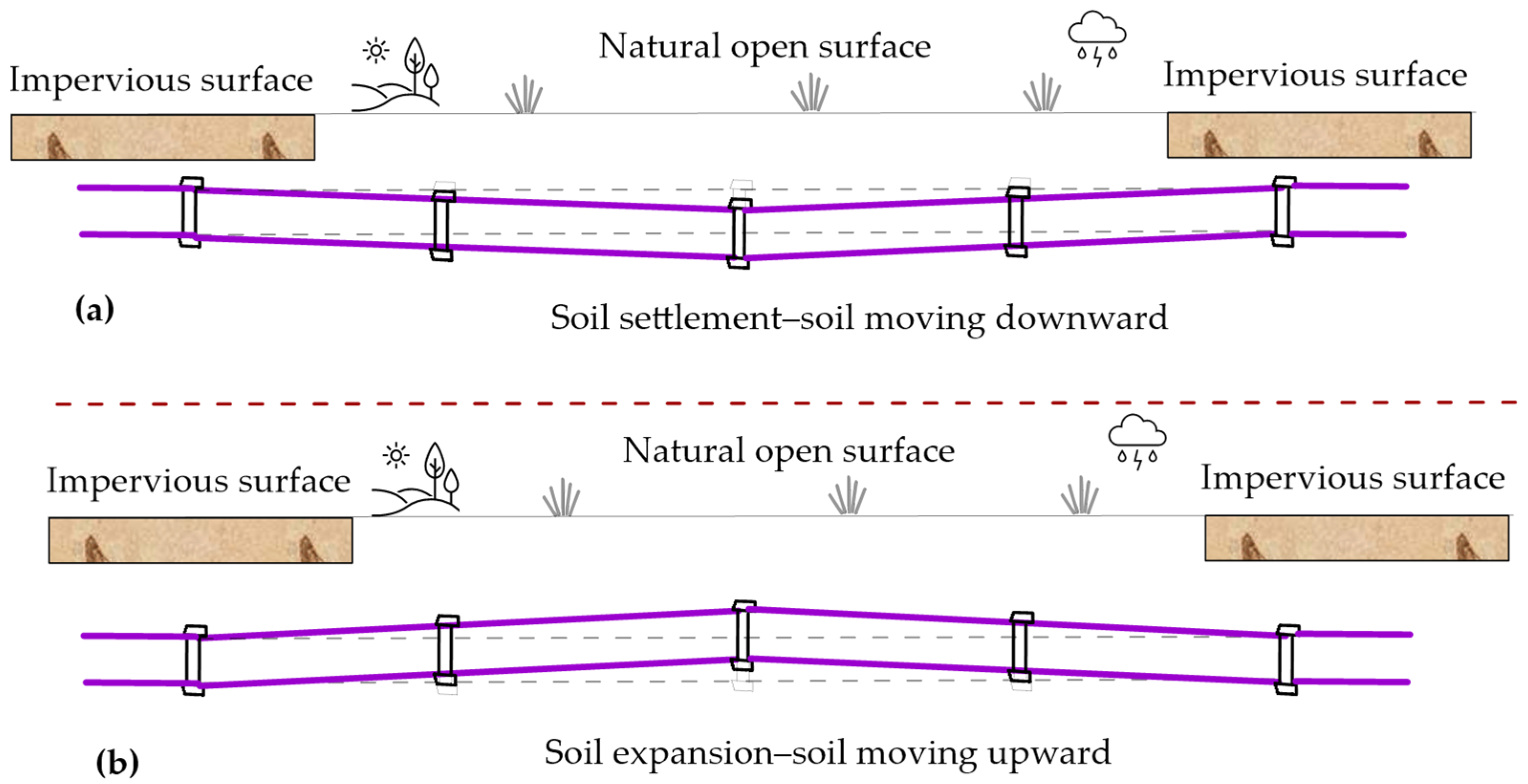

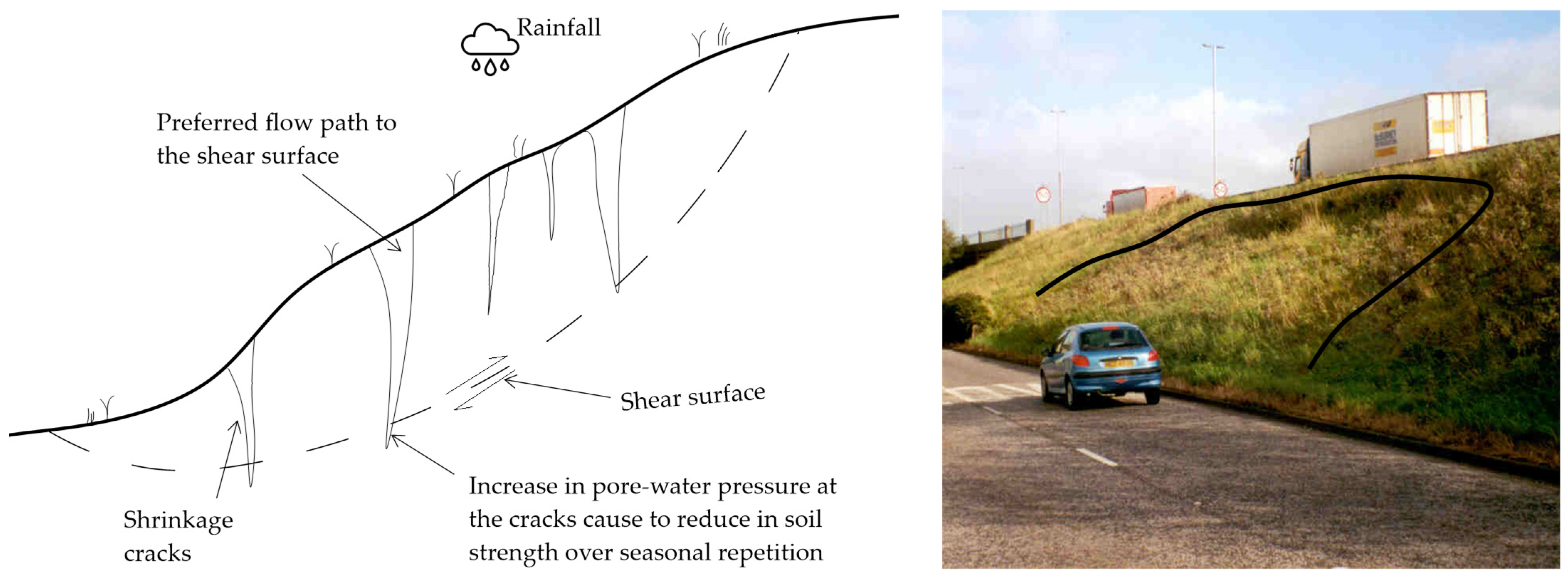
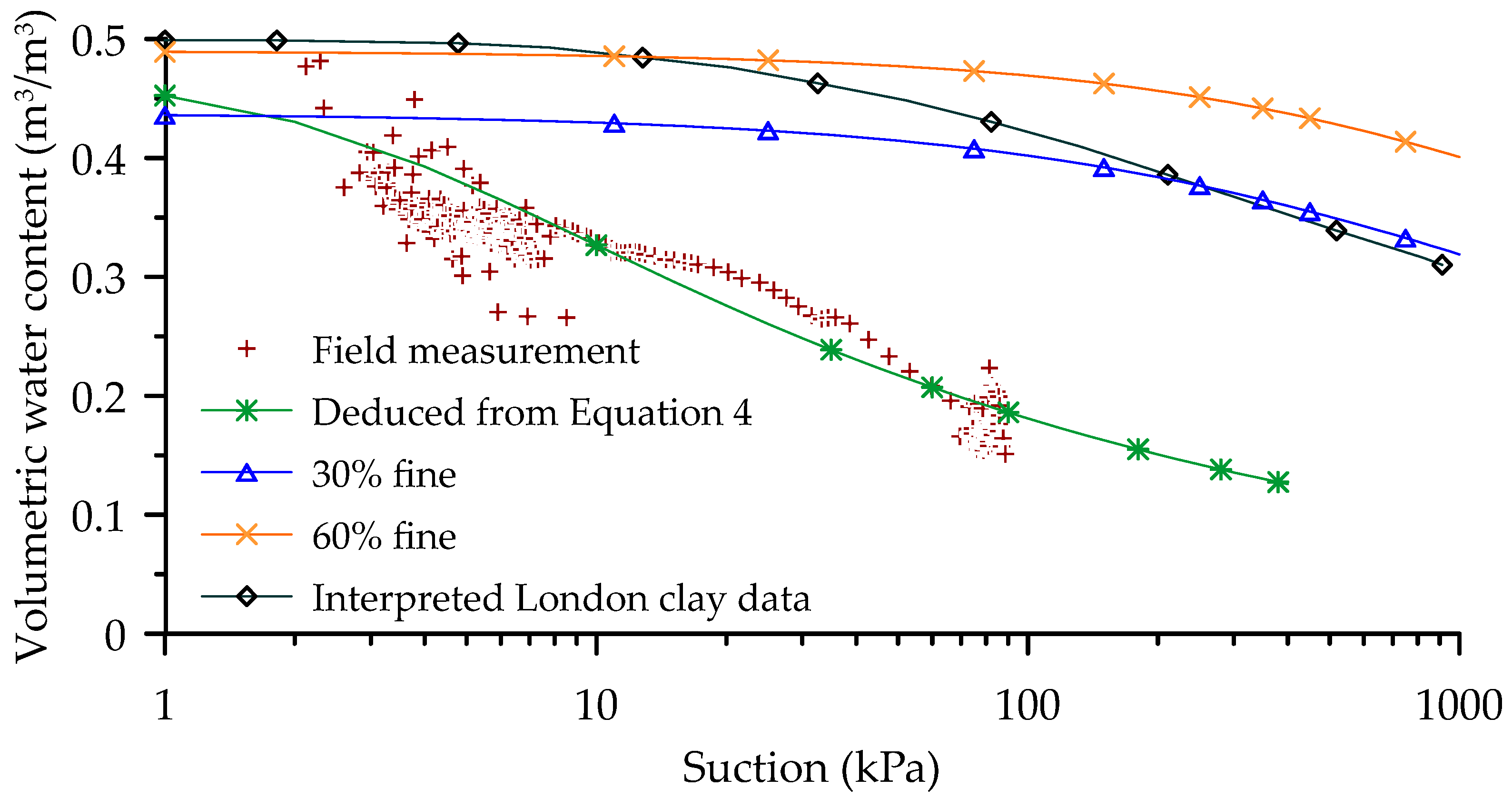
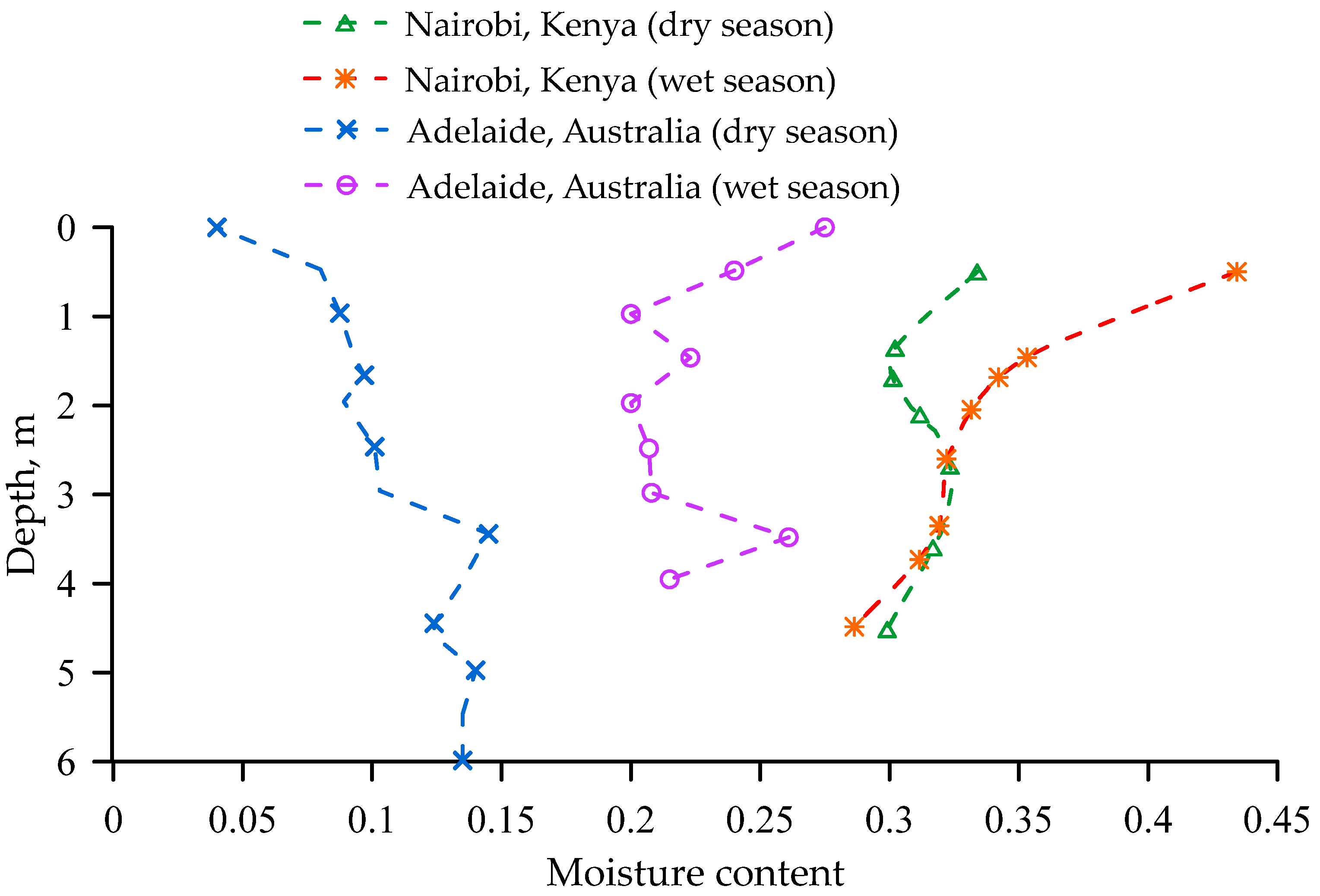
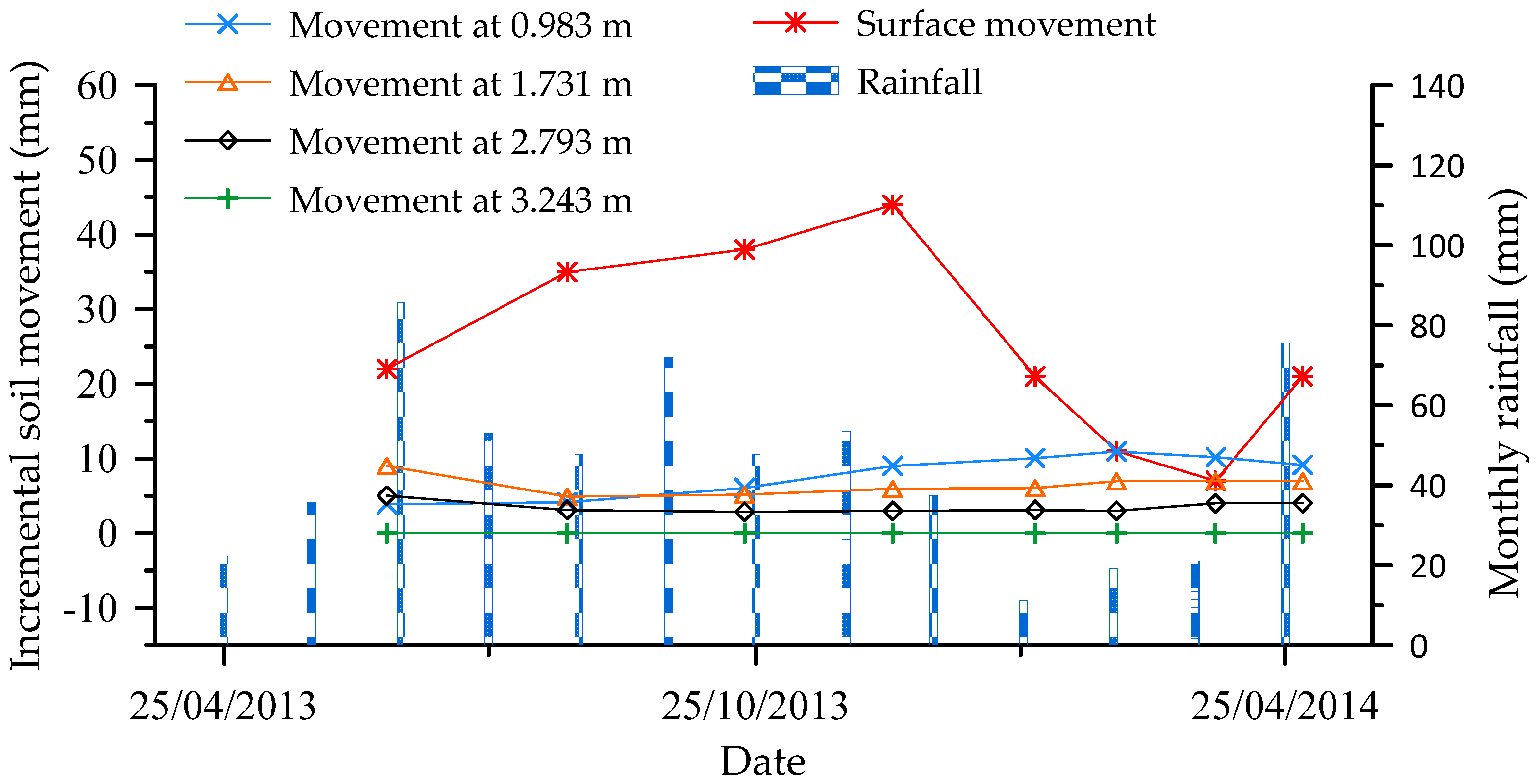
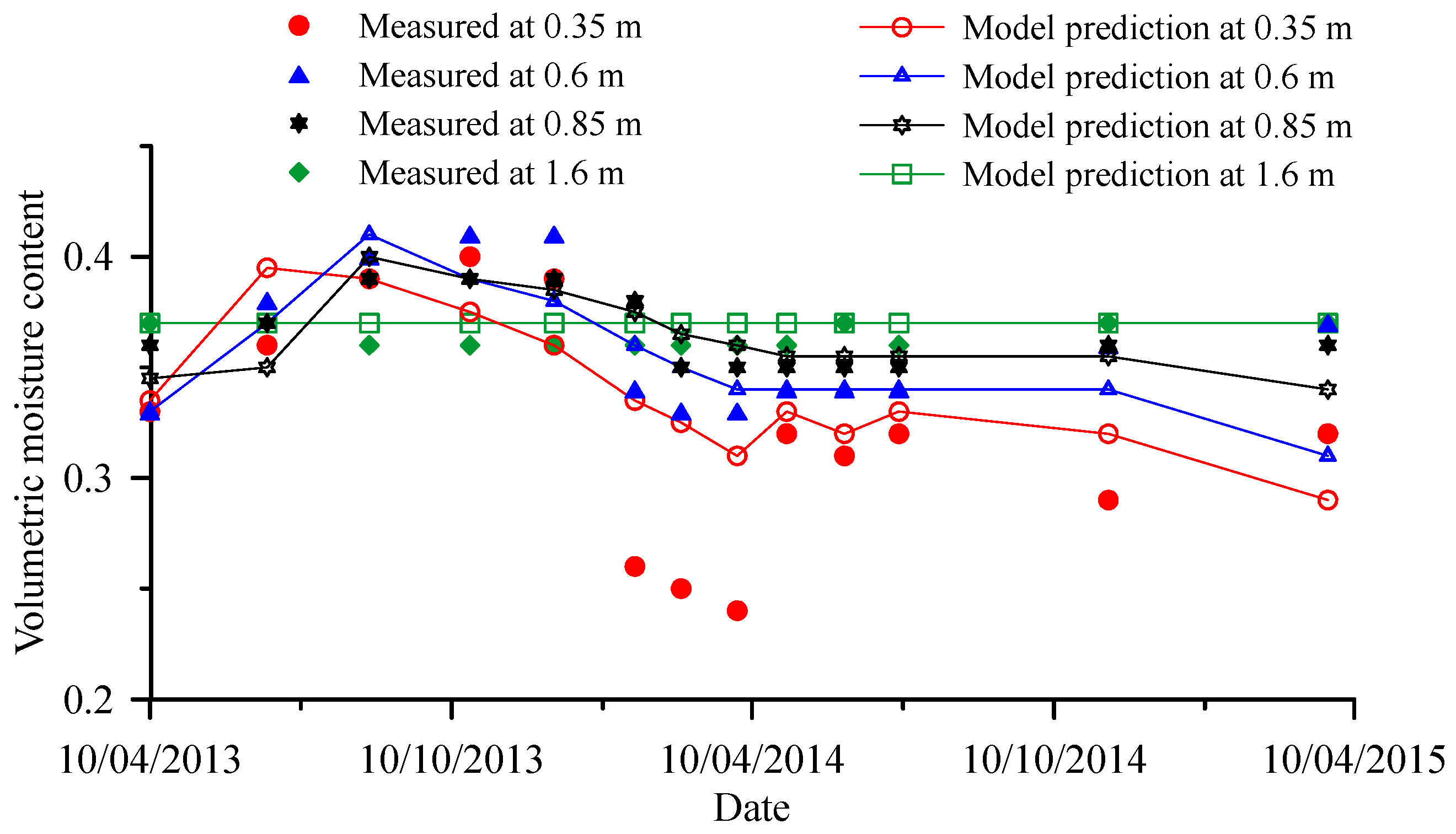

| Phenomenon | Soil Reaction | Result |
|---|---|---|
| Heaving/ | Ground movement | Building failure |
| Shrink–swell | Buried pipe failure | |
| Road failure | ||
| Cracking | Increased permeability | Contamination of underlying groundwater through the easy movement of contaminants |
| Slope instability | Slope movement | Distortion of structures founded on slopes, e.g., road disconnection or interruption of water supply distribution networks |
| Expansiveness | Liquid Limit (LL), % | Plasticity Index (PI) | Weighted PI (PI × % < 425 µm) | Ipt (% Strain/pF) | Modified PI (%) |
|---|---|---|---|---|---|
| Very high | >70 | >45 | >3200 | 4 to 7 for PI > 55 | - |
| High | >70 | >45 | 2200 to 3200 | 2 to 4 for PI < 55 | ≥40 |
| Moderate/ medium | 50 to 70 | 25 to 45 | 1200 to 2200 | 1 to 2 | ≥20 to <40 |
| Low | <50 | <25 | <1200 | <1 | ≥10 to <20 |
| TMI | Hs (m) |
|---|---|
| >10 | 1.5 |
| ≥−5 to 10 | 1.8 |
| ≥−15 to ≤−5 | 2.3 |
| ≥−25 to ≤−15 | 3 |
| ≥−40 to ≤−25 | 4 |
| ≤−40 | >4 |
| Liquid Limit, % | Plasticity Index, % | Soil Suction at Natural Water Content ( ), kPa | Swell Potential, % | Potential Soil Type |
|---|---|---|---|---|
| >60 | >35 | >429 | >1.5 | High |
| 50 to 60 | 25 to 35 | 161 to 429 | 0.5 to 1.5 | Marginal |
| <50 | <25 | <161 | <0.5 | Low |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Devkota, B.; Karim, M.R.; Rahman, M.M.; Nguyen, H.B.K. Accounting for Expansive Soil Movement in Geotechnical Design—A State-of-the-Art Review. Sustainability 2022, 14, 15662. https://doi.org/10.3390/su142315662
Devkota B, Karim MR, Rahman MM, Nguyen HBK. Accounting for Expansive Soil Movement in Geotechnical Design—A State-of-the-Art Review. Sustainability. 2022; 14(23):15662. https://doi.org/10.3390/su142315662
Chicago/Turabian StyleDevkota, Bikash, Md Rajibul Karim, Md Mizanur Rahman, and Hoang Bao Khoi Nguyen. 2022. "Accounting for Expansive Soil Movement in Geotechnical Design—A State-of-the-Art Review" Sustainability 14, no. 23: 15662. https://doi.org/10.3390/su142315662
APA StyleDevkota, B., Karim, M. R., Rahman, M. M., & Nguyen, H. B. K. (2022). Accounting for Expansive Soil Movement in Geotechnical Design—A State-of-the-Art Review. Sustainability, 14(23), 15662. https://doi.org/10.3390/su142315662









