Hysteresis Behavior Modeling of Hard Rock Based on the Mechanism and Relevant Characteristics
Abstract
1. Introduction
2. Experimental Foundation for the Constitutive Model
2.1. Rock Samples and Experimental Scheme
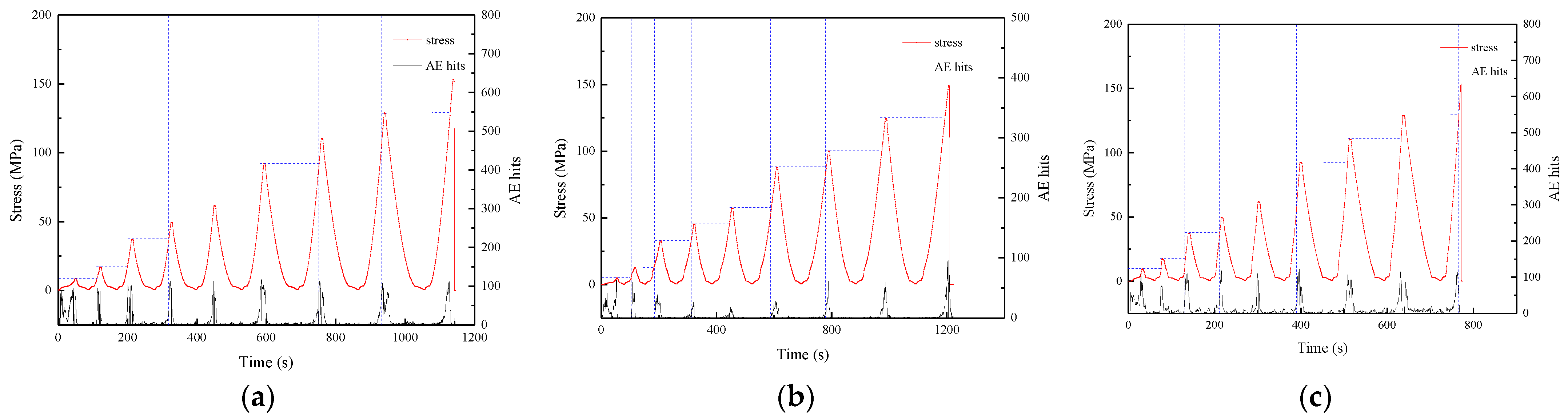
2.2. Results and Analysis of Mechanical Behavior
3. Constitutive Model and Relative Numerical Implementation
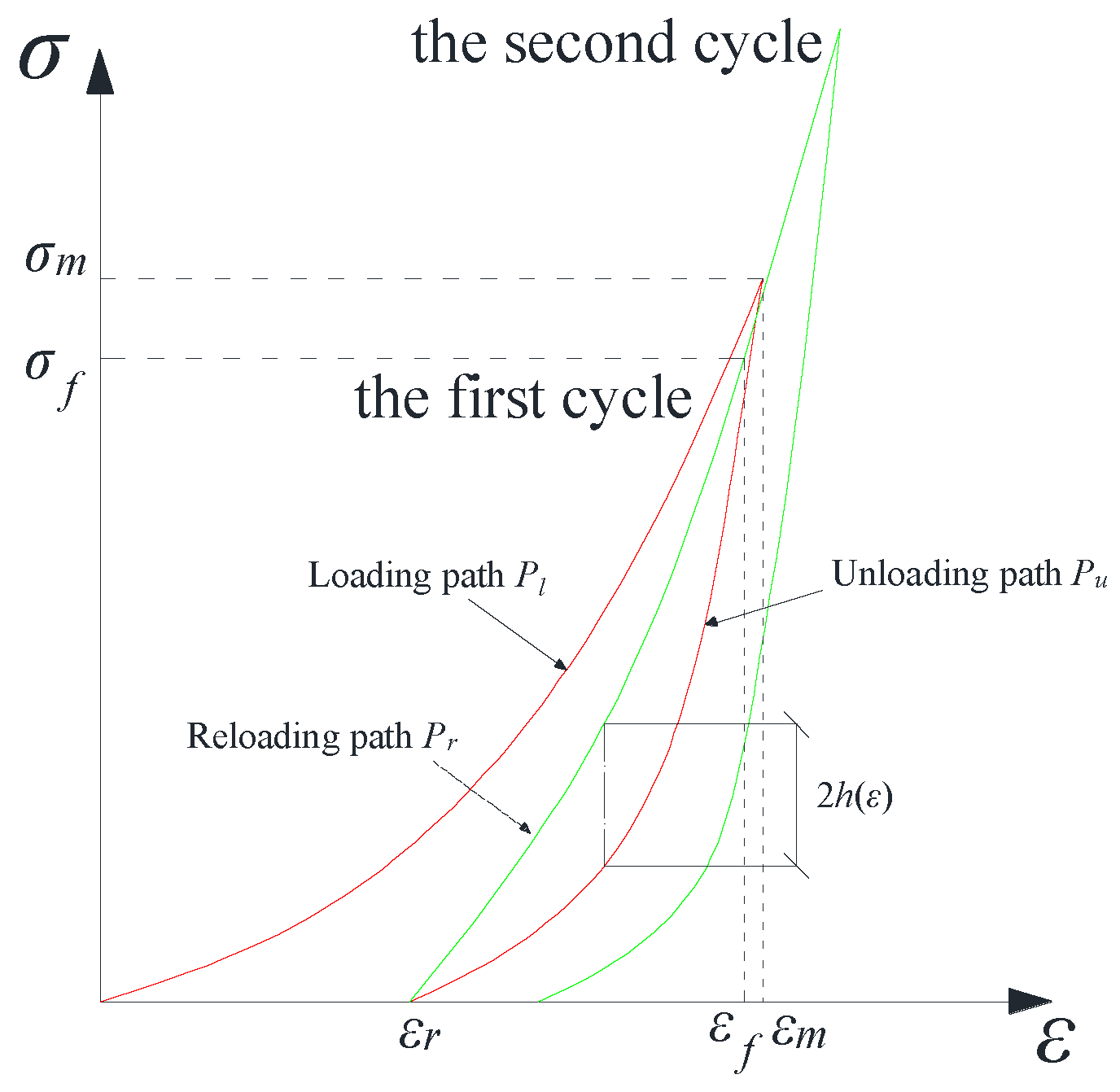
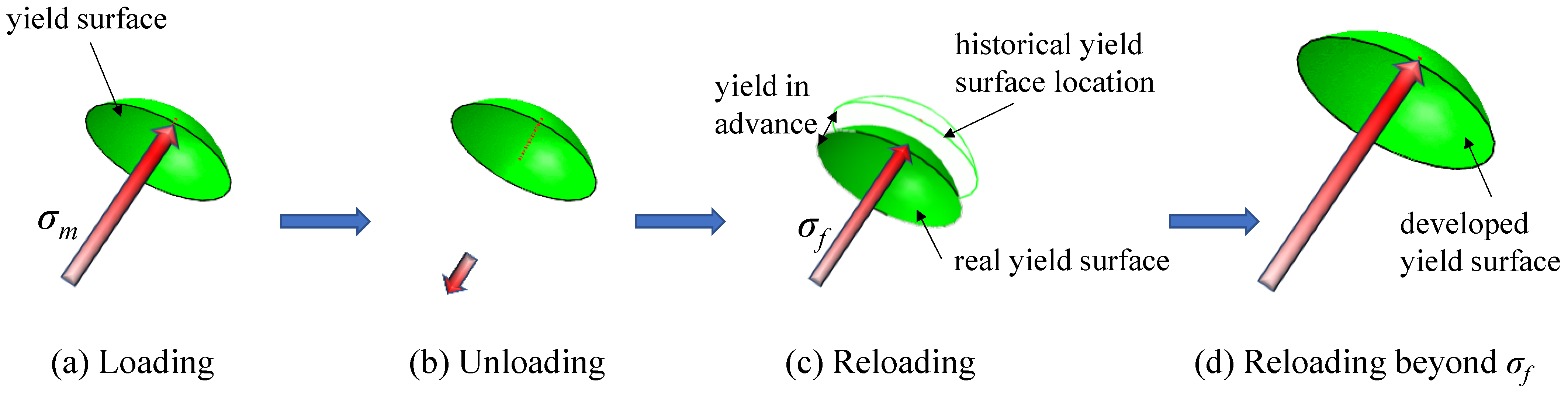
3.1. Basic Hypothesis
- (a)
- The elastic deformation and plastic deformation occur simultaneously during the initial loading and the reloading process from σ to σmi for the subsequent cycles.
- (b)
- Only elastic deformation occurs during unloading and reloading from 0 to σ for each cycle.
- (c)
- The value of friction is dominated by roughness and contact pressure between surfaces in general; in this study, these two factors are considered to be representative of plastic deformation (damage degree of rock sample) and stress level, respectively. Therefore, the greater the damage and stress are, the greater the friction. In addition, friction performs negative work to the facility during the loading process but performs negative work on the rock sample during the unloading process.
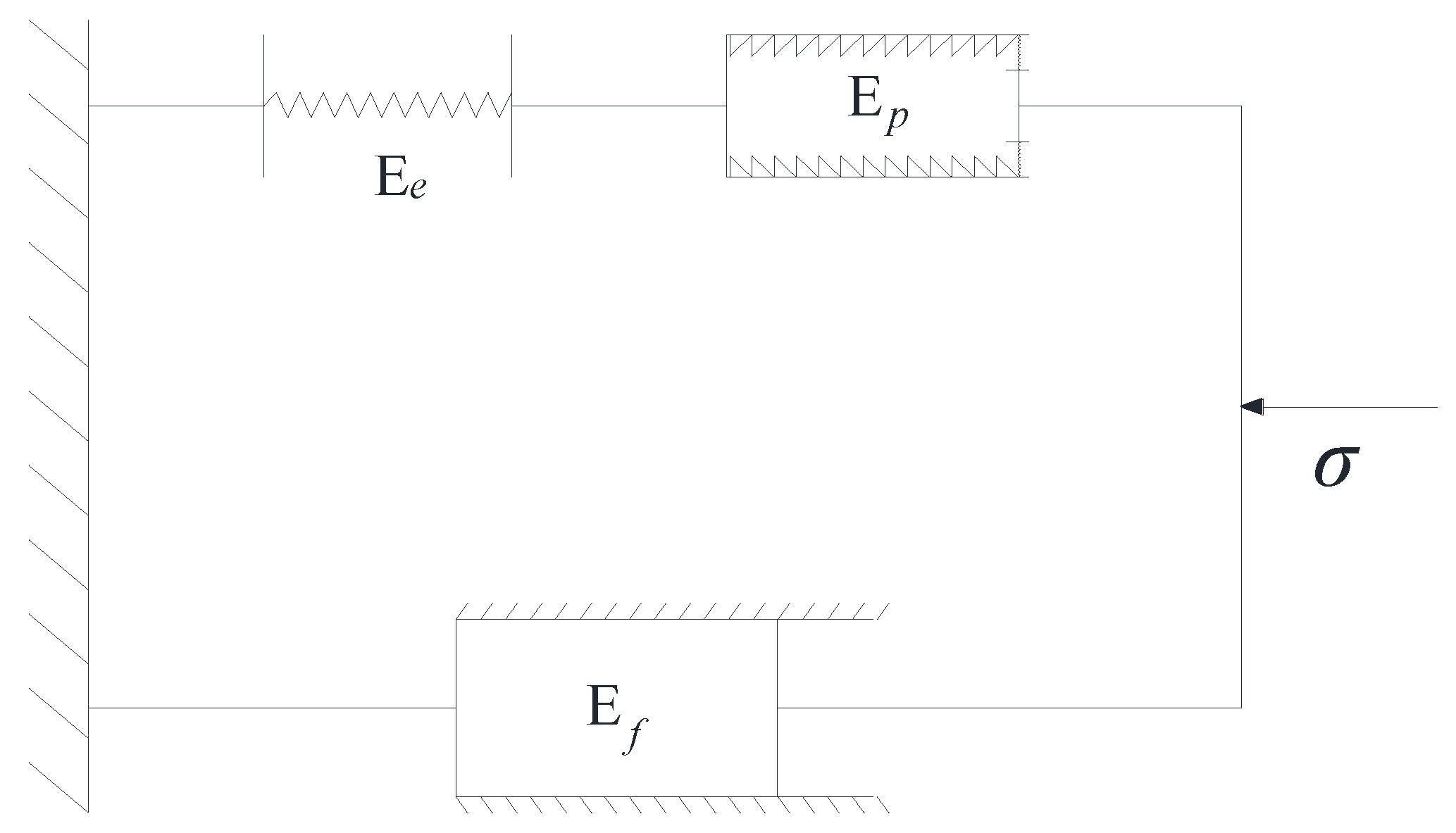
3.2. Constitutive Model
3.3. Procedure of Computer Implementation
3.4. Constitutive Law of Elements in the Proposed Model
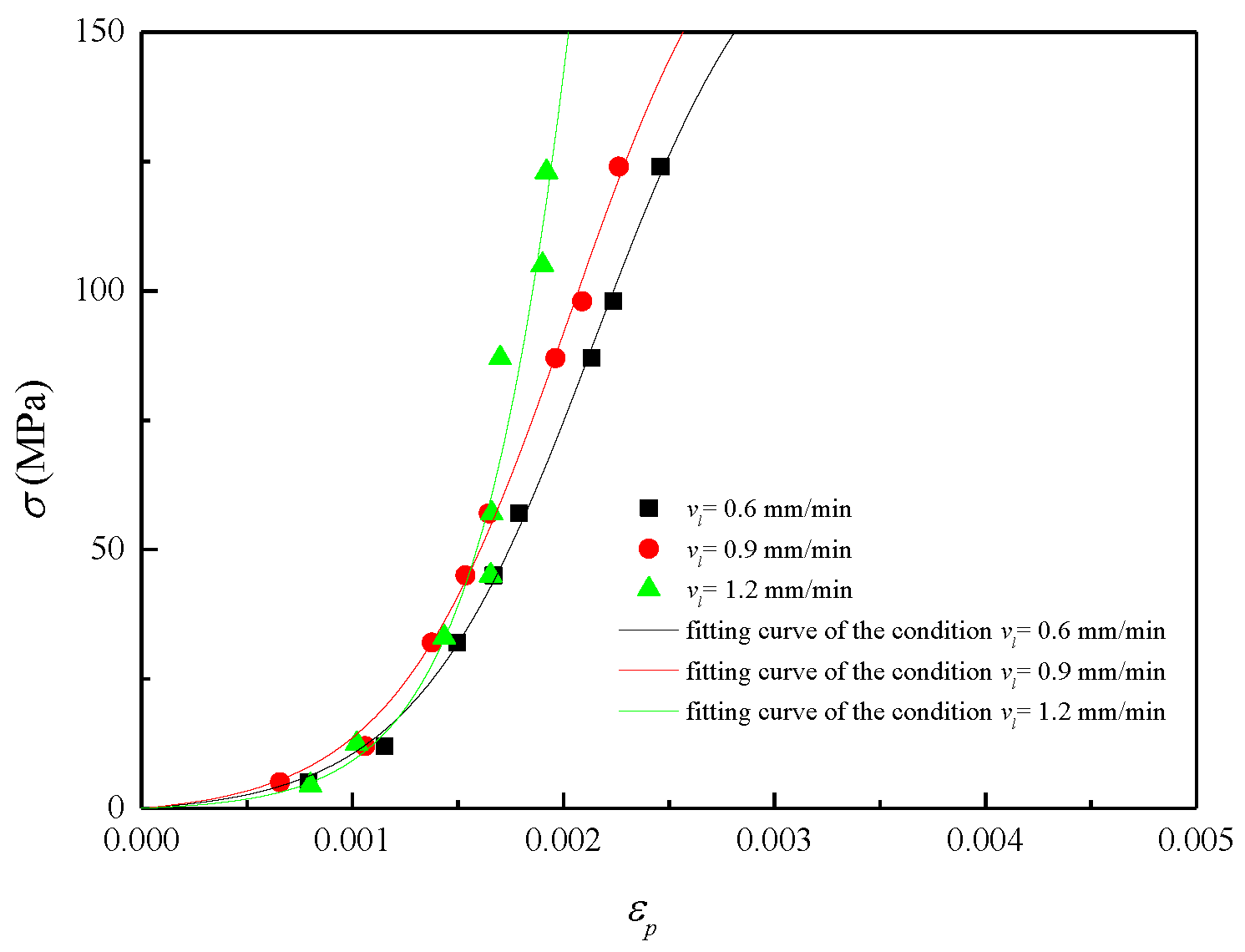
3.4.1. Determination of f(εe)
3.4.2. Determination of g(εp)
3.4.3. Determination of h(εp, f)
4. Parameter Calibration
4.1. Calibration Procedure
- (a)
- Based on the reference values, three different values of each parameter are designated as the labels of the sample set (Table 7). The combination of different parameters results in a total of 2187 samples, of which 90 percent are used for training, and 10 percent are used for validation. By substituting these parameters into the algorithm of the constitutive model, the corresponding strain values can be derived as the features of the sample set.
- (b)
- Considering an order-of-magnitude difference between each parameter, the Min-Max normalization is used for data preprocessing.
- (c)
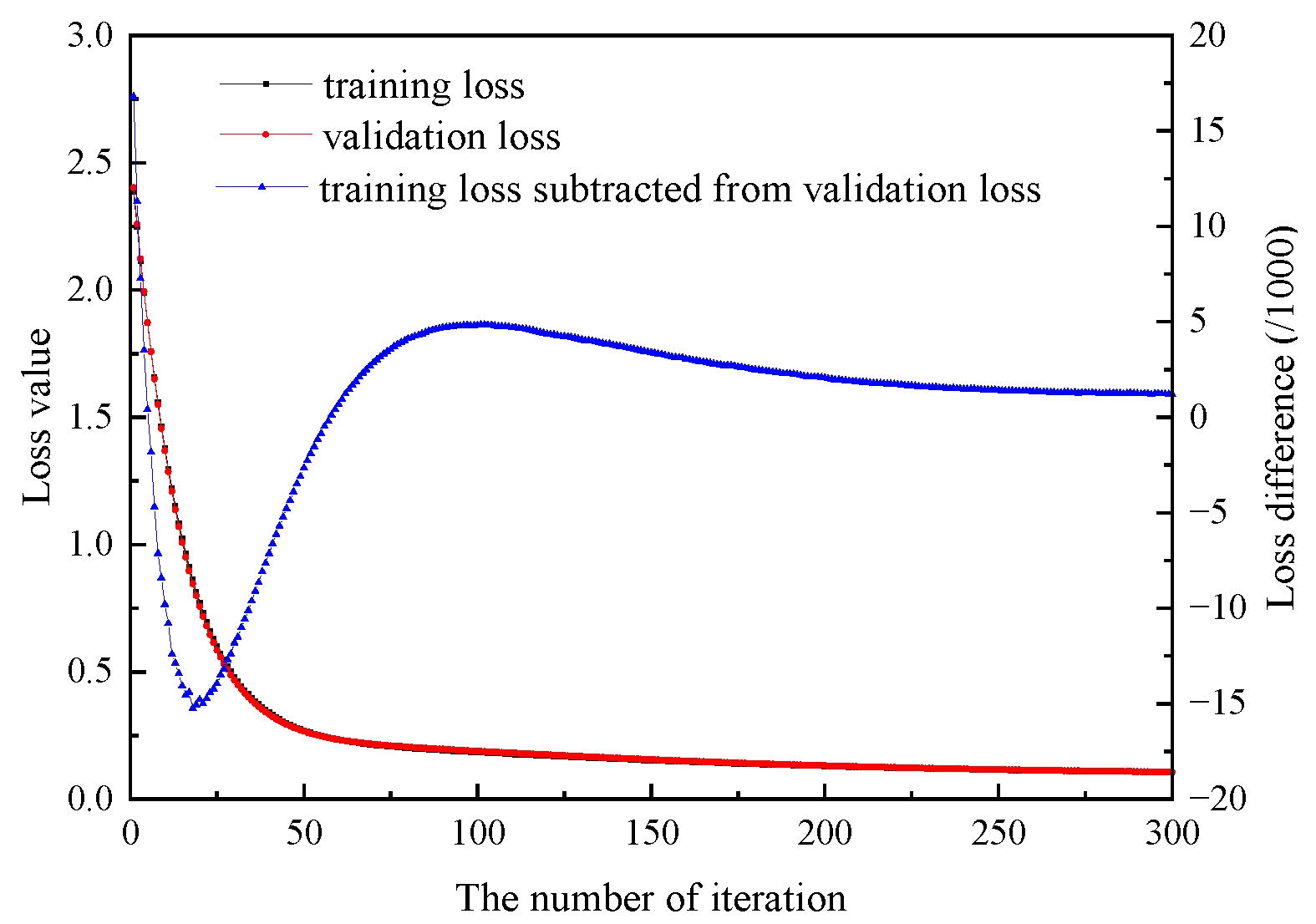
- Constructing a regression model using the BP neural network, which contains one input layer with 17 neurons, one hidden layer with 12 neurons, and one output layer with 7 neurons. The ReLU activation function is used in the hidden layer so as to simulate the nonlinear relation between the input and output, and the Adam optimizer is used to improve the quality of backpropagation. After each iteration, the training loss and validation loss are recorded and saved.
- (d)
- Setting termination criteria in terms of loss value (magnitude of loss< 0.1 and the change of loss <0.005). If the criteria are satisfied, we can conduct the calibration by introducing the experimental strain data into the qualified regression model. If not, a new iteration begins.
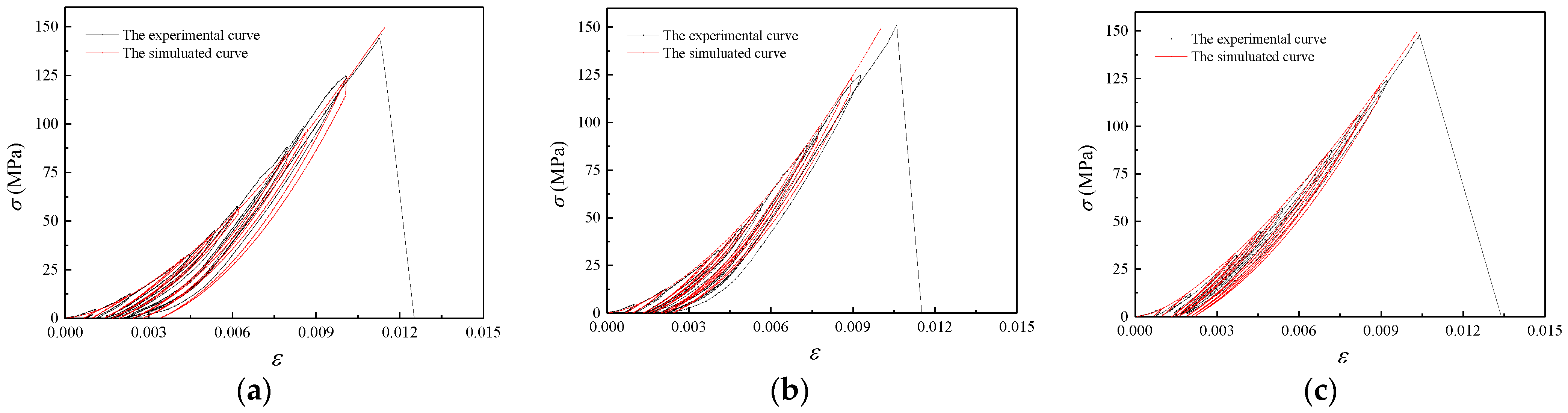
4.2. Results
5. Conclusions
- (1)
- By combining the uniaxial cyclic compression on the granite sample with AE monitoring, it is found that the loading process exhibits both elastic deformation and plastic deformation regardless of the stage that the rock sample is currently in. In addition, the mechanical behaviors during the unloading process and reloading process before the Kaiser point are primarily elastic deformation, accompanied by the obstruction of friction between inner cracks. When the loading exceeds the Kaiser point, the plastic strain will continue to develop.
- (2)
- The cyclic behavior of granite is simulated by a proposed comprehensive body that consists of an elastic element Ee, a plastic element Ep, and a friction element Ef, in which Ef is connected in parallel with the serial combination of Ee and Ep. The opposite effect of Ef during the unloading process and reloading process produces the massing effect, and the plastic deformation during reloading is prompted prior to the historical maximum stress, which brings about the ratcheting effect and Felicity effect.
- (3)
- In terms of hard rock such as granite, the elasticity reflected by the tangential modulus is affected by elastic strain and plastic strain. The elastic strain dominates the variation process of elasticity, while plastic strain determines the lower limit. Specifically, the tangential modulus of the elastic element exhibits a linear positive correlation with elastic strain, while the lower limit decreases linearly with the plastic strain. In addition, the plastic deformation grows from fast to slow throughout the deformation process, which can be simulated by the Logistic equation.
- (4)
- The proposed model and corresponding parameters are validated by comparison with the experimental stress–strain curves under three different conditions, and the strain (peak strain and residual strain in each cycle) differences between simulated results and experimental results for different conditions are basically less than 18%, 6%, and 13%, respectively, which strengthens the reliability. In addition, it is worth noting that even though the proposed model holds for the uniaxial compression case, the modeling flow could be extended to other applications, such as triaxial compression, and this paper provides a reference for future research on the cyclic behavior of other materials.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bagde, M.; Petroš, V. Fatigue properties of intact sandstone samples subjected to dynamic uniaxial cyclical loading. Int. J. Rock Mech. Min. Sci. 2005, 42, 237–250. [Google Scholar] [CrossRef]
- Chen, H.; Cong, T.N.; Yang, W.; Tan, C.; Li, Y.; Ding, Y. Progress in electrical energy storage system: A critical review. Prog. Nat. Sci. 2009, 19, 291–312. [Google Scholar] [CrossRef]
- Li, C.; Liu, N.; Liu, W.; Feng, R. Study on Characteristics of Energy Storage and Acoustic Emission of Rock under Different Moisture Content. Sustainability 2021, 13, 1041. [Google Scholar] [CrossRef]
- Gong, F.-Q.; Wu, C.; Luo, S.; Yan, J.-Y. Load–unload response ratio characteristics of rock materials and their application in prediction of rockburst proneness. Bull. Eng. Geol. Environ. 2019, 78, 5445–5466. [Google Scholar] [CrossRef]
- Zhu, X.; Li, Y.; Wang, C.; Sun, X.; Liu, Z. Deformation Failure Characteristics and Loading Rate Effect of Sandstone Under Uniaxial Cyclic Loading and Unloading. Geotech. Geol. Eng. 2019, 37, 1147–1154. [Google Scholar] [CrossRef]
- Imre, B.; Räbsamen, S.; Springman, S.M. A coefficient of restitution of rock materials. Comput. Geosci. 2008, 34, 339–350. [Google Scholar] [CrossRef]
- Chen, Y.P.; Wang, S.J.; Wang, E.Z. Quantitative Study on Stress-strain Hysteretic Behaviors in Rocks. Chin. J. Rock Mech. Eng. 2007, 26, 4066–4073. [Google Scholar]
- Wen, T.; Tang, H.; Wang, Y.; Ma, J.; Fan, Z. Mechanical Characteristics and Energy Evolution Laws for Red Bed Rock of Badong Formation under Different Stress Paths. Adv. Civ. Eng. 2019, 2019, 8529329. [Google Scholar] [CrossRef]
- Xi, D.Y.; Chen, Y.P.; Tao, Y.Z.; Liu, Y.C. Nonlinear Elastic Hysteric Characteristics of Rocks. Chin. J. Rock Mech. Eng. 2006, 25, 1086–1093. [Google Scholar]
- Meng, Q.; Zhang, M.; Han, L.; Pu, H.; Nie, T. Effects of Acoustic Emission and Energy Evolution of Rock Specimens Under the Uniaxial Cyclic Loading and Unloading Compression. Rock Mech. Rock Eng. 2016, 49, 3873–3886. [Google Scholar] [CrossRef]
- Meng, Q.; Zhang, M.; Han, L.; Pu, H.; Chen, Y. Acoustic Emission Characteristics of Red Sandstone Specimens Under Uniaxial Cyclic Loading and Unloading Compression. Rock Mech. Rock Eng. 2018, 51, 969–988. [Google Scholar] [CrossRef]
- Wang, T.; Wang, C.; Xue, F.; Wang, L.; Teshome, B.H.; Xue, M. Acoustic Emission Characteristics and Energy Evolution of Red Sandstone Samples under Cyclic Loading and Unloading. Shock Vib. 2021, 2021, 8849137. [Google Scholar] [CrossRef]
- Li, Y.; Zhao, T.; Li, Y.; Chen, Y. A five-parameter constitutive model for hysteresis shearing and energy dissipation of rock joints. Int. J. Min. Sci. Technol. 2022, in press. [CrossRef]
- McCall, K.R.; Guyer, R.A. Equation of state and wave propagation in hysteretic nonlinear elastic materials. Geophys. Res. 1994, 99, 23887–23897. [Google Scholar] [CrossRef]
- Scalerandi, M.; Gliozzi, A.; Idjimarene, S. Power laws behavior in multi-state elastic models with different constraints in the statistical distribution of elements. Commun. Nonlinear Sci. Numer. Simul. 2014, 19, 3628–3641. [Google Scholar] [CrossRef]
- Chen, X.; Huang, Y.; Chen, C.; Lu, J.; Fan, X. Experimental study and analytical modeling on hysteresis behavior of plain concrete in uniaxial cyclic tension. Int. J. Fatigue 2017, 96, 261–269. [Google Scholar] [CrossRef]
- Wang, X.; Song, L.; Gao, X.; Chang, X. Effect of loading rate on the nonlinear elastic response of concrete. Eur. J. Environ. Civ. Eng. 2021, 25, 909–923. [Google Scholar] [CrossRef]
- Hua, D.; Jiang, Q. Three-Dimensional Modeling of Hysteresis in Rocks. J. Geophys. Res. Solid Earth 2022, 127, e2021JB023230. [Google Scholar] [CrossRef]
- Hashiguchi, K. Generalized plastic flow rule. Int. J. Plast. 2005, 21, 321–351. [Google Scholar] [CrossRef]
- Kong, L.; Zheng, Y.R.; Yao, Y.P. Subloading surface cyclic plastic model for soil based on Generalized plasticity (I): Theory and model. Rock Soil Mech. 2003, 24, 141–145. [Google Scholar] [CrossRef]
- Kong, L.; Zheng, Y.R.; Yao, Y.P. Subloading surface cyclic plastic model for soil based on Generalized plasticity (II): Constitutive equation and identification. Rock Soil Mech. 2003, 24, 349–354. [Google Scholar] [CrossRef]
- Zhou, Y.Q.; Sheng, Q.; Zhu, Z.Q.; Fu, X.D. Subloading surface model for rock based on modified Drucker-Prager criterion. Rock Soil Mech. 2017, 38, 400–418. [Google Scholar] [CrossRef]
- Zhou, Y.; Sheng, Q.; Li, N.; Fu, X.; Zhang, Z.; Gao, L. A constitutive model for rock materials subjected to triaxial cyclic compression. Mech. Mater. 2020, 144, 103341. [Google Scholar] [CrossRef]
- Valanis, K.C.; Fan, J. A numerical algorithm for endochronic plasticity and comparison with experiment. Comput. Struct. 1984, 19, 717–724. [Google Scholar] [CrossRef]
- Cerfontaine, B.; Charlier, R.; Collin, F.; Taiebat, M. Validation of a New Elastoplastic Constitutive Model Dedicated to the Cyclic Behaviour of Brittle Rock Materials. Rock Mech. Rock Eng. 2017, 50, 2677–2694. [Google Scholar] [CrossRef]
- Liu, D.; He, M.; Cai, M. A damage model for modeling the complete stress–strain relations of brittle rocks under uniaxial compression. Int. J. Damage Mech. 2018, 27, 1000–1019. [Google Scholar] [CrossRef]
- Wu, Y.L.; Chen, J.; Zeng, S.M. The Acoustic Emission Technique Research on Dynamic Damage Characteristics of the Coal Rock. Procedia Eng. 2011, 26, 1076–1082. [Google Scholar] [CrossRef][Green Version]
- Kong, X.; Wang, E.; He, X.; Zhao, E.; Zhao, C. Mechanical characteristics and dynamic damage evolution mechanism of coal samples in compressive loading experiments. Eng. Fract. Mech. 2019, 210, 160–169. [Google Scholar] [CrossRef]
- Xiao, J.-Q.; Ding, D.-X.; Jiang, F.-L.; Xu, G. Fatigue damage variable and evolution of rock subjected to cyclic loading. Int. J. Rock Mech. Min. Sci. 2010, 47, 461–468. [Google Scholar] [CrossRef]
- Liu, E.; He, S. Effects of cyclic dynamic loading on the mechanical properties of intact rock samples under confining pressure conditions. Eng. Geol. 2012, 125, 81–91. [Google Scholar] [CrossRef]
- Wang, Z.; Li, S.; Qiao, L.; Zhao, J. Fatigue Behavior of Granite Subjected to Cyclic Loading Under Triaxial Compression Condition. Rock Mech. Rock Eng. 2013, 46, 1603–1615. [Google Scholar] [CrossRef]
- Chen, Y.; Zuo, J.; Guo, B.; Guo, W. Effect of cyclic loading on mechanical and ultrasonic properties of granite from Maluanshan Tunnel. Bull. Eng. Geol. Environ. 2020, 79, 299–311. [Google Scholar] [CrossRef]
- Li, X.W.; Yao, Z.S.; Huang, X.W.; Liu, Z.X.; Zhao, X.; Mu, K.H. Investigation of deformation and failure characteristics and energy evolution of sandstone under cyclic loading and unloading. Rock Soil Mech. 2021, 42, 1693–1704. [Google Scholar]
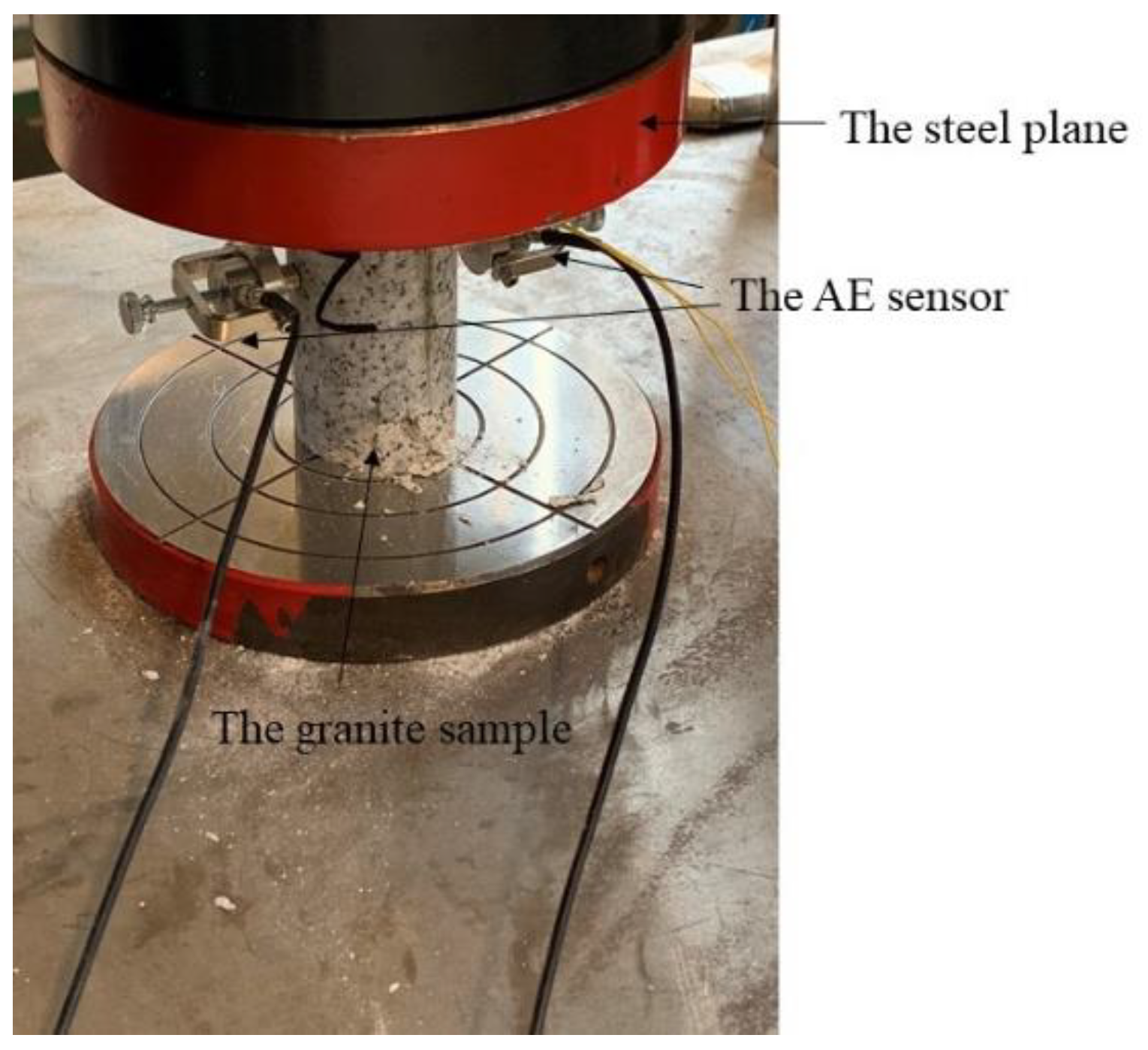


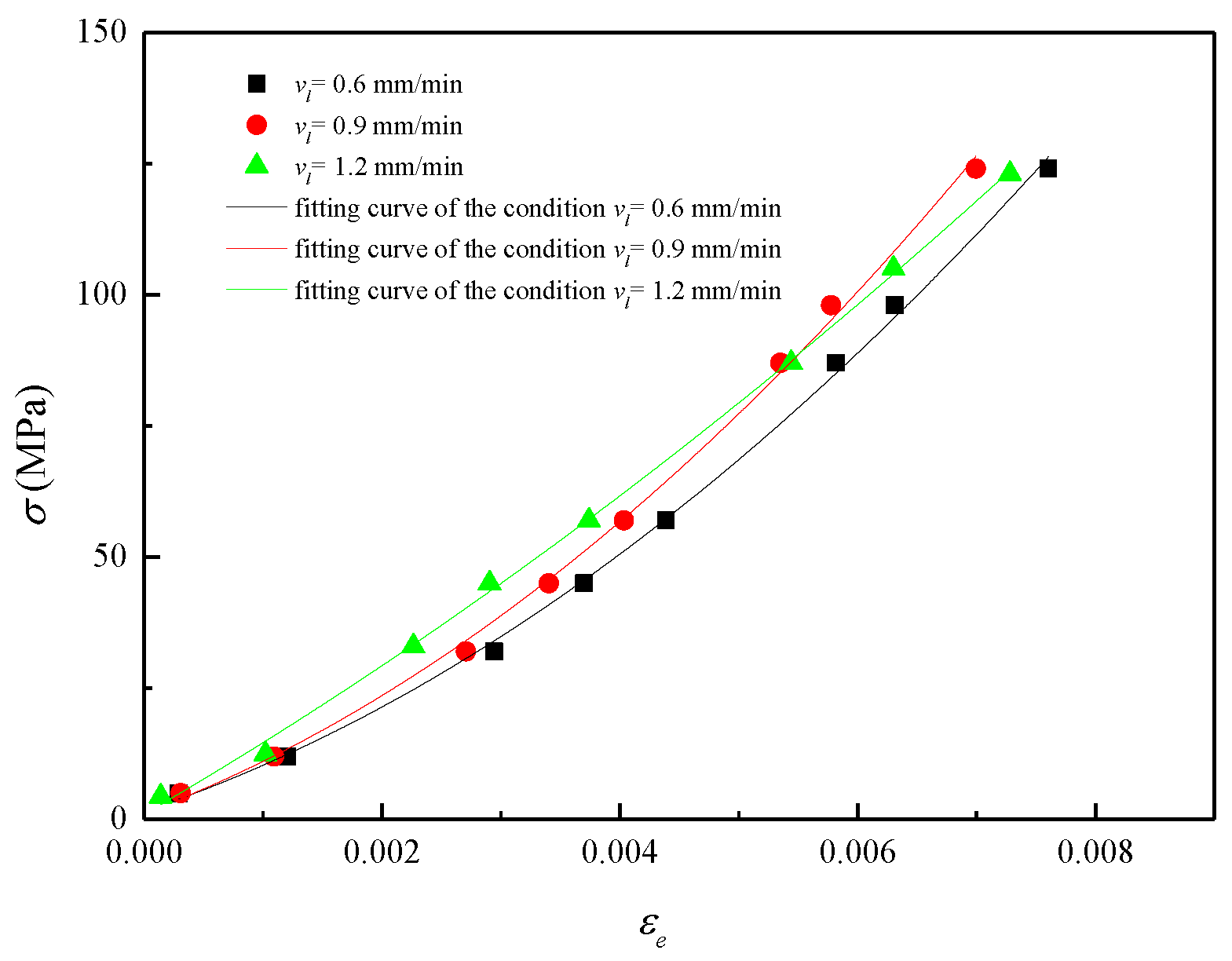
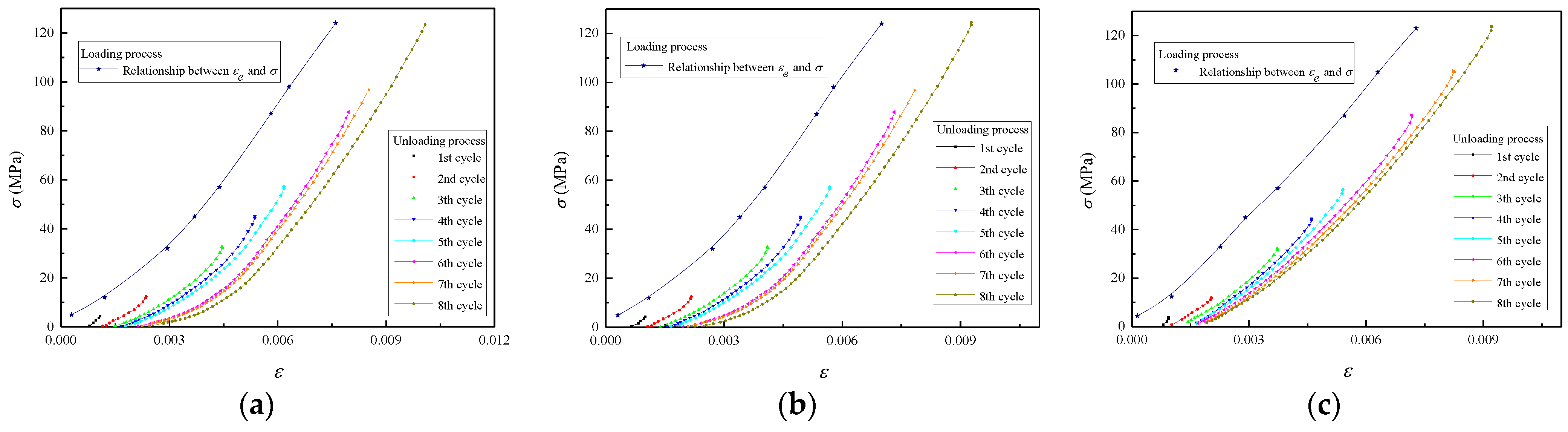
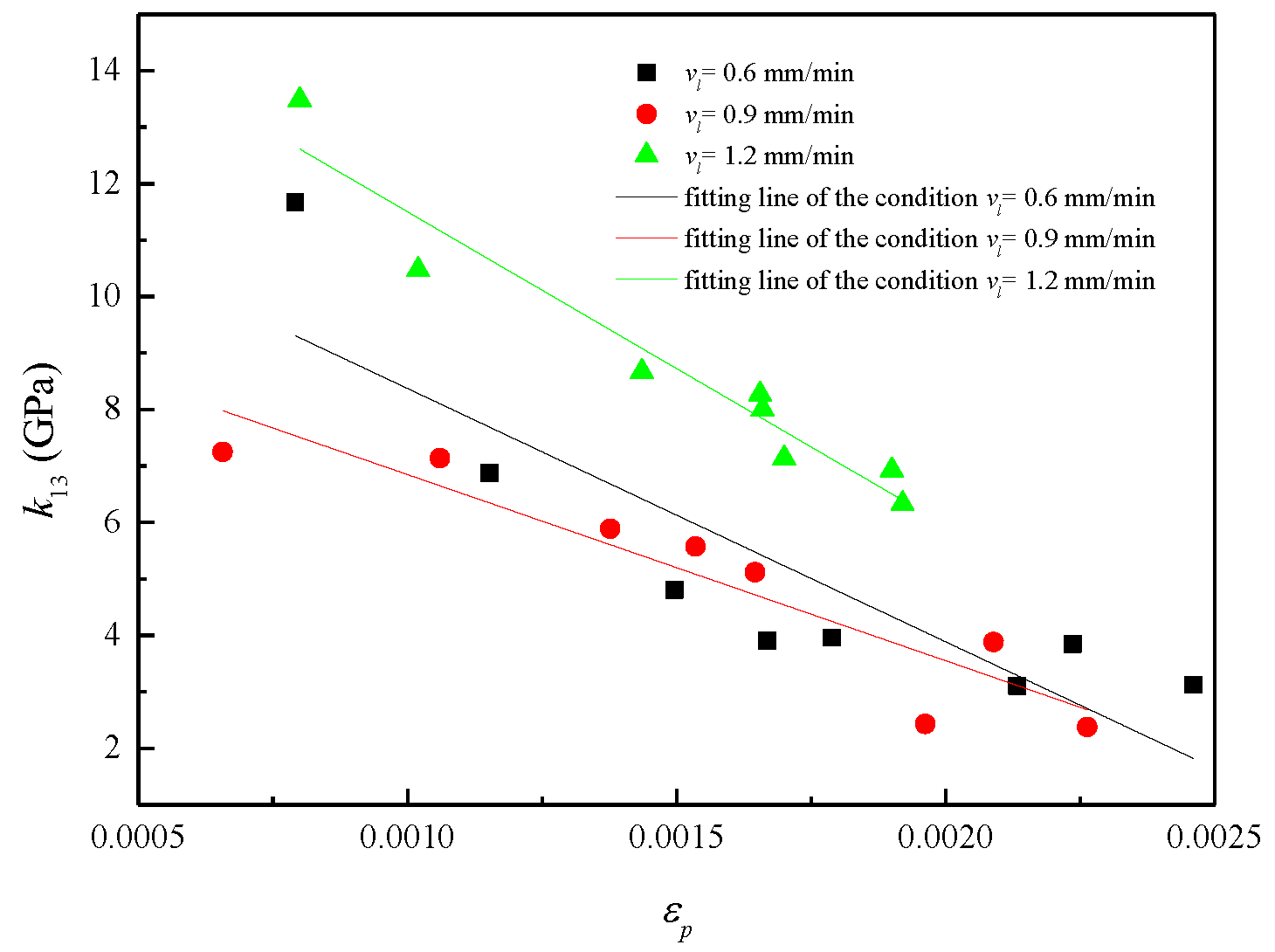

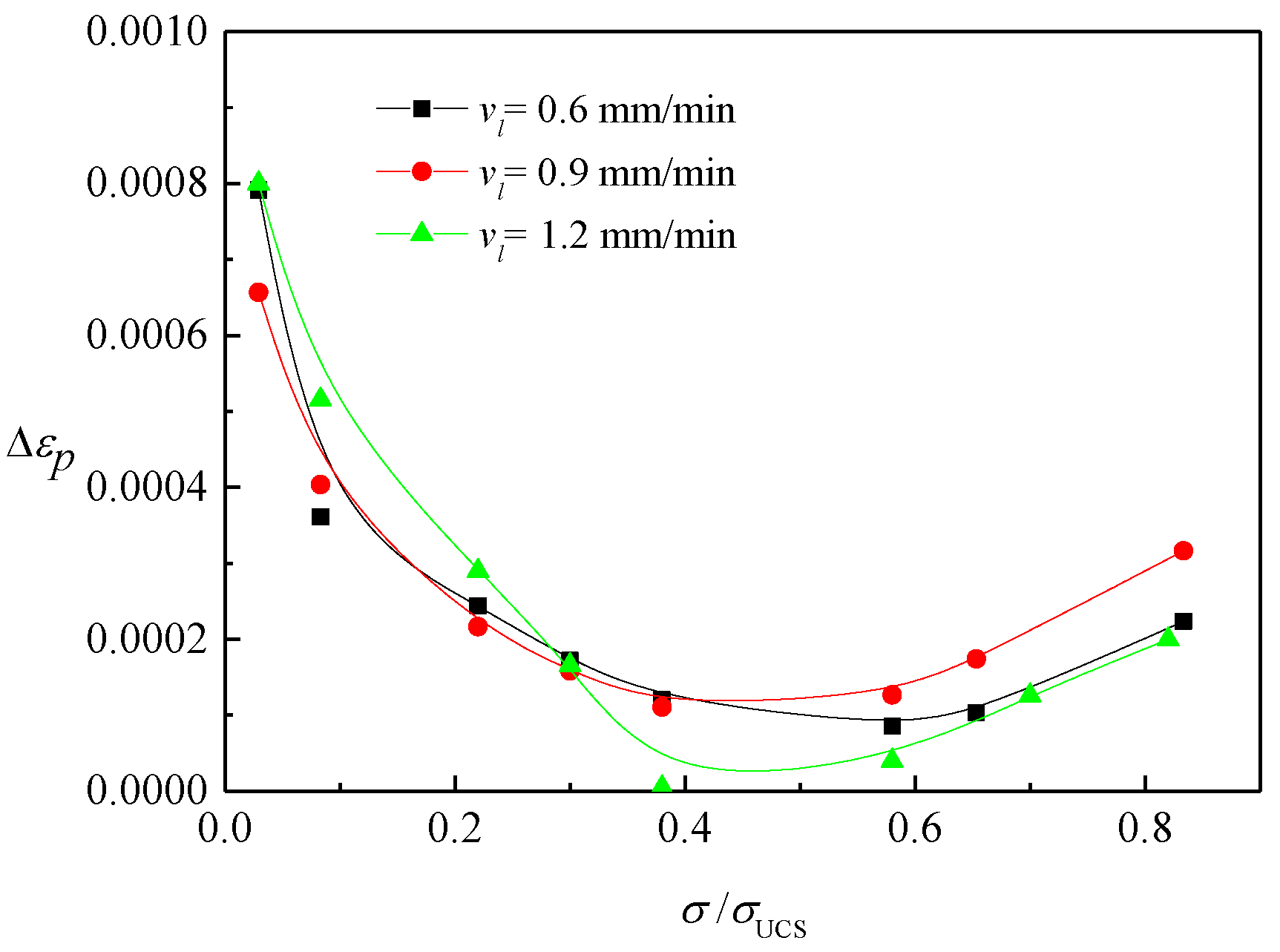



| Quartz (%) | K-Feldspar (%) | Plagioclase (%) | Biotite (%) | Others (%) |
|---|---|---|---|---|
| 35.11 | 28.21 | 30.64 | 5.45 | 0.59 |
| Water Content (%) | Density (g/cm3) | Dry Density (g/cm3) | Velocity of Longitudinal Waves (m/s) | Schmidt Hardness | UCS (MPa) | Uniaxial Tensile Strength (MPa) |
|---|---|---|---|---|---|---|
| 0.09 | 2.7 | 2.6 | 3077 | 69 | 150.0 | 4.8 |
| k11 | k12 | R2 | |
|---|---|---|---|
| vl = 0.6 mm/min | 8391.26 | 1,075,623.84 | 0.9977 |
| vl = 0.9 mm/min | 9152.61 | 1,270,575.23 | 0.9974 |
| vl = 1.2 mm/min | 13,657.93 | 452,678.39 | 0.9986 |
| k13’ | b13’ | R | |
|---|---|---|---|
| vl = 0.6 mm/min | −4,487,920 | 12,859.92 | −0.8751 |
| vl = 0.9 mm/min | −3,298,220 | 10,145.63 | −0.9333 |
| vl = 1.2 mm/min | −5,555,450 | 17,057.99 | −0.9681 |
| k2 | a | b | R2 | |
|---|---|---|---|---|
| vl = 0.6 mm/min | 183.34 | 5.02 | 2337.40 | 0.9967 |
| vl = 0.9 mm/min | 192.88 | 4.87 | 2401.32 | 0.9944 |
| vl = 1.2 mm/min | 702.44 | 7.16 | 2896.71 | 0.9244 |
| Cycle 2 | Cycle 3 | Cycle 4 | Cycle 5 | Cycle 6 | Cycle 7 | Cycle 8 | Cycle 9 | |
|---|---|---|---|---|---|---|---|---|
| vl = 0.6 mm/min | 1.16 | 0.98 | 0.97 | 0.95 | 0.93 | 0.91 | 0.89 | 0.87 |
| vl = 0.9 mm/min | 1.13 | 0.98 | 0.97 | 0.97 | 0.96 | 0.95 | 0.94 | 0.91 |
| vl = 1.2 mm/min | 1.08 | 0.98 | 0.98 | 0.97 | 0.97 | 0.95 | 0.95 | 0.93 |
| k11 | k12 | k2 | a | b | d | k3 | |
|---|---|---|---|---|---|---|---|
| vl = 0.6 mm/min | 7000, 8000, 9000 | 9 × 105, 1.0 × 106, 1.1 × 106 | 150, 200, 250 | 3, 5, 7 | 2000, 3000, 4000 | 0.0001, 0.0005, 0.0009 | 10, 20, 30 |
| vl = 0.9 mm/min | 7000, 8000, 9000 | 1.1 × 106, 1.2 × 106, 1.3 × 106 | 150, 200, 250 | 3, 5, 7 | 2000, 3000, 4000 | 0.0001, 0.0005, 0.0009 | 10, 20, 30 |
| vl = 1.2 mm/min | 12,000, 13,000, 14,000 | 4 × 105, 5 × 105, 6 × 105 | 650, 700, 750 | 5, 7, 9 | 2000, 3000, 4000 | 0.0001, 0.0005, 0.0009 | 10, 20, 30 |
| k11 | k12 | k2 | a | b | d | k3 | |
|---|---|---|---|---|---|---|---|
| vl = 0.6 mm/min | 7279 | 1,039,693 | 164.6 | 5.7 | 3356.2 | 0.00051 | 17.8 |
| vl = 0.9 mm/min | 8509 | 1,142,430 | 241.3 | 6.2 | 3573.3 | 0.00024 | 15.2 |
| vl = 1.2 mm/min | 12,237 | 569,263 | 702 | 7.2 | 3300 | 0.00013 | 13.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fu, H.; Li, J.; Li, G.; Li, D. Hysteresis Behavior Modeling of Hard Rock Based on the Mechanism and Relevant Characteristics. Sustainability 2022, 14, 10412. https://doi.org/10.3390/su141610412
Fu H, Li J, Li G, Li D. Hysteresis Behavior Modeling of Hard Rock Based on the Mechanism and Relevant Characteristics. Sustainability. 2022; 14(16):10412. https://doi.org/10.3390/su141610412
Chicago/Turabian StyleFu, Helin, Jie Li, Guoliang Li, and Dongping Li. 2022. "Hysteresis Behavior Modeling of Hard Rock Based on the Mechanism and Relevant Characteristics" Sustainability 14, no. 16: 10412. https://doi.org/10.3390/su141610412
APA StyleFu, H., Li, J., Li, G., & Li, D. (2022). Hysteresis Behavior Modeling of Hard Rock Based on the Mechanism and Relevant Characteristics. Sustainability, 14(16), 10412. https://doi.org/10.3390/su141610412





