Numerical Simulation of Fracture Propagation during Refracturing
Abstract
:1. Introduction
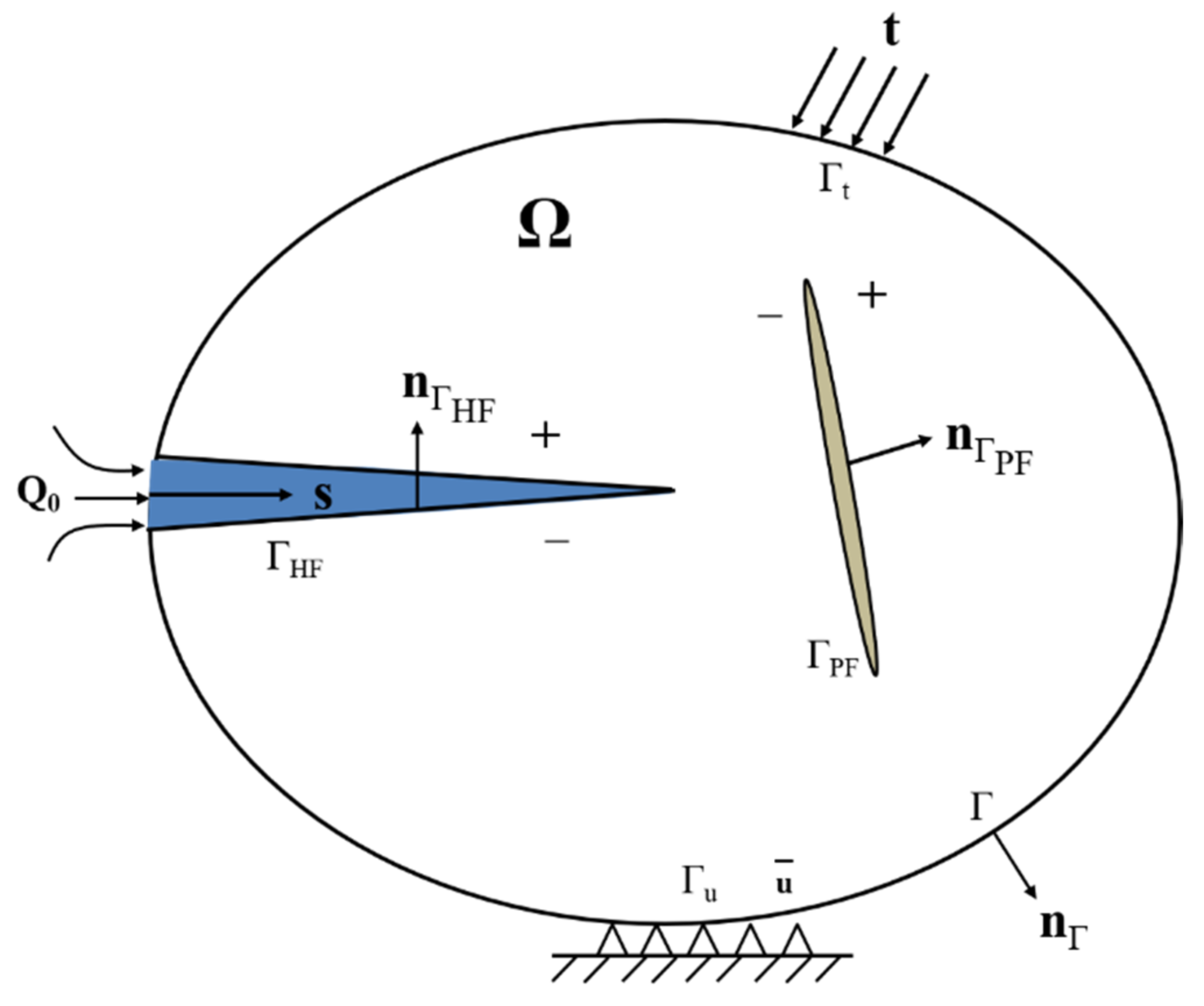
2. Method and Theory
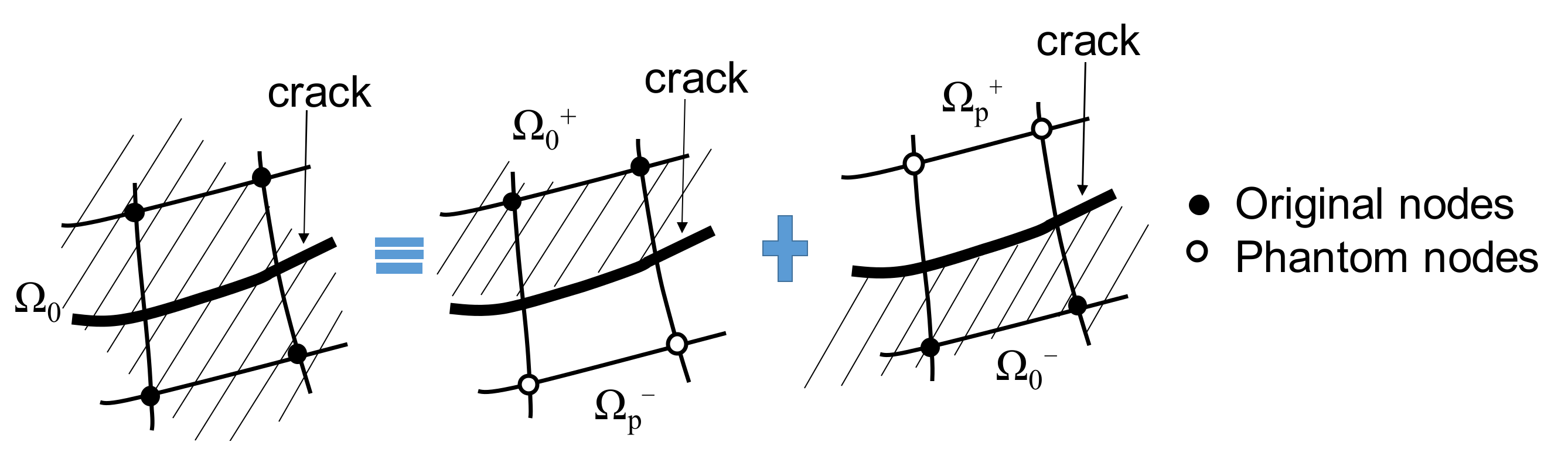
2.1. Enrichment Displacement Functions
2.2. Fluid Flow within HFs
2.3. Traction–Separation Constitutive Behavior
3. Numerical Simulation
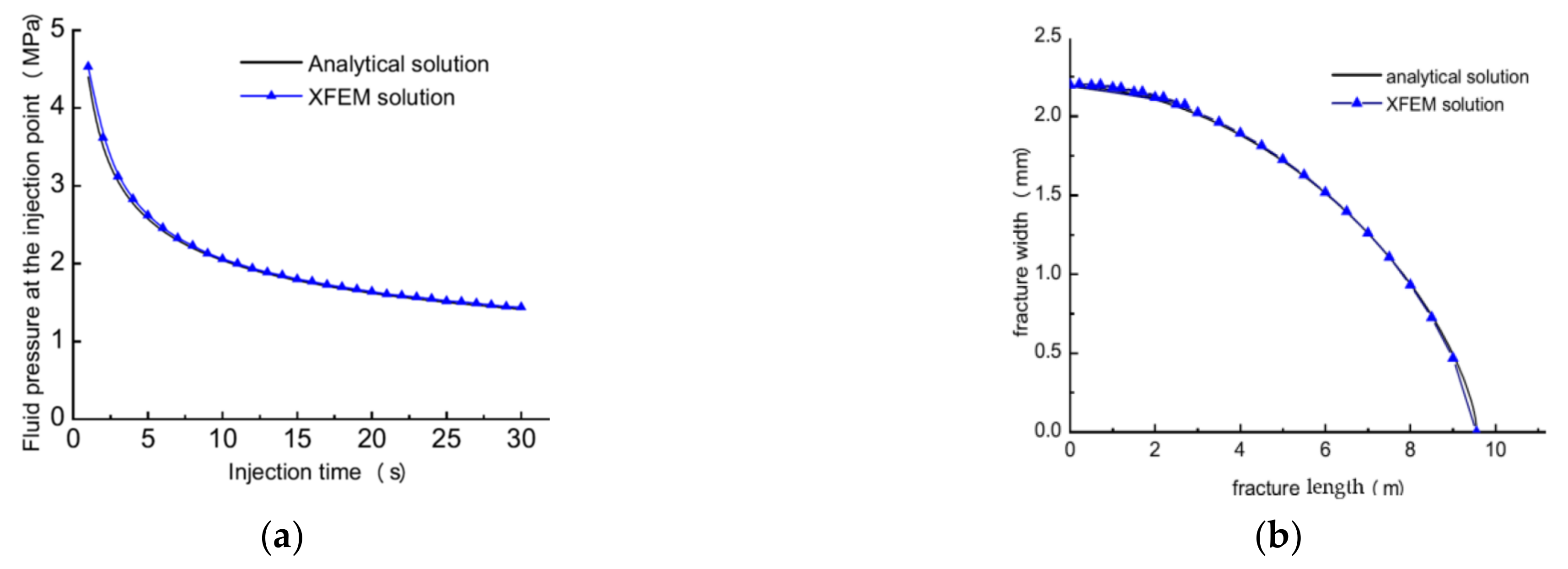
3.1. Model Verification
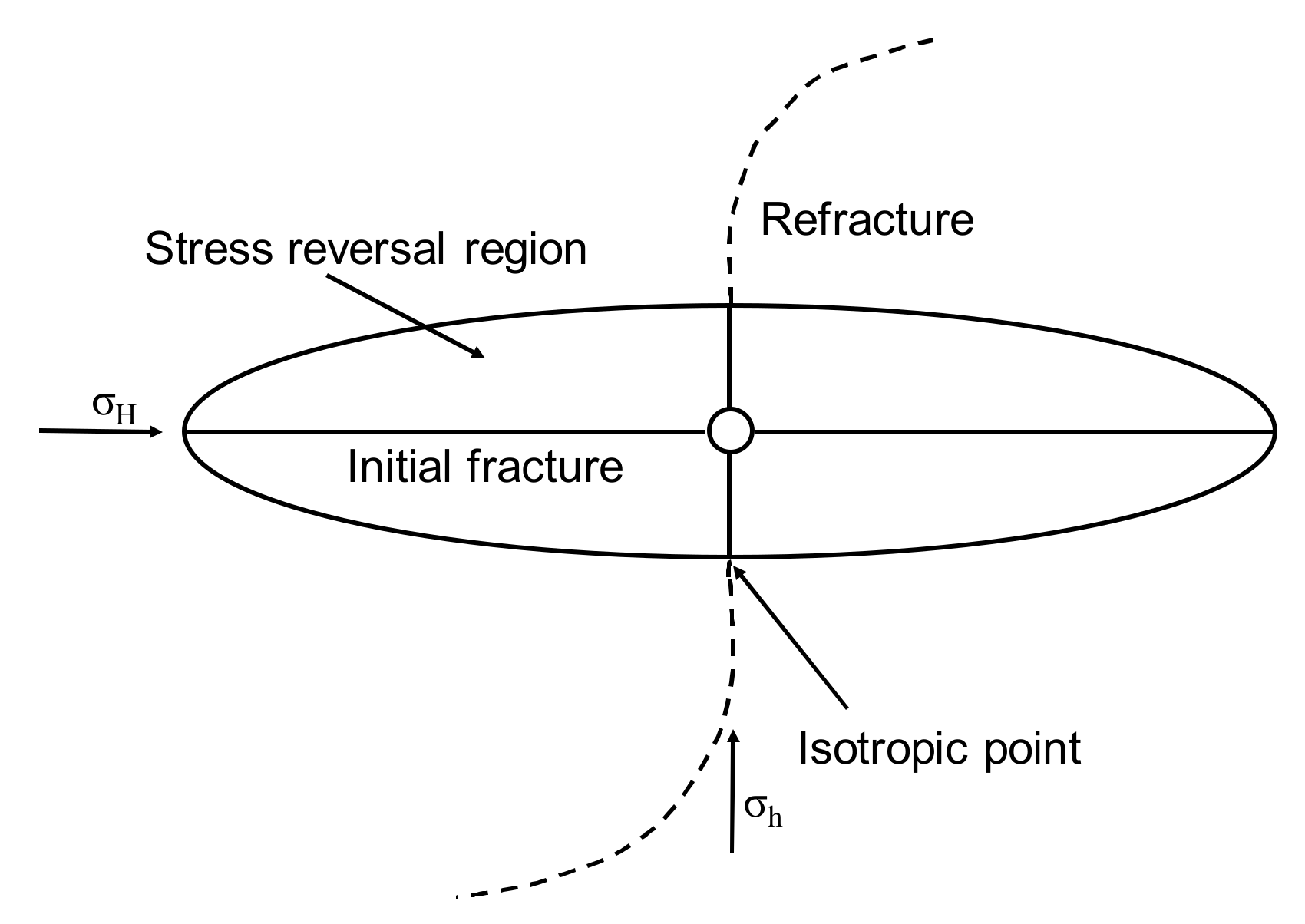
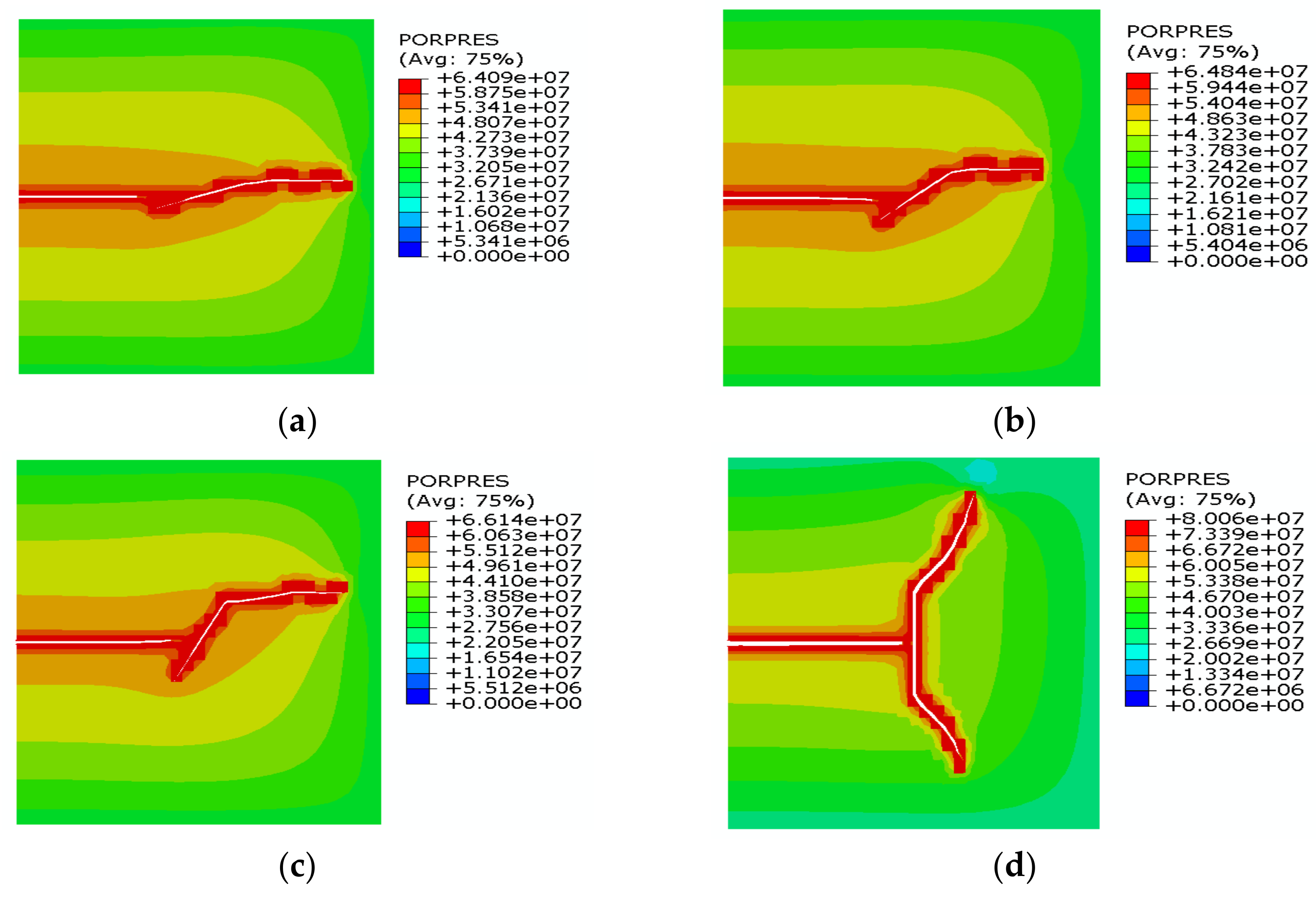
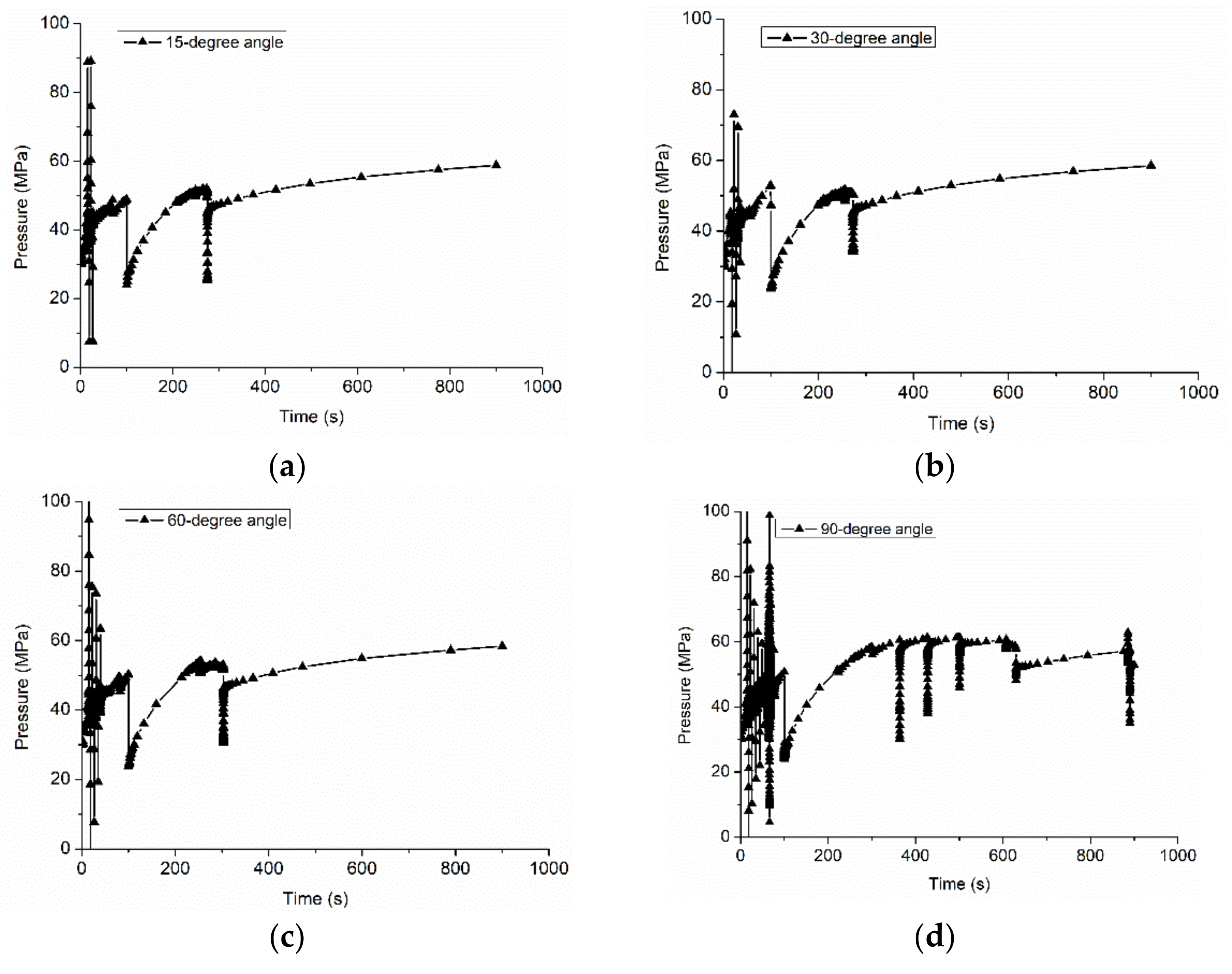
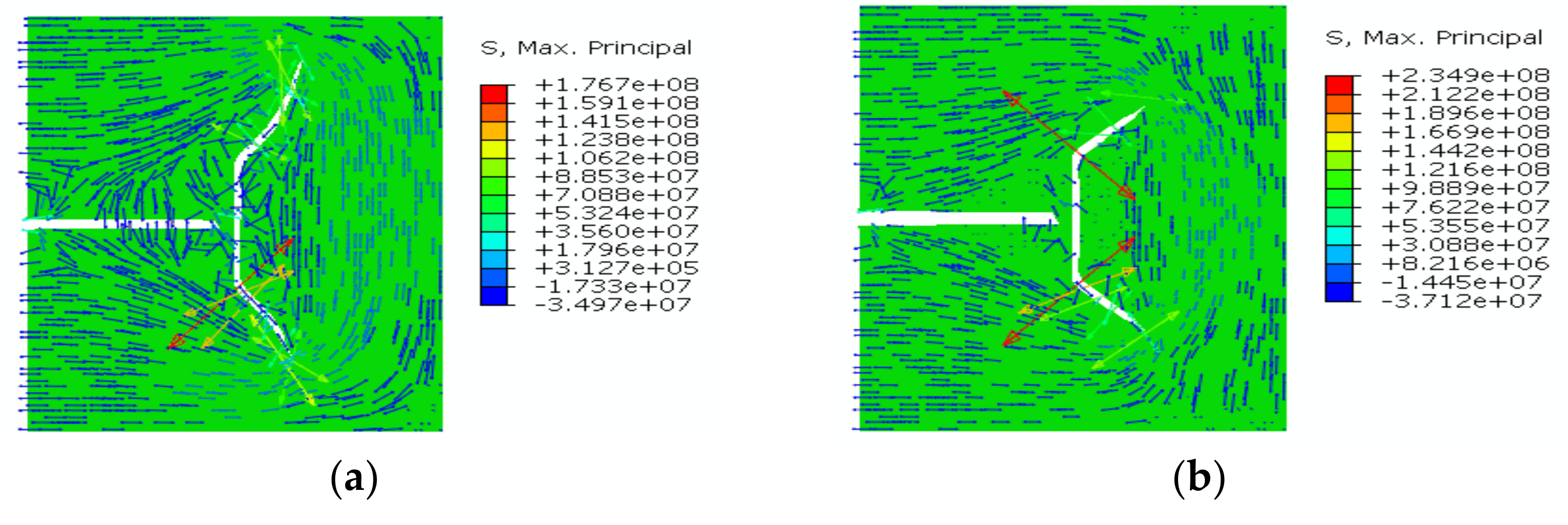
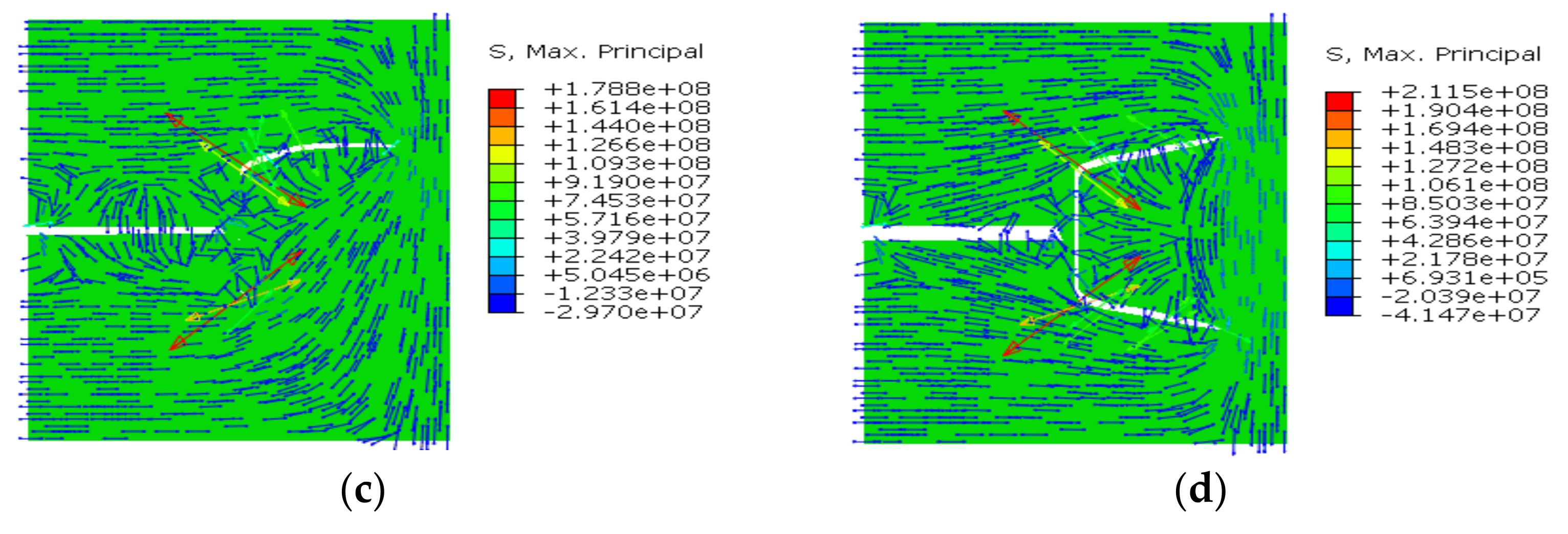
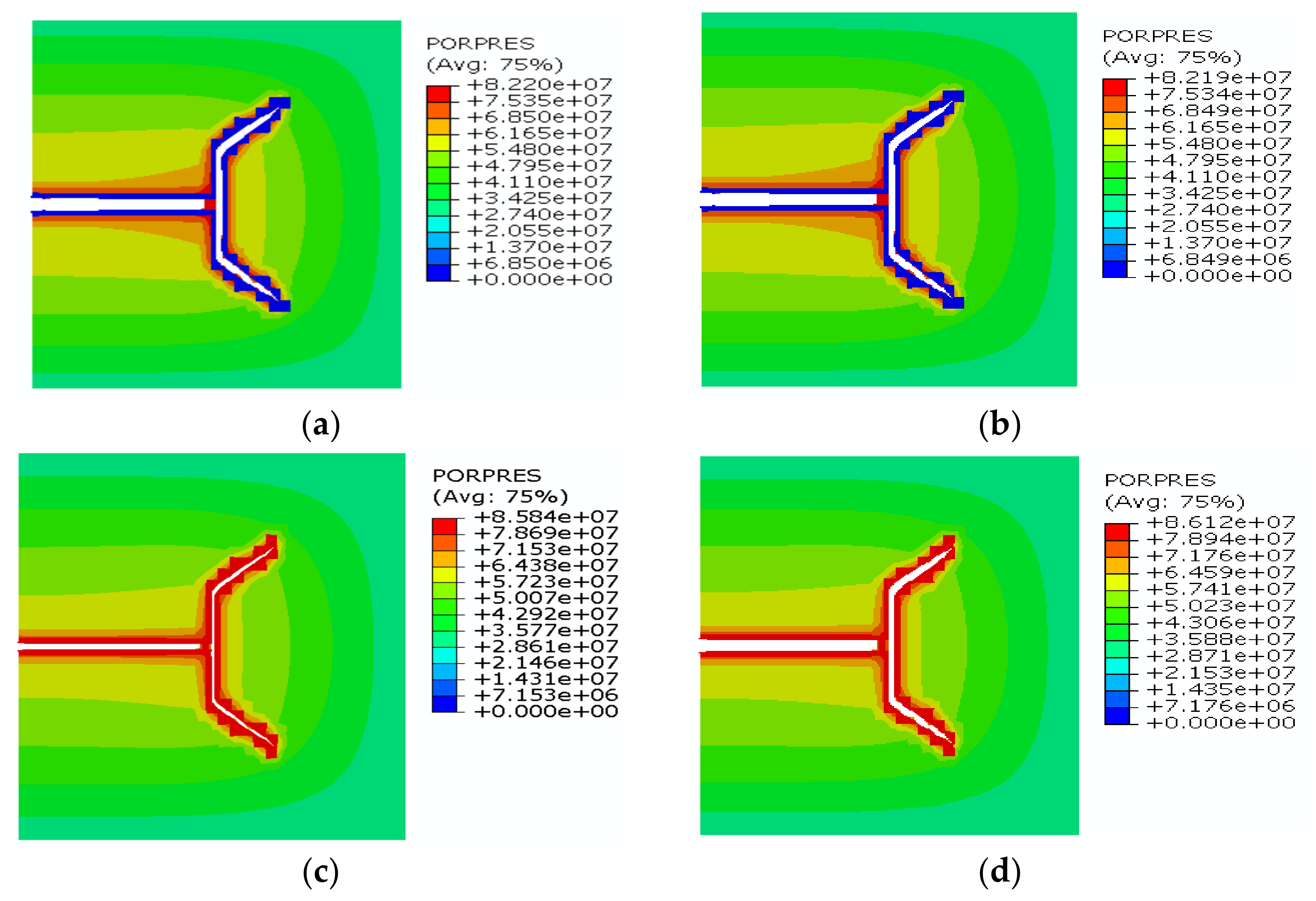
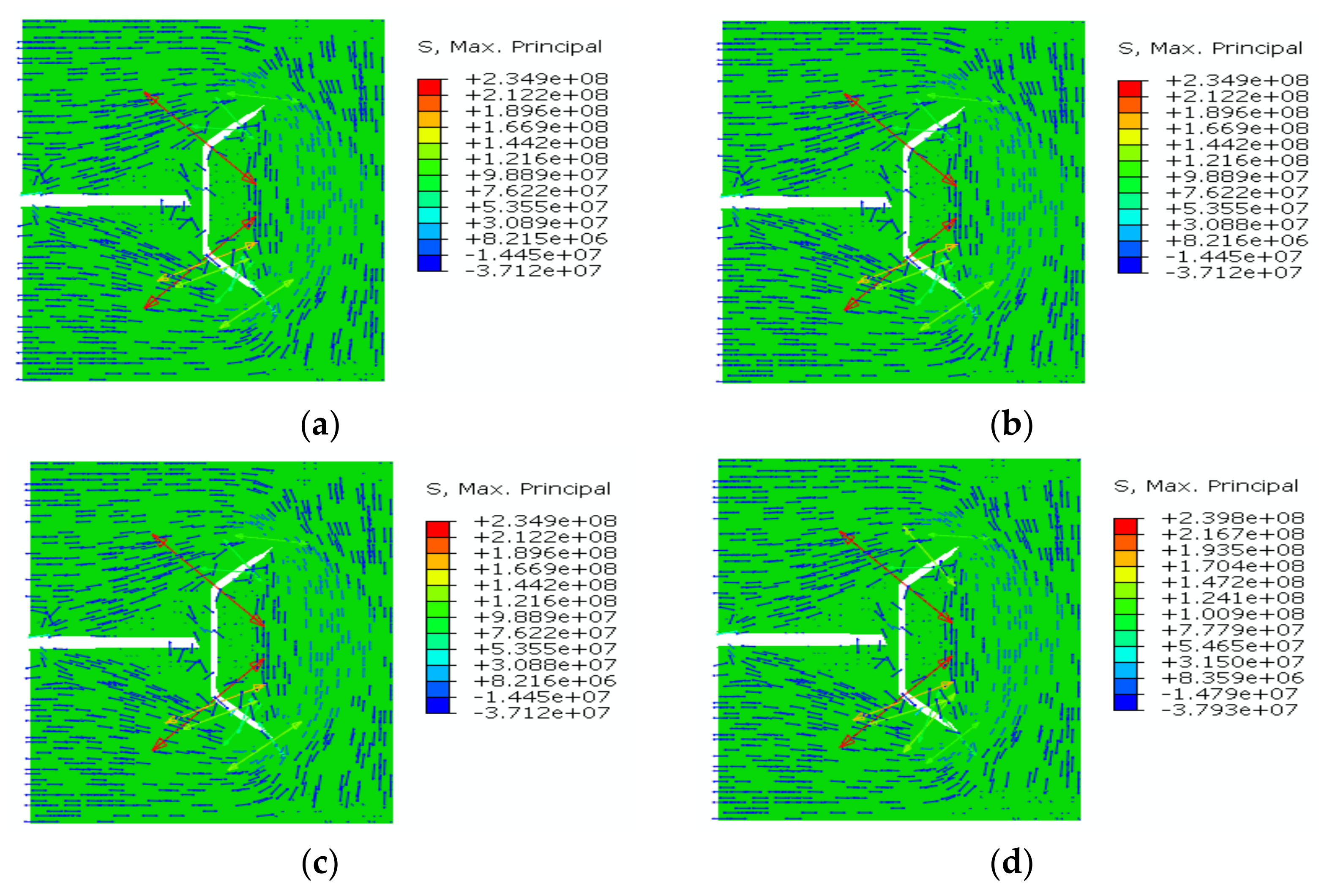
3.2. The Impact of Approaching Angle on Fracture Reorientation
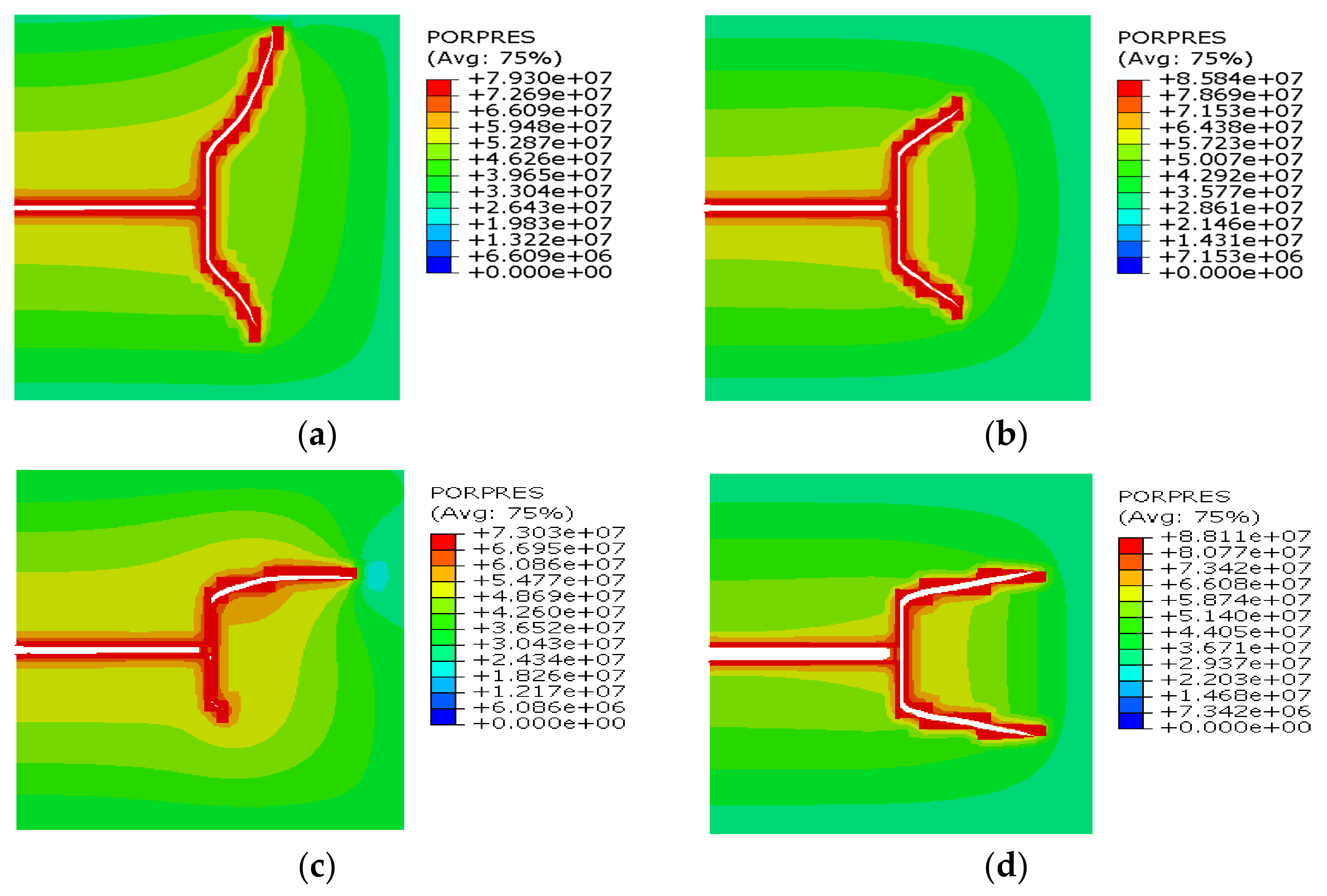
3.3. The Impact of Stress Difference on Fracture Reorientation
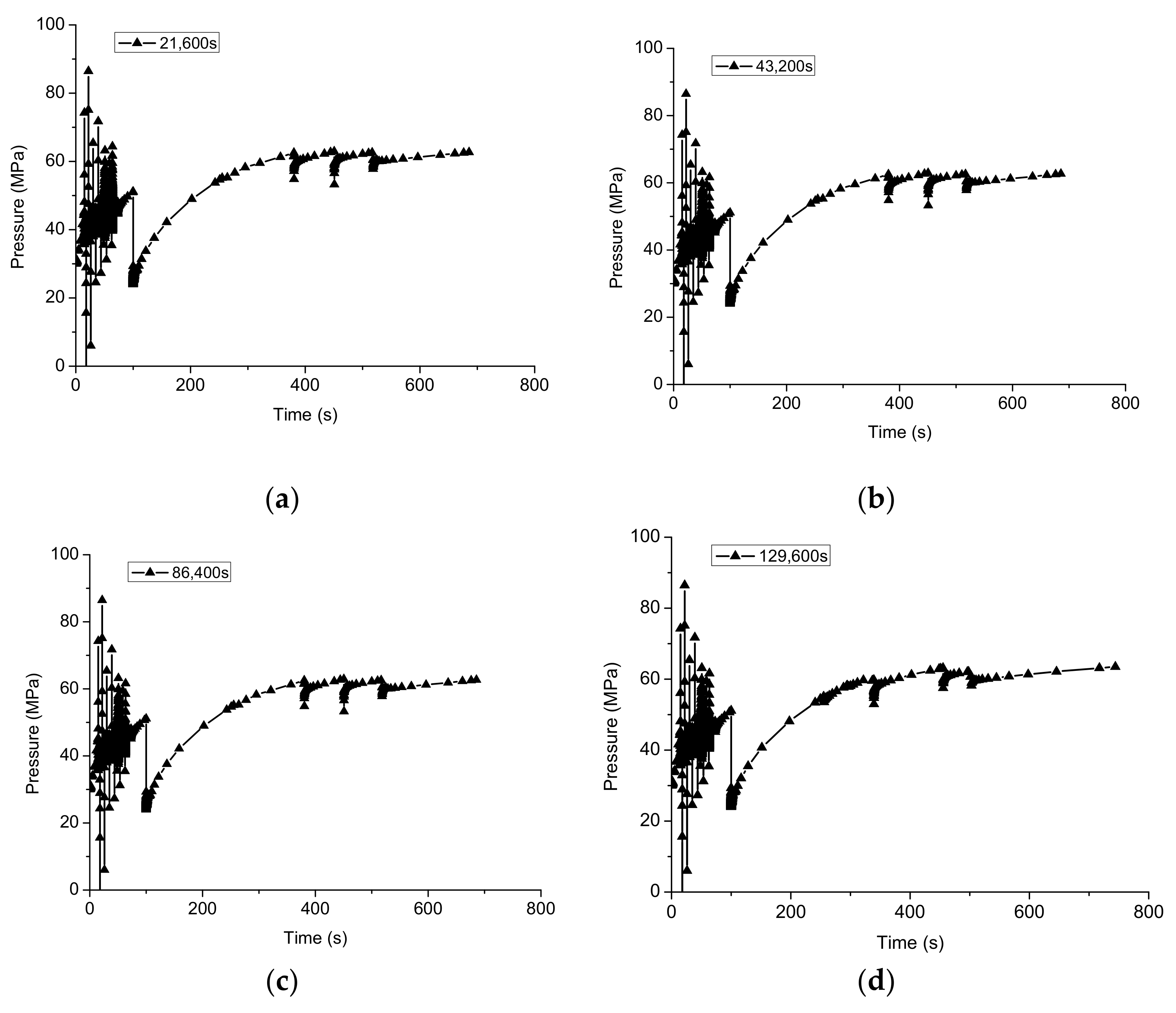
3.4. The Impact of Production Time on Fracture Reorientation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Palmer, I.D. Induced Stresses Due to Propped Hydraulic Fracture in Coalbed Methane Wells. In Proceedings of the Society of Petroleum Engineers, Oklahoma City, OK, USA, 1 January 1993. [Google Scholar]
- Too, J.L.; Cheng, A.; Linga, P. Fracturing Methane Hydrate in Sand: A Review of the Current Status. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 20 March 2018. [Google Scholar]
- Gale, J.F.W.; Laubach, S.E.; Olson, J.E.; Eichhubl, P.; Fall, A. Natural Fractures in Shale: A Review and New Observations. AAPG Bull. 2014, 98, 2165–2216. [Google Scholar] [CrossRef]
- Economides, M.J.; Nolte, K.G. (Eds.) Reservoir Stimulation, 3rd ed.; Wiley: Chichester, UK; New York, NY, USA, 2000; ISBN 978-0-471-49192-7. [Google Scholar]
- Zhang, Q.; Hou, B.; Lin, B.; Liu, X.; Gao, Y. Integration of Discrete Fracture Reconstruction and Dual Porosity/Dual Permeability Models for Gas Production Analysis in a Deformable Fractured Shale Reservoir. J. Nat. Gas Sci. Eng. 2021, 93, 104028. [Google Scholar] [CrossRef]
- Hou, B.; Chang, Z.; Fu, W.; Muhadasi, Y.; Chen, M. Fracture Initiation and Propagation in a Deep Shale Gas Reservoir Subject to an Alternating-Fluid-Injection Hydraulic-Fracturing Treatment. SPE J. 2019, 24, 1839–1855. [Google Scholar] [CrossRef]
- Dahi-Taleghani, A. Fracture Re-Initiation As a Possible Branching Mechanism during Hydraulic Fracturing. In Proceedings of the American Rock Mechanics Association, Salt Lake City, UT, USA, 1 January 2010. [Google Scholar]
- Dahi-Taleghani, A.; Olson, J.E. How Natural Fractures Could Affect Hydraulic-Fracture Geometry. SPE J. 2014, 19, 161–171. [Google Scholar] [CrossRef]
- Dahi-Taleghani, A.; Olson, J.E. Numerical Modeling of Multistranded-Hydraulic-Fracture Propagation: Accounting for the Interaction between Induced and Natural Fractures. SPE J. 2011, 16, 575–581. [Google Scholar] [CrossRef]
- Maxwell, S.C.; Urbancic, T.I.; Steinsberger, N.; Zinno, R. Microseismic Imaging of Hydraulic Fracture Complexity in the Barnett Shale. In Proceedings of the Society of Petroleum Engineers, San Antonio, TX, USA, 1 January 2002. [Google Scholar]
- Yu, H.; Taleghani, A.D.; Lian, Z. On How Pumping Hesitations May Improve Complexity of Hydraulic Fractures, a Simulation Study. Fuel 2019, 249, 294–308. [Google Scholar] [CrossRef]
- Yu, H.; Dahi Taleghani, A.; Lian, Z.; Lin, T. On How Asymmetric Stimulated Rock Volume in Shales May Impact Casing Integrity. Energy Sci. Eng. 2020, 8, 1524–1540. [Google Scholar] [CrossRef]
- Zhou, F.; Liu, Y.; Yang, X.; Zhang, F.; Xiong, C.; Liu, X. Case Study: YM204 Obtained High Petroleum Production by Acid Fracture Treatment Combining Fluid Diversion and Fracture Reorientation. In Proceedings of the Society of Petroleum Engineers, Scheveningen, The Netherlands, 1 January 2009. [Google Scholar]
- Palisch, T.T.; Vincent, M.C.; Handren, P.J. Slickwater Fracturing: Food for Thought. In Proceedings of the Society of Petroleum Engineers, Alexandria, VA, USA, 1 January 2008. [Google Scholar]
- Warpinski, N.R.; Branagan, P.T. Altered-Stress Fracturing. J. Pet. Technol. 1989, 41, 990–997. [Google Scholar] [CrossRef]
- Siebrits, E.; Elbel, J.L.; Detournay, E.; Detournay-Piette, C.; Christianson, M.; Robinson, B.M.; Diyashev, I.R. Parameters Affecting Azimuth and Length of a Secondary Fracture During a Refracture Treatment. In Proceedings of the Society of Petroleum Engineers, New Orleans, LA, USA, 1 January 1998. [Google Scholar]
- Roussel, N.P.; Sharma, M.M. Quantifying Transient Effects in Altered-Stress Refracturing of Vertical Wells. SPE J. 2010, 15, 770–782. [Google Scholar] [CrossRef]
- Sneddon, I.N.; Elliot, H.A. The Opening of a Griffith Crack under Internal Pressure. Q. Appl. Math. 1946, 4, 262–267. [Google Scholar] [CrossRef] [Green Version]
- Elbel, J.L.; Mack, M.G. Refracturing: Observations and Theories. In Proceedings of the Society of Petroleum Engineers, Oklahoma City, OK, USA, 1 January 1993. [Google Scholar]
- Li, X.; Wang, J.; Elsworth, D. Stress Redistribution and Fracture Propagation during Restimulation of Gas Shale Reservoirs. J. Pet. Sci. Eng. 2017, 154, 150–160. [Google Scholar] [CrossRef]
- Bruno, M.S.; Nakagawa, F.M. Pore Pressure Influence on Tensile Fracture Propagation in Sedimentary Rock. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1991, 28, 261–273. [Google Scholar] [CrossRef]
- Berchenko, I.; Detournay, E. Deviation of Hydraulic Fractures through Poroelastic Stress Changes Induced by Fluid Injection and Pumping. Int. J. Rock Mech. Min. Sci. 1997, 34, 1009–1019. [Google Scholar] [CrossRef]
- Boone, T.J.; Wawrzynek, P.A. Exploiting Poroelastic Effects and Permeability Contrasts to Control Fracture Orientation. In Proceedings of the Society of Petroleum Engineers, Delft, The Netherlands, 1 January 1994. [Google Scholar]
- Dalkhaa, C.; Azzolina, N.A.; Chakhmakhchev, A.; Kurz, B.A.; Sorensen, J.A.; Gorecki, C.D.; Harju, J.A. Refracturing in the Bakken—An Analysis of Data from Across North Dakota. In Proceedings of the OnePetro, Houston, TX, USA, 20 June 2022. [Google Scholar]
- Wright, C.A.; Conant, R.A. Hydraulic Fracture Reorientation in Primary and Secondary Recovery from Low-Permeability Reservoirs. In Proceedings of the Society of Petroleum Engineers, Dallas, TX, USA, 1 January 1995. [Google Scholar]
- Wang, Y.; Yao, Y.; Wang, L.; Hu, Y.; Wu, H.; Wang, H. Case Study: Analysis of Refracturing Crack Orientation-Angle and Extension-Length in Tight Gas Reservoir, Sulige Gasfield of China. In Proceedings of the OnePetro, Madrid, Spain, 6 June 2022. [Google Scholar]
- Wang, D.-B.; Zhou, F.-J.; Li, Y.-P.; Yu, B.; Martyushev, D.; Liu, X.-F.; Wang, M.; He, C.-M.; Han, D.-X.; Sun, D.-L. Numerical Simulation of Fracture Propagation in Russia Carbonate Reservoirs during Refracturing. Pet. Sci. 2022, in press. [Google Scholar] [CrossRef]
- Chen, J.; Zhang, Q.; Zhang, J. Numerical Simulations of Temporary Plugging-Refracturing Processes in a Conglomerate Reservoir Under Various In-Situ Stress Difference Conditions. Front. Phys. 2022, 9, 825. [Google Scholar] [CrossRef]
- Melenk, J.M.; Babuška, I. The Partition of Unity Finite Element Method: Basic Theory and Applications. Comput. Methods Appl. Mech. Eng. 1996, 139, 289–314. [Google Scholar] [CrossRef] [Green Version]
- Belytschko, T.; Black, T. Elastic Crack Growth in Finite Elements with Minimal Remeshing. Int. J. Numer. Methods Eng. 1999, 45, 601–620. [Google Scholar] [CrossRef]
- Abaqus 6.14-1 Documentation; Dassault Systemes Simulia Corporation: Providence, RI, USA, 2014.
- Song, J.; Areias, P.M.A.; Belytschko, T. A Method for Dynamic Crack and Shear Band Propagation with Phantom Nodes. Int. J. Numer. Methods Eng. 2006, 67, 868–893. [Google Scholar] [CrossRef]
- Ma, T.; Zhang, K.; Shen, W.; Guo, C.; Xu, H. Discontinuous and Continuous Galerkin Methods for Compressible Single-Phase and Two-Phase Flow in Fractured Porous Media. Adv. Water Resour. 2021, 156, 104039. [Google Scholar] [CrossRef]
- Xu, Y.; Sheng, G.; Zhao, H.; Hui, Y.; Zhou, Y.; Ma, J.; Rao, X.; Zhong, X.; Gong, J. A New Approach for Gas-Water Flow Simulation in Multi-Fractured Horizontal Wells of Shale Gas Reservoirs. J. Pet. Sci. Eng. 2021, 199, 108292. [Google Scholar] [CrossRef]
- Rice, J.R.; Cleary, M.P. Some Basic Stress Diffusion Solutions for Fluid—Saturated Elastic Porous Media with Compressible Constituents. Rev. Geophys. 1976, 14, 227–241. [Google Scholar] [CrossRef]
- Barenblatt, G.I. The Mathematical Theory of Equilibrium Cracks in Brittle Fracture. In Advances in Applied Mechanics; Dryden, H.L., von Kármán, T., Kuerti, G., van den Dungen, F.H., Howarth, L., Eds.; Elsevier: Amsterdam, The Netherlands, 1962; Volume 7, pp. 55–129. [Google Scholar]
- Benzeggagh, M.L.; Kenane, M. Measurement of Mixed-Mode Delamination Fracture Toughness of Unidirectional Glass/Epoxy Composites with Mixed-Mode Bending Apparatus. Compos. Sci. Technol. 1996, 56, 439–449. [Google Scholar] [CrossRef]
- Geertsma, J.; De Klerk, F. A Rapid Method of Predicting Width and Extent of Hydraulically Induced Fractures. J. Pet. Technol. 1969, 21, 1571–1581. [Google Scholar] [CrossRef]
- Detournay, E. Propagation Regimes of Fluid-Driven Fractures in Impermeable Rocks. Int. J. Geomech. 2004, 4, 35–45. [Google Scholar] [CrossRef]
- Bunger, A.P.; Detournay, E.; Garagash, D.I. Toughness-Dominated Hydraulic Fracture with Leak-Off. Int. J. Fract. 2005, 134, 175–190. [Google Scholar] [CrossRef]
- Irwin, G. Fracture Strength Relative to Onset and Arrest of Crack Propagation. In Proceedings of the Proc ASTM, Philadelphia, PA, USA, 1 January 1958; Volume 58, pp. 640–657. [Google Scholar]
- Roussel, N.P.; Sharma, M.M. Role of Stress Reorientation in the Success of Refracture Treatments in Tight Gas Sands. SPE Prod. Oper. 2012, 27, 346–355. [Google Scholar] [CrossRef]




| Parameters | Values |
|---|---|
| Elastic modulus, E | 15,000 MPa |
| Poisson’s ratio, ν | 0.25 |
| Critical fracture energy, GC | 250 Pa·m |
| Injection rate, Qinj | 0.001 m2/s |
| Fluid viscosity, μ | 1 mPa·s |
| Tensile strength, Tmax | 3 MPa |
| Filtration coefficient, cL | 5.879 × 10−13 Pa/(m·s) |
| Rock porosity, | 0.1 |
| Rock permeability, k | 0.01 mD |
| Original pore pressure, pp | 30 MPa |
| Far-field stress, σH/σh/σv | 15/12/18 MPa |
| Injection rate, Qinj | 2 × 10−3 m2/s |
| Injection time, tinj | 100 s |
| Production rate, Qprod | 2 × 10−4 m2/s |
| Production time, tprod | 86,400 s |
| Parameters | Values |
|---|---|
| Elastic modulus, E | 20,000 MPa |
| Poisson’s ratio, ν | 0.22 |
| Fracture toughness, KIC | 100 kPa·m1/2 |
| Flow rate, Qinj | 0.06 m2/min |
| Fluid viscosity, μ | 100 mPa·s |
| Dimensionless parameter, Km | 0.313 |
| Time, t | 0.5 min |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, D.; Dahi Taleghani, A.; Yu, B.; Wang, M.; He, C. Numerical Simulation of Fracture Propagation during Refracturing. Sustainability 2022, 14, 9422. https://doi.org/10.3390/su14159422
Wang D, Dahi Taleghani A, Yu B, Wang M, He C. Numerical Simulation of Fracture Propagation during Refracturing. Sustainability. 2022; 14(15):9422. https://doi.org/10.3390/su14159422
Chicago/Turabian StyleWang, Daobing, Arash Dahi Taleghani, Bo Yu, Meng Wang, and Chunming He. 2022. "Numerical Simulation of Fracture Propagation during Refracturing" Sustainability 14, no. 15: 9422. https://doi.org/10.3390/su14159422
APA StyleWang, D., Dahi Taleghani, A., Yu, B., Wang, M., & He, C. (2022). Numerical Simulation of Fracture Propagation during Refracturing. Sustainability, 14(15), 9422. https://doi.org/10.3390/su14159422








