Condition-Based Multi-State-System Maintenance Models for Smart Grid System with Stochastic Power Supply and Demand
Abstract
:1. Introduction
- Development of MSS reliability model for the generic smart grid system with HPS.
- Establishment of the power grid measures that simultaneously account for the stochastic behavior of the power supply and power demand.
- Formulation of PM models for the generic smart-grid system with HPS using the MSS reliability models.
- Design of a DOE scheme to simulate the normal/extreme settings related to climate changes, thereby evaluating its impact on the established power grid measurements.
- Although a generic smart grid with an HPS was considered, the ramifications of this study are adaptable and expandable to the specific smart grid structures with appropriate modifications, thereby extending the practicability.
2. Related Work
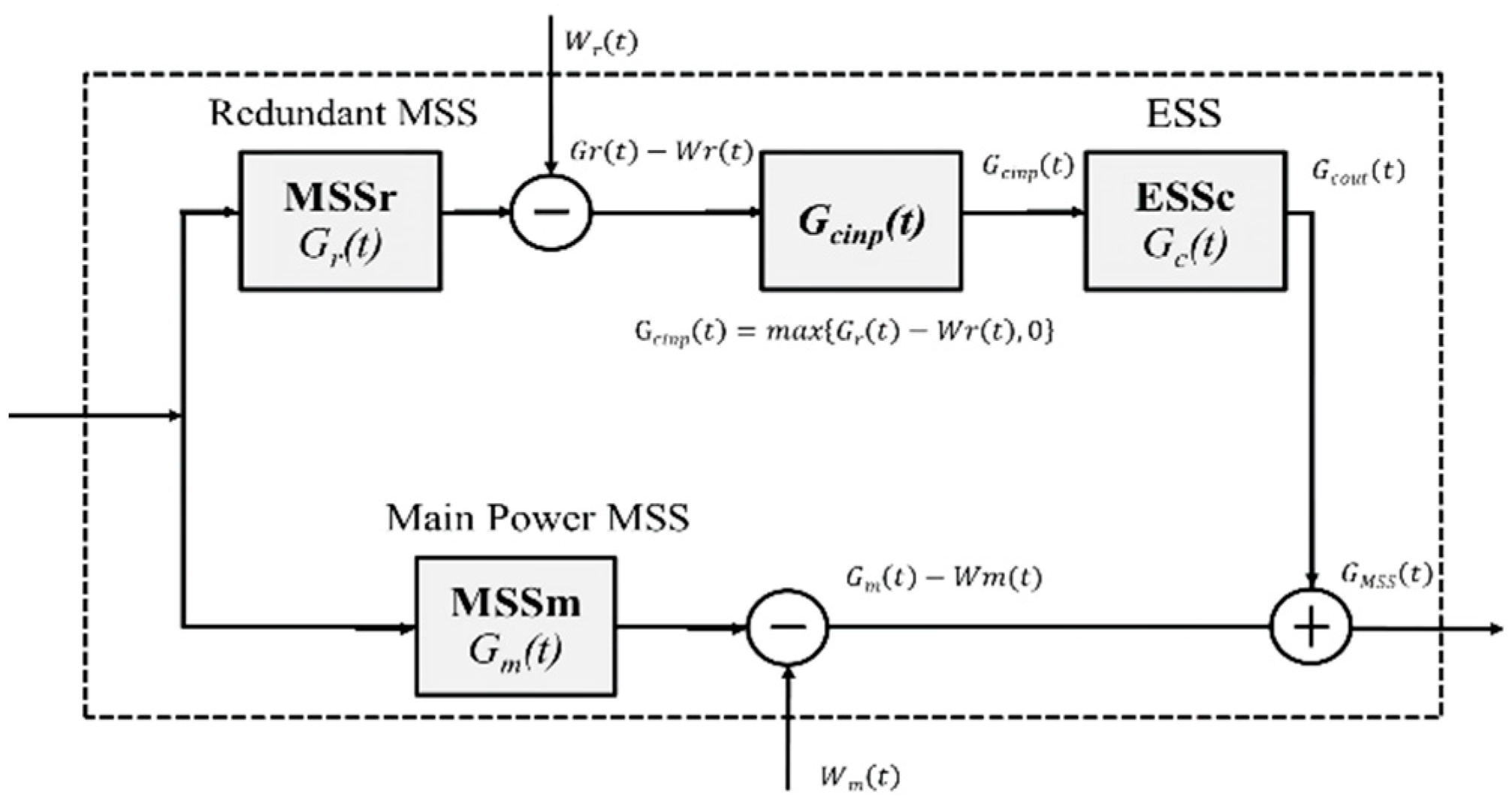
3. Materials and Methods
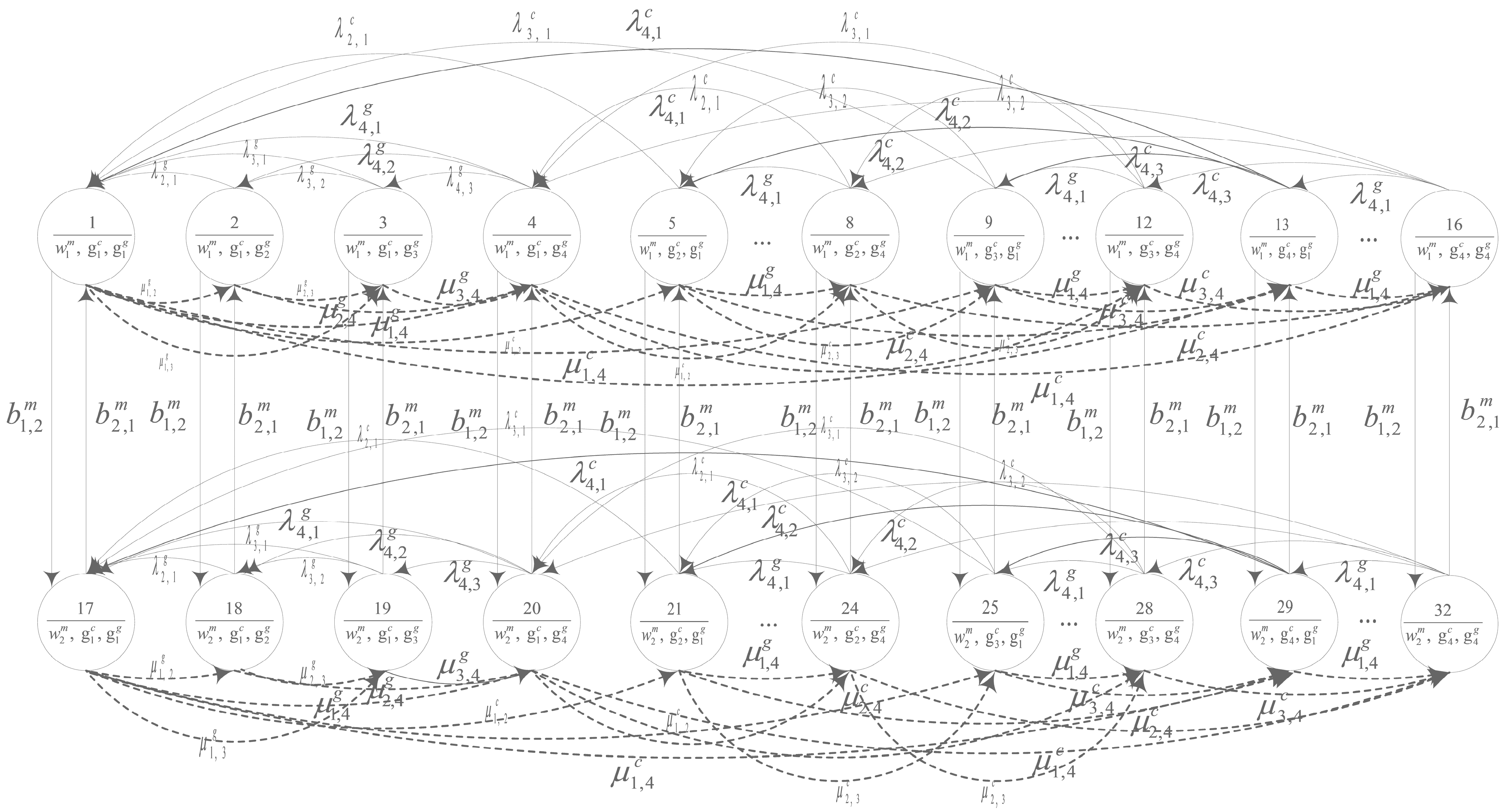
3.1. Simulation of the Smart Grid
- Power efficiency-related measures
- 2.
- Total maintenance cost
3.2. Construction and Optimization of Four PM Models
3.2.1. Construction of Two Single-Objective PM Models
3.2.2. Construction of Two Bi-Objective PM Models
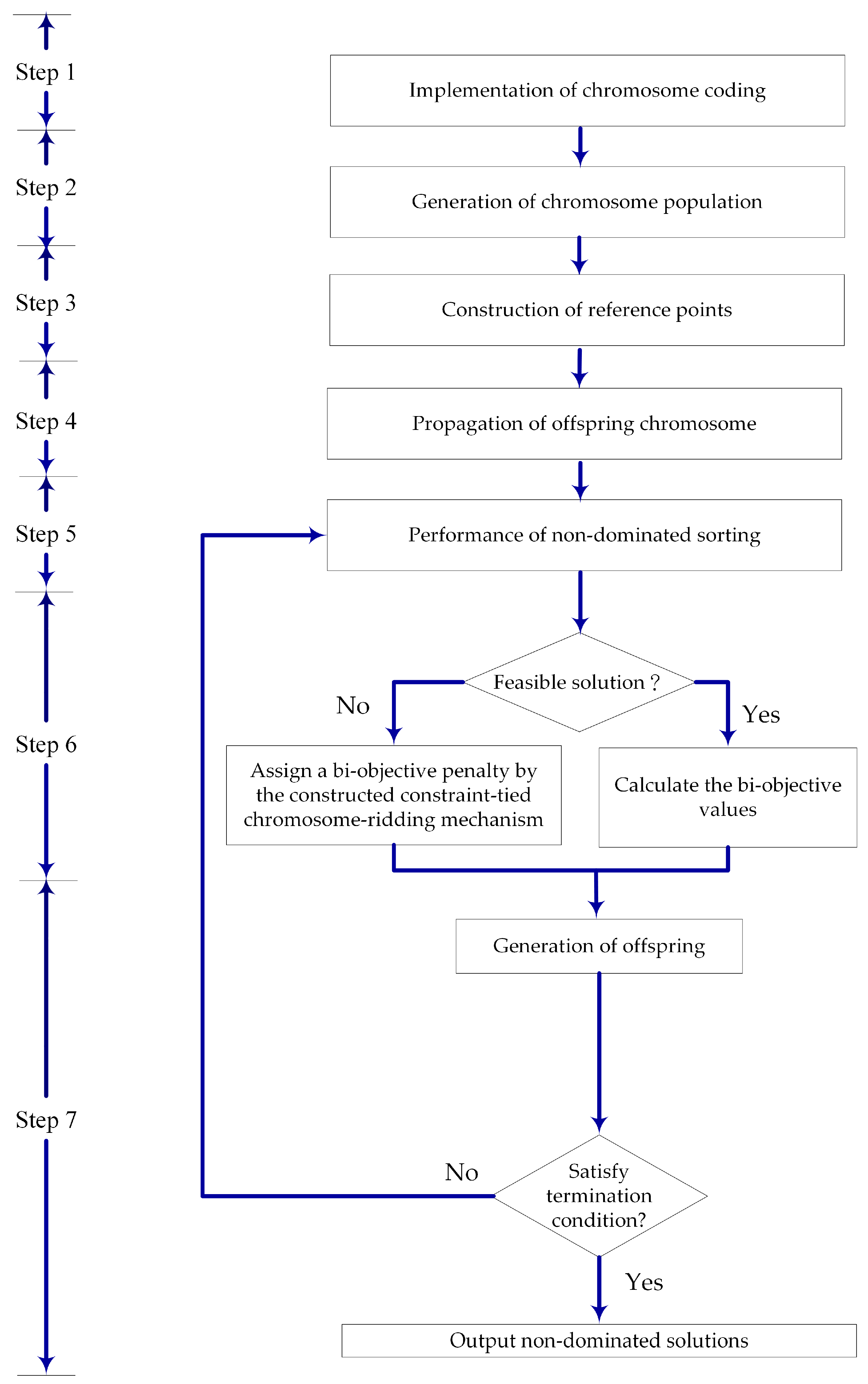
3.3. Single-Objective PM Optimization
3.4. Multi-Objective PM Optimization
4. Results and Discussion
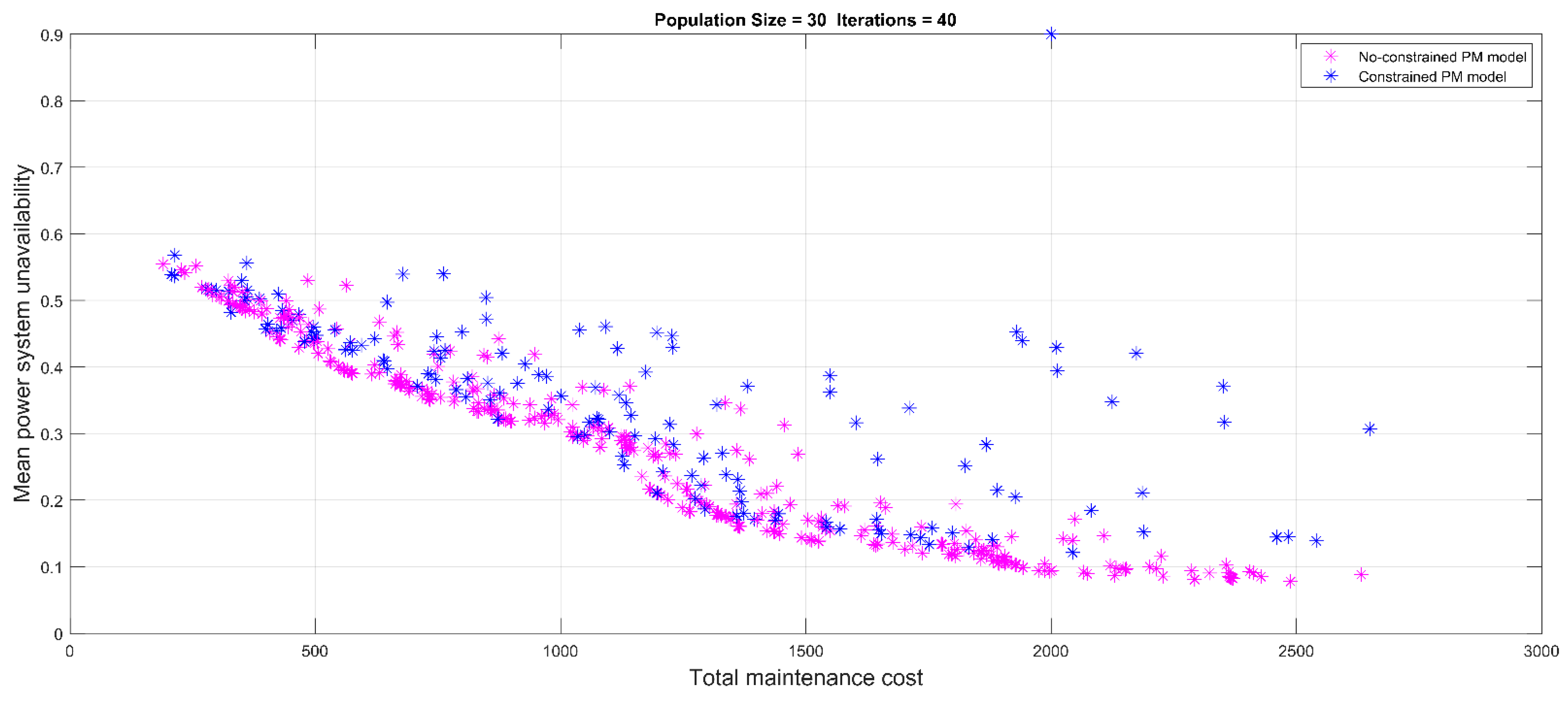
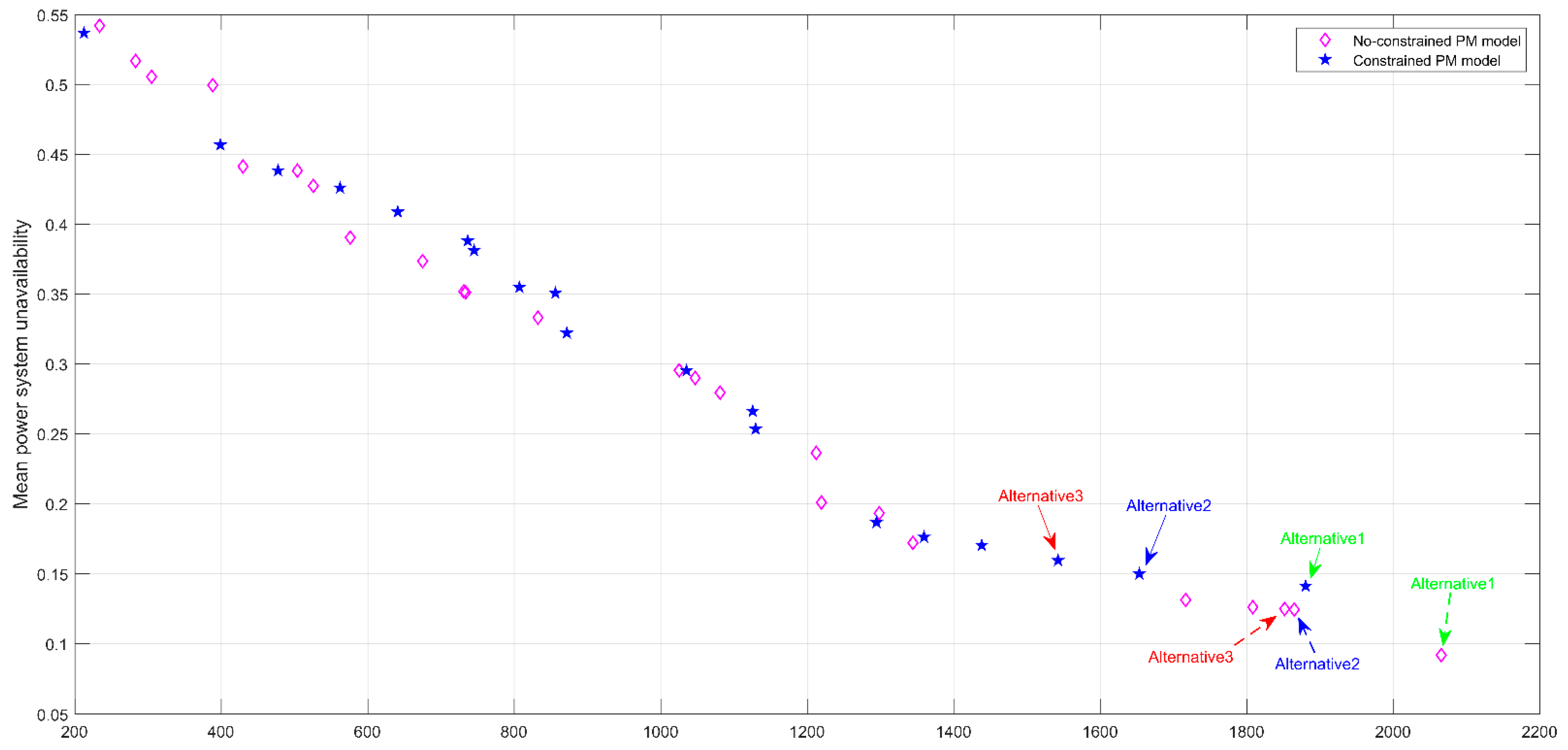
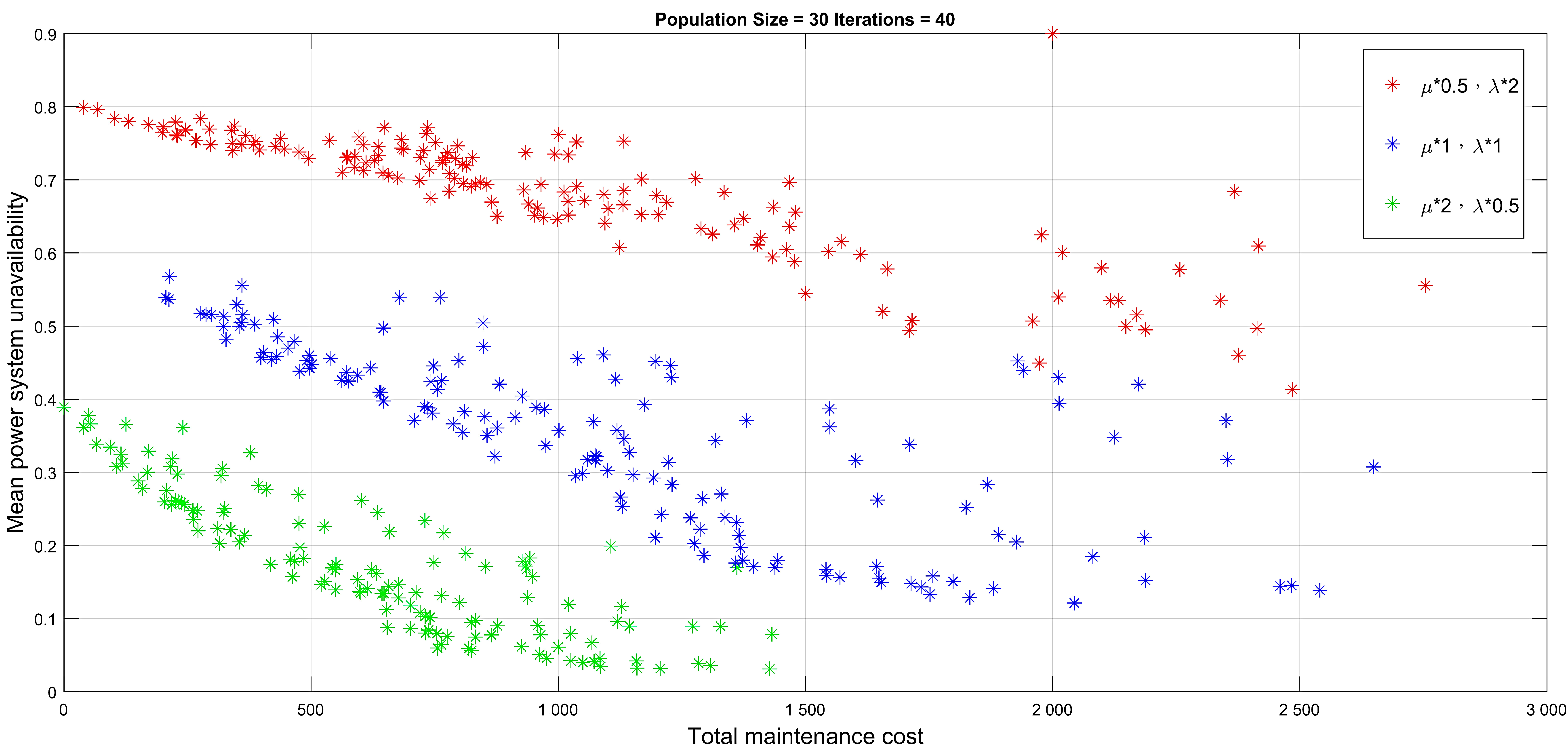
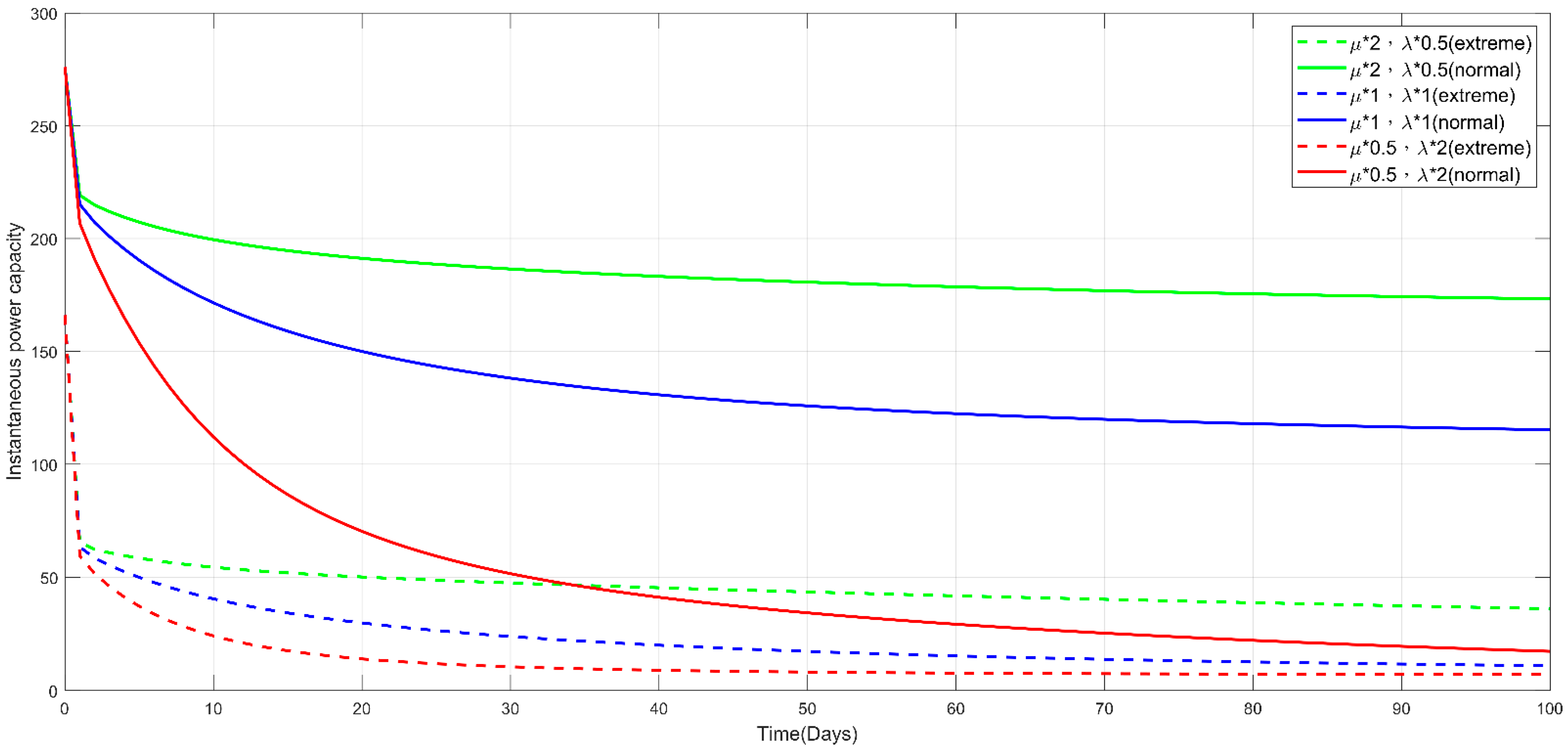
- As can be seen from Figure 21 and Figure 22, a high degradation rate combined with low repair rates, (corresponding to 1 and 4 in Table 6 for the experiments with the normal and climates, respectively, and both colored red) led to a lower measured performance in terms of the mean power system unavailability and total PM cost. This is in contrast to the case of the inverse combination, which corresponds to 3 and 6, both colored green. The comparison results were mathematically and practically appropriate, given that a high degradation rate with a low repair rate decreases the reliability of the smart grid, whereas a lacking workforce and limited maintenance resources contribute to inefficient repair. In comparison, the alternative, with a low degradation combined with high repair rates, provided a robust and stable system that demonstrated a superior power measurement performance with a low PM cost in a smart grid.
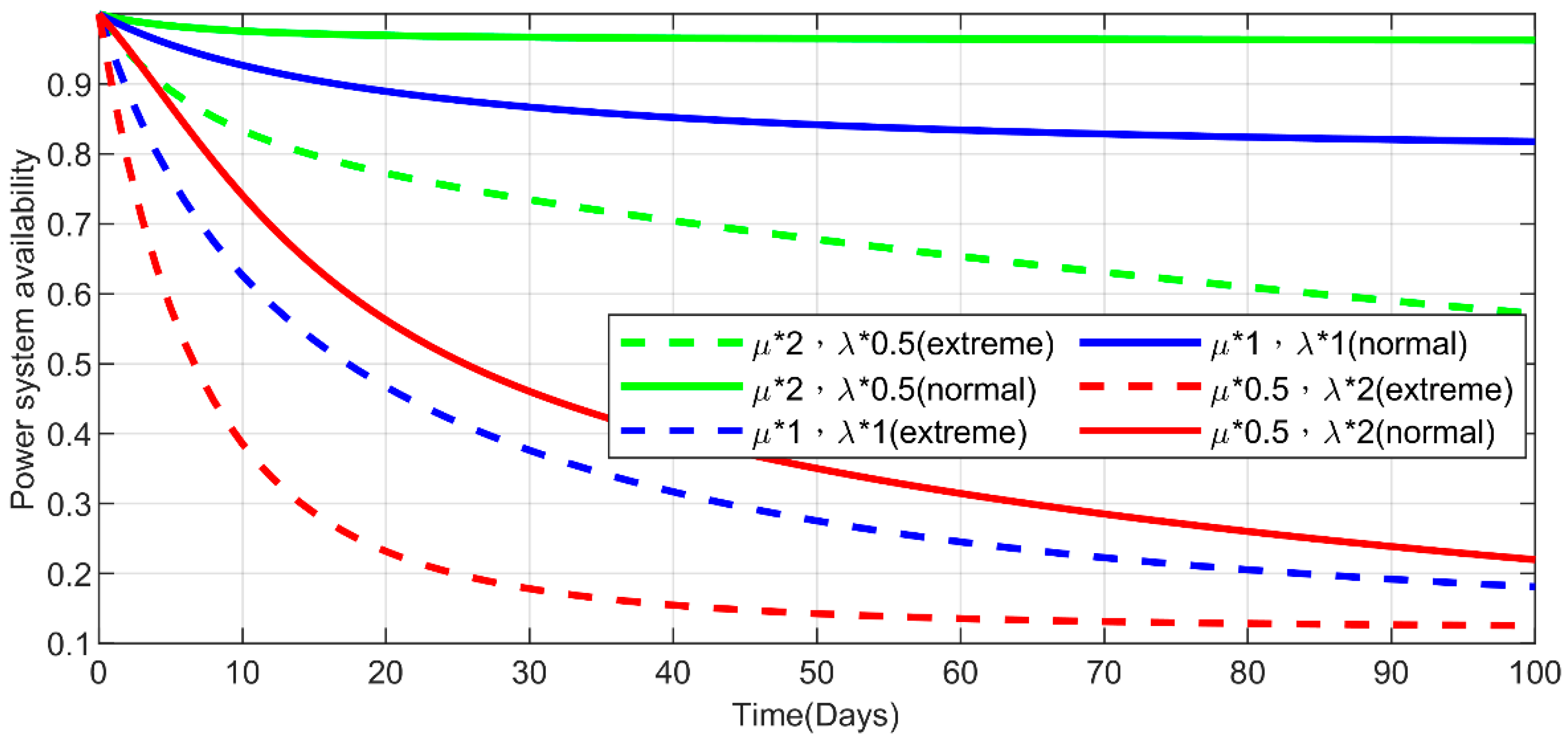
- As shown in Figure 23, the simulated normal climate (corresponding to 1, 2, and 3 in Table 6, as indicated by circle dots) outperformed the simulated extreme climate (corresponding to 4, 5, and 6, as indicated by stellular dots) in terms of the power system unavailability and PM cost, regardless of the different combinations of degradation rates and repair rates. For example, given the combination of degradation rate and repair rate at 0.5 and 2 under normal climates, the power system unavailability and PM cost are determined at 0.0324 and 1159, which is lower than that of 0.3023 and 1213 under extreme climates. This highlights the extent to which climate change influences the power grid performance, and in turn, poses a safety risk to humans amidst hurricanes, flash floods, droughts, and extreme high temperatures, among other natural disasters. Moreover, these disasters presently occur at an abnormally high frequency. Hence, research has been conducted to curb green gas emissions; thus preventing the planet temperature from rising to 1.5 °C above the preindustrial level, which leads to most catastrophic disasters.
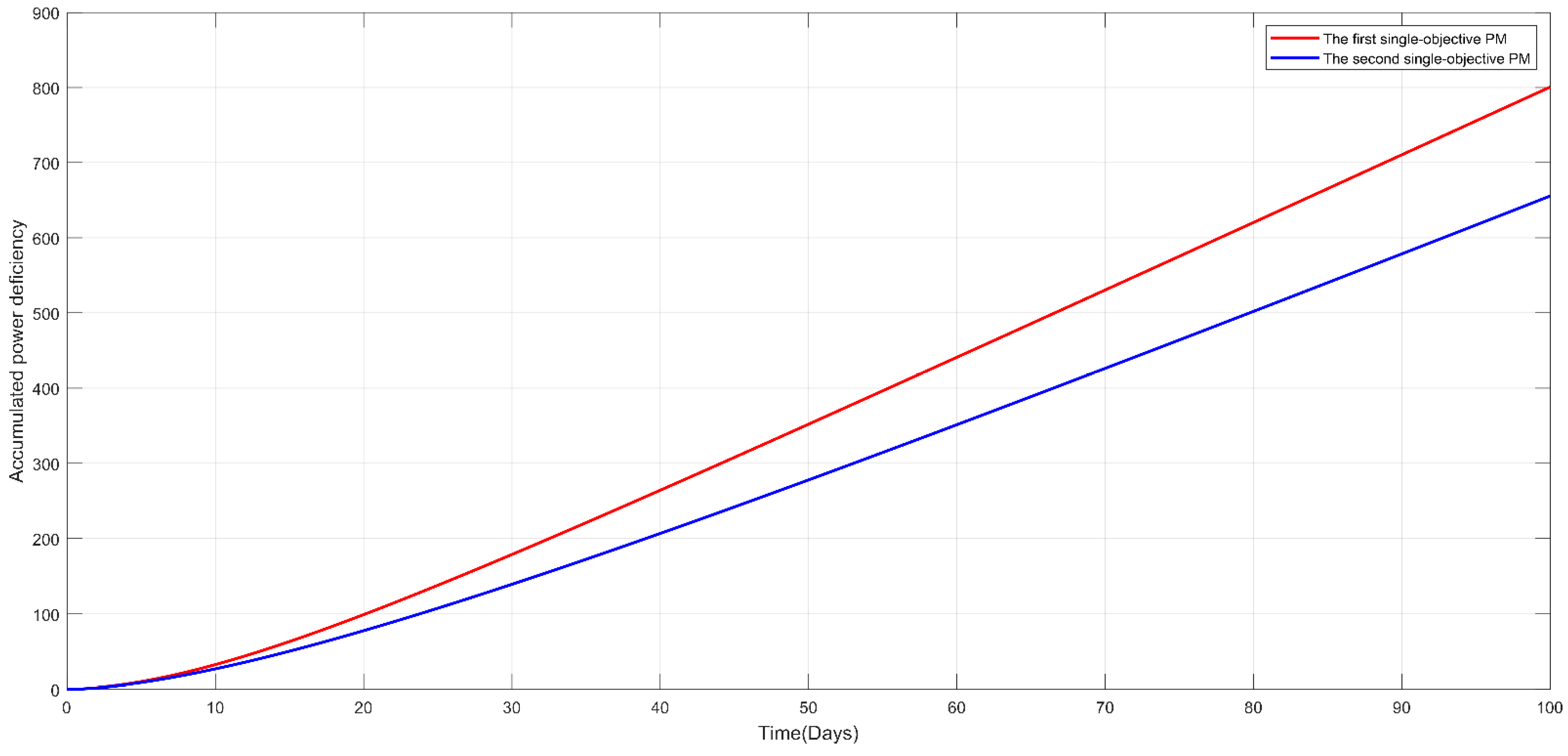
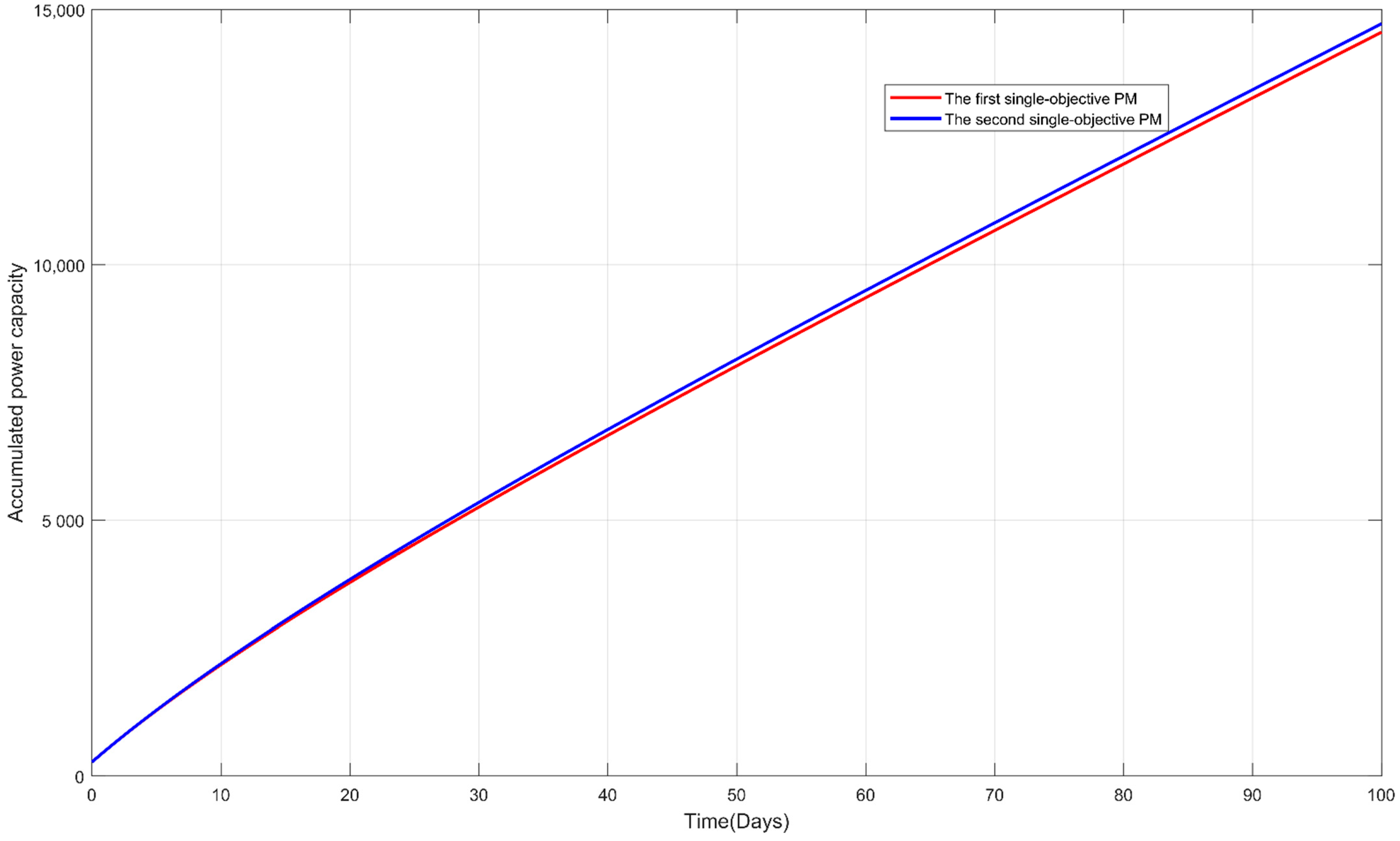
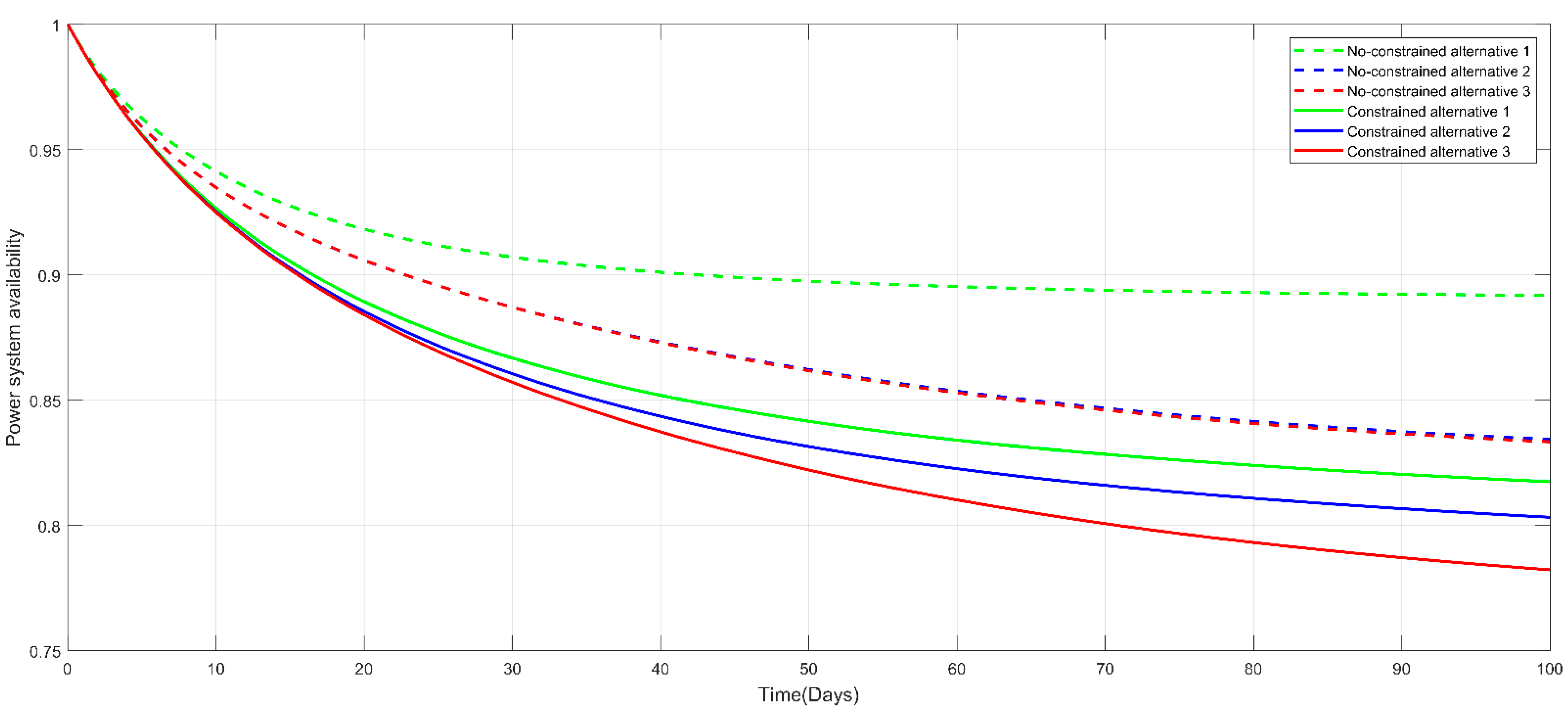
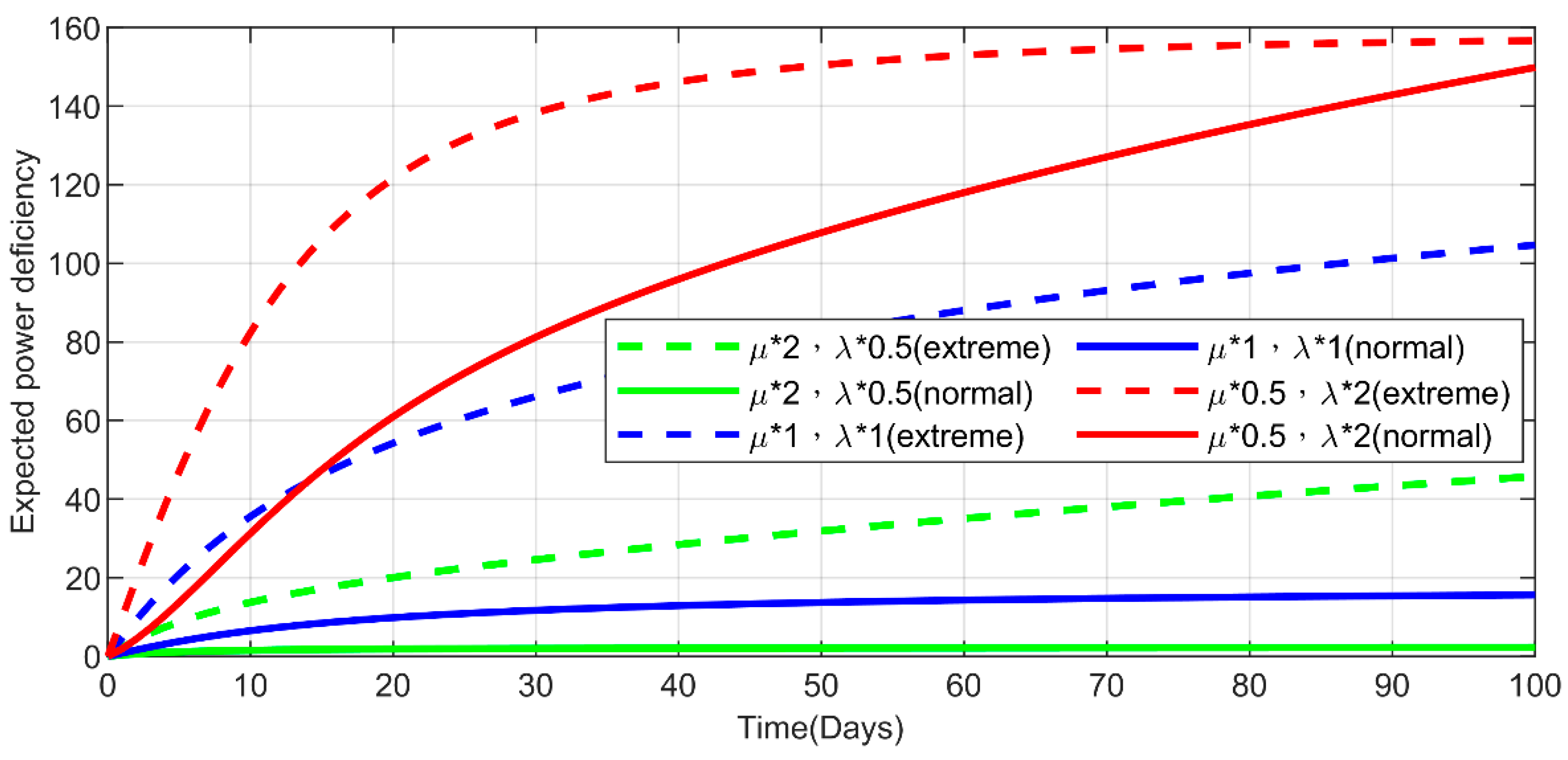
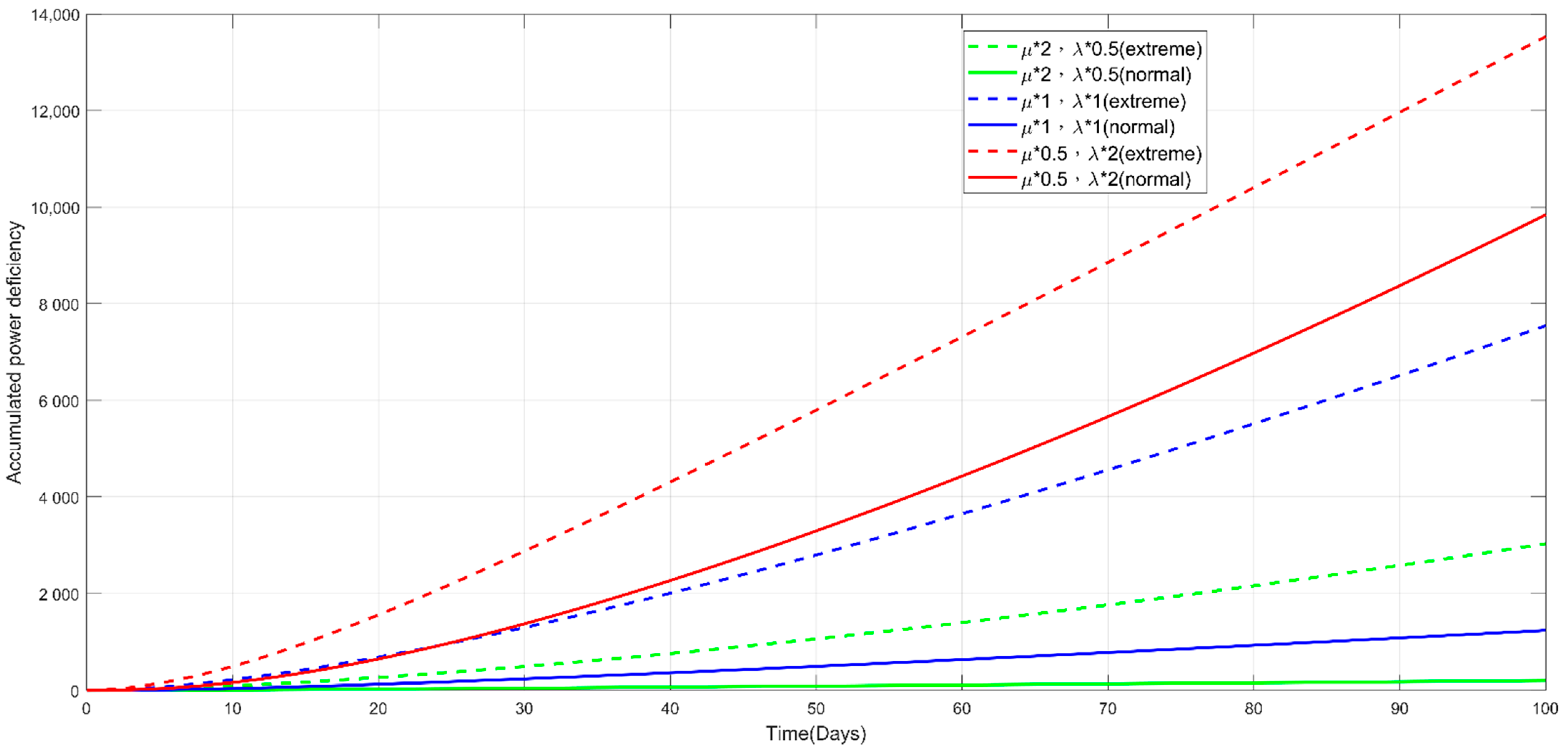
- Figure 24, Figure 25, Figure 26, Figure 27 and Figure 28 present the other power measures from different perspectives, namely, the power system availability, expected power deficiency, accumulated power deficiency, instantaneous power capacity, and accumulated power capacity. All present the same conclusion, as depicted in the second point, through differentiated diagrams reflecting the power-grid performances across various measures.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lisnianski, A.; Elmakias, D.; Laredo, D.; Ben Haim, H. A multi-state Markov model for a short-term reliability analysis of a power generating unit. Reliab. Eng. Syst. Saf. 2012, 98, 1–6. [Google Scholar] [CrossRef]
- Howlader, A.M.; Izumi, Y.; Uehara, A.; Urasaki, N.; Senjyu, T.; Saber, A.Y. A robust H∞ controller based frequency control approach using the wind-battery coordination strategy in a small power system. Int. J. Electr. Power Energy Syst. 2014, 58, 190–198. [Google Scholar] [CrossRef]
- Pan, I.; Das, S. Kriging based surrogate modeling for fractional order control of microgrids. IEEE Trans. Smart Grid 2015, 6, 36–44. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Xu, Y.; Fang, S.; Zheng, X.; Feng, X. Robust coordination of a hybrid AC/DC multi-energy ship microgrid with flexible voyage and thermal loads. IEEE Trans. Smart Grid 2020, 11, 2782–2793. [Google Scholar] [CrossRef]
- Hou, J.; Sun, J.; Hofmann, H.F. Mitigating power fluctuations in electric ship propulsion with hybrid energy storage system: Design and analysis. IEEE J. Ocean. Eng. 2018, 43, 93–107. [Google Scholar] [CrossRef]
- Conteh, F.; Takahashi, H.; Hemeida, A.; Krishnan, N.; Mikhaylov, A.; Senjyu, T. Analysis of hybrid grid-connected renewable power generation for sustainable electricity supply in Sierra Leone. Sustainability 2021, 13, 11435. [Google Scholar] [CrossRef]
- Shahzad, M.; Qadir, A.; Ullah, N.; Mahmood, Z.; Saad, N.M.; Ali, S.S. Optimization of on-grid hybrid renewable energy system: A case study on Azad Jammu and Kashmir. Sustainability 2022, 14, 5757. [Google Scholar] [CrossRef]
- Kharrich, M.; Kamel, S.; Hassan, M.H.; ElSayed, S.K.; Taha, I.B.M. An improved heap-based optimizer for optimal design of a hybrid microgrid considering reliability and availability constraints. Sustainability 2021, 13, 10419. [Google Scholar] [CrossRef]
- Zahraoui, Y.; Alhamrouni, I.; Mekhilef, S.; Basir Khan, M.R.; Seyedmahmoudian, M.; Stojcevski, A.; Horan, B. Energy management system in microgrids: A comprehensive review. Sustainability 2021, 13, 10492. [Google Scholar] [CrossRef]
- Choi, Y.-J.; Oh, B.-C.; Acquah, M.A.; Kim, D.-M.; Kim, S.-Y. Optimal operation of a hybrid power system as an island microgrid insouth-Korea. Sustainability 2021, 13, 5022. [Google Scholar] [CrossRef]
- Shang, C.; Srinivasan, D.; Reindl, T. Economic and environmental generation and voyage scheduling of all-electric ships. IEEE Trans. Power Syst. 2016, 31, 4087–4096. [Google Scholar] [CrossRef]
- Boveri, A.; Silvestro, F.; Molinas, M.; Skjong, E. Optimal sizing of energy storage systems for shipboard applications. IEEE Trans. Energy Convers. 2019, 34, 801–811. [Google Scholar] [CrossRef] [Green Version]
- Fang, S.; Xu, Y.; Li, Z.; Zhao, T.; Wang, H. Two-step multi-objective management of hybrid energy storage system in all-electric ship microgrids. IEEE Trans. Veh. Technol. 2019, 68, 3361–3373. [Google Scholar] [CrossRef]
- Wang, X.; Shipurkar, U.; Haseltalab, A.; Polinder, H.; Claeys, F.; Negenborn, R.R. Sizing and control of a hybrid ship propulsion system using multi-objective double-layer optimization. IEEE Access 2021, 9, 72587–72601. [Google Scholar] [CrossRef]
- Bao, M.; Ding, Y.; Singh, C.; Shao, C. A multi-state model for reliability assessment of integrated gas and power systems utilizing universal generating function techniques. IEEE Trans. Smart Grid 2019, 10, 6271–6283. [Google Scholar] [CrossRef]
- Karngala, A.K.; Singh, C. Reliability assessment framework for the distribution system including distributed energy resources. IEEE Trans. Sustain. Energy 2021, 12, 1539–1548. [Google Scholar] [CrossRef]
- Shadmand, M.B.; Balog, R.S. Multi-objective optimization and design of Photovoltaic-wind hybrid system for community Smart DC microgrid. IEEE Trans. Smart Grid 2014, 5, 2635–2643. [Google Scholar] [CrossRef]
- Huang, C.H.; Wang, C.; Chen, G. Multiobjective multistate system preventive maintenance model with human reliability. Int. J. Aerosp. Eng. 2021, 2021, 6623810. [Google Scholar] [CrossRef]
- Chen, G.L.; Wang, C.; Huang, C. Improved availability index for repairable fuzzy multi-state systems. IEEE Access 2020, 8, 212927–212938. [Google Scholar] [CrossRef]
- Caballé, N.C.; Castro, I.T.; Pérez, C.J.; Lanza-Gutiérrez, J.M. A condition-based maintenance of a dependent degradation-threshold-shock model in a system with multiple degradation processes. Reliab. Eng. Syst. Saf. 2015, 134, 98–109. [Google Scholar] [CrossRef]
- Castro, I.T.; Caballé, N.C.; Pérez, C.J. A condition-based maintenance for a system subject to multiple degradation processes and external shocks. Int. J. Syst. Sci. 2015, 46, 1692–1704. [Google Scholar] [CrossRef]
- Zhai, Q.; Ye, Z.; Yang, J.; Zhao, Y. Measurement errors in degradation-based burn-in. Reliab. Eng. Syst. Saf. 2016, 150, 126–135. [Google Scholar] [CrossRef]
- Manoharan, M.; Nair, V.G. Evaluation of system performance measures of multi state degraded system with minimal repair. Reliab. Theory Appl. 2017, 12, 76–83. [Google Scholar]
- Bhesdadiya, R.H.; Trivedi, I.N.; Jangir, P.; Jangir, N.; Kumar, A. An NSGA-III algorithm for solving multi-objective economic/environmental dispatch problem. Cogent Eng. 2016, 3, 1269383. [Google Scholar] [CrossRef]
- Guo, C.; Ye, C.; Ding, Y.; Lin, Z.; Wang, P. Risk-based many-objective configuration of power system fault current limiters utilising NSGA-III. IET Gener. Transm. Distrib. 2020, 14, 5646–5654. [Google Scholar] [CrossRef]
- Yang, X.; Zhang, Y. Optimal transmission line assignment considering reliabilities and assignment cost in the multi-state network. IEEE Access 2020, 8, 105690–105700. [Google Scholar] [CrossRef]
- Alizadeh Afrouzy, Z.; Paydar, M.M.; Nasseri, S.H.; Mahdavi, I. A meta-heuristic approach supported by NSGA-II for the design and plan of supply chain networks considering new product development. J. Ind. Eng. Int. 2018, 14, 95–109. [Google Scholar] [CrossRef] [Green Version]
- Behmanesh, R.; Rahimi, I.; Gandomi, A.H. Evolutionary many-objective algorithms for combinatorial optimization problems: A comparative study. Arch. Comput. Methods Eng. 2021, 28, 673–688. [Google Scholar] [CrossRef]
- Li, Y.; Kou, Y.; Li, Z. An improved nondominated sorting genetic algorithm III method for solving multiobjective weapon-target assignment part I: The value of fighter combat. Int. J. Aerosp. Eng. 2018, 2018, 8302324. [Google Scholar] [CrossRef] [Green Version]
- Ni, X.; Dong, Z.; Xie, W.; Jia, W.; Duan, C.; Yao, H. Research on the multi-objective cooperative competition mechanism of Jinsha river downstream cascade reservoirs during the flood season based on optimized NSGA-III. Water 2019, 11, 849. [Google Scholar] [CrossRef] [Green Version]
- Bi, X.; Wang, C. An improved NSGA-III algorithm based on objective space decomposition for many-objective optimization. Soft Comput. 2017, 21, 4269–4296. [Google Scholar] [CrossRef]
- Liu, Q.; Liu, X.; Wu, J.; Li, Y. An improved NSGA-III algorithm using genetic K-means clustering algorithm. IEEE Access 2019, 7, 185239–185249. [Google Scholar] [CrossRef]
- Lisnianski, A.; Fennkel, I.; Ding, Y. Multi-State System Reliability Analysis and Optimization for Engineers and Industrial Managers; Springer: Berlin/Heidelberg, Germany, 2010; pp. 273–281. [Google Scholar]
- Zheng, J.H.; Wu, C.Q.; Huang, J.; Liu, Y.; Wu, Q.H. Multi-objective optimization for coordinated day-ahead scheduling problem of integrated electricity-natural gas system with microgrid. IEEE Access 2020, 8, 86788–86796. [Google Scholar] [CrossRef]
- Wang, C.H.; Tsai, S.W. Optimizing bi-objective imperfect preventive maintenance model for series-parallel system using established hybrid genetic algorithm. J. Intell. Manuf. 2014, 25, 603–616. [Google Scholar] [CrossRef]
- Montgomery, D.C. Design and Analysis of Experiments, 6th ed.; Wiley: New York, NY, USA, 2005. [Google Scholar]



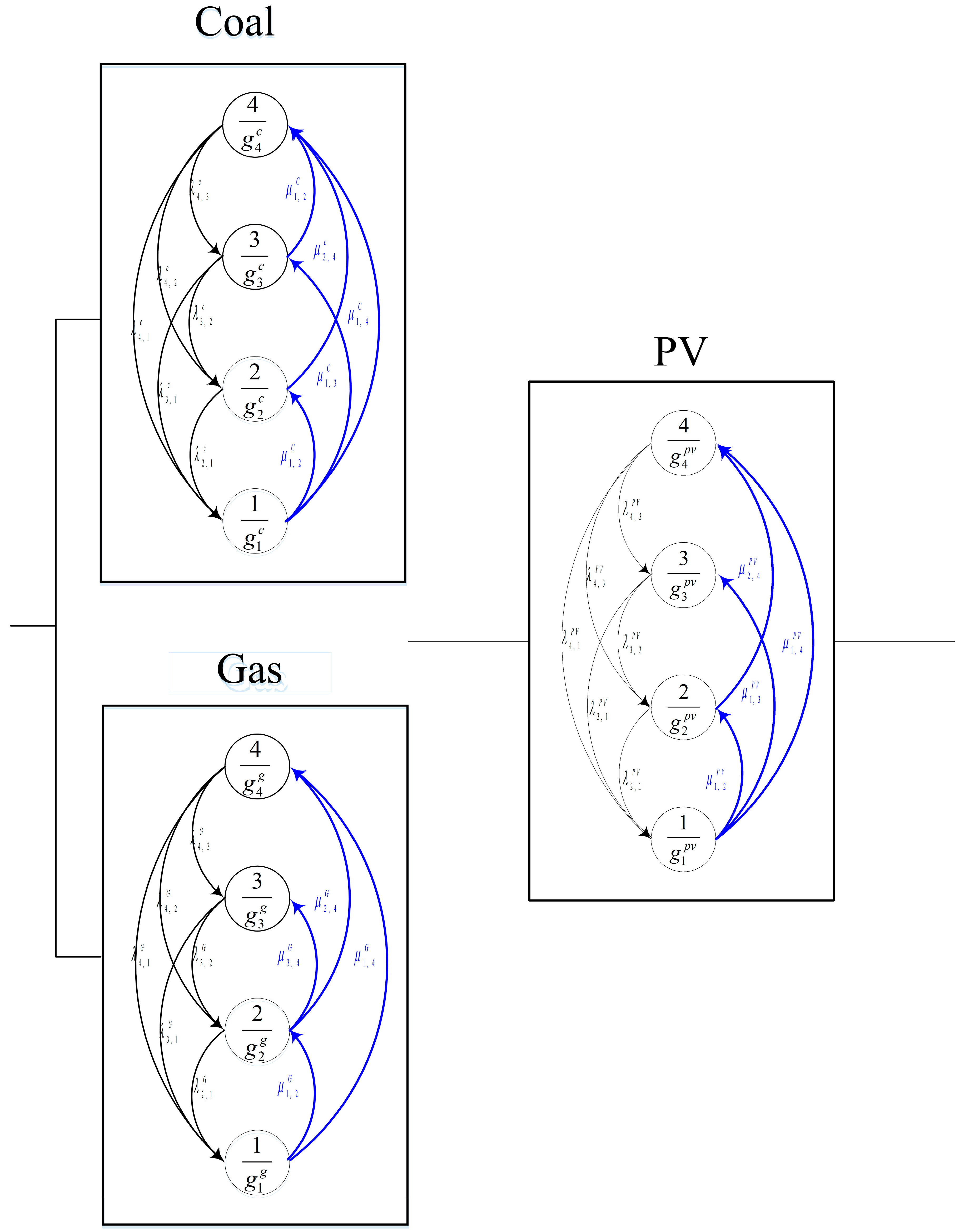
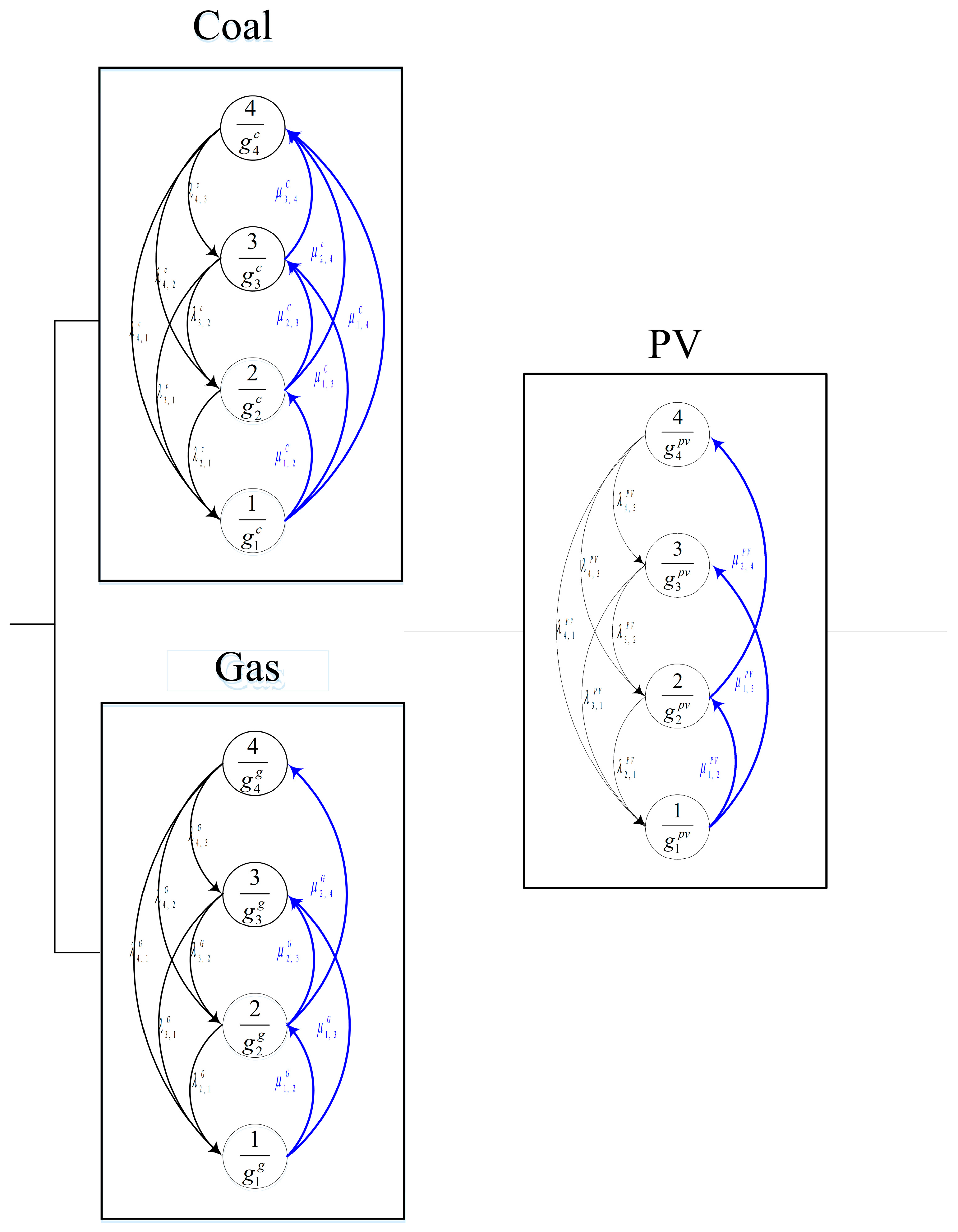
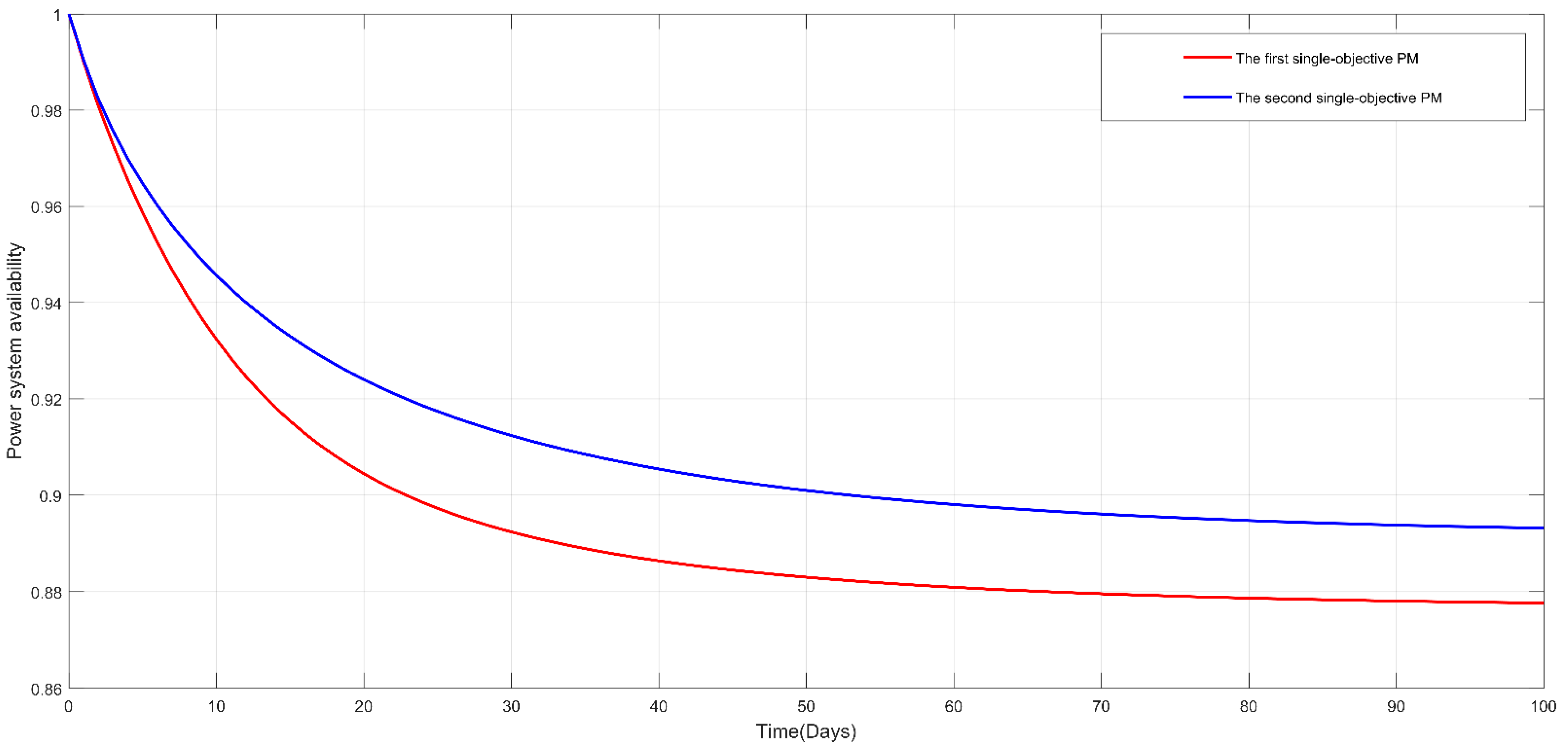
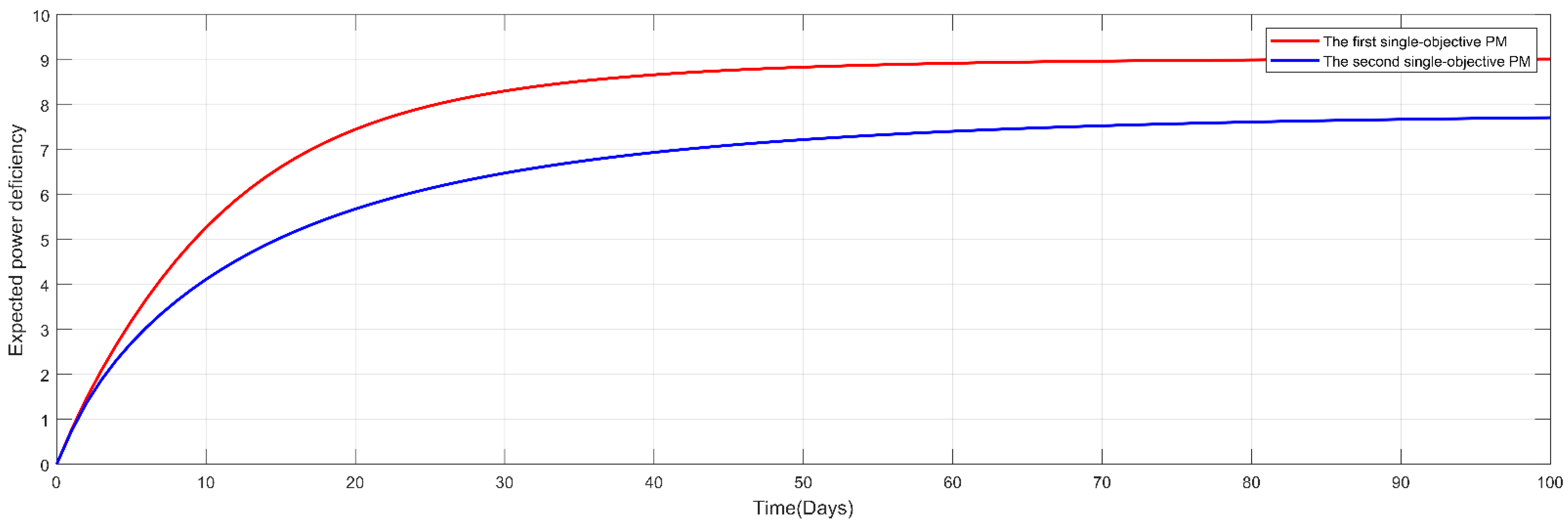




| The PM Models | Mean Power System Availability | Total Maintenance Cost |
|---|---|---|
| First single-objective PM model | 0.8951 | 2000 |
| Second single-objective PM model | 0.9113 | 2173 |
| Non-Constrained PM | Constrained PM | |||
|---|---|---|---|---|
| First PM alternative | 0.0918 | 2066 | 0.1411 | 1880 |
| Second PM alternative | 0.1244 | 1865 | 0.1499 | 1653 |
| Third PM alternative | 0.1248 | 1852 | 0.1595 | 1542 |
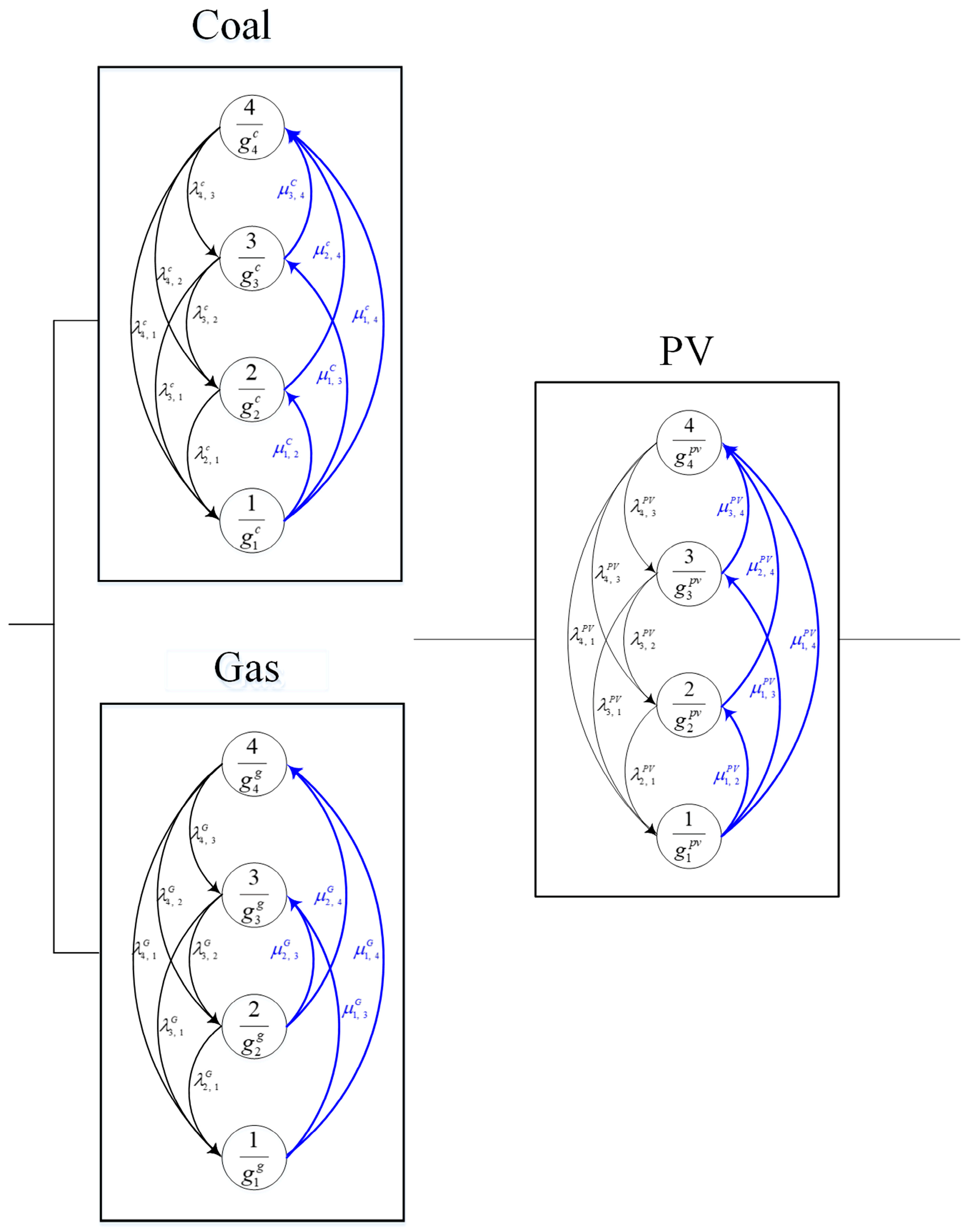
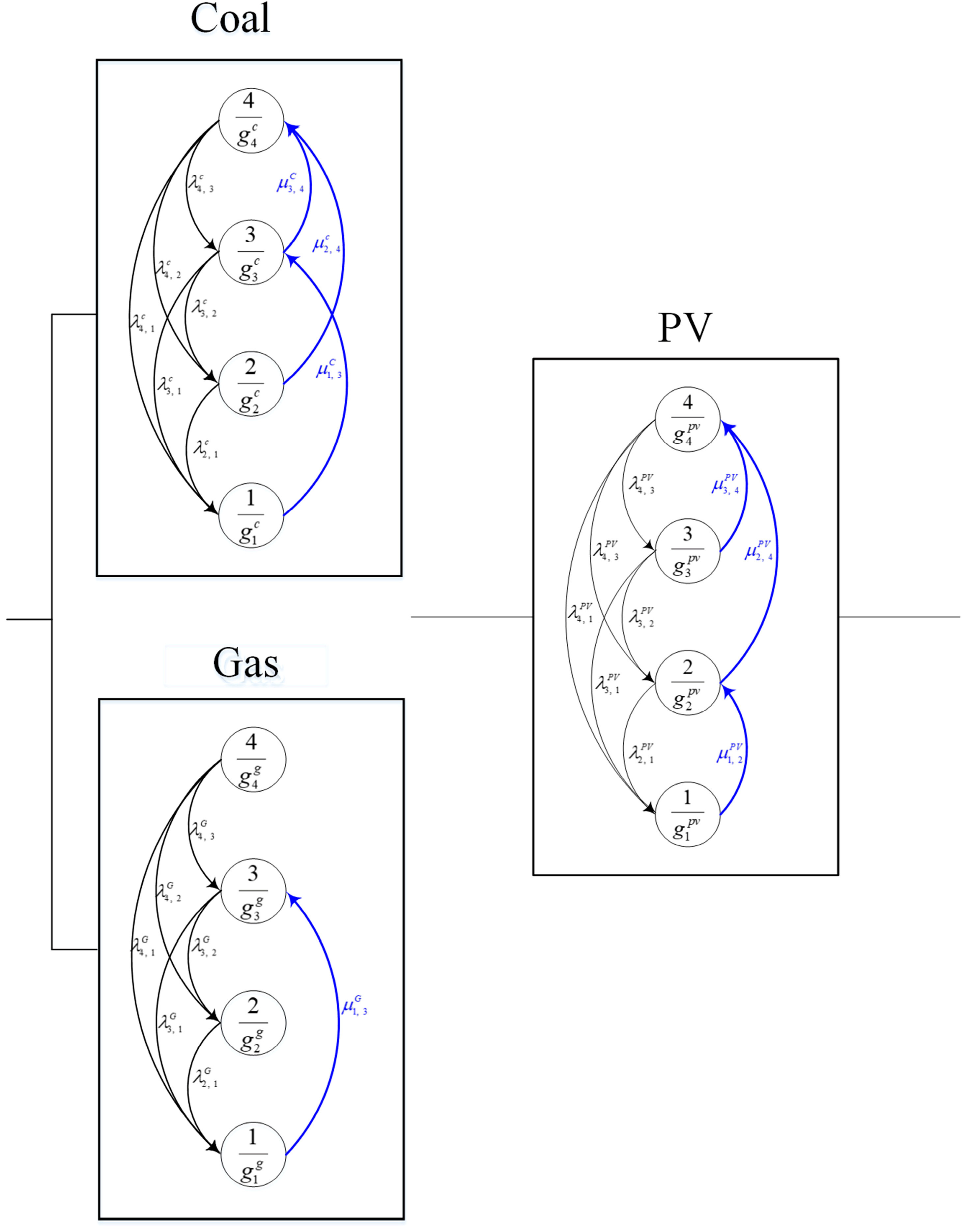
| Transition Rates | Coal | Gas | PV | ||
|---|---|---|---|---|---|
| Apparatus | Degradation rates | 0.0144 | 0.0234 | 0.0108 | |
| 0.0081 | 0.0045 | 0.0081 | |||
| 0.0045 | 0.0063 | 0.0072 | |||
| 0.0405 | 0.0036 | 0.0216 | |||
| 0.0032 | 0.0018 | 0.0063 | |||
| 0.0045 | 0.0045 | 0.0081 | |||
| Minimum repair rates | 0.2628 | 0.1116 | 0.0504 | ||
| 0.2196 | 0.3474 | 0.045 | |||
| 0.0108 | 0.0162 | 0.009 | |||
| 0.144 | 0.0684 | 0.0216 | |||
| 0.162 | 0.153 | 0.0684 | |||
| 0.144 | 0.0594 | 0.0576 | |||
| Power demand | Low–High rate | 1.52 | |||
| High–Low rate | 2.43 | ||||
| Apparatus States | Coal | Gas | PV |
|---|---|---|---|
| 1 | 0 | 0 | 0 |
| 2 | 204 | 136 | 112 |
| 3 | 282 | 194 | 173 |
| 4 | 360 | 228 | 228 |
| Apparatuses Costs | Coal | Gas | PV | |
|---|---|---|---|---|
| Minimum repair costs | 250 | 230 | 240 | |
| 600 | 450 | 530 | ||
| 650 | 600 | 630 | ||
| 400 | 300 | 350 | ||
| 500 | 400 | 450 | ||
| 300 | 200 | 250 | ||
| Climate Statuses | Rates | |||||
|---|---|---|---|---|---|---|
| 1 | Normal climate | 2 | 0.5 | 0.5782 | 0.4218 | 1665 |
| 2 | 1 | 1 | 0.1411 | 0.8589 | 1880 | |
| 3 | 0.5 | 2 | 0.0324 | 0.9676 | 1159 | |
| 4 | Extreme climate | 2 | 0.5 | 0.7885 | 0.2115 | 2592 |
| 5 | 1 | 1 | 0.6508 | 0.3492 | 1657 | |
| 6 | 0.5 | 2 | 0.3023 | 0.6977 | 1213 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, C.-H.; Huang, C.-H.; You, D.-G. Condition-Based Multi-State-System Maintenance Models for Smart Grid System with Stochastic Power Supply and Demand. Sustainability 2022, 14, 7848. https://doi.org/10.3390/su14137848
Wang C-H, Huang C-H, You D-G. Condition-Based Multi-State-System Maintenance Models for Smart Grid System with Stochastic Power Supply and Demand. Sustainability. 2022; 14(13):7848. https://doi.org/10.3390/su14137848
Chicago/Turabian StyleWang, Chun-Ho, Chao-Hui Huang, and Deng-Guei You. 2022. "Condition-Based Multi-State-System Maintenance Models for Smart Grid System with Stochastic Power Supply and Demand" Sustainability 14, no. 13: 7848. https://doi.org/10.3390/su14137848
APA StyleWang, C.-H., Huang, C.-H., & You, D.-G. (2022). Condition-Based Multi-State-System Maintenance Models for Smart Grid System with Stochastic Power Supply and Demand. Sustainability, 14(13), 7848. https://doi.org/10.3390/su14137848






