Improving Stress-Strain Behavior of Waste Aggregate Concrete Using Affordable Glass Fiber Reinforced Polymer (GFRP) Composites
Abstract
:1. Introduction
2. Experimental Program
2.1. Test Matrix
2.2. Material Properties
2.3. Preparation of Test Specimens
2.4. Instrumentation & Test Setup
3. Experimental Results
3.1. Ultimate Failure Modes
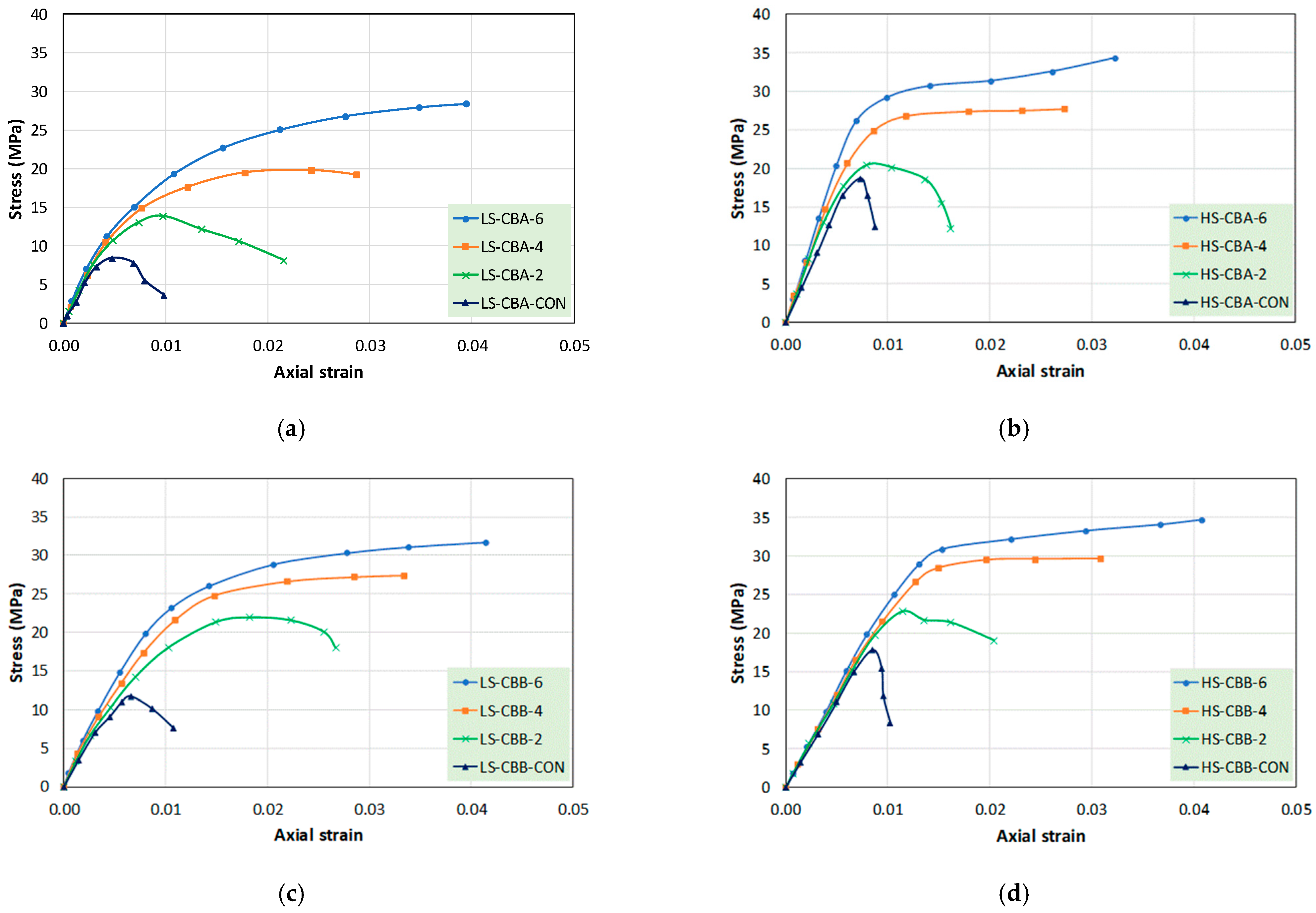
3.2. Axial Load-Deflection Curves
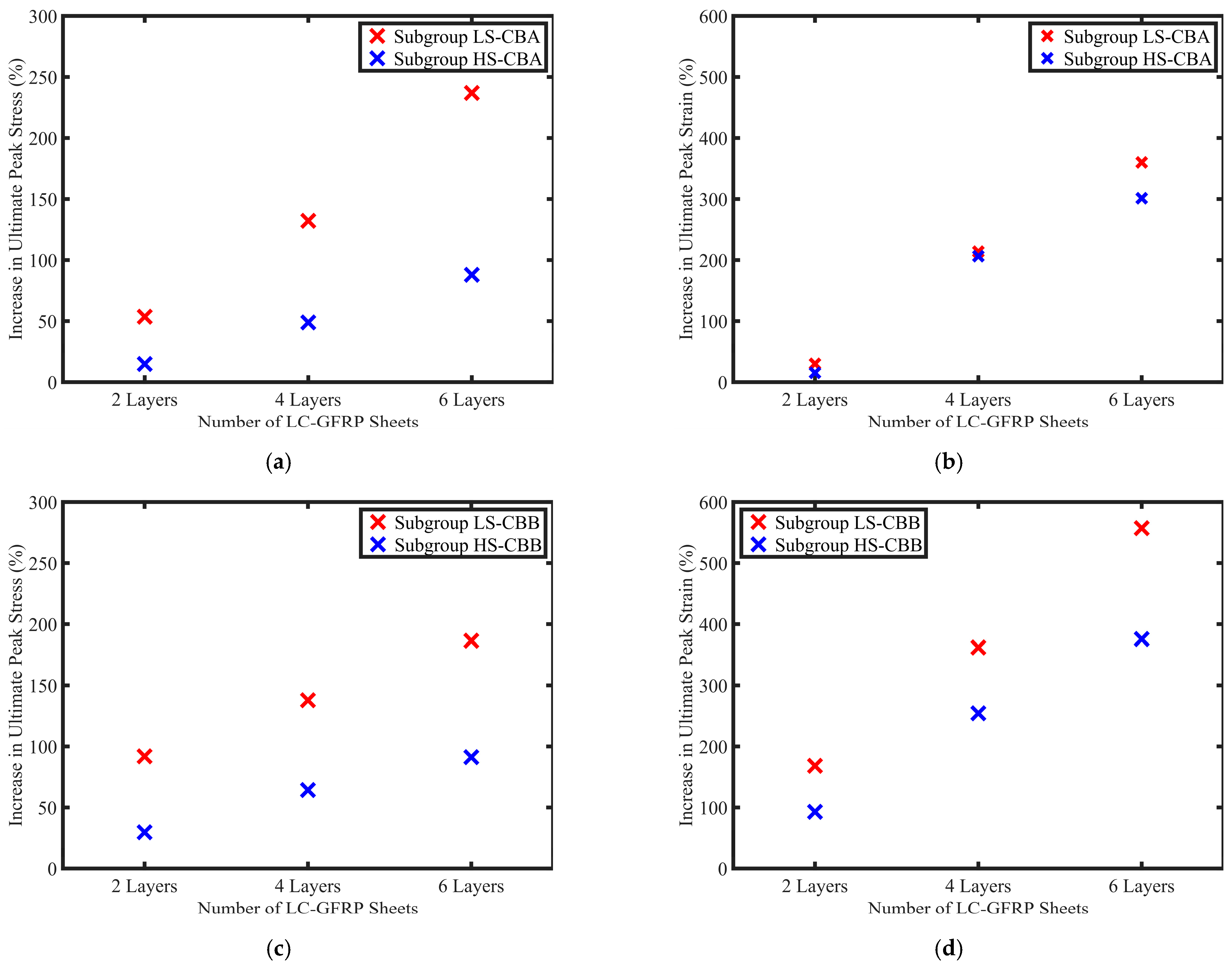
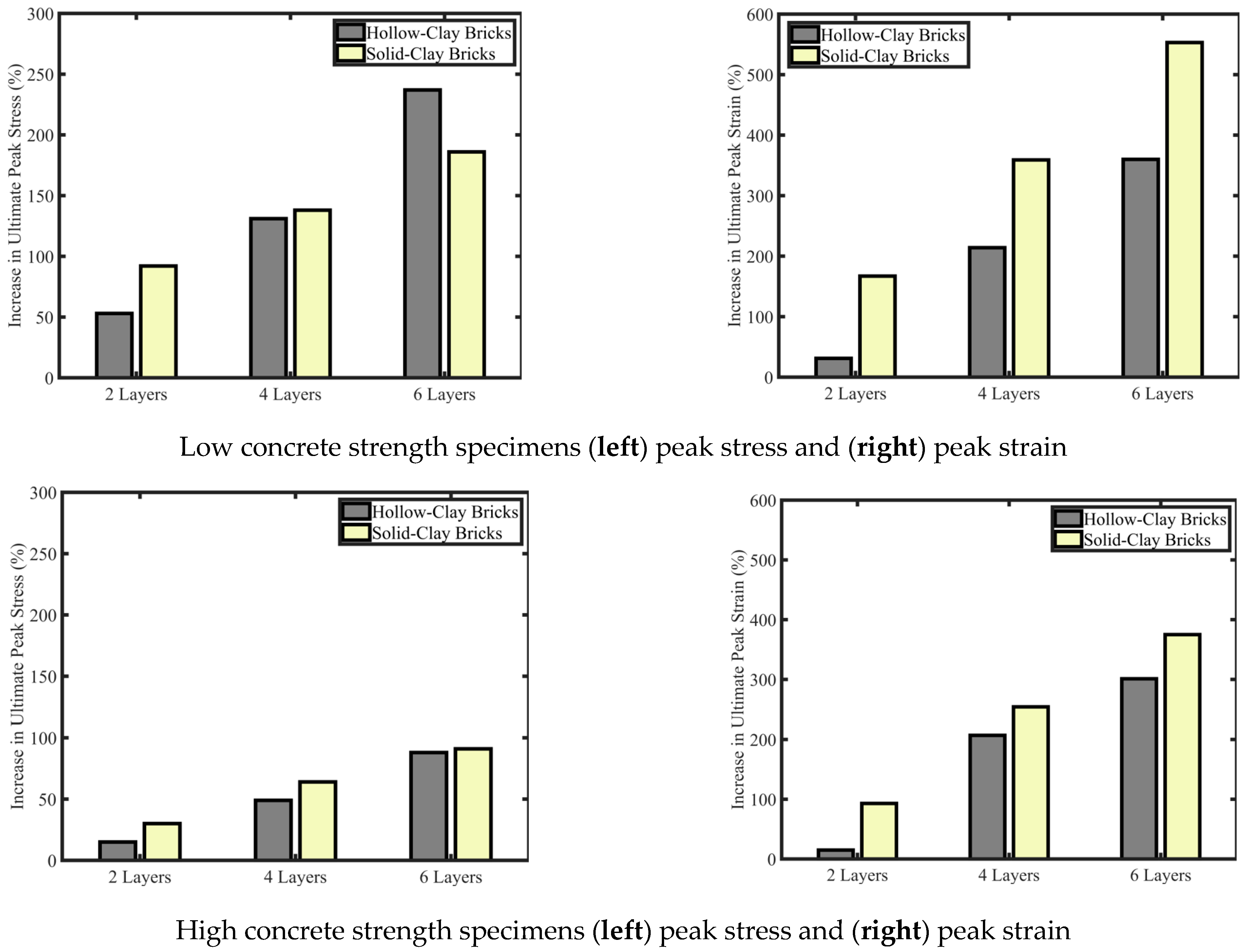
3.3. Effect of LS-GFRP Layers & Concrete Strength
3.4. Effect of Type of Bricks
4. Analytical Investigations
4.1. Compressive Strength Models
4.2. Ultimate Strain Models
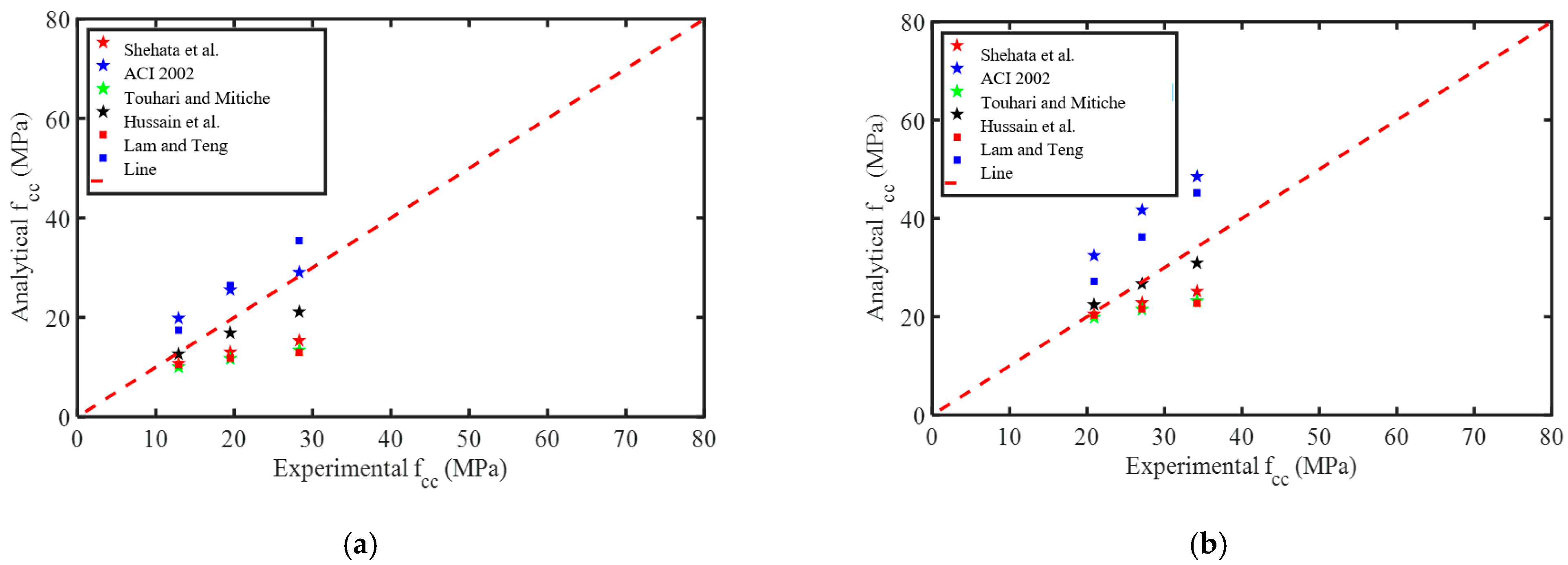
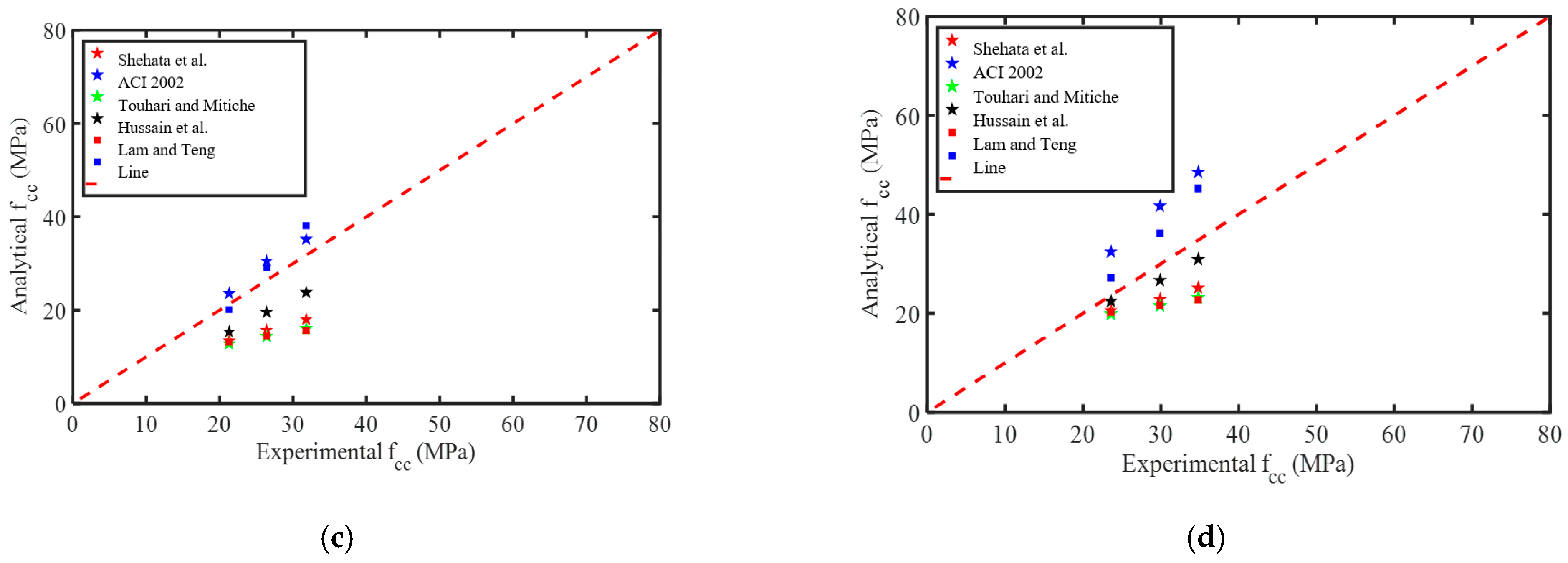
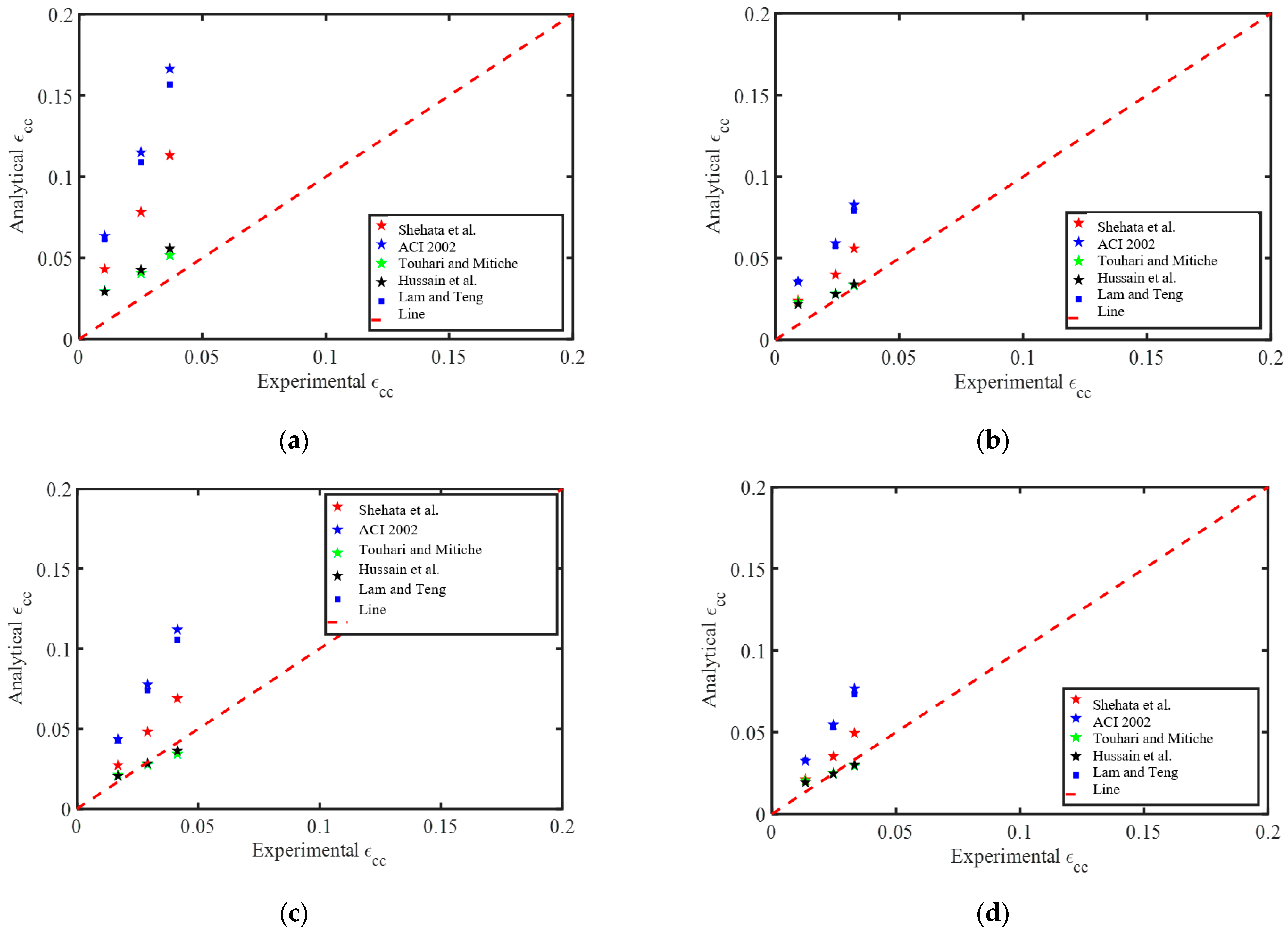
4.3. Assessment of Existing Stress-Strain Models
5. Conclusions and Suggestions for Future Research
- LC-GFRP sheets were able to enhance peak axial stress and corresponding strain of RBAC specimens. This improvement was found to correlate positively with the number of external LC-GFRP layers. For the case of 4 and 6 LC-GFRP layers, a bilinear stress-strain relation was observed exhibiting significant axial ductility.
- The increase in ultimate stress and strain of RBAC specimens was dependent upon the inherent unconfined concrete strength. For low strength specimens, increase in both ultimate compressive stress and corresponding strain was higher than that observed in high strength concrete specimens.
- For solid clay brick aggregate concrete, increase in ultimate compressive stress and corresponding strain was found higher than that in hollow-clay brick aggregate concrete. Therefore, it can be established that for same specimen type i.e., concrete strength, size, and mix ratio, specimens constructed with hollow brick aggregates may require higher LC-GFRP amounts to reach similar strength levels as those of solid-clay brick aggregate concrete.
- Several existing confined axial stress-strain models were assessed to check their accuracy for LC-GFRP confined specimens. It was found that the model of Hussain et al. [39] provided closest approximations of experimental ultimate compressive stresses. Whereas none of the existing models could predict experimental peak strains with good accuracy.
- The proposed LC-GFRP composites can be widely used to enhance the strength and ductility of reinforced concrete columns, beams, beam-column joints and to replace the existing high-cost carbon fiber reinforced polymer composites.
- Future studies must be carried to study the influence of steel reinforcement on the strength of the LC-GFRP composites confined reinforced concrete columns and use of brick aggregates on the adhesion of the reinforcing steel to the concrete.
Author Contributions
Funding
Institutional Review Board Statement
Acknowledgments
Conflicts of Interest
References
- Zheng, H.; Yuan-Feng, L. Probe into the Resource Utilization of Abandoned. Google Scholar, Henan Buildings. 2019. Available online: https://scholar.google.com/scholar?hl=en&as_sdt=0%2C5&q=Probe+into+the+resource+utilization+of+abandoned+clay+bricks+in+construction&btnG= (accessed on 28 April 2022).
- Xiao, J.; Li, W.; Fan, Y.; Huang, X. An overview of study on recycled aggregate concrete in China (1996–2011). Constr. Build. Mater. 2012, 31, 364–383. [Google Scholar] [CrossRef]
- Hamad, B.S.; Dawi, A.H. Sustainable normal and high strength recycled aggregate concretes using crushed tested cylinders as coarse aggregates. Case Stud. Constr. Mater. 2017, 7, 228–239. [Google Scholar] [CrossRef]
- Ohemeng, E.A.; Ekolu, S.O. Comparative analysis on costs and benefits of producing natural and recycled concrete aggregates: A South African case study. Case Stud. Constr. Mater. 2020, 13, e00450. [Google Scholar] [CrossRef]
- Hussain, I.; Ali, B.; Akhtar, T.; Jameel, M.S.; Raza, S.S. Comparison of mechanical properties of concrete and design thickness of pavement with different types of fiber-reinforcements (steel, glass, and polypropylene). Case Stud. Constr. Mater. 2020, 13, e00429. [Google Scholar] [CrossRef]
- Poon, C.S.; Kou, S.C.; Lam, L. Use of recycled aggregates in molded concrete bricks and blocks. Constr. Build. Mater. 2002, 16, 281–289. [Google Scholar] [CrossRef]
- Sagoe-Crentsil, K.; Brown, T.; Taylor, A. Performance of concrete made with commercially produced coarse recycled concrete aggregate. Cem. Concr. Res. 2001, 31, 707–712. [Google Scholar] [CrossRef]
- RILEM—Specifications for Concrete with Recycled Aggregates. Mater. Struct. 1994, 27, 557–559. Available online: https://www.rilem.net/publication/publication/279?id_papier=6137 (accessed on 28 April 2022). [CrossRef]
- Manfredi, S.; Pant, R.; Pennington, D.W.; Versmann, A. Supporting environmentally sound decisions for waste man-agement with LCT and LCA. Int. J. Life Cycle Assess. 2011, 16, 937–939. [Google Scholar] [CrossRef]
- Chaiyasarn, K.; Hussain, Q.; Joyklad, P.; Rodsin, K. New hybrid basalt/E-glass FRP jacketing for enhanced confinement of recycled aggregate concrete with clay brick aggregate. Case Stud. Constr. Mater. 2021, 14, e00507. [Google Scholar] [CrossRef]
- Ge, Z.; Wang, Y.; Sun, R.; Wu, X.; Guan, Y. Influence of ground waste clay brick on properties of fresh and hardened concrete. Constr. Build. Mater. 2015, 98, 128–136. [Google Scholar] [CrossRef]
- Zhao, C.; Zheng, C.; Zhao, M.; Tu, Y.; Liu, J. Multivariate autoregressive models and kernel learning algorithms for classifying driving mental fatigue based on electroencephalographic. Expert Syst. Appl. 2011, 38, 1859–1865. [Google Scholar] [CrossRef]
- Gao, C.; Huang, L.; Yan, L.; Jin, R.; Chen, H. Mechanical properties of recycled aggregate concrete modified by nano-particles. Constr. Build. Mater. 2020, 241, 118030. [Google Scholar] [CrossRef]
- Khalaf, F.M. Using Crushed Clay Brick as Coarse Aggregate in Concrete. J. Mater. Civ. Eng. 2006, 18, 518–526. [Google Scholar] [CrossRef]
- Khalaf, F.M.; DeVenny, A.S. Performance of Brick Aggregate Concrete at High Temperatures. J. Mater. Civ. Eng. 2004, 16, 556–565. [Google Scholar] [CrossRef]
- Hansen, T.C. Recycling of Demolished Concrete and Masonry: Report of Technical Committee 37-DRC, Demolition and Reuse of Concrete, E & FN Spon. 1992. Available online: https://www.routledge.com/Recycling-of-Demolished-Concrete-and-Masonry/Hansen/p/book/9780419158202 (accessed on 28 April 2022).
- Khalaf, F.M.; Devenny, A.S. Recycling of Demolished Masonry Rubble as Coarse Aggregate in Concrete: Review. J. Mater. Civ. Eng. 2004, 16, 331–340. [Google Scholar] [CrossRef]
- Akhtaruzzaman, A.A.; Hasnat, A. Properties of Concrete Using Crushed Brick as Aggregate. Concr. Int. 1983, 5, 58–63. [Google Scholar]
- Debieb, F.; Kenai, S. The use of coarse and fine crushed bricks as aggregate in concrete. Constr. Build. Mater. 2008, 22, 886–893. [Google Scholar] [CrossRef]
- Yang, Y.-F.; Ma, G.-L. Experimental behaviour of recycled aggregate concrete filled stainless steel tube stub columns and beams. Thin-Wall. Struct. 2013, 66, 62–75. [Google Scholar] [CrossRef]
- Adamson, M.; Razmjoo, A.; Poursaee, A. Durability of concrete incorporating crushed brick as coarse aggregate. Constr. Build. Mater. 2015, 94, 426–432. [Google Scholar] [CrossRef] [Green Version]
- Vrijders, J.; Desmyter, J. Een Hoogwaardig Gebruik van Puingranulaten Stimuleren, OVAM Mechelen. 2008. Available online: https://www.ovam.be/sites/default/files/FILE1297949793382080915_Hoogwaardig_gebruik_puingranulaten.pdf (accessed on 22 April 2022).
- Poon, C.S.; Chan, D. Feasible use of recycled concrete aggregates and crushed clay brick as unbound road sub-base. Constr. Build. Mater. 2006, 20, 578–585. [Google Scholar] [CrossRef]
- Gao, C.; Huang, L.; Yan, L.; Kasal, B.; Li, W. Behavior of glass and carbon FRP tube encased recycled aggregate concrete with recycled clay brick aggregate. Compos. Struct. 2016, 155, 245–254. [Google Scholar] [CrossRef]
- Tang, Z.; Li, W.; Tam, V.W.; Yan, L. Mechanical performance of CFRP-confined sustainable geopolymeric recycled concrete under axial compression. Eng. Struct. 2020, 224, 111246. [Google Scholar] [CrossRef]
- Hassan, S.H.; Rasheed, L.S.; Salih, S.A. The repair of recycled crushed brick aggregate RC beams with shear deficiencies with CFRP. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1067, 012048. [Google Scholar] [CrossRef]
- Chen, G.M.; Zhang, J.J.; Jiang, T.; Lin, C.J.; He, Y.H. Compressive Behavior of CFRP-Confined Recycled Aggregate Concrete in Different-Sized Circular Sections. J. Compos. Constr. 2018, 22, 04018021. [Google Scholar] [CrossRef]
- Islam, M.; Choudhury, M.S.I.; Amin, A.F.M.S. Dilation Effects in FRP-Confined Square Concrete Columns Using Stone, Brick, and Recycled Coarse Aggregates. J. Compos. Constr. 2015, 20, 04015017. [Google Scholar] [CrossRef]
- Yooprasertchai, E.; Dithaem, R.; Arnamwong, T.; Sahamitmongkol, R.; Jadekittichoke, J.; Joyklad, P.; Hussain, Q. Remediation of punching shear failure using glass fiber reinforced polymer (GFRP) rods. Polymers 2021, 13, 2369. [Google Scholar] [CrossRef] [PubMed]
- Iskander, M.; Hassan, M. State of the Practice Review in FRP Composite Piling. J. Compos. Constr. 1998, 2, 116–120. [Google Scholar] [CrossRef]
- Yoddumrong, P.; Rodsin, K.; Katawaethwarag, S. Seismic strengthening of low-strength RC concrete columns using low-cost glass fiber reinforced polymers (GFRPs). Case Stud. Constr. Mater. 2020, 13, e00383. [Google Scholar] [CrossRef]
- Rodsin, K.; Hussain, Q.; Suparp, S.; Nawaz, A. Compressive behavior of extremely low strength concrete confined with low-cost glass FRP composites. Case Stud. Constr. Mater. 2020, 13, e00452. [Google Scholar] [CrossRef]
- ASTM C1314-21; Standard Test Method for Compressive Strength of Masonry Prisms. ASTM International: West Conshohocken, PA, USA, 2021.
- ASTM C140/C140M-22a; Standard Test Methods for Sampling and Testing Concrete Masonry Units and Related Units. 2022. Available online: https://www.astm.org/c0140-c0140m-22a.html (accessed on 23 April 2022).
- ASTM D3013-13; Standard Specification for Epoxy Molding Compounds. 2021. Available online: https://www.astm.org/d3013-13.html (accessed on 30 April 2022).
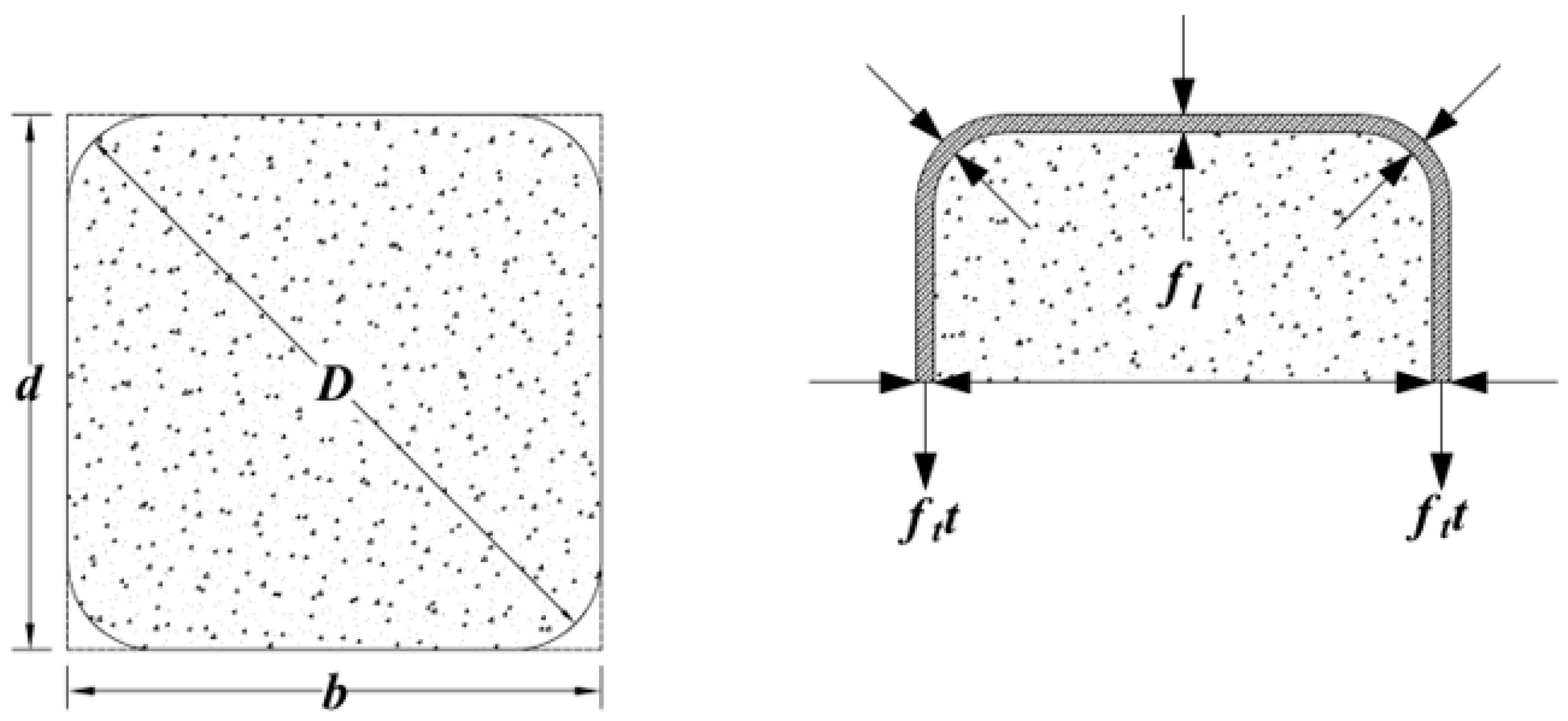
- Mostofinejad, D.; Moshiri, N.; Mortazavi, N. Effect of corner radius and aspect ratio on compressive behavior of rec-tangular concrete columns confined with CFRP. Mater. Struct. Mater. Construct. 2015, 48, 107–122. [Google Scholar] [CrossRef]
- Wang, L.-M.; Wu, Y.-F. Effect of corner radius on the performance of CFRP-confined square concrete columns: Test. Eng. Struct. 2008, 30, 493–505. [Google Scholar] [CrossRef]
- Soudki, K.; Alkhrdaji, T. Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Concrete Structures (ACI 440.2R-02). In Structures Congress and Exposition; ASCE: Reston, VA, USA, 2005; pp. 1–8. [Google Scholar] [CrossRef] [Green Version]
- Hussain, Q.; Ruangrassamee, A.; Tangtermsirikul, S.; Joyklad, P.; Wijeyewickrema, A.C. Low-Cost Fiber Rope Reinforced Polymer (FRRP) Confinement of Square Columns with Different Corner Radii. Buildings 2021, 11, 355. [Google Scholar] [CrossRef]
- Saleem, S.; Hussain, Q.; Pimanmas, A. Compressive Behavior of PET FRP–Confined Circular, Square, and Rectangular Concrete Columns. J. Compos. Constr. 2016, 21, 04016097. [Google Scholar] [CrossRef]
- Shehata, I.A.E.M.; Carneiro, L.; Shehata, L.C.D. Strength of short concrete columns confined with CFRP sheets. Mater. Struct. 2002, 35, 50–58. [Google Scholar] [CrossRef]
- Pimanmas, A.; Hussain, Q.; Panyasirikhunawut, A.; Rattanapitikon, W. Axial strength and deformability of concrete confined with natural fibre-reinforced polymers. Mag. Concr. Res. 2018, 71, 55–70. [Google Scholar] [CrossRef]
- Richart, F.E.; Brandtzæg, A.; Brown, R.L. A Study of the Failure of Concrete under Combined Compressive Stresses, Bulletins—Engineering Experiment Station. 1928. Available online: http://hdl.handle.net/2142/4277 (accessed on 6 March 2022).
- Miyauchi, K. Estimation of Strengthening Effects with Crbon Feber Sheet for Concrete Column. In Proceedings of the 3rd International Symposium on Non-Metallic (FRP) Reinforcement for Concrete Structures, Sapporo, Japan, 14–16 October 1997; Japan Concrete Institute: Tokyo, Japan, 1997; pp. 217–224. Available online: https://ci.nii.ac.jp/naid/80009963999/ (accessed on 6 March 2022).
- Benzaid, R.; Mesbah, H.; Chikh, N.E. FRP-confined Concrete Cylinders: Axial Compression Experiments and Strength Model. J. Reinf. Plast. Compos. 2010, 29, 2469–2488. [Google Scholar] [CrossRef]
- Behavior of RC Deep Beams Strengthened in Shear using Glass Fiber Reinforced Polymer with Mechanical Anchors. In Proceedings of the International Conference on Environment and Civil Engineering (ICEACE’2015), Pattaya, Thailand, 24–25 April 2015. [CrossRef]
- Touhari, M.; Mitiche, R.K. Strength model of FRP confined concrete columns based on analytical analysis and exper-imental test. Int. J. Struct. Integr. 2020, 11, 82–106. [Google Scholar] [CrossRef]
- Mirmiran, A.; Shahawy, M.; Samaan, M.; El Echary, H.; Mastrapa, J.C.; Pico, O. Effect of Column Parameters on FRP-Confined Concrete. J. Compos. Constr. 1998, 2, 175–185. [Google Scholar] [CrossRef]
- Lam, L.; Teng, J.G. Strength Models for Fiber-Reinforced Plastic-Confined Concrete. J. Struct. Eng. 2002, 128, 612–623. [Google Scholar] [CrossRef]


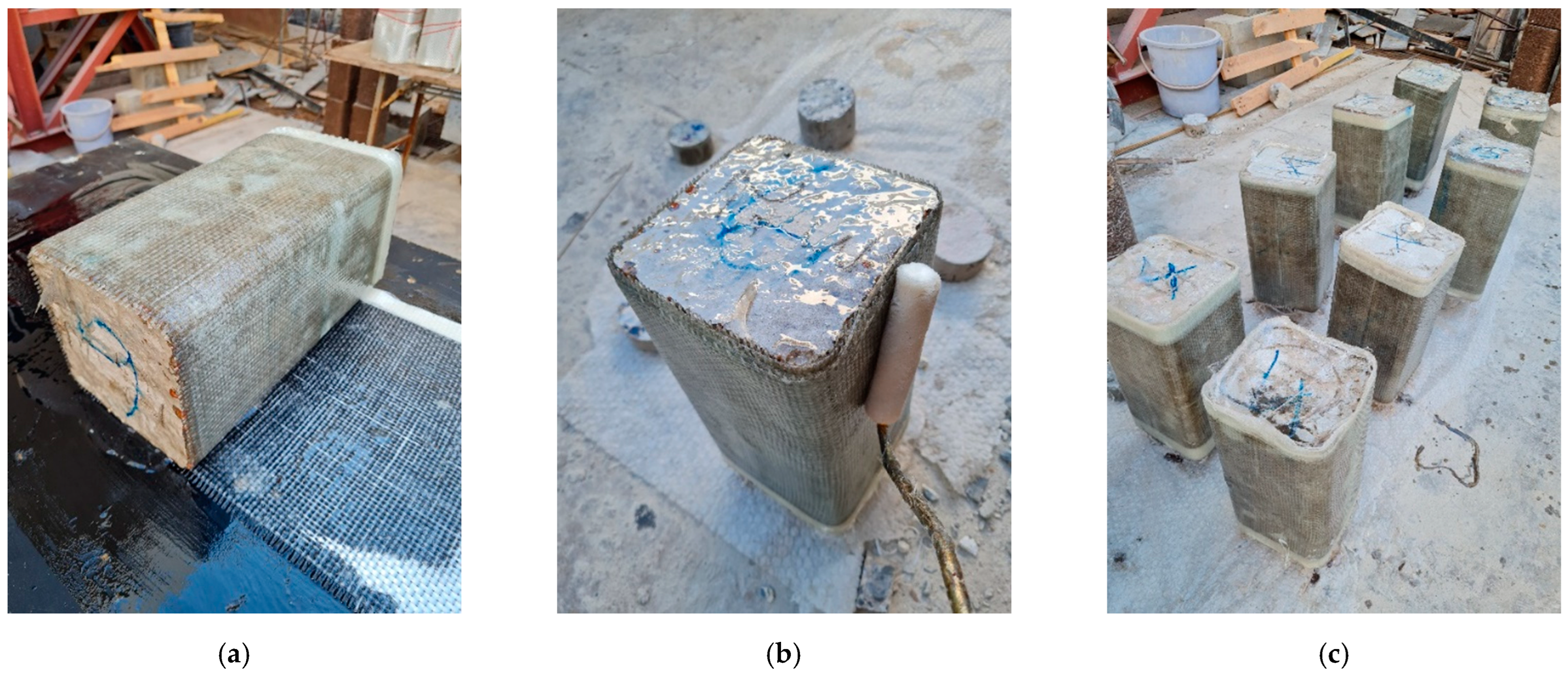
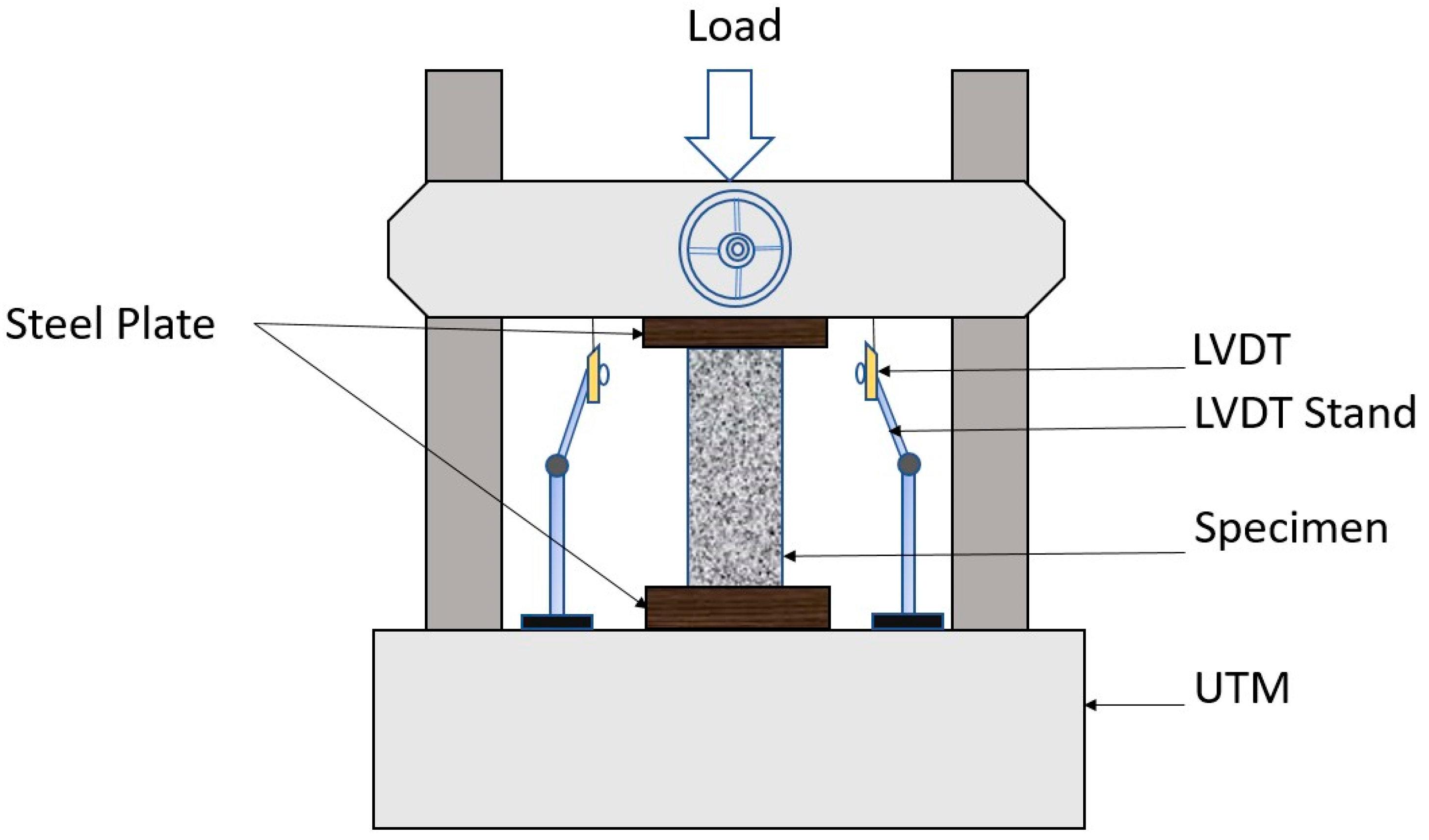


| Specimens | Concrete Strength | Brick Type | GFRP Layers | Number of Specimens |
|---|---|---|---|---|
| LS-CBA-CON | LS | Type A | - | 2 |
| LS-CBA-2L | LS | Type A | 2 | 2 |
| LS-CBA-4L | LS | Type A | 4 | 2 |
| LS-CBA-6L | LS | Type A | 6 | 2 |
| HS-CBA-CON | HS | Type A | - | 2 |
| HS-CBA-2L | HS | Type A | 2 | 2 |
| HS-CBA-4L | HS | Type A | 4 | 2 |
| HS-CBA-6L | HS | Type A | 6 | 2 |
| LS-CBB-CON | LS | Type B | - | 2 |
| LS-CBB-2L | LS | Type B | 2 | 2 |
| LS-CBB-4L | LS | Type B | 4 | 2 |
| LS-CBB-6L | LS | Type B | 6 | 2 |
| HS-CBB-CON | HS | Type B | - | 2 |
| HS-CBB-2L | HS | Type B | 2 | 2 |
| HS-CBB-4L | HS | Type B | 4 | 2 |
| HS-CBB-6L | HS | Type B | 6 | 2 |
| Mix Ingredients (kg/m3) | Low Strength Concrete (15 MPa) | High Strength Concrete (35 MPa) |
|---|---|---|
| Cement | 242 | 444 |
| Fine aggregates | 726 | 605 |
| Natural coarse aggregates | 605 | 504 |
| Clay brick aggregates | 605 | 504 |
| Type of Bricks | Compressive Strength of Bricks (MPa) | Water Absorption of Bricks (%) | |
|---|---|---|---|
| Type A | 120 | 3.14 | 23.27 |
| Type B | 140 | 8.10 | 16.58 |
| Composite | Tensile Stress (MPa) | Ultimate Strain (%) | Elastic Modulus (GPa) | Standard Deviation |
|---|---|---|---|---|
| Epoxy | 17.20 | 0.632 | 2.72 | 1.09 |
| LC-GFRP | 377.64 | 2.040 | 18.70 | 1.91 |
| Specimens | Ultimate Stress (MPa) | Increase in Ultimate Stress (%) | Ultimate Strain | Increase in Ultimate Strain (%) |
|---|---|---|---|---|
| LS-CBA-CON | 8.40 | - | 0.0080 | - |
| LS-CBA-2L | 12.9 | 53 | 0.0104 | 31 |
| LS-CBA-4L | 19.5 | 131 | 0.0251 | 214 |
| LS-CBA-6L | 28.3 | 237 | 0.0368 | 360 |
| HS-CBA-CON | 18.2 | - | 0.0079 | - |
| HS-CBA-2L | 20.9 | 15 | 0.0091 | 15 |
| HS-CBA-4L | 27.1 | 49 | 0.0242 | 207 |
| HS-CBA-6L | 34.2 | 88 | 0.0317 | 301 |
| LS-CBB-CON | 11.1 | - | 0.0063 | - |
| LS-CBB-2L | 21.3 | 92 | 0.0169 | 167 |
| LS-CBB-4L | 26.4 | 138 | 0.0291 | 359 |
| LS-CBB-6L | 31.8 | 186 | 0.0414 | 553 |
| HS-CBB-CON | 18.2 | - | 0.0070 | - |
| HS-CBB-2L | 23.6 | 30 | 0.0135 | 93 |
| HS-CBB-4L | 29.9 | 64 | 0.0248 | 254 |
| HS-CBB-6L | 34.8 | 91 | 0.0333 | 375 |
| ID | Model | Ultimate Stress | Ultimate Strain |
|---|---|---|---|
| 1 | Shehata et al. [41] | ||
| 2 | ACI 2002 [38] | ||
| 3 | Touhari and Mitiche [47] | ||
| 4 | Hussain et al. [39] | ||
| 5 | Mirmiran et al. [48] | - | |
| 7 | Lam and Teng [49] |
| ID/Model | Shihata et al. [41] | ACI 2002 [28] | Touhari and Mitiche [47] | Hussain et al. [39] | Mirmiran et al. [48] | Lam & Teng [49] | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| LS-CBA-2L | 12.9 | 0.83 | 4.14 | 1.53 | 6.10 | 0.78 | 2.83 | 0.98 | 2.81 | 0.81 | - | 1.35 | 5.92 | |
| LS-CBA-4L | 19.5 | 0.67 | 3.11 | 1.31 | 4.58 | 0.60 | 1.62 | 0.87 | 1.69 | 0.61 | - | 1.35 | 4.34 | |
| LS-CBA-6L | 28.3 | 0.54 | 3.07 | 1.02 | 4.52 | 0.47 | 1.40 | 0.75 | 1.51 | 0.46 | - | 1.25 | 4.25 | |
| HS-CBA-2L | 20.9 | 0.98 | 2.63 | 1.55 | 3.89 | 0.95 | 2.55 | 1.08 | 2.40 | 0.97 | - | 1.30 | 3.91 | |
| HS-CBA-4L | 27.1 | 0.84 | 1.65 | 1.54 | 2.44 | 0.79 | 1.17 | 0.98 | 1.15 | 0.80 | - | 1.34 | 2.37 | |
| HS-CBA-6L | 34.2 | 0.73 | 1.76 | 1.42 | 2.61 | 0.68 | 1.05 | 0.90 | 1.07 | 0.67 | - | 1.32 | 2.49 | |
| LS-CBB-2L | 21.3 | 0.63 | 1.61 | 1.11 | 2.58 | 0.60 | 1.25 | 0.72 | 1.21 | 0.62 | - | 0.94 | 2.52 | |
| LS-CBB-4L | 26.4 | 0.60 | 1.65 | 1.16 | 2.67 | 0.55 | 0.95 | 0.74 | 0.98 | 0.55 | - | 1.10 | 2.54 | |
| LS-CBB-6L | 31.8 | 0.57 | 1.66 | 1.11 | 2.70 | 0.54 | 0.83 | 0.75 | 0.88 | 0.49 | - | 1.20 | 2.55 | |
| HS-CBB-2L | 23.6 | 0.87 | 1.57 | 1.37 | 2.41 | 0.83 | 1.52 | 0.95 | 1.43 | 0.86 | - | 1.15 | 2.42 | |
| HS-CBB-4L | 29.9 | 0.76 | 1.43 | 1.39 | 2.20 | 0.73 | 1.01 | 0.89 | 0.99 | 0.72 | - | 1.21 | 2.14 | |
| HS-CBB-6L | 34.8 | 0.72 | 1.49 | 1.40 | 2.30 | 0.70 | 0.89 | 0.88 | 0.90 | 0.65 | - | 1.30 | 2.20 | |
| Mean = | 0.73 | 2.14 | 1.33 | 3.25 | 0.68 | 1.42 | 0.87 | 1.42 | 0.68 | - | 1.23 | 3.14 | ||
| Standard Deviation = | 0.13 | 0.88 | 0.18 | 1.23 | 0.14 | 0.65 | 0.11 | 0.61 | 0.15 | - | 0.12 | 1.18 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rodsin, K.; Ali, N.; Joyklad, P.; Chaiyasarn, K.; Al Zand, A.W.; Hussain, Q. Improving Stress-Strain Behavior of Waste Aggregate Concrete Using Affordable Glass Fiber Reinforced Polymer (GFRP) Composites. Sustainability 2022, 14, 6611. https://doi.org/10.3390/su14116611
Rodsin K, Ali N, Joyklad P, Chaiyasarn K, Al Zand AW, Hussain Q. Improving Stress-Strain Behavior of Waste Aggregate Concrete Using Affordable Glass Fiber Reinforced Polymer (GFRP) Composites. Sustainability. 2022; 14(11):6611. https://doi.org/10.3390/su14116611
Chicago/Turabian StyleRodsin, Kittipoom, Nazam Ali, Panuwat Joyklad, Krisada Chaiyasarn, Ahmed W. Al Zand, and Qudeer Hussain. 2022. "Improving Stress-Strain Behavior of Waste Aggregate Concrete Using Affordable Glass Fiber Reinforced Polymer (GFRP) Composites" Sustainability 14, no. 11: 6611. https://doi.org/10.3390/su14116611
APA StyleRodsin, K., Ali, N., Joyklad, P., Chaiyasarn, K., Al Zand, A. W., & Hussain, Q. (2022). Improving Stress-Strain Behavior of Waste Aggregate Concrete Using Affordable Glass Fiber Reinforced Polymer (GFRP) Composites. Sustainability, 14(11), 6611. https://doi.org/10.3390/su14116611








