Brief Literature Review and Classification System of Reliability Methods for Evaluating the Stability of Earth Slopes
Abstract
1. Introduction
2. Historical Background
3. Contemporary Research
3.1. Methodology
3.2. System Reliability
3.3. Uncertainties of Soil Parameters
4. Applications
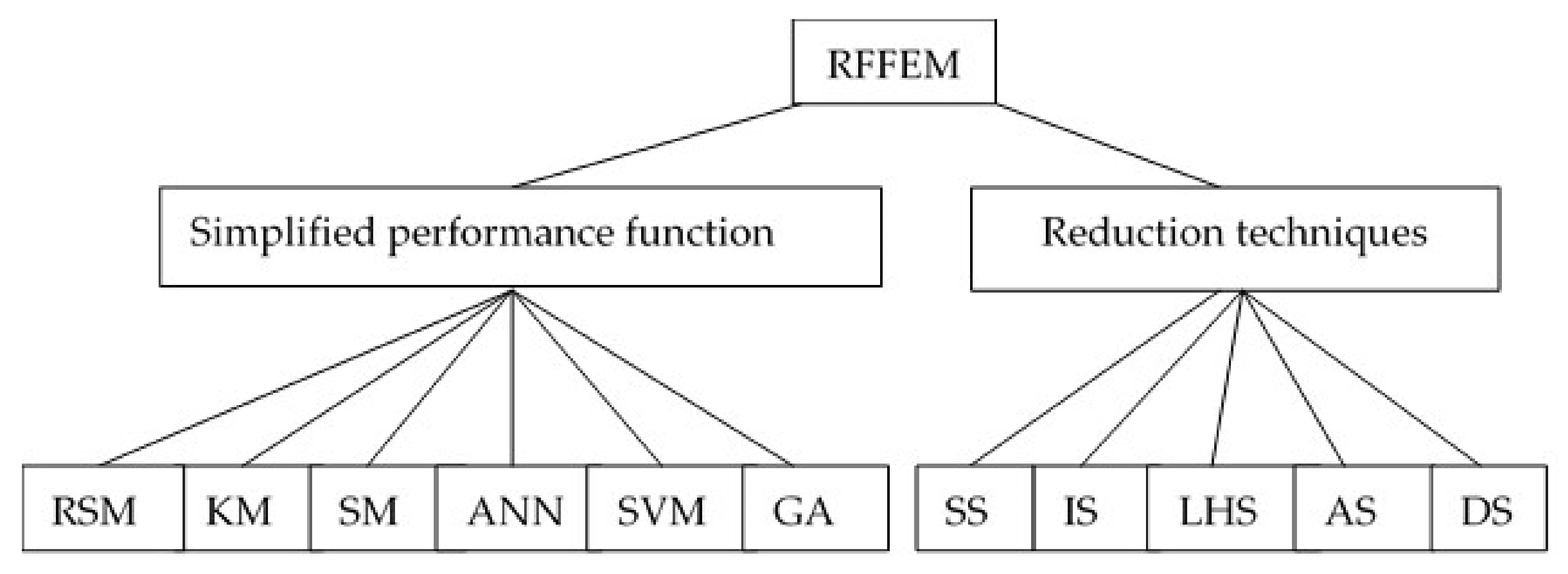
5. Classification System
- level 1—partial factor approach—employs only one “characteristic” value of each uncertainty parameter;
- level 2—estimates two values of each uncertainty parameter, usually the mean value, standard deviation, and the correlation between these parameters;
- level 3—best estimate of the probability of failure—knowledge of the join distribution of all uncertain parameters is required;
- level 4—reliability methods appropriate for structures of major economic importance, taking into account the structures’ economic value, including the consequences of their failure.
6. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Fellenius, W. Calculations of the Stability of Earth Dams. In Proceedings of the Second Congress of Large Dams, Washington, DC, USA, 7–12 September 1936; Volume 4, pp. 445–463. [Google Scholar]
- Bishop, A.W. The use of the slip circle in the stability analysis of slopes. Geotechnique 1955, 5, 7–17. [Google Scholar] [CrossRef]
- Janbu, N. Slope Stability Computation, Embankment- Dam Engineering: Casagrande Volume; John Wiley & Sons: New York, NY, USA, 1973; pp. 47–86. [Google Scholar]
- Nonveiller, E. The stability analysis of slopes with a slide surface of general shape. In Proceedings of the 6th International Conference on Soil Mechanics and Foundation Engineering, Montreal, QC, Canada, 8–15 September 1965; Volume 2, pp. 522–525. [Google Scholar]
- Spencer, E. A method of analysis of the stability of embankments assuming parallel inter-slice forces. Geotechnique 1967, 17, 11–26. [Google Scholar] [CrossRef]
- Morgenstern, N.R.; Price, V.E. The Analysis of the Stability of General Slip Surfaces. Geotechnique 1965, 15, 77–93. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Humpheson, C.; Lewis, R.W. Associated and Non-Associated Visco-Plasticity and Plasticity in Soil Mechanics. Geotechnique 1975, 25, 671–689. [Google Scholar] [CrossRef]
- Dawson, E.M.; Roth, W.H.; Drescher, A. Slope Stability Analysis by Strength Reduction. Geotechnique 1999, 49, 835–840. [Google Scholar] [CrossRef]
- Griffiths, D.V.; Lane, P.A. Slope stability analysis by finite elements. Geotechnics 1999, 49, 387–403. [Google Scholar] [CrossRef]
- Cheng, Y.M. Location of critical failure surface and some further studies on slope stability analysis. Comput. Geotech. 2003, 30, 255–267. [Google Scholar] [CrossRef]
- Drucker, D.C.; Prager, W. Soil mechanics and plastic analysis or limit design. Q. Appl. Math. 1952, 10, 157–165. [Google Scholar] [CrossRef]
- Chen, W.F.; Giger, M.W.; Fang, H.Y. On the limit analysis of stability of slopes. Soils Found. 1969, 9, 23–32. [Google Scholar] [CrossRef]
- Donald, I.B.; Chen, Z.Y. Slope stability analysis by the upper bound approach: Fundamentals and methods. Can. Geotech. J. 1997, 34, 853–862. [Google Scholar] [CrossRef]
- Michalowski, R.L. Slope stability analysis: A kinematical approach. Géotechnique 1995, 45, 283–293. [Google Scholar] [CrossRef]
- Przewłócki, J. Some comments on slope stability evaluation. Part I: Deterministic analysis. Inżynieria Morska Geotech. 2004, 2, 141–149. [Google Scholar]
- Wu, T.H.; Kraft, L.M. Safety Analysis of Slopes. J. Soil Mech. Found. Div. 1970, 96, 609–630. [Google Scholar] [CrossRef]
- Cornell, C.A. First-order uncertainty analysis of soil deformation and stability. In Proceedings of the 1st International Conference Applications of Statistics and Probability in Soil and Structural, Hong Kong, 13–16 September 1971; pp. 129–144. Available online: https://trid.trb.org/view/128568 (accessed on 16 July 1974).
- Alonso, E.E. Risk analysis of slopes and its application to slopes in Canadian sensitive clays. Geotechique 1976, 26, 453–472. [Google Scholar] [CrossRef]
- Tang, W.H.; Yucemen, M.S.; Ang, A.H.S. Probability based short term design of soil slopes. Can. Geotech. J. 1976, 13, 201–215. [Google Scholar] [CrossRef]
- Vanmarcke, E.H. Reliability of earth slopes. J. Geotech. Eng. 1977, 103, 1227–1246. [Google Scholar]
- Chowdhury, R.N.; Grivas, D. Probabilistic model of progressive failure of slopes. J. Geot. Eng. 1982, 108, 803–917. [Google Scholar] [CrossRef]
- Tobutt, D.C. Monte Carlo simulation methods for slope stability. Comput. Geosci. 1982, 8, 199–209. [Google Scholar] [CrossRef]
- Chowdhury, R.N.; Tang, W.H.; Sidi, I. Reliability model of progressive slope failure. Géotechnique 1987, 37, 467–481. [Google Scholar] [CrossRef]
- El-Ramly, H.; Morgenstern, N.R.; Cruden, D.M. Probabilistic slope stability analysis for practice. Can. Geotech. 2002, 39, 665–683. [Google Scholar] [CrossRef]
- Griffiths, D.V.; Fenton, G.A. Probabilistic Slope Stability Analysis by Finite Elements. J. Geotech. Geoenviron. Eng. 2004, 130, 507–518. [Google Scholar] [CrossRef]
- Christian, J.T.; Ladd, C.C.; Baecher, G.B. Reliability applied to slope stability analysis. J. Geot. Eng. 1994, 120, 2180–2207. [Google Scholar] [CrossRef]
- Hassan, A.M.; Wolff, T.F. Search algorithm for minimum reliability index of earth slopes. J. Geotech. Geoenviron. Eng. 1999, 125, 301–308. [Google Scholar] [CrossRef]
- Low, B.K.; Tang, W.H. Probabilistic Slope Analysis Using Janbu’s Generalized Procedure of Slices. Comput. Geotech. 1997, 21, 121–142. [Google Scholar] [CrossRef]
- Low, B.K.; Gilbert, R.B.; Wright, S.G. Slope reliability analysis using generalized method of slices. J. Geotech. Geoenviron. Eng. 1998, 124, 350–362. [Google Scholar] [CrossRef]
- Cho, S.E. Effects of spatial variability of soil properties on slope stability. Eng. Geol. 2007, 92, 97–109. [Google Scholar] [CrossRef]
- Cho, S.E. First-order reliability analysis of slope considering multiple failure modes. Eng. Geol. 2013, 154, 98–105. [Google Scholar] [CrossRef]
- Knabe, W.; Przewłócki, J. Probabilistic Slope Stability Analysis; Institute of Hydro-Engineering of Polish Academy of Sciences: Gdańsk, Poland, 1990. [Google Scholar]
- Przewłócki, J. Some comments on slope stability evaluation. Part II: Probabilistic analysis. Inżynieria Morska Geotech. 2004, 3, 141–149. [Google Scholar]
- Cornell, C.A. Reliability-based earthquake-resistant design—The future. In Proceedings of the Eleventh World Conference on Earthquake Engineering, Acapulco, Mexico, 23–28 June 1966. [Google Scholar]
- Refice, A.; Capolongo, D. Probabilistic modeling of uncertainties in earthquake-induced landslide hazard assessment. Comput. Geosci. 2002, 28, 735–749. [Google Scholar] [CrossRef]
- Zhang, J.; Huang, H.W.; Juang, C.H.; Li, D.Q. Extension of Hassan and Wolff method for system reliability analysis of soil slopes. Eng. Geol. 2013, 160, 81–88. [Google Scholar] [CrossRef]
- Zhang, L.L.; Zhang, L.M.; Tang, W.H. Rainfall-induced slope failure considering variability of soil properties. Geotechnique 2006, 55, 215–220. [Google Scholar] [CrossRef]
- Zhang, J.; Huang, H.W.; Zhang, L.M.; Zhu, H.H.; Shi, B. Probabilistic prediction of rainfall-induced slope failure using a mechanics-based model. Eng. Geol. 2014, 168, 129–140. [Google Scholar] [CrossRef]
- Bray, J.D.; Travasarou, T. Simplified Procedure for Estimating Earthquake-Induced Deviatoric Slope Displacements. J. Geotech. Geoenvironmental Eng. 2007, 133, 381–392. [Google Scholar] [CrossRef]
- Wasowski, J.; Keefer, D.K.; Lee, C.T. Toward the next generation of research on earthquake-induced landslides: Current issues and future challenges. Eng. Geol. 2011, 122, 1–8. [Google Scholar] [CrossRef]
- Rathje, E.M.; Saygili, G. Estimating Fully Probabilistic Seismic Sliding Displacements of Slopes from a Pseudoprobabilistic Approach. J. Geotech. Geoenviron. Eng. 2011, 137, 208–217. [Google Scholar] [CrossRef]
- Rathje, E.M.; Wang, Y.; Stafford, P.J.; Antonakos, G.; Saygili, G. Probabilistic assessment of the seismic performance of earth slopes. Bull. Earthq. Eng. 2014, 12, 1071–1090. [Google Scholar] [CrossRef]
- Santoso, A.M.; Phoon, K.K.; Quek, S.T. Effects of soil spatial variability on rainfall-induced landslides. Comput. Struct. 2011, 89, 893–900. [Google Scholar] [CrossRef]
- Chiu, C.F.; Yan, W.M.; Yuen, K.V. Reliability analysis of soil-water characteristics curve and its application to slope stability analysis. Eng. Geol. 2012, 135, 83–91. [Google Scholar] [CrossRef]
- Tan, X.H.; Hu, N.; Li, D.; Shen, M.F.; Hou, X.L. Time-Variant Reliability Analysis of Unsaturated Soil Slopes Under Rainfall. Geotech. Geol. Eng. 2013, 31, 319–327. [Google Scholar] [CrossRef]
- Lee, D.; Lai, M.; WU, J.; Chi, Y.; Ko, W.; Lee, B. Slope management criteria for Alishan Highway based on database of heavy rainfall-induced slope failures. Eng. Geol. 2013, 162, 97–107. [Google Scholar] [CrossRef]
- Dou, H.Q.; Han, T.C.; Gong, X.N.; Qiu, Z.Y.; Li, Z.N. Effects of the spatial variability of permeability on rainfall-induced landslides. Eng. Geol. 2015, 192, 92–100. [Google Scholar] [CrossRef]
- Du, W.; Wang, G. A one-step Newmark displacement model for probabilistic seismic slope displacement hazard analysis. Eng. Geol. 2016, 205, 12–23. [Google Scholar] [CrossRef]
- Vega, J.A.; Hidalgo, C.A. Quantitative risk assessment of landslides triggered by earthquakes and rainfall based on direct costs of urban buildings. Geomorphology 2016, 273, 217–235. [Google Scholar] [CrossRef]
- Xiao, J.; Gong, W.; Martin, J.R.; Shen, M.; Luo, Z. Probabilistic seismic stability analysis of slope at a given site in a specified exposure time. Eng. Geol. 2016, 212, 53–62. [Google Scholar] [CrossRef]
- Xiong, M.; Huang, Y. Stochastic seismic response and dynamic reliability analysis of slopes: A review. Soil Dyn. Earthq. Eng. 2017, 100, 458–464. [Google Scholar] [CrossRef]
- Pang, R.; Xu, B.; Kong, X.; Zhou, Y.; Zou, D. Seismic performance evaluation of high CFRD slopes subjected to near-fault ground motions based on generalized probability density evolution method. Eng. Geol. 2018, 246, 391–401. [Google Scholar] [CrossRef]
- Wang, Y.; Cao, Z.; Au, S.K. Practical reliability analysis of slope stability by advanced Monte Carlo simulations in a spreadsheet. Can. Geotech. J. 2011, 48, 162–172. [Google Scholar] [CrossRef]
- Ali, A.; Lyamin, A.V.; Huang, J.; Li, J.H.; Cassidy, M.J.; Sloan, S.W. Probabilistic stability assessment using adaptive limit analysis and random fields. Acta Geotech. 2017, 12, 937–948. [Google Scholar] [CrossRef]
- Griffiths, D.V.; Huang, J.; Fenton, G.A. Comparison of slope reliability method of analysis. In Proceedings of the Geoflorida Conference Advances in Analysis, Modeling and Design, West Palm Beach, FL, USA, 20–24 February 2010; pp. 1952–1961. [Google Scholar]
- Farah, K.; Ltifi, M.; Hassis, H. A Study of Probabilistic FEMs for a Slope Reliability Analysis Using the Stress Fields. Open Civ. Eng. J. 2015, 9, 196–206. [Google Scholar] [CrossRef][Green Version]
- Johari, A.; Fooladi, H. Comparative study of stochastic slope stability analysis based on conditional and unconditional random field. Comput. Geotech. 2020, 125, 103707. [Google Scholar] [CrossRef]
- Liu, S.Y.; Shao, L.T.; Li, H.J. Slope stability analysis using the limit equilibrium method and two finite element methods. Comput. Geotech. 2015, 63, 291–298. [Google Scholar] [CrossRef]
- Li, L.; Chu, X.S. Multiple response surfaces for slope reliability analysis. Int. J. Numer. Anal. Methods Geomech. 2015, 39, 175–192. [Google Scholar] [CrossRef]
- Zhang, J.; Huang, H.W.; Phoon, K.K. Application of the Kriging-based response surface method to the system reliability of soil slopes. J. Geotech. Geoenviron. Eng. 2013, 139, 651–655. [Google Scholar] [CrossRef]
- Kang, F.; Qing Xu, Q.; Li, J. Slope reliability analysis using surrogate models via new support vector machines with swarm intelligence. Appl. Math. Model. 2016, 40, 6105–6120. [Google Scholar] [CrossRef]
- Cho, S.E. Probabilistic stability analyses of slopes using the ANN-based response surface. Comput. Geotech. 2009, 36, 787–797. [Google Scholar] [CrossRef]
- Kang, F.; Li, J.S.; Li, J.J. System reliability analysis of slopes using least squares support vector machines with particle swarm optimization. Neurocomputing 2016, 209, 46–56. [Google Scholar] [CrossRef]
- Tun, Y.W.; Pedroso, D.M.; Scheuermann, A.; Williams, D.J. Probabilistic reliability analysis of multiple slopes with genetic algorithms. Comput. Geotech. 2016, 77, 68–76. [Google Scholar] [CrossRef]
- Ching, J.; Phoon, K.-K.; Hu, Y.-G. Efficient Evaluation of Reliability for Slopes with Circular Slip Surfaces Using Importance Sampling. J. Geotech. Geoenviron. Eng. 2009, 135, 768–777. [Google Scholar] [CrossRef]
- Li, L.; Wang, Y.; Cao, Z. Probabilistic slope stability analysis by risk aggregation. Eng. Geol. 2014, 176, 57–65. [Google Scholar] [CrossRef]
- Li, D.Q.; Xiao, T.; Cao, Z.J.; Phoon, K.K.; Zhou, C.B. Efficient and consistent reliability analysis of soil slope stability using both limit equilibrium analysis and finite element analysis. Appl. Math. Model. 2016, 40, 5216–5229. [Google Scholar] [CrossRef]
- Huang, J.; Fenton, G.; Griffiths, D.V.; Li, D.; Zhou, C. On the efficient estimation of small failure probability in slopes. Landslides 2016, 14, 491–498. [Google Scholar] [CrossRef]
- Li, D.Q.; Yang, Z.H.; Cao, Z.J.; Au, S.K.; Phoon, K.K. System reliability analysis of slope stability using generalized Subset Simulation. Appl. Math. Model. 2017, 46, 650–664. [Google Scholar] [CrossRef]
- He, X.; Xu, H.; Sabetamal, H.; Sheng, D. Machine learning aided stochastic reliability analysis of spatially variable slopes. Comput. Geotech. 2020, 126, 103711. [Google Scholar] [CrossRef]
- Dyson, A.P.; Tolooiyan, A. Prediction and classification for finite element slope stability analysis by random field comparison. Comput. Geotech. 2019, 109, 117–129. [Google Scholar] [CrossRef]
- Dyson, A.P.; Tolooiyan, A. Comparative Approaches to Probabilistic Finite Element Methods for Slope Stability Analysis. Simul. Model. Pract. Theory 2020, 100, 102061. [Google Scholar] [CrossRef]
- Wang, B.; Feng, X.; Pan, P.; Li, S. Slope failure analysis using the material point method. Yanshilixue Yu Gongcheng Xuebao Chin. J. Rock Mech. Eng. 2017, 36, 2146–2155. [Google Scholar] [CrossRef]
- Liu, X.; Wang, Y.; Li, D.Q. Investigation of slope failure mode evolution during large deformation in spatially variable soils by random limit equilibrium and material point methods. Comput. Geotech. 2019, 111, 301–312. [Google Scholar] [CrossRef]
- Wong, F.S. Slope reliability and response surface method. J. Geotech. Eng. 1985, 111, 32–53. [Google Scholar] [CrossRef]
- Xu, B.; Low, B.K. Probabilistic stability analyses of embankments based on finite-element method. J. Geotech. Geoenviron. Eng. 2006, 132, 1444–1454. [Google Scholar] [CrossRef]
- Li, D.Q.; Zheng, D.; Cao, Z.J.; Tang, X.S.; Phoon, K.K. Response Surface method fo slope stability: Review and comparison. Eng. Geolog. 2016, 203, 3–14. [Google Scholar] [CrossRef]
- Hu, B.; Su, G.S.; Jiang, J.; Xiao, Y. Gaussian process-based response surface method for slope reliability analysis. Advances in Civ. Eng. 2019, 2019. [Google Scholar] [CrossRef]
- Li, D.; Chen, Y.; Lu, W.; Zhou, C. Stochastic response surface method for reliability analysis of rock slopes involving correlated non-normal variables. Comput. Geotech. 2011, 38, 58–68. [Google Scholar] [CrossRef]
- Liu, Z.; Choi, J.C.; Nadim, F.; Lacaasse, S. Reliability analysis of sensitive clay slope with the response surface method. In Proceedings of the GeoShanghai 2018 International Conference: Geoenvironment and Geohazard, Shanghai, China, 27–30 May 2018; pp. 63–72. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, L.M.; Tang, W.H. New methods for system reliability analysis of soil slopes. Can. Geotech. J. 2011, 48, 1138–1148. [Google Scholar] [CrossRef]
- Tan, X.H.; Shen, M.F.; Hou, X.L.; Li, D.; Hu, N. Response Surface Method of Reliability Analysis and its Application in Slope Stability Analysis. Geotech. Geol. Eng. 2013, 31, 1011–1025. [Google Scholar] [CrossRef]
- Zhou, X.P.; Huang, X.C. Reliability analysis of slopes using UD-based response surface methods combined with LASSO. Eng. Geol. 2018, 233, 111–123. [Google Scholar] [CrossRef]
- Ji, J.; Low, B.K. Stratified Response Surfaces for System Probabilistic Evaluation of Slopes. J. Geotech. Geoenviron. Eng. 2012, 138, 1398–1406. [Google Scholar] [CrossRef]
- Banaki, R.; Ahmad, F.; Tabarroki, M.; Yahaya, A.S. Probabilistic Analyses of Slopes: A State of the Art Review—Inpressco. Int. J. Curr. Eng. Technol. 2013, 3, 58–63. [Google Scholar]
- Rosenblueth, E.Y.-I. Point estimates for probability moments. Proc. Nat. Acad. Sci. USA 1975, 72, 3812–3814. [Google Scholar] [CrossRef]
- Harr, M.E. Probabilistic estimates for multivariate analyses. Appl. Math. Model. 1989, 13, 313–318. [Google Scholar] [CrossRef]
- Hong, H.P. An efficient point estimate method for probabilistic analysis. Reliab. Eng. Syst. Saf. 1998, 59, 261–267. [Google Scholar] [CrossRef]
- Baecher, G.B.; Christian, J.T. Reliability and Statistics in Geotechnical Engineering; John Wiley & Sons: Chichester, UK, 2003. [Google Scholar]
- Przewłócki, J. Problems of Stochastic Soil Mechanics—Reliability Analysis; Dolnośląskie Wydawnictwo Edukacyjne: Wrocław, Poland, 2006. [Google Scholar]
- Gibson, W. Probabilistic methods for slope analysis and design. Aust. Geomech. 2011, 46, 1–11. [Google Scholar]
- Wang, J.P.; Huang, D. Rosen Point: A Microsoft Excel-based program for the Rosenblueth point estimate method and an application in slope stability analysis. Comput. Geosci. 2012, 48, 239–243. [Google Scholar] [CrossRef]
- Ahmadabadi, M.; Poisel, R. Assessment of the application of point estimate methods in the probabilistic stability analysis of slopes. Comput. Geotech. 2015, 69, 540–550. [Google Scholar] [CrossRef]
- Yang, Z.; Ching, J. A novel simplified geotechnical reliability analysis method. Appl. Math. Model. 2019, 74, 337–349. [Google Scholar] [CrossRef]
- Winkelmann, K.; Zabuski, L.; Przewłócki, J.; Górski, J. Reliability-Based Stability Analysis of a Baltic Cliff by the Combined Response Surface Method. Geotech. Geol. Eng. 2020, 38, 5549–5563. [Google Scholar] [CrossRef]
- Zhang, T.; Zhou, X.P.; Liu, X.F. Reliability analysis of slopes using the improved stochastic response surface methods with multicollinearity. Eng. Geol. 2020, 271, 105617. [Google Scholar] [CrossRef]
- Wang, M.; Wang, X.; Liu, Q.; Shen, F.; Jin, J. A novel multi-dimensional cloud model coupled with connection numbers theory for evaluation of slope stability. Appl. Math. Model. 2020, 77, 426–438. [Google Scholar] [CrossRef]
- Liu, L.L.; Cheng, Y.M.; Pan, Q.J.; Dias, D. Incorporating stratigraphic boundary uncertainty into reliability analysis of slopes in spatially variable soils using one-dimensional conditional Markov chain model. Comput. Geotech. 2020, 118, 103321. [Google Scholar] [CrossRef]
- Oka, Y.; Wu, T.H. System reliability of slope stability. J. Geot. Eng. 1990, 116, 1185–1189. [Google Scholar] [CrossRef]
- Chowdhury, R.N.; Xu, D.W. Geotechnical system reliability of slopes. Reliab. Eng. Syst. Saf. 1995, 47, 141–151. [Google Scholar] [CrossRef]
- Huang, J.; Griffiths, D.V.; Fenton, G.A. System reliability of slopes by RFEM. Soils Found. 2010, 50, 343–353. [Google Scholar] [CrossRef]
- Liu, L.L.; Cheng, Y.M. Efficient system reliability analysis of soil slopes using multivariate adaptive regression splines-based. Comput. Geotech. 2016, 79, 41–54. [Google Scholar] [CrossRef]
- Ma, J.Z.; Zhang, J.; Huang, H.W.; Zhang, L.L.; Huang, J.S. Identification of representative slip surfaces for reliability analysis of soil slopes based on shear strength reduction. Comput. Geotech. 2017, 85, 199–206. [Google Scholar] [CrossRef]
- Zhang, J.; Huang, H.W. Risk assessment of slope failure considering multiple slip surfaces. Comput. Geotech. 2016, 74, 188–195. [Google Scholar] [CrossRef]
- Liu, L.L.; Cheng, Y.M.; Zhang, S.H. Conditional random field reliability analysis of a cohesion-frictional slope. Comput. Geotech. 2017, 82, 173–186. [Google Scholar] [CrossRef]
- Duan, X.; Zhang, J.; Huang, H.; Zeng, P.; Zhang, L. System reliability analysis of soil slopes through constrained optimization. Landslides 2020, 18, 655–666. [Google Scholar] [CrossRef]
- Malkawi, A.I.; Hassan, W.F.; Abdulla, F.A. Uncertainty and reliability analysis applied to slope stability. Struct. Saf. 2000, 22, 161–187. [Google Scholar] [CrossRef]
- Wang, Y.; Cao, Z.; Au, S.K. Efficient Monte Carlo Simulation of parameter sensitivity in probabilistic slope stability analysis. Comput. Geotech. 2010, 37, 1015–1022. [Google Scholar] [CrossRef]
- Javankhoshdel, S.; Bathurst, R.J. cohesive and cohesive-frictional (c-ϕ) soils. Can. Geotech. J. 2014, 51, 1033–1045. [Google Scholar] [CrossRef]
- Salgado, R.; Kim, D. Reliability Analysis of Load and Resistance Factor Design of Slopes. J. Geotech. Geoenviron. Eng. 2014, 140, 57–73. [Google Scholar] [CrossRef]
- Cao, Z.; Wang, Y.; Li, D. Probabilistic Approaches for Geotechnical Site Characterization and Slope Stability Analysis; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Wu, X.Z. Probabilistic slope stability analysis by a copula-based sampling method. Comput. Geosci. 2013, 17, 739–755. [Google Scholar] [CrossRef]
- Tang, X.S.; Li, D.Q.; Zhou, C.B.; Phoon, K.K. Copula-based approaches for evaluating slope reliability under incomplete probability information. Struct. Saf. 2015, 52, 90–99. [Google Scholar] [CrossRef]
- Tang, X.S.; Wang, M.X.; Li, D.Q. Modeling multivariate cross-correlated geotechnical random fields using vine copulas for slope reliability analysis. Comput. Geotech. 2020, 127, 103784. [Google Scholar] [CrossRef]
- Li, D.Q.; Qi, X.H.; Cao, Z.J.; Tang, X.S.; Phoon, K.K.; Zhou, C.B. Evaluating slope stability uncertainty using coupled Markov chain. Comput. Geotech. 2016, 73, 72–80. [Google Scholar] [CrossRef]
- Deng, Z.P.; Li, D.Q.; Qi, X.H.; Cao, Z.J.; Phoon, K.K. Reliability evaluation of slope considering geological uncertainty and inherent variability of soil parameters. Comput. Geotech. 2017, 92, 121–131. [Google Scholar] [CrossRef]
- Jha, S.K.; Ching, J. Simplified reliability method for spatially variable undrained engineered slopes. Soils Found. 2013, 53, 708–719. [Google Scholar] [CrossRef]
- Jiang, S.H.; Li, D.Q.; Zhang, L.M.; Zhou, C.B. Slope reliability analysis considering spatially variable shear strength parameters using a non-intrusive stochastic finite element method. Eng. Geol. 2014, 168, 120–128. [Google Scholar] [CrossRef]
- Li, K.S.; Lumb, P. Probabilistic design of slopes. Can. Geotech. J. 1987, 24, 520–531. [Google Scholar] [CrossRef]
- Low, B.K.; Lacasse, S.; Nadim, F. Slope reliability analysis accounting for spatial variation. Georisk 2007, 1, 177–189. [Google Scholar] [CrossRef]
- Suchomel, R.; Mašin, D. Comparison of different probabilistic methods for predicting stability of a slope in spatially variable c–u soil. Comput. Geotech. 2010, 37, 132–140. [Google Scholar] [CrossRef]
- Griffiths, D.V.; Huang, J.; Fenton, G.A. Influence of spatial variability on slope reliability using 2-D random field. J. Geotech. Geoenviron. Eng. 2009, 135, 1367–1378. [Google Scholar] [CrossRef]
- Ji, J.; Zhang, C.; Gao, Y.; Kodikara, J. Effect of 2D spatial variability on slope reliability: A simplified FORM analysis. Geosci. Front. 2018, 9, 1631–1638. [Google Scholar] [CrossRef]
- Kim, J.M.; Sitar, N. Reliability approach to slope stability analysis with spatially correlated soil properties. Soils Found. 2013, 53, 1–10. [Google Scholar] [CrossRef]
- Mahmoudi, E.; Schmüdderich, C.; Hölter, R.; Zhao, C.; Wichtmann, T.; König, M. Stochastic field simulation of slope stability problems: Improvement and reduction of computational effort. Comput. Methods Appl. Mech. Eng. 2020, 369, 113167. [Google Scholar] [CrossRef]
- Jiang, S.H.; Papaioannou, I.; Straub, D. Bayesian updating of slope reliability in spatially variable soils with in-situ measurements. Eng. Geol. 2018, 239, 310–320. [Google Scholar] [CrossRef]
- Li, X.Y.; Zhang, L.M.; Gao, L.; Zhu, H. Simplified slope reliability analysis considering spatial soil variability. Eng. Geol. 2017, 216, 90–97. [Google Scholar] [CrossRef]
- Liu, L.; Deng, Z.; Zhang, S.; Cheng, Y. Simplified framework for system reliability analysis of slopes in spatially variable soils. Eng. Geol. 2018, 239, 330–343. [Google Scholar] [CrossRef]
- Luo, X.; Cheng, T.; Li, X.; Zhou, J. Slope safety factor search strategy for multiple sample points for reliability analysis. Eng. Geol. 2012, 129–130, 27–37. [Google Scholar] [CrossRef]
- Low, B.K.; Tang, W.H. Efficient spreadsheet algorithm for first-order reliability method. J. Eng. Mech. 2007, 133, 1378–1387. [Google Scholar] [CrossRef]
- Low, B.K.; Phoon, K.K. Reliability-based design and its complementary role to Eurocode 7 design approach. Comput. Geotech. 2015, 65, 30–44. [Google Scholar] [CrossRef]
- Hack, R.; Price, D.; Rengers, N. A new approach to rock slope stability—A probability classification (SSPC). Bull. Eng. Geol. Environ. 2003, 62, 167–184. [Google Scholar] [CrossRef]
- Lindsay, P.; Campbell, R.N.; Fergusson, D.A.; Gillard, G.R.; Moore, T.A. Slope stability probability classification, Waikato Coal Measures, New Zealand. Int. J. Coal Geol. 2001, 45, 127–145. [Google Scholar] [CrossRef]
- Heidelbe, B. Assessment of rock slope stability by probabilistic Slope e Stability Probability Classification m method along highway cut slopes in Adilcevaz-Bitlis (Turkey). J. Mt. Sci. 2016, 13, 1893–1909. [Google Scholar]
- Ersöz, T.; Topal, T. Assessment of rock slope stability with the effects of weathering and excavation by comparing deterministic methods and slope stability probability classification (SSPC). Environ. Earth Sci. 2018, 77, 1–18. [Google Scholar] [CrossRef]
- Melchers, R.E. Structural Reliability Analysis and Prediction; John Wiley and Sons: New York, NY, USA, 1999. [Google Scholar]
- Shuppener, B. Eurocode 7: Geotechnical Design-Part 1: General Rules-Its Implementation in the European Member States. In Proceedings of the 14th European Conference on Soil Mechanics and Geotechnical Engineering, Madrid, Spain, 24–27 September 2007; Volume 2, pp. 279–289. [Google Scholar]
| Random Soil Model | SRV | RF | |||||
|---|---|---|---|---|---|---|---|
| Deterministic Method | LEM | FEM/DEM/ MPM | LA | LEM | FEM/DEM | LA | |
| Reliability Level | |||||||
| 1 | SRVLEM1 | SRVFEM1 | SRVLA1 | RFLEM1 | - | - | |
| 2 | SRVLEM2 | SRVFEM2 | SRVLA2 | RFLEM2 | RFFEM2 | RFLA2 | |
| 3 | SRVLEM3 | SRVFEM3 | SRVLA3 | RFLEM3 | RFFEM3 | RFLA3 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Przewłócki, J. Brief Literature Review and Classification System of Reliability Methods for Evaluating the Stability of Earth Slopes. Sustainability 2021, 13, 9090. https://doi.org/10.3390/su13169090
Przewłócki J. Brief Literature Review and Classification System of Reliability Methods for Evaluating the Stability of Earth Slopes. Sustainability. 2021; 13(16):9090. https://doi.org/10.3390/su13169090
Chicago/Turabian StylePrzewłócki, Jarosław. 2021. "Brief Literature Review and Classification System of Reliability Methods for Evaluating the Stability of Earth Slopes" Sustainability 13, no. 16: 9090. https://doi.org/10.3390/su13169090
APA StylePrzewłócki, J. (2021). Brief Literature Review and Classification System of Reliability Methods for Evaluating the Stability of Earth Slopes. Sustainability, 13(16), 9090. https://doi.org/10.3390/su13169090






