Under the framework of traditional game theory research, the research object is often assumed to be completely rational, which ignores the fact that human beings have analytical reasoning and learning ability in economic activities [
1]. The evolutionary game theory adopted in this study holds that participants are only rational economic men with limited rationality, and they usually obtain relevant strategies based on a transfer mechanism rather than rational choice. Each strategy choice of participants has its corresponding strategic revenue and the probability of participants choosing the strategy. In the process of multiple dynamic games, the theory transforms human behavior into a gradual evolutionary process with learning ability and adaptability. It shows that each higher-yield strategy in the game will replace the lower-yield strategy and finally, forms a stable evolutionary equilibrium strategy through repeated games. Obviously, the equilibrium emphasized by evolutionary game is the result of learning and adjustment rather than the result of selection, which is consistent with the simulation and simulation characteristics of SD. SD is a science that closely combines system science theory with computer simulation to study the structure and behavior of system feedback. It can combine other theories for interdisciplinary researches. The research method combining SD and evolutionary game theory has been applied in engineering project management and other fields; for example, Liu et al. combined multi-agent game with SD to discuss the long-term dynamic game process of stakeholders in coal mine safety production project under bounded rationality [
22]. Xie et al. proposed the behavior strategy model of central government, local government and farmers in cultivated land protection project based on evolutionary game, and simulated the system changes under different strategies through SD simulation [
23]. Thus, the combination of SD and evolutionary game theory is helpful to the dynamic study of the optimal strategy of each player, which makes the whole evolutionary game process reach equilibrium.
2.1. Analysis of Game Players’ Relationships
The term “PPP” is typically used to describe a long-term contractual arrangement for the provision of a public asset and related services in exchange for performance-based payments linked to the asset’s availability and/or use and the delivery of the related services. All contracts signed by government departments and social institutions for the operation of public infrastructure and the provision of public services are regarded as PPP models. The level of PPP development and measures adopted by member states of the European Union vary greatly. Some countries, such as Austria, Denmark, Germany, Ireland, Italy, the Netherlands, Portugal and the United Kingdom, set up PPP specialized institutions for management. Some countries, such as France, Germany, Greece, Portugal, Spain and Turkey, set up mechanisms or systems to constrain PPP through a series of laws. China has set up China Public Private Partnerships Center to manage PPP projects. Although this paper takes China as an example, the relevant formulas and models involved are universal and will be explained later.
In the PPP project, the government, as policy makers and regulators, stipulates the quantity and quality standards of the projects, and entrusts investors to build PPP projects and provide them with public consumption. The investors, as producers of PPP projects, can understand the public demand and production cost, product quality, and other information. As the ultimate consumer of the PPP project, the public directly senses the value of the project and feeds it back to the government. The game antagonistic relationship of the three parties is that the backbone of the PPP is made by government + investors. Then, the government is linked primarily to the public since they share a common interest. Thus, the main confrontation is government + public versus investors. Investor players are backed by their lending banks (and sub-contractors) that represent a key stakeholder in capital-intensive problems. The specific relationship among the three parties of the game is analyzed as follows.
(1) The game between the government and investors
In the process of PPP project outsourcing, the government wants to outsource the management rights of PPP projects to enterprise with market experience and provide more specialized services for the public. It is mainly decided by how the government supervises the investors. Because of information asymmetry and inconsistent objectives, investors will have more opportunistic behavioral tendencies if the government cannot effectively monitor the behavior of investment portfolios (although both the government and investors hope that the PPP project can go on smoothly, their goals are different. The government’s goal is to maximize the benefits brought by PPP as much as possible, while investors’ goal is to maximize the operating benefits of PPP project itself, which makes the two payment functions different) [
24]. For example, it is possible for investors to take the benefits of the public and the government to maximize their interests. At this time, the government needs to adopt supervision mechanism and carry out certain punishment to transfer the risk caused by information asymmetry. However, opportunistic private behaviour is mitigated by competition in the tender, and possibly by pay-for-performance remuneration pattern.
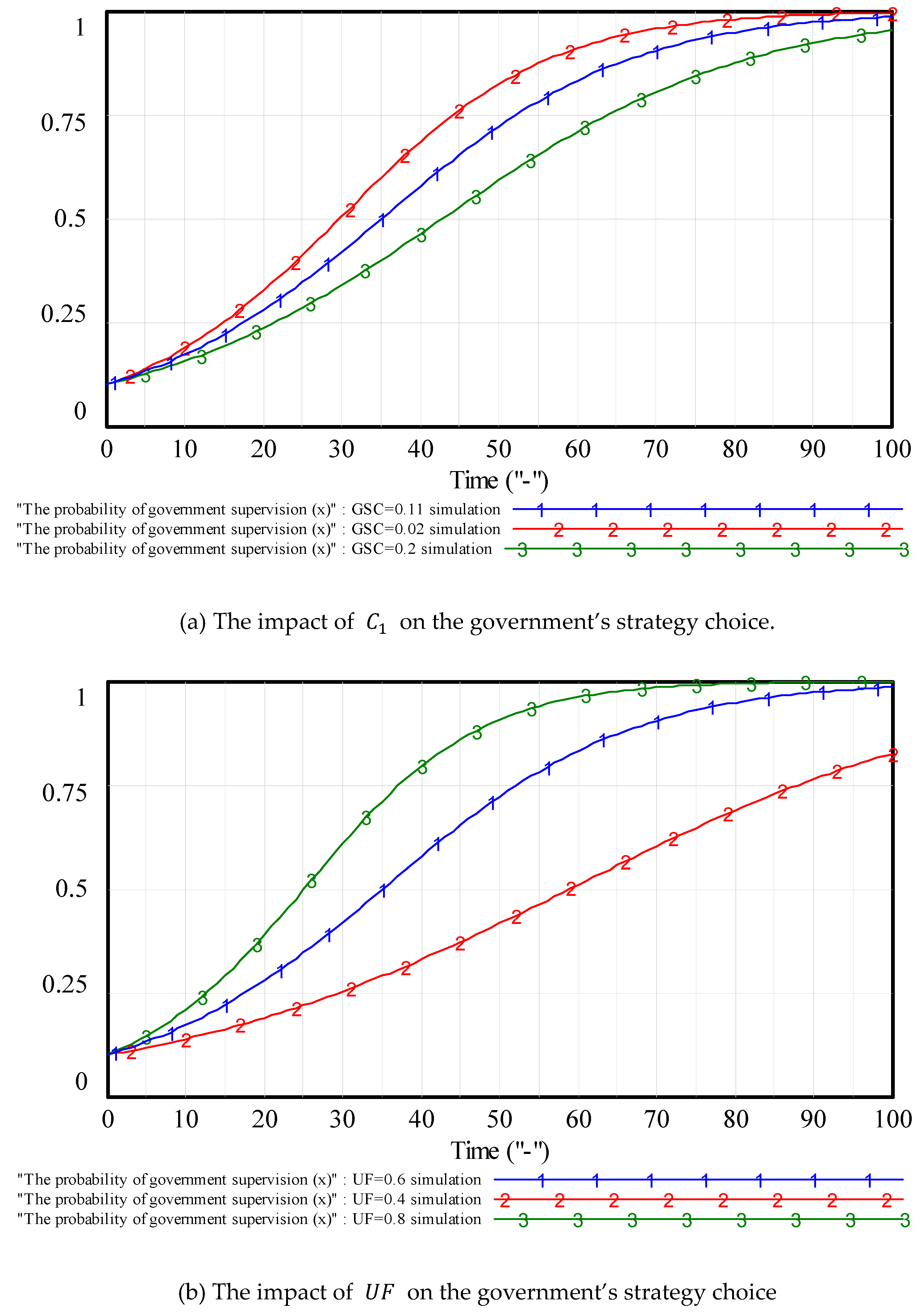
The government must pay costs for the implementation of supervision. Moreover, the more the government invests in supervision, the greater the supervision of the investors, the greater the punishment, and the more likely investors choose to make efforts. The more investors invest in project’s risk management and control, the higher the service quality of power construction, the more they can be well received by the public, and the more likely they are to be recognized by the government and obtain long-term contracts. On the contrary, if the government fails to supervise, investors’ efforts will lead to public dissatisfaction, thereby bringing losses to the government. Therefore, the costs and profits of supervision are the focuses of game between the government and investors.
(2) The game between the government and the public
In the process of outsourcing the PPP project to the investor, the government and investors formulate a contract to stipulate the rights and responsibilities of both parties. This explicit contract is achievable in practice. Although there is a principal-agent relationship between the government and the public, the implicit contract relationship actually affects the realization of the contract. Therefore, in the outsourcing contract, there is no direct relationship between the government and the public. However, the purpose of the government’s PPP project is to meet the needs of the public. As consumers, the public should have the most say in the results of the PPP project. Moreover, from the perspective of practice, public satisfaction has increasingly attracted the attention of government departments, and the public’s evaluation has become more and more important in government decision-making [
25]. As the main responsible person of service outsourcing, the government has the responsibility of finding suitable investors for the public to replace the government’s functions in fulfilling PPP projects [
26]. Therefore, it is necessary for the public to participate in the process of outsourcing PPP projects. Whitaker points out that many public services require the active participation of the general public, especially those who will be directly beneficiaries of services [
27]. Public participation will help improve the quality of PPP projects and increase public satisfaction and trust in the public sector.
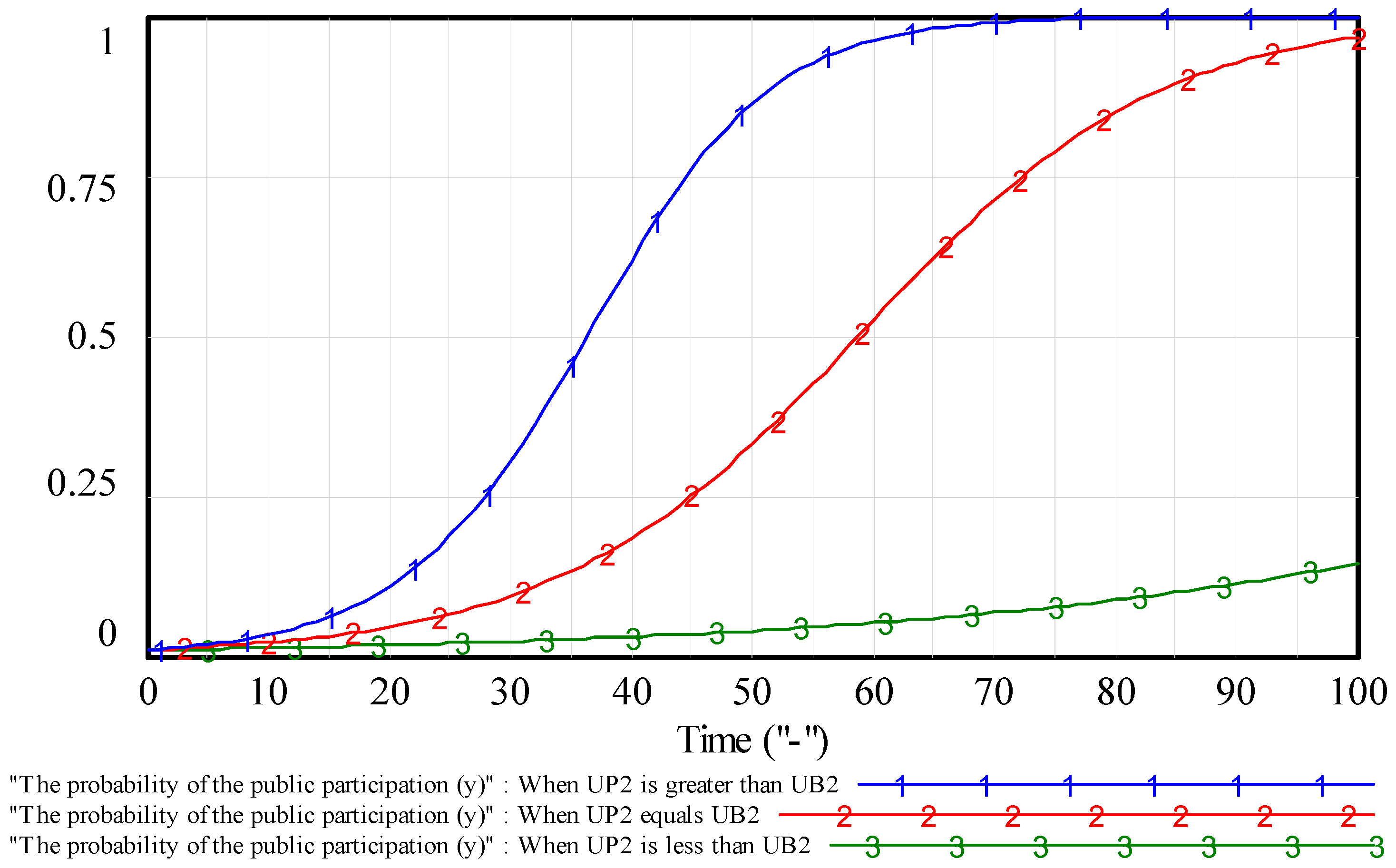
Since the government must consider the wishes of the public in the process of providing PPP projects, the government’s strategy is to “encourage the public’s participation” and “disallow the public’s participation” in the face of public participation. From the government’s point of view, public satisfaction should be the starting point and end result of the government’s provision of PPP projects. Therefore, the choice of “encouraging public participation” is the best decision. From the perspective of the public, there are two strategic choices of “participation” and “no participation”. The participation strategy can enhance the perceived value of the public to the project, and then express the objective consumption intention to the government, so as to maintain or improve the PPP project. However, public participation will incur participation costs and opportunity costs (we collectively refer to the public’s participation costs), as well as the loss of profits that investors do not strive to bring to the public. If the public does not participate in the project, it will not benefit from the service and incur participation costs. Therefore, the trade-off between cost and benefit is the main influencing factor of public participation [
28]. When the PPP project brings losses to the public, the government should provide corresponding compensation. Therefore, the government should pay attention not only to the benefits obtained through PPP projects, but also to meeting the value compensation expectations of public participation.
(3) The game between the government+ public versus investors
Although investors and the government directly sign the PPP project contract and accept the supervision and management of the government, the target of investors is the public, whose evaluation is the main basis for the government to approve investors or not. Thus, although there is no direct contractual relationship between the public and investors, there is a direct interest relationship.
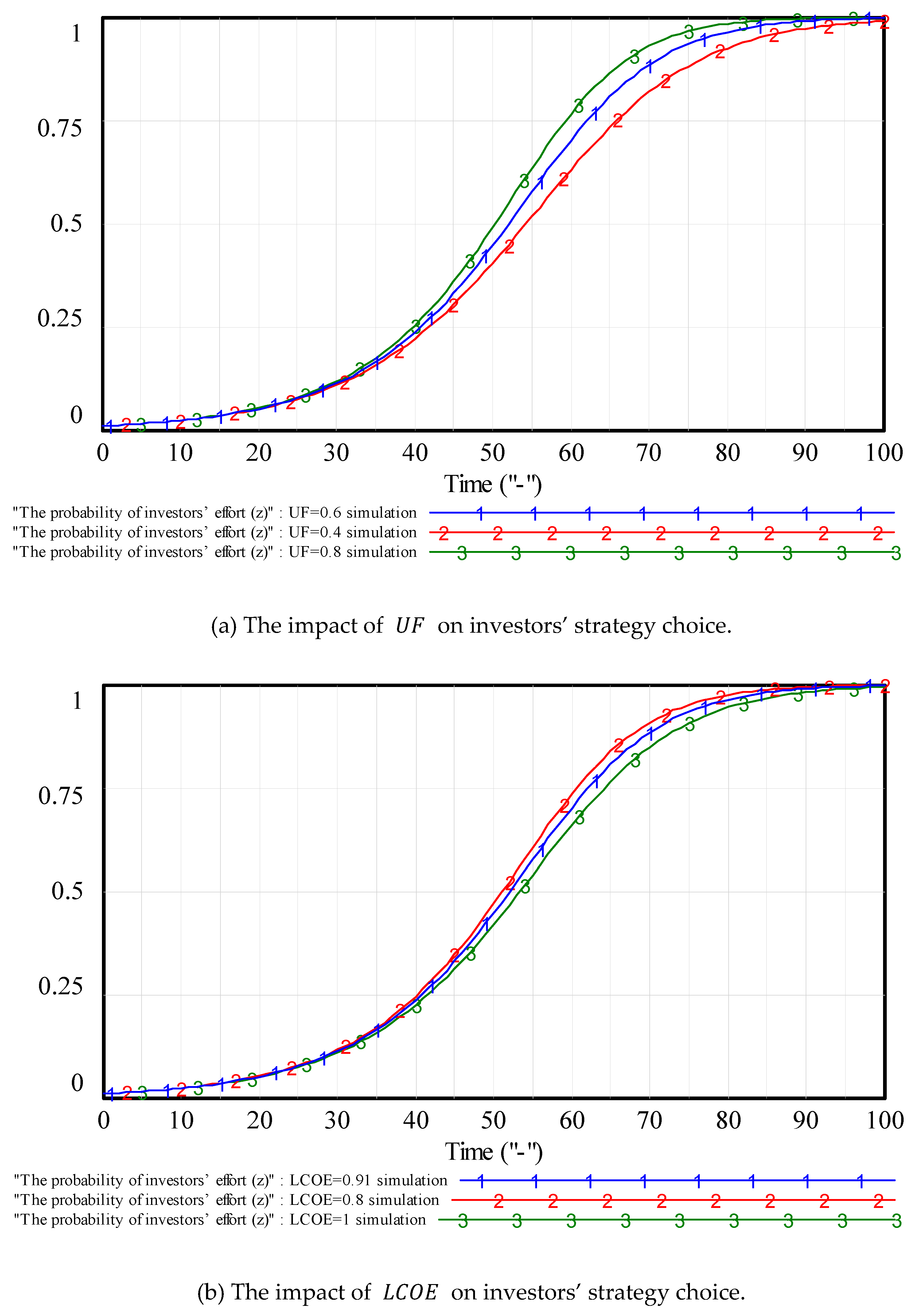
Whether investors make efforts is a factor that cannot be ignored by the public. However, for investors, in the absence of government supervision, they do not want to make more efforts, because investors do not bear the cost of harming the public interest, and will not actively raise the level of service efforts. Under the conditions of government supervision, investors will increase the cost of efforts, or bear the penalty cost of not working hard. Both of these costs are closely related to the perceived value of the public. Therefore, investors’ effort cost and penalty cost are the focuses of the game between investors and the public.
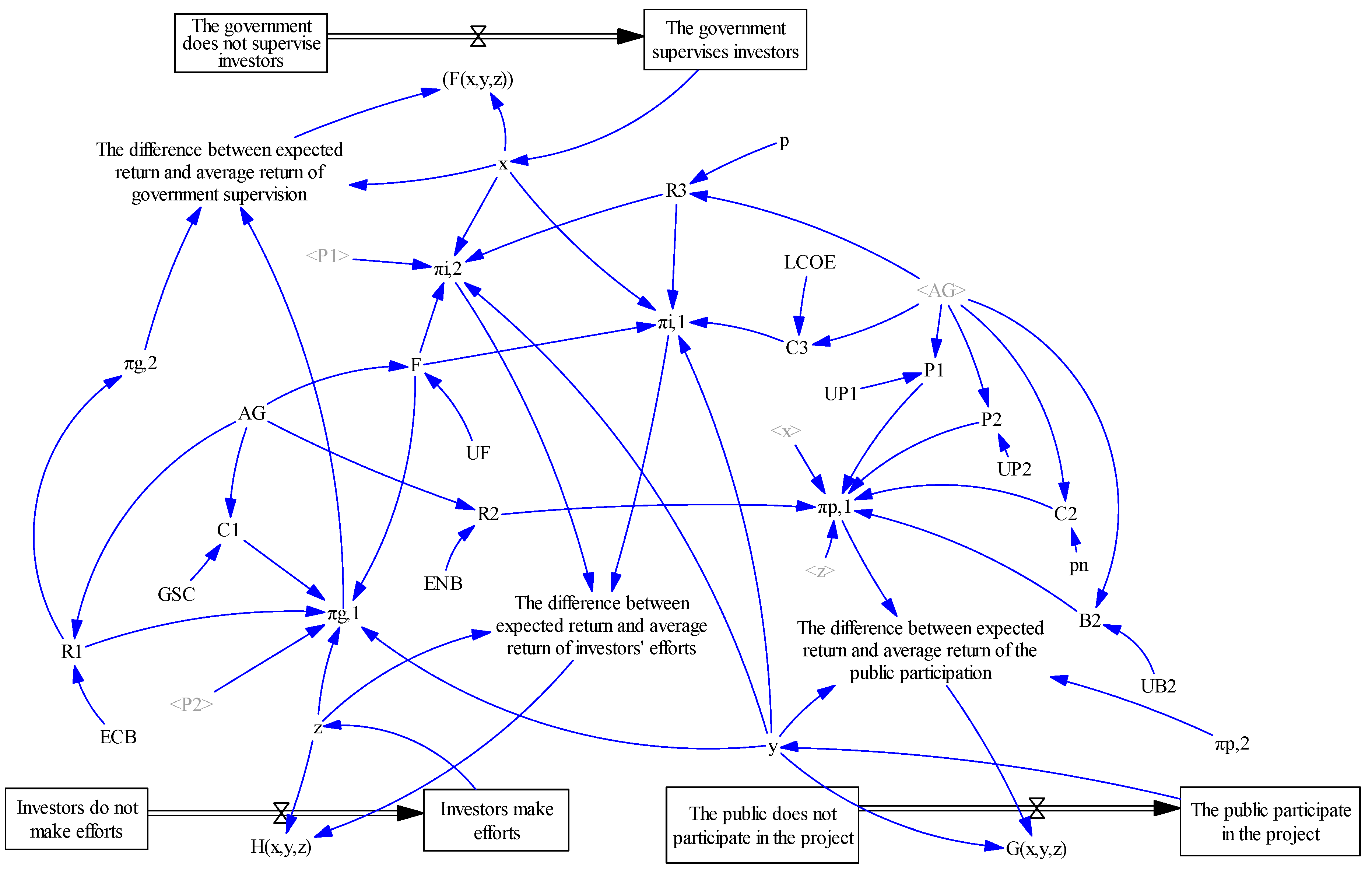
In summary, in the outsourcing of PPP projects, the government’s choice of strategies is {encourage public participation, supervise investors; encourage public participation, not to supervise investors}, and the public’s strategy is {participation; no participation}, investors’ strategy is {effort; no effort}. This study uses , , , , , and to indicate the probability that the government supervises investors, the public participates in the project, and investors make efforts, respectively. Moreover, .