Flow–Vegetation Interaction in a Living Shoreline Restoration and Potential Effect to Mangrove Recruitment
Abstract
1. Introduction
2. Materials and Methods
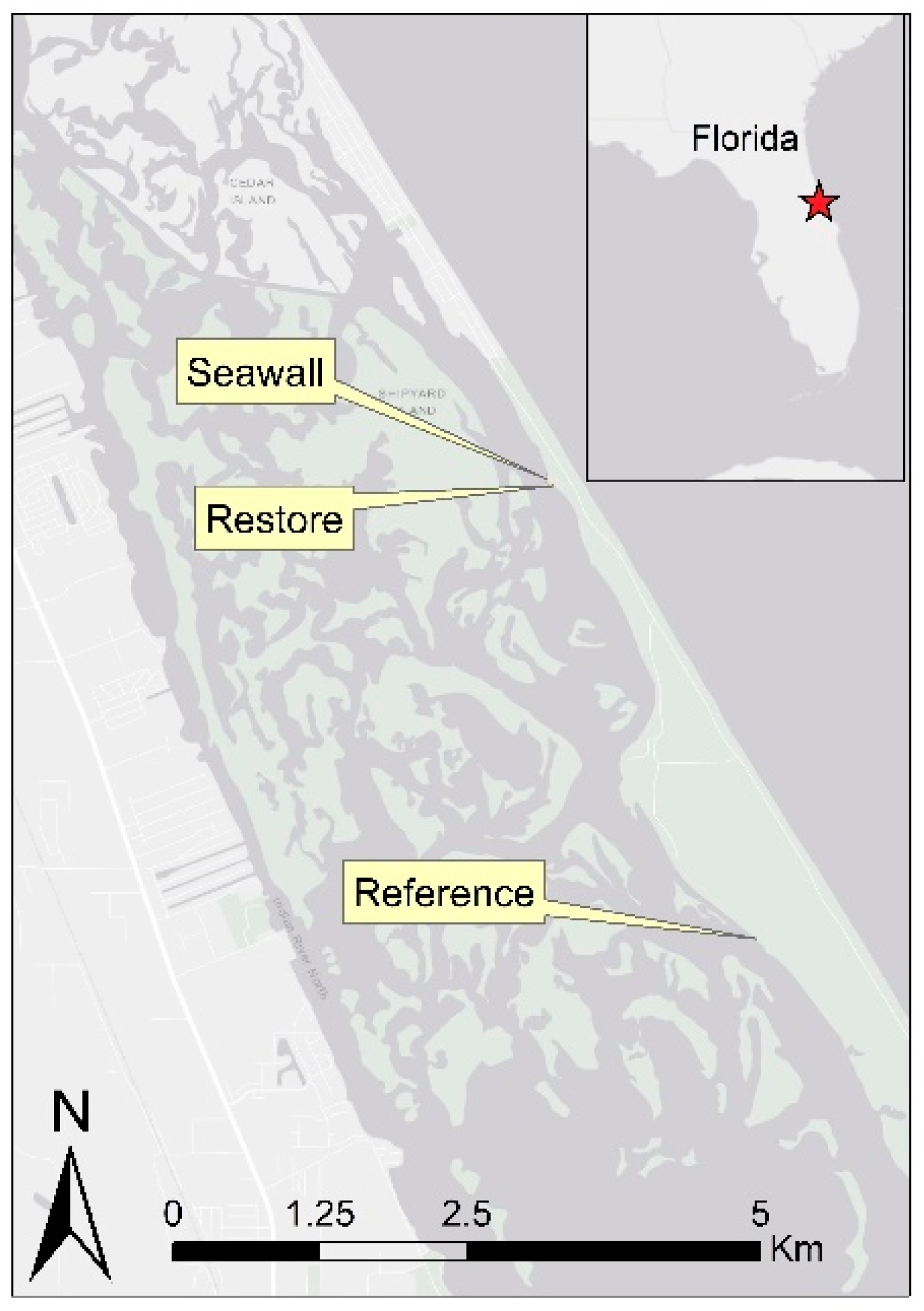
2.1. Study Site Description
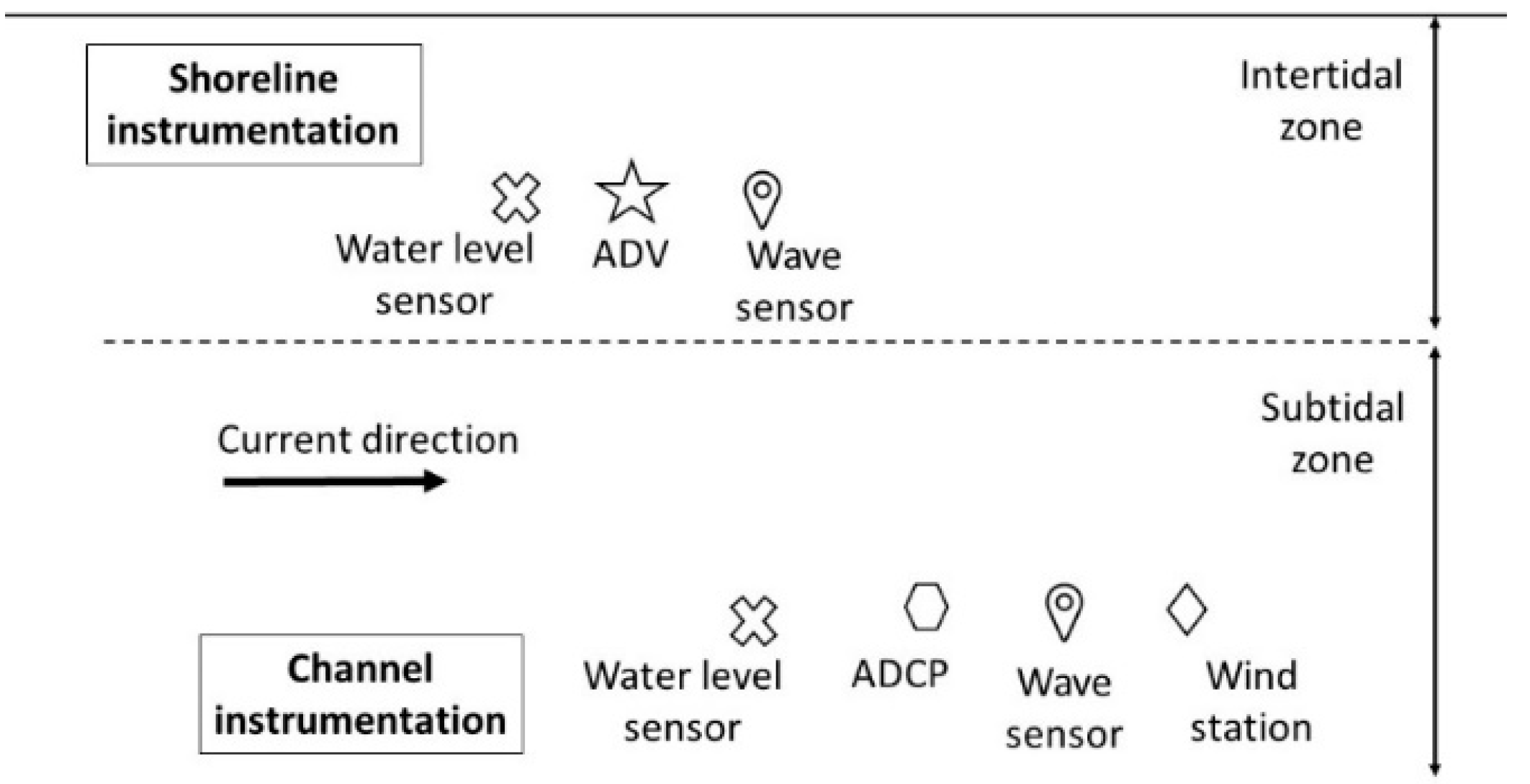
2.2. Field Data Collection
2.3. Analysis of Hydrodynamic Data
2.3.1. Post-Processing and Quality Control
2.3.2. Turbulent Kinetic Energy Dissipation
3. Results
3.1. Vegetation Characteristics and Shoreline Morphologies
3.2. Mean Current Attenuation from Channel to Shoreline
3.3. Wave Attenuation from Channel to Shoreline
3.4. Comparison of Turbulent Kinetic Energy Dissipation across Sites
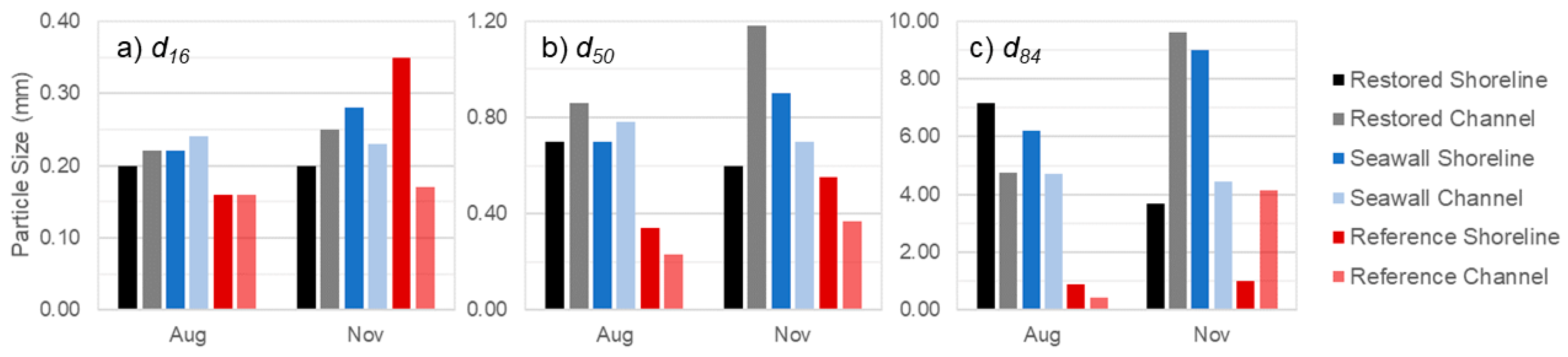
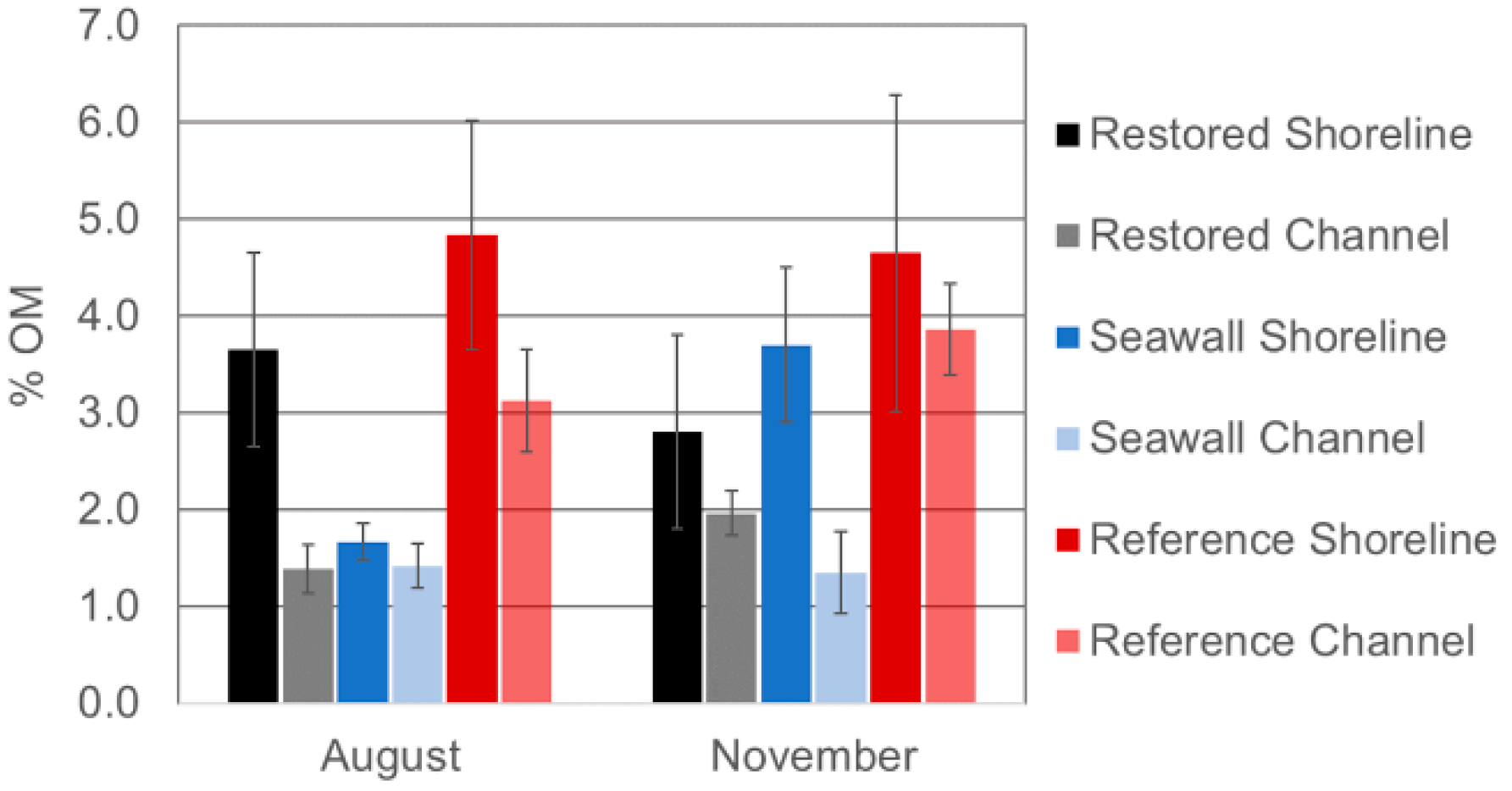
3.5. Comparison of Sediments across Sites
4. Discussion
4.1. Differential Hydrodynamic Effects of Bare Sediment, Restored Vegetation, and Natural Vegetation
Turbulent Kinetic Energy Dissipation Rate
4.2. Mangrove Recruitment into Restored Shorelines
4.3. Why Did Vegetation at the Restored Site Thrive While Hybrid Restoration at the Seawall Did Not?
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
References
- Lotze, H.K.; Lenihan, H.S.; Bourque, B.J.; Bradbury, R.H.; Cooke, R.G.; Kay, M.C.; Kidwell, S.M.; Kirby, M.X.; Peterson, C.H.; Jackson, J.B. Depletion, degradation, and recovery potential of estuaries and coastal seas. Science 2006, 312, 1806–1809. [Google Scholar] [CrossRef] [PubMed]
- Gittman, R.K.; Scyphers, S.B.; Smith, C.S.; Neylan, I.P.; Grabowski, J.H. Ecological consequences of shoreline hardening: A meta-analysis. Biosci. J. 2016, 66, 763–773. [Google Scholar] [CrossRef] [PubMed]
- Gittman, R.K.; Fodrie, F.J.; Popowich, A.M.; Keller, D.A.; Bruno, J.F.; Currin, C.A.; Piehler, M.F. Engineering away our natural defenses: An analysis of shoreline hardening in the US. Front. Ecol. Environ. 2015, 13, 301–307. [Google Scholar] [CrossRef]
- Small, C.; Nicholls, R.J. A global analysis of human settlement in coastal zones. J. Coast. Res. 2003, 19, 584–599. [Google Scholar]
- Hinkel, J.; Lincke, D.; Vafeidis, A.T.; Perrette, M.; Nicholls, R.J.; Tol, R.S.; Marzeion, B.; Fettweis, X.; Ionescu, C.; Levermann, A. Coastal flood damage and adaptation costs under 21st century sea-level rise. Proc. Natl. Acad. Sci. USA 2014, 111, 3292–3297. [Google Scholar] [CrossRef] [PubMed]
- Young, I.R.; Zieger, S.; Babanin, A.V. Global trends in wind speed and wave height. Science 2011, 332, 451–455. [Google Scholar] [CrossRef] [PubMed]
- Bilkovic, D.M.; Mitchell, M.; Mason, P.; Duhring, K. The role of living shorelines as estuarine habitat conservation strategies. Coast. Manag. 2016, 44, 161–174. [Google Scholar] [CrossRef]
- Gedan, K.B.; Kirwan, M.L.; Wolanski, E.; Barbier, E.B.; Silliman, B.R. The present and future role of coastal wetland vegetation in protecting shorelines: Answering recent challenges to the paradigm. Clim. Chang. 2011, 106, 7–29. [Google Scholar] [CrossRef]
- Gittman, R.K.; Popowich, A.M.; Bruno, J.F.; Peterson, C.H. Marshes with and without sills protect estuarine shorelines from erosion better than bulkheads during a Category 1 hurricane. Ocean Coast. Manag. 2014, 102, 94–102. [Google Scholar] [CrossRef]
- Temmerman, S.; Meire, P.; Bouma, T.J.; Herman, P.M.; Ysebaert, T.; De Vriend, H.J. Ecosystem-based coastal defence in the face of global change. Nature 2013, 504, 79–83. [Google Scholar] [CrossRef]
- Smith, C.S.; Gittman, R.K.; Neylan, I.P.; Scyphers, S.B.; Morton, J.P.; Fodrie, F.J.; Peterson, C.H. Hurricane damage along natural and hardened estuarine shorelines: Using homeowner experiences to promote nature-based coastal protection. Mar. Policy 2017, 81, 350–358. [Google Scholar] [CrossRef]
- Swann, L. The use of living shorelines to mitigate the effects of storm events on Dauphin Island, Alabama, USA. In Proceedings of the American Fisheries Society Symposium, Ottawa, ON, Canada, 8–12 September 2008; Volume 64, p. 11. [Google Scholar]
- Mcleod, E.; Chmura, G.L.; Bouillon, S.; Salm, R.; Björk, M.; Duarte, C.M.; Lovelock, C.E.; Schlesinger, W.H.; Silliman, B.R. A blueprint for blue carbon: Toward an improved understanding of the role of vegetated coastal habitats in sequestering CO2. Front. Ecol. Environ. 2011, 9, 552–560. [Google Scholar] [CrossRef]
- Ridge, J.T.; Rodriguez, A.B.; Fodrie, F.J. Salt Marsh and Fringing Oyster Reef Transgression in a Shallow Temperate Estuary: Implications for Restoration, Conservation and Blue Carbon. Estuaries Coast. 2017, 40, 1013–1027. [Google Scholar] [CrossRef]
- Donnelly, M.; Shaffer, M.; Connor, S.; Sacks, P.; Walters, L. Using mangroves to stabilize coastal historic sites: Deployment success versus natural recruitment. Hydrobiologia 2017, 803, 389–401. [Google Scholar] [CrossRef]
- Struve, J.; Falconer, R.A. Hydrodynamic and Water Quality Processes in Mangrove Regions. J. Coast. Res. 2001, 27, 65–75. [Google Scholar]
- Lewis, R.R. Ecological engineering for successful management and restoration of mangrove forests. Ecol. Eng. 2005, 24, 403–418. [Google Scholar] [CrossRef]
- Balke, T.; Bouma, T.; Horstman, E.; Webb, E.; Erftemeijer, P.; Herman, P. Windows of opportunity: Thresholds to mangrove seedling establishment on tidal flats. Mar. Ecol. Prog. Ser. 2011, 440, 1–9. [Google Scholar] [CrossRef]
- Balke, T.; Swales, A.; Lovelock, C.E.; Herman, P.M.J.; Bouma, T.J. Limits to seaward expansion of mangroves: Translating physical disturbance mechanisms into seedling survival gradients. J. Exp. Mar. Biol. Ecol. 2015, 467, 16–25. [Google Scholar] [CrossRef]
- Gittman, R.K.; Peterson, C.H.; Currin, C.A.; Joel Fodrie, F.; Piehler, M.F.; Bruno, J.F. Living shorelines can enhance the nursery role of threatened estuarine habitats. Ecol. Appl. 2016, 26, 249–263. [Google Scholar] [CrossRef]
- Smith, C.S.; Puckett, B.; Gittman, R.K.; Peterson, C.H. Living shorelines enhanced the resilience of saltmarshes to Hurricane Matthew. Ecol. Appl. 2016, 28, 871–877. [Google Scholar] [CrossRef]
- Nepf, H.M. Hydrodynamics of vegetated channels. J. Hydraul. Res. 2012, 50, 262–279. [Google Scholar] [CrossRef]
- Mendez, F.J.; Losada, I.J. An empirical model to estimate the propagation of random breaking and nonbreaking waves over vegetation fields. Coast. Eng. 2004, 51, 103–118. [Google Scholar] [CrossRef]
- Yang, J.Q.; Chung, H.; Nepf, H.M. The onset of sediment transport in vegetated channels predicted by turbulent kinetic energy. Geophys. Res. Lett. 2016, 43, 11261–11268. [Google Scholar] [CrossRef]
- Yang, J.Q.; Nepf, H.M. A Turbulence-Based Bed-Load Transport Model for Bare and Vegetated Channels. Geophys. Res. Lett. 2018, 45, 10–428. [Google Scholar] [CrossRef]
- Tinoco, R.O.; Coco, G. Turbulence as the Main Driver of Resuspension in Oscillatory Flow Through Vegetation. J. Geophys. Res. Earth Surf. 2018, 123, 891–904. [Google Scholar] [CrossRef]
- Bouma, T.J.; van Duren, L.A.; Temmerman, S.; Claverie, T.; Blanco-Garcia, A.; Ysebaert, T.; Herman, P.M.J. Spatial flow and sedimentation patterns within patches of epibenthic structures: Combining field, flume and modelling experiments. Cont. Shelf Res. 2007, 27, 1020–1045. [Google Scholar] [CrossRef]
- Chang, W.-Y.; Constantinescu, G.; Tsai, W.F. On the flow and coherent structures generated by a circular array of rigid emerged cylinders placed in an open channel with flat and deformed bed. J. Fluid Mech. 2017, 831, 1–40. [Google Scholar] [CrossRef]
- Neary, V.S.; Constantinescu, S.G.; Bennett, S.J.; Diplas, P. Effects of vegetation on turbulence, sediment transport, and stream morphology. J. Hydraul. Eng. 2011, 138, 765–776. [Google Scholar] [CrossRef]
- Luhar, M.; Nepf, H.M. From the blade scale to the reach scale: A characterization of aquatic vegetative drag. Adv. Water Resour. 2013, 51, 305–316. [Google Scholar] [CrossRef]
- Horstman, E.M.; Bryan, K.R.; Mullarney, J.C.; Pilditch, C.A.; Eager, C.A. Are flow-vegetation interactions well represented by mimics? A case study of mangrove pneumatophores. Adv. Water Resour. 2018, 111, 360–371. [Google Scholar] [CrossRef]
- Walters, L.J.; Roman, A.; Stiner, J.; Weeks, D. Water Resource Management Plan, Canaveral National Seashore; National Park Service: Titusville, FL, USA, 2001.
- Down, C.; Withrow, R. Vegetation and Other Parameters in the Brevard County Bar-Built Estuaries; Project Report 06-73; Brevard County Health Department: Melbourne, FL, USA, 1978. [Google Scholar]
- Hellmann, R. Canaveral National Seashore: Archeological Overview and Assessment; Southeast Archeological Center, National Park Service: Tallahassee, FL, USA, 2013.
- Garvis, S.; Sacks, P.L. Walters. Assessing the Formation, Movement and Restoration of Dead Intertidal Oyster Reefs Over Time Using Remote Sensing in Canaveral National Seashore and Mosquito Lagoon, Florida. J. Shellf. Res. 2015, 34, 251–258. [Google Scholar] [CrossRef]
- Walters, L.; Donnelly, M.; Sacks, P.; Campbell, D. Lessons learned from living shoreline stabilization in popular tourist areas: Boat wakes, volunteer support, and protecting historic structures. In Living Shorelines: The Science and Management of Nature-Based Coastal Protection; Bilkovic, D.M., Mitchell, M.M., la Peyre, M.K., Toft, J.D., Eds.; CRC Press: Boca Raton, FL, USA, 2017; pp. 235–248. [Google Scholar]
- Koca, K.; Noss, C.; Anlanger, C.; Brand, A.; Lorke, A. Performance of the Vectrino Profiler at the sediment–water interface. J. Hydraul. Res. 2017, 55, 573–581. [Google Scholar] [CrossRef]
- Thomas, R.E.; Schindfessel, L.; McLelland, S.J.; Crelle, S.; Mulder, T.D. Bias in mean velocities and noise in variances and covariances measured using a multistatic acoustic profiler: The Nortek Vectrino Profiler. Meas. Sci. Technol. 2017, 28, 075302. [Google Scholar] [CrossRef]
- Pieterse, A.; Puleo, J.A.; McKenna, T.E.; Figlus, J. In situ measurements of shear stress, erosion and deposition in man-made tidal channels within a tidal saltmarsh. Estuar. Coast. Shelf Sci. 2017, 192, 29–41. [Google Scholar] [CrossRef]
- Puleo, J.A.; Lanckriet, T.; Blenkinsopp, C. Bed level fluctuations in the inner surf and swash zone of a dissipative beach. Mar. Geol. 2014, 349, 99–112. [Google Scholar] [CrossRef]
- Mori, N.; Suzuki, T.; Kakuno, S. Noise of acoustic Doppler velocimeter data in bubbly flows. J. Eng. Mech. 2007, 133, 122–125. [Google Scholar] [CrossRef]
- Goring, D.G.; Nikora, V.I. Despiking acoustic Doppler velocimeter data. J. Hydraul. Eng. 2002, 128, 117–126. [Google Scholar] [CrossRef]
- Wahl, T.L. Discussion of “Despiking acoustic Doppler velocimeter data”. J. Hydraul. Eng. 2003, 129, 484–487. [Google Scholar] [CrossRef]
- Wiles, P.J.; Rippeth, T.P.; Simpson, J.H.; Hendricks, P.J. A novel technique for measuring the rate of turbulent dissipation in the marine environment. Geophys. Res. Lett. 2006, 33, L21608. [Google Scholar] [CrossRef]
- Pope, S.B. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Mullarney, J.C.; Henderson, S.M.; Norris, B.K.; Bryan, K.R.; Fricke, A.T.; Sandwell, D.R.; Culling, D.P. A question of scale: How turbulence around aerial roots shapes the seabed morphology in mangrove forests of the Mekong Delta. Oceanography 2017, 30, 34–47. [Google Scholar] [CrossRef]
- Norris, B.K.; Mullarney, J.C.; Bryan, K.R.; Henderson, S.M. Turbulence within Natural Mangrove Pneumatophore Canopies. J. Geophys. Res. Oceans 2019. accepted for publication. [Google Scholar]
- Stocking, J.B.; Rippe, J.P.; Reidenbach, M.A. Structure and dynamics of turbulent boundary layer flow over healthy and algae-covered corals. Coral Reefs 2016, 35, 1047–1059. [Google Scholar] [CrossRef]
- Breithaupt, J.L.; Duga, E.M.; Witt, R.; Filyaw, N.; Friedland, M.J.; Donnelly; Walters, L.J.; Chambers, L.G. Carbon and nutrient fluxes from seagrass and mangrove wrack on organic and mineral sediment shorelines. Estuar. Coast. Shelf Sci. 2019. in review. [Google Scholar]
- Bouma, T.J.; Vries, M.D.; Low, E.; Kusters, L.; Herman, P.M.J.; Tanczos, I.C.; Van Regenmortel, S. Flow hydrodynamics on a mudflat and in salt marsh vegetation: Identifying general relationships for habitat characterisations. Hydrobiologia 2005, 540, 259–274. [Google Scholar] [CrossRef]
- Tinoco, R.O.; Coco, G. Observations of the effect of emergent vegetation on sediment resuspension under unidirectional currents and waves. Earth Surf. Dyn. 2014, 2, 83–96. [Google Scholar] [CrossRef]
- Paquier, A.E.; Haddad, J.; Lawler, S.; Ferreira, C.M. Quantification of the attenuation of storm surge components by a coastal wetland of the US Mid Atlantic. Estuaries Coast. 2017, 40, 930–946. [Google Scholar] [CrossRef]
- Siniscalchi, F.; Nikora, V.I.; Aberle, J. Plant patch hydrodynamics in streams: Mean flow, turbulence, and drag forces. Water Resour. Res. 2012, 48. [Google Scholar] [CrossRef]
- Nepf, H.M.; Vivoni, E.R. Flow structure in depth-limited, vegetated flow. J. Geophys. Res. Oceans 2000, 105, 28547–28557. [Google Scholar] [CrossRef]
- Chen, Y.; Li, Y.; Cai, T.; Thompson, C.; Li, Y. A comparison of biohydrodynamic interaction within mangrove and saltmarsh boundaries. Earth Surf. Process. Landf. 2016, 41, 1967–1979. [Google Scholar] [CrossRef]
- Koch, E.W.; Barbier, E.B.; Silliman, B.R.; Reed, D.J.; Perillo, G.M.; Hacker, S.D.; Halpern, B.S. Non-linearity in ecosystem services: Temporal and spatial variability in coastal protection. Front. Ecol. Environ. 2009, 7, 29–37. [Google Scholar] [CrossRef]
- Yager, E.M.; Schmeeckle, M.W. The influence of vegetation on turbulence and bed load transport. J. Geophys. Res. Earth Surf. 2013, 118, 1585–1601. [Google Scholar] [CrossRef]
- Kitsikoudis, V.; Kibler, K.M.; Walters, L.J. In-situ measurements of turbulent flow over intertidal natural and degraded oyster reefs in an estuarine lagoon. Ecol. Eng. 2019. in review. [Google Scholar]
- Maza, M.; Adler, K.; Ramos, D.; Garcia, A.M.; Nepf, H. Velocity and drag evolution from the leading edge of a model mangrove forest. J. Geophys. Res. Oceans 2017, 122, 9144–9159. [Google Scholar] [CrossRef]
- Kitsikoudis, V.; Yagci, O.; Kirca, V.S.O.; Kellecioglu, D. Experimental investigation of channel flow through idealized isolated tree-like vegetation. Environ. Fluid Mech. 2016, 16, 1283–1308. [Google Scholar] [CrossRef]
- Lanckriet, T.; Puleo, J.A. Near-bed turbulence dissipation measurements in the inner surf and swash zone. J. Geophys. Res. Oceans 2013, 118, 6634–6647. [Google Scholar] [CrossRef]
- Brinkkemper, J.A.; Lanckriet, T.; Grasso, F.; Puleo, J.A.; Ruessink, B.G. Observations of turbulence within the surf and swash zone of a field-scale sandy laboratory beach. Coast. Eng. 2016, 113, 62–72. [Google Scholar] [CrossRef]
- Pieterse, A.; Puleo, J.A.; McKenna, T.E.; Aiken, R.A. Near-bed shear stress, turbulence production and dissipation in a shallow and narrow tidal channel. Earth Surf. Process. Landf. 2015, 40, 2059–2070. [Google Scholar] [CrossRef]
- Norris, B.K.; Mullarney, J.C.; Bryan, K.R.; Henderson, S.M. The effect of pneumatophore density on turbulence: A field study in a Sonneratia-dominated mangrove forest, Vietnam. Cont. Shelf Res. 2017, 147, 114–127. [Google Scholar] [CrossRef]
- Kamali, B.; Hashim, R. Mangrove restoration without planting. Ecol. Eng. 2011, 37, 387–391. [Google Scholar] [CrossRef]
- Peterson, J.M.; Bell, S.S. Tidal events and salt-marsh structure influence black mangrove (Avicennia germinans) recruitment across an ecotone. Ecology 2012, 93, 1648–1658. [Google Scholar] [CrossRef]
- Donnelly, M.J.; Walters, L.J. Trapping of Rhizophora mangle by coexisting early successional species. Estuaries Coast. 2014, 37, 1562–1571. [Google Scholar] [CrossRef]

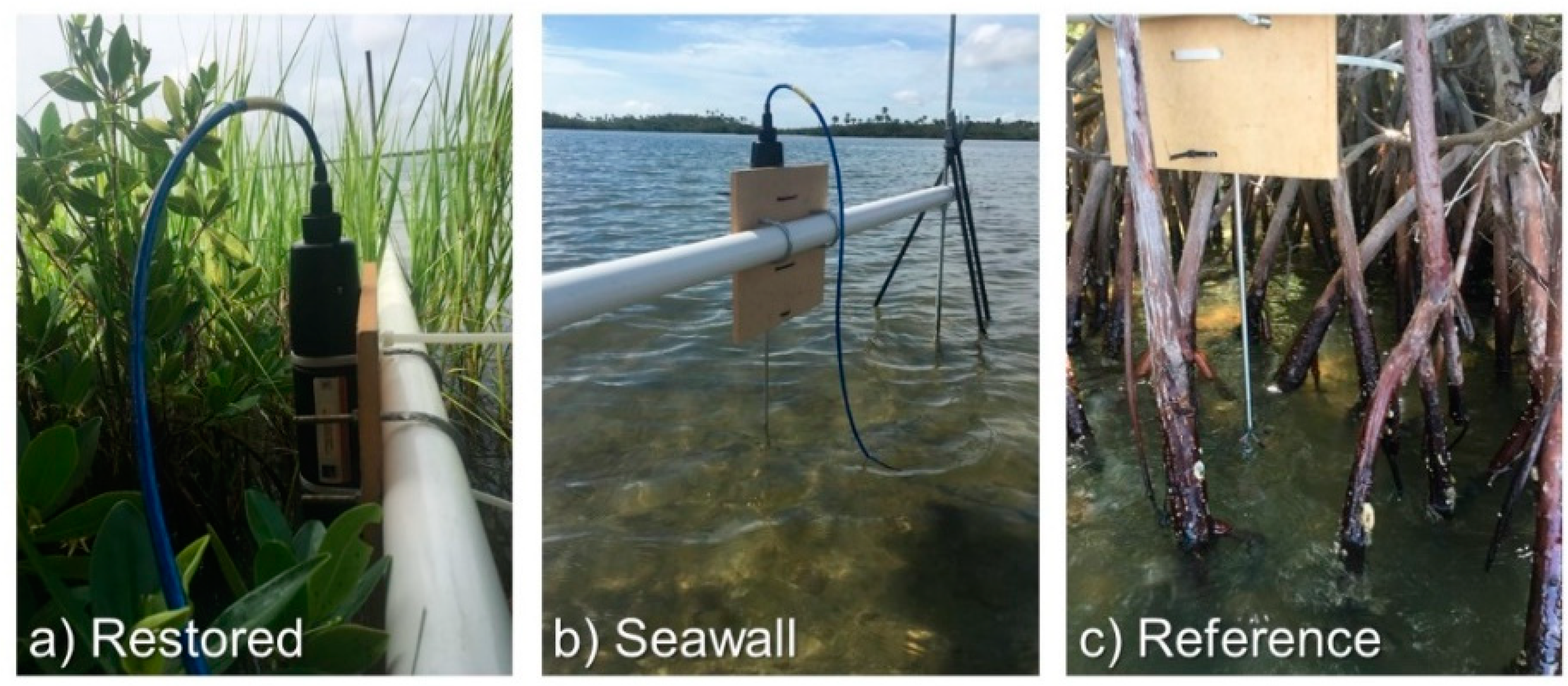
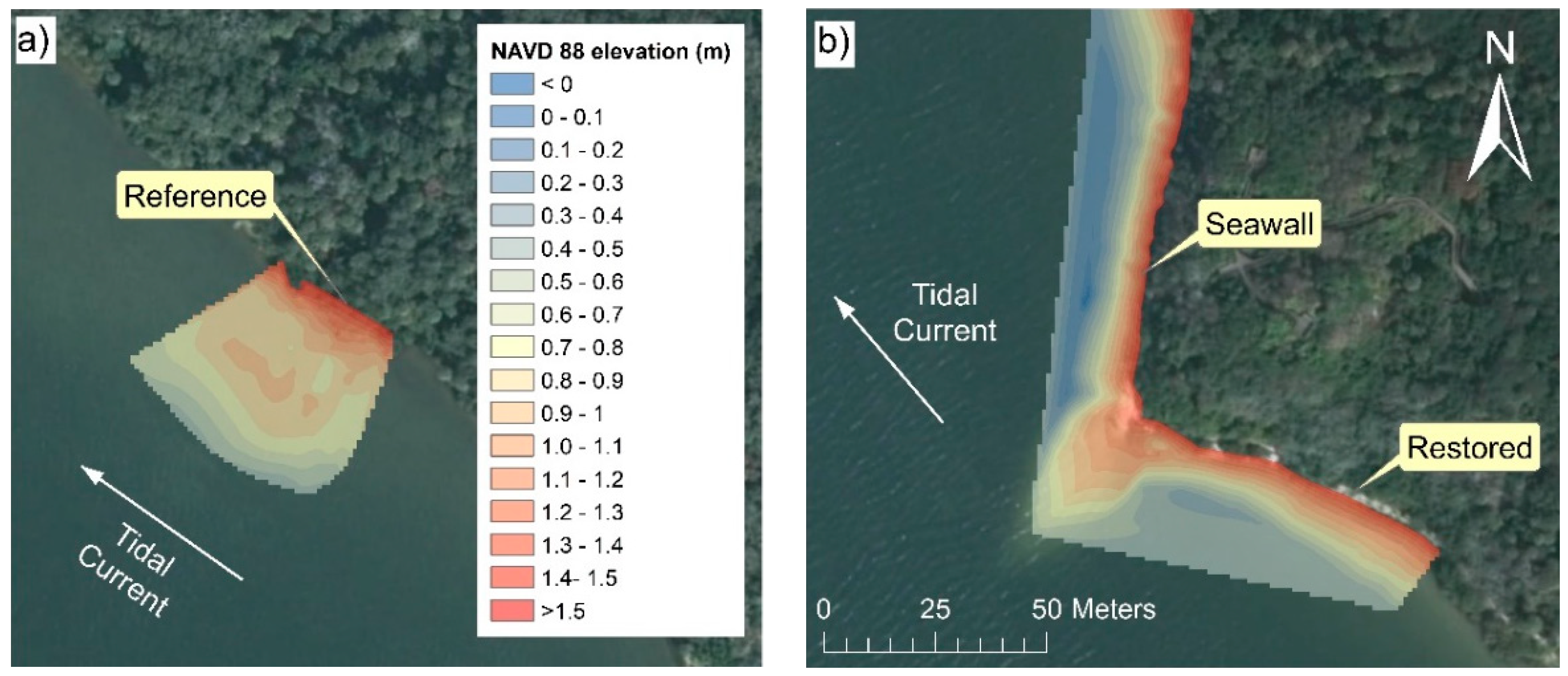
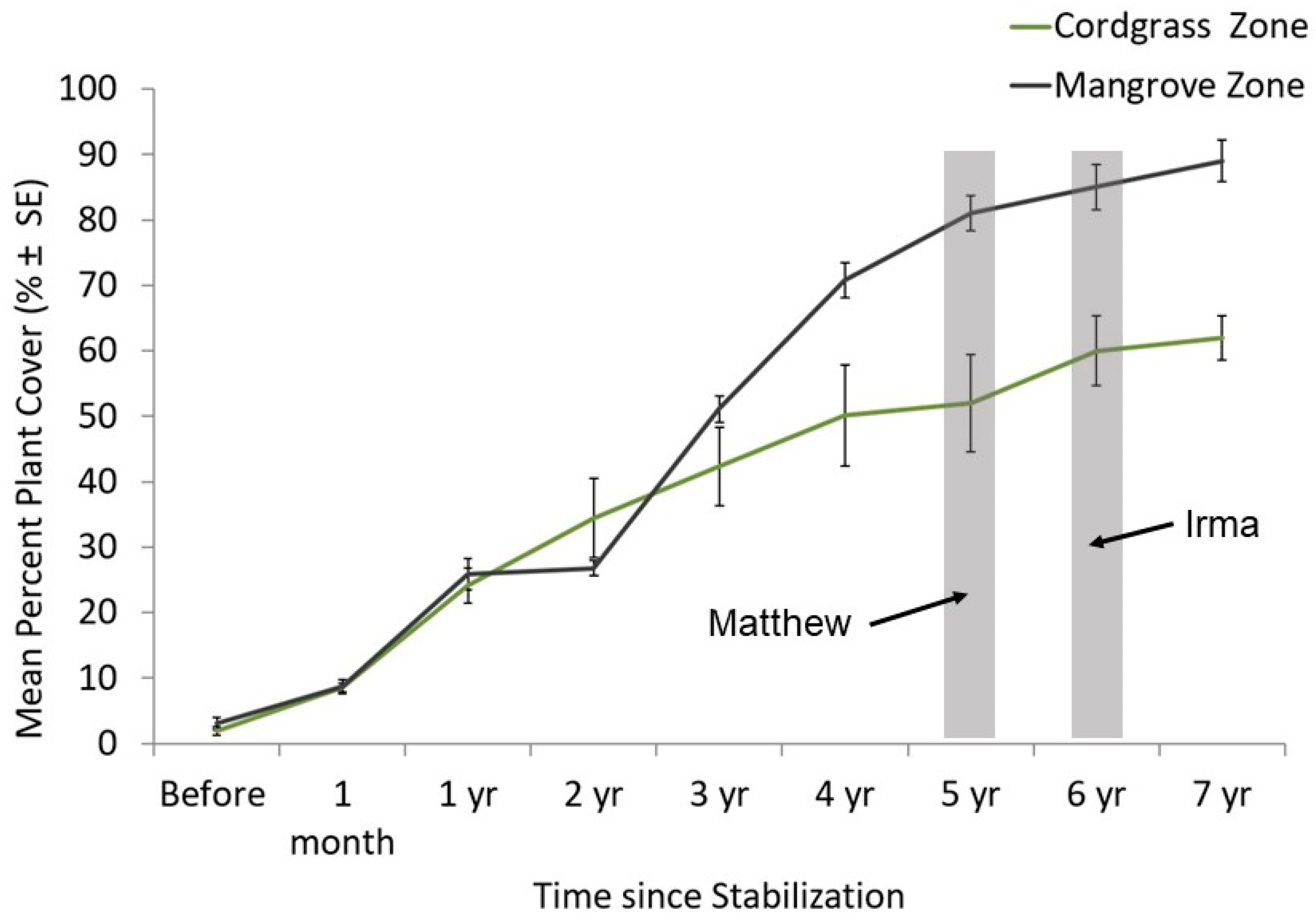
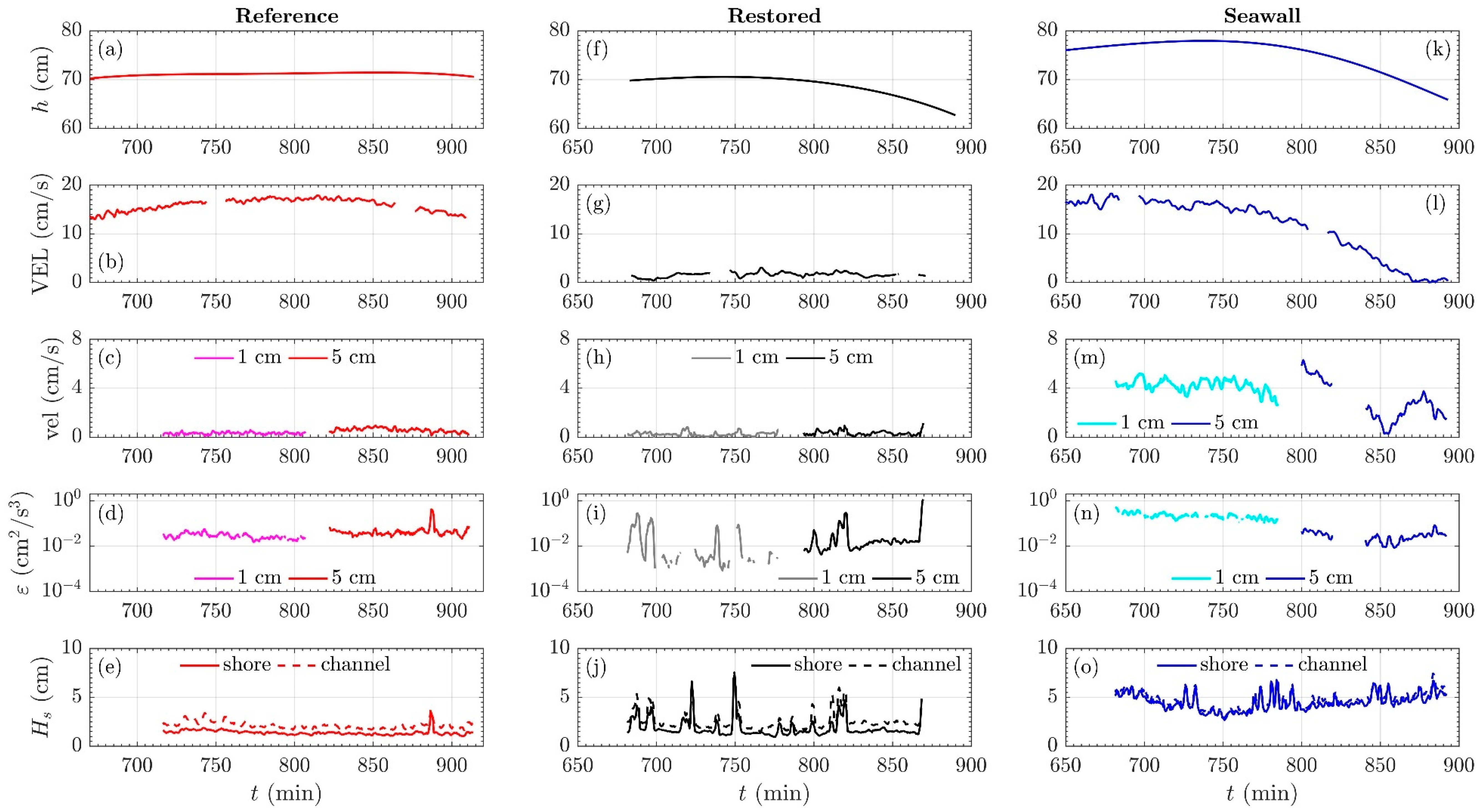
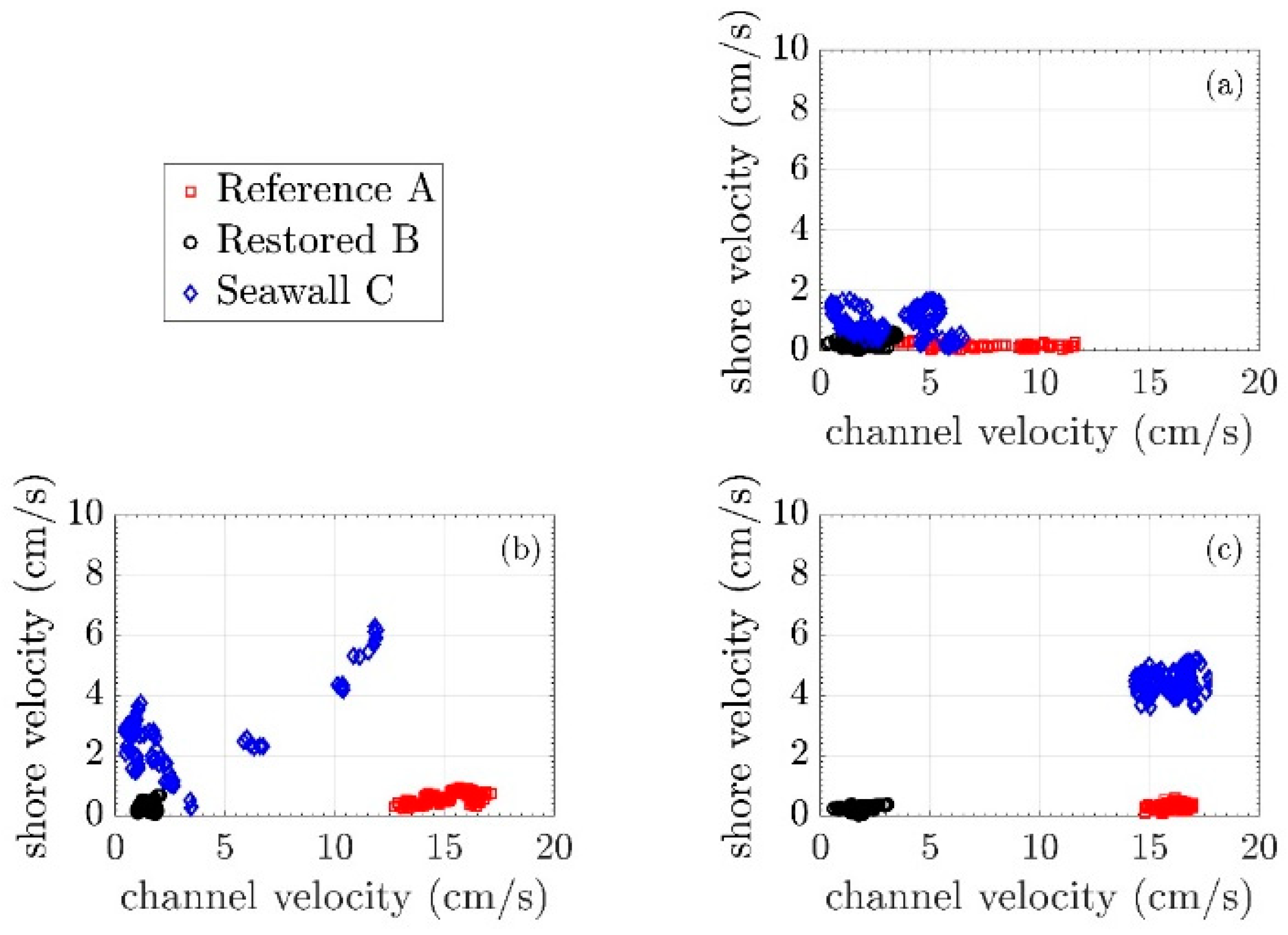
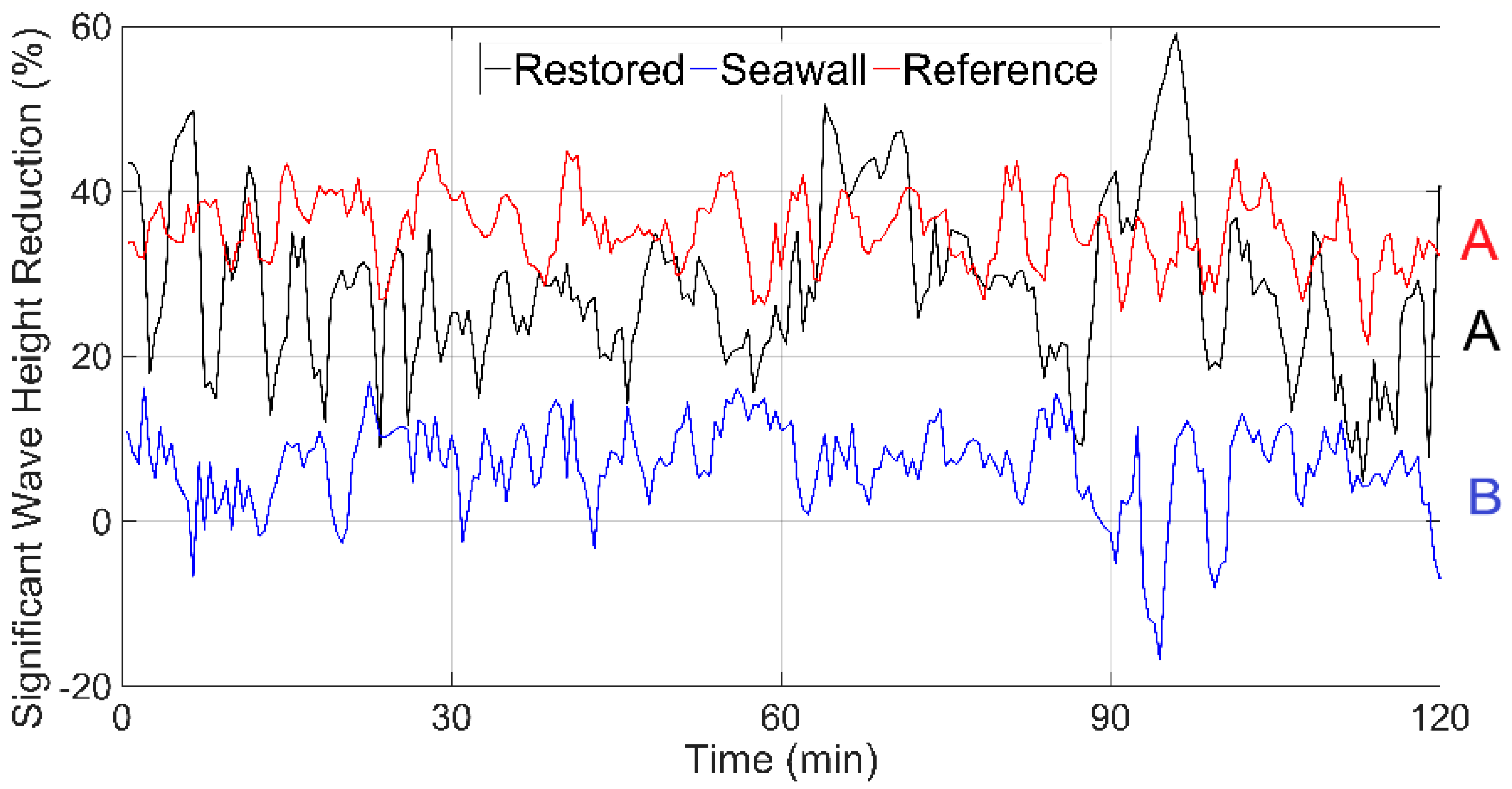

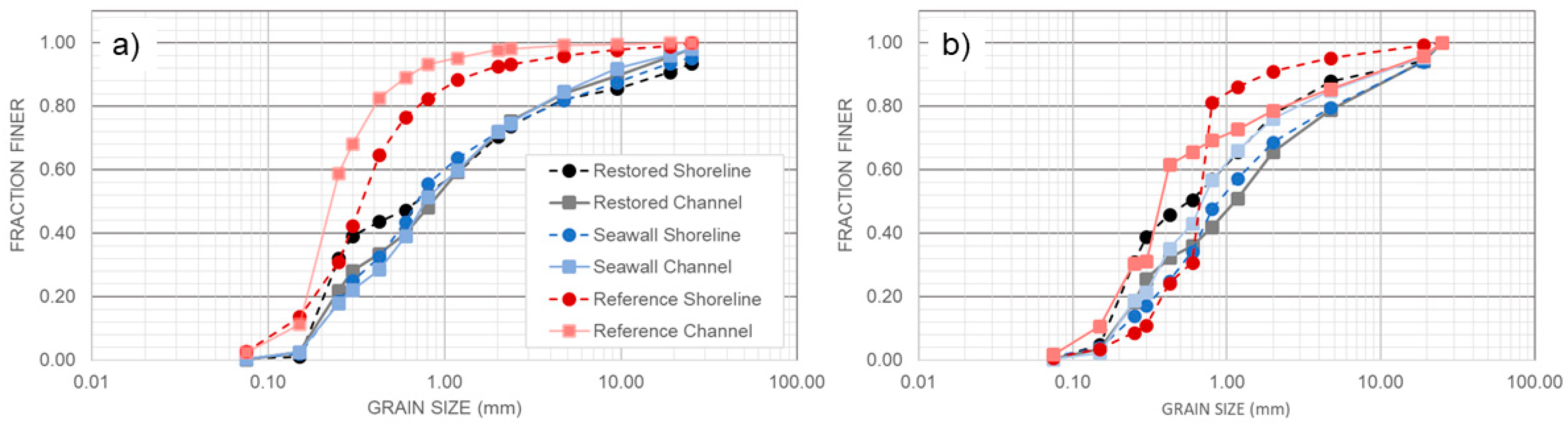
| Site | Slope (m/m) Mean ± SE | Stem Density (n) (Elements/m2) | Frontal Area (α) (m2/m3) | Solid Volume Fraction (ϕ) (m3/m3) | Mangrove Seedlings Mean ± SE | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Intertidal Shoreline | Subtidal Nearshore | Overall | mean, CV | Probe | Mean, CV | PROBE | Mean, CV | Probe | Number per Transect m | Diameter (mm) | Cohort Age (years) | |
| Restored | 0.050 ± 0.001 | 0.051 ± 0.001 | 0.050 ± 0.001 | 97, 0.79 | 252 | 0.94, 0.59 | 1.62 | 0.012, 1.11 | 0.009 | 0.0 ± 0.0 | 0.0 ± 0.0 | - |
| Seawall | 0.130 ± 0.005 | 0.061 ± 0.001 | 0.110 ± 0.003 | 24, 1.64 | 0 | 0.20, 1.55 | 0.00 | 0.002, 1.59 | 0.000 | 0.0 ± 0.0 | 0.0 ± 0.0 | - |
| Reference | 0.056 ± 0.001 | 0.008 ± 0.001 | 0.027 ± 0.001 | 83, 0.39 | 116 | 2.49, 0.56 | 3.12 | 0.068, 0.74 | 0.070 | 4.0 ± 0.8 | 13.0 ± 0.5 | 1 |
| Waves | Current | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Nov 2017 Significant Wave Height (cm) | Aug 2017 1.0 cm Above Bed (cm/s) | Nov 2017 1.0 cm Above Bed (cm/s) | Nov 2017 5.0 cm Above Bed (cm/s) | |||||||||
| Channel | Shoreline | Percent Change | Channel | Shoreline | Percent Change | Channel | Shoreline | Percent Change | Channel | Shoreline | Percent Change | |
| Restored | 2.4 ± 0.2 | 1.4 ± 0.1 | 36 ± 8 | 1.9 ± 0.8 | 0.3 ± 0.1 | 83.8 ± 12.0 | 1.6 ± 0.5 | 0.2 ± 0.1 | 86.9 ± 8.1 | 1.5 ± 0.3 | 0.3 ± 0.1 | 80.1 ± 9.3 |
| Seawall | 4.8 ± 0.8 | 4.5 ± 0.8 | 7 ± 6 | 3.9 ± 1.5 + | 0.9 ± 0.4 + | 74.4 ± 16.1 + | 16.0 ± 0.9 | 4.4 ± 0.4 | 72.3 ± 2.5 | 5.8 ± 4.1 + | 2.9 ± 1.8 + | 36.4 ± 39.3 + |
| Reference | 2.2 ± 0.4 | 1.4 ± 0.3 | 35 ± 6 | 7.6 ± 2.3 | 0.2 ± 0.1 | 97.6 ± 1.8 | 16.1 ± 0.5 | 0.3 ± 0.1 | 98.2 ± 0.5 | 15.2 ± 1.2 | 0.6 ± 0.2 | 96.2 ± 1.0 |
| Mean Square | df | F-Stat | p-Value | |
|---|---|---|---|---|
| Wave Attenuation Rate | 9.20 * | 2 | 2694.57 | <0.001 |
| Current Attenuation 1.0 cm Above Bed | 3.32 * | 2 | 475.94 | <0.001 |
| Current Attenuation 5.0 cm Above Bed | 6.23 * | 2 | 216.89 | <0.001 |
| Normalized TKE Dissipation Rate | 0.68 * | 2 | 1660.89 | <0.001 |
| Comparison | Wave Attenuation Rate | Current Attenuation Rate | Normalized TKE Dissipation Rate | |||||
|---|---|---|---|---|---|---|---|---|
| 1.0 cm Above Bed | 5.0 cm Above Bed | 5.0 cm Above Bed | ||||||
| Mean ± SE Difference (%) | p-Value | Mean ± SE Difference (%) | p-Value | Mean ± SE Difference (%) | p-Value | Mean ± SE Difference (m−1) | p-Value | |
| Restored-Seawall | 29.0 ± 0.7 * | <0.001 | 12.3 ± 0.9 * | <0.001 | 43.7 ± 3.1 * | <0.001 | 0.06 ± 0.003 * | <0.001 |
| Restored-Reference | 0.5 ± 0.7 | 0.780 | −12.5 ± 0.9 * | <0.001 | −16.1 ± 2.3 * | <0.001 | −0.08 ± 0.010 * | <0.001 |
| Reference-Seawall | 28.6 ± 0.4 * | <0.001 | 24.8 ± 0.8 * | <0.001 | 59.8 ± 2.9 * | <0.001 | 0.14 ± 0.002 * | <0.001 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kibler, K.M.; Kitsikoudis, V.; Donnelly, M.; Spiering, D.W.; Walters, L. Flow–Vegetation Interaction in a Living Shoreline Restoration and Potential Effect to Mangrove Recruitment. Sustainability 2019, 11, 3215. https://doi.org/10.3390/su11113215
Kibler KM, Kitsikoudis V, Donnelly M, Spiering DW, Walters L. Flow–Vegetation Interaction in a Living Shoreline Restoration and Potential Effect to Mangrove Recruitment. Sustainability. 2019; 11(11):3215. https://doi.org/10.3390/su11113215
Chicago/Turabian StyleKibler, Kelly M., Vasileios Kitsikoudis, Melinda Donnelly, David W. Spiering, and Linda Walters. 2019. "Flow–Vegetation Interaction in a Living Shoreline Restoration and Potential Effect to Mangrove Recruitment" Sustainability 11, no. 11: 3215. https://doi.org/10.3390/su11113215
APA StyleKibler, K. M., Kitsikoudis, V., Donnelly, M., Spiering, D. W., & Walters, L. (2019). Flow–Vegetation Interaction in a Living Shoreline Restoration and Potential Effect to Mangrove Recruitment. Sustainability, 11(11), 3215. https://doi.org/10.3390/su11113215







