Evaluation of Cleaner Production for Gold Mines Employing a Hybrid Multi-Criteria Decision Making Approach
Abstract
:1. Introduction
2. Materials and Methods
2.1. Evaluation Criteria of Cleaner Production for Gold Mines
2.2. Probabilistic Linguistic Term Sets
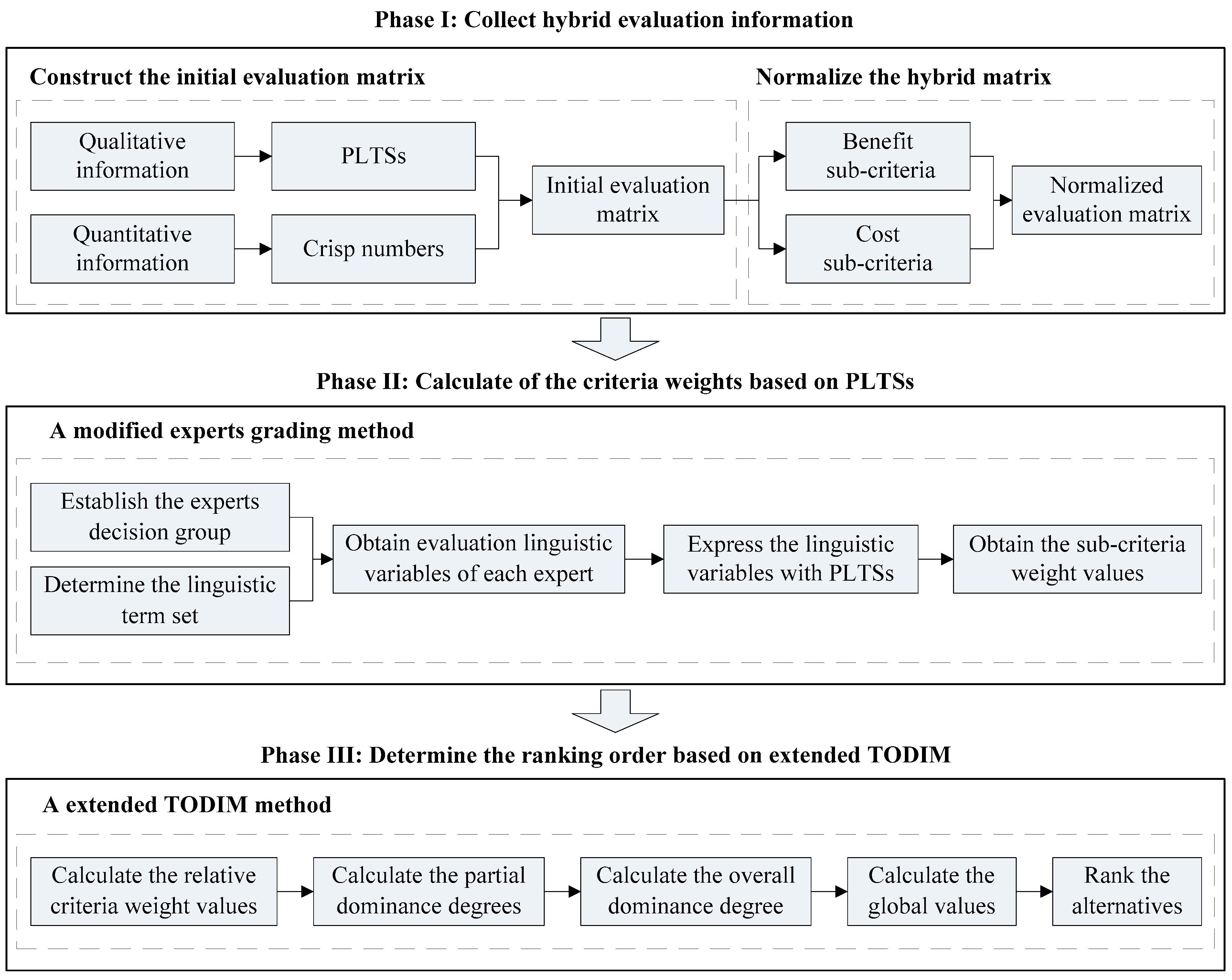
3. Hybrid Multi-Criteria Decision Making Approach
3.1. Phase I: Collect Hybrid Evaluation Information
3.2. Phase II: Calculate the Criteria Weights based on PLTSs
3.3. Phase III: Determine the Ranking Order Based on Extended TODIM
4. Case Study
5. Discussions
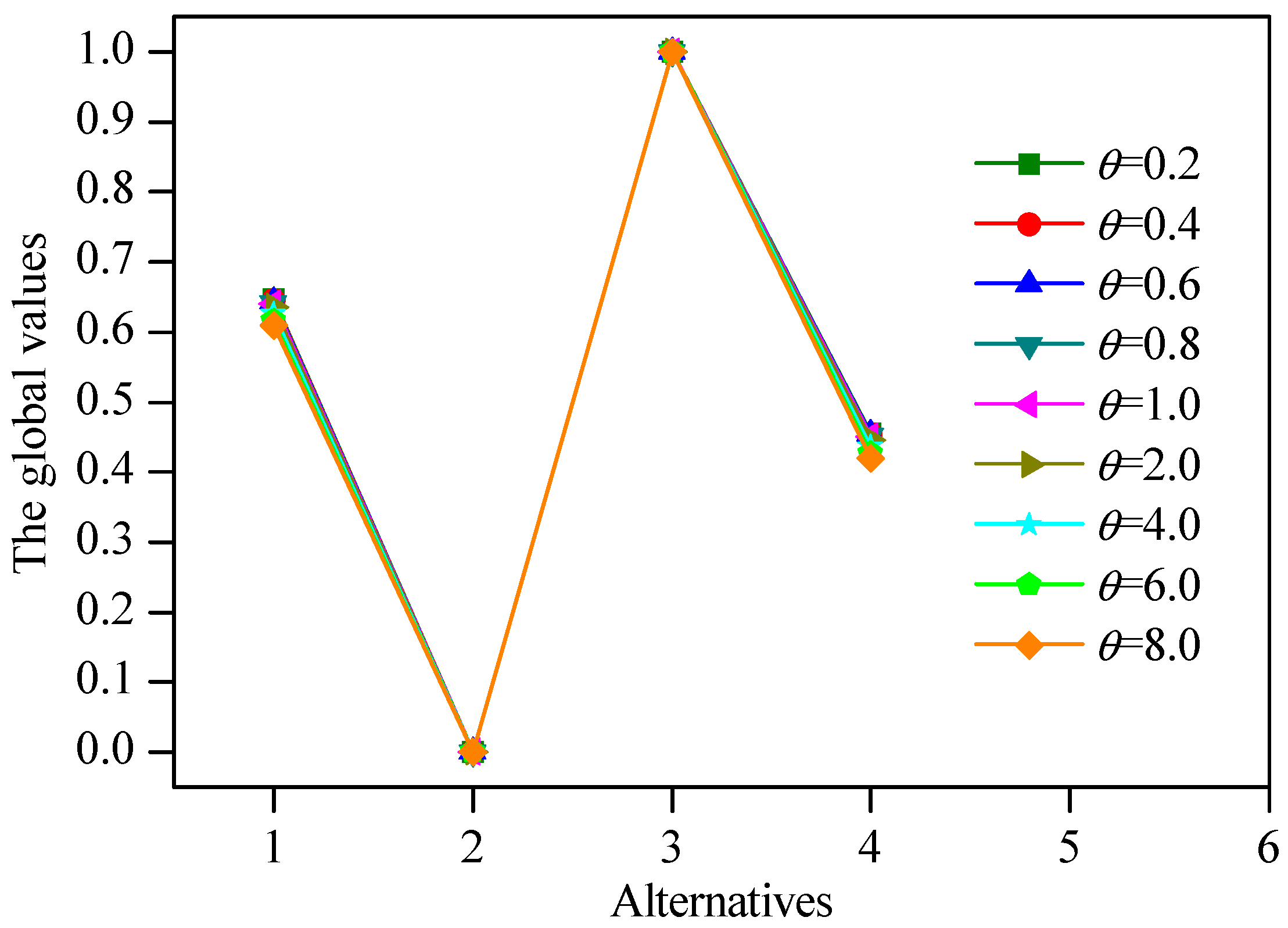
5.1. Sensitivity Analysis
5.2. Comparison Analysis
5.3. Managerial Implication
6. Conclusions
Author Contributions
Funding
Acknowledgement
Conflicts of Interest
References
- Harris, R.D.F.; Shen, J. The intrinsic value of gold: An exchange rate-free price index. J. Int. Money Financ. 2017, 79, 203–217. [Google Scholar] [CrossRef]
- Kumah, A. Sustainability and gold mining in the developing world. J. Clean. Prod. 2006, 14, 315–323. [Google Scholar] [CrossRef]
- Gorman, M.R.; Dzombak, D.A. A review of sustainable mining and resource management: Transitioning from the life cycle of the mine to the life cycle of the mineral. Resour. Conserv. Recycl. 2018, 137, 281–291. [Google Scholar] [CrossRef]
- Assawincharoenkij, T.; Hauzenberger, C.; Ettinger, K.; Sutthirat, C. Mineralogical and geochemical characterization of waste rocks from a gold mine in northeastern Thailand: Application for environmental impact protection. Environ. Sci. Pollut. Res. 2018, 25, 3488–3500. [Google Scholar] [CrossRef] [PubMed]
- Kiventerä, J.; Lancellotti, I.; Catauro, M.; Dal Poggetto, F.; Leonelli, C.; Illikainen, M. Alkali activation as new option for gold mine tailings inertization. J. Clean. Prod. 2018, 187, 76–84. [Google Scholar] [CrossRef]
- Xiao, R.; Wang, S.; Li, R.H.; Wang, J.J.; Zhang, Z.Q. Soil heavy metal contamination and health risks associated with artisanal gold mining in Tongguan, Shaanxi, China. Ecotoxicol. Environ. Saf. 2017, 141, 17–24. [Google Scholar] [CrossRef] [PubMed]
- Mayala, L.P.; Veiga, M.M.; Khorzoughi, M.B. Assessment of mine ventilation systems and air pollution impacts on artisanal tanzanite miners at Merelani, Tanzania. J. Clean. Prod. 2016, 116, 118–124. [Google Scholar] [CrossRef]
- Euler, D.S. Application of ventilation management programs for improved mine safety. Int. J. Min. Sci. Technol. 2017, 27, 647–650. [Google Scholar]
- Jordaan, M.A.; Mimba, M.E.; NguemheFils, S.C.; Edith-Etakah, B.T.; Shapi, M.; Penaye, J.; Davies, T.C. Occurrence and levels of potentially harmful elements (PHEs) in natural waters of the gold mining areas of the Kette-Batouri region of Eastern Cameroon. Environ. Monit. Assess. 2018, 190, 416. [Google Scholar] [CrossRef] [PubMed]
- Tomiyasu, T.; Kodamatani, H.; Hamada, Y.K.; Matsuyama, A.; Imura, R.; Taniguchi, Y.; Hidayati, N.; Rahajoe, J.S. Distribution of total mercury and methylmercury around the small-scale gold mining area along the Cikaniki River, Bogor, Indonesia. Environ. Sci. Pollut. Res. 2017, 24, 2643–2652. [Google Scholar] [CrossRef] [PubMed]
- Carvalho, P.C.S.; Neiva, A.M.R.; Silva, M.M.V.G.; Santos, A.C.T. Human health risks in an old gold mining area with circum-neutral drainage, central Portugal. Environ. Geochem. Health 2017, 39, 43–62. [Google Scholar] [CrossRef] [PubMed]
- Drace, K.; Kiefer, A.M.; Veiga, M.M.; Williams, M.K.; Ascari, B.; Knapper, K.A.; Logan, K.M.; Breslin, V.M.; Skidmore, A.; Bolt, D.A.; et al. Mercury-free, small-scale artisanal gold mining in Mozambique: Utilization of magnets to isolate gold at clean tech mine. J. Clean. Prod. 2012, 32, 88–95. [Google Scholar] [CrossRef]
- Gutiérrez Aguilar, C.M.; Panameño, R.; Perez Velazquez, A.; Angel Álvarez, B.E.; Kiperstok, A.; César, S.F. Cleaner production applied in a small furniture industry in Brazil: Addressing focused changes in design to reduce waste. Sustainability 2017, 9, 1867. [Google Scholar] [CrossRef]
- Matos, L.M.; Anholon, R.; da Silva, D.; Ordoñez, R.E.C.; Quelhas, O.L.G.; Leal Filho, W.; de Santa-Eulalia, L.A. Implementation of cleaner production: A ten-year retrospective on benefits and difficulties found. J. Clean. Prod. 2018, 187, 409–420. [Google Scholar] [CrossRef]
- Malinauskienė, M.; Kliopova, I.; Slavickaitė, M.; Staniškis, J.K. Integrating resource criticality assessment into evaluation of cleaner production possibilities for increasing resource efficiency. Clean Technol. Environ. 2016, 18, 1333–1344. [Google Scholar] [CrossRef]
- Hilson, G.; Nayee, V. Environmental management system implementation in the mining industry: A key to achieving cleaner production. Int. J. Miner. Process. 2002, 64, 19–41. [Google Scholar] [CrossRef]
- Song, S.Q.; Zhou, Y.Z. Demonstration research on cleaner production of mines. China Popul. Resour. Environ. 2003, 13, 106–110. [Google Scholar]
- Hilson, G. Defining “cleaner production” and “pollution prevention” in the mining context. Miner. Eng. 2003, 16, 305–321. [Google Scholar] [CrossRef]
- Rajaram, R.; Dutta, S.; Parameswaran, K. Sustainable Mining Practices: A Global Perspective; Taylor & Francis: London, UK, 2005. [Google Scholar]
- Fan, Y.V.; Varbanov, P.S.; Klemeš, J.J.; Nemet, A. Process efficiency optimisation and integration for cleaner production. J. Clean. Prod. 2018, 174, 177–183. [Google Scholar] [CrossRef]
- Severo, E.A.; de Guimarães, J.C.F.; Dorion, E.C.H. Cleaner production, social responsibility and eco-innovation: Generations’ perception for a sustainable future. J. Clean. Prod. 2018, 186, 91–103. [Google Scholar] [CrossRef]
- Liang, W.Z.; Zhao, G.Y.; Luo, S.Z. Linguistic neutrosophic Hamacher aggregation operators and the application in evaluating land reclamation schemes for mines. PLoS ONE 2018, 13, e0206178. [Google Scholar] [CrossRef] [PubMed]
- Zhang, P.L.; Duan, N.; Dan, Z.G.; Shi, F.F.; Wang, H.F. An understandable and practicable cleaner production assessment model. J. Clean. Prod. 2018, 187, 1094–1102. [Google Scholar] [CrossRef]
- Tseng, M.L.; Lin, Y.H.; Chiu, A.S. Fuzzy AHP-based study of cleaner production implementation in Taiwan PWB manufacturer. J. Clean. Prod. 2009, 17, 1249–1256. [Google Scholar] [CrossRef]
- Peng, W.G.; Li, C.G. Fuzzy-Soft set in the field of cleaner production evaluation for aviation industry. Commun. Inf. Sci. Manag. Eng. 2012, 2, 39–43. [Google Scholar]
- Basappaji, K.M.; Nagesha, N. Assessment of cleaner production level in agro based industries—A fuzzy logic approach. Energy Proced. 2014, 54, 127–134. [Google Scholar] [CrossRef]
- Gong, B.G.; Guo, D.D.; Zhang, X.Q.; Cheng, J.S. An approach for evaluating cleaner production performance in iron and steel enterprises involving competitive relationships. J. Clean. Prod. 2017, 142, 739–748. [Google Scholar] [CrossRef]
- Dong, L.J.; Shu, W.W.; Li, X.B.; Zhang, J.M. Quantitative evaluation and case studies of cleaner mining with multiple indexes considering uncertainty factors for phosphorus mines. J. Clean. Prod. 2018, 183, 319–334. [Google Scholar] [CrossRef]
- Liang, W.Z.; Zhao, G.Y.; Luo, S.Z. An integrated EDAS-ELECTRE method with picture fuzzy information for cleaner production evaluation in gold mines. IEEE Access 2018, 6, 65747–65759. [Google Scholar] [CrossRef]
- Liang, W.Z.; Zhao, G.Y.; Luo, S.Z. Selecting the optimal mine ventilation system via a decision making framework under hesitant linguistic environment. Symmetry 2018, 10, 283. [Google Scholar] [CrossRef]
- Luo, S.Z.; Cheng, P.F.; Wang, J.Q.; Huang, Y.J. Selecting project delivery systems based on simplified neutrosophic linguistic preference relations. Symmetry 2017, 9, 151. [Google Scholar] [CrossRef]
- Liang, W.Z.; Zhao, G.Y.; Wu, H. Evaluating investment risks of metallic mines using an extended TOPSIS method with linguistic neutrosophic numbers. Symmetry 2017, 9, 149. [Google Scholar] [CrossRef]
- Rodriguez, R.M.; Martinez, L.; Herrera, F. Hesitant fuzzy linguistic term sets for decision making. IEEE Trans. Fuzzy Syst. 2012, 20, 109–119. [Google Scholar] [CrossRef]
- Yager, R.R. Multicriteria decision making with ordinal/linguistic intuitionistic fuzzy sets for mobile apps. IEEE Trans. Fuzzy Syst. 2016, 24, 590–599. [Google Scholar] [CrossRef]
- Liang, W.Z.; Zhao, G.Y.; Hong, C.S. Selecting the optimal mining method with extended multi-objective optimization by ratio analysis plus the full multiplicative form (MULTIMOORA) approach. Neural Comput. Appl. 2018. [Google Scholar] [CrossRef]
- Pang, Q.; Wang, H.; Xu, Z.S. Probabilistic linguistic term sets in multi-attribute group decision making. Inf. Sci. 2016, 369, 128–143. [Google Scholar] [CrossRef]
- Wei, G.W. Grey relational analysis model for dynamic hybrid multiple attribute decision making. Knowl. Based Syst. 2011, 24, 672–679. [Google Scholar] [CrossRef]
- Zhang, X.L.; Xing, X.M. Probabilistic linguistic VIKOR method to evaluate green supply chain initiatives. Sustainability 2017, 9, 1231. [Google Scholar] [CrossRef]
- Peng, H.G.; Zhang, H.Y.; Wang, J.Q. Cloud decision support model for selecting hotels on TripAdvisor.com with probabilistic linguistic information. Int. J. Hosp. Manag. 2018, 68, 124–138. [Google Scholar] [CrossRef]
- Liao, H.C.; Jiang, L.S.; Xu, Z.S.; Xu, J.P.; Herrera, F. A linear programming method for multiple criteria decision making with probabilistic linguistic information. Inf. Sci. 2017, 415, 341–355. [Google Scholar] [CrossRef]
- Gomes, L.F.A.M.; Lima, M.M.P.P. TODIM: Basics and application to multicriteria ranking of projects with environmental impacts. Found. Comput. Decis. Sci. 1992, 16, 113–127. [Google Scholar]
- Liang, W.Z.; Zhao, G.Y.; Wu, H.; Chen, Y. Assessing the risk degree of goafs by employing hybrid TODIM method under uncertainty. Bull. Eng. Geol. Environ. 2018. [Google Scholar] [CrossRef]
- Tosun, Ö.; Akyüz, G. A fuzzy TODIM approach for the supplier selection problem. Int. J. Comput. Int. Syst. 2015, 8, 317–329. [Google Scholar] [CrossRef]
- Zindani, D.; Maity, S.R.; Bhowmik, S.; Chakraborty, S. A material selection approach using the TODIM (TOmada de Decisao Interativa Multicriterio) method and its analysis. Int. J. Mater. Res. 2017, 108, 345–354. [Google Scholar] [CrossRef]
- Wu, M.Q.; An, Y.L.; Zhou, X.D.; Xiao, Y. Establishment of the index system of cleaner production assessment of phosplate minesddphosplate mines of Guiyang a case study. Environ. Sci. Manag. 2009, 34, 189–194. [Google Scholar]
- Zhou, H.L.; Yang, S.; Chen, J.H. Research and application of cleaner production evaluation index system in gold mine. Gold Sci. Technol. 2017, 25, 93–100. [Google Scholar]
- Liang, W.Z.; Zhao, G.Y.; Hong, C.S. Performance Assessment of Circular Economy for Phosphorus Chemical Firms Based on VIKOR-QUALIFLEX Method. J. Clean. Prod. 2018, 196, 1365–1378. [Google Scholar] [CrossRef]
- Liang, W.Z.; Zhao, G.Y.; Wu, H.; Dai, B. Risk assessment of rockburst via an extended MABAC method under fuzzy environment. Tunn. Undergr. Space Technol. 2019, 83, 533–544. [Google Scholar] [CrossRef]
- Basu, A.J.; van Zyl, D.J. Industrial ecology framework for achieving cleaner production in the mining and minerals industry. J. Clean. Prod. 2006, 14, 299–304. [Google Scholar] [CrossRef]
- Xu, Z.S. Deviation measures of linguistic preference relations in group decision making. Omega 2005, 33, 249–254. [Google Scholar] [CrossRef]
- Gou, X.J.; Xu, Z.S.; Liao, H.C. Multiple criteria decision making based on Bonferroni means with hesitant fuzzy linguistic information. Soft Comput. 2017, 21, 6515–6529. [Google Scholar] [CrossRef]
- Gou, X.J.; Xu, Z.S. Novel basic operational laws for linguistic terms, hesitant fuzzy linguistic term sets and probabilistic linguistic term sets. Inf. Sci. 2016, 372, 407–427. [Google Scholar] [CrossRef]
- Krohling, R.A.; de Souza, T.T. Combining prospect theory and fuzzy numbers to multi-criteria decision making. Expert Syst. Appl. 2012, 39, 11487–11493. [Google Scholar] [CrossRef]
- Qin, Q.D.; Liang, F.Q.; Li, L.; Chen, Y.W.; Yu, G.F. A TODIM-based multi-criteria group decision making with triangular intuitionistic fuzzy numbers. Appl. Soft Comput. 2017, 55, 93–107. [Google Scholar] [CrossRef]
- Gomes, L.F.A.M. An application of the TODIM method to the multicriteria rental evaluation of residential properties. Eur. J. Oper. Res. 2009, 193, 204–211. [Google Scholar] [CrossRef]
- Zhao, H.R.; Zhao, H.R.; Guo, S. Evaluating the comprehensive benefit of eco-industrial parks by employing multi-criteria decision making approach for circular economy. J. Clean. Prod. 2017, 142, 2262–2276. [Google Scholar] [CrossRef]
- Liu, P.D. A novel method for hybrid multiple attribute decision making. Knowl.-Based Syst. 2009, 22, 388–391. [Google Scholar]
- Fan, Z.P.; Zhang, X.; Chen, F.D.; Liu, Y. Extended TODIM method for hybrid multiple attribute decision making problems. Knowl.-Based Syst. 2013, 42, 40–48. [Google Scholar] [CrossRef]
- Hossain, M.U.; Poon, C.S.; Dong, Y.H.; Lo, I.M.; Cheng, J.C. Development of social sustainability assessment method and a comparative case study on assessing recycled construction materials. Int. J. Life Cycle Assess. 2018, 23, 1654–1674. [Google Scholar] [CrossRef]
- Wang, F.; Li, H. Novel method for hybrid multiple attribute decision making based on TODIM method. J. Syst. Eng. Electron. 2015, 26, 1023–1031. [Google Scholar] [CrossRef]

| DMs | Education | Positional Titles | Employment Position | Working Years |
|---|---|---|---|---|
| M.S. | Senior Engineer | Mine manager | 21 | |
| M.S. | Senior Engineer | Deputy mine manager | 18 | |
| M.S. | Senior Engineer | Engineering technologist | 19 | |
| Ph.D. | Engineer | Deputy mine manager | 23 | |
| Ph.D. | Engineer | Engineering technologist | 22 | |
| Ph.D. | Engineer | Senior adviser | 30 | |
| Ph.D. | Senior Engineer | Mine manager | 25 | |
| Ph.D. | Senior Engineer | Mine manager | 33 | |
| Ph.D. | Senior Engineer | Deputy mine manager | 29 | |
| Ph.D. | Senior Engineer | Senior adviser | 35 |
| (m3/t) | 0.37 | 0.42 | 0.29 | 0.31 |
| (kgce/t) | 3.31 | 4.54 | 3.94 | 5.65 |
| (%) | 76 | 69 | 73 | 65 |
| (%) | 71 | 58 | 84 | 67 |
| (%) | 28 | 21 | 36 | 27 |
| (%) | 100 | 91 | 100 | 96 |
| (%) | 92 | 85 | 94 | 100 |
| (%) | 73 | 93 | 90 | 80 |
| (%) | 86 | 92 | 77 | 82 |
| (%) | 74 | 89 | 86 | 93 |
| (%) | 21 | 19 | 12 | 18 |
| (%) | 13 | 19 | 17 | 15 |
| (m3/t) | 0.3846 | 0.0000 | 1.0000 | 0.8462 |
| (kgce/t) | 1.0000 | 0.4744 | 0.7308 | 0.0000 |
| (%) | 1.0000 | 0.3636 | 0.7273 | 0.0000 |
| (%) | 0.5000 | 0.0000 | 1.0000 | 0.3462 |
| (%) | 0.4667 | 0.0000 | 1.0000 | 0.4000 |
| (%) | 1.0000 | 0.0000 | 1.0000 | 0.5556 |
| (%) | 0.4667 | 0.0000 | 0.6000 | 1.0000 |
| (%) | 0.0000 | 1.0000 | 0.8500 | 0.3500 |
| (%) | 0.6000 | 1.0000 | 0.0000 | 0.3333 |
| (%) | 0.0000 | 0.7895 | 0.6316 | 1.0000 |
| (%) | 0.0000 | 0.6667 | 1.0000 | 0.7778 |
| (%) | 1.0000 | 0.0000 | 0.3333 | 0.6667 |
| Very high | High | High | High | Very high | 0.0774 | |||
| High | High | Very high | Very high | Very high | 0.0839 | |||
| Medium | Slightly high | High | Medium | Slightly high | 0.0258 | |||
| High | Medium | Slightly high | High | Very high | 0.0516 | |||
| High | Very high | Very high | High | Slightly high | 0.0710 | |||
| Slightly high | Medium | High | Slightly high | Medium | 0.0258 | |||
| Very high | High | Slightly high | High | Slightly high | 0.0581 | |||
| High | Very high | High | Very high | high | 0.0774 | |||
| Very high | Very high | high | High | High | 0.0774 | |||
| Very high | High | Very high | High | Slightly high | 0.0710 | |||
| Very high | Very high | High | High | High | 0.0774 | |||
| High | High | High | High | Very high | 0.0710 | |||
| Very high | Very high | Slightly high | High | High | 0.0710 | |||
| High | High | Slightly high | Slightly high | Slightly high | 0.0452 | |||
| Slightly high | Slightly high | Slightly high | Slightly high | High | 0.0387 | |||
| High | High | Very high | Very high | High | 0.0774 |
| 0 | –11.7793 | –22.1894 | –14.9051 | |
| –26.9445 | 0 | –29.9559 | –25.5474 | |
| –12.7369 | –7.41886 | 0 | −9.90802 | |
| −20.3134 | −12.4199 | −26.099 | 0 |
| Ranking Results | The Optimal Alternative | The Worst Alternative | |
|---|---|---|---|
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, W.; Luo, S.; Zhao, G. Evaluation of Cleaner Production for Gold Mines Employing a Hybrid Multi-Criteria Decision Making Approach. Sustainability 2019, 11, 146. https://doi.org/10.3390/su11010146
Liang W, Luo S, Zhao G. Evaluation of Cleaner Production for Gold Mines Employing a Hybrid Multi-Criteria Decision Making Approach. Sustainability. 2019; 11(1):146. https://doi.org/10.3390/su11010146
Chicago/Turabian StyleLiang, Weizhang, Suizhi Luo, and Guoyan Zhao. 2019. "Evaluation of Cleaner Production for Gold Mines Employing a Hybrid Multi-Criteria Decision Making Approach" Sustainability 11, no. 1: 146. https://doi.org/10.3390/su11010146
APA StyleLiang, W., Luo, S., & Zhao, G. (2019). Evaluation of Cleaner Production for Gold Mines Employing a Hybrid Multi-Criteria Decision Making Approach. Sustainability, 11(1), 146. https://doi.org/10.3390/su11010146






