Study on Adaptive-Passive and Semi-Active Eddy Current Tuned Mass Damper with Variable Damping
Abstract
1. Introduction
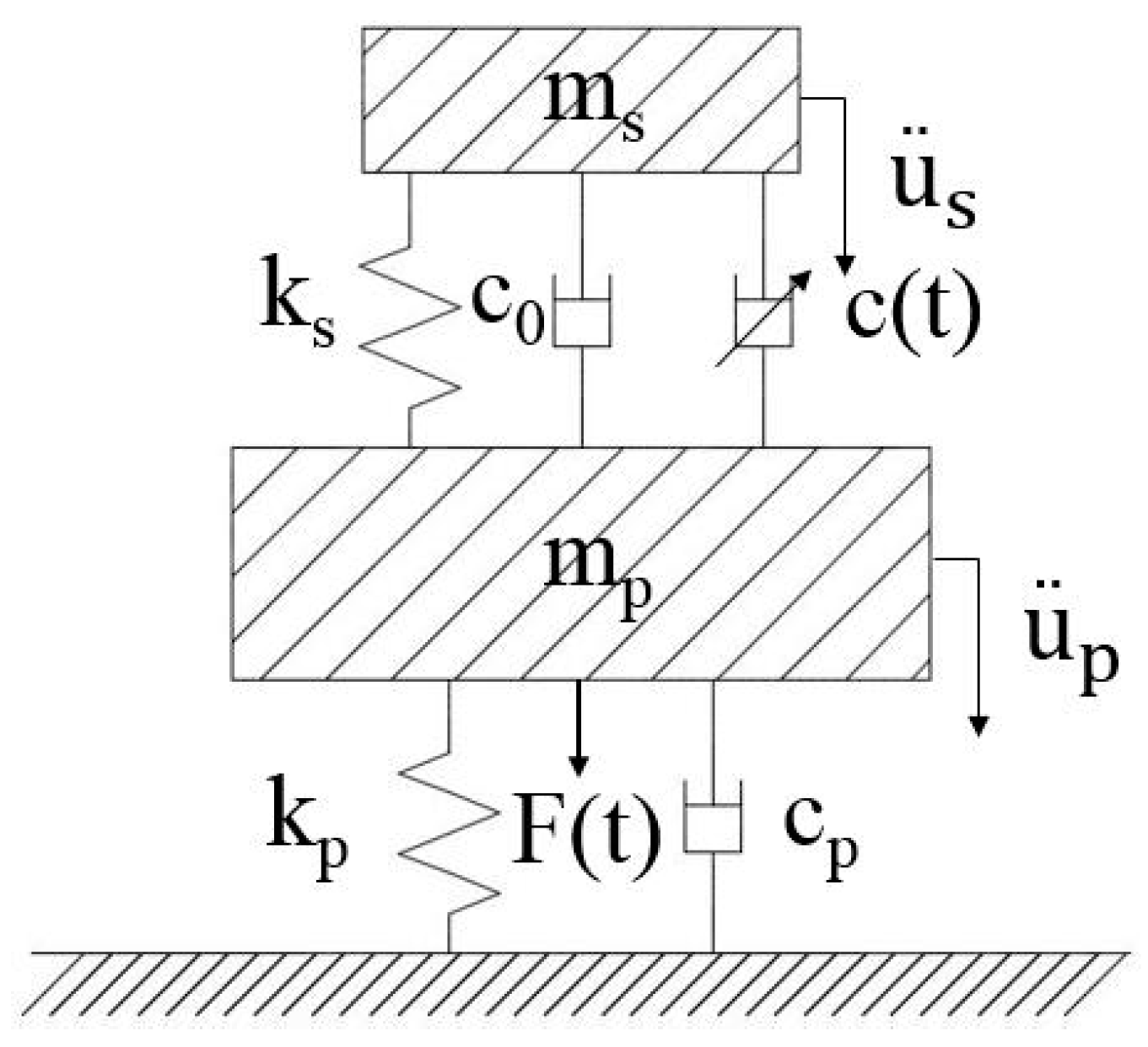
2. Mechanical Analysis of Adaptive-Passive ECTMD
2.1. Mechanical Model of Adaptive-Passive ECTMD
2.2. Finite Element Model of Adaptive-Passive ECTMD
3. Parametric Study
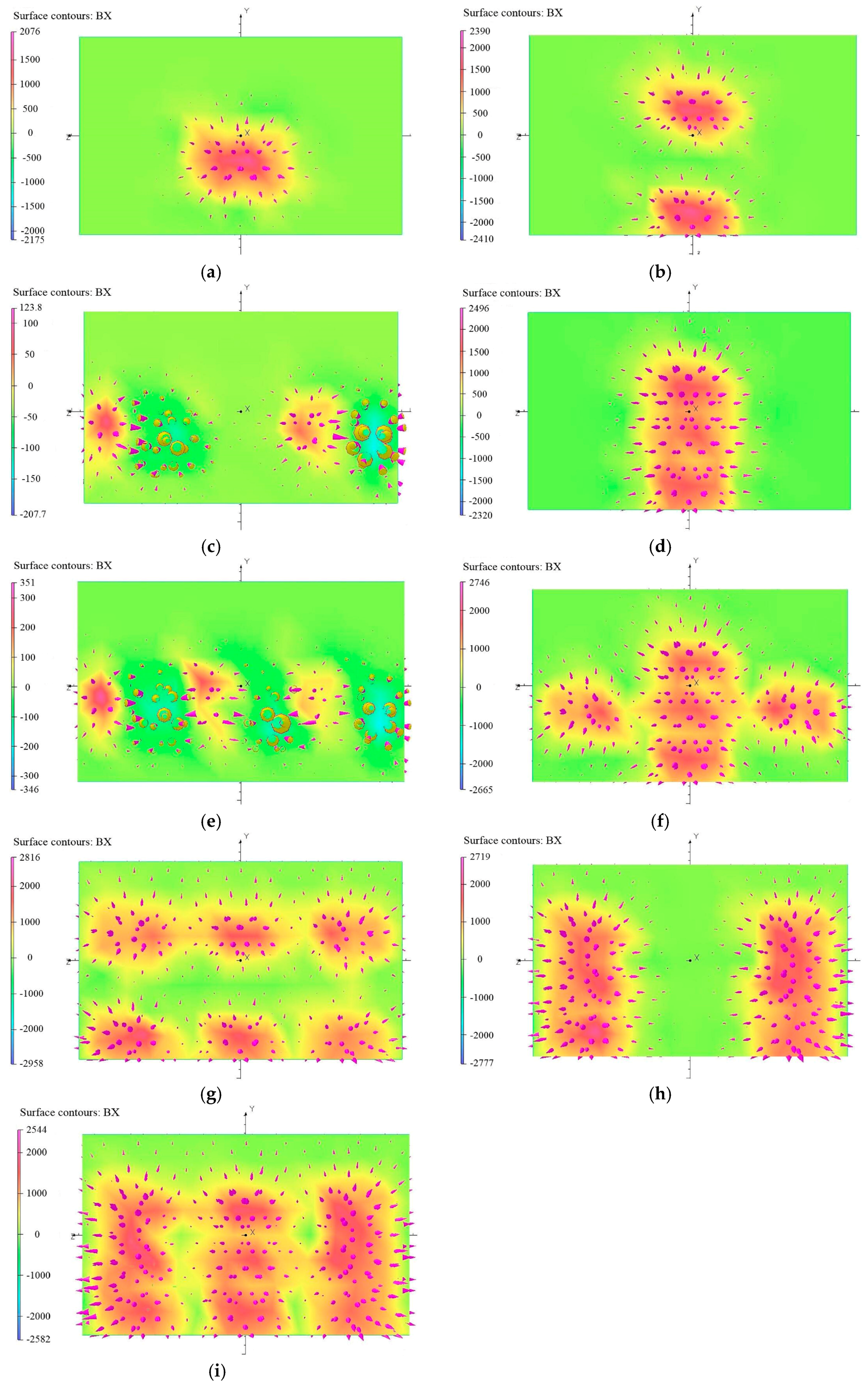
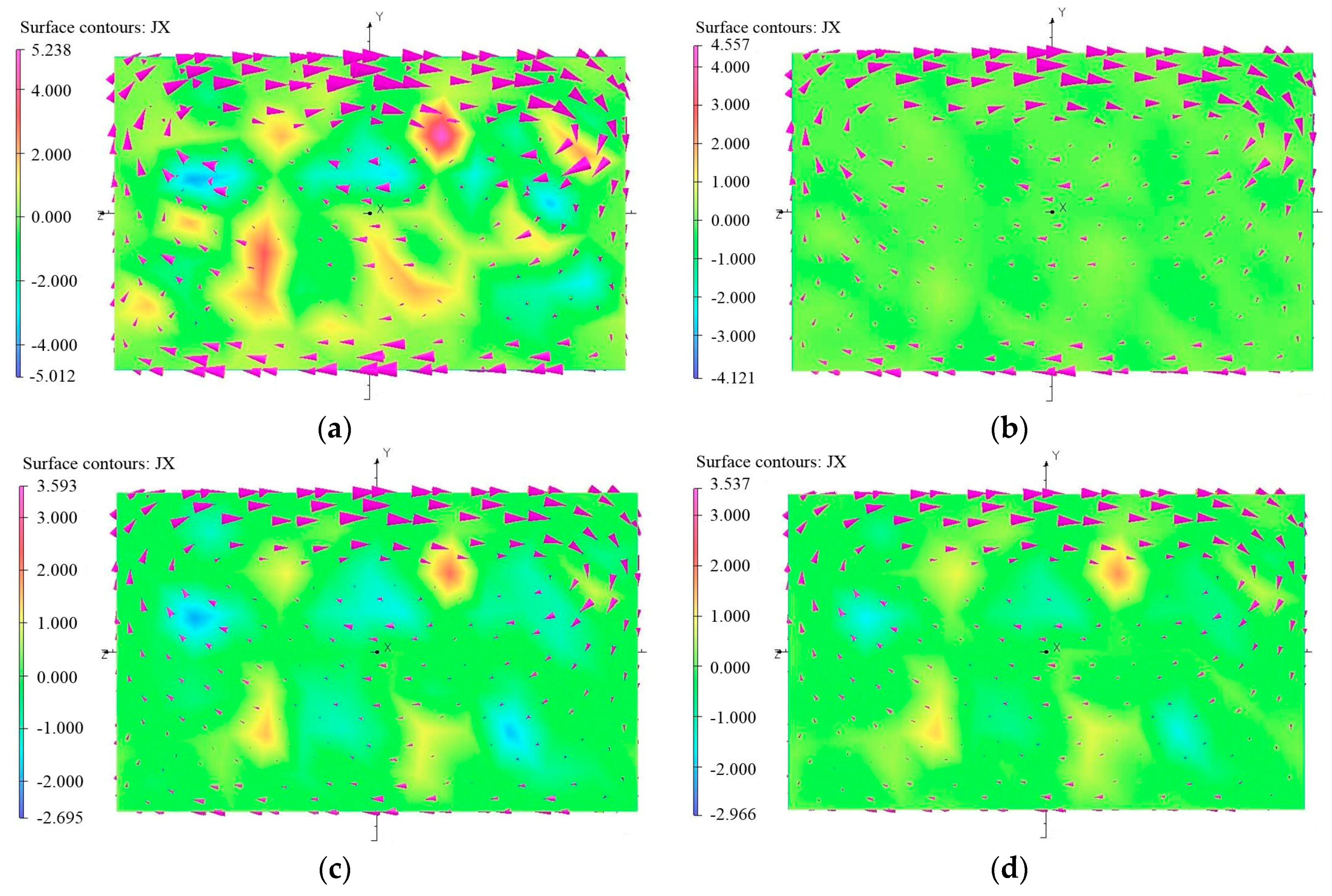
3.1. Effect of the Magnets’ Adsorption Position
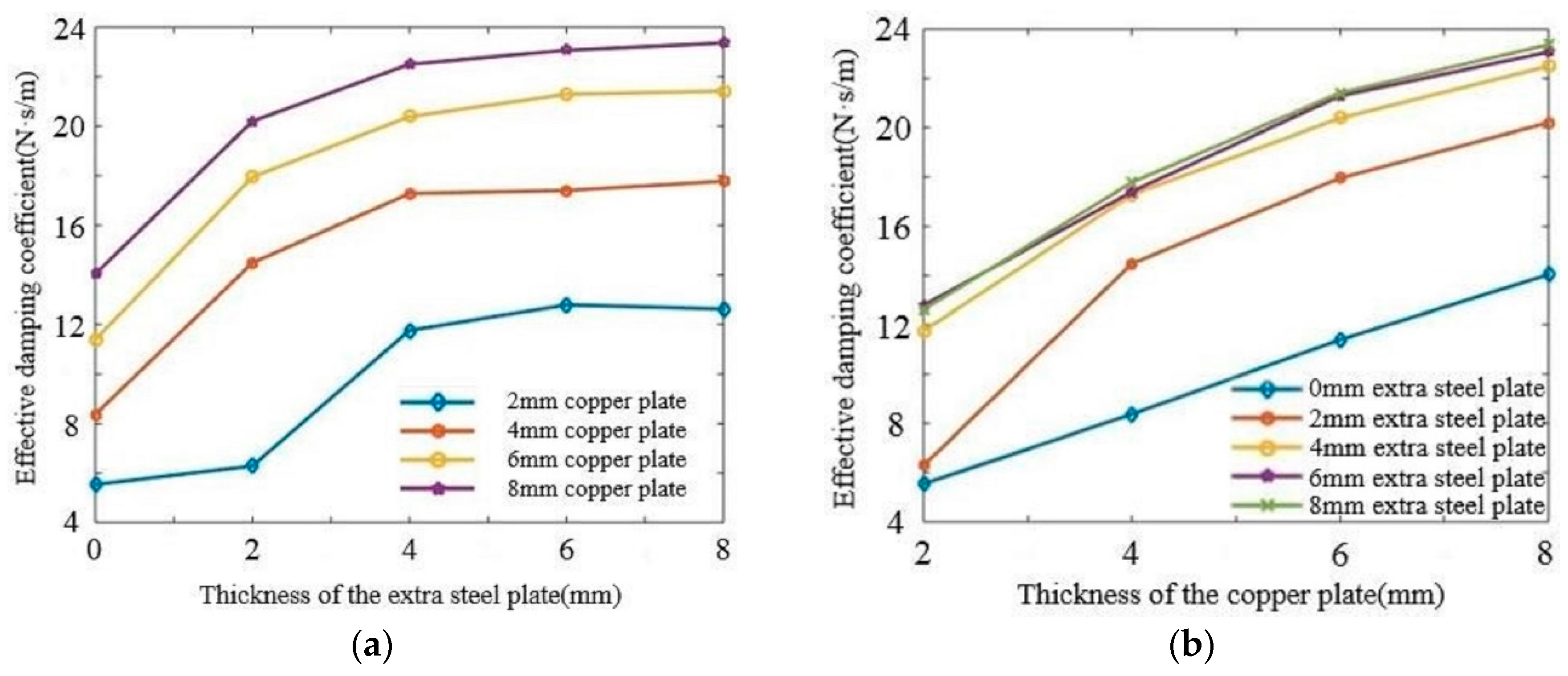
3.2. Effect of Thickness of Conductive Plates and Extra Steel Plates
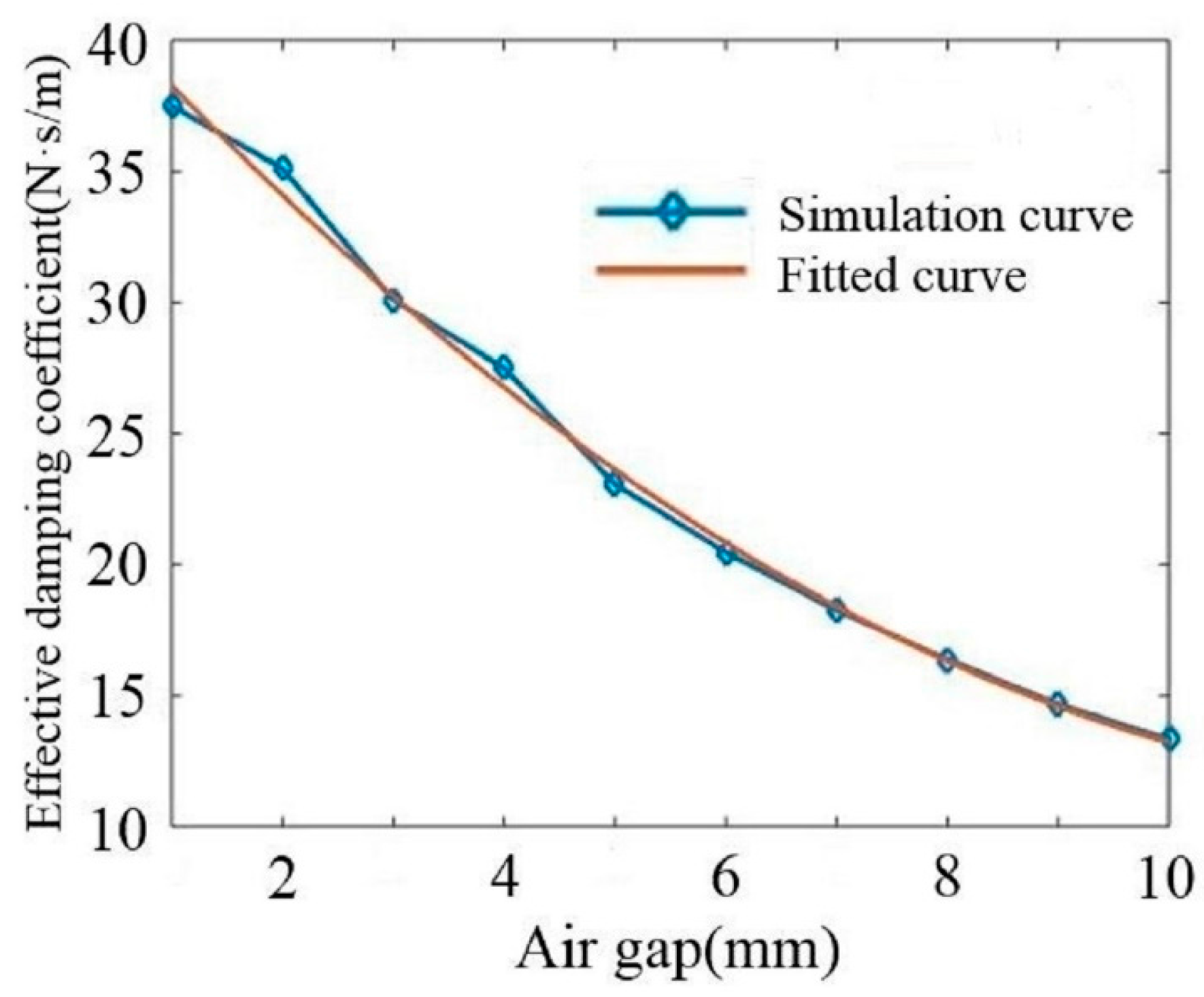
3.3. Effect of the Air Gap
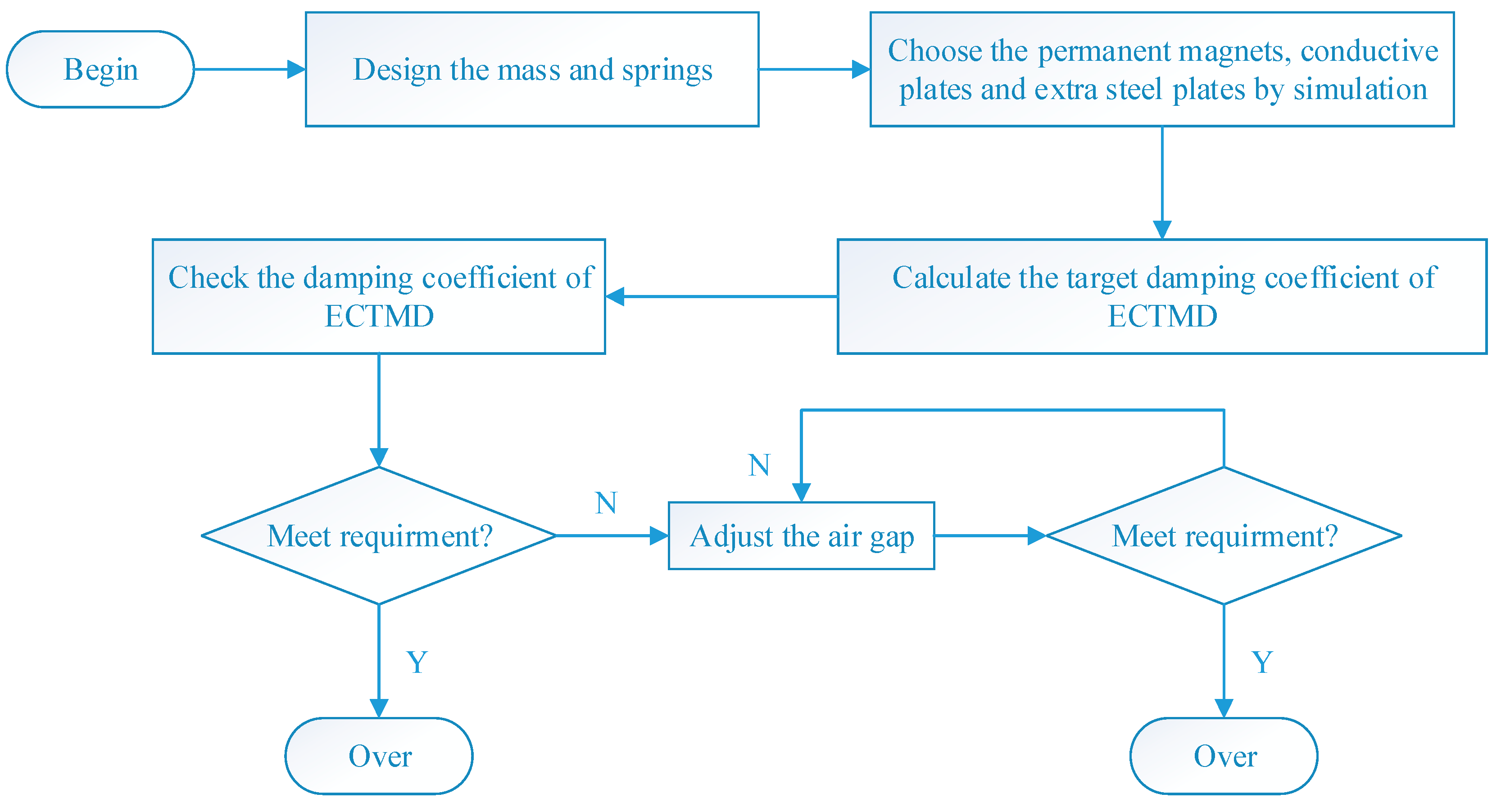
4. Design Method of Adaptive-Passive ECTMD
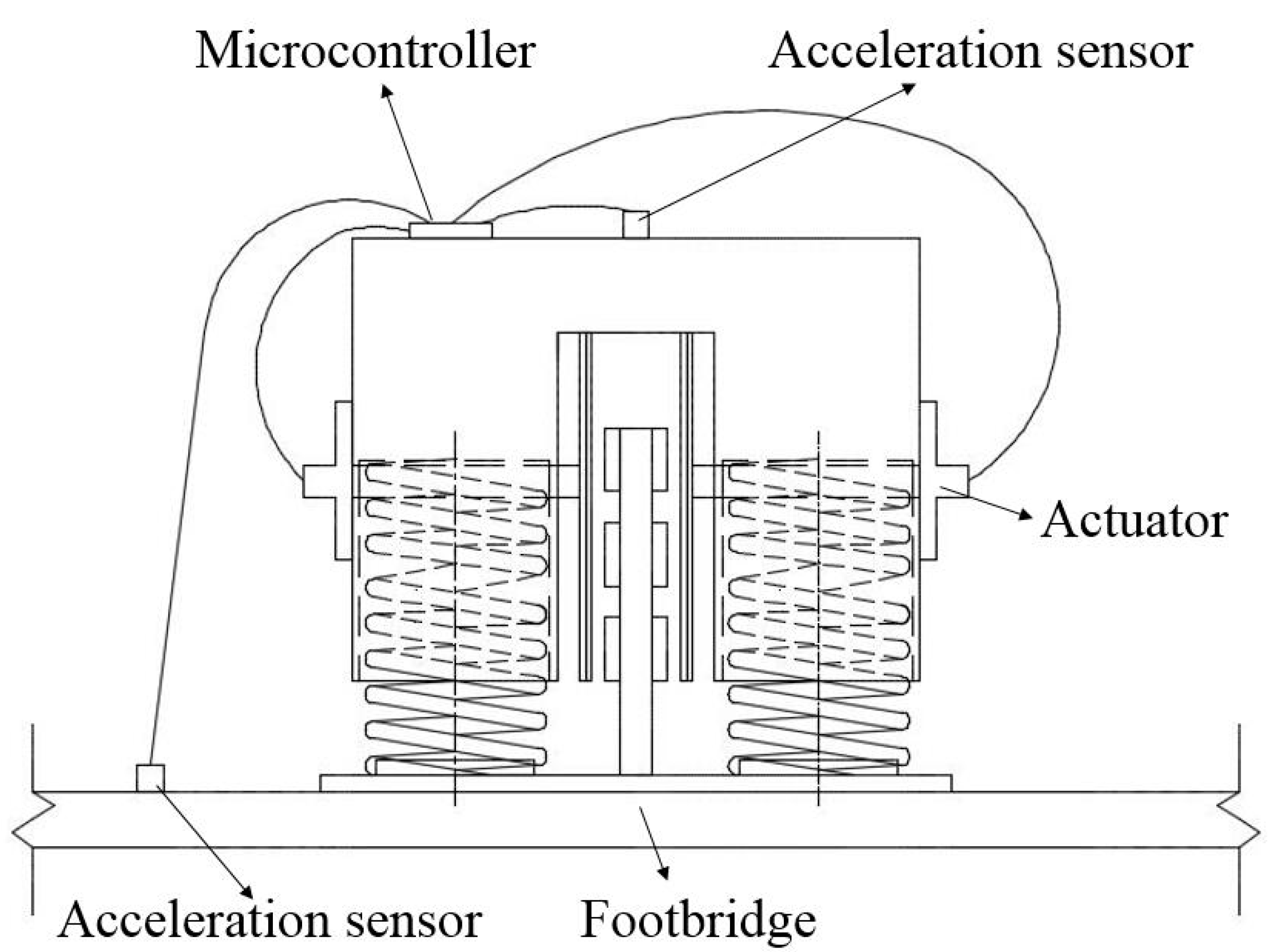
5. Semi-Active ECTMD with Variable Damping Element
5.1. Control Algorithm
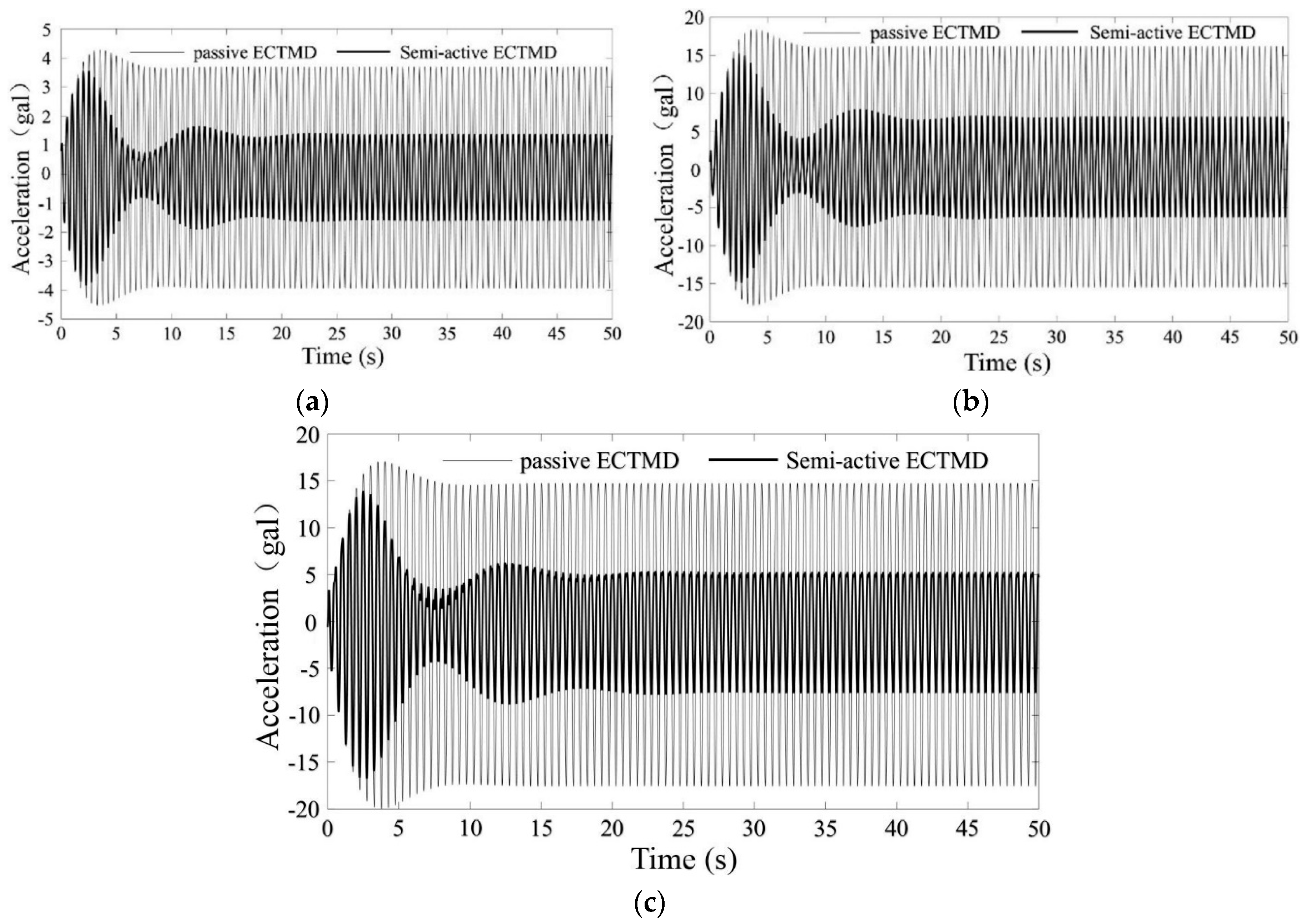
5.2. Case Study
6. Conclusions
- The proposed adaptive–passive ECTMD has the advantages of being friendly to the environment, suitable for assembly production, economical, free of additional stiffness, etc. The most important advantage is that the damping ratio can be adjusted very easily through adjusting the air gap between conductive plates and magnets. Therefore, it meets the sustainability requirement.
- Four important parameters, e.g., adsorption position of permanent magnets, thickness of the conductive plate, thickness of the extra steel plate and the air gap between permanent magnets and the conductive plate, influence the damping effects. However, the absorption position of permeant magnets has a relatively larger influence on the magnetic induction intensity.
- The proposed two new evaluation indexes (beneficial magnetic induction intensity and adverse magnetic induction intensity) are useful indications of the damping mechanism of the device. The effective damping coefficient as a function of the air gap and the fitting function can easily be obtained. With this fitting function, the damping ratio of the proposed ECTMD can be adjusted both passively and semi-actively easily through adjusting the air gap.
- The passive ECTMD can be conveniently upgraded to a semi-active one because the adjusting mechanism for air gap is very easy to be implemented. By using the LQG control algorithm, this semi-active ECTMD has much better control effects over the optimized passive one under harmonic and human-induced excitations. Both devices can be used in engineering projects based on the client’s demands.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Lu, Z.; Chen, X.; Zhang, D.; Dai, K. Experimental and analytical study on the performance of particle tuned mass dampers under seismic excitation. Earthq. Eng. Struct. Dyn. 2017, 46, 697–714. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, D.; Masri, S.F.; Lu, X. An experimental study of vibration control of wind-excited high-rise buildings using particle tuned mass dampers. Smart Struct. Syst. 2016, 18, 93–115. [Google Scholar] [CrossRef]
- Dai, K.; Wang, J.; Mao, R.; Lu, Z.; Chen, S. Experimental investigation on dynamic characterization and seismic control performance of a TLPD system. Struct. Des. Tall Spec. Build. 2017, 26, e1350. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, Z.; Masri, S.F.; Lu, X. Particle impact dampers: Past, Present, and Future. Struct. Control Health Monit. 2018, 25, e2508. [Google Scholar] [CrossRef]
- Lu, Z.; Chen, X.; Zhou, Y. An equivalent method for optimization of particle tuned mass damper based on experimental parametric study. J. Sound Vib. 2017. [Google Scholar] [CrossRef]
- Lu, Z.; Huang, B.; Zhou, Y. Theoretical study and experimental validation on the energy dissipation mechanism of particle dampers. Struct. Control Health Monit. 2017. [Google Scholar] [CrossRef]
- Brownjohn, J.; Fok, P.; Roche, M.; Moyo, P. Long span steel pedestrian bridge at Singapore Changi Airport—Part 1: Prediction of vibration serviceability problems. Eng. Strict. 2004, 82, 21–27. [Google Scholar]
- Li, Q.; Fan, J.; Nie, J.; Li, Q.; Chen, Y. Crowd-induced random vibration of footbridge and vibration control using multiple tuned mass dampers. J. Sound Vib. 2010, 329, 4068–4092. [Google Scholar] [CrossRef]
- Domaneschi, M.; Martinelli, L. Refined optimal passive control of buffeting-induced wind loading of a suspension bridge. Wind Struct. Int. J. 2014, 18, 1–20. [Google Scholar] [CrossRef]
- Domaneschi, M.; Martinelli, L.; Po, E. Control of Wind Buffeting Vibrations in a Suspension Bridge by TMD: Hybridization and robustness issues. Comput. Struct. 2015, 155, 3–17. [Google Scholar] [CrossRef]
- Domaneschi, M.; Martinelli, L. Earthquake resilience-based control solutions for the extended benchmark cable-stayed bridge. J. Struct. Eng. 2016, 142, C4015009. [Google Scholar] [CrossRef]
- Domaneschi, M.; Perotti, F.; Martinelli, L. Wind and earthquake protection of cable-supported bridges. Bridg. Eng. 2016, 9307, 1–15. [Google Scholar] [CrossRef]
- Casciati, F.; Giuliano, F. Performance of multi-TMD in the towers of suspension bridges. J. Vib. Control 2009, 15, 821–847. [Google Scholar] [CrossRef]
- Wang, Z.; Chen, Z.; Wang, J. Feasibility study of a large-scale tuned mass damper with eddy current damping mechanism. Earthq. Eng. Eng. Vib. 2012, 11, 391–401. [Google Scholar] [CrossRef]
- Hu, Y.; Liu, L.; Rahimi, S. Seismic vibration control of 3D steel frames with irregular plans using eccentrically placed MR dampers. Sustainability 2017, 9, 1255. [Google Scholar] [CrossRef]
- Pluk, K.; Beek, T.; Jansen, J.; Lomonova, E. Modeling and measurements on a finite rectangular conducting plate in an eddy current damper. IEEE Trans. Ind. Electron. 2014, 61, 4061–4072. [Google Scholar] [CrossRef]
- Ebrahimi, B.; Khamesee, M.; Golnaraghi, M. Design and modeling of a magnetic shock absorber based on eddy current damping effect. J. Sound Vib. 2008, 315, 875–889. [Google Scholar] [CrossRef]
- Lu, X.; Zhang, Q.; Weng, D.; Zhou, Z.; Wang, S.; Mahin, S.; Ding, S.; Qian, F. Improving performance of a super tall building using a new eddy current tuned mass damper. Struct. Control Health Monit. 2017, 24, e1882. [Google Scholar] [CrossRef]
- Bourquin, F.; Caruso, G.; Peigney, M.; Siegert, D. Magnetically tuned mass dampers for optimal vibration damping of large structures. Smart Mater. Struct. 2014, 23, 85009. [Google Scholar] [CrossRef]
- Bae, J.; Kwak, M.; Inman, D. Vibration suppression of a cantilever beam using eddy current damper. J. Sound Vib. 2005, 284, 805–824. [Google Scholar] [CrossRef]
- Sodano, H.; Inman, D. Non-contact vibration control system employing an active eddy current damper. J. Sound Vib. 2007, 305, 596–613. [Google Scholar] [CrossRef]
- Sodano, H.; Inman, D. Modeling of a new active eddy current vibration control system. J. Dyn. Syst. Meas. Control 2008, 130, 21009. [Google Scholar] [CrossRef]
- Sodano, H.; Bae, J.; Inman, D.; Belvin, W. Concept and model of eddy current damper for vibration suppression of a beam. J. Sound Vib. 2005, 288, 1177–1196. [Google Scholar] [CrossRef]
- Amjadian, M.; Agrawal, A. A passive electromagnetic eddy current friction damper (PEMECFD): Theoretical and analytical modeling. Struct. Control Health Monit. 2017, 24, e1978. [Google Scholar] [CrossRef]
- Maddah, A.; Hojjat, Y.; Karafi, M.; Ashory, M. Reduction of magneto rheological dampers stiffness by incorporating of an eddy current damper. J. Sound Vib. 2017, 396, 51–68. [Google Scholar] [CrossRef]
- Chen, J.; Lu, G.; Li, Y.; Wang, T.; Wang, W.; Song, G. Experimental study on robustness of an eddy current-tuned mass damper. Appl. Sci. 2017, 7, 895. [Google Scholar] [CrossRef]
- Wang, W.; Dalton, D.; Hua, X.; Wang, X.; Chen, Z.; Song, G. Experimental study on vibration control of a submerged pipeline model by eddy current tuned mass damper. Appl. Sci. 2017, 7, 987. [Google Scholar] [CrossRef]
- Eason, R.P.; Sun, C.; Dick, A.J.; Nagarajaiah, S. Attenuation of a linear oscillator using a nonlinear and a semi-active tuned mass damper in series. J. Sound Vib. 2013, 332, 154–166. [Google Scholar] [CrossRef]
- Sun, C.; Nagarajaiah, S.; Dick, A.J. Family of smart tuned mass damper with variable frequency under harmonic excitation and ground motions: Closed-form evaluation. Smart Struct. Syst. 2014, 13, 319–341. [Google Scholar] [CrossRef]
- Li, C.; Cao, B. Hybrid active tuned mass dampers for structures under the ground acceleration. Struct. Control Health Monit. 2015, 22, 757–773. [Google Scholar] [CrossRef]
- Lin, G.; Lin, C.; Lu, L.Y.; Ho, Y. Experimental verification of seismic vibration control using semi-active friction tuned mass damper. Earthq. Eng. Struct. Dyn. 2012, 41, 813–830. [Google Scholar] [CrossRef]
- Nagarajaiah, S. Adaptive passive, semi-active, smart tuned mass dampers: Identification and control using empirical mode decomposition, Hilbert transform, and short-term Fourier transform. Struct. Control Health Monit. 2009, 16, 800–841. [Google Scholar] [CrossRef]
- Nagarajaiah, S.; Varadarajan, N. Short time Fourier transform algorithm for wind response control of buildings with variable stiffness TMD. Eng. Struct. 2005, 27, 431–441. [Google Scholar] [CrossRef]
- Lin, P.Y.; Chung, L.L. Semi-active control of building structures with semi-active tuned mass damper. Comput. Aided Civ. Infrastruct. Eng. 2005, 20, 35–51. [Google Scholar] [CrossRef]
- Sun, C.; Nagarajaiah, S. Study on semi-active tuned mass damper with variable damping and stiffness under seismic excitations. Struct. Control Health Monit. 2014, 21, 890–906. [Google Scholar] [CrossRef]
- Lin, G.; Lin, C.; Chen, B.; Soong, T. Vibration control performance of tuned mass dampers with resettable variable stiffness. Eng. Struct. 2015, 83, 187–197. [Google Scholar] [CrossRef]
- Emiliano, M.; Alessandro, D. Robust design of mass-uncertain rolling-pendulum TMDs for the seismic protection of buildings. Mech. Syst. Signal Process. 2009, 23, 127–147. [Google Scholar]
- Chang, M.; Lin, C.; Ueng, J.; Hsieh, K.; Wang, J. Experimental study on adjustable tuned mass damper to reduce floor vibrations due to machinery. Struct. Control Health Monit. 2013, 21, 890–906. [Google Scholar] [CrossRef]
- Berardengo, M.; Cigada, A.; Guanziroli, F.; Manzoni, S. Modelling and control of an adaptive tuned mass damper based on shape memory alloys and eddy currents. J. Sound Vib. 2015, 349, 18–38. [Google Scholar] [CrossRef]
- Rizos, D.; Feltrin, G.; Motavalli, M. Structural identification of a prototype pre-stressable leaf-spring based adaptive tuned mass damper: Nonlinear characterization and classification. Mech. Syst. Signal Process. 2011, 25, 205–221. [Google Scholar] [CrossRef]
- Pietrosanti, D.; Angelis, M.; Basili, M. Optimal design and performance evaluation of systems with tuned mass damper inerter (TMDI). Earthq. Eng. Struct. Dyn. 2017, 46, 1367–1388. [Google Scholar] [CrossRef]
- Brzeski, P.; Perlikowski, P. Effects of play and inerter nonlinearities on the performance of tuned mass damper. Nonlinear Dyn. 2017, 88, 1027–1041. [Google Scholar] [CrossRef]
- Marian, L.; Giaralis, A. Optimal design of a novel tuned mass-damper-inerter (TMDI) passive vibration control configuration for stochastically support-excited structural systems. Probab. Eng. Mech. 2014, 38, 156–164. [Google Scholar] [CrossRef]
- Giaralis, A.; Petrini, F. Wind-induced vibration mitigation in tall buildings using the tuned mass-damper-inerter. J. Struct. Eng. 2017, 143, 4017127. [Google Scholar] [CrossRef]
- Lazar, I.; Neild, S.; Wagg, D. Vibration suppression of cables using tuned inerter dampers. Eng. Struct. 2016, 122, 62–71. [Google Scholar] [CrossRef]
- OPERA-3D User Guide; QUENCH Code; Cobham Technical Services, Vector Fields Limited: Kidlington, UK, 2013.
- Lu, Z.; Masri, S.; Lu, X. Studies of the performance of particle dampers attached to a two-degree-of-freedom system under random excitation. J. Vib. Control 2011, 17, 1454–1471. [Google Scholar]
- Ni, Y.; Chen, Y.; Ko, J.; Cao, D. Neuro-control of cable vibration using semi-active magneto-rheological dampers. Eng. Struct. 2002, 24, 295–307. [Google Scholar] [CrossRef]
- Zhu, W.; Luo, M.; Dong, L. Semi-active control of wind excited building structures using MR/ER dampers. Probab. Eng. Mech. 2004, 19, 279–285. [Google Scholar] [CrossRef]
- Montazeri, A.; Poshtan, J.; Choobdar, A. Performance and robust stability trade-off in minimax LQG control of vibrations in flexible structures. Eng. Struct. 2009, 31, 2407–2413. [Google Scholar] [CrossRef]
- Dong, X.; Yu, M.; Liao, C.; Chen, W. Comparative research on semi-active control strategies for magneto-rheological suspension. Nonlinear Dyn. 2010, 59, 433–453. [Google Scholar] [CrossRef]
- Gu, Z.; Oyadiji, O. Application of MR damper in structural control using ANFIS method. Comput. Struct. 2008, 86, 427–436. [Google Scholar] [CrossRef]
- Ozbulut, O.; Bitaraf, M.; Hurlebaus, S. Adaptive control of base-isolated structures against near-field earthquakes using variable friction dampers. Eng. Struct. 2011, 33, 3143–3154. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, D.; Zhou, Y. Experimental parametric study on wind-induced vibration control of particle tuned mass damper on a benchmark high-rise building. Struct. Des. Tall Spec. Build. 2017, 26, e1359. [Google Scholar] [CrossRef]
- Casciati, F.; Casciati, S.; Faravelli, L. A contribution to the modelling of human induced excitation on pedestrian bridges. Struct. Saf. 2017, 66, 51–61. [Google Scholar] [CrossRef]
- Zivanovic, S.; Pavic, A.; Reynolds, P. Vibration serviceability of footbridges under human-induced excitation: A literature review. J. Sound Vib. 2005, 279, 1–74. [Google Scholar] [CrossRef]
- Díaz, I.; Reynolds, P. Acceleration feedback control of human-induced floor vibrations. Eng. Struct. 2010, 32, 163–173. [Google Scholar] [CrossRef]
- Lu, X.; Ding, K.; Shi, W.; Weng, D. Tuned mass dampers for human-induced vibration control of the Expo Culture Centre at the World Expo 2010 in Shanghai, China. Struct. Eng. Mech. 2012, 43, 607–621. [Google Scholar] [CrossRef]
- Lu, Z.; Chen, X.; Li, X.; Li, P. Optimization and application of multiple tuned mass dampers in the vibration control of pedestrian bridges. Struct. Eng. Mech. 2017, 62, 55–64. [Google Scholar] [CrossRef]
- Occhiuzzi, A.; Spizzuoco, M.; Ricciardelli, F. Loading models and response control of footbridges excited by running pedestrians. Struct. Control Health Monit. 2010, 15, 349–368. [Google Scholar] [CrossRef]
- Racic, V.; Brownjohn, J.M.W.; Pavic, A. Reproduction and application of human bouncing and jumping forces from visual marker data. J. Sound Vib. 2010, 239, 3397–3416. [Google Scholar] [CrossRef]
- Shi, W.; Wang, L.; Lu, Z. Study on self-adjustable tuned mass damper with variable mass. Struct. Control Health Monit. 2017. [Google Scholar] [CrossRef]

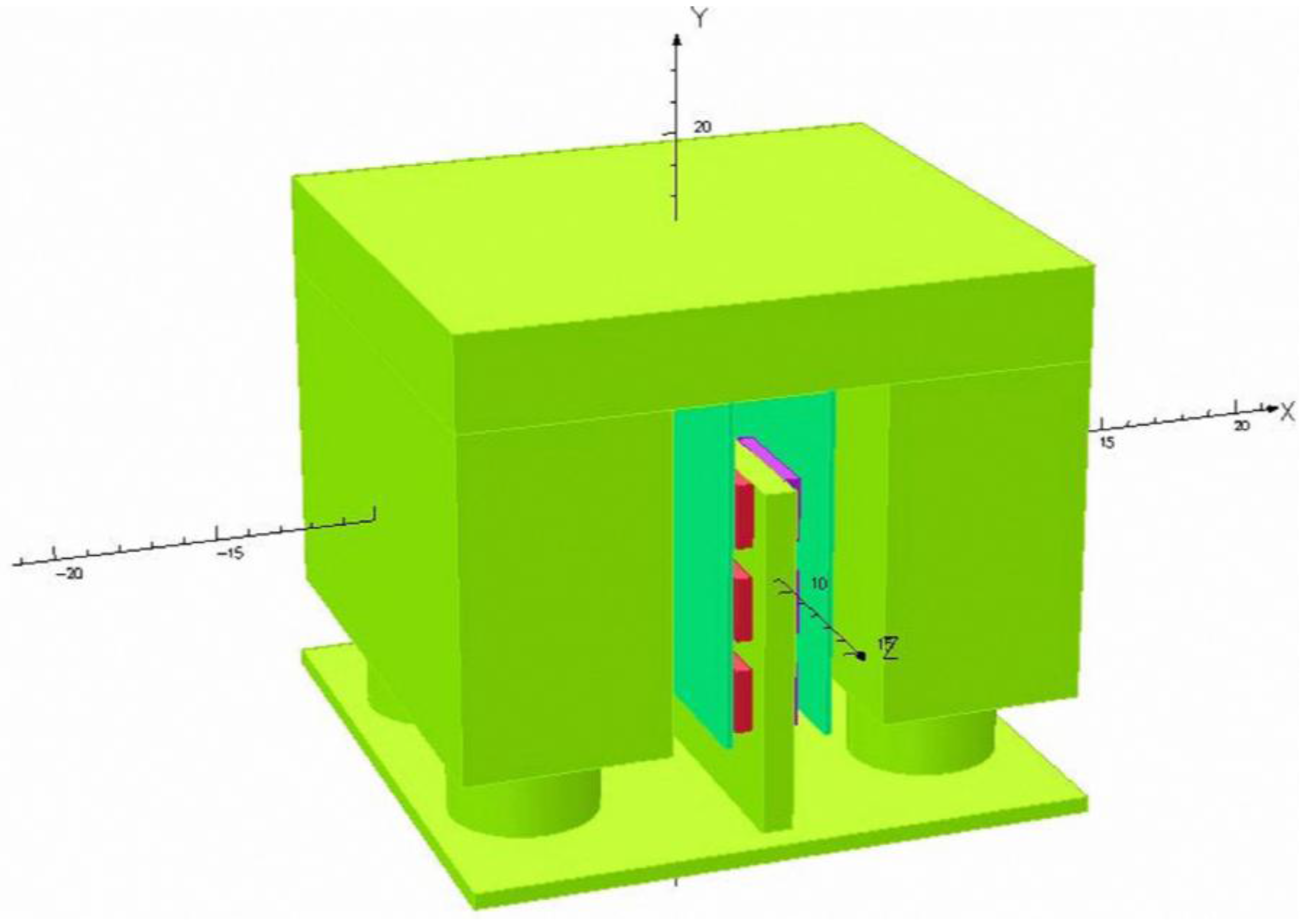
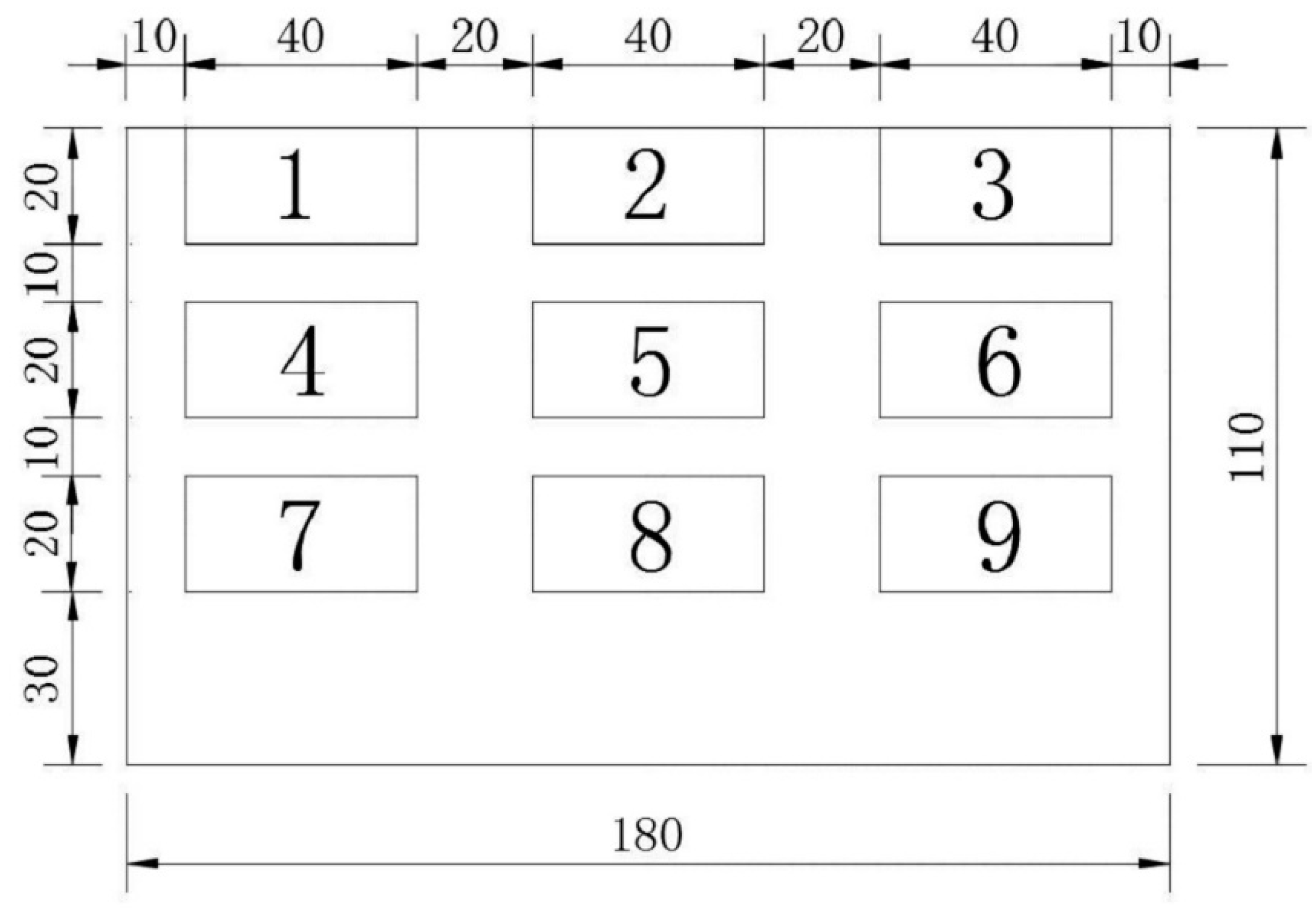

| Case | Position |
|---|---|
| 1 | 5 |
| 2 | 2, 8 |
| 3 | 4, 6 |
| 4 | 2, 5, 8 |
| 5 | 4, 5, 6 |
| 6 | 2, 4, 5, 6,8 |
| 7 | 1, 2, 3, 7, 8, 9 |
| 8 | 1, 4, 7, 3, 6, 9 |
| 9 | 1, 2, 3, 4, 5, 6, 7, 8, 9 |
| Case | Minimum/Gauss | Maximum/Gauss | Surface Integral/Gauss·cm2 | Average/Gauss |
|---|---|---|---|---|
| 1 | −234.2 | 1852.6 | 17,631.6 | 89.048 |
| 2 | −118.5 | 1952.1 | 34,816.4 | 175.840 |
| 3 | −207.7 | 123.8 | −920.8 | −4.651 |
| 4 | −224.3 | 1999.1 | 52,860.2 | 266.971 |
| 5 | −195.4 | 136.5 | −1779.9 | −8.989 |
| 6 | −525.5 | 2266.9 | 84,455.9 | 426.545 |
| 7 | −342.6 | 20,004.6 | 92,551.0 | 467.429 |
| 8 | −843.5 | 2104.2 | 92,216.6 | 465.740 |
| 9 | −971.2 | 2019.0 | 132,199.2 | 667.673 |
| Case | Thickness of Copper Plate/mm | Thickness of Extra Steel Plates/mm | Lorentz Force/N | Instantaneous Eddy Power/×103 W | Total Damping Coefficient /(N·s/m) | Effective Damping Coefficient /(N·s/m) | Damping Loss Coefficient /% |
|---|---|---|---|---|---|---|---|
| 1 | 2 | 0 | 0.127 | 2.216 | 6.350 | 5.540 | 12.756 |
| 2 | 2 | 2 | 0.245 | 2.515 | 12.250 | 6.288 | 48.673 |
| 3 | 2 | 4 | 0.280 | 4.702 | 14.000 | 11.755 | 16.036 |
| 4 | 2 | 6 | 0.302 | 5.116 | 15.100 | 12.790 | 15.298 |
| 5 | 2 | 8 | 0.299 | 5.050 | 14.950 | 12.625 | 15.552 |
| 6 | 4 | 0 | 0.194 | 3.348 | 9.700 | 8.370 | 13.711 |
| 7 | 4 | 2 | 0.337 | 5.794 | 16.850 | 14.485 | 14.036 |
| 8 | 4 | 4 | 0.395 | 6.913 | 19.750 | 17.283 | 12.494 |
| 9 | 4 | 6 | 0.396 | 6.962 | 19.800 | 17.405 | 12.096 |
| 10 | 4 | 8 | 0.405 | 7.111 | 20.250 | 17.778 | 12.209 |
| 11 | 6 | 0 | 0.260 | 4.556 | 13.000 | 11.390 | 12.385 |
| 12 | 6 | 2 | 0.409 | 7.181 | 20.450 | 17.953 | 12.213 |
| 13 | 6 | 4 | 0.459 | 8.155 | 22.950 | 20.388 | 11.166 |
| 14 | 6 | 6 | 0.478 | 8.517 | 23.900 | 21.293 | 10.910 |
| 15 | 6 | 8 | 0.481 | 8.567 | 24.050 | 21.418 | 10.946 |
| 16 | 8 | 0 | 0.314 | 5.616 | 15.700 | 14.040 | 10.573 |
| 17 | 8 | 2 | 0.451 | 8.082 | 22.550 | 20.205 | 10.400 |
| 18 | 8 | 4 | 0.498 | 9.001 | 24.900 | 22.503 | 9.629 |
| 19 | 8 | 6 | 0.509 | 9.227 | 25.450 | 23.068 | 9.361 |
| 20 | 8 | 8 | 0.516 | 9.344 | 25.800 | 23.360 | 9.457 |
| Case | Air Gap/mm | Effective Damping Coefficient /(N·s/m) |
|---|---|---|
| 1 | 1 | 37.500 |
| 2 | 2 | 35.120 |
| 3 | 3 | 30.110 |
| 4 | 4 | 27.500 |
| 5 | 5 | 23.068 |
| 6 | 6 | 20.478 |
| 7 | 7 | 18.260 |
| 8 | 8 | 16.383 |
| 9 | 9 | 14.668 |
| 10 | 10 | 13.325 |
| Walking | 0.4 | 0 | 0.1 | 1.57 | 0.1 | 1.57 |
| Running | 1.6 | 0 | 0.7 | 0.00 | 0.2 | 0.00 |
| Jumping | 1.7 | 0 | 1.1 | 1.73 | 0.5 | 1.73 |
| Simulation Conditions | Case | 1.8 Hz | 2.0 Hz | 2.2 Hz |
|---|---|---|---|---|
| Harmonic Excitations | Passive ECTMD/gal | 5.47 | 9.86 | 6.64 |
| Semi-active ECTMD/gal | 5.06 | 3.81 | 6.18 | |
| Reduction/% | 7.50 | 61.36 | 6.93 | |
| Walking excitations | Passive ECTMD/gal | 2.26 | 3.94 | 2.97 |
| Semi-active ECTMD/gal | 2.14 | 1.61 | 2.74 | |
| Reduction/% | 5.31 | 59.14 | 7.74 | |
| Running excitations | Passive ECTMD/gal | 8.88 | 16.20 | 11.17 |
| Semi-active ECTMD/gal | 8.37 | 6.91 | 10.53 | |
| Reduction/% | 5.74 | 57.35 | 5.73 | |
| Jumping excitations | Passive ECTMD/gal | 10.47 | 17.57 | 12.98 |
| Semi-active ECTMD/gal | 9.86 | 7.63 | 12.28 | |
| Reduction/% | 5.83 | 56.57 | 5.39 |
| Case | 1.0 Hz | 1.5 Hz | 2.0 Hz | 2.5 Hz | 3.0 Hz | |
|---|---|---|---|---|---|---|
| Without TMD/gal | 0.35 | 1.71 | 25.12 | 3.80 | 2.07 | |
| Passive ECTMD/gal | 0.34 | 1.36 | 9.86 | 3.10 | 1.92 | |
| Semi-active ECTMD/gal | 0.33 | 1.32 | 3.81 | 2.93 | 1.83 | |
| Reduction/% | Comparing to without TMD | 5.71 | 22.81 | 84.83 | 22.89 | 11.59 |
| Comparing to passive ECTMD | 2.94 | 2.94 | 61.36 | 5.48 | 4.69 | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, W.; Wang, L.; Lu, Z.; Gao, H. Study on Adaptive-Passive and Semi-Active Eddy Current Tuned Mass Damper with Variable Damping. Sustainability 2018, 10, 99. https://doi.org/10.3390/su10010099
Shi W, Wang L, Lu Z, Gao H. Study on Adaptive-Passive and Semi-Active Eddy Current Tuned Mass Damper with Variable Damping. Sustainability. 2018; 10(1):99. https://doi.org/10.3390/su10010099
Chicago/Turabian StyleShi, Weixing, Liangkun Wang, Zheng Lu, and Hui Gao. 2018. "Study on Adaptive-Passive and Semi-Active Eddy Current Tuned Mass Damper with Variable Damping" Sustainability 10, no. 1: 99. https://doi.org/10.3390/su10010099
APA StyleShi, W., Wang, L., Lu, Z., & Gao, H. (2018). Study on Adaptive-Passive and Semi-Active Eddy Current Tuned Mass Damper with Variable Damping. Sustainability, 10(1), 99. https://doi.org/10.3390/su10010099






