Modulation of Vestibular Microphonics: A Historical Note
Abstract
:1. Introduction
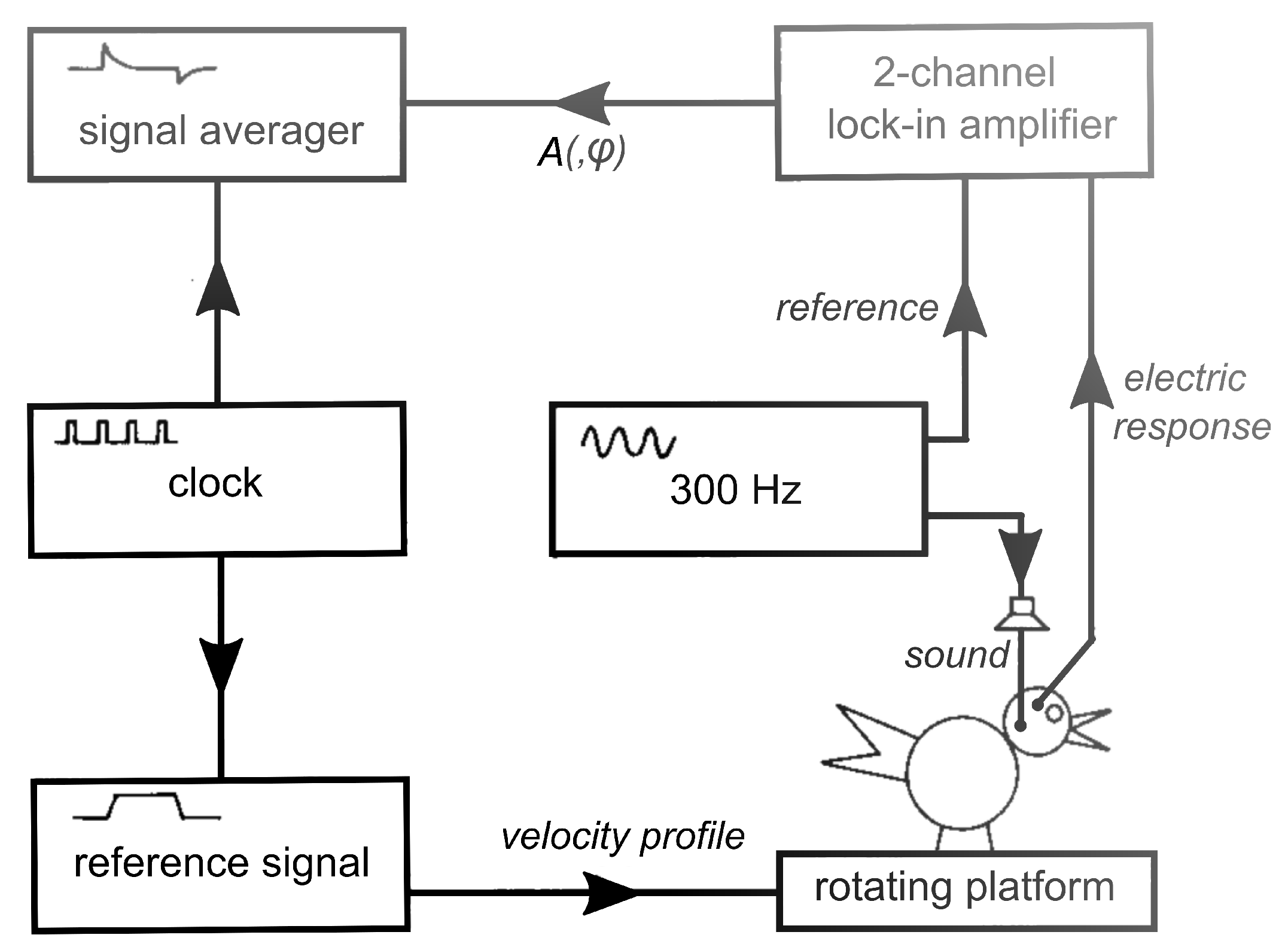
2. Materials and Methods
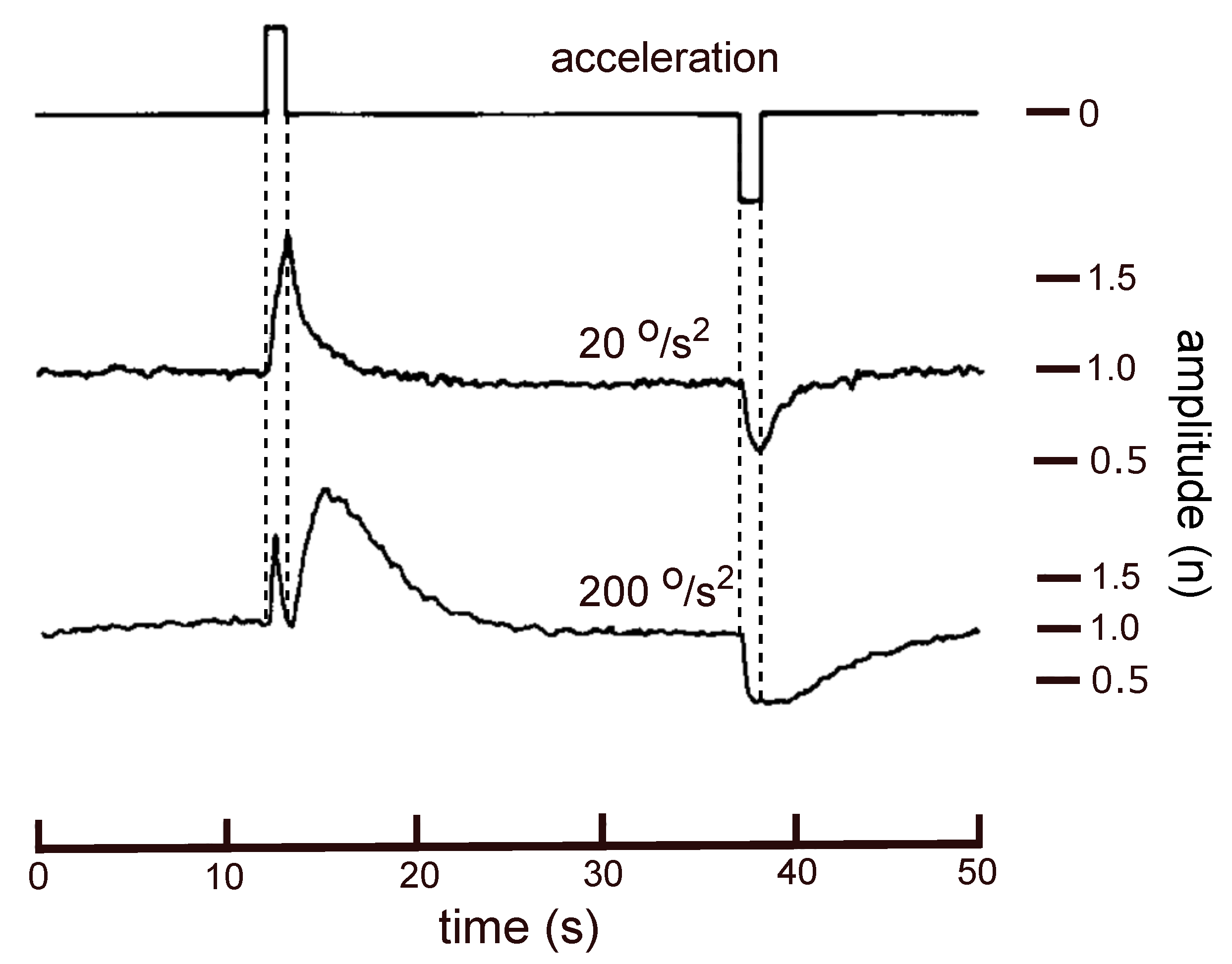
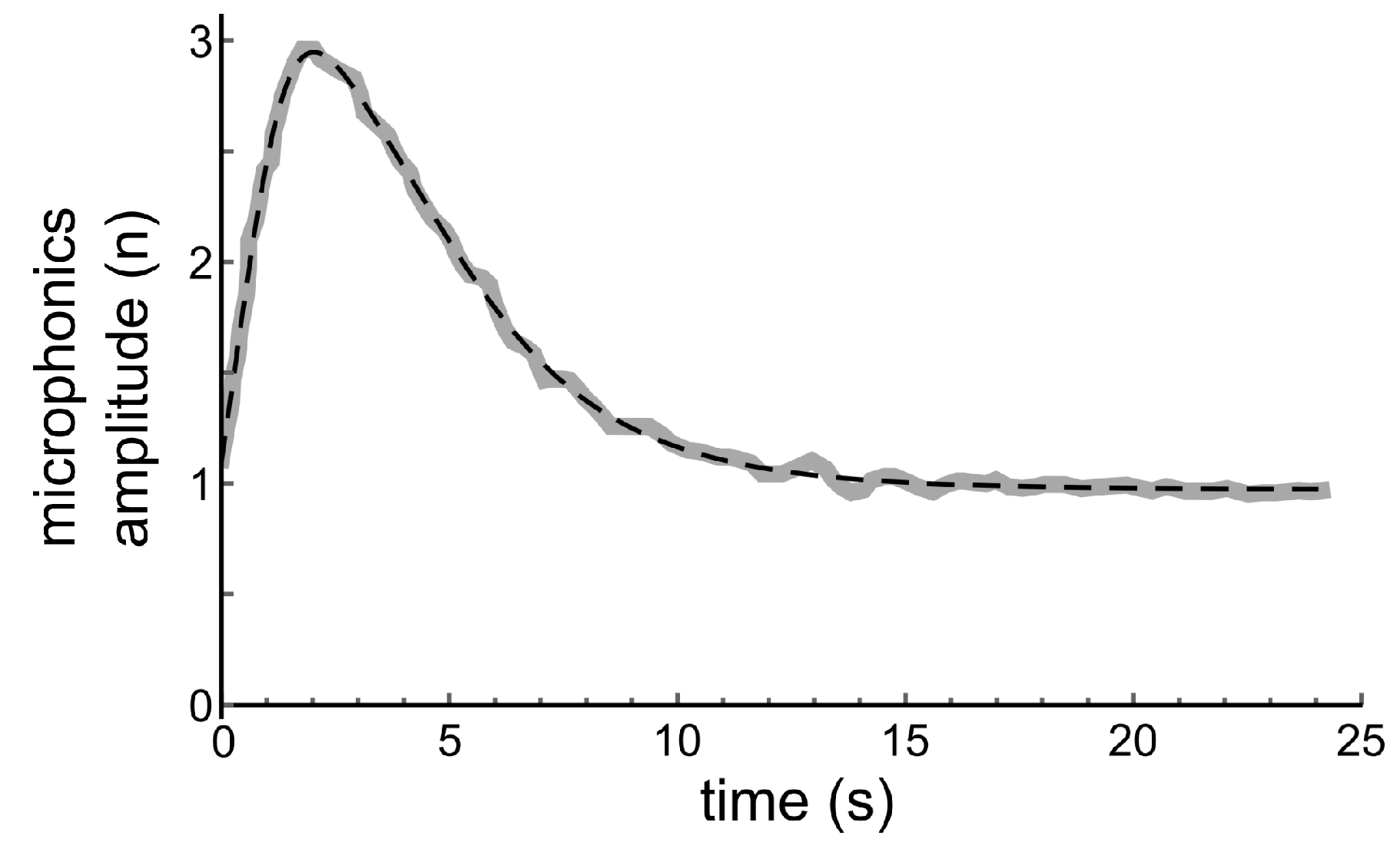
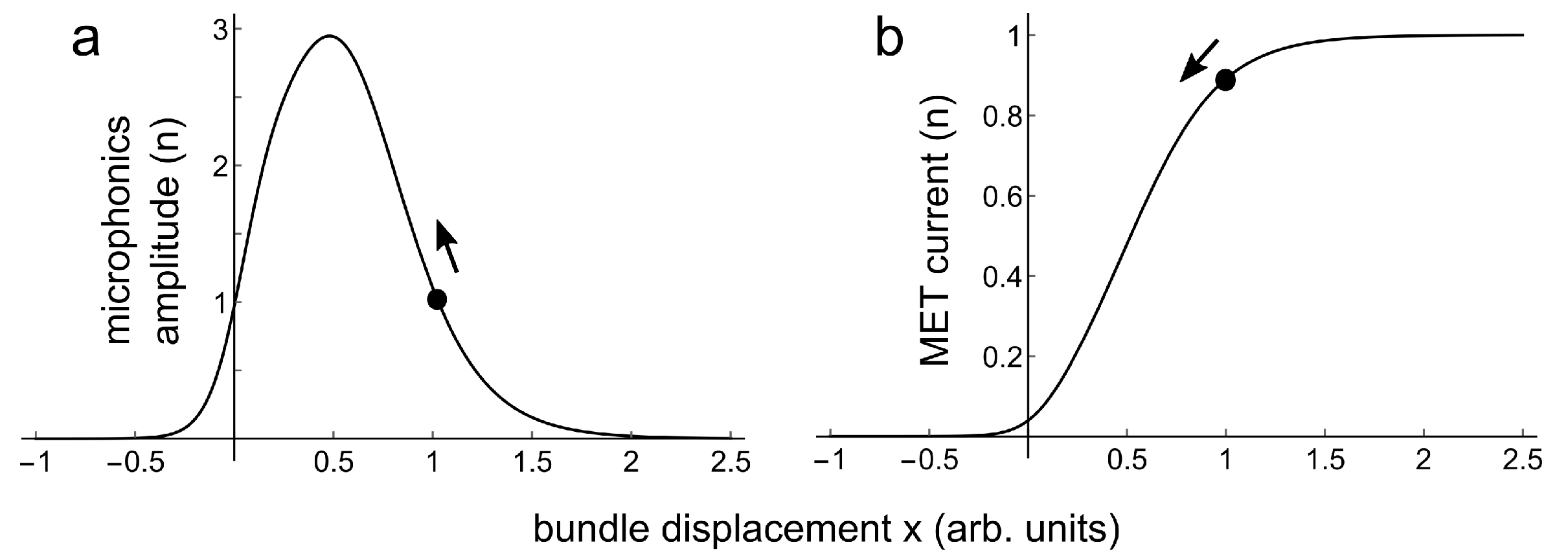
3. Analysis of a Result
4. Conclusions
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pastras, C.J.; Stefani, S.P.; Curthoys, I.S.; Camp, A.J.; Brown, D.J. Utricular sensitivity during hydrodynamic displacements of the macula. J. Ass. Res. Otolaryngol. 2020, 21, 409–423. [Google Scholar] [CrossRef] [PubMed]
- Wit, H.P.; Tideman, B.J.; Segenhout, J.M. Modulation of microphonics: A new method to study the vestibular system. Adv. Oto-Rhino-Laryng. (Karger Basel) 1988, 42, 59–64. [Google Scholar]
- De Vries, H.L.; Bleeker, D.J.W. The microphonic activity of the labyrinth of the pigeon: Part II: The response of the cristae in the semicircular canals. Acta Otolaryngol. 1949, 37, 298–306. [Google Scholar] [CrossRef] [PubMed]
- Wit, H.P.; Kahmann, H.F.; Segenhout, J.M. Vestibular microphonic potentials in pigeons. Arch. Oto-Rhino-Laryngol. 1986, 243, 146–150. [Google Scholar] [CrossRef] [PubMed]
- Brown, D.J.; Pastras, C.J.; Curthoys, I.S. Electrophysiological measurements of peripheral vestibular function—A review of electrovestibulography. Front. Syst. Neurosci. 2017, 11, 34. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wever, E.G.; Bray, C.W. Action currents in the auditory nerve in response to acoustic stimulation. Proc. Natl. Acad. Sci. USA 1930, 16, 344–350. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tullio, P. Das Ohr und Die Entstehung von Sprache und Schrift; Urban und Schwarzenegger: Berlin, Germany, 1929. [Google Scholar]
- Mikaelian, D. Vestibular response to sound: Single unit recording from the vestibular nerve in fenestrated deaf mice (Df/Df). Acta Oto-Laryngol. 1964, 58, 409–422. [Google Scholar] [CrossRef] [PubMed]
- Young, E.D.; Fernandez, C.; Goldberg, J.M. Responses of squirrel monkey vestibular neurons to audio-frequency sound and head vibration. Acta Oto-Laryngol. 1977, 84, 352–360. [Google Scholar] [CrossRef] [PubMed]
- Wit, H.P.; Bleeker, J.D.; Mulder, H.H. Responses of pigeon vestibular nerve fibers to sound and vibration with audiofrequencies. J. Acoust. Soc. Am. 1984, 75, 202–208. [Google Scholar] [CrossRef] [PubMed]
- Pastras, C.J.; Stefani, S.P.; Camp, A.J.; Curthoys, I.S.; Brown, D.J. Summating potentials from the utricular macula of anaesthesized guinea pigs. Hearing Res. 2021, 406, 108259. [Google Scholar] [CrossRef] [PubMed]
- Géléoc, G.S.G.; Lennan, G.W.T.; Richardson, G.P.; Kros, C.J. A quantitative comparison of mechanoelectrical transduction in vestibular and auditory hair cells of neonatal mice. Proc. R. Soc. Lond. 1997, 264, 611–621. [Google Scholar] [CrossRef] [PubMed] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wit, H.P. Modulation of Vestibular Microphonics: A Historical Note. Audiol. Res. 2021, 11, 384-388. https://doi.org/10.3390/audiolres11030036
Wit HP. Modulation of Vestibular Microphonics: A Historical Note. Audiology Research. 2021; 11(3):384-388. https://doi.org/10.3390/audiolres11030036
Chicago/Turabian StyleWit, Hero P. 2021. "Modulation of Vestibular Microphonics: A Historical Note" Audiology Research 11, no. 3: 384-388. https://doi.org/10.3390/audiolres11030036
APA StyleWit, H. P. (2021). Modulation of Vestibular Microphonics: A Historical Note. Audiology Research, 11(3), 384-388. https://doi.org/10.3390/audiolres11030036





