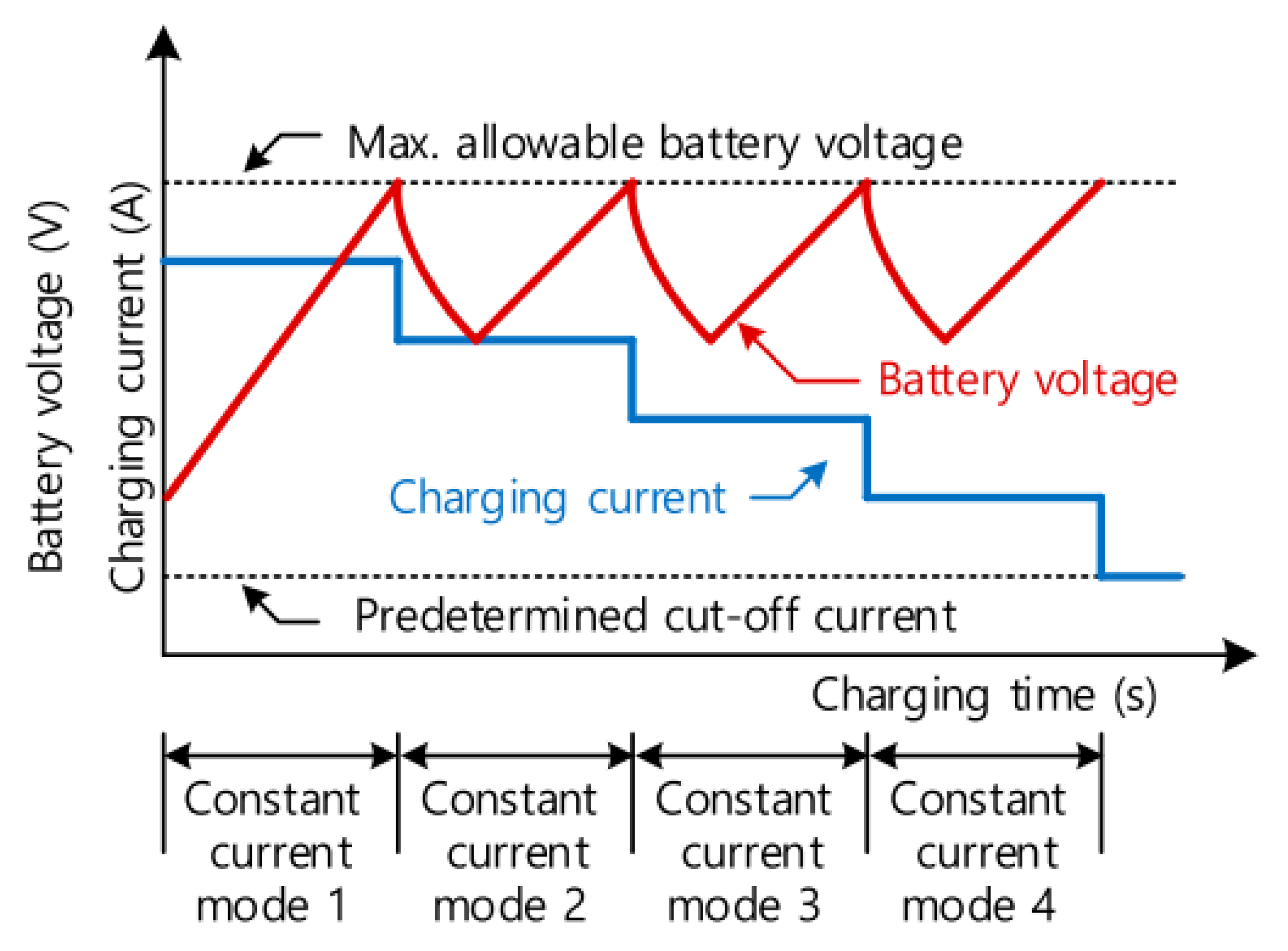
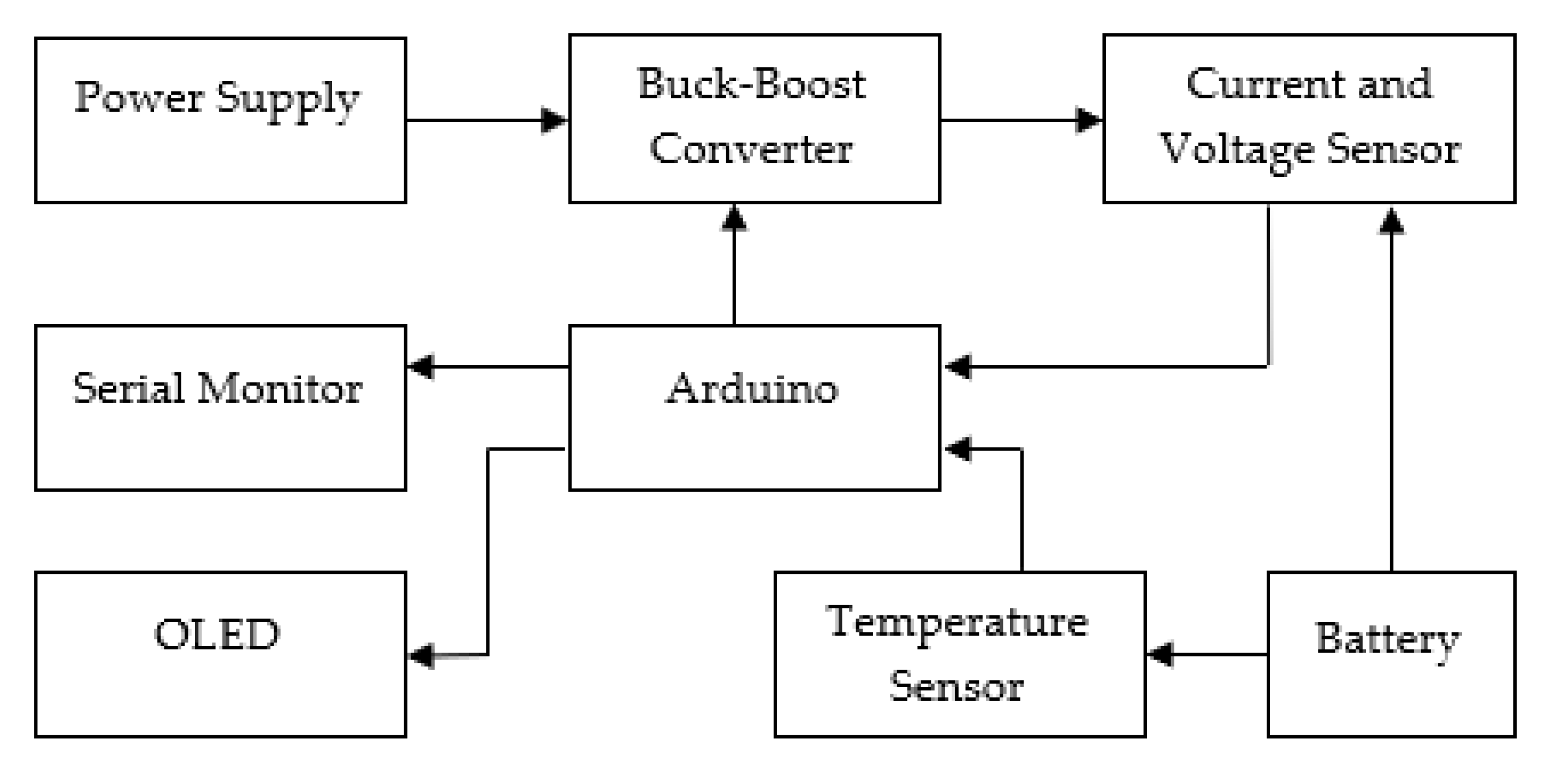
3.2.3. Comparison between CCCV and MSCC
- (a)
Charging 2S and 4S batteries
Figure 10 shows the charging processes of 2-series (2S) and 4-series (4S) batteries with CCCV and MSCC methods. The utilization of a 2S battery was to perform the buck mode, whereas the 4S battery was to perform the boost mode of the charger. According to the figure, both voltage and current show a constant pattern before the drastic change in the CCCV method. On the other side, in the MSCC method, the charging current’s pattern was like a step. The overall results showed a similar charging time for both 2S and 4S; the MSCC method required 45 min to fully charge the battery, which was reasonably faster than the CCCV method (55 min). A more comprehensive and detailed analysis will be provided in the next part.
- (b)
Charging voltages
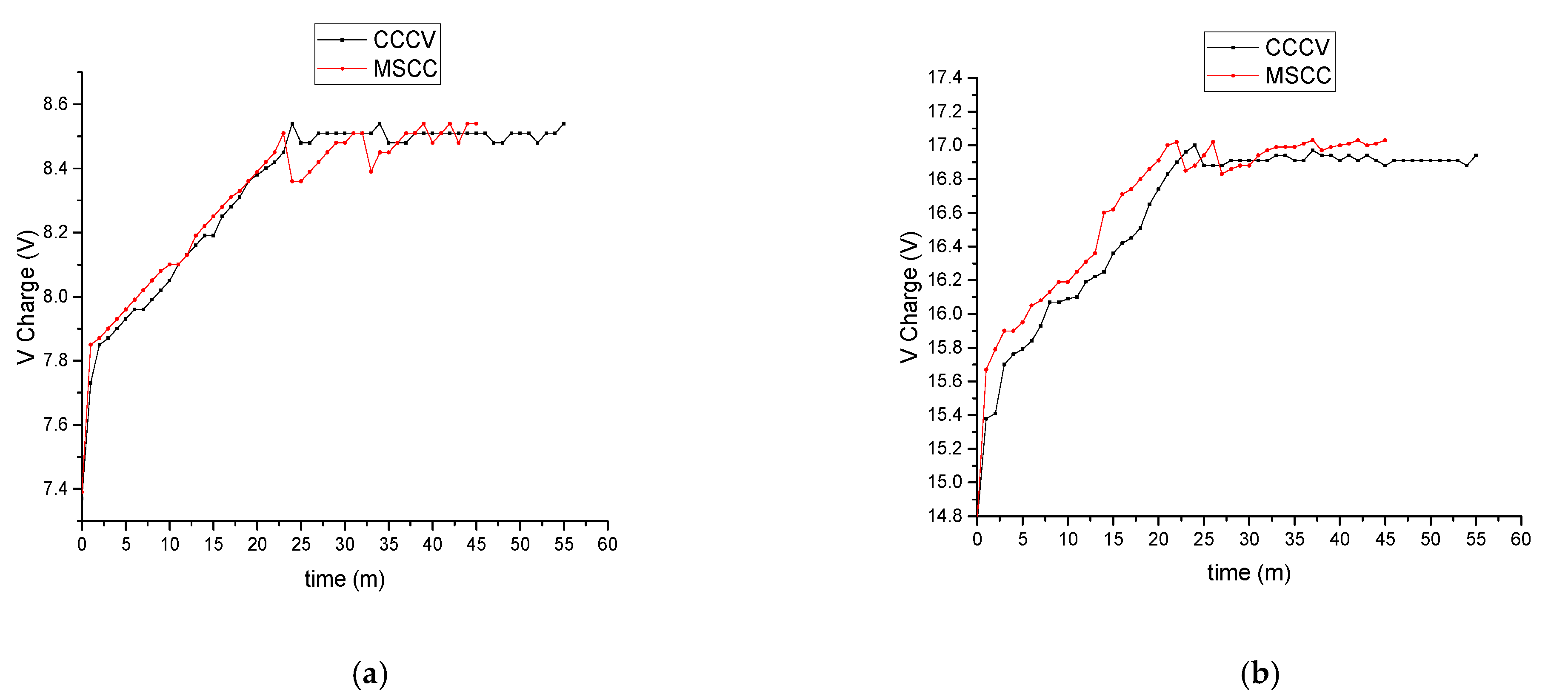
A comparison of charging voltage using CCCV and MSCC methods is shown in
Figure 11. Based on
Figure 11a, the voltage would slowly increase to stabilize the charging current at 2 A when charging a 2S battery using the CCCV method. When the voltage reached the cut-off at 8.51 V, the charging system entered CV mode, and the voltage would be stable at 8.51 V with a voltage ripple of 0–0.03 V.
Meanwhile, the initial charging voltage using the MSCC method will increase slowly to stabilize the charging current at 2 A, as shown in
Figure 11a. The charging system continued to the second step when the voltage reached the cut-off at 8.51 V, with a decreasing pattern, following the pattern of the charging current, which would later be stable at 1.42 A. After the charging current reached the second step of the charging strategy, the voltage rose again until it reached the second cut-off at 8.51 V. Then, the charging system continued to the third step of the fast-charging strategy, and the voltage would be dropped again until the charging current reached the next value of the current’s threshold. When the voltage reached the third cut-off at 8.54 V, the system then entered the fourth step of the fast-charging strategy with a decreasing trend until the charging current reached 0.73 A. The charging process would be continued until the charging voltage reached the fourth cut-off at 8.54 V, with a current of 0.52 A. At the fifth step of the charging strategy, the voltage would be maintained to stabilize the charging current at 0.52 A until the battery became fully charged. At this stage, the battery temperature decreased as the charging stages changed.
Similarly, the pattern of the charging voltage for the 4S battery is shown in
Figure 11b. In CCCV mode, the voltage would increase slowly to stabilize the charging current at 2 A. After reaching the cut-off voltage at 17.00 V, the charging system entered CV mode, and the voltage would be stable at 16.94 V with a ripple of 0–0.06 V.
In the MSCC mode, the initial charging voltage will increase slowly as well to stabilize the charging current at 2 A. The results were very much similar to the application for the 2S battery. The only difference was the cut-off value of the voltage. In the 4S battery, the voltage cut-off was around 17 V. The pattern of the charging voltage was similar to the previous results of the application for a 2S battery.
According to
Figure 11a,b, the charging voltage pattern for CCCV was considered reasonably smooth, whereas MSCC had a saw-tooth pattern since the charging current was changed after reaching the voltage threshold. However, the MSCC method performed a faster charging completion than the CCCV method based on the time taken by the batteries to be fully charged.
- (c)
Charging Current
In the application of the CCCV method for 2S batteries, the charging current was stable at ±2 A until the 26th minute; this indicates that the charging system was in the CC mode. Then, the charging current slowly dropped, indicating that the charging system was in CV mode, as shown in
Figure 12a. Similarly, in the application of the MSCC method, the charging current was stable at ±2 A until the 23rd minute; this indicates that the charging system was in the first step of the CC mode. Then, the charging current was lowered and stabilized at ±1.4 A until the 32nd minute, indicating that the charging system was in the second step. The current was reduced and stabilized at ±1.0 A until the 39th minute, indicating the third step of the fast-charging strategy. Later, the charging current was lowered and stabilized at ±0.73 A until the 42nd minute, indicating that the charging system was in the fourth step. The charging current was then lowered and re-stabilized at ±0.52 A until the battery was fully charged at the 45th minute, which was in the fifth stage.
Meanwhile,
Figure 12b shows the results for applying both methods for 4S batteries. When the battery was charged using the CCCV method, the charging current was stable at ±2 A until the 24th minute; this indicates that the charging was in the CC mode. After that, the current slowly dropped, indicating the charging system operated in CV mode. Using the MSCC method, the charging current was stable at ±2 A until the 23rd minute, which means the charging system was at the first step of the CC mode. At the 23rd minute, the charging current was reduced and stabilized at ±1.4 A until the 37th minute, indicating that the charging system was in the second step of the fast-charging strategy. After that, the charging current was reduced and stabilized at ±1.0 A until the 42nd minute, in the third stage. The charging current was reduced and stabilized at ±0.73 A until the 42nd minute, indicating that the charging is in the fourth stage. Eventually, the charging current was lowered and stabilized at ±0.52 A until the battery was fully charged at the 45th minute. This was considered the fifth step of the fast-charging strategy using the MSCC method.
Unlike the pattern of charging voltage, some differences can be clearly seen in the patterns of charging current for both methods. The charging current of the MSCC method had a step-like pattern that prevented a drastic change in current drop. However, in the CCCV method, the charging current had a drastic drop in its pattern. In these results, the current in the MSCC method was lower than in the CCCV method. This phenomenon does not mean the MSCC charging current capacity is lower than CCCV; this happens due to battery voltage in MSCC reaching the cut-off voltage (8.4 V for 2S and 16.8 for 4S); therefore, based on the MSCC algorithm, the current value is changed to the next step value. If the battery voltage has not reached the cut-off voltage yet, the MSCC current will continue at the determined current step, which will be higher than the CCCV current.
- (d)
Battery temperature
A detailed comparison of the battery temperature in the charging system for a 2S battery using CCCV and MSCC methods is shown in
Figure 13a. According to the figure, the battery temperature of a charging system using the CCCV method rose slowly in CC mode. Then, after reaching 32.7 °C, which was the highest temperature, the battery temperature was maintained stably for a while before decreasing in CV mode at the 33rd minute.
Meanwhile, in the MSCC method, the battery temperature also rose slowly in the first step of the CC mode but was not as drastic as in the CCCV method. The battery temperature reached its maximum, which was 32.2 °C at the 26th minute, and then decreased as the mode changed. The decrease in battery temperature in the MSCC method was performed faster than in the CCCV method, as the temperature was not maintained at the same value for a long time.
Figure 13b was a detailed comparison of the battery temperature for charging 4S batteries. Similar to the results of applying the CCCV method for charging the 2S battery, the battery’s temperature increased slowly in CC mode, was stable at its maximum (32 °C) for a while, and then decreased during the CV mode at the 32nd minute. The pattern of the battery temperature while charging the 4S battery using the MSCC method was also similar to the results of charging the 2S battery. The battery temperature also increased slowly at the first step of CC mode; however, the battery temperature was slightly higher than the results of the CCCV method during this period. Then, the battery temperature reached its maximum at the 23rd minute, which was 31.6 °C, and then decreased as the mode changed. During this period, the battery temperature was lower than the results of the CCCV method.
- (e)
Battery Voltage
The difference between the charging voltage and the battery voltage is that the charging voltage is measured when there is a current flow to the battery from the charger. In contrast, the battery voltage is measured every 5 min when the charger is disconnected since the battery current must be zero to estimate SoC using the OCV method.
Figure 14 shows the comparison of the battery voltage among the applications of CCCV and MSCC methods.
Figure 14a shows the results for charging 2S batteries. In the CCCV method, the initial battery voltage was 7.37 V, and the battery voltage slowly increased to reach 8.42 V. This indicates that the charging system had successfully charged the battery. Since the current entering the battery was restricted in the CV mode, the battery voltage would rise slowly.
Whereas when charging with MSCC, with an initial battery voltage of 7.38 V, the battery voltage increased faster with a higher voltage than the results of the CCCV method. The battery voltage rose slowly in the fourth stage due to the small charging current. The battery voltage did not exceed 8.4 V; this indicates that the system had successfully charged the battery fully and managed to keep the battery from overcharging.
Similarly, the battery voltage of a charging system for the 4S battery using CCCV and MSCC methods increased slowly and successfully reached 16.8 V, with initial voltages of 14.77 and 14.78 V, respectively. According to
Figure 14b, in applying the CCCV method to charge 4S batteries, the battery voltage rose slowly when entering the CV mode since the current to the battery was reduced. In different ways, during the fourth stage of charging using the MSCC method, the battery voltage rose slowly because the current entering the charging was small. The battery voltage did not exceed 16.8 V; this indicates that the system has fully charged the battery and managed to keep the battery from overcharging. The charging time taken to charge 4S batteries was the same as for 2S batteries.