Electrostatic Charge Effects on Pharmaceutical Aerosol Deposition in Human Nasal–Laryngeal Airways
Abstract
:1. Introduction
2. Methods
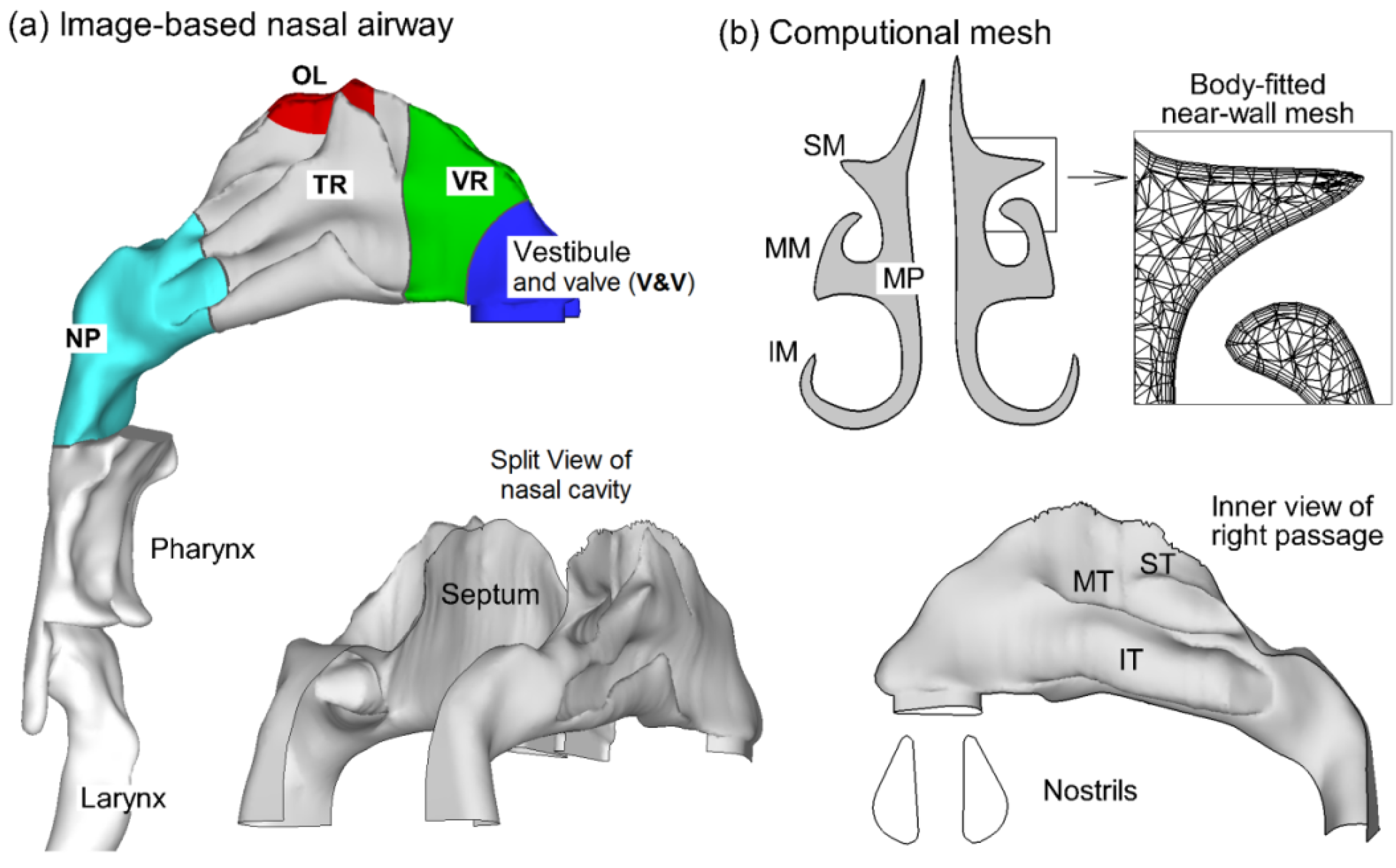
2.1. Nasal–Laryngeal Airway Model
2.2. Fluid-Particle Electrostatic Model


2.3. Charge Level in Pharmaceutical Aerosols
2.4. Numerical Method and Grid Sensitivity Analysis
3. Results and Discussion
3.1. Validation of Electrostatic Deposition Model
3.2. Nasal Airflows
3.3. Charged Particle Depositions
4. Conclusions
- For submicron aerosols, electrostatic charge exerted a discernible but not significant effect on both total and sub-regional depositions;
- For micrometer particles, which could acquire high levels of electrostatic charge, both total and local deposition values could be altered substantially depending on the aerosol charge;
- With increasing charge levels, the high-deposition-region shifted toward the front of the nasal passage;
- Boltzmann charge had a negligible effect on the nasal deposition of both submicron and micrometer aerosols.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Bologa, A. Electrohydrodynamic instability of droplets as an influencing factor for aerosol space charge generation. J. Electrost. 2001, 51, 470–475. [Google Scholar] [CrossRef]
- Karner, S.; Urbanetz, N.A. The impact of electrostatic charge in pharmaceutical powders with specific focus on inhalation-powders. J. Aerosol Sci. 2011, 42, 428–445. [Google Scholar] [CrossRef]
- Chan, T.L.; Lippmann, M.; Cohen, V.; Schlesinger, R.B. Effect of electrostatic charges on particle deposition in a hollow cast of the human larynx-tracheobronchial tree. J. Aerosol Sci. 1978, 9, 463–468. [Google Scholar] [CrossRef]
- Kwok, P.C.; Noakes, T.; Chan, H.-K. Effect of moisture on the electrostatic charge properties of metered dose inhaler aerosols. J. Aerosol Sci. 2008, 39, 211–226. [Google Scholar] [CrossRef]
- Cohen, B.S.; Xiong, J.Q.; Asgharian, B.; Ayres, L. Deposition of inhaled charged ultrafine particles in a simple tracheal model. J. Aerosol Sci. 1995, 26, 1149–1160. [Google Scholar] [CrossRef]
- Fry, F.A. Charge distribution on polystyrene aerosols and deposition in the human nose. J. Aerosol Sci. 1970, 1, 135–146. [Google Scholar] [CrossRef]
- Kwok, P.C.; Glover, W.; Chan, H.K. Electrostatic charge characteristics of aerosols produced from metered dose inhalers. J. Pharm. Sci. 2005, 94, 2789–2799. [Google Scholar] [CrossRef]
- Yu, C.P.; Chandra, K. Precipitation of submicron charged particles in human lung airways. Bull. Math. Biol. 1977, 39, 471–478. [Google Scholar]
- Xi, J.; Longest, P.W.; Martonen, T.B. Effects of the laryngeal jet on nano- and microparticle transport and deposition in an approximate model of the upper tracheobronchial airways. J. Appl. Physiol. 2008, 104, 1761–1777. [Google Scholar] [CrossRef]
- Hinds, W.C. Aerosol Technology: Properties, Behavior, and Measurement of Airborne Particles; John Wiley and Sons: New York, NY, USA, 1999. [Google Scholar]
- Chen, Y.K.; Yu, C.P. Particle deposition from duct flows by combined mechanisms. Aerosol Sci. Technol. 1993, 19, 389–395. [Google Scholar] [CrossRef]
- Xi, J.; Longest, P.W. Numerical predictions of submicrometer aerosol deposition in the nasal cavity using a novel drift flux approach. Int. J. Heat Mass Transf. 2008, 51, 5562–5577. [Google Scholar] [CrossRef]
- Si, X.A.; Xi, J.; Kim, J.; Zhou, Y.; Zhong, H. Modeling of release position and ventilation effects on olfactory aerosol drug delivery. Respir. Physiol. Neurobiol. 2013, 186, 22–32. [Google Scholar] [CrossRef]
- Morsi, S.A.; Alexander, A.J. An investigation of particle trajectories in two-phase flow systems. J. Fluid Mech. 1972, 55, 193–208. [Google Scholar] [CrossRef]
- Li, A.; Ahmadi, G. Dispersion and deposition of spherical particles from point sources in a turbulent channel flow. Aerosol Sci. Technol. 1992, 16, 209–226. [Google Scholar] [CrossRef]
- Xi, J.; Longest, P.W. Effects of oral airway geometry characteristics on the diffusional deposition of inhaled nanoparticles. J. Biomech. Eng. 2008, 130, 011008:1–011008:16. [Google Scholar]
- Byron, P.R.; Peart, J.; Staniforth, J.N. Aerosol electrostatics I: Properties of fine powders before and after aerosolization by dry powder inhalers. Pharma. Res. 1997, 14, 698–705. [Google Scholar] [CrossRef]
- Vinchurkar, S.; Longest, P.W.; Peart, J. CFD simulations of the Andersen cascade impactor: Model development and effects of aerosol charge. J. Aerosol Sci. 2009, 40, 807–822. [Google Scholar] [CrossRef]
- Longest, P.W.; Xi, J. Effectiveness of direct Lagrangian tracking models for simulating nanoparticle deposition in the upper airways. Aerosol Sci. Technol. 2007, 41, 380–397. [Google Scholar] [CrossRef]
- Xi, J.; Longest, P.W. Evaluation of a drift flux model for simulating submicrometer aerosol dynamics in human upper tracheobronchial airways. Ann. Biomed. Eng. 2008, 36, 1714–1734. [Google Scholar] [CrossRef]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Xi, J.; Si, X.; Longest, W. Electrostatic Charge Effects on Pharmaceutical Aerosol Deposition in Human Nasal–Laryngeal Airways. Pharmaceutics 2014, 6, 26-35. https://doi.org/10.3390/pharmaceutics6010026
Xi J, Si X, Longest W. Electrostatic Charge Effects on Pharmaceutical Aerosol Deposition in Human Nasal–Laryngeal Airways. Pharmaceutics. 2014; 6(1):26-35. https://doi.org/10.3390/pharmaceutics6010026
Chicago/Turabian StyleXi, Jinxiang, Xiuhua Si, and Worth Longest. 2014. "Electrostatic Charge Effects on Pharmaceutical Aerosol Deposition in Human Nasal–Laryngeal Airways" Pharmaceutics 6, no. 1: 26-35. https://doi.org/10.3390/pharmaceutics6010026
APA StyleXi, J., Si, X., & Longest, W. (2014). Electrostatic Charge Effects on Pharmaceutical Aerosol Deposition in Human Nasal–Laryngeal Airways. Pharmaceutics, 6(1), 26-35. https://doi.org/10.3390/pharmaceutics6010026




