Antibodies Processed Using High Dilution Technology Distantly Change Structural Properties of IFNγ Aqueous Solution
Abstract
:1. Introduction
2. Materials and Methods
2.1. Preparation of Samples
- A water-lactose mixture obtained from fluidised lactose saturated with extremely diluted solutions of antibodies to IFNγ (see below), hereinafter referred to as saturated lactose.
- A water-lactose blend obtained from fluidised lactose saturated with extremely diluted solutions of water, designated as a control sample.
- 7 mL of water was added to 40 experimental tablets (300 mg each) of each sample and left at room temperature (humidity 35%) for 15 min in sterile glass vials with screw caps (Glastechnik Gräfenroda, Geratal, Germany). Then the resulting paste was mixed with a spatula.
- An aqueous solution of IFNγ (1 mg/mL) to the volume of 2 mL in a 5 mL polystyrene test tube was immersed in a vial with a water-lactose mixture. Thus, the part of the test tube filled with IFNγ was completely surrounded by a water-lactose mixture. There was no direct contact between the IFNγ aqueous solution and the water-lactose mixture–they were separated from each other by the wall of the test tube.
- The vial (with the immersed polystyrene test tube of IFNγ inside it) was immersed in a Ministat 230 liquid thermostat (Huber, Offenburg, Germany), set at 37.0 °C. After one hour, 0.5 mL of the IFNγ solution was taken out of the tube for spectral analysis (sample 1, see Section 2.2). The rest of the sample was left incubated under the same conditions. After analysing the first sample, a second sample was taken for spectral analysis in a similar way. The total incubation time of the second sample was 1.5–2 h. After analysing the second sample, a third sample was taken which was incubated for 2.5–3 h. Thus, we analysed not only the effect of the saturated lactose and the control on the IFNγ aqueous solution but also the effect’s dependence on the incubation time.
2.2. THz-TDS
2.3. Spectral Data Analysis
2.4. Statistical Data Analysis
3. Results
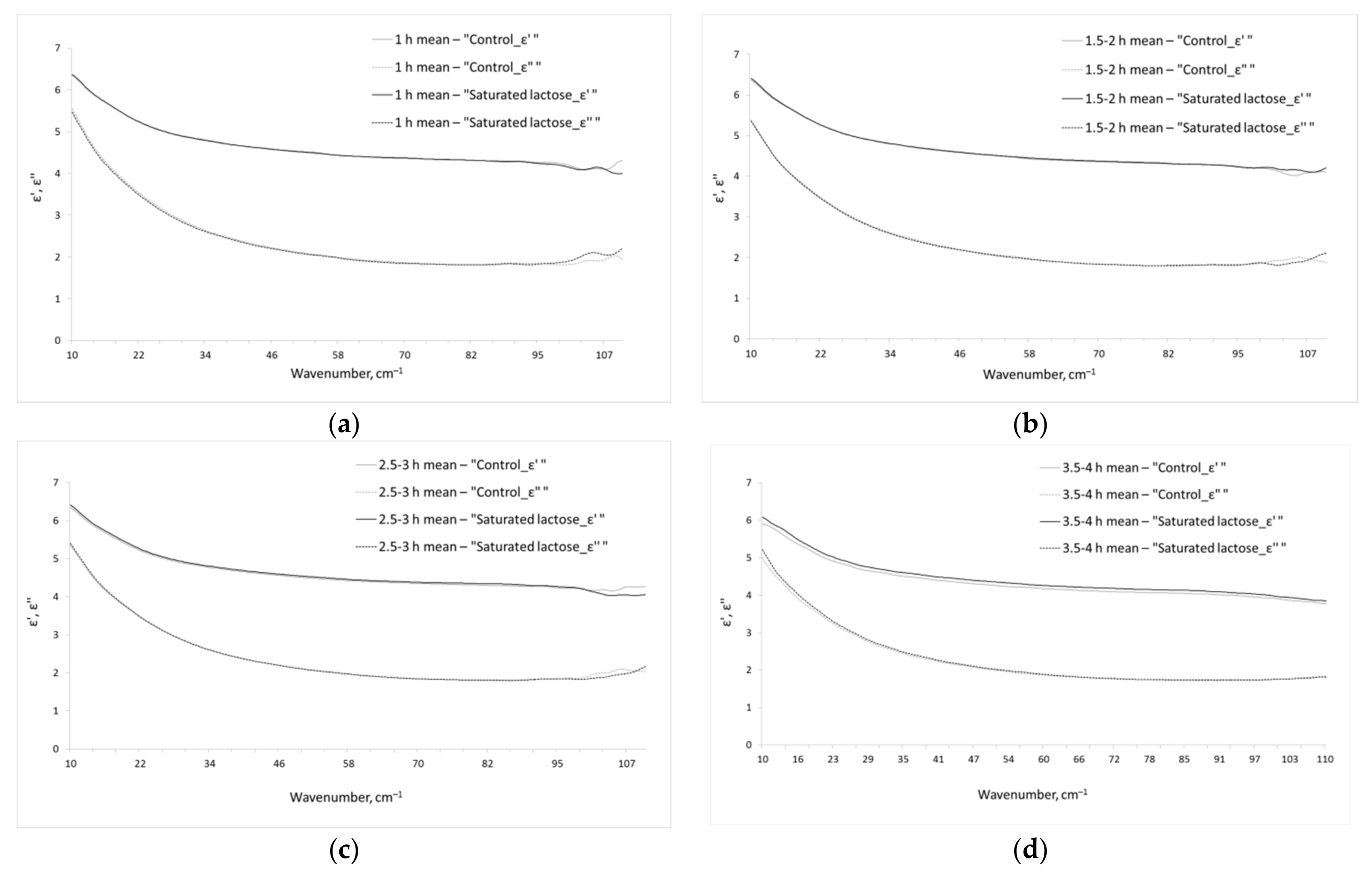
3.1. Dielectric Permittivities of Aqueous Phase of Analyzed IFNγ Solutions
3.2. Parameters of the Model Dielectric Permittivity of the Aqueous Phase of IFNγ Solutions
4. Discussion
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chikramane, P.S.; Kalita, D.; Suresh, A.K.; Kane, S.G.; Bellare, J.R. Why extreme dilutions reach non-zero asymptotes: A nanoparticulate hypothesis based on froth flotation. Langmuir 2012, 28, 15864–15875. [Google Scholar] [CrossRef] [PubMed]
- Gudkov, S.V.; Penkov, N.V.; Baimler, I.V.; Lyakhov, G.A.; Pustovoy, V.I.; Simakin, A.V.; Sarimov, R.M.; Scherbakov, I.A. Effect of Mechanical Shaking on the Physicochemical Properties of Aqueous Solutions. Int. J. Mol. Sci. 2020, 21, 8033. [Google Scholar] [CrossRef]
- Fesenko, E.E.; Gluvstein, A.Y. Changes in the state of water, induced by radiofrequency electromagnetic fields. FEBS Lett. 1995, 367, 53–55. [Google Scholar] [CrossRef] [Green Version]
- Novikov, V.V.; Yablokova, E.V.; Fesenko, E.E. The role of water in the effect of weak combined magnetic fields on production of reactive oxygen species (ROS) by neutrophils. Appl. Sci. 2020, 10, 3326. [Google Scholar] [CrossRef]
- Demangeat, J.L. NMR water proton relaxation in unheated and heated ultrahigh aqueous dilutions of histamine: Evidence for an air-dependent supramolecular organization of water. J. Mol. Liq. 2009, 144, 32–39. [Google Scholar] [CrossRef]
- Bunkin, N.F.; Shkirin, A.V.; Ninham, B.W.; Chirikov, S.N.; Chaikov, L.L.; Penkov, N.V.; Kozlov, V.A.; Gudkov, S.V. Shaking-Induced Aggregation and Flotation in Immunoglobulin Dispersions: Differences between Water and Water–Ethanol Mixtures. ACS Omega 2020, 5, 14689–14701. [Google Scholar] [CrossRef] [PubMed]
- Bunkin, N.F.; Shkirin, A.V.; Penkov, N.V.; Chirikov, S.N.; Ignatiev, P.S.; Kozlov, V.A. The physical nature of mesoscopic inhomogeneities in highly diluted aqueous suspensions of protein particles. Phys. Wave Phenom. 2019, 27, 102–112. [Google Scholar] [CrossRef]
- Jin, Q.; Yiwen, E.; Williams, K.; Dai, J.; Zhang, X.C. Observation of broadband terahertz wave generation from liquid water. Appl. Phys. Lett. 2017, 111, 071103. [Google Scholar] [CrossRef]
- Zhao, H.; Tan, Y.; Zhang, R.; Shalaby, M.; Zhang, C.; Zhao, Y.; Zhang, X.-C. Ultrafast hydrogen bond dynamics of liquid water revealed by terahertz-induced transient birefringence. Light Sci. Appl. 2020, 9, 136. [Google Scholar] [CrossRef]
- Don, E.; Farafonova, O.; Pokhil, S.; Barykina, D.; Nikiforova, M.; Shulga, D.; Borshcheva, A.; Tarasov, S.; Ermolaeva, T.; Epstein, O. Use of piezoelectric immunosensors for detection of interferon-gamma interaction with specific antibodies in the presence of released-active forms of antibodies to interferon-gamma. Sensors 2016, 16, 96. [Google Scholar] [CrossRef] [Green Version]
- Don, E.S.; Bobrovnik, S.A.; Sherriff, G.; Myslivets, A.A.; Tarasov, S.A.; Epstein, O.I. Advanced approach to activity evaluation for released-active forms of antibodies to interferon-gamma by enzyme-linked immunoassay. J. Immunoass. Immunochem. 2019, 40, 250–268. [Google Scholar] [CrossRef]
- Don, E.S.; Emelyanova, A.G.; Yakovleva, N.N.; Petrova, N.V.; Nikiforova, M.V.; Gorbunov, E.A.; Tarasov, S.A.; Morozov, S.G.; Epstein, O.I. Dose-Dependent Antiviral Activity of Released-Active Form of Antibodies to Interferon-Gamma against influenza A/California/07/09(H1N1) in Murine Model. J. Med. Virol. 2017, 89, 759–766. [Google Scholar] [CrossRef]
- Don, E.; Van der Meide, N.; Egorov, V.; Putilovskiy, M.; Tarasov, S. The level of natural autoantibodies to IFN-gamma in varicella infection treated with antiviral drug Anaferon for children: A pilot study. Immunol. Lett. 2020, 222, 90–94. [Google Scholar] [CrossRef]
- Tarasov, S.A.; Gorbunov, E.A.; Don, E.S.; Emelyanova, A.G.; Kovalchuk, A.L.; Yanamala, N.; Schleker, A.S.S.; Klein-Seetharaman, J.; Groenestein, R.; Tafani, J.-P.; et al. Insights into the mechanism of action of highly diluted biologics. J. Immunol. 2020, 205, 1345–1354. [Google Scholar] [CrossRef]
- Woods, K.N. New insights into the microscopic interactions associated with the physical mechanism of action of highly diluted biologics. Sci. Rep. 2021, 11, 13774. [Google Scholar] [CrossRef]
- Penkov, N.; Penkova, N. Analysis of Emission Infrared Spectra of Protein Solutions in Low Concentrations. Front. Phys. 2020, 8, 611. [Google Scholar] [CrossRef]
- Nazarov, M.M.; Cherkasova, O.P.; Shkurinov, A.P. Study of the dielectric function of aqueous solutions of glucose and albumin by THz time-domain spectroscopy. Quantum Electron. 2016, 46, 488–495. [Google Scholar] [CrossRef]
- Cherkasova, O.P.; Nazarov, M.M.; Konnikova, M.; Shkurinov, A.P. THz Spectroscopy of Bound Water in Glucose: Direct Measurements from Crystalline to Dissolved State. J. Infrared Millim. Terahz Waves 2020, 41, 1057–1068. [Google Scholar] [CrossRef]
- Penkov, N.V.; Shvirst, N.E.; Yashin, V.A.; Fesenko, E.E. Calculation of the portion of free water molecules in water solutions by means of spectral analysis. Biophysics 2013, 58, 739–742. [Google Scholar] [CrossRef]
- Penkov, N.V.; Yashin, V.A.; Shvirst, N.E.; Fesenko, E.E., Jr.; Fesenko, E.E. On peculiarities of temperature dependences of water spectra in the terahertz frequency domain. Biophysics 2014, 59, 220–222. [Google Scholar] [CrossRef]
- Penkov, N.V.; Shvirst, N.E.; Yashin, V.A.; Fesenko, E.E. On singularities of molecular relaxation in water solutions. Biofizika 2013, 58, 933–941. (In Russian) [Google Scholar] [CrossRef]
- Penkov, N.; Yashin, V.; Fesenko, E.E., Jr.; Manokhin, A.; Fesenko, E. A Study of the Effect of a Protein on the Structure of Water in Solution Using Terahertz Time-Domain Spectroscopy. Appl. Spectrosc. 2018, 72, 257–267. [Google Scholar] [CrossRef] [PubMed]
- Penkov, N.; Shvirst, N.; Yashin, V.; Fesenko, E., Jr.; Fesenko, E. Terahertz Spectroscopy Applied for Investigation of Water Struc-ture. J. Phys. Chem. B. 2015, 119, 12664–12670. [Google Scholar] [CrossRef] [PubMed]
- Penkov, N.V.; Yashin, V.A.; Fesenko, E.E., Jr.; Fesenko, E.E. Calculation of the amount of free water molecules in aqueous solutions by means of spectral parameters from the terahertz frequency domain taking into account processes of screening. Biophysics 2014, 59, 347–350. [Google Scholar] [CrossRef]
- Asaki, M.L.T.; Redondo, A.; Zawodzinski, T.A.; Zawodzinski, A.J. Dielectric relaxation of electrolyte solutions using terahertz transmission spectroscopy. J. Chem. Phys. 2002, 116, 8469–8482. [Google Scholar] [CrossRef] [Green Version]
- Peng, Y.; Shi, C.; Zhu, Y.; Gu, M.; Zhuang, S. Terahertz spectroscopy in biomedical field: A review on signal-to-noise ratio improvement. PhotoniX 2020, 1, 12. [Google Scholar] [CrossRef] [Green Version]
- Penkov, N.V.; Yashin, V.A.; Belosludtsev, K.N. Hydration Shells of DPPC Liposomes from the Point of View of Terahertz Time-Domain Spectroscopy. Appl. Spectrosc. 2021, 75, 189–198. (In English) [Google Scholar] [CrossRef] [PubMed]
- Penkov, N.V.; Penkova, N. Key Differences of the Hydrate Shell Structures of ATP and Mg·ATP Revealed by Terahertz Time-Domain Spectroscopy and Dynamic Light Scattering. J. Phys. Chem. B 2021, 125, 4375–4382. (In English) [Google Scholar] [CrossRef]
- Pershin, S.M. Conversion of ortho-para H2O isomers in water and a jump in erythrocyte fluidity through a microcapillary at a temperature of 36.6 ± 0.3 °C. Phys. Wave Phenom. 2021, 17, 241–250. [Google Scholar] [CrossRef]
- Dutta Banik, S.; Nordblad, M.; Woodley, J.M.; Peters, G.H. Effect of Water Clustering on the Activity of Candida antarctica Lipase B in Organic Medium. Catalysts 2017, 7, 227. [Google Scholar] [CrossRef] [Green Version]
- Noskov, S.Y.; Kiselev, M.G.; Kolker, A.M. The role of bounded water in protein-ligand association processes. Biophysics 2010, 55, 39–45. [Google Scholar] [CrossRef]
- Sun, L.; Zhao, L.; Peng, R.-Y. Research progress in the effects of terahertz waves on biomacromolecules. Mil. Med. Res. 2021, 8, 28. [Google Scholar] [CrossRef]
- Yamazaki, S.; Harata, M.; Idehara, T.; Konagaya, K.; Yokoyama, G.; Hoshina, H.; Ogawa, Y. Actin polymerization is activated by terahertz irradiation. Sci. Rep. 2018, 8, 9990. [Google Scholar] [CrossRef]
- Vasylieva, A.; Doroshenko, I.; Vaskivskyi, Y.; Chernolevska, Y.; Pogorelov, V. FTIR study of condensed water structure. J. Mol. Struct. 2018, 1167, 232–238. [Google Scholar] [CrossRef]
- Shi, L.; Gruenbaum, S.M.; Skinner, J.L. Interpretation of IR and Raman line shapes for H2O and D2O ice Ih. Phys. Chem. B 2012, 116, 13821–13830. [Google Scholar] [CrossRef]
- Yakovenko, A.A.; Yashin, V.A.; Kovalev, A.E.; Fesenko, E.E. Structure of the vibrational absorption spectra of water in the visible region. Biophysics 2002, 47, 891–895. [Google Scholar]
- Scherer, J.R.; Go, M.K.; Kint, S. Raman spectra and structure of water from −10 to 90. deg. J. Phys. Chem. 1974, 78, 1304–1313. [Google Scholar] [CrossRef]
- Walrafen, G.E.; Hokmabadi, M.S.; Yang, W.H. Raman isosbestic points from liquid water. J. Chem. Phys. 1986, 85, 6964–6969. [Google Scholar] [CrossRef]
- Kraiski, A.V.; Mel’nik, N.N. Low-frequency Raman spectra in water and in weak aqueous solutions. Spatial inhomogeneity in hydrogen peroxide solution. Biophysics 2012, 57, 750–756. [Google Scholar] [CrossRef]
- Slatinskaya, O.V.; Pyrkov, Y.N.; Filatova, S.A.; Guryev, D.A.; Penkov, N.V. Study of the Effect of Europium Acetate on the Intermolecular Properties of Water. Front. Phys. 2021, 9, 63. [Google Scholar] [CrossRef]
- Noguchi, T.; Sugiura, M. Structure of an active water molecule in the water-oxidizing complex of photosystem II as studied by FTIR spectroscopy. Biochemistry 2000, 39, 10943–10949. [Google Scholar] [CrossRef] [PubMed]
- Sommers, G.M.; Andrade, M.F.C.; Zhang, L.; Wang, H.; Car, R. Raman spectrum and polarizability of liquid water from deep neural networks. Phys. Chem. Chem. Phys. 2020, 22, 10592–10602. [Google Scholar] [CrossRef] [PubMed]
- Fadeev, V.V.; Burikov, S.A.; Volkov, P.A.; Lapshin, V.B.; Syroeshkin, A.V. Raman scattering and fluorescence spectra of water from the sea surface microlayer. Oceanology 2009, 49, 205–210. [Google Scholar] [CrossRef]
- Burikov, S.A.; Dolenko, T.A.; Velikotnyi, P.A.; Sugonyaev, A.V.; Fadeev, V.V. The effect of hydration of ions of inorganic salts on the shape of the Raman stretching band of water. Opt. Spectrosc. 2005, 98, 235–239. [Google Scholar] [CrossRef]
- Penkov, N.; Fesenko, E. Development of Terahertz Time-Domain Spectroscopy for Properties Analysis of Highly Diluted Antibodies. Appl. Sci. 2020, 10, 7736. [Google Scholar] [CrossRef]
- Theuer, M.; Harsha, S.S.; Molter, D.; Torosyan, G.; Beigang, R. Terahertz time-domain spectroscopy of gases, liquids, and solids. Chemphyschem 2011, 12, 2695–2705. (In English) [Google Scholar] [CrossRef]
- Choy, T.C. Effective Medium Theory: Principle and Applications; Oxford University Press: Oxfordshire, UK, 2016. [Google Scholar]
- Fischer, H.; Polikarpov, I.; Craievich, A.F. Average protein density is a molecular-weight-dependent function. Protein Sci. 2004, 13, 2825–2828. [Google Scholar] [CrossRef]
- Markelz, A.G.; Roitberg, A.; Heilweil, E.J. Pulsed terahertz spectroscopy of DNA, bovine serum albumin and collagen between 0.1 and 2.0 THz. Chem. Phys. Lett. 2000, 320, 42–48. [Google Scholar] [CrossRef]
- Von Hippel, A.R. The dielectric relaxation spectra of water, ice, and aqueous solutions, and their interpretation. I. Critical survey of the sta-tus-quo for water. IEEE Trans. Electr. Insul. 1988, 23, 801–816. [Google Scholar] [CrossRef]
- Laage, D.; Hynes, J.T. A Molecular Jump Mechanism of Water Reorientation. Science 2006, 311, 832–835. [Google Scholar] [CrossRef]
- Yada, H.; Nagai, M.; Tanaka, K. Origin of the fast relaxation component of water and heavy water revealed by terahertz time domain attenuated total reflection spectroscopy. Chem. Phys. Lett. 2008, 464, 166–170. [Google Scholar] [CrossRef]
- Zasetsky, A.Y. Dielectric relaxation in liquid water: Two fractions or two dynamics? Phys. Rev. Lett. 2011, 107, 117601. [Google Scholar] [CrossRef]
- Nielsen, O.F. Low-frequency spectroscopic studies of interactions in liquids. Annu. Rep. Prog. Chem. Sect. C Phys. Chem. 1993, 90, 3–44. [Google Scholar] [CrossRef]
- Nielsen, O.F. Low-frequency spectroscopic studies and intermolecular vibrational energy transfer in liquids. Annu. Rep. Prog. Chem. Sect. C Phys. Chem. 1996, 93, 57–99. [Google Scholar] [CrossRef]
- Ellison, W.J. Permittivity of pure water, at standard atmospheric pressure, over the frequency range 0–25 THz and the temperature range 0–100 °C. J. Phys. Chem. Ref. Data 2007, 36, 1–18. [Google Scholar] [CrossRef]
- Buchner, R.; Barthel, J.; Stauber, J. The dielectric relaxation of water between 0 °C and 35 °C. Chem. Phys. Lett. 1999, 306, 57–63. [Google Scholar] [CrossRef]
- Von Hippel, A.R. The Dielectric Relaxation Spectra of Water, Ice, and Aqueous Solutions, and Their Interpretation. 2. Tentative Interpretation of the Relaxation Spectrum of Water in the Time and Frequency Domain. IEEE Trans. Electr. Insul. 1988, 23, 817–823. [Google Scholar] [CrossRef]
- Djikaev, Y.S.; Ruckenstein, E. Dependence of the number of hydrogen bonds per water molecule on its distance to a hydrophobic surface and a thereupon-based model for hydrophobic attraction. J. Chem. Phys. 2010, 133, 194105. [Google Scholar] [CrossRef]
- Rossi, C.; Foletti, A.; Magnani, A.; Lamponi, S. New perspectives in cell communication: Bioelectromagnetic interactions. Semin. Cancer Biol. 2011, 21, 207–214. [Google Scholar] [CrossRef]
- Thomas, Y.; Schiff, M.; Belkadi, L.; Jurgens, P.; Kahhak, L.; Benveniste, J. Activation of human neutrophils by electronically transmitted phorbol-myristate acetate. Med. Hypotheses 2000, 54, 9–33. [Google Scholar] [CrossRef]
- Hwang, S.G.; Hong, J.K.; Sharma, A.; Pollack, G.H.; Bahng, G. Exclusion zone and heterogeneous water structure at ambient temperature. PLoS ONE 2018, 13, e0195057. [Google Scholar] [CrossRef]
- Montagnier, L.; Aissa, J.; Del Giudice, E.; Lavallee, C.; Tedeschi, A.; Vitiello, G. DNA waves and water. J. Phys. Conf. Ser. 2011, 306, 012007. [Google Scholar] [CrossRef]
- Dong, X.; Wang, S.; Yu, P.; Yang, F.; Zhao, J.; Wu, L.Z.; Tung, C.H.; Wang, J. Ultrafast Vibrational Energy Transfer through the Covalent Bond and Intra-and Intermolecular Hydrogen Bonds in a Supramolecular Dimer by Two-Dimensional Infrared Spectroscopy. J. Phys. Chem. B 2020, 124, 544–555. [Google Scholar] [CrossRef]
- De Ninno, A.; Pregnolato, M. Electromagnetic homeostasis and the role of low-amplitude electromagnetic fields on life organization. Electromagn. Biol. Med. 2017, 36, 115–122. [Google Scholar] [CrossRef]
- Jerman, I.; Ružič, R.; Krašovec, R.; Skarja, M.; Mogilnicki, L. Electrical Transfer of Molecule Information into Water, Its Storage, and Bioeffects on Plants and Bacteria. Electromagn. Biol. Med. 2005, 24, 341–353. [Google Scholar] [CrossRef]
- Riemenschneider, J. Spectroscopic Investigations on Pure Water and Aqueous Salt Solutions in the Mid Infrared Region. Ph.D. Thesis, University of Rostock, Rostock, Germany, 2011; pp. 1–112. [Google Scholar]
- Yukhnevich, G.V. Infrared Spectroscopy of Water; Nauka: Moscow, Russia, 1973. (In Russian) [Google Scholar]
- Taricska, N.; Bokor, M.; Menyhárd, D.K.; Tompa, K.; Perczel, A. Hydration shell differentiates folded and disordered states of a Trp-cage miniprotein, allowing characterization of structural heterogeneity by wide-line NMR measurements. Sci. Rep. 2019, 9, 2947. [Google Scholar] [CrossRef]
- Fenimore, P.W.; Frauenfelder, H.; McMahon, B.H.; Young, R.D. Bulk-solvent and hydration-shell fluctuations, similar to α-and β-fluctuations in glasses, control protein motions and functions. Proc. Natl. Acad. Sci. USA 2004, 101, 14408–14413. [Google Scholar] [CrossRef] [Green Version]
- Tcypkin, A.; Ponomareva, E.; Putilin, S.; Smirnov, S.; Shtumpf, S.A.; Melnik, M.; Yiwen, E.; Kozlov, S.; Zhang, X. Concentration dependence of terahertz generation in jets of water and ethanol mixtures. Infrared Millim.-Wave Terahertz Technol. V 2018, 10826, 1082603. [Google Scholar] [CrossRef]
- Belovolova, L.V.; Glushkov, M.V.; Vinogradov, E.A.; Babintsev, V.A.; Golovanov, V.I. Ultraviolet Fluorescence of Water and Highly Diluted Aqueous Media. Phys. Wave Phenom. 2009, 17, 21–31. [Google Scholar] [CrossRef]
- Penkov, N.V. Temporal Dynamics of the Scattering Properties of Deionized Water. Phys. Wave Phenom. 2020, 28, 135–139. [Google Scholar] [CrossRef]
- Styrkas, A.D.; Nikishinal, N.G. Mechanochemical processes in water. High Energy Chem. 2007, 41, 396–402. [Google Scholar] [CrossRef]
- Stebnovskii, S.V. Dynamo-optical effect in homogeneous Newtonian fluids. Tech. Phys. 2002, 47, 1369–1372. [Google Scholar] [CrossRef]
| Incubation Time | Sample | Δε1 | Δε2 | τ2*10, ps | A/103, cm−2 | A/ω2 | ω, cm−1 | γ, cm−1 |
|---|---|---|---|---|---|---|---|---|
| 1 h | Control | 71.03 ± 4.08 | 2.63 ± 0.13 | 3.06 ± 0.28 | 72.00 ± 20.62 | 1.68 ± 0.15 | 204.50 ± 21.69 | 190.00 ± 54.59 |
| Saturated lactose | 67.52 ± 4.49 | 2.76 ± 0.07 | 3.12 ± 0.16 | 57.00 ± 13.67 | 1.64 ± 0.11 | 185.33 ± 15.88 | 154.17 ± 37.34 | |
| 1.5–2 h | Control | 67.08 ± 3.97 | 2.74 ± 0.15 | 3.13 ± 0.15 | 70.67 ± 21.61 | 1.68 ± 0.16 | 202.33 ± 22.57 | 189.17 ± 46.20 |
| Saturated lactose | 66.18 ± 2.70 | 2.77 ± 0.15 | 3.19 ± 0.25 | 70.67 ± 11.15 | 1.70 ± 0.07 | 203.00 ± 11.85 | 188.33 ± 29.10 | |
| 2.5–3 h | Control | 67.30 ± 5.97 | 2.68 ± 0.12 | 2.92 ± 0.16 | 50.67 ± 7.23 | 1.58 ± 0.08 | 178.67 ± 9.18 | 134.17 ± 19.85 |
| Saturated lactose | 66.65 ± 2.43 | 2.79 ± 0.15 | 3.22 ± 0.15 * (p = 0.007) | 67.33 ± 11.57 * (p = 0.01) | 1.70 ± 0.10 | 198.33 ± 12.99 * (p = 0.01) | 180.00 ± 28.64 * (p = 0.009) | |
| 3.5–4 h | Control | 67.91 ± 8.60 | 2.34 ± 0.22 | 2.74 ± 0.21 | 53.84 ± 22.82 | 1.46 ± 0.36 | 188.17 ± 16.87 | 174.57 ± 32.99 |
| Saturated lactose | 67.24 ± 9.26 | 2.49 ± 0.22 * (p = 0.042) | 3.03 ± 0.28 * (p < 0.001) | 74.10 ± 23.93 * (p = 0.035) | 1.57 ± 0.21 | 212.94 ± 26.93 * (p = 0.004) | 222.34 ± 46.03 * (p < 0.001) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Penkov, N. Antibodies Processed Using High Dilution Technology Distantly Change Structural Properties of IFNγ Aqueous Solution. Pharmaceutics 2021, 13, 1864. https://doi.org/10.3390/pharmaceutics13111864
Penkov N. Antibodies Processed Using High Dilution Technology Distantly Change Structural Properties of IFNγ Aqueous Solution. Pharmaceutics. 2021; 13(11):1864. https://doi.org/10.3390/pharmaceutics13111864
Chicago/Turabian StylePenkov, Nikita. 2021. "Antibodies Processed Using High Dilution Technology Distantly Change Structural Properties of IFNγ Aqueous Solution" Pharmaceutics 13, no. 11: 1864. https://doi.org/10.3390/pharmaceutics13111864
APA StylePenkov, N. (2021). Antibodies Processed Using High Dilution Technology Distantly Change Structural Properties of IFNγ Aqueous Solution. Pharmaceutics, 13(11), 1864. https://doi.org/10.3390/pharmaceutics13111864






