Do Stands Self-Thin Through a Common Point? An Additional Concept for the Self-Thinning Rule
Abstract
1. Introduction
2. Data and Methods
3. Results
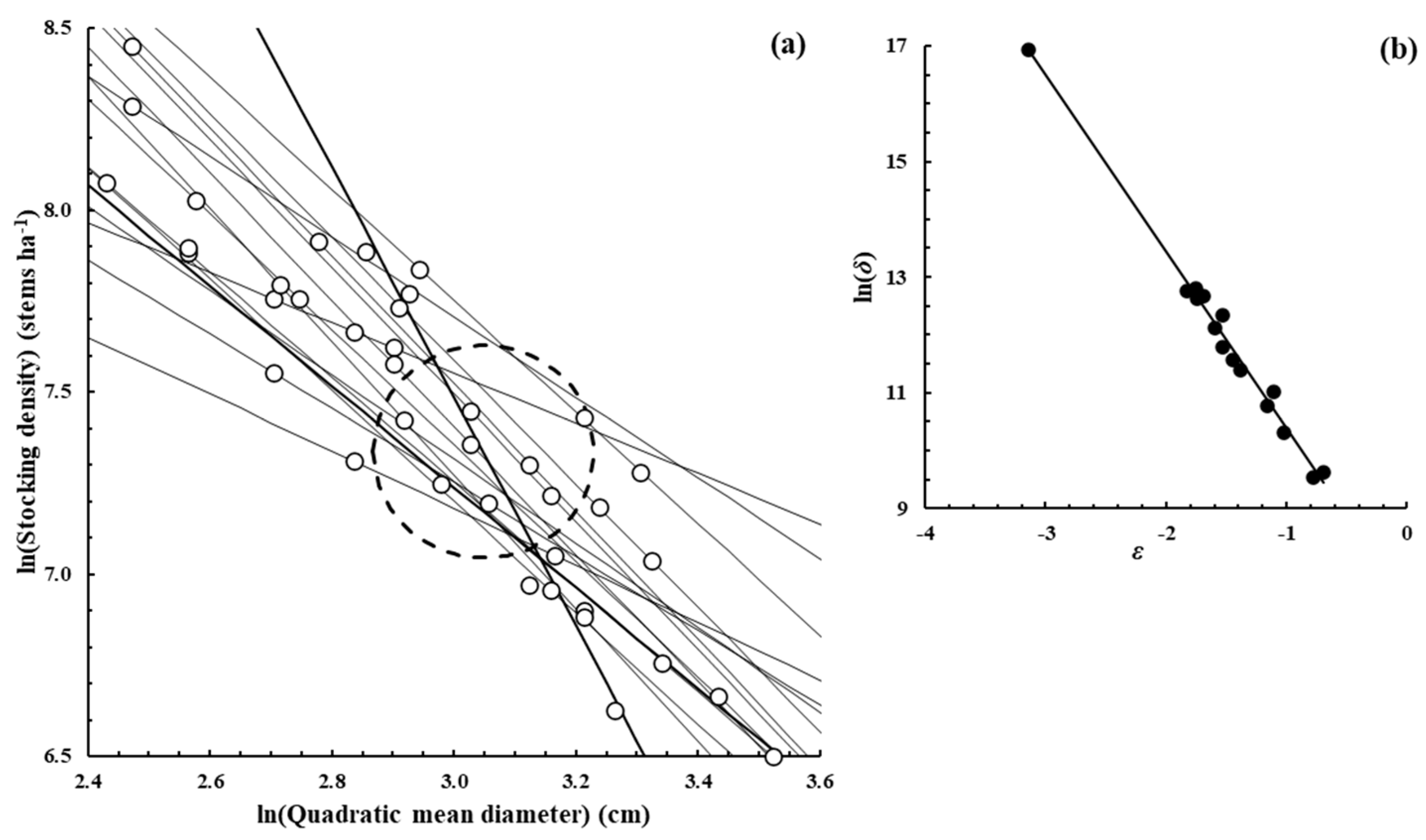
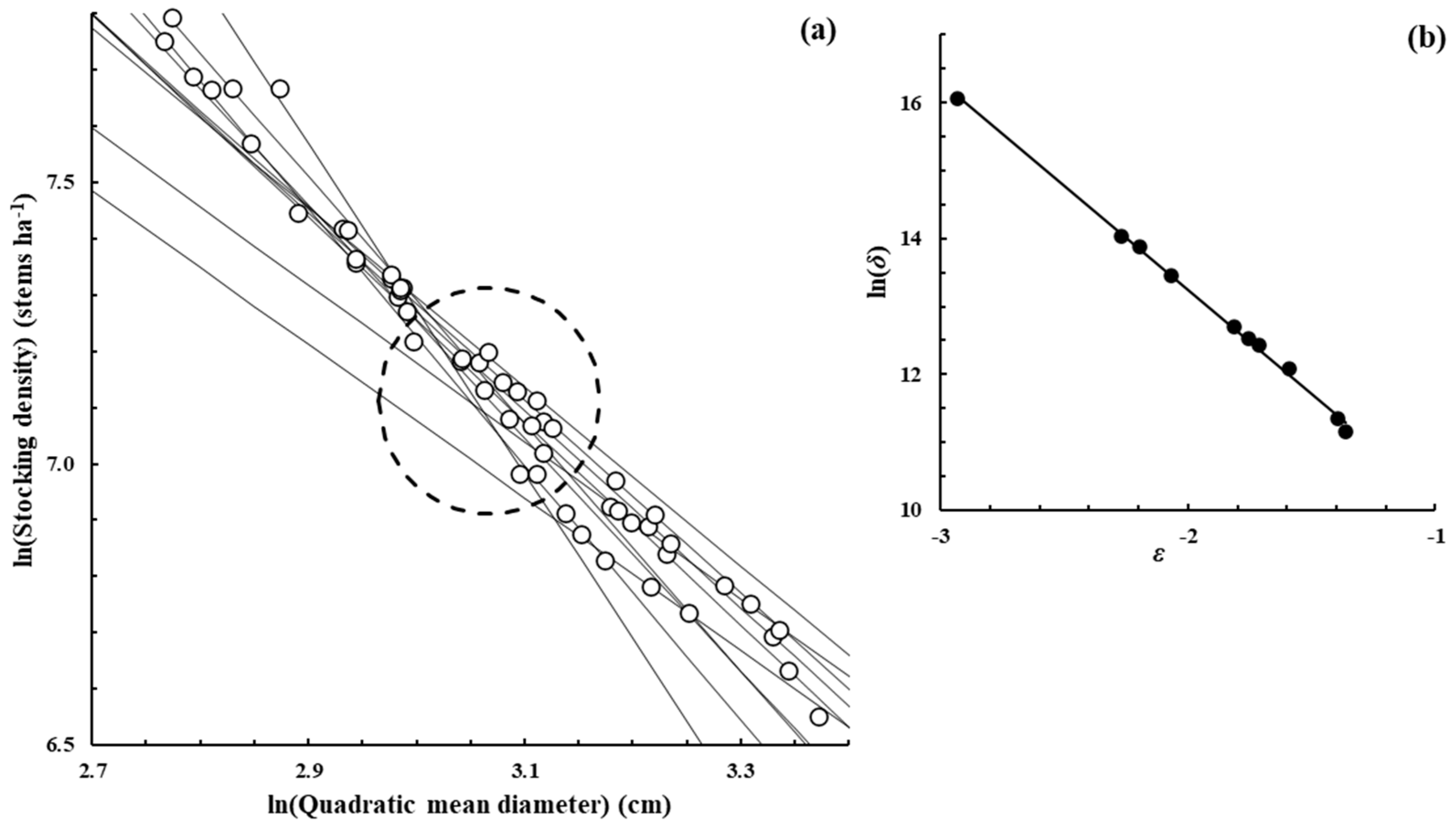
3.1. Dynamic Thinning Lines in E. obliqua Forest
3.2. What Might Determine a Common Intersection Point?
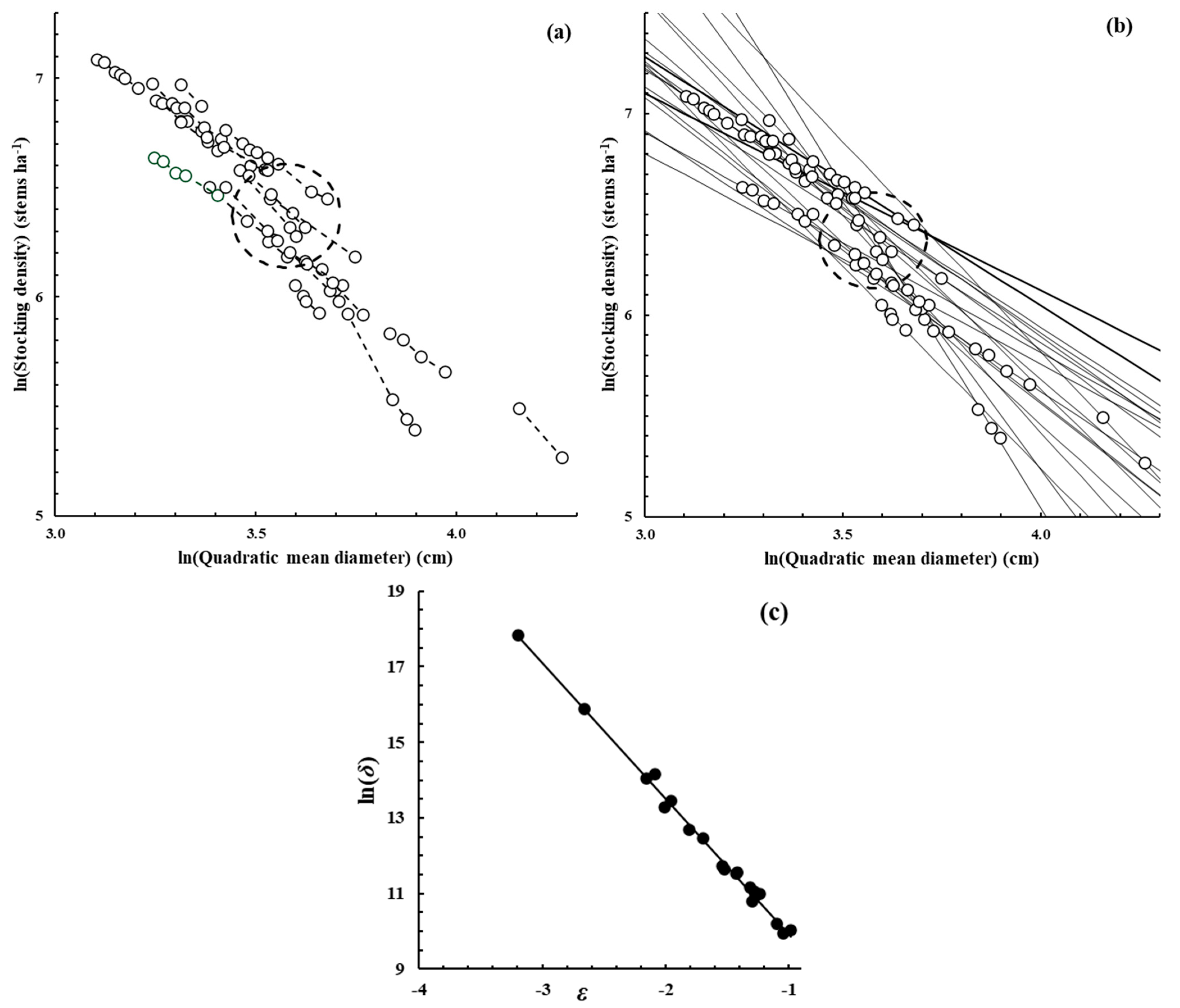
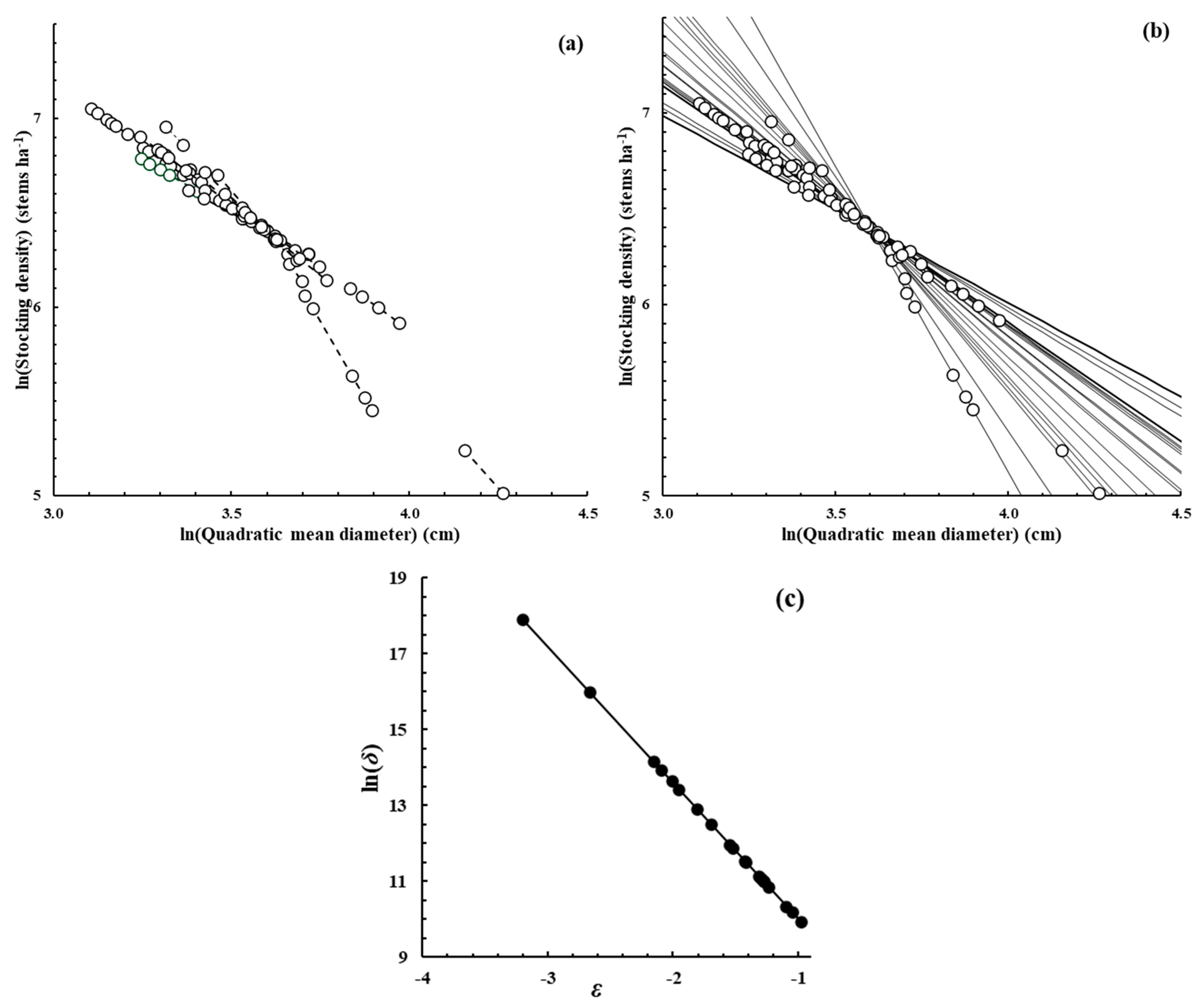
3.3. Common Intersection Points for Other Species
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
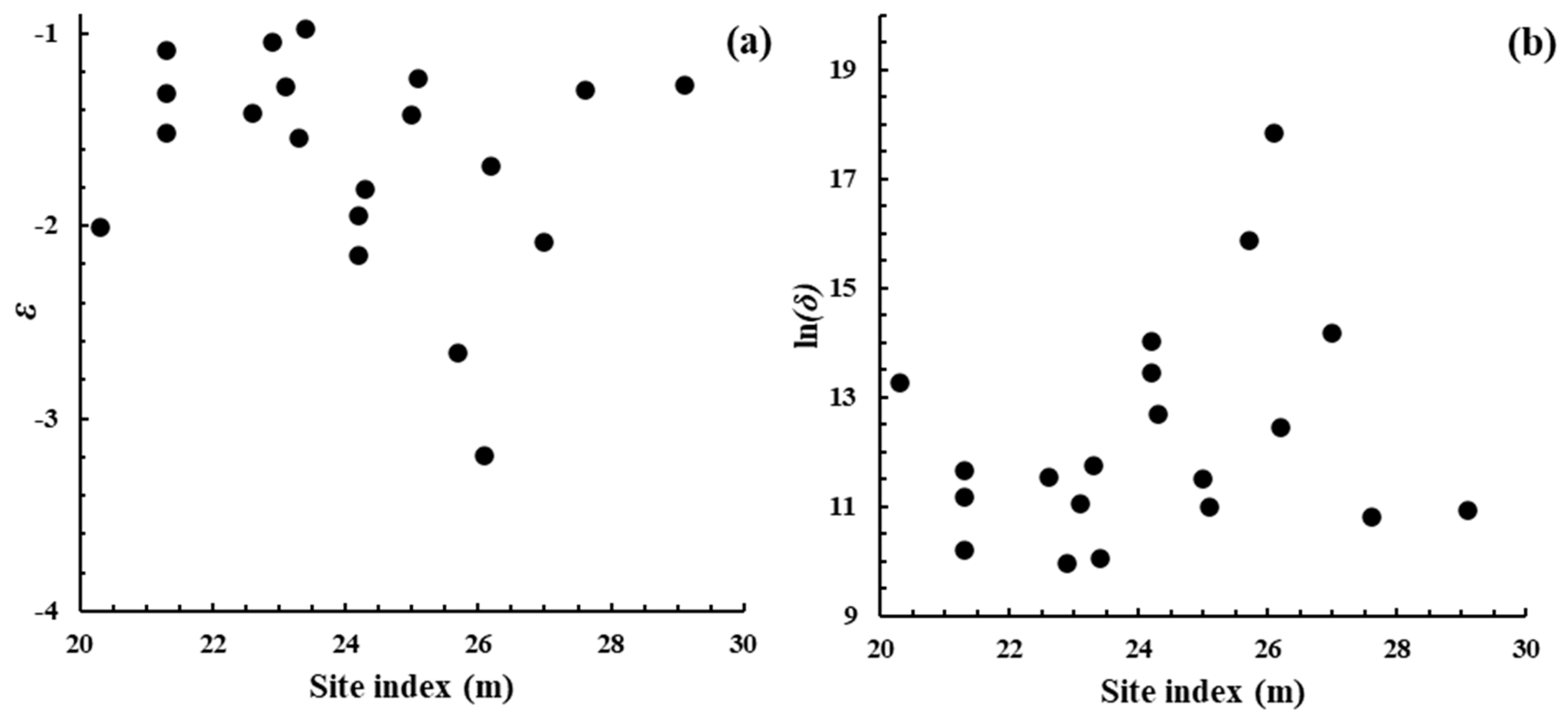
Appendix A. Site Index Effects in E. obliqua
Appendix B. Dynamic Thinning Lines of Other Species
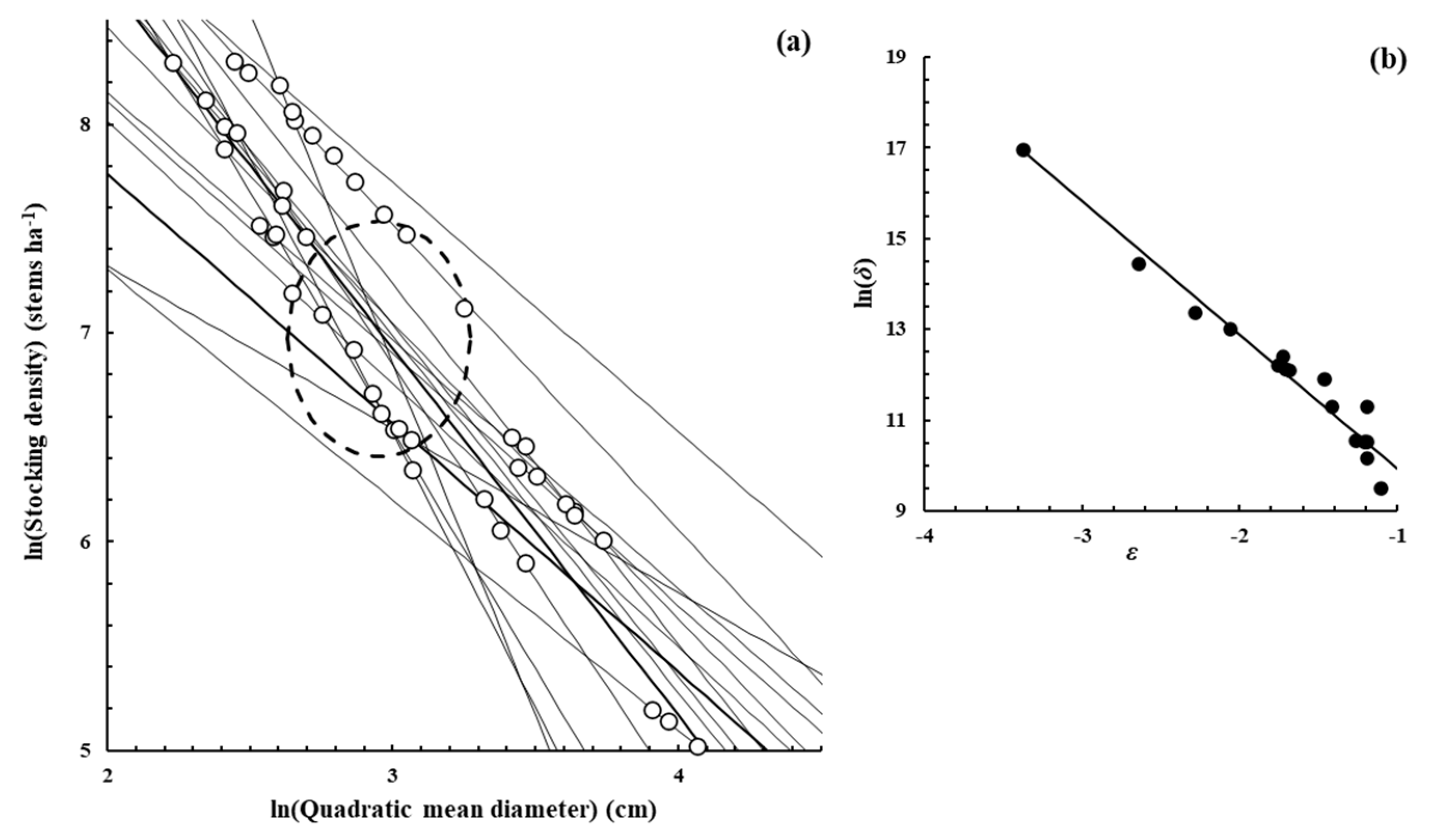
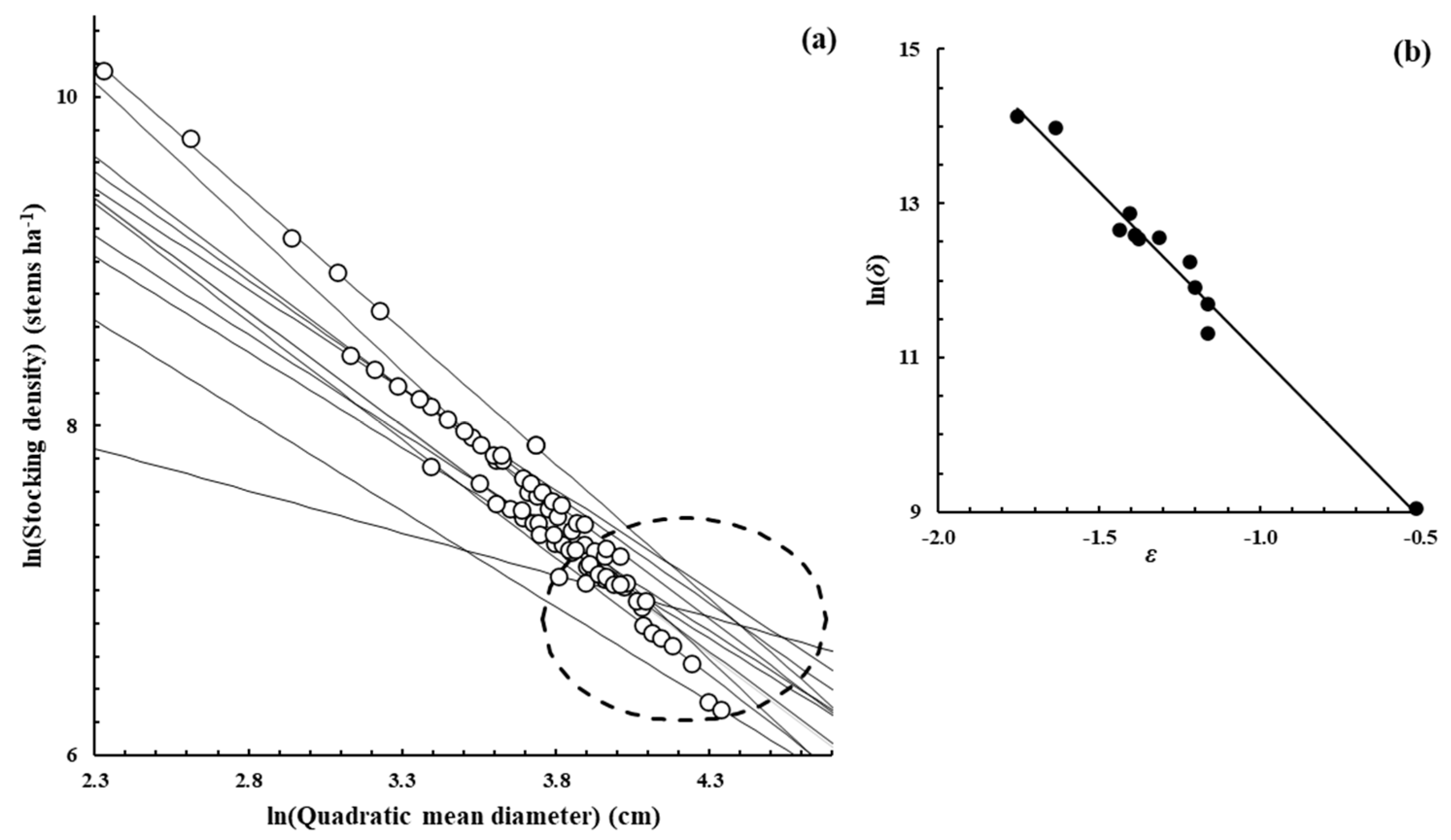
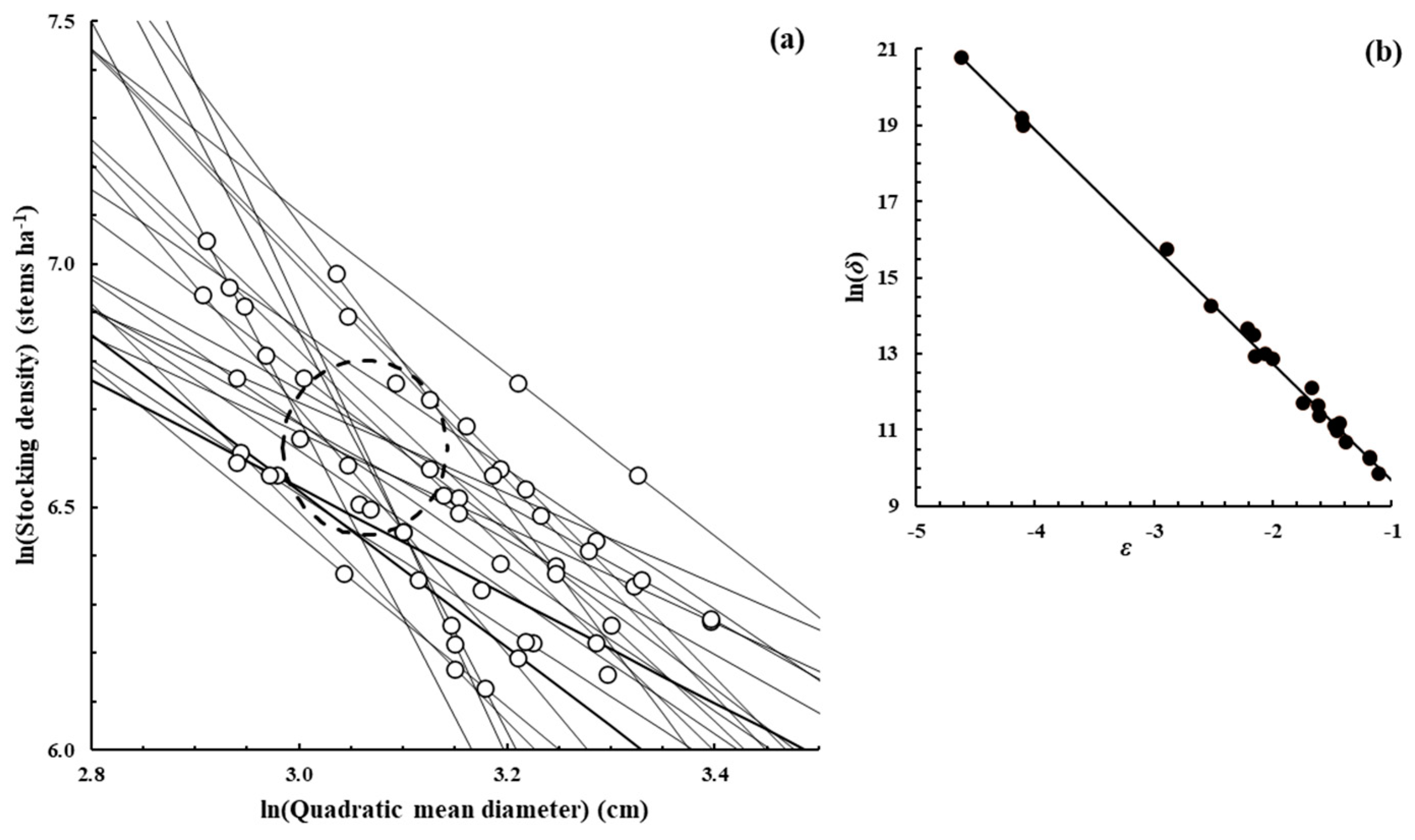

References
- Yoda, K.; Kira, T.; Ogawa, H.; Hozumi, K. Self-thinning in overcrowded pure stands under cultivated and natural conditions. J. Biol. Osaka City Univ. 1963, 14, 107–129. [Google Scholar]
- Gorham, E. Shoot height, weight and standing crop in relation to density of monospecific stands. Nature 1979, 279, 148–150. [Google Scholar] [CrossRef]
- White, J. The allometric interpretation of the self-thinning rule. J. Theor. Biol. 1981, 89, 475–500. [Google Scholar] [CrossRef]
- Reineke, L.H. Perfecting a stand-density index for even-aged forests. J. Agric. Res. 1933, 46, 627–638. [Google Scholar]
- West, P.W. Tree and Forest Measurement, 3rd ed.; Springer International Publishing: Cham, Switzerland, 2015. [Google Scholar]
- Weller, D.E. Will the real self-thinning rule stand up?—A reply to Osawa and Sugita. Ecology 1990, 71, 1204–1207. [Google Scholar] [CrossRef]
- VanderSchaaf, C.L.; Burkhart, H.E. Comparison of methods to estimate Reineke’s maximum size-density relationship species boundary line slope. For. Sci. 2007, 53, 435–442. [Google Scholar] [CrossRef]
- Pavel, M.A.A.; Barreiro, S.; Tomé, M. The importance of using permanent plots data to fit the self-thinning line: An example for maritime pine stands in Portugal. Forests 2023, 14, 1354. [Google Scholar] [CrossRef]
- Caicoya, A.T.; Biber, P.; del Rio, M.; Ruiz-Peinado, R.; Arcangeli, C.; Matthews, R.; Pretzsch, H. Self-thinning of Scots pine across Europe changes with solar radiation, precipitation and temperature but does not show trends in time. For. Ecol. Manag. 2024, 552, 121585. [Google Scholar]
- Puettmann, K.J.; Hann, D.W.; Hibbs, D.E. Evaluation of the size-density relationships for pure red alder and Douglas-fir stands. For. Sci. 1993, 39, 7–27. [Google Scholar] [CrossRef]
- Pretzsch, H.; Biber, P. A re-evaluation of Reineke’s rule and stand density index. For. Sci. 2005, 51, 304–320. [Google Scholar] [CrossRef]
- de Montigny, L.; Nigh, G. Density frontiers for even-aged Douglas-fir and western hemlock stands in coastal British Columbia. For. Sci. 2007, 53, 675–682. [Google Scholar] [CrossRef]
- Comeau, P.G.; White, M.; Kerr, G.; Hale, S.E. Maximum density-size relationships for Sitka spruce and coastal Douglas-fir in Britain and Canada. Forestry 2010, 83, 461–468. [Google Scholar] [CrossRef]
- Charru, M.; Seynave, I.; Morneau, F.; Rivoire, M.; Bontemps, J.-D. Significant differences and curvilinearity in the self-thinning relationships of 11 temperate tree species assessed from forest inventory data. Ann. For. Sci. 2012, 69, 195–205. [Google Scholar] [CrossRef]
- Brunet-Navarro, P.; Sterck, F.J.; Vayreda, J.; Martinez-Vilalta, J.; Mohren, G.M.J. Self-thinning in four pine species: An evaluation of potential climate impacts. Ann. For. Sci. 2016, 73, 1025–1034. [Google Scholar] [CrossRef]
- Riofrío, J.; del Río, M.; Bravo, F. Mixing effects on growth efficiency in mixed pine forests. Forestry 2016, 90, 381–392. [Google Scholar] [CrossRef]
- Condés, S.; Vallet, P.; Bielak, K.; Bravo-Oviedo, A.; Voll, L.; Ducey, M.J.; Pach, M.; Pretzsch, H.; Sterba, H.; Vayreda, J.; et al. Climate influences on the maximum size-density relationship in Scots pine (Pinus sylvestris L.) and European beech (Fagus sylvatica L.) stands. For. Ecol. Manag. 2017, 385, 295–307. [Google Scholar] [CrossRef]
- Aguirre, A.; del Rio, M.; Condes, S. Intra- and inter-specific variation of the maximum size-density relationship along an aridity gradient in Iberian pinewoods. For. Ecol. Manag. 2018, 411, 90–100. [Google Scholar] [CrossRef]
- Quiñonez-Barraza, G.; Tamarit-Urias, J.C.; Martínez-Salvador, M.; García-Cuevas, X.; Santos-Posadas, H.M.; Santiago-García, W. Maximum density and density management diagram for mixed-species forests in Durango, Mexico. Revista Chapingo Serie Ciencias Forestales y del Ambiente 2018, 24, 73–90. [Google Scholar] [CrossRef]
- Lee, D.; Choi, J. Evaluating maximum stand density and size-density relationships based on the Competition Density Rule in Korean pines and Japanese larch. For. Ecol. Manag. 2019, 446, 204–213. [Google Scholar] [CrossRef]
- Pretzsch, H. The effect of tree crown allometry on community dynamics in mixed-species stands versus monocultures. A review and perspectives for modeling and silvicultural regulation. Forests 2019, 10, 810. [Google Scholar] [CrossRef]
- Ningre, F.; Ottorini, J.-M.; Le Goff, N. Modeling size-density trajectories for even-aged oak (Quercus petraea Liebl.) stands and comparison with beech (Fagus silvatica L.) in France. Ann. For. Sci. 2019, 76, 73. [Google Scholar] [CrossRef]
- de Prado, D.R.; San Martín, R.; Bravo, F.; de Aza, C.H. Potential climatic influence on maximum stand carrying capacity for 15 Mediterranean coniferous and broadleaf species. For. Ecol. Manag. 2020, 460, 117824. [Google Scholar] [CrossRef]
- Donald, C.M. Competition among pasture plants. I. Interspecific competition among annual pasture plants. Aust. J. Agric. Res. 1951, 2, 355–376. [Google Scholar] [CrossRef]
- Shinozaki, K.; Kira, T. Intraspecific competition among higher plants VII. Logistic theory of the C-D effect. J. Inst. Polytech. 1956, 12, 69–82. [Google Scholar]
- Tang, S.; Meng, F.-R.; Meng, C.H. The impact of initial stand density and site index on maximum density index and self-thinning index in a stand self-thinning model. For. Ecol. Manag. 1995, 75, 61–68. [Google Scholar] [CrossRef]
- Bi, H.Q. The self-thinning surface. For. Sci. 2001, 47, 361–370. [Google Scholar] [CrossRef]
- Bi, H.Q. Stochastic frontier analysis of a classic self-thinning experiment. Austral Ecol. 2004, 29, 408–417. [Google Scholar] [CrossRef]
- Pittman, S.D.; Turnblom, E.C. A study of self-thinning using coupled allometric equations: Implications for coastal Douglas-fir stand dynamics. Can. J. For. Res. 2003, 33, 1661–1669. [Google Scholar] [CrossRef]
- Poage, N.J.; Marshall, D.D.; McClellan, M.H. Maximum stand-density index of 40 western hemlock–Sitka spruce stands in southeast Alaska. Western J. App. For. 2007, 22, 99–104. [Google Scholar] [CrossRef]
- Weiskittel, A.; Gould, P.; Temesgen, H. Sources of variation in the self-thinning boundary line for three species with varying levels of shade tolerance. For. Sci. 2009, 55, 84–93. [Google Scholar] [CrossRef]
- Schütz, J.P.; Zingg, A. Improving estimations of maximal stand density by combining Reineke’s size-density rule and the yield level, using the example of spruce (Picea abies (L.) Karst.) and European Beech (Fagus sylvatica L.). Ann. For. Sci. 2010, 67, 507. [Google Scholar] [CrossRef]
- Reyes-Hernandez, V.; Comeau, P.G.; Bokalo, M. Static and dynamic maximum size-density relationships for mixed trembling aspen and white spruce stands in western Canada. For. Ecol. Manag. 2013, 289, 300–311. [Google Scholar] [CrossRef]
- Zhang, J.W.; Oliver, W.W.; Powers, R.F. Reevaluating the self-thinning boundary line for ponderosa pine (Pinus ponderosa) forests. Can. J. For. Res. 2013, 43, 963–971.9604. [Google Scholar] [CrossRef]
- Zhang, X.; Cao, Q.V.; Duan, A.; Zhan, G.J. Self-thinning trajectories of Chinese fir plantations in Southern China. For. Sci. 2016, 62, 594–599. [Google Scholar] [CrossRef]
- Zhang, X.Q.; Lu, L.L.; Cao, Q.V.; Duan, A.G.; Zhang, J.G. Climate-sensitive self-thinning trajectories of Chinese fir plantations in south China. Can. J. For. Res. 2018, 48, 1388–1397. [Google Scholar] [CrossRef]
- Kweon, D.; Comeau, P.G. Effects of climate on maximum size-density relationships in Western Canadian trembling aspen stands. For. Ecol. Manag. 2017, 406, 281–289. [Google Scholar] [CrossRef]
- Forrester, D.I.; Baker, T.G.; Elms, S.R.; Hobi, M.L.; Ouyang, S.; Wiedmann, J.C.; Xiang, W.; Zell, J.; Pulkkinen, M. Self-thinning tree mortality models that account for vertical stand structure, species mixing and climate. For. Ecol. Manag. 2021, 487, 118936. [Google Scholar] [CrossRef]
- Le Goff, N.; Ningre, F.; Ottorini, J.M. Modeling size-density trajectories of even-aged ash (Fraxinus excelsior L.) stands in France. A baseline to assess the impact of Chalara ash dieback. Ann. For. Sci. 2021, 78, 3. [Google Scholar] [CrossRef]
- Marqués, L.; Weng, E.; Bugmann, H.; Forrester, D.I.; Rohner, B.; Hobi, M.L.; Trotsiuk, V.; Stocker, B.D. Tree growth enhancement drives a persistent biomass gain in unmanaged temperate forest. AGU Adv. 2023, 4, e2022AV000859. [Google Scholar] [CrossRef]
- Dong, L.B.; Chen, G.M.; Chung, W.; Liu, Z.G. Variations on the maximum density-size lines to climate and site factors for Larix spp. plantations in northeast China. Ecol. Model. 2024, 498, 110913. [Google Scholar] [CrossRef]
- Lee, D.; Siipilehto, J.; Hynynen, J. Comparison and analysis of self-thinning models based on diameter-based maximum size-density relationships. For. Ecol. Manag. 2025, 575, 122374. [Google Scholar] [CrossRef]
- Mrad, A.; Manzoni, S.; Oren, R.; Vico, G.; Lindh, M.; and Katul, G. Recovering the metabolic self-thinning, and constant final yield rules in mono-specific stands. Front. For. Glob. Chang. 2020, 3, 62. [Google Scholar] [CrossRef]
- Avery, T.E.; Burkhart, H.E. Forest Measurements, 5th ed.; McGraw Hill: New York, NY, USA, 2002. [Google Scholar]
- Burkhart, H.E. Comparison of maximum size-density relationships based on alternate stand attributes for predicting tree numbers and stand growth. For. Ecol. Manag. 2013, 289, 404–408. [Google Scholar] [CrossRef]
- Mattay, J.P.; West, P.W. A Collection of Growth and Yield Data from Eight Eucalypt Species Growing in Even-Aged, Monoculture Forest. CSIRO Division of Forestry, User Series No 18; Canberra. 1994. Available online: http://sciwest.byethost6.com/Mattay&West1994EucalyptdataCSIRO.pdf (accessed on 15 December 2024).
- West, P.W.; Mattay, J.P. Yield prediction models and comparative growth rates for six eucalypt species. Aust. For. 1993, 56, 211–225. [Google Scholar] [CrossRef]
- Bacon, D.W.; Watts, D.G. Estimating the transition between two intersecting straight lines. Biometrika 1971, 58, 525–534. [Google Scholar] [CrossRef]
- Deleuze, C.; Pain, O.; Dhôte, J.-F.; Hervé, J.-C. A flexible radial increment model for individual trees in pure even-aged stands. Ann. For. Sci. 2004, 61, 327–335. [Google Scholar] [CrossRef][Green Version]
- West, P.W.; Ratkowsky, D.A. Models relating individual tree basal area growth rates to tree basal areas in even-aged, monoculture forest stands. J. For. 2022, 9, 21–38. [Google Scholar] [CrossRef]
- Trouvé, R.; Nitschke, C.R.; Robinson, A.P.; Baker, P.J. Estimating the self-thinning line from mortality data. For. Ecol. Manag. 2017, 402, 122–134. [Google Scholar] [CrossRef]
- Ningre, F.; Ottorini, J.-M.; Le Goff, N. Modelling size-density trajectories for even-aged beech (Fagus sylvatica L.) stands in France. Ann. For. Sci 2016, 73, 765–776. [Google Scholar] [CrossRef]
- Salas-Eljatib, C.; Weiskittel, A.R. Evaluation of modeling strategies for assessing self-thinning behavior and carrying capacity. Ecol. Evol. 2018, 8, 10768–10779. [Google Scholar] [CrossRef]
- VanderSchaaf, C.L. Estimating individual stand size-density trajectories and a maximum size-density relationship species boundary line slope. For. Sci. 2010, 56, 327–335. [Google Scholar] [CrossRef]
- Gower, S.T.; McMurtrie, R.E.; Murty, D. Aboveground net primary production decline with stand age: Potential causes. Trends Ecol. Evol. 1996, 11, 378–382. [Google Scholar] [CrossRef] [PubMed]
- Ryan, M.G.; Binkley, D.; Fownes, J.H. Age-related decline in forest productivity: Pattern and process. Adv. Ecol. Res. 1997, 27, 213–262. [Google Scholar]
- Davis, L.S.; Johnson, K.N.; Bettinger, P.S.; Howard, T.E. Forest Management, 4th ed.; McGraw-Hill: New York, NY, USA, 2001. [Google Scholar]
- Skovsgaard, J.P.; Vanclay, J.K. Forest site productivity: A review of spatial and temporal variability in natural site conditions. Forestry 2013, 86, 305–315. [Google Scholar] [CrossRef]
- Binkley, D. Acorn review: The persistent mystery of declining growth in older forests. For. Ecol. Manag. 2023, 538, 121004. [Google Scholar] [CrossRef]
- Pretzsch, H.; del Rio, M.; Arcangeli, C.; Bielak, K.; Dudzinska, M.; Forrester, D.I.; Kohnle, U.; Ledermann, T.; Matthews, R.; Nagel, R.; et al. Competition-based mortality and tree losses. An essential component of net primary productivity. For. Ecol. Manag. 2023, 544, 21204. [Google Scholar] [CrossRef]
- West, P.W. A review of the growth behaviour of stands and trees in even-aged, monospecific forest. Ann. For. Sci. 2024, 81, 34. [Google Scholar] [CrossRef]
- West, P.W. Do increasing respiratory costs explain the decline with age in forest growth rate? J. For. Res. 2020, 31, 693–712. [Google Scholar] [CrossRef]
| Species | Location | Number of Plots | Number of Measurements | Age (Years) | Data Source |
|---|---|---|---|---|---|
| Eucalyptus obliqua | Tasmania, Australia | 20 | 81 | 32–53–89 | Present work |
| Eucalyptus camaldulensis | Victoria, Australia | 17 | 47 | 9–32–70 | [51] |
| Fagus sylvatica | Northwest France | 17 | 105 | 15–33–39 | [22,52] |
| Quercus petraea | Northwest France | 12 | 75 | 14–29–38 | [22] |
| Populus tremuloides | Boreal regions, Canada | 22 | 54 | - | [37] |
| Nothofagus spp. | Southcentral Chile | 16 | 39 | - | [53] |
| Pinus pinaster | Portugal | 9 | 40 | 24–33–50 | [8] |
| Pinus taeda | Mississippi, USA | 10 | 54 | 8–18–30 | [54] |
| Species | Correlation Coefficient | ln(N0) | ln(D0) |
|---|---|---|---|
| Eucalyptus obliqua | −0.997 | 6.37 (6.14–6.61) | 3.58 (3.44–3.71) |
| Eucalyptus camaldulensis | −0.981 | 6.97 (6.41–7.54) | 2.95 (2.63–3.27) |
| Fagus sylvatica | −0.959 | 6.27 (5.42–7.11) | 4.88 (4.09–5.68) |
| Quercus petraea | −0.988 | 6.82 (6.21–7.44) | 4.22 (3.76–4.68) |
| Populus tremuloides | −0.998 | 6.62 (6.44–6.80) | 3.06 (2.98–3.14) |
| Nothofagus spp. | −0.995 | 7.34 (7.04–7.63) | 3.05 (2.87–3.23) |
| Pinus pinaster | −0.987 | 7.92 (7.23–8.61) | 2.75 (2.35–3.14) |
| Pinus taeda | −0.999 | 7.11 (6.91–7.31) | 3.07 (2.97–3.17) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
West, P.W.; Ratkowsky, D.A. Do Stands Self-Thin Through a Common Point? An Additional Concept for the Self-Thinning Rule. Forests 2025, 16, 199. https://doi.org/10.3390/f16020199
West PW, Ratkowsky DA. Do Stands Self-Thin Through a Common Point? An Additional Concept for the Self-Thinning Rule. Forests. 2025; 16(2):199. https://doi.org/10.3390/f16020199
Chicago/Turabian StyleWest, P. W., and D. A. Ratkowsky. 2025. "Do Stands Self-Thin Through a Common Point? An Additional Concept for the Self-Thinning Rule" Forests 16, no. 2: 199. https://doi.org/10.3390/f16020199
APA StyleWest, P. W., & Ratkowsky, D. A. (2025). Do Stands Self-Thin Through a Common Point? An Additional Concept for the Self-Thinning Rule. Forests, 16(2), 199. https://doi.org/10.3390/f16020199







