Genetic Parameter Estimates for the Growth and Morphological Traits of Castanopsis hystrix Families and the Genotype × Environment Interaction Effects
Abstract
:1. Introduction
2. Materials and Methods
2.1. C. hystrix Families and Layout of the Experimental Forests
2.2. Overview of the Experimental Sites
2.3. Determination of Traits
2.4. Data Analysis
2.4.1. Estimation of Genetic Parameters
Estimations of Single-Location Genetic Variance and Heritability
Estimation of Multiple-Location Genetic Variance and Heritability
Estimation of the Genetic Coefficient of Variances and Environmental Coefficient Variances
Estimation of Genetic Gains
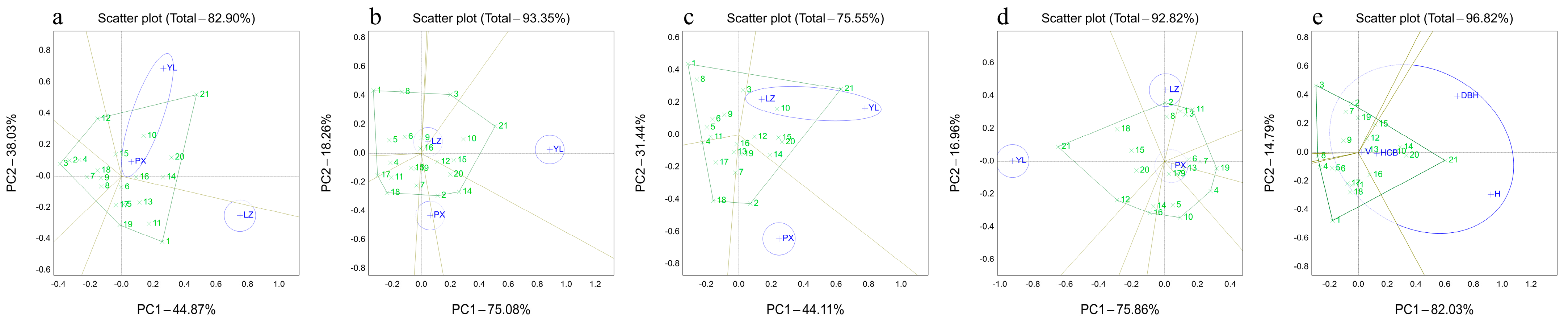
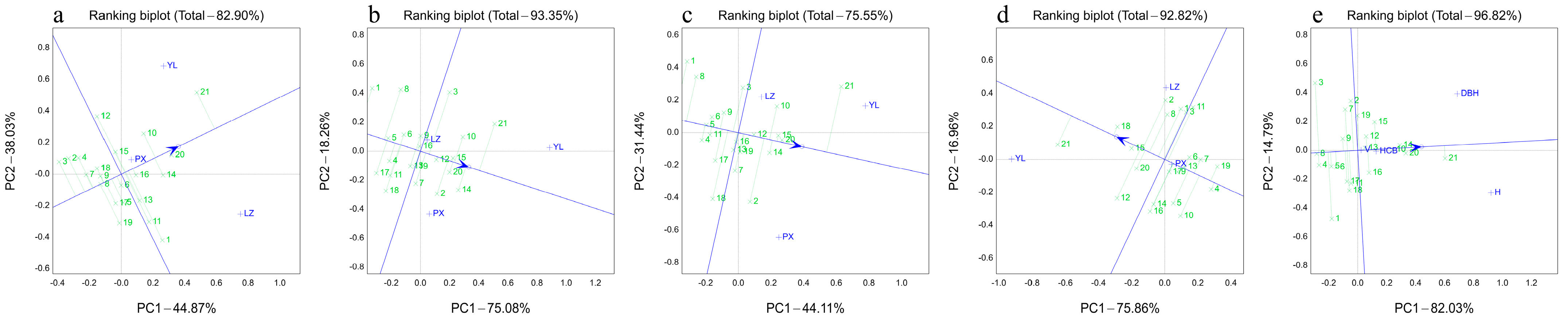
2.4.2. BLUP–GGE Analysis of the Growth and Morphological Traits
3. Results
3.1. Genetic Variances in the Growth Traits of C. hystrix
3.2. Genetic Variances in the Morphological Traits of C. hystrix
3.3. Estimating the Genetic Parameters for the Growth and Morphological Traits of C. hystrix
3.4. BLUP
3.5. GGE-BLUP
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, Y.J.; Suontama, M.; Burdon, R.D.; Dungey, H.S. Genotype by environment interactions in forest tree breeding: Review of methodology and perspectives on research and application. Tree Genet. Genomes 2017, 13, 60. [Google Scholar]
- Lee, K.; Kim, I.S.; Lee, S.W. Estimation of genetic parameters on growth characteristics of a 35-year-old Pinus koraiensis progeny trial in South Korea. J. For. Res. 2020, 32, 2227–2236. [Google Scholar]
- Munhoz, L.; Biernaski, F.; Peres, F.; Dias, A.; Tabarussi, E. Predicted genetic gains for growth traits and genotype × environment interaction in Pinus greggii: New perspectives for genetic improvement in Brzzil. An. Da Acad. Bras. De Ciencisa 2021, 93 (Suppl. S3), e20101486. [Google Scholar]
- Baltunis, B.S.; Brawner, J.T. Clonal stability in Pinus radiata across New Zealand and Australia 1: Growth and form traits. New For. 2010, 40, 305–322. [Google Scholar]
- Suontama, M.; Low, C.B.; Stovold, G.T.; Miller, M.A.; Fleet, K.R.; Li, Y.; Dungey, H.S. Genetic parameters and genetic gains across three breeding cycles for growth and form traits of Eucalyptus regnans in New Zealand. Tree Genet. Genomes 2015, 11, 133. [Google Scholar]
- Yin, M.Y.; Guo, J.J.; Wang, C.S.; Zhao, Z.G.; Zeng, J. Genetic parameter estimates and genotype × environment interactions of growth and quality traits for Betula alnoides Buch.-Ham. Ex, D. Don in four provenance-family trials in Southern China. Forests 2019, 10, 1036. [Google Scholar]
- Shelbourne, C.J. Genotype-Environment Interaction: Its Study and Its Implications in Forest Tree Improvement; The IUFRO Genetics and SABRAO Joint Symposium: Tokyo, Japan, 1972; pp. 1–28. [Google Scholar]
- Zas, R.; Merlo, E.; Fernández-López, J. Genotype by environment interaction in maritime pine families in Galicia, Northwest Spain. Silvae Genet. 2004, 53, 175–182. [Google Scholar]
- Skrøppa, T. A critical evaluation of methods available to estimate the genotype × environment interaction. Stud. For. Suec. 1982, 166, 3–14. [Google Scholar]
- Burdon, R.D. Genetic correlation as a concept for studying genotype-environment interaction in forest tree breeding. Silvae Genetica. 1977, 26, 168–175. [Google Scholar]
- Richard, C. Statistical analysis of regional yield trials: AMMI analysis of factorial designs. Agric. Syst. 1996, 51, 242–244. [Google Scholar]
- Yan, W.K.; Hunt, L.A.; Sheng, Q.L.; Szlavnics, Z. Cultivar Evaluation and Mega-Environment Investigation Based on the GGE Biplot. Crop Sci. 2000, 40, 597–605. [Google Scholar]
- Cullis, B.R.; Smith, A.B.; Coombes, N.E. On the design of early generation variety trials with correlated data. J. Agric. Biol. Environ. Stat. 2006, 11, 381–393. [Google Scholar]
- Ling, J.; Xiao, Y.; Hu, J.; Wang, F.; Ouyang, F.; Wang, J.; Weng, Y.; Zhang, H. Genotype by environment interaction analysis of growth of Picea koraiensis families at different sites using BLUP-GGE. New For. 2021, 52, 113–127. [Google Scholar] [CrossRef]
- Li, Y.X.; Zhang, X.X.; Zhang, Q.H.; Jiang, L.P.; Han, R.; Sun, S.Q.; Hu, X.Q.; Pei, X.N.; Zhan, C.L.; Zhao, X.Y. G × E analysis of growth traits of Betula platyphylla clones at three separated sites in Northeastern China. Phyton-Int. J. Exp. Bot. 2022, 91, 2055–2068. [Google Scholar]
- Li, N.; Yang, Y.M.; Xu, F.; Chen, X.Y.; Wei, R.Y.; Li, Z.Y.; Pan, W.; Zhang, W.H. Genetic diversity and population structure analysis of Castanopsis hystrix and construction of a core collection using phenotypic traits and molecular markers. Genes 2022, 13, 2383. [Google Scholar] [CrossRef]
- Jiang, J.H.; Lv, J.X. Preliminary research of Castanopsis hystrix and Betula alnoides plantation wood applied in furniture and decoration. Sci. Silvae Sin. 2008, 44, 136–140, (In Chinese with English Abstract). [Google Scholar]
- Zhu, J.Y.; Shen, W.H.; Jiang, Y.; Lu, L.H.; Tan, Y.B.; Liu, X. Genetic variation and superior family selection of Castanopsis hystrix families. J. Trop. Subtrop. Bot. 2014, 22, 270–280, (In Chinese with English Abstract). [Google Scholar]
- Fang, B.J. Analysis and selection of genetic variation on growth traits among provenances/families of Castanopsis hystrix. Subtrop. Agric. Res. 2021, 17, 22–29, (In Chinese with English Abstract). [Google Scholar]
- Fu, L.Y.; Sharma, R.P.; Hao, K.J.; Tang, S.Z. A generalized interregional nonlinear mixed-effects crown width model for Prince Rupprecht larch in northern China. For. Ecol. Manag. 2017, 389, 364–373. [Google Scholar]
- Fu, L.Y.; Sun, H.; Sharma, R.P.; Lei, Y.C.; Zhang, H.R.; Tang, S.Z. Nonlinear mixed-effects crown width models for individual trees of Chinese fir (Cunninghamia lanceolata) in south-central China. For. Ecol. Manag. 2013, 302, 210–220. [Google Scholar]
- Pang, L.F.; Ma, Y.P.; Sharma, R.P.; Rice, S.; Song, X.; Fu, L. Developing an improved parameter estimation method for the segmented taper equation through combination of constrained two-dimensional optimum seeking and least square regression. Forests 2016, 7, 194. [Google Scholar] [CrossRef] [Green Version]
- Bian, L.M.; Shi, J.S.; Zheng, R.H.; Chen, J.H.; Wu, H.X. Genetic parameters and genotype-environment interactions of Chinese fir (Cunninghamia lanceolata) in Fujian province. Can. J. For. Res. 2014, 44, 582–592. [Google Scholar] [CrossRef]
- Zhao, X.Y.; Xia, H.; Wang, X.W.; Wang, C.; Liang, D.Y.; Li, K.L.; Liu, G.F. Variance and stability analysis of growth characters in half-sib Betula platyphylla families at three different sites in China. Euphytica 2016, 208, 173–186. [Google Scholar] [CrossRef]
- Murillo, O.; Resende, V.; Badilla, Y.; Gamboa, J.P. Genotype by environment interaction and teak (Tectona grandis L.) selection in Costa Rica. Silvae Genet. 2019, 68, 116–121. [Google Scholar] [CrossRef] [Green Version]
- Diao, S.; Hou, Y.M.; Xie, Y.H.; Sun, X.M. Age trends of genetic parameters, early selection and family by site interactions for growth traits in Larix kaempferi open-pollinated families. BMC Genet. 2016, 17, 104–115. [Google Scholar] [CrossRef] [Green Version]
- Souza, B.M.; Aguiar, A.V.D.; Dambrat, H.M.; Galucha, S.C.; Tambarussi, E.V.; Sestrem, M.S.C.D.S.; Tomigian, D.S.; Freitas, M.L.M.; Venson, I.; Torres-Dini, D. Effects of previous land use on genotype-by-environment interactions in two loblolly pine progeny tests. For. Ecol. Manag. 2022, 503, 119762. [Google Scholar] [CrossRef]
- Jiang, Y.; Xiao, Y.Q. Survey on natural factor in original habitat of Castanopsis hystrix of native forest in Guangxi. Guangxi For. Sci. 2007, 36, 202–205, (In Chinese with English Abstract). [Google Scholar]
- Liu, G.J.; Jia, H.Y.; Xu, J.M.; Niu, C.H.; Zeng, J.; Lan, G.; Zhu, M.F.; Li, W.Z. Effects of soil physicochemical properties on growth and heartwood formation of Castanopsis hystrix plantation. For. Res. 2021, 34, 88–97, (In Chinese with English Abstract). [Google Scholar]
- Krishnamoorthy, M.; Palanisamy, K.; Francis, A.P.; Girrrsan, K. Impact of environmental factors and altitude on growth and reproductive characteristics of teak (Tectona grandis Linn. f.) in Southern India. J. For. Environ. Sci. 2016, 32, 353–366. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Xue, J.; Clinton, P.W.; Dungey, H.S. Genetic parameters and clone by environment interactions for growth and foliar nutrient concentrations in radiate pine on 14 widely diverse New Zealand sites. Tree Genet. Genomes 2015, 11, 10. [Google Scholar] [CrossRef]
- Vargas-Hernandez, J.J.; Adams, W.T.; Joyce, D.G. Quantitative genetic structure of stem form and branching traits in Douglas fir seedlings and implications for early selection. Silvae Genatica 2003, 52, 36–44. [Google Scholar]
- Yuan, C.Z.; Zhang, Z.; Jin, G.Q.; Zheng, Y.; Zhou, Z.C.; Sun, L.S.; Tong, H.B. Genetic parameters and genotype by environment interactions influencing growth and productivity in Masson pine in east and central China. For. Ecol. Manag. 2021, 487, 118991. [Google Scholar] [CrossRef]
| Family Codes | Provenance | Latitude (N) | Longitude (E) | Altitude (m) | Average Annual Temperature (°C) | Average Annual Rainfall (mm) |
|---|---|---|---|---|---|---|
| 1 | Bobai | 22°16′–22°28′ | 109°32′–111°17′ | 290 | 21.9 | 1756.0 |
| 2–7 | Donglan | 24°10′–24°30′ | 107°05′–107°43′ | 294 | 20.1 | 1546.0 |
| 8–13 | Pubei | 22°14′–22°41′ | 109°13′–109°14′ | 230 | 21.5 | 1757.4 |
| 14–18 | Rong County | 22°28′–23°08′ | 110°15′–110°53′ | 214 | 21.3 | 1660.0 |
| 19–21 | PX | 21°51′–22°08′ | 106°41′–106°59′ | 300 | 21.3 | 1376.5 |
| Experimental Site | Latitude (N) | Longitude (E) | Altitude (m) | Soil (g/kg) | Temperature (°C) | Average Annual Rainfall (mm) | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| pH | Hydrolysis (N) | Fast-Acting (p) | Fast-Acting (K) | Average | Extreme Low | Extreme High | Cumulative (>10 °C) | |||||
| YL | 22°48′ | 110°28′ | 178 | 4.10 | 157.8 | 1.65 | 22.12 | 22.7 | −2.3 | 36.8 | 7175.7 | 1698.9 |
| LZ | 24°13′ | 109°07′ | 149 | 6.07 | 78.06 | 3.60 | 31.26 | 21.9 | −8.0 | 38.9 | 6642.9 | 1345.0 |
| PX | 22°17′ | 106°42′ | 550 | 4.25 | 160.05 | 2.52 | 20.37 | 20.2 | 6.2 | 34.2 | 7575.5 | 1376.5 |
| Site | Source | Degrees of Freedom | Tree Height | DBH | V | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Sum of Squares | F | p | Sum of Squares | F | p | Sum of Squares | F | p | |||
| YL | Block | 2 | 33.14 | 4.13 * | 0.0018 | 131.00 | 6.07 ** | 0.003 | 0.073 | 6.46 ** | 0.002 |
| Family | 20 | 31.71 | 3.94 ** | <0.001 | 59.91 | 2.78 ** | <0.001 | 0.031 | 2.75 ** | <0.001 | |
| Interaction effect | 40 | 12.22 | 1.52 * | 0.041 | 32.00 | 1.48 | 0.052 | 0.002 | 1.36 | 0.103 | |
| Error | 126 | 8.02 | 21.57 | 0.011 | |||||||
| Total | 188 | 11.70 | 29.03 | 0.015 | |||||||
| LZ | Block | 2 | 86.30 | 11.26 ** | <0.001 | 13.79 | 6.78 ** | 0.002 | 0.038 | 9.29 ** | <0.001 |
| Family | 20 | 34.84 | 4.55 ** | <0.001 | 6.43 | 3.16 ** | <0.001 | 0.015 | 3.76 ** | <0.001 | |
| Interaction effect | 40 | 13.93 | 1.82 ** | 0.007 | 4.9 | 2.01 ** | 0.002 | 0.007 | 1.83 ** | 0.006 | |
| Error | 126 | 7.67 | 2.03 | 0.004 | |||||||
| Total | 188 | 12.72 | 3.06 | 0.006 | |||||||
| PX | Block | 2 | 20.25 | 2.45 ** | 0.002 | 344.12 | 20.36 ** | <0.001 | 0.052 | 1.70 * | 0.002 |
| Family | 20 | 145.22 | 17.6 ** | <0.001 | 30.35 | 1.80 ** | 0.030 | 0.784 | 26.62 ** | <0.001 | |
| Interaction effect | 40 | 9.70 | 1.18 | 0.254 | 22.65 | 1.34 | 0.120 | 0.037 | 1.22 | 0.254 | |
| Error | 126 | 14.95 | 20.94 | 0.030 | |||||||
| Total | 188 | 14.76 | 13.31 | 0.036 | |||||||
| Family | Tree Height | DBH | V | ||||||
|---|---|---|---|---|---|---|---|---|---|
| YL | LZ | PX | YL | LZ | PX | YL | LZ | PX | |
| 1 | 10.54 | 20.80 | 16.87 | 10.96 | 15.28 | 18.84 | 0.05 | 0.24 | 0.22 |
| 2 | 12.39 | 14.37 | 20.37 | 15.93 | 13.38 | 24.81 | 0.13 | 0.10 | 0.48 |
| 3 | 12.58 | 14.04 | 15.89 | 17.18 | 13.92 | 19.26 | 0.17 | 0.10 | 0.24 |
| 4 | 12.91 | 14.94 | 17.94 | 12.27 | 12.94 | 22.51 | 0.08 | 0.11 | 0.33 |
| 5 | 11.66 | 18.09 | 16.93 | 12.13 | 14.67 | 21.63 | 0.07 | 0.18 | 0.33 |
| 6 | 12.47 | 17.67 | 17.14 | 13.42 | 13.97 | 21.37 | 0.09 | 0.16 | 0.31 |
| 7 | 12.08 | 15.61 | 19.01 | 14.19 | 14.63 | 24.40 | 0.11 | 0.14 | 0.43 |
| 8 | 12.29 | 16.17 | 15.89 | 13.26 | 14.80 | 18.96 | 0.08 | 0.14 | 0.22 |
| 9 | 12.37 | 16.67 | 18.39 | 14.73 | 13.83 | 21.64 | 0.11 | 0.14 | 0.30 |
| 10 | 15.23 | 18.33 | 19.26 | 18.07 | 15.00 | 21.67 | 0.20 | 0.17 | 0.31 |
| 11 | 11.00 | 19.73 | 18.49 | 12.22 | 14.00 | 23.75 | 0.07 | 0.19 | 0.37 |
| 12 | 15.23 | 15.17 | 18.77 | 15.92 | 14.22 | 22.98 | 0.16 | 0.12 | 0.34 |
| 13 | 11.83 | 18.86 | 19.00 | 13.76 | 15.50 | 23.58 | 0.10 | 0.20 | 0.41 |
| 14 | 13.46 | 19.62 | 19.96 | 17.61 | 13.78 | 25.08 | 0.15 | 0.20 | 0.45 |
| 15 | 13.77 | 16.73 | 19.28 | 17.24 | 14.28 | 23.11 | 0.19 | 0.15 | 0.39 |
| 16 | 12.93 | 18.20 | 20.04 | 14.60 | 14.67 | 22.19 | 0.11 | 0.17 | 0.38 |
| 17 | 11.02 | 17.60 | 20.56 | 11.08 | 14.94 | 23.38 | 0.07 | 0.17 | 0.40 |
| 18 | 12.31 | 16.01 | 22.24 | 11.89 | 13.28 | 25.14 | 0.06 | 0.12 | 0.51 |
| 19 | 10.20 | 18.17 | 20.03 | 14.09 | 15.98 | 24.11 | 0.11 | 0.19 | 0.43 |
| 20 | 14.73 | 19.74 | 19.03 | 16.88 | 15.11 | 24.03 | 0.18 | 0.21 | 0.42 |
| 21 | 18.41 | 19.98 | 19.50 | 20.53 | 16.06 | 22.37 | 0.29 | 0.23 | 0.39 |
| Total | 12.83 | 17.44 | 18.73 | 14.67 | 14.49 | 22.58 | 0.12 | 0.16 | 0.36 |
| Source | Degrees of Freedom | Tree Height | DBH | V | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Sum of Squares | F | p | Sum of Squares | F | p | Sum of Squares | F | p | ||
| Site | 2 | 2153.50 | 112.49 ** | <0.001 | 8791.07 | 433.48 ** | <0.001 | 6.48 | 281.49 ** | <0.001 |
| Site × Block | 6 | 1098.87 | 19.13 ** | <0.001 | 1532.01 | 25.18 ** | <0.001 | 2.49 | 36.11 ** | <0.001 |
| Family | 20 | 627.31 | 3.28 ** | <0.001 | 626.66 | 3.09 ** | <0.001 | 0.74 | 3.22 ** | <0.001 |
| Site × Family | 39 | 917.76 | 2.46 ** | <0.001 | 875.89 | 2.21 ** | <0.001 | 0.71 | 1.58 ** | 0.018 |
| Site × Block × Family | 118 | 1890.67 | 1.67 ** | <0.001 | 2128.43 | 1.78 ** | <0.001 | 2.36 | 1.74 ** | 0.001 |
| Error | 381 | 3646.86 | 3863.42 | 4.38 | ||||||
| Total | 566 | 10,335.00 | 17,817.50 | 17.15 | ||||||
| Site | Source | Degrees of Freedom | HCB | CW | Branch Angle | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Sum of Squares | F | p | Sum of Squares | F | p | Sum of Squares | F | p | |||
| YL | Block | 2 | 117.96 | 17.71 ** | <0.001 | 2.74 | 9.79 ** | <0.001 | 1012.0 | 0.2 | 0.822 |
| Family | 20 | 179.16 | 2.69 ** | <0.001 | 7.74 | 2.76 ** | <0.001 | 52,837.0 | 1.02 | 0.438 | |
| Interaction effect | 40 | 229.11 | 1.72 ** | 0.012 | 9.96 | 1.78 ** | 0.009 | 106,135.0 | 1.03 | 0.437 | |
| Error | 126 | 419.49 | 17.63 | 324,763.0 | |||||||
| Total | 188 | 945.73 | 38.07 | 484,747.0 | |||||||
| LZ | Block | 2 | 29.06 | 7.03 ** | 0.001 | 0.20 | 0.07 | 0.934 | 342.7 | 1.16 | 0.318 |
| Family | 20 | 73.39 | 1.77 * | 0.03 | 24.33 | 0.83 | 0.678 | 2169.0 | 0.73 | 0.786 | |
| Interaction effect | 40 | 94.96 | 1.15 | 0.278 | 33.69 | 0.57 | 0.978 | 5528.8 | 0.93 | 0.588 | |
| Error | 126 | 260.5 | 185.50 | 18,660.0 | |||||||
| Total | 188 | 457.91 | 243.72 | 26,700.6 | |||||||
| PX | Block | 2 | 23.40 | 12.27 ** | <0.001 | 0.40 | 0.94 | 0.394 | 631.2 | 1.24 | 0.293 |
| Family | 20 | 33.06 | 1.73 * | 0.036 | 5.33 | 1.25 | 0.225 | 3470.8 | 0.68 | 0.839 | |
| Interaction effect | 40 | 41.93 | 1.1 | 0.339 | 6.51 | 0.76 | 0.836 | 7320.2 | 0.72 | 0.886 | |
| Error | 126 | 120.19 | 26.86 | 32,107.3 | |||||||
| Total | 188 | 218.59 | 39.10 | 43,529.5 | |||||||
| Family | HCB | CW | Branch Angle | ||||||
|---|---|---|---|---|---|---|---|---|---|
| YL | LZ | PX | YL | LZ | PX | YL | LZ | PX | |
| 1 | 4.93 | 6.53 | 8.11 | 1.40 | 2.22 | 2.47 | 45.22 | 32.78 | 57.67 |
| 2 | 4.21 | 6.69 | 8.29 | 1.27 | 2.83 | 2.10 | 43.56 | 32.33 | 61.22 |
| 3 | 4.49 | 6.48 | 8.12 | 1.11 | 2.83 | 2.56 | 45.89 | 23.56 | 54.22 |
| 4 | 6.08 | 5.16 | 8.31 | 1.19 | 3.17 | 2.15 | 50.44 | 35.67 | 67.89 |
| 5 | 7.58 | 4.94 | 8.97 | 1.40 | 2.17 | 2.46 | 59.00 | 27.78 | 59.00 |
| 6 | 7.28 | 5.70 | 8.21 | 1.15 | 2.50 | 2.23 | 52.22 | 28.89 | 63.11 |
| 7 | 7.12 | 5.69 | 8.63 | 1.33 | 3.00 | 2.25 | 65.11 | 28.22 | 54.00 |
| 8 | 6.89 | 6.46 | 8.47 | 1.42 | 2.67 | 1.95 | 51.11 | 28.33 | 61.89 |
| 9 | 5.24 | 5.51 | 9.34 | 1.34 | 3.61 | 2.27 | 52.78 | 28.56 | 53.78 |
| 10 | 6.47 | 4.68 | 8.20 | 1.52 | 2.83 | 2.35 | 50.56 | 28.11 | 61.33 |
| 11 | 7.30 | 6.60 | 8.67 | 1.84 | 3.22 | 2.08 | 52.33 | 33.89 | 54.89 |
| 12 | 7.06 | 4.99 | 8.48 | 1.61 | 2.50 | 2.17 | 47.00 | 32.78 | 61.00 |
| 13 | 6.98 | 5.53 | 8.18 | 1.21 | 2.78 | 2.31 | 41.56 | 27.56 | 50.44 |
| 14 | 8.34 | 4.91 | 8.78 | 1.96 | 2.94 | 2.27 | 46.00 | 25.89 | 54.56 |
| 15 | 4.96 | 5.86 | 8.44 | 1.34 | 2.72 | 2.30 | 44.44 | 22.33 | 60.00 |
| 16 | 6.21 | 4.71 | 7.57 | 1.33 | 2.72 | 2.14 | 42.78 | 29.89 | 56.11 |
| 17 | 5.52 | 5.42 | 7.92 | 1.28 | 2.89 | 2.12 | 42.56 | 26.67 | 57.89 |
| 18 | 4.53 | 6.21 | 8.17 | 1.29 | 2.56 | 2.36 | 39.67 | 27.11 | 65.44 |
| 19 | 3.84 | 5.59 | 8.90 | 1.33 | 3.33 | 2.36 | 42.67 | 34.22 | 61.33 |
| 20 | 5.06 | 5.56 | 9.19 | 1.36 | 2.33 | 2.48 | 44.44 | 27.11 | 61.00 |
| 21 | 4.61 | 5.89 | 8.18 | 1.71 | 2.39 | 2.64 | 51.11 | 30.89 | 63.33 |
| Total | 5.94 | 5.67 | 8.43 | 1.4 | 2.77 | 2.29 | 48.12 | 29.17 | 59.05 |
| Source | Degrees of Freedom | HCB | CW | Branch Angle | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Sum of Square | F | p | Sum of Square | F | p | Sum of Square | F | p | ||
| Site | 2 | 878.00 | 207.38 ** | <0.001 | 183.5 | 150.80 ** | 0.001 | 91,473.8 | 46.04 ** | <0.001 |
| Site × Block | 6 | 170.43 | 13.42 ** | <0.001 | 3.34 | 0.92 | 0.484 | 1985.5 | 0.33 | 0.919 |
| Family | 20 | 85.59 | 2.02 ** | 0.006 | 8.96 | 0.74 | 0.788 | 23,519.8 | 1.18 | 0.265 |
| Site × F | 40 | 200.03 | 2.36 ** | <0.001 | 28.43 | 1.17 | 0.230 | 34,957.5 | 0.88 | 0.681 |
| Site × Block × Family | 120 | 366.00 | 1.44 ** | 0.005 | 50.16 | 0.69 | 0.992 | 118,984.3 | 1.00 | 0.495 |
| Error | 378 | 800.18 | 229.99 | 375,530.0 | ||||||
| Total | 566 | 2500.22 | 504.39 | 646,450.9 | ||||||
| Site | Trait | CVg | CVe | ||||
|---|---|---|---|---|---|---|---|
| YL | Tree height | 2.17 | 1.4 | 8.02 | 11.47 | 22.07 | 0.78 |
| DBH | 3.1 | 3.48 | 21.57 | 12.01 | 31.66 | 0.67 | |
| V | 0.0017 | 0.0013 | 0.0112 | 34.21 | 88.19 | 0.74 | |
| HCB | 0.359 | 0.799 | 3.329 | 10.09 | 30.72 | 0.53 | |
| LZ | Tree height | 0.28 | 0.73 | 1.96 | 3.04 | 8.03 | 0.5 |
| DBH | 2.26 | 2.35 | 7.36 | 10.37 | 18.49 | 0.72 | |
| V | 0.00084 | 0.00121 | 0.00402 | 17.88 | 39.63 | 0.64 | |
| HCB | 0.17 | nsf | 2.141 | 7.27 | 25.81 | 0.83 | |
| PX | Tree height | 1.26 | 0.55 | 5.25 | 3.79 | 12.23 | 0.83 |
| DBH | 0.68 | 2.19 | 16.9 | 9.22 | 18.21 | 0.41 | |
| V | 0.00156 | 0.00258 | 0.03059 | 18.11 | 48.58 | 0.54 | |
| HCB | 0.0752 | nsf | 0.977 | 3.25 | 11.73 | 0.83 |
| Trait | CVg | CVe | |||||
|---|---|---|---|---|---|---|---|
| Tree height | 0.30 | 2.11 | 0.82 | 9.60 | 3.38 | 18.96 | 0.35 |
| DBH | 0.31 | 2.59 | 0.48 | 10.14 | 3.23 | 18.46 | 0.38 |
| V | 0.0071 | 0.00022 | 0.00273 | 0.0116 | 12.49 | 50.49 | 0.62 |
| HCB | 0.027 | 0.311 | 0.217 | 2.117 | 2.46 | 21.78 | 0.19 |
| Family | YL | LZ | PX | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| H | DBH | V | HCB | H | DBH | V | HCB | H | DBH | V | HCB | |
| 1 | −1.652 | −2.21 | −0.044 | −0.247 | 2.466 | 0.46 | 0.049 | 0.359 | −1.121 | −1.558 | −0.06 | −0.132 |
| 2 | −0.315 | 0.813 | 0.005 | −0.009 | −2.256 | −0.645 | −0.044 | 0.424 | 0.983 | 0.947 | 0.049 | −0.06 |
| 3 | −0.213 | 1.503 | 0.029 | −0.328 | −2.493 | −0.328 | −0.045 | 0.336 | −1.709 | −1.386 | −0.049 | −0.128 |
| 4 | 0.061 | −1.434 | −0.027 | −0.711 | −1.832 | −0.897 | −0.039 | −0.215 | −0.473 | −0.019 | −0.013 | −0.05 |
| 5 | −0.839 | −1.514 | −0.032 | −0.122 | 0.476 | 0.105 | 0.01 | −0.303 | −1.081 | −0.387 | −0.012 | 0.218 |
| 6 | −0.259 | −0.743 | −0.022 | −0.379 | 0.166 | −0.302 | 0.001 | 0.012 | −0.954 | −0.499 | −0.02 | −0.091 |
| 7 | −0.537 | −0.278 | −0.009 | −0.541 | −1.343 | 0.086 | −0.019 | 0.007 | 0.168 | 0.774 | 0.026 | 0.081 |
| 8 | −0.386 | −0.843 | −0.023 | −0.034 | −0.593 | 0.137 | −0.01 | 0.327 | −1.79 | −1.506 | −0.061 | 0.013 |
| 9 | −0.331 | 0.051 | −0.005 | −0.178 | −0.764 | −0.089 | −0.013 | −0.067 | −0.206 | −0.383 | −0.024 | 0.372 |
| 10 | 1.72 | 2.035 | 0.045 | −0.247 | 0.329 | 0.04 | 0.001 | −0.414 | 0.411 | −0.214 | −0.013 | −0.096 |
| 11 | −1.308 | −1.461 | −0.034 | −0.422 | 1.683 | −0.283 | 0.021 | 0.387 | −0.121 | 0.44 | 0.001 | 0.095 |
| 12 | 1.72 | 0.752 | 0.023 | 0.724 | −1.669 | −0.154 | −0.03 | −0.284 | 0.178 | 0.219 | −0.003 | 0.018 |
| 13 | −0.712 | −0.544 | −0.016 | −0.303 | 1.038 | 0.59 | 0.024 | −0.058 | 0.161 | 0.429 | 0.02 | −0.105 |
| 14 | 0.548 | 1.762 | 0.019 | 0.173 | 1.601 | −0.412 | 0.023 | −0.317 | 0.724 | 0.958 | 0.031 | 0.141 |
| 15 | 0.671 | 1.543 | 0.04 | 0.505 | −0.519 | −0.121 | −0.007 | 0.077 | 0.328 | 0.233 | 0.011 | 0.004 |
| 16 | 0.075 | −0.039 | −0.006 | 0.204 | 0.557 | 0.105 | 0.005 | −0.4 | 0.687 | −0.104 | 0.008 | −0.355 |
| 17 | −1.292 | −2.145 | −0.031 | −0.078 | 0.117 | 0.266 | 0.004 | −0.104 | 1.147 | 0.448 | 0.019 | −0.21 |
| 18 | −0.37 | −1.66 | −0.036 | 0.718 | −1.049 | −0.703 | −0.026 | 0.225 | 1.641 | 0.722 | 0.042 | −0.11 |
| 19 | −1.88 | −0.298 | −0.009 | −0.792 | 0.533 | 0.867 | 0.022 | −0.034 | 0.623 | 0.51 | 0.023 | 0.191 |
| 20 | 1.362 | 1.324 | 0.033 | 0.442 | 1.691 | 0.363 | 0.03 | −0.048 | 0.244 | 0.653 | 0.025 | 0.309 |
| 21 | 3.993 | 3.51 | 0.101 | 1.626 | 1.862 | 0.913 | 0.046 | 0.091 | 0.16 | −0.276 | −0.001 | −0.105 |
| Family | H (m) | DBH (cm) | V (cm3) | HCB (m) | Coefficient of Advantages | Rank |
|---|---|---|---|---|---|---|
| 21 | 19.30 | 22.99 | 0.30 | 6.23 | 0.0001 | 1 |
| 20 | 17.83 | 18.67 | 0.27 | 6.60 | 0.1595 | 2 |
| 14 | 17.68 | 18.82 | 0.27 | 7.34 | 0.2292 | 3 |
| 10 | 17.61 | 18.25 | 0.23 | 6.45 | 0.3262 | 4 |
| Average value of selected families | 18.10 | 19.68 | 0.27 | 6.66 | ||
| Group average | 16.33 | 17.25 | 0.21 | 6.68 | ||
| Genetic gains (%) | 21.29 | 22.92 | 21.25 | −0.14 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, G.; Tian, Z.; Jia, H.; Shen, W.; Li, Z.; Tang, L.; Zhao, H.; Xu, J. Genetic Parameter Estimates for the Growth and Morphological Traits of Castanopsis hystrix Families and the Genotype × Environment Interaction Effects. Forests 2023, 14, 1619. https://doi.org/10.3390/f14081619
Liu G, Tian Z, Jia H, Shen W, Li Z, Tang L, Zhao H, Xu J. Genetic Parameter Estimates for the Growth and Morphological Traits of Castanopsis hystrix Families and the Genotype × Environment Interaction Effects. Forests. 2023; 14(8):1619. https://doi.org/10.3390/f14081619
Chicago/Turabian StyleLiu, Guangjin, Zuwei Tian, Hongyan Jia, Wenhui Shen, Zuhe Li, Li Tang, Hongpeng Zhao, and Jianmin Xu. 2023. "Genetic Parameter Estimates for the Growth and Morphological Traits of Castanopsis hystrix Families and the Genotype × Environment Interaction Effects" Forests 14, no. 8: 1619. https://doi.org/10.3390/f14081619
APA StyleLiu, G., Tian, Z., Jia, H., Shen, W., Li, Z., Tang, L., Zhao, H., & Xu, J. (2023). Genetic Parameter Estimates for the Growth and Morphological Traits of Castanopsis hystrix Families and the Genotype × Environment Interaction Effects. Forests, 14(8), 1619. https://doi.org/10.3390/f14081619





