Molecular Dynamics Study on Mechanical Properties of Cellulose with Water Molecules Diffusion Behavior at Different Oxygen Concentrations
Abstract
:1. Introduction
2. Materials and Methods
2.1. Modeling
2.2. Dynamic Simulation
3. Results and Discussion
3.1. Balance of the System
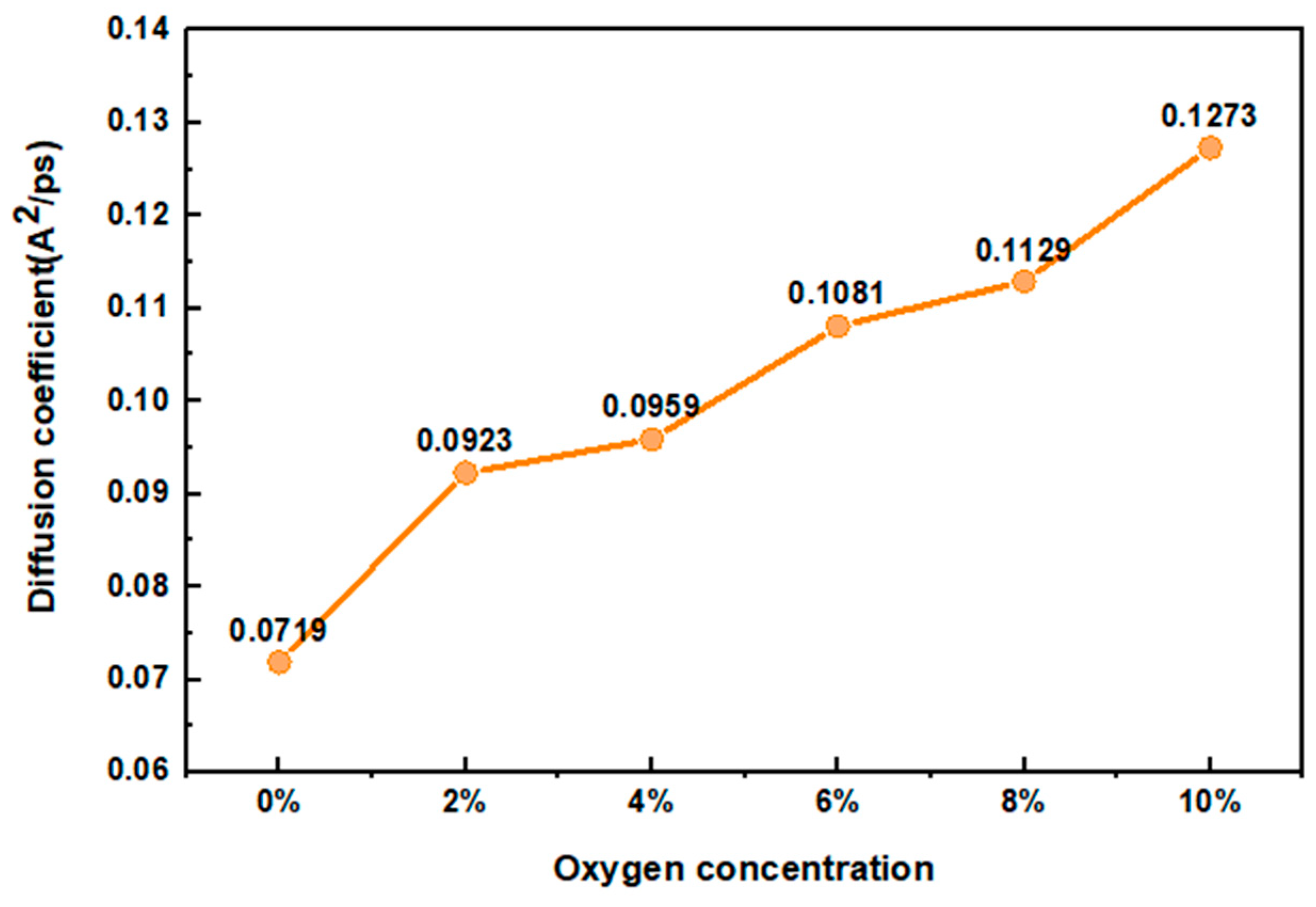
3.2. Diffusion Coefficient of Water Molecules
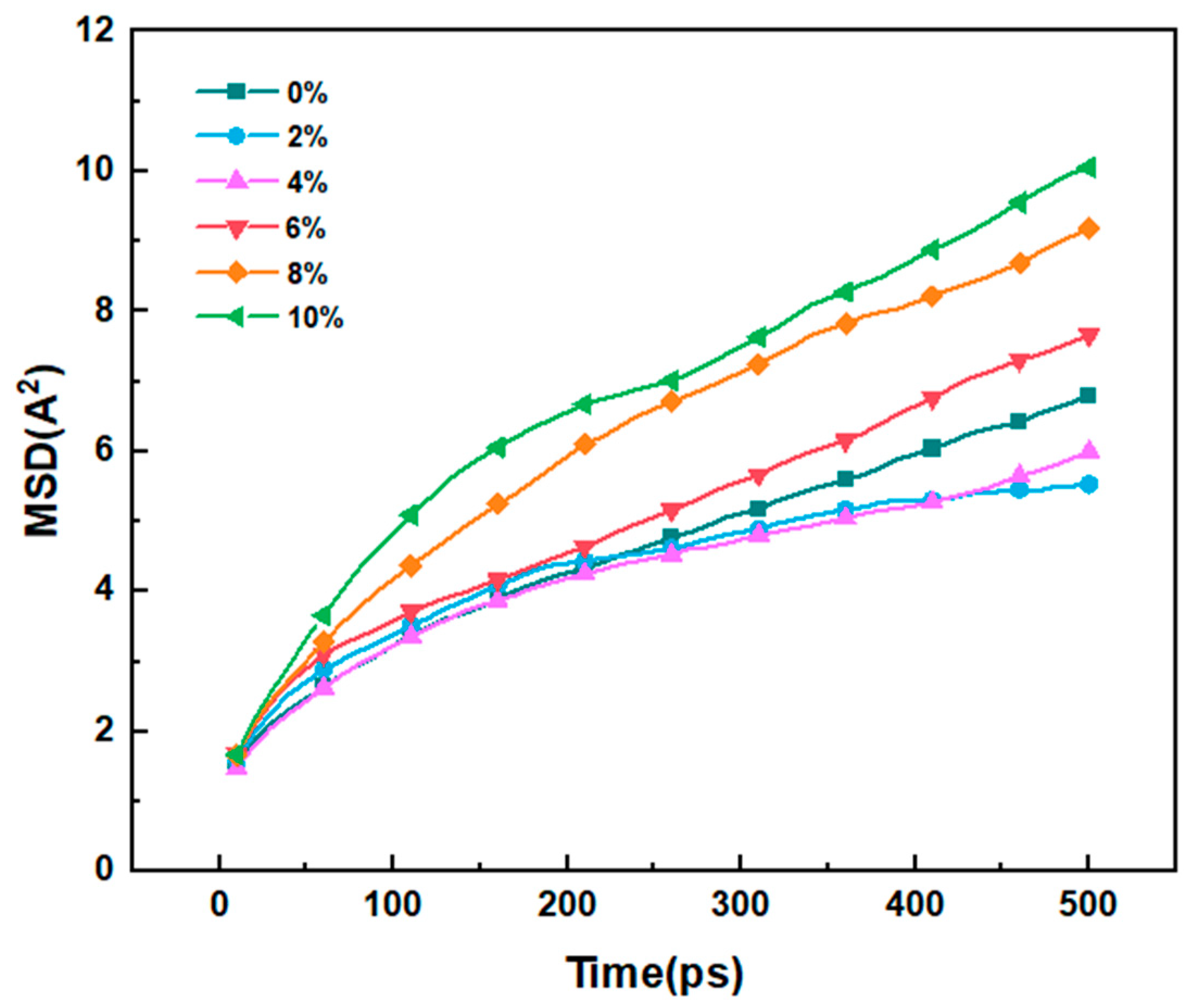
3.3. MSD of Wood Cellulose Chains
3.4. Lattice Parameters and Density
3.5. Hydrogen Bonding
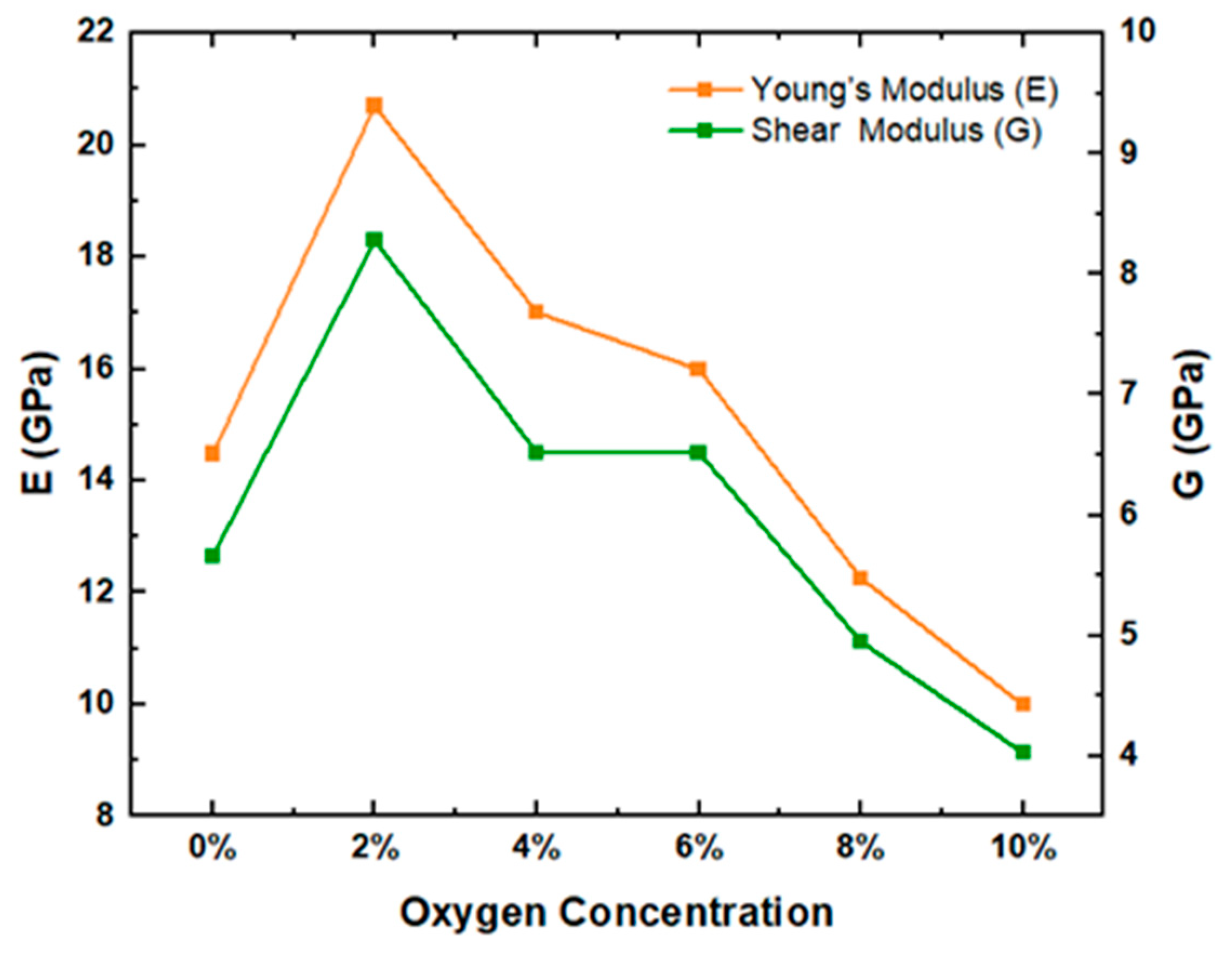
3.6. Mechanical Properties
4. Conclusions
- Water molecules become more flexible in the presence of oxygen, which causes the diffusion coefficient of water molecules to gradually rise with oxygen concentration. This aids in minimizing energy loss during heat treatment, thus improving the processing efficiency. At the same time, the increase in water molecule diffusion coefficient causes a simultaneous rise in cell volume and a corresponding fall in density.
- The MSD of cellulose chains decreases and then increases with the increase in oxygen concentration, which indicates that the thermal stability of cellulose chains is better at low oxygen concentrations, and oxygen concentrations that are too high will lead to the destruction of the internal structure of cellulose chains and greatly reduce the stability. This is related to the number of hydrogen bonds within the cellulose chain. The formation of intermolecular hydrogen bonds increases the molecular interactions within the cellulose chains and enhances the stability of the structure.
- Young’s modulus and shear modulus of cellulose chains first rise and then decline with oxygen concentration, indicating that the rigidity and distortion resistance of cellulose chains improve and then fall, peaking at 2% oxygen concentration. This change trend is compatible with the cellulose chains’ hydrogen bonding and MSD change trends, which further indicate that the internal structure of cellulose chains is more stable at low oxygen concentrations. These results indicate that an appropriate increase in oxygen concentration can help to potentially improve the stiffness and resistance to deformation of wood, and also confirm the significance of this paper’s research, which provides additional theoretical support for the development of wood heat treatment processes.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kamperidou, V.; Barboutis, I.; Vasileiou, V. Effect of Thermal Treatment on Colour and Hygroscopic Properties of Poplar Wood. In Proceedings of the 23rd International Scientific Conference: Wood is Good—With Knowledge and Technology to a Competitive Forestry and Wood Technology Sector, Zagreb, Croatia, 12 October 2012; pp. 59–67. [Google Scholar]
- Mohebby, B.; Ilbeighi, F.; Kazemi-Najafi, S. Influence of hydrothermal modification of fibers on some physical and mechanical properties of medium density fiberboard (MDF). Eur. J. Wood Wood Prod. 2008, 66, 213–218. [Google Scholar] [CrossRef]
- Zhou, F.; Fu, Z.; Gao, X.; Zhou, Y. Changes in the wood-water interactions of mahogany wood due to heat treatment. Holzforschung 2020, 74, 853–863. [Google Scholar] [CrossRef]
- Fu, Z.; Zhou, Y.; Gao, X.; Liu, H.; Zhou, F. Changes of water related properties in radiata pine wood due to heat treatment. Constr. Build. Mater. 2019, 227, 116692. [Google Scholar] [CrossRef]
- Burmester, A. Effect of heat-pressure-treatments of semi-dry wood on its dimensional stability. Holz Als Roh- Werkst. 1973, 31, 237–243. [Google Scholar] [CrossRef]
- Cao, Y.; Lu, J.; Huang, R.; Jiang, J. Increased dimensional stability of Chinese fir through steam-heat treatment. Eur. J. Wood Wood Prod. 2012, 70, 441–444. [Google Scholar] [CrossRef]
- Cao, Y.; Lu, J.; Huang, R.; Zhao, Y.; Wu, Y. Evaluation of decay resistance for steam-heat-treated wood. Bioresources 2011, 6, 4696–4704. [Google Scholar]
- Giebeler, E. Dimensional stabilization of wood by moisture-heat-pressure-treatment. Holz Als Roh- Werkst. 1983, 41, 87–94. [Google Scholar] [CrossRef]
- Bruno, E.; Helena, F.; Hélder, V.; José, F.; Idalina, D.; Luísa, C.; Dennis, J.; Lina, N. Termite Resistance, Chemical and Mechanical Characterization of Paulownia tomentosa Wood before and after Heat Treatment. Forests 2021, 12, 1114. [Google Scholar]
- Lee, S.H.; Ashaari, Z.; Jamaludin, F.R.; Yee, C.N.; Ahamad, W.N. Physico-mechanical properties of particleboard made from heat-treated rubberwood particles. Eur. J. Wood Wood Prod. 2017, 75, 655–658. [Google Scholar] [CrossRef]
- Lu, C.; Liu, Y.; Jiang, H.; Lu, Q. Impact of heat treatment on the surface color and glossiness of young eucalyptus wood. Wood Res. 2022, 67, 348–360. [Google Scholar] [CrossRef]
- Jiang, J.; Lu, J.; Zhou, Y.; Huang, R.; Zhao, Y.; Jiang, J. Optimization of processing variables during heat treatment of oak (Quercus mongolica) wood. Wood Sci. Technol. 2014, 48, 253–267. [Google Scholar] [CrossRef]
- Lee, S.H.; Rossky, P.J. A comparison of the structure and dynamics of liquid water at hydrophobic and hydrophilic surfaces—A molecular dynamics simulation study. J. Chem. Phys. 1994, 100, 3334–3345. [Google Scholar] [CrossRef]
- Khazraji, A.C.; Robert, S. Interaction Effects between Cellulose and Water in Nanocrystalline and Amorphous Regions: A Novel Approach Using Molecular Modeling. J. Nanomater. 2013, 2013, 409676. [Google Scholar] [CrossRef]
- Meier, R.J.; Maple, J.R.; Hwang, M.J.; Hagler, A.T. Molecular Modeling Urea- and Melamine-Formaldehyde Resins. 1. A Force Field for Urea and Melamine. J. Phys. Chem. 1995, 99, 5445–5456. [Google Scholar] [CrossRef]
- Hou, T.; Zhang, W.; Xu, X. Binding Affinities for a Series of Selective Inhibitors of Gelatinase-A Using Molecular Dynamics with a Linear Interaction Energy Approach. J. Phys. Chem. B 2001, 105, 5304–5315. [Google Scholar] [CrossRef]
- Fukuda, M.; Kuwajima, S. Molecular dynamics simulation of water diffusion in atactic and amorphous isotactic polypropylene. J. Chem. Phys. 1998, 108, 3001–3009. [Google Scholar] [CrossRef]
- Tanaka, F.; Fukui, N. The behavior of cellulose molecules in aqueous environments. Cellulose 2004, 11, 33–38. [Google Scholar] [CrossRef]
- Liao, R.-J.; Zhu, M.-Z.; Zhou, X.; Yang, L.-J.; Yan, J.-M.; Sun, C.-X. Molecular Dynamics Simulation of the Diffusion Behavior of Water Molecules in Oil and Cellulose Composite Media. Acta Phys.-Chim. Sin. 2011, 27, 815–824. [Google Scholar]
- Theodorou, D.N.; Suter, U.W. Detailed molecular structure of a vinyl polymer glass. Macromolecules 1985, 18, 1467–1478. [Google Scholar] [CrossRef]
- Mazeau, K.; Heux, L. Molecular dynamics simulations of bulk native crystalline and amorphous structures of cellulose. J. Phys. Chem. B 2008, 107, 2394–2403. [Google Scholar] [CrossRef]
- Paajanen, A.; Vaari, J. High-temperature decomposition of the cellulose molecule: A stochastic molecular dynamics study. Cellulose 2017, 24, 2713–2725. [Google Scholar] [CrossRef]
- Wang, X.; Tang, C.; Wang, Q.; Li, X.; Hao, J. Selection of Optimal Polymerization Degree and Force Field in the Molecular Dynamics Simulation of Insulating Paper Cellulose. Energies 2017, 10, 1377. [Google Scholar] [CrossRef]
- Wang, W.; Ma, W.; Wu, M.; Sun, L. Effect of Water Molecules at Different Temperatures on Properties of Cellulose Based on Molecular Dynamics Simulation. Bioresources 2022, 17, 269–280. [Google Scholar] [CrossRef]
- Onyon, P.F. Polymer Handbook. Nature 1972, 238, 56. [Google Scholar] [CrossRef]
- Liu, W.K.; Karpov, E.G.; Park, H.S. Nano Mechanics and Materials: Theory, Multiscale Methods and Applications; John Wiley & Sons: Chichester, UK, 2006. [Google Scholar]
- Maple, J.R.; Hwang, M.J.; Stockfisch, T.P.; Hagler, A.T. Derivation of Class II Force Fields. III. Characterization of a Quantum Force Field for Alkanes. Isr. J. Chem. 2013, 34, 195–231. [Google Scholar] [CrossRef]
- Nosé, S. Constant Temperature Molecular Dynamics Methods. Prog. Theor. Phys. Suppl. 1991, 103, 1–46. [Google Scholar] [CrossRef]
- Berendsen, H.J.C.P.; Postma, J.; Gunsteren, W.; Dinola, A.D.; Haak, J.R. Molecular-Dynamics with Coupling to An External Bath. J. Chem. Phys. 1984, 81, 3684–3690. [Google Scholar] [CrossRef]
- Andersen, H.C. Molecular dynamics simulations at constant pressure and/or temperature. J. Chem. Phys. 1980, 72, 2384–2393. [Google Scholar] [CrossRef]
- Ewald, P.P. Die Berechnung optischer und elektrostatischer Gitterpotentiale. Ann. Phys. 1921, 369, 253–287. [Google Scholar] [CrossRef]
- Allen, M.; Tildesley, D.; Press, U. Computer Simulation of Liquids; Oxford University: Oxford, UK, 1989. [Google Scholar]
- Sangiovanni, D.G.; Hellman, O.; Alling, B.; Abrikosov, I.A. Efficient and accurate determination of lattice-vacancy diffusion coefficients via non equilibrium ab initio molecular dynamics. Phys. Rev. B. 2016, 93, 094305. [Google Scholar] [CrossRef]
- Brown, W.R.; Jenkins, R.B.; Park, G.S. The sorption and diffusion of small molecules in amorphous and crystalline polybutadienes. J. Polym. Ence Polym. Symp. 2010, 41, 45–67. [Google Scholar] [CrossRef]
- Einstein, A.; Einstein, E. Zur Elektrodinamik bewegter KÖrper. Ann. Phys. 1905, 17, 891–921. [Google Scholar] [CrossRef]
- Yang, L.; Qi, C.; Wu, G.; Liao, R.; Wang, Q.; Gong, C.; Gao, J. Molecular dynamics simulation of diffusion behaviour of gas molecules within oil–paper insulation system. Mol. Simul. 2013, 39, 988–999. [Google Scholar] [CrossRef]
- Du, D.; Tang, C.; Zhang, J.; Hu, D. Effects of Hydrogen Sulfide on the Mechanical and Thermal Properties of Cellulose Insulation Paper: A Molecular Dynamics Simulation. Mater. Chem. Phys. 2019, 240, 122153. [Google Scholar] [CrossRef]
- Xu, B.; Chen, Z.; Ma, Q. Effect of high-voltage electric field on formaldehyde diffusion within building materials. Build. Environ. 2016, 95, 214–218. [Google Scholar] [CrossRef]
- Ouyang, F.; Wang, W. Effect of Thermo-Hydro-Mechanical Treatment on Mechanical Properties of Wood Cellulose: A Molecular Dynamics Simulation. Forests 2022, 13, 903. [Google Scholar] [CrossRef]
- Li, X.; Tang, C.; Wang, J.; Tian, W.; Hu, D. Analysis and mechanism of adsorption of naphthenic mineral oil, water, formic acid, carbon dioxide, and methane on meta-aramid insulation paper. J. Mater. Sci. 2019, 54, 8556–8570. [Google Scholar] [CrossRef]
- Li, X.; Tang, C.; Wang, Q.; Li, X.P.; Hao, J. Molecular simulation research on the micro effect mechanism of interfacial properties of nano SiO2/meta-aramid fiber. Int. J. Heat Technol. 2017, 35, 123–129. [Google Scholar] [CrossRef]
- Hinterstoisser, B.; Akerholm, M.; Salmen, L. Load distribution in native cellulose. Biomacromolecules 2003, 4, 1232–1237. [Google Scholar] [CrossRef] [PubMed]
- Zhang, N.; Shen, Z.; Chen, C.; He, G.; Hao, C. Effect of hydrogen bonding on self-diffusion in methanol/water liquid mixtures: A molecular dynamics simulation study. J. Mol. Liq. 2015, 203, 90–97. [Google Scholar] [CrossRef]
- Fu, S.Y.; Lauke, B.; Li, R.K.Y.; Mai, Y.W. Effects of PA6,6/PP ratio on the mechanical properties of short glass fiber reinforced and rubber-toughened polyamide 6,6/polypropylene blends. Compos. Part B-Eng. 2006, 37, 182–190. [Google Scholar] [CrossRef]
- Pugh, S.F. XCII. Relations between the elastic moduli and the plastic properties of polycrystalline pure metals. Philos. Mag. 2009, 45, 823–843. [Google Scholar]
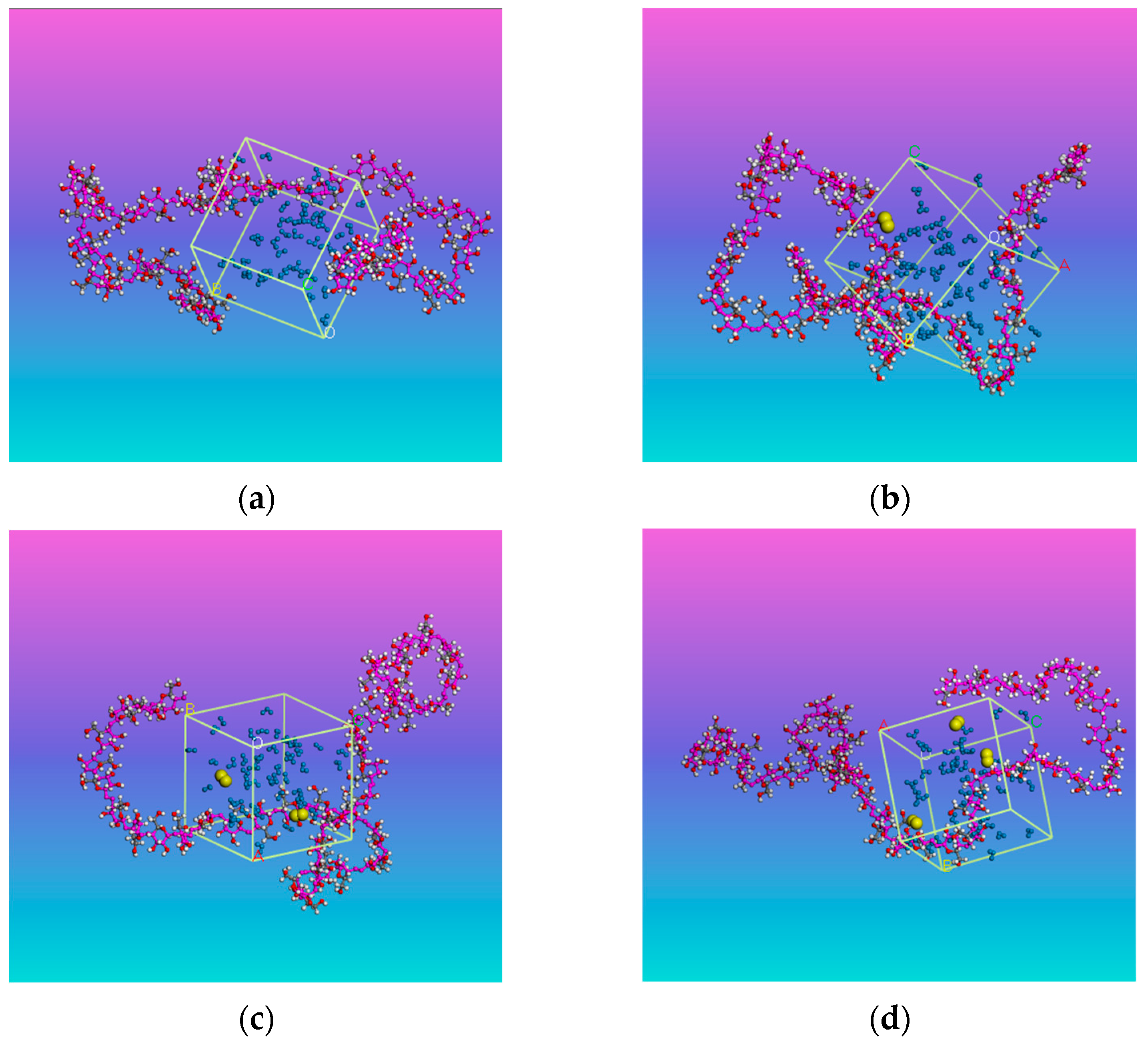
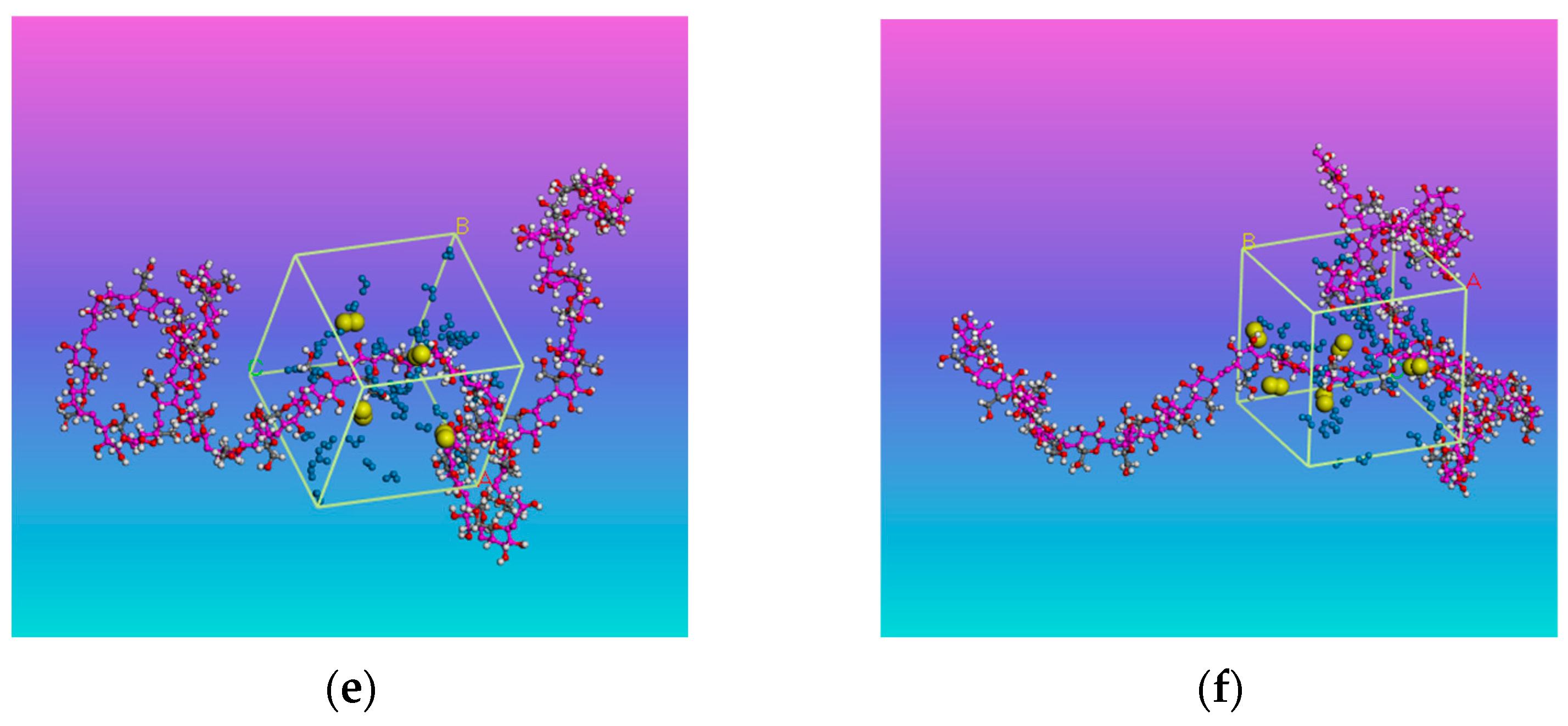
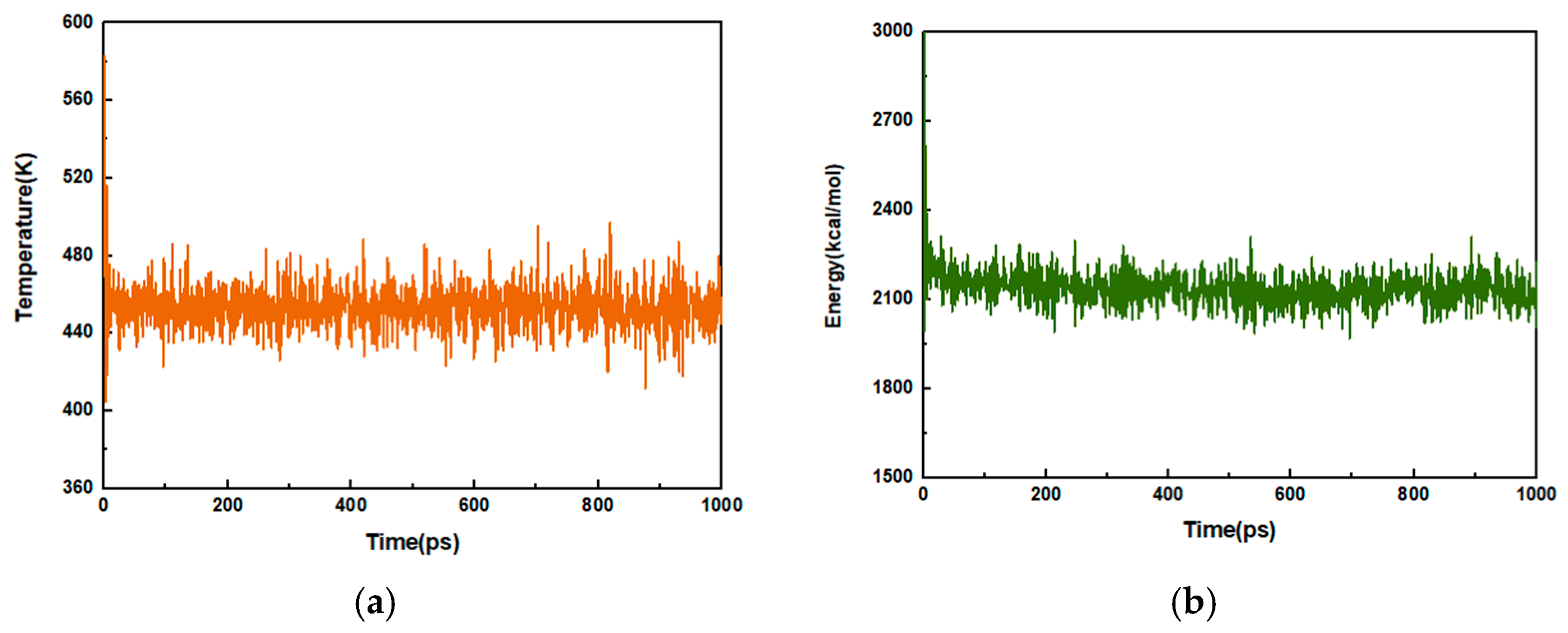
| Oxygen Concentration | 0% | 2% | 4% | 6% | 8% | 10% |
|---|---|---|---|---|---|---|
| Fluctuation Value | 2.22% | 2.71% | 3.10% | 2.92% | 2.37% | 2.79% |
| Oxygen Concentration | m | D | R-Square |
|---|---|---|---|
| 0% | 0.4314 | 0.0719 | 0.9980 |
| 2% | 0.5539 | 0.0923 | 0.9987 |
| 4% | 0.5755 | 0.0959 | 0.9989 |
| 6% | 0.6484 | 0.1081 | 0.9978 |
| 8% | 0.6772 | 0.1129 | 0.9995 |
| 10% | 0.7637 | 0.1273 | 0.9993 |
| Oxygen Concentration | Cell Parameters | Volume (A3) | ||||
|---|---|---|---|---|---|---|
| the Length | the Width | the Height | Final | Average | Std.Dev. | |
| 0% | 21.11 | 21.11 | 21.11 | 9404.397 | 9483.821 | 145.470 |
| 2% | 21.16 | 21.16 | 21.16 | 9475.331 | 9535.602 | 121.343 |
| 4% | 21.19 | 21.19 | 21.19 | 9520.700 | 9597.114 | 109.067 |
| 6% | 21.24 | 21.24 | 21.24 | 9577.419 | 9633.354 | 125.889 |
| 8% | 21.31 | 21.31 | 21.31 | 9671.309 | 9655.824 | 115.276 |
| 10% | 21.37 | 21.37 | 21.37 | 9776.236 | 9756.931 | 162.700 |
| Oxygen Concentration | Density (g/cm3) | ||
|---|---|---|---|
| Final | Average | Std.Dev. | |
| 0% | 1.361 | 1.350 | 0.020 |
| 2% | 1.353 | 1.345 | 0.017 |
| 4% | 1.349 | 1.339 | 0.015 |
| 6% | 1.344 | 1.336 | 0.017 |
| 8% | 1.333 | 1.335 | 0.016 |
| 10% | 1.321 | 1.324 | 0.021 |
| Oxygen Concentration | Number of Hydrogen Bonds | |||
|---|---|---|---|---|
| between Cellulose Chains | between Water Molecular | between Water–Cellulose | Total | |
| 0% | 73 | 30 | 91 | 194 |
| 2% | 96 | 29 | 90 | 215 |
| 4% | 91 | 25 | 81 | 197 |
| 6% | 87 | 28 | 75 | 190 |
| 8% | 90 | 21 | 70 | 181 |
| 10% | 83 | 22 | 70 | 175 |
| Oxygen Concentration | λ | μ | G (GPa) | E (GPa) | γ (GPa) | K/G |
|---|---|---|---|---|---|---|
| 0% | 7.15 | 5.66 | 5.66 | 14.48 | 0.28 | 1.28 |
| 2% | 8.24 | 8.28 | 8.28 | 20.70 | 0.25 | 1.00 |
| 4% | 10.13 | 6.52 | 6.52 | 17.01 | 0.30 | 1.5 |
| 6% | 5.41 | 6.52 | 6.52 | 15.99 | 0.23 | 0.85 |
| 8% | 4.47 | 4.95 | 4.95 | 12.26 | 0.24 | 0.93 |
| 10% | 3.70 | 4.03 | 4.03 | 9.99 | 0.24 | 0.96 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Y.; Wang, W.; Jiang, X. Molecular Dynamics Study on Mechanical Properties of Cellulose with Water Molecules Diffusion Behavior at Different Oxygen Concentrations. Forests 2023, 14, 371. https://doi.org/10.3390/f14020371
Guo Y, Wang W, Jiang X. Molecular Dynamics Study on Mechanical Properties of Cellulose with Water Molecules Diffusion Behavior at Different Oxygen Concentrations. Forests. 2023; 14(2):371. https://doi.org/10.3390/f14020371
Chicago/Turabian StyleGuo, Yuanyuan, Wei Wang, and Xuewei Jiang. 2023. "Molecular Dynamics Study on Mechanical Properties of Cellulose with Water Molecules Diffusion Behavior at Different Oxygen Concentrations" Forests 14, no. 2: 371. https://doi.org/10.3390/f14020371
APA StyleGuo, Y., Wang, W., & Jiang, X. (2023). Molecular Dynamics Study on Mechanical Properties of Cellulose with Water Molecules Diffusion Behavior at Different Oxygen Concentrations. Forests, 14(2), 371. https://doi.org/10.3390/f14020371





