Modulating the Acoustic Vibration Performance of Wood by Introducing a Periodic Annular Groove Structure
Abstract
:1. Introduction
2. Materials and Methods
2.1. Structural Design
2.2. Finite Element Simulation
2.3. Equations for the Equivalent Parameters
2.4. Experimental Test
3. Results and Discussion
3.1. Results of the Experimental Tests
3.2. Results of Finite Element Simulation
3.2.1. Relationship between f and Structural Parameters
3.2.2. Relationship between E′ and structural parameters
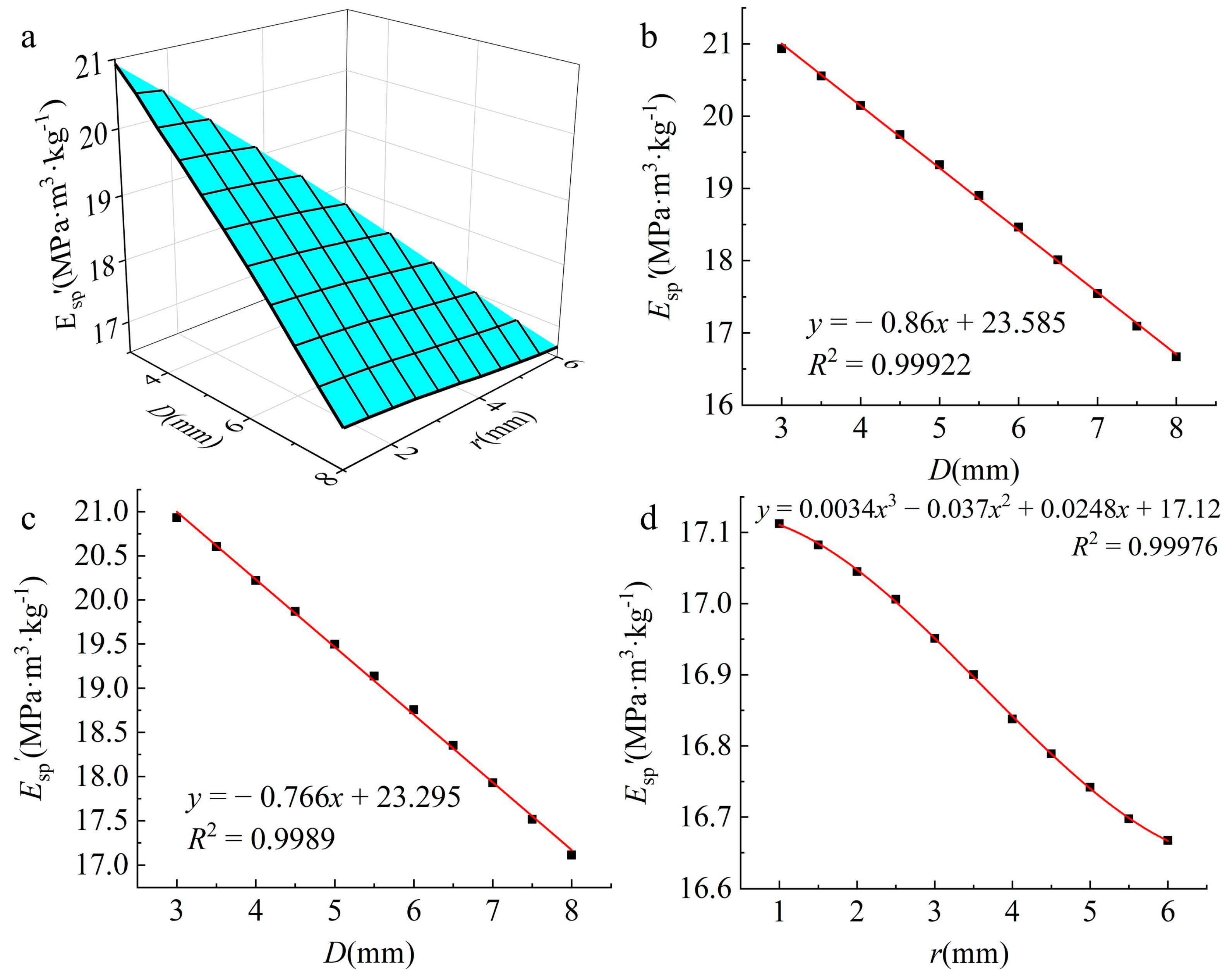
3.2.3. Relationship between Esp′ and Structural Parameters
3.2.4. Relationship between w′ and Structural Parameters
3.2.5. Relationship between R′ and Structural Parameters
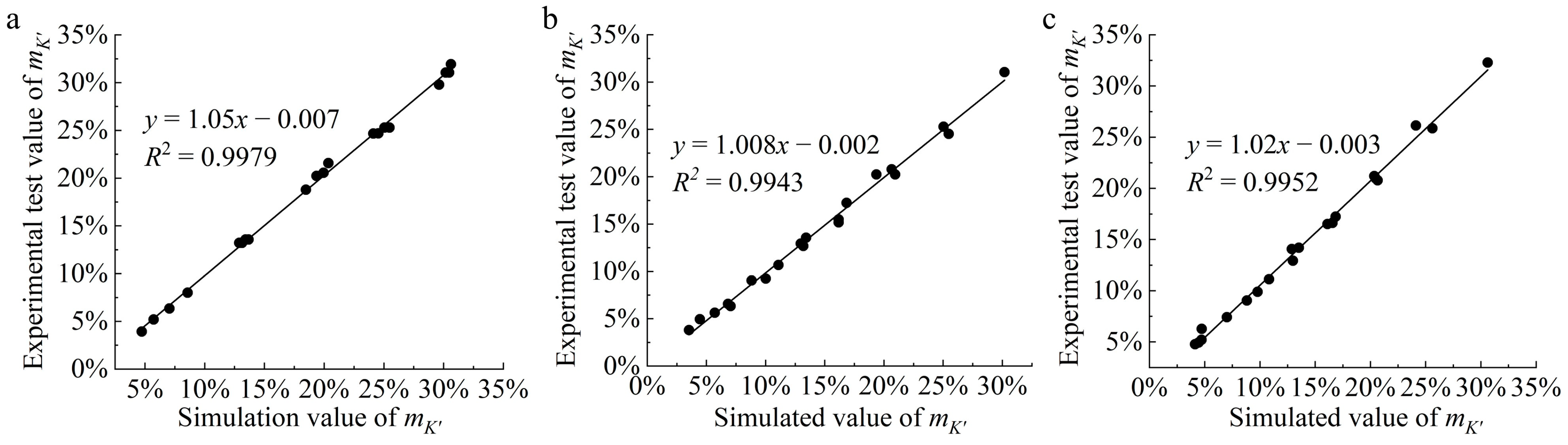
3.3. Reliability Evaluation of the Finite Element Simulation Results
3.4. Overall Evaluation of Results
4. Conclusions
- (1)
- It is feasible to modify the acoustic vibration performance of wood using periodic circular groove structures. When the groove depth and ring width are constant, the wood’s frequency, equivalent dynamic MOE, equivalent specific dynamic MOE, equivalent acoustic radiation quality constant, and equivalent acoustic impedance exhibit noticeable linear negative correlations with the outer circle radius. When the groove depth and outer circle radius are constant, the relationships between the wood’s five acoustic vibration parameters and the inner circle radius are close to cubic functions. When the groove depth and inner circle are constant, except for the relationship between the equivalent sound radiation quality factor and the outer circle radius, which approximates a quadratic function, the other acoustic vibration performance-related parameters are linearly and negatively correlated with the outer circle radius. Therefore, periodic circular groove structures can be used to adjust the acoustic vibration performance of wood, reducing the differences resulting from the variability among individual wood samples.
- (2)
- There are different relationships between various acoustic vibration performance parameters and the periodic annular groove structure parameters. However, apart from the equivalent sound radiation quality factor, the other parameters can first be roughly selected based on the outer circle radius, followed by fine-tuning with the inner circle radius. In contrast, the equivalent sound radiation quality factor is better suited for initial selection based on the inner circle radius, followed by fine-tuning with the outer circle radius, enabling the design of the wood’s acoustic vibration performance.
- (3)
- Following the concept of metamaterials, we experimentally verified the extent to which the frequency, equivalent dynamic MOE, equivalent ratio of dynamic MOE, equivalent sound radiation quality factor, and equivalent acoustic impedance of wood are influenced by structural changes. Overall, the observed trend in each parameter closely aligned with the finite element simulation results. There was a high degree of correlation between the experimental test results and finite element simulation results. Thus, it is considered that finite element techniques can be used to investigate the effect of the structure of periodic annular grooves on the acoustic vibration performance of wood.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jiang, Z.; Deng, L.; Song, R.; Chen, F.; Liu, X. Research Progress on The Acoustic Vibration Performance of Wood and Bamboo. World For. Res. 2021, 34, 1–7. [Google Scholar] [CrossRef]
- Viala, R.; Placet, V.; Cogan, S. Simultaneous Non-Destructive Identification of Multiple Elastic and Damping Properties of Spruce Tonewood to Improve Grading. J. Cult. Herit. 2020, 42, 108–116. [Google Scholar] [CrossRef]
- Yoshikawa, S. Acoustical Classification of Woods for String Instruments. J. Acoust. Soc. Am. 2007, 122, 568–573. [Google Scholar] [CrossRef] [PubMed]
- Huang, Y.; Meng, S.; Zhao, P.; Yue, M. A Method for Identifying Wood Grades of Chinese Zither Panel Based on Near Infrared Spectroscopy. Spectrosc. Spect. Anal. 2019, 39, 723. [Google Scholar] [CrossRef]
- Liu, M.; Peng, L.; Lyu, S.; Lyu, J.; Gao, Y.; Fan, Z. Research Progress of Wood Treatment to Improve Acoustic Vibration Performance for Making Musical Instruments. China Wood Ind. 2020, 34, 29–33. [Google Scholar] [CrossRef]
- Akl, W.; Elsabbagh, A.; Baz, A. Acoustic Metamaterials with Circular Sector Cavities and Programmable Densities. J. Acoust. Soc. Am. 2012, 132, 2857–2865. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, H.; Cheng, H.; Tian, J.; Chen, S. Multidimensional Manipulation of Wave Fields Based on Artificial Microstructures. Opto-Electron. Adv. 2020, 3, 200002. [Google Scholar] [CrossRef]
- Dalela, S.; Balaji, P.S.; Jena, D.P. A Review on Application of Mechanical Metamaterials for Vibration Control. Mech. Adv. Mater. Struct. 2022, 29, 3237–3262. [Google Scholar] [CrossRef]
- Liu, X.; Hu, G. Elastic Metamaterials Making Use of Chirality: A Review. J. Mech. Eng. 2016, 62, 403–418. [Google Scholar] [CrossRef]
- Wu, W.; Hu, W.; Qian, G.; Liao, H.; Xu, X.; Berto, F. Mechanical Design and Multifunctional Applications of Chiral Mechanical Metamaterials: A Review. Mater. Des. 2019, 180, 107950. [Google Scholar] [CrossRef]
- Kadic, M.; Milton, G.W.; Van Hecke, M.; Wegener, M. 3D Metamaterials. Nat. Rev. Phys. 2019, 1, 198–210. [Google Scholar] [CrossRef]
- Hedayati, R.; Bodaghi, M. Acoustic Metamaterials and Acoustic Foams: Recent Advances. Appl. Sci. 2022, 12, 3096. [Google Scholar] [CrossRef]
- Yu, X.; Zhou, J.; Liang, H.; Jiang, Z.; Wu, L. Mechanical Metamaterials Associated with Stiffness, Rigidity and Compressibility: A Brief Review. Prog. Mater. Sci. 2018, 94, 114–173. [Google Scholar] [CrossRef]
- Zhou, J.; Li, L.T. Metamaterial technology and its application prospects. Strateg. Study CAE 2018, 20, 69–74. [Google Scholar] [CrossRef]
- Sun, H.; Yan, F.; Gu, H.; Li, Y. Acoustic Metamaterial with Negative Parameter. In Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, San Diego, CA, USA, 9 March 2014. [Google Scholar] [CrossRef]
- Cui, H.; Liu, C.; Hu, H. Research on Low-Frequency Noise Control Based on Fractal Coiled Acoustic Metamaterials. Shock Vib. 2022, 2022, 2083563. [Google Scholar] [CrossRef]
- Kumar, S.; Pueh Lee, H. Recent Advances in Active Acoustic Metamaterials. Int. J. Appl. Mech. 2019, 11, 1950081. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, X.M.; Lin, P.; Lee, H.P. Sound Insulation Properties of Membrane-type Acoustic Metamaterials. Noise Vib. Control 2021, 41, 42–48. [Google Scholar] [CrossRef]
- Mir, F.; Saadatzi, M.; Ahmed, R.U.; Banerjee, S. Acoustoelastic MetaWall Noise Barriers for Industrial Application with Simultaneous Energy Harvesting Capability. Appl. Acoust. 2018, 139, 282–292. [Google Scholar] [CrossRef]
- Zhu, Y.; Assouar, B. Multifunctional Acoustic Metasurface Based on an Array of Helmholtz Resonators. Phys. Rev. B 2019, 99, 174109. [Google Scholar] [CrossRef]
- Viala, R.; Placet, V.; Cogan, S. Model-Based Evidence of the Dominance of the Guitar Brace Design over Material and Climatic Variability for Dynamic Behaviors. Appl. Acoust. 2021, 182, 108275. [Google Scholar] [CrossRef]
- Stanciu, M.D.; Rosca, I.C.; Mihălcică, M.; Bucur, V. Dynamic Response of Wooden Plates in Different Stages of Guitar Manufacturing. Eur. J. Wood Wood Prod. 2022, 80, 997–1013. [Google Scholar] [CrossRef]
- Brauchler, A.; Ziegler, P.; Eberhard, P. An Entirely Reverse-Engineered Finite Element Model of a Classical Guitar in Comparison with Experimental Data. J. Acoust. Soc. Am. 2021, 149, 4450–4462. [Google Scholar] [CrossRef] [PubMed]
- Gonzalez, S.; Salvi, D.; Baeza, D.; Antonacci, F.; Sarti, A. A Data-Driven Approach to Violin Making. Sci. Rep. 2021, 11, 9455. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, Y.; Shen, J.; Liu, M. Effect of Ribs on Vibration Transmission of Piano Soundboard. J. Northeast For. Univ. 2009, 37, 44–45. [Google Scholar] [CrossRef]
- Stanciu, M.D.; Dinulică, F.; Bucur, V.; Gliga, V.G.; Nastac, S.M.; Câmpean, M. Changing the Vibrational Behavior of the Wooden Thin Arched Plates—The Maestro Violins Experimental Study Case. Thin Wall. Struct. 2022, 174, 109042. [Google Scholar] [CrossRef]
- Bader, R.; Fischer, J.; Münster, M.; Kontopidis, P. Metamaterials in Musical Acoustics: A Modified Frame Drum. J. Acoust. Soc. Am. 2019, 145, 3086–3094. [Google Scholar] [CrossRef]
- Oñate, C.E.; Arancibia, A.; Cartes, G.; Beas, C.F. Seeking for Spectral Manipulation of the Sound of Musical Instruments Using Metamaterials. In Proceedings of the 15th International Audio Mostly Conference, Graz, Austria, 15 September 2020. [Google Scholar] [CrossRef]
- Gonzalez, S.; Chacra, E.; Carreño, C.; Espinoza, C. Wooden Mechanical Metamaterials: Towards Tunable Wood Plates. Mater. Des. 2022, 221, 110952. [Google Scholar] [CrossRef]
- Lercari, M.; Gonzalez, S.; Espinoza, C.; Longo, G.; Antonacci, F.; Sarti, A. Using Mechanical Metamaterials in Guitar Top Plates: A Numerical Study. Appl. Sci. 2022, 12, 8619. [Google Scholar] [CrossRef]
- Bui, T.A.; Lardeur, P.; Oudjene, M.; Park, J. Numerical Modelling of the Variability of the Vibration Frequencies of Multi-Layered Timber Structures Using the Modal Stability Procedure. Compos. Struct. 2022, 285, 115226. [Google Scholar] [CrossRef]
- Hawryszków, P.; Biliszczuk, J. Vibration Serviceability of Footbridges Made of the Sustainable and Eco Structural Material: Glued-Laminated Wood. Materials 2022, 15, 1529. [Google Scholar] [CrossRef]
- Wang, F.; Yang, L.Q.; Sun, J.; Zhu, X. Application of Finite Element Method to Modal Analysis of Wood. J. Northeast For. Univ. 2008, 36, 24–25. [Google Scholar] [CrossRef]
- Zhang, Y.; Ge, Y.; Miao, Y.; Liu, Z.; Li, Y.; Tian, M.; Li, X.; Wang, C. Vibration Mode of Guzheng Soundboard with Composite Structure. J. Beijing For. Univ. 2022, 44, 132–141. [Google Scholar] [CrossRef]
- Ni, Z. Vibration Mechanics; Xi’an Jiaotong University Press: Xi’an, China, 1989; pp. 65–67. [Google Scholar]
- Brémaud, I.; El Kaïm, Y.; Guibal, D.; Minato, K.; Thibaut, B.; Gril, J. Characterisation and Categorisation of the Diversity in Viscoelastic Vibrational Properties between 98 Wood Types. Ann. Forest. Sci. 2012, 69, 373–386. [Google Scholar] [CrossRef]
- Wegst, U.G.K. Wood for Sound. Am. J. Bot. 2006, 93, 1439–1448. [Google Scholar] [CrossRef]
- Ahmed, S.A.; Adamopoulos, S. Acoustic Properties of Modified Wood under Different Humid Conditions and Their Relevance for Musical Instruments. Appl. Acoust. 2018, 140, 92–99. [Google Scholar] [CrossRef]
- Mania, P.; Gąsiorek, M. Acoustic Properties of Resonant Spruce Wood Modified Using Oil-Heat Treatment (OHT). Materials 2020, 13, 1962. [Google Scholar] [CrossRef]
- Hassan, K.T.S.; Tippner, J. Acoustic Properties Assessment of Neem (Azadirachta Indica A. Juss.) Wood from Trees Irrigated with Secondarily Treated Wastewater. Bioresources 2019, 14, 2919–2930. [Google Scholar] [CrossRef]
- Abdolahian Sohi, A.M.; Khademi-Eslam, H.; Hemmasi, A.H.; Roohnia, M.; Talaiepour, M. Nondestructive Detection of the Effect of Drilling on Acoustic Performance of Wood. Bioresources 2011, 6, 2632–2646. [Google Scholar] [CrossRef]
- Zvoníček, T.; Vašina, M.; Pata, V.; Smolka, P. Three-Dimensional Printing Process for Musical Instruments: Sound Reflection Properties of Polymeric Materials for Enhanced Acoustical Performance. Polymers 2023, 15, 2025. [Google Scholar] [CrossRef]
- Tallarico, D.; Haslinger, S.G. Trapped Modes and Negative Refraction in a Locally Resonant Metamaterial: Transient Insights into Manufacturing Bounds for Ultrasonic Applications. Appl. Sci. 2021, 11, 7576. [Google Scholar] [CrossRef]
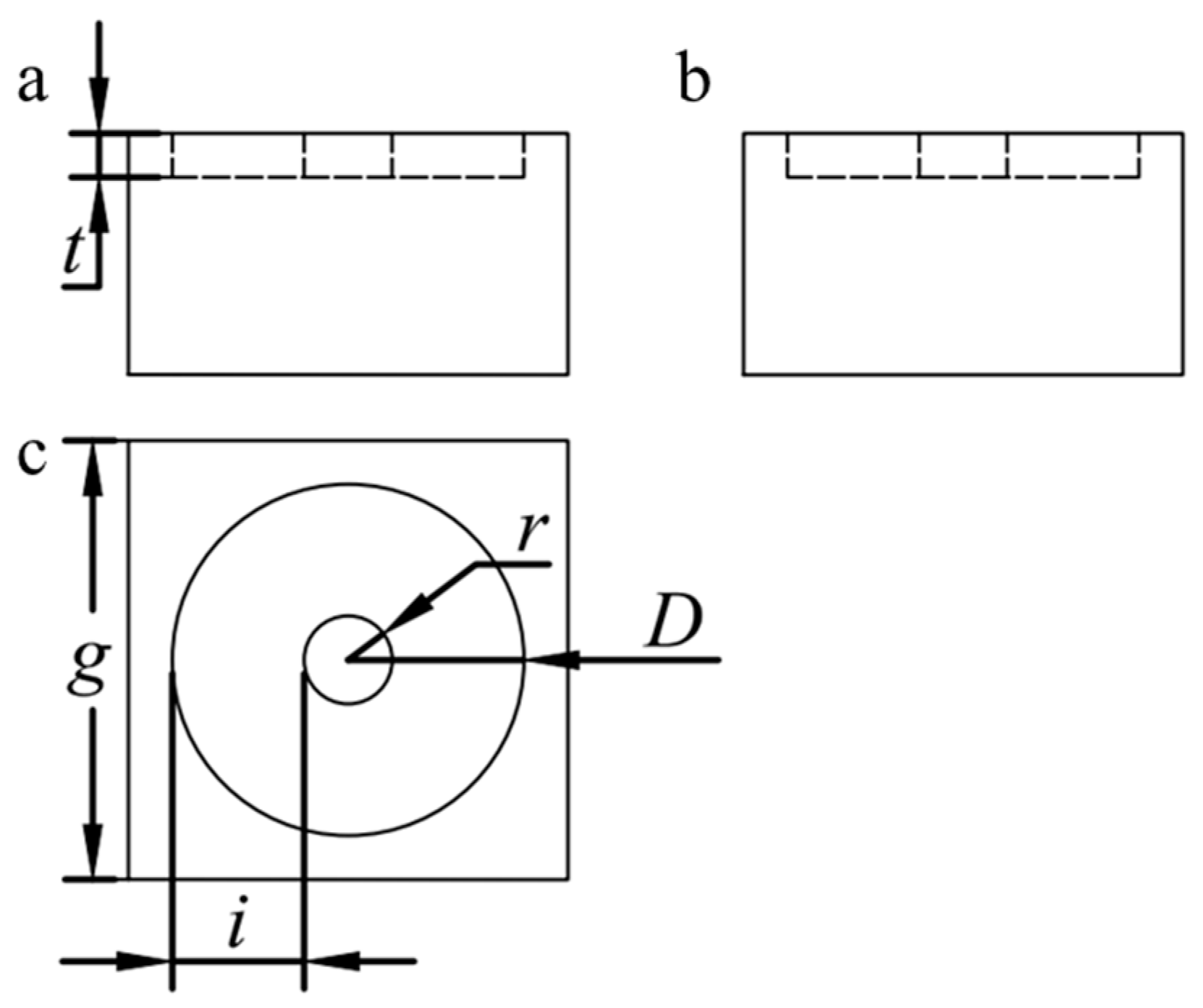

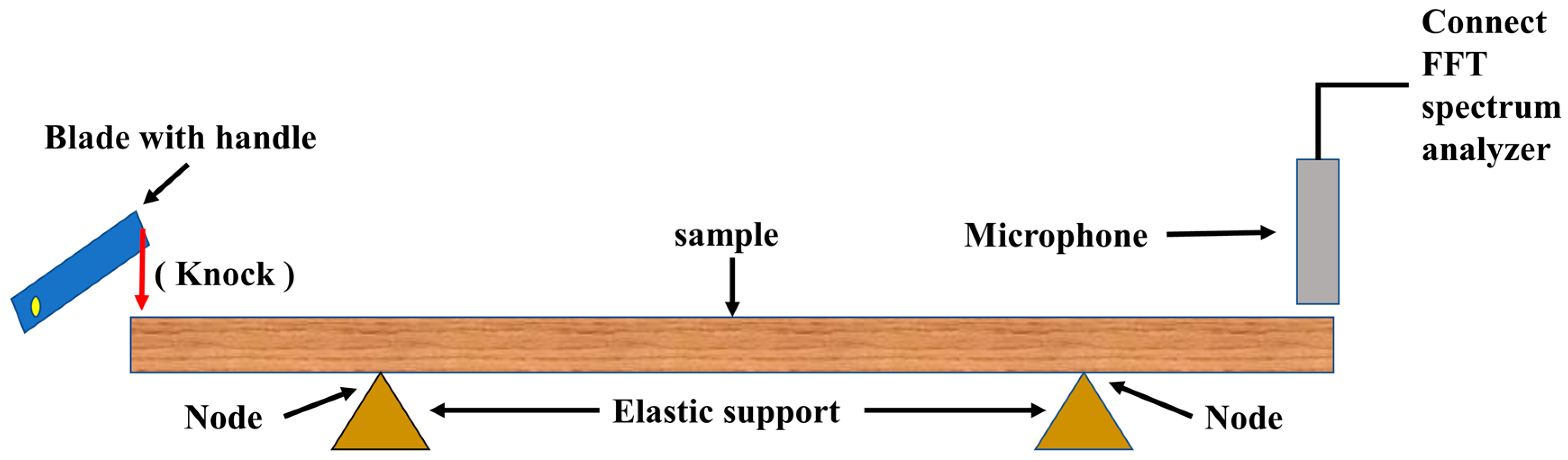


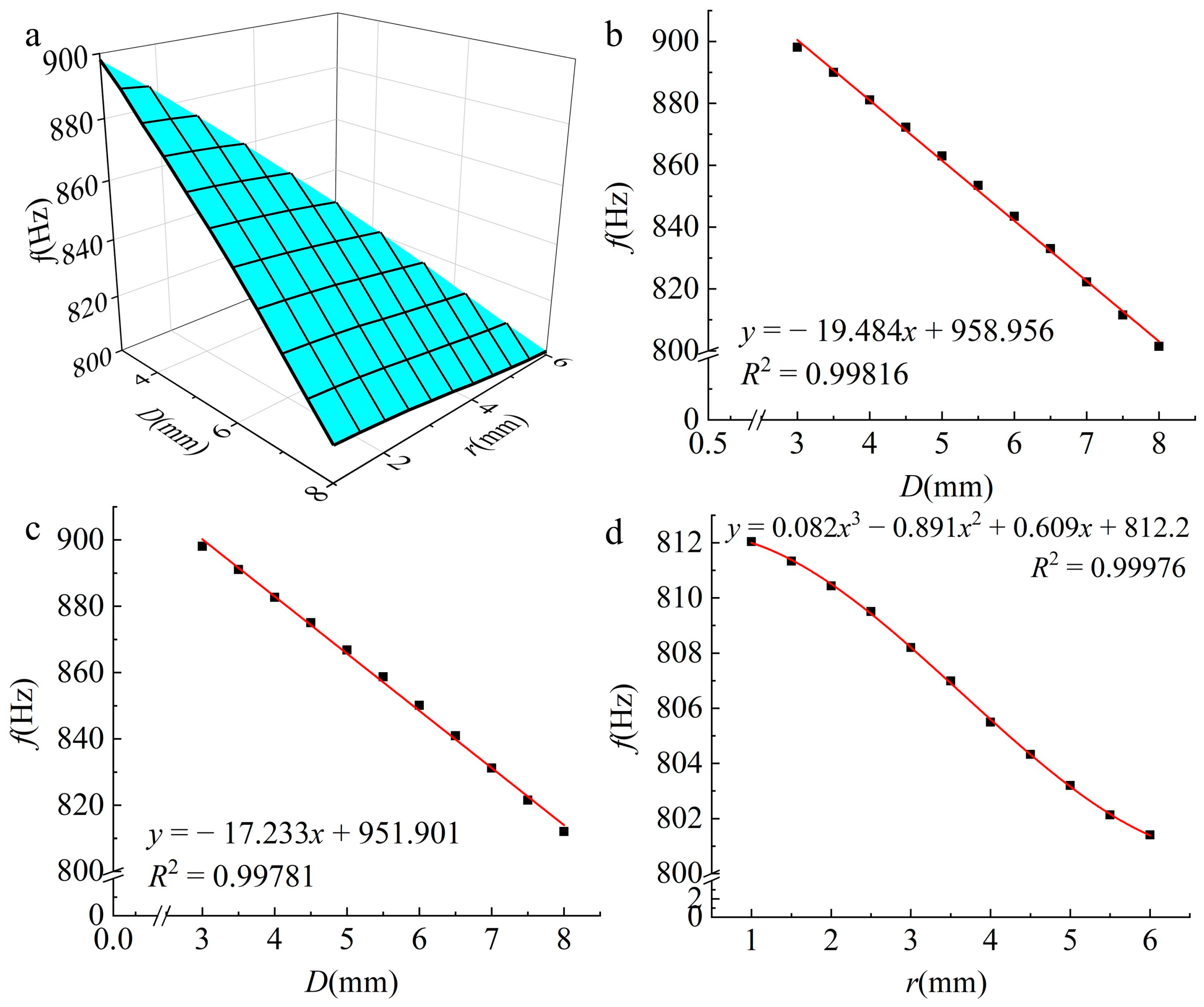
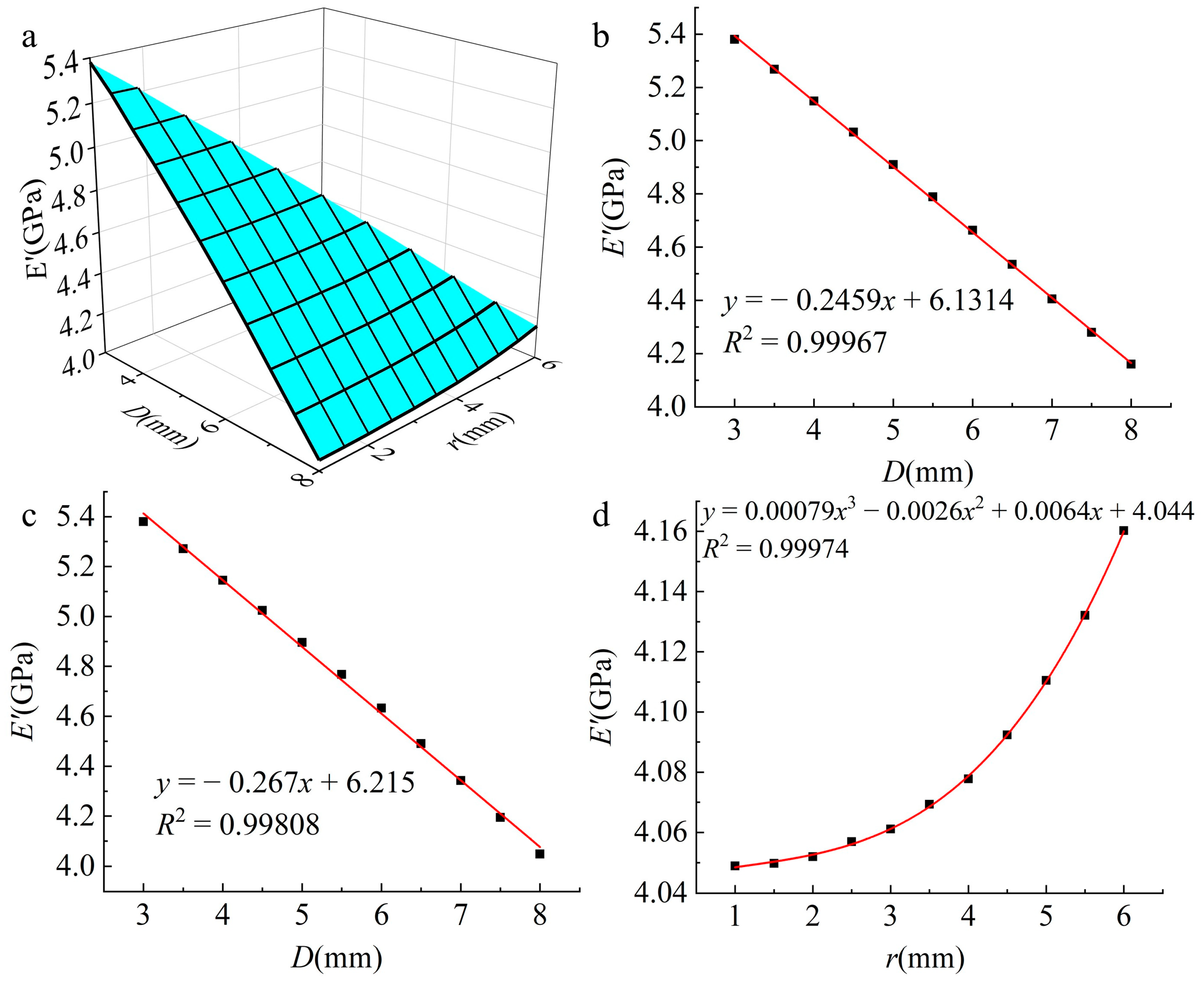


| Specimen Number | Length (mm) | Width (mm) | Height (mm) | Density (g·cm−3) |
|---|---|---|---|---|
| A | 240.6 | 20.15 | 11.00 | 0.2327 |
| B | 240.5 | 20.06 | 10.95 | 0.2038 |
| C | 240.6 | 20.14 | 10.98 | 0.2025 |
| D | 240.6 | 20.14 | 10.96 | 0.2307 |
| Radius of Outer Circle D (mm) | Radius of Inner Circle r (mm) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 3.0 | 1.0 (2.0) | ||||||||||
| 3.5 | 1.0 (2.5) | 1.5 (2.0) | |||||||||
| 4.0 | 1.0 (3.0) | 1.5 (2.5) | 2.0 (2.0) | ||||||||
| 4.5 | 1.0 (3.5) | 1.5 (3.0) | 2.0 (2.5) | 2.5 (2.0) | |||||||
| 5.0 | 1.0 (4.0) | 1.5 (3.5) | 2.0 (3.0) | 2.5 (2.5) | 3.0 (2.0) | ||||||
| 5.5 | 1.0 (4.5) | 1.5 (4.0) | 2.0 (3.5) | 2.5 (3.0) | 3.0 (2.5) | 3.5 (2.0) | |||||
| 6.0 | 1.0 (5.0) | 1.5 (4.5) | 2.0 (4.0) | 2.5 (3.5) | 3.0 (3.0) | 3.5 (2.5) | 4.0 (2.0) | ||||
| 6.5 | 1.0 (5.5) | 1.5 (5.0) | 2.0 (4.5) | 2.5 (4.0) | 3.0 (3.5) | 3.5 (3.0) | 4.0 (2.5) | 4.5 (2.0) | |||
| 7.0 | 1.0 (6.0) | 1.5 (5.5) | 2.0 (5.0) | 2.5 (4.5) | 3.0 (4.0) | 3.5 (3.5) | 4.0 (3.0) | 4.5 (2.5) | 5.0 (2.0) | ||
| 7.5 | 1.0 (6.5) | 1.5 (6.0) | 2.0 (5.5) | 2.5 (5.0) | 3.0 (4.5) | 3.5 (4.0) | 4.0 (3.5) | 4.5 (3.0) | 5.0 (2.5) | 5.5 (2.0) | |
| 8.0 | 1.0 (7.0) | 1.5 (6.5) | 2.0 (6.0) | 2.5 (5.5) | 3.0 (5.0) | 3.5 (4.5) | 4.0 (4.0) | 4.5 (3.5) | 5.0 (3.0) | 5.5 (2.5) | 6.0 (2.0) |
| Density (g·cm−3) | Young’s Modulus (GPa) | Shear Modulus (GPa) | Poisson’s Ratio | ||||||
|---|---|---|---|---|---|---|---|---|---|
| EL | ET | ER | GLT | GLR | GRT | μLT | μLR | μRT | |
| 0.260 | 6.20 | 1.03 | 2.96 | 0.300 | 0.200 | 0.033 | 0.15 | 0.02 | 0.08 |
| Specimen Number | D/mm | r/mm | i/mm |
|---|---|---|---|
| A5 | 5 | 2 | 3 |
| A6 | 6 | 2 | 4 |
| A7 | 7 | 2 | 5 |
| A8 | 8 | 2 | 6 |
| B5 | 8 | 5 | 3 |
| B4 | 8 | 4 | 4 |
| B3 | 8 | 3 | 5 |
| B2 | 8 | 2 | 6 |
| C0 | 7 | 3 | 4 |
| F0 | 5 | 1 | 4 |
| Specimen Number | mf (%) | mE′ (%) | mEsp′ (%) | mR′ (%) | mw′ (%) |
|---|---|---|---|---|---|
| A5 | 7.41 | 16.52 | 14.19 | 4.78 | 9.88 |
| A6 | 9.04 | 20.79 | 17.23 | 4.94 | 12.93 |
| A7 | 11.11 | 25.87 | 20.96 | 5.21 | 16.62 |
| A8 | 14.07 | 32.28 | 26.14 | 6.27 | 21.20 |
| B5 | 13.56 | 29.79 | 25.28 | 8.00 | 18.78 |
| B4 | 13.56 | 31.04 | 25.28 | 6.33 | 20.23 |
| B3 | 13.22 | 31.06 | 24.69 | 5.20 | 20.56 |
| B2 | 13.22 | 31.93 | 24.66 | 3.94 | 21.57 |
| C0 | 10.67 | 24.52 | 20.24 | 5.63 | 15.48 |
| D0 | 6.56 | 15.18 | 12.68 | 3.81 | 9.23 |
| D/mm | r/mm | i/mm | mf | mE′ | mEsp′ | mR′ | mw′ |
|---|---|---|---|---|---|---|---|
| 5 | 2 | 3 | 7.01% | 16.12% | 13.52% | 4.13% | 9.80% |
| 6 | 2 | 4 | 8.81% | 20.65% | 16.85% | 4.45% | 12.98% |
| 7 | 2 | 5 | 10.83% | 25.60% | 20.49% | 4.71% | 16.56% |
| 8 | 5 | 3 | 13.67% | 29.62% | 25.47% | 8.58% | 18.48% |
| 8 | 4 | 4 | 13.42% | 30.18% | 25.05% | 7.05% | 19.36% |
| 8 | 3 | 5 | 13.13% | 30.47% | 24.54% | 5.73% | 19.96% |
| 8 | 2 | 6 | 12.89% | 30.63% | 24.12% | 4.73% | 20.36% |
| 7 | 3 | 4 | 11.09% | 25.47% | 20.95% | 5.71% | 16.17% |
| 5 | 1 | 4 | 6.83% | 16.17% | 13.20% | 3.53% | 10.03% |
| Specimen Number | Xf | XE′ | XEsp′ | XR′ | Xw′ |
|---|---|---|---|---|---|
| A5 | −5.40% | −2.45% | −4.69% | −13.53% | −0.88% |
| A6 | −2.47% | −0.66% | −2.23% | −10.04% | 0.39% |
| A7 | −2.49% | −1.04% | −2.23% | −9.54% | −0.33% |
| A8 | −8.39% | −5.12% | −7.72% | −24.59% | −3.97% |
| B5 | 0.83% | −0.57% | 0.77% | 7.23% | −1.61% |
| B4 | −1.00% | −2.77% | −0.92% | 11.36% | −4.29% |
| B3 | −0.65% | −1.91% | −0.61% | 10.13% | −2.93% |
| B2 | −2.47% | −4.08% | −2.17% | 20.21% | −5.64% |
| C0 | 3.99% | 3.87% | 3.53% | 1.42% | 4.43% |
| F0 | 4.20% | 6.55% | 4.05% | −7.43% | 8.65% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L.; He, L.; Liang, Y.; Zhang, J.; Zhang, H.; Zhou, J.; Cui, H.; Li, M.; Miao, Y.; Liu, Z. Modulating the Acoustic Vibration Performance of Wood by Introducing a Periodic Annular Groove Structure. Forests 2023, 14, 2360. https://doi.org/10.3390/f14122360
Zhang L, He L, Liang Y, Zhang J, Zhang H, Zhou J, Cui H, Li M, Miao Y, Liu Z. Modulating the Acoustic Vibration Performance of Wood by Introducing a Periodic Annular Groove Structure. Forests. 2023; 14(12):2360. https://doi.org/10.3390/f14122360
Chicago/Turabian StyleZhang, Liang, Lan He, Yuwei Liang, Juncheng Zhang, Haiyang Zhang, Jing Zhou, Haotian Cui, Mingrui Li, Yuanyuan Miao, and Zhenbo Liu. 2023. "Modulating the Acoustic Vibration Performance of Wood by Introducing a Periodic Annular Groove Structure" Forests 14, no. 12: 2360. https://doi.org/10.3390/f14122360
APA StyleZhang, L., He, L., Liang, Y., Zhang, J., Zhang, H., Zhou, J., Cui, H., Li, M., Miao, Y., & Liu, Z. (2023). Modulating the Acoustic Vibration Performance of Wood by Introducing a Periodic Annular Groove Structure. Forests, 14(12), 2360. https://doi.org/10.3390/f14122360







