Long-Term Productivity of Monospecific and Mixed Oak (Quercus petraea [Matt.] Liebl. and Quercus robur L.) Stands in Germany: Growth Dynamics and the Effect of Stand Structure
Abstract
:1. Introduction
- Is there a discernible long-term growth trend in oak stands over the last century?
- How does productivity of monospecific and mixed oak stands depend on stand structure, site conditions and stand development phase?
- How is productivity of individual oak trees related to their social status, vertical stand structure, mixture type and age?
- What is the contribution of small tree individuals to stand growth?
2. Materials and Methods
2.1. Tree Species
2.2. Research Plots
2.3. Productivity and Growth Dominance
2.4. Individual Tree and Stand Characteristics
2.5. Statistical Analyses
3. Results
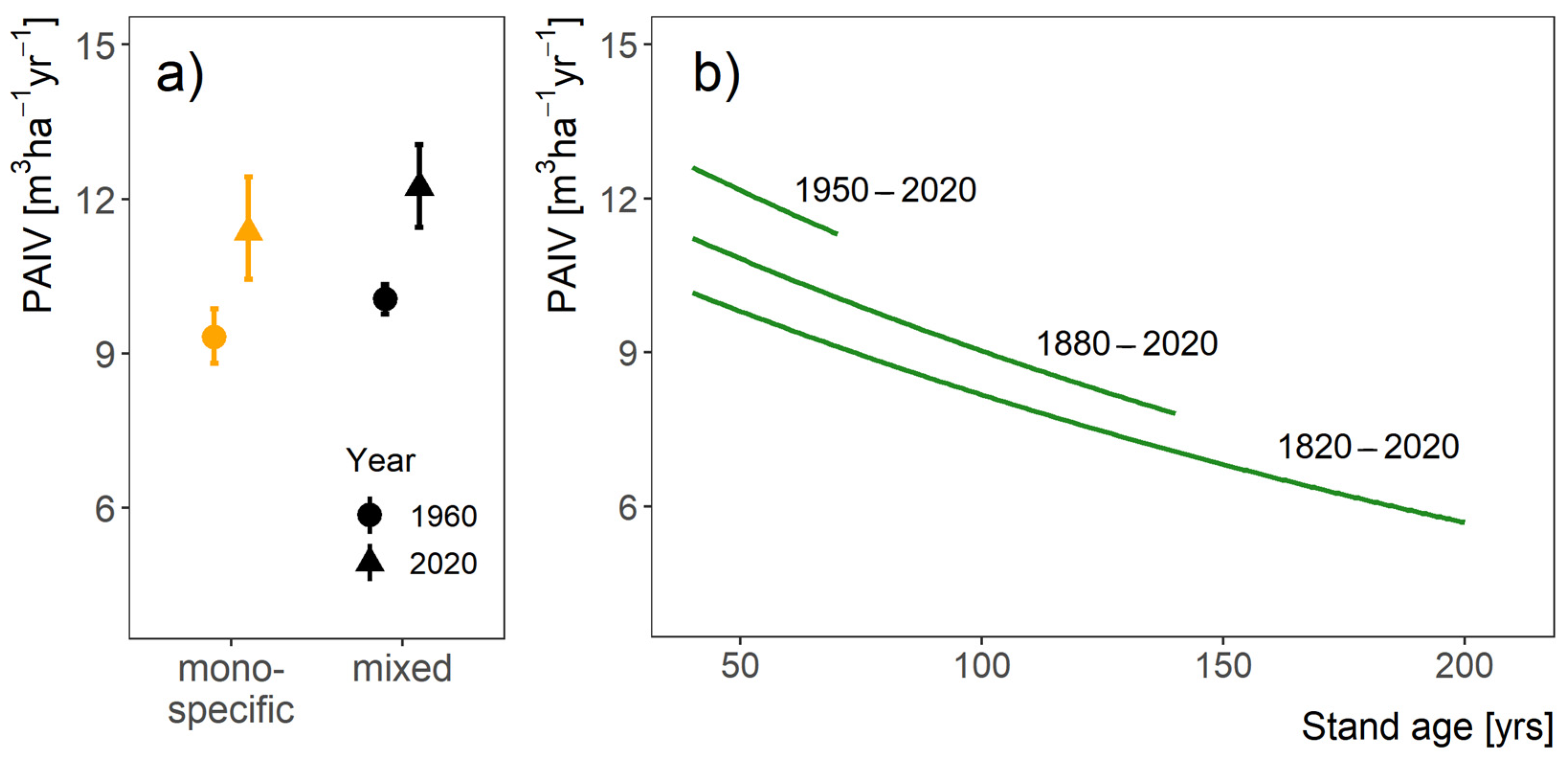
3.1. Long-Term Growth Trends of Oak Stands
3.2. Stand Productivity as Modulated by Stand Characteristics
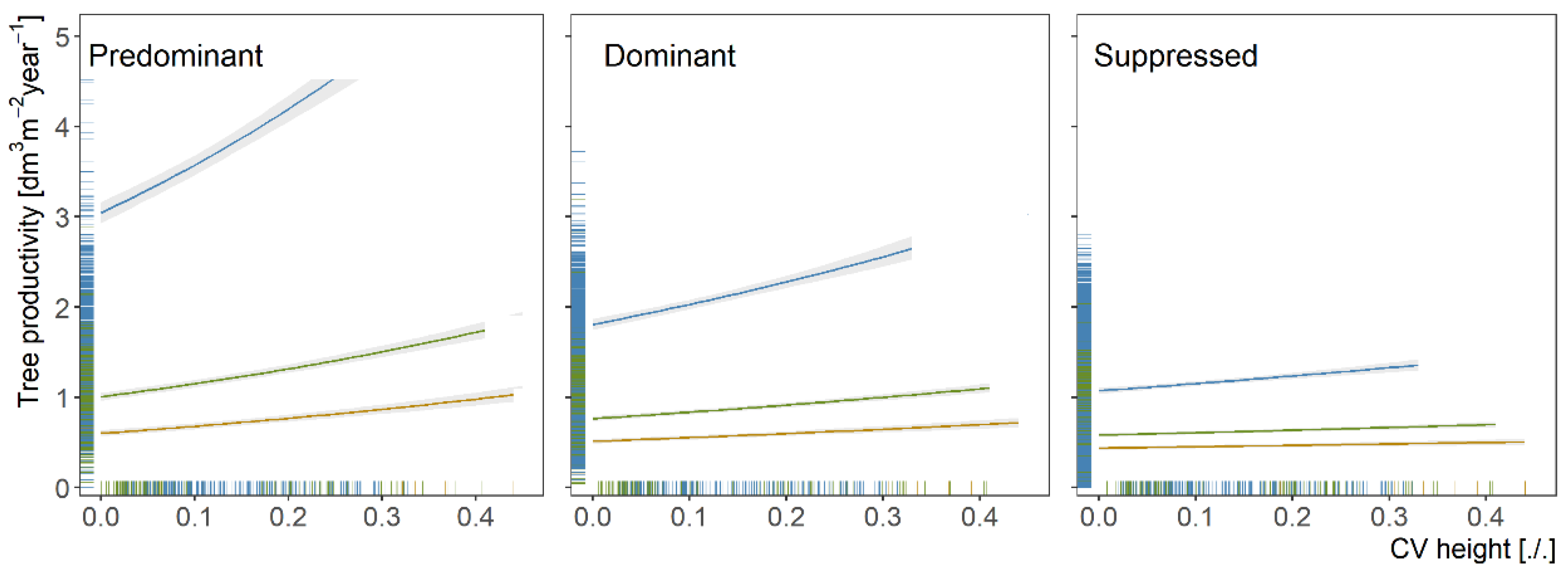
3.3. Individual Tree Productivity of Oaks Depending on Tree and Stand Characteristics
3.4. Stand Growth Partitioning between Trees of Different Sizes
4. Discussion
4.1. Long-Term Growth Trends
4.2. Stand Productivity
4.3. Tree Productivity
4.4. Growth Partitioning
4.5. Implications for Silvicultural Management Strategies
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bolte, A.; Ammer, C.; Löf, M.; Madsen, P.; Nabuurs, G.-J.; Schall, P.; Spathelf, P.; Rock, J. Adaptive forest management in central Europe: Climate change impacts, strategies and integrative concept. Scand. J. For. Res. 2009, 24, 473–482. [Google Scholar] [CrossRef]
- Lindner, M. Developing adaptive forest management strategies to cope with climate change. Tree Physiol. 2000, 20, 299–307. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lindner, M.; Maroschek, M.; Netherer, S.; Kremer, A.; Barbati, A.; Garcia-Gonzalo, J.; Seidl, R.; Delzon, S.; Corona, P.; Kolström, M.; et al. Climate change impacts, adaptive capacity, and vulnerability of European forest ecosystems. For. Ecol. Manag. 2010, 259, 698–709. [Google Scholar] [CrossRef]
- Brockerhoff, E.G.; Barbaro, L.; Castagneyrol, B.; Forrester, D.I.; Gardiner, B.; González-Olabarria, J.R.; Lyver, P.O.; Meurisse, N.; Oxbrough, A.; Taki, H.; et al. Forest biodiversity, ecosystem functioning and the provision of ecosystem services. Biodivers. Conserv. 2017, 26, 3005–3035. [Google Scholar] [CrossRef] [Green Version]
- Pretzsch, H.; del Río, M.; Biber, P.; Arcangeli, C.; Bielak, K.; Brang, P.; Dudzinska, M.; Forrester, D.I.; Klädtke, J.; Kohnle, U.; et al. Maintenance of long-term experiments for unique insights into forest growth dynamics and trends: Review and perspectives. Eur. J. For. Res. 2019, 138, 165–185. [Google Scholar] [CrossRef] [Green Version]
- Pretzsch, H.; Biber, P.; Schütze, G.; Uhl, E.; Rötzer, T. Forest stand growth dynamics in Central Europe have accelerated since 1870. Nat. Commun. 2014, 5, 4967. [Google Scholar] [CrossRef] [Green Version]
- Pretzsch, H.; Bielak, K.; Block, J.; Bruchwald, A.; Dieler, J.; Ehrhart, H.-P.; Kohnle, U.; Nagel, J.; Spellmann, H.; Zasada, M.; et al. Productivity of mixed versus pure stands of oak (Quercus petraea (Matt.) Liebl. and Quercus robur L.) and European beech (Fagus sylvatica L.) along an ecological gradient. Eur. J. For. Res. 2013, 132, 263–280. [Google Scholar] [CrossRef]
- Pretzsch, H.; Steckel, M.; Heym, M.; Biber, P.; Ammer, C.; Ehbrecht, M.; Bielak, K.; Bravo, F.; Ordóñez, C.; Collet, C.; et al. Stand growth and structure of mixed-species and monospecific stands of Scots pine (Pinus sylvestris L.) and oak (Q. robur L., Quercus petraea (Matt.) Liebl.) analysed along a productivity gradient through Europe. Eur. J. For. Res. 2019, 139, 349–367. [Google Scholar] [CrossRef] [Green Version]
- Río, M.; Sterba, H. Comparing volume growth in pure and mixed stands of Pinus sylvestris and Quercus pyrenaica. Ann. For. Sci. 2009, 66, 502. [Google Scholar] [CrossRef] [Green Version]
- Liang, J.; Crowther, T.W.; Picard, N.; Wiser, S.; Zhou, M.; Alberti, G.; Schulze, E.-D.; McGuire, A.D.; Bozzato, F.; Pretzsch, H.; et al. Positive biodiversity-productivity relationship predominant in global forests. Science 2016, 354, aaf8957. [Google Scholar] [CrossRef] [Green Version]
- Vandermeer, J.H. The Ecology of Intercropping; Cambridge University Press: Cambridge, UK, 1989; ISBN 9780521345927. [Google Scholar]
- Pretzsch, H.; Block, J.; Dieler, J.; Dong, P.H.; Kohnle, U.; Nagel, J.; Spellmann, H.; Zingg, A. Comparison between the productivity of pure and mixed stands of Norway spruce and European beech along an ecological gradient. Ann. For. Sci. 2010, 67, 712. [Google Scholar] [CrossRef] [Green Version]
- Pretzsch, H.; Biber, P. Tree species mixing can increase maximum stand density. Can. J. For. Res. 2016, 46, 1179–1193. [Google Scholar] [CrossRef] [Green Version]
- Condés, S.; Del Rio, M.; Sterba, H. Mixing effect on volume growth of Fagus sylvatica and Pinus sylvestris is modulated by stand density. For. Ecol. Manag. 2013, 292, 86–95. [Google Scholar] [CrossRef] [Green Version]
- Brunner, A.; Forrester, D.I. Tree species mixture effects on stem growth vary with stand density—An analysis based on individual tree responses. For. Ecol. Manag. 2020, 473, 118334. [Google Scholar] [CrossRef]
- Zeller, L.; Pretzsch, H. Effect of forest structure on stand productivity in Central European forests depends on developmental stage and tree species diversity. For. Ecol. Manag. 2019, 434, 193–204. [Google Scholar] [CrossRef]
- Forrester, D.I. Linking forest growth with stand structure: Tree size inequality, tree growth or resource partitioning and the asymmetry of competition. For. Ecol. Manag. 2019, 447, 139–157. [Google Scholar] [CrossRef]
- Forrester, D.I.; Pretzsch, H. Tamm Review: On the strength of evidence when comparing ecosystem functions of mixtures with monocultures. For. Ecol. Manag. 2015, 356, 41–53. [Google Scholar] [CrossRef]
- Manso, R.; Morneau, F.; Ningre, F.; Fortin, M. Effect of climate and intra- and inter-specific competition on diameter increment in beech and oak stands. Forestry 2015, 88, 540–551. [Google Scholar] [CrossRef]
- del Río, M.; Condés, S.; Pretzsch, H. Analyzing size-symmetric vs. size-asymmetric and intra- vs. inter-specific competition in beech (Fagus sylvatica L.) mixed stands. For. Ecol. Manag. 2014, 325, 90–98. [Google Scholar] [CrossRef] [Green Version]
- Ammer, C. Diversity and forest productivity in a changing climate. New Phytol. 2019, 221, 50–66. [Google Scholar] [CrossRef] [Green Version]
- del Río, M.; Pretzsch, H.; Alberdi, I.; Bielak, K.; Bravo, F.; Brunner, A.; Condés, S.; Ducey, M.J.; Fonseca, T.; von Lüpke, N.; et al. Characterization of the structure, dynamics, and productivity of mixed-species stands: Review and perspectives. Eur. J. For. Res. 2016, 135, 23–49. [Google Scholar] [CrossRef]
- Forrester, D.I. The spatial and temporal dynamics of species interactions in mixed-species forests: From pattern to process. For. Ecol. Manag. 2014, 312, 282–292. [Google Scholar] [CrossRef]
- Binkley, D. A hypothesis about the interaction of tree dominance and stand production through stand development. For. Ecol. Manag. 2004, 190, 265–271. [Google Scholar] [CrossRef]
- Binkley, D.; Kashian, D.M.; Boyden, S.; Kaye, M.W.; Bradford, J.B.; Arthur, M.A.; Fornwalt, P.J.; Ryan, M.G. Patterns of growth dominance in forests of the Rocky Mountains, USA. For. Ecol. Manag. 2006, 236, 193–201. [Google Scholar] [CrossRef]
- Pothier, D. Relationships between patterns of stand growth dominance and tree competition mode for species of various shade tolerances. For. Ecol. Manag. 2017, 406, 155–162. [Google Scholar] [CrossRef]
- Fernández-Tschieder, E.; Binkley, D. Linking competition with Growth Dominance and production ecology. For. Ecol. Manag. 2018, 414, 99–107. [Google Scholar] [CrossRef]
- Pretzsch, H. The social drift of trees. Consequence for growth trend detection, stand dynamics, and silviculture. Eur. J. For. Res. 2021, 140, 703–719. [Google Scholar] [CrossRef]
- Albert, M.; Nagel, R.-V.; Nuske, R.; Sutmöller, J.; Spellmann, H. Tree Species Selection in the Face of Drought Risk—Uncertainty in Forest Planning. Forests 2017, 8, 363. [Google Scholar] [CrossRef]
- Leuschner, C.; Ellenberg, H. Ecology of Central European Forests: Vegetation Ecology of Central Europe; Springer: Cham, Switzerland, 2017; Volume 1, ISBN 978-3-319-43040-9. [Google Scholar]
- Pretzsch, H.; Schütze, G.; Uhl, E. Resistance of European tree species to drought stress in mixed versus pure forests: Evidence of stress release by inter-specific facilitation: Drought stress release by inter-specific facilitation. Plant Biol. 2013, 15, 483–495. [Google Scholar] [CrossRef]
- Steckel, M.; del Río, M.; Heym, M.; Aldea, J.; Bielak, K.; Brazaitis, G.; Černý, J.; Coll, L.; Collet, C.; Ehbrecht, M.; et al. Species mixing reduces drought susceptibility of Scots pine (Pinus sylvestris L.) and oak (Quercus robur L., Quercus petraea (Matt.) Liebl.)—Site water supply and fertility modify the mixing effect. For. Ecol. Manag. 2020, 461, 117908. [Google Scholar] [CrossRef]
- Attocchi, G. Silviculture of Oak for High-Quality Wood Production: Effects of Thinning on Crown Size, Volume Growth and Stem Quality in Even-Aged Stands of Pedunculate Oak (Quercus robur L.) in Northern Europe; Southern Swedish Forest Research Centre, Swedish University of Agricultural Sciences: Alnarp, Sweden, 2015; ISBN 978-91-576-8277-2. [Google Scholar]
- Kenk, G.K. New perspectives in German oak silviculture. Ann. For. Sci. 1993, 50, 563–570. [Google Scholar] [CrossRef] [Green Version]
- von Lüpke, B. Silvicultural methods of oak regeneration with special respect to shade tolerant mixed species. For. Ecol. Manag. 1998, 106, 19–26. [Google Scholar] [CrossRef]
- Löf, M.; Brunet, J.; Filyushkina, A.; Lindbladh, M.; Skovsgaard, J.P.; Felton, A. Management of oak forests: Striking a balance between timber production, biodiversity and cultural services. Int. J. Biodivers. Sci. Ecosyst. Serv. Manag. 2016, 12, 59–73. [Google Scholar] [CrossRef] [Green Version]
- Mölder, A.; Meyer, P.; Nagel, R.-V. Integrative management to sustain biodiversity and ecological continuity in Central European temperate oak (Quercus robur, Q. petraea) forests: An overview. For. Ecol. Manag. 2019, 437, 324–339. [Google Scholar] [CrossRef]
- Bayerische Staatsforsten AöR. Waldbauhandbuch Bayersiche Staatsforsten: Richtlinie zur Baumartenwahl; Bayerische Staatsforsten: Regensburg, Germany, 2020. [Google Scholar]
- Bayerisches Staatsministerium für Ernährung, Landwirtschaft und Forsten. Baumarten für den Klimawald; Leitlinien der Bayerischen Forstverwaltung; Bayerisches Staatsministerium für Ernährung, Landwirtschaft und Forsten: Munich, Germany, 2020. [Google Scholar]
- Leroy, B.M.L.; Lemme, H.; Braumiller, P.; Hilmers, T.; Jacobs, M.; Hochrein, S.; Kienlein, S.; Müller, J.; Pretzsch, H.; Stimm, K.; et al. Relative impacts of gypsy moth outbreaks and insecticide treatments on forest resources and ecosystems: An experimental approach. Ecol. Solut. Evid. 2021, 2, e12045. [Google Scholar] [CrossRef]
- Field, E.; Castagneyrol, B.; Gibbs, M.; Jactel, H.; Barsoum, N.; Schönrogge, K.; Hector, A. Associational resistance to both insect and pathogen damage in mixed forests is modulated by tree neighbour identity and drought. J. Ecol. 2020, 108, 1511–1522. [Google Scholar] [CrossRef]
- Maleki, K.; Zeller, L.; Pretzsch, H. Oak often needs to be promoted in mixed beech-oak stands—The structural processes behind competition and silvicultural management in mixed stands of European beech and sessile oak. iForest 2020, 13, 80–88. [Google Scholar] [CrossRef] [Green Version]
- Stimm, K.; Heym, M.; Uhl, E.; Tretter, S.; Pretzsch, H. Height growth-related competitiveness of oak (Quercus petraea (Matt.) Liebl. and Quercus robur L.) under climate change in Central Europe. Is silvicultural assistance still required in mixed-species stands? For. Ecol. Manag. 2021, 482, 118780. [Google Scholar] [CrossRef]
- Beinhofer, B. Comparing the financial performance of traditionally managed beech and oak stands with roomy established and pruned stands. Eur. J. For. Res. 2010, 129, 175–187. [Google Scholar] [CrossRef]
- Weaver, G.T.; Spiecker, H. Silviculture of high-quality oaks: Questions and future research needs. Ann. Sci For. 1993, 50, 531–534. [Google Scholar] [CrossRef] [Green Version]
- Aas, G. Quercus petraea (Matt.) Liebl., Traubeneiche. In Enzyklopädie der Holzgewächse; Roloff, A., Weisgerber, H., Lang, U., Stimm, B., Eds.; Wiley-VCH: Weinheim, Germany, 2000. [Google Scholar]
- Aas, G. Quercus robur L., Stieleiche. In Enzyklopädie der Holzgewächse; Roloff, A., Weisgerber, H., Lang, U., Stimm, B., Eds.; Wiley-VCH: Weinheim, Germany, 2002; pp. 1–15. [Google Scholar]
- Jüttner, O. Eichenertragstafeln. In Ertragstafeln der Wichtigsten Baumarten; Schober, R., Ed.; JD Sauerländer´s Verlag: Frankfurt am Main, Germany, 1955; pp. 12–25, 134–138. [Google Scholar]
- Johann, K. DESER-Norm 1993. Normen der Sektion Ertragskunde im Deutschen Verband Forstlicher Forschungsanstalten zur Aufbereitung von waldwirtschaftlichen Dauerversuchen. Ber. Der Jahrestag. Des Dtsch. Verb. Forstl. Sekt. Ertragskunde 1993, 96–104. [Google Scholar]
- Schwaiger, F.; Poschenrieder, W.; Biber, P.; Pretzsch, H. Species Mixing Regulation with Respect to Forest Ecosystem Service Provision. Forests 2018, 9, 632. [Google Scholar] [CrossRef] [Green Version]
- West, P.W. Calculation of a Growth Dominance Statistic for Forest Stands. For. Sci. 2014, 60, 1021–1023. [Google Scholar] [CrossRef]
- Reineke, L.H. Perfecting a stand-density index for even-aged forests. J. Agric. Res. 1933, 46, 627–638. [Google Scholar]
- Kramer, H.; Akça, A. Leitfaden zur Waldmesslehre, 3rd ed.; Sauerländer: Frankfurt am Main, Germany, 1995; ISBN 9783793908807. [Google Scholar]
- Burschel, P.; Huss, J. Grundriß des Waldbaus: Ein Leitfaden für Studium und Praxis, 2nd ed.; Parey: Berlin, Germany, 1997; ISBN 3826330455. [Google Scholar]
- Zuur, A.F.; Ieno, E.N.; Walker, N.; Saveliev, A.A.; Smith, G.M. (Eds.) Mixed Effects Models and Extensions in Ecology with R; Springer: New York, NY, USA, 2009; ISBN 978-0-387-87457-9. [Google Scholar]
- R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2018. [Google Scholar]
- Bates, D.; Maechler, M.; Bolker, B.; Walker, S. Fitting Linear Mixed-Effects Models Using lme4. J. Stat. Softw. 2015, 67, 1–48. [Google Scholar] [CrossRef]
- Wood, S.N. Generalized Additive Models: An Introduction with R, 2nd ed.; Chapman and Hall/CRC: Boca Raton, FL, USA, 2017; ISBN 9781315370279. [Google Scholar]
- Wickham, H. Ggplot2: Elegant Graphic.cs for Data Analysis, 2nd ed.; Springer: New York, NY, USA, 2016; ISBN 978-3-319-24277-4. [Google Scholar]
- Spiecker, H. Overview of Recent Growth Trends in European Forests. In Forest Growth Responses to the Pollution Climate of the 21st Century; Sheppard, L.J., Cape, J.N., Eds.; Springer: Dordrecht, The Netherlands, 1999; pp. 33–46. ISBN 978-94-017-1578-2. [Google Scholar]
- Bontemps, J.-D.; Hervé, J.-C.; Dhôte, J.-F. Long-Term Changes in Forest Productivity: A Consistent Assessment in Even-Aged Stands. For. Sci. 2009, 55, 549–564. [Google Scholar] [CrossRef]
- Bontemps, J.-D.; Hervé, J.-C.; Duplat, P.; Dhôte, J.-F. Shifts in the height-related competitiveness of tree species following recent climate warming and implications for tree community composition: The case of common beech and sessile oak as predominant broadleaved species in Europe. Oikos 2012, 121, 1287–1299. [Google Scholar] [CrossRef] [Green Version]
- Pretzsch, H.; Biber, P.; Schütze, G.; Bielak, K. Changes of forest stand dynamics in Europe. Facts from long-term observational plots and their relevance for forest ecology and management. For. Ecol. Manag. 2014, 316, 65–77. [Google Scholar] [CrossRef]
- Hilmers, T.; Avdagić, A.; Bartkowicz, L.; Bielak, K.; Binder, F.; Bončina, A.; Dobor, L.; Forrester, D.I.; Hobi, M.L.; Ibrahimspahić, A.; et al. The productivity of mixed mountain forests comprised of Fagus sylvatica, Picea abies, and Abies alba across Europe. For. An. Int. J. For. Res. 2019, 92, 512–522. [Google Scholar] [CrossRef] [Green Version]
- Vannoppen, A.; Kint, V.; Ponette, Q.; Verheyen, K.; Muys, B. Tree species diversity impacts average radial growth of beech and oak trees in Belgium, not their long-term growth trend. For. Ecosyst. 2019, 6, 252. [Google Scholar] [CrossRef] [Green Version]
- Fajvan, M.A.; Rentch, J.; Gottschalk, K. The effects of thinning and gypsy moth defoliation on wood volume growth in oaks. Trees 2008, 22, 257–268. [Google Scholar] [CrossRef]
- Forrester, D.I.; Bauhus, J. A Review of Processes Behind Diversity—Productivity Relationships in Forests. Curr. For. Rep. 2016, 2, 45–61. [Google Scholar] [CrossRef] [Green Version]
- Pretzsch, H.; Schütze, G. Effect of tree species mixing on the size structure, density, and yield of forest stands. Eur. J. For. Res. 2016, 135, 1–22. [Google Scholar] [CrossRef]
- Dieler, J.; Uhl, E.; Biber, P.; Müller, J.; Rötzer, T.; Pretzsch, H. Effect of forest stand management on species composition, structural diversity, and productivity in the temperate zone of Europe. Eur. J. For. Res. 2017, 136, 739–766. [Google Scholar] [CrossRef]
- Pretzsch, H. Canopy space filling and tree crown morphology in mixed-species stands compared with monocultures. For. Ecol. Manag. 2014, 327, 251–264. [Google Scholar] [CrossRef] [Green Version]
- Torresan, C.; del Río, M.; Hilmers, T.; Notarangelo, M.; Bielak, K.; Binder, F.; Boncina, A.; Bosela, M.; Forrester, D.I.; Hobi, M.L.; et al. Importance of tree species size dominance and heterogeneity on the productivity of spruce-fir-beech mountain forest stands in Europe. For. Ecol. Manag. 2020, 457, 117716. [Google Scholar] [CrossRef]
- Hein, S.; Dhôte, J.-F. Effect of species composition, stand density and site index on the basal area increment of oak trees (Quercus sp.) in mixed stands with beech (Fagus sylvatica L.) in northern France. Ann. For. Sci. 2006, 63, 457–467. [Google Scholar] [CrossRef] [Green Version]
- Pretzsch, H.; Schütze, G. Crown allometry and growing space efficiency of Norway spruce (Picea abies L. Karst.) and European beech (Fagus sylvatica L.) in pure and mixed stands. Plant Biol. 2005, 7, 628–639. [Google Scholar] [CrossRef] [Green Version]
- Dieler, J.; Pretzsch, H. Morphological plasticity of European beech (Fagus sylvatica L.) in pure and mixed-species stands. For. Ecol. Manag. 2013, 295, 97–108. [Google Scholar] [CrossRef]
- Zapater, M.; Hossann, C.; Bréda, N.; Bréchet, C.; Bonal, D.; Granier, A. Evidence of hydraulic lift in a young beech and oak mixed forest using 18O soil water labelling. Trees 2011, 25, 885–894. [Google Scholar] [CrossRef]
- Pretzsch, H. Grundlagen der Waldwachstumsforschung; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar]
- Pretzsch, H. The course of tree growth. Theory and reality. For. Ecol. Manag. 2020, 478, 118508. [Google Scholar] [CrossRef]
- Del Río, M.; Schütze, G.; Pretzsch, H. Temporal variation of competition and facilitation in mixed species forests in Central Europe. Plant Biol. 2014, 16, 166–176. [Google Scholar] [CrossRef] [PubMed]
- Vanhellemont, M.; Bijlsma, R.-J.; de Keersmaeker, L.; Vandekerkhove, K.; Verheyen, K. Species and structural diversity affect growth of oak, but not pine, in uneven-aged mature forests. Basic Appl. Ecol. 2018, 27, 41–50. [Google Scholar] [CrossRef]
- Pretzsch, H.; Biber, P. A re-evaluation of Reineke’s rule and stand density index. For. Sci. 2005, 51, 304–320. [Google Scholar]
- de Groote, S.R.; Vanhellemont, M.; Baeten, L.; van den Bulcke, J.; Martel, A.; Bonte, D.; Lens, L.; Verheyen, K. Competition, tree age and size drive the productivity of mixed forests of pedunculate oak, beech and red oak. For. Ecol. Manag. 2018, 430, 609–617. [Google Scholar] [CrossRef]
- Toïgo, M.; Perot, T.; Courbaud, B.; Castagneyrol, B.; Gégout, J.-C.; Longuetaud, F.; Jactel, H.; Vallet, P.; Hector, A. Difference in shade tolerance drives the mixture effect on oak productivity. J. Ecol. 2018, 106, 1073–1082. [Google Scholar] [CrossRef]
- Baret, M.; Pepin, S.; Ward, C.; Pothier, D. Long-term changes in stand growth dominance as related to resource acquisition and utilization in the boreal forest. For. Ecol. Manag. 2017, 400, 408–416. [Google Scholar] [CrossRef]
- Knoke, T.; Ammer, C.; Stimm, B.; Mosandl, R. Admixing broadleaved to coniferous tree species: A review on yield, ecological stability and economics. Eur. J. For. Res. 2008, 127, 89–101. [Google Scholar] [CrossRef]
- Paul, C.; Brandl, S.; Friedrich, S.; Falk, W.; Härtl, F.; Knoke, T. Climate change and mixed forests: How do altered survival probabilities impact economically desirable species proportions of Norway spruce and European beech? Ann. For. Sci. 2019, 76, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Puettmann, K.J.; Messier, C. Simple Guidelines to Prepare Forests for Global Change: The Dog and the Frisbee. NWSC 2019, 93, 209. [Google Scholar] [CrossRef]
- Brändle, M.; Brandl, R. Species richness of insects and mites on trees: Expanding Southwood. J. Anim. Ecol. 2001, 70, 491–504. [Google Scholar] [CrossRef]
- Manos, P.S.; Stanford, A.M. The Historical Biogeography of Fagaceae: Tracking the Tertiary History of Temperate and Subtropical Forests of the Northern Hemisphere. Int. J. Plant Sci. 2001, 162, S77–S93. [Google Scholar] [CrossRef]
- van der Plas, F.; Ratcliffe, S.; Ruiz-Benito, P.; Scherer-Lorenzen, M.; Verheyen, K.; Wirth, C.; Zavala, M.A.; Ampoorter, E.; Baeten, L.; Barbaro, L.; et al. Continental mapping of forest ecosystem functions reveals a high but unrealised potential for forest multifunctionality. Ecol. Lett. 2018, 21, 31–42. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Penone, C.; Allan, E.; Soliveres, S.; Felipe-Lucia, M.R.; Gossner, M.M.; Seibold, S.; Simons, N.K.; Schall, P.; van der Plas, F.; Manning, P.; et al. Specialisation and diversity of multiple trophic groups are promoted by different forest features. Ecol. Lett. 2019, 22, 170–180. [Google Scholar] [CrossRef] [PubMed]
- Pretzsch, H.; Zenner, E.K. Toward managing mixed-species stands: From parametrization to prescription. For. Ecosyst. 2017, 4, 1–17. [Google Scholar] [CrossRef] [Green Version]
- Pretzsch, H.; Poschenrieder, W.; Uhl, E.; Brazaitis, G.; Makrickiene, E.; Calama, R. Silvicultural prescriptions for mixed-species forest stands. A European review and perspective. Eur. J. For. Res. 2021, 140, 1267–1294. [Google Scholar] [CrossRef]
- Pretzsch, H.; del Río, M. Density regulation of mixed and mono-specific forest stands as a continuum: A new concept based on species-specific coefficients for density equivalence and density modification. Forestry 2020, 93, 1–15. [Google Scholar] [CrossRef]
- Thurm, E.A.; Pretzsch, H. Growth–density relationship in mixed stands—Results from long-term experimental plots. For. Ecol. Manag. 2021, 483, 118909. [Google Scholar] [CrossRef]
- Petritan, I.C.; Marzano, R.; Petritan, A.M.; Lingua, E. Overstory succession in a mixed Quercus petraea–Fagus sylvatica old growth forest revealed through the spatial pattern of competition and mortality. For. Ecol. Manag. 2014, 326, 9–17. [Google Scholar] [CrossRef]
- Mosandl, R.; Abt, A. Waldbauverfahren in Eichenwäldern gestern und heute. AFZ-Der Wald 2016, 20, 28–32. [Google Scholar]
- Krahl-Urban, J. Die Eichen: Forstliche Monographie der Traubeneiche und der Stieleiche; P. Parey: Hamburg, Germany, 1959. [Google Scholar]




| N | n | S [ha] | Per | Int [yrs] | hq 100 [m] | Prec [mm] | Temp [°C] | Alt [m a.s.l.] | |
|---|---|---|---|---|---|---|---|---|---|
| Long-term Experiments | 32 | 112 | 0.37 | 1898–2020 | 7 | 26.1 | 772 | 8.2 | 364 |
| 0.03–1.0 | 3–22 | 16.9–34.5 | 570–1019 | 7.4–9.5 | 37–534 | ||||
| Strict Forest Reserves (SFR) | 25 | 25 | 0.9 | 1977–2017 | 14 | 25.7 | 774 | 8.1 | 429 |
| 0.1–1.8 | 3–29 | 15.6–33.6 | 643–1174 | 7.7–8.9 | 286–579 | ||||
| Temporary Experiments | 5 | 10 | 0.16 | 2007–2017 | 5 | 24.7 | 728 | 9.2 | 391 |
| 0.06–0.28 | 5–5 | 19.6–30.8 | 715–734 | 8.8–9.6 | 320–479 |
| PAIV | Vol | Age | SDI | Propoak | SI | GDC | cvh | ||
|---|---|---|---|---|---|---|---|---|---|
| [N = 785] | [m³ ha−1 year−1] | [m³ ha−1] | [years] | [n ha−1] | [%] | [m] | [./.] | [./.] | |
| mono- specific [n = 390] | mean | 9.15 | 278.37 | 96 | 200 | 99 | 24.46 | 0.02 | 0.13 |
| min | 2.92 | 42.03 | 17 | 75 | 90 | 16.91 | −0.20 | 0.00 | |
| max | 20.91 | 729.62 | 229 | 399 | 100 | 32.17 | 0.19 | 0.44 | |
| sd | 3.43 | 124.60 | 45 | 63 | 2 | 3.74 | 0.06 | 0.08 | |
| mixed [n = 395] | mean | 10.94 | 428.02 | 117 | 266 | 63 | 26.86 | −0.03 | 0.24 |
| min | 2.90 | 73.66 | 27 | 102 | 2 | 15.63 | −0.53 | 0.03 | |
| max | 26.90 | 1139.87 | 360 | 549 | 90 | 34.53 | 0.20 | 0.58 | |
| sd | 3.32 | 175.79 | 51 | 94 | 23 | 3.19 | 0.10 | 0.10 |
| iv/cpa | Age | rel_d | cpa | ||
|---|---|---|---|---|---|
| [N = 67.479] | [dm³ m−2 year−1] | [years] | [./.] | [m²] | |
| mono- specific [n = 40.827] | mean | 0.92 | 79 | 0.62 | 17.21 |
| min | 0.00 | 22 | 0.15 | 1.91 | |
| max | 4.52 | 234 | 1.00 | 232.97 | |
| sd | 0.52 | 38 | 0.16 | 17.04 | |
| mixed [n = 26.652] | mean | 0.95 | 106 | 0.62 | 31.46 |
| min | 0.00 | 28 | 0.09 | 2.01 | |
| max | 5.70 | 371 | 1.00 | 252.07 | |
| sd | 0.45 | 48 | 0.16 | 26.94 | |
| Parameters | Variables | est | se | p-Value | sig. | Random Effects | sd |
|---|---|---|---|---|---|---|---|
| a0 | 2.244 | 0.029 | <0.001 | *** | bi | 0.14 | |
| a1 | ln (V) | 0.382 | 0.031 | <0.001 | *** | bij | 0.00 |
| a2 | ln (SDI) | 0.285 | 0.040 | <0.001 | *** | εijt | 0.26 |
| a3 | Age | −0.004 | 0.000 | <0.001 | *** | ||
| a4 | MT [mono] | −0.073 | 0.033 | 0.025 | * | n obs | 728 |
| a5 | Year | 0.003 | 0.000 | <0.001 | *** | pseudo-R² (m/c) | 0.62/0.71 |
| a6 | MT [mono] * Year | 0.000 | 0.001 | 0.933 | n.s. | RMSE | 0.25 |
| Parameters | Variables | est | se | p-Value | sig. | Random Effects | sd |
|---|---|---|---|---|---|---|---|
| a0 | 2.175 | 0.094 | <0.001 | *** | bj | 0.14 | |
| a1 | ln (V) | 0.108 | 0.036 | 0.003 | ** | bjt | 0.22 |
| a2 | ln (SI) | 1.047 | 0.114 | <0.001 | *** | εijt | 0.12 |
| a3 | Age | −0.000 | 0.000 | 0.250 | ns | ||
| a4 | ln (SDI) | 0.355 | 0.051 | <0.001 | *** | n obs | 785 |
| a5 | MT [mono] | −0.027 | 0.023 | 0.258 | ns | pseudo-R² (m/c) | 0.42/0.92 |
| a6 | lay [2nd] | 0.059 | 0.018 | 0.001 | *** | RMSE | 0.07 |
| a7 | Age * ln (SDI) | 0.002 | 0.001 | <0.001 | *** | ||
| a8 | ln (SDI) * MT [mono] | 0.110 | 0.051 | 0.014 | * | ||
| a9 | ln (SDI) * lay [2nd] | −0.209 | 0.038 | <0.001 | *** |
| Parameters | Variables | est | se | p-Value | sig. | Random Effects | sd |
|---|---|---|---|---|---|---|---|
| a0 | −0.122 | 0.040 | 0.002 | ** | bj | 0.21 | |
| a1 | ln (Age) | −0.209 | 0.019 | <0.001 | *** | bjf | 0.29 |
| a2 | ln (SDI) | −0.234 | 0.025 | <0.001 | *** | bjft | 0.31 |
| a3 | rel_d | 2.636 | 0.037 | <0.001 | *** | εijft | 0.46 |
| a4 | MT [mono] | −0.061 | 0.011 | <0.001 | *** | ||
| a5 | cvh | 0.260 | 0.061 | <0.001 | *** | n obs | 67.479 |
| a6 | ln (Age) * ln (SDI) | −0.782 | 0.027 | <0.001 | *** | pseudo-R² (m/c) | 0.38/0.70 |
| a7 | ln (Age) * rel_d | −1.493 | 0.041 | <0.001 | *** | RMSE | 0.42 |
| a8 | ln (Age) * MT [mono] | −0.298 | 0.024 | <0.001 | *** | ||
| a9 | ln (Age) * cvh | −0.231 | 0.093 | 0.013 | * | ||
| a10 | rel_d * MT [mono] | 0.669 | 0.048 | <0.001 | *** | ||
| a11 | rel_d * ln (SDI) | −0.126 | 0.058 | 0.030 | * | ||
| a12 | rel_d * cvh | 2.953 | 0.197 | <0.001 | *** | ||
| a13 | cvh * ln (SDI) | −0.341 | 0.109 | 0.002 | ** | ||
| a14 | MT [mono] * ln (SDI) | 0.136 | 0.027 | <0.001 | *** | ||
| a15 | MT [mono] * cvh | 0.273 | 0.085 | 0.001 | ** |
| Parameters | Variables | est | se | p-Value | sig. | Random Effects | sd |
|---|---|---|---|---|---|---|---|
| a0 | 0.021 | 0.007 | 0.005 | ** | bi | 0.01 | |
| a1 | Age | −0.003 | 0.000 | <0.001 | *** | bij | 0.04 |
| a2 | MT [mixed] | −0.060 | 0.008 | <0.001 | *** | εijk | 0.06 |
| a3 | ln (SDI) | 0.037 | 0.015 | 0.012 | * | ||
| a4 | Age * MT [mixed] | −0.001 | 0.000 | <0.001 | *** | n obs | 740 |
| a5 | Age * ln (SDI) | −0.001 | 0.000 | 0.093 | ns | pseudo-R² (m/c) | 0.45/0.60 |
| a6 | MT [mixed] * ln (SDI) | 0.051 | 0.019 | 0.007 | ** | RMSE | 0.06 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stimm, K.; Heym, M.; Nagel, R.-V.; Uhl, E.; Pretzsch, H. Long-Term Productivity of Monospecific and Mixed Oak (Quercus petraea [Matt.] Liebl. and Quercus robur L.) Stands in Germany: Growth Dynamics and the Effect of Stand Structure. Forests 2022, 13, 724. https://doi.org/10.3390/f13050724
Stimm K, Heym M, Nagel R-V, Uhl E, Pretzsch H. Long-Term Productivity of Monospecific and Mixed Oak (Quercus petraea [Matt.] Liebl. and Quercus robur L.) Stands in Germany: Growth Dynamics and the Effect of Stand Structure. Forests. 2022; 13(5):724. https://doi.org/10.3390/f13050724
Chicago/Turabian StyleStimm, Kilian, Michael Heym, Ralf-Volker Nagel, Enno Uhl, and Hans Pretzsch. 2022. "Long-Term Productivity of Monospecific and Mixed Oak (Quercus petraea [Matt.] Liebl. and Quercus robur L.) Stands in Germany: Growth Dynamics and the Effect of Stand Structure" Forests 13, no. 5: 724. https://doi.org/10.3390/f13050724
APA StyleStimm, K., Heym, M., Nagel, R.-V., Uhl, E., & Pretzsch, H. (2022). Long-Term Productivity of Monospecific and Mixed Oak (Quercus petraea [Matt.] Liebl. and Quercus robur L.) Stands in Germany: Growth Dynamics and the Effect of Stand Structure. Forests, 13(5), 724. https://doi.org/10.3390/f13050724






