Mechanical Behavior of GFRP Dowel Connections to Cross Laminated Timber-CLT Panels
Abstract
:1. Introduction
2. Materials and Methods
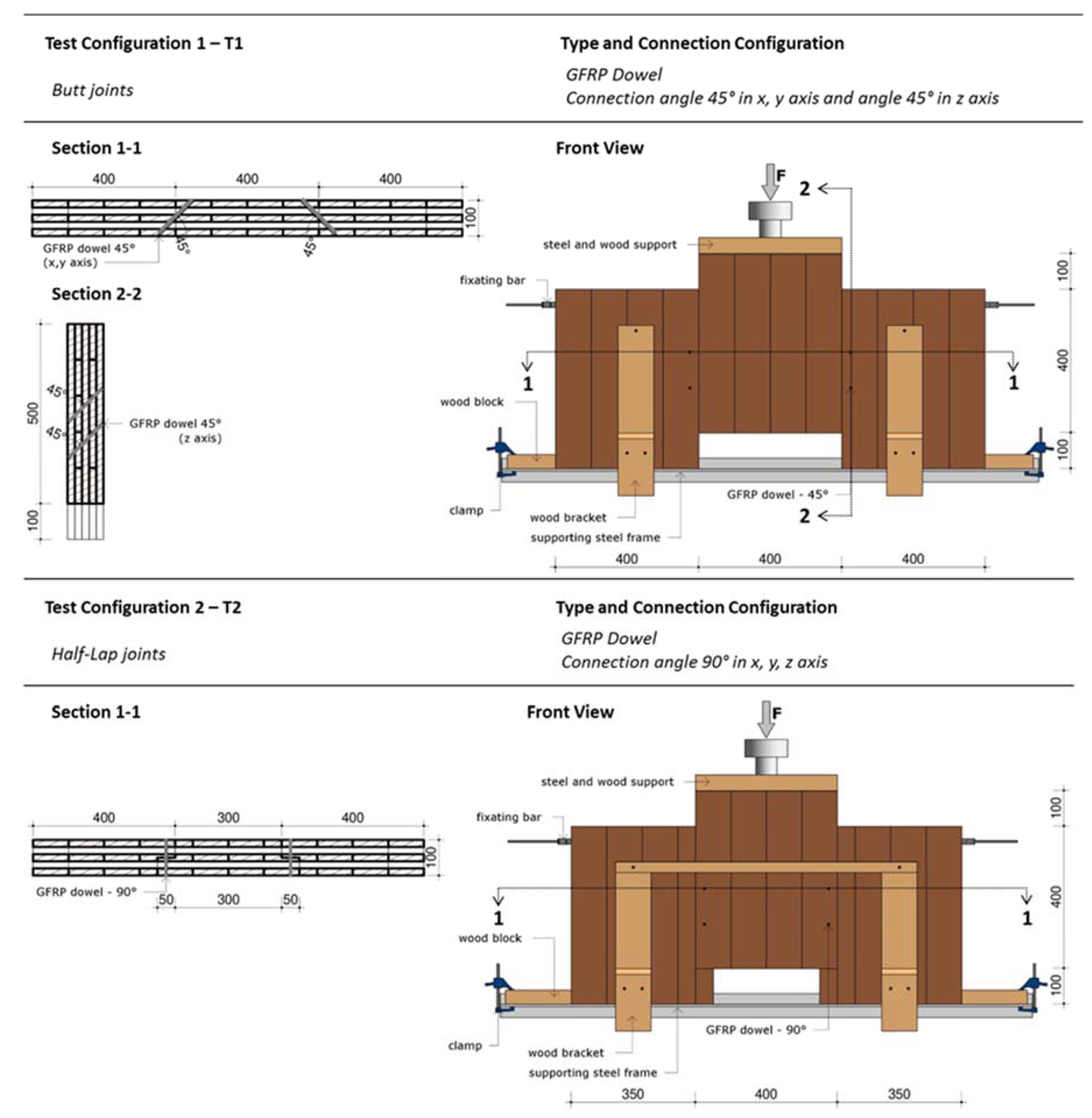
2.1. Specimen Description
2.1.1. NDT Ultrasonic Test
2.1.2. Manufacture of CLT Panels and GFRP Dowels
2.2. Testing
3. Results
3.1. Butt-End Connections Results
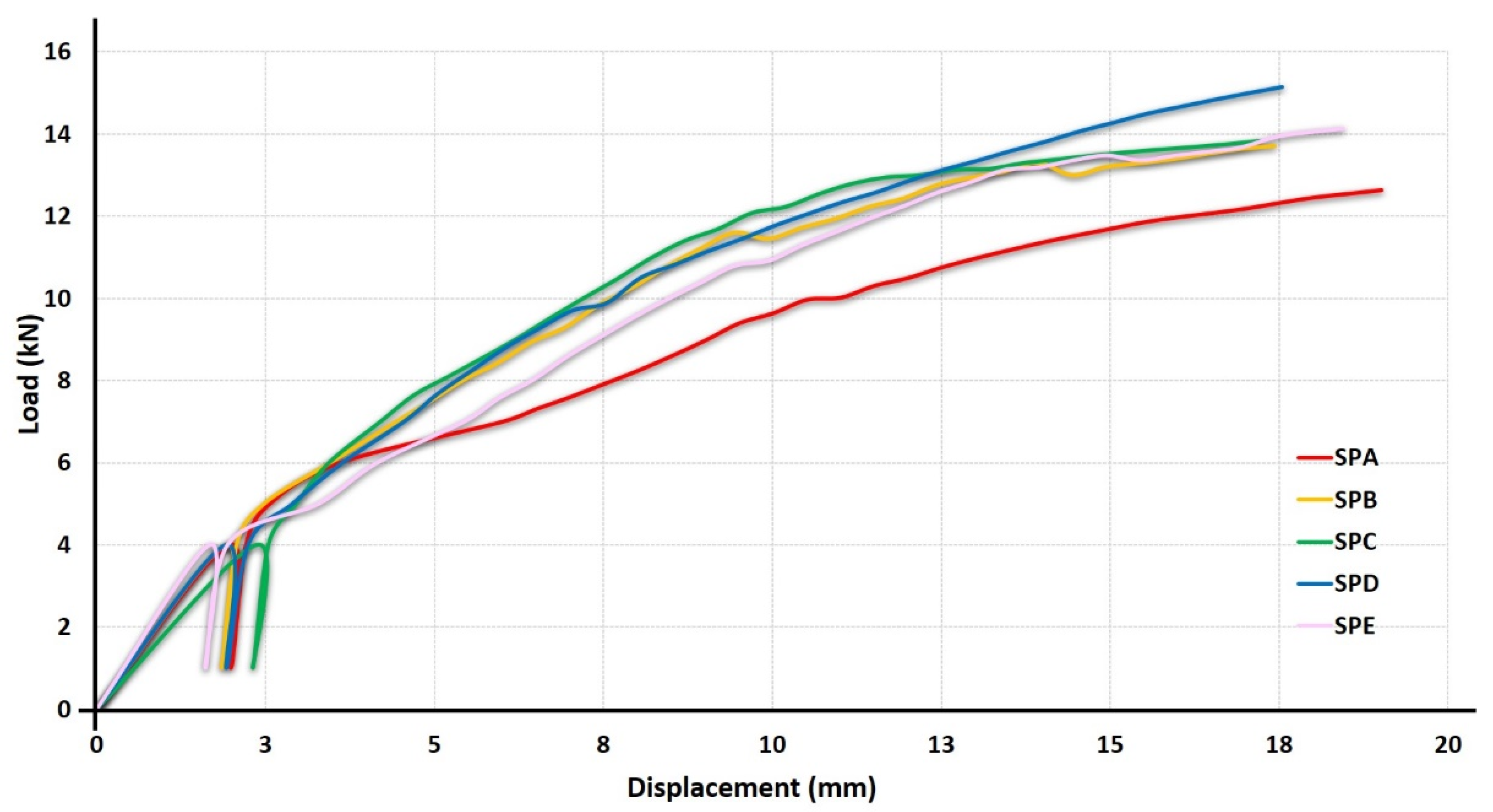
3.2. Half-Lap Connections Results
4. Discussion
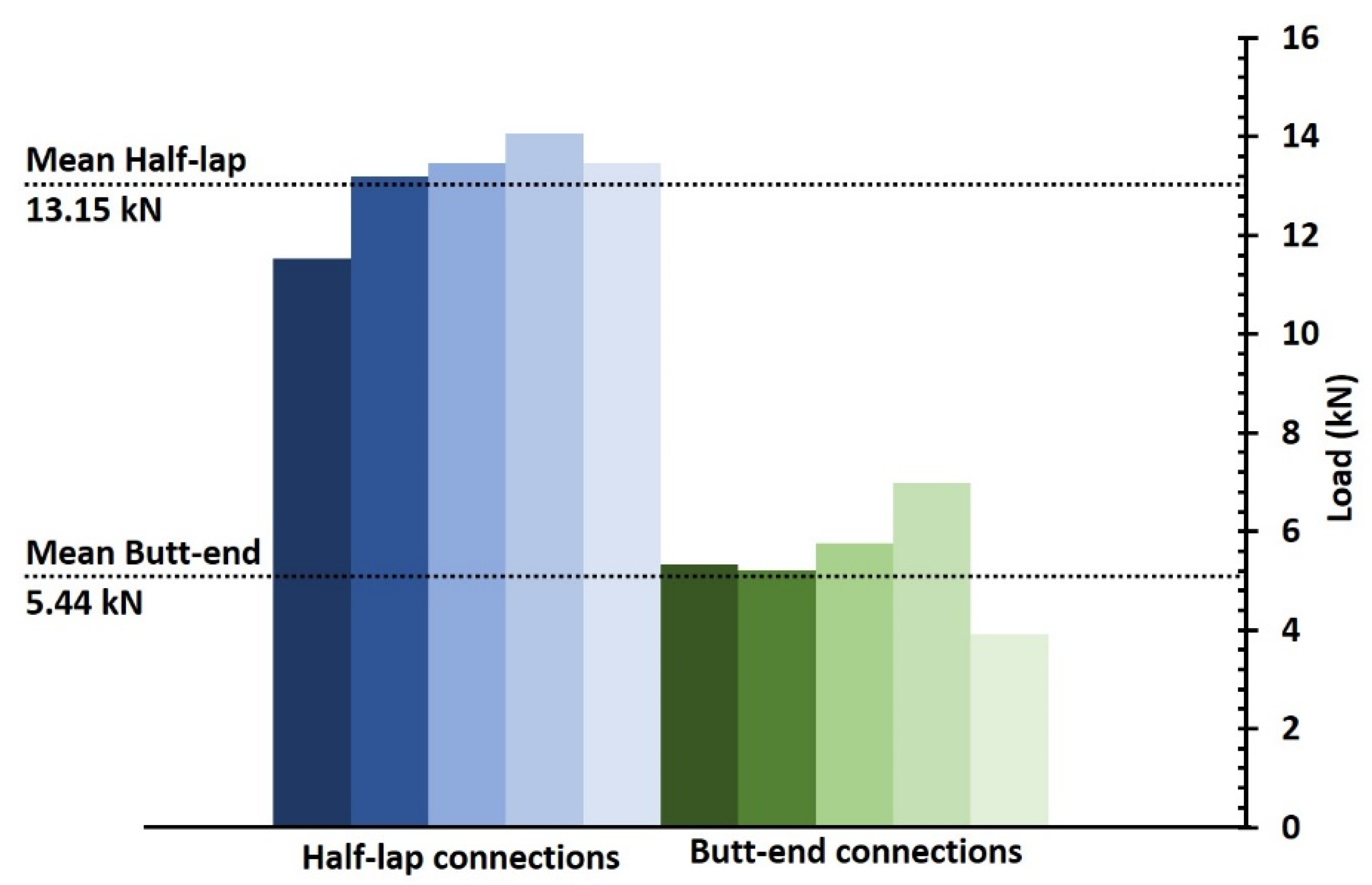
4.1. Comparison between Butt-End Connections and Half-Lap Connections
4.2. Comparison between Experimental Results and Literature Review
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cherry, R.; Manalo, A.; Karunasena, W.; Stringer, G. Out-of-grade sawn pine: A state-of-the-art review on challenges and new opportunities in cross laminated timber (CT). Constr. Build. Mater. 2019, 211, 858–868. [Google Scholar] [CrossRef]
- Sanborn, K.; Gentry, T.; Koch, Z.; Valkenburg, A.; Conley, C.; Stewart, L. Ballistic performance of Cross-laminated Timber (CLT). Int. J. Impact Eng. 2019, 128, 11–23. [Google Scholar] [CrossRef]
- Hossain, A.; Danzig, I.; Tannert, T. Cross-Laminated Timber Shear Connections with Double-Angled Self-Tapping Screw Assemblies. J. Struct. Eng. 2016, 142, 04016099. [Google Scholar] [CrossRef]
- Popovski, M.; Schneider, J.; Schweinsteiger, M. Lateral load resistance of cross-laminated wood panels. In Proceedings of the 11th World Conference on Timber Engineering 2010, WCTE 2010, Trentino, Italy, 20–24 June 2010; Volume 4, pp. 3394–3403. [Google Scholar]
- Fragiacomo, M.; Dujic, B.; Sustersic, I. Elastic and ductile design of multi-storey crosslam massive wooden buildings under seismic actions. Eng. Stuct. 2011, 33, 3043–3053. [Google Scholar] [CrossRef]
- Fragiacomo, M.; Dujic, B.; Sustersic, I. Strength and deformation characteristics of typical X-Lam connections. In Proceedings of the World Conference on Timber Engineering, Auckland, New Zealand, 15–19 July 2012; p. 10. [Google Scholar]
- Branco, J.M.; Kekeliak, M.; Lourenco, P. In-plane stiffness of timber floors strengthened with CLT. Eur. J. Wood Wood Prod. 2015, 73, 313–323. [Google Scholar] [CrossRef] [Green Version]
- Aloisio, A.; Pasca, D.; Tomasi, R.; Fragiacomo, M. Dynamic identification and model updating of an eight-storey CLT building. Eng. Struct. 2020, 213, 110593. [Google Scholar] [CrossRef]
- Jockwer, R.; Wiehle, P.; Palma, P.; Klippel, M.; Wapp, A.; Frangi, A.; Hebel, D. Structural behaviour and design of timber connections with dowels and slotted-in plates made of bamboo composite. In Proceedings of the WCTE 2018—World Conference on Timber Engineering, Seoul, Korea, 20–23 August 2018. [Google Scholar]
- Drake, R. The Advancement of Structural Connection Techniques for Timber Buildings. Ph.D. Thesis, University of Bath, Bath, UK, 2003. [Google Scholar]
- Thomson, A.; Harris, R.; Walker, P.; Ansell, M. Development of non-metallic timber connections for contemporary applications. In Proceedings of the 11th World Conference on Timber Engineering 2010, WCTE 2010, Trentino, Italy, 20–24 June 2010; Volume 4, pp. 2995–3002. [Google Scholar]
- Thomson, A.; Harris, R.; Ansell, M.; Walker, P. Experimental performance of non-metallic mechanically fastened timber connections. Struct. Eng. 2010, 88, 25–32. [Google Scholar]
- 1995-1-1 EN; Eurocode 5: Design of Timber Structures—Part 1-1: General–Common Rules and Rules for Buildings; European Committee for Standardization: Brussels, Belgium, 2004.
- ASTM D4475-2; Standard Test Method for Apparent Horizontal Shear Strength of Pultruded Reinforced Plastic Rods By the Short-Beam Method. ASTM International: West Conshohocken, PA, USA, 2008.
- Brandon, D.; Ansell, M.; Bregulla, J.; Harris, R.; Walker, P. Behaviour of non-metallic shear connections in fire. In Proceedings of the WCTE 2014—World Conference on Timber Engineering, Proceedings, Quebec City, QC, Canada, 10–14 August 2014. [Google Scholar]
- Brandon, D.; Ansell, M.P.; Harris, R.; Walker, P.; Bregulla, J. Modelling of Non-metallic Timber Connections at Elevated Temperatures. In Materials and Joints in Timber Structures: Recent Developments of Technology; Springer: Dordrecht, the Netherlands, 2014; Volume 9, pp. 231–241. [Google Scholar]
- Brandon, D.; Maluk, C.; Ansell, M.P.; Harris, R.; Walker, P.; Bisby, L.; Bregulla, J. Fire performance of metal-free timber connections. Proc. Inst. Civ. Eng. Constr. Mater. 2015, 168, 173–186. [Google Scholar] [CrossRef] [Green Version]
- Palma, P.; Kobel, P.; Minor, A.; Frangi, A. Dowelled timber connections with internal members of densified veneer wood and fibre-reinforced polymer dowels. In Proceedings of the WCTE 2016—World Conference on Timber Engineering, Vienna, Austria, 22–25 August 2016. [Google Scholar]
- NBR 7190; Projeto de Estruturas de Madeira; Associação Brasileira de Normas Técnicas–ABNT: Rio de Janeiro, Brazilia, 1997; p. 107.
- ANSI/APA PRG 320; Standard for Performance-Rated Cross-Laminated Timber. APA–The Engineered Wood Association: Tacoma, WA, US, 2018.
- EN 26891; Timber Structures—Joints Made With Mechanical Fasteners—General Principles for the Determination of Strength and Deformation Characteristics; European Committee for Standardization: Brussels, Belgium, 2004.
- Piggott, M. Load Bearing Fibre Composites, 2nd ed.; Kluwer Academic Publishers: Torronto, ON, Canada, 2002. [Google Scholar]






| Configuration | Specimen | Grouping of Panels | MOEd [10⁻⁶ MPa] | |
|---|---|---|---|---|
| Mean | COV (%) | |||
| T1—Half-lap | SPA | Panel 1 + Panel 5 + Panel 3 | 5584 | 9 |
| SPB | Panel 4 + Panel 2 + Panel 6 | 5452 | 11 | |
| SPC | Panel 7 + Panel 14 + Panel 9 | 5402 | 17 | |
| SPD | Panel 8 + Panel 10 + Panel 12 | 5340 | 11 | |
| SPE | Panel 11 + Panel 13 + Panel 15 | 5316 | 12 | |
| Twin half-lap | Panel 16 + Panel 20 + Panel 18 | 5434 | 12 | |
| T2—Butt-end | SP1 | Panel 17 + Panel 19 + Panel 21 | 5321 | 2 |
| SP2 | Panel 22 + Panel 23 + Panel 24 | 5422 | 13 | |
| SP3 | Panel 27 + Panel 29 + Panel 30 | 5310 | 9 | |
| SP4 | Panel 25 + Panel 26 + Panel 28 | 5321 | 2 | |
| SP5 | Panel 31 + Panel 34 + Panel 35 | 5416 | 2 | |
| Twin butt-end | Panel 32 + Panel 33 + Panel 36 | 5403 | 11 | |
| Specimen | Fest (kN) | Fmax (kN) | Dmax (mm) | kser (kN/mm) | |
|---|---|---|---|---|---|
| EN 26891 (1991) | Regression | ||||
| SP1 | 5 | 5.33 | 11.54 | 1.97 | 2.66 |
| SP2 | 5.22 | 14.71 | 3.87 | 5.51 | |
| SP3 | 5.77 | 14.41 | 3.00 | 4.32 | |
| SP4 | 6.97 | 14.75 | 2.73 | 3.63 | |
| SP5 | 3.92 | 11.91 | 3.20 | 4.44 | |
| Mean | 5.44 | 13.46 | 2.95 | 4.11 | |
| COV (%) | 20.17 | 11.87 | 23.50 | 25.64 | |
| Specimen | Fest (kN) | Fmax (kN) | Dmax (mm) | kser (kN/mm) | |
|---|---|---|---|---|---|
| EN 26891 (1991) | Regression | ||||
| SPA | 10 | 11.54 | 14.51 | 1.51 | 2.03 |
| SPB | 13.20 | 14.94 | 1.56 | 2.08 | |
| SPC | 13.47 | 14.70 | 1.27 | 1.68 | |
| SPD | 14.07 | 14.55 | 1.56 | 2.11 | |
| SPE | 13.47 | 14.95 | 1.79 | 2.38 | |
| Mean | 13.15 | 14.73 | 1.54 | 2.06 | |
| COV (%) | 7.26 | 1.42 | 12.05 | 12.17 | |
| Properties | Gavric et al. [6] | Branco et al. [7] | Experimental GFRP | ||
|---|---|---|---|---|---|
| Half-Lap | Nail 90° | Nail 45° | Half-Lap | Butt-End | |
| Fmax (kN) | 5.25 | 3.51 | 2.48 | 13.15 | 5.44 |
| Dmax (mm) | 15.98 | 14.03 | 13.90 | 14.73 | 13.46 |
| kser (kN/mm) | 1.24 | 1.20 | 1.37 | 1.54 | 2.95 |
| Connections | 4 screws | 2 nails | 4 GFRP dowels | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
de Almeida, A.C.; de Melo Moura, J.D. Mechanical Behavior of GFRP Dowel Connections to Cross Laminated Timber-CLT Panels. Forests 2022, 13, 320. https://doi.org/10.3390/f13020320
de Almeida AC, de Melo Moura JD. Mechanical Behavior of GFRP Dowel Connections to Cross Laminated Timber-CLT Panels. Forests. 2022; 13(2):320. https://doi.org/10.3390/f13020320
Chicago/Turabian Stylede Almeida, Amanda Ceinoti, and Jorge Daniel de Melo Moura. 2022. "Mechanical Behavior of GFRP Dowel Connections to Cross Laminated Timber-CLT Panels" Forests 13, no. 2: 320. https://doi.org/10.3390/f13020320
APA Stylede Almeida, A. C., & de Melo Moura, J. D. (2022). Mechanical Behavior of GFRP Dowel Connections to Cross Laminated Timber-CLT Panels. Forests, 13(2), 320. https://doi.org/10.3390/f13020320






