Form and Volume of the Stem of Tectona grandis L.f. in the Central-WESTERN Region of Brazil
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Area and Data
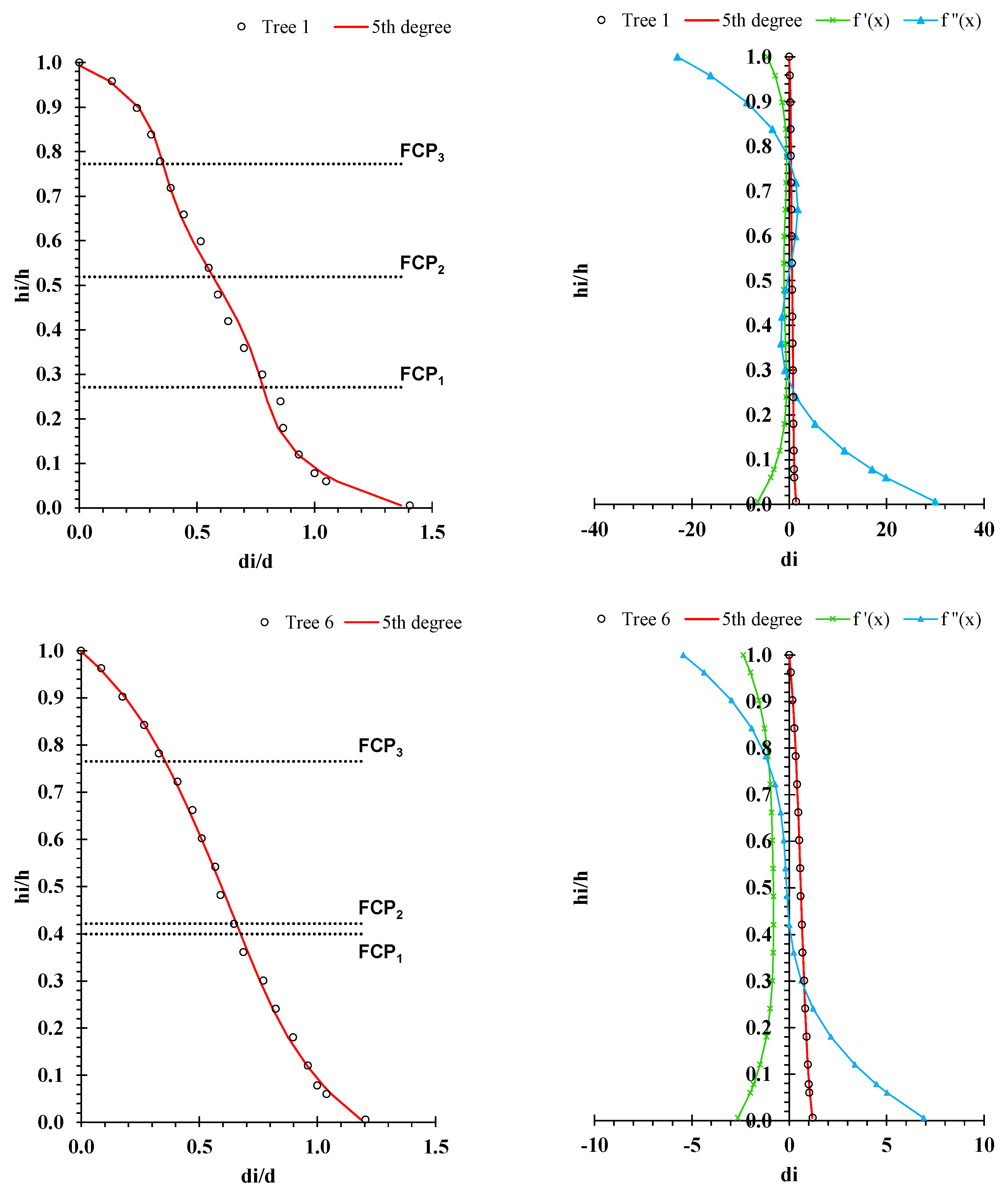
2.2. Study of the form Change Points of the Stems
2.3. Models Evaluated
2.4. Date Stratification and Change in the Model Variables
2.5. Statistical Evaluation of the Models
2.6. Volume
3. Results and Discussion
3.1. Descriptive Study of the Stem Forms
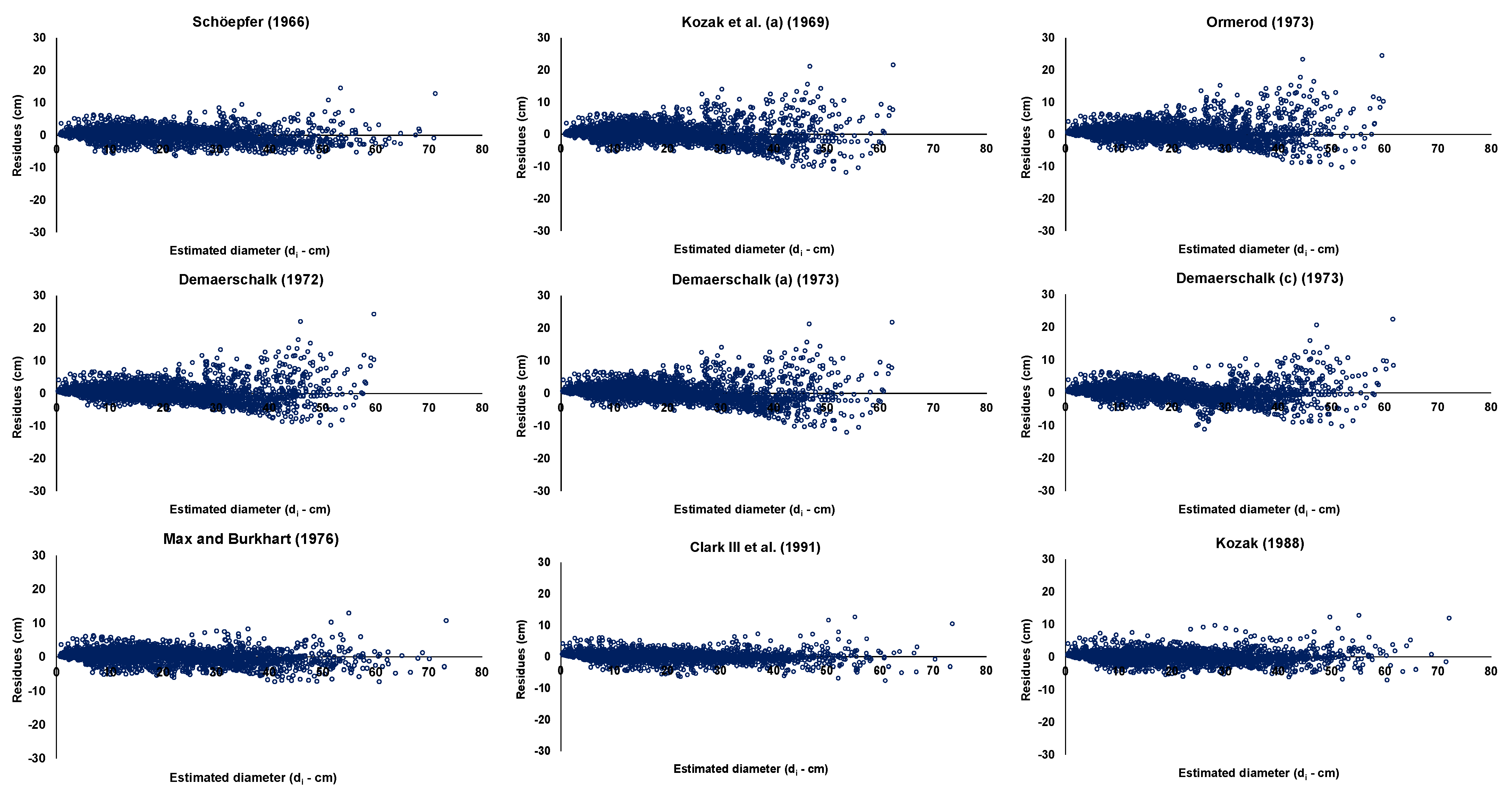
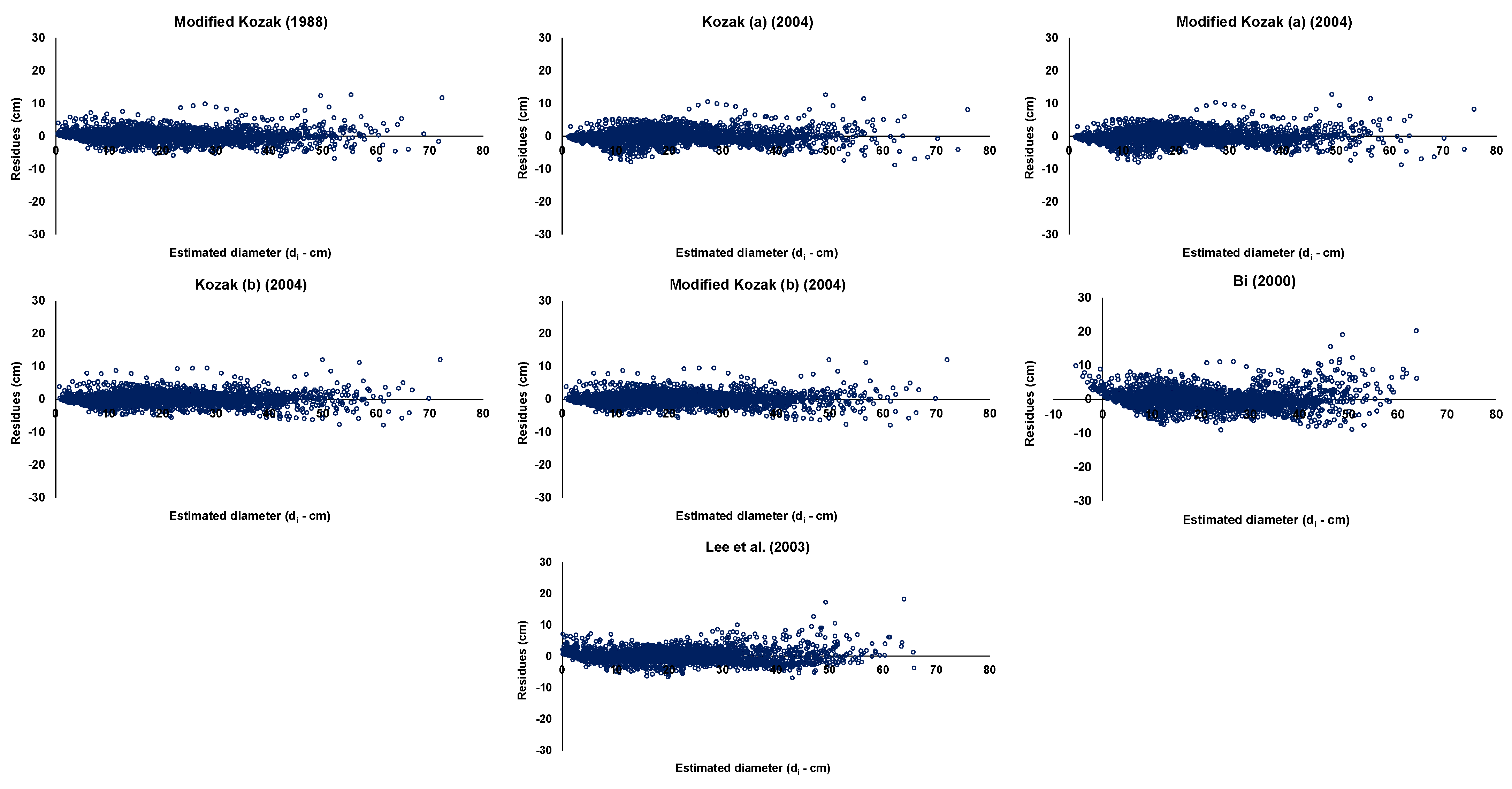
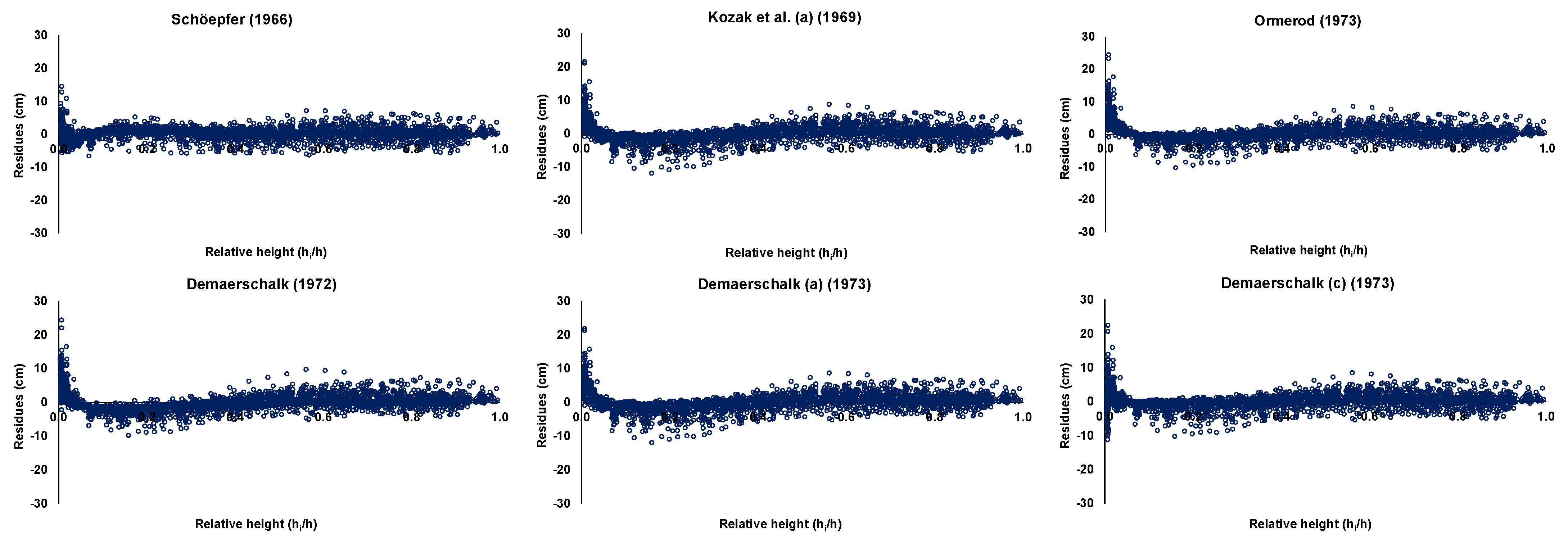
3.2. Adjustment of the Taper Models without Data Stratification
3.3. Stratification as a Variability Reducer in Models Adjustment
3.4. Proposed Modifications to the Clark III et al. Model (1991)
3.5. Volume
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- APGIV. An update of the Angiosperm Phylogeny Group classification for the orders and families of flowering plants: APG IV. Bot. J. Linn. Soc. 2016, 181, 1–20. [Google Scholar] [CrossRef]
- Moya, R.; Bond, B.; Quesada, H. A review of heartwood properties of Tectona grandis trees from fast-growth plantations. Wood Sci. Technol. 2014, 48, 411–433. [Google Scholar] [CrossRef]
- International Union of Forest Research Organizations (IUFRO). The Global Teak Study. Analysis. In Evaluation and Future Potential of Teak Resources; IUFRO: Vienna, Austria, 2017; 108p. [Google Scholar]
- Adu-Bredu, S.; Ofori, D.A.; Ræbild, A.; Hansen, J.K.; Koffi, A.; Vigneron, P.; Kjær, E.D. Trait variations in 28-year-old teak (Tectona grandis) provenance field trials in Ghana, West Africa. South. For. A J. For. Sci. 2018, 81, 57–68. [Google Scholar] [CrossRef]
- Cañadas-L, A.; Andrade-Candell, J.; Domínguez-A, J.M.; Molina-H, C.; Schnabel-D, O.; Vargas-Hernández, J.J.; Wehenkel, C. Growth and Yield Models for Teak Planted as Living Fences in Coastal Ecuador. Forests 2018, 9, 55. [Google Scholar] [CrossRef]
- Dantani, A.; Shamaki, S.B.; Gupa, M.A.; Sa’idu, M.; Mukhtar, R.B.; Umar, M.; Abubakar, B.; Abubakar, A. Allometric Models for Estimating Site Index of Teak (Tectona grandis Linn F.) in Kanya Forest Plantation, Kebbi State, Nigeria. Asian J. Environ. Ecol. 2019, 10, 1–7. [Google Scholar] [CrossRef]
- Fernández-Sólis, D.; Berrocal, A.; Moya, R. Heartwood formation and prediction of heartwood parameters in Tectona grandis L.f. trees growing in forest plantations in Costa Rica. Bois For. Des Trop. 2018, 335, 25–37. [Google Scholar] [CrossRef]
- Medeiros, R.A.; De Paiva, H.N.; D’Ávila, F.S.; Leite, H.G. Growth and yield of teak stands at different spacing. Pesq. Agropec. Bras. 2018, 53, 1109–1118. [Google Scholar] [CrossRef]
- Moya, R.; Gaitán-Álvarez, J.; Ortiz-Malavassi, E.; Berrocal, A.; Fernández, D. Equations for predicting heartwood merchantable volume and tradable sawlog in Tectona grandis. J. Trop. For. Sci. 2020, 32, 379–390. [Google Scholar] [CrossRef]
- Seppänen, P.; Mäkinen, A. Comprehensive yield model for plantation teak in Panama. Silva Fenn. 2020, 54, 1–25. [Google Scholar] [CrossRef]
- Tewari, V.P.; Bilas Singh, B. Total Wood Volume Equations for Tectona grandis Linn F. Stands in Gujarat, India. J. Environ. Sci. 2020, 34, 313–320. [Google Scholar] [CrossRef]
- Torres, D.A.; del Valle, J.I.; Restrepo, G. Teak growth, yield- and thinnings’ simulation in volume and biomass in Colombia. Ann. For. Res. 2020, 63, 53–70. [Google Scholar] [CrossRef]
- Vaides-López, E.; Alvarado-Hernández, A.; Moya-Fernández, R. Site characteristics that determine the growth and productivity of teak (Tectona grandis L.f.) of young plantations in Guatemala. Agron. Costarric. 2019, 43, 135–148. [Google Scholar]
- Yang, B.; Jia, H.; Zhao, Z.; Pang, S.; Cai, D. Horizontal and vertical distributions of heartwood for teak plantation. Forests 2020, 11, 225. [Google Scholar] [CrossRef]
- Indústria Brasileira de Árvores (IBA). Relatório IBÁ; IBÁ: Brasília, DF, Brazil, 2019; 80p. [Google Scholar]
- MacFarlane, D.W.; Weiskittel, A.R. A new method for capturing stem taper variation for trees of diverse morphological types. Can. J. For. Res. 2016, 46, 804–815. [Google Scholar] [CrossRef]
- Fonweban, J.; Gardiner, B.; Mac Donald, E.; Auty, D. Taper functions for Scots pine (Pinus sylvestris L.) and Sitka spruce (Picea sitchensis (Bong.) Carr.) in Northern Britain. Forestry 2011, 84, 49–60. [Google Scholar] [CrossRef]
- Choochuen, T.; Suksavate, W.; Meunpong, P. Development of a taper equation for teak (Tectona grandis L.f.) growing in Western Thailand. Environ. Nat. Resour. J. 2021, 19, 176–185. [Google Scholar] [CrossRef]
- Favalessa, C.M.C.; Ubialli, J.A.; Caldeira, S.F.; Drescher, R. Funções de afilamento não segmentadas e segmentadas para Tectona grandis na região centro-sul matogrossense. Pesq. Flor. Bras. 2012, 32, 373–387. [Google Scholar] [CrossRef]
- Koirala, A.; Montes, C.R.; Bullock, B.P.; Wagle, B.H. Developing taper equations for planted teak (Tectona grandis L.f.) trees of central lowland Nepal. Trees For. People 2021, 5, 100103. [Google Scholar] [CrossRef]
- Lanssanova, R.; Machado, S.; Orso, G.; Pelissari, A.; Filho, A.F.; Silva, F. Calibration of a mixed-effect stem taper model for Tectona grandis. J. Trop. For. Sci. 2020, 32, 341–348. [Google Scholar] [CrossRef]
- Pelissari, A.L.; Roveda, M.; Caldeira, S.F.; Sanquetta, C.R.; Corte, A.P.D.; Rodrigues, C.K. Geostatistical modeling of timber volume spatial variability for Tectona grandis L.F. precision forestry. Cerne 2017, 23, 115–122. [Google Scholar] [CrossRef]
- Júnior, I.D.S.T.; de Souza, J.R.M.; Lopes, L.S.D.S.; Fardin, L.P.; Casas, G.G.; Neto, R.R.D.O.; Leite, R.V.; Leite, H.G. Machine learning and regression models to predict multiple tree stem volumes for teak. South For. 2021, 83, 294–302. [Google Scholar] [CrossRef]
- Wijenayake, P.R.; Hiroshima, T.; Yamamoto, H. Comparative Study on Commercial Log Production Managed under Different Conditions: Evaluating Plantation Grown Teak of Sri Lanka. J. For. Plan. 2019, 25, 27–37. [Google Scholar] [CrossRef]
- Kozak, A.; Munro, D.D.; Smith, J.H.G. Taper functions and their application in forest inventory. For. Chron. 1969, 45, 278–283. [Google Scholar] [CrossRef]
- Scolforo, H.F.; McTague, J.P.; Raimundo, M.R.; Weiskittel, A.; Carrero, O.; Scolforo, J.R.S. Comparison of taper functions applied to eucalypts of varying genetics in Brazil: Application and evaluation of the penalized mixed spline approach. Can. J. For. Res. 2018, 48, 568–580. [Google Scholar] [CrossRef]
- Muhairwe, C.K. Examination and Modeling of Tree Form and Taper Over Time or Interior Lodgepole Pine. Ph.D. Thesis, University of British Columbia, Vancouver, BC, Canada, 1993. [Google Scholar]
- Max, T.A.; Burkhart, H.E. Segmented polynomial regression applied to taper equations. For. Sci. 1976, 22, 283–289. [Google Scholar]
- Parresol, B.R.; Hotvedt, J.E.; Cao, Q.V. A volume and taper prediction system for bald cypress. Can. J. For. Res. 1987, 17, 250–259. [Google Scholar] [CrossRef]
- Clark, A., III; Souter, R.A.; Schlaegel, B.E. Stem Profile Equations for Southern Tree Species; USDA Forest Service: Atlanta, GA, USA, 1991; Volume 282, 113p. [Google Scholar]
- Kozak, A. My last words on taper equations. For. Chron. 2004, 80, 507–515. [Google Scholar] [CrossRef]
- Bi, H. Trigonometric variable-form taper equations for Australian Eucalyptus. For. Sci. 2000, 46, 397–409. [Google Scholar]
- Bernardi, L.K.; Thiersch, M.F.B.M.; Arteaga, A.J.M.; Almeida, A.A.A.; Pádua, F.A.; Thiersch, C.R. Diferentes modelos para o afilamento do tronco de Eucalyptus sp. para o cenário florestal brasileiro. Ciênc. Florest. 2021, 31, 1364–1382. [Google Scholar] [CrossRef]
- McTague, J.P.; Weiskittel, A.R. Evolution, history, and use of stem taper equations: A review of their development, application, and implementation. Can. J. For. Res. 2021, 51, 210–235. [Google Scholar] [CrossRef]
- Alvares, C.A.; Stape, J.L.; Sentelhas, P.C.; Moraes, G.J.L.; Sparovek, G. Köppen’s climate classification map for Brazil. Meteorol. Z. 2013, 22, 711–728. [Google Scholar] [CrossRef]
- Kozak, A. A variable exponent taper equation. Can. J. For. Res. 1988, 18, 1363–1368. [Google Scholar] [CrossRef]
- Assmann, E. The Principles of Forest Yield Study; Pergamon Press: New York, NY, USA, 1970; 506p. [Google Scholar]
- Péllico Netto, S.; Brena, D. Inventário Florestal; Universidade Federal do Paraná: Curitiba, Brazil, 1997; 316p. [Google Scholar]
- Prodan, M.; Peters, R.; Cox, F. Mensura Florestal; IICA: San José, Costa Rica, 1997; 586p. [Google Scholar]
- Schöepfer, W. Automatisierung Des Massem, Sorten Und Wertberechnung Stenender Waldbestande Schriftenreihe Bad; Wurtt-Forstl: Koblenz, Germany, 1966. [Google Scholar]
- Wolfram Alpha LLC. Wolfram Alpha. Available online: http://www.wolframalpha.com/input/?i=2%2B2 (accessed on 5 September 2022).
- Demaerschalk, J.P. Converting volume equations to compatible taper equations. For. Sci. 1972, 18, 241–245. [Google Scholar] [CrossRef]
- Demaerschalk, J.P. Integrated systems for the estimation of tree taper and volume. Can. J. For. Res. 1973, 3, 90–94. [Google Scholar] [CrossRef]
- Ormerod, D.W. A simple bole model. For. Chron. 1973, 49, 136–138. [Google Scholar] [CrossRef]
- Cao, Q.V.; Burkhart, H.E.; Max, T.A. Evaluating of two methods for cubic-volume prediction of Loblolly Pine to any merchantable limit. For. Sci. 1980, 26, 71–80. [Google Scholar]
- Byrne, J.C.; Reed, D.D. Complex compatible taper and volume estimation systems for Red and Loblolly Pine. For. Sci. 1986, 32, 423–443. [Google Scholar]
- Lee, W.K.; Seo, J.H.; Son, Y.M.; Lee, K.H.; von Gadow, K. Modeling stem profiles for Pinus densiflora in Korea. For. Ecol. Manag. 2003, 172, 69–77. [Google Scholar] [CrossRef]
- Môra, R.; Silva, G.F.; Gonçalves, F.G.; Soares, C.P.B.; Chichorro, J.F.; Curto, R.A. Análise de diferentes formas de ajuste de funções de afilamento. Sci. For. 2014, 42, 237–249. [Google Scholar]
- Lanssanova, L.R.; Machado, S.D.A.; Garrett, A.T.D.A.; Bonete, I.P.; Pelissari, A.L.; Filho, A.F.; da Silva, F.A.; Ciarnoschi, L.D. Mixed-effect non-linear modelling for diameter estimation along the stem of Tectona grandis in mid-western Brazil. South. For. 2018, 81, 167–173. [Google Scholar] [CrossRef]
- Souza, C.A.M.; Finger, C.A.G.; Schneider, P.R.; Silva, G.F.; Thomas, C. Eficiência de um modelo de afilamento ajustado sem e com estratificação por classe de quociente de forma para formação dos sortimentos de Pinus taeda L. Ciênc. Florest. 2012, 22, 125–135. [Google Scholar] [CrossRef]
- Finger, C.A.G. Fundamentos Da Biometria Florestal; UFSM/CEPEF/FATEC: Santa Maria, Brazil, 1992; 269p. [Google Scholar]
- Jiang, L.; Brooks, J.R.; Wang, J. Compatible taper and volume equations for yellow-poplar in West Virginia. For. Ecol. Manag. 2005, 213, 399–409. [Google Scholar] [CrossRef]
- Newnham, R.M. A Variable-Form Taper Function; Forestry Canada Petawawa National Forestry Institute: Chalk River, ON, Canada, 1988; p. PI-X-83. [Google Scholar]
- SAS Statistical System, version 9.3; SAS Institute Inc.: Cary, NC, USA, 2016.
- Schneider, P.R.; Schneider, P.S.P.; Souza, C.A.M. Análise de Regressão Aplicada à Engenharia Florestal; FACOS-UFSM: Santa Maria, Brasil, 2009; 294p. [Google Scholar]
- Özçelik, R.; Brooks, J.R.; Jiang, L. Modeling stem profile of Lebanon cedar, Brutian pine and Cilicica fir in Southern Turkey using nonlinear mixed-effects models. Eur. J. For. Res. 2011, 130, 613–621. [Google Scholar] [CrossRef]
- Larson, P.R. Stem form development of forest trees. For. Sci. 1963, 9, a0001-42. [Google Scholar] [CrossRef]
- Smith, D.M.; Larson, B.C.; Kelty, M.J.; Ashton, P.K.S. The Pratice of Silviculture Applied Forest Ecology; John Wiley & Sons: New York, NY, USA, 1997; 537p. [Google Scholar]
- Siqueira, T.A.S. Serapilheira E a Regeneração Por Alto Fuste Após a Colheita de Povoamento de Teca. Master’s Thesis, (Mestrado em Ciências Ambientais e Florestais). Universidade Federal de Mato Grosso, Cuiabá, MT, Brasil, 2017. [Google Scholar]
- Gill, J.; King, G. What to Do When Your Hessian Is Not Invertible Alternatives to Model Respecification in Nonlinear Estimation. Sociol. Methods Res. 2004, 33, 54–87. [Google Scholar] [CrossRef]
- Müller, M.D.; Salles, T.T.; Paciullo, D.S.C.; Brighenti, A.M.; De Castro, C.R.T. Equações de altura, volume e afilamento para eucalipto e acácia estabelecidos em sistema silvipastoril. Floresta 2014, 44, 473–484. [Google Scholar] [CrossRef]
- Môra, R. Funções de Afilamento de Forma Variável E Modelagem de Efeitos Mistos Em Fustes de Pinus Taeda E Eucalyptus Saligna. Ph.D. Thesis, (Doutorado em Engenharia Florestal). Universidade Federal do Paraná, Curitiba, PR, Brasil, 2015. [Google Scholar]
- Demaerschalk, J.P.; Kozak, A. The whole-bole system: A conditioned dual-equation system for precise prediction of tree profiles. Can. J. For. Res. 1977, 7, 488–497. [Google Scholar] [CrossRef]
- Muhairwe, C.K. Taper equations for Eucalyptus pilularis and Eucalyptus grandis for the north coast in New South Wales, Australia. For. Ecol. Manag. 1999, 113, 251–269. [Google Scholar] [CrossRef]
- Newnham, R. Variable-form taper functions for four Alberta tree species. Can. J. For. Res. 1992, 22, 210–223. [Google Scholar] [CrossRef]
- Favalessa, C.M.C.; Ubialli, J.A.; Caldeira, S.F.; Drescher, R.; Acosta, F.C. Equações de sortimentos para Tectona grandis na região centro-sul de Mato Grosso. Pesq. Flor. Bras. 2012, 32, 389–399. [Google Scholar] [CrossRef]
- Fischer, F. Eficiência Dos Modelos Polinomiais E Das Razões de Volume NA Estimativa Volumétrica Dos Sortimentos E Do Perfil Do Fuste de Pinus Taeda. Master’s Thesis, (Mestrado em Engenharia Florestal). Universidade Federal de Lavras, Lavras, MG, Brasil, 1997. [Google Scholar]
- Assis, A.L.; Soares, J.R.S.; Mello, J.M.; Weimar, F.A.; Donizete, A.O. Comparação de modelos polinomiais segmentados e não segmentados na estimativa de diâmetros e volumes ao longo do fuste. Cerne 2001, 7, 20–42. [Google Scholar]
- Müller, I. Forma de Tronco E Sortimentos de Madeira Para Eucalyptus Grandis Hill Ex Maiden, Manejado Em Alto Fuste, NA Região Sudeste Do Estado Do Rio Grande Do Sul. Ph.D. Thesis, (Doutorado em Engenharia Florestal). Universidade Federal do Paraná, Curitiba, PR, Brasil, 2004. [Google Scholar]
- Friedl, R.A. Dinâmica E Prognose NA Forma Dos Fustes Em Povoamentos Plantados de Araucaria Angustifolia (Bert.) O. Ktze. Master’s Thesis, (Mestrado em Engenharia Florestal). Universidade Federal do Paraná, Curitiba, PR, Brasil, 1989. [Google Scholar]
- Figueiredo Filho, A.; Retslaff, F.A.S.; Kohler, S.V.; Becker, M.; Brandes, D. Efeito da Idade no Afilamento e Sortimento em Povoamentos de Araucaria angustifólia. Flor. Amb. 2015, 22, 50–59. [Google Scholar] [CrossRef]
- Kohler, S.V.; Kohler, H.S.; Figueiredo Filho, A.; Arce, J.E.; Machado, S.A. Evolução do sortimento em povoamentos de Pinus Taeda nos estados do Paraná e Santa Catarina. Floresta 2015, 45, 545–554. [Google Scholar] [CrossRef]
- Finger, C.A.G.; Zanon, M.L.B.; Schneider, P.R.; Klein, J.E.M.; Coelho, M.C.B. Funções de forma para Eucalyptus dunnii Maiden implantados na depressão central e encosta do sudeste do Rio Grande do Sul. Ciênc. Rural 1995, 25, 399–403. [Google Scholar] [CrossRef]


| Farm | Coordinates | Total Area | Useful Area | t0 | t | n | h100 | |||
|---|---|---|---|---|---|---|---|---|---|---|
| S | W | (ha) | (m2 tree−1) | (Years) | (Tree) | (cm) | (m) | (m) | ||
| Aguaçu | 15°37′ | 55°33′ | 118.5 | 6 | 2001 | 12 | 52 | 25.02 (±5.16) | 19.0 (±1.7) | 21.3 |
| Soroteca | 15°37′ | 58°18′ | 60.0 | 9 | 2000 | 8 | 63 | 18.24 (±2.48) | 17.0 (±1.3) | 17.5 |
| Teca do Brasil | 16°12′ | 56°22′ | 1260.0 | 9 | 1999–2003 | 9 to 16 | 224 | 23.04 (±8.64) | 18.7 (±3.5) | 17.9 |
| Teca do Jauru | 15°25′ | 58°37′ | 6.8 | 3 | 1979 | 36 | 31 | 40.55 (±5.16) | 24.3 (±3.2) | 27.6 |
| Class | Diameter Class (d—cm) | n | FA | CV | ||
|---|---|---|---|---|---|---|
| Lower Limit | Center of Class | Upper Limit | (Trees) | (Trees) | (%) | |
| 1 | 10.0 | ├ | 15.0 | 33 | 20 | 28 |
| 2 | 15.0 | ├ | 20.0 | 3 | 20 | 9 |
| 3 | 20.0 | ├ | 25.0 | 9 | 20 | 14 |
| 4 | 25.0 | ├ | 30.0 | 2 | 20 | 8 |
| 5 | 30.0 | ├ | 35.0 | 10 | 20 | 15 |
| 6 | 35.0 | ├ | 40.0 | 12 | 16 | 16 |
| 7 | 40.0 | ├ | 45.0 | 9 | 18 | 14 |
| 8 | 45.0 | ├ | 50.0 | 5 | 9 | 9 |
| 9 | 50.0 | ├ | 55.0 | 4 | 2 | 2 |
| 10 | 55.0 | ├ | 60.0 | 27 | 3 | 12 |
| SUM | 148 | |||||
| Class | Diameter Class at Breast Height (d—cm) | Absolute Frequency | ||
|---|---|---|---|---|
| Lower Limit | Center of Class | Upper Limit | (Trees) | |
| 1 | 10.0 | ├ | 15.0 | 20 |
| 2 | 15.0 | ├ | 20.0 | 20 |
| 3 | 20.0 | ├ | 25.0 | 20 |
| 4 | 25.0 | ├ | 30.0 | 20 |
| 5 | 30.0 | ├ | 35.0 | 20 |
| 6 | 35.0 | ├ | 40.0 | 16 |
| 7 | 40.0 | ├ | 45.0 | 18 |
| 8 | 45.0 | ├ | 50.0 | 9 |
| 9 | 50.0 | ├ | 55.0 | 2 |
| 10 | 55.0 | ├ | 60.0 | 3 |
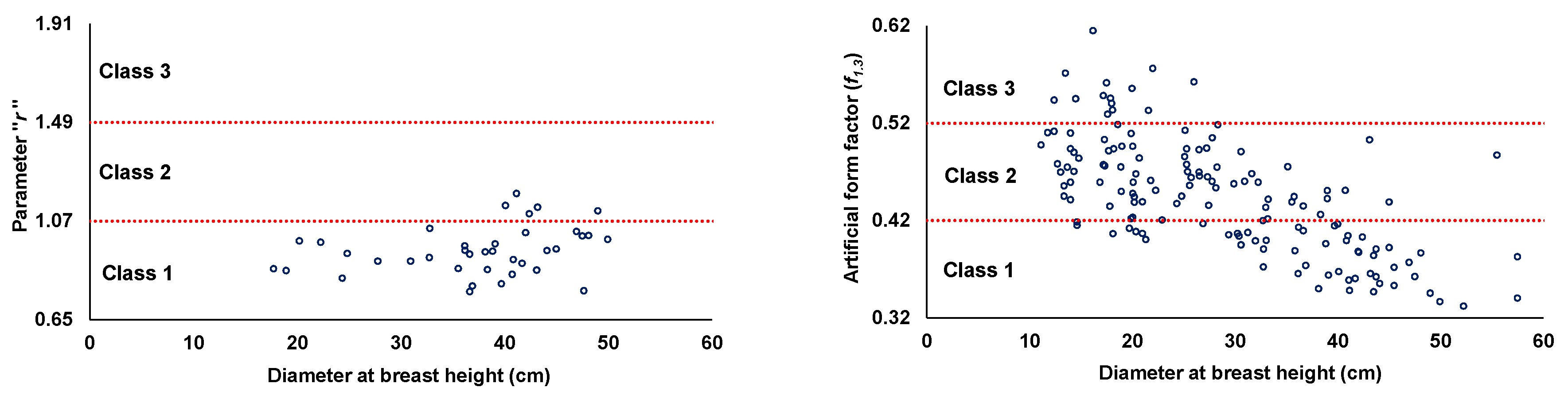
| Class | Parameter “r” | Absolute Frequency | ||
| Lower Limit | Center of Class | Upper Limit | (trees) | |
| 1 | 0.30 | ├ | 0.80 | 85 |
| 2 | 0.80 | ├ | 1.30 | 45 |
| 3 | 1.30 | ├ | 1.80 | 18 |
| Class | Artificial Form Factor (f1.3) | Absolute Frequency | ||
| Lower Limit | Center of Class | Upper Limit | (trees) | |
| 1 | 0.30 | ├ | 0.38 | 32 |
| 2 | 0.38 | ├ | 0.46 | 100 |
| 3 | 0.46 | ├ | 0.55 | 16 |
| Clark III et al. [30] | |
|---|---|
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 | |
| 8 |
| Class DBH | Average Values in Each Diameter Class—Schöepfer Model (1966) [40] | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| FCP1 | FCP2 | FCP3 | y1 (di) | x1 (hi) | y2 (di) | x2 (hi) | y3 (di) | x3 (hi) | |
| 1 | 0.26 | 0.50 | 0.69 | 11.01 | 3.6 | 8.25 | 6.9 | 7.16 | 9.5 |
| 2 | 0.28 | 0.55 | 0.76 | 14.00 | 4.8 | 11.20 | 9.4 | 6.03 | 13.1 |
| 3 | 0.28 | 0.57 | 0.73 | 16.37 | 5.1 | 14.08 | 10.4 | 7.91 | 13.4 |
| 4 | 0.29 | 0.61 | 0.76 | 21.21 | 6.1 | 18.79 | 13.0 | 11.04 | 16.1 |
| 5 | 0.29 | 0.64 | 0.79 | 24.21 | 6.5 | 18.14 | 14.4 | 9.69 | 17.9 |
| 6 | 0.27 | 0.54 | 0.73 | 28.32 | 7.0 | 22.63 | 14.3 | 13.59 | 19.2 |
| 7 | 0.27 | 0.54 | 0.76 | 31.11 | 7.2 | 26.98 | 14.6 | 12.53 | 19.9 |
| 8 | 0.27 | 0.56 | 0.72 | 33.63 | 7.3 | 23.25 | 15.3 | 15.56 | 19.5 |
| 9 | 0.31 | 0.65 | 0.69 | 33.02 | 8.8 | 41.97 | 19.1 | 18.34 | 19.6 |
| 10 | 0.30 | 0.57 | 0.68 | 40.82 | 7.7 | 57.26 | 16.0 | 24.11 | 17.2 |
| Minimum | 0.26 | 0.50 | 0.68 | 11.01 | 3.6 | 8.25 | 6.9 | 6.03 | 9.5 |
| Maximum | 0.31 | 0.65 | 0.79 | 40.82 | 8.8 | 57.26 | 19.1 | 24.11 | 19.9 |
| Mean | 0.28 | 0.57 | 0.73 | 25.37 | 6.4 | 24.26 | 13.3 | 12.59 | 16.5 |
| Model | Estimated Parameters | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| β0 | β1 | β2 | β3 | β4 | β5 | β6 | β7 | β8 | β9 | |
| Single equation | ||||||||||
| Schöepfer [40] | 1.277 * | −5.658 * | 24.241 * | −50.413 * | 46.499 * | −15.931 * | ||||
| Kozak et al. (a) (1969) [25] | −2.219 * | 1.025 * | ||||||||
| Ormerod [44] | 0.841 * | |||||||||
| Demaerschalk [42] | 0.182 * | 0.913 * | 0.888 * | −0.895 * | ||||||
| Demaerschalk (a) [43] | 1.185 * | 1.778 * | ||||||||
| Demaerschalk (c) [43] | 8686.5 * | 40.665 * | 1.089 * | 1.658 * | ||||||
| Segmented | ||||||||||
| Max and Burkhart [28] | 0.087 * | 0.713 * | −2.936 * | 1.411 * | 113.1 * | −1.203 * | ||||
| Clark III et al. [30] | 40.875 * | 8.587 * | 0.898 * | 928.1 * | 0.655 * | 1.806 * | ||||
| Variable-exponent | ||||||||||
| Kozak (1988) [36] | 1.091 * | 0.962 * | 0.999 * | 0.321 * | −0.177 * | −0.394 ns | 0.292 * | 0.055 * | ||
| Modified Kozak (1988) [36] | 1.086 * | 0.963 * | 0.999 * | 0.481 * | −0.215 * | 0.083 * | 0.057 * | |||
| Kozak (a) (2004) [31] | 1.331 * | 0.981 * | 0.522 * | −0.048 ns | 0.024 * | −0.251 * | ||||
| Modified Kozak (a) (2004) [31] | 1.345 * | 0.977 * | 0.511 * | 0.025 * | −0.268 * | |||||
| Kozak (b) (2004) [31] | 1.107 * | 0.953 * | 0.021 ns | 0.759 * | −1.287 * | −0.009 * | 1.034 * | 2.520 * | 0.145 * | −1.573 * |
| Modified Kozak (b) (2004) [31] | 1.140 * | 0.963 * | 0.755 * | −1.322 * | −0.009 * | 1.010 * | 2.797 * | 0.149 * | −1.567 * | |
| Bi [32] | 28.51 * | −13.49 * | −2.129 * | −15.034 * | 1.045 * | −14.99 * | 9.758 * | |||
| Lee et al. [47] | 1.700 * | 0.907 * | 4.123 * | −5.715 * | 2.853 * | |||||
| Model | SQRes | Syx% | R²aj. |
|---|---|---|---|
| Single equation | |||
| Schöepfer [40] | 11,378.8 | 9.63 | 0.97 |
| Kozak et al. (a) (1969) [25] | 22,940.1 | 13.67 | 0.95 |
| Ormerod [44] | 24,617.8 | 14.16 | 0.94 |
| Demaerschalk [42] | 22,492.0 | 13.54 | 0.95 |
| Demaerschalk (a) [43] | 23,764.0 | 13.92 | 0.94 |
| Demaerschalk (c) [43] | 18,077.2 | 12.14 | 0.96 |
| Segmented | |||
| Max and Burkhart [28] | 10,658.6 | 9.33 | 0.97 |
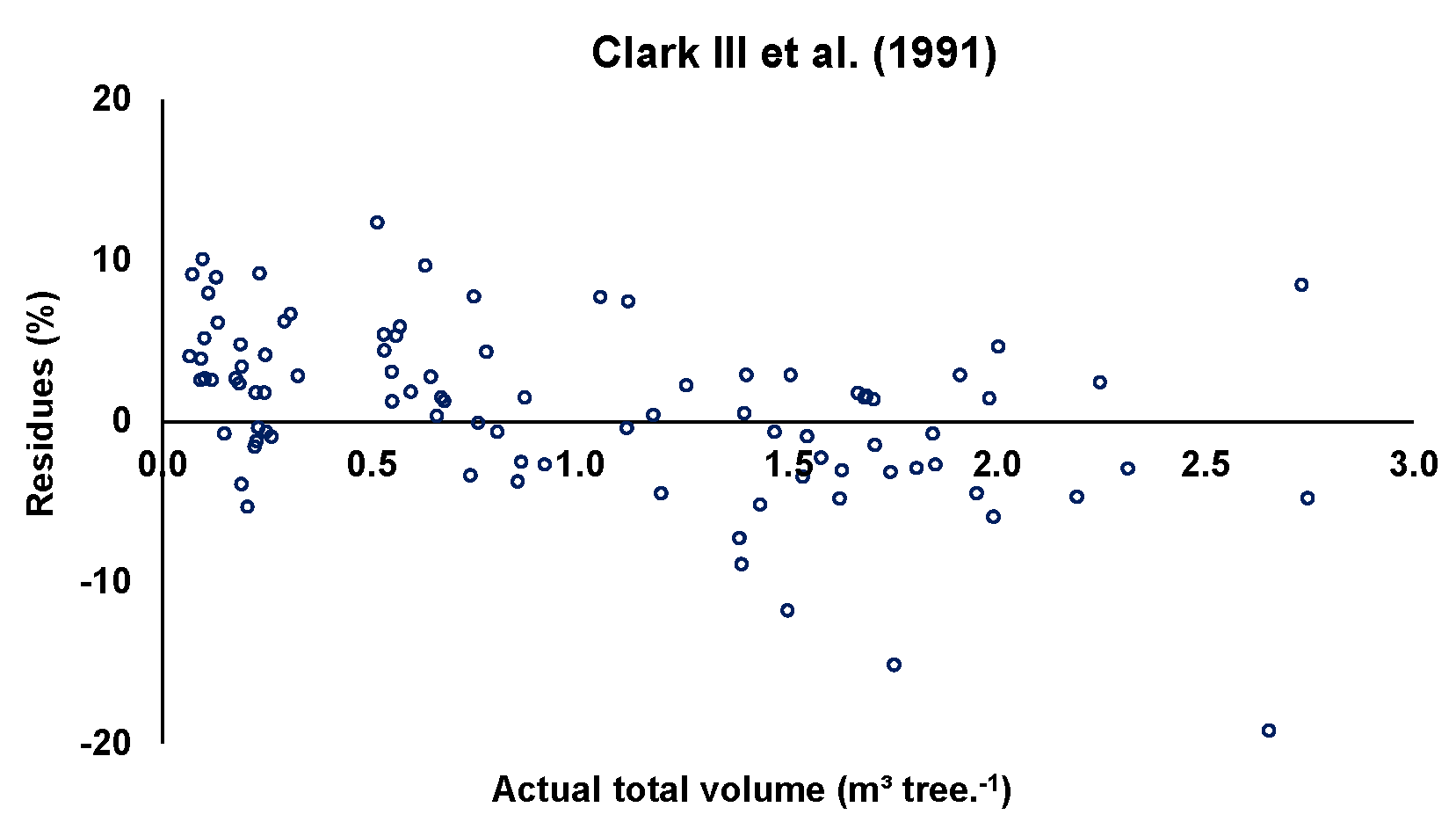
| Clark III et al. [30] | 6447.5 | 7.25 | 0.98 |
| Variable-exponent | |||
| Modified Kozak (1988) [36] | 8906.2 | 8.53 | 0.98 |
| Modified Kozak (a) (2004) [31] | 11,415.0 | 9.65 | 0.97 |
| Modified Kozak (b) (2004) [31] | 9054.2 | 8.60 | 0.98 |
| Bi [32] | 18,587.1 | 12.32 | 0.96 |
| Lee et al. [47] | 11,378.8 | 9.63 | 0.97 |
| Class of DBH | N | DBH Class | Estimated Parameters | SQRes | Syx% | R²aj. | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Li | Ls | β0 | β1 | β2 | β3 | β4 | β5 | |||||
| 1 | 20 | 10 | 15 | 25.531 * | 4.445 * | 0.899 * | 501.7 ns | 0.929 * | 4.384 ns | 78.1 | 6.82 | 0.99 |
| 2 | 20 | 15 | 20 | 37.490 * | 5.288 * | 0.215 ns | 4440.9 * | 0.935 * | 3.927 * | 113.5 | 4.79 | 0.98 |
| 3 | 20 | 20 | 25 | 39.231 * | 7.109 * | 1.047 * | −289.4 ns | 0.701 * | 1.797 * | 247.6 | 5.51 | 0.97 |
| 4 | 20 | 25 | 30 | 52.951 * | 8.742 * | 0.834 * | 6780.6 ns | 0.834 * | 3.509 * | 886.3 | 6.95 | 0.98 |
| 5 | 20 | 30 | 35 | 46.319 * | 8.370 * | 1.000 * | 22981.6 * | 0.600 * | 1.880 * | 745.8 | 6.29 | 0.98 |
| 6 | 16 | 35 | 40 | 50.722 * | 10.062 * | 0.893 * | 8047.0 ns | 0.721 * | 1.964 * | 731.3 | 5.87 | 0.98 |
| 7 | 18 | 40 | 45 | 37.376 * | 7.378 * | 1.097 * | −15525.2 ns | 0.560 * | 1.465 * | 1470.9 | 7.14 | 0.98 |
| 8 | 9 | 45 | 50 | 30.956 * | 14.406 * | −0.489 ns | 132825.0 * | 0.590 * | 1.540 * | 466.1 | 5.32 | 0.99 |
| 9 | 2 | 50 | 55 | −3.137 ns | 15.709 * | 0.567 ns | −11842.3 ns | 0.591 * | 1.758 * | 52.6 | 4.25 | 0.99 |
| 10 | 3 | 55 | 60 | 34.699 * | 12.687 * | 3.099 ns | −381868.0 ns | 0.647 * | 1.899 * | 424.6 | 9.30 | 0.99 |
| No Stratification | 40.874 * | 8.585 * | 0.898 * | 929.1 * | 0.655 * | 1.806 * | 6449.1 | 7.25 | 0.98 | |||
| Class of r | N | Parameter “r” | Estimated Parameters | SQRes | Syx% | R²aj. | ||||||
| Li | Ls | β0 | β1 | β2 | β3 | β4 | β5 | |||||
| 1 | 85 | 0.3 | 0.8 | 42.646 * | 9.684 * | 0.924 * | 1745.9 * | 0.726 * | 2.028 * | 4379.6 | 7.27 | 0.98 |
| 2 | 45 | 0.8 | 1.3 | 42.861 * | 8.045 * | 0.877 * | 676.7 * | 0.551 * | 1.635 * | 1595.8 | 6.58 | 0.99 |
| 3 | 18 | 1.3 | 1.8 | 17.687 * | 5.595 * | 0.433 * | 1846.7 * | 0.589 * | 1.433 * | 177.4 | 5.82 | 0.99 |
| No Stratification | 40.874 * | 8.585 * | 0.898 * | 929.1 * | 0.655 * | 1.806 * | 6449.1 | 7.25 | 0.98 | |||
| Class of f1.3 | N | f1.3 | Estimated Parameters | SQRes | Syx% | R²aj. | ||||||
| Li | Ls | β0 | β1 | β2 | β3 | β4 | β5 | |||||
| 1 | 32 | 0.30 | 0.38 | 47.673 * | 11.816 * | 0.841 * | 4840.6 * | 0.579 * | 1.468 * | 1600.2 | 5.95 | 0.99 |
| 2 | 100 | 0.38 | 0.46 | 39.092 * | 7.531 * | 0.925 * | 513.1 * | 0.697 * | 2.071 * | 3659.3 | 6.96 | 0.98 |
| 3 | 16 | 0.46 | 0.54 | 23.057 * | 4.215 * | 0.761 * | 1166.7 * | 0.957 * | 9.291 * | 415.7 | 7.57 | 0.98 |
| No Stratification | 40.874 * | 8.585 * | 0.898 * | 929.1 * | 0.655 * | 1.806 * | 6449.1 | 7.25 | 0.98 | |||
| Model | Estimated Parameters | SQRes | Syx% | R²aj. | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| β0 | β1 | β2 | β3 | β4 | β5 | β6 | ||||
| Clark III et al. [30] | 40.875 * | 8.587 * | 0.898 * | 928.2 * | 0.655 * | 1.806 * | 6449.6 | 7.25 | 0.98 | |
| Modification 1 | 41.837 * | 8.587 * | 0.943 * | 0.655 * | 1.806 * | 6500.3 | 7.28 | 0.98 | ||
| Modification 2 | 47.265 * | 8.587 * | 0.655 * | 1.806 * | 6512.1 | 7.29 | 0.98 | |||
| Modification 3 | Hessian matrix | |||||||||
| Modification 4 | 40.875 * | 7.799 * | 0.898 * | 928.1 * | 0.603 * | 1.719 * | 6378.8 | 7.21 | 0.98 | |
| Modification 5 | 40.875 * | 8.587 * | 0.898 * | 928.1 * | 0.628 * | 1.707 * | 0.083 * | 6358.2 | 7.20 | 0.98 |
| Modification 6 | 40.875 * | 7.799 * | 0.898 * | 928.1 * | 0.548 * | 1.565 * | 0.348 * | 6118.4 | 7.07 | 0.99 |
| Modification 7 | 40.875 * | 8.587 * | 0.898 * | 928.1 * | 0.610 * | 1.646 * | 0.017 * | 6252.9 | 7.14 | 0.99 |
| Modification 8 | 40.875 * | 7.799 * | 0.898 * | 928.1 * | 0.548 * | 1.564 * | 0.020 * | 6139.3 | 7.08 | 0.99 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rocha, K.J.d.; Finger, C.A.G.; Favalessa, C.M.C.; Caldeira, S.F.; Fleig, F.D. Form and Volume of the Stem of Tectona grandis L.f. in the Central-WESTERN Region of Brazil. Forests 2022, 13, 1818. https://doi.org/10.3390/f13111818
Rocha KJd, Finger CAG, Favalessa CMC, Caldeira SF, Fleig FD. Form and Volume of the Stem of Tectona grandis L.f. in the Central-WESTERN Region of Brazil. Forests. 2022; 13(11):1818. https://doi.org/10.3390/f13111818
Chicago/Turabian StyleRocha, Karen Janones da, César Augusto Guimarães Finger, Cyro Matheus Cometti Favalessa, Sidney Fernando Caldeira, and Frederico Dimas Fleig. 2022. "Form and Volume of the Stem of Tectona grandis L.f. in the Central-WESTERN Region of Brazil" Forests 13, no. 11: 1818. https://doi.org/10.3390/f13111818
APA StyleRocha, K. J. d., Finger, C. A. G., Favalessa, C. M. C., Caldeira, S. F., & Fleig, F. D. (2022). Form and Volume of the Stem of Tectona grandis L.f. in the Central-WESTERN Region of Brazil. Forests, 13(11), 1818. https://doi.org/10.3390/f13111818







