Streamflow Variability Indicated by False Rings in Bald Cypress (Taxodium distichum (L.) Rich.)
Abstract
1. Introduction
2. Materials and Methods
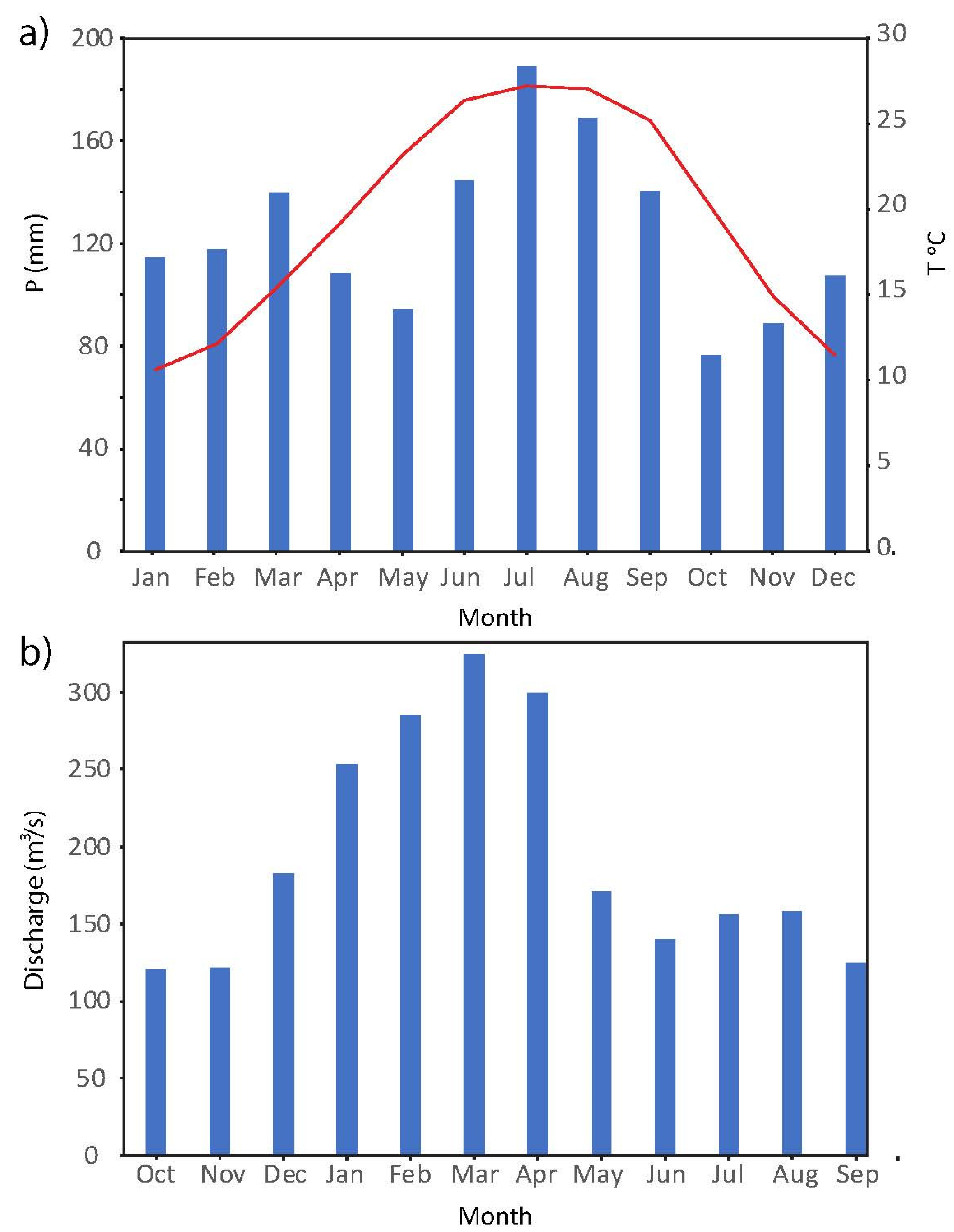
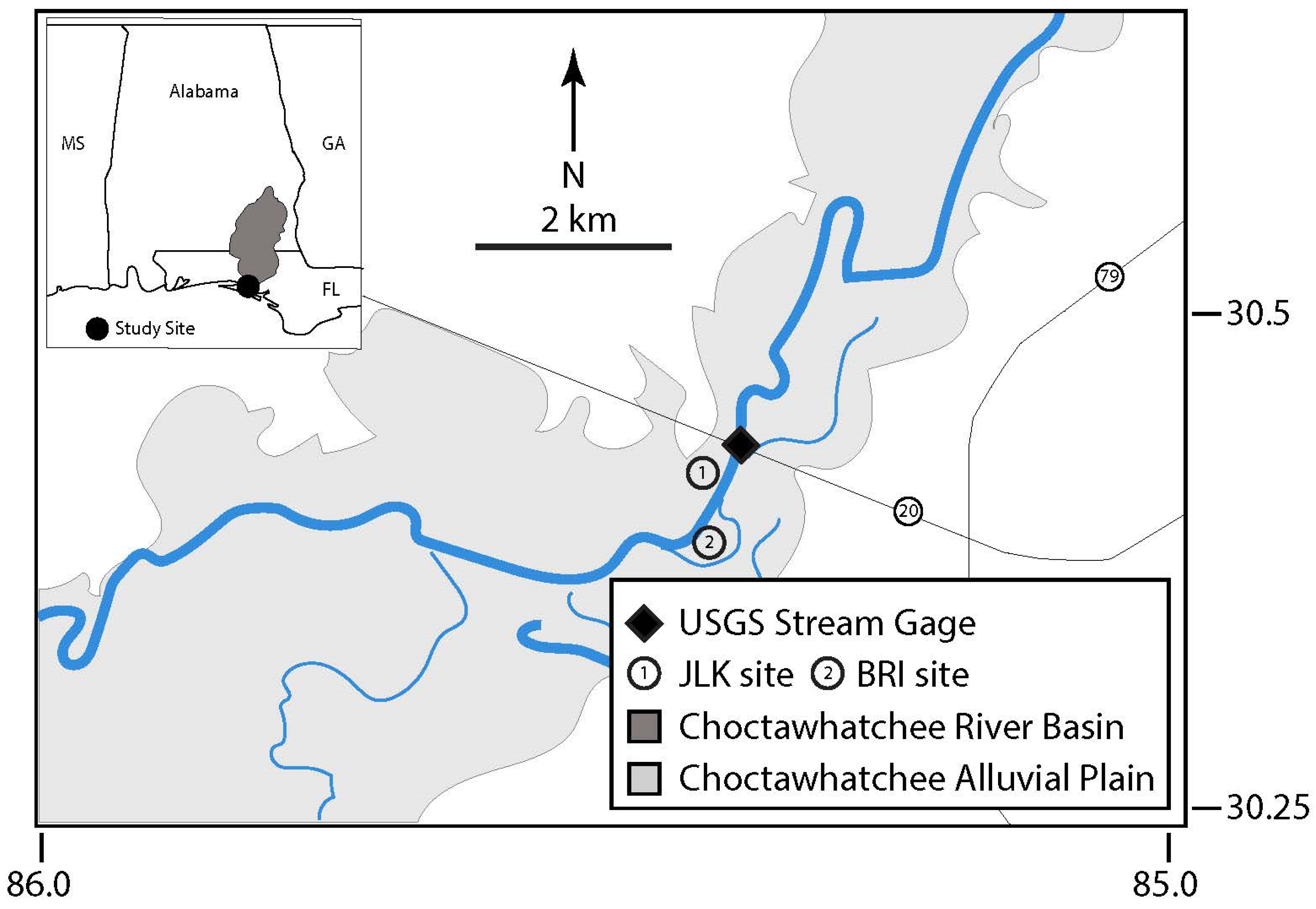
2.1. Study Area
2.2. Tree Ring Data
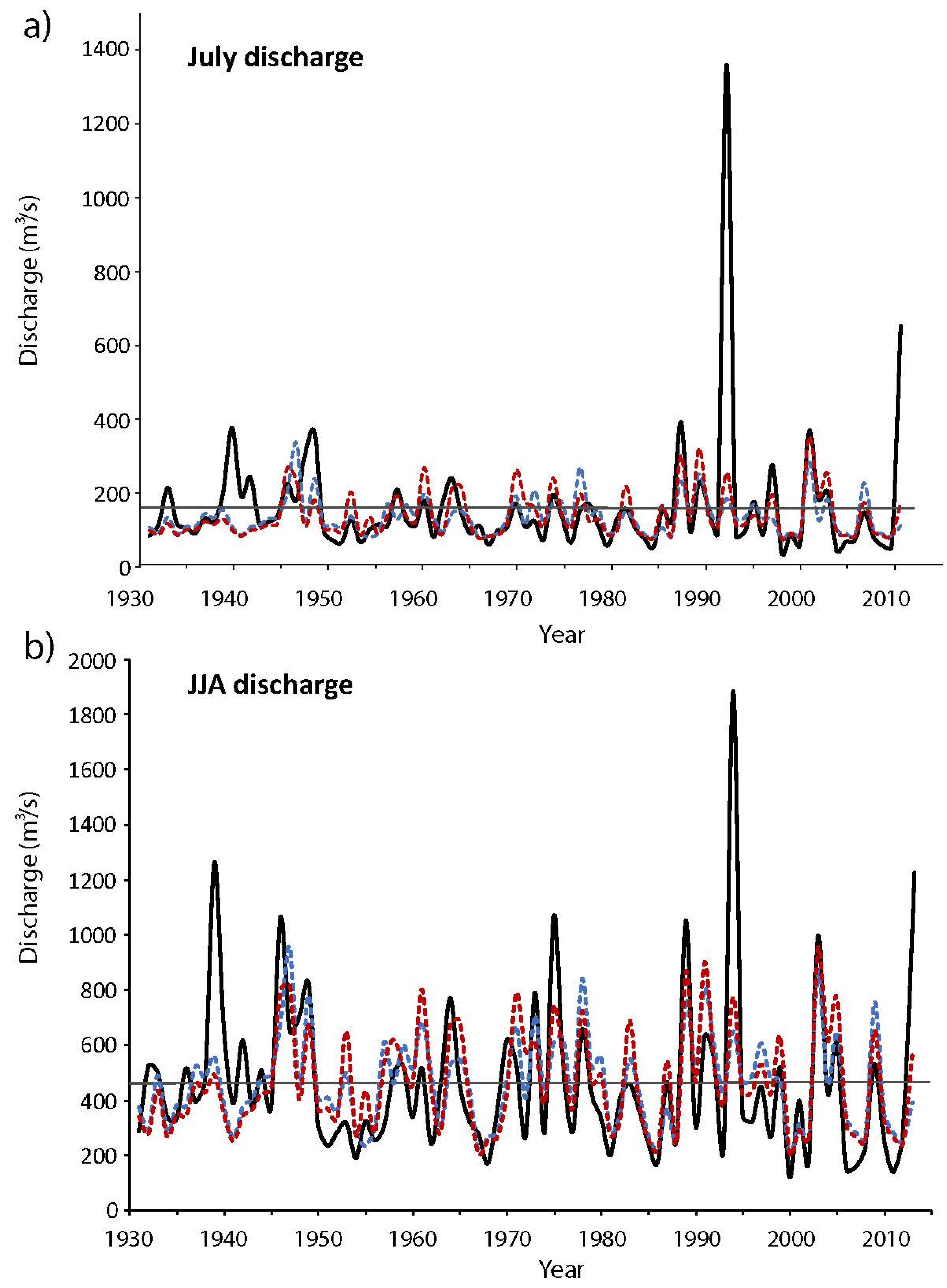
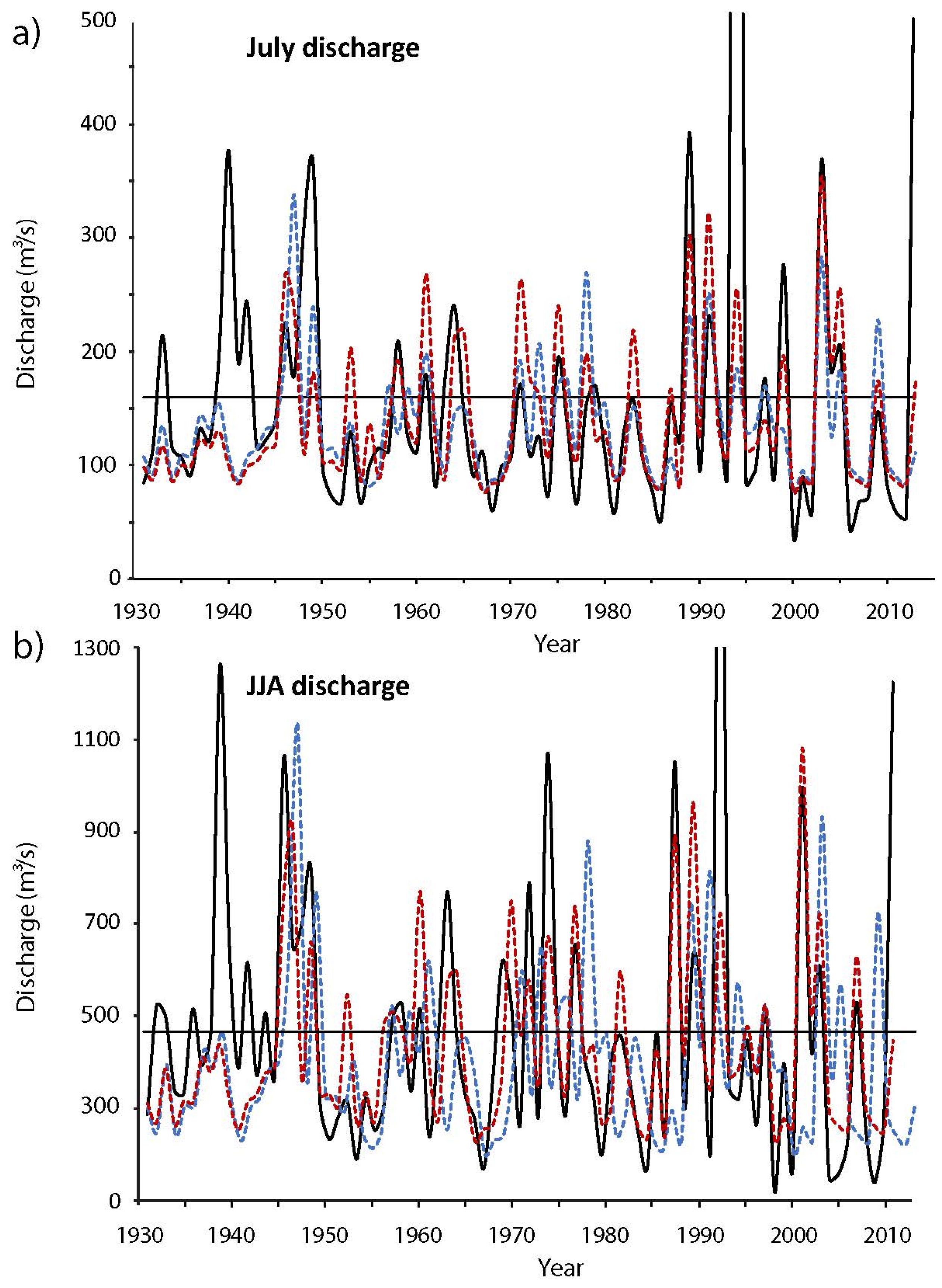
2.3. Climate and Streamflow Data
2.4. Regression Equations: Using Ring Width and False Rings to Predict Summer Streamflow
2.5. False Ring Association with Summer Storms/Tropical Cyclones
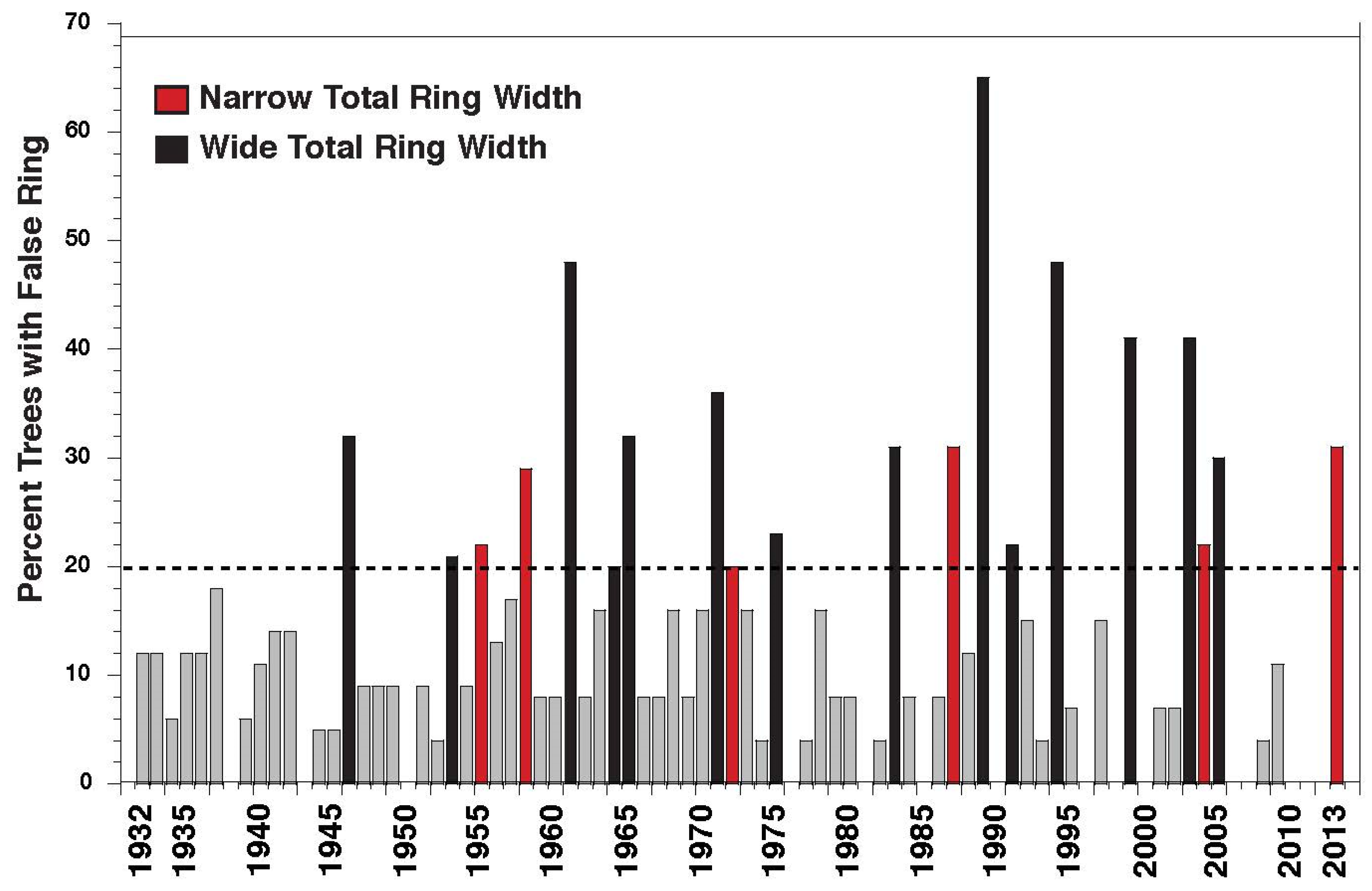
3. Results
3.1. Ring Width Chronology
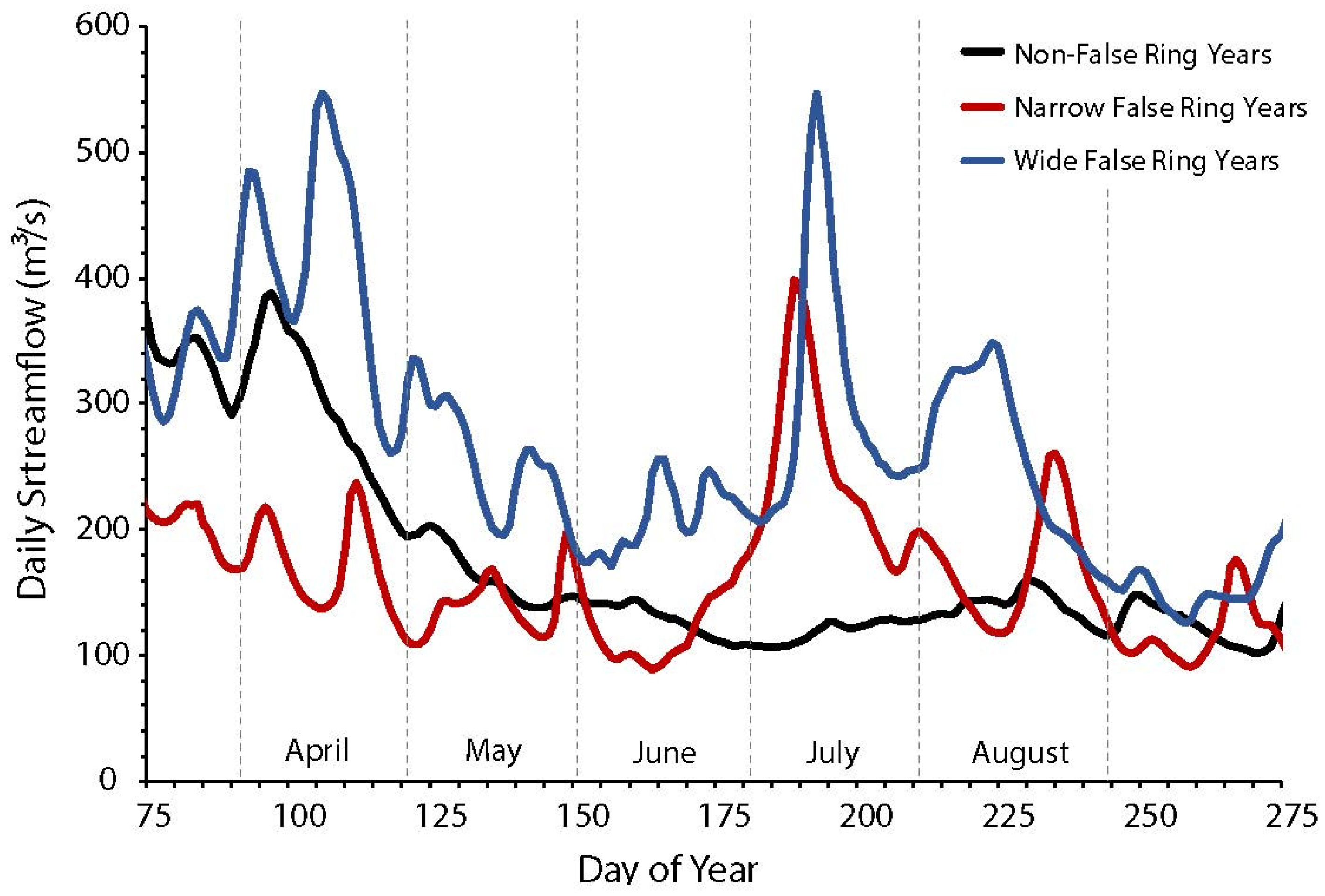
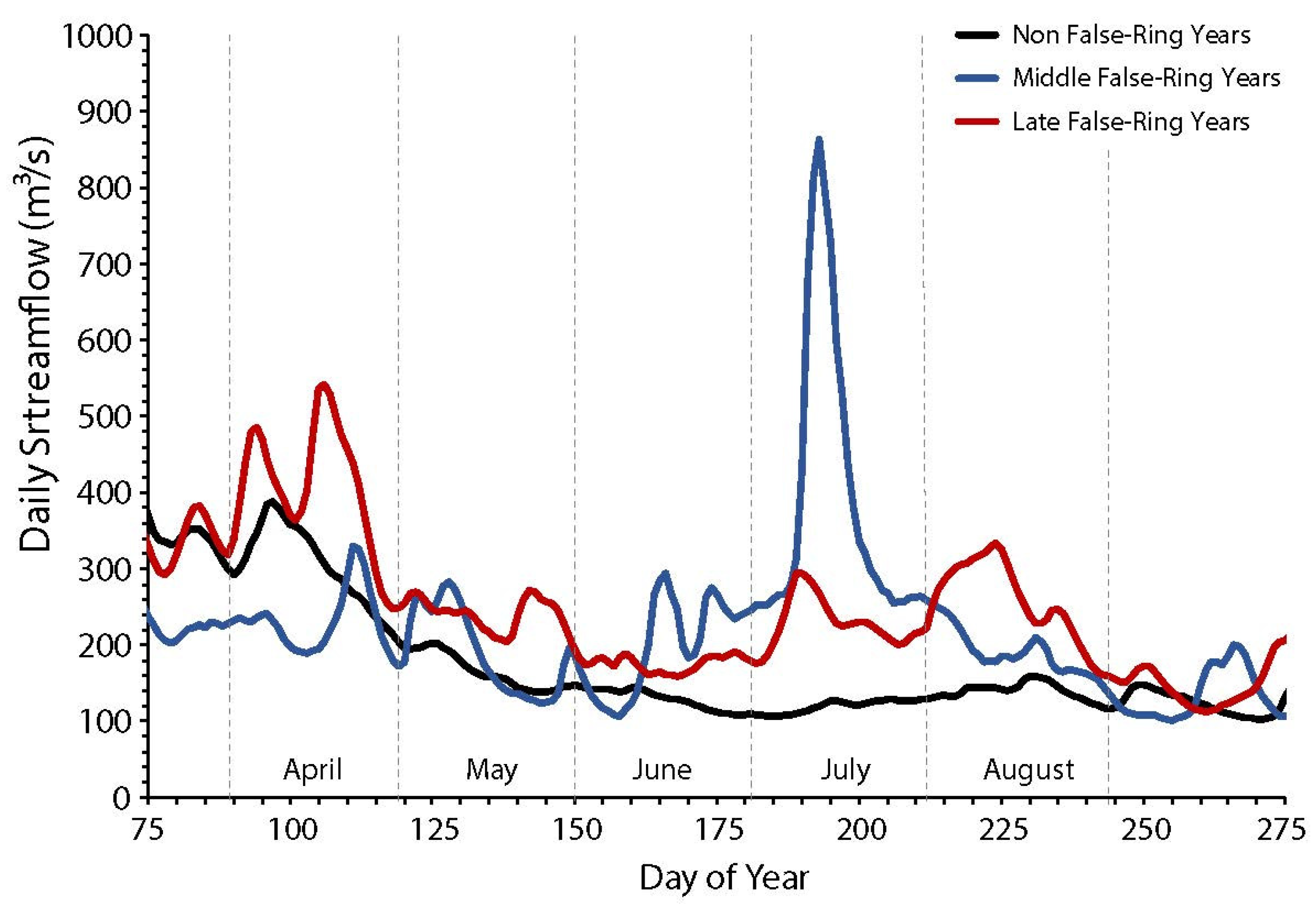
3.2. False Ring Chronology
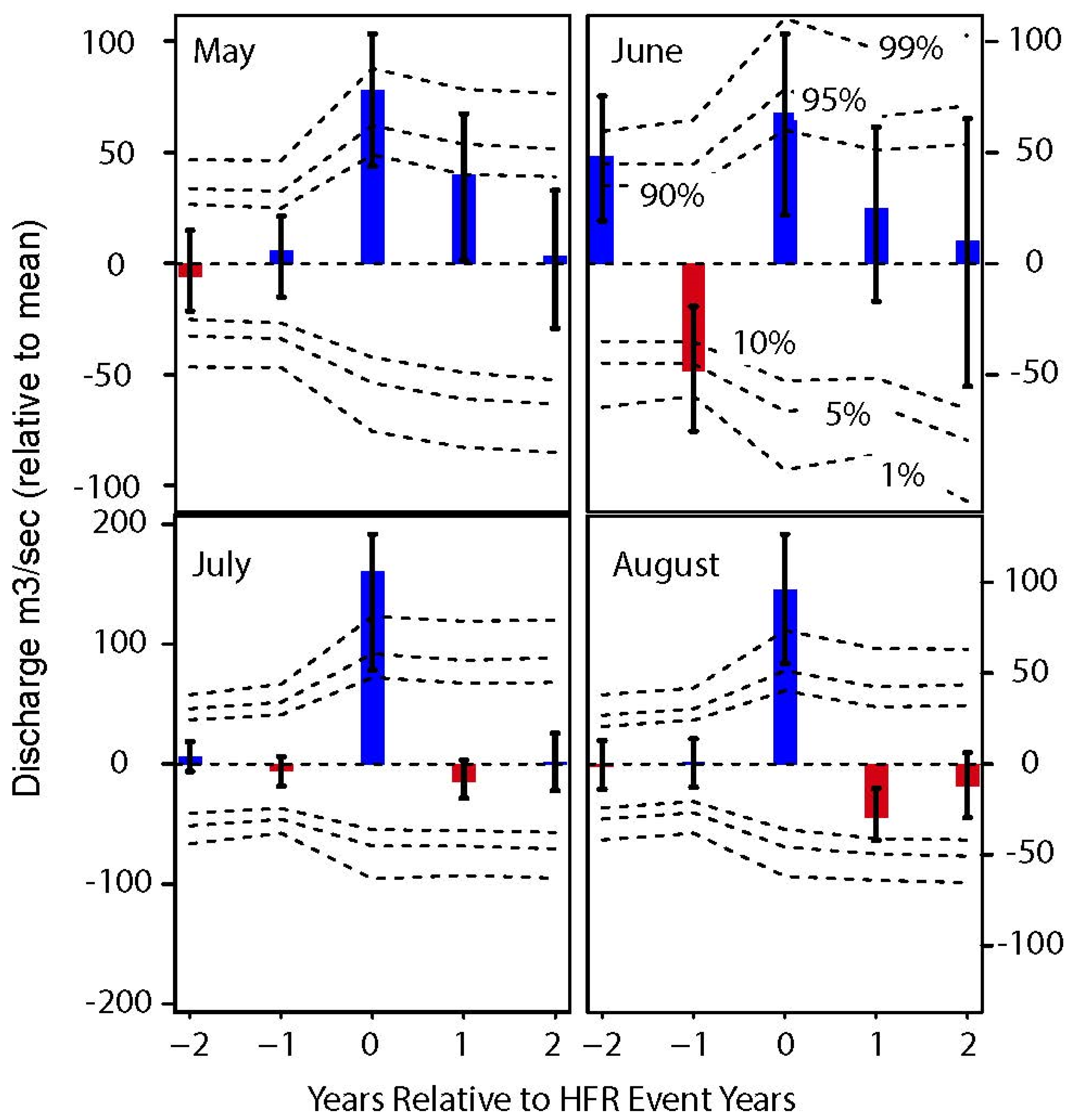
3.3. Hydroclimatic Significance of False Rings
3.4. Estimating Streamflow with Ring Width and FR
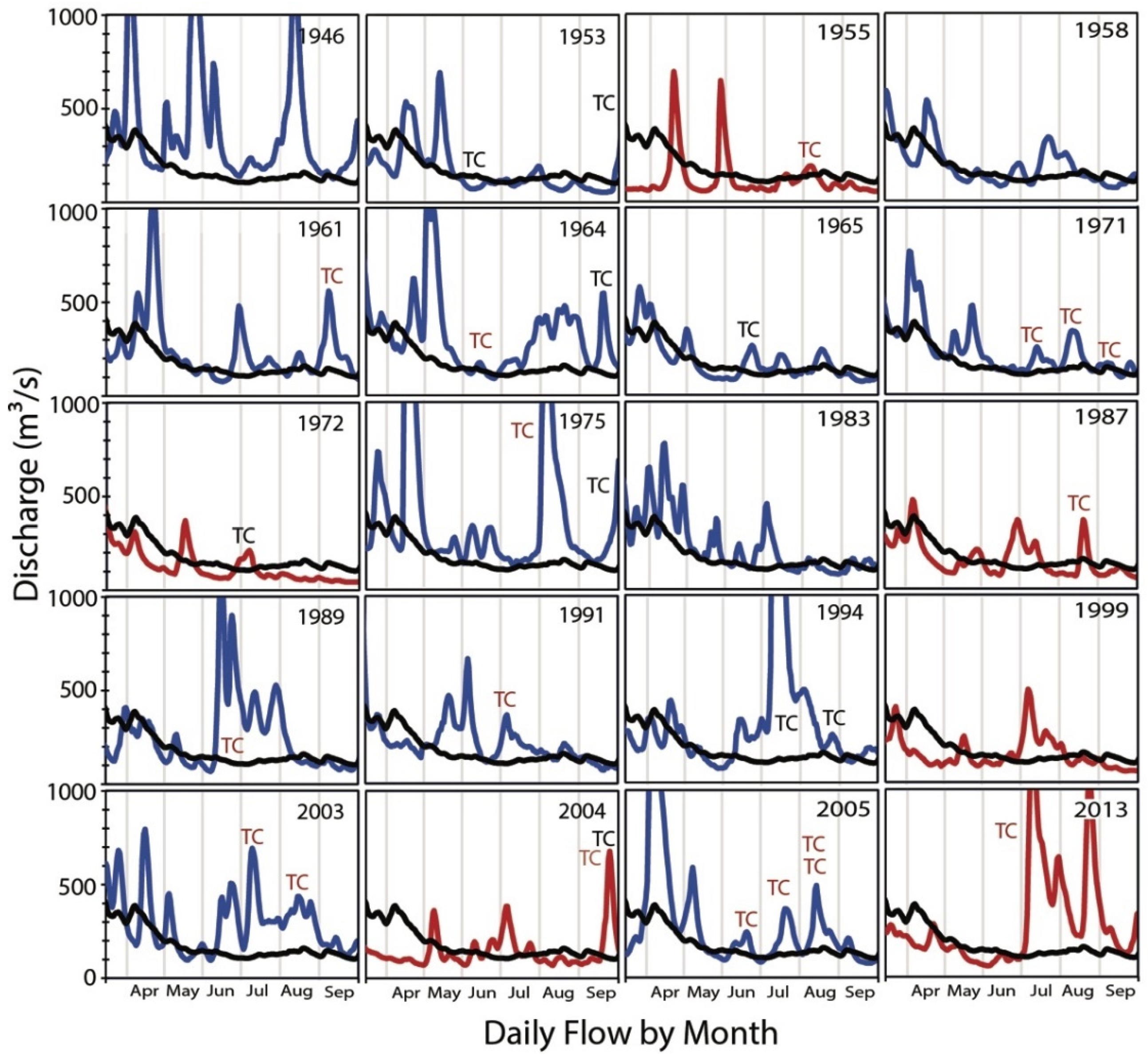
3.5. False Rings and Tropical Cyclone Activity
4. Discussion
4.1. Use of False Rings for Streamflow Reconstructions
4.2. False Ring Association with Summer Storms/Tropical Cyclones
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
References
- Schulman, E. Classification of false annual rings in west Texas pines. Tree-Ring Bull. 1939, 6, 11–13. [Google Scholar]
- Fritts, H.C. Tree Rings and Climate; The Blackburn Press: Caldwell, NJ, USA, 1976. [Google Scholar]
- Beaufait, W.R.; Nelson, T.C. Ring counts in second-growth bald cypress. J. For. 1957, 55, 588. [Google Scholar]
- Bowers, L.J.; Gosselink, J.G.; Patrick, W.H., Jr.; Choong, E.T. Investigation of six anatomical and four statistical features of bald-cypress (Taxodium distichum) tree rings. For. Ecol. Manag. 1990, 33, 503–508. [Google Scholar] [CrossRef]
- Young, P.J.; Megonigal, J.P.; Sharitz, R.R.; Day, F.P. False ring formation in baldcypress (Taxodium distichum) saplings under two flooding regimes. Wetlands 1993, 13, 293–298. [Google Scholar] [CrossRef]
- Villalba, R.; Veblen, T.T. A tree-ring record of dry spring-wet summer events in the forest-steppe ecotone, Northern Patagonia, Argentina. In Tree Rings, Environment and Humanity: 107–116. Radiocarbon; Dean, J.S., Meko, D.M., Swetnam, T.W., Eds.; University of Arizona: Tucson, AZ, USA, 1996. [Google Scholar]
- Priya, P.B.; Bhat, K.M. False ring formation in teak (Tectona grandis L.f.) and the influence of environmental factors. For. Ecol. Manag. 1998, 108, 215–222. [Google Scholar] [CrossRef]
- Wimmer, R.; Strumia, G.; Holawe, F. Use of false rings in Austrian pine to reconstruct early growing season precipitation. Can. J. For. Res. 2000, 30, 1691–1697. [Google Scholar] [CrossRef]
- Masiokas, M.; Villalba, R. Climatic significance of intra-annual bands in the wood of Nothofagus pumillo in southern Patagonia. Trees 2004, 18, 696–704. [Google Scholar] [CrossRef]
- Edmondson, J.R. The meterological significance of false rings in eastern redcedar (Juniperus virginiana L.) from the Southern Great Plains, USA. Tree-Ring Res. 2010, 66, 19–34. [Google Scholar] [CrossRef]
- Griffin, D.; Meko, D.M.; Touchan, R.; Leavitt, S.W.; Woodhouse, C.A. Latewood Chronology Development for Summer-Moisture Reconstruction in the US Southwest. Tree-Ring Res. 2011, 67, 87–101. [Google Scholar] [CrossRef]
- Harley, G.L.; Grissino-Mayer, H.D.; Franklin, J.A.; Anderson, C.; Köse, N. Cambial activity of Pinus elliottii var. densa reveals influence of solar radiation on seasonal growth dynamics in the Florida Keys. Trees–Struct. Funct. 2012, 26, 1449–1459. [Google Scholar] [CrossRef]
- Harley, G.L.; Maxwell, J.T.; Larson, E.; Grissino-Mayer, H.D.; Henderson, J.; Huffman, J. Suwannee River flow variability 1550–2005 CE reconstructed from a multispecies tree-ring network. J. Hydrol. 2017, 544, 438–451. [Google Scholar] [CrossRef]
- De Micco, V.; Campelo, F.; De Luis, M.; Bräuning, A.; Grabner, M.; Battipaglia, G.; Cherubini, P. Intra-annual density fluctuations in tree rings: How, when, where, and why? IAWA J. Int. Assoc. Wood Anat. 2016, 37, 232–259. [Google Scholar] [CrossRef]
- Copenheaver, C.A.; Pokorski, E.A.; Currie, J.E.; Abrams, M.D. Causation of false ring formation in Pinus banksiana: A comparison of age, canopy class, climate and growth rate. For. Ecol. Manag. 2006, 236, 348–355. [Google Scholar] [CrossRef]
- Copenheaver, C.A.; Matiuk, J.D.; Nolan, L.J.; Franke, M.E.; Block, P.R.; Reed, W.P.; Kidd, K.R.; Martini, G. False Ring Formation in Bald Cypress (Taxodium distichum). Wetlands 2017, 37, 1037–1044. [Google Scholar] [CrossRef]
- Babst, F.; Wright, W.E.; Szejner, P.; Wells, L.; Belmecheri, S.; Monson, R.K. Blue intensity parameters derived from Ponderosa pine tree rings characterize intra-annual density fluctuations and reveal seasonally divergent water limitations. Trees 2016, 30, 1403–1415. [Google Scholar] [CrossRef]
- Mitchell, T.J.; Knapp, P.A.; Ortegren, J.T. Tropical cyclone frequency inferred from intra-annual density fluctuations in longleaf pine in Florida, USA. Clim. Res. 2019, 78, 249–259. [Google Scholar] [CrossRef]
- Stahle, D.W.; Cleaveland, M.K.; Hehr, J.G. North-Carolina climate changes reconstructed from tree rings—AD 372 to 1985. Science 1988, 240, 1517–1519. [Google Scholar] [CrossRef]
- Stahle, D.W.; Cleaveland, M.K. Reconstruction and analysis of spring rainfall over the Southeast U.S. for the past 1000 years. Bull. Am. Meteorol. Soc. 1992, 73, 1947–1961. [Google Scholar] [CrossRef]
- Stahle, D.W.; Cleaveland, M.K.; Blanton, D.B.; Therrell, M.D.; Gay, D.A. The lost colony and Jamestown droughts. Science 1998, 280, 564–567. [Google Scholar] [CrossRef]
- Stahle, D.W.; Burnett, D.J.; Villaneuva, J.; Cerano, J.; Fye, F.K.; Griffin, R.D.; Cleaveland, M.K.; Stahle, D.K.; Edmondson, J.R.; Wolff, K.P. Tree-ring analysis of ancient baldcypress trees and subfossil wood. Quat. Sci. Rev. 2012, 34, 1–15. [Google Scholar] [CrossRef]
- Stahle, D.K.; Burnette, D.J.; Stahle, D.W. A moisture balance reconstruction for the drainage basin of Albemarle Sound, North Carolina. Estuar. Coasts 2013, 36, 1340–1353. [Google Scholar] [CrossRef]
- Cleaveland, M.K. A 963-year reconstruction of summer (JJA) streamflow in the White River, Arkansas, USA, from tree-rings. Holocene 2000, 10, 33–41. [Google Scholar] [CrossRef]
- Keim, R.F.; Amos, J.B. Dendrochronological analysis of bald cypress (Taxodium distichum) response to climate and contrasting flood regimes. Can. J. For. Res. 2012, 42, 423–436. [Google Scholar] [CrossRef]
- Palta, M.M.; Doyle, T.W.; Jackson, C.R.; Meyer, J.L.; Sharitz, R.R. Changes in diameter growth of Taxodium distichum in response to flow alteration in the Savannah River. Wetlands 2012, 32, 59–71. [Google Scholar] [CrossRef]
- U.S. Geological Surve. National Water Information System Data Available on the World Wide Web (USGS Water Data for the Nation). 2016. Available online: http://waterdata.usgs.gov/nwis//inventory/?site_no=02361000 (accessed on 10 June 2018).
- Stahle, D.W.; Cleaveland, M.K. NOAA/WDS Paleoclimatology–Stahle–Choctawhatchee River–TADI–ITRDB FL001. NOAA Natl. Cent. Environ. Inf. 2002. [Google Scholar] [CrossRef]
- Winsberg, M.D. Florida Weather; University of Central Florida Press: Orlando, FL, USA, 1990; p. 177. [Google Scholar]
- USDA Soil Survey of Walton County, Florida; U.S. Department of Agriculture, Natural Resources Conservation Service: Washington, DC, USA, 1989.
- Speer, J.H. Fundamentals of Tree-Ring Research; The University of Arizona Press: Tucson, AZ, USA, 2010. [Google Scholar]
- Stokes, M.A.; Smiley, T.L. An Introduction to Tree Ring Dating; University of Arizona Press: Tucson, AZ, USA, 1996. [Google Scholar]
- Stahle, D.W.; Diaz, J.V.; Cleaveland, M.K.; Therrell, M.D.; Paull, G.J.; Burns, B.T.; Salinas, W.; Suzan, H.; Fule, P.Z. Recent tree-ring research in Mexico. In Dendrochronología en America Latina; Roig, F.A., Ed.; EDIUNC: Mendoza, Argentina, 2000; pp. 285–306. [Google Scholar]
- Stahle, D.W.; Cleaveland, M.K.; Grissino-Mayer, H.; Griffin, R.D.; Fye, F.K.; Therrell, M.D.; Burnette, D.J.; Meko, D.M.; Villanueva Diaz, J. Cool and warm season precipitation reconstructions over western New Mexico. J. Clim. 2009, 22, e3729–e3750. [Google Scholar] [CrossRef]
- Holmes, R.L. Computer-assisted quality control in tree-ring dating and measurement. Tree-Ring Bull. 1983, 44, 69–78. [Google Scholar]
- Bunn, A.G. A dendrochronology program library in R (dplR). Dendrochronologia 2008, 26, 115–124. [Google Scholar] [CrossRef]
- R Development Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2009; ISBN 3-900051-07-0. Available online: http://www.R-project.org (accessed on 5 January 2020).
- Cook, E.R.; Kairiukstis, L.A. (Eds.) Methods of Dendrochronology: Applications in the Environmental Sciences; Springer Science & Business Media: New York, NY, USA, 2013. [Google Scholar]
- Meko, D.M.; Baisan, C.H. Pilot study of latewood-width of conifers as an indicator of variability of summer rainfall in the North American Monsoon region. Int. J. Climatol. 2001, 21, 697–708. [Google Scholar] [CrossRef]
- Battipaglia, G.; De Micco, V.; Brand, W.A.; Linke, P.; Aronne, G.; Saurer, M.; Cherubini, P. Variations of vessel diameter and δ13C in false rings of Arbutus unedo L. reflect different environmental conditions. New Phytol. 2010, 188, 1099–1112. [Google Scholar] [CrossRef]
- Schroder, J. Dendrogeomorphological analysis of mass movement on Table Cliffs Plateau, Utah. Quat. Res. 1978, 9, 168–185. [Google Scholar] [CrossRef]
- Rao, M.P.; Cook, E.R.; Cook, B.I.; Anchukaitis, K.J.; D’Arrigo, R.D.; Krusic, P.J.; LeGrande, A.N. A double bootstrap approach to Superposed Epoch Analysis to evaluate response uncertainty. Dendrochronologia 2019, 55, 119–124. [Google Scholar] [CrossRef]
- Knapp, K.R.; Kruk, M.C.; Levinson, D.H.; Diamond, H.J.; Neumann, C.J. The International Best Track Archive for Climate Stewardship (IBTrACS): Unifying tropical cyclone best track data. Bull. Am. Meteorol. Soc. 2010, 91, 363–376. [Google Scholar] [CrossRef]
- Knapp, K.R.; Diamond, H.J.; Kossin, J.P.; Kruk, M.C.; Schreck, C.J. International Best Track Archive for Climate Stewardship (IBTrACS) Project, Version 4; NOAA National Centers for Environmental Information. Available online: https//doi.org/10.25921/82ty-9e16 (accessed on 13 February 2020).
- Landsea, C.W.; Franklin, J.L. Atlantic Hurricane Database Uncertainty and Presentation of a New Database Format. Mon. Weather Rev. 2013, 141, 3576–3592. [Google Scholar] [CrossRef]
- Roth, D. Tropical Cyclone Rainfall Data. HPC 2018. Available online: https://www.wpc.ncep.noaa.gov/tropical/rain/tcrainfall.html (accessed on 5 January 2020).
- Schoner, R.W.; Molansky, S. Rainfall Associated with Hurricanes; National Hurricane Research Project. Report Num. 3; NOAA/NHRL: Coral Gables, FL, USA, 1956; p. 305.
- Stahle, D.W. The Tree-Ring Record of False Spring in the Southcentral USA. Ph.D. Dissertation, Arizona State University, Tempe, AZ, USA, 1990. [Google Scholar]
- Davidson, G.R.; Laine, B.C.; Galicki, S.J.; Threlkeld, S.T. Root-Zone Hydrology: Why Bald Cypress in Flooded Wetlands Grow More When it Rains. Tree-Ring Res. 2006, 62, 3–12. [Google Scholar] [CrossRef]
- NOAA. Weekly Climate Bulletin; No. 89/26; National Oceanic and Atmospheric Administration, National Weather Service, National Meteorological Center: Washington, DC, USA, 1989; p. 2.
- Knapp, P.A.; Maxwell, J.T.; Soulé, P.T. Tropical cyclone rainfall variability in coastal North Carolina derived from longleaf pine (Pinus palustris Mill.): AD 1771–2014. Clim. Chang. 2016, 135, 311–323. [Google Scholar] [CrossRef]
- Trouet, V.; Harley, G.L.; Domínguez-Delmás, M. Shipwreck rates reveal Caribbean tropical cyclone response to past radiative forcing. Proc. Natl. Acad. Sci. USA 2016, 113, 3169–3174. [Google Scholar] [CrossRef] [PubMed]
- Tucker, C.S.; Trepanier, J.C.; Harley, G.L.; DeLong, K.L. Recording tropical cyclone activity from 1909 to 2014 along the northern Gulf of Mexico using maritime slash pine trees (Pinus elliottii var. elliottii Engelm.). J. Coast. Res. 2018, 34, 328–340. [Google Scholar] [CrossRef]
- Novak, K.; Saz-Sanchez, M.A.; Cufar, K.; Raventos, J.; de Luis, M. Age, climate and intra-annual density fluctuations in Pinus halepensis in Spain. IAWA J. 2013, 34, 459–474. [Google Scholar] [CrossRef]
- De Micco, V.; Battipaglia, G.; Cherubini, P.; Aronne, G. Comparing methods to analyse anatomical features of tree rings with and without Intra-Annual-Density-Fluctuations (IADFs). Dendrochronologia 2014, 32, 1–6. [Google Scholar] [CrossRef]
- Vose, R.S.; Applequist, S.; Squires, M.; Durre, I.; Menne, M.J.; Williams, C.N., Jr.; Fenimore, C.; Gleason, K.; Arndt, D. Improved Historical Temperature and Precipitation Time Series for U.S. Climate Divisions. J. Appl. Meteor. Climatol. 2014, 53, 1232–1251. [Google Scholar] [CrossRef]

| EPS | |||||||
|---|---|---|---|---|---|---|---|
| TRW | 28 | 14.6 | 1.68 | 0.68 | 0.41 | 0.47 | 0.93 |
| EW | 28 | 14.6 | 1.69 | 0.68 | 0.41 | 0.47 | 0.93 |
| LW | 28 | 14.6 | 1.69 | 0.35 | 0.14 | 0.19 | 0.77 |
| Year | FR% | Type | EW | LW | TRW | TC 50 NM | TC Rainfall Data |
|---|---|---|---|---|---|---|---|
| 1989 | 65 | M | 1.707 | 0.917 | 1.700 | TS “Allison” 6/24 | |
| 1994 | 48 | M | 1.420 | 1.046 | 1.407 | TS “Alberto” 7/03, TS “Beryl” 8/16 | TS “Alberto” 7/03, TS “Beryl” 8/16 |
| 1961 | 48 | L | 1.505 | 1.156 | 1.496 | H “Carla” 9/9–15 | |
| 1999 | 40 | M | 0.932 | 1.042 | 0.950 | No TC rainfall | |
| 2003 | 41 | L | 2.019 | 1.130 | 1.966 | TS “Bill” 6/27–7/03, TD “Seven” 7/25 | |
| 1971 | 36 | L | 1.464 | 1.227 | 1.455 | TD “2a” 7/5, TD “Eleven” 8/29, H “Fern” 9/1 | |
| 1965 | 32 | L | 1.128 | 1.11 | 1.139 | TS “Unnamed” 6/11 | TS “Unnamed” 6/11 |
| 1946 | 32 | L | 1.503 | 1.112 | 1.481 | No TC rainfall | |
| 1983 | 31 | L | 1.142 | 1.013 | 1.140 | No TC rainfall | |
| 1987 | 31 | M | 0.643 | 1.040 | 0.676 | TS “Unnamed” 8/09 | |
| 2013 | 31 | L | 0.723 | 0.864 | 0.736 | TS “Andrea” 6/05 | |
| 2005 | 30 | L | 1.425 | 1.293 | 1.399 | TS “Arlene” 6/10, H “Cindy” 7/03, H “Dennis” 7/08, H “Katrina” 8/24 | |
| 1958 | 29 | L | 0.898 | 1.013 | 0.918 | No TC rainfall | |
| 1975 | 23 | L | 1.295 | 1.123 | 1.300 | H “Eloise” 9/13 | TD “4” 7/27, H “Eloise” 9/13 |
| 1991 | 22 | L | 1.841 | 1.087 | 1.808 | No TC rainfall | |
| 2004 | 22 | M | 0.893 | 1.013 | 0.911 | H “Frances” 9/07 | H “Ivan” 9/26 |
| 1955 | 22 | M | 0.306 | 0.735 | 0.329 | TD “Brenda” 8/1 | |
| 1953 | 21 | L | 1.003 | 0.974 | 1.016 | TS “Alice” 6/07, H “Florence” 9/26 | TS “Alice” 6/07, H “Florence” 9/26 |
| 1964 | 20 | M | 1.084 | 0.959 | 1.085 | H “Dora” 9/11 | TS “Unnamed” 6/4, H “Dora” 9/11 |
| 1972 | 20 | L | 0.763 | 1.064 | 0.781 | H “Agnes” 6/20 | H “Agnes” 6/20 |
| 0.958 | 0.945 | 0.960 | |||||
| 0.408 | 0.203 | 0.396 |
| Variance Explained, R2 | ||||
|---|---|---|---|---|
| June Q | July Q | August Q | Total JJA Q | |
| TRW | 61 | 30 | 19 | 43 |
| TRW + FRb | 61 | 43 | 22 | 49 |
| Improvement (%) | 0 | 43 | 16 | 14 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Therrell, M.D.; Elliott, E.A.; Meko, M.D.; Bregy, J.C.; Tucker, C.S.; Harley, G.L.; Maxwell, J.T.; Tootle, G.A. Streamflow Variability Indicated by False Rings in Bald Cypress (Taxodium distichum (L.) Rich.). Forests 2020, 11, 1100. https://doi.org/10.3390/f11101100
Therrell MD, Elliott EA, Meko MD, Bregy JC, Tucker CS, Harley GL, Maxwell JT, Tootle GA. Streamflow Variability Indicated by False Rings in Bald Cypress (Taxodium distichum (L.) Rich.). Forests. 2020; 11(10):1100. https://doi.org/10.3390/f11101100
Chicago/Turabian StyleTherrell, Matthew D., Emily A. Elliott, Matthew D. Meko, Joshua C. Bregy, Clay S. Tucker, Grant L. Harley, Justin T. Maxwell, and Glenn A. Tootle. 2020. "Streamflow Variability Indicated by False Rings in Bald Cypress (Taxodium distichum (L.) Rich.)" Forests 11, no. 10: 1100. https://doi.org/10.3390/f11101100
APA StyleTherrell, M. D., Elliott, E. A., Meko, M. D., Bregy, J. C., Tucker, C. S., Harley, G. L., Maxwell, J. T., & Tootle, G. A. (2020). Streamflow Variability Indicated by False Rings in Bald Cypress (Taxodium distichum (L.) Rich.). Forests, 11(10), 1100. https://doi.org/10.3390/f11101100






