Generalized Nonlinear Mixed-Effects Individual Tree Diameter Increment Models for Beech Forests in Slovakia
Abstract
1. Introduction
2. Materials and Methods
2.1. Data Materials
2.2. Data Analysis
2.2.1. Tree and Stand Variables
2.2.2. Tree Diameter Growth: A Theoretical Context and Modelling Approach
2.2.3. Extension of Chapman-Richards Function
2.2.4. Model Estimation and Evaluation
2.2.5. Calibrated Response or Localized Diameter Increment Prediction
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Garcia, O. The state-space approach in growth modeling. Can. J. For. Res. 1994, 24, 1894–1903. [Google Scholar] [CrossRef]
- Amaro, A.; Reed, D.; Soares, P. Modelling forest systems; CABI Publishing: Wallingford, Oxon, UK, 2003; p. 432. [Google Scholar]
- Vanclay, J.K. Modelling forest growth and yield: Applications to mixed tropical forests; CAB International: Wallingford, Oxon, UK, 1994; p. 312. [Google Scholar]
- Pretzsch, H. Forest dynamics, growth and yield: from measurement to model; Springer Verlag: Berlin/Heidelberg, Germany, 2009; p. 664. [Google Scholar]
- Weiskittel, A.R.; Hann, D.W.; Kershaw, J.A.; Vanclay, J.K. Forest growth and yield modeling; Wiley: New York, NY, USA, 2011; p. 424. [Google Scholar]
- Hasenauer, H.E. Sustainable forest management: growth models for Europe; Springer-Verlag: Berlin/Heidelberg, Germany, 2006; p. 388. [Google Scholar]
- Adame, P.; Hynynen, J.; Cañellas, I.; del Río, M. Individual-tree diameter growth model for rebollo oak (Quercus pyrenaica Willd.) coppices. For. Ecol. Manag. 2008, 255, 1011–1022. [Google Scholar] [CrossRef]
- Crecente-Campo, F.; Soares, P.; Tomé, M.; Dieguez-Aranda, U. Modelling annual individual-tree growth and mortality of Scots pine with data obtained at irregular measurement intervals and containing missing observations. For. Ecol. Manag. 2010, 260, 1965–1974. [Google Scholar] [CrossRef]
- Subedi, N.; Sharma, M. Individual-tree diameter growth models for black spruce and jack pine plantations in northern Ontario. For. Ecol. Manag. 2011, 261, 2140–2148. [Google Scholar] [CrossRef]
- Vospernik, S. Possibilities and limitations of individual-tree growth models—A review on model evaluations. Die Bodenkultur: J. Land Manag., Food Environ. 2017, 68, 103–112. [Google Scholar] [CrossRef][Green Version]
- Sánchez-González, M.; del Río, M.; Canellas, I.; Montero, G. Distance independent tree diameter growth model for cork oak stands. For. Ecol. Manag. 2006, 225, 262–270. [Google Scholar] [CrossRef]
- Hasenauer, H. Concepts within tree growth modeling. In Sustainable forest management: Growth models for Europe; Hasenauer, H., Ed.; Springer Verlag: Berlin/Heidelberg, Germany, 2006; p. 398. [Google Scholar]
- Condés, S.; Sterba, H. Comparing an individual tree growth model for Pinus halepensis Mill. in the Spanish region of Murcia with yield tables gained from the same area. Eur. J. For. Res. 2008, 127, 253–261. [Google Scholar] [CrossRef]
- Sterba, H.; Monserud, R.A. Applicability of the forest stand growth simulator PROGNAUS for the Austrian part of the Bohemian Massif. Ecol. Model. 1997, 98, 23–34. [Google Scholar] [CrossRef]
- Hasenauer, H.; Kindermann, G.; Steinmetz, P. The tree growth model MOSES 3.0. In Sustaianble forest management, Growth models for Europe; Hasenauer, H., Ed.; Springer Verlag: Berlin/Heidelberg, Germany, 2006; p. 388. [Google Scholar]
- Pinheiro, J.C.; Bates, D.M. Mixed-effects models in S and S-PLUS; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Saud, P.; Lynch, T.B.; Anup, K.C.; Guldin, J.M. Using quadratic mean diameter and relative spacing index to enhance height-diameter and crown ratio models fitted to longitudinal data. Forestry 2016, 89, 215–229. [Google Scholar] [CrossRef]
- Fu, L.; Sharma, R.P.; Hao, K.; Tang, S. A generalized interregional nonlinear mixed-effects crown width model for Prince Rupprecht larch in northern China. For. Ecol. Manag. 2017, 389, 364–373. [Google Scholar] [CrossRef]
- Hall, D.B.; Bailey, R.L. Modeling and prediction of forest growth variables based on multilevel nonlinear mixed models. For. Sci. 2001, 47, 311–321. [Google Scholar]
- Fu, L.; Sun, H.; Sharma, R.P.; Lei, Y.; Zhang, H.; Tang, S. Nonlinear mixed-effects crown width models for individual trees of Chinese fir (Cunninghamia lanceolata) in south-central China. For. Ecol. Manag. 2013, 302, 210–220. [Google Scholar] [CrossRef]
- Sharma, R.P.; Breidenbach, J. Modeling height-diameter relationships for Norway spruce, Scots pine, and downy birch using Norwegian national forest inventory data. For. Sci. Technol. 2015, 11, 44–53. [Google Scholar] [CrossRef]
- Sharma, R.P.; Vacek, Z.; Vacek, S.; Podrázský, V.; Jansa, V. Modelling individual tree height to crown base of Norway spruce (Picea abies (L.) Karst.) and European beech (Fagus sylvatica L.). PLoS ONE 2017, 12, e0186394. [Google Scholar] [CrossRef]
- Sharma, R.P.; Vacek, Z.; Vacek, S. Individual tree crown width models for Norway spruce and European beech in Czech Republic. For. Ecol. Manag. 2016, 366, 208–220. [Google Scholar] [CrossRef]
- Bosela, M.; Štefančík, I.; Petráš, R.; Vacek, S. The effects of climate warming on the growth of European beech forests depend critically on thinning strategy and site productivity. Agric. For. Meteorol. 2016, 222, 21–31. [Google Scholar] [CrossRef]
- Geßler, A.; Keitel, C.; Kreuzwieser, J.; Matyssek, R.; Seiler, W.; Rennenberg, H. Potential risks for European beech (Fagus sylvatica L.) in a changing climate. Trees 2007, 21, 1–11. [Google Scholar] [CrossRef]
- Knoke, T.; Ammer, C.; Stimm, B.; Mosandl, R. Admixing broadleaved to coniferous tree species: a review on yield, ecological stability and economics. Eur. J. For. Res. 2008, 127, 89–101. [Google Scholar] [CrossRef]
- Barna, M.; Ján, K.; Bublinec, E. Beech and Beech Ekosystems of Slovakia; VEDA: Bratislava, Slovak Republic, 2011; p. 634. [Google Scholar]
- Green report. Správa o lesnom hospodárstve v Slovenskej republike za rok 2016. Bratislava MPRV SR: 68. , 2017. Available online: https://www.enviroportal.sk/environmentalne-temy/vplyvy-na-zp/lesnictvo/dokumenty/spravy-o-lesnom-hospodarstve-v-slovenskej-republike (accessed on 23 May 2019).
- Vacek, Z.; Vacek, S.; Bílek, L.; Remeš, J.; Štefančík, I. Changes in horizontal structure of natural beech forests on an altitudinal gradient in the Sudetes. Dendrobiology 2015, 73, 33–45. [Google Scholar] [CrossRef]
- Petritan, A.M.; Von Lüpke, B.; Petritan, I.C. Effects of shade on growth and mortality of maple (Acer pseudoplatanus), ash (Fraxinus excelsior) and beech (Fagus sylvatica) saplings. Forestry 2007, 80, 397–412. [Google Scholar] [CrossRef]
- Bolte, A.; Hilbrig, L.; Grundmann, B.; Kampf, F.; Brunet, J.; Roloff, A. Climate change impacts on stand structure and competitive interactions in a southern Swedish spruce–beech forest. Eur. J. For. Res. 2010, 12, 261–276. [Google Scholar] [CrossRef]
- Bosela, M.; Tobin, B.; Šebeň, V.; Petráš, R.; Larocque, G.R. Different mixtures of Norway spruce, silver fir, and European beech modify competitive interactions in central European mature mixed forests. Can. J. For. Res. 2015, 45, 1577–1586. [Google Scholar] [CrossRef]
- Pretzsch, H.; del Rio, M.; Schütze, G.; Ammer, C.; Annighöfer, P.; Avdagic, A.; Barbeito, I.; Bielak, K.; Brazaitis, G.; Coll, L.; et al. Mixing of Scots pine (Pinus sylvestris L.) and European beech (Fagus sylvatica L.) enhances structural heterogeneity, and the effect increases with water availability. For. Ecol. Manag. 2016, 373, 149–166. [Google Scholar] [CrossRef]
- Hanewinkel, M.; Cullmann, D.A.; Schelhaas, M.J.; Nabuurs, G.J.; Zimmermann, N.E. Climate change may cause severe loss in the economic value of European forest land. Nat. Clim. Chang. 2013, 3, 203. [Google Scholar] [CrossRef]
- Boncina, A.; Kadunc, A.; Robic, D. Effects of selective thinning on growth and development of beech (Fagus sylvatica L.) forest stands in south-eastern Slovenia. Ann. For. Sci. 2007, 64, 47–57. [Google Scholar] [CrossRef]
- Štefančík, I.; Vacek, Z.; Sharma, R.P.; Vacek, S.; Rösslová, M. Effect of thinning regimes on development and growth of crop trees in Fagus sylvatica stands of Central Europe over 50 years. Dendrobiology 2018, 79, 141–155. [Google Scholar] [CrossRef]
- Štefančík, I. Rast, štruktúra a produkcia bukových porastov srozdielnym režimom výchovy (Vedecká monografia); NLC: Zvolen, 2015; p. 148. Available online: http://sclib.svkk.sk/sck01/Record/000499076 (accessed on 23 May 2019).
- Sharma, R.P.; Brunner, A.; Eid, T.; Øyen, B.-H. Modelling dominant height growth from national forest inventory individual tree data with short time series and large age errors. For. Ecol. Manag. 2011, 262, 2162–2175. [Google Scholar] [CrossRef]
- Hossfeld, J.W. Mathematik für Forstmänner, Őkonomen und Cameralisten; Nabu Press: Gotha, Germany, 1822; p. 310. [Google Scholar]
- Cieszewski, C.J. Comparing fixed- and variable-base-age site equations having single versus multiple asymptotes. For. Sci. 2002, 48, 7–23. [Google Scholar]
- Zhao, D.; Kane, M.; Borders, B.E. Crown ratio and relative spacing relationships for loblolly pine plantations. Open J. For. 2012, 2, 101–115. [Google Scholar] [CrossRef]
- Sharma, R.P.; Bíllek, L.; Vacek, Z.; Vacek, S. Modelling crown width-diameter relationship for Scots pine in the central Europe. Trees 2017, 31, 1875–1889. [Google Scholar] [CrossRef]
- Fonseca, T.F.; Duarte, J.C. A silvicultural stand density model to control understory in maritime pine stands. iForest 2017, 10, 829–836. [Google Scholar] [CrossRef]
- Schelhaas, M.-J.; Hengeveld, G.M.; Heidema, N.; Thürig, E.; Rohner, B.; Vacchiano, G.; Vayreda, J.; Redmond, J.; Socha, J.; Fridman, J.; et al. Species-specific, pan-European diameter increment models based on data of 2.3 million trees. For. Ecosyst. 2018, 5, 21. [Google Scholar] [CrossRef]
- West, P.W. Tree and forest measurement; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Zeide, B. Analysis of growth equations. For. Sci. 1993, 39, 594–616. [Google Scholar] [CrossRef]
- Zeide, B. Accuracy of equations describing diameter growth. Can. J. For. Res. 1989, 19, 1283–1286. [Google Scholar] [CrossRef]
- Gyawali, A.; Sharma, R.P.; Bhandari, S.K. Individual tree basal area growth models for Chir pine (Pinus roxberghii Sarg.) in western Nepal. J. For. Sci. 2015, 61, 535–543. [Google Scholar] [CrossRef]
- Tomé, J.; Tomé, M.; Barreiro, S.; Paulo, J.A. Age-independent difference equations for modelling tree and stand growth. Can. J. For. Res. 2006, 36, 1621–1630. [Google Scholar] [CrossRef]
- Richards, F.J. A flexible growth function for empirical use. J. Exp. Bot. 1959, 10, 290–300. [Google Scholar] [CrossRef]
- Chapman, D.G. Statistical Problems in Dynamics of Exploited Fisheries Populations. Proceedings of the Fourth Berkeley Symposium on Mathematical Statistics and Probability; Neyman, J., Ed.; University of California Press: Berkeley, CA, USA, 1961; pp. 153–168. [Google Scholar]
- Bertalanffy, L. Quantitative laws in metabolism and growth. Quart. Rev. Biol. 1957, 32, 217–231. [Google Scholar] [CrossRef]
- Weibull, W. A statistical distribution function of wide applicability. J. Appl. Mech. 1951, 18, 293–296. [Google Scholar]
- Gompertz, B. On the nature of the function expressive of the law of human mortality and on a new model of determining life contingencies. Phil. Trans. R. Soc. 1825, 115, 513–585. [Google Scholar]
- Korf, V. A mathematical definition of stand volume growth law (In Czech). Lesnicka Prace 1939, 18, 337–339. [Google Scholar]
- Näslund, M. Skogsforsö ksastaltens gallringsforsök i tallskog (Thinning experiments in pine forest conducted by the forest experiment station). Medd. fran Statens Skogsforsöksanstalt 1936, 29, 1–169. [Google Scholar]
- Levakovic, A. An analytical form of growth law. Glasnik za Sumske Pokuse (In Serbo-Croat.) 1935, 4, 283–310. [Google Scholar]
- Fu, L.; Sharma, R.P.; Wang, G.; Tang, S. Modelling a system of nonlinear additive crown width models applying seemingly unrelated regression for Prince Rupprecht larch in northern China. For. Ecol. Manag. 2017, 386, 71–80. [Google Scholar] [CrossRef]
- Sharma, R.P.; Vacek, Z.; Vacek, S. Generalized nonlinear mixed-effects individual tree crown ratio models for Norway spruce and European beech. Forests 2018, 9, 555. [Google Scholar] [CrossRef]
- Vonesh, E.F.; Chinchilli, V.M. Linear and nonlinear models for the analysis of repeated measurements; Marcel Dekker: New York, NY, USA, 1997. [Google Scholar]
- SAS Institute Inc. SAS/ETS1 9.1.3 User’s Guide; SAS Institute Inc.: Cary, NC, USA, 2012. [Google Scholar]
- Littell, R.C.; Milliken, G.A.; Stroup, W.W.; Wolfinger, R.D.; Schabenberger, O. SAS for mixed models, 2nd ed.; SAS Institute: Cary, NC, USA, 2006; p. 814. [Google Scholar]
- Nakagawa, S.; Schielzeth, H. A general and simple method for obtaining R2 from generalized linear mixed-effects models. Methods Ecol. Evol. 2013, 4, 133–142. [Google Scholar] [CrossRef]
- Akaike, H. A new look at statistical model identification. IEEE Trans. Autom. Control 1974, 19, 716–723. [Google Scholar] [CrossRef]
- Calama, R.; Montero, G. Interregional nonlinear height-diameter model with random coefficients for stone pine in Spain. Can. J. For. Res. 2004, 34, 150–163. [Google Scholar] [CrossRef]
- Crecente-Campo, F.; Tomé, M.; Soares, P.; Dieguez-Aranda, U. A generalized nonlinear mixed-effects height-diameter model for Eucalyptus globulus L. in northwestern Spain. For. Ecol. Manag. 2010, 259, 943–952. [Google Scholar] [CrossRef]
- Sharma, R.P.; Vacek, Z.; Vacek, S.; Kučera, M. Modelling individual tree height-diameter relationships for multi-layered and multi-species forests in central Europe. Trees 2018, 33, 103–119. [Google Scholar] [CrossRef]
- Carmean, W.H.; Lenthall, D.J. Height growth and site index curves ofr jack pine in north central Ontario. Can. J. For. Res. 1989, 19, 215–224. [Google Scholar] [CrossRef]
- Goelz, J.C.G.; Burk, T.E. Development of a well-behaved site index equation-Jack pine in North central Ontario. Can. J. For. Res. 1992, 22, 776–784. [Google Scholar] [CrossRef]
- Huang, S.; Titus, S.J. An age-independent individual tree height prediction model for boreal spruce-aspen stands in Alberta. Can. J. For. Res. 1994, 24, 1295–1301. [Google Scholar] [CrossRef]
- Burkhart, H.E.; Tomé, M. Modeling forest trees and stands; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Fang, Z.X.; Bailey, R.L. Height-diameter models for tropical forests on Hainan Island in southern China. For. Ecol. Manag. 1998, 110, 315–327. [Google Scholar] [CrossRef]
- Sharma, M.; Parton, J. Height-diameter equations for boreal tree species in Ontario using a mixed-effects modeling approach. For. Ecol. Manag. 2007, 249, 187–198. [Google Scholar] [CrossRef]
- Monserud, R.A.; Sterba, H. A basal area increment model for individual trees growing in even- and uneven-aged forest stands in Austria. For. Ecol. Manag. 1996, 80, 57–80. [Google Scholar] [CrossRef]
- Cienciala, E.; Russ, R.; Santruckova, H.; Altman, J.; Kopacek, J.; Hůnová, I.; Štěpánek, P.; Oulehle, F.; Tumajer, J.; Ståhl, G.R. Discerning environmental factors affecting current tree growth in Central Europe. Sci. Total Environ. 2016, 573, 541–554. [Google Scholar] [CrossRef]
- Zhao, L.; Li, C.; Tang, S. Individual-tree diameter growth model for fir plantations based on multi-level linear mixed effects models across southeast China. J. For. Res. 2013, 18, 305–315. [Google Scholar] [CrossRef]
- Dormann, C.F.; Elith, J.; Bacher, S.; Buchmann, C.; Carl, G.; Carre, G.; Marquez, J.R.G.; Gruber, B.; Lafourcade, B.; Leitao, P.J. Collinearity: A review of methods to deal with it and a simulation study evaluating their performance. Ecography 2013, 36, 27–46. [Google Scholar] [CrossRef]
- Mäkinen, H.; Nöjd, P.; Isomäki, A. Radial, height and volume increment variation in Picea abies (L.) Karst. Stands with varying thinning intensities. Scand. J. For. Res. 2002, 17, 304–316. [Google Scholar] [CrossRef]
- Sharma, R.P.; Vacek, Z.; Vacek, S. Modeling individual tree height to diameter ratio for Norway spruce and European beech in Czech Republic. Trees 2016, 30, 1969–1982. [Google Scholar] [CrossRef]
- Wonn, H.T.; O’Hara, K.L. Height: Diameter ratios and stability relationships for four northern rocky mountain tree species. West. J. Appl. For. 2001, 16, 87–94. [Google Scholar]
- Kim, M.; Lee, W.-K.; Kim, Y.-S.; Lim, C.-H.; Song, C.; Park, T.; Son, Y.; Son, Y.-M. Impact of thinning intensity on the diameter and height growth of Larix kaempferi stands in central Korea. For. Sci. Technol. 2016, 12, 77–87. [Google Scholar]
- Binkley, D.; Stape, J.L.; Ryan, M.G. Thinking about efficiency of resource use in forests. For. Ecol. Manag. 2004, 193, 5–16. [Google Scholar] [CrossRef]
- Bayer, D.; Seifert, S.; Pretzsch, H. Structural crown properties of Norway spruce (Picea abies [L.] Karst.) and European beech (Fagus sylvatica [L.]) in mixed versus pure stands revealed by terrestrial laser scanning. Trees 2013, 27, 1035–1047. [Google Scholar] [CrossRef]
- Pretzsch, H. Canopy space filling and tree crown morphology in mixed-species stands compared with monocultures. For. Ecol. Manag. 2014, 327, 251–264. [Google Scholar] [CrossRef]
- Pretzsch, H.; Block, J.; Dieler, J.; Dong, P.H.; Kohnle, U.; Nagel, J.; Spellmann, H.; Zingg, A. Comparison between the productivity of pure and mixed stands of Norway spruce and European beech along an ecological gradient. Ann. For. Sci. 2010, 67, 712. [Google Scholar] [CrossRef]
- Sterba, H.; del Rio, M.; Brunner, A.; Condes, S. Effect of species proportion definition on the evaluation of growth in pure vs. mixed stands. For. Syst. 2014, 23, 547–559. [Google Scholar] [CrossRef]
- Pretzsch, H.; Forrester, D.I.; Rötzer, T. Representation of species mixing in forest growth models. A review and perspective. Ecol. Model. 2015, 313, 276–292. [Google Scholar] [CrossRef]
- Sharma, R.P.; Vacek, Z.; Vacek, S. Modelling tree crown-to-bole diameter ratio for Norway spruce and European beech. Silva Fenn. 2017, 51, 1740. [Google Scholar] [CrossRef]
- Kozak, A.; Kozak, R. Does cross validation provide additional information in the evaluation of regression models? Can. J. For. Res. 2003, 33, 976–987. [Google Scholar] [CrossRef]
- Yang, Y.Q.; Monserud, R.A.; Huang, S.M. An evaluation of diagnostic tests and their roles in validating forest biometric models. Can. J. For. Res. 2004, 34, 619–629. [Google Scholar] [CrossRef]


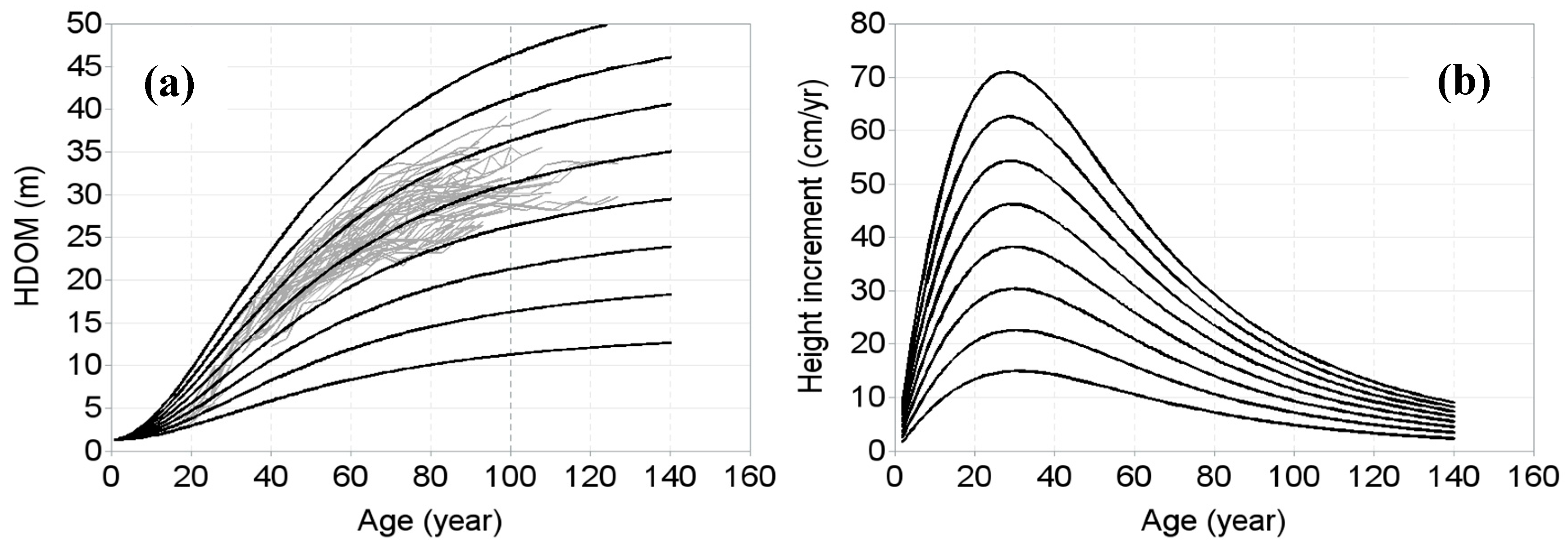
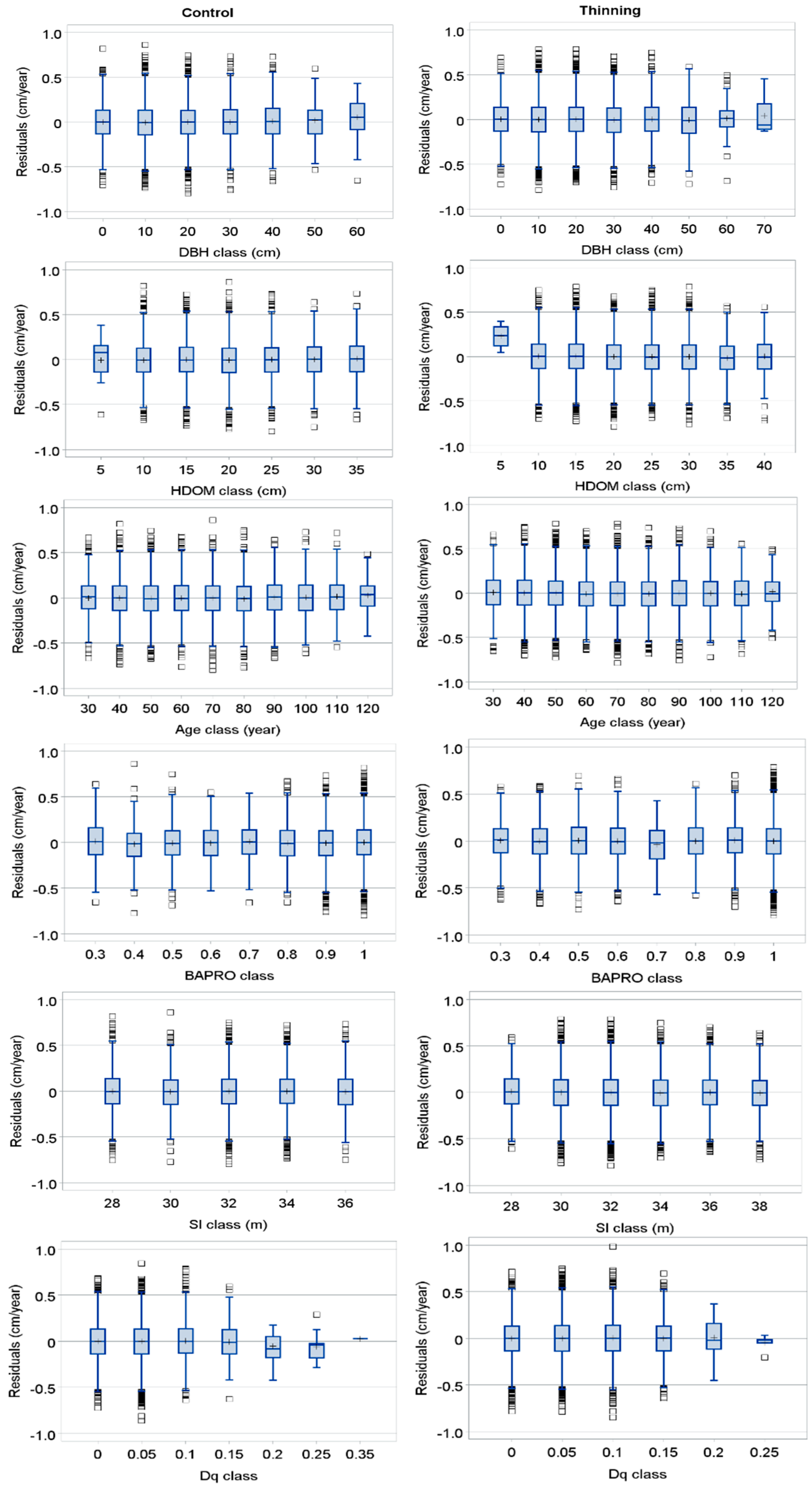

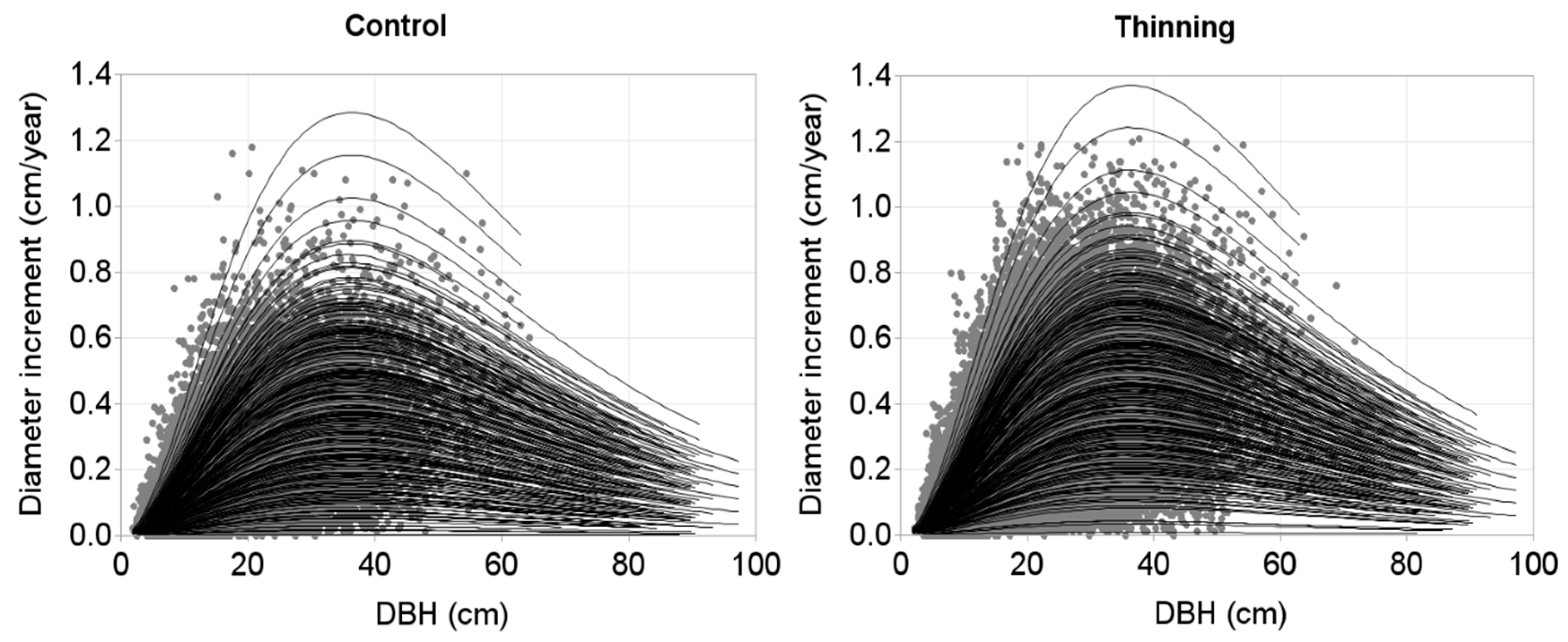
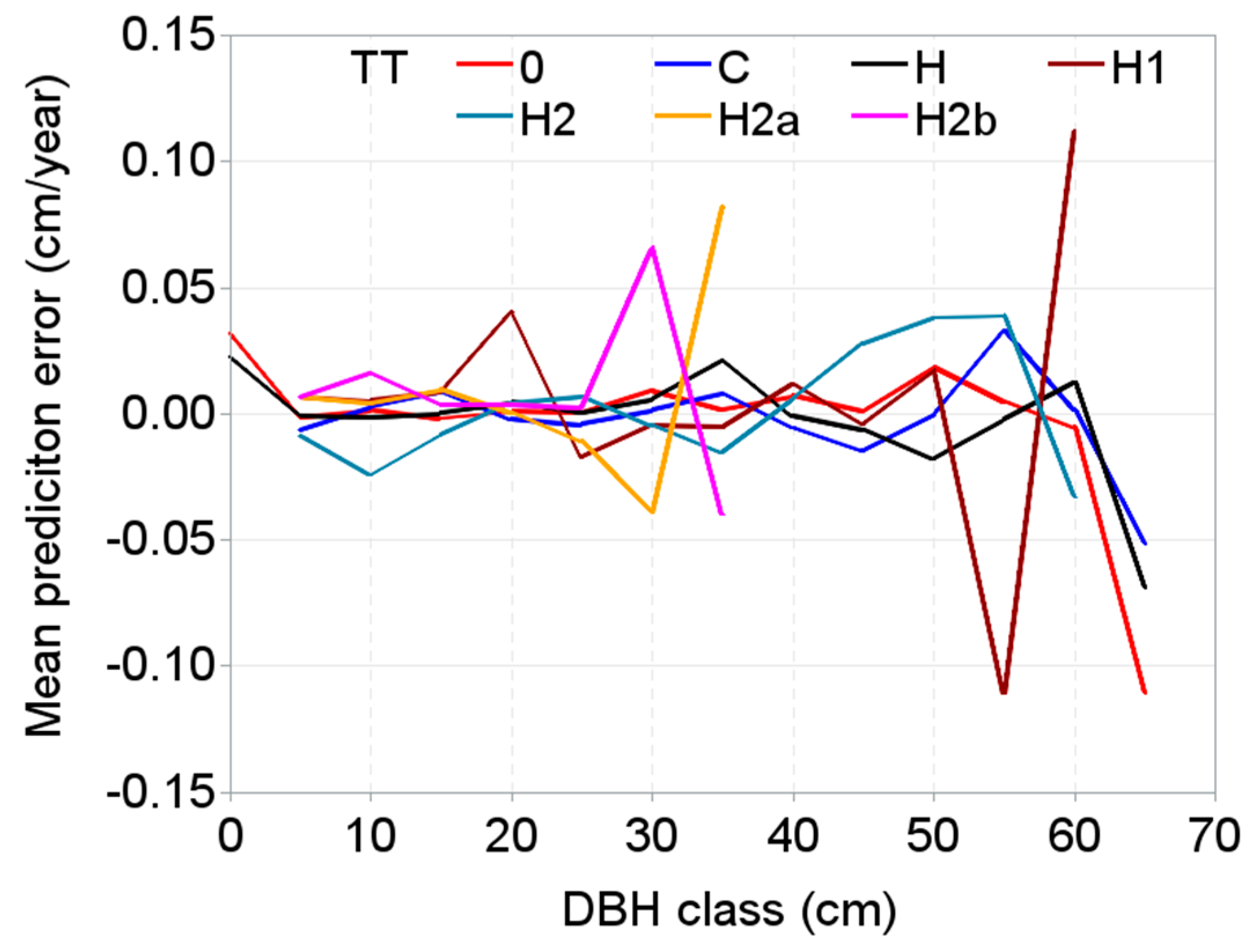
| Plot Names/Plot Number | Number of Measurements | Year of First and Last Measurements | Age Span (year) | Elevation (m a.s.l.) | Mean Annual Temperature (°C) | Mean Annual Precipitation (mm) | Soil Types |
|---|---|---|---|---|---|---|---|
| Jalna/C,H,O | 13 | 1959, 2017 | 36–94 | 610 | 6.2 | 800 | Eutric Cambisol |
| Konus/C,H,O | 12 | 1961, 2014 | 30–83 | 510 | 6.5 | 900 | Eutric Cambisol |
| Kalsa/C,H,O | 12 | 1961, 2014 | 37–90 | 520 | 6 | 790 | Stagni–Eutric Cambisol |
| Kalsa/H2 | 10 | 1969, 2014 | 45–90 | 520 | 6 | 790 | |
| Zalobin/C,H,O | 12 | 1962, 2015 | 39–92 | 250 | 7.9 | 660 | Stagni–Eutric Cambisol |
| Zalobin/H1,Hx | 8 | 1980, 2015 | 57–92 | 250 | 7.9 | 660 | |
| Zlata Idka/C,H,O | 13 | 1960, 2018 | 40–98 | 700 | 6.7 | 780 | Haplic Cambisol |
| Ciganka/C,H,H2,O | 11 | 1967, 2017 | 60–110 | 560 | 5.5 | 918 | Haplic (Dystric) Cambisol |
| Lukov/H,O | 12 | 1962, 2016 | 45–99 | 550 | 5.5 | 690 | Haplic Cambisol |
| Lukov/C | 11 | 1966, 2016 | 49–99 | 550 | 5.5 | 690 | Haplic Cambisol |
| Stagiar/I,II,III,IV | 7 | 1984, 2014 | 38–68 | 620 | 6.6 | 925 | Haplic Cambisol |
| Stara Pila/H,O | 10 | 1973, 2018 | 15–65 | 690–720 | 6.8 | 1100 | Cambisol |
| Motycky/H,O | 10 | 1972, 2017 | 41–93 | 810–870 | 5.8 | 1080 | Calcaric Cambisol |
| Korytnica/H,O | 11 | 1968, 2018 | 50–108 | 930–970 | 4.2 | 1200 | Cambisol |
| Hrable/H,O | 10 | 1969, 2014 | 74–127 | 820–840 | 6.0 | 900 | Dystric Cambisol |
| Variables | Statistics (Mean ± Standard Deviation (Range)) | |
|---|---|---|
| Control | Thinning | |
| Number of sample plots | 13 | 28 |
| Number of observations of beech | 45,982 | 85,500 |
| Number of beech sample trees | 8231 | 18,321 |
| Number of beech sample trees per plot | 561 ± 313 (74–1394) | 580 ± 364 (32–1170) |
| Number of stems per hectare (N ha−1) | 2503 ± 1441 (435–11978) | 19 ± 1322 (224–5707) |
| Stand basal area per hectare (BA, m2 ha−1) | 39.6 ± 5.8 (0.83–55.9) | 31.6 ± 4.6 (0.57–56.2) |
| BA of trees lager than a subject tree (BAL, m2 ha−1) | 30.8 ± 6.2 (0–55.4) | 33.1 ± 9.3 (0–53.2) |
| Basal area proportion of beech (BAPRO) | 0.8 ± 0.16 (0.23–1.0) | 0.88 ± 0.19 (0.25–1.0) |
| Quadratic mean DBH per plot (QMD, cm) | 15.1 ± 6.7 (2.8–37.2) | 15.5 ± 7.3 (2.3–43.9) |
| Arithmetic mean DBH per plot (AMD, cm) | 13.5 ± 6.2 (2.4–36.0) | 13.8 ± 6.9 (2.6–43.1) |
| Ratio of DBH to QMD (Dq) | 0.21 ± 0.13 (0.01–0.41) | 0.23 ± 0.11 (0.01–0.37) |
| Relative spacing index (RSI) | 0.11 ± 0.04 (0.05–1.3) | 0.13 ± 0.06 (0.03–1.3) |
| Dominant height per plot (HDOM, m) | 19.4 ± 6.3 (4.3–33.2) | 20.5 ± 6.5 (3.5–40.1) |
| HDOM at age of 100 year (site index—SI, m) | 31.8 ± 2.6 (27.6–36.2) | 32.6 ± 2.5 (28.6–38.5) |
| Dominant diameter (DDOM, cm) | 14.8 ± 6.3 (3.7–36.1) | 16.8 ± 7.7 (3.9–48.8) |
| Mean height per plot (m) | 19.4 ± 6.3 (4.3–34.9) | 20.5 ± 6.5 (3.5–40.1) |
| Stand age (year) | 62.9 ± 19.7 (26.0–119) | 62.4 ± 18.5 (26.0–119) |
| Height (m) | 20.5 ± 8.0 (2.8–41.1) | 22.2 ± 8.4 (2.9–43.7) |
| Height range per plot (m) | 20.1 ± 5.1 (3.3–34.6) | 18.6 ± 7.3 (3.1–36.6) |
| DBH range per plot (cm) | 35.5 ± 11.6 (2.8–65.7) | 33.3 ± 9.9 (3.8–62.5) |
| Diameter at breast height (DBH, cm) | 16.3 ± 9.7 (2.1–64.5) | 17.5 ± 11.1 (2.2–66.2) |
| DBH increment (cm year−1) | 0.16 ± 0.14 (0.01–1.19) | 0.22 ± 0.19 (0.01–1.21) |
| Height-to-DBH ratio (HDR, m cm−1) | 1.24 ± 0.32 (0.5–3.3) | 1.13 ± 0.31 (0.5–3.8) |
| Designation | Integral Form | Differential Form | Reference |
|---|---|---|---|
| F1 | Richards [50], Chapman [51] | ||
| F2 | , | Bertanlaffy [52] | |
| F3 | , | Korf [55] | |
| F4 | , | Weibull [53] | |
| F5 | , | Gompertz [54] | |
| F6 | , | Näslund [56] | |
| F7 | Levakovic [57] | ||
| F8 | Hossfeld II [39] |
| Function | RMSE | R2adj |
|---|---|---|
| F1 | 0.1569 | 0.4098 |
| F2 | 0.1588 | 0.4025 |
| F3 | 0.1584 | 0.4053 |
| F4 | 0.1584 | 0.4053 |
| F5 | 0.1583 | 0.4055 |
| F6 | 0.1589 | 0.4016 |
| F7 | 0.1593 | 0.3997 |
| F8 | 0.1594 | 0.3991 |
| Components | Age Independent Model (Equation (6)) | Age Dependent Model (Equation (7)) | ||
|---|---|---|---|---|
| Estimates | p-Value | Estimates | p-Value | |
| Fixed | ||||
| α1 | −0.23486 (0.0114) | 0.0001 | 31.24818 (1.6507) | 0.0001 |
| α2 | 0.929377 (0.0113) | 0.0001 | −0.73081 (0.0224) | 0.0001 |
| α3 | −0.0773 (0.0316) | 0.0145 | −1.06174 (0.0368) | 0.0001 |
| α4 | 0.165254 (0.00293) | 0.0001 | 0.065613 (0.00260) | 0.0001 |
| α5 | 18.82619 (0.3430) | 0.0001 | 13.76482 (0.3738) | 0.0001 |
| α6 | 0.634192 (0.0128) | 0.0001 | 0.650947 (0.0147) | 0.0001 |
| α7 | −0.03812 (0.000519) | 0.0001 | ||
| b2 | 0.023836 (0.000368) | 0.0001 | 0.026481 (0.000399) | 0.0001 |
| b3 | 3.468139 (0.0179) | 0.0001 | 3.192778 (0.0176) | 0.0001 |
| Variance | ||||
| σ2ui1 | 4.7254 | 6.9270 | ||
| σui1ui2 | 0.4981 | 0.8419 | ||
| σ2ui2 | 0.0877 | 0.9727 | ||
| σ2 | 0.01215 | 0.01121 | ||
| 0.3071 | 0.2964 | |||
| Fit statistics | ||||
| 0.6288 | 0.6539 | |||
| 0.6566 | 0.6796 | |||
| RMSE | 0.1196 | 0.1141 | ||
| AIC | −23,933 | −24,107 | ||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sharma, R.P.; Štefančík, I.; Vacek, Z.; Vacek, S. Generalized Nonlinear Mixed-Effects Individual Tree Diameter Increment Models for Beech Forests in Slovakia. Forests 2019, 10, 451. https://doi.org/10.3390/f10050451
Sharma RP, Štefančík I, Vacek Z, Vacek S. Generalized Nonlinear Mixed-Effects Individual Tree Diameter Increment Models for Beech Forests in Slovakia. Forests. 2019; 10(5):451. https://doi.org/10.3390/f10050451
Chicago/Turabian StyleSharma, Ram P., Igor Štefančík, Zdeněk Vacek, and Stanislav Vacek. 2019. "Generalized Nonlinear Mixed-Effects Individual Tree Diameter Increment Models for Beech Forests in Slovakia" Forests 10, no. 5: 451. https://doi.org/10.3390/f10050451
APA StyleSharma, R. P., Štefančík, I., Vacek, Z., & Vacek, S. (2019). Generalized Nonlinear Mixed-Effects Individual Tree Diameter Increment Models for Beech Forests in Slovakia. Forests, 10(5), 451. https://doi.org/10.3390/f10050451






