Noncircular Sources-Based Sparse Representation Algorithm for Direction of Arrival Estimation in MIMO Radar with Mutual Coupling
Abstract
:1. Introduction
2. Problem Formulation
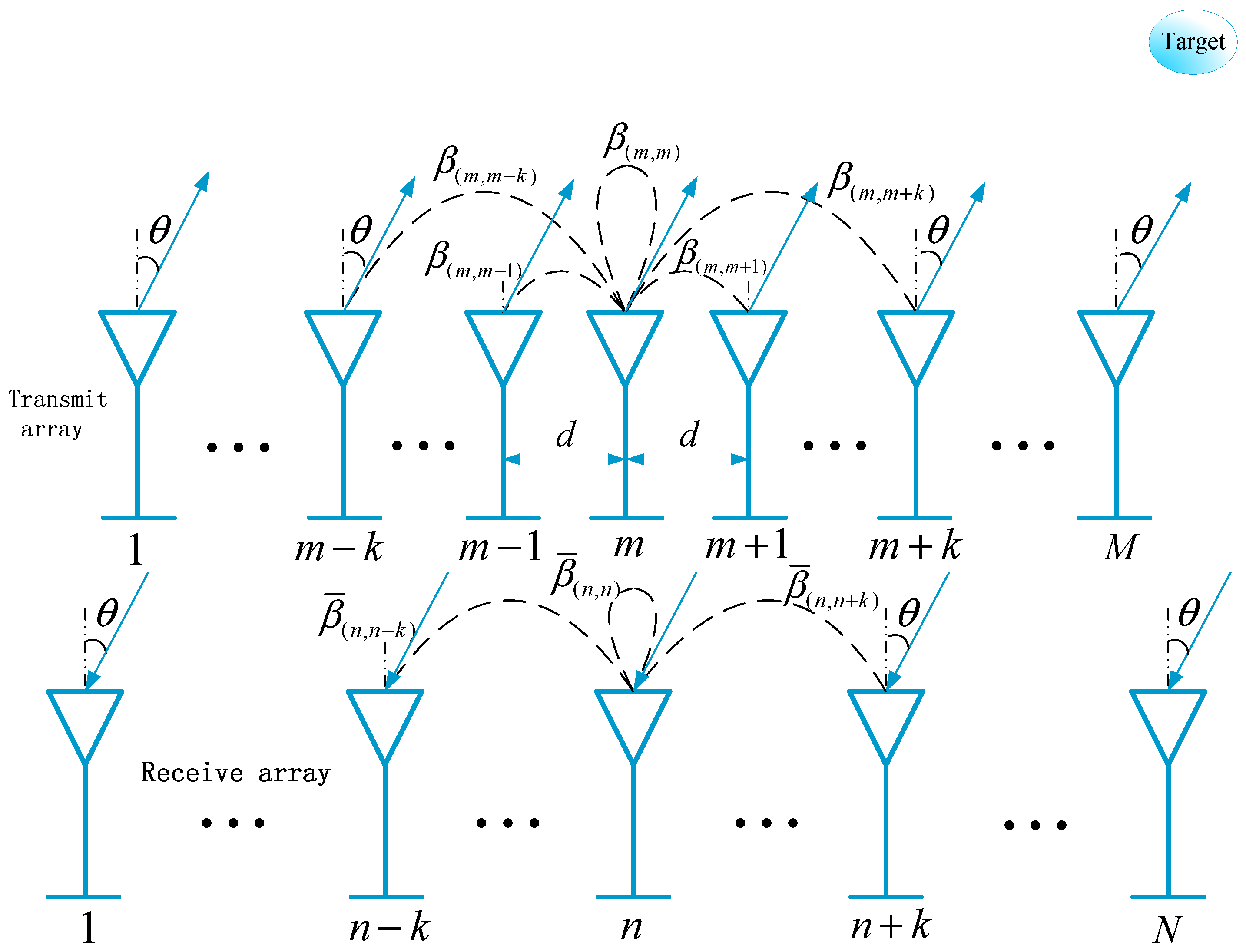
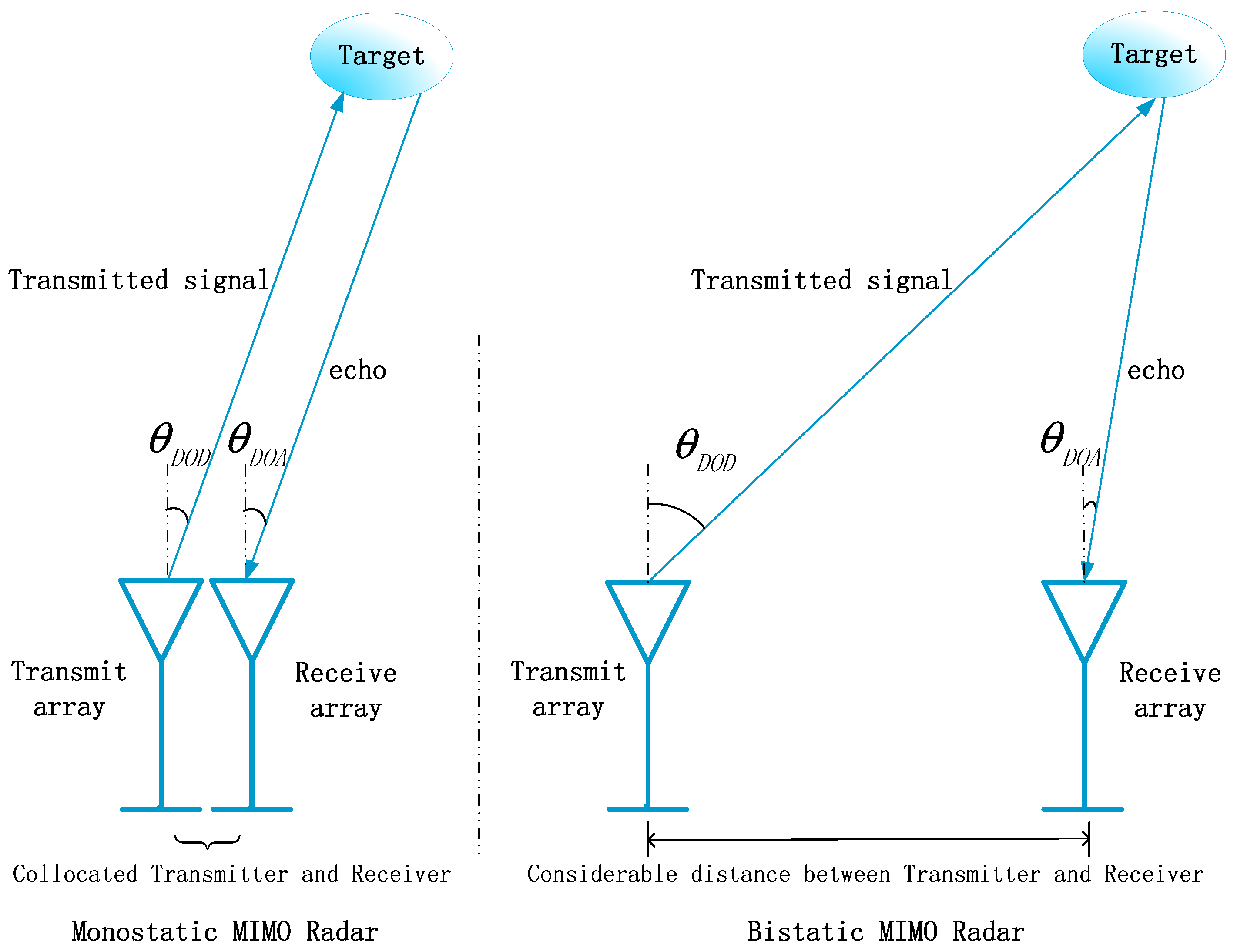
2.1. MIMO Radar System Model with Mutual Coupling
2.2. Noncircular Signals
3. The Proposed Algorithm
3.1. Mutual Coupling Elimination
3.2. Noncircular Signal-Based Extended Matrix Construction
3.3. Joint Reweighted Sparse Representation-Based DOA Estimation Scheme
4. Related Remarks
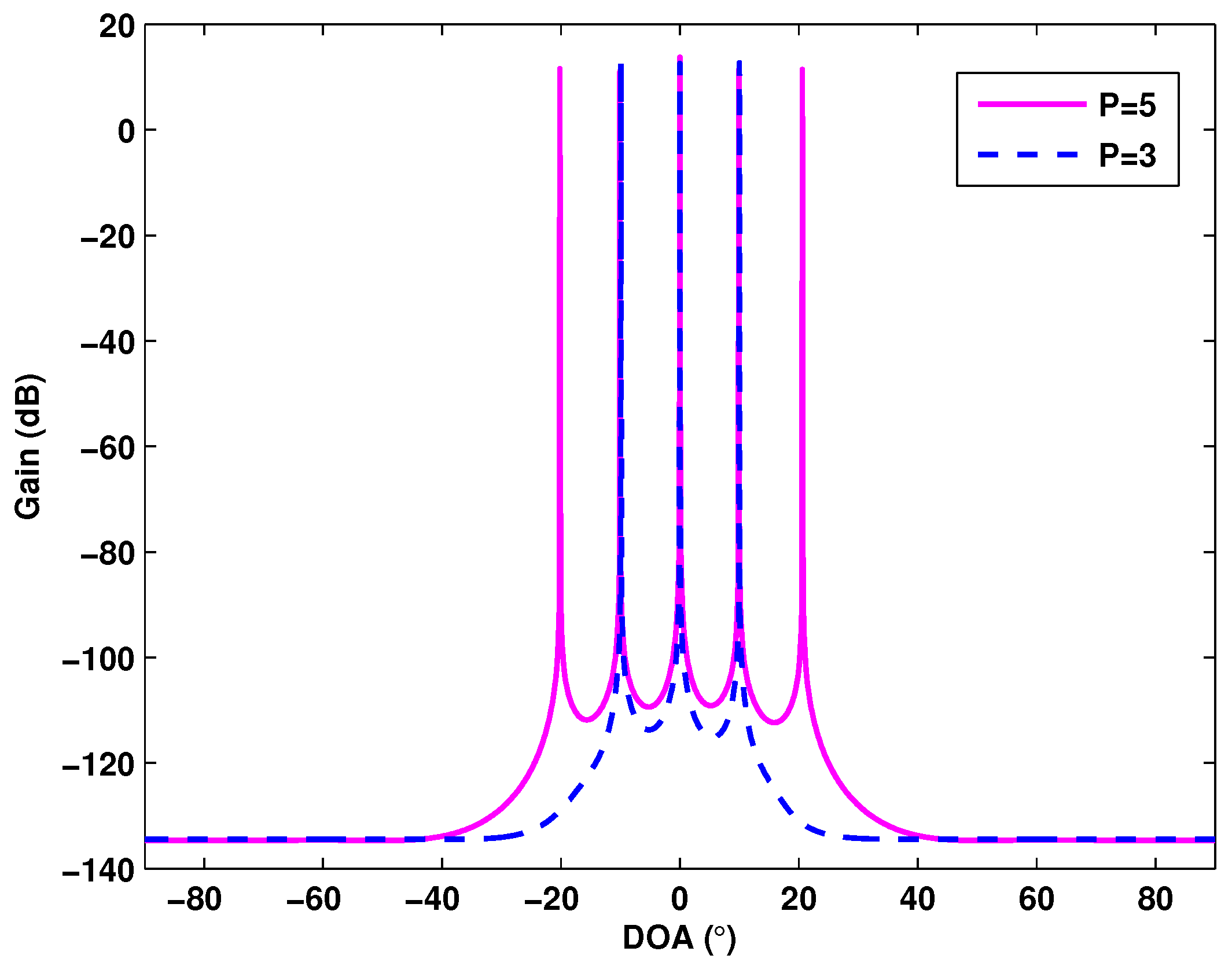
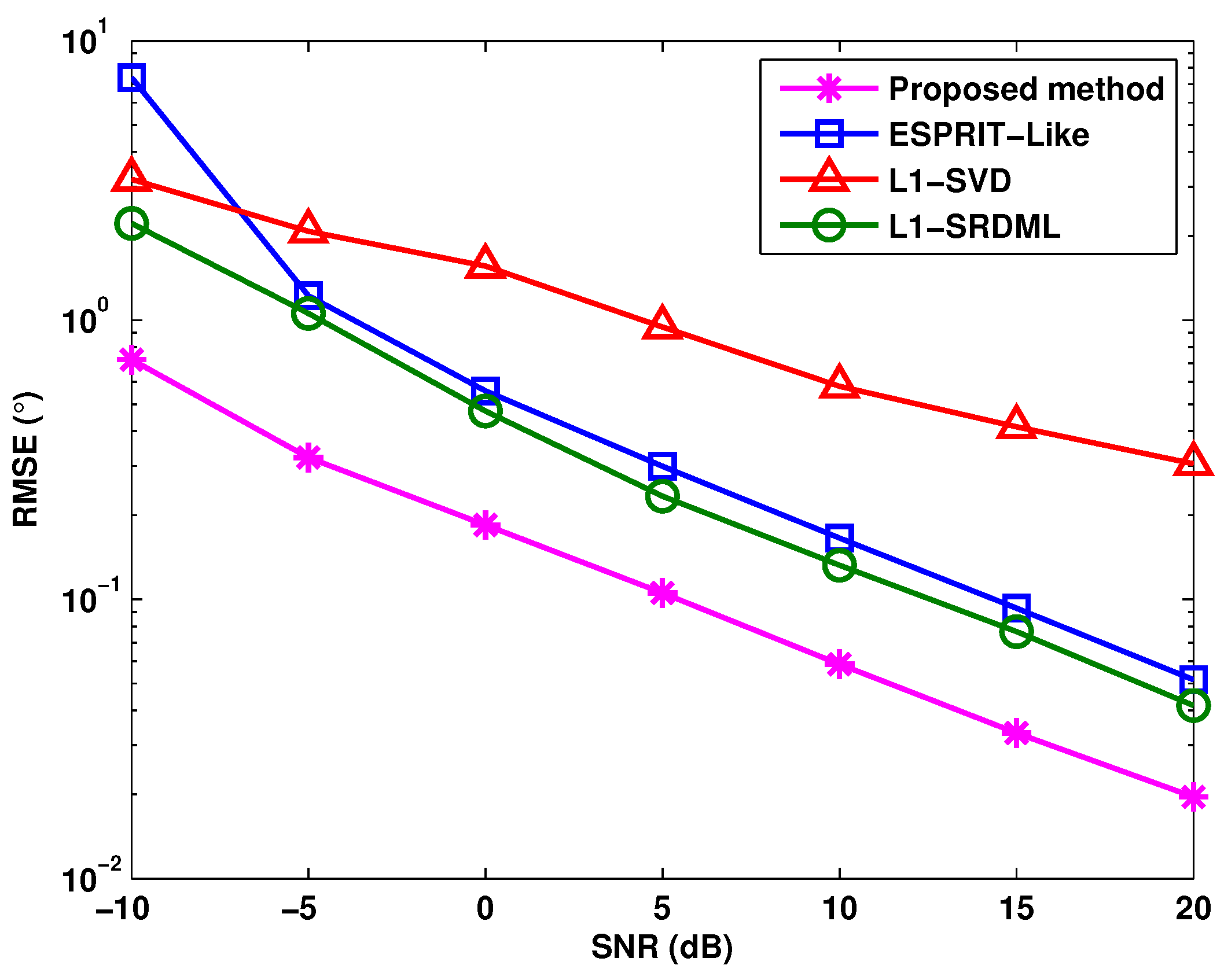
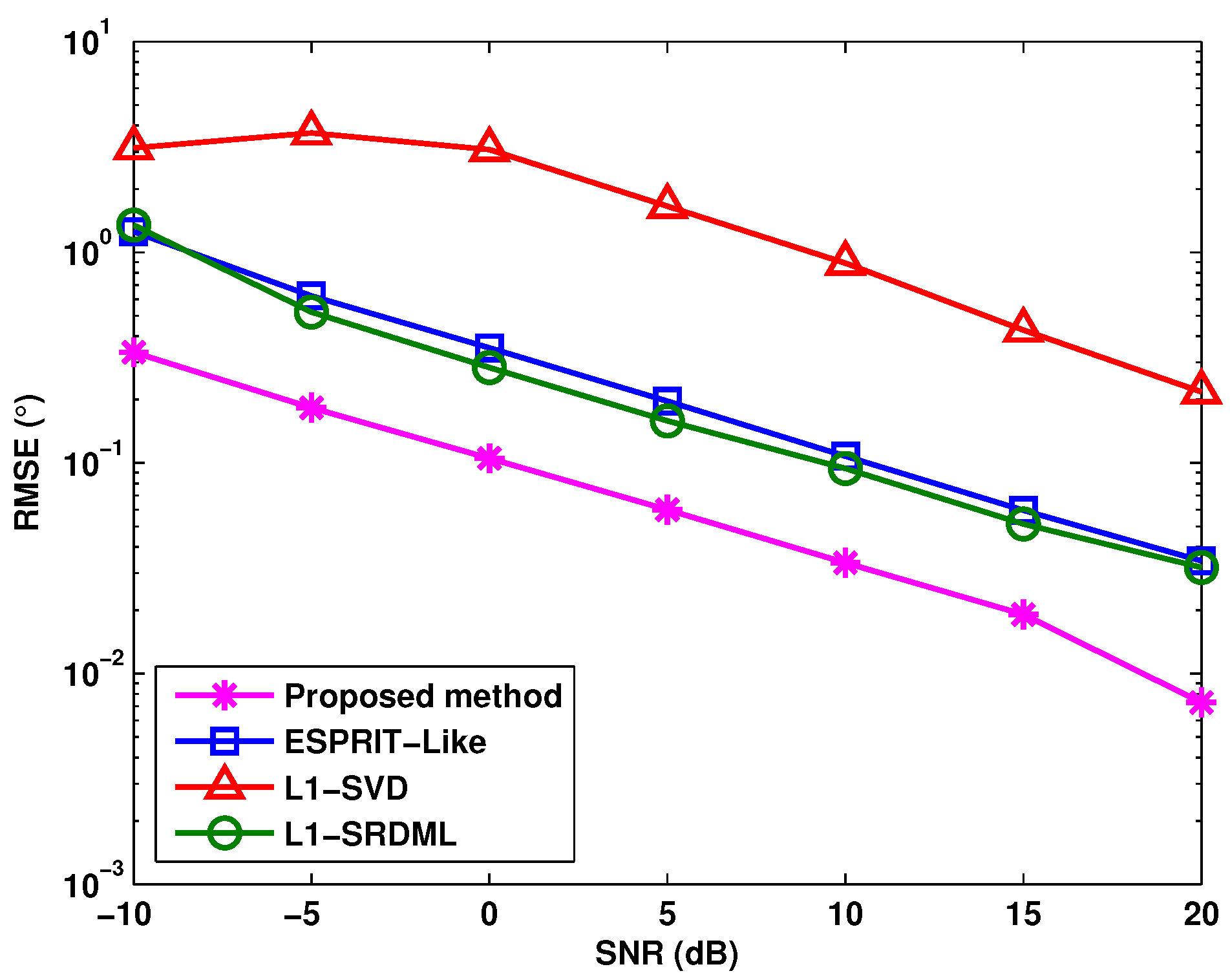
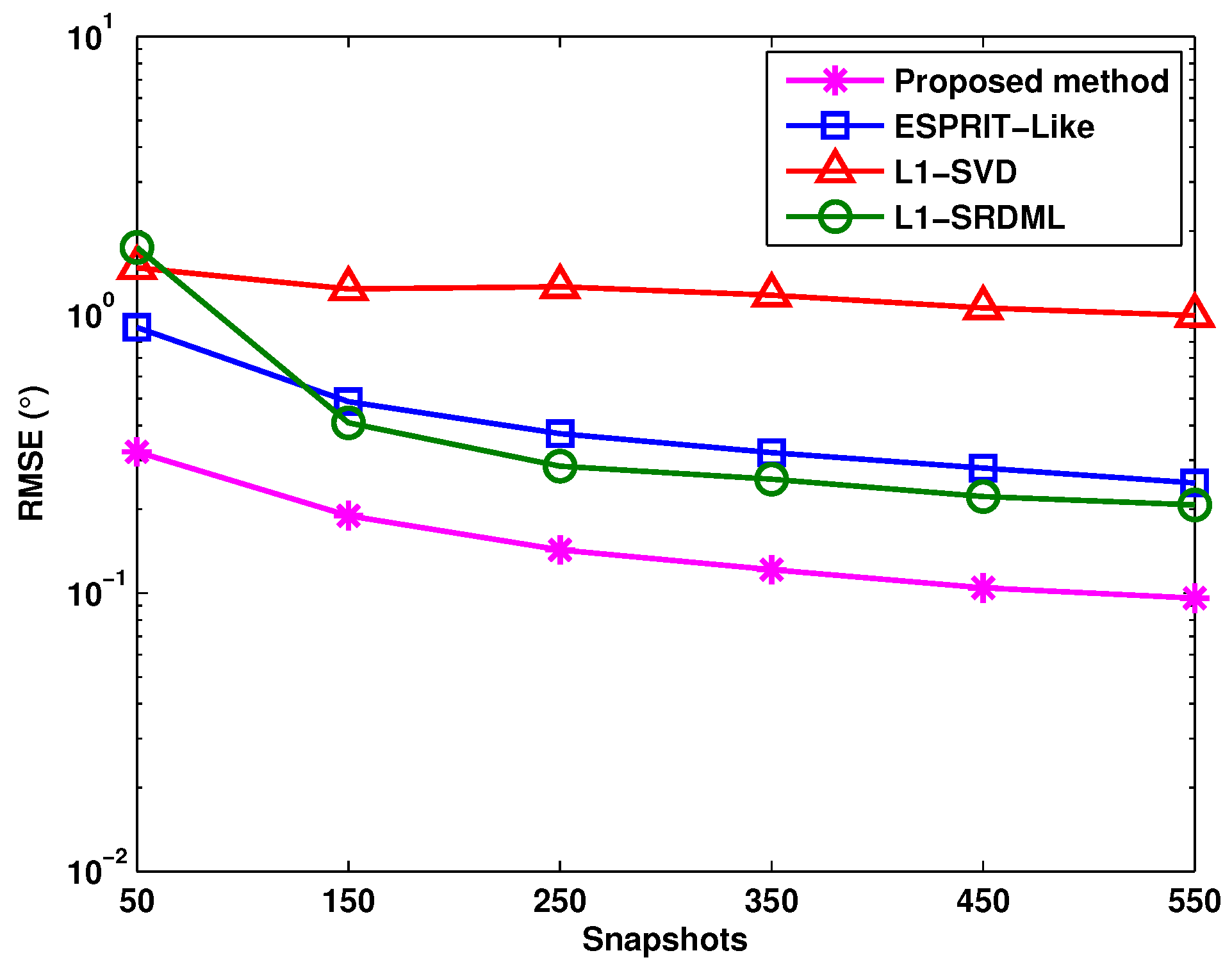
5. Simulation Results
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Fishler, E.; Haimovich, A.; Blum, R. Performance of MIMO radar systems: Advantages of angular diversity. In Proceedings of the Thirty-Eighth Asilomar Conference on Signals, Systems and Computers, Pacific Grove, CA, USA, 7–10 November 2004; pp. 305–309.
- Wang, X.; Jeske, D.; Serpedin, E. An overview of a class of clock synchronization algorithms for wireless sensor networks: A statistical signal processing perspective. Algorithms 2015, 8, 590–620. [Google Scholar] [CrossRef]
- Gao, X.; Zhang, X.F.; Feng, G.P.; Wang, Z.Q.; Xu, D.Z. On the MUSIC-derived aprroaches of angle estimation for bistatic MIMO radar. In Proceedings of the 2009 International Conference on Wireless Networks and Information Systems, TBD, Shanghai, China, 28–29 December 2009; pp. 343–346.
- Chen, D.; Chen, B.; Qin, G. Angle estimation using ESPRIT in MIMO radar. Electron. Lett. 2008, 44, 770–771. [Google Scholar]
- Zhang, X.; Huang, Y.; Chen, C.; Li, J.; Xu, D. Reduced-complexity Capon for derection of arrival estimation in a monostatic mutiple-input multiple-output radar. IET Radar Sonar Navig. 2012, 8, 796–801. [Google Scholar] [CrossRef]
- He, J.; Gao, M.W.; Zhang, L.; Wu, H. Sparse signal recovery from fixed low-rank subspace via compressive measurement. Algorithms 2013, 6, 871–882. [Google Scholar] [CrossRef]
- Burns, B.L.; Wilson, N.E.; Thomas, M.A. Group sparse reconstruction of multi-dimensional spectroscopic imaging in human brain in vivo. Algorithms 2014, 7, 276–294. [Google Scholar] [CrossRef]
- Liu, Y.J.; Tao, T.Y. A CS recovery algorithm for model and time delay identification of MISO-FIR systems. Algorithms 2015, 8, 743–753. [Google Scholar] [CrossRef]
- Malioutov, D.; Cetin, M.; Willsky, A.S. A sparse signal reconstruction perspective for source localization with sensor arrays. IEEE Trans. Signal Process. 2005, 53, 3010–3022. [Google Scholar] [CrossRef]
- Yin, J.; Chen, T. Direction-of-arrival estimation using a sparse representation of array covariance vectors. IEEE Trans. Signal Process. 2011, 59, 4489–4493. [Google Scholar] [CrossRef]
- Wang, X.P.; Wang, W.; Li, X.; Liu, J. Real-valued covariance vector sparsity-inducing DOA estimation for monostatic MIMO radar. Sensors 2015, 15, 28271–28286. [Google Scholar] [CrossRef] [PubMed]
- Dai, J.; Xu, X.; Zhao, D. Direction-of-arrival estimation via real-valued sparse representation. IEEE Antennas Wirel. Propag. Lett. 2013, 12, 376–379. [Google Scholar] [CrossRef]
- Liu, X.; Liao, G. Direction finding and mutual coupling estimation for bistatic MIMO radar. Signal Process. 2012, 92, 517–522. [Google Scholar] [CrossRef]
- Zheng, Z.D.; Zhang, J.; Zhang, J.Y. Joint DOD and DOA estimation of bistatic MIMO radar in the presence of unknown mutual coupling. Signal Process. 2012, 92, 3039–3048. [Google Scholar] [CrossRef]
- Dai, J.; Zhao, D.; Ji, X. A sparse representation method for DOA estimation with unknown mutual coupling. IEEE Antennas Wirel. Propag. Lett. 2012, 11, 1210–1213. [Google Scholar] [CrossRef]
- Liu, J.; Wang, X.P.; Zhou, W.D. Covariance vector sparsity-aware DOA estimation for monostatic MIMO radar with unknown mutual coupling. Signal Process. 2016, 119, 21–27. [Google Scholar] [CrossRef]
- Bencheikh, M.L.; Wang, Y. Non circular ESPRIT-RootMUSIC joint DOA-DOD estimation in bistatic MIMO radar. In Proceedings of the 2011 7th International Workshop on Systems, Signal Processing and Their Applications (WOSSPA), Tipaza, Algeria, 9–11 May 2011; pp. 51–54.
- Picinbono, B. On circularity. IEEE Trans. Signal Process. 1994, 42, 3473–3482. [Google Scholar] [CrossRef]
- Yang, M.L.; Chen, B.X.; Yang, X.Y. Conjugate ESPRIT algorithm for bistatic MIMO radar. Electron. Lett. 2010, 46, 1692–1694. [Google Scholar] [CrossRef]
- Wang, W.; Wang, X.; Song, H.; Ma, Y. Conjugate ESPRIT for DOA estimation in monostatic MIMO radar. Signal Process. 2013, 93, 2070–2075. [Google Scholar] [CrossRef]
- Li, J.; Stoica, P. MIMO radar with colocated antennas. IEEE Signal Process. Mag. 2007, 24, 106–114. [Google Scholar] [CrossRef]
- Wang, W.J.; Ren, S.W.; Ding, Y.T.; Wang, H.Y. An efficient algorithm for direction finding against unknown mutual coupling. Sensors 2014, 14, 20064–20077. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.X.; Trinkle, M.; Ng, B.W.-H. DOA estimation under unknown mutual coupling and multipath with improved effective array aperture. Sensors 2015, 15, 30856–30869. [Google Scholar] [CrossRef] [PubMed]
- Friedlander, B.; Weiss, A.J. Direction finding in the presence of mutual coupling. IEEE Trans. Antenn. Propag. 1991, 39, 273–284. [Google Scholar] [CrossRef]
- Haardt, M.; Romer, F. Enhancements of unitary ESPRIT for non-circular sources. In Proceedings of the IEEE International Conference on Acoustics, Speech and Signal Processing, Montreal, QC, Canada, 17–21 May 2004; pp. 1520–6149.
- Abeida, H.; Delmas, J.P. MUSIC-like estimation of direction of arrival for noncircular sour. IEEE Trans. Signal Process. 2006, 54, 2678–2690. [Google Scholar] [CrossRef]
- Sturm, J.F. Using SeDuMi, A Matlab toolbox for optimization ove symmetric cones. Optim. Method Softw. 2009, 11–12, 625–653. [Google Scholar]
- Grant, M.; Boyd, S. CVX: MATLAB Software for Disciplined Convex Programming, Version 2.1; CVX Research: Austin, TX, USA, 2012.
- Taboga, M. Lectures on Probability Theory and Mathematical Statistics; Amazon CreateSpace: North Charleston, SC, USA, 2012; pp. 469–473. [Google Scholar]
- Wax, M.; Kailath, T. Detection of signals by information theoretic criteria. IEEE Trans. Acoust. Speech Signal Process. 1985, 33, 387–392. [Google Scholar] [CrossRef]

© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, W.; Liu, J.; Zhu, P.; Gong, W.; Hou, J. Noncircular Sources-Based Sparse Representation Algorithm for Direction of Arrival Estimation in MIMO Radar with Mutual Coupling. Algorithms 2016, 9, 61. https://doi.org/10.3390/a9030061
Zhou W, Liu J, Zhu P, Gong W, Hou J. Noncircular Sources-Based Sparse Representation Algorithm for Direction of Arrival Estimation in MIMO Radar with Mutual Coupling. Algorithms. 2016; 9(3):61. https://doi.org/10.3390/a9030061
Chicago/Turabian StyleZhou, Weidong, Jing Liu, Pengxiang Zhu, Wenhe Gong, and Jiaxin Hou. 2016. "Noncircular Sources-Based Sparse Representation Algorithm for Direction of Arrival Estimation in MIMO Radar with Mutual Coupling" Algorithms 9, no. 3: 61. https://doi.org/10.3390/a9030061
APA StyleZhou, W., Liu, J., Zhu, P., Gong, W., & Hou, J. (2016). Noncircular Sources-Based Sparse Representation Algorithm for Direction of Arrival Estimation in MIMO Radar with Mutual Coupling. Algorithms, 9(3), 61. https://doi.org/10.3390/a9030061




