Algorithms 2009, 2(1), 437-447; doi:10.3390/a2010437
Article

Resonance in Interacting Induced-Dipole Polarizing Force Fields: Application to Force-Field Derivatives

Francisco Torrens 1,* email and Gloria Castellano 2 email
1 Institut Universitari de Ciència Molecular, Universitat de València, Edifici d’Instituts de Paterna, P. O. Box 22085, E-46071 València, Spain 2 Instituto Universitario de Medio Ambiente y Ciencias Marinas, Universidad Católica de Valencia San Vicente Mártir, Guillem de Castro-94, E-46003 València, Spain
* Author to whom correspondence should be addressed.
Received: 5 December 2008; in revised form: 15 January 2009 / Accepted: 27 February 2009 / Published: 10 March 2009
(This article belongs to the Special Issue Algorithms and Molecular Sciences)
PDF Full-text Download PDF Full-Text [198 KB, uploaded 10 March 2009 10:44 CET]
Abstract: The Silberstein model of the molecular polarizability of diatomic molecules, generalized by Applequist et al. for polyatomic molecules, is analyzed. The atoms are regarded as isotropically polarizable points located at their nuclei, interacting via the fields of their induced dipoles. The use of additive values for atom polarizabilities gives poor results, in some cases leading to artificial predictions of absorption bands. 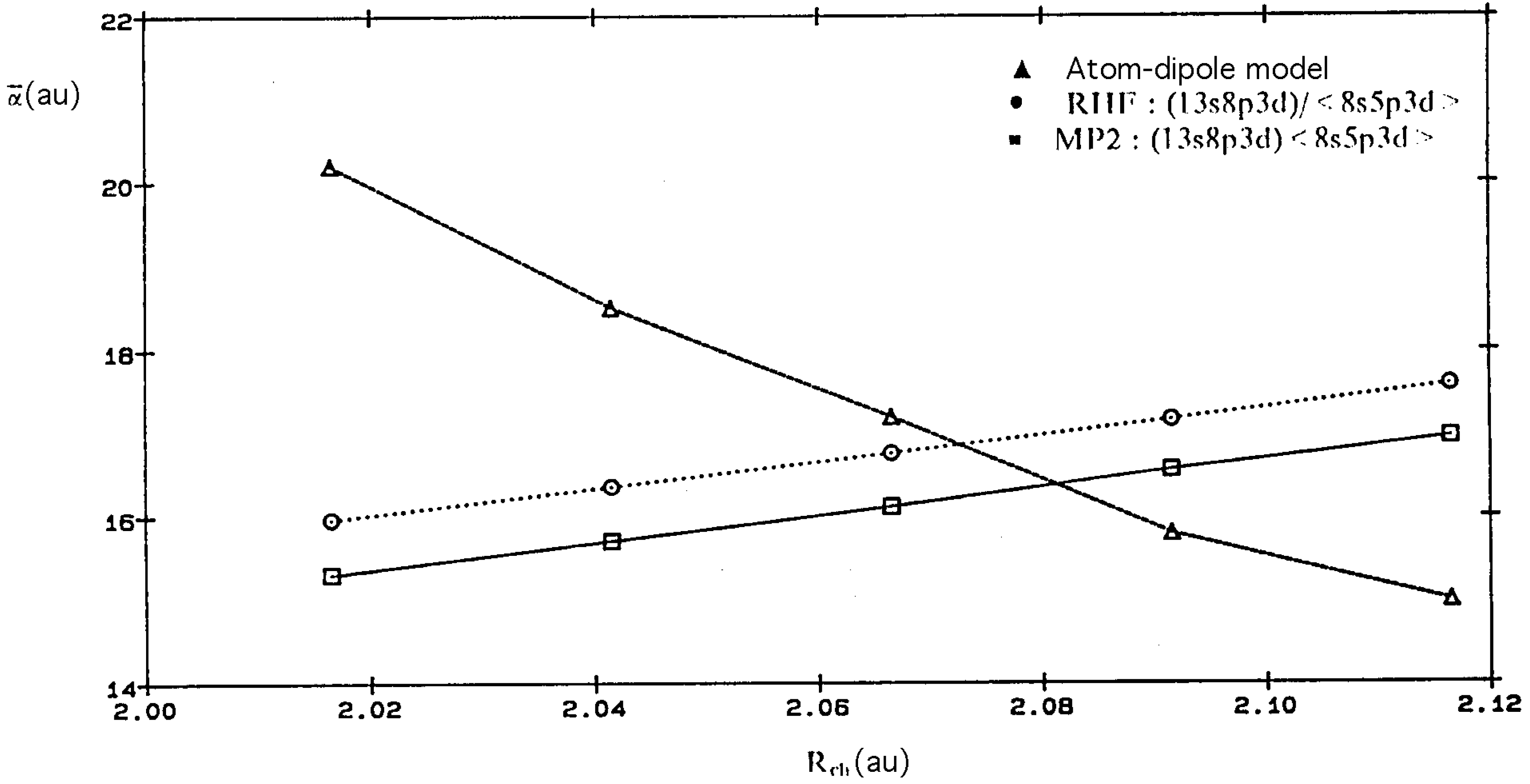The molecular polarizability of methane and its derivative are computed. The agreement with experimental mean molecular polarizabilities is within 1–5%. A hypothesis is indispensable for a suitable representation of polarizability derivative.
Keywords: interacting induced-dipole polarization; polarizability; resonance; polarizing force field; derivative; dipole moment; electric field; electric field gradient

Article Statistics

Click here to load and display the download statistics.

Cite This Article

MDPI and ACS Style

Torrens, F.; Castellano, G. Resonance in Interacting Induced-Dipole Polarizing Force Fields: Application to Force-Field Derivatives. Algorithms 2009, 2, 437-447.

AMA Style

Torrens F, Castellano G. Resonance in Interacting Induced-Dipole Polarizing Force Fields: Application to Force-Field Derivatives. Algorithms. 2009; 2(1):437-447.

Chicago/Turabian Style

Torrens, Francisco; Castellano, Gloria. 2009. "Resonance in Interacting Induced-Dipole Polarizing Force Fields: Application to Force-Field Derivatives." Algorithms 2, no. 1: 437-447.

Algorithms EISSN 1999-4893 Published by MDPI AG, Basel, Switzerland RSS E-Mail Table of Contents Alert