Review on Polymers for Thermoelectric Applications
Abstract
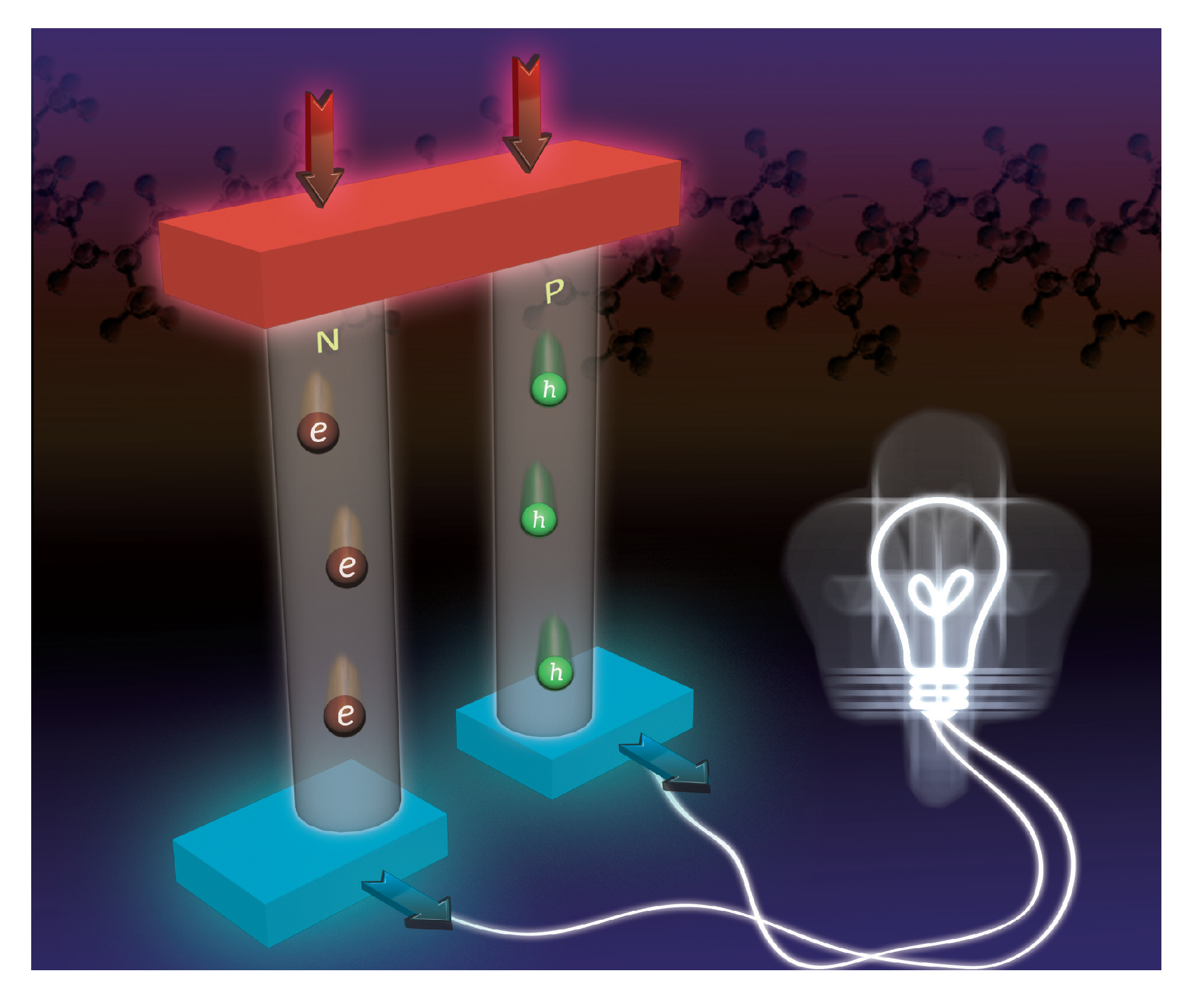
:1. Introduction
| Polymer | Structure | Polymer | Structure |
|---|---|---|---|
| Polyacetylene | 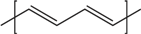 | Polyaniline | 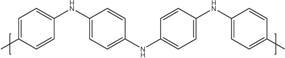 |
| PEDOT | 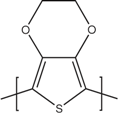 | Polypyrrole | 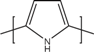 |
| Polyalkyl thiophenes |  | Poly(2-7carbazoles) | 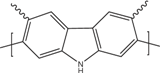 |
2. Effect of the Doping Level on the Thermoelectric Properties of Conductive Polymers
2.1. Chemical Doping and De-Doping
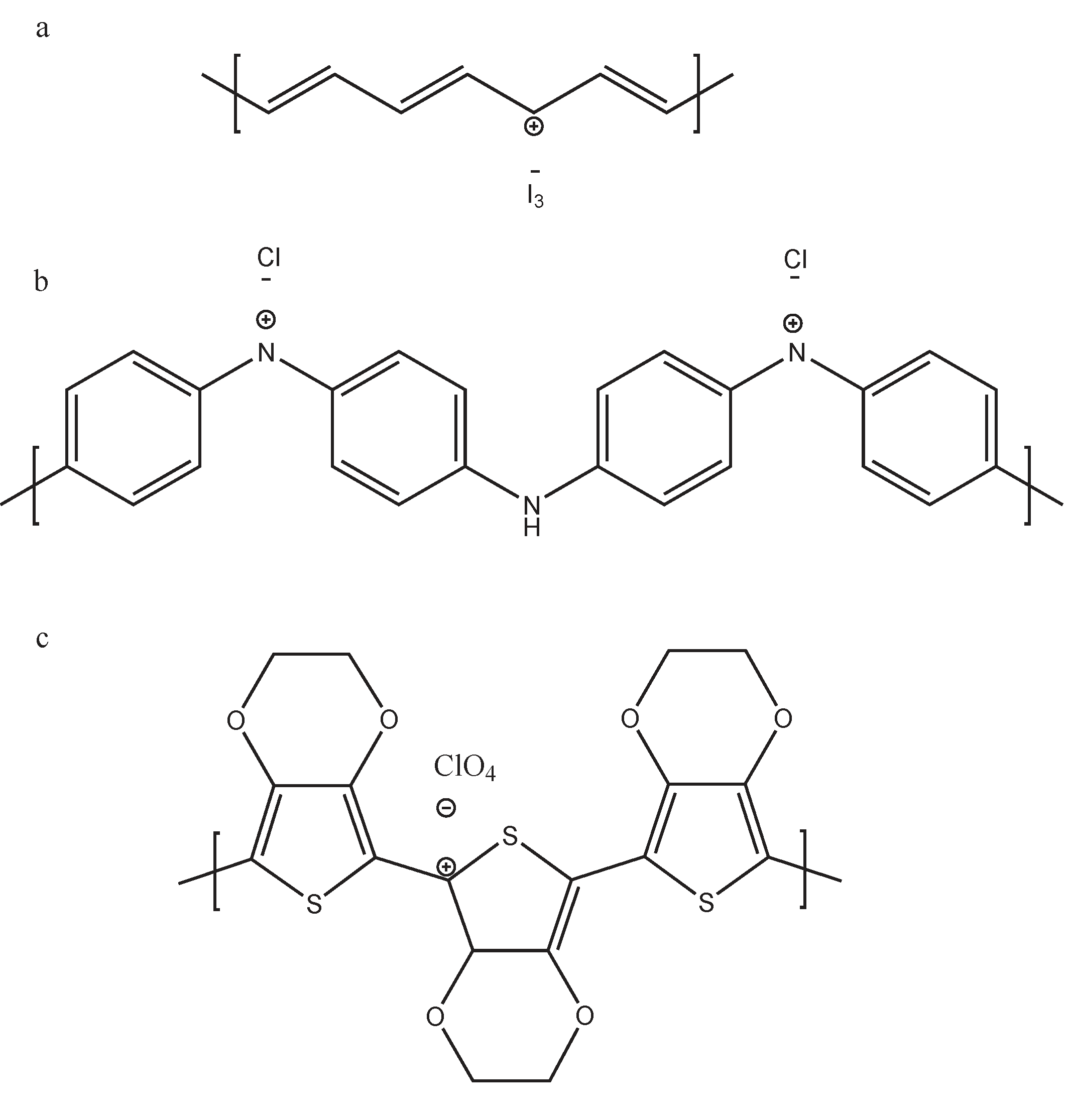
| Polymer | Dopant | References | Polymer | Dopant | References |
|---|---|---|---|---|---|
| PEDOT | 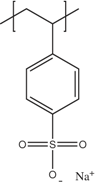 | [13,14,21,22,23] | PANI | 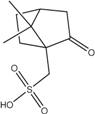 | [24,25,26] |
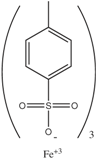 | [14,15,16] | H2SO4 | [27,28] | ||
| LiClO4 | [10,29,30] | HCl | [31] | ||
| BF4 | [32,33,34] | H3PO4 | [35] | ||
| PF6− | [10,29,36] | Polycarbazoles | FeCl3 | [37] | |
| Polyalkyl thiophenes | FeCl3 | [38] | Polyacetylene | I2 vapour | [39,40] |
| I2 vapour | [38] |
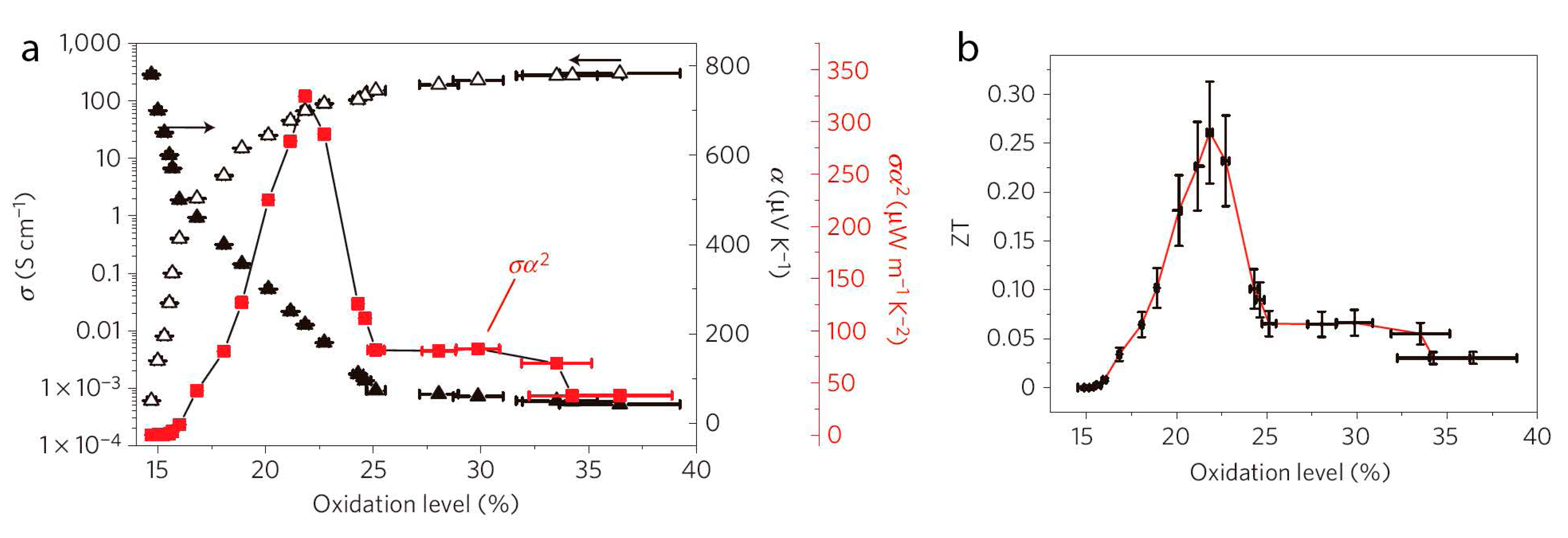
2.2. Electrochemical Doping/De-Doping
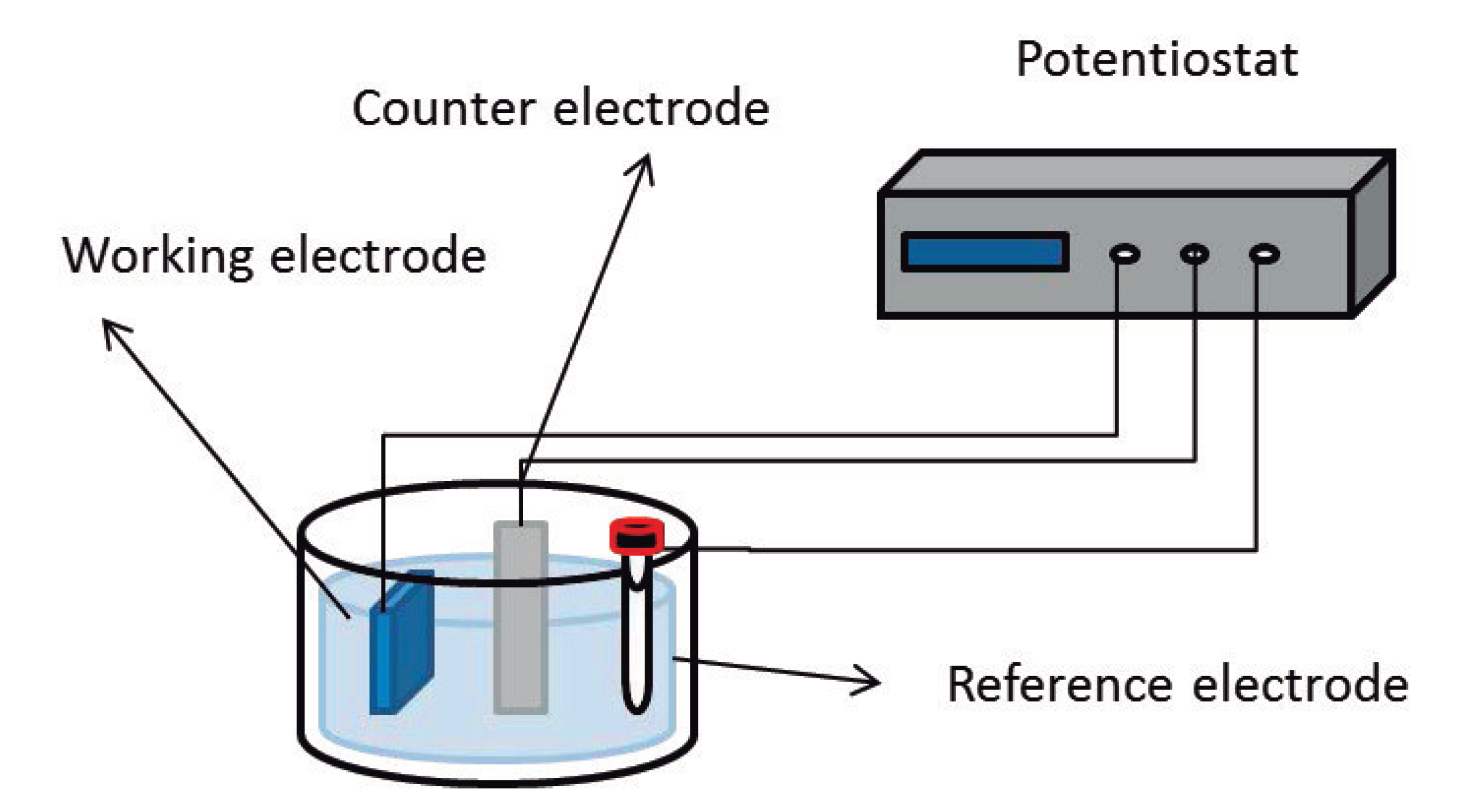
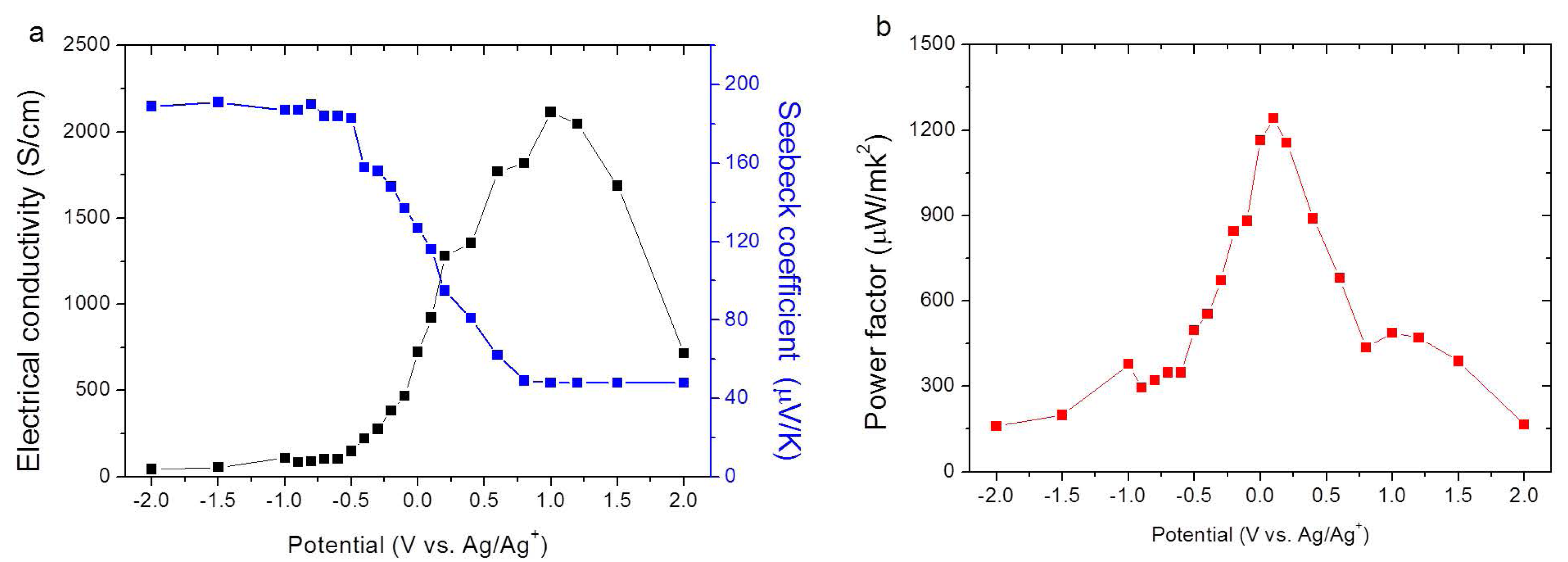
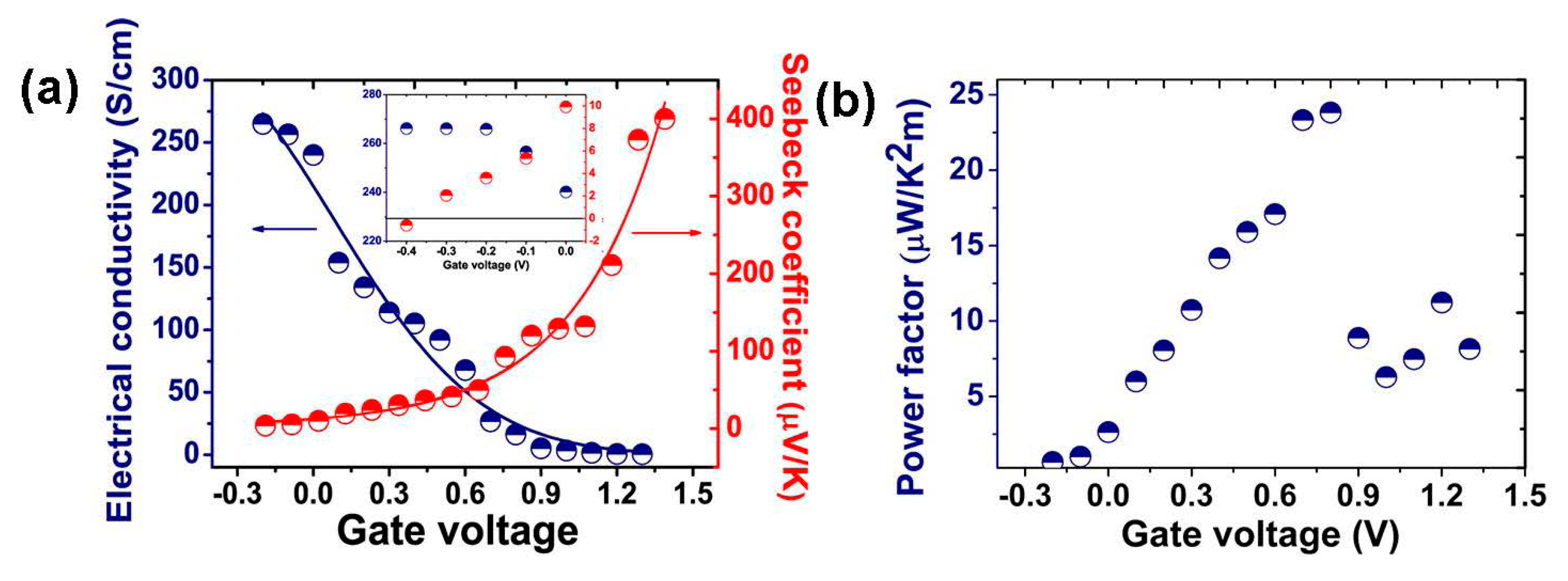
2.3. Secondary Doping
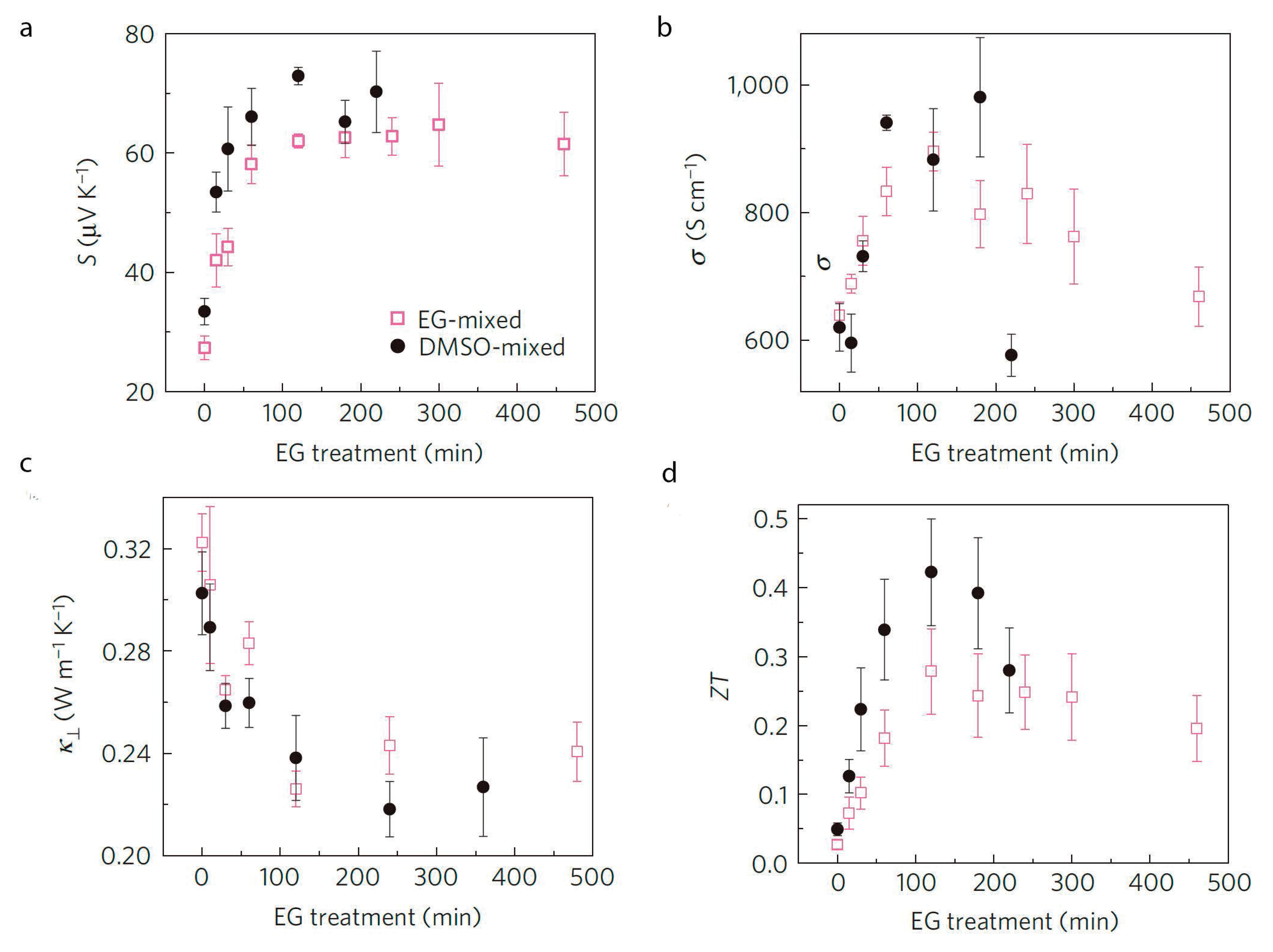


2.4. Effect of pH on the Thermoelectric Properties of PEDOT:PSS
2.5. Co-polymers and Polymer Blends

3. Improvement of the Figure of Merit Using Polymer Composites
3.1. Carbon Nanotubes
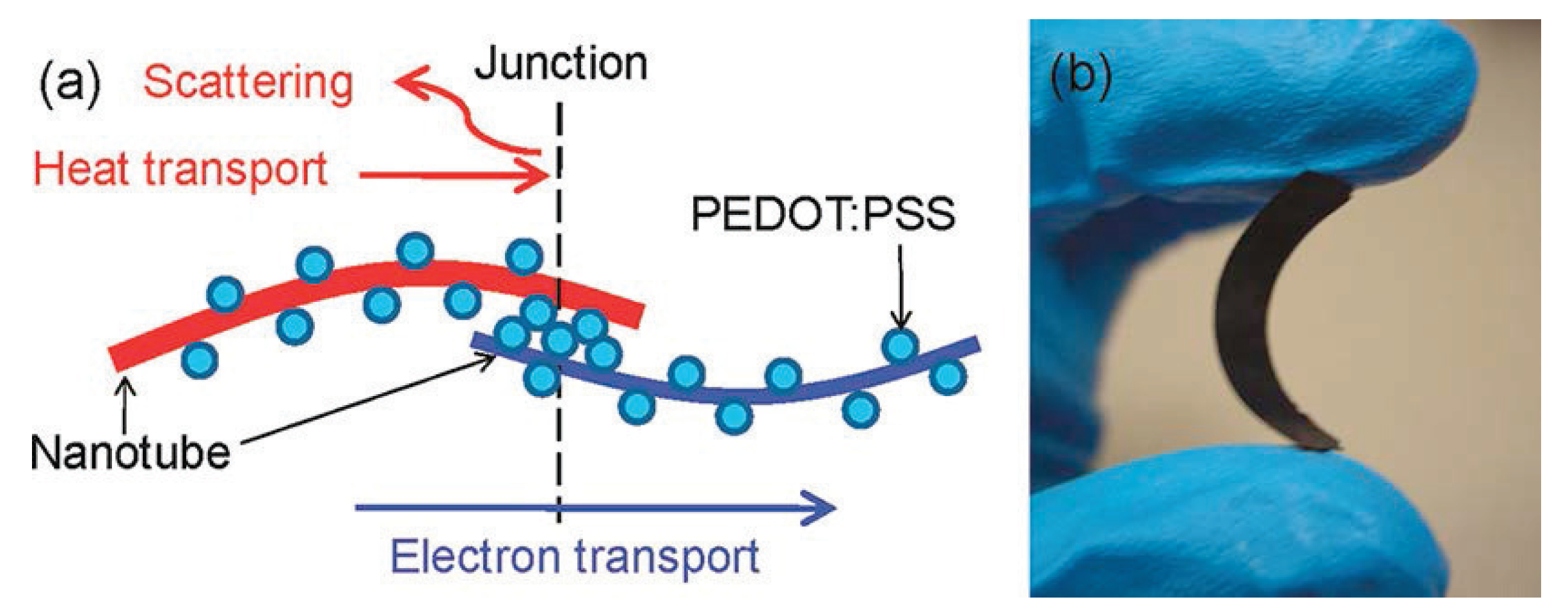
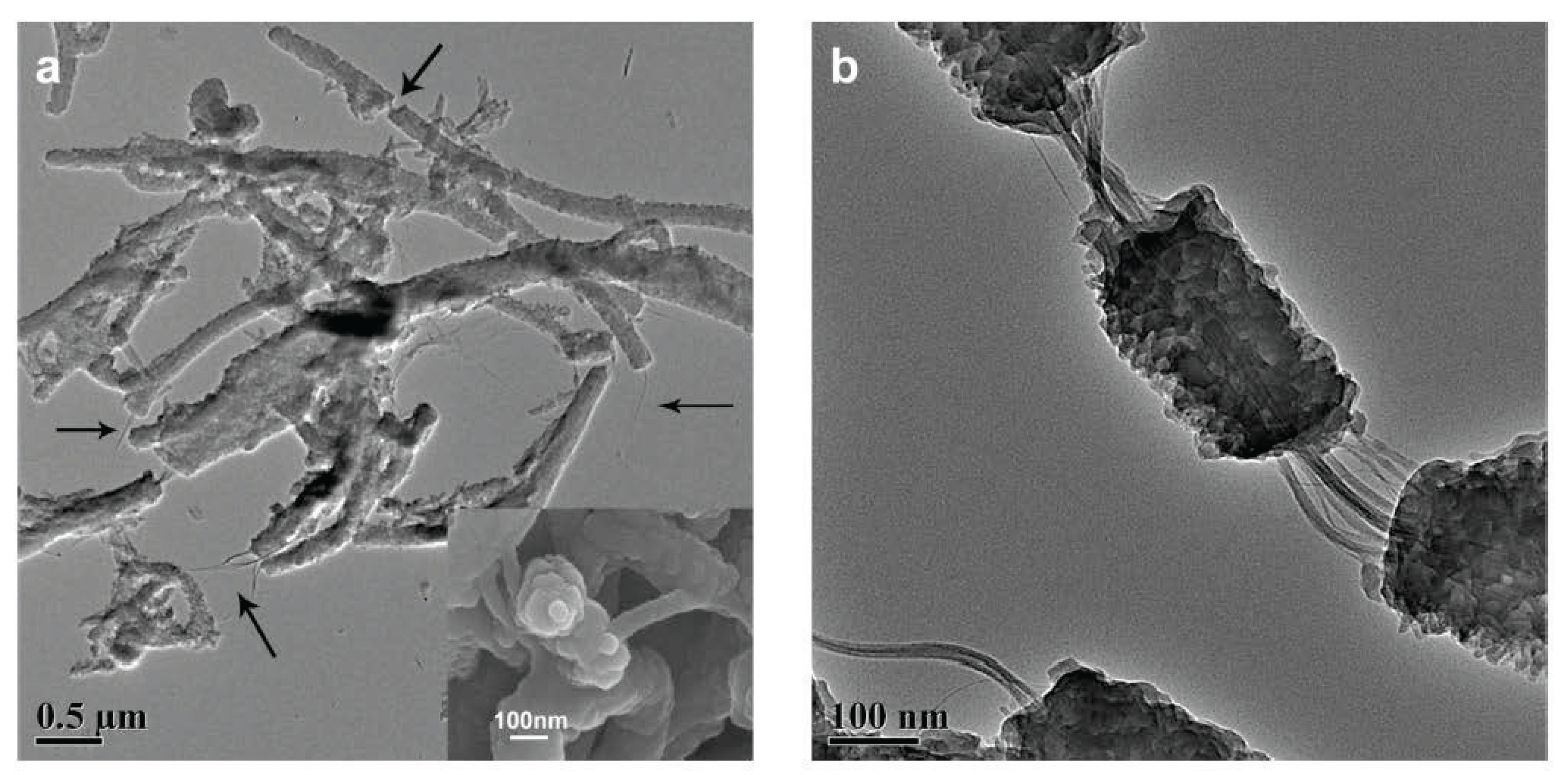
3.2. Graphene/Graphite
3.3. Inorganic Nanoparticles
| System | σ(S·cm−1) | S (µV·K−1) | κ(W·m−1·K−1) | PF (µW·m−1·K−2) | ZT | Refs. |
|---|---|---|---|---|---|---|
| PEDOT:Tos/ PEO-PPO-PEO electrochem. reduct. | ∼1200 | ∼100 | 1270 | ∼1.02 | [16] | |
| PEDOT:PSS + EG treat. | ∼980 | ∼70 | 0.23 | 469 | 0.4 | [48] |
| PEDOS-C6 electrochem. reduct. | ∼200 | ∼110 | 354.7 | [83] | ||
| PEDOT:PSS + EG treat. + hydrazine reduct. | ∼1300 | ∼49 | 0.3 | 320 | 0.3 | [13] |
| PEDOT:Tos + TDEA reduct. | ∼80 | ∼290 | 0.37 | 324 | 0.25 | [13] |
| PEDOT:BTFMSI + hydrazine reduct. | ∼1080 | ∼37 | 0.19 | 147 | 0.22 | [10] |
| PEDOT:PSS electrochem. reduct. | ∼25 | ∼90 | 0.17 | 23.5 | 0.041 | [21] |
| PEDOT:PSS + DMSO 5% | 298 | 12.65 | 4.78 | ∼0.001 | [44] | |
| PANI/CSA-doping in m-cresol | 220 | ∼20 | 11 | [25] | ||
| PANI doped with H3PO4 | 40 | ∼7 | 0.19 | [17] | ||
| polyselenophene and its copolymers with 3-methylthiophene | 0.1–54 | 20–98 | 2–12 | 0.034 | [38] | |
| Copolymer of 1,12-bis(carbazolyl) dodecane and thieno[3,2-b]thiophene and its copolymers with 3-methylthiophene | 4 × 10−5−0.4 | 75–169 | ∼0.17–0.33 | [52] | ||
| Phenylenevinylene block copolymers and their blends with MEH-PPV | 6 × 10−6−14.4 | 7–531 | ∼10−5–1.33 | [45] | ||
| PEDOT:PSS + Polythiophenes Bilayered nanofilms | 125–200 | 11–17 | ∼1.5–6 | [53] |
| System | σ(S·cm−1) | S(µV·K−1) | κ(W·m−1·K−1) | PF (µW·m−1·K−2) | ZT | Refs. |
|---|---|---|---|---|---|---|
| SWCNT/PEDOT:PSS, DMSO, GA | 400 | 27 | ↑0.4 | 25 | ∼0.02 | [56] |
| CNT/PEDOT stabilizer TCPP | 980 | 70 | 500 | [58] | ||
| CNT/PVAc | 48 | 45 | 0.34 | 0.006 | [55] | |
| SWCNT/PEDOT:PSS PVAc | 1000 | 41 | 0.2–0.4 | 160 | [57] | |
| SWCNT/PEDOT:PSS Layered structure | 241 | 38.9 | 21.1 | [84] | ||
| SWCNT/PANI | 125 | 40 | 0.2 | 0.004 | [59] | |
| 3D-CNT/PANI | 40.35 | 23 | 0.29 | 0.0022 | [59] | |
| CNT-PANI nanofibers | 15 | 10 | 0.16 | 0.0022 | [85] | |
| PANI coated CNT/PANI | 28 | 21.6 | 0.4 | 0.001 | [86] | |
| poly(3-hexylthiophene) SWCNTs | 1000 | 29 | 98 | [61] | ||
| MWCNT/polithiophene | 6 | 25 | 0.6 | 8.7 × 10−4 | [87] | |
| PANI/graphite composites | 100 | 10 | 1.2 | 4.18 | 1.37 × 10−3 | [63] |
| PANI/graphene nanosheets pellet | ∼60 | ∼30 | 5.6 | [64] | ||
| PANI/graphene nanosheets film | ∼8 | ∼42 | 1.47 | [64] | ||
| PANI/graphene nanoplatelets mechanical blending | 123 | 34 | 14 | [66] | ||
| PEDOT:PSS/expanded graphite | 213 | 15 | 5.31 | [68] | ||
| PEDOT:PSS/graphene fullerene | 700 | 25 | 0.4 | 0.06 | [69] |
| System | σ (S·cm−1) | S (µV·K−1) | κ (W·m−1·K−1) | PF (µW·m−1·K−2) | ZT | Refs. | |||
|---|---|---|---|---|---|---|---|---|---|
| PEDOT:PSS PAA Bi2Te3 | 380 | 79 | 0.36 | ∼240 | 0.2 | [77] | |||
| PEDOT:PSS Bi2Te3 | 250 | 150 | 0.558 | 131 | 0.08 | [74] | |||
| PANI Bi2Te3 nanorods p-type | 11.626 | 39 | 0.11 | 1.8 | 0.004 | [74] | |||
| PANI Bi2Te3 nanorods n-type | 23 | −70 | 10 | [74] | |||||
| PEDOT:PSS Bi2Te3 films | 421 | 18.6 | 0.07 | 9.9 | 0.04 | [76] | |||
| PEDOT:PSS Te | 19.3 | 163 | 0.22–0.3 | 70.9 | 0.1 | [78] | |||
| PEDOT:PSS Te nanowire | ∼15 | 260 | 100 | [79] | |||||
| PEDOT:PSS Te nanowire | ∼12 | 170 | 35 | [80] | |||||
| PEDOT:PSS Gold nanorod | ∼2000 | 12 | 20 | [80] | |||||
| PH3T Bi2Te3 | ∼4.5 | 118 | 6.3 | [88] | |||||
4. Theoretical Models of Thermoelectric Transport in Polymers
4.1. Phenomenological Models
4.2. Models Based on ab Initio Techniques
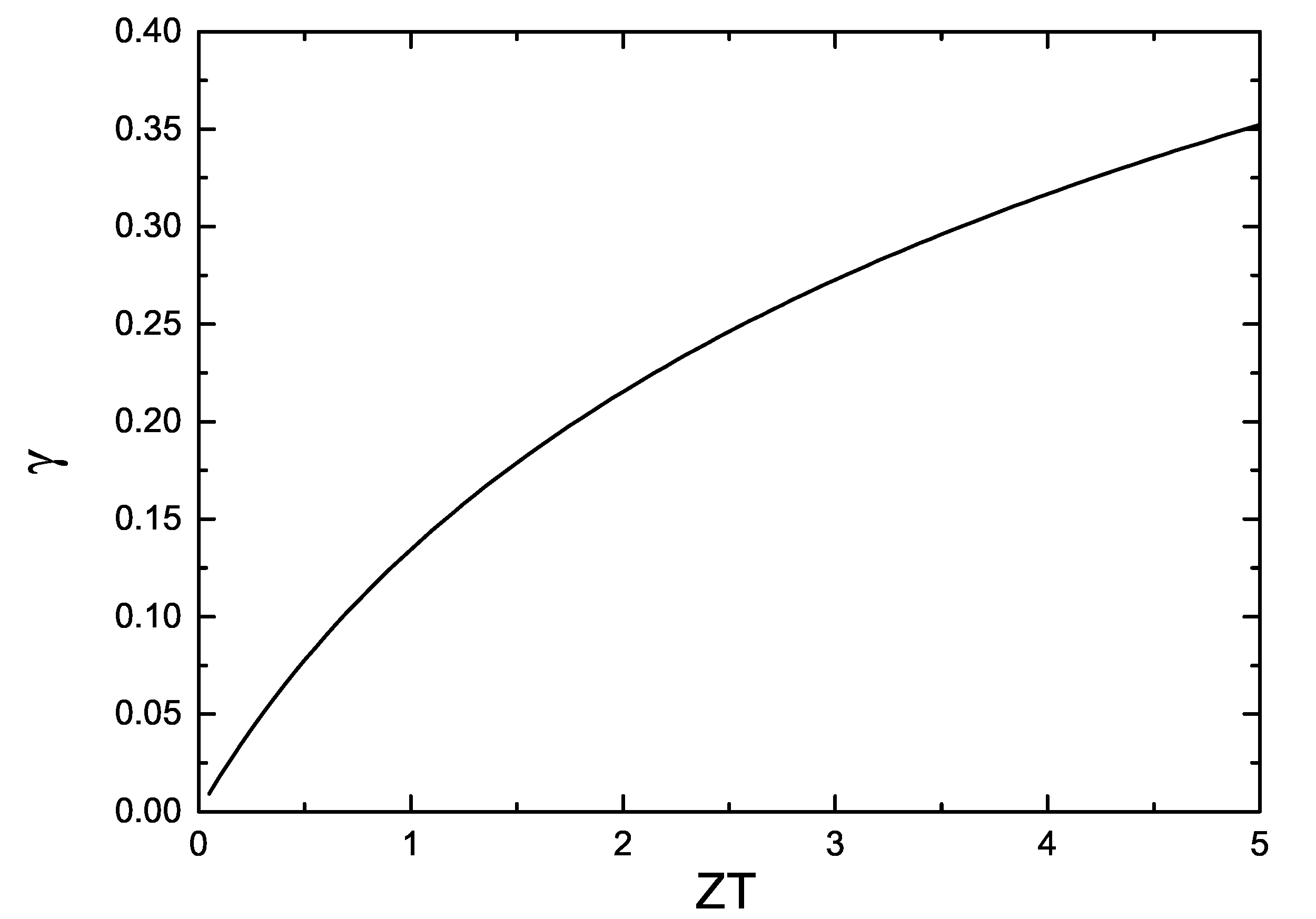
5. Summary
Acknowledgments
Author Contributions
Abbreviation List
| PEDOT | poly(3,4-ethylenedioxythiophene) |
| PPy | polypyrrole |
| PANI | polyaniline |
| Tos | p-toluenesulfonate |
| PSS | polystyrenesulfonate |
| MEH-PPV | poly-(2-methoxy-5-(2-ethylhexyloxy)phenylenevinylene) |
| CNT | carbon nanotube |
| GN | grafene |
| TCPP | meso-tetra(4-carboxyphenyl) porphine |
| PEDOS-C6 | poly(3,4-ethylenedioxyselenophene) |
| SWCNT | singlewall carbon nanotube |
| DWCNT | doublewall carbon nanotube |
| MWCNT | multiwall carbon nanotube |
| P3HT | poly(3-hexylthiophene) |
| Pth | polythiophene |
| P3MeT | poly(3-Metylthiophene) |
| PEO-PPO-PEO | poly(ethylene glycol)-block-poly(propylene glycol)-blockpoly(ethylene glycol) triblock copolymer |
| CSA | camphorsulfonic acid |
| EG | ethylene glycol |
| DMSO | dimethylsulfoxide |
| PVA | polyvinyl alcohol |
| PEI | polyethyleneimine |
| PVAc | poly(vinyl acetate) |
| PAA | poly(acrylic acid) |
| BTFMSI | bis(trifluoromethylsulfonyl)imide |
| TDEA | tetrakis(dimethylamino)ethylene |
Conflicts of Interest
References
- Kim, D.; Kim, Y.; Choi, K.; Grunlan, J.C.; Yu, C. Improved thermoelectric behavior of nanotube-filled polymer composites with poly(3,4-ethylenedioxythiophene) poly(styrenesulfonate). ACS Nano 2010, 4, 513–523. [Google Scholar] [CrossRef] [PubMed]
- Kim, G.H.; Hwang, D.H.; Woo, S.I. Thermoelectric properties of nanocomposite thin films prepared with poly(3,4-ethylenedioxythiophene) poly(styrenesulfonate) and graphene. Phys. Chem. Chem. Phys. 2012, 14, 3530–3536. [Google Scholar] [CrossRef] [PubMed]
- Goupil, C.; Seifert, W.; Zabrocki, K.; Mueller, E.; Snyder, G.J. Thermodynamics of thermoelectric phenomena and applications. Entropy 2011, 13, 1481–1517. [Google Scholar] [CrossRef]
- Cantarero, A.; Àlvarez, F.X. Thermoelectric effects: Semiclassical and quantum approaches from the boltzmann transport equation. In Nanoscale Thermoelectrics; Springer International Publishing: Berlin/Heidelberg, Germany, 2014; pp. 1–39. [Google Scholar]
- Heremans, J.P. Low dimensional thermoelectricity. Acta Phys. Pol. A 2005, 108, 609–634. [Google Scholar]
- Hicks, L.D.; Dresselhaus, M.S. Effect of quantum-well structures on the thermoelectric figure of merit. Phys. Rev. B 1993, 47, 12727–12731. [Google Scholar] [CrossRef]
- Martín-González, M.; Caballero-Calero, O.; Díaz-Chao, P. Nanoengineering thermoelectrics for 21st century: Energy harvesting and other trends in the field. Renew. Sustain. Energy Rev. 2013, 24, 288–305. [Google Scholar] [CrossRef]
- De Tomás, C.; Cantarero, A.; Lopeandia, A.; Àlvarez, F.X. From kinetic to collective behavior in thermal transport on semiconductors and semiconductor nanostructures. J. Appl. Phys. 2014, 115, 164314. [Google Scholar]
- Wagner, M.; Span, G.; Holzer, S.; Grasser, T. Thermoelectric power generation using large-area Si/SiGe pn-junctions with varying Ge content. Semicond. Sci. Technol. 2007, 22, S173–S176. [Google Scholar]
- Culebras, M.; Cantarero, A.; Gómez, C.M. Enhanced thermoelectric performance of PEDOT with different counter ions optimized by chemical reduction. J. Mater. Chem. A 2014, 2, 10109–10115. [Google Scholar] [CrossRef]
- Nolas, G.S.; Morelli, D.T.; Tritt, T.M. Skutterudites: A phonon-glass-electron crystal approach to advanced thermoelectric energy conversion applications. Annu. Rev. Mater. Sci. 1999, 29, 1–29. [Google Scholar] [CrossRef]
- Kuznetsov, V.L.; Kuznetsova, L.A.; Kaliazin, A.E.; Rowe, D.M. Preparation and thermoelectric properties of A8IIB16IIIB30IV clathrate compounds. J. Appl. Phys. 2000, 87, 7871–7875. [Google Scholar] [CrossRef]
- Lee, S.H.; Park, H.; Kim, S.; Son, W.; Cheong, I.W.; Kim, J.H. Transparent and flexible organic semiconductor nanofilms with enhanced thermoelectric efficiency. J. Mater. Chem. A 2014, 2, 7288–7294. [Google Scholar] [CrossRef]
- Bubnova, O.; Khan, Z.U.; Wang, H.; Braun, S.; Evans, D.R.; Fabretto, M.; Hojati-Talemi, P.; Dagnelund, D.; Arlin, J.B.; Geerts, Y.H.; et al. Semi-metallic polymers. Nat. Mater. 2014, 13, 190–194. [Google Scholar]
- Bubnova, O.; Khan, Z.U.; Malti, A.; Braun, S.; Fahlman, M.; Berggren, M.; Crispin, X. Optimization of the thermoelectric figure of merit in the conducting polymer poly(3,4-ethylenedioxythiophene). Nat. Mater. 2011, 10, 429–433. [Google Scholar] [CrossRef] [PubMed]
- Park, T.; Park, C.; Kim, B.; Shin, H.; Kim, E. Flexible PEDOT electrodes with large thermoelectric power factors to generate electricity by the touch of fingertips. Energy Environ. Sci. 2013, 6, 788–792. [Google Scholar] [CrossRef]
- Limelette, P.; Schmaltz, B.; Brault, D.; Gouineau, M.; Autret-Lambert, C.; Roger, S.; Grimal, V.; Tran Van, F. Conductivity scaling and thermoelectric properties of polyaniline hydrochloride. J. Appl. Phys. 2014, 115, 033712:1–033712:6. [Google Scholar] [CrossRef]
- Yamaguchi, I.; Nagano, T. Synthesis, chemical, and thermoelectric properties of n-type ÏǍ-conjugated polymer composed of 1,2,4-triazole and pyridine rings and its metal complexes. J. Appl. Polym. Sci. 2014, 131, 39928:1–39928:7. [Google Scholar] [CrossRef]
- Garreau, S.; Louarn, G.; Buisson, J.; Froyer, G.; Lefrant, S. In situ spectroelectrochemical raman studies of poly(3,4-ethylenedioxythiophene) (PEDT). Macromolecules 1999, 32, 6807–6812. [Google Scholar] [CrossRef]
- Chen, X.; Inganas, O. Three-step redox in polythiophenes: Evidence from electrochemistry at an ultramicroelectrode. J. Phys. Chem. 1996, 100, 15202–15206. [Google Scholar] [CrossRef]
- Bubnova, O.; Berggren, M.; Crispin, X. Tuning the thermoelectric properties of conducting polymers in an electrochemical transistor. J. Am. Chem. Soc. 2012, 134, 16456–16459. [Google Scholar] [CrossRef] [PubMed]
- Aleshin, A.; Williams, S.; Heeger, A. Transportproperties of poly(3,4-ethylenedioxythiophene)/ poly(styrenesulfonate). Synth. Met. 1998, 94, 173–177. [Google Scholar] [CrossRef]
- Jiang, F.-X.; Xu, J.-K.; LU, B.-Y.; Xie, Y.; Huang, R.-J.; Li, L.-F. Thermoelectric performance of poly(3,4-ethylenedioxythiophene): Poly(styrenesulfonate). Chin. Phys. Lett. 2008, 25, 2202–2205. [Google Scholar] [CrossRef]
- Jin, J.; Wang, Q.; Haque, M.A. Doping dependence of electrical and thermal conductivity of nanoscale polyaniline thin films. J. Phys. D-Appl. Phys. 2010, 43, 205302. [Google Scholar]
- Yao, Q.; Wang, Q.; Wang, L.; Wang, Y.; Sun, J.; Zeng, H.; Jin, Z.; Huang, X.; Chen, L. The synergic regulation of conductivity and Seebeck coefficient in pure polyaniline by chemically changing the ordered degree of molecular chains. J. Mater. Chem. A 2014, 2, 2634–2640. [Google Scholar] [CrossRef]
- Yao, Q.; Chen, L.; Xu, X.; Wang, C. The high thermoelectric properties of conducting polyaniline with pecial submicron-fibre structure. Chem. Lett. 2005, 34, 522–523. [Google Scholar] [CrossRef]
- Sydulu, B.S.; Palaniappan, S.; Srinivas, P. Nano fibre polyaniline containing long chain and small molecule dopants and carbon composites for supercapacitor. Electrochim. Acta 2013, 95, 251–259. [Google Scholar] [CrossRef]
- Yu, Q.Z.; Shi, M.M.; Deng, M.; Wang, M.; Chen, H.Z. Morphology and conductivity of polyaniline sub-micron fibers prepared by electrospinning. Mater. Sci. Eng. B Adv. Funct. Solid-State Mater. 2008, 150, 70–76. [Google Scholar] [CrossRef]
- Sakurai, S.; Kawamata, Y.; Takahashi, M.; Kobayashi, K. Improved photocurrent of a poly (3,4-ethylenedioxythiophene)-ClO4-/TiO2 thin film-modified counter electrode for dye-sensitized solar cells. J. Oleo Sci. 2011, 60, 639–646. [Google Scholar] [CrossRef] [PubMed]
- Pandey, G.P.; Rastogi, A.C. Synthesis and characterization of pulsed polymerized poly(3,4-ethylenedioxythiophene) electrodes for high-performance electrochemical capacitors. Electrochim. Acta 2013, 87, 158–168. [Google Scholar] [CrossRef]
- Li, J.; Tang, X.; Li, H.; Yan, Y.; Zhang, Q. Synthesis and thermoelectric properties of hydrochloric acid-doped polyaniline. Synth. Met. 2010, 160, 1153–1158. [Google Scholar] [CrossRef]
- Liu, K.; Pang, H.; Zhang, J.; Huang, H.; Liu, Q.; Chu, Y. Synthesis and characterization of a highly stable poly (3,4-ethylenedioxythiophene)-gold nanoparticles composite film and its application to electrochemical dopamine sensors. RSC Adv. 2014, 4, 8415–8420. [Google Scholar] [CrossRef]
- Astratine, L.; Magner, E.; Cassidy, J.; Betts, A. Electrodeposition and characterisation of copolymers based on pyrrole and 3,4-ethylenedioxythiophene in BMIM BF4 using a microcell configuration. Electrochim. Acta 2014, 115, 440–448. [Google Scholar] [CrossRef]
- Hu, C.W.; Lee, K.M.; Vittal, R.; Yang, D.J.; Ho, K.C. A high contrast hybrid electrochromic device containing PEDOT, heptyl viologen, and radical provider TEMPO. J. Electrochem. Soc. 2010, 157, P75–P78. [Google Scholar]
- Yoon, C.; Kim, J.; Sung, H.; Lee, H. Electrical conductivity and thermopower of phosphoric acid doped polyaniline. Synth. Met. 1997, 84, 789–790. [Google Scholar] [CrossRef]
- Michalski, R.; Sikora, A.; Adamus, J.; Marcinek, A. Mechanistic aspects of radiation-induced oligomerization of 3,4-ethylenedioxythiophene in ionic liquids. J. Phys. Chem. A 2010, 114, 11552–11559. [Google Scholar] [CrossRef] [PubMed]
- Levesque, I.; Gao, X.; Klug, D.; Tse, J.; Ratcliffe, C.; Leclerc, M. Highly soluble poly(2,7-carbazolenevinylene) for thermoelectrical applications: From theory to experiment. React. Funct. Polym. 2005, 65, 23–36. [Google Scholar] [CrossRef]
- Lu, B.; Chen, S.; Xu, J.; Zhao, G. Thermoelectric performances of different types of polyselenophene and its copolymers with 3-methylthiophene via electropolymerization. Synth. Met. 2013, 183, 8–15. [Google Scholar] [CrossRef]
- Zuzok, R.; Kaiser, A.; Pukacki, W.; Roth, S. Thermoelectric-power and conductivity of iodine-doped new polyacetylene. J. Chem. Phys. 1991, 95, 1270–1275. [Google Scholar] [CrossRef]
- Bi, K.; Weathers, A.; Matsushita, S.; Pettes, M.T.; Goh, M.; Akagi, K.; Shi, L. Iodine doping effects on the lattice thermal conductivity of oxidized polyacetylene nanofibers. J. Appl. Phys. 2013, 114, 19430–2. [Google Scholar] [CrossRef]
- Shirakawa, H.; Louis, E.; Macdiarmid, A.; Chiang, C.; Heeger, A. Synthesis of electrically conducting organic polymers-halogen derivatives of polyacetylene, (CH)x. J. Chem. Soc. Chem. Commun. 1977. [CrossRef]
- Rahman, S.; Abul-Hamayel, M.; Aleem, B. Electrochemically synthesized polypyrrole films as primer for protective coatings on carbon steel. Surf. Coat. Technol. 2006, 200, 2948–2954. [Google Scholar] [CrossRef]
- Gao, Z.; Yang, W.; Wang, J.; Yan, H.; Yao, Y.; Ma, J.; Wang, B.; Zhang, M.; Liu, L. Electrochemical synthesis of layer-by-layer reduced graphene oxide sheets/polyaniline nanofibers composite and its electrochemical performance. Electrochim. Acta 2013, 91, 185–194. [Google Scholar] [CrossRef]
- Chang, K.C.; Jeng, M.S.; Yang, C.C.; Chou, Y.W.; Wu, S.K.; Thomas, M.A.; Peng, Y.C. The thermoelectric performance of poly(3,4-ethylenedioxythiophene)/poly(4-styrenesulfonate) thin films. J. Electron. Mater. 2009, 38, 1182–1188. [Google Scholar] [CrossRef]
- Yue, R.; Chen, S.; Liu, C.; Lu, B.; Xu, J.; Wang, J.; Liu, G. Synthesis, characterization, and thermoelectric properties of a conducting copolymer of 1,12-bis(carbazolyl)dodecane and thieno[3,2-b]thiophene. J. Solid State Electrochem. 2012, 16, 117–126. [Google Scholar] [CrossRef]
- Scholdt, M.; Do, H.; Lang, J.; Gall, A.; Colsmann, A.; Lemmer, U.; Koenig, J.D.; Winkler, M.; Boettner, H. Organic semiconductors for thermoelectric applications. J. Electron. Mater. 2010, 39, 1589–1592. [Google Scholar] [CrossRef]
- Liu, C.; Jiang, F.; Huang, M.; Yue, R.; Lu, B.; Xu, J.; Liu, G. Thermoelectric performance of poly(3,4-ethylenedioxy-thiophene)/poly(Styrenesulfonate) pellets and films. J. Electron. Mater. 2011, 40, 648–651. [Google Scholar] [CrossRef]
- Kim, G.H.; Shao, L.; Zhang, K.; Pipe, K.P. Engineered doping of organic semiconductors for enhanced thermoelectric efficiency. Nat. Mater. 2013, 12, 719–723. [Google Scholar] [CrossRef] [PubMed]
- Yoon, C.; Kim, J.; Sung, H.; Lee, H. Electrical conductivity and thermopower of phosphoric acid doped polyaniline. Synth. Met. 1997, 84, 789–790. [Google Scholar] [CrossRef]
- Tsai, T.C.; Chang, H.C.; Chen, C.H.; Huang, Y.C.; Whang, W.T. A facile dedoping approach for effectively tuning thermoelectricity and acidity of PEDOT:PSS films. Org. Electron. 2014, 15, 641–645. [Google Scholar] [CrossRef]
- Kong, F.; Liu, C.; Song, H.; Xu, J.; Huang, Y.; Zhu, H.; Wang, J. Effect of solution pH value on thermoelectric performance of freestanding PEDOT:PSS films. Synth. Met. 2013, 185, 31–37. [Google Scholar] [CrossRef]
- Taylor, P.S.; Korugic-Karasz, L.; Wilusz, E.; Lahti, P.M.; Karasz, F.E. Thermoelectric studies of oligophenylenevinylene segmented block copolymers and their blends with MEH-PPV. Synth. Met. 2013, 185, 109–114. [Google Scholar] [CrossRef]
- Shi, H.; Liu, C.; Xu, J.; Song, H.; Lu, B.; Jiang, F.; Zhou, W.; Zhang, G.; Jiang, Q. Facile fabrication of PEDOT:PSS/polythiophenes bilayered nanofilms on pure organic electrodes and their thermoelectric performance. ACS Appl. Mater. Interfaces 2013, 5, 12811–12819. [Google Scholar] [CrossRef] [PubMed]
- Meyyappan, M. Carbon Nanotubes. Science and Applications; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Yu, C.; Kim, Y.S.; Kim, D.; Grunlan, J.C. Thermoelectric behavior of segregated-network polymer nanocomposites. Nano Lett. 2009, 9, 1283. [Google Scholar]
- Kim, D.; Kim, Y.; Choi, K.; Grunlan, J.C.; Yu, C. Improved thermoelectric behavior of nanotube-filled polymer composites with poly(3,4-ethylenedioxythiophene) poly(styrenesulfonate). ACS Nano 2010, 4, 513–523. [Google Scholar] [CrossRef] [PubMed]
- Yu, C.; Choi, K.; Yin, L.; Grunlan, J.C. Light-Weight flexible carbon nanotube based organic composites with large thermoelectric power factors. ACS Nano 2011, 5, 7885–7892. [Google Scholar] [CrossRef] [PubMed]
- Moriarty, G.P.; Briggs, K.; Stevens, B.; Yu, C.; Grunlan, J.C. Fully organic nanocomposites with high thermoelectric power factors by using a dual-stabilizer preparation. Energy Technol. 2013, 1, 265–272. [Google Scholar] [CrossRef]
- Yao, Q.; Chen, L.; Zhang, W.; Liufu, S.; Chen, X. Enhanced thermoelectric performance of single-walled carbon nanotubes/polyaniline hybrid nanocomposites. ACS Nano 2010, 4, 2445–2451. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Gui, X.; Wang, Z.; Li, Z.; Xiang, R.; Wang, K.; Wu, D.; Xia, X.; Zhou, Y.; Wang, Q.; et al. Superlow thermal conductivity 3D carbon nanotube network for thermoelectric applications. ACS Appl. Mater. Interfaces 2012, 4, 81–86. [Google Scholar] [CrossRef]
- Bounioux, C.; Diaz-Chao, P.; Campoy-Quiles, M.; Martin-Gonzalez, M.S.; Goni, A.R.; Yerushalmi-Rozene, R.; Mueller, C. Thermoelectric composites of poly(3-hexylthiophene) and carbon nanotubes with a large power factor. Energy Environ. Sci. 2013, 6, 918–925. [Google Scholar] [CrossRef]
- Rastegaralam, M.; Piao, M.; Kim, G.; Dettlaff-Weglikowska, U.; Roth, S. Influence of chemical treatment on the electrical conductivity and thermopower of expanded graphite foils. Phys. Status Solidi C-Curr. Top. Solid State Phys. 2013, 10, 1183–1187. [Google Scholar]
- Wang, L.; Wang, D.; Zhu, G.; Li, J.; Pan, F. Thermoelectric properties of conducting polyaniline/graphite composites. Mater. Lett. 2011, 65, 1086–1088. [Google Scholar] [CrossRef]
- Du, Y.; Shen, S.Z.; Yang, W.; Donelson, R.; Cai, K.; Casey, P.S. Simultaneous increase in conductivity and Seebeck coefficient in a polyaniline/graphene nanosheets thermoelectric nanocomposite. Synth. Met. 2012, 161, 2688–2692. [Google Scholar] [CrossRef]
- Lu, Y.; Song, Y.; Wang, F. Thermoelectric properties of graphene nanosheets-modified polyaniline hybrid nanocomposites by an in situ chemical polymerization. Mater. Chem. Phys. 2013, 138, 238–244. [Google Scholar] [CrossRef]
- Abad, B.; Alda, I.; Diaz-Chao, P.; Kawakami, H.; Almarza, A.; Amantia, D.; Gutierrez, D.; Aubouy, L.; Martin-Gonzalez, M. Improved power factor of polyaniline nanocomposites with exfoliated graphene nanoplatelets (GNPs). J. Mater. Chem. A 2013, 1, 10450–10457. [Google Scholar] [CrossRef]
- Kim, G.H.; Hwang, D.H.; Woo, S.I. Thermoelectric properties of nanocomposite thin films prepared with poly(3,4-ethylenedioxythiophene) poly(styrenesulfonate) and graphene. Phys. Chem. Chem. Phys. 2012, 14, 3530–3536. [Google Scholar] [CrossRef] [PubMed]
- Culebras, M.; Gomez, C.M.; Cantarero, A. Thermoelectric measurements of PEDOT:PSS/expanded graphite composites. J. Mater. Sci. 2013, 48, 2855–2860. [Google Scholar] [CrossRef]
- Zhang, K.; Zhang, Y.; Wang, S. Enhancing thermoelectric properties of organic composites through hierarchical nanostructures. Sci. Rep. 2013, 3, 3448. [Google Scholar]
- Piao, M.; Kim, G.; Kennedy, G.P.; Roth, S.; Dettlaff-Weglikowska, U. Preparation and characterization of expanded graphite polymer composite films for thermoelectric applications. Phys. Status Solidi B-Basic Solid State Phys. 2013, 250, 2529–2534. [Google Scholar] [CrossRef]
- Gangopadhyay, R.; De, A.; Das, S. Transport properties of polypyrrole-ferric oxide conducting nanocomposites. J. Appl. Phys. 2000, 87, 2363–2371. [Google Scholar]
- Zhao, X.; Hu, S.; Zhao, M.; Zhu, T. Thermoelectric properties of Bi0.5Sb1.5Te polyaniline hybrids prepared by mechanical blending. Mater. Lett. 2002, 52, 147–149. [Google Scholar] [CrossRef]
- Liu, H.; Wang, J.; Hu, X.; Boughton, R.; Zhao, S.; Li, Q.; Jiang, M. Structure and electronic transport properties of polyanilineNaFe4P12 composite. Chem. Phys. Lett. 2002, 352, 185–190. [Google Scholar] [CrossRef]
- Zhang, B.; Sun, J.; Katz, H.E.; Fang, F.; Opila, R.L. Promising thermoelectric properties of commercial PEDOT:PSS materials and their Bi2Te3 Powder Composites. ACS Appl. Mater. Interfaces 2010, 2, 3170–3178. [Google Scholar] [CrossRef] [PubMed]
- Chatterjee, K.; Mitra, M.; Kargupta, K.; Ganguly, S.; Banerjee, D. Synthesis, characterization and enhanced thermoelectric performance of structurally ordered cable-like novel polyaniline-bismuth telluride nanocomposite. Nanotechnology 2013, 24, 215703. [Google Scholar] [CrossRef]
- Song, H.; Liu, C.; Zhu, H.; Kong, F.; Lu, B.; Xu, J.; Wang, J.; Zhao, F. Improved thermoelectric performance of free-standing PEDOT:PSS-Bi2Te3 films with low thermal conductivity. J. Electron. Mater. 2013, 42, 1268–1274. [Google Scholar] [CrossRef]
- Kato, K.; Hagino, H.; Miyazaki, K. Fabrication of Bismuth Telluride Thermoelectric Films Containing Conductive Polymers Using a Printing Method. J. Electron. Mater. 2013, 42, 1313–1318. [Google Scholar] [CrossRef]
- See, K.C.; Feser, J.P.; Chen, C.E.; Majumdar, A.; Urban, J.J.; Segalman, R.A. Water-Processable polymer-nanocrystal hybrids for thermoelectrics. Nano Lett. 2010, 10, 4664–4667. [Google Scholar] [CrossRef] [PubMed]
- Yee, S.K.; Coates, N.E.; Majumdar, A.; Urban, J.J.; Segalman, R.A. Thermoelectric power factor optimization in PEDOT:PSS tellurium nanowire hybrid composites. Phys. Chem. Chem. Phys. 2013, 15, 4024–4032. [Google Scholar] [PubMed]
- Coates, N.E.; Yee, S.K.; McCulloch, B.; See, K.C.; Majumdar, A.; Segalman, R.A.; Urban, J.J. Effect of interfacial properties on polymer-nanocrystal thermoelectric transport. Adv. Mater. 2013, 25, 1629–1633. [Google Scholar] [CrossRef] [PubMed]
- Stanford, M.; Wang, H.; Ivanov, I.; Hu, B. High Seebeck effects from conducting polymer: Poly(3,4-ethylenedioxythiophene): poly(styrenesulfonate) based thin-film device with hybrid metal/polymer/metal architecture. Appl. Phys. Lett. 2012, 101, 173304. [Google Scholar]
- Yoshida, A.; Toshima, N. Gold nanoparticle and gold nanorod embedded PEDOT:PSS thin films as organic thermoelectric materials. J. Electron. Mater. 2014, 43, 1492–1497. [Google Scholar] [CrossRef]
- Kim, B.; Shin, H.; Park, T.; Lim, H.; Kim, E. NIR-Sensitive poly(3,4-ethylenedioxyselenophene) derivativesfortransparentphoto-thermo-electricconverters. Adv. Mater. 2013, 25, 5483–5489. [Google Scholar]
- Song, H.; Liu, C.; Xu, J.; Jiang, Q.; Shi, H. Fabrication of a layered nanostructure PEDOT:PSS/SWCNTs composite and its thermoelectric performance. RSC Adv. 2013, 3, 22065–22071. [Google Scholar] [CrossRef]
- Wang, Q.; Yao, Q.; Chang, J.; Chen, L. Enhanced thermoelectric properties of CNT/PANI composite nanofibers by highly orienting the arrangement of polymer chains. J. Mater. Chem. 2012, 22, 17612–17618. [Google Scholar] [CrossRef]
- Yan, H.; Kou, K. Enhanced thermoelectric properties in polyaniline composites with polyaniline-coated carbon nanotubes. J. Mater. Sci. 2014, 49, 1222–1228. [Google Scholar] [CrossRef]
- Wang, L.; Jia, X.; Wang, D.; Zhu, G.; Li, J. Preparation and thermoelectric properties of polythiophene/multiwalled carbon nanotube composites. Synth. Met. 2013, 181, 79–85. [Google Scholar] [CrossRef]
- He, M.; Ge, J.; Lin, Z.; Feng, X.; Wang, X.; Lu, H.; Yang, Y.; Qiu, F. Thermopower enhancement in conducting polymer nanocomposites via carrier energy scattering at the organic-inorganic semiconductor interface. Energy Environ. Sci. 2012, 5, 8351–8358. [Google Scholar] [CrossRef]
- Wang, D.; Shi, W.; Chen, J.; Xi, J.; Shuai, Z. Modeling thermoelectric transport in organic materials. Phys. Chem. Chem. Phys. 2012, 14, 16505–16520. [Google Scholar] [CrossRef] [PubMed]
- D’Agosta, R. Towards a dynamical approach to the calculation of the figure of merit of thermoelectric nanoscale devices. Phys. Chem. Chem. Phys. 2012, 15, 1758–1765. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Cruz, L.; Phyllips, P. Granular-rod model for electronic conduction in polyaniline. Phys. Rev. B 1993, 47, 1840–1845. [Google Scholar] [CrossRef]
- Wang, X.; Shapiro, B.; Smela, E. Development of a model for charge transport in conjugated polymers. J. Phys. Chem. C 2009, 113, 382–401. [Google Scholar] [CrossRef]
- Wang, Z.H.; Ray, A.; MacDiarmid, A.G.; Epstein, A.J. Electron localization and charge transport in poly(o-toluidine): A model polyaniline derivetive. Phys. Rev. B 1991, 43, 4373–4384. [Google Scholar] [CrossRef]
- Desai, T.; Keblinski, P.; Kumar, S.K. Molecular dynamics simulation of polymer transport in nanocomposites. J. Chem. Phys. 2005, 122, 134910. [Google Scholar] [CrossRef]
- Pal, S.; Balasubramanian, G.; Puri, I.K. Reducing thermal transport in electrically conducting polymers: Effects of ordered mixing of polymer chains. Appl. Phys. Lett. 2013, 102, 023109. [Google Scholar] [CrossRef]
- Savin, A.V.; Kosevich, Y.A.; Cantarero, A. Semiquantum molecular dynamics simulation of thermal properties and heat transport in low-dimensional nanostructures. Phys. Rev. B 2012, 86, 064305. [Google Scholar] [CrossRef]
- Hafner, J. Ab-initio simulations of materials using VASP: Density-functional theory and beyond. J. Comp. Chem. 2008, 29, 2044–2078. [Google Scholar] [CrossRef]
- Holzwarth, N.; Matthews, G.; Dunning, R.; Tackett, A.; Zeng, Y. Comparison of the projector augmented-wave, pseudopotential, and linearized augmented-plane-wave formalisms for density-functional calculations of solids. Phys. Rev. B 1997, 55, 2005–2017. [Google Scholar] [CrossRef]
- Perdew, J.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
- Bardeen, J.; Shockely, W. Deformation potentials and mobilities in nonpolar crystals. Phys. Rev. B 1950, 80, 72–80. [Google Scholar]
- Wang, J.; Wolf, R.M.; Caldwell, J.; Kollman, P.; Case, D. Development and testing of a general amber force field. J. Comp. Chem. 2004, 25, 1157–1174. [Google Scholar] [CrossRef]
- Jorgensen, W.L.; Madura, J.D.; Swenson, C.J. Optimized intermolecular potential functions for liquid hydrocarbons. J. Am. Chem. Soc. 1984, 106, 6638–6646. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comp. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Wang, D.; Tang, L.; Long, M.; Shuai, Z. Anisotropic thermal transport in organic molecular crystals from nonequilibrium molecular dynamics simulations. J. Phys. Chem. C 2011, 115, 5940–5946. [Google Scholar] [CrossRef]
- Wang, J.S.; Wang, J.; Lue, J.T. Quantum thermal transport in nanostructures. Eur. Phys. J. B 2008, 62, 381–404. [Google Scholar] [CrossRef]
- Yand, K.; Cahangirov, S.; Cantarero, A.; Rubio, A.; D’Agosta, R. Thermoelectric properties of atomically thin silicene and germanene nanostructures. Phys. Rev. B 2014, 89, 125403. [Google Scholar]
- Mao, R.; Kong, B.D.; Kim, K.W.; Jayasekera, T.; Calzolari, A.; Buongiorno Nardelli, M. Phonon engineering in nanostructures: Controlling interfacial thermal resistance in multilayer-graphene/dielectric heterojunctions. Appl. Phys. Lett. 2012, 101, 113111. [Google Scholar]
- Calzolari, A.; Jayasekera, T.; Kim, K.W.; Nardelli, M.B. Ab initio thermal transport properties of nanostructures form density functional perturbation theory. J. Phys. Cond. Matter 2012, 24, 492204. [Google Scholar] [CrossRef]
- Pizzi, G.; Volja, D.; Krzinsky, B.; Fornari, M.; Marzari, N. BOLTZ WANN: A code for the evaluation of thermoelectric and electronic transport properties with a mxiamally-localized Wannier function basis. Comp. Phys. Commun. 2014, 185, 422–429, and references therein. [Google Scholar] [CrossRef]
- Bao, W.S.; Meguid, S.A.; Zhu, Z.H.; Weng, G.J. Tunneling resistance and its efffect on the electrical conductivity of carbon nanotube nanocomposites. J. Appl. Phys. 2012, 111, 093726. [Google Scholar] [CrossRef]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Culebras, M.; Gómez, C.M.; Cantarero, A. Review on Polymers for Thermoelectric Applications. Materials 2014, 7, 6701-6732. https://doi.org/10.3390/ma7096701
Culebras M, Gómez CM, Cantarero A. Review on Polymers for Thermoelectric Applications. Materials. 2014; 7(9):6701-6732. https://doi.org/10.3390/ma7096701
Chicago/Turabian StyleCulebras, Mario, Clara M. Gómez, and Andrés Cantarero. 2014. "Review on Polymers for Thermoelectric Applications" Materials 7, no. 9: 6701-6732. https://doi.org/10.3390/ma7096701
APA StyleCulebras, M., Gómez, C. M., & Cantarero, A. (2014). Review on Polymers for Thermoelectric Applications. Materials, 7(9), 6701-6732. https://doi.org/10.3390/ma7096701






