1. Introduction
In recent years, the study of microwave absorbing materials has attracted much attention for electromagnetic interference (EMI) shielding and electromagnetic (EM) wave absorbing applications, for both commercial and defense purposes [
1,
2,
3]. Traditional spinel-type ferrites have been extensively studied, however they have some disadvantages such as low permeability, caused by Snoek’s limit, and large density hinder their further development [
4]. On the other hand, dielectric absorbers have potential as microwave absorbents due to advantages such as low density, controllable dielectric loss ability, and so on [
5]. However, compared with ferrite materials, dielectric fillers such as carbon fiber and multi-walled carbon nanotubes are used to control complex permittivity only [
6]. The complex permeability and permittivity of dielectric dissipation materials are out of balance, and their impedance matching characteristic is poor [
7]. Recently, many studies have been carried out to investigate the complementary behavior of magnetic or dielectric microwave absorber coatings by mixing multi-walled carbon nanotubes or metallic magnetic materials [
8,
9]. Several groups have reported excellent microwave absorption properties of heterogeneously structured nanocomposites, such as Fe
3O
4-poly(3,4-ethylenedoxythiophene) (PEDOT) microspheres [
10], hierarchical magnetic yolk/shell microspheres [
11], nanocomposites,
etc. These heterogeneous structures exhibit interesting chemistry as well as effective interfaces and material-dependent properties, endowing such structures with enhanced properties, or satisfactory synergistic effects for microwave absorption enhancement. However, up until now very little attention has been directed toward the magnetic particle-filled ordered mesoporous carbon materials for microwave absorption applications.
The purpose of this study was to investigate the electromagnetic and microwave absorption properties of hybrid nanorods consisting of ordered, mesoporous carbon (OMC) and α-Fe2O3, which may adjust the dielectric properties of α-Fe2O3and OMC and contribute to the microwave absorption properties. Most interestingly, it exhibited a broader absorption bandwidth and lighter weight in the studied range of 2–18 GHz than the as-synthesized dendritic α-Fe2O3 micro-pines. It was found that a multi-loss combination in the unique α-Fe2O3/OMC hybrid structures contributed to enhanced microwave absorption.
2. Results and Discussion
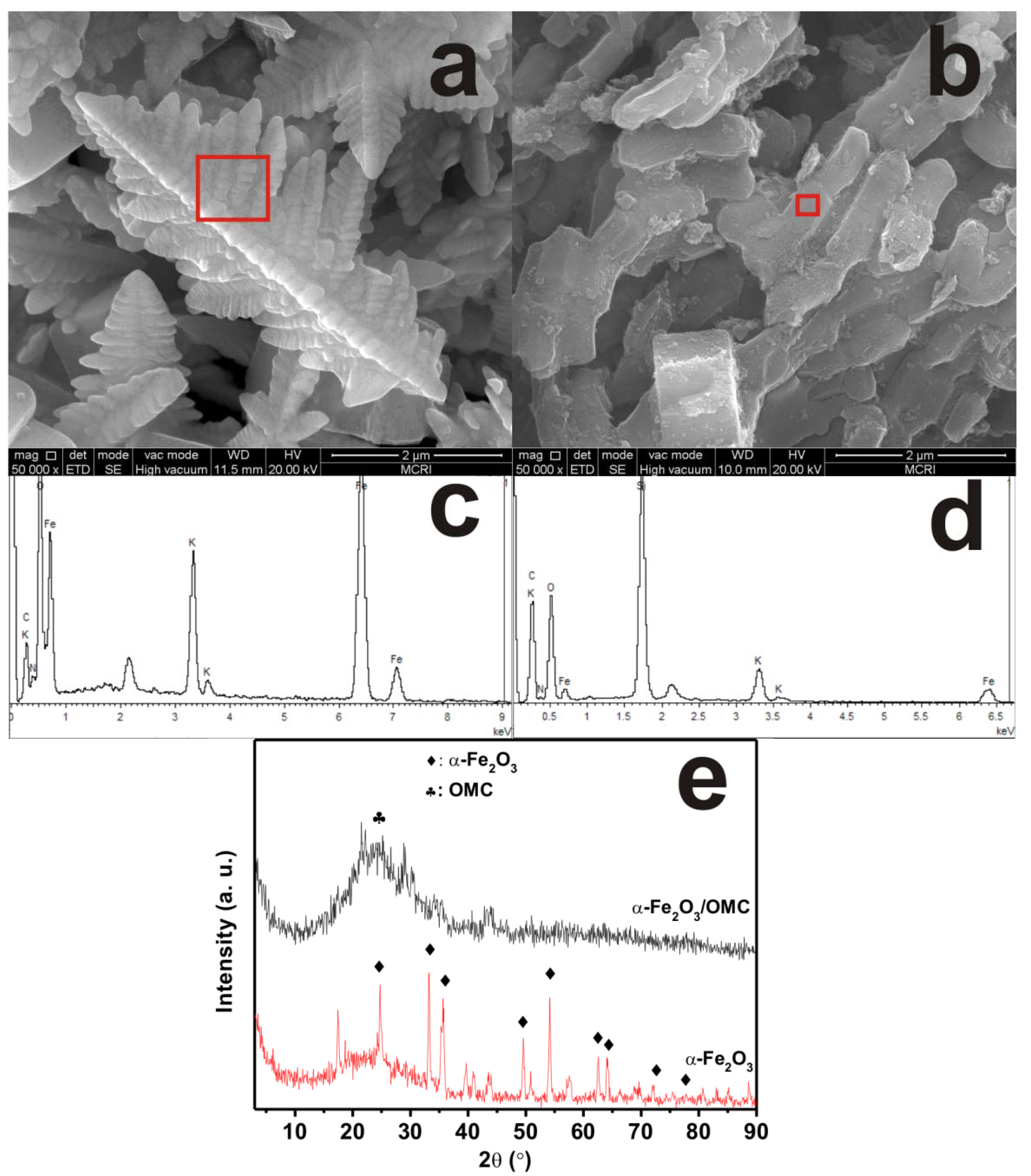
Representative SEM images of the as-synthesized products are shown in
Figure 1a,b respectively. It can be clearly seen that dendritic Fe
2O
3 micropines can be formed by using a facial hydrothermal reaction at low temperatures [
12]. As shown in
Figure 1a, the high magnification image of a single dendrite shows a hierarchical structure with tertiary branches. The lengths of the dendrite trunks are 3–5 μm, and those of the branch trunk range from 50 nm to 1 μm, which is very consistent with the report of Sun
et al. [
12]. When OMC is added to the solution of 0.1 mol L
−1 K
3[Fe(CN)
6] in a Teflon-sealed autoclave, Fe
2O
3-filled OMC structures are obtained, as shown in
Figure 1b. Compared with the electron microscopy of undoped OMC [
13], it is found that the Fe
2O
3-filled OMC structures are relatively thick, suggesting that Fe
2O
3 nanoparticles are mostly embedded in the carbon walls of OMC.
Figure 1c,d show the main peaks of O, Fe, and C elements in the energy dispersive spectroscopy (EDS) spectrum, indicating that the nanorods contain a Fe
2O
3 component.
Figure 1e shows the XRD pattern of the dendritic Fe
2O
3 micropines, in which all diffraction peaks indicated by Miller indices can be indexed to α-Fe
2O
3. There are also peaks which are not well defined. These peaks may be assigned to the intermediate products when preparing dendritic Fe
2O
3 micropines, which would not contribute to the absorption properties of the composites. It suggests that α-Fe
2O
3 dendrites are highly crystalline. However, the α-Fe
2O
3-filled OMC nanocomposites are relatively amorphous, exhibiting weak diffraction peaks for α-Fe
2O
3 in the XRD pattern, expect for a diffraction hump of carbon around 15°–35°.
Figure 1.
Structural characterizations of dendritic α-Fe2O3 micropines and α-Fe2O3/OMC nanorods: (a,b) scanning electron microscope (SEM) images; (c,d) energy dispersive spectroscopy (EDS) spectrum; and (e) X-ray diffraction on a diffractometer (XRD) patterns.
Figure 1.
Structural characterizations of dendritic α-Fe2O3 micropines and α-Fe2O3/OMC nanorods: (a,b) scanning electron microscope (SEM) images; (c,d) energy dispersive spectroscopy (EDS) spectrum; and (e) X-ray diffraction on a diffractometer (XRD) patterns.
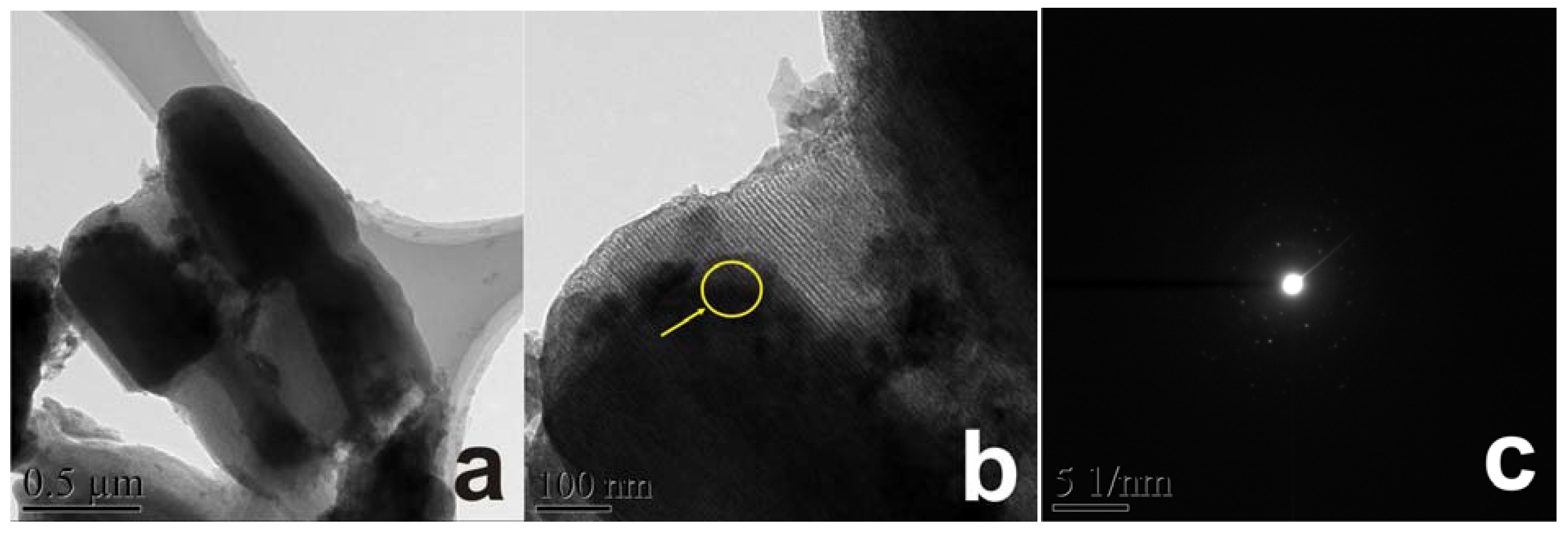
The morphology of as-prepared α-Fe
2O
3/OMC hybrid structure was investigated in detail by TEM, and shown in
Figure 2.
Figure 2a shows a low magnification TEM micrograph of α-Fe
2O
3/OMC hybrid nanorods, in which all the nanorods seem to have a core-shell structure. However, the α-Fe
2O
3 core regime is formed by filling the mesopores of OMC with α-Fe
2O
3 nanoparticles. The formation of the OMC shell may have been caused by not completely filling the α-Fe
2O
3 nanoparticles. Strictly, it is not really a core-shell structure. As shown in
Figure 2b, the α-Fe
2O
3 appears black and OMC is light colored in the image, because hematite has a higher mass–thickness contrast. The bright dot patterns from SAED of the sample given in
Figure 2c can be well-indexed to the crystalline phase of hematite.
Figure 2.
(a,b) transmission electron microscope (TEM) images and (c) SAED of α-Fe2O3/OMC nanorods.
Figure 2.
(a,b) transmission electron microscope (TEM) images and (c) SAED of α-Fe2O3/OMC nanorods.
The electromagnetic (EM) parameters (relative complex permittivity,
ε =
ε′ −
jε′′ and relative complex permeability,
μr =
μ′ −
jμ′′) of the wax composites containing 50 wt % of the as-synthesized samples were measured at room temperature.
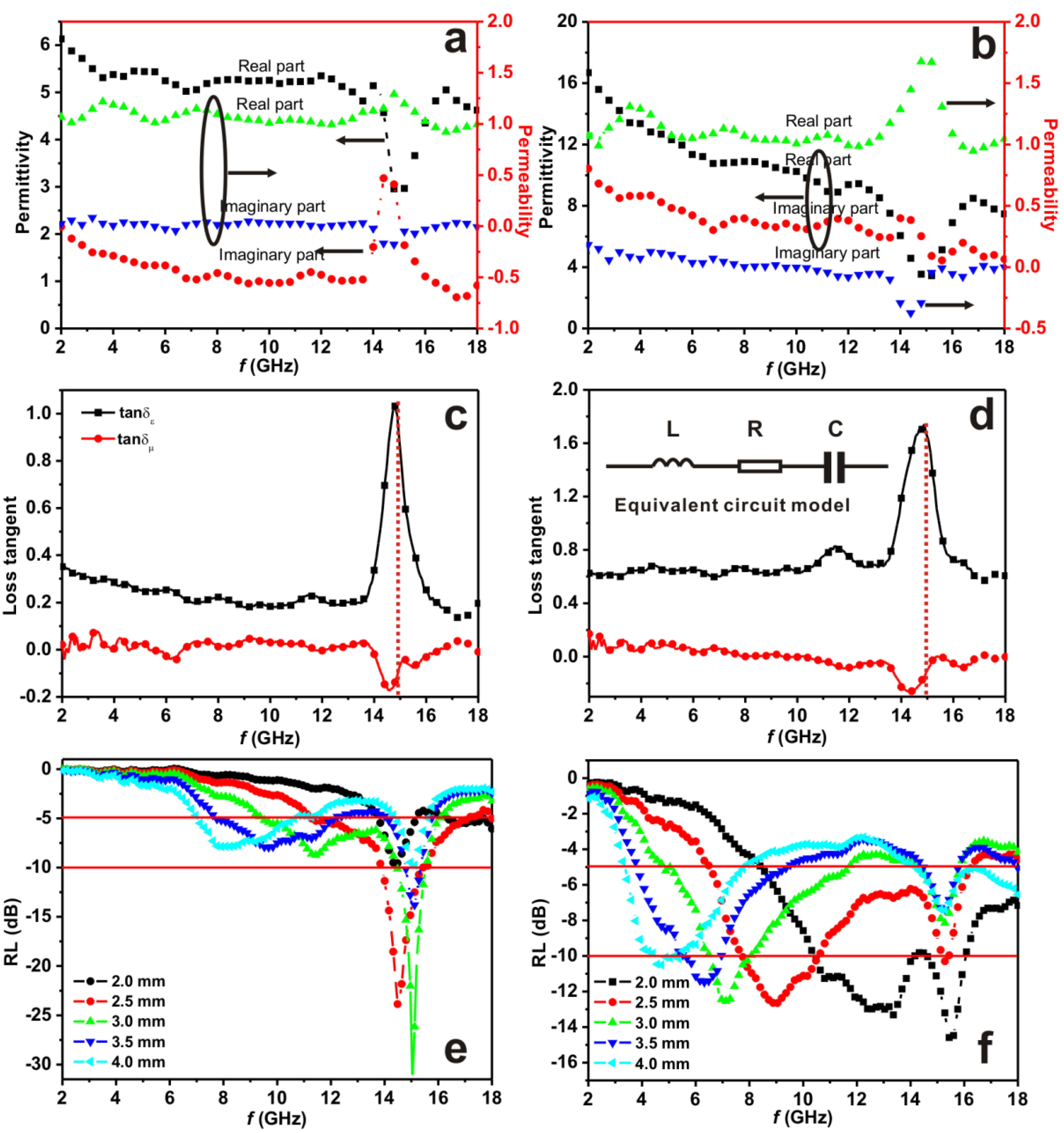
Figure 3a,b show the measured
εr and
μr in the range of 2–18 GHz for the two samples. The
ε′ and
ε′′ values of α-Fe
2O
3 microdendrites are in the range 3–6 and 0.5–3 over a frequency region of 2–18 GHz. However, those values are much lower than those of α-Fe
2O
3/OMC hybrid nanorods (4–17 for
ε′ and 4.5–11.5 for
ε′′). It suggests that α-Fe
2O
3/OMC hybrid nanorods have a strong dielectric loss when exposed to EM waves. The dielectric loss tangent (
) of the two samples is shown in
Figure 3c,d. The
values of α-Fe
2O
3/OMC hybrid nanorods larger than 0.6 are distributed between 2 and 18 GHz and exhibit a peak value of 1.7 at 15 GHz, whereas the values for α-Fe
2O
3 microdendrites are in the range 0.2–0.4 over the frequency range 2–14 GHz, indicating a strong dielectric resonance at the high frequency range in the case of α-Fe
2O
3/OMC hybrid nanorods. In general, high dielectric loss results from the inherent physical properties of OMC and their special heterogeneous structures. Ordered mesoporous carbon (OMC) materials have large surface areas, so the number of surface atoms with unsaturated bonds is greatly increased, resulting in an increase of dipoles. Consequently, the dipole polarizations could contribute to dielectric loss. Furthermore the microstructure of α-Fe
2O
3 nanoparticles filling a carbon matrix would provide additional interfaces around the nanoparticles, and the interfacial polarization associated with relaxation could give rise to dielectric loss as well. The above two dielectric loss aspects can be confirmed by the
Cole-Cole semicircle, as shown in
Figure 2S(a) and
Figure 2S(b) [
13,
14,
15], because the
Cole-Cole semicircle is a typical feature of dielectric relaxation. In addition, because of the addition of the conductive material OMC, the increase in electric conductivity of the α-Fe
2O
3/OMC hybrid nanorods results in an enhanced conductance loss, as shown in
Figure 2S(a) and
Figure 2S(b) (red dashed lines) [
16,
17].
Figure 3.
(
a,
b) relative complex permittivity and permeability; (
c,
d) dielectric and magnetic tangent loss; (
e,
f) reflection loss of α-Fe
2O
3 microdendrites and α-Fe
2O
3/OMC nanorods respectively. The inset in
Figure 3d is a
LRC equivalent circuit model.
Figure 3.
(
a,
b) relative complex permittivity and permeability; (
c,
d) dielectric and magnetic tangent loss; (
e,
f) reflection loss of α-Fe
2O
3 microdendrites and α-Fe
2O
3/OMC nanorods respectively. The inset in
Figure 3d is a
LRC equivalent circuit model.
The
μ′ and
μ′′ values of the two samples (
Figure 3a,b) indicate that
μ′ is close to one, while the
μ′′ is almost equal to zero in the frequency range 2–18 GHz, expect for a resonant frequency at around 15 GHz. Moreover, the
μ′′ value is negative at this resonant frequency. Considerable attempts have been made to obtain an exact solution of the phenomenon that causes the values of permeability
μ′′ to be negative at some frequencies. Chiu [
18] has pointed out that it is meaningless and may be due to noise, although we have previously explained the phenomenon as the outward radiation of magnetic energy, resulting in a conversion of the incident wave to other forms of energy [
13,
14,
15]. So, in the present work we consider that this phenomenon may be related to the eddy currents on the surface of α-Fe
2O
3/OMC structures and α-Fe
2O
3 dendrites which can produce an induced magnetic field, and in turn radiate EM waves, leading to an increase in the total magnetic energy and hence a negative
μ′′ in the measured frequency range [
15]. According to the references [
13,
14,
15], if the values of
is kept constant while the frequency is varied, the magnetic loss only results from eddy current loss. From
Figure 2S(c) and
Figure 2S(d) it can be found that an eddy current may occur on the surface of α-Fe
2O
3/OMC composites. The magnetic loss tangent (
) of the two samples is simply the inverse to that of
, as shown in
Figure 3c,d, which can be explained by the
LRC equivalent circuit model (see the inset in
Figure 3d), where
L,
R, and
C are the inductance, resistance and capacitance respectively. In particular, the eddy current effect regarded as inductance, the dielectric relaxation polarization playing a role as capacitance, and the conductance loss acted as resistance all contribute to the
LRC equivalent circuit model. The inverse change trend is attributed to the capacitance leading or lagging behind the inductance by an angle of 90° [
15].
On the basis of transmission line theory [
19] the reflection loss (RL) can be calculated; the results are shown in
Figure 3e,f. It can be seen clearly that the α-Fe
2O
3/OMC hybrid nanorods with a coating layer thickness of 2.0 mm exhibit a broader absorption bandwidth than the α-Fe
2O
3 microdendrites, corresponding to an RL below −10 dB with the same thickness, which can reach up to 6.0 GHz (from 10.5 GHz to 16.5 GHz). It is also noted that the absorption peak value of α-Fe
2O
3 microdendrites is much higher than that of the α-Fe
2O
3/OMC hybrid nanorods, but its absorption bandwidth is too narrow to be widely applied.
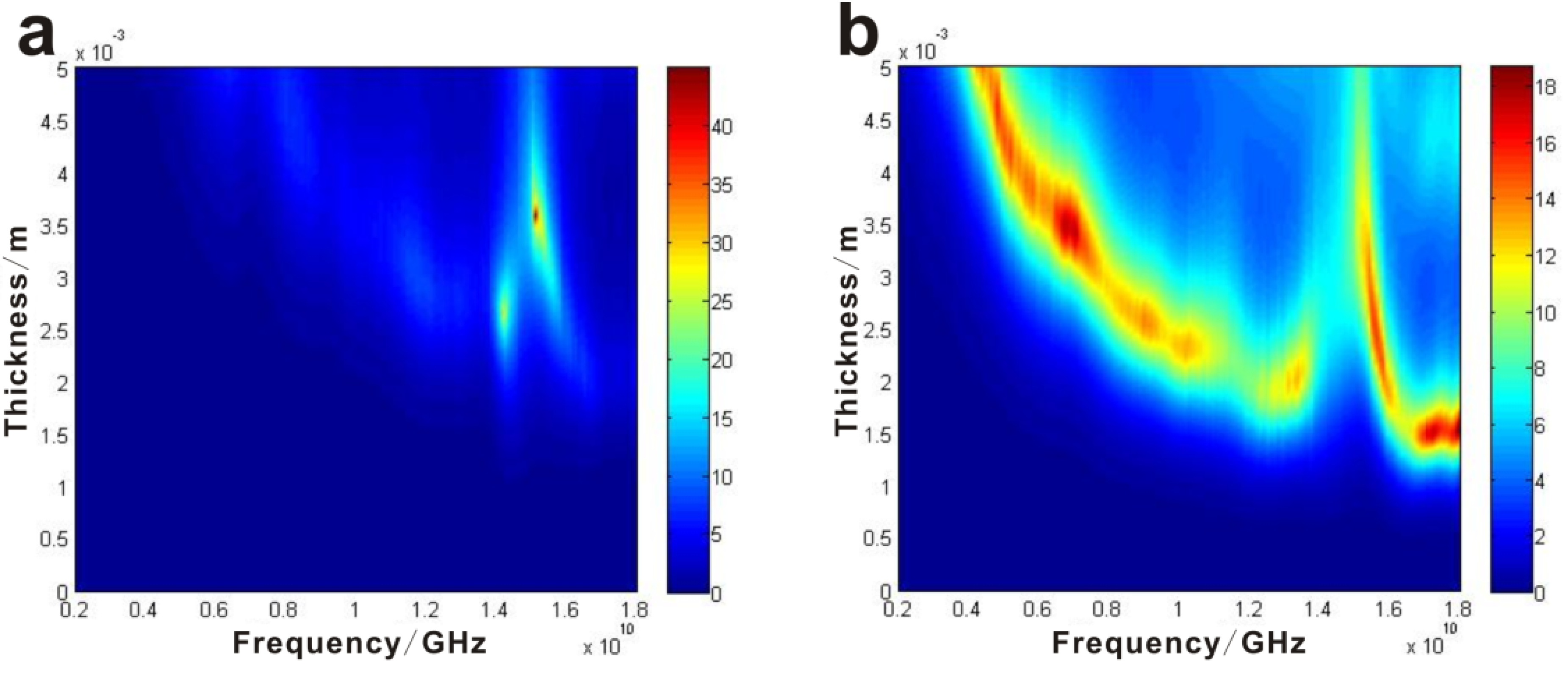
Figure 4a,b show the color map of the reflection loss values calculated from the measured EM parameters of nanocomposites. It is obvious that for α-Fe
2O
3/OMC hybrid nanorods, RL exceeds −10 dB in the range of 4–18 GHz for absorber thicknesses of 1.5–5.0 mm. Compared with the recent representative nanocomposites, the as-synthesized α-Fe
2O
3/OMC hybrid nanorods present a similar microwave absorption performance with thin thickness and broad absorption range, as shown in
Table 1. However, we report a novel synthetic approach that can be used to prepare magnetic particle-filled ordered mesoporous carbon materials for microwave absorption application. The enhanced microwave properties of the α-Fe
2O
3/OMC hybrid nanorods are attributed to a major dielectric loss and a minor eddy current loss, leading to the microwave absorption enhancement.
Figure 4.
Reflection loss map of (a) α-Fe2O3 microdendrites and (b) α-Fe2O3/OMC nanorods.
Figure 4.
Reflection loss map of (a) α-Fe2O3 microdendrites and (b) α-Fe2O3/OMC nanorods.
Table 1.
Microwave absorption performance of some representative nanocomposites.
Table 1.
Microwave absorption performance of some representative nanocomposites.
| Samples | Minimum RL value (dB) | Frequency range (GHz) (RL < −10 dB) | dm (mm) (RL < −10 dB) | Percentage (wt % or vol %) | References |
|---|
| Fe3O4/PEDOT | −30 | ~8–13 | 4 mm | 20 (vol %) | [10] |
| Porous Fe3O4/carbon | −27.9 | ~13–18 | 2 mm | 55 (wt %) | [16] |
| α-Fe2O3 | −23.9 | 13.8–15.5 | 2.5 mm | 50 (wt %) | this work |
| α-Fe2O3/OMC | −15.0 | 10.5–16.5 | 2 mm | 50 (wt %) | this work |
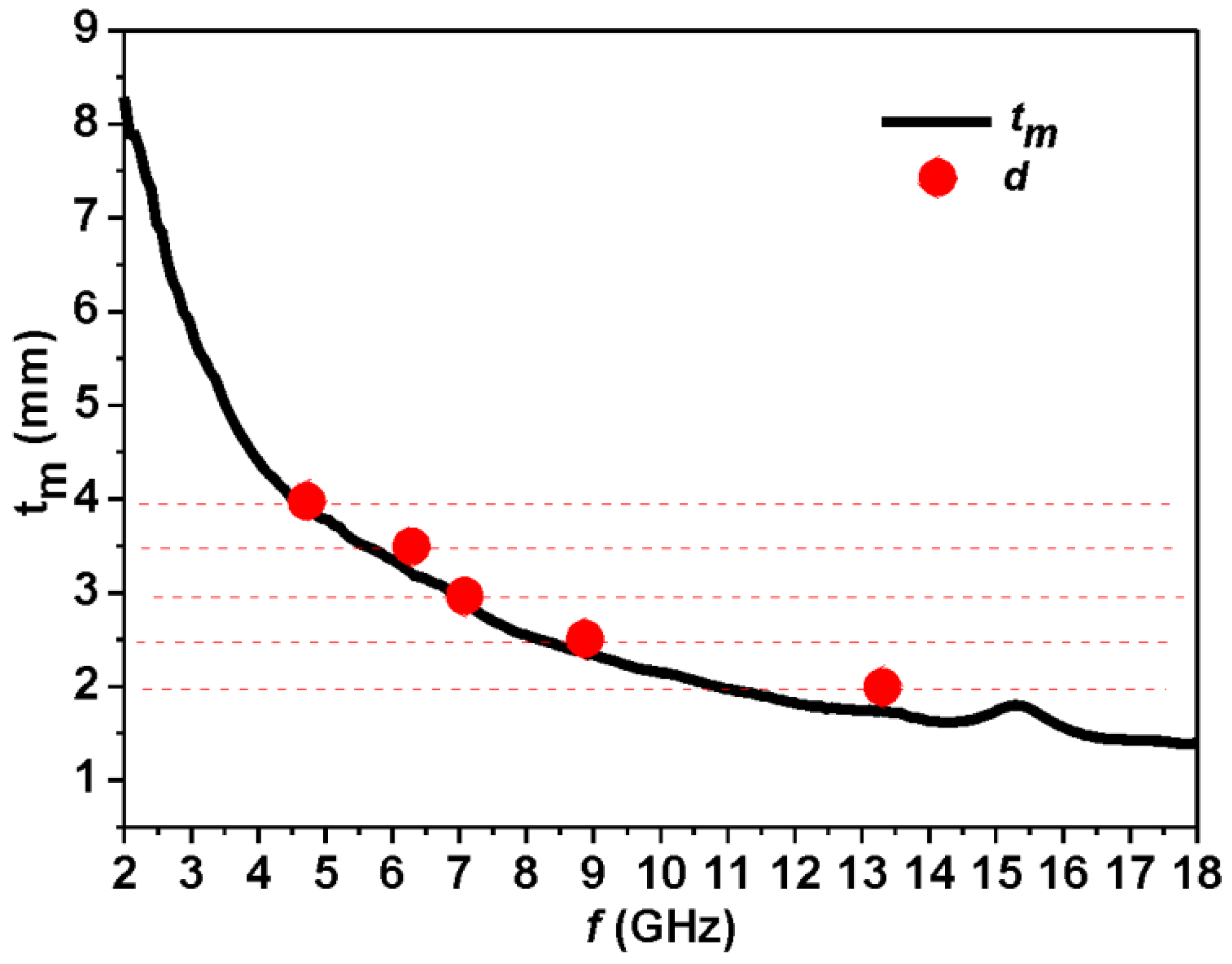
Besides the major dielectric loss, electromagnetic waves could be absorbed via a so-called “geometrical effect” [
17]. The “geometrical effect” means that when the thickness of absorber (
tm) satisfies the following Equation (1), the reflected waves are totally canceled in the air-absorber interface:
where
is the wavelength in the absorber at some specific frequency,
fm;
and
are the moduli of
and
respectively; and
is the wavelength in free space. The calculated frequency dependence of
tm is shown in
Figure 5 (black line) for the α-Fe
2O
3/OMC hybrid nanorods sample. The thickness (denoted as
d)—corresponding to the minimum RL in
Figure 4f—is also plotted in
Figure 5 with red circles. The calculated thickness
tm is consistent with
d, which implies that the corresponding
fm and the matching thickness
d is coincident with the quarter wavelength matching condition. Therefore, the above calculations justify that the selective absorption properties of the α-Fe
2O
3/OMC hybrid nanorods at certain frequencies is due to the geometric effect.
Figure 5.
The frequency dependence of matching thickness d and calculated thickness tm for α-Fe2O3/OMC hybrid nanorods.
Figure 5.
The frequency dependence of matching thickness d and calculated thickness tm for α-Fe2O3/OMC hybrid nanorods.