An Innovative Tube Hydro-Joining Process Combining Piercing, Hole Flanging and Nut Inlaying
Abstract
1. Introduction
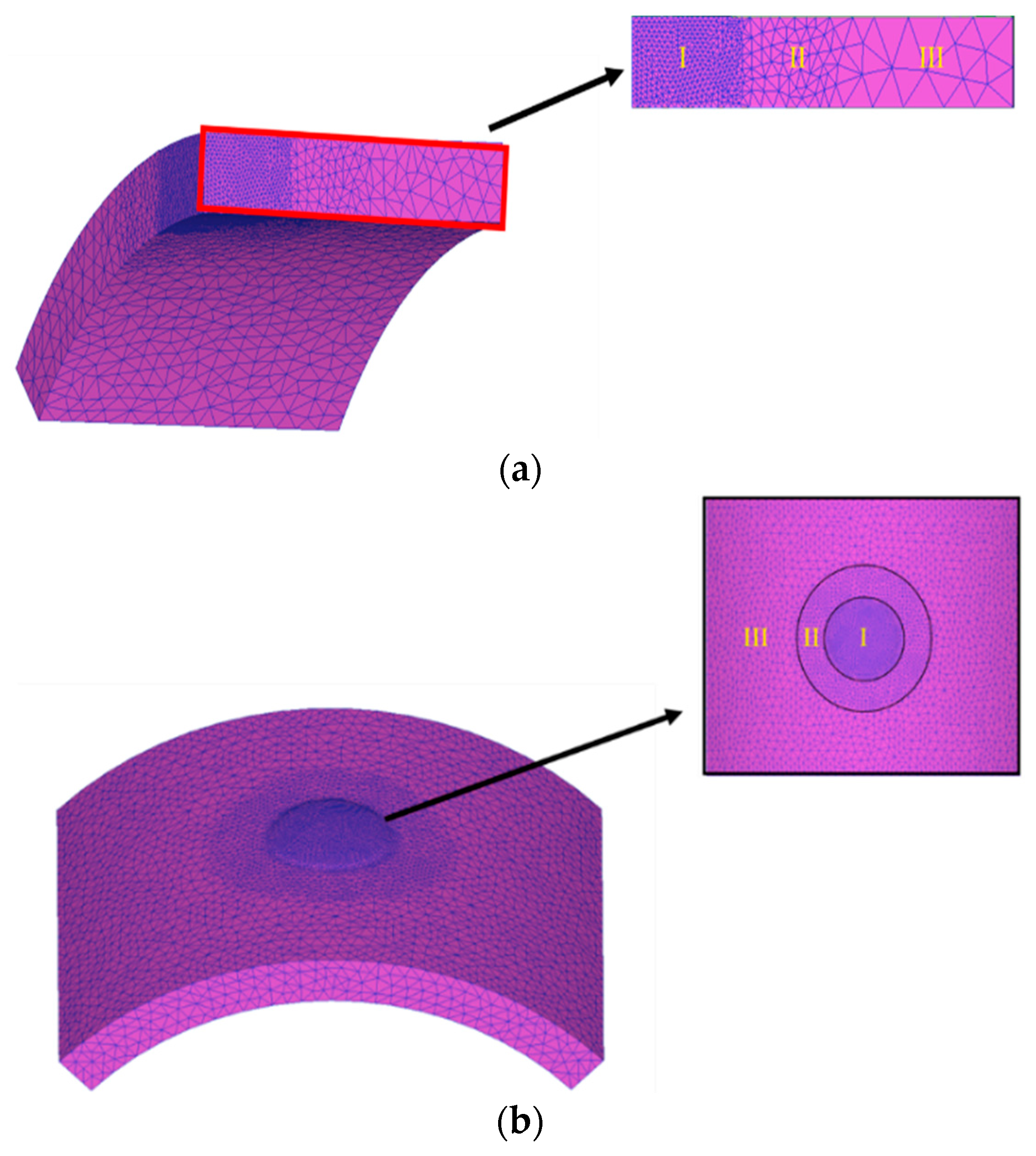
2. Finite Element Simulation Setup
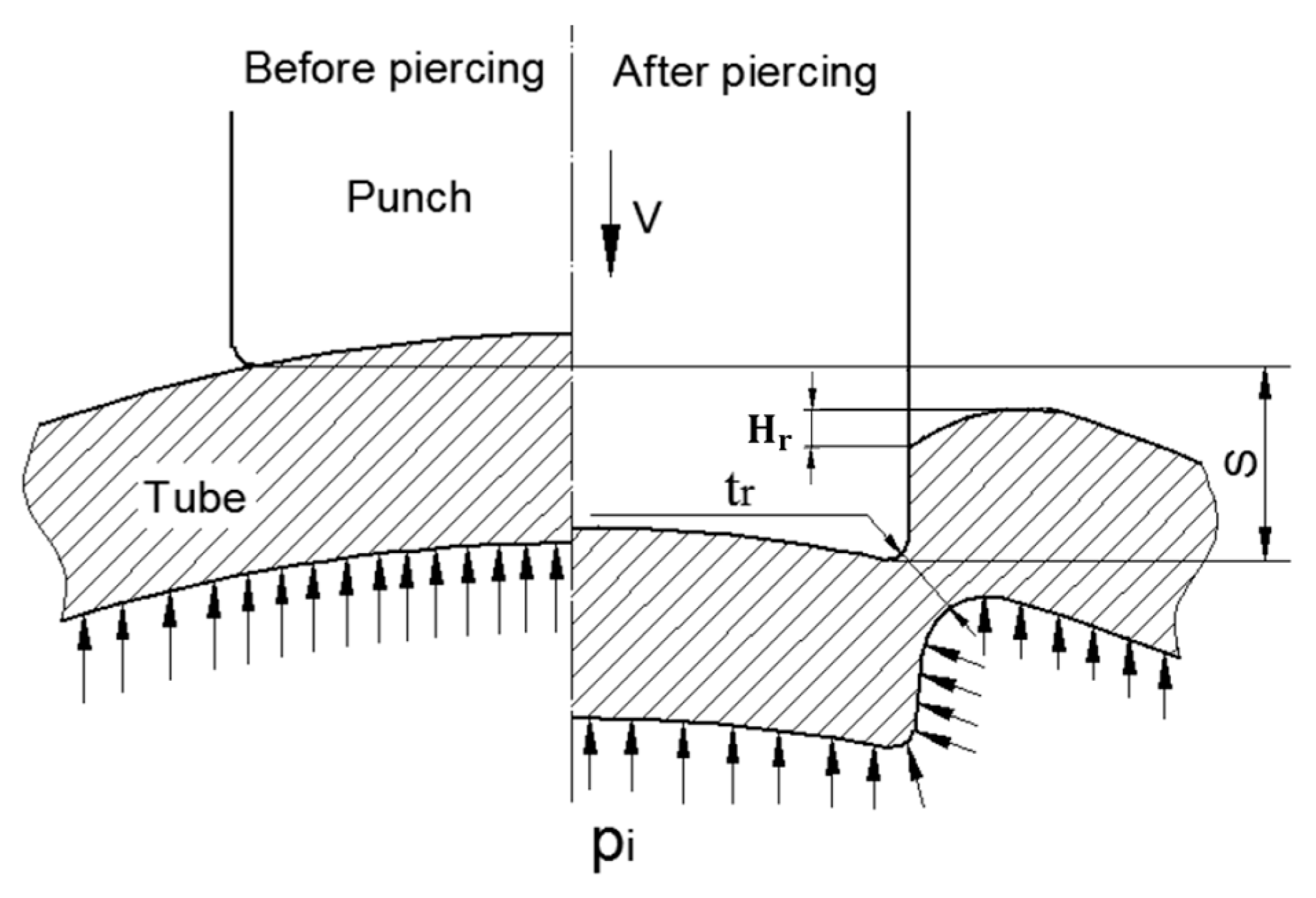
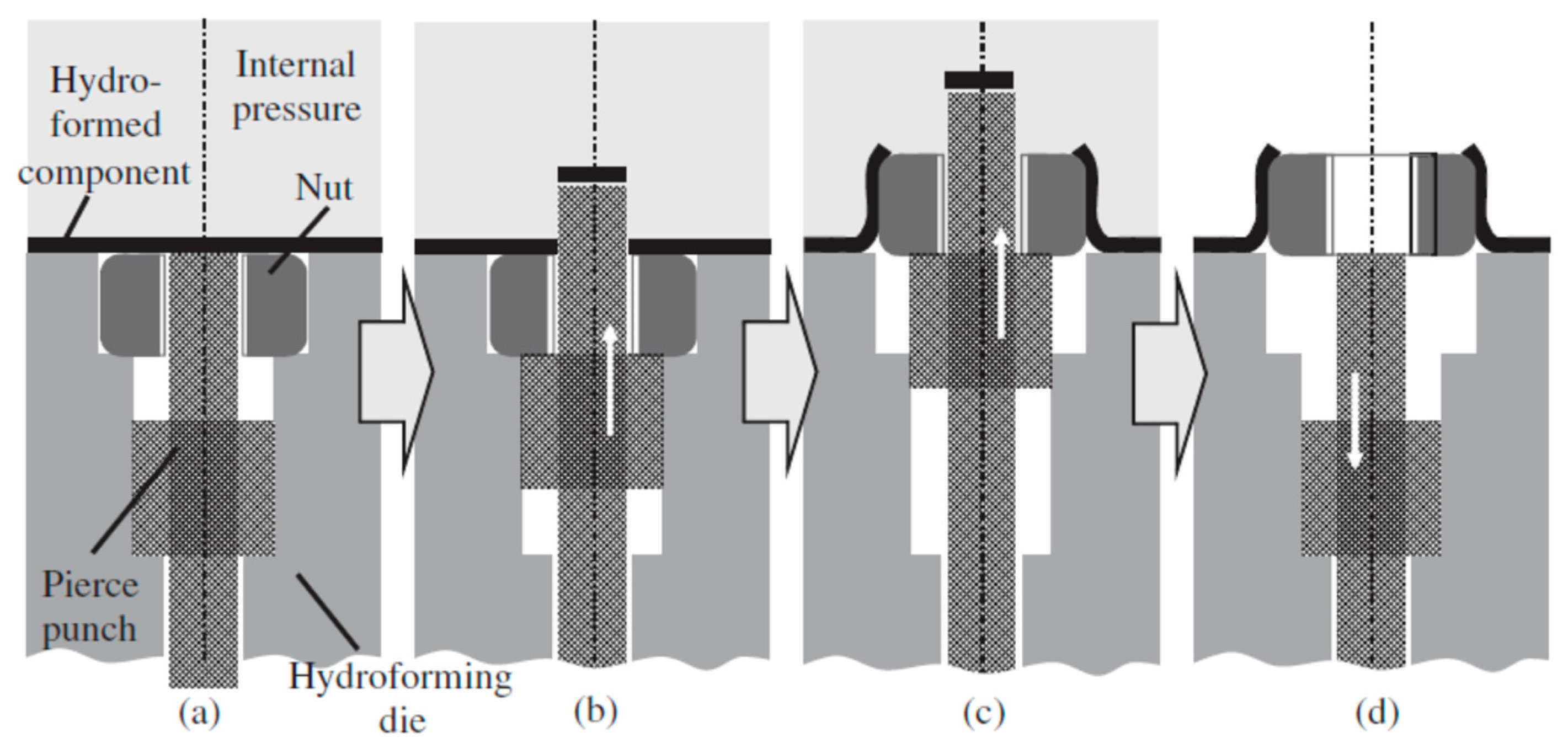
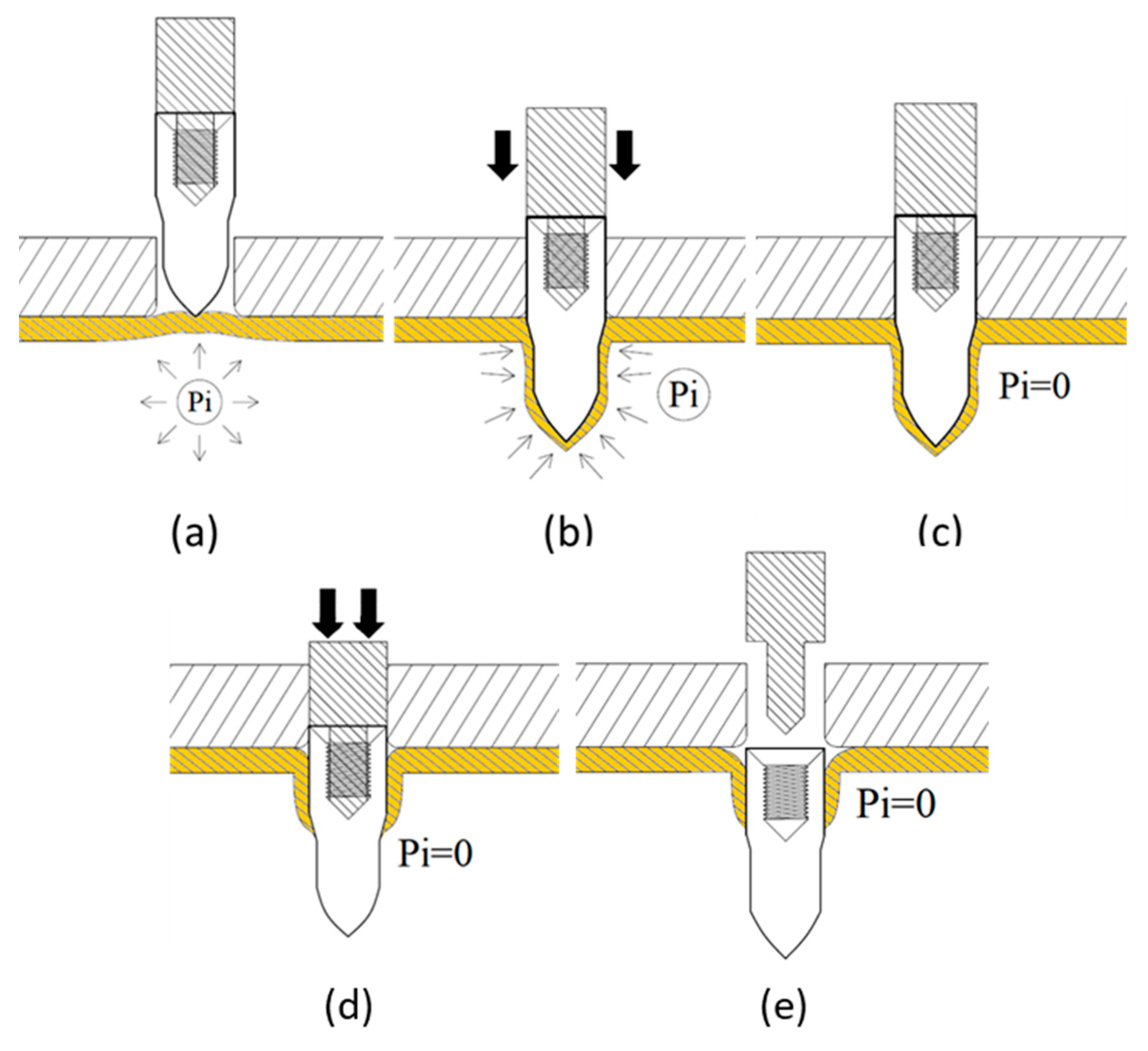
2.1. Hydro-Piercing and Nut Inlaying
2.2. Simulation Conditions
3. Finite Element Simulation Results and Discussion
3.1. Forming Parameter Settings
3.2. Effects of Nut Fit Zone Parameter Changes on Pull Out Load
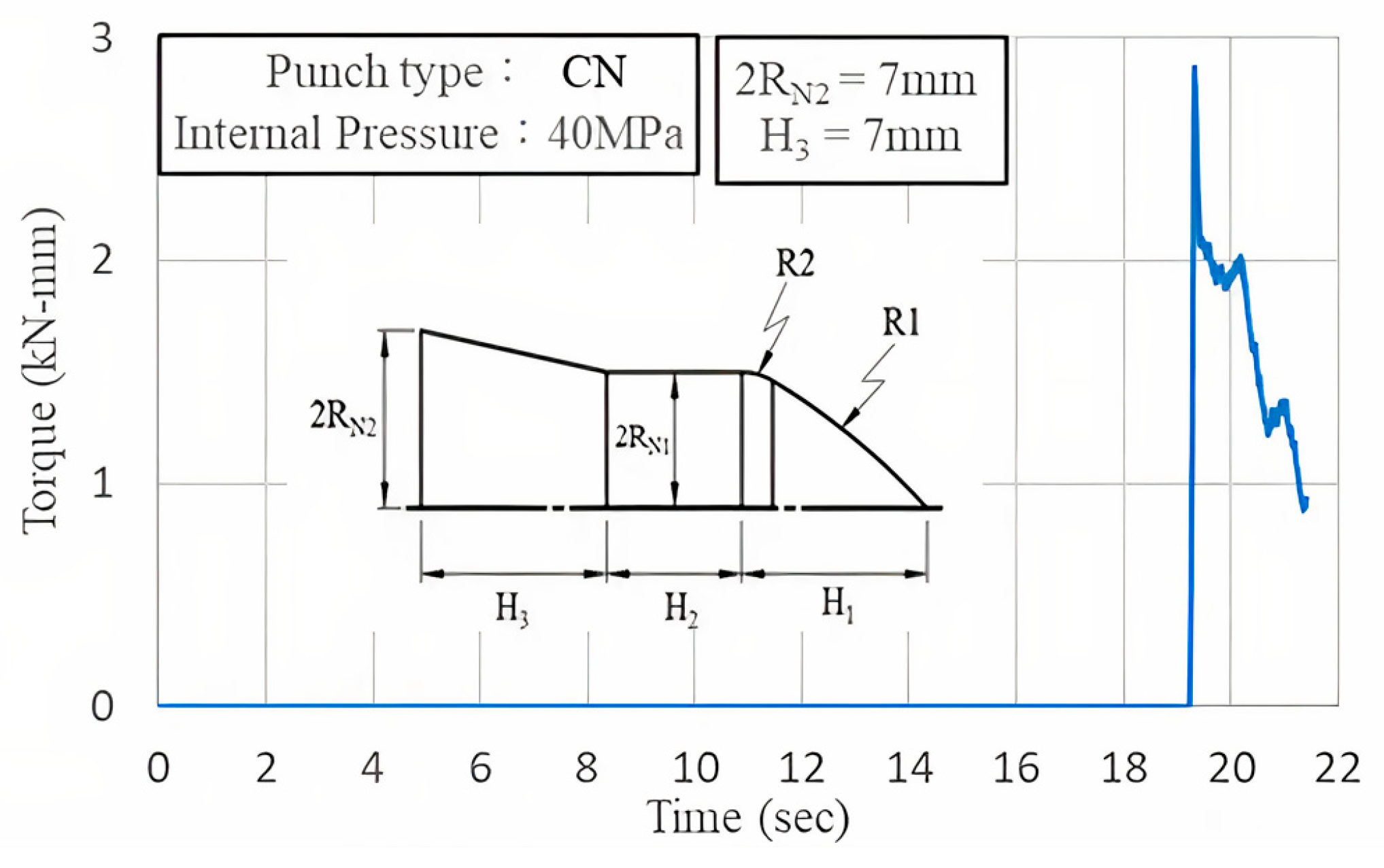
3.3. Torque Analyses and Discussion
4. Nut Hydro-Joining Experiments
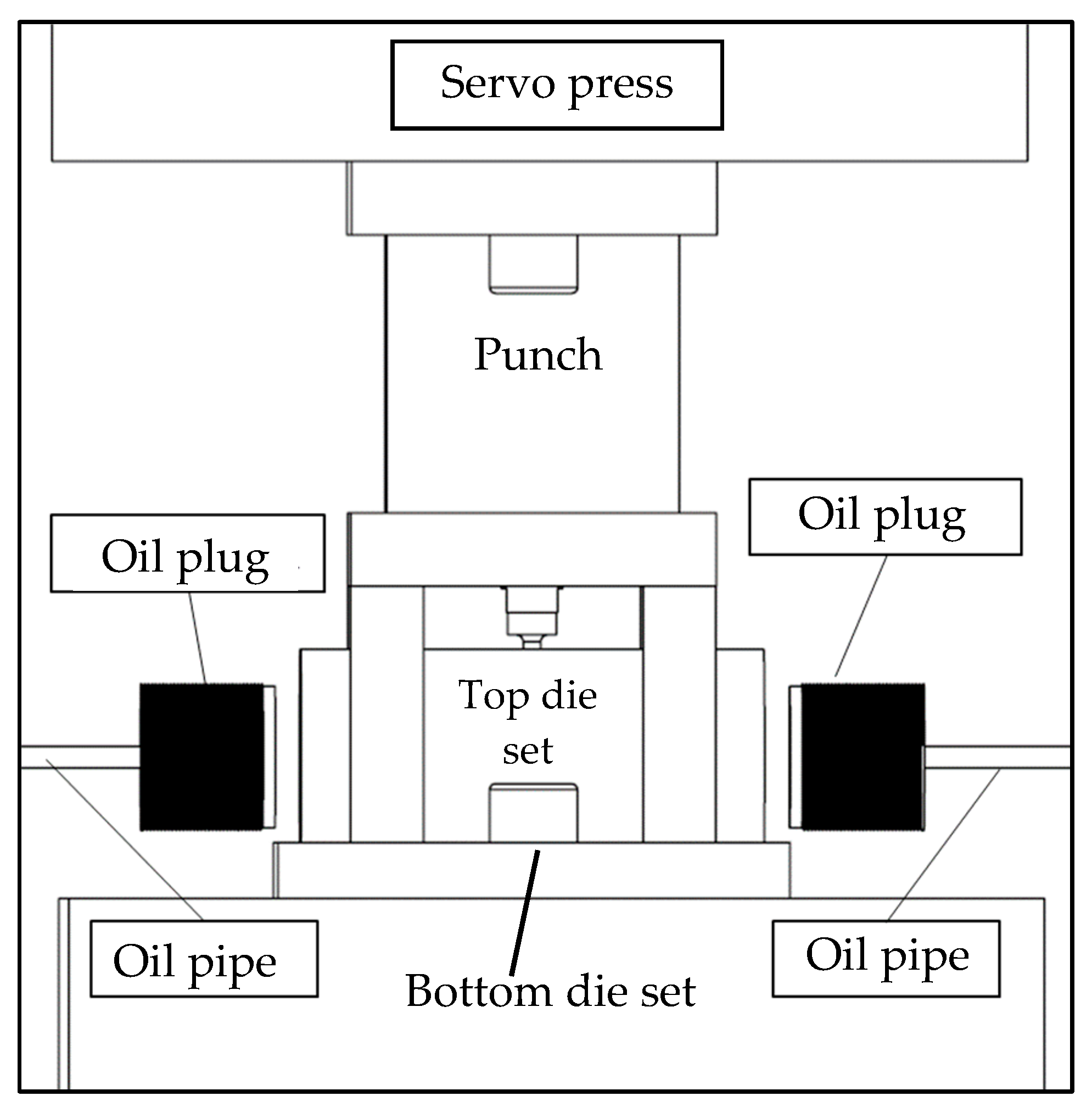
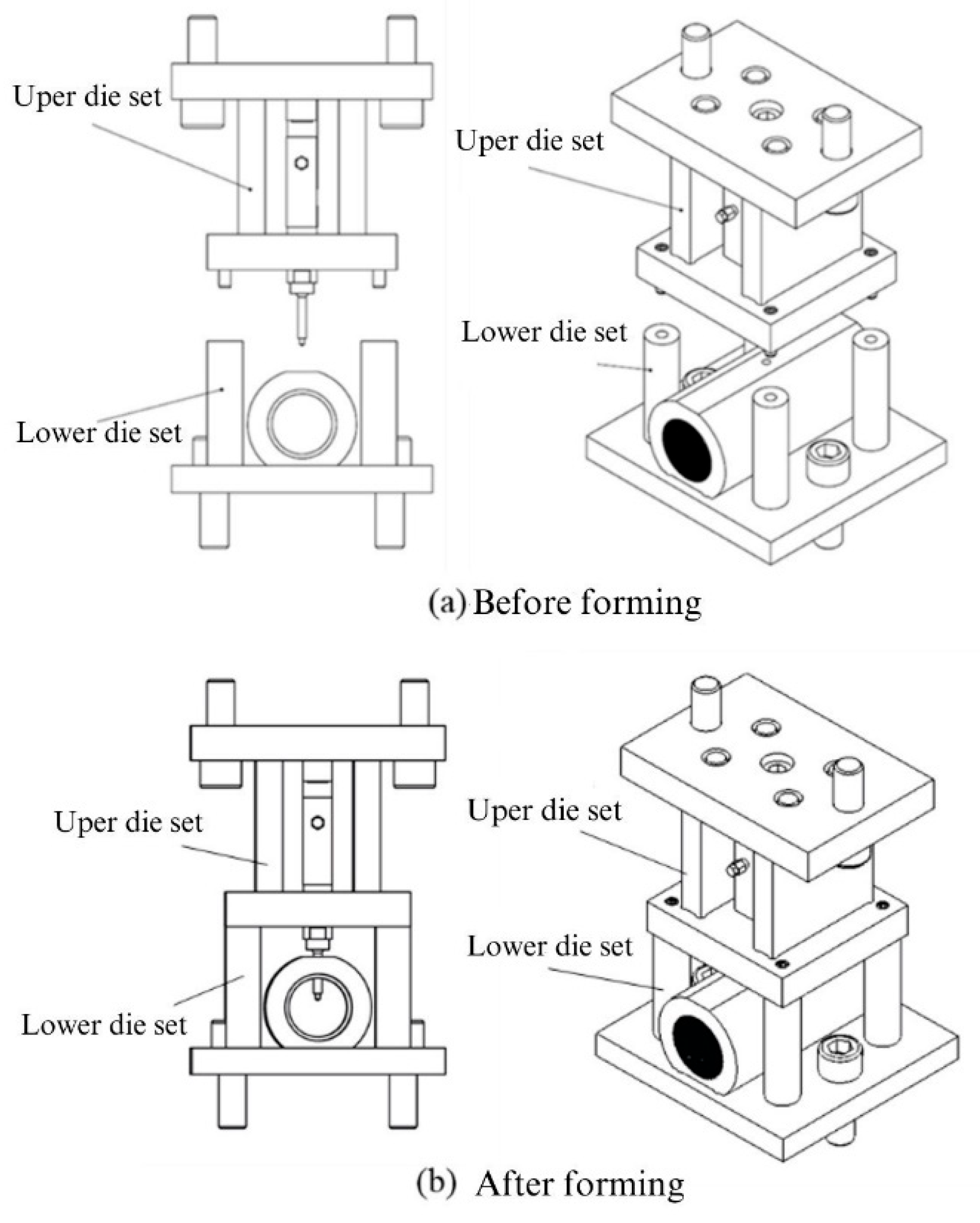
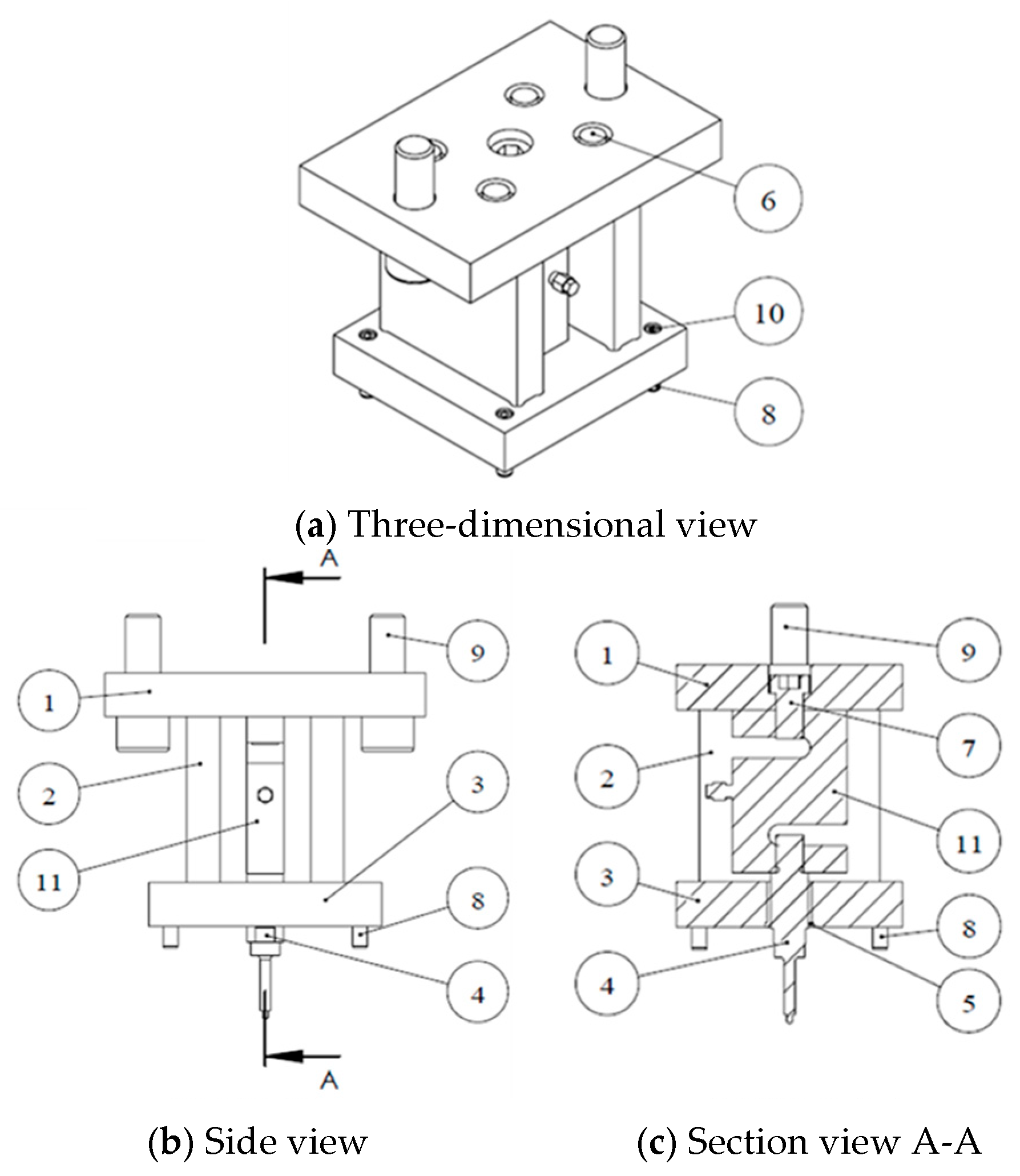
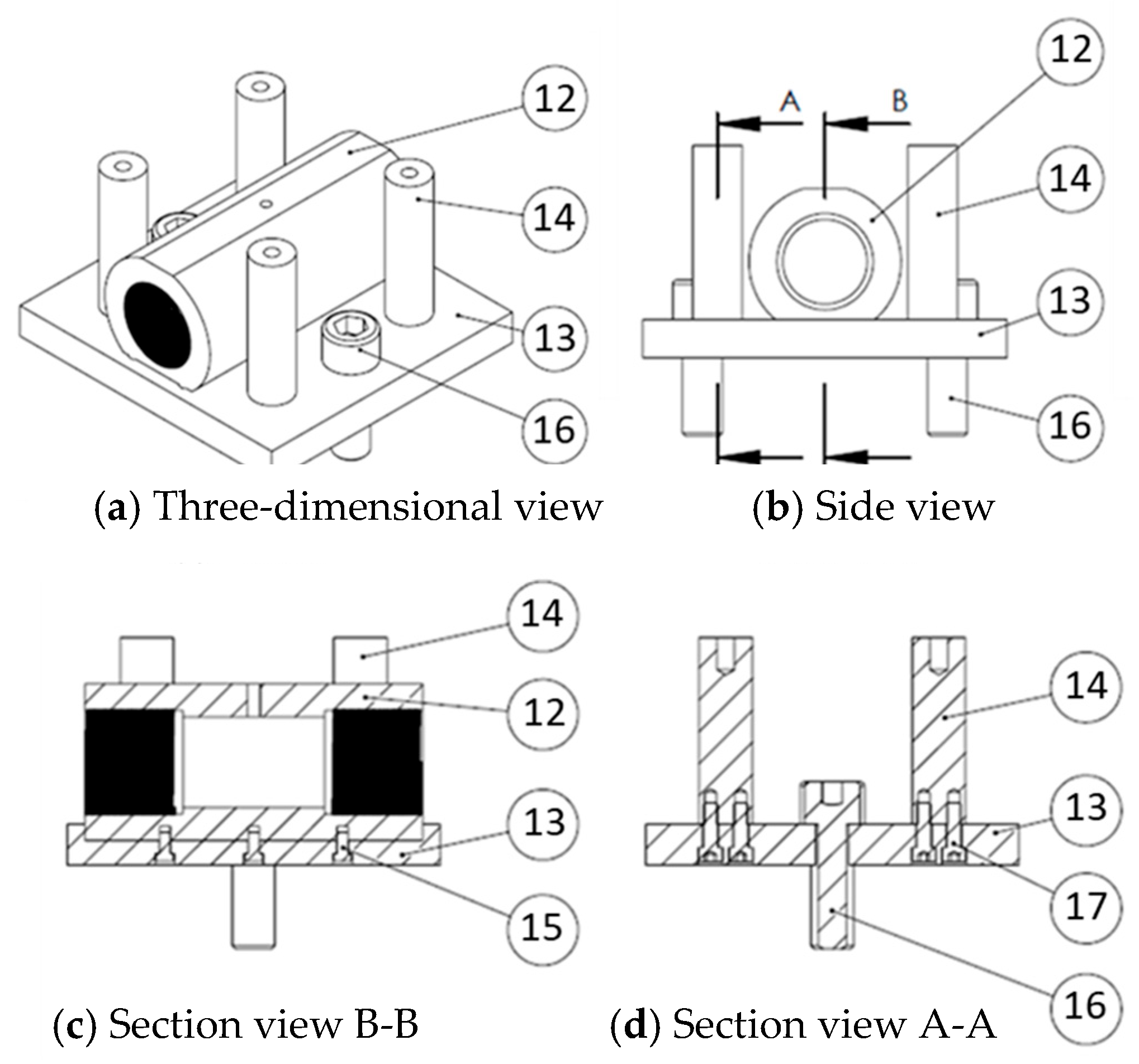
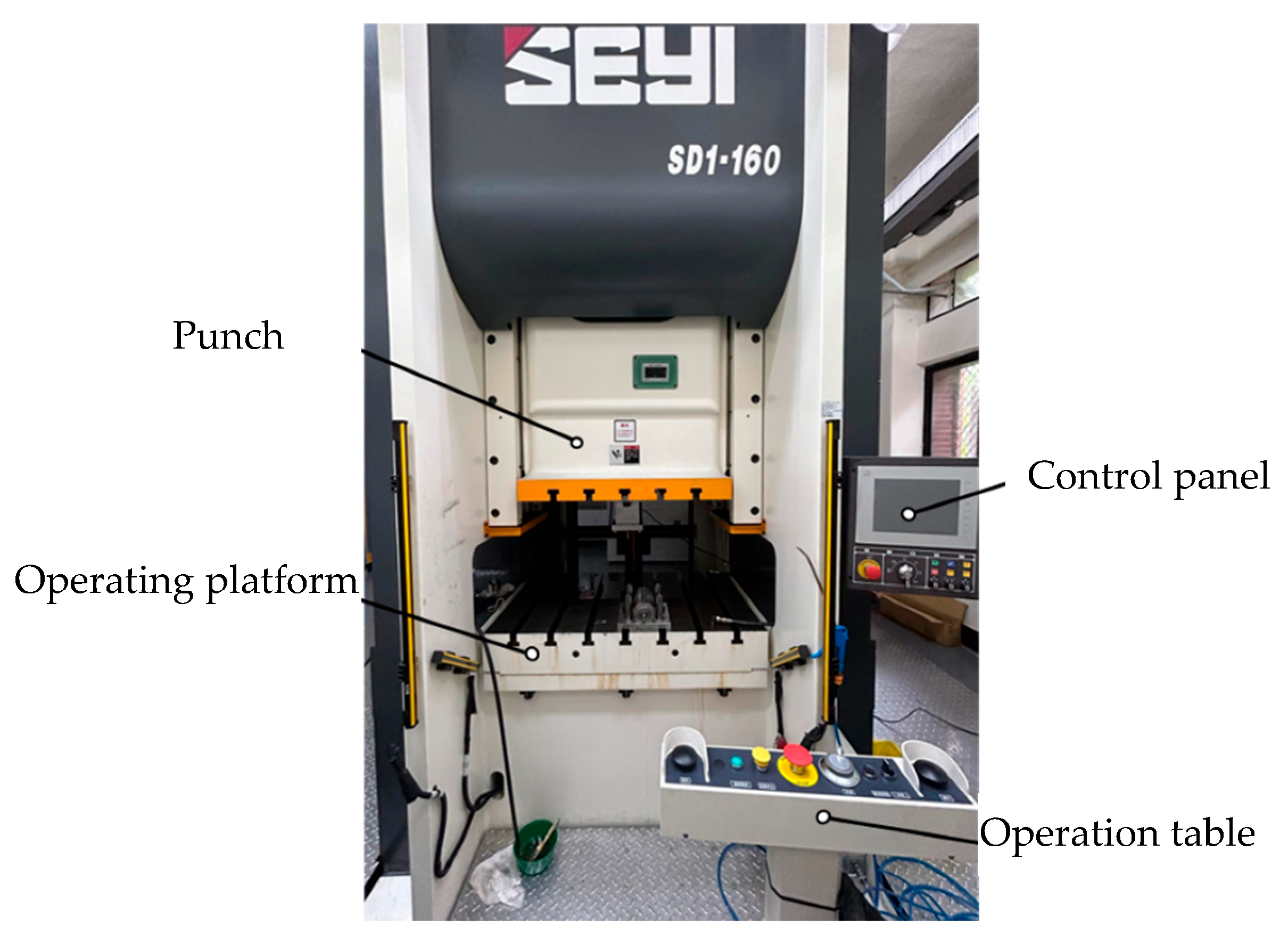
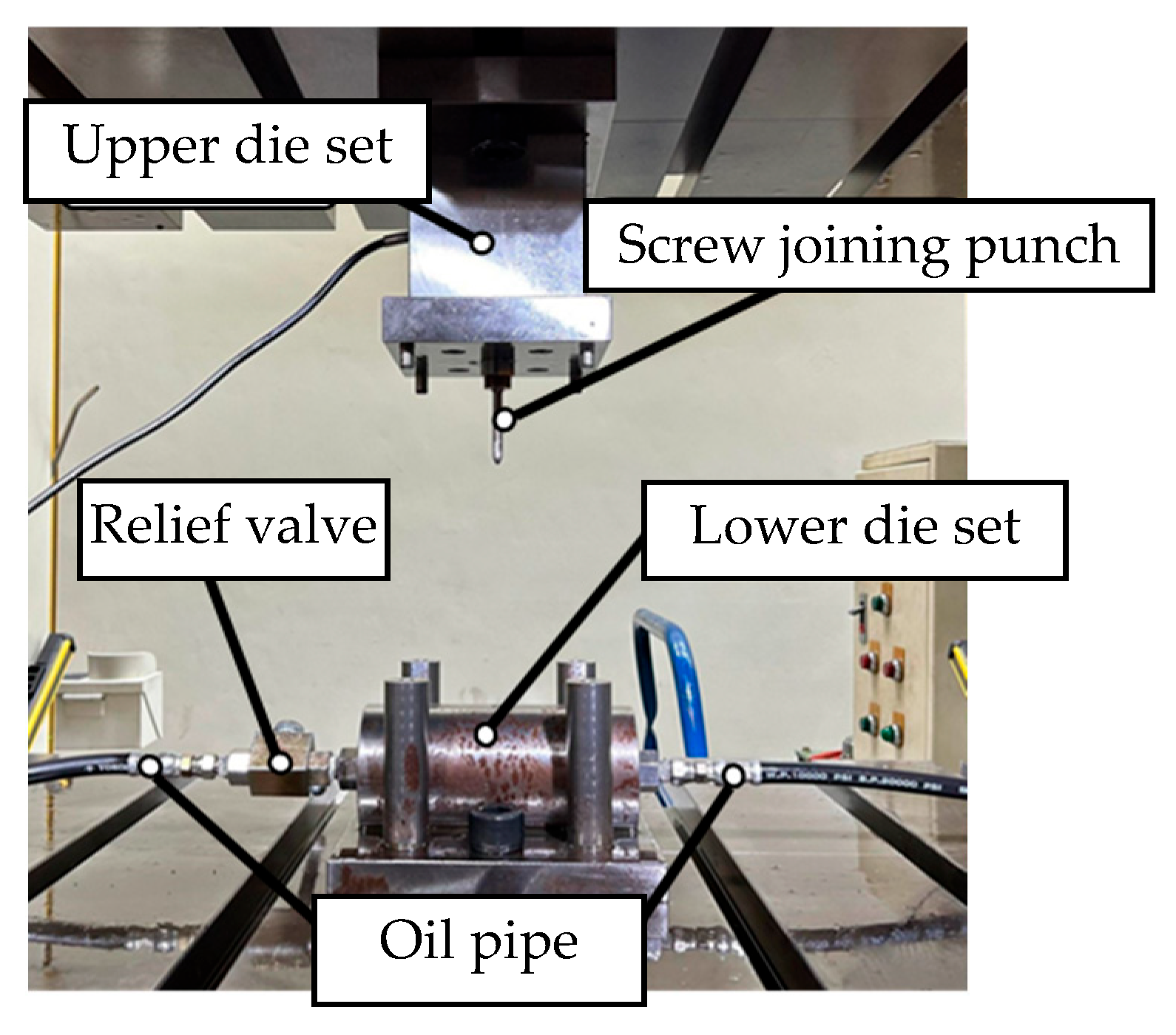
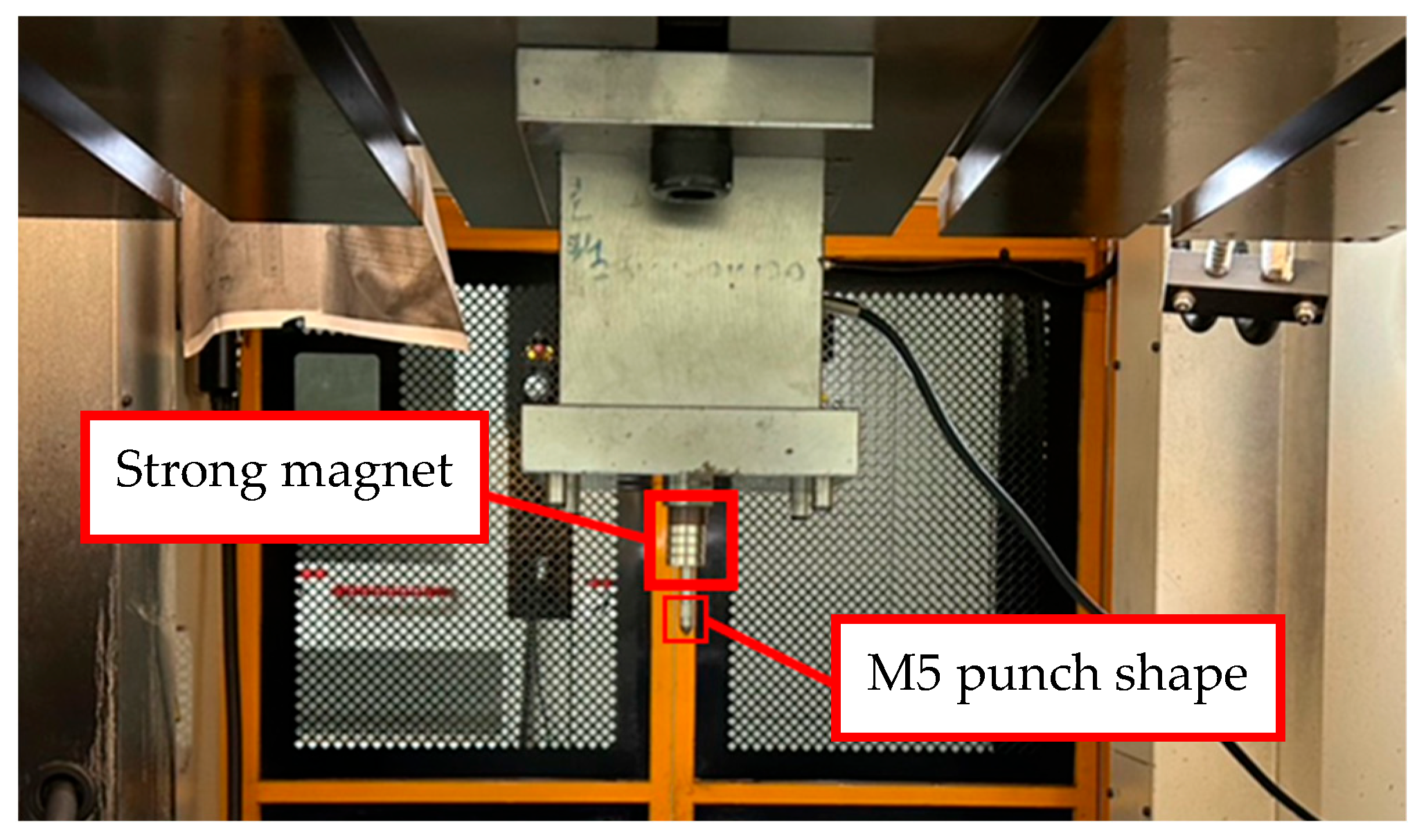
4.1. Experimental Die Design and Equipment Overview
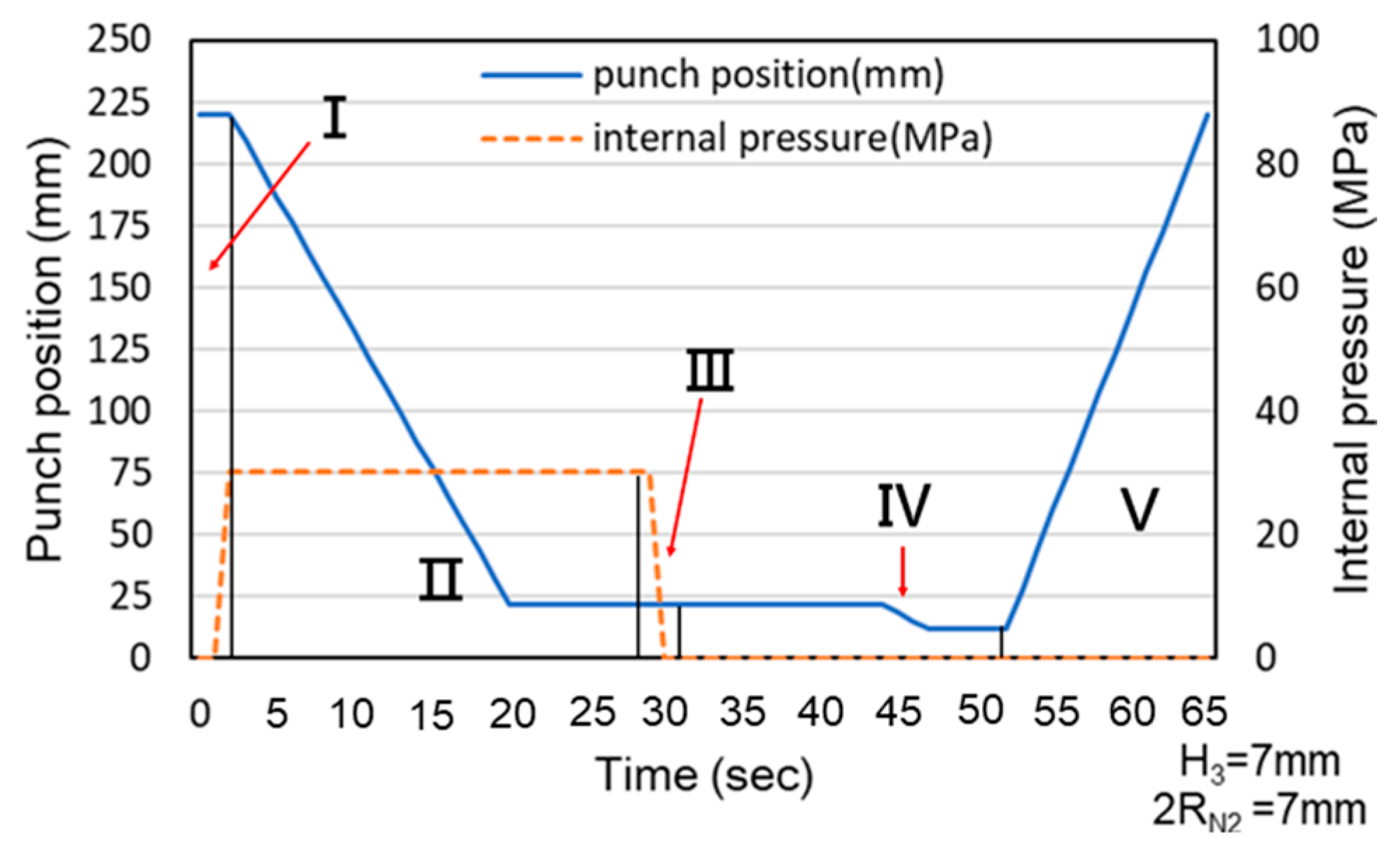
4.2. Experimental Procedure and Design
4.3. Pull out Load Experiment Results
4.4. Nut Inlaying Experiment
5. Conclusions
- (1)
- The pull out load steadily increased as the internal pressure increased, but at an internal pressure of 70 MPa, the material overflowed into the die cavity, affecting the pull out load simulations.
- (2)
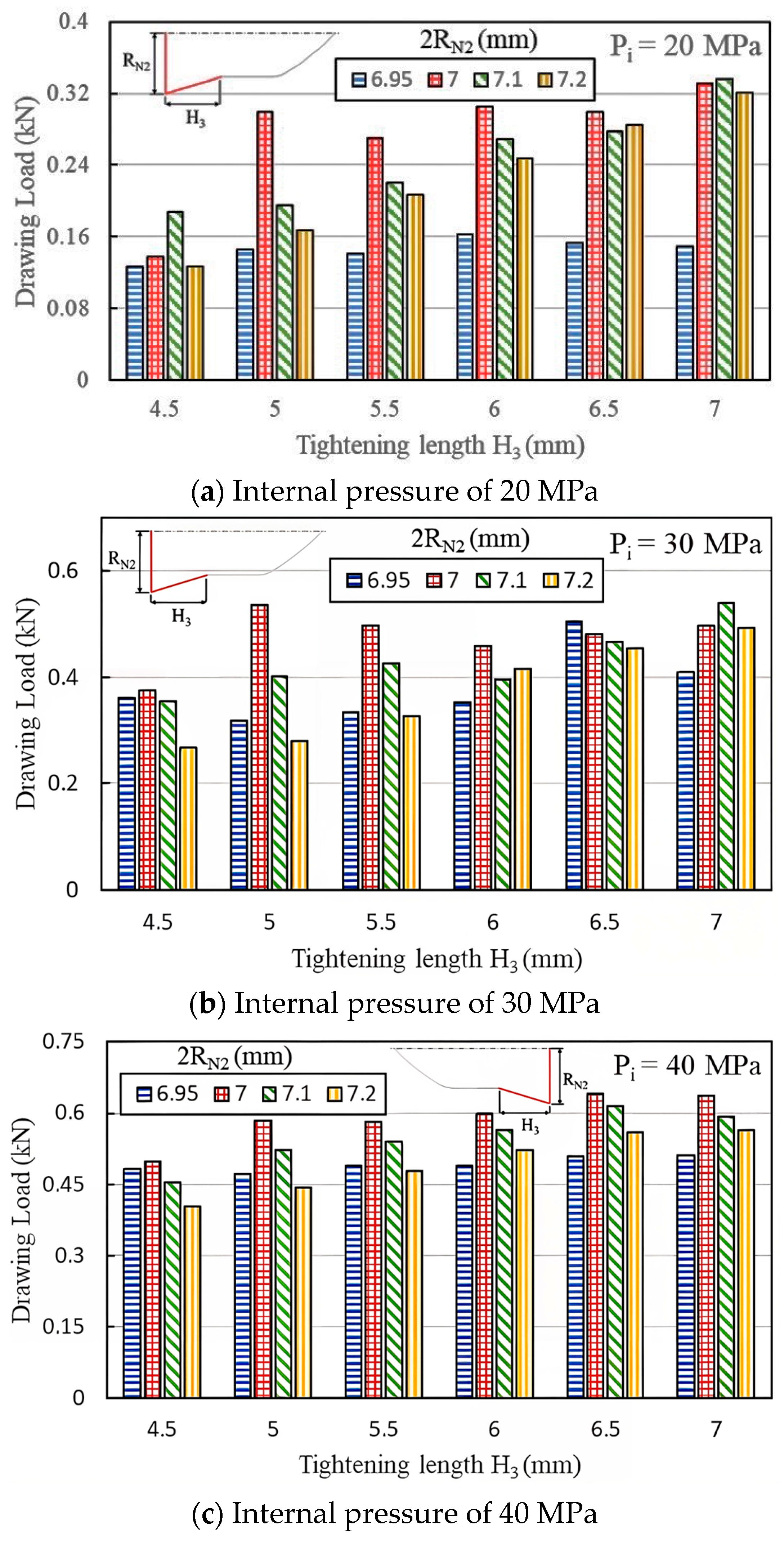
- The load slightly increased as the fit zone height increased, likely due to the increased punch stroke length caused by the fit zone height changes, which increased the contact area between the nuts and the tubes, increasing the pull out load. By contrast, as the fit zone diameter decreased from 7.2 mm to 7 mm, the pull out load increased. The pull out load increased until the fit zone diameter reached 6.95 mm; subsequently, it began to decrease. Therefore, this study concluded that the optimal parameters for a large load are a fit zone diameter of 7 mm and an internal pressure of 60 MPa, which result in a 906 N pull out load.
- (3)
- The nut torque simulation results revealed that due to nut geometry, the rotation was smooth, and the torque typically was below or between torque grades 8.8 and 10.9, failing to meet the standards for pneumatic tools and professional operations.
- (4)
- In the hydro-joining experiment results, the error in the maximum pull out load was relatively large at 30 MPa, approaching 50%, whereas the error at 50 MPa was only 5%. Substantial rollover was observed, likely due to the lack of internal pressure support during the nut inlaying stage after pressure release, which led to excessive punch strokes. This study also observed that the failure zone in the experiments occurred in the annular region rather than at the tip region as in the simulations, contributing to the larger observed error in the experiments.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| 2Rh | Die hole diameter |
| Pi | Internal pressure |
| Pp | Punch position |
| T | Time |
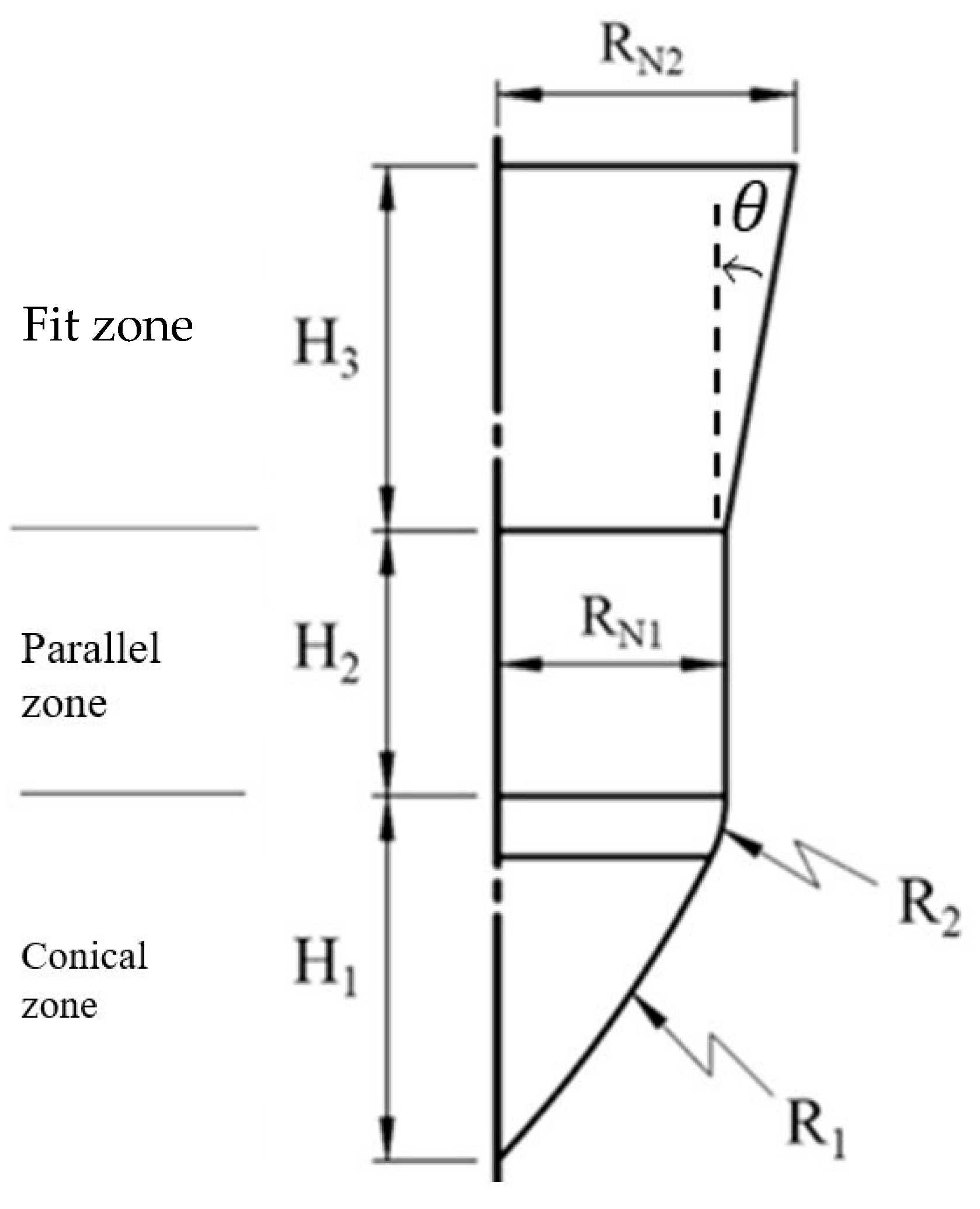
| RN1 | Top of fit zone radius |
| RN2 | Fit zone radius |
| H3 | Fit zone height |
| H2 | Parallel zone height |
| H1 | Conical zone height |
| R1 | Profile radius of conical zone |
| R2 | Corner radius |
References
- Dohmann, F.; Hartl, C. Tube hydroforming—Research and practical application. J. Mater. Process. Technol. 1997, 71, 174–186. [Google Scholar] [CrossRef]
- Ahmetoglu, M.; Altan, T. Tube hydroforming: State-of-the-art and future trends. J. Mater. Process. Technol. 2000, 98, 25–33. [Google Scholar] [CrossRef]
- Mizumura, M.; Sato, K.; Kuriyama, Y. Development of nut-inlaying technique in hydroformed component by hydro-burring. Mater. Trans. 2012, 53, 801–806. [Google Scholar] [CrossRef]
- Mizumura, M.; Sato, K.; Suehiro, M.; Wada, M.; Iguchi, K.; Kuriyama, Y. Development of new hydroforming methods. Nippon. Steel Tech. Rep. 2013, 103, 39–46. [Google Scholar]
- Fracz, W.; Stachowicz, F.; Trzepiecinski, T. Investigations of thickness distribution in hole expanding of thin steel sheets. Arch. Civ. Mech. Eng. 2012, 12, 279–283. [Google Scholar] [CrossRef]
- Kacem, A.; Krichen, A.; Manach, P.Y.; Thuillier, S.; Yoon, J.W. Failure prediction in the hole-flanging process of aluminium alloys. Engineeting Fract. Mech. 2013, 99, 261–265. [Google Scholar] [CrossRef]
- Thipprakmas, S.; Jin, M.; Murakawa, M. Study on flanged shapes in fineblanked-hole flanging process using finite element method. J. Mater. Process. Technol. 2007, 193, 128–133. [Google Scholar] [CrossRef]
- Smith, L.M. Hydroforming for Advanced Manufacturing; Woodhead Publishing Limited: Dearborn, MI, USA, 2008. [Google Scholar]
- Wu, Z.G.; Li, S.H.; Zhang, W.G.; Wang, W.R. Ductile fracture simulation of hydropiercing process based on various criteria in 3D modeling. Mater. Des. 2010, 31, 3661–3671. [Google Scholar] [CrossRef]
- Qian, L.; Ji, W.; Sun, C.; Fang, G.; Lian, J. Prediction of edge fracture during hole-flanging of advanced high-strength steel considering blanking pre-damage. Eng. Fract. Mech. 2021, 248, 107721. [Google Scholar] [CrossRef]
- Hwang, Y.M.; Pham, H.N.; Tsui, H.S.R. Investigation of Punch Shape and Loading Path Design in Hydro-Flanging Processes of Aluminum Alloy Tubes. Metals 2021, 11, 636. [Google Scholar] [CrossRef]
- Liu, W.; Hao, J.; Liu, G.; Gao, G.L.; Yuan, S.J. Influence of punch shape on geometrical profile and quality of hole piercing-flanging under high pressure. Int. J. Adv. Manuf. Technol. 2016, 86, 1253–1262. [Google Scholar] [CrossRef]
- Choi, S.K.; Kim, W.T.; Moon, Y.H. Analysis of deformation surrounding a hole produced by tube hydro-piercing. J. Eng. Manuf. 2004, 218, 1091–1097. [Google Scholar] [CrossRef]
- Hwang, Y.M.; Wu, R.K. Process and loading path design for hydraulic compound forming of rectangular tubes. Int. J. Adv. Manuf. Technol. 2017, 91, 2135–2142. [Google Scholar] [CrossRef]
- Hwang, Y.M.; Dai, W.H.; Chen, C.C. Investigation of punch shape design in tube hydro-piercing processes. Int. J. Adv. Manuf. Technol. 2020, 110, 2211–2220. [Google Scholar] [CrossRef]
- Hwang, Y.M.; Tsai, Y.J. Movable die and loading path design in tube hydroforming of irregular bellows. Metals 2020, 10, 1518. [Google Scholar] [CrossRef]
- Park, J.Y.; Han, S.W.; Jeong, H.S.; Cho, J.R.; Moon, Y.H. Advanced sealing system to prevent leakage in hydroforming. J. Mater. Process. Technol. 2017, 247, 103–110. [Google Scholar] [CrossRef]
- Yu, X.Y.; Chen, J.; Chen, J.S. Interaction Effect of Cracks and Anisotropic Influence on Degradation of Edge Stretchability in Hole-expansion of Advanced High Strength Steel. Int. J. Mech. Sci. 2016, 105, 348–359. [Google Scholar] [CrossRef]
- Kumar, S.; Ahmed, M.; Panthi, S.K. Effect of Punch Profile on Deformation Behaviour of AA5052 Sheet in Stretch Flanging Process. Arch. Civ. Mech. Eng. 2020, 20, 18. [Google Scholar] [CrossRef]
- Patne, S.; Karale, A.; Mohankumar, V.; Rane, S. Bolt pre-load CAE analysis and validation: FEA simulation of hex bolt tightening torque for IDU assembly of 2-wheeler in MSC Nastran and practical validation. Mater. Today 2023, 72, 1925–1928. [Google Scholar] [CrossRef]
- Zhang, Q.; Wu, C.D.; Zhao, S.D. Numerical Simulation on Hydroforming Tube-like Eccentric Shaft Part by Using Movable die. In Proceedings of the 5th International Conference on Tube Hydroforming, Hokkaido, Japan, 24–27 July 2011; pp. 149–152. [Google Scholar]
- Katsuya, S.; Minoru, Y.; Masato, S. Hole-making of aluminum circular pipe by impact hydraulic pressure. In Proceedings of the 70th Japanese Joint Conference for the Technology of Plasticity, Tokyo, Japan, 12 October 2019; pp. 309–310. [Google Scholar]
- Manabe, K.; Fuchizawa, S. Tube forming technology: Toward the highest card for weight savings. J. Jpn. Soc. Technol. Plast. 2011, 52, 33–41. [Google Scholar] [CrossRef]
- Sun, L.; Wang, X.; Wang, Q.; Fan, Z.; Ling, C.; Liu, X.; Chu, G. Experimental and numerical investigation on the square hole hydro-piercing process. Metals 2023, 13, 1107. [Google Scholar] [CrossRef]
- Uhe, B.; Meschut, G. Advanced self-piercing riveting of ultra-high-strength steel through rivets with modified material properties. J. Manuf. Process. 2024, 125, 354–363. [Google Scholar] [CrossRef]





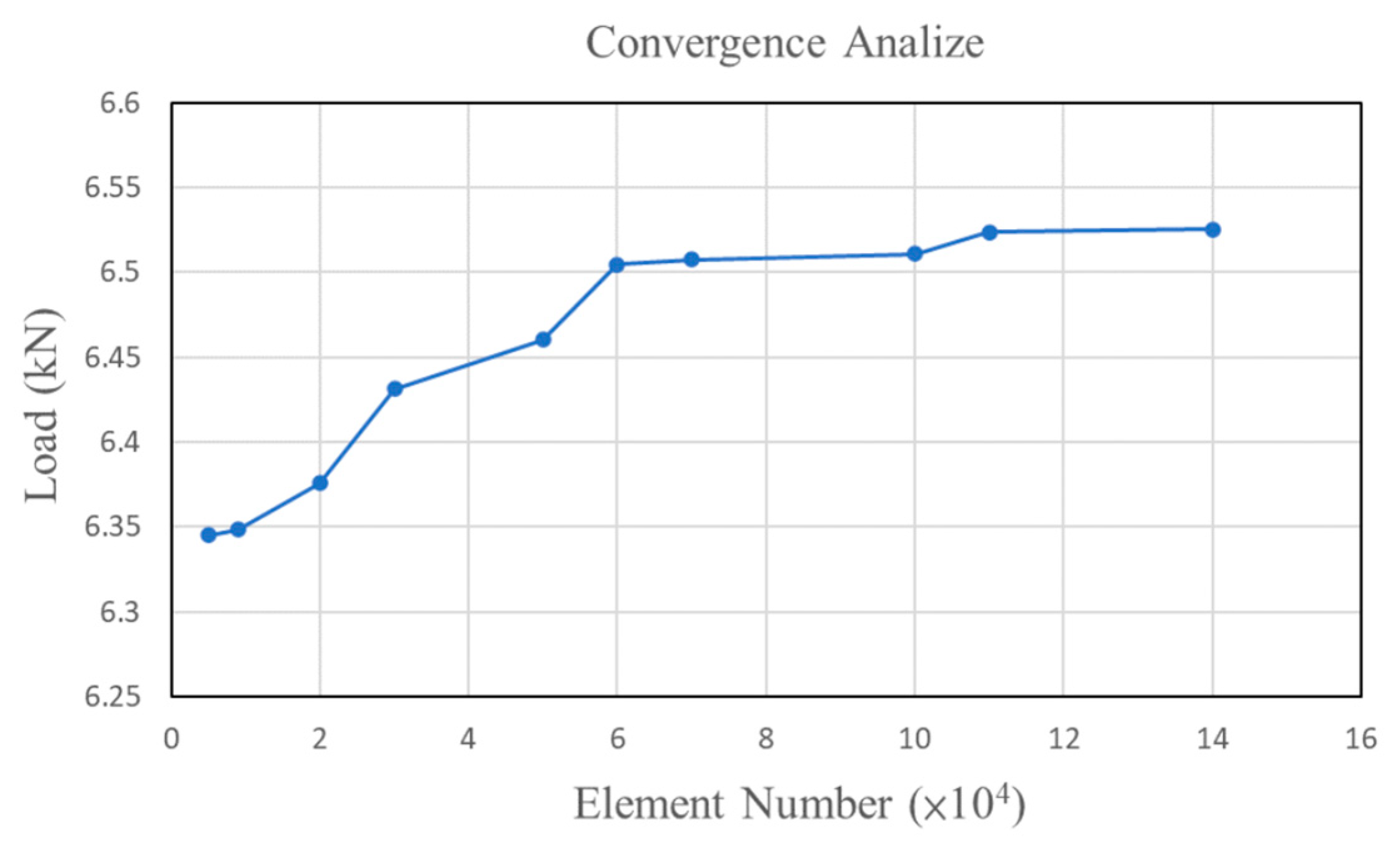
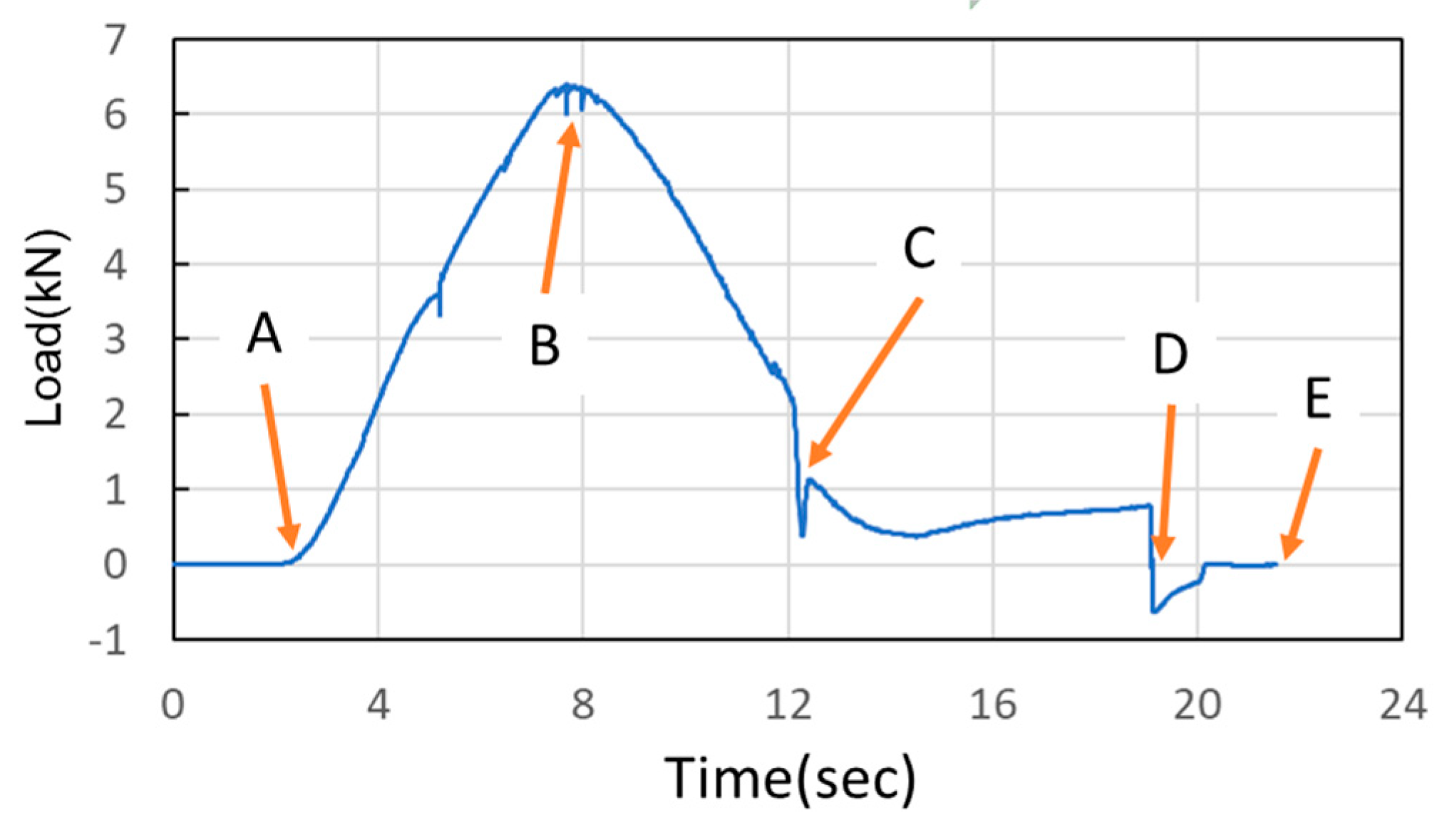



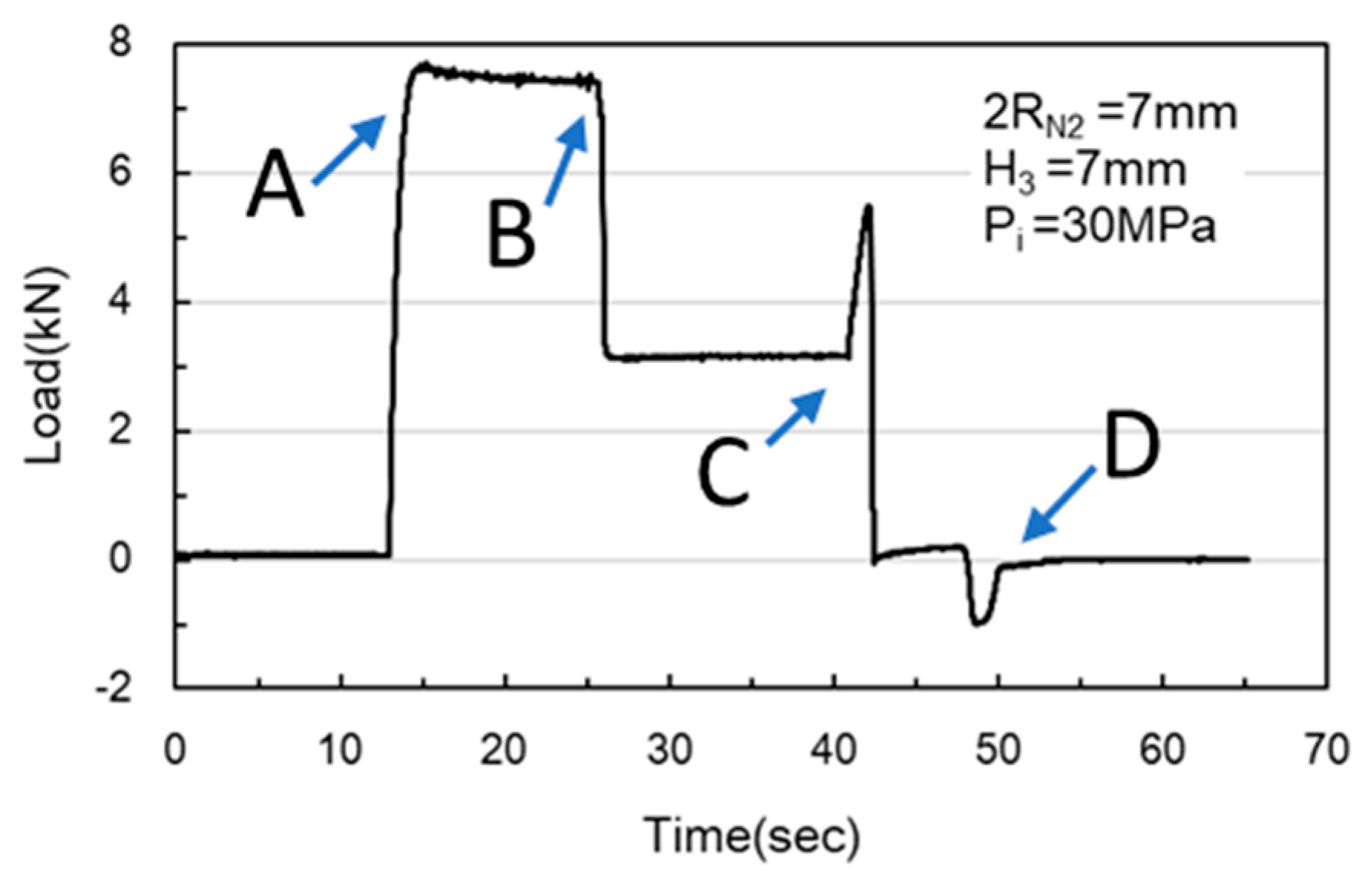

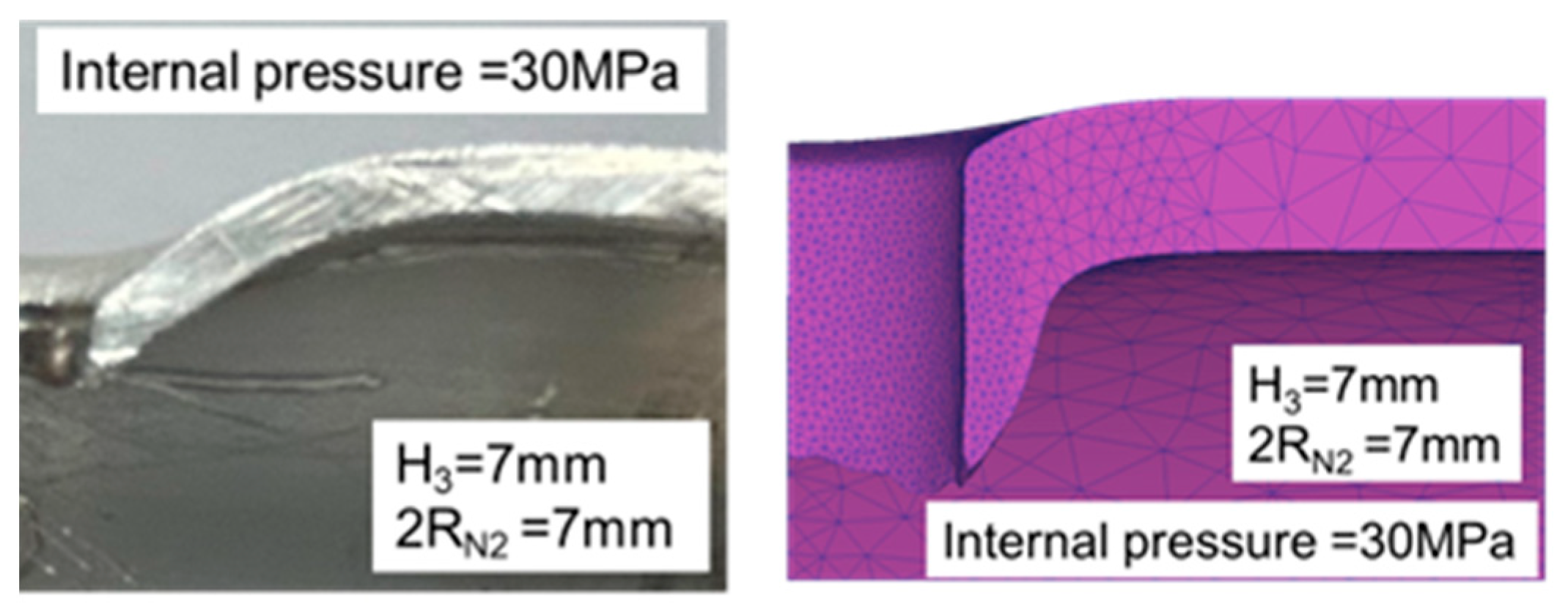
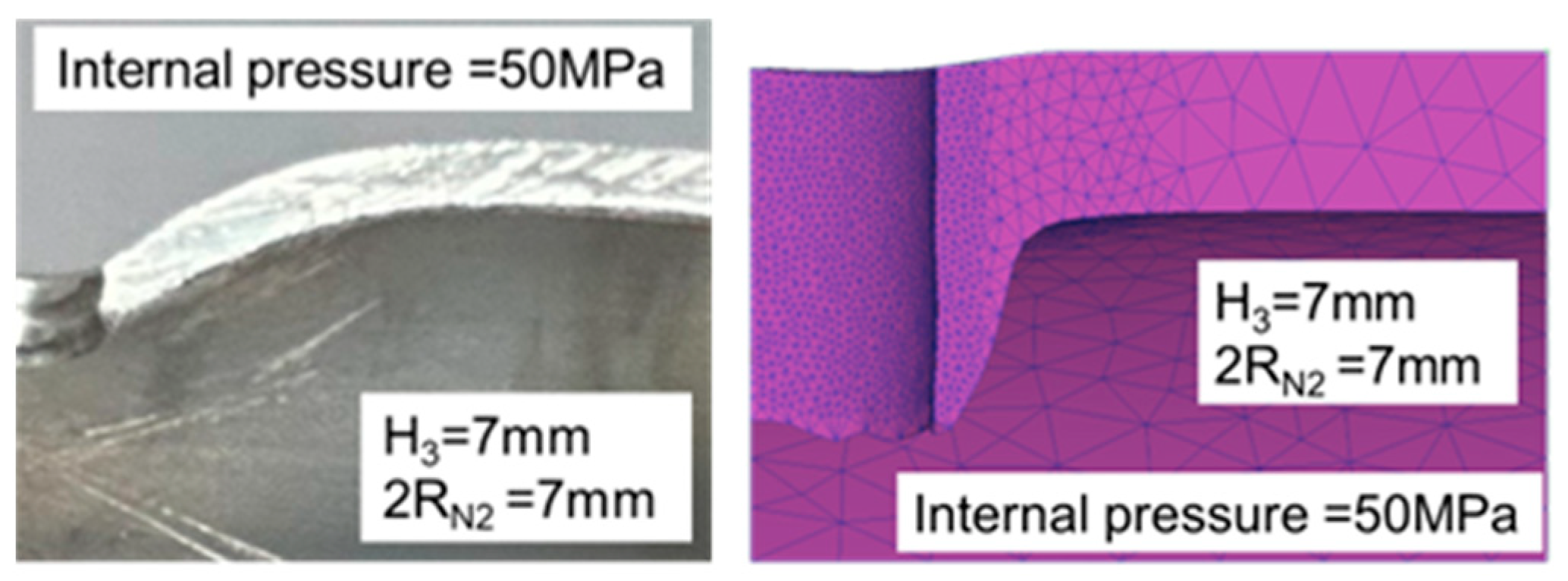


| Nut Diameter 2RN [mm] | 6.9 |
| Nut height H3 + H2 [mm] | 4 |
| Nut height H1 [mm] | 4 |
| Nut height HN [mm] | 5.5 |
| Position of Center R1 | |
| X10 [mm] | −14.58 |
| Z10 [mm] | 13.69 |
| Position of Center R2 | |
| X20 [mm] | 1.45 |
| Z20 [mm] | 5.5 |
| FEM Software | DEFORM 3D | |
|---|---|---|
| flow stress | ||
| Failure criteria | Normalized C and L: | |
| Critical value Cf | 1.42 | |
| Young’s modulus E [GPa] | 68.9 | |
| Poisson’s ratio | 0.33 | |
| Coefficient of friction | Nut and Tube | 0.15 |
| Die and Tube | 0.2 | |
| Punch velocity V [mm/s] | 1 | |
| Internal pressure pi [MPa] | 20, 30, 40, 50, 60, 70 | |
| Temperature T | Room temperature | |
| Simulation Type | Quarter Model |
|---|---|
| Internal pressure, Pi [MPa] | 20, 30, 40, 50, 60, 65, 70 |
| Die hole diameter, 2Rh [mm] | 7.2 |
| Nut fit zone height, H3 [mm] | 4.5, 5, 5.5, 6, 6.5, 7 |
| Nut parallel zone height, H2 [mm] | 4 |
| Nut conical zone height, H1 [mm] | 5.5 |
| Nut diameter, 2RN2 [mm] (clearance value) | 6.95, 7, 7.1, 7.2 (0.125, 0.1, 0.05, 0) |
| Nut diameter, 2RN1 [mm] | 6.9 |
| Rounded corner, R2 [mm] | 2 |
| Rounded corner, R1 [mm] | 20 |
| Fit zone height, H3 [mm] | 4.5 | 5 | 5.5 | 6 | 6.5 | 7 |
| Punch stroke length [mm] | 14 | 14.5 | 15 | 15.5 | 16 | 16.5 |
| Simulation Type | Full 3D Model |
|---|---|
| Internal pressure, Pi [MPa] | 20, 30, 40, 50, 60 |
| Die hole diameter, 2Rh [mm] | 7.2 |
| Fit zone height, H3 [mm] | 5, 6, 7 |
| Parallel zone height, H2 [mm] | 4 |
| Conical zone height, H1 [mm] | 5.5 |
| Diameter, 2RN2 [mm] (clearance value) | 6.95, 7, 7.1 (0.125, 0.1, 0.05) |
| Diameter, 2RN1 [mm] | 6.9 |
| Rounded corner, R2 [mm] | 2 |
| Rounded corner, R1 [mm] | 20 |
| No. | Name | Material | Quantity |
|---|---|---|---|
| 1 | Upper die plate | S45C | 1 |
| 2 | Load cell-fixing die | S45C | 2 |
| 3 | Load cell-fixing die | S45C | 1 |
| 4 | Nut punch | SKD11 | 1 |
| 5 | Guide sleeve | SUJ2 | 1 |
| 6 | M10×30 hexagon bolt | SCM435 | 8 |
| 7 | M18×30 hexagon bolt | SCM435 | 1 |
| 8 | Guide pin | SUJ2 | 4 |
| 9 | M24×70 hexagon bolt | SCM435 | 2 |
| 10 | M6×16 hexagon bolt | SCM435 | 4 |
| 11 | Load cell | 1 |
| No. | Name | Material | Quantity |
|---|---|---|---|
| 12 | Tube-holding sleeve | Recycled steel | 1 |
| 13 | Lower die plate | S45C | 1 |
| 14 | guide pillar | SS41 | 4 |
| 15 | M6×12 hexagon bolt | SCM435 | 3 |
| 16 | M24×70 hexagon bolt | SCM435 | 2 |
| 17 | M8×25 hexagon bolt | SCM435 | 8 |
| Simulation [kN] | Experiment [kN] | Error Value | |
|---|---|---|---|
| 30 MPa | 5.87 | 7.71 | 24% |
| 50 MPa | 6.94 | 8.56 | 19% |
| Simulation [kN] | Experiment [kN] | Error Value | |
|---|---|---|---|
| 30 MPa | 0.51 | 1.004 | 49% |
| 50 MPa | 0.75 | 0.711 | 5% |
| Pi = 0 MPa 2RN2 = 7 mm H3 = 7 mm |   |
| Pi = 30 MPa 2RN2 = 7 mm H3 = 7 mm |   |
| Pi = 50 MPa 2RN2 = 7 mm H3 = 7 mm |   |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hwang, Y.-M.; Pham, H.-N.; Ho, Z.-W.; Wang, Y.-J. An Innovative Tube Hydro-Joining Process Combining Piercing, Hole Flanging and Nut Inlaying. Materials 2025, 18, 1990. https://doi.org/10.3390/ma18091990
Hwang Y-M, Pham H-N, Ho Z-W, Wang Y-J. An Innovative Tube Hydro-Joining Process Combining Piercing, Hole Flanging and Nut Inlaying. Materials. 2025; 18(9):1990. https://doi.org/10.3390/ma18091990
Chicago/Turabian StyleHwang, Yeong-Maw, Hong-Nhan Pham, Ze-Wei Ho, and Yu-Jen Wang. 2025. "An Innovative Tube Hydro-Joining Process Combining Piercing, Hole Flanging and Nut Inlaying" Materials 18, no. 9: 1990. https://doi.org/10.3390/ma18091990
APA StyleHwang, Y.-M., Pham, H.-N., Ho, Z.-W., & Wang, Y.-J. (2025). An Innovative Tube Hydro-Joining Process Combining Piercing, Hole Flanging and Nut Inlaying. Materials, 18(9), 1990. https://doi.org/10.3390/ma18091990






