Effect of Magnesium Salt Whiskers on the Mechanical Properties of Phosphogypsum Building Blocks
Abstract
1. Introduction
2. Calculation and Experimental Methods
2.1. Calculation Methods
2.2. Computational Model
2.3. Experimental Method
3. Results and Discussion
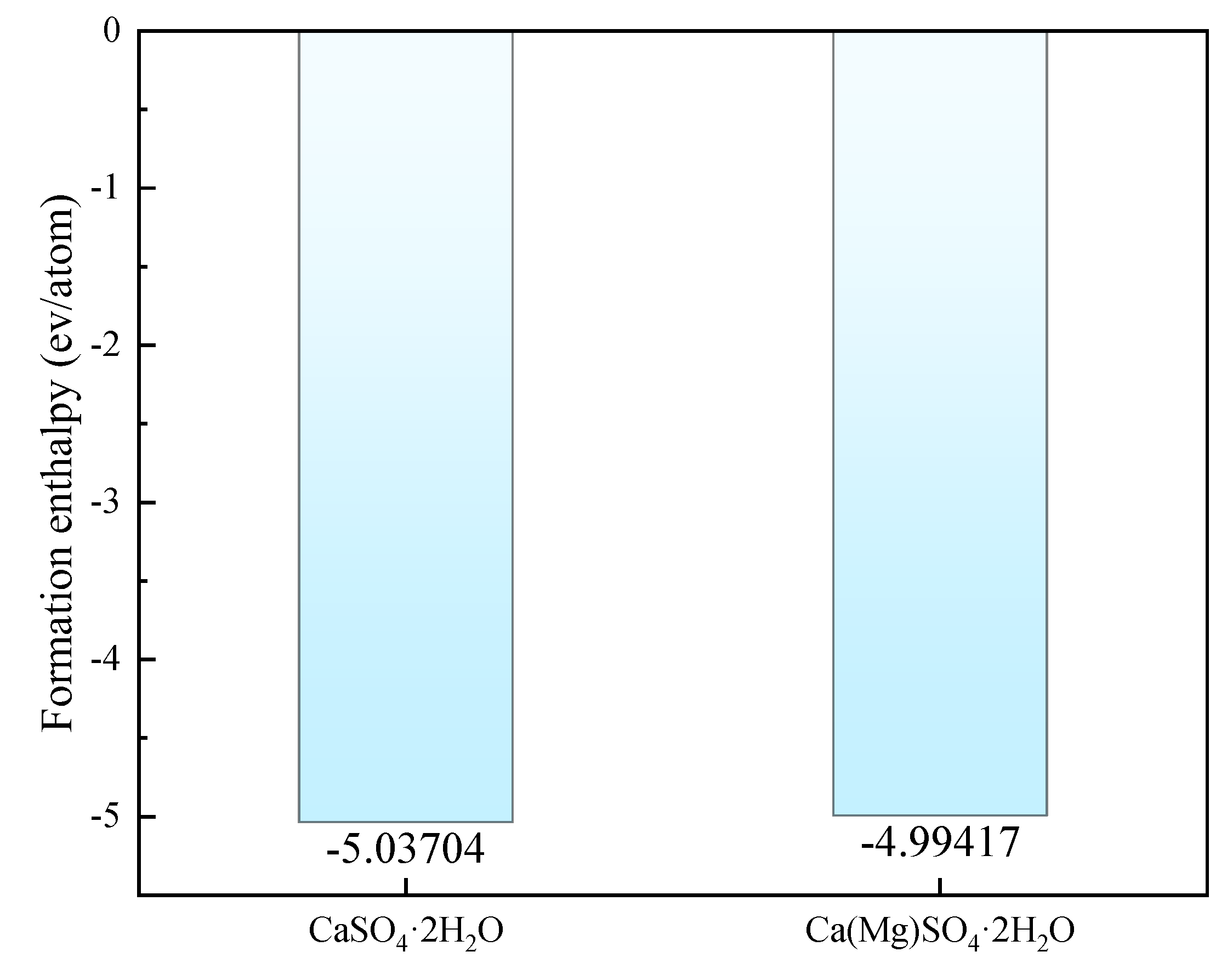
3.1. Crystal Structure Stability
3.2. Influence of Mg Doping on the Mechanical Properties of CaSO4·2H2O
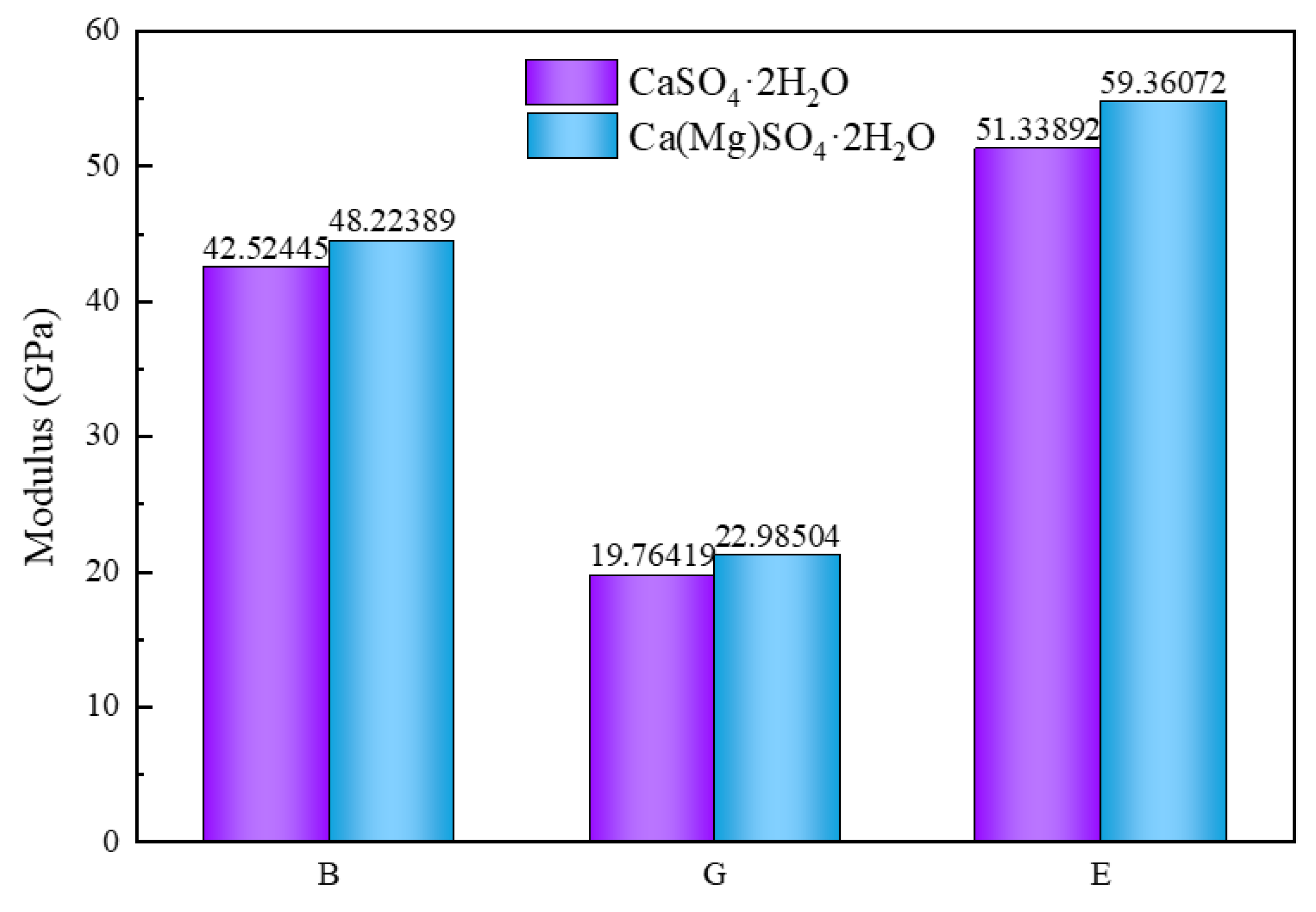
3.2.1. Elastic Modulus and Mechanical Performance Stability
3.2.2. Hardness
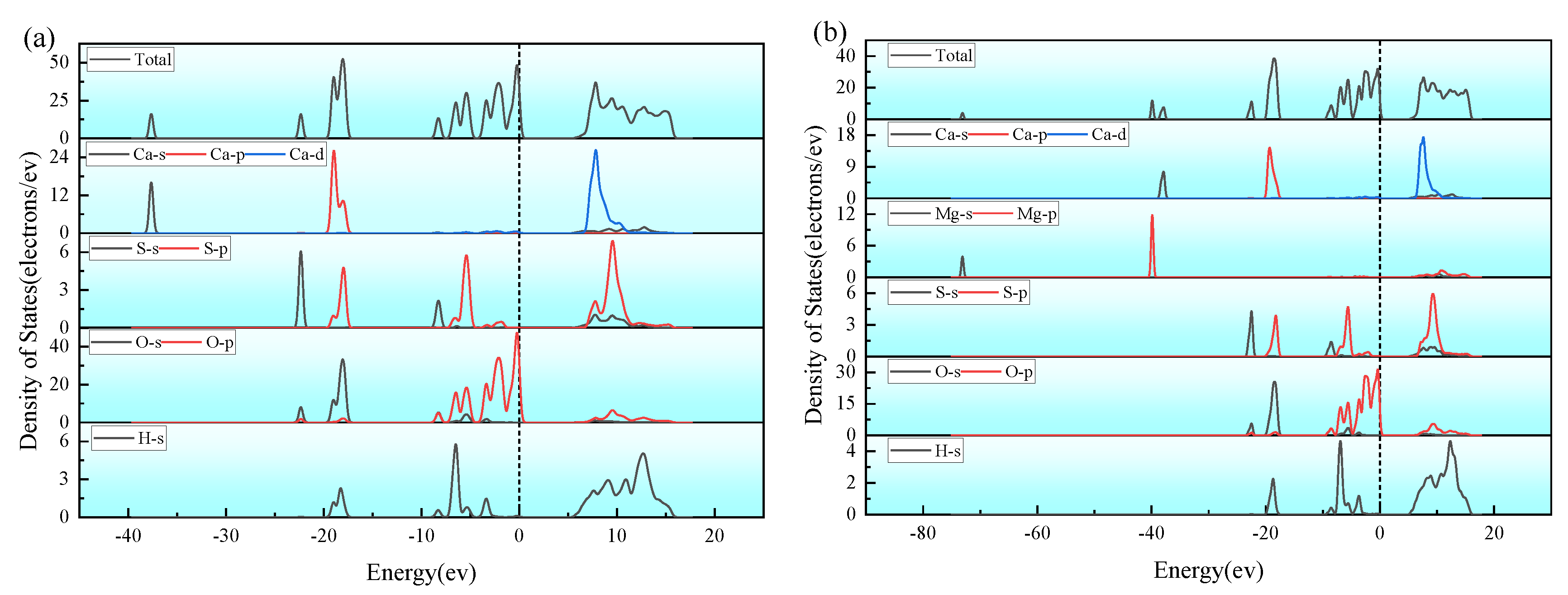
3.3. The Impact of Mg Doping on the Electronic Properties of CaSO4·2H2O
3.4. Interfacial Properties of CaSO4·2H2O with Magnesium Salts (Mg(OH)2, MgCl2, MgSO4)
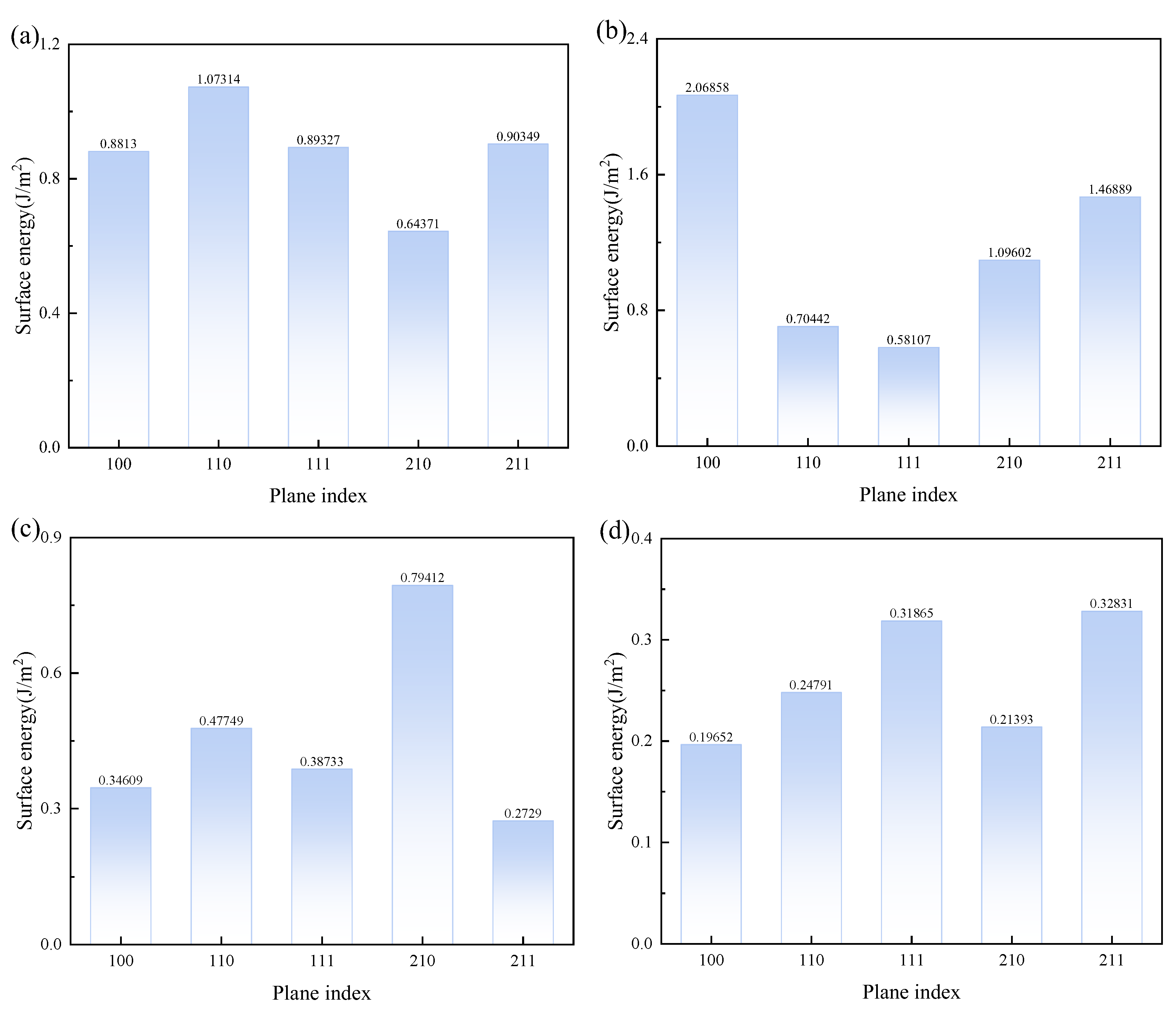
3.4.1. Surface Energy of CaSO4·2H2O with MgSO4, Mg(OH)2, and MgCl2
3.4.2. Interface Stability of CaSO4·2H2O/MgSO4, CaSO4·2H2O/Mg(OH)2, and CaSO4·2H2O/MgCl2
3.4.3. Analysis of Interface Electronic Structure
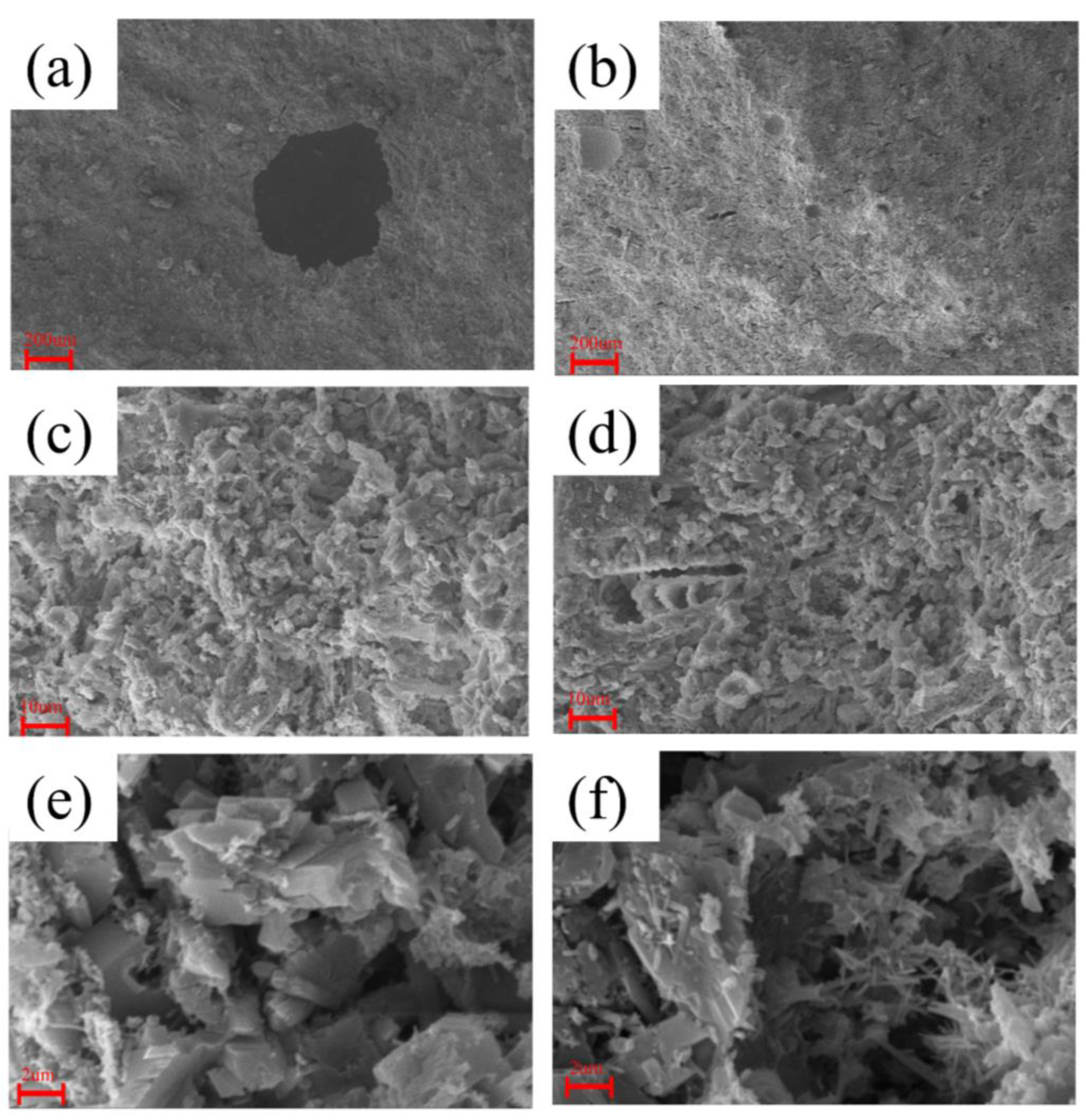
3.5. Experimental Results
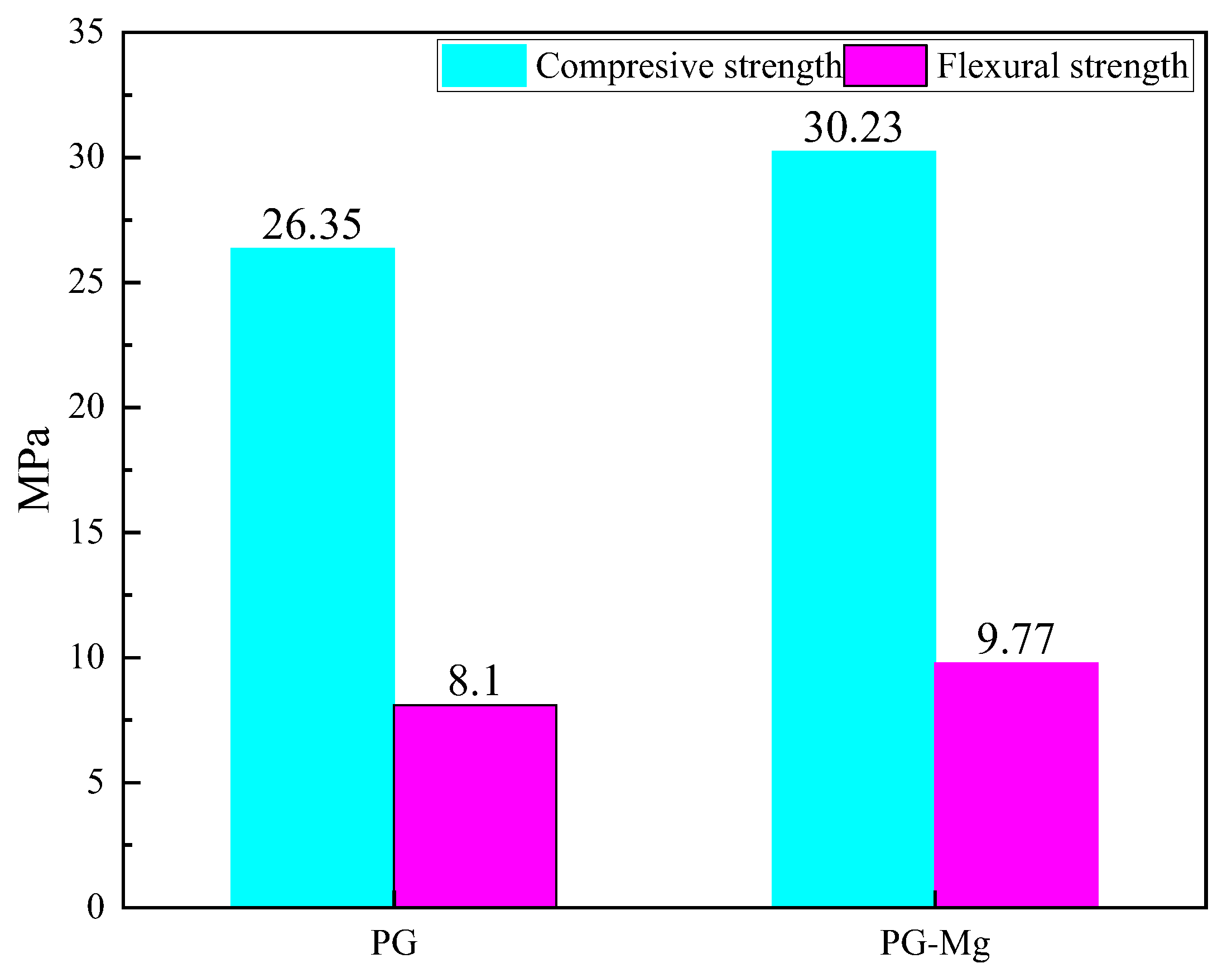
3.5.1. Impact of Magnesium Salts on the Mechanical Properties of Bricks
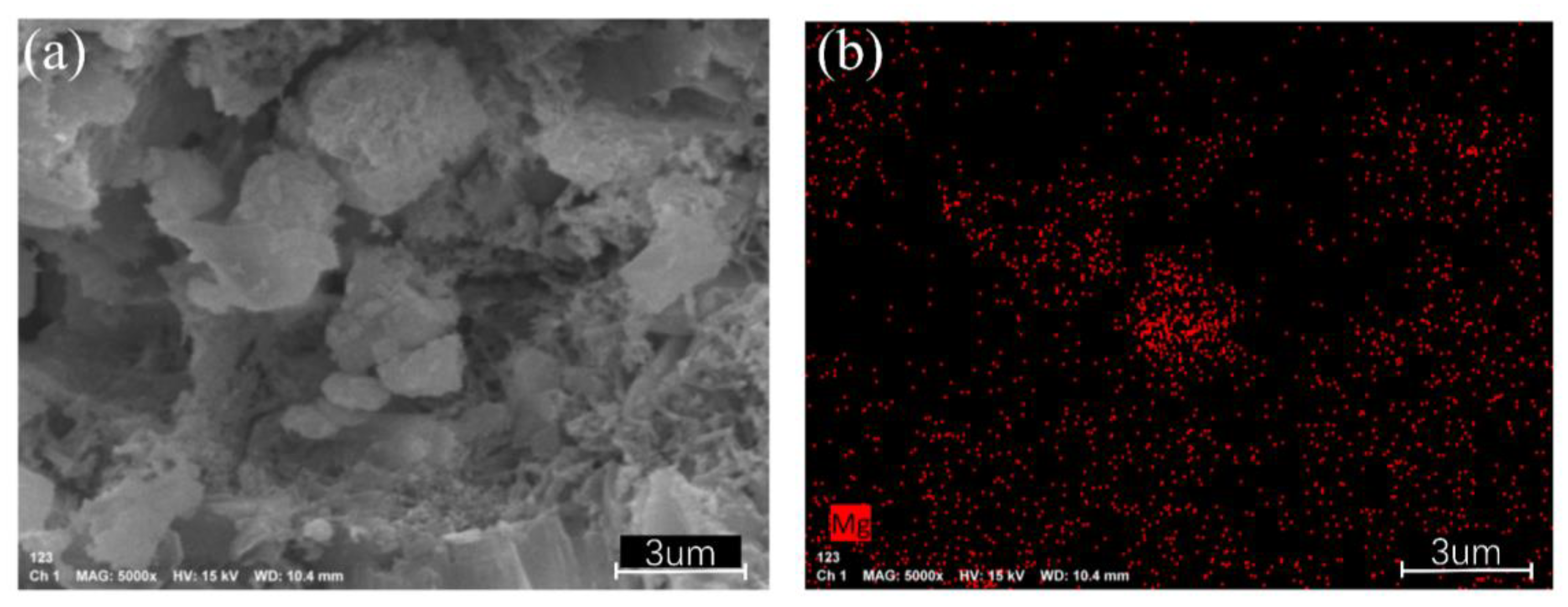
3.5.2. Microscopic Morphology
4. Discussion
- (1)
- The volumetric modulus, shear modulus, and Young’s modulus of CaSO4⋅2H2O are 42.52445, 19.76419, and 51.33892 GPa, respectively. After the addition of Mg, these properties increased by 13.43803%, 16.29639%, and 15.62518%, respectively.
- (2)
- Before Mg doping, the Poisson’s ratio of CaSO4·2H2O is 0.29879, and after doping, it is slightly reduced to 0.29484. The hardness of CaSO4·2H2O is 3.18363 GPa, while after Mg doping, the hardness of Ca(Mg)SO4·2H2O reaches 3.6273 GPa, representing a 13.9359% increase in hardness.
- (3)
- The order of interface binding stability between CaSO4⋅2H2O and different magnesium salts is as follows: CaSO4⋅2H2O/Mg(OH)2 > CaSO4⋅2H2O/MgSO4 > CaSO4⋅2H2O/MgCl2.
- (4)
- After doping magnesium salt whiskers into phosphogypsum, the compressive strength increased by 14.72%; the compressive strength of pure phosphogypsum blocks is 26.35 MPa. After doping with Mg salt, the compressive strength increases to 30.23 MPa, an improvement of 14.72%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tayibi, H.; Choura, M.; López, F.A.; Alguacil, F.J.; López-Delgado, A. Environmental impact and management of phosphogypsum. J. Environ. Manag. 2009, 90, 2377–2386. [Google Scholar] [CrossRef] [PubMed]
- Lütke, S.F.; Oliveira, M.L.S.; Silva, L.F.O.; Cadaval, T.R.S.; Dotto, G.L. Nanominerals assemblages and hazardous elements assessment in phosphogypsum from an abandoned phosphate fertilizer industry. Chemosphere 2020, 256, 127138. [Google Scholar] [CrossRef]
- Meskini, S.; Mechnou, I.; Benmansour, M.; Remmal, T.; Samdi, A. Environmental investigation on the use of a phosphogypsum-based road material: Radiological and leaching assessment. J. Environ. Manag. 2023, 345, 118597. [Google Scholar] [CrossRef]
- Baolin, K.; Qin, Z.; Xianhai, L.; Zhihui, S. Adsorption and solidification of cadmium by calcium sulfate dihydrate (gypsum) in an aqueous environment: A dispersion-corrected DFT and ab initio molecular dynamics study. Phys. Chem. Chem. Phys. 2022, 24, 9521–9533. [Google Scholar] [CrossRef] [PubMed]
- Xiao, J.; Lu, T.; Zhuang, Y.; Jin, H. A Novel Process to Recover Gypsum from Phosphogypsum. Materials 2022, 15, 1944. [Google Scholar] [CrossRef]
- Outbakat, M.B.; El Mejahed, K.; El Gharous, M.; El Omari, K.; Beniaich, A. Effect of Phosphogypsum on Soil Physical Properties in Moroccan Salt-Affected Soils. Sustainability 2022, 14, 13087. [Google Scholar] [CrossRef]
- Wu, F.; Ren, Y.; Qu, G.; Liu, S.; Chen, B.; Liu, X.; Zhao, C.; Li, J. Utilization path of bulk industrial solid waste: A review on the multi-directional resource utilization path of phosphogypsum. J. Environ. Manag. 2022, 313, 114957. [Google Scholar] [CrossRef]
- Bilal, E.; Bellefqih, H.; Bourgier, V.; Mazouz, H.; Dumitraş, D.-G.; Bard, F.; Laborde, M.; Caspar, J.P.; Guilhot, B.; Iatan, E.-L.; et al. Phosphogypsum circular economy considerations: A critical review from more than 65 storage sites worldwide. J. Clean. Prod. 2023, 414, 137561. [Google Scholar] [CrossRef]
- Yang, L.; Zhang, Y.; Yan, Y. Utilization of original phosphogypsum as raw material for the preparation of self-leveling mortar. J. Clean. Prod. 2016, 127, 204–213. [Google Scholar] [CrossRef]
- Mashifana, T.; Okonta, F.N.; Ntuli, F. Geotechnical Properties and Application of Lime Modified Phosphogypsum Waste. Mater. Sci. 2018, 24, 312–318. [Google Scholar] [CrossRef]
- Weiksnar, K.D.; Townsend, T.G. Enhancing the chemical performance of phosphogypsum as a road base material by blending with common aggregates. Resour. Conserv. Recycl. 2024, 200, 107300. [Google Scholar] [CrossRef]
- Attallah, M.F.; Metwally, S.S.; Moussa, S.I.; Soliman, M.A. Environmental impact assessment of phosphate fertilizers and phosphogypsum waste: Elemental and radiological effects. Microchem. J. 2019, 146, 789–797. [Google Scholar] [CrossRef]
- Garbaya, H.; Jraba, A.; Khadimallah, M.A.; Elaloui, E. The Development of a New Phosphogypsum-Based Construction Material: A Study of the Physicochemical, Mechanical and Thermal Characteristics. Materials 2021, 14, 7369. [Google Scholar] [CrossRef]
- Meskini, S.; Samdi, A.; Ejjaouani, H.; Remmal, T. Valorization of phosphogypsum as a road material: Stabilizing effect of fly ash and lime additives on strength and durability. J. Clean. Prod. 2021, 323, 129161. [Google Scholar] [CrossRef]
- Chen, S.; Chen, J.; He, X.; Su, Y.; Jin, Z.; Fan, J.; Qi, H.; Wang, B. Comparative analysis of colloid-mechanical microenvironments on the efficient purification of phosphogypsum. Constr. Build. Mater. 2023, 392, 132037. [Google Scholar] [CrossRef]
- Ren, K.; Cui, N.; Zhao, S.; Zheng, K.; Ji, X.; Feng, L.; Cheng, X.; Xie, N. Low-Carbon Sustainable Composites from Waste Phosphogypsum and Their Environmental Impacts. Crystals 2021, 11, 719. [Google Scholar] [CrossRef]
- Silva, L.F.O.; Oliveira, M.L.S.; Crissien, T.J.; Santosh, M.; Bolivar, J.; Shao, L.; Dotto, G.L.; Gasparotto, J.; Schindler, M. A review on the environmental impact of phosphogypsum and potential health impacts through the release of nanoparticles. Chemosphere 2022, 286, 131513. [Google Scholar] [CrossRef] [PubMed]
- Qin, X.; Cao, Y.; Guan, H.; Hu, Q.; Liu, Z.; Xu, J.; Hu, B.; Zhang, Z.; Luo, R. Resource utilization and development of phosphogypsum-based materials in civil engineering. J. Clean. Prod. 2023, 387, 135858. [Google Scholar] [CrossRef]
- Rashad, A.M. Phosphogypsum as a construction material. J. Clean. Prod. 2017, 166, 732–743. [Google Scholar] [CrossRef]
- Yang, Q.; Xiang, Z.; Liu, T.; Deng, C.; Zhang, H. Study on the Mechanical Properties of Cast-In-Situ Phosphogypsum as Building Material for Structural Walls. Materials 2023, 16, 1481. [Google Scholar] [CrossRef]
- Diwa, R.R.; Tabora, E.U.; Palattao, B.L.; Haneklaus, N.H.; Vargas, E.P.; Reyes, R.Y.; Ramirez, J.D. Evaluating radiation risks and resource opportunities associated with phosphogypsum in the Philippines. J. Radioanal. Nucl. Chem. 2021, 331, 967–974. [Google Scholar] [CrossRef]
- Ma, L.; Zhang, H.; Wang, X.; Chen, L. Application and Development Prospects of Phosphogypsum in Different Phases: A Review. Preprints 2023. [Google Scholar] [CrossRef]
- Xue, G.; Yilmaz, E.; Song, W.; Cao, S. Mechanical, flexural and microstructural properties of cement-tailings matrix composites: Effects of fiber type and dosage. Compos. Part B Eng. 2019, 172, 131–142. [Google Scholar] [CrossRef]
- Flores Medina, N.; Barbero-Barrera, M.M. Mechanical and physical enhancement of gypsum composites through a synergic work of polypropylene fiber and recycled isostatic graphite filler. Constr. Build. Mater. 2017, 131, 165–177. [Google Scholar] [CrossRef]
- Ang, C.N.; Wang, Y.C. Effect of moisture transfer on specific heat of gypsum plasterboard at high temperatures. Constr. Build. Mater. 2009, 23, 675–686. [Google Scholar] [CrossRef]
- Jia, R.; Wang, Q.; Feng, P. A comprehensive overview of fibre-reinforced gypsum-based composites (FRGCs) in the construction field. Compos. Part B Eng. 2021, 205, 108540. [Google Scholar] [CrossRef]
- Romero-Hermida, M.I.; Flores-Ales, V.; Hurtado-Bermudez, S.J.; Santos, A.; Esquivias, L. Environmental Impact of Phosphogypsum-Derived Building Materials. Int. J. Environ. Res. Public Health 2020, 17, 4248. [Google Scholar] [CrossRef]
- Nizevičienė, D.; Vaičiukynienė, D.; Michalik, B.; Bonczyk, M.; Vaitkevičius, V.; Jusas, V. The treatment of phosphogypsum with zeolite to use it in binding material. Constr. Build. Mater. 2018, 180, 134–142. [Google Scholar] [CrossRef]
- Wu, F.; Jin, C.; Qu, G.; Liu, Y.; Wang, C.; Chen, B.; Liu, S.; Li, J. Enhancement of phosphogypsum mechanical block with the addition of iron and aluminum salts. J. Build. Eng. 2022, 52, 104397. [Google Scholar] [CrossRef]
- Wang, D.; Chen, C.; Wang, Y.; Jiu, S.; Chen, Y. Influence of modified calcium sulfate hemihydrate whisker on the physical, mechanical, and microscopic properties of gypsum matrix composites. Constr. Build. Mater. 2023, 394, 132280. [Google Scholar] [CrossRef]
- Rovero, L.; Galassi, S.; Misseri, G. Experimental and analytical investigation of bond behavior in glass fiber-reinforced composites based on gypsum and cement matrices. Compos. Part B Eng. 2020, 194, 108051. [Google Scholar] [CrossRef]
- Zhu, C.; Zhang, J.; Peng, J.; Cao, W.; Liu, J. Physical and mechanical properties of gypsum-based composites reinforced with PVA and PP fibers. Constr. Build. Mater. 2018, 163, 695–705. [Google Scholar] [CrossRef]
- Skripnyak, N.V.; Ponomareva, A.V.; Belov, M.P.; Syutkin, E.A.; Khvan, A.V.; Dinsdale, A.T.; Abrikosov, I.A. Mixing enthalpies of alloys with dynamical instability: Bcc Ti-V system. Acta Mater. 2020, 188, 145–154. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, D.; Nagaumi, H.; Zhou, Y.; Yu, W.; Chong, X.; Li, X.; Zhang, H. Morphology, thermal stability, electronic structure and mechanical properties of α-AlFeMnSi phases with varying Mn/Fe atomic ratios: Experimental studies and DFT calculations. J. Alloys Compd. 2022, 901, 163523. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhu, S.; Dai, F.-Z.; Xiang, H.; Liu, Y.; Liu, L.; Ma, Z.; Wu, S.; Liu, F.; Sun, K.; et al. Theoretical predictions and experimental verification on the phase stability of enthalpy-stabilized HE TMREB2s. J. Mater. Sci. Technol. 2022, 121, 154–162. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, M.; Cavin, J.; Flores, K.; Mishra, R. A Fast and Robust Method for Predicting the Phase Stability of Refractory Complex Concentrated Alloys using Pairwise Mixing Enthalpy. Acta Mater. 2022, 241, 118389. [Google Scholar] [CrossRef]
- Ullah, H.M.N.; Rizwan, M.; Ali, S.S.; Usman, Z.; Cao, C. A DFT study of optical, elastic, mechanical, and overall water-splitting photocatalytic properties of pristine and Cd substituted BaZrO3: A lead free environment friendly material. Mater. Sci. Eng. B 2022, 286, 116041. [Google Scholar] [CrossRef]
- Zeng, Q.; Wang, Y.; Yang, C.; Guan, K.; Liu, J.; Deng, Q.; Gao, Y. First-principles study on predicting the crystal structures, mechanical properties and electronic structures of HfCxN1-x. J. Eur. Ceram. Soc. 2021, 41, 3037–3044. [Google Scholar] [CrossRef]
- Song, X.; Ding, Y.; Zhang, J.; Jiang, C.; Liu, Z.; Lin, C.; Zheng, W.; Zeng, Y. Thermophysical and mechanical properties of cubic, tetragonal and monoclinic ZrO2. J. Mater. Res. Technol. 2023, 23, 648–655. [Google Scholar] [CrossRef]
- Yan, M.; Wei, H.; Shi, X.; Jiang, Y. The influence of rare earth elements (Y, Sc) on the interfacial binding properties between the precipitation phases Fe2B, FeB and the matrix Fe in boron steel. Vacuum 2023, 215, 112287. [Google Scholar] [CrossRef]
- Wu, Y.; Ma, L.; Zhou, X.; Duan, Y.; Shen, L.; Peng, M. Insights to electronic structures, elastic properties, fracture toughness, and thermal properties of M23C6 carbides. Int. J. Refract. Met. Hard Mater. 2022, 109, 105985. [Google Scholar] [CrossRef]
- Li, Y.; Liu, Y.; Yang, J. First principle calculations and mechanical properties of the intermetallic compounds in a laser welded steel/aluminum joint. Opt. Laser Technol. 2020, 122, 105875. [Google Scholar] [CrossRef]
- Wang, G.; Jiang, Y.; Li, Z.; Chong, X.; Feng, J. Balance between strength and ductility of dilute Fe2B by high-throughput first-principles calculations. Ceram. Int. 2021, 47, 4758–4768. [Google Scholar] [CrossRef]
- Bafekry, A.; Stampfl, C.; Ghergherehchi, M.; Farjami Shayesteh, S. A first-principles study of the effects of atom impurities, defects, strain, electric field and layer thickness on the electronic and magnetic properties of the C2N nanosheet. Carbon 2020, 157, 371–384. [Google Scholar] [CrossRef]
- Li, Z.; Lv, Q.; Wei, H.; Zhou, M.; Zhang, F.; Shan, Q. Effects of RE (Nd, Ce, Y, La) on TiC(100)/Fe(110) interface based on first-principles calculations. Vacuum 2022, 205, 111449. [Google Scholar] [CrossRef]
- Li, Z.; Peng, M.; Wei, H.; Zhang, W.; Lv, Q.; Zhang, F.; Shan, Q. First-principles study on surface corrosion of 6082 aluminum alloy in H+ and Cl− medium. J. Mol. Struct. 2023, 1294, 136570. [Google Scholar] [CrossRef]
- Yuan, X.; Xiao, Y.; Wang, G.; Zhang, L. TiN inducing ferrite nucleation based on the bcc-Fe/TiN interfaces formation at atomic scale by first-principles calculation. Comput. Mater. Sci. 2021, 197, 110570. [Google Scholar] [CrossRef]
- Zhang, W.; Li, Z.; Wei, H.; Xiang, X.; Zhang, F.; Shan, Q. Interfacial structure of WC-Fe metal-matrix composite (WC/Fe3W3C and Fe/Fe3W3C) stability, electronic and mechanical properties from first-principles calculations. Mater. Today Commun. 2022, 33, 104470. [Google Scholar] [CrossRef]
- Zahra, T.; Thamboo, J.; Asad, M. Compressive strength and deformation characteristics of concrete block masonry made with different mortars, blocks and mortar beddings types. J. Build. Eng. 2021, 38, 102213. [Google Scholar] [CrossRef]
- Tawfeeq, W.M.; Ali, T.K.M.; Al-Kumzari, Y.; Al-Hosni, M.; Al-Fazari, K.; Al-Bedwawi, M.; Al-Bashkardi, A. Flexural performance of reinforced concrete beams made by using recycled block aggregates and fibers. Innov. Infrastruct. Solut. 2020, 6, 38. [Google Scholar] [CrossRef]
- Zhao, Z.; Courard, L.; Groslambert, S.; Jehin, T.; LÉOnard, A.; Xiao, J. Use of recycled concrete aggregates from precast block for the production of new building blocks: An industrial scale study. Resour. Conserv. Recycl. 2020, 157, 104786. [Google Scholar] [CrossRef]
- Jian, S.; Yang, X.; Gao, W.; Li, B.; Gao, X.; Huang, W.; Tan, H.; Lei, Y. Study on performance and function mechanisms of whisker modified flue gas desulfurization (FGD) gypsum. Constr. Build. Mater. 2021, 301, 124341. [Google Scholar] [CrossRef]
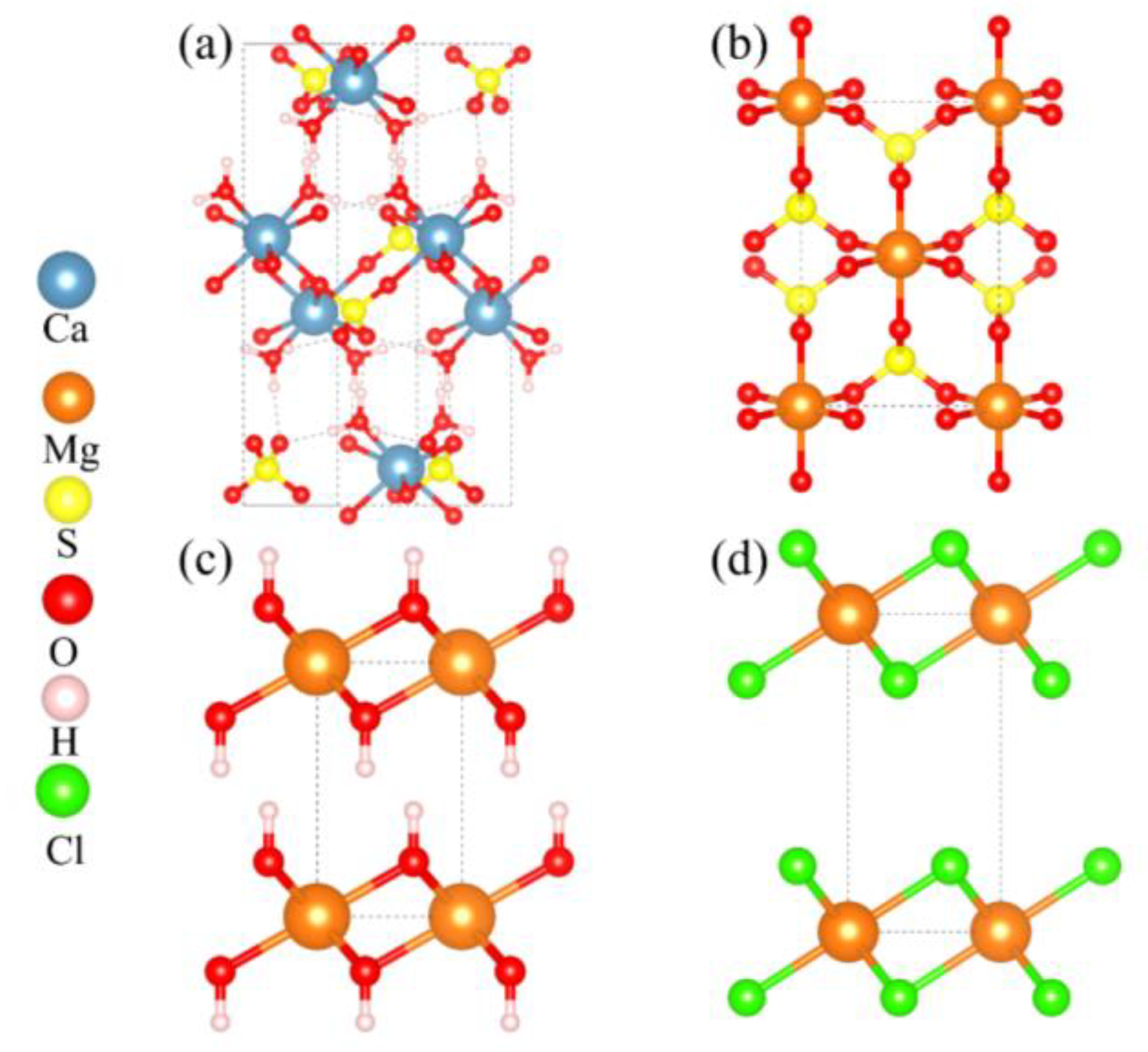
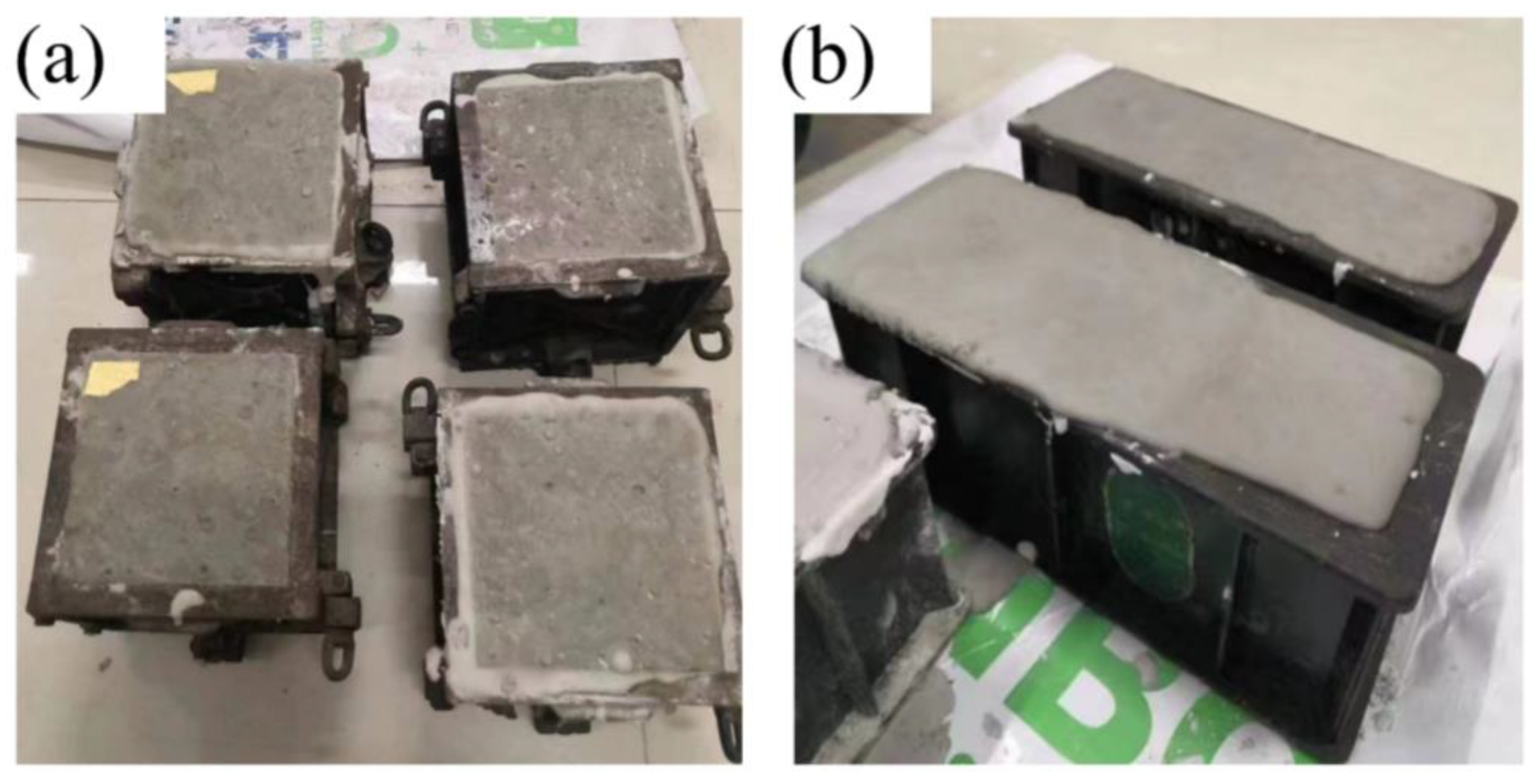



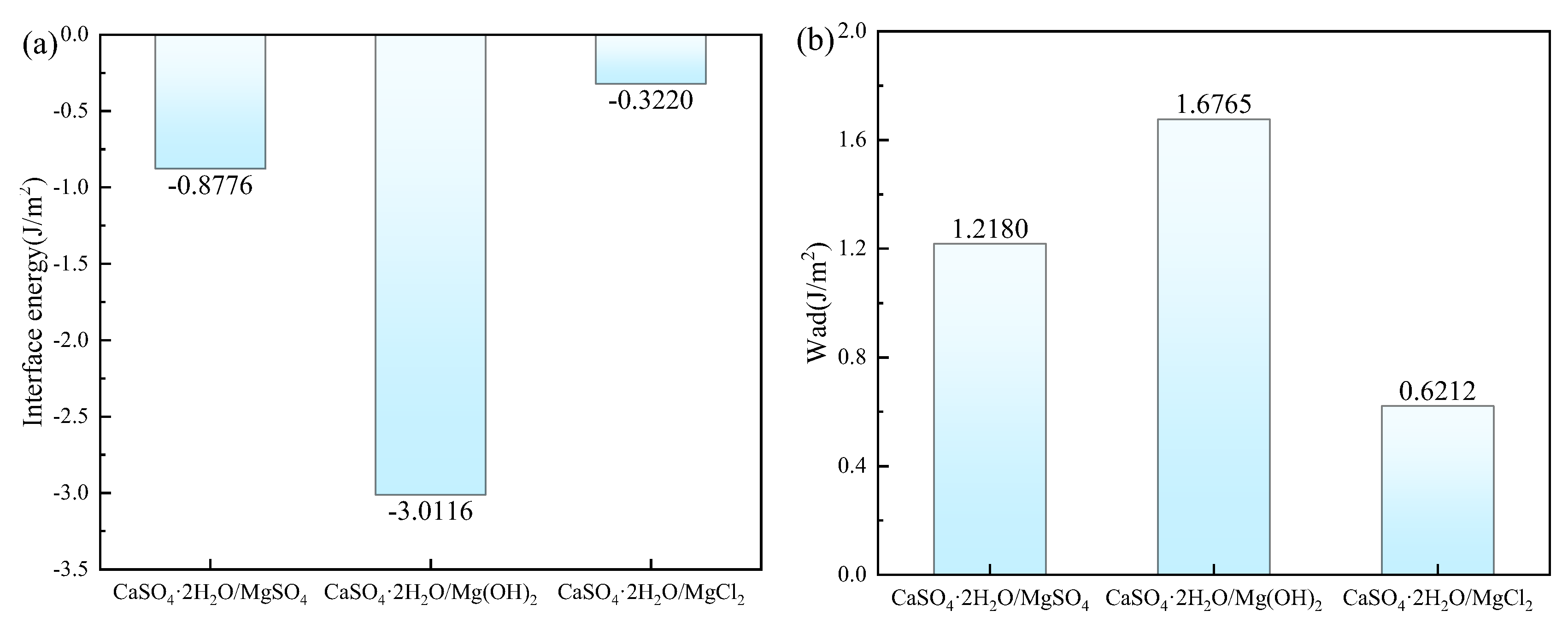

| CaSO4·2H2O | Retarder (SG-12P) | Water Reducer (PC-1050) | MSW | |
|---|---|---|---|---|
| PG | Remainder | 0.2% | 2.3% | — |
| PG-Mg | Remainder | 0.2% | 2.3% | 0.6% |
| Species | C11 | C12 | C13 | C22 | C23 | C33 | C44 | C55 | C66 |
|---|---|---|---|---|---|---|---|---|---|
| CaSO4⋅2H2O | 80.94 | 32.48 | 29.93 | 63.40 | 31.22 | 56.33 | 19.60 | 26.27 | 20.97 |
| Ca(Mg)SO4⋅2H2O | 88.78 | 25.61 | 49.68 | 59.76 | 37.52 | 92.35 | 25.51 | 36.37 | 19.72 |
| CaSO4·2H2O | Ca(Mg)SO4·2H2O | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Bond | (A) | Quantity | Bond | (A) | Quantity | Bond | |||
| H-H | −0.0483 | 2.2855 | 24 | H-H | −0.0459 | 2.2465 | 27 | H-H | −0.0483 |
| H-O | 0.238 | 1.7114 | 40 | H-O | 0.17704 | 1.9927 | 54 | H-O | 0.238 |
| H-S | −0.02 | 2.8971 | 8 | H-S | −0.0225 | 2.8879 | 8 | H-S | −0.02 |
| H-Ca | −0.05 | 2.9642 | 8 | H-Ca | −0.05 | 2.9542 | 6 | H-Ca | −0.05 |
| 44 | H-Mg | −0.11 | 2.6965 | 4 | |||||
| O-O | −0.05 | 2.8898 | 16 | O-O | −0.1018 | 2.6427 | 51 | O-O | −0.05 |
| O-S | 0.545 | 1.4991 | 32 | O-S | 0.5538 | 1.4986 | 16 | O-S | 0.545 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, J.; Yan, M.; Gu, K.; Li, X.; Wei, H. Effect of Magnesium Salt Whiskers on the Mechanical Properties of Phosphogypsum Building Blocks. Materials 2025, 18, 1152. https://doi.org/10.3390/ma18051152
He J, Yan M, Gu K, Li X, Wei H. Effect of Magnesium Salt Whiskers on the Mechanical Properties of Phosphogypsum Building Blocks. Materials. 2025; 18(5):1152. https://doi.org/10.3390/ma18051152
Chicago/Turabian StyleHe, Jiang, Maiping Yan, Kaizhi Gu, Xiangming Li, and He Wei. 2025. "Effect of Magnesium Salt Whiskers on the Mechanical Properties of Phosphogypsum Building Blocks" Materials 18, no. 5: 1152. https://doi.org/10.3390/ma18051152
APA StyleHe, J., Yan, M., Gu, K., Li, X., & Wei, H. (2025). Effect of Magnesium Salt Whiskers on the Mechanical Properties of Phosphogypsum Building Blocks. Materials, 18(5), 1152. https://doi.org/10.3390/ma18051152






