Analysis of Multi-Zone Reaction Mechanisms in BOF Steelmaking and Comprehensive Simulation
Abstract
1. Introduction
2. Multi-Zone Reaction Division of BOF Steelmaking
3. Analysis of the Main Reaction Zones in BOF Steelmaking
3.1. Analysis of the Gas–Liquid Reactions in the Jet Impact Zone
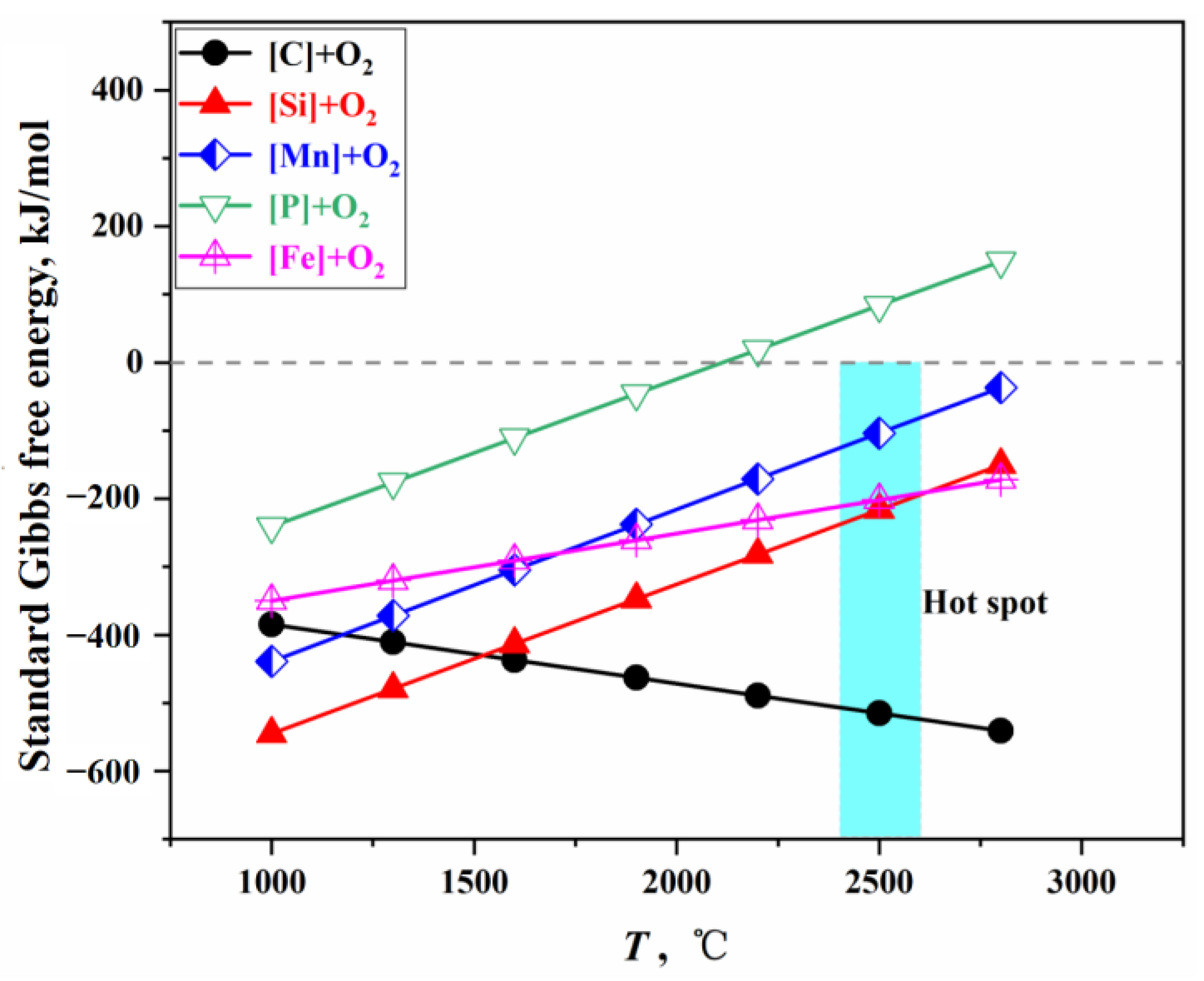
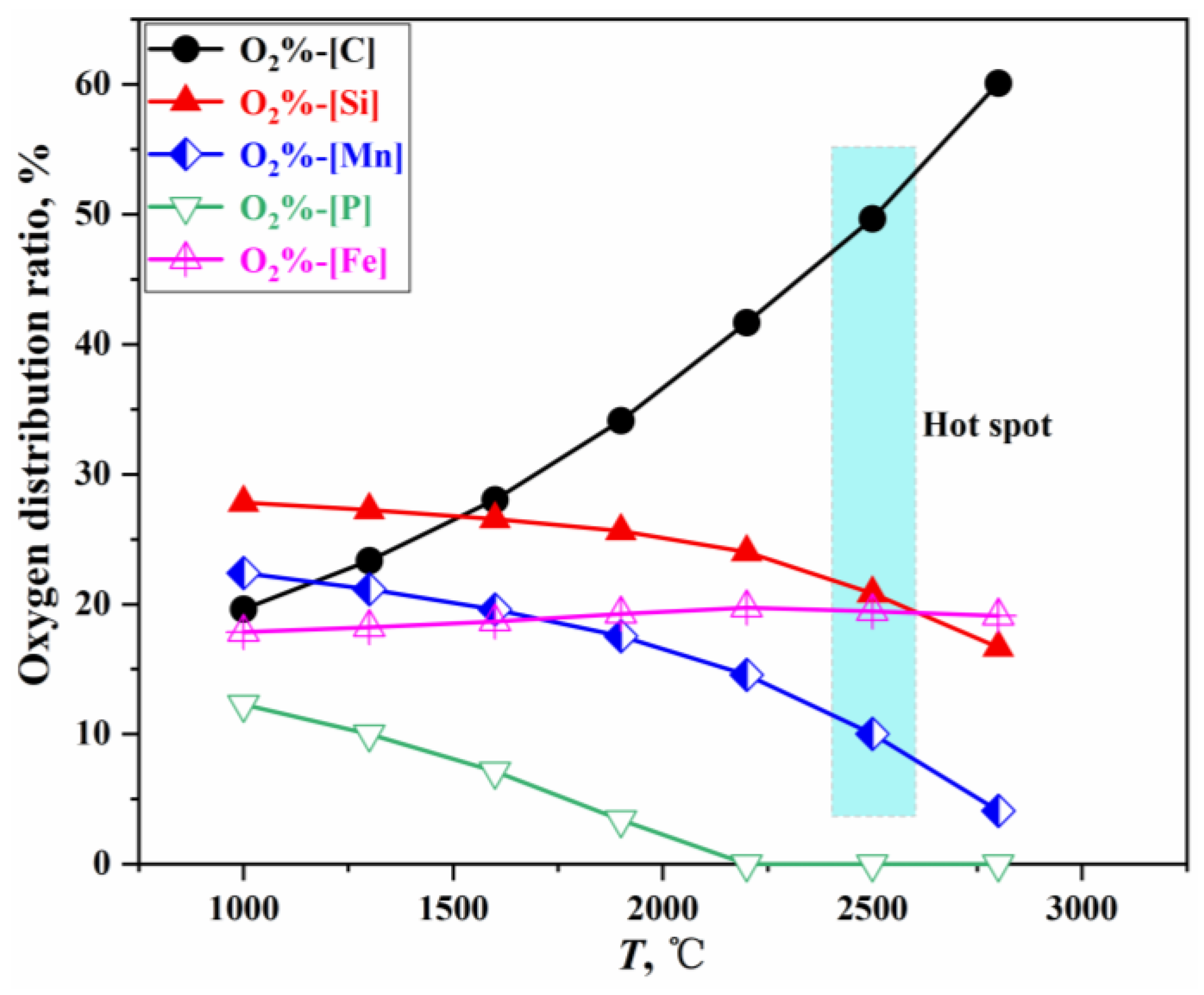
3.1.1. Thermodynamics of the Gas–Liquid Reactions
3.1.2. Dynamics Analysis of the Impact Zone
3.2. Analysis of the Slag–Metal Reaction at the Molten Bath Surface Zone
3.2.1. Thermodynamics of the Slag–Metal Reactions
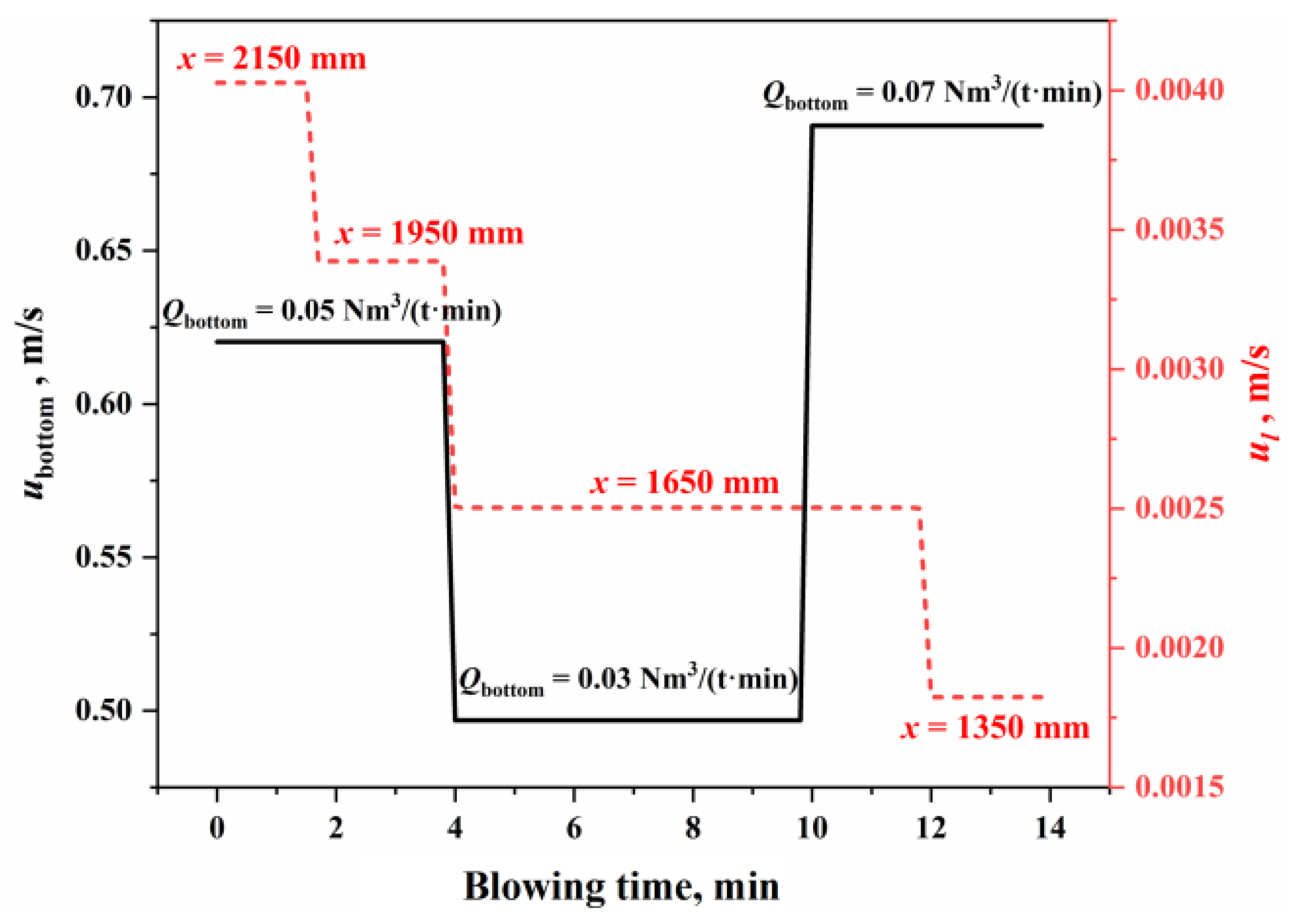
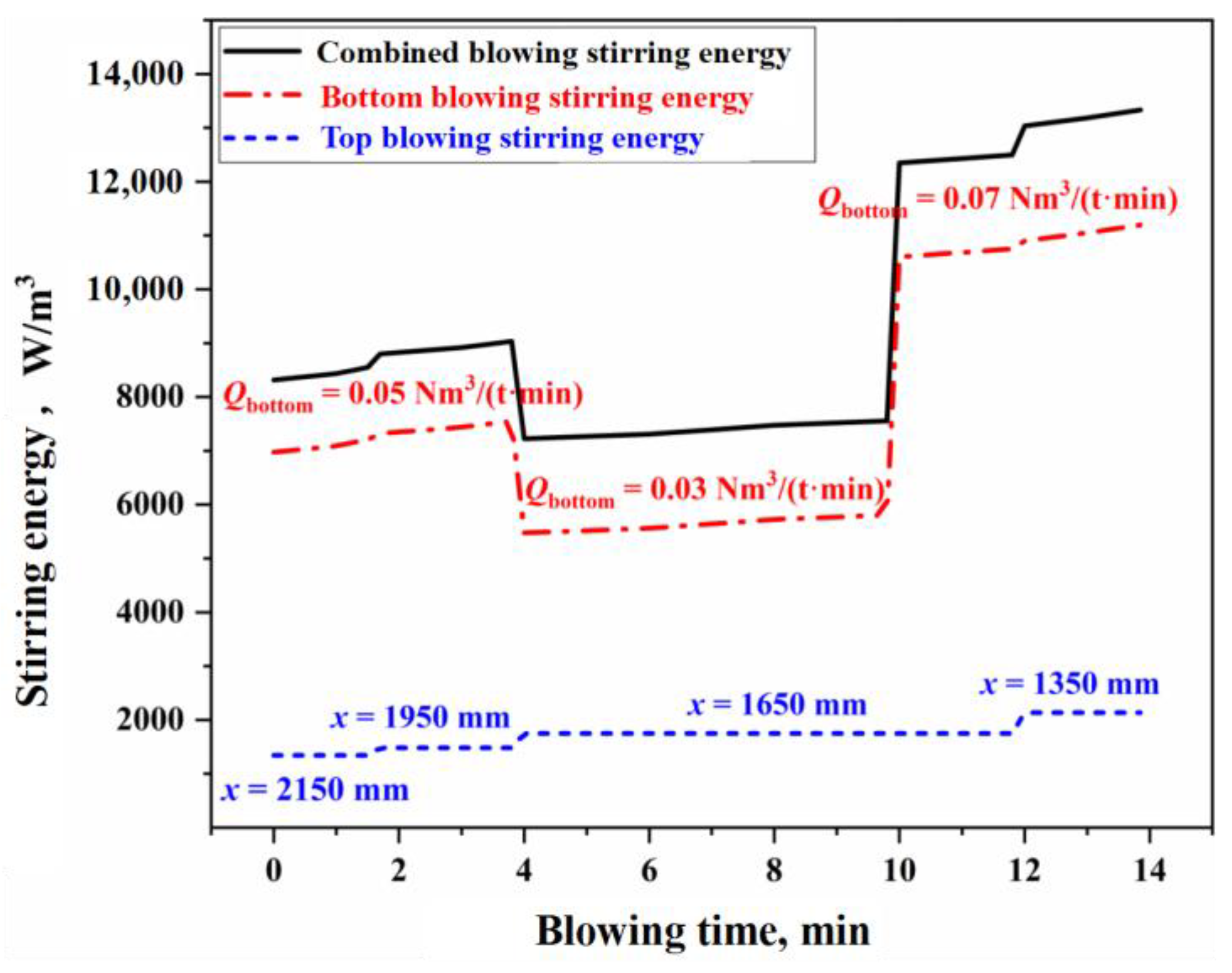
3.2.2. Dynamics Analysis of the Molten Bath Surface Zone
3.3. Analysis of the Metal Droplet Reaction in the Emulsified Zone
3.3.1. Dynamics of the Decarburization Reactions of Metal Droplets
3.3.2. Effective Reaction Amount of Molten Metal in the Emulsified Zone
4. Analysis of the Auxiliary Reaction Zones in BOF Steelmaking
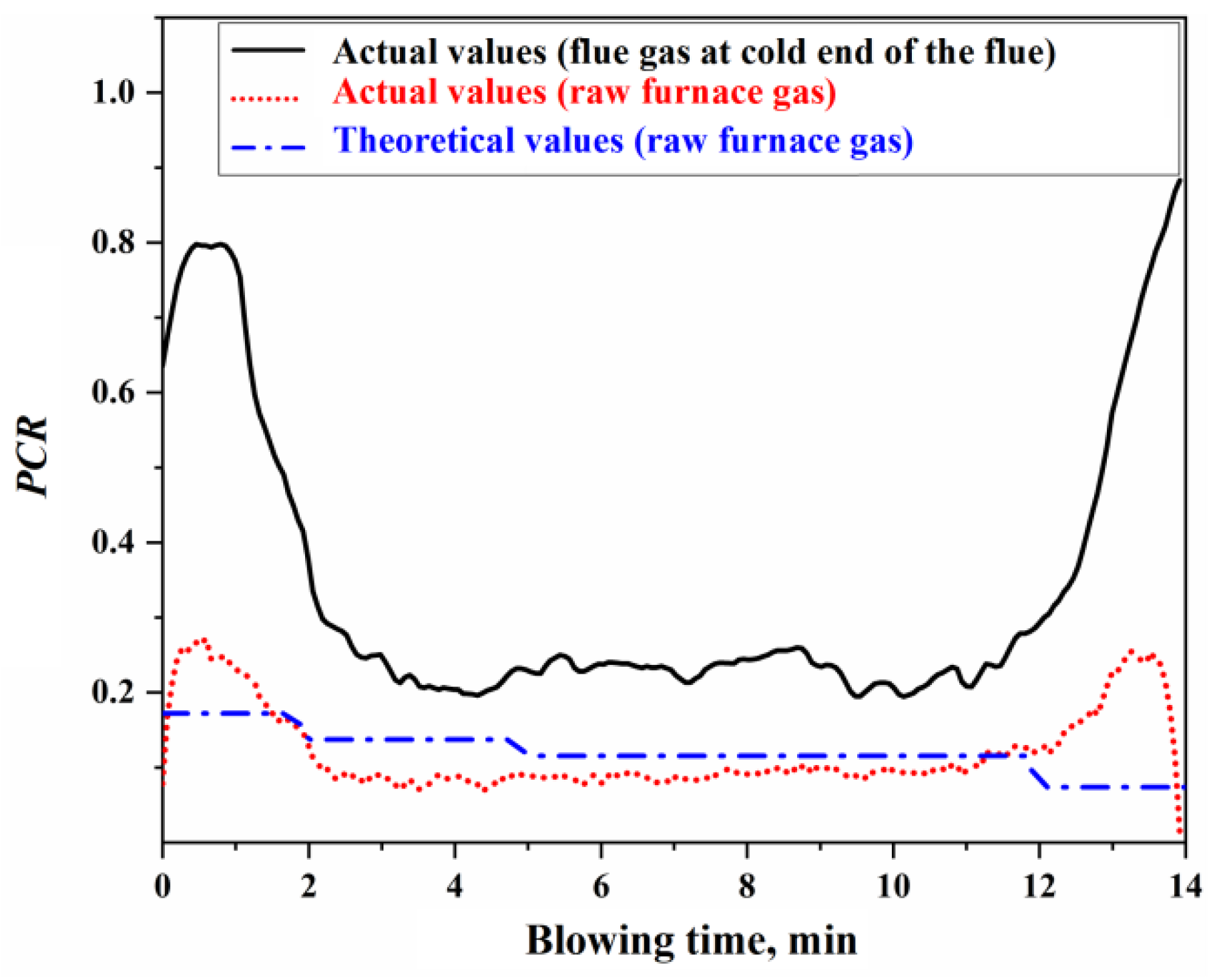
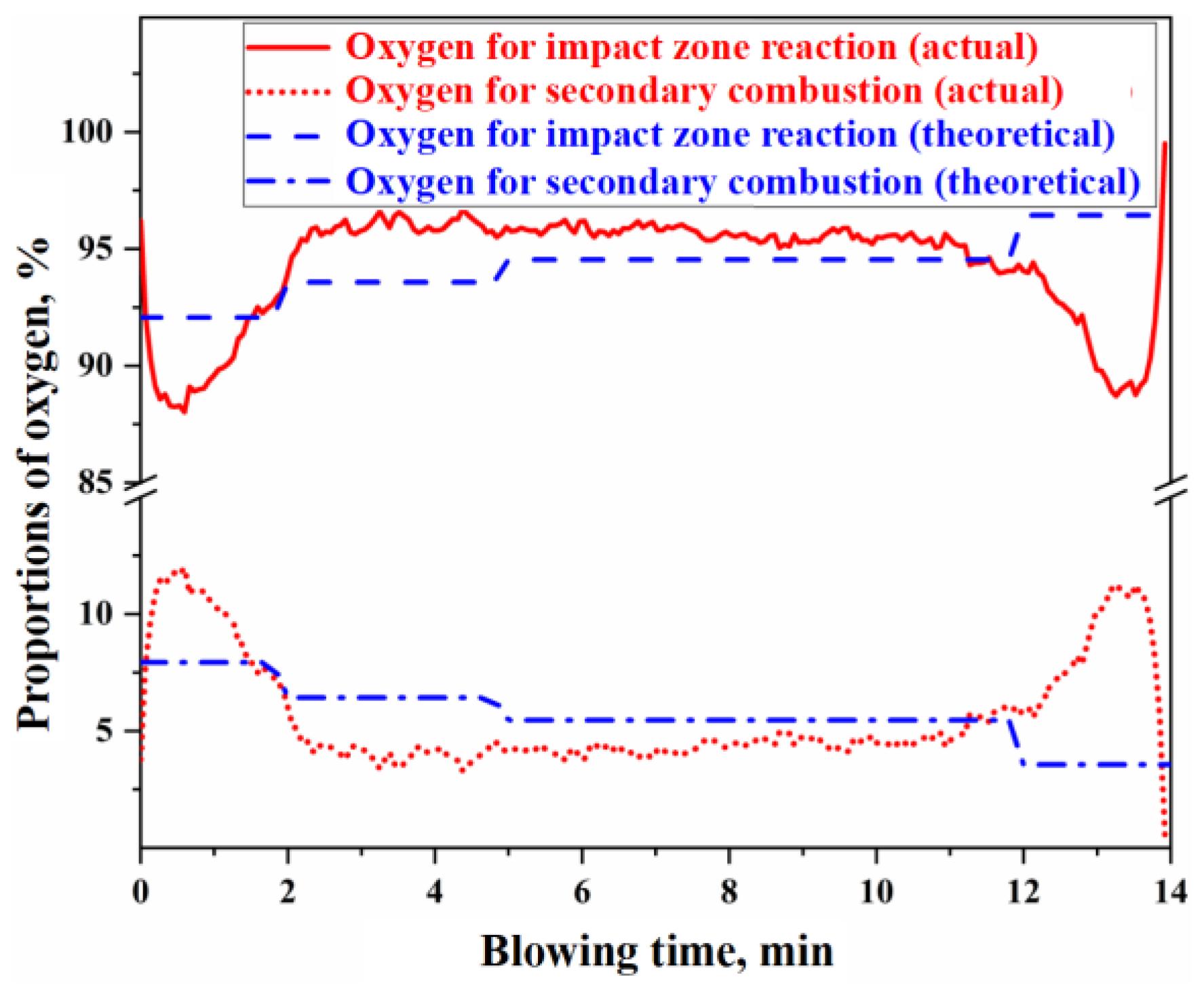
4.1. Analysis of the Secondary Combustion Reaction in the Gas Phase Mixing Zone
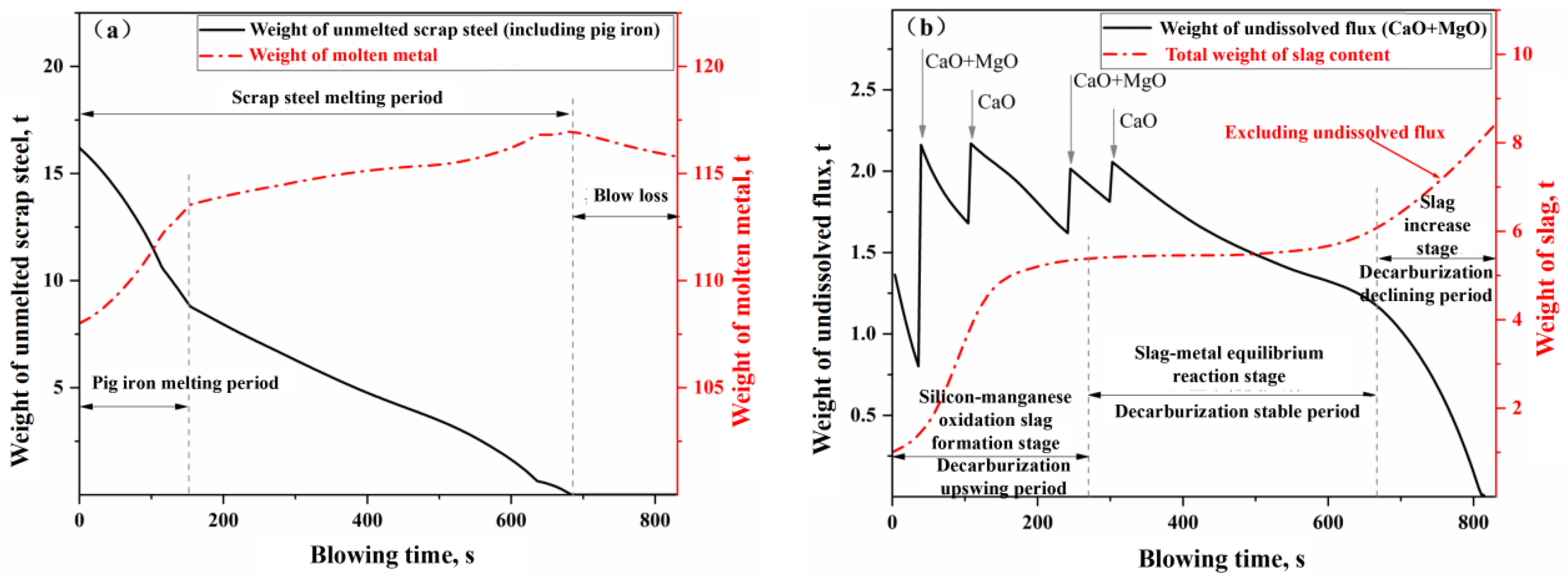
4.2. Analysis of the Scrap Steel Melting Reaction in the Molten Metal Mixing Zone
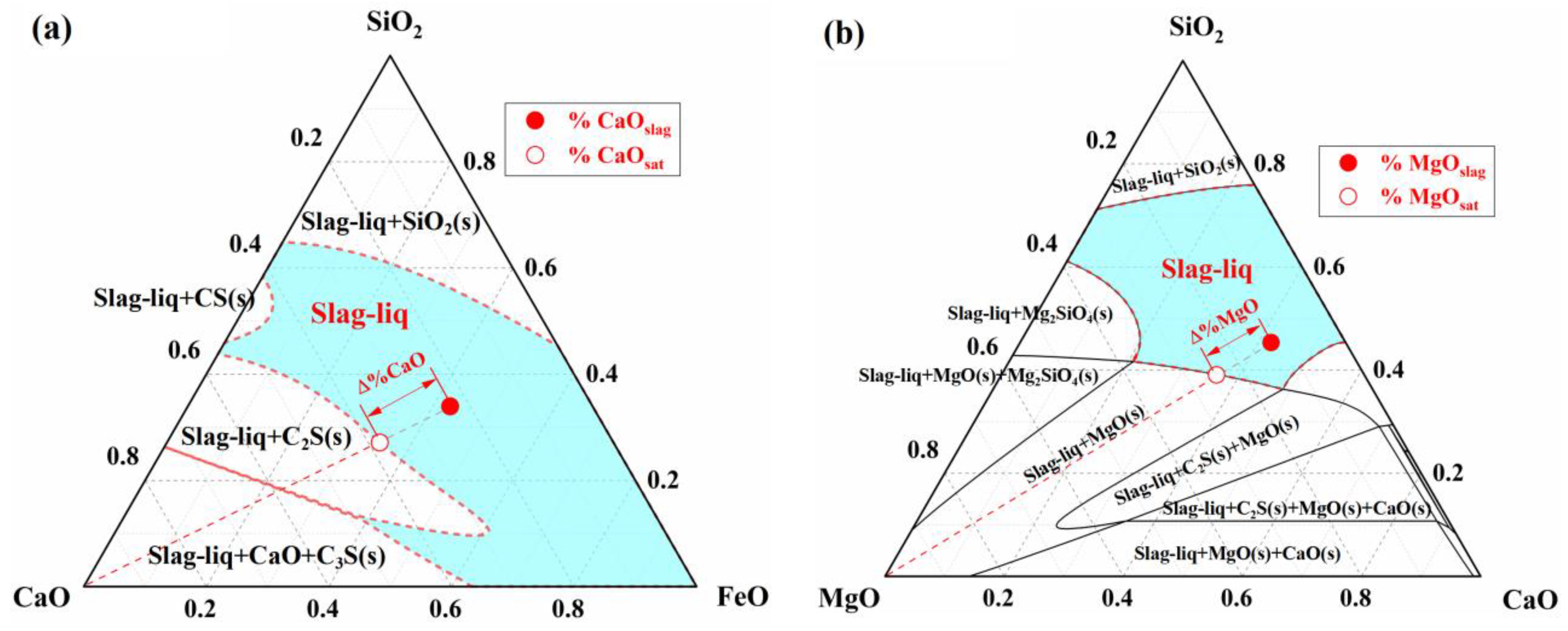
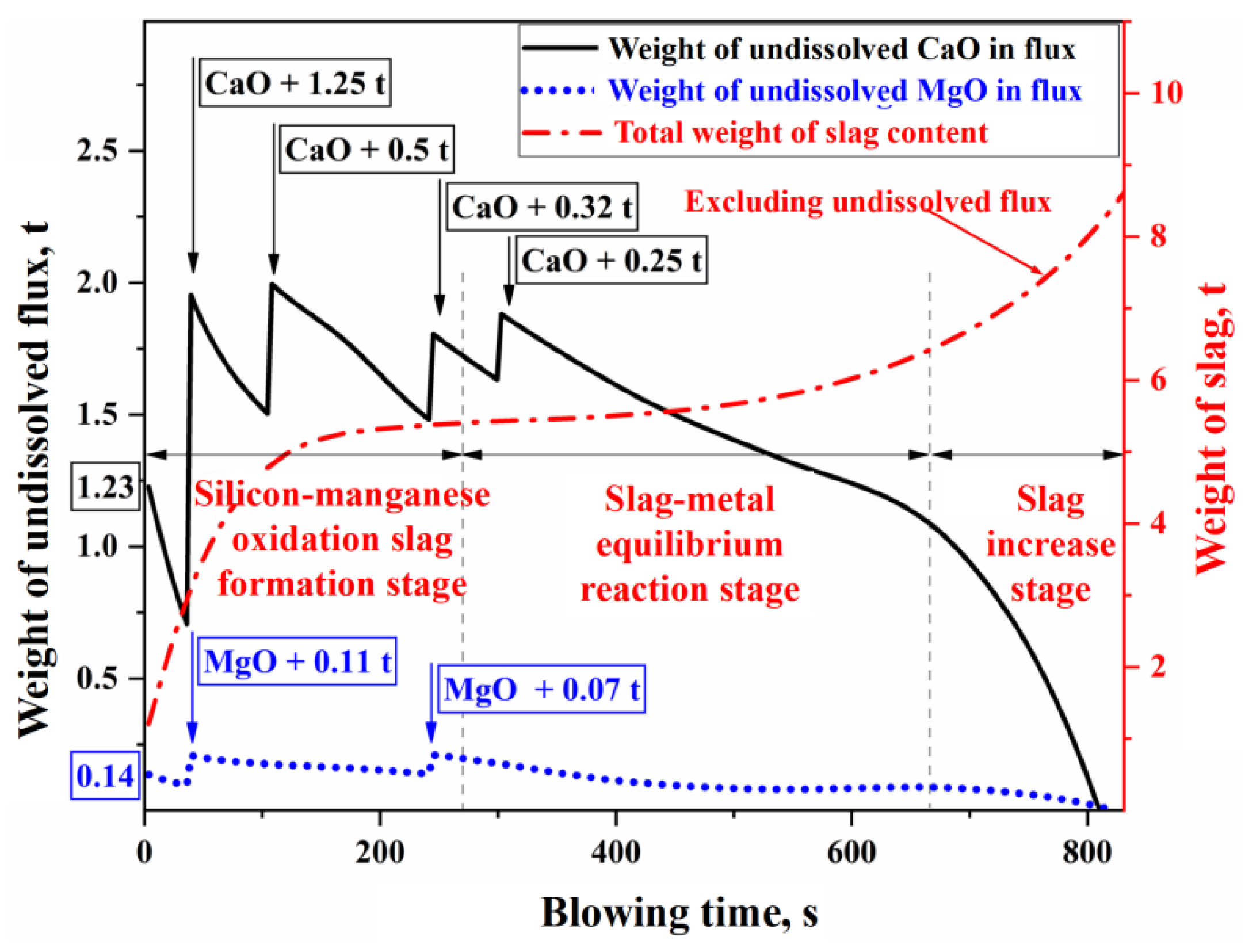
4.3. Analysis of the Flux Dissolution Reaction in the Slag Homogenization Zone
5. Comprehensive Simulation of Multi-Zone Reactions in BOF Steelmaking
5.1. Establishment of a Comprehensive Model of the Multi-Region Reaction Mechanism
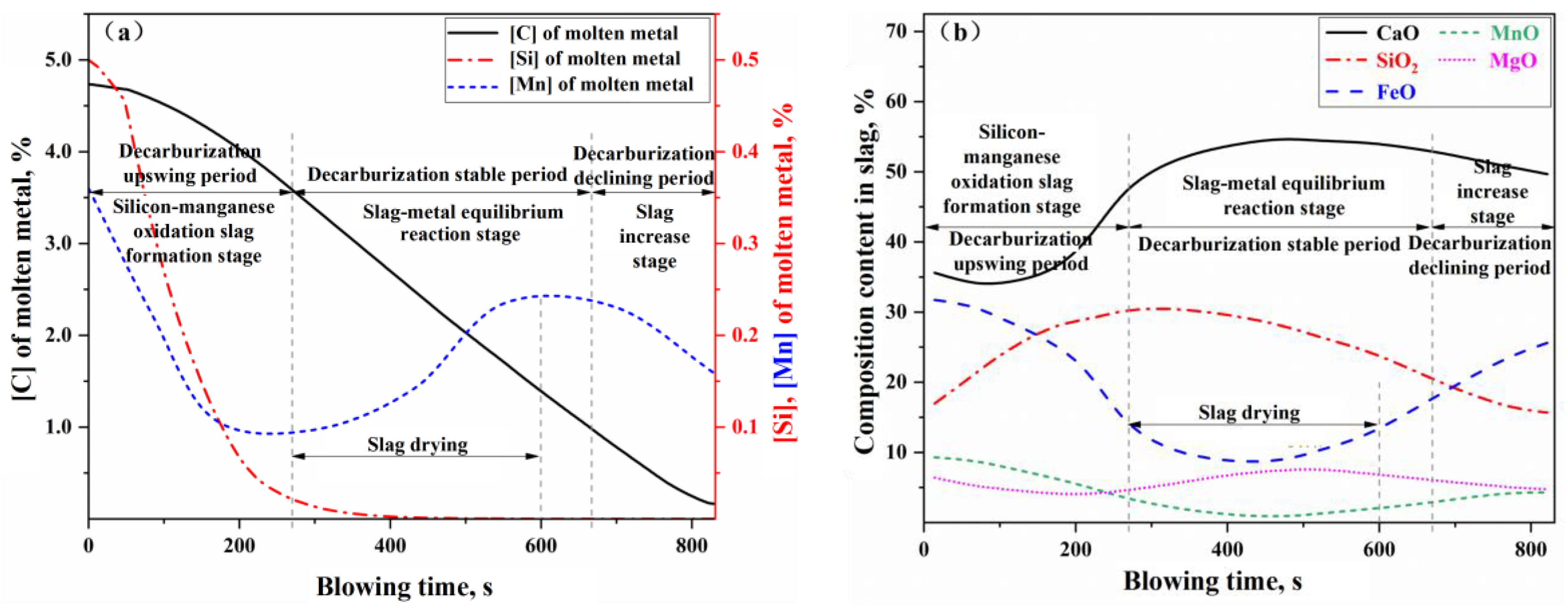
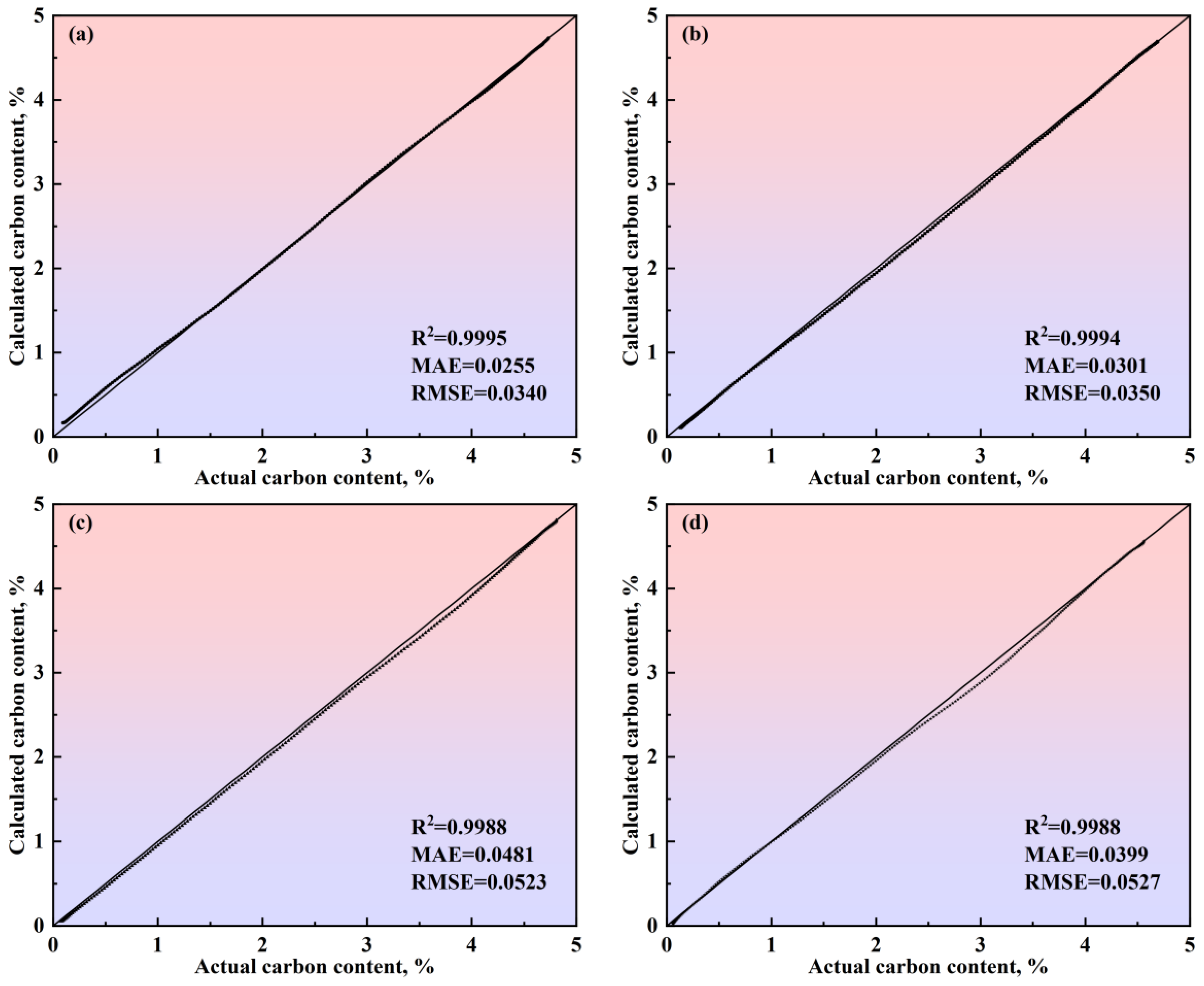
5.2. Discussion on the Simulation Results of the BOF Steelmaking Process
5.3. Evaluation of the Comprehensive Model of the Multi-Region Reaction Mechanism
6. Conclusions
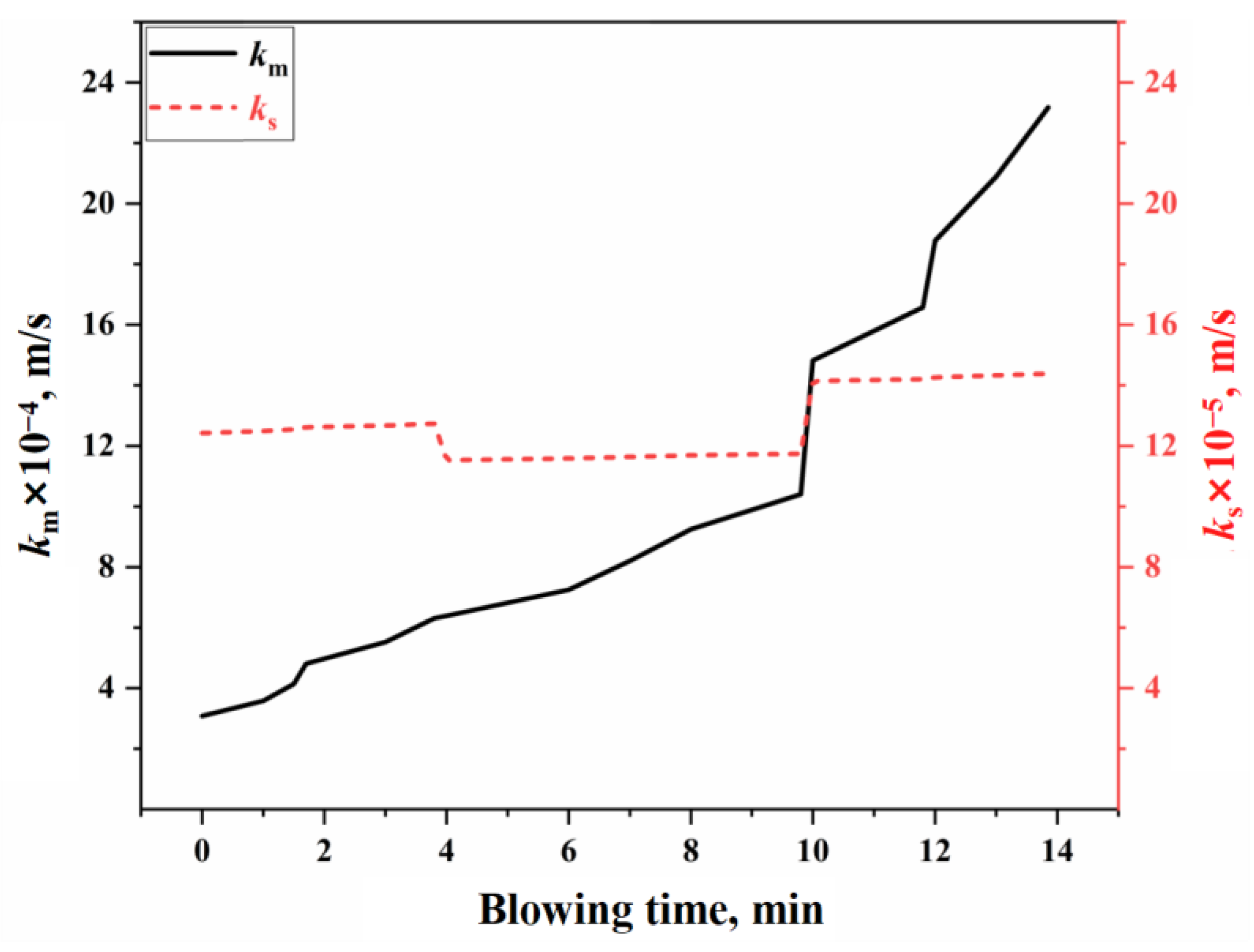
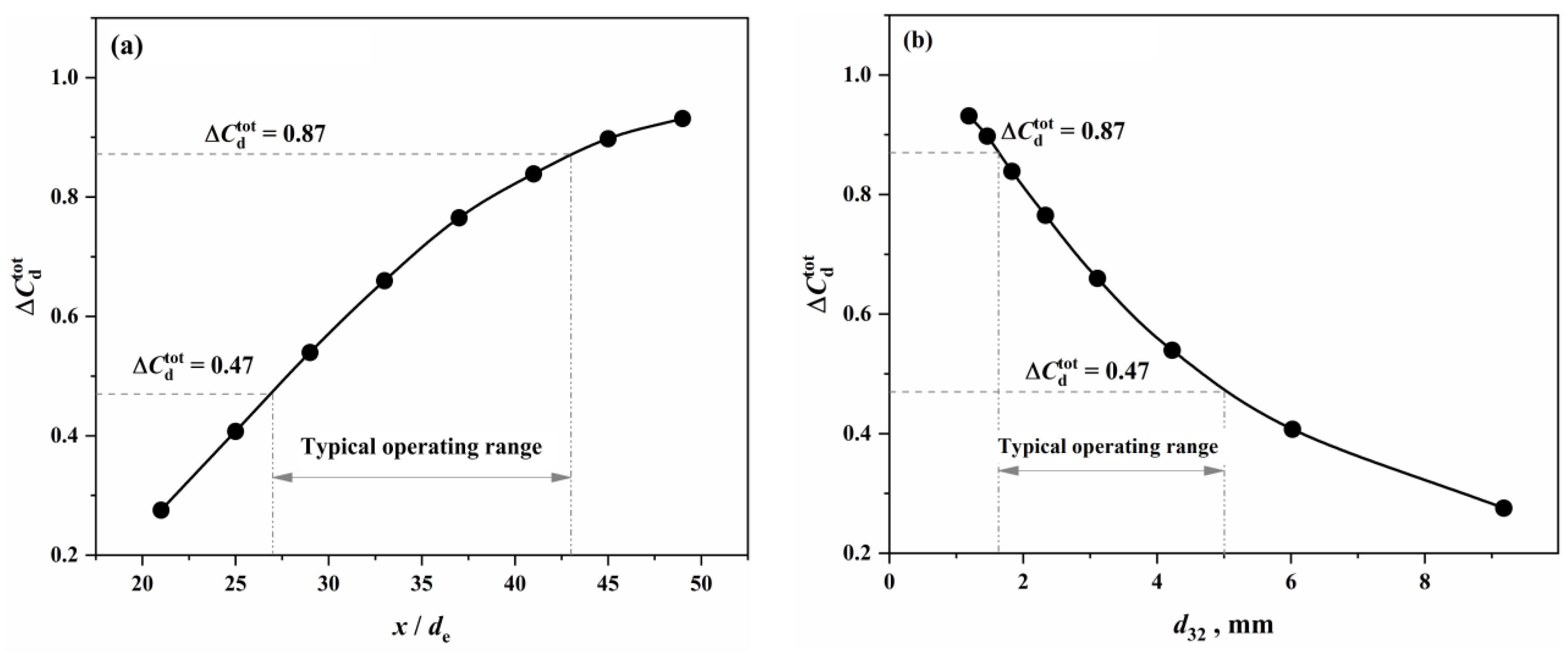
- The oxygen distribution ratio among the elements in the gas–liquid reactions of the jet impact zone is determined by the Gibbs free energy contributions of each reaction. The kinetic conditions for the slag–metal reactions in the molten bath surface zone are primarily controlled by bottom blowing, which contributes approximately 80% to the overall stirring energy. Under typical operating conditions, the effective mass transfer coefficient on the molten metal side is (3.08–23.18) × 10−4 m/s, while, on the slag side, it is (12.42–14.38) × 10−5 m/s. Additionally, the overall effective decarburization rate of the droplets ranges from 0.47 to 0.87.
- In the three auxiliary reaction zones, approximately 6% of the top-blown oxygen is utilized for the secondary combustion of the furnace gas in the gas phase homogenization zone. The melting of scrap steel is influenced by both the carbon concentration and thermal drives, while the melting of pig iron is primarily controlled by thermal mechanisms. The dissolution rate of CaO and MgO fluxes in the slag homogenization zone is determined by the concentration gradient and the stirring intensity of the BOF. The dissolution rates of the fluxes are relatively fast in the early and late stages of the blowing process, while they are slower during the mid-process.
- The multiphase reaction zone was divided into three main reaction zones and three auxiliary reaction zones in the converter, and the reaction mechanisms of the six zones were analyzed from both thermodynamic and kinetic perspectives. Based on this, a multi-zone reaction mechanism model was established, and the accuracy of the model was verified according to actual production data. The results show that the calculated decarburization rate curves and carbon content curves derived from this comprehensive model closely match both the overall profiles and local morphological features of the actual curves from the furnace operation. The relative deviation of the cumulative decarburization rate (i.e., total decarburization amount) ranges from −0.66% to 1.68%, while the absolute deviation of the calculated carbon content curve compared to the actual curve is less than 0.12%. The prediction error for the final carbon content is −0.028–0.073%.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Cao, L.; Wang, Y.; Liu, Q.; Feng, X. Physical and mathematical modeling of multiphase flows in a converter. ISIJ Int. 2018, 58, 573–584. [Google Scholar] [CrossRef]
- Sun, J.; Zhang, J.; Jiang, R.; Feng, X.; Liu, Q. Numerical simulation of droplet splashing behavior in steelmaking converter based on VOF-to-DPM hybrid model and AMR technique. Metall. Mater. Trans. B 2024, 55, 1098–1116. [Google Scholar] [CrossRef]
- Mamoru, M.; Shigeo, A.; Iwao, M. Mathematical model of LD converter process taken account of oxidation of phosphorus and manganese of rate of lime solution. Tetsu–Hagane 1970, 56, 1677–1686. [Google Scholar]
- Shigeo, A.; Iwao, M. Theoretical analysis of LD converter operation by mathematical model considered scrap-melting process. Tetsu–Hagane 1971, 57, 1331–1339. [Google Scholar]
- Lytvynyuk, Y.; Schenk, J.; Hiebler, M.; Sormann, A. Thermodynamic and kinetic model of the converter steelmaking process. Part 1: The description of the BOF model. Steel Res. Int. 2014, 85, 537–543. [Google Scholar] [CrossRef]
- Lytvynyuk, Y.; Schenk, J.; Hiebler, M.; Sormann, A. Thermodynamic and kinetic model of the converter steelmaking process. Part 2: The model validation. Steel Res. Int. 2014, 85, 544–563. [Google Scholar] [CrossRef]
- Dogan, N.; Brooks, G.A.; Rhamdhani, M.A. Comprehensive model of oxygen steelmaking Part 1: Model development and validation. ISIJ Int. 2011, 51, 1086–1092. [Google Scholar] [CrossRef]
- Dogan, N.; Brooks, G.A.; Rhamdhani, M.A. Comprehensive model of oxygen steelmaking Part 2: Application of bloated droplet theory for decarburization in emulsion zone. ISIJ Int. 2011, 51, 1093–1101. [Google Scholar] [CrossRef]
- Dogan, N.; Brooks, G.A.; Rhamdhani, M.A. Comprehensive model of oxygen steelmaking Part 3: Decarburization in impact zone. ISIJ Int. 2011, 51, 1102–1109. [Google Scholar] [CrossRef]
- Brooks, G.A.; Rhamdhani, M.A.; Coley, K.S.; Subagyo Pan, Y. Transient kinetics of slag metal reactions. Metall. Mater. Trans. B 2009, 40, 353–362. [Google Scholar] [CrossRef]
- Cicutti, C.; Valdez, M.; Perez, T.; Donayo, R.; Petroni, J. Analysis of slag foaming during the operation of an industrial converter. Lat. Am. Appl. Res. 2002, 32, 237–240. [Google Scholar]
- Cicutti, C.; Valdez, M.; Pérez, T.; Petroni, J.; Gomez, A.; Donayo, R.; Ferro, L. Study of slag-metal reactions in an LD-LBE converter. In Proceedings of the 6th International Conference on Molten Slags, Fluxes and Salts, Helsinki, Finland, 12–17 June 2000; pp. 367–384. [Google Scholar]
- Rout, B.K.; Brooks, G.; Subagyo Rhamdhani, M.A.; Li, Z. Modeling of droplet generation in a top blowing steelmaking process. Metall. Mater. Trans. B 2016, 47, 3350–3361. [Google Scholar] [CrossRef]
- Rout, B.K.; Brooks, G.A.; Li, Z.; Rhamdhani, M.A. Analysis of desiliconization reaction kinetics in a BOF. In Proceedings of the AISTech 2016 Iron and Steel Technology Conference, Pittsburgh, PA, USA, 16–19 May 2016; pp. 1019–1026. [Google Scholar]
- Rout, B.K.; Brooks, G.A.; Li, Z.; Rhamdhani, A. Dynamic modeling of oxygen steelmaking process: A multi-zone kinetic approach. In Proceedings of the AISTech 2017 Iron and Steel Technology Conference, Nashville, TN, USA, 8–11 May 2017; pp. 1315–1326. [Google Scholar]
- Rout, B.K.; Brooks, G.; Akbar Rhamdhani, M.; Li, Z.; Schrama, F.N.; Overbosch, A. Dynamic model of basic oxygen steelmaking process based on multi-zone reaction kinetics: Modeling of decarburization. Metall. Mater. Trans. B 2018, 49, 1022–1033. [Google Scholar] [CrossRef]
- Rout, B.K.; Brooks, G.; Rhamdhani, M.A.; Li, Z.; Schrama, F.N.; Sun, J. Dynamic model of basic oxygen steelmaking process based on multi-zone reaction kinetics: Model derivation and validation. Metall. Mater. Trans. B 2018, 49, 537–557. [Google Scholar] [CrossRef]
- Rout, B.K.; Brooks, G.; Akbar Rhamdhani, M.; Li, Z.; Schrama, F.N.; van der Knoop, W. Dynamic model of basic oxygen steelmaking process based on multi-zone reaction kinetics: Modeling of manganese removal. Metall. Mater. Trans. B 2018, 49, 2191–2208. [Google Scholar] [CrossRef]
- Chou, K.; Pal, U.B.; Reddy, R.G. A general model for BOP decarburization. ISIJ Int. 1993, 33, 862–868. [Google Scholar] [CrossRef]
- Narita, K.; Makino, T.; Matsumoto, H.; Hikosaka, A.; Katsuda, J.I. Oxidation mechanism of silicon in hot metal. Tetsu–Hagane 1983, 69, 1722–1729. [Google Scholar] [CrossRef]
- Suito, H.; Inoue, R. Thermodynamic assessment of maganese distribution in hot metal and steel. ISIJ Int. 1995, 35, 266–271. [Google Scholar] [CrossRef]
- Biswas, J.; Ghosh, S.; Ballal, N.B.; Basu, S. A dynamic mixed-control model for BOF metal-slag-gas reactions. Metall. Mater. Trans. B 2021, 52, 1309–1321. [Google Scholar] [CrossRef]
- Biswas, J.; Gu, K.Z.; Coley, K.S. A decarburization model for a Fe-C droplet reacting in oxidizing slag. Metall. Mater. Trans. B 2021, 52, 3888–3906. [Google Scholar] [CrossRef]
- Sarkar, R.; Gupta, P.; Basu, S.; Ballal, N.B. Dynamic modeling of LD converter steelmaking: Reaction modeling using Gibbs’ free energy minimization. Metall. Mater. Trans. B 2015, 46, 961–976. [Google Scholar] [CrossRef]
- Kruskopf, A.; Visuri, V. A gibbs energy minimization approach for modeling of chemical reactions in a basic oxygen furnace. Metall. Mater. Trans. B 2017, 48, 3281–3300. [Google Scholar] [CrossRef][Green Version]
- Piro, M.H.A.; Simunovic, S.; Besmann, T.M.; Lewis, B.J.; Thompson, W.T. The thermochemistry library thermochimica. Comput. Mater. Sci. 2013, 67, 266–272. [Google Scholar] [CrossRef]
- Van Ende, M.A.; Kim, Y.M.; Cho, M.K.; Choi, J.; Jung, I.H. A kinetic model for the Ruhrstahl Heraeus (RH) degassing process. Metall. Mater. Trans. B 2011, 42, 477–489. [Google Scholar] [CrossRef]
- Jung, I.H.; Hudon, P.; Kim, W.Y.; Van Ende, M.A.; Rahman, M.; Curiel, G.G. Thermodynamic database of P2O5-containing oxide systems for the dephosphorization process in steelmaking. High Temp. Mater. Process. 2013, 32, 247–254. [Google Scholar] [CrossRef]
- Jung, I.H.; Hudon, P.; Van Ende, M.A.; Kim, W.Y. Thermodynamic database for P2O5-containing slags and its application to the dephosphorization process. In Proceedings of the AISTech 2014 Iron and Steel Technology Conference, Indianapolis, IN, USA, 5–8 May 2014; pp. 1257–1268. [Google Scholar]
- Van Ende, M.A.; Jung, I.H. Development of a thermodynamic database for mold flux and application to the continuous casting process. ISIJ Int. 2014, 54, 489–495. [Google Scholar] [CrossRef]
- Van Ende, M.A.; Jung, I.H. A kinetic BOF process simulation model. In Proceedings of the Asia Steel International Conference 2015, Yokohama, Japan, 5–8 October 2015; pp. 596–597. [Google Scholar]
- Van Ende, M.A.; Jung, I.H. A kinetic process simulation model for basic oxygen furnace (BOF): Importance of slag chemistry for BOF operation. CAMP-ISIJ 2015, 28, 527–530. [Google Scholar]
- Van Ende, M.A.; Jung, I.H. A kinetic ladle furnace process simulation model: Effective equilibrium reaction zone model using FactSage macro processing. Metall. Mater. Trans. B 2017, 48, 28–36. [Google Scholar] [CrossRef]
- Van Ende, M.A.; Jung, I.H. Applications of thermodynamic database to the kinetic steelmaking process simulations. In Computational Materials System Design; Shin, D., Saal, J., Eds.; Springer International Publishing: Cham, Switzerland, 2018; pp. 47–66. [Google Scholar]
- Jung, I.H.; Van Ende, M.A. Computational thermodynamic calculations: FactSage from CALPHAD thermodynamic database to virtual process simulation. Metall. Mater. Trans. B 2020, 51, 1851–1874. [Google Scholar] [CrossRef]
- Krainer, V.H. Zur Metallurgie der Sauerstoffaufblasverfahren. Tetsu–Hagane 1965, 51, 1194–1207. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Huang, X.H. Principle of Ferrous Metallurgy, 4th ed.; Metallurgical Industry Press: Beijing, China, 2013. [Google Scholar]
- Tu, H. Research on Dynamic Process Model of Converter Based on Furnace Gas Detection. Ph.D. Thesis, Shanghai University, Shanghai, China, 2002. [Google Scholar]
- Ding, R.; Blanpain, B.; Jones, P.T.; Wollants, P. Modeling of the vacuum oxygen decarburization refining process. Metall. Mater. Trans. B 2000, 31, 197–206. [Google Scholar] [CrossRef]
- Wei, J.; Zhu, D. Mathematical modeling of the argon-oxygen decarburization refining process of stainless steel: Part I. Mathematical model of the process. Metall. Mater. Trans. B 2002, 33, 111–119. [Google Scholar] [CrossRef]
- Wei, J.-H.; Zhu, D.-P. Mathematical modeling of the argon-oxygen decarburization refining process of stainless steel: Part II. Application of the model to industrial practice. Metall. Mater. Trans. B 2002, 33, 121–127. [Google Scholar] [CrossRef]
- Danckwerts, P.V. Significance of liquid-film coefficients in gas absorption. Ind. Eng. Chem. 1951, 43, 1460–1467. [Google Scholar] [CrossRef]
- Hino, M.; Ito, K. Thermodynamic Data for Steelmaking; Tohoku University Press: Sendai, Japan, 2010. [Google Scholar]
- Hwang, H.Y.; Irons, G.A. A water model study of impinging gas jets on liquid surfaces. Metall. Mater. Trans. B 2012, 43, 302–315. [Google Scholar] [CrossRef]
- Krishnapisharody, K.; Irons, G.A. An analysis of recirculatory flow in gas-stirred ladles. Steel Res. Int. 2010, 81, 880–885. [Google Scholar] [CrossRef]
- Kitamura, S.Y.; Kitamura, T.; Shibata, K.; Mizukami, Y.; Mukawa, S.; Nakagawa, J. Effect of stirring energy, temperature and flux composition on hot metal dephosphorization kinetics. ISIJ Int. 1991, 31, 1322–1328. [Google Scholar] [CrossRef]
- Lin, W.H. Study on Process Behavior Analysis and Decarburization Control of BOF Steelmaking. Ph.D. Thesis, University of Science and Technology Beijing, Beijing, China, 2023. [Google Scholar]
- Li, R.; Harris, R.L. Interaction of gas jets with model process liquids. In Proceedings of the Pyrometallurgy 95, London, UK, 31 May 1995; pp. 107–124. [Google Scholar]
- Subagyo; Brooks, G.A.; Coley, K.S.; Irons, G.A. Generation of droplets in slag-metal emulsions through top gas blowing. ISIJ Int. 2003, 43, 983–989. [Google Scholar] [CrossRef]
- Koria, S.C.; Lange, K.W. A new approach to investigate the drop size distribution in basic oxygen steelmaking. Metall. Mater. Trans. B 1984, 15, 109–116. [Google Scholar] [CrossRef]
- Deo, B.; Boom, R. Fundamentals of Steelmaking Metallurgy; Prentice Hall International: London, UK, 1993. [Google Scholar]
- Hirai, M.; Tsujino, R.; Mukai, T.; Harada, T.; Omori, M. The Mechanism of post combustion in converter. Tetsu–Hagane 1987, 73, 1117–1124. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Kitamura, S.Y.; Shibata, H.; Maruoka, N. Kinetic model of hot metal dephosphorization by liquid and solid coexisting slags. Steel Res. Int. 2008, 79, 586–590. [Google Scholar] [CrossRef]
- Pahlevani, F.; Kitamura, S.; Shibata, H.; Maruoka, N. Simulation of steel refining process in converter. Steel Res. Int. 2010, 81, 617–622. [Google Scholar] [CrossRef]
- Xin, Z.-C.; Zhang, J.-S.; Zheng, J.; Jin, Y.; Liu, Q. A hybrid modeling method based on expert control and deep neural network for temperature prediction of molten steel in LF. ISIJ Int. 2022, 62, 532–541. [Google Scholar] [CrossRef]


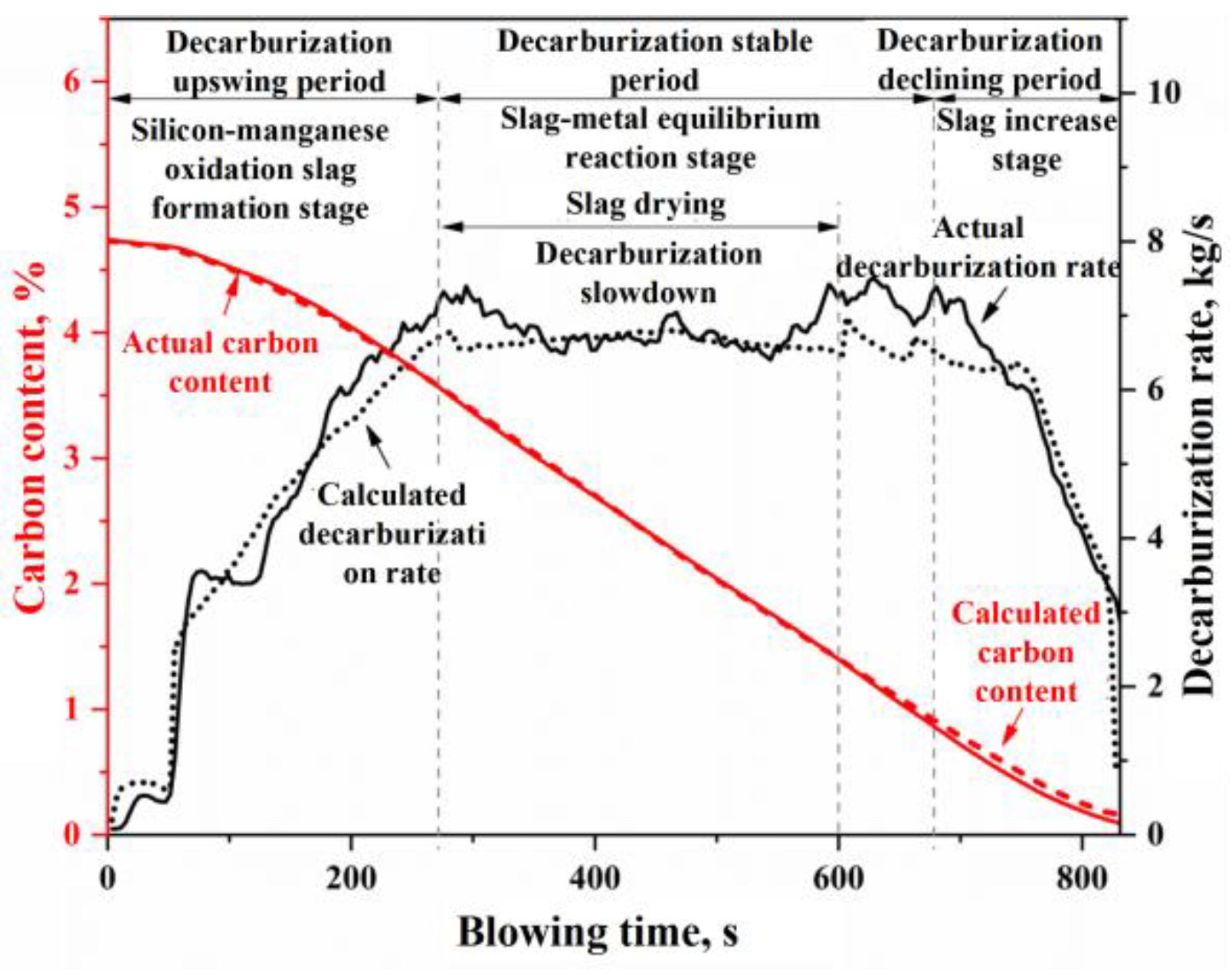
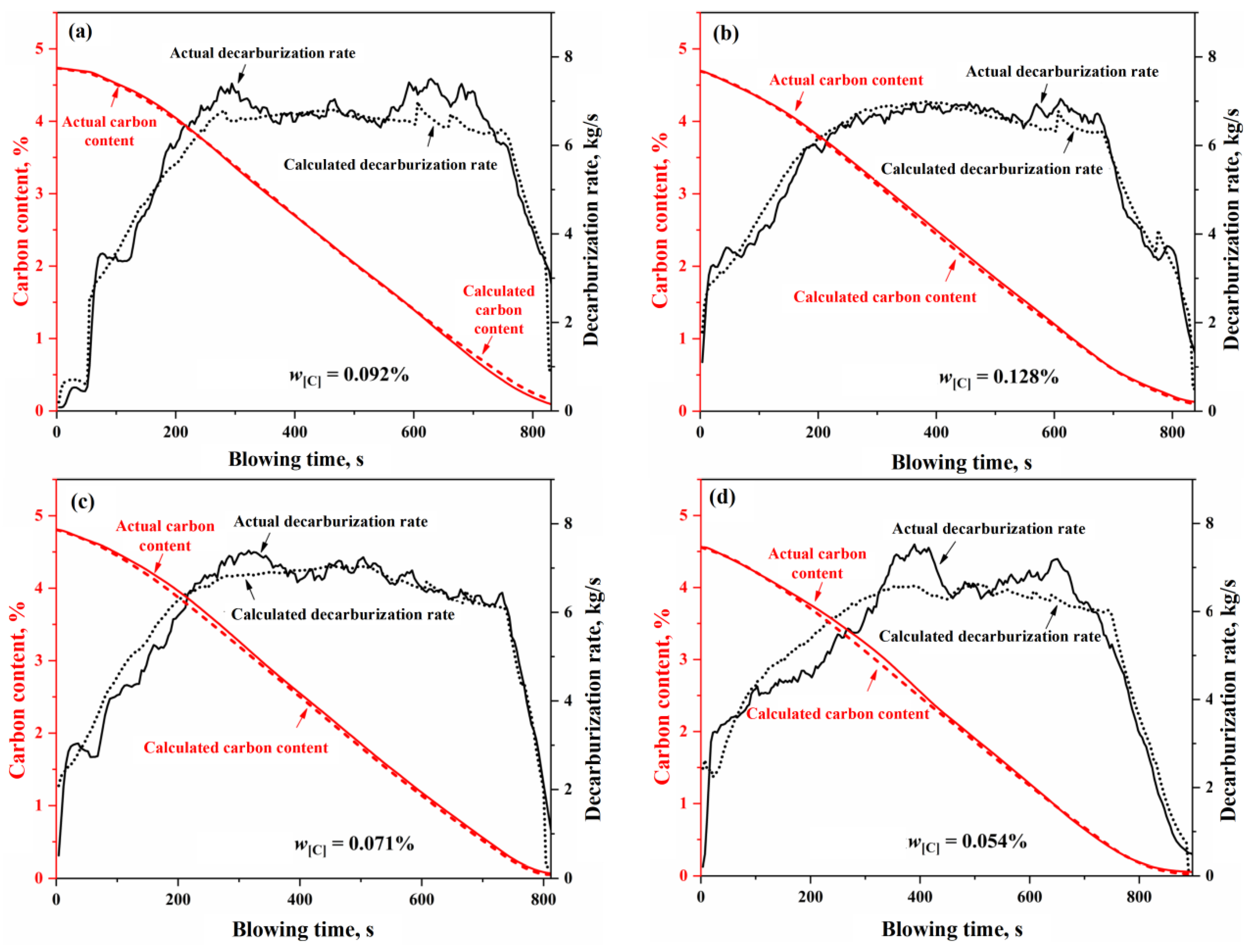
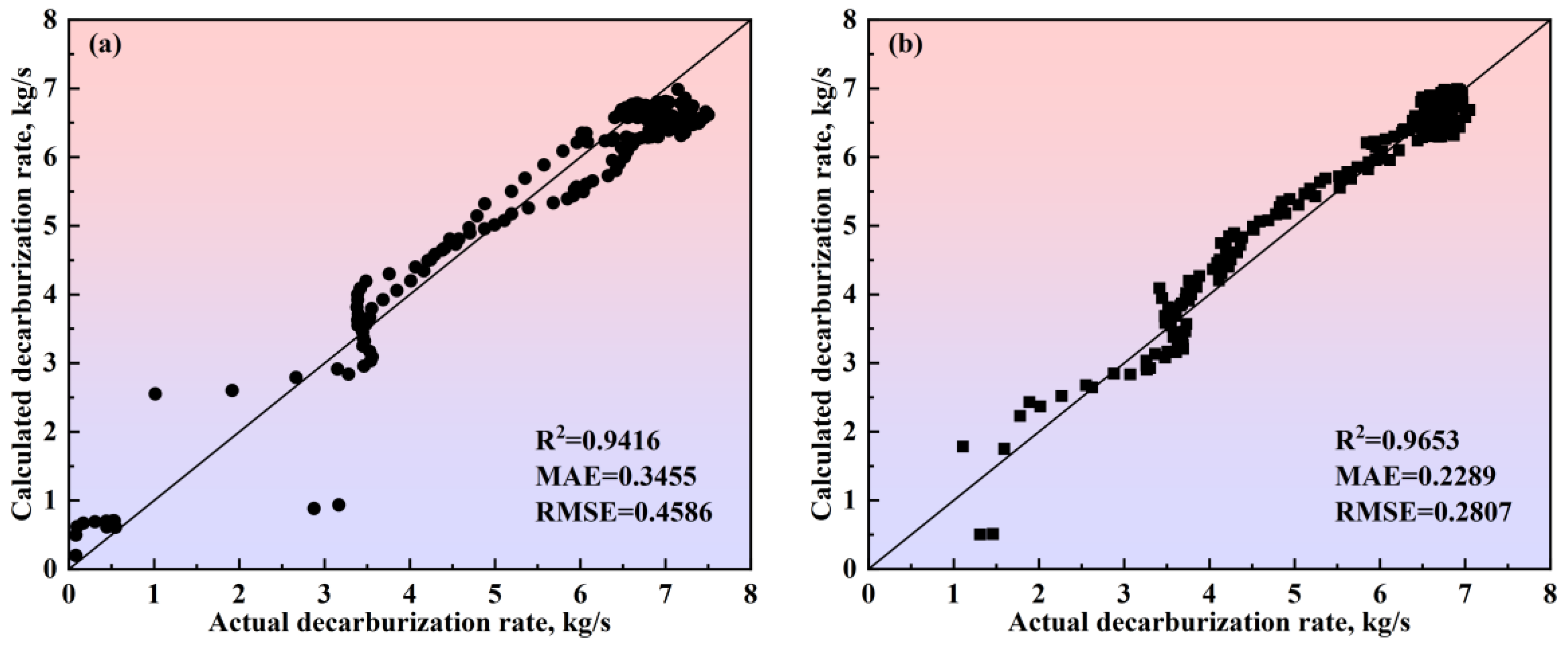
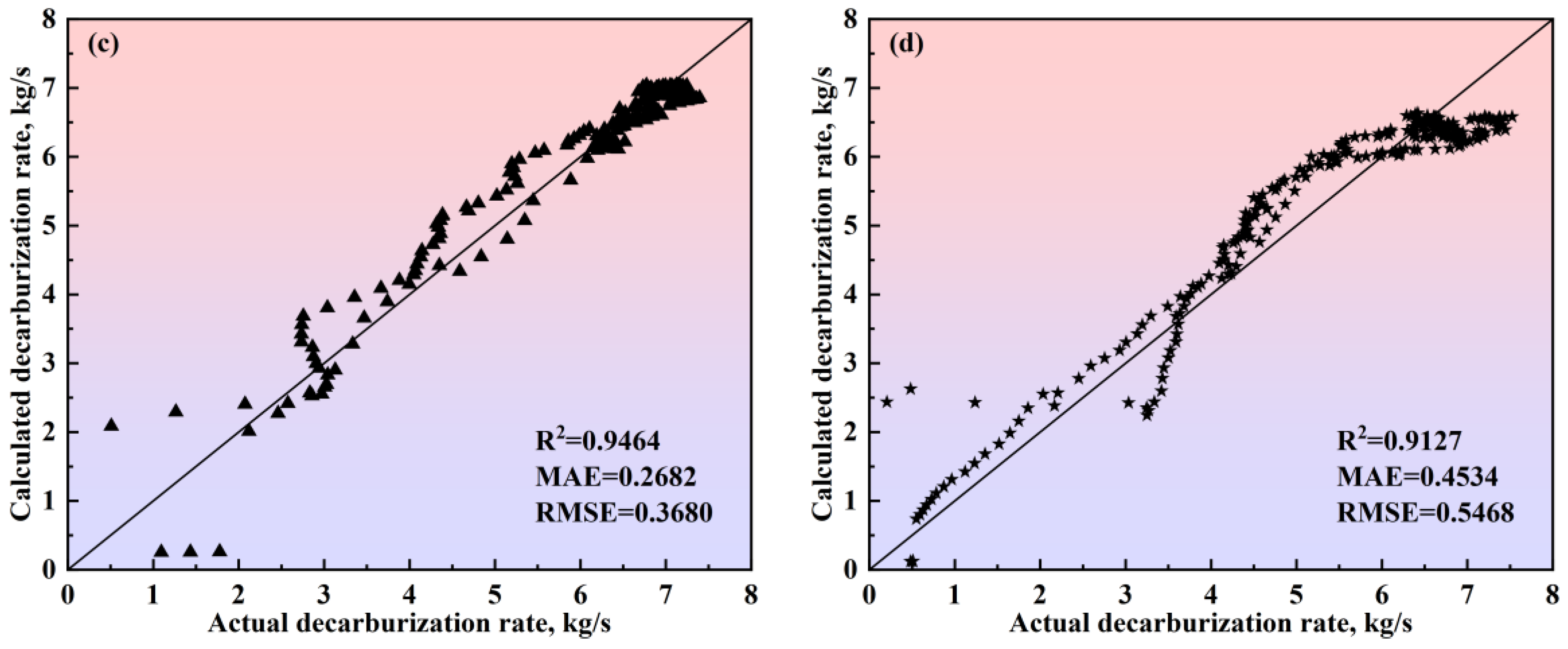
| Name | Value | Name | Value | Name | Value |
|---|---|---|---|---|---|
| Weight of molten iron, t | 108.0 | [C] of molten iron, % | 4.73 | [C] of molten metal at the end of blowing process, % | 0.092 |
| Weight of scrap steel, t | 16.2 | [Mn] of molten iron, % | 0.36 | [Mn] of molten metal at the end of blowing process, % | 0.163 |
| Weight of lime, kg | 3524 | [Si] of molten iron, % | 0.50 | [Si] of molten metal at the end of blowing process, % | 0.002 |
| Weight of dolomite, kg | 1028 | Temperature of molten iron, °C | 1325 | Temperature of molten metal at the end of blowing process, °C | 1640 |
| Weight of ore, kg | 622 | Total amount of oxygen blown, Nm3 | 5316 | Bottom blowing gas intensity, Nm3/(t·min) | 0.03~0.07 |
| Heat | Grade of Steel | Target [C] of Molten Metal at the End of Blowing Process | Calculated [C] of Molten Metal at the End of Blowing Process, % | Actual [C] of Molten Metal at the End of Blowing Process, % | Remark |
|---|---|---|---|---|---|
| A | B/Q345B | 0.165 | 0.092 | Abnormal blowing, the slag becomes dry | |
| B | 65Mn | 0.109 | 0.128 | Normal blowing | |
| C | 510L | 0.055 | 0.071 | Normal blowing | |
| D | 200IF | 0.026 | 0.054 | Normal blowing |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xin, Z.; Liu, Q.; Zhang, J.; Lin, W. Analysis of Multi-Zone Reaction Mechanisms in BOF Steelmaking and Comprehensive Simulation. Materials 2025, 18, 1038. https://doi.org/10.3390/ma18051038
Xin Z, Liu Q, Zhang J, Lin W. Analysis of Multi-Zone Reaction Mechanisms in BOF Steelmaking and Comprehensive Simulation. Materials. 2025; 18(5):1038. https://doi.org/10.3390/ma18051038
Chicago/Turabian StyleXin, Zicheng, Qing Liu, Jiangshan Zhang, and Wenhui Lin. 2025. "Analysis of Multi-Zone Reaction Mechanisms in BOF Steelmaking and Comprehensive Simulation" Materials 18, no. 5: 1038. https://doi.org/10.3390/ma18051038
APA StyleXin, Z., Liu, Q., Zhang, J., & Lin, W. (2025). Analysis of Multi-Zone Reaction Mechanisms in BOF Steelmaking and Comprehensive Simulation. Materials, 18(5), 1038. https://doi.org/10.3390/ma18051038









