Mechanisms of Strength Degradation of Dental Zirconia Due to Glazing: Dependence on Glaze Thickness
Abstract
1. Introduction
2. Materials and Methods
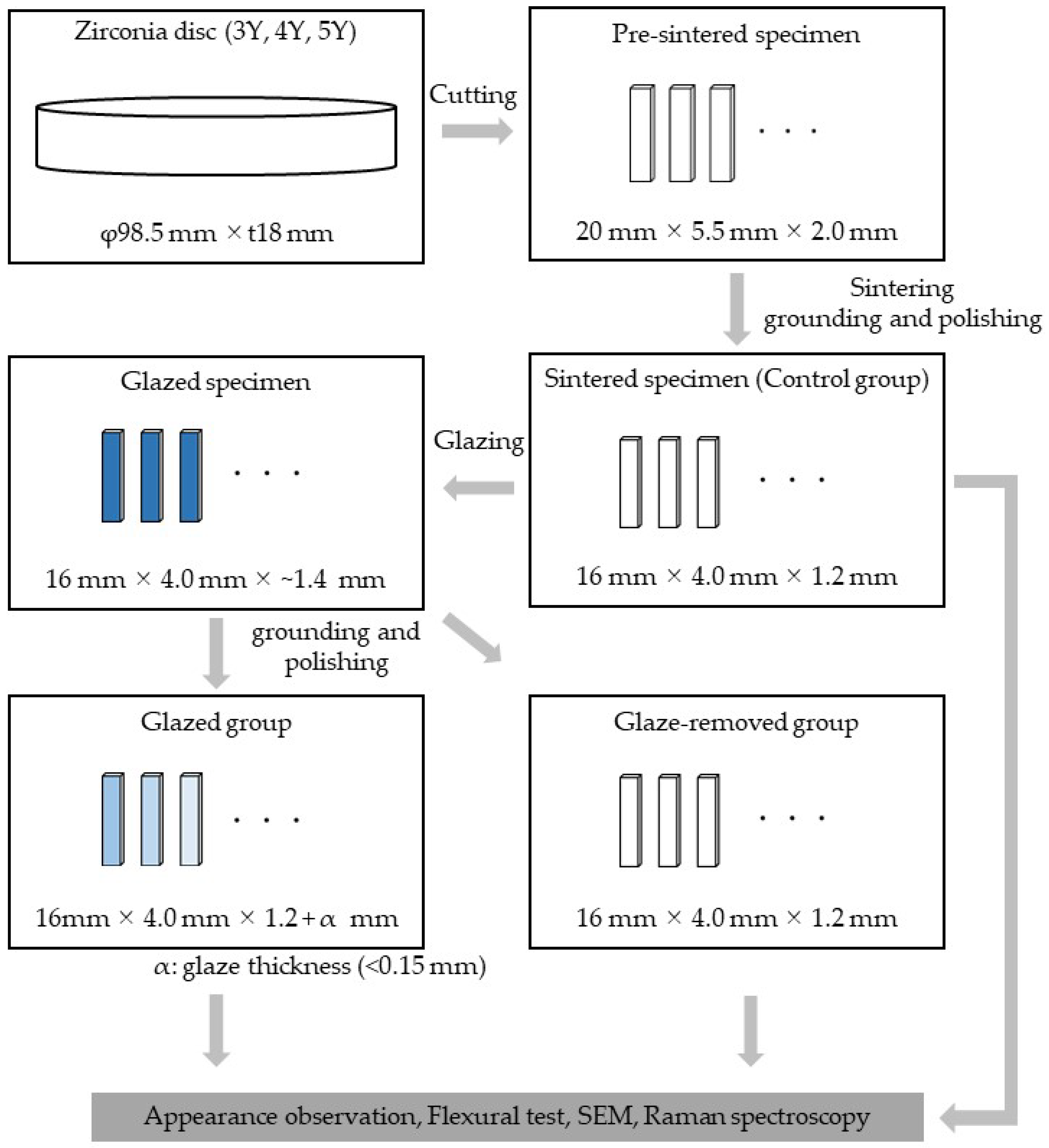
2.1. Preparation of Test Piece
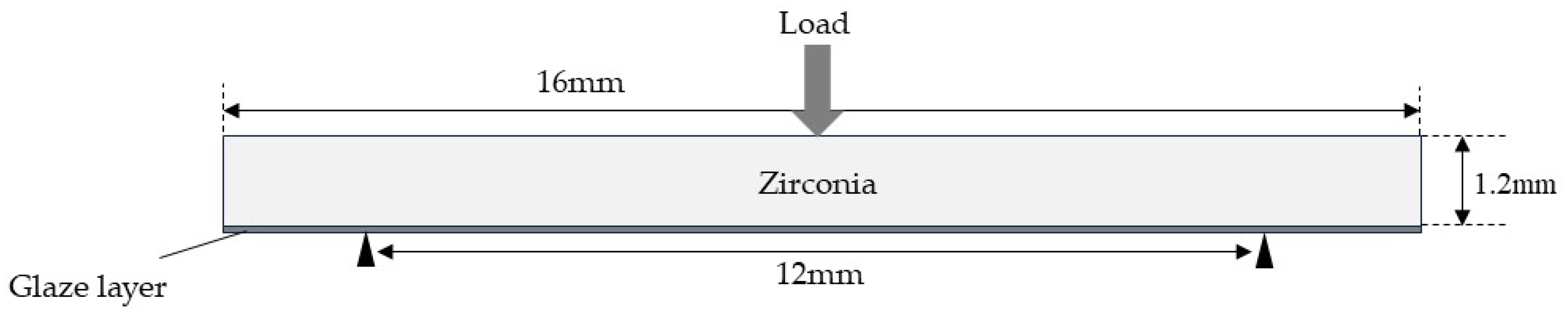
2.2. Three-Point Flexural Test
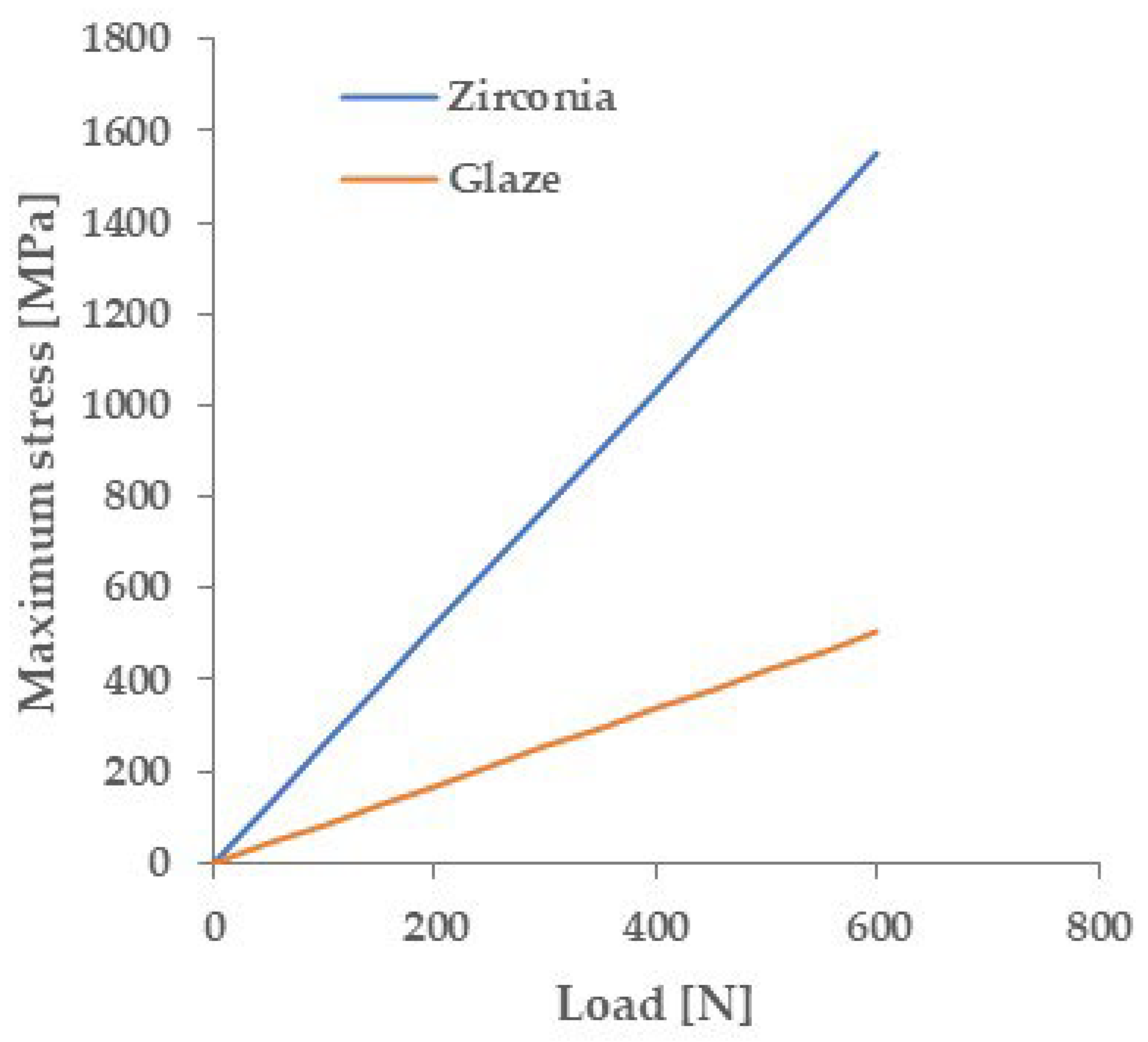
2.3. Finite Element Method (FEM) Analysis
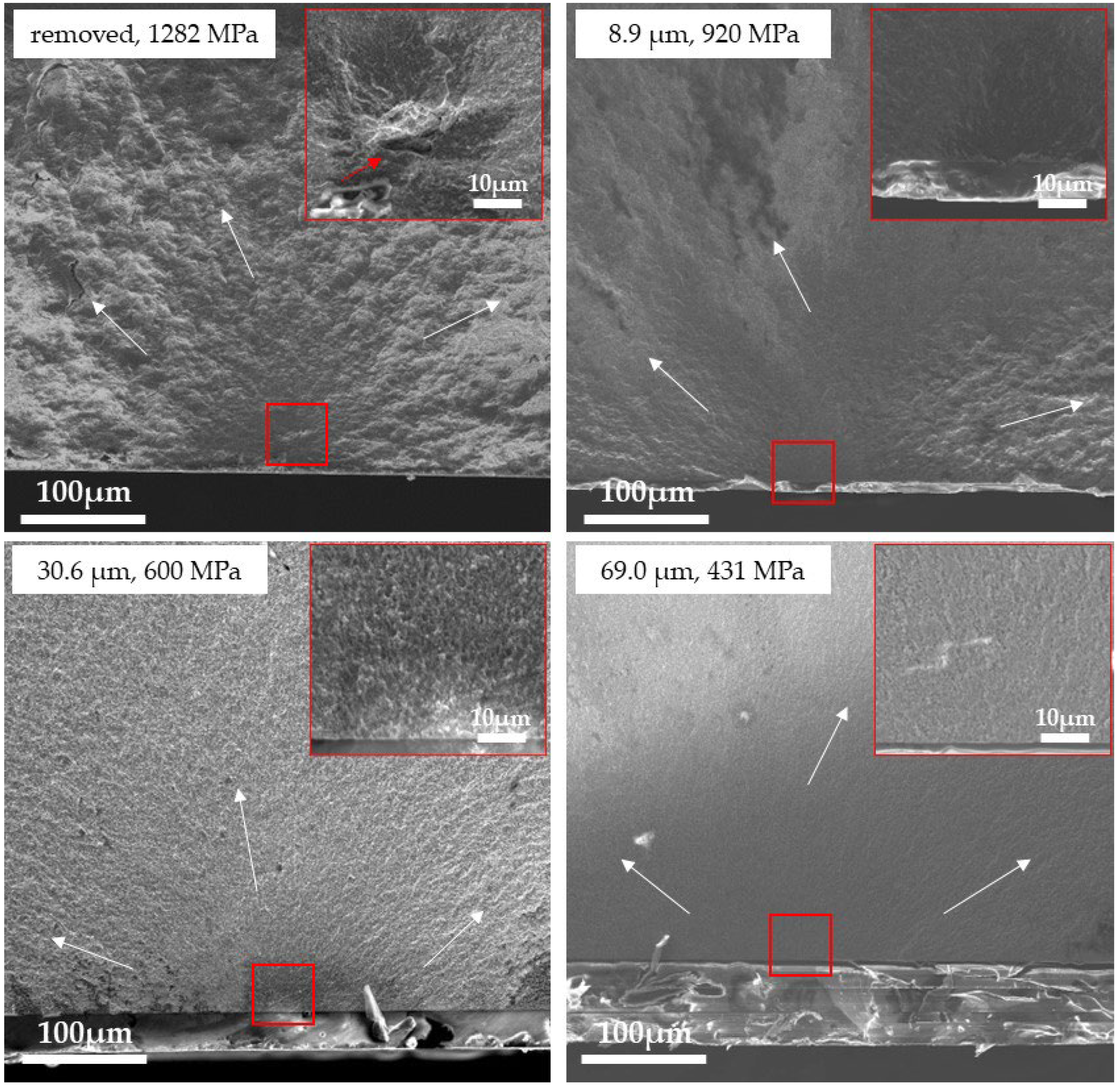
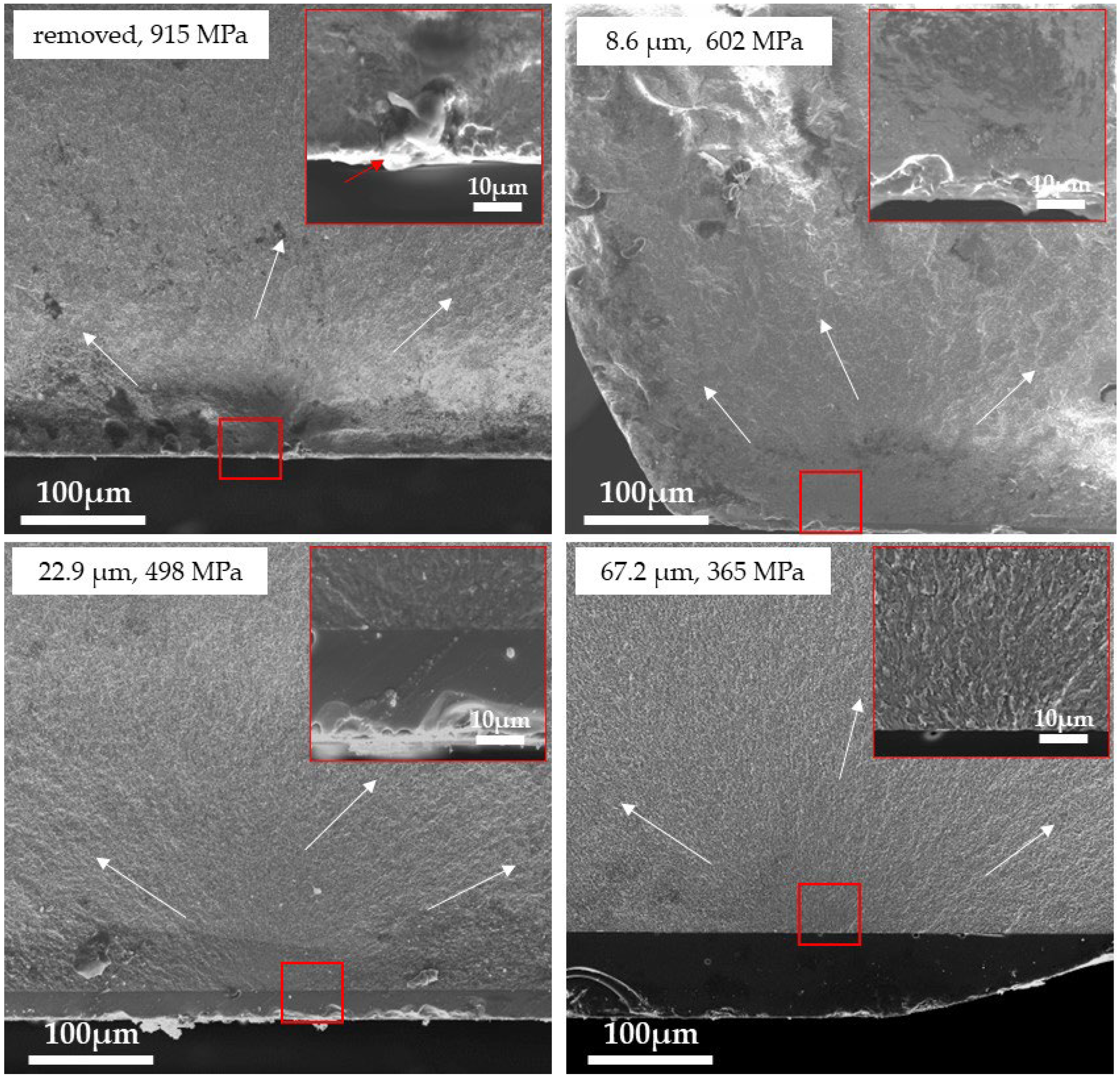
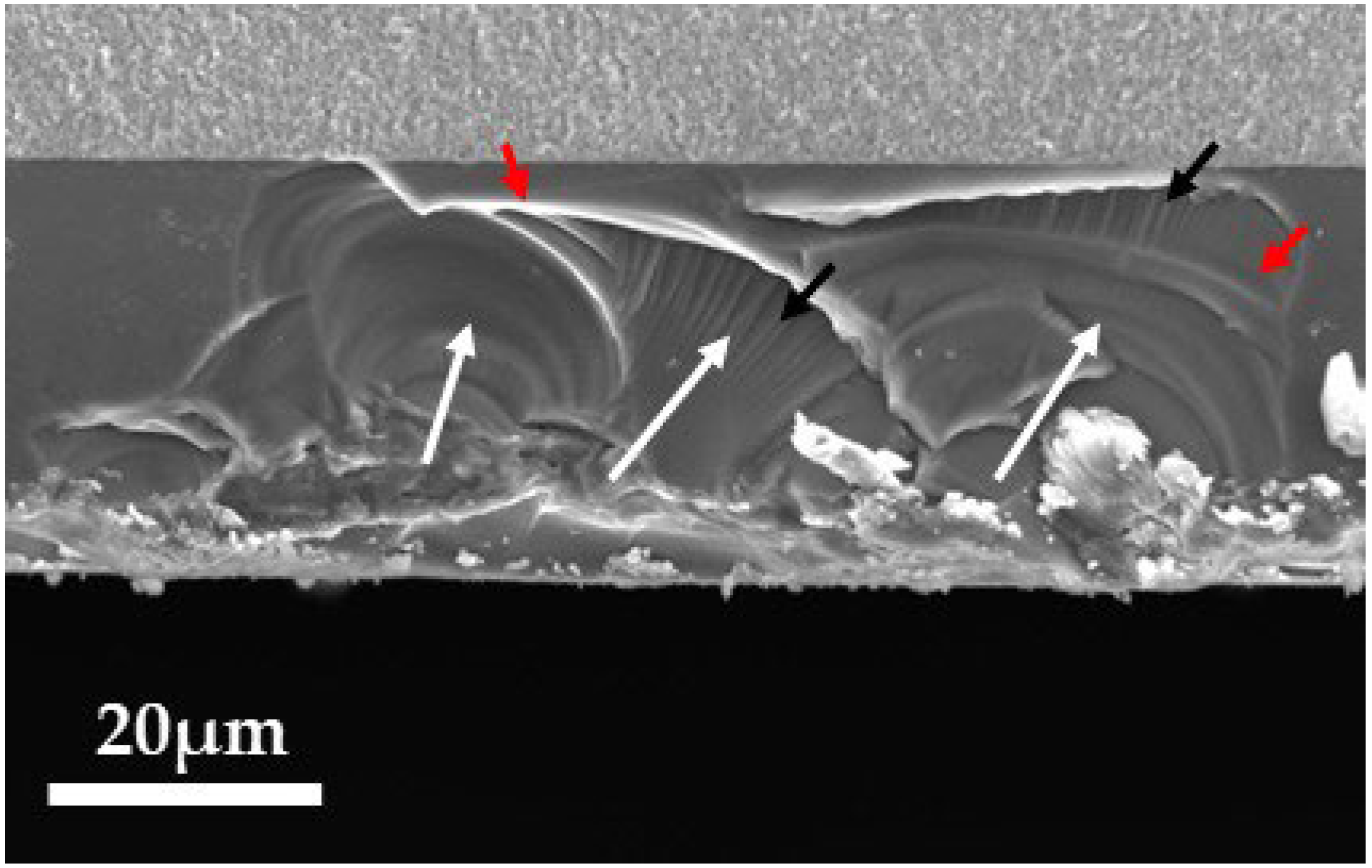
2.4. Fracture Surface Observation
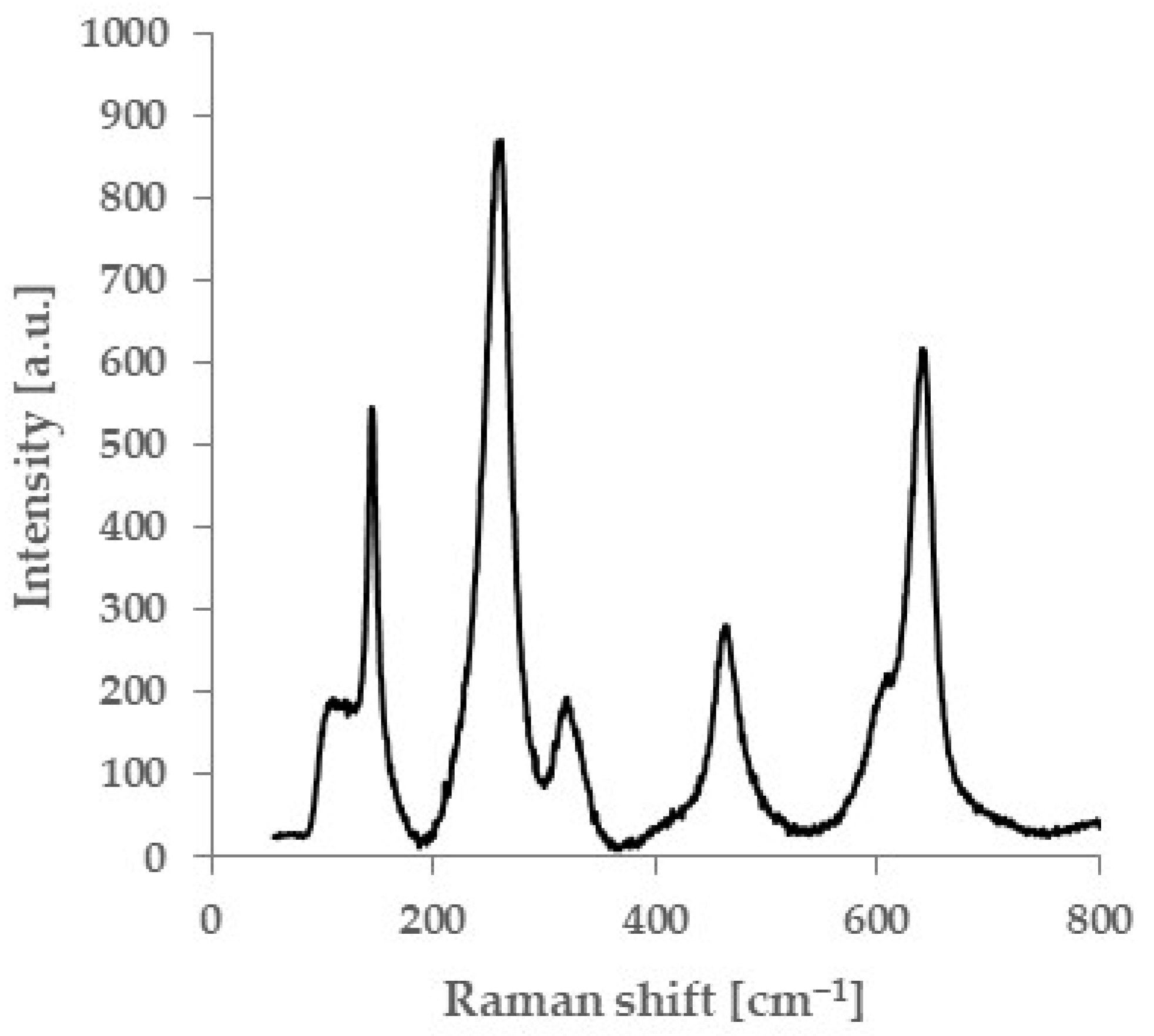
2.5. Raman Spectroscopy
2.6. Statistical Analysis
3. Results
3.1. Appearance Observation of the Fractured Specimen
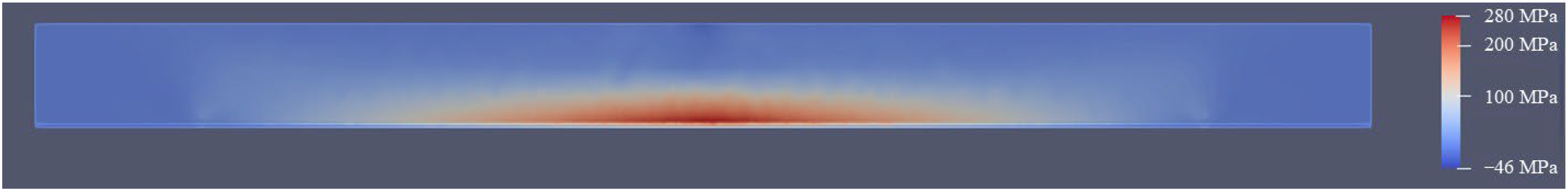
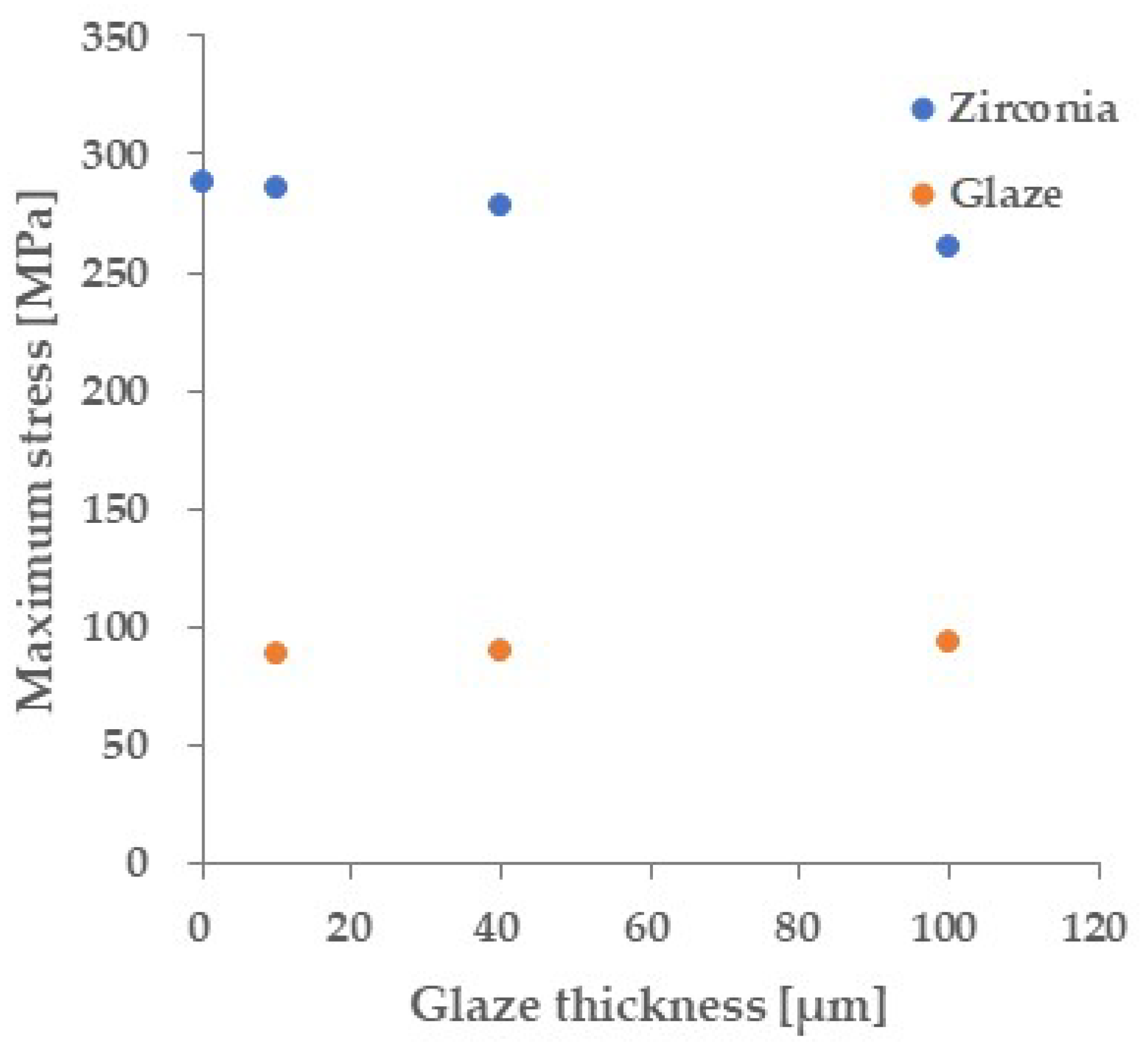
3.2. FEM Analysis
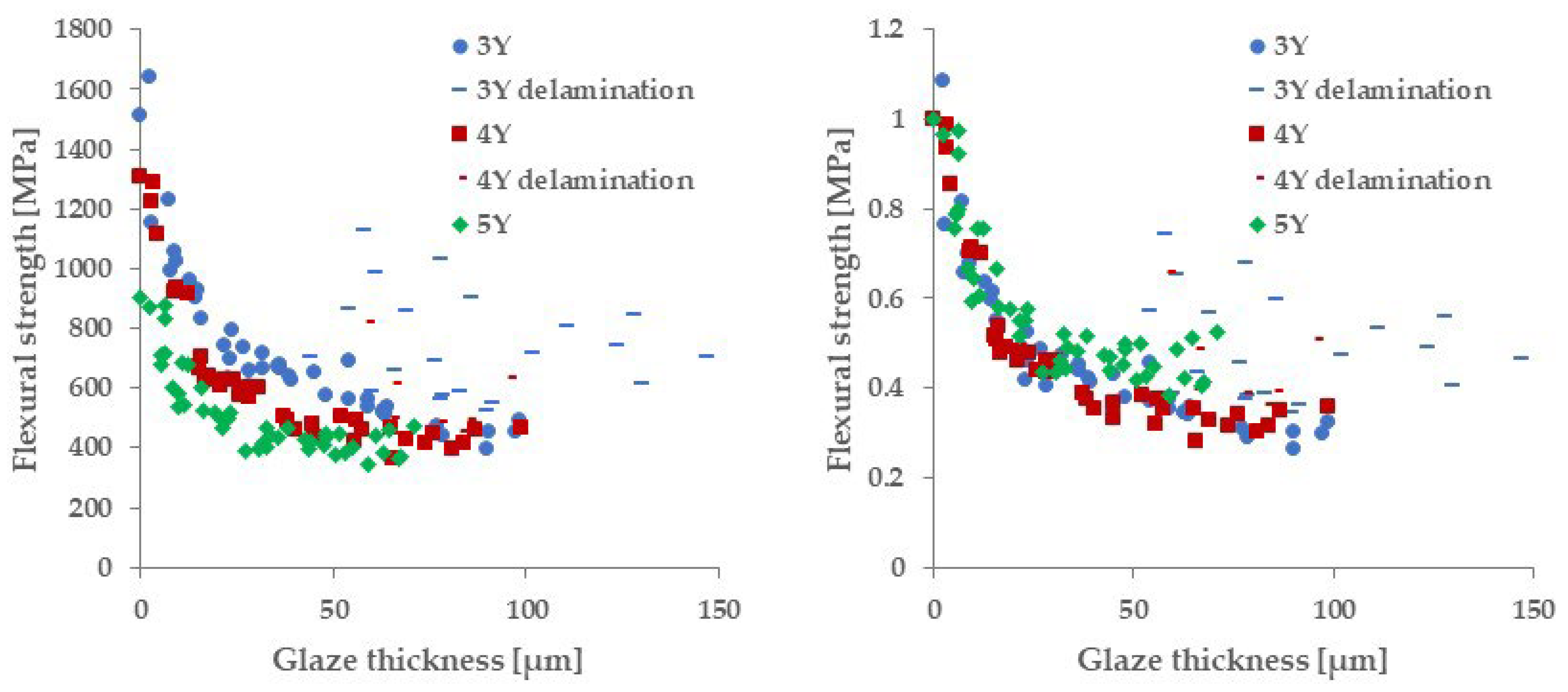
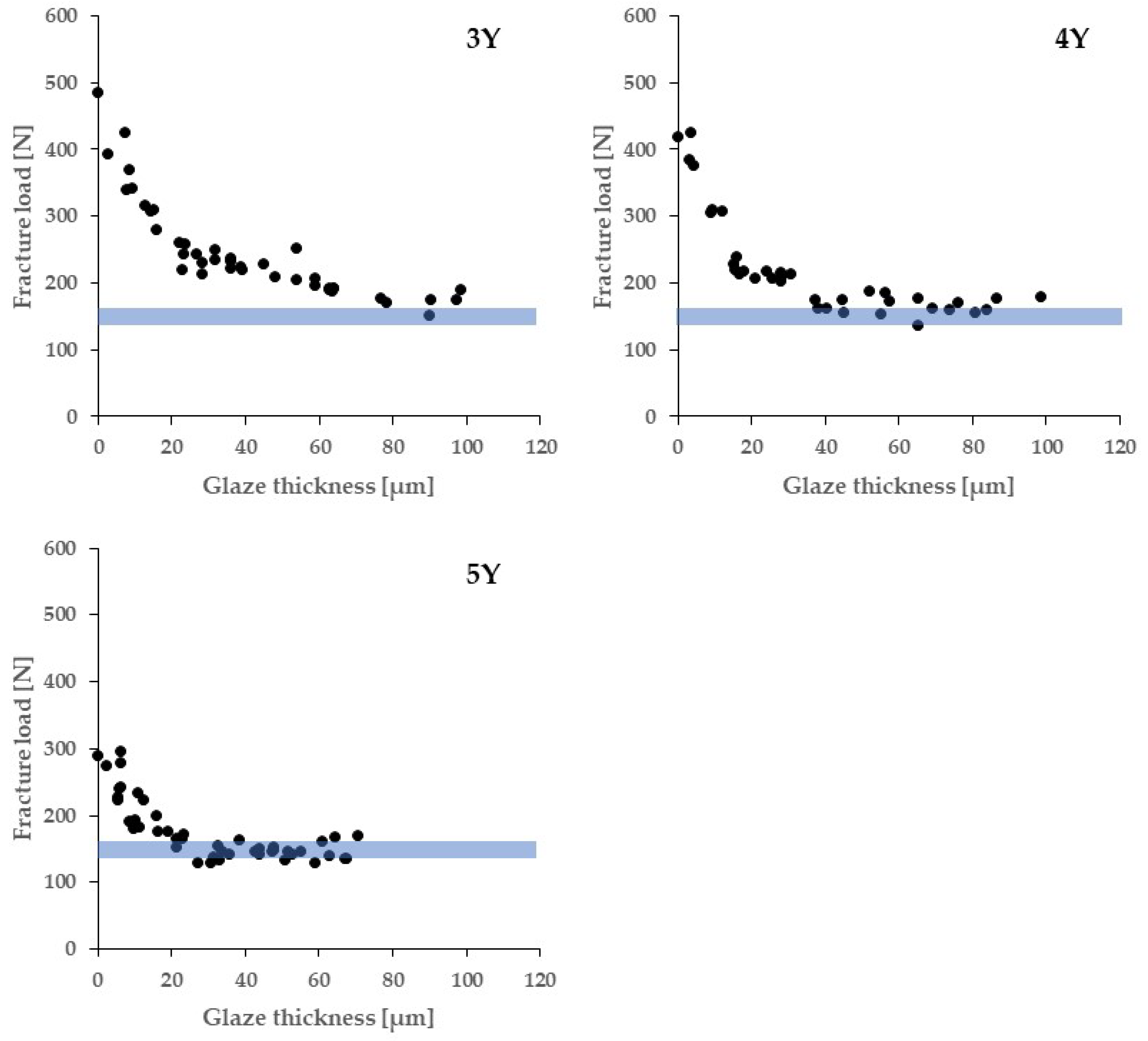
3.3. Three-Point Flexural Test
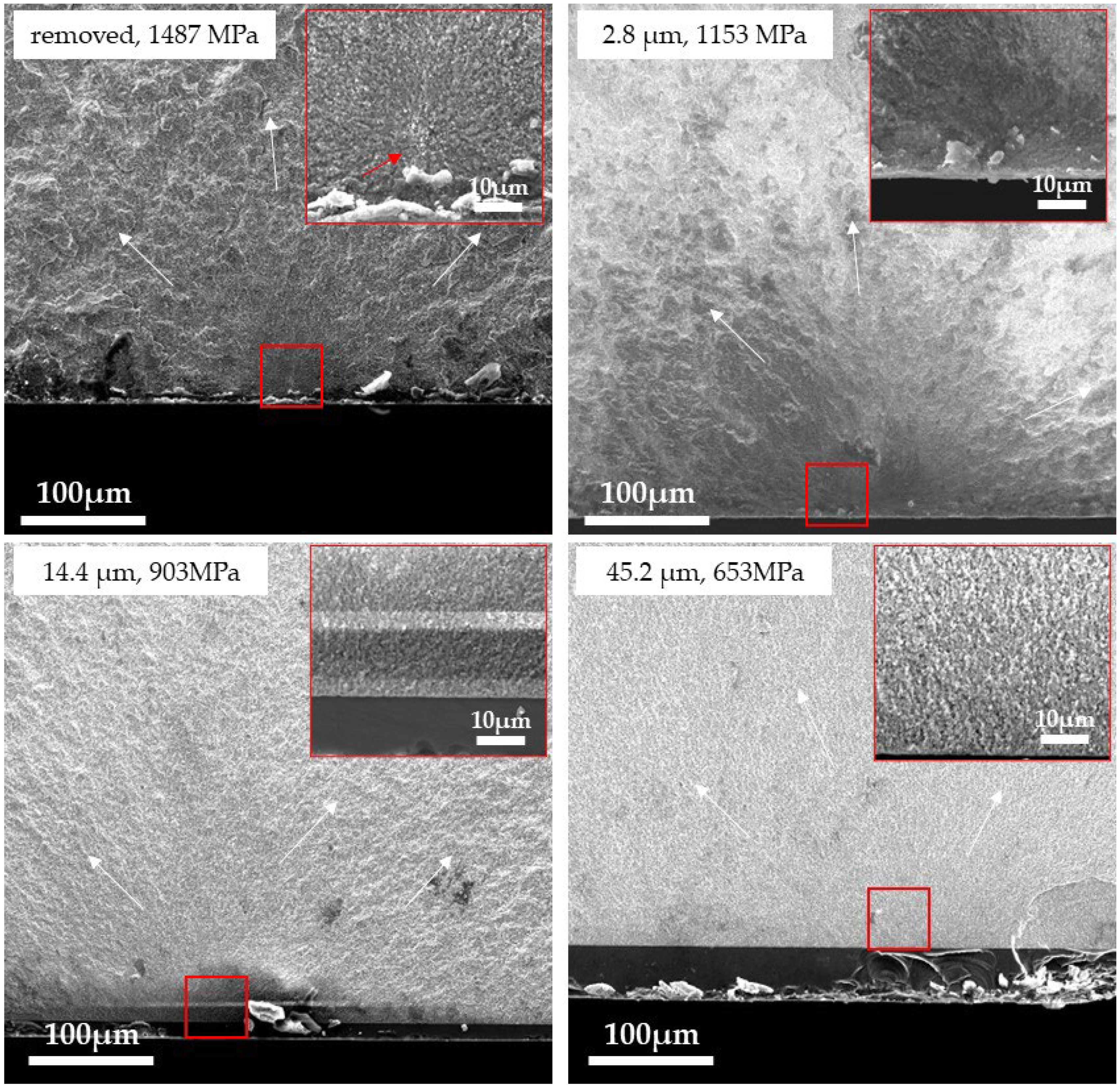
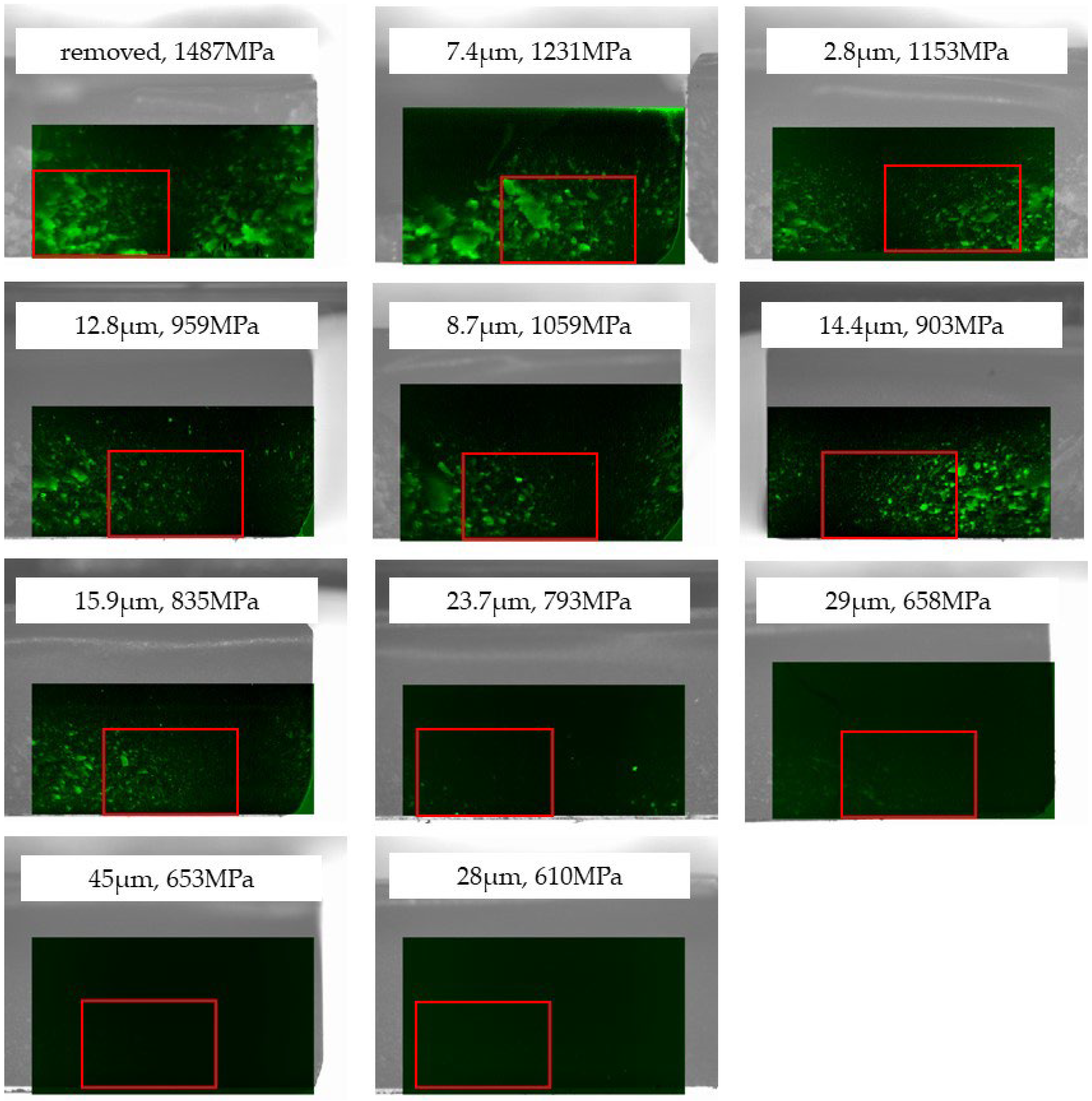
3.4. Fracture Surface Observation
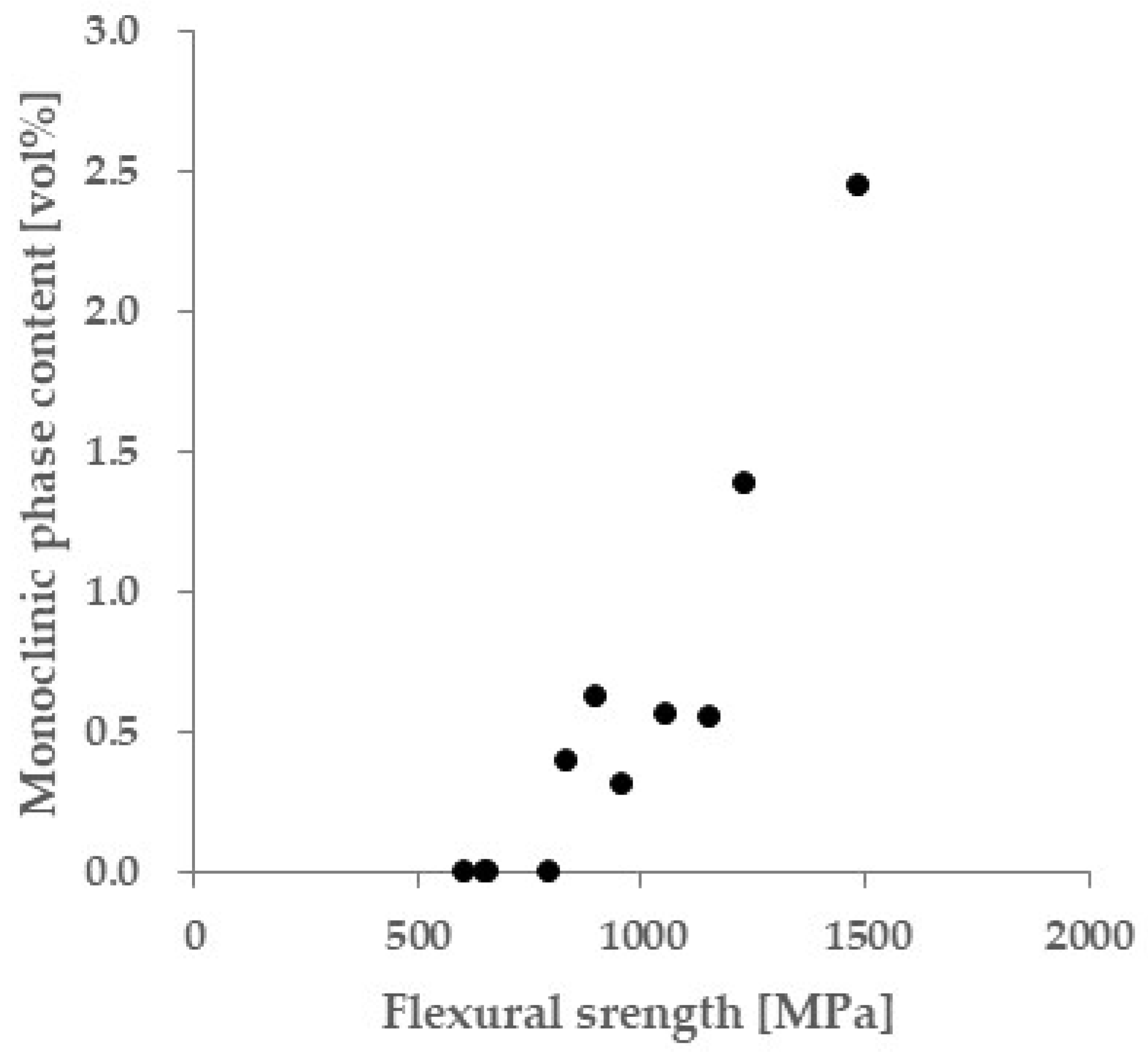
3.5. Raman Spectroscopy
4. Discussion
4.1. Chemical and Crystallographical Effect of Glazing
4.2. Mechanical Effect of Glazing
4.3. Crack Initiation and Propagation
4.4. Dependence of Strength on Glaze Thickness
4.4.1. Mechanism of Strength Decrease with Increasing Glaze Layer Thickness
4.4.2. Region with Constant Fracture Load
4.5. Tetragonal-to-Monoclinic Phase Transformation
4.6. Delamination of Glaze Layer
4.7. Clinical Impact
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sulaiman, T.A.; Suliman, A.A.; Abdulmajeed, A.A.; Zhang, Y. Zirconia restoration types, properties, tooth preparation design, and bonding. A narrative review. J. Esthet. Restor. Dent. 2024, 36, 78–84. [Google Scholar] [CrossRef] [PubMed]
- Alqutaibi, A.Y.; Ghulam, O.; Krsoum, M.; Binmahmoud, S.; Taher, H.; Elmalky, W.; Zafar, M.S. Revolution of Current Dental Zirconia: A Comprehensive Review. Molecules 2022, 27, 1699. [Google Scholar] [CrossRef] [PubMed]
- Kongkiatkamon, S.; Peampring, C. Comparison of Regular and Speed Sintering on Low-Temperature Degradation and Fatigue Resistance of Translucent Zirconia Crowns for Implants: An In Vitro Study. J. Funct. Biomater. 2022, 13, 281. [Google Scholar] [CrossRef] [PubMed]
- Rana, M.S.; Meng, F.; Zhang, R.; Huang, L.; Qian, K. Integrating zirconia-gold hybrid into aptasensor capable of ultrasensitive and robust carcinoembryonic antigen determination. Electrochim. Acta 2024, 507, 145063. [Google Scholar] [CrossRef]
- Li, Z. Advancements of biomaterial in hip replacement technology incorporating ceramic materials. J. Orthop. 2025, 62, 27–35. [Google Scholar] [CrossRef]
- Ciszyński, M.; Chwaliszewski, B.; Simka, W.; Dominiak, M.; Gedrange, T.; Hadzik, J. Zirconia Dental Implant Designs and Surface Modifications: A Narrative Review. Materials 2024, 17, 4202. [Google Scholar] [CrossRef]
- Dal Piva, A.M.; Tribst, J.P.; Werner, A.; Anami, L.C.; Bottino, M.A.; Kleverlaan, C.J. Three-body wear effect on different CAD/CAM ceramics staining durability. J. Mech. Behav. Biomed. Mater. 2020, 103, 103579. [Google Scholar] [CrossRef]
- Bittar, B.F.; Miranda, J.S.; Simões, A.C.; de Carvalho Ramos, N.; Machado, J.P.; Zhang, Y.; Souza, R.O.A.; Leite, F.P.P. Effect of extrinsic pigmentation and surface treatments on biaxial flexure strength after cyclic loading of a translucent ZrO2 ceramic. Dent. Mater. 2019, 35, 1644–1653. [Google Scholar] [CrossRef]
- Kim, H.-K.; Kim, S.-H.; Lee, J.-B.; Han, J.-S.; Yeo, I.-S. Effect of polishing and glazing on the color and spectral distribution of monolithic zirconia. J. Adv. Prosthodont. 2013, 5, 296–304. [Google Scholar] [CrossRef]
- Yener, E.S.; Özcan, M.; Kazazoğlu, E. The effect of glazing on the biaxial flexural strength of different zirconia core materials. Acta Odontol. Latinoam. 2011, 24, 133–140. [Google Scholar]
- Eltanahy, M.; Wahsh, M.; Mohamed, F. The Effect of Surface Grinding and Finishing on the Surface Roughness And Flexural Strength of Cubic Zirconia. Egypt. Dent. J. 2023, 69, 497–504. [Google Scholar] [CrossRef]
- Doğru, G.; Demiralp, E.; Koçak, E.; Örkcü, H.H.; Aydin, C.; Yilmaz, H. Influence of Finishing Procedures on Surface Roughness and Biaxial Flexural Strength of High-translucent 4Y-PSZ, 5Y-PSZ, and 6Y-PSZ Monolithic Zirconia. Clin. Exp. Health Sci. 2023, 13, 166–175. [Google Scholar] [CrossRef]
- Singh, R.G.; Li, K.C.; Lyons, K.M.; Waddell, J.N. Effect of two brands of glaze material on the flexural strength and probability of failure of high translucent monolithic zirconia. Materials 2021, 14, 7022. [Google Scholar] [CrossRef] [PubMed]
- Lu, Y.; de Oliveira Dal Piva, A.M.; Tribst, J.P.M.; Feilzer, A.J.; Kleverlaan, C.J. Does glaze firing affect the strength of advanced lithium disilicate after simulated defects? Clin. Oral Investig. 2023, 27, 6429–6438. [Google Scholar] [CrossRef]
- Hatanaka, G.R.; Polli, G.S.; Adabo, G.L. The mechanical behavior of high-translucent monolithic zirconia after adjustment and finishing procedures and artificial aging. J. Prosthet. Dent. 2020, 123, 330–337. [Google Scholar] [CrossRef]
- Kumchai, H.; Juntavee, P.; Sun, A.F.; Nathanson, D. Effect of glazing on flexural strength of full-contour zirconia. Int. J. Dent. 2018, 2018, 8793481. [Google Scholar] [CrossRef]
- Lohbauer, U.; Scherrer, S.S.; Della Bona, A.; Tholey, M.; van Noort, R.; Vichi, A.; Kelly, J.R.; Cesar, P.F. ADM guidance-Ceramics: All-ceramic multilayer interfaces in dentistry. Dent. Mater. 2017, 33, 585–598. [Google Scholar] [CrossRef]
- Hsueh, C.-H.; Kelly, J. Simple solutions of multilayered discs subjected to biaxial moment loading. Dent. Mater. 2009, 25, 506–513. [Google Scholar] [CrossRef]
- Swain, M. Unstable cracking (chipping) of veneering porcelain on all-ceramic dental crowns and fixed partial dentures. Acta Biomater. 2009, 5, 1668–1677. [Google Scholar] [CrossRef]
- Ban, S. Classification and properties of dental zirconia as implant fixtures and superstructures. Materials 2021, 14, 4879. [Google Scholar] [CrossRef]
- Kui, A.; Manziuc, M.; Petruțiu, A.; Buduru, S.; Labuneț, A.; Negucioiu, M.; Chisnoiu, A. translucent zirconia in fixed prosthodontics—An integrative overview. Biomedicines 2023, 11, 3116. [Google Scholar] [CrossRef] [PubMed]
- ISO 6872:2024; Dentistry—Ceramic Materials. ISO: Geneva, Switzerland, 2024.
- Yan, J.; Kaizer, M.R.; Zhang, Y. Load-bearing capacity of lithium disilicate and ultra-translucent zirconias. J. Mech. Behav. Biomed. Mater. 2018, 88, 170–175. [Google Scholar] [CrossRef] [PubMed]
- Quinn, G.D. Fractography of Ceramics and Glasses; National Institute of Standards and Technology: Washington, DC, USA, 2007; Volume 960. [Google Scholar]
- Clarke, D.; Adar, F. Measurement of the crystallographically transformed zone produced by fracture in ceramics containing tetragonal zirconia. J. Am. Ceram. Soc. 1982, 65, 284–288. [Google Scholar] [CrossRef]
- Lunt, A.; Salvati, E.; Baimpas, N.; Dolbnya, I.; Neo, T.K.; Korsunsky, A.M. Investigations into the interface failure of yttria partially stabilised zirconia-porcelain dental prostheses through microscale residual stress and phase quantification. Dent. Mater. 2019, 35, 1576–1593. [Google Scholar] [CrossRef] [PubMed]
- Chevalier, J.; Gremillard, L.; Virkar, A.V.; Clarke, D.R. The tetragonal-monoclinic transformation in zirconia: Lessons learned and future trends. J. Am. Ceram. Soc. 2009, 92, 1901–1920. [Google Scholar] [CrossRef]
- Mainjot, A.K.; Schajer, G.S.; Vanheusden, A.J.; Sadoun, M.J. Influence of veneer thickness on residual stress profile in veneering ceramic: Measurement by hole-drilling. Dent. Mater. 2012, 28, 160–167. [Google Scholar] [CrossRef]
- Nakamura, T.; Usami, H.; Ohnishi, H.; Takeuchi, M.; Nishida, H.; Sekino, T.; Yatani, H. The effect of adding silica to zirconia to counteract zirconia’s tendency to degrade at low temperatures. Dent. Mater. J. 2011, 30, 330–335. [Google Scholar] [CrossRef][Green Version]
- Samodurova, A.; Kocjan, A.; Swain, M.V.; Kosmač, T. The combined effect of alumina and silica co-doping on the ageing resistance of 3Y-TZP bioceramics. Acta Biomater. 2015, 11, 477–487. [Google Scholar] [CrossRef]
- Hsueh, C.; Luttrell, C.; Becher, P. Modelling of bonded multilayered disks subjected to biaxial flexure tests. Int. J. Solids Struct. 2006, 43, 6014–6025. [Google Scholar] [CrossRef]
- Lobo, C.M.M.; Sacorague, S.C.M.C.; Silva, N.R.d.; Costa, A.K.F.; Alves, L.M.M.; Bottino, M.A.; Özcan, M.; Melo, R.M.d. Effect of glazing application side and mechanical cycling on the biaxial flexural strength and Weibull characteristics of a Y-TZP ceramic. J. Appl. Oral Sci. 2020, 28, e20200438. [Google Scholar] [CrossRef]
- Huang, C.; Hsueh, C. Piston-on-three-ball versus piston-on-ring in evaluating the biaxial strength of dental ceramics. Dent. Mater. 2011, 27, e117–e123. [Google Scholar] [CrossRef] [PubMed]
- Chai, H.; Lee, J.J.-W.; Mieleszko, A.J.; Chu, S.J.; Zhang, Y. On the interfacial fracture of porcelain/zirconia and graded zirconia dental structures. Acta Biomater. 2014, 10, 3756–3761. [Google Scholar] [CrossRef] [PubMed]
- Mukherjee, S.; Jayaram, V.; Biswas, S. Validation of stresses and stress intensity factor in a notched bilayer system under four point bending, as determined by the solution of the Navier’s equation. Int. J. Mech. Sci. 2006, 48, 1287–1294. [Google Scholar] [CrossRef]
- Morrell, R. Fractography of Brittle Materials; National Physical Laboratory: Teddington, UK, 2008. [Google Scholar]
- Garvie, R.C.; Hannink, R.; Pascoe, R. Ceramic steel? Nature 1975, 258, 703–704. [Google Scholar] [CrossRef]
- Gupta, T.K.; Lange, F.; Bechtold, J. Effect of stress-induced phase transformation on the properties of polycrystalline zirconia containing metastable tetragonal phase. J. Mater. Sci. 1978, 13, 1464–1470. [Google Scholar] [CrossRef]
- Evans, A.; Heuer, A. Transformation toughening in ceramics: Martensitic transformations in crack-tip stress fields. J. Am. Ceram. Soc. 1980, 63, 241–248. [Google Scholar] [CrossRef]
- Evans, A.G. Perspective on the development of high-toughness ceramics. J. Am. Ceram. Soc. 1990, 73, 187–206. [Google Scholar] [CrossRef]
- McMeeking, R.; Evans, A. Mechanics of transformation-toughening in brittle materials. J. Am. Ceram. Soc. 1982, 65, 242–246. [Google Scholar] [CrossRef]
- He, M.Y.; Evans, A.G.; Hutchinson, J.W. Crack deflection at an interface between dissimilar elastic materials: Role of residual stresses. Int. J. Solids Struct. 1994, 31, 3443–3455. [Google Scholar] [CrossRef]
- Dimitriadis, K.; Tulyaganov, D.U.; Agathopoulos, S. Evaluation of bond strength between zirconia milled ceramic material and veneered dental porcelain. Eur. J. Oral Sci. 2024, 132, e12989. [Google Scholar] [CrossRef]
- Wang, G.; Zhang, S.; Bian, C.; Kong, H. Interface toughness of a zirconia-veneer system and the effect of a liner application. J. Prosthet. Dent. 2014, 112, 576–583. [Google Scholar] [CrossRef] [PubMed]
- Anusavice, K.J.; Shen, C.; Rawls, H.R. Phillips’ Science of Dental Materials; Elsevier Health Sciences: Amsterdam, The Netherlands, 2012. [Google Scholar]
- Alberto, L.H.; Kalluri, L.; Esquivel-Upshaw, J.F.; Duan, Y. Three-Dimensional Finite Element Analysis of Different Connector Designs for All-Ceramic Implant-Supported Fixed Dental Prostheses. Ceramics 2022, 5, 34–43. [Google Scholar] [CrossRef] [PubMed]
- Alberto, L.H.; Kalluri, L.; Esquivel-Upshaw, J.F.; Duan, Y. Finite element analysis of an implant-supported FDP with different connector heights. Symmetry 2022, 14, 2334. [Google Scholar] [CrossRef]



| 3Y | 4Y | 5Y | GP | ||
|---|---|---|---|---|---|
| Control | Flexural strength | 1502.2 A,a | 1301.9 B,b | 919.7 C,c | 129.2 D |
| Standard deviation | 169.0 | 125.8 | 121.1 | 6.9 | |
| Glaze-removed | Flexural strength | 1511.7 A,a | 1294.2 B,b | 901.2 C,c | — |
| Standard deviation | 120.2 | 126.5 | 65.4 | — |
| Source | SS | MS | F | p | *: p < 0.05 **: p < 0.01 |
|---|---|---|---|---|---|
| Material | 3,664,159.80 | 1,832,079.90 | 117.1856 | <0.001 | ** |
| Glazing → removal procedure | 459.56 | 459.56 | 0.0294 | 0.8645 | |
| Material * Glazing → removal procedure | 1989.51 | 994.76 | 0.0636 | 0.9384 | |
| Error | 844,236.02 | 15,634.00 | |||
| Total | 4,510,844.89 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nonaka, K.; Teramae, M.; Pezzotti, G. Mechanisms of Strength Degradation of Dental Zirconia Due to Glazing: Dependence on Glaze Thickness. Materials 2025, 18, 684. https://doi.org/10.3390/ma18030684
Nonaka K, Teramae M, Pezzotti G. Mechanisms of Strength Degradation of Dental Zirconia Due to Glazing: Dependence on Glaze Thickness. Materials. 2025; 18(3):684. https://doi.org/10.3390/ma18030684
Chicago/Turabian StyleNonaka, Kazumichi, Mitsuji Teramae, and Giuseppe Pezzotti. 2025. "Mechanisms of Strength Degradation of Dental Zirconia Due to Glazing: Dependence on Glaze Thickness" Materials 18, no. 3: 684. https://doi.org/10.3390/ma18030684
APA StyleNonaka, K., Teramae, M., & Pezzotti, G. (2025). Mechanisms of Strength Degradation of Dental Zirconia Due to Glazing: Dependence on Glaze Thickness. Materials, 18(3), 684. https://doi.org/10.3390/ma18030684





