Determination of Osmotic Flow in Water Transport in an Illitic Clay
Abstract
1. Introduction
2. Experimental Procedure
3. Theoretical Background
3.1. The Transport Model
3.2. Evaluation of Uncertainties
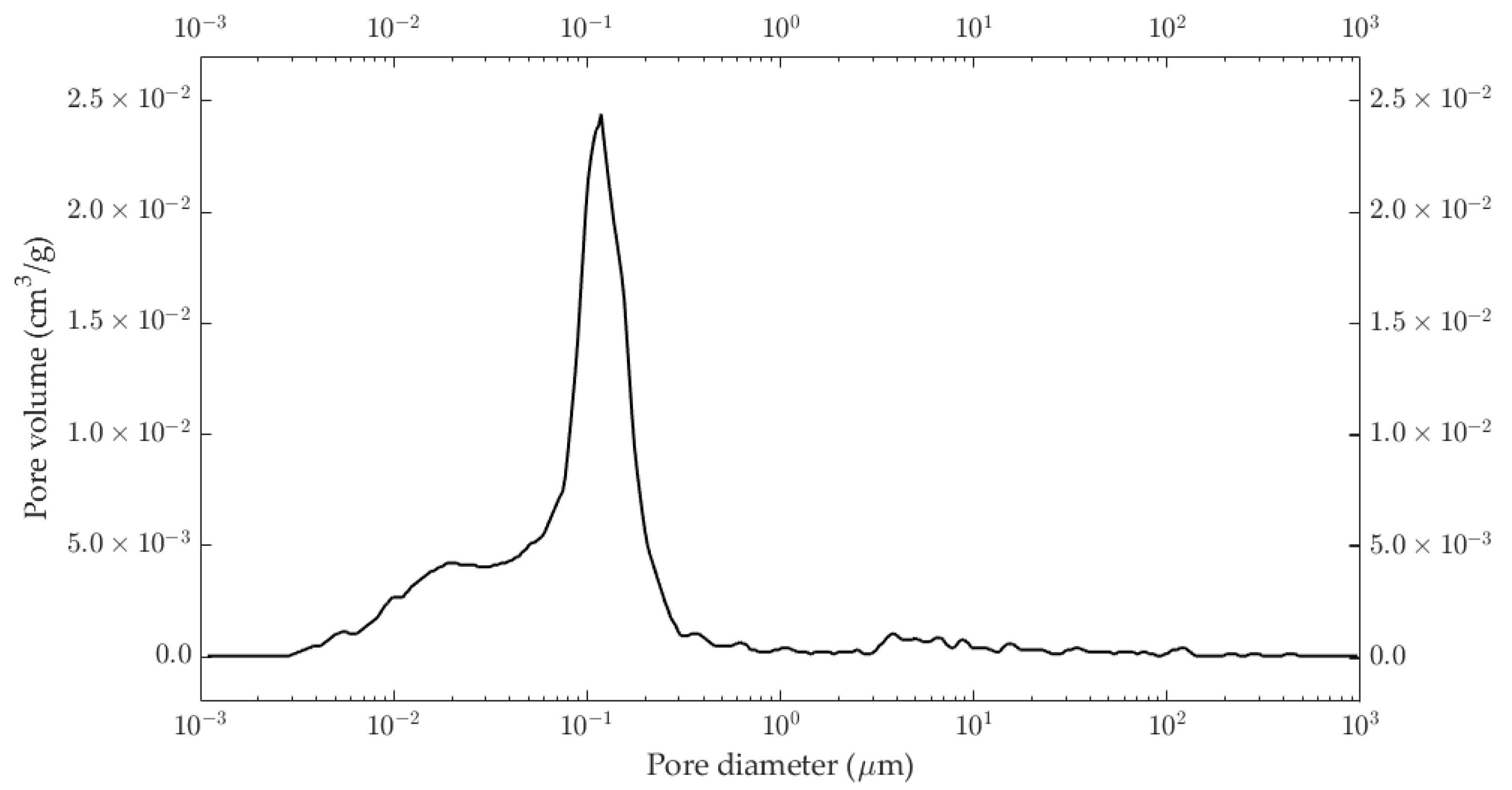
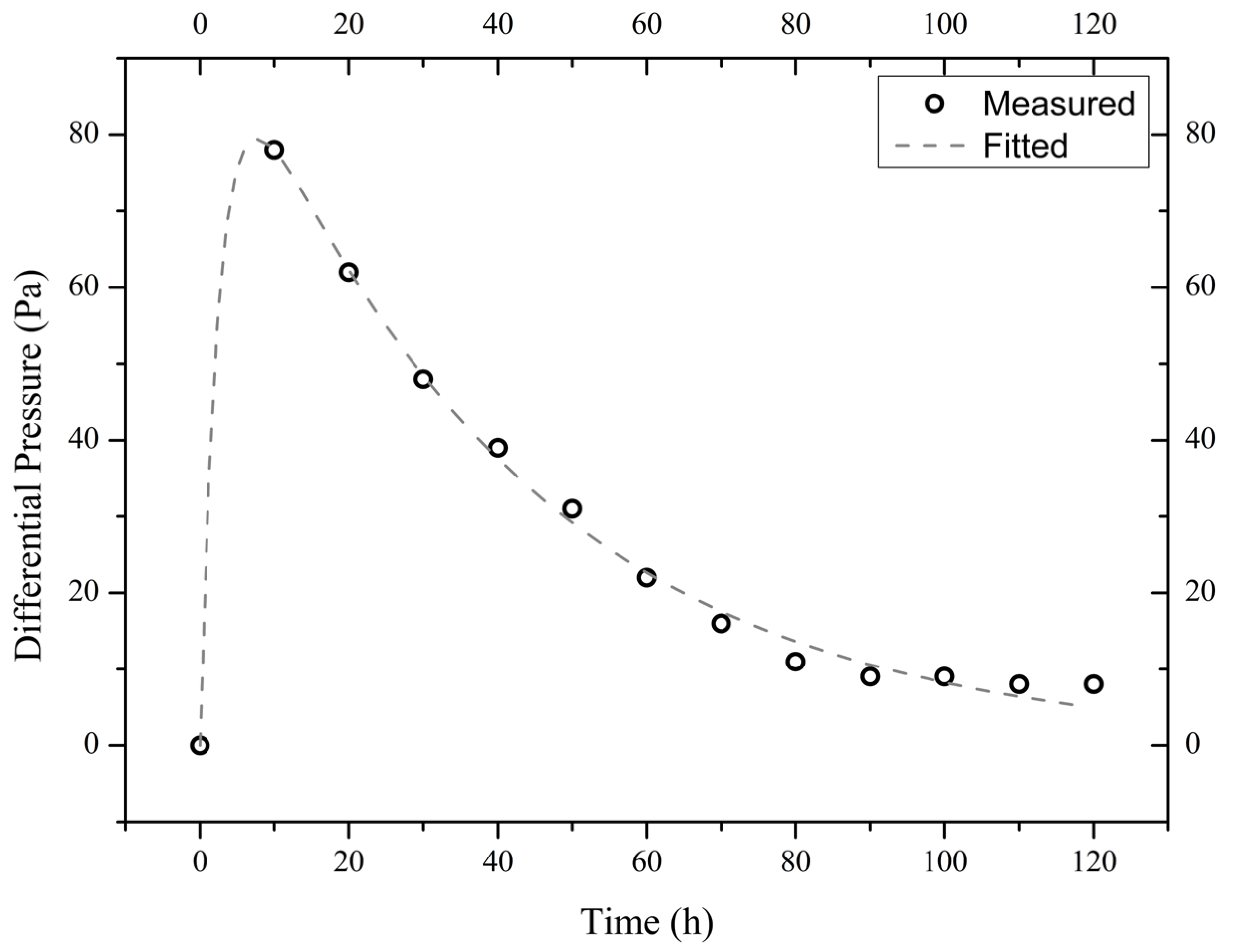
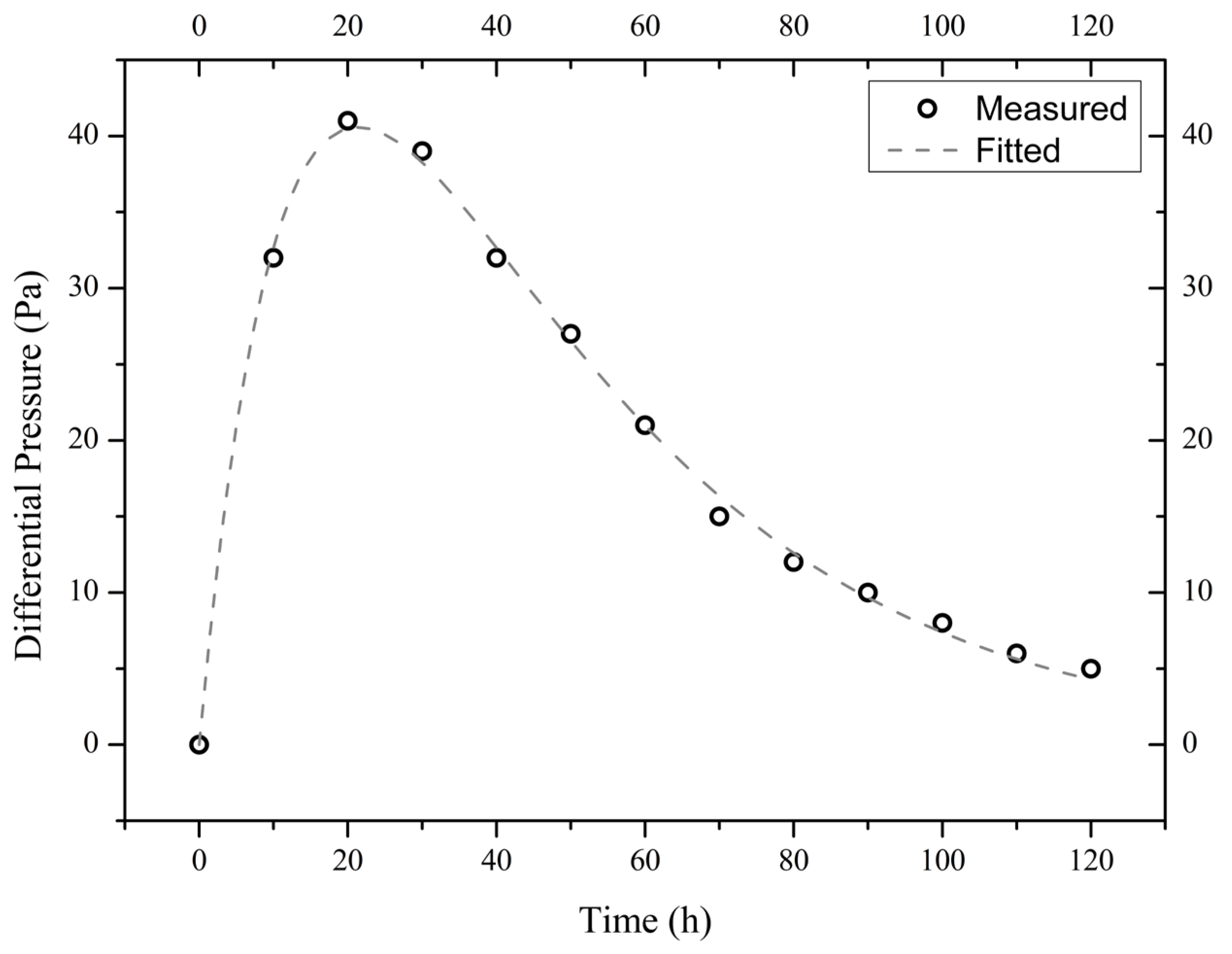
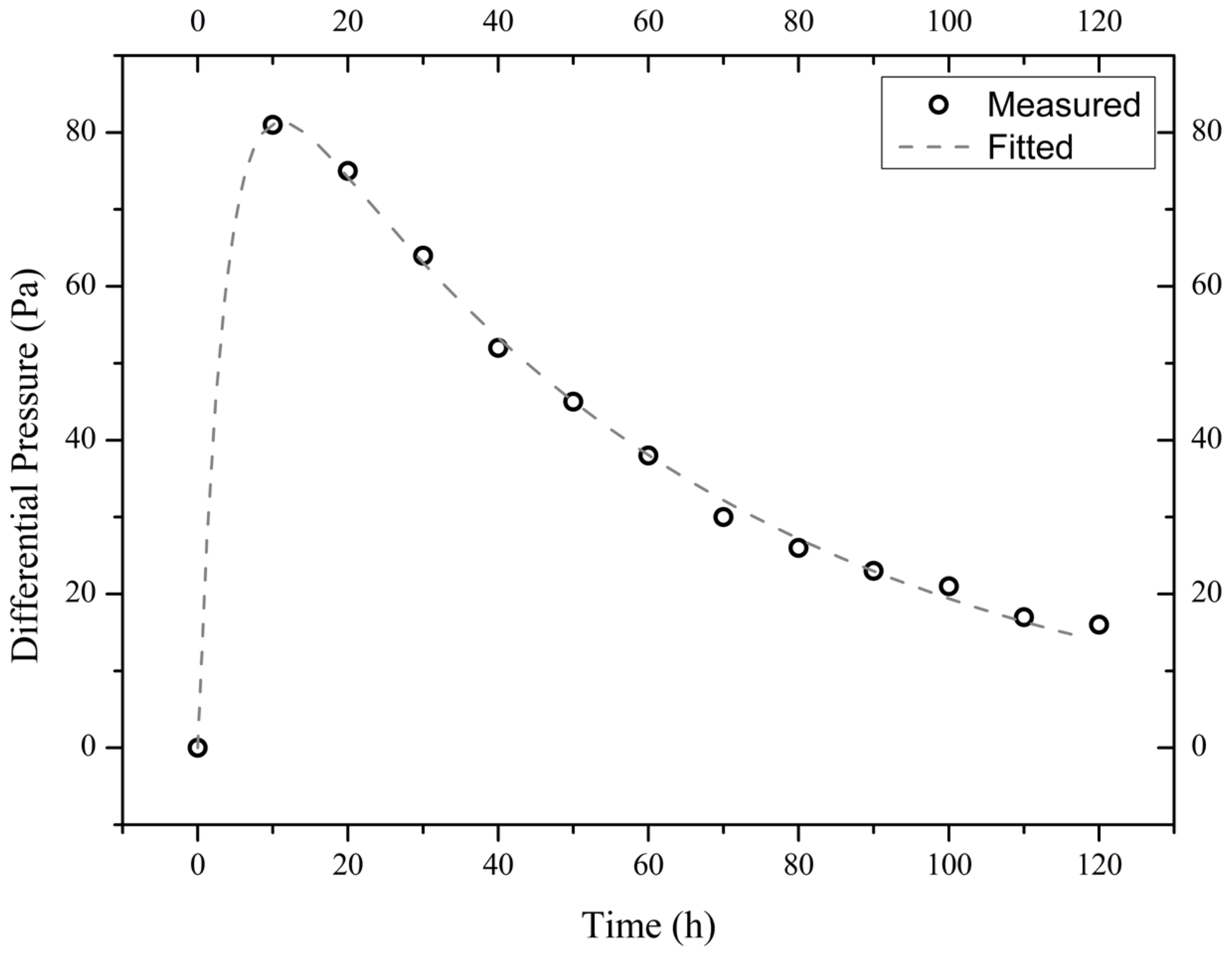
4. Results
4.1. Time Evolutions
4.2. Flow of Water
4.3. Uncertainties
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Borg, F.G. What Is Osmosis? Explanation and Understanding of a Physical Phenomenon. arXiv 2003. [Google Scholar] [CrossRef]
- Grathwohl, P. Diffusion in Natural Porous Media: Contaminant Transport, Sorption/Desorption and Dissolution Kinetics. In Topics in Environmental Fluid Mechanics, 1st ed.; Springer: Boston, MA, USA, 1998; p. 223. [Google Scholar]
- Mitchell, J.K.; Soga, K. Fundamentals of Soil Behavior, 3rd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2005; p. 592. [Google Scholar]
- Malusis, M.A.; Shackelford, C.D. Chemico-Osmotic Efficiency of a Geosynthetic Clay Liner. J. Geotech. Geoenviron. Eng. 2002, 128, 97–106. [Google Scholar] [CrossRef]
- Olsen, H.W. Liquid Movement Through Kaolinite under Hydraulic, Electric, and Osmotic Gradients. Am. Assoc. Pet. Geol. Bull. 1972, 56, 2022–2028. [Google Scholar]
- Neuzil, C.E. Osmotic Generation of ‘Anomalous’ Fluid Pressures in Geological Environments. Nature 2000, 403, 182–184. [Google Scholar] [CrossRef]
- Murray, H.H. Structure and composition of the clay minerals and their physical and chemical properties. Dev. Clay Sci. 2007, 3, 7–31. [Google Scholar]
- Grim, R.E. Clay Mineralogy, 2nd ed.; McGraw-Hill: New York, NY, USA, 1968; p. 596. [Google Scholar]
- Fritz, S.J. Ideality of Clay Membranes in Osmotic Processes: A Review. Clays Clay Miner. 1986, 34, 214–223. [Google Scholar] [CrossRef]
- Sherwood, J.D.; Craster, B. Transport of Water and Ions Through a Clay Membrane. J. Colloid Interface Sci. 2000, 230, 349–358. [Google Scholar] [CrossRef]
- Bader, S.; Kooi, H. Modelling of Solute and Water Transport in Semi-Permeable Clay Membranes: Comparison with Experiments. Adv. Water Resour. 2005, 28, 203–214. [Google Scholar] [CrossRef]
- Garavito, A.M.; Kooi, H.; Neuzil, C.E. Numerical Modeling of a Long-Term in Situ Chemical Osmosis Experiment in the Pierre Shale, South Dakota. Adv. Water Resour. 2006, 29, 481–492. [Google Scholar] [CrossRef]
- Garavito, A.M.; De Cannière, P.; Kooi, H. In Situ Chemical Osmosis Experiment in the Boom Clay at the Mol Underground Research Laboratory. Phys. Chem. Earth 2007, 32, 421–433. [Google Scholar] [CrossRef]
- Mokni, N.; Olivella, S.; Valcke, E.; Mariën, A.; Smets, S.; Li, X. Deformation and Flow Driven by Osmotic Processes in Porous Materials: Application to Bituminised Waste Materials. Transp. Porous Media 2011, 86, 635–662. [Google Scholar] [CrossRef]
- Kang, J.B.; Shackelford, C.D. Membrane Behavior of Compacted Clay Liners. J. Geotech. Geoenviron. Eng. 2010, 136, 1368–1382. [Google Scholar] [CrossRef]
- Mokni, N.; Olivella, S.; Alonso, E.E.E. Swelling in Clayey Soils Induced by the Presence of Salt Crystals. Appl. Clay Sci. 2010, 47, 105–112. [Google Scholar] [CrossRef]
- Mokni, N.; Olivella, S.; Li, X.; Smets, S.; Valcke, E. Deformation Induced by Dissolution of Salts in Porous Media. Phys. Chem. Earth 2008, 33, S436–S443. [Google Scholar] [CrossRef]
- Guarena, N.; Dominijanni, A.; Manassero, M. Reflection coefficient of a natural sodium bentonite in aqueous mixed electrolyte solutions: Positive and negative anomalous osmosis. Can. Geotech. J. 2024; Early Access. [Google Scholar] [CrossRef]
- Zheng, J.Z.; Zhang, Z.H.; Tian, G.L. Membrane Behavior of Kaolin Bentonite Mixtures. J. Geotech. Geoenviron. Eng. 2022, 148, 06022010. [Google Scholar] [CrossRef]
- Song, Z.Y.; Wei, C.F.; Cai, G.Q.; Zhang, Z.H.; Du, X.L. Membrane behavior of clay considering the effect of fixed charges. Sci. Total Environ. 2023, 856, 159196. [Google Scholar] [CrossRef]
- Fritz, C.J.; Scalia, J.; Shackelford, C.D.; Malusis, M.A. Determining Maximum Chemico-Osmotic Pressure Difference across Clay Membranes. J. Geotech. Geoenviron. Eng. 2020, 146, 06019018. [Google Scholar] [CrossRef]
- Zhang, Z.H.; Yang, H.W.; Song, Z.Y. Membrane behavior of clay under mixed solution conditions. Sci. Total Environ. 2024, 921, 171105. [Google Scholar] [CrossRef]
- Zhang, Z.H.; Yang, H.W.; Song, Z.Y. Membrane efficiency model of clay under multiionic conditions. J. Clean. Prod. 2023, 429, 139518. [Google Scholar] [CrossRef]
- Mazzieri, F.; Bernardo, D. Chemico-Osmotic Coefficients of Geosynthetic Clay Liners under Different Confinement Conditions. In Proceedings of the Geo-Congress on Sustainable Infrastructure Solutions from the Ground Up, Los Angeles, CA, USA, 26–29 March 2023. [Google Scholar]
- Bernardo, D.; Domizi, J.; Fratalocchi, E.; Mazzieri, F. Chemico-Osmotic efficiency of geosynthetic clay liners: Testing apparatus and preliminary results. In Proceedings of the 12th International Conference on Geosynthetics (12ICG), Rome, Italy, 17–21 September 2023. [Google Scholar]
- Tong, S.; Sample-Lord, K.M.; Rahman, S.A.B.; Yesiller, N.; Hanson, J.L. Diffusion and membrane behavior of an exhumed geosynthetic clay liner. In Proceedings of the 12th International Conference on Geosynthetics (12ICG), Rome, Italy, 17–21 September 2023. [Google Scholar]
- Malusis, M.A.; Scalia, J.; Norris, A.S.; Shackelford, C.D. Effect of chemico-osmosis on solute transport in clay barriers. Environ. Geotech. 2020, 7, 447–456. [Google Scholar] [CrossRef]
- Rosanne, R.; Paszkuta, M.; Tevissen, E.; Adler, P.M. Thermodiffusion in Compact Clays. J. Colloid Interface Sci. 2003, 267, 194–203. [Google Scholar] [CrossRef] [PubMed]
- Rosanne, M.; Paszkuta, M.; Adler, P.M. Thermodiffusional Transport of Electrolytes in Compact Clays. J. Colloid Interface Sci. 2006, 299, 797–805. [Google Scholar] [CrossRef] [PubMed]
- Soler, J.M. The Effect of Coupled Transport Phenomena in the Opalinus Clay and Implications for Radionuclide Transport. J. Contam. Hydrol. 2001, 53, 63–84. [Google Scholar] [CrossRef] [PubMed]
- Gonçalvès, J.; Trémosa, J. Estimating Thermo-Osmotic Coefficients in Clay-Rocks: I. Theoretical Insights. J. Colloid Interface Sci. 2010, 342, 166–174. [Google Scholar] [CrossRef]
- Trémosa, J.; Gonçalvès, J.; Matray, J.M.; Violette, S. Estimating Thermo-Osmotic Coefficients in Clay-Rocks: II. In Situ Experimental Approach. J. Colloid Interface Sci. 2010, 342, 175–184. [Google Scholar] [CrossRef]
- Zubair, M.M.; Saleem, H.; Zaidi, S.J. Recent progress in reverse osmosis modeling: An overview. Desalination 2023, 564, 116705. [Google Scholar] [CrossRef]
- Qasim, M.; Badrelzaman, M.; Darwish, N.N.; Darwish, N.A.; Hilal, N. Reverse osmosis desalination: A state-of-the-art review. Desalination 2019, 459, 59–104. [Google Scholar] [CrossRef]
- Medved, I.; Černý, R. Osmosis in Porous Media: A Review of Recent Studies. Microporous Mesoporous Mater. 2013, 170, 299–317. [Google Scholar] [CrossRef]
- Húlan, T.; Trník, A.; Štubňa, I.; Bačík, P.; Kaljuvee, T.; Vozár, L. Thermomechanical Analysis of Illite from Füzérradvány. Mater. Sci. 2015, 21, 429–434. [Google Scholar] [CrossRef][Green Version]
- Staverman, A.J.; Smith, J.A.M. Physical Chemistry: Enriching Topics from Colloid and Surface Sciences, 1st ed.; Theorex: La Jolla, CA, USA, 1975; p. 343. [Google Scholar]
- Kedem, O.; Katchalsky, A. Thermodynamic Analysis of the Permeability of Biological Membranes to Non-Electrolytes. Biochim. Biophys. Acta 1958, 27, 229–246. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes. I. Phys. Rev. 1931, 37, 405–426. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes. II. Phys. Rev. 1931, 38, 2265–2279. [Google Scholar] [CrossRef]
- JCGM 100:2008; Evaluation of Measurement Data—Guide to the Expression of Uncertainty in Measurement. Joint Committee for Guides in Metrology. JCGM: Paris, France, 2008.


| Measured Quantity | Value | uc | Unit | Relative uc (%) | |
|---|---|---|---|---|---|
| Exposed area S | 1.59 × 10−3 | 3.5 × 10−6 | m2 | 0.2 | |
| Surface area of the reservoir Ab | 4 × 10−2 | 1.5 × 10−4 | m2 | 0.4 | |
| Sample thickness | 5 × 10−3 | 1 × 10−4 | m | 2.0 | |
| Total number of water moles nw0 | 332.96 | 0.57 | – | 0.2 | |
| Temperature T | 295 | 2 | K | 0.7 | |
| Initial mole fraction difference Δxsi | 2.99 × 10−2 | 5.6 × 10−5 | – | 0.2 | |
| Mole fraction in equilibrium xs0 | 1.50 × 10−2 | 3.8 × 10−5 | – | 0.3 | |
| I900-1 | A1 | 106.83 | 4.09 | Pa | 3.8 |
| A2 | 7.31 × 10−6 | 2.9 × 10−7 | s−1 | 4.0 | |
| A3 | 8.07 × 10−5 | 8.2 × 10−6 | s−1 | 10.2 | |
| Osmotic efficiency σ | 3.99 × 10−4 | 4.7× 10−5 | – | 11.8 | |
| Molar membrane permeability k | 5.03 × 10−4 | 2.0 × 10−5 | mol∙Pa−1∙s−1∙m−2 | 4.0 | |
| Molar salt diffusivity D | 7.53 | 0.76 | mol∙m−2∙s−1 | 10.1 | |
| Effective salt diffusivity Ds | 6.73 × 10−7 | 7.0 × 10−8 | m2∙s−1 | 10.4 | |
| Effective water diffusivity Dw | 6.21 × 10−7 | 6.4 × 10−8 | m2∙s−1 | 10.3 | |
| Darcy permeability kD | 0.046 | 0.002 | Darcy | 4.3 | |
| I900-2 | A1 | 116.49 | 21.81 | Pa | 18.3 |
| A2 | 7.63 × 10−6 | 6.5 × 10−7 | s−1 | 8.5 | |
| A3 | 2.04 × 10−5 | 2.4 × 10−6 | s−1 | 11.8 | |
| Osmotic efficiency σ | 8.1 × 10−5 | 1.8 × 10−5 | – | 22.2 | |
| Molar membrane permeability k | 5.25 × 10−4 | 4.5 × 10−5 | mol∙Pa−1∙s−1∙m−2 | 8.6 | |
| Molar salt diffusivity D | 1.94 | 0.22 | mol∙m−2∙s−1 | 11.3 | |
| Effective salt diffusivity Ds | 1.74 × 10−7 | 2.0 × 10−8 | m2∙s−1 | 11.5 | |
| Effective water diffusivity Dw | 1.61 × 10−7 | 1.9 × 10−8 | m2∙s−1 | 11.8 | |
| Darcy permeability kD | 0.048 | 0.004 | Darcy | 8.3 | |
| I900-3 | A1 | 104.62 | 3.90 | Pa | 3.7 |
| A2 | 4.86 × 10−6 | 2.0 × 10−7 | s−1 | 4.1 | |
| A3 | 7.41 × 10−5 | 1.3 × 10−5 | s−1 | 17.5 | |
| Osmotic efficiency σ | 5.54 × 10−4 | 1.05 × 10−4 | – | 19.0 | |
| Molar membrane permeability k | 3.35 × 10−4 | 1.4 × 10−5 | mol∙Pa−1∙s−1∙m−2 | 4.2 | |
| Molar salt diffusivity D | 6.91 | 1.21 | mol∙m−2∙s−1 | 17.5 | |
| Effective salt diffusivity Ds | 6.22 × 10−7 | 1.1 × 10−7 | m2∙s−1 | 17.7 | |
| Effective water diffusivity Dw | 5.74 × 10−7 | 1.0 × 10−7 | m2∙s−1 | 17.4 | |
| Darcy permeability kD | 0.031 | 0.001 | Darcy | 3.2 | |
| Sample | Darcy Flow | Osmosis | Diffusion | Ratio |
|---|---|---|---|---|
| (×10−3 mol∙m−2∙s−1) | (%:%:%) | |||
| I900-1 | 38.7 | 1634.5 | 207.6 | 2.1:86.9:11.0 |
| I900-2 | 21.2 | 346.3 | 53.5 | 5.0:82.3:12.7 |
| I900-3 | 26.7 | 1511.5 | 190.4 | 1.6:87.4:11.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mánik, M.; Medveď, I.; Keppert, M.; Suchorab, Z.; Trník, A. Determination of Osmotic Flow in Water Transport in an Illitic Clay. Materials 2025, 18, 338. https://doi.org/10.3390/ma18020338
Mánik M, Medveď I, Keppert M, Suchorab Z, Trník A. Determination of Osmotic Flow in Water Transport in an Illitic Clay. Materials. 2025; 18(2):338. https://doi.org/10.3390/ma18020338
Chicago/Turabian StyleMánik, Marek, Igor Medveď, Martin Keppert, Zbigniew Suchorab, and Anton Trník. 2025. "Determination of Osmotic Flow in Water Transport in an Illitic Clay" Materials 18, no. 2: 338. https://doi.org/10.3390/ma18020338
APA StyleMánik, M., Medveď, I., Keppert, M., Suchorab, Z., & Trník, A. (2025). Determination of Osmotic Flow in Water Transport in an Illitic Clay. Materials, 18(2), 338. https://doi.org/10.3390/ma18020338








