The purpose of fitting models is to gain insights into the mechanisms underlying the observed behaviour. As the complexity of the models grows, however, the number of parameters to be explored goes up, and this brings two problems. First, the process of best-fitting these parameters becomes increasingly uncertain, with multiple local optima. This makes it much more difficult to find and recognise the global best fit. The second problem is that increasing doubt is cast on whether the chosen model, even if it is the global best fit, is a true representation of what is actually happening. Other local optima with significantly different parameter values may give fits that are almost as good.
4.2.1. Elastic Stretching
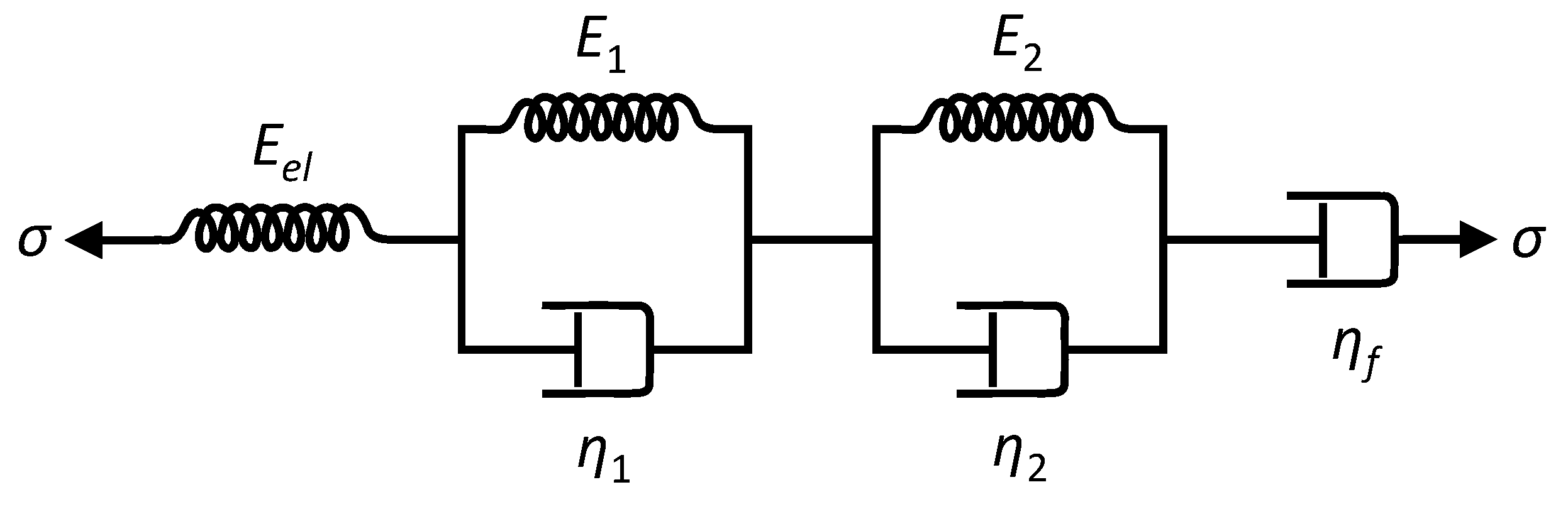
The model described in
Section 3.4 and Equation (
4) was fitted to the strain versus time response for each individual step of the stepped-stress tests. Two Kelvin–Voigt stages were used in those cases where adding a second Kelvin–Voigt stage significantly improved the fit; otherwise, just one Kelvin–Voigt stage was used. The corresponding elastic stretching component
for each step was then estimated as the difference between the fitted
coefficient for that step and the strain immediately before the corresponding stress step was applied. These individual step estimates could then be summed to give estimates of the total elastic strain.
For strings N7 and N21, a consequence of the tension modulation cycles applied at the end of each step to measure the Young’s modulus (
Section 3.2) was that the string frequency at the end of the Young’s modulus test was usually slightly different from the target frequency for that step. This in turn meant that the strain level recorded at the start of the next step, immediately before the next applied stress change, was slightly wrong. To attempt to correct for this, the fitted model for each step was used to estimate the expected strain level at the start time of the next step, prior to calculating the elastic stretching components.
Similar corrections were not required for string N20, since the frequency modulation used with that string, to continuously monitor the Young’s modulus, always finished back at the required target frequency, while the tests run with the other strings did not include any form of Young’s modulus test and ran directly from one target frequency to the next, as listed in
Table 1.
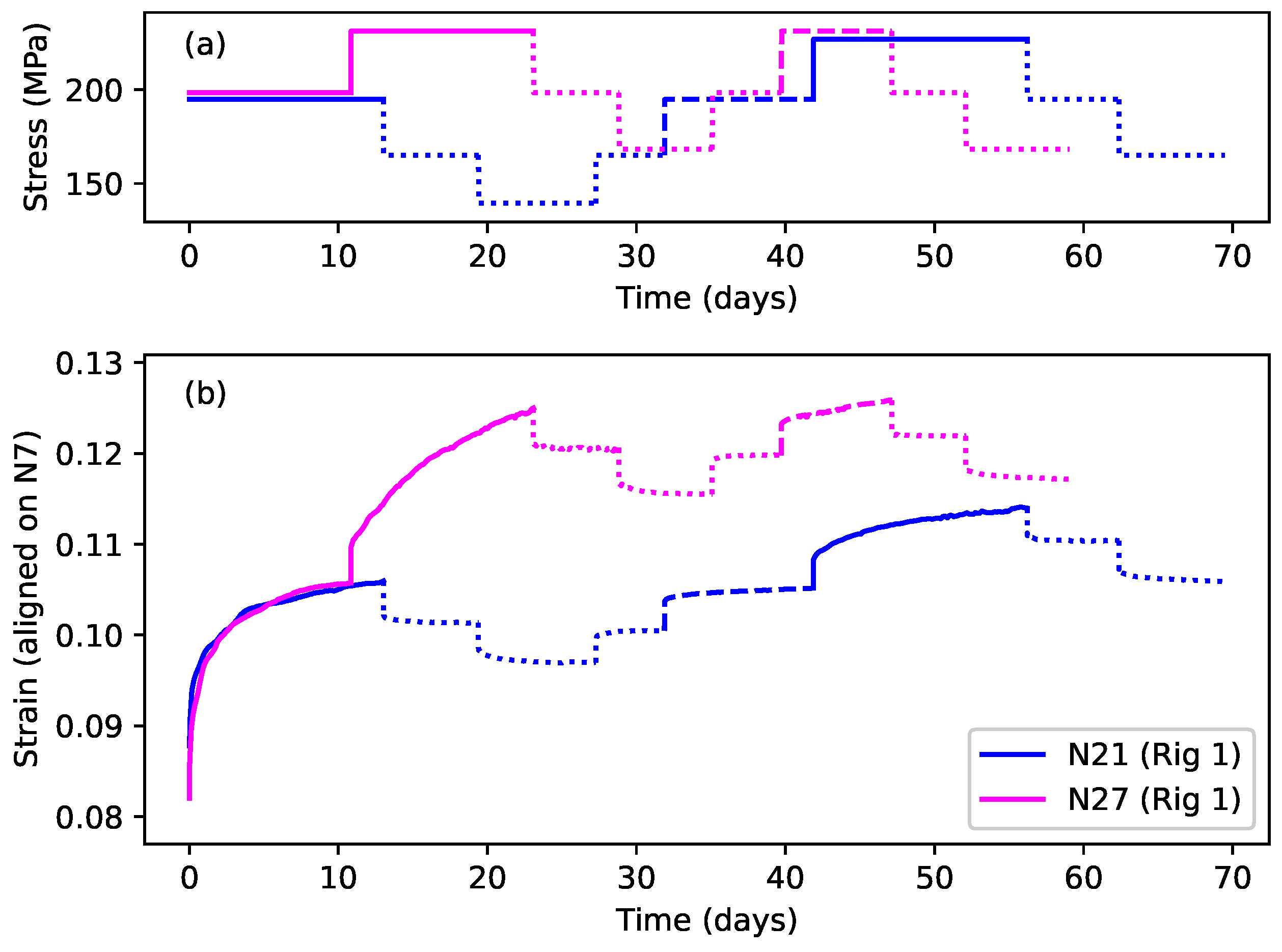
For string N21 step 10, the form of the strain response to the period of elevated temperature was different to the other steps (
Figure 5c), so in this case the expected strain level, at the start of step 11, was estimated by fitting a slightly different function, equivalent to a single Kelvin–Voigt stage, to just the tail section of the step 10 response.
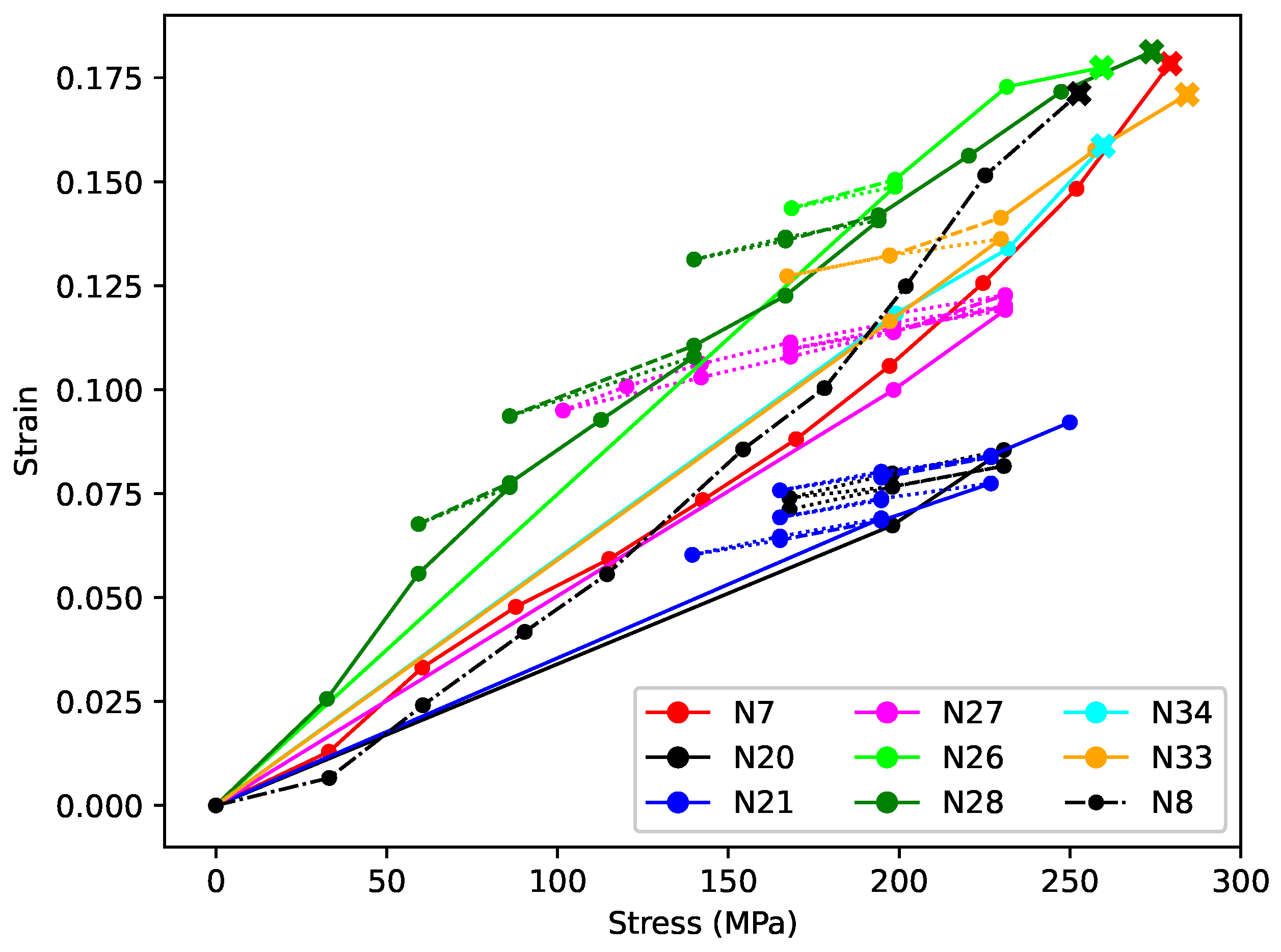
Figure 7 shows the total elastic strain
plotted against the applied stress
. As for
Figure 6, this is again the opposite of the normal convention, but logical in this context. To aid in the comparison, the plots have been aligned vertically in the same way as for
Figure 4b and
Figure 5b, with the common alignment point being the strain reached at the end of the first step with an applied stress of close to 200 MPa (corresponding to a target fundamental frequency of 425 or 429 Hz), and the strain at the end of string N7 step 7 used as the reference level. The remaining spread in the responses at around 200 MPa is due to the applied stress sequences for most of the strings passing through 200 MPa on multiple occasions (
Figure 5a), each time with slightly different strain response levels.
The responses clearly displayed a common trend, with the elastic stiffness increasing with the applied stress and strain, as indicated by the gradual flattening of the strain versus stress responses.
From the tensile Young’s modulus tests conducted at the end of each step with string N7 (
Section 3.2), it became clear very early on that these nylon instrument strings experienced significant strain hardening, with the Young’s modulus increasing with the applied stress. Subsequent studies [
6] led to an expression for the measure of Young’s modulus
at 20 °C, obtained from tension-modulation tests:
where
(expressed in MPa) is the notional stress, and
(expressed in kg/m
3) is the bulk density of the unstretched string.
Murphy [
11] found a reasonable match between the fitted stiffness of the series spring in some of his models and the corresponding value of
. If, as might be expected, the Young’s modulus for the elastic stretching obeyed a similar relation
, then the total elastic strain up to an applied stress
should be:
Since the tests run with strings N7 and N28 spanned a wide range of stress levels, functions of this form could be fitted to their total elastic strain versus applied stress responses. Including an additional residual offset
to allow for the vagaries of the initial string stretching was found to improve the fits obtained, and the last data point, where the string broke, was excluded in both cases. The dashed lines included in
Figure 7 show the fitted functions. The fitted coefficients were not an exact match for the values predicted by Equation (
10), but they were reasonably close, especially for string N7, and the quality of the fits (
= 0.9996 for string N7 and 0.9905 for string N28) certainly supported the use of Equation (
11) as a model for the elastic stretching component
.
4.2.2. Recoverable Creep
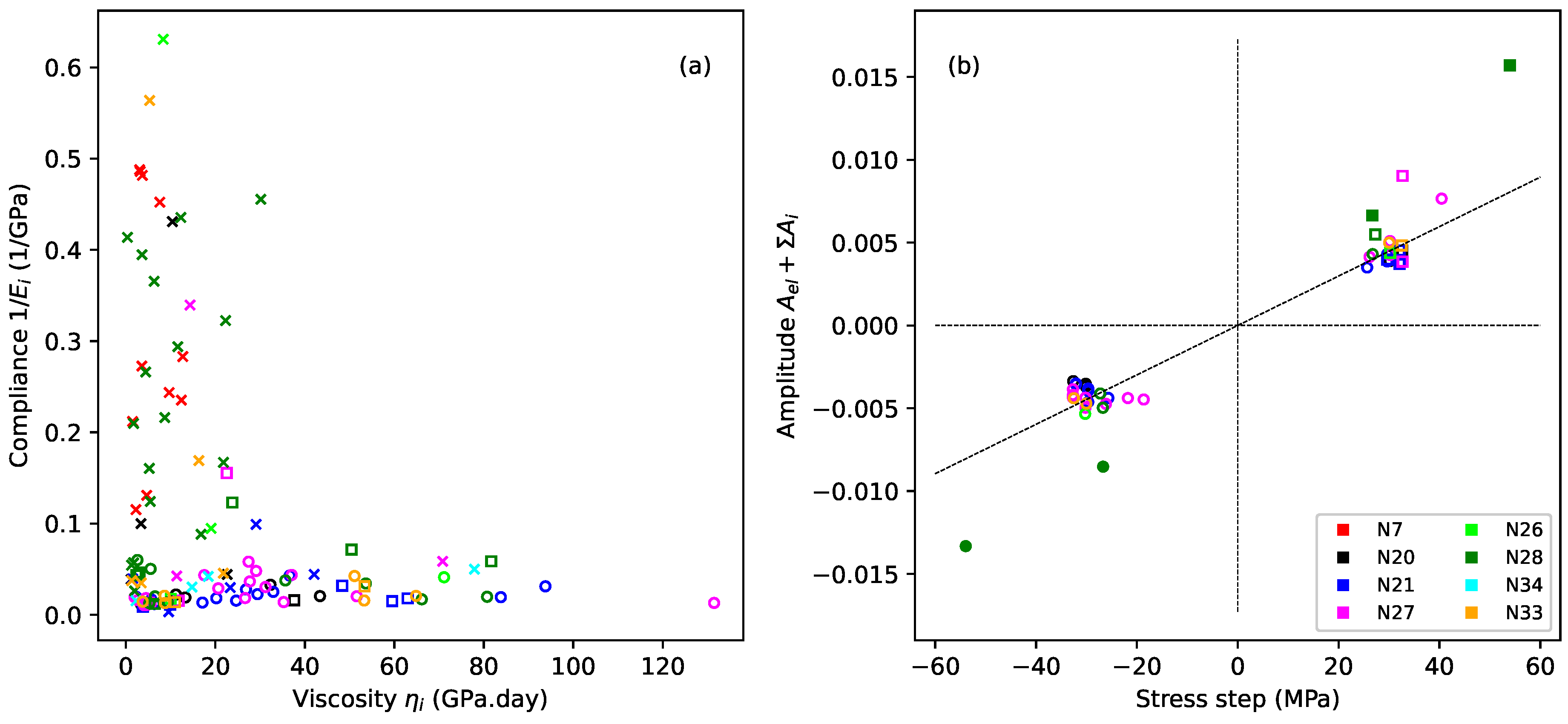
Figure 8 explores the fitted coefficients for the Kelvin–Voigt stages from the step-by-step model fitting exercise described in the previous section. Crosses and squares indicate, respectively, steps where the applied stress exceeded or matched the previous maximum; circles indicate steps where the applied stress was below the previous maximum.
Figure 8a plots the compliance
against the viscosity
for all steps except the last steps where the string broke (all strings except N20, N21, and N27). The viscosity values in
Figure 8a, and later in
Table 2, have been expressed in units of GPa.day. While this is not a formal SI unit, it gives an immediate intuitive appreciation of the time constants involved.
There was a clear distinction between the steps where the applied stress exceeded the previous maximum (crosses) and steps where this was not the case, with the former generally having much higher compliance values and only a modest range of relatively low viscosities, while the latter almost uniformly had lower compliance values, less than 0.1/GPa, but a much wider range of viscosities. This confirms that whatever was happening during the steps exhibiting the plastic creep episodes must have been quite different from the behaviour during the recoverable creep episodes.
Attempts to analyse the contributions from the different fitted Kelvin–Voigt stages were frustrated by the degree of variation in the fitted coefficients.
Figure 8b therefore takes a different approach and plots the total amplitude of all the Kelvin–Voigt stages plus the fitted elastic strain for each step,
, against the change in the applied stress, but now only for those steps where the applied stress did not exceed the previous maximum, and again excluding the last steps where the string broke. Most of the points are tightly and symmetrically clustered, supporting the view that the creep behaviour during these steps was both symmetric and fully recoverable.
The fitted line in
Figure 8b shows the average gradient for all the steps where the applied stress was less than the corresponding previous maximum (circles), excluding the points for string N28 shown with the filled markers. The trend was not as clear when the fitted elastic strain was excluded from the amplitude calculations. It seems entirely plausible that, working with real measured data, sampled at discrete time points, the split of fitted amplitudes between the elastic stretching component and the Kelvin–Voigt stages may have been somewhat noisy, so that the underlying trend only appeared in its clearest form when plotting the combined amplitude in this way.
The anomalous nature of the excluded points for string N28 was already apparent in the strain plot in
Figure 5b and in the gradient variations in
Figure 6. For that string, the strain changes during the last period when the applied stress was reduced (starting from step 12 on day 99) were very similar to those seen with the other strings. The strain changes during the other two earlier periods of reduced stress (steps 4, 5, 8, and 9), however, were disproportionately larger. These four steps are the ones indicated with filled markers in
Figure 8b. The stress reduction applied to string N27 (
Figure 5a) came down almost as far (N27 step 11) as for string N28 step 8 without causing the same degree of strain reduction. Indeed, as will be seen in
Figure 9c, the strain response of string N27 displayed a very high degree of consistency during the long sequence of reduced-stress steps applied to it. Perhaps the behaviour of string N28 when these earlier stress reductions were applied was influenced in some significant way by the overall stretching state of the string (explored below in
Section 4.2.3), but this is only conjecture.
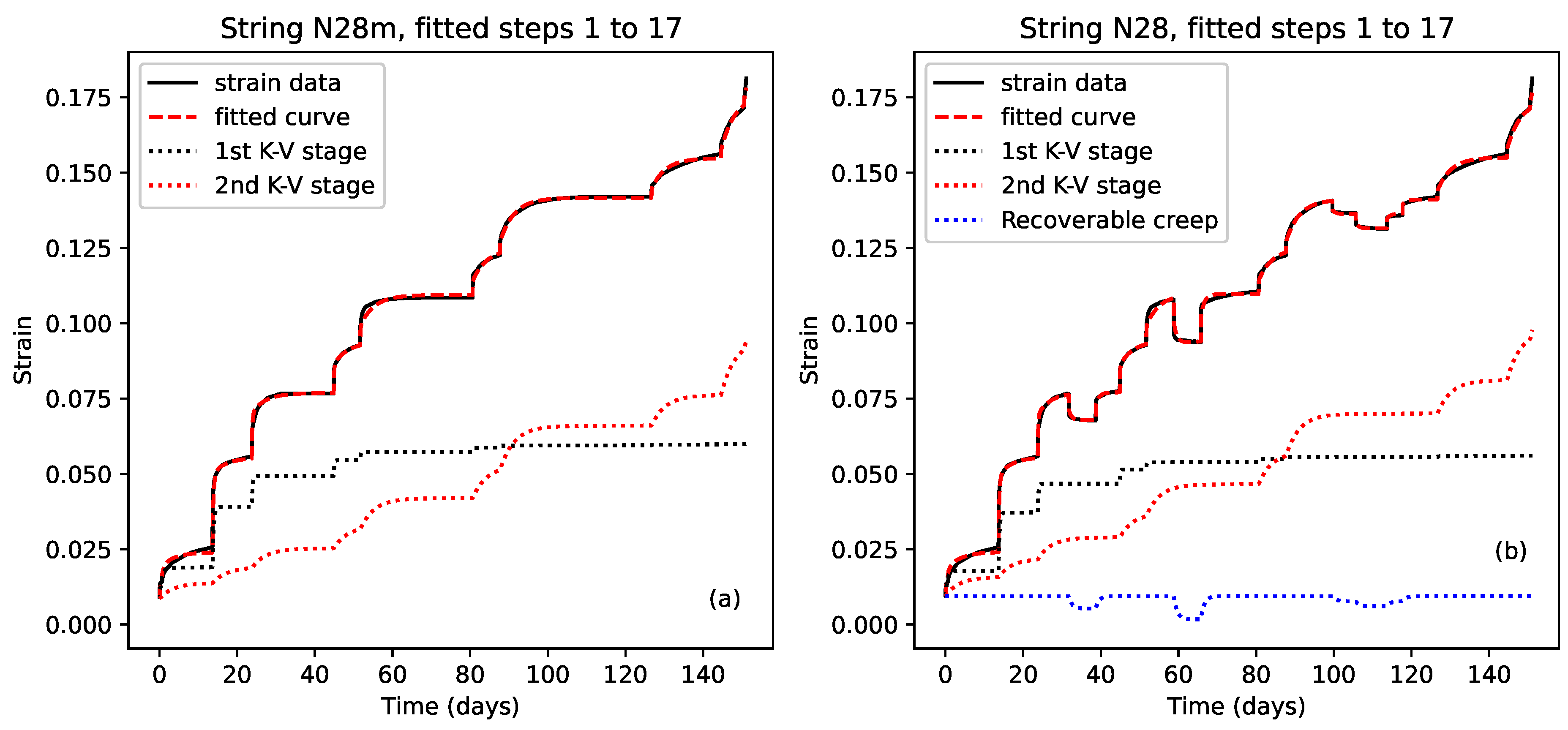
Following on from the above findings,
Figure 9 shows some results from fitting a single model to sequences of steps where the applied stress did not exceed the previous maximum. The model was essentially that given in Equations (
3) and (
4), with the variation in the elastic stretching component modelled using Equation (
11). The results from the step-by-step model fitting and analysis initially suggested that a single Kelvin–Voigt stage might be sufficient, but it was found that for the best multi-step fit, two stages were needed, one to model the observed recoverable creep behaviour, and one, working with the series dashpot, to model a slower trend.
The first fitted step, step
m, for these step sequences displaying recoverable creep, was necessarily partway along the full step sequence. Consequently, fitted values of
for the Kelvin–Voigt stages, for use in Equation (
6), were not available for the first fitted step. Furthermore, since the plastic creep behaviour of the preceding steps was very different from the recoverable creep behaviour being modelled, estimates for
could not sensibly be made.
The start point for the model evaluation of the Kelvin–Voigt stages and the series dashpot was therefore relocated to the start of the first fitted step, using the expressions given in Equations (
7) and (
9). An amended offset
was included to cover both the initial residual strain offset
and the non-elastic stretching prior to the start of the fitted steps. The model coefficients
and
were treated as constants, independent of the applied stress. Examining the single step fitting results for the steps immediately before the fitted sequences suggested that any ongoing contributions from their viscoelastic (Kelvin–Voigt) components should be very small, validating the use of Equation (
7).
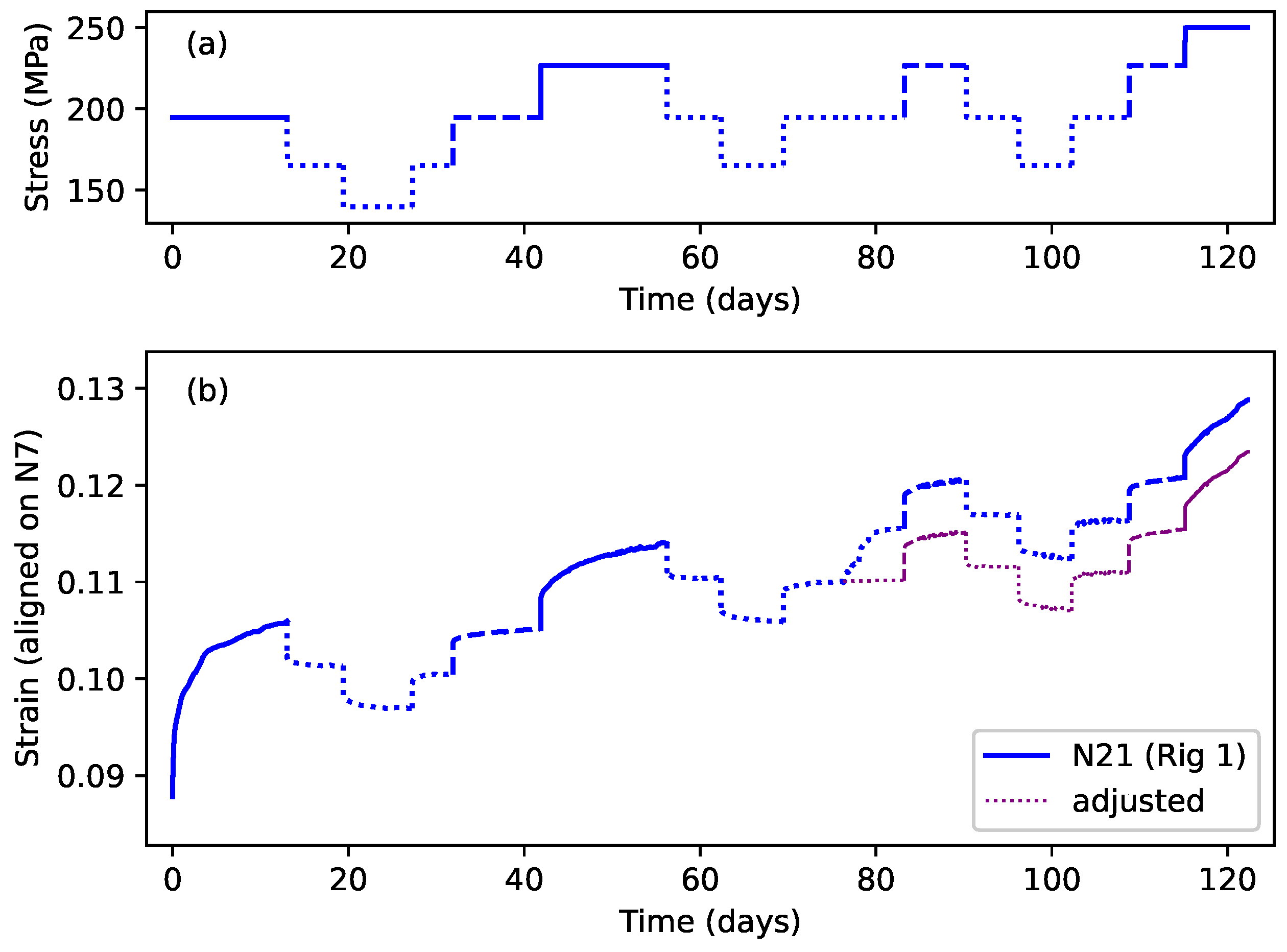
The fits shown in
Figure 9 are for the longest available sequences of steps where the applied stress did not exceed the previous maximum. For string N21, the additional strain triggered by the temporary increase in temperature at step 10 (
Section 3.2) was replaced by extrapolating the model fitted to step 9, during the step-by-step modelling exercise, over the duration of step 10, and then adjusting the strain data for the subsequent steps, so that the step 11 response followed on from the extended step 9 response. This modified strain response is shown as the dotted purple line in
Figure 10b. It was found that the fit quality across the transition point between the extended step 9 and step 11 could be improved by allowing the fitting algorithm to apply a small strain level ‘tweak’ to the steps after that point. The required tweak was very small at only 0.00056.
Attempts to auto-fit the elastic stretching coefficients
and
were unsuccessful; the fitting function appeared to have difficulty choosing between the elastic stretching component and a Kelvin–Voigt stage. These coefficients were instead varied manually, around the values obtained from the fits for strings N7 and N28 (shown as the dashed lines in
Figure 7) and those given by Equation (
10), for the Young’s modulus measure
from [
6]. Of the combinations tried, the best results were obtained when the values of
and
matched those given by Equation (
10).
The fits shown in
Figure 9 are excellent, with the dashed red lines of the modelled responses closely matching the black lines of the measured strain responses. The fit to string N27 (
Figure 9c) provided particularly strong evidence for the recoverable nature of the creep associated with stress steps at or below the level of the previous maximum applied stress, covering a wide overall range of stress levels, including one case of a double step (step 12).
The dotted blue lines in
Figure 9 (offset for clarity) show the contributions from the primary Kelvin–Voigt stage, while the dotted orange lines show the combined contributions from the second Kelvin–Voigt stage and the series dashpot. For the single step fits, this slower trend would have been covered just by the series dashpot in many cases. The dotted orange line in
Figure 9c also gave a particularly clear illustration of the type of lag which should be expected in the response of a Kelvin–Voigt stage having a large time constant (approximately 3 weeks in this case) and correspondingly slow response.
It was not clear whether these slower components of the fitted model were related to the previous, or perhaps ongoing, plastic creep behaviour of the studied strings, or to some other component of the strain response. It was this aspect of the string behaviour, though, that led to the cautionary statement made in the Introduction that the observed ‘plastic’ deformation may not in fact have been truly permanent. The negative slope of the response for string N20 (
Figure 9a) presumably indicates some form of energy release from the previous stretching during the first two stress steps applied to that string. It is not clear why this string should have behaved quite so markedly in this way, but it is perhaps worth noting that this was the string for which a frequency modulation cycle was applied throughout its testing.
Extending the model to cover any steps where the applied stress exceeded the previous maximum did not produce good results. The model did, however, appear to be able to cover those steps where the stress matched the previous maximum in most cases. One exception was string N27 step 15 (
Figure 9c), and careful examination showed that the fit actually started to fail during the preceding step 14.
For string N21,
Figure 9b shows a fairly good fit result spanning steps both before (steps 7–9) and after (steps 11–15) step 10, when the additional heating was applied. While somewhat closer fits could be obtained for shorter sequences without step 10, this result does support the observation that the additional temperature-induced stretching did not appear to have any great effect on the subsequent string stretching behaviour.
Table 2 shows sets of fitted coefficients from fitting the multi-step model to different step sequences. The first five rows are for the fits shown in
Figure 9 plus a sequence from string N28; the next two compare the fits obtained for shorter sequences from string N21, before and after the additional heating was applied at step 10; and the last set shows how the fitted coefficients varied as the length of the fitted sequence was changed for string N27. The
and
coefficients are for the primary Kelvin–Voigt stage, represented by the dotted blue lines in
Figure 9, while the
,
, and
coefficients are for the second Kelvin–Voigt stage and the series dashpot, for which the combined contribution is represented by the dotted orange lines. The viscosity values have again been given in units of GPa.day, allowing the time constants
(in days) for the Kelvin–Voigt stages to be obtained directly by dividing the viscosity values by the corresponding Young’s modulus values.
There is considerable variation between the different strings, but the overall magnitude of each coefficient is fairly consistent. Looking at the results for string N27, fitting to different step sequence lengths, it can be seen that the coefficients varied with the number of fitted steps, but appeared to settle down somewhat as the sequences became longer. Comparing the results for the two shorter step sequences from string N21, from before and after step 10, with the longer sequence spanning step 10, the coefficients for the two shorter sequences are fairly similar, further supporting the observation that the additional heating applied during step 10 did not affect the underlying string behaviour. The coefficients for the longer sequence are rather different, and the scale of the differences is perhaps rather larger than might be expected from the different sequence lengths, based on the results just discussed for string N27, but this was presumably a consequence of residual inconsistencies following the adjustments to the step 10 response.
4.2.3. Plastic Creep
The models already described for fitting the elastic stretching and recoverable creep components were simple and robust, with model coefficients that were independent of the applied stress, suggesting that the fitted models were likely to be a good match for the real underlying behaviour of the strings. Even then, as discussed earlier, it was necessary to fit the coefficients for the elastic stretching model separately from those for the recoverable creep.
The plastic stretching behaviour was quickly found to be more complex.
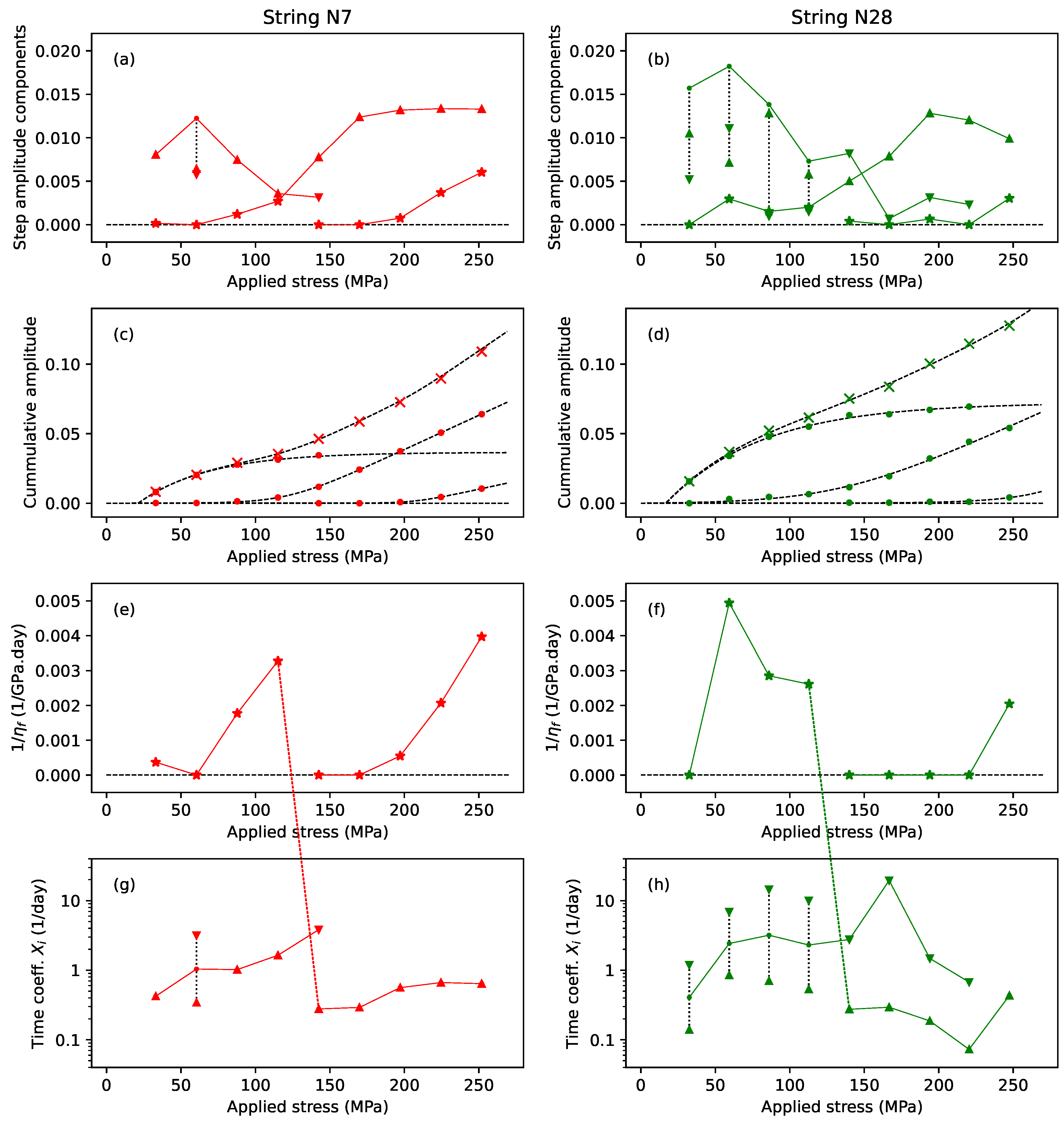
Figure 11 explores the fitted coefficients from the step-by-step model fitting exercise for strings N7 (left) and N28 (right) as a function of the applied stress. Up- and down-triangles (▲,▼) mark the coefficients for the slower (or only) and faster Kelvin–Voigt stages, respectively, while stars (★) mark the contributions from the series dashpot.
Figure 11a,b collect together the various non-elastic amplitude contributions to the strain at each step. For the series dashpot, the values shown are the amplitude contributions at the end of each step,
. The solid lines trace selected sequences through these coefficients, to be described in some detail shortly. The dotted vertical black lines indicate where the coefficients
and
of two Kelvin–Voigt stages have been summed to give the corresponding point in the marked sequence.
Figure 11c,d show the cumulative amplitude (filled circles) along the sequences shown in
Figure 11a,b, and the total cumulative amplitude (crosses). The dashed black lines show the results of fitting functions to these points (Equations (
12) and (
13) below).
Figure 11e,f show the inverse of the viscosity for the series dashpot
, giving a measure of the dashpot fluidity, or speed of response.
Figure 11g,h show the time coefficients
for the Kelvin–Voigt stages, and use a logarithmic
y-axis to better accommodate the range of values. The solid lines, and the lines jumping between subplots, trace the same sequences shown in
Figure 11a,b. The marked sequence points for the second point in
Figure 11g and the first four points in
Figure 11h were placed at the geometric mean (the mid-point on a logarithmic scale) of the corresponding
and
coefficients. This geometric mean is not intended as a specific prediction; it simply indicates that the sequence was expected to run somewhere in this range.
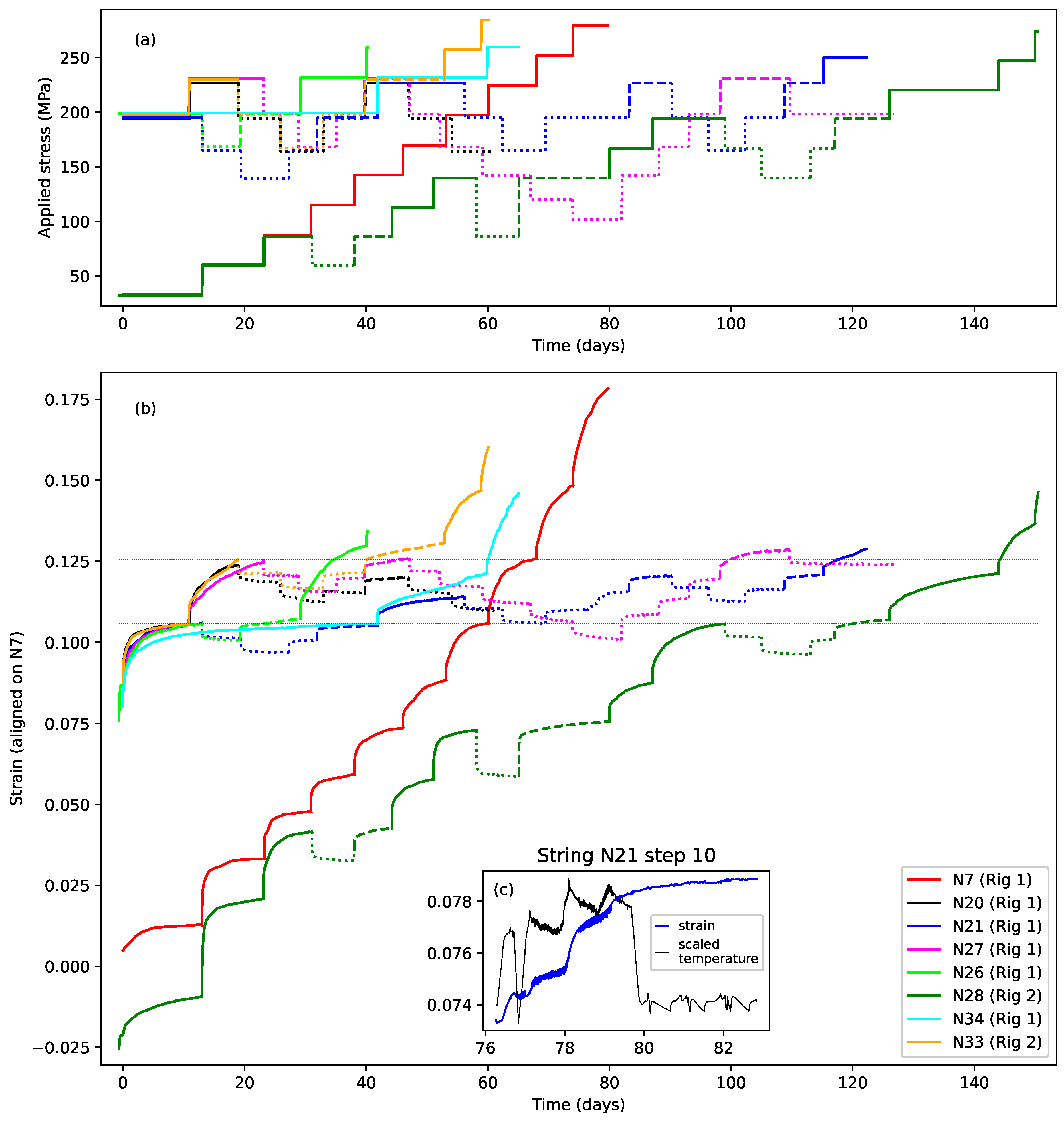
The data selection for string N7 was straightforward, since every step appeared to exhibit plastic stretching, with no periods of reduced stress in the applied stress sequence, and no apparent instances of the plastic creep constraint seen with some of the other strings. The plots for string N7 therefore show the results for all the first nine steps, with just the last step, where the string broke, excluded. For string N28, the data selection was complicated by the presence of the reduced-tension episodes. For
Figure 11b, to best display the full extent of the plastic stretching, the amplitude values shown include the contributions from any subsequent steps at less than or the same applied stress level. Hence, the values shown at 86 MPa are the sums of the amplitude contributions for steps 3–5 inclusive, and similarly at 140 MPa (steps 7–9) and 194 MPa (steps 11–15). Conversely, for
Figure 11f,h, to explore the timing behaviour during the main part of the plastic creep, only the values from those steps where the applied stress exceeded the previous maximum are shown; so the results from steps 4 and 5, 8 and 9, and 12–15 were excluded. The last step, where the string broke, was also excluded.
Looking first at the total cumulative amplitude plot (crosses) for string N7 in
Figure 11c, there was a clear inflection point at around 100 MPa, indicating some sort of transition in the stretching behaviour. Looking at the amplitude contributions from the series dashpot (stars) in
Figure 11a, there appeared to be a discontinuity, with the dashpot contribution rising for the first four steps, and then dropping back to start again from the fifth or sixth step. A corresponding discontinuity was present in
Figure 11e, with the fluidity of the dashpot generally increasing (speeding up) with the applied stress, but again restarting at the discontinuity.
This behaviour suggested that the fitted dashpot might in fact be modelling the early behaviour of two different stretching components (see
Section 3.4). Looking again at
Figure 11a, there was a minimum in the amplitude of the
coefficients (up-triangles) for the slower (or only) fitted Kelvin–Voigt stage at the same point as the discontinuity in the dashpot contributions. A similar transition could be seen in the
time coefficients (
Figure 11g), with these coefficients again generally increasing (speeding up) before dropping back at the discontinuity, after which there was no clear trend.
From these observations, it was hypothesised that the plastic creep consisted of three separate stretching components, indicated by the linked sequences shown in
Figure 11a: an initial component modelled by the Kelvin–Voigt stages for the first four steps, and continuing on to the faster of the two Kelvin–Voigt stages at step 5; a second component initially modelled by the series dashpot for the first four steps, and then being modelled by the slower (or only) Kelvin–Voigt stage from step 5 onwards; and a third component modelled by the series dashpot from around step 6 or 7.
The behaviour for string N28 was somewhat less clear than for string N7, especially in the timing behaviour, but a similar pattern could be discerned. In particular, the same discontinuity could be seen in the dashpot contributions, in the same stress range between the fourth and fifth points, and a similar minimum could be seen in the coefficient values at the fifth point, with a smooth progression from the dashpot amplitude contributions prior to that point.
The analysis for string N28 was complicated by the presence of many more steps where two Kelvin–Voigt stages were fitted. The most likely explanation for why this should be the case was simply the difference in the retuning and sampling intervals for the two strings: hourly for string N7 and every five minutes for string N28. Indeed, the primary reason for shortening the retuning period following the testing of string N7 was a concern that the hourly sampling rate was simply not fast enough to capture the faster aspects of the stretching behaviour. The apparent constraint of the plastic creep for some steps would also have reduced some of the amplitude contributions.
Looking at the cumulative amplitude plots for the selected sequences (
Figure 11c,d), it appeared that the three stretching components were similar for both strings, and could be characterised as three phases in the overall stretching history. During the first phase, which will be referred to as the initial ‘straightening’ phase, the cumulative amplitude of the fitted
coefficients, and hence the underlying compliance, increased with the applied stress in a diminishing manner to reach a limit somewhere in the region of 170–240 MPa. This could be modelled as a function of the applied stress using a single Kelvin–Voigt stage of amplitude:
where
were all constants. This function is zero for
, rising to a limit of
for
. The time coefficients during this phase could be modelled approximately as a linearly increasing function of the applied stress.
During the second stretching phase, there was virtually no contribution during the first two steps (with an applied stress of less than 65 MPa), after which the cumulative amplitude increased slowly at first before rising almost linearly with the applied stress from step 5 or 6 onwards (above about 160 MPa), essentially taking over from the initial straightening phase and giving rise to the inflection points in the total cumulative amplitude plots (crosses) in
Figure 11c,d. This could also be modelled as a function of the applied stress using a Kelvin–Voigt stage, this time of amplitude:
where
were also constants. This ‘ln-exp’ function is zero when
, rises slowly to
when
, and approaches a straight line with gradient
for
. Such an effect might be associated with a more-or-less constant compliance linked with a stress threshold. A third stretching phase, which appeared to be similar in character to the second and could be modelled in the same way, then came in at around step 7 (above about 200 MPa). The behaviour of the corresponding time coefficients for both these phases was less clear, but could be modelled approximately as constant values.
In formulating this three-phase model, there were at least three possible sources of error. The selection of which data to include in the selected sequences is perhaps the most obvious, along with the functions chosen to model the resulting cumulative amplitude responses. The choice of how many Kelvin–Voigt stages to use during the initial step-by-step modelling could also have an impact: adding or removing a Kelvin–Voigt stage affected the distribution of the strain amplitude across the remaining model components, including the series dashpot element. Overall, though, the results were plausible: the same model was derived for both strings, the transition points occurred in the same way and at the same stress levels, and the cumulative amplitude responses for the selected sequences varied smoothly with the applied stress.
Referring back to
Figure 5, string N28 stretched somewhat further than string N7 during the first few steps, while during the later steps, when not constrained, the responses of the two strings appeared fairly similar. From
Figure 11c,d this difference would correspond with differences in the extent of the stretching during the initial straightening phase. If the above analysis is correct, and assuming the other strings behaved in a similar way, then the corresponding straightening phase for each string would have been largely completed in response to the first applied stress step at about 200 MPa, with the observed differences in the residual offsets corresponding to intrinsic string differences during this initial straightening, as postulated earlier.
Figure 12a shows the results of fitting a model based on the above analysis to the first nine steps of the string N7 strain response, while
Figure 12b shows the results of fitting the same model to all 10 steps. The form of the model was again that given in Equations (
3) and (
4), with the variation in the elastic stretching component modelled using Equations (
10) and (
11). This time, however, since the model fitting started from the first step, the expression given in Equation (
6) could be used to model the response of the Kelvin–Voigt stages to the applied sequence of stress steps, and an offset was only needed to cover the initial residual strain offset
.
Two Kelvin–Voigt stages were again required, each covering elements of the plastic creep across a different part of the applied stress range. This was a rather different approach to the model described earlier for the recoverable creep, in which the two Kelvin–Voigt stages were active across the full range of applied stress levels, each covering quite different aspects of the stretching behaviour (the recoverable creep and some slower stretching component).
One Kelvin–Voigt stage was used to cover the initial straightening phase, with its amplitude
modelled as a function of the form shown in Equation (
12), and its time coefficient
allowed to vary linearly, increasing (speeding up) with the applied stress. The second Kelvin–Voigt stage was used to cover both the second and third stretching components shown in
Figure 11c,d (while not shown in those plots, the cumulative sum of these two stretching components had a very similar form to that of the second stretching component on its own). Its amplitude was a function of the form shown in Equation (
13), and it was modelled as having a constant time coefficient.
It was found that good fits could be obtained without including the series dashpot. This was encouraging since experience with these types of instrument strings had shown that they always eventually stopped creeping, at least until they got close to their breaking points [
6].
The solid black and dashed red lines in
Figure 12 show the measured and fitted strain responses, while the dotted black and red lines show the contributions from the separate Kelvin–Voigt stages. Both fits are very good, but the fit in
Figure 12a is slightly better over the later steps (steps 7–9). The contributions from the two Kelvin–Voigt stages are also different in the two plots, with the results shown in
Figure 12a being a closer match to the behaviour expected from the examination of the step-by-step model coefficients (
Figure 11c). It seems likely that the string stretching during the last step was accelerating towards breaking, distorting the fit over the later steps in
Figure 12b.
It became clear that the fitting error surface had multiple local minima: the results obtained were sensitive to the starting point used by the fitting function. Indeed, in order to find these fits, two forms of the model had to be used initially. First, a model with fixed coefficients for the main stretching Kelvin–Voigt stage, derived from the step-by-step fitting results, was used to obtain a fit for the initial straightening phase, fitting to just the first few steps of the string N7 strain response. Then, these coefficients were fixed, and an improved fit was found for the main stretching Kelvin–Voigt stage. This process was iterated until a fit could be obtained for the full strain response, and then fine-tuned by allowing both sets of coefficients to vary.
As indicated earlier, no claim can be made regarding the physical accuracy of the amplitude expressions given in Equations (
12) and (
13) except that they gave a reasonably good match to the behaviour found in the step-by-step fitting results. Consequently, while the model obtained seems qualitatively plausible, it may not reflect the underlying physics correctly.
Attempts to fit a model to the measured strain response from string N28 were made more complicated by the apparent reduction in some of the step responses due to some form of constraint mechanism, and the presence of the recoverable creep episodes, which, as noted above, varied in amplitude in a more complex manner than seen with the other strings.
To explore the plastic creep constraint effect, a version of the string N28 strain response was created with the recoverable creep steps removed in a similar way to the removal of the enhanced-heating step from the string N21 response (
Section 4.2.2). A model similar to that used for string N7 was fitted to this modified response, with the result shown in
Figure 13a. The amplitude of the main stretching phase
was scaled by an additional creep constraint factor
. This was initially modelled as a linear function of the time since the last overall increase in the applied stress, subject to a time threshold and constrained to the range 0–1:
where
was the start time of the last step with an applied stress in excess of the previous maximum, and
and
were constants. Each time the constraint factor was applied, the amplitudes of the subsequent steps had to be adjusted down accordingly, so that the amplitude at each step
n was calculated as:
It was discovered, however, that this constraint factor was actually only required following the last episode of reduced stress, for step 16; it appeared that the strain response during step 10, following the applied stress reduction during step 8, had not in fact been constrained. Similarly, the response during step 17 did not appear to have been constrained. The model was therefore simplified to take a single value of
to use with just step 16. When the constraint mechanism was required (for step 16), it was quite significant with
(for the fit shown in
Figure 13b), indicating fairly strongly that this form of constraint was indeed not required for the other steps.
The best fitting results were obtained when both the initial straightening and the main stretching phases were allowed to continue running during those periods where the applied stress had been reduced (modelled as if the applied stress was unchanged). This appeared to be the approach most consistent with the observed behaviour, described above in
Section 4.1.
To model the full strain response for string N28, the model developed so far was supplemented by a third Kelvin–Voigt stage to model the recoverable creep episodes. This took the same approach as used earlier for the multi-step fits shown in
Figure 9, using the expression of Equation (
7) with
and
restarted from the start of each recoverable creep episode. In accordance with the earlier observations about the variable size of these recoverable creep episodes, three separate model coefficients were used for the amplitude values
, while a single common value was used for the time coefficient
.
Figure 13b shows the fit obtained with this combined model. The fit is not quite as precise as for some of the earlier results, but is still very good. As before, the solid black and dashed red lines show the measured and fitted strain responses, while the dotted black and red lines show the contributions from the Kelvin–Voigt stages modelling the initial ‘straightening’ and main stretching phases. The dotted blue line shows the fitted result for the recoverable creep components. As expected by this point, these had progressively falling amplitudes, with fitted coefficients
of 0.16, 0.14, and 0.06/GPa.
The contribution from the Kelvin–Voigt stage modelling the initial straightening phase (dotted black lines) was very similar in
Figure 13a,b. The contribution from the Kelvin–Voigt stage modelling the main stretching phase (dotted red lines), however, was somewhat larger in
Figure 13b than in
Figure 13a. Whether this was due to some interaction with the fit for the recoverable creep components, or a consequence of the way the recoverable creep components were removed from the modified strain response shown in
Figure 13a, or some combination of these factors, is not known. In both
Figure 13a,b, this Kelvin–Voigt stage also came in with a much stronger contribution almost from the start of the response than for that fitted for string N7. To some extent, this was expected from the analysis of the single-step fitting results, but not to the extent seen here. Why this should be the case is not known, but it may simply be a feature of the particular fitting solution obtained.
While the usefulness of the final model was reduced somewhat by the need to more-or-less tailor fit the recoverable creep components and the plastic creep constraint function, it nevertheless helped to provide further insights into these elements of the string behaviour.