Predicting Strut Geometry of PCL and DMSO2 Biocomposites from Nozzle to Deposition in Bio-Scaffold 3D Printing
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
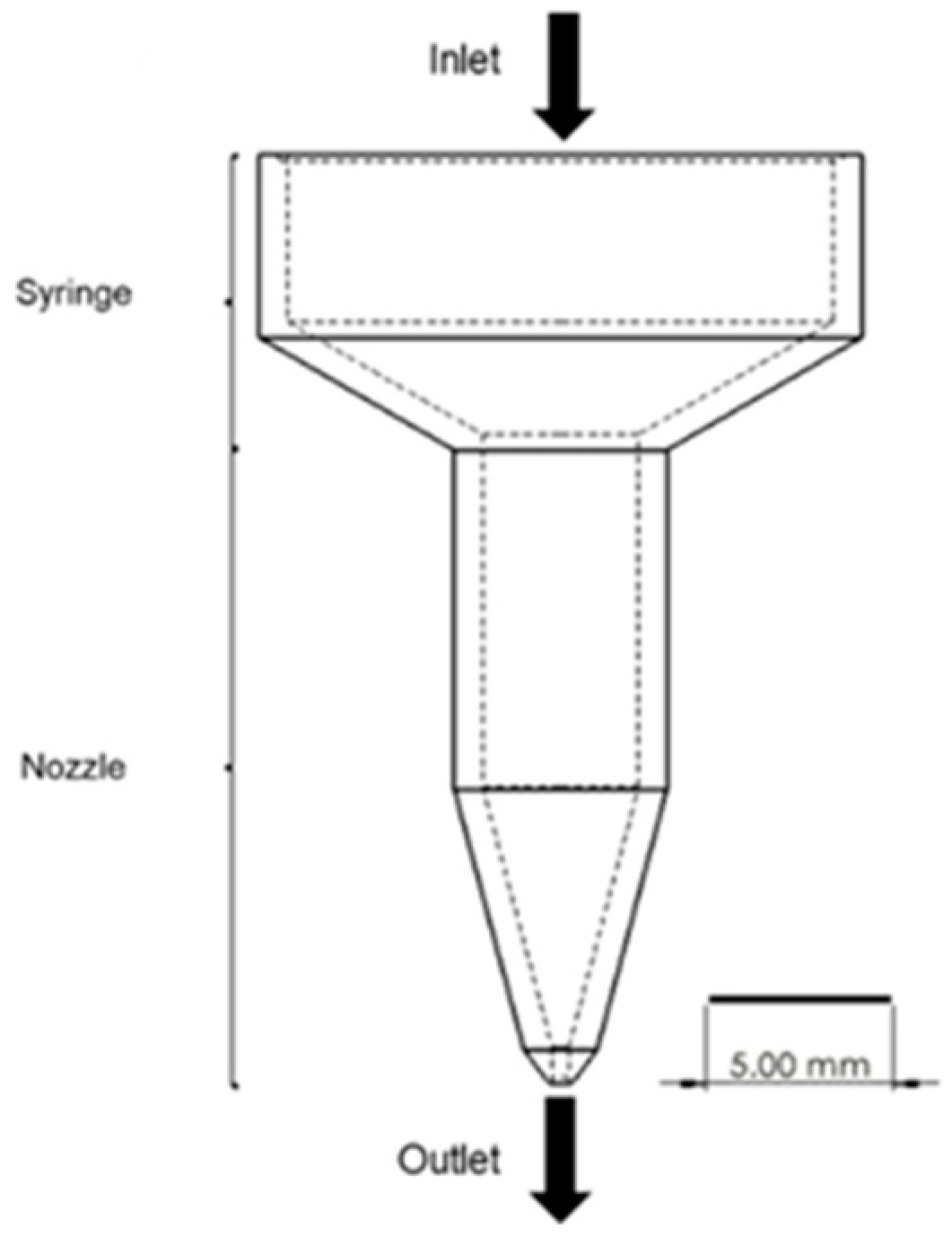
2.2. Printing Equipment and Process
2.3. Modeling
2.3.1. Viscosity Model
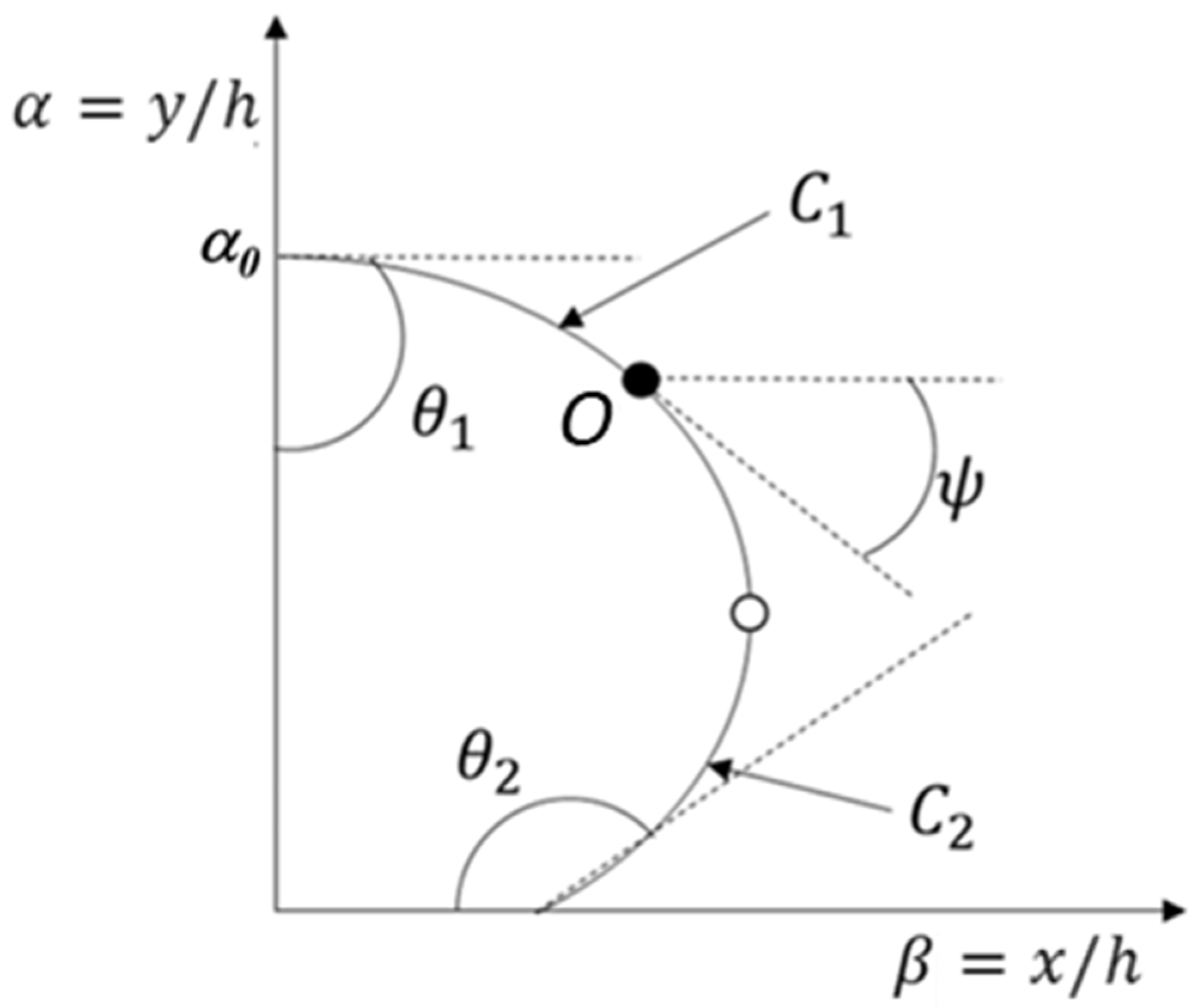
2.3.2. Stacking Model
3. Results and Discussion
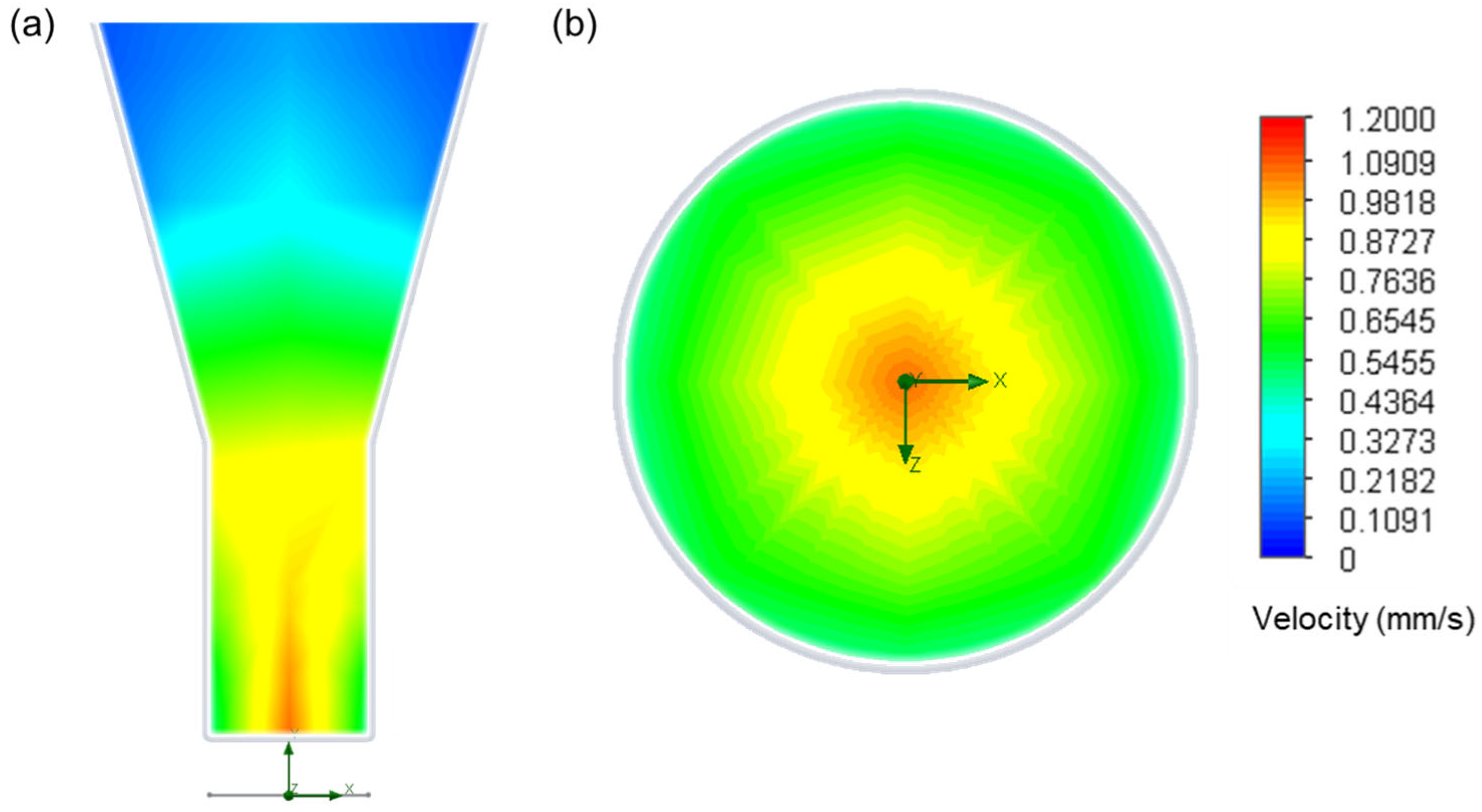
3.1. Numerical Analysis for Extrusion Velocity
3.2. Numerical Analysis for Strut Diameter
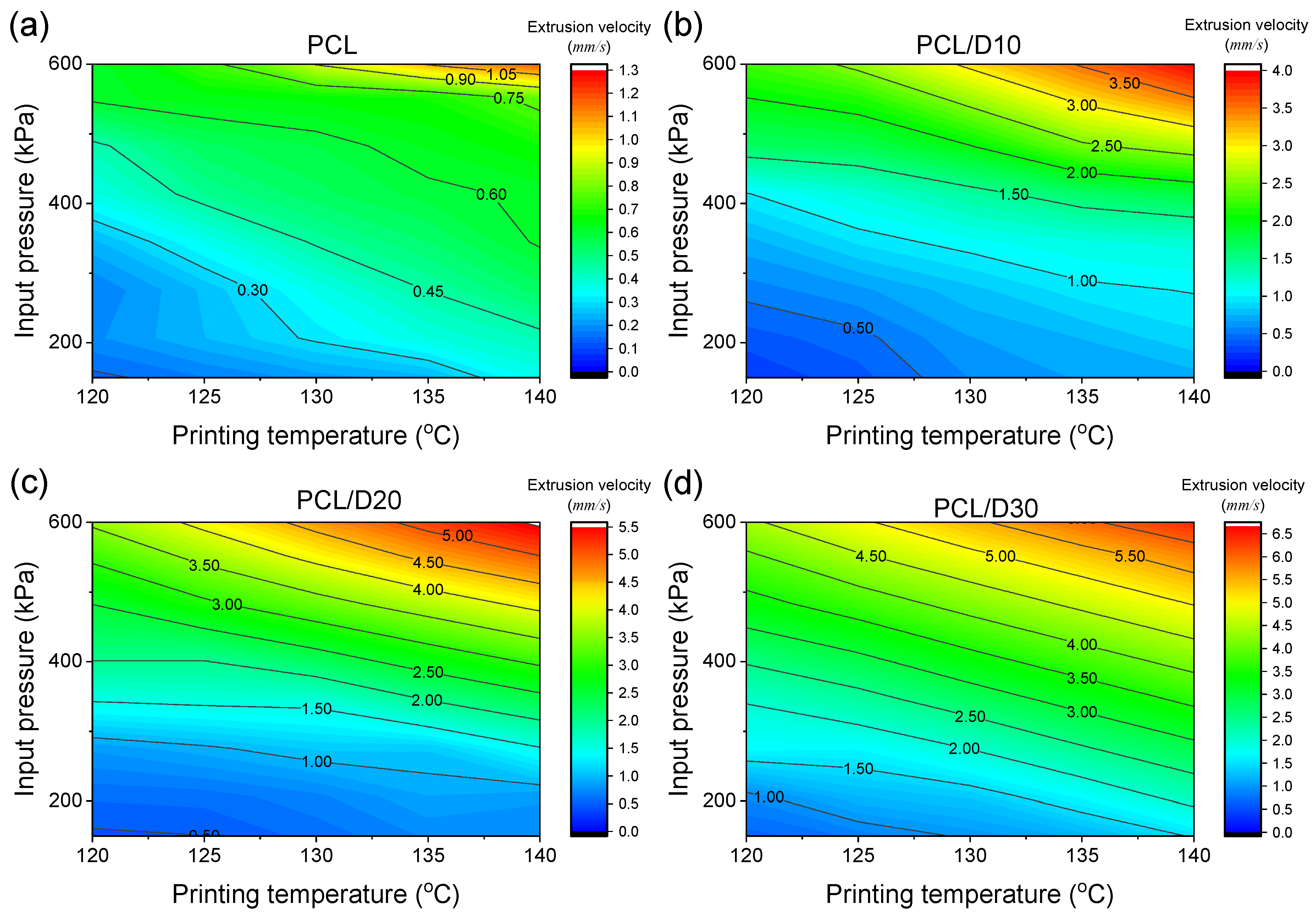
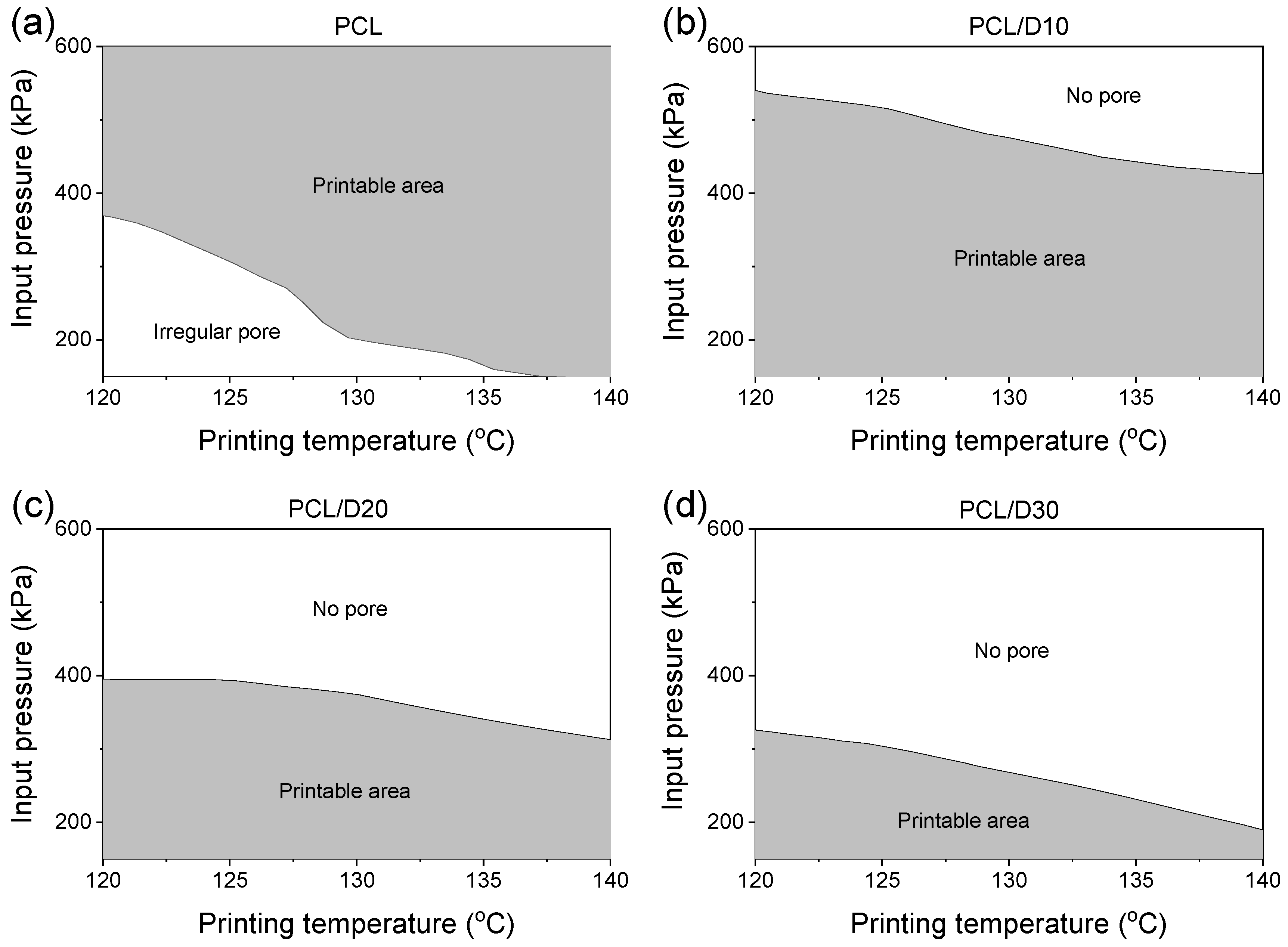
3.3. Printing Condition Effect on the Extrusion Velocity and Strut Diameter
3.4. Material Property Effect on the Extrusion Velocity and Strut Diameter
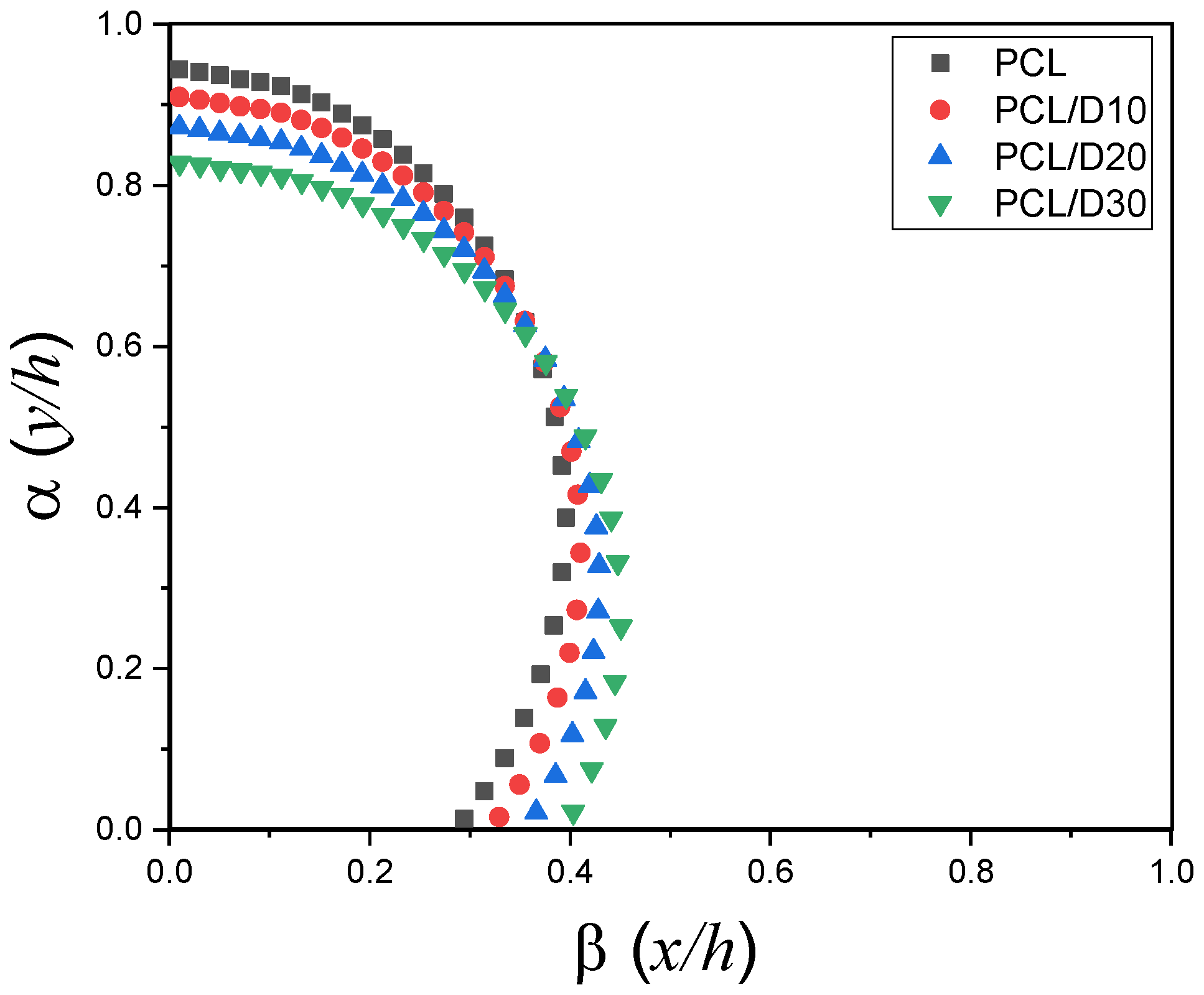
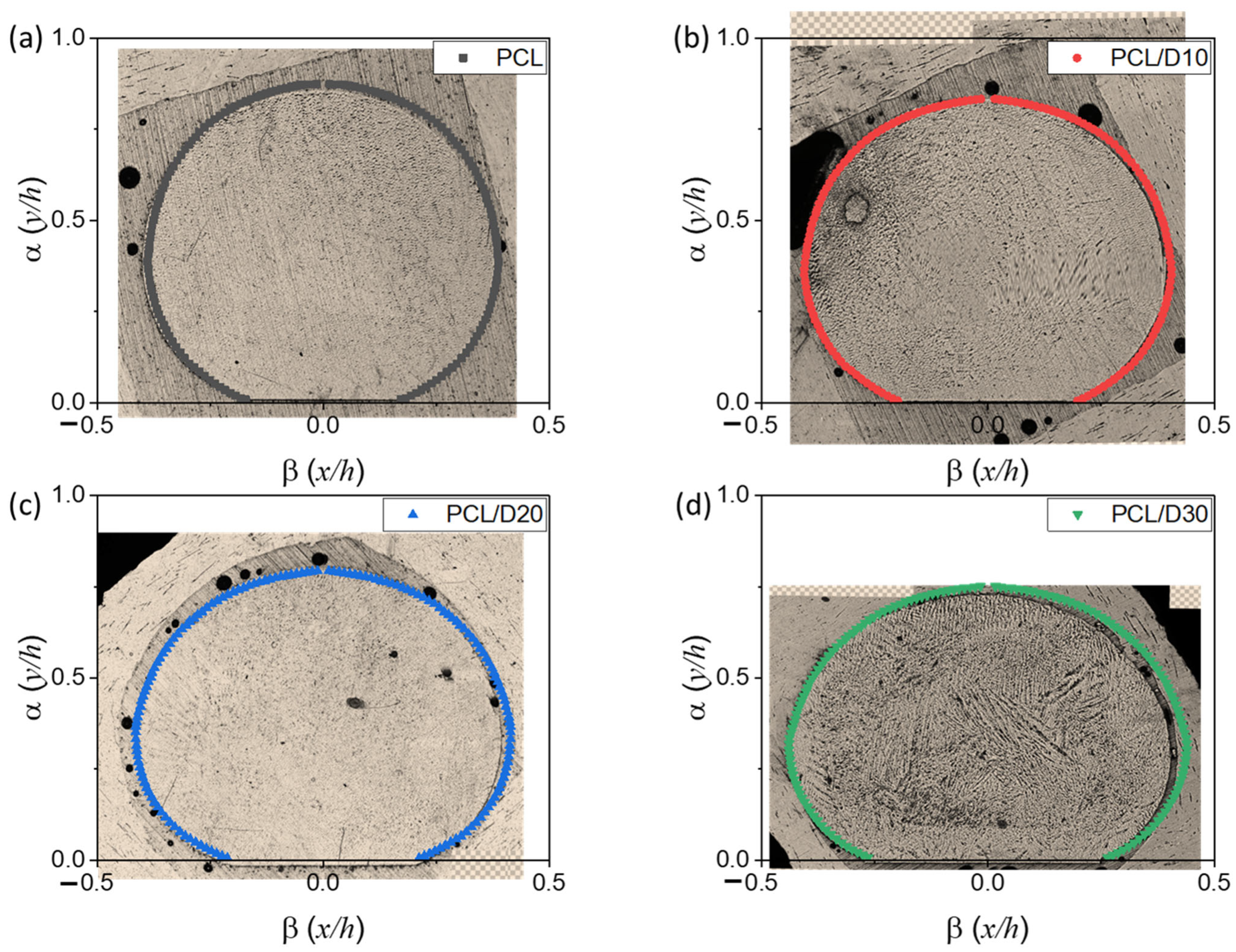
3.5. 3D Scaffold Fabrication Under Optimal Printing Conditionsr
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Frantz, C.; Stewart, K.M.; Weaver, V.M. The extracellular matrix at a glance. J. Cell Sci. 2010, 123, 4195–4200. [Google Scholar] [CrossRef]
- Hollister, S.J. Porous scaffold design for tissue engineering. Nat. Mater. 2005, 4, 518–524. [Google Scholar] [CrossRef] [PubMed]
- Jang, J.W.; Min, K.E.; Kim, C.; Shin, J.; Lee, J.; Yi, S. Scaffold Characteristics, Fabrication Methods, and Biomaterials for the Bone Tissue Engineering. Int. J. Precis. Eng. Manuf. 2023, 24, 511–529. [Google Scholar] [CrossRef]
- Marin, E.; Boschetto, F.; Pezzotti, G. Biomaterials and biocompatibility: An historical overview. J. Biomed. Mater. Res. Part A 2020, 108, 1617–1633. [Google Scholar] [CrossRef] [PubMed]
- Mitragotri, S.; Lahann, J. Physical approaches to biomaterial design. Nat. Mater. 2009, 8, 15–23. [Google Scholar] [CrossRef]
- Nair, L.S.; Laurencin, C.T. Biodegradable polymers as biomaterials. Prog. Polym. Sci. 2007, 32, 762–798. [Google Scholar] [CrossRef]
- Backes, E.H.; Harb, S.V.; Beatrice, C.A.G.; Shimomura, K.M.B.; Passador, F.R.; Costa, L.C.; Pessan, L.A. Polycaprolactone usage in additive manufacturing strategies for tissue engineering applications: A review. J. Biomed. Mater. Res. Part B Appl. Biomater. 2022, 110, 1479–1503. [Google Scholar] [CrossRef]
- Zhu, C.; Gemeda, H.B.; Duoss, E.B.; Spadaccini, C.M. Toward multiscale, multimaterial 3D printing. Adv. Mater. 2024, 36, 2314204. [Google Scholar] [CrossRef]
- Duty, C.; Ajinjeru, C.; Kishore, V.; Compton, B.; Hmeidat, N.; Chen, X.; Liu, P.; Hassen, A.A.; Lindahl, J.; Kunc, V. What makes a material printable? A viscoelastic model for extrusion-based 3D printing of polymers. J. Manuf. Process. 2018, 35, 526–537. [Google Scholar] [CrossRef]
- Min, K.-E.; Jang, J.-W.; Shin, J.; Kim, C.; Yi, S. Development of Prediction Method for Dimensional Stability of 3D-Printed Objects. Appl. Sci. 2023, 13, 11027. [Google Scholar] [CrossRef]
- Pennings, S.; Liu, K.J.; Qian, H. The stem cell niche: Interactions between stem cells and their environment. Stem Cells Int. 2018, 2018, 4879379. [Google Scholar] [CrossRef]
- Donnaloja, F.; Jacchetti, E.; Soncini, M.; Raimondi, M.T. Natural and synthetic polymers for bone scaffolds optimization. Polymers 2020, 12, 905. [Google Scholar] [CrossRef]
- Ganguly, S.; Tang, X.S. 3D Printing of High Strength Thermally Stable Sustainable Lightweight Corrosion-Resistant Nanocomposite by Solvent Exchange Postprocessing. ACS Sustain. Chem. Engineering. 2024, 13, 423–435. [Google Scholar] [CrossRef]
- Siddiqui, N.; Asawa, S.; Birru, B.; Baadhe, R.; Rao, S. PCL-Based Composite Scaffold Matrices for Tissue Engineering Applications. Mol. Biotechnol. 2018, 60, 506–532. [Google Scholar] [CrossRef] [PubMed]
- Patrício, T.; Domingos, M.; Gloria, A.; D’Amora, U.; Coelho, J.F.; Bartolo, P.J. Fabrication and characterisation of PCL and PCL/PLA scaffolds for tissue engineering. Rapid Prototyp. J. 2014, 20, 145–156. [Google Scholar] [CrossRef]
- Agrawal, G.; Negi, Y.S.; Pradhan, S.; Dash, M.; Samal, Y.S. Wettability and contact angle of polymeric biomaterials. In Characterization of Polymeric Biomaterials; Elsevier: Amsterdam, The Netherlands, 2017; pp. 57–81. [Google Scholar]
- Udofia, E.N.; Zhou, W. Microextrusion based 3D printing—A review. In 2018 International Solid Freeform Fabrication Symposium; University of Texas at Austin: Austin, TX, USA, 2018. [Google Scholar]
- Gautam, S.; Sharma, C.; Purohit, S.D.; Singh, H.; Dinda, A.K.; Potdar, P.D.; Chou, C.-F.; Mishra, N.C. Gelatin-polycaprolactone-nanohydroxyapatite electrospun nanocomposite scaffold for bone tissue engineering. Mater. Sci. Eng. C 2021, 119, 111588. [Google Scholar] [CrossRef] [PubMed]
- Murugan, S.; Parcha, S.R. Fabrication techniques involved in developing the composite scaffolds PCL/HA nanoparticles for bone tissue engineering applications. J. Mater. Sci. Mater. Med. 2021, 32, 93. [Google Scholar] [CrossRef]
- Petretta, M.; Gambardella, A.; Desando, G.; Cavallo, C.; Bartolotti, I.; Shelyakova, T.; Goranov, V.; Brucale, M.; Dediu, V.A.; Fini, M.; et al. Multifunctional 3D-printed magnetic polycaprolactone/hydroxyapatite scaffolds for bone tissue engineering. Polymers 2021, 13, 3825. [Google Scholar] [CrossRef]
- Wang, F.; Tankus, E.B.; Santarella, F.; Rohr, N.; Sharma, N.; Märtin, S.; Michalscheck, M.; Maintz, M.; Cao, S.; Thieringer, F.M. Fabrication and characterization of PCL/HA filament as a 3D printing material using thermal extrusion technology for bone tissue engineering. Polymers 2022, 14, 669. [Google Scholar] [CrossRef]
- Jang, J.-W.; Min, K.-E.; Kim, C.; Wern, C.; Yi, S. PCL and DMSO2 Composites for Bio-Scaffold Materials. Materials 2023, 16, 2481. [Google Scholar] [CrossRef]
- Min, K.-E.; Jang, J.-W.; Yi, S.; Kim, C. Role of binder on yield strength of polycaprolactone/dimethylsulfone composites for bio-applications. J. Mater. Res. Technol. 2023, 27, 462–471. [Google Scholar] [CrossRef]
- Kyle, S.; Jessop, Z.M.; Al-Sabah, A.; Whitaker, I.S. ‘Printability’of candidate biomaterials for extrusion based 3D printing: State-of-the-art. Adv. Healthc. Mater. 2017, 6, 1700264. [Google Scholar] [CrossRef] [PubMed]
- Ouyang, L.; Yao, R.; Zhao, Y.; Sun, W. Effect of bioink properties on printability and cell viability for 3D bioplotting of embryonic stem cells. Biofabrication 2016, 8, 035020. [Google Scholar] [CrossRef] [PubMed]
- He, Y.; Yang, F.; Zhao, H.; Gao, Q.; Xia, B.; Fu, J. Research on the printability of hydrogels in 3D bioprinting. Sci. Rep. 2016, 6, 29977. [Google Scholar] [CrossRef]
- Zidan, A.; Alayoubi, A.; Coburn, J.; Asfari, S.; Ghammraoui, B.; Cruz, C.N.; Ashraf, M. Extrudability analysis of drug loaded pastes for 3D printing of modified release tablets. Int. J. Pharm. 2019, 554, 292–301. [Google Scholar] [CrossRef]
- Comminal, R.; Jafarzadeh, S.; Serdeczny, M.; Spangenberg, J. Estimations of Interlayer Contacts in Extrusion Additive Manufacturing Using a CFD Model. In International Conference on Additive Manufacturing in Products and Applications; Springer: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Yu, M.; Yeow, Y.J.; Lawrence, L.; Claudio, P.P.; Day, J.B.; Salary, R. Characterization of the Functional Properties of Polycaprolactone Bone Scaffolds Fabricated Using Pneumatic Micro-Extrusion. J. Micro-Nano-Manuf. 2021, 9, 030905. [Google Scholar] [CrossRef]
- Min, K.-E.; Jang, J.-W.; Kim, J.-K.; Yi, S.; Kim, C. Prediction of Curing Time/Shear Strength of Non-Conductive Adhesives Using a Neural Network Model. Appl. Sci. 2022, 12, 12150. [Google Scholar] [CrossRef]
- Min, K.-E.; Jang, J.-W.; Kang, S.; Kim, C.; Yi, S. Identification of Solder Joint Failure Modes Using Machine Learning. IEEE Trans. Compon. Packag. Manuf. Technol. 2023, 13, 2032–2039. [Google Scholar] [CrossRef]
- Serdeczny, M.P.; Comminal, R.; Mollah, M.T.; Pedersen, D.B.; Spangenberg, J. Numerical modeling of the polymer flow through the hot-end in filament-based material extrusion additive manufacturing. Addit. Manuf. 2020, 36, 101454. [Google Scholar] [CrossRef]
- Gosset, A.; Barreiro-Villaverde, D.; Permuy, J.C.B.; Lema, M.; Ares-Pernas, A.; López, M.J.A. Experimental and numerical investigation of the extrusion and deposition process of a poly (lactic acid) strand with fused deposition modeling. Polymers 2020, 12, 2885. [Google Scholar] [CrossRef]
- Lee, J.; Walker, J.; Natarajan, S.; Yi, S. Prediction of geometric characteristics in polycaprolactone (PCL) scaffolds produced by extrusion-based additive manufacturing technique for tissue engineering. Rapid Prototyp. J. 2019, 26, 238–248. [Google Scholar] [CrossRef]
- Maples, R.E. Petroleum Refinery Process Economics; PennWell Corporation: Tulsa, OK, USA, 2000. [Google Scholar]
- Heinrich, S.M.; Liedtke, P.E.; Nigro, N.J.; Elkouh, A.F.; Lee, P.S. Effect of chip and pad geometry on solder joint formation in SMT. J. Electron. Packag. 1993, 115, 433–439. [Google Scholar] [CrossRef]
- Jang, J.-W.; Min, K.-E.; Kim, C.; Wern, C.; Yi, S. Rheological Properties and 3D Printing Behavior of PCL and DMSO2 Composites for Bio-Scaffold. Materials 2024, 17, 2459. [Google Scholar] [CrossRef] [PubMed]
- Ramlan, I.B.; Darlis, N.B. Comparison between solidworks and ansys flow simulation on aerodynamic studies. J. Ind. Eng. Innov. 2019, 1, 1–10. [Google Scholar]
- Kirkpatrick, M.P.; Armfield, S.W.; Kent, J.H. A representation of curved boundaries for the solution of the Navier–Stokes equations on a staggered three-dimensional Cartesian grid. J. Comput. Physics. 2003, 184, 1–36. [Google Scholar] [CrossRef]
- Fang, Q.; Hanna, M.A. Rheological properties of amorphous and semicrystalline polylactic acid polymers. Ind. Crops Prod. 1999, 10, 47–53. [Google Scholar] [CrossRef]
- Lee, S.; Kareko, L.; Jun, J. Study of thermoplastic PLA foam extrusion. J. Cell. Plast. 2008, 44, 293–305. [Google Scholar] [CrossRef]
- Yousefzade, O.; Jeddi, J.; Vazirinasab, E.; Garmabi, H. Poly (lactic acid) phase transitions in the presence of nano calcium carbonate: Opposing effect of nanofiller on static and dynamic measurements. J. Thermoplast. Compos. Mater. 2019, 32, 312–327. [Google Scholar] [CrossRef]





| Material | Appearance | Molecular Weight (g/mol) | Density (kg/m3) | Viscosity @120 °C (Pa∙s) | CTE * (10−6/°C) | Surface Tension (N/m) |
|---|---|---|---|---|---|---|
| PCL | Powder | 50,000 | 1145 | 2211.35 | 165 | 0.040 |
| DMSO2 | Powder | 94.13 | 1450 | 0.00114 | 88 | 0.060 |
| Temp. (°C) | PCL | PCL/D10 | PCL/D20 | PCL/D30 |
|---|---|---|---|---|
| 120 | 1286.6 | 863.0 | 696.9 | 333.8 |
| 125 | 1094.4 | 816.3 | 662.1 | 316.6 |
| 130 | 1018.9 | 762.7 | 622.2 | 286.5 |
| 135 | 946.0 | 749.7 | 577.1 | 251.1 |
| 140 | 889.5 | 720.1 | 526.9 | 229.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jang, J.-W.; Min, K.-E.; Park, J.-H.; Kim, C.; Yi, S. Predicting Strut Geometry of PCL and DMSO2 Biocomposites from Nozzle to Deposition in Bio-Scaffold 3D Printing. Materials 2025, 18, 2380. https://doi.org/10.3390/ma18102380
Jang J-W, Min K-E, Park J-H, Kim C, Yi S. Predicting Strut Geometry of PCL and DMSO2 Biocomposites from Nozzle to Deposition in Bio-Scaffold 3D Printing. Materials. 2025; 18(10):2380. https://doi.org/10.3390/ma18102380
Chicago/Turabian StyleJang, Jae-Won, Kyung-Eun Min, Jun-Hee Park, Cheolhee Kim, and Sung Yi. 2025. "Predicting Strut Geometry of PCL and DMSO2 Biocomposites from Nozzle to Deposition in Bio-Scaffold 3D Printing" Materials 18, no. 10: 2380. https://doi.org/10.3390/ma18102380
APA StyleJang, J.-W., Min, K.-E., Park, J.-H., Kim, C., & Yi, S. (2025). Predicting Strut Geometry of PCL and DMSO2 Biocomposites from Nozzle to Deposition in Bio-Scaffold 3D Printing. Materials, 18(10), 2380. https://doi.org/10.3390/ma18102380








