Exploiting the Modulation Effects of Epitaxial Vanadium Film in a Quasi-BIC-Based Terahertz Metamaterial
Abstract
1. Introduction
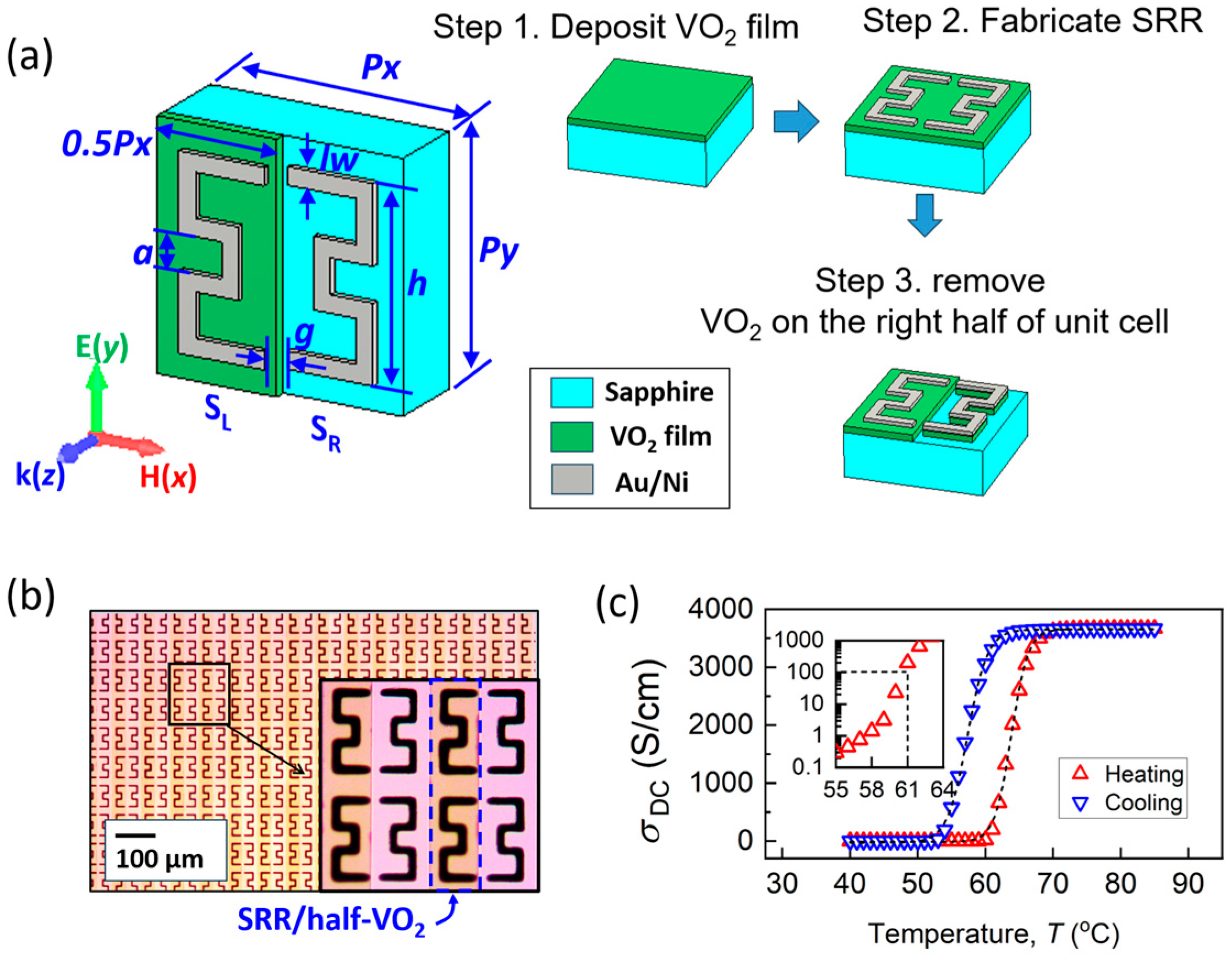
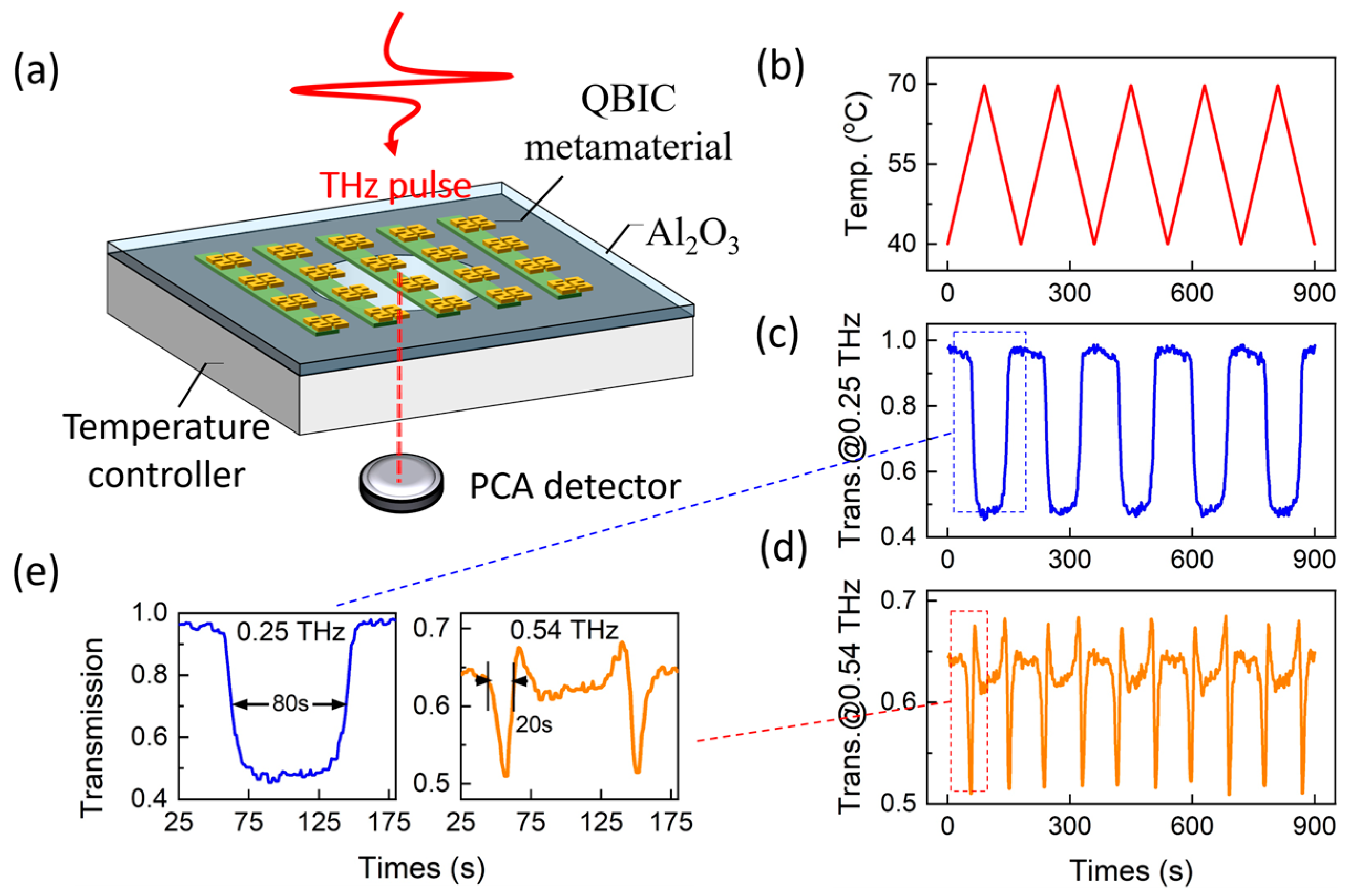
2. Materials and Methods
3. Results
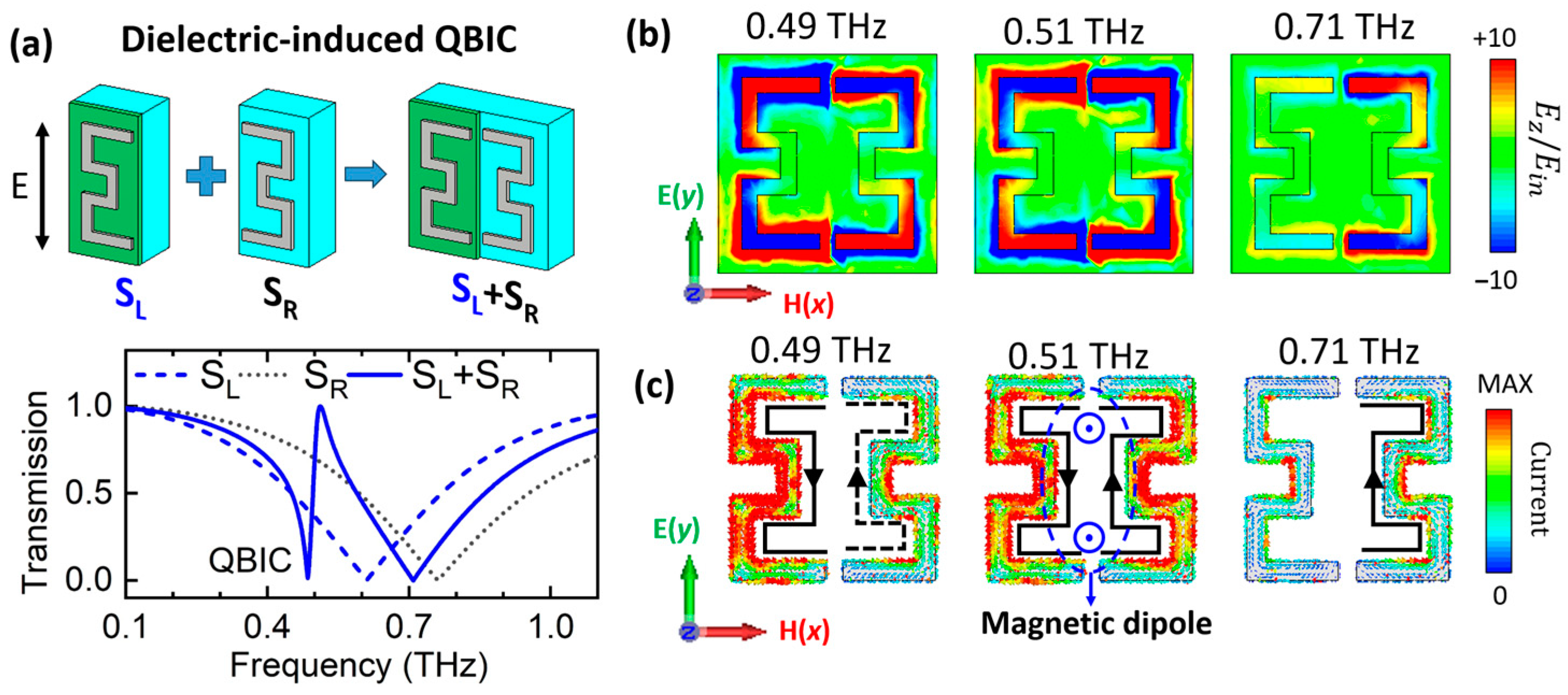
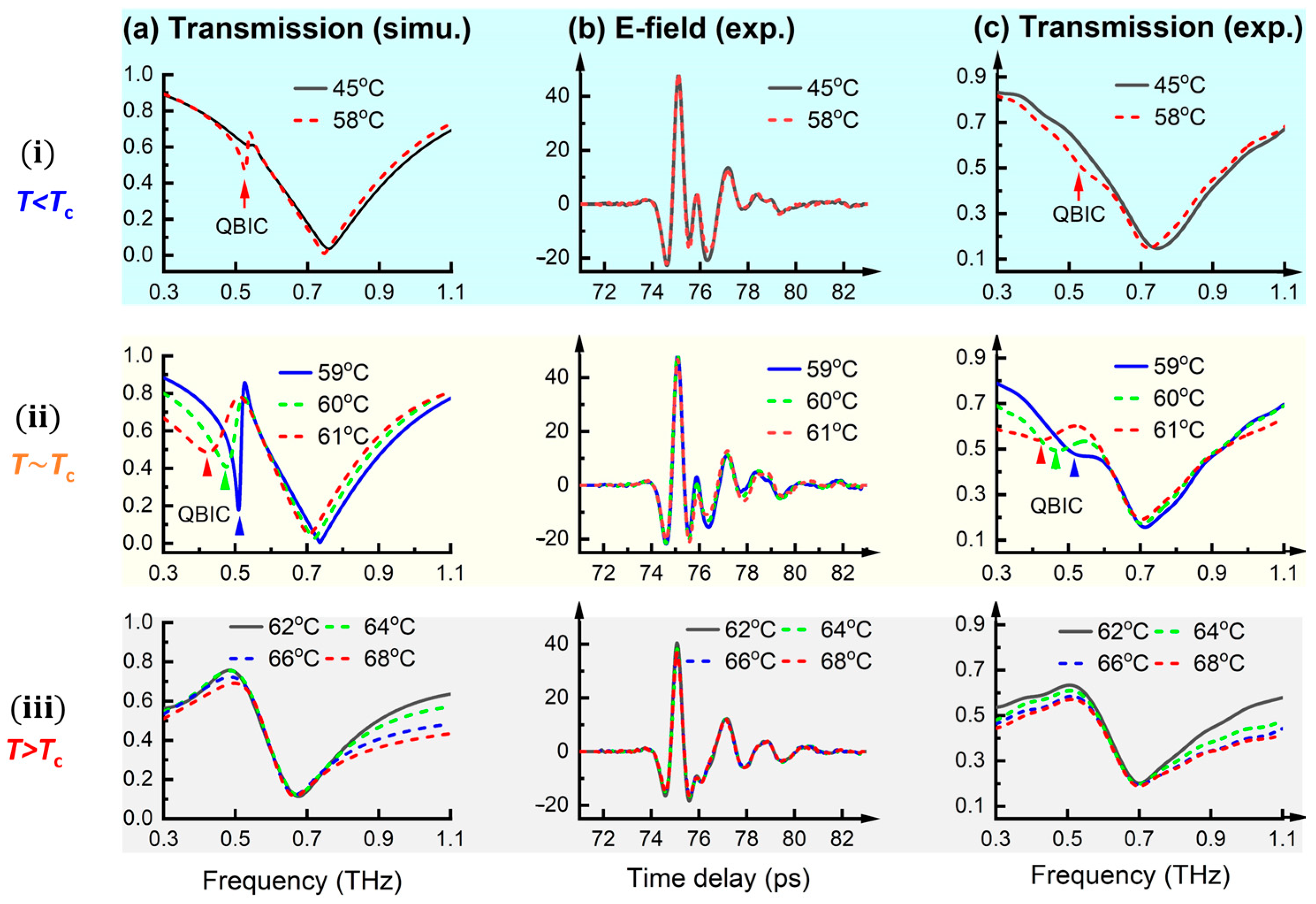
3.1. Mechanisms for QBIC
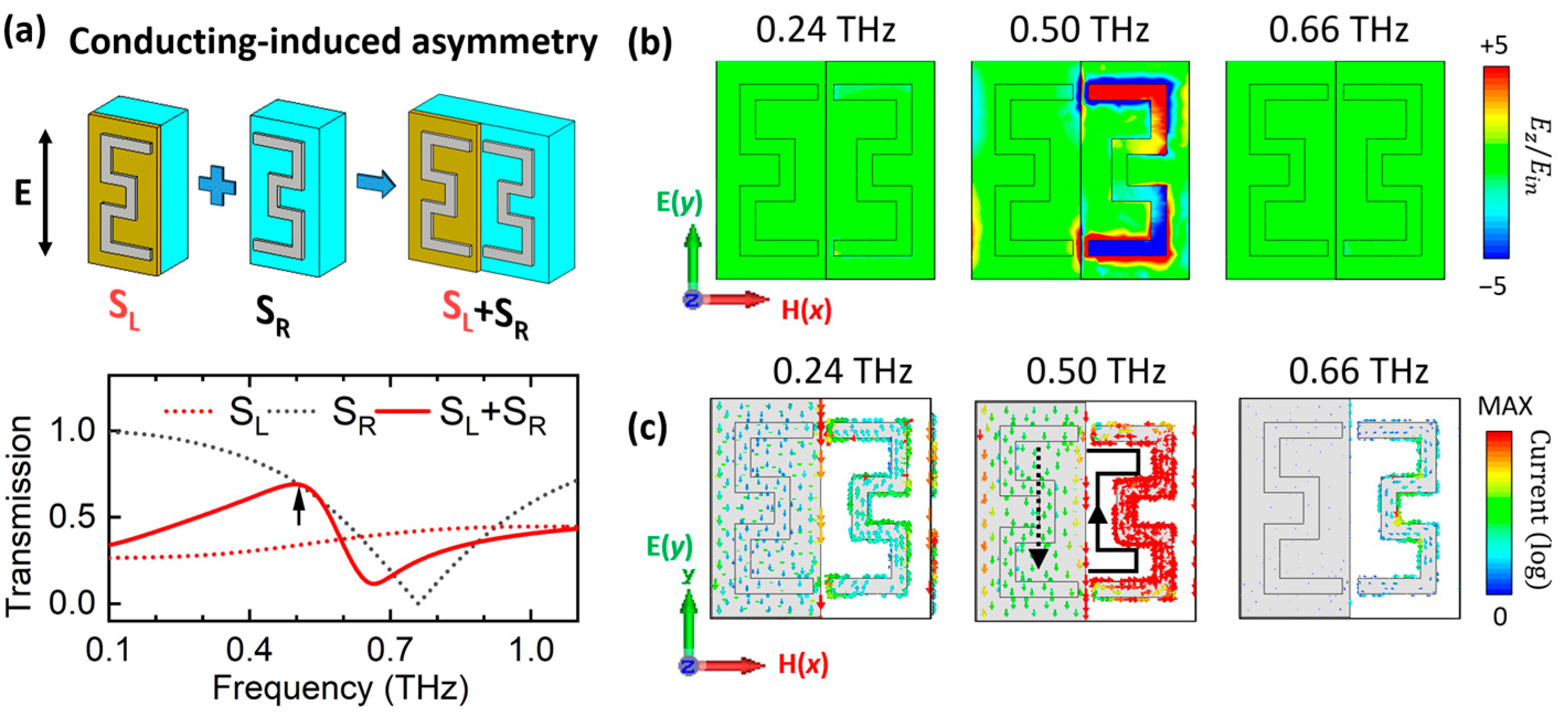
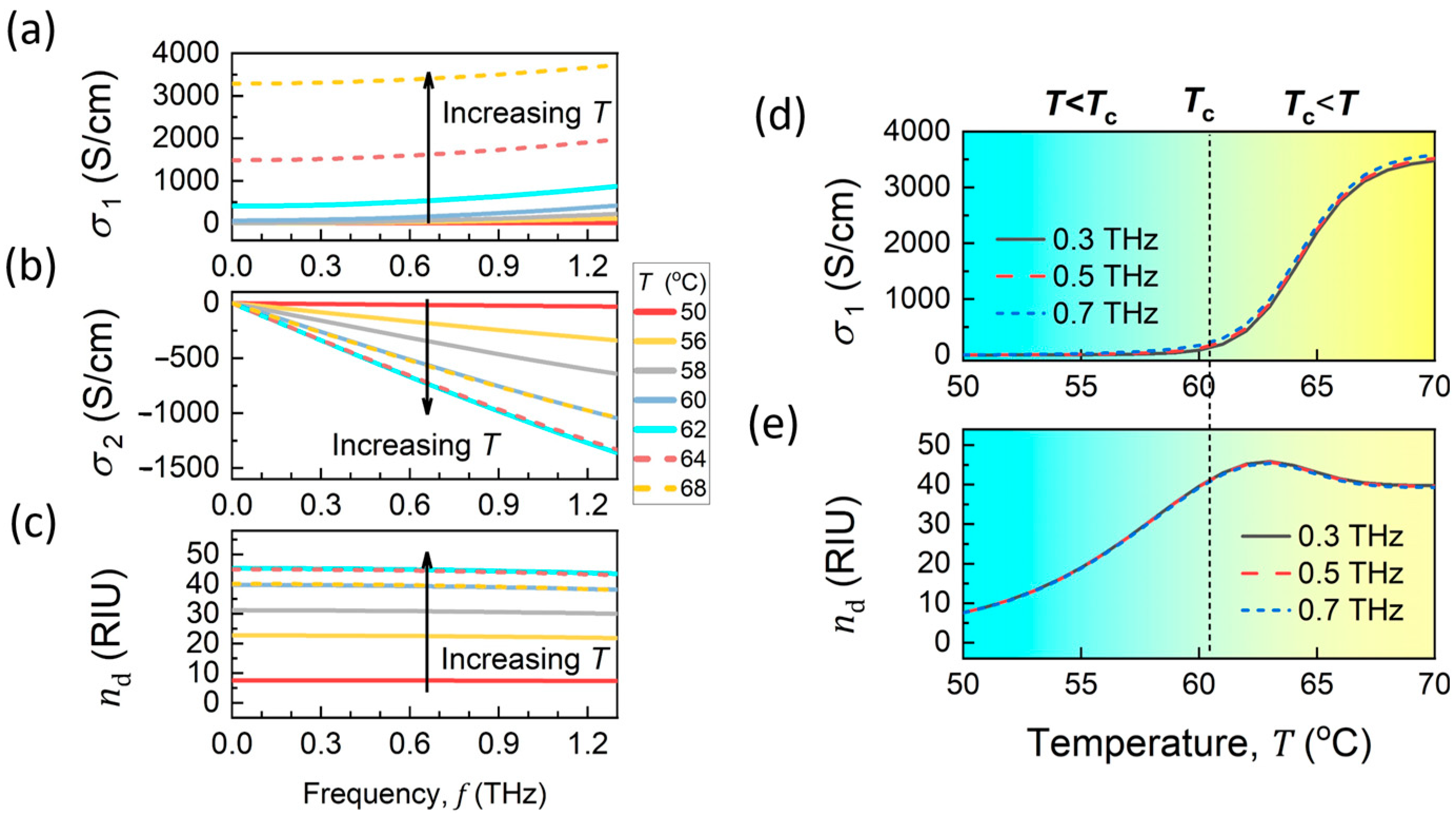
3.2. Cooperative Permittivity–Conductivity-Induced Modulation Effects
3.3. THz Properties of VO2 Film
3.4. Experimental Spectra
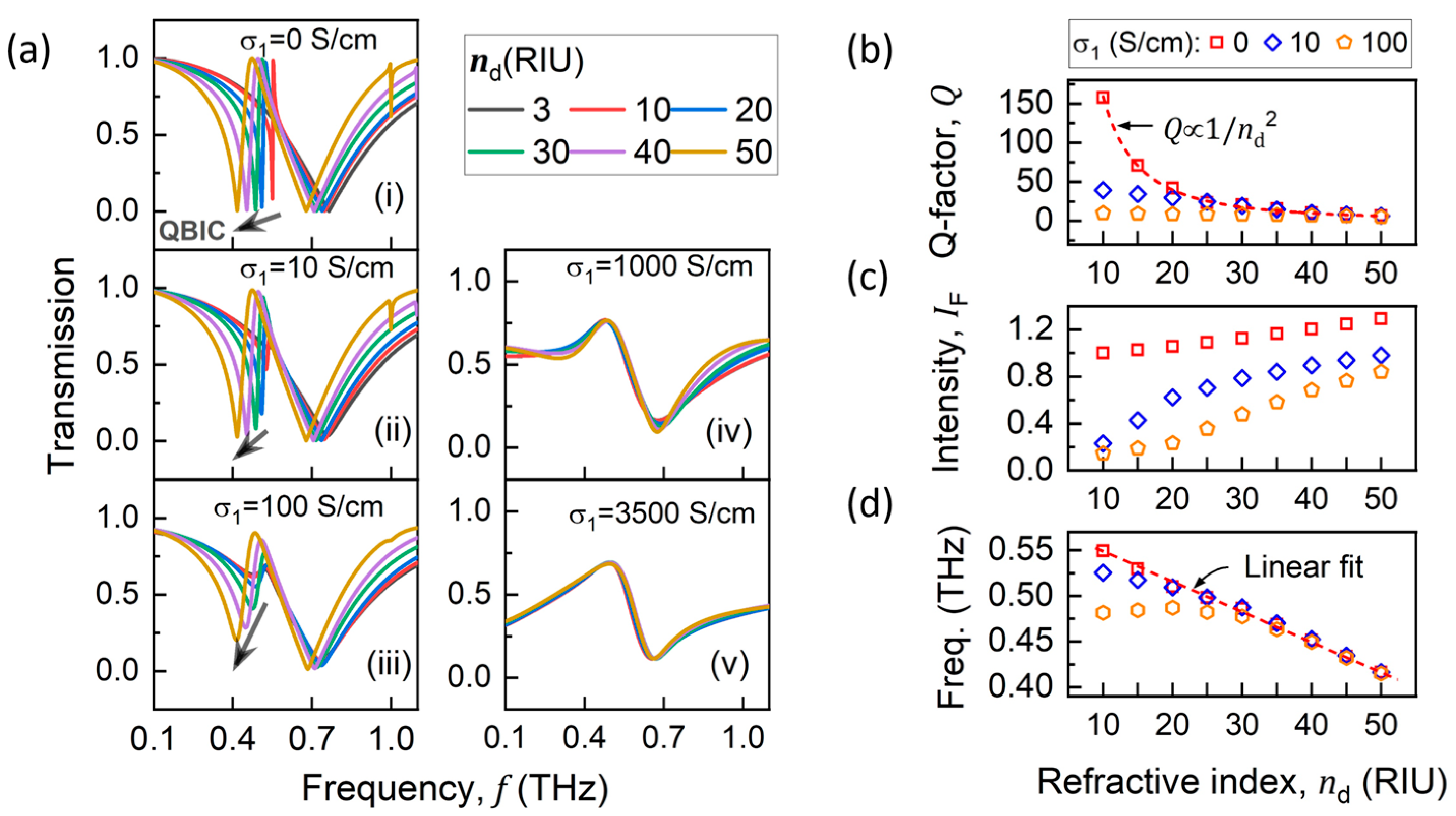
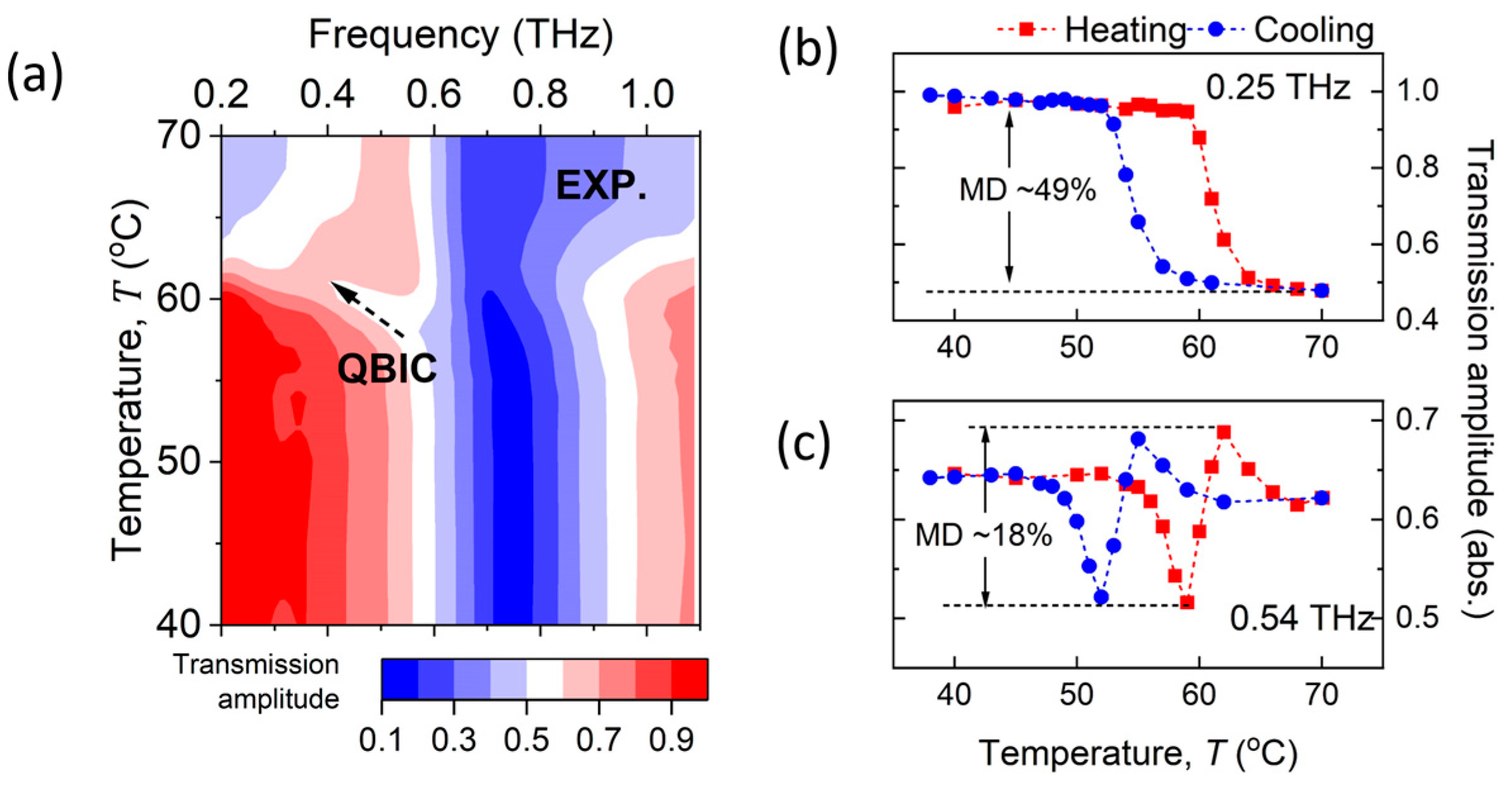
3.5. Frequency-Dependent Modulation Effects
3.6. PCMs with Permittivity Tunability
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Akyildiz, I.F.; Han, C.; Hu, Z.; Nie, S.; Jornet, J.M. Terahertz Band Communication: An Old Problem Revisited and Research Directions for the Next Decade. IEEE Trans. Commun. 2022, 70, 4250–4285. [Google Scholar] [CrossRef]
- Akyildiz, I.F.; Jornet, J.M.; Han, C. Terahertz Band: Next Frontier for Wireless Communications. Phys. Commun. 2014, 12, 16–32. [Google Scholar] [CrossRef]
- Elayan, H.; Amin, O.; Shihada, B.; Shubair, R.M.; Alouini, M.S. Terahertz Band: The Last Piece of Rf Spectrum Puzzle for Communication Systems. IEEE Open J. Commun. Soc. 2020, 1, 1–32. [Google Scholar] [CrossRef]
- Rappaport, T.S.; Xing, Y.; Kanhere, O.; Ju, S.; Madanayake, A.; Mandal, S.; Alkhateeb, A.; Trichopoulos, G.C. Wireless Communications and Applications above 100 Ghz: Opportunities and Challenges for 6g and Beyond. IEEE Access 2019, 7, 78729–78757. [Google Scholar] [CrossRef]
- Dang, S.; Amin, O.; Shihada, B.; Alouini, M.-S. What Should 6g Be? Nat. Electron. 2020, 3, 20–29. [Google Scholar] [CrossRef]
- Thomas, S.; Virdi, J.S.; Babakhani, A.; Roberts, I.P. A Survey on Advancements in Thz Technology for 6g: Systems, Circuits, Antennas, and Experiments. IEEE Open J. Commun. Soc. 2025, 6, 1998–2016. [Google Scholar] [CrossRef]
- Li, S.; Zhang, Z.; Rupakula, B.; Rebeiz, G.M. An Eight-Element 140-Ghz Wafer-Scale If Beamforming Phased-Array Receiver with 64-Qam Operation in Cmos Rfsoi. IEEE J. Solid-State Circuits 2022, 57, 385–399. [Google Scholar] [CrossRef]
- Song, H.J.; Lee, N. Terahertz Communications: Challenges in the Next Decade. IEEE Trans. Terahertz Sci. Technol. 2022, 12, 105–117. [Google Scholar] [CrossRef]
- F3: The Path to 6G: Architectures, Circuits, Technologies for Sub-Thz Communications, Sensing and Imaging. In Proceedings of the 2022 IEEE International Solid-State Circuits Conference (ISSCC), San Francisco, CA, USA, 20–26 February 2022.
- Mittleman, D.M. Twenty Years of Terahertz Imaging. Opt. Express 2018, 26, 9417–9431. [Google Scholar] [CrossRef]
- Castro-Camus, E.; Koch, M.; Mittleman, D.M. Recent Advances in Terahertz Imaging: 1999 to 2021. Appl. Phys. B 2021, 128, 12. [Google Scholar] [CrossRef]
- Rakita, A.; Nikolić, N.; Mildner, M.; Matiasek, J.; Elbe-Bürger, A. Re-Epithelialization and Immune Cell Behaviour in an Ex Vivo Human Skin Model. Sci. Rep. 2020, 10, 1. [Google Scholar] [CrossRef] [PubMed]
- Mikerov, M.; Shrestha, R.; van Dommelen, P.; Mittleman, D.M.; Koch, M. Analysis of Ancient Ceramics Using Terahertz Imaging and Photogrammetry. Opt. Express 2020, 28, 22255–22263. [Google Scholar] [CrossRef] [PubMed]
- Pizzuto, A.; Mittleman, D.M.; Klarskov, P. Laser Thz Emission Nanoscopy and Thz Nanoscopy. Opt. Express 2020, 28, 18778–18789. [Google Scholar] [CrossRef] [PubMed]
- Xu, S.; Guo, Y.; Liang, X.; Lu, H. Intelligent Rapid Detection Techniques for Low-Content Components in Fruits and Vegetables: A Comprehensive Review. Foods 2024, 13, 1116. [Google Scholar] [CrossRef]
- Si, W.; Zhou, W.; Liu, X.; Wang, K.; Liao, Y.; Yan, F.; Ji, X. Recent Advances in Broadband Photodetectors from Infrared to Terahertz. Micromachines 2024, 15, 427. [Google Scholar] [CrossRef]
- Nauta, B. 1.2 Racing Down the Slopes of Moore’s Law. In Proceedings of the 2024 IEEE International Solid-State Circuits Conference (ISSCC), San Francisco, CA, USA, 18–22 February 2024. [Google Scholar]
- Ong, S.N.; Chan, L.H.K.; Chew, K.W.J.; Lim, C.K.; Oo, W.L.; Bellaouar, A.; Zhang, C.; Chow, W.H.; Chen, T.; Rassel, R.; et al. 22nm Fd-Soi Technology with Back-Biasing Capability Offers Excellent Performance for Enabling Efficient, Ultra-Low Power Analog and Rf/Millimeter-Wave Designs. In Proceedings of the 2019 IEEE Radio Frequency Integrated Circuits Symposium (RFIC), Boston, MA, USA, 2–4 June 2019. [Google Scholar]
- Mei, X.; Yoshida, W.; Lange, M.; Lee, J.; Zhou, J.; Liu, P.-H.; Leong, K.; Zamora, A.; Padilla, J.; Sarkozy, S.; et al. First Demonstration of Amplification at 1 Thz Using 25-Nm Inp High Electron Mobility Transistor Process. IEEE Electron Device Lett. 2015, 36, 327–329. [Google Scholar] [CrossRef]
- Pekarik, J.; Jain, V.; Kenney, C.; Holt, J.; Khokale, S.; Saroop, S.; Johnson, J.B.; Stein, K.; Ontalus, V.; Durcan, C.; et al. Sige Hbts with SiGe HBTs with fT/fMAX~375/510 GHz Integrated in 45nm Pdsoi Cmos. In Proceedings of the 2021 IEEE BiCMOS and Compound Semiconductor Integrated Circuits and Technology Symposium (BCICTS), Monterey, CA, USA, 5–8 December 2021. [Google Scholar]
- Zhou, S.; Chen, K.; Cole, M.T.; Li, Z.; Li, M.; Chen, J.; Lienau, C.; Li, C.; Dai, Q. Ultrafast Electron Tunneling Devices—From Electric-Field Driven to Optical-Field Driven. Adv. Mater. 2021, 33, 2101449. [Google Scholar] [CrossRef]
- Taghinejad, M.; Xia, C.; Hrton, M.; Lee, K.-T.; Kim, A.S.; Li, Q.; Guzelturk, B.; Kalousek, R.; Xu, F.; Cai, W.; et al. Determining Hot-Carrier Transport Dynamics from Terahertz Emission. Science 2023, 382, 299–305. [Google Scholar] [CrossRef]
- Nagatsuma, T.; Ducournau, G.; Renaud, C.C. Advances in Terahertz Communications Accelerated by Photonics. Nat. Photonics 2016, 10, 371–379. [Google Scholar] [CrossRef]
- Razeghi, M.; Lu, Q. Room Temperature Terahertz and Frequency Combs Based on Intersubband Quantum Cascade Laser Diodes: History and Future. Photonics 2025, 12, 79. [Google Scholar] [CrossRef]
- Mehdi, I.; Siles, J.V.; Lee, C.; Schlecht, E. Thz Diode Technology: Status, Prospects, and Applications. Proc. IEEE 2017, 105, 990–1007. [Google Scholar] [CrossRef]
- Li, X.; Feng, J. Microfabrication Technologies for Interaction Circuits of Thz Vacuum Electronic Devices. Micromachines 2024, 15, 1357. [Google Scholar] [CrossRef] [PubMed]
- Habibighahfarokhi, F.; Sergaeva, O.; Carletti, L.; Franceschini, P.; Tognazzi, A.; Locatelli, A.; Leon, U.A.; Della Valle, G.; De Angelis, C.; Rocco, D. Nonlinear Dielectric Metasurfaces for Terahertz Applications. Photonics 2025, 12, 370. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, Y.; Guo, X.; Chen, T.; Liang, H.; Hao, X.; Hou, X.; Kou, W.; Zhao, Y.; Zhou, T.; et al. A Review of Thz Modulators with Dynamic Tunable Metasurfaces. Nanomaterials 2019, 9, 965. [Google Scholar] [CrossRef]
- Fan, R.; Xiong, B.; Peng, R.; Wang, M. Constructing Metastructures with Broadband Electromagnetic Functionality. Adv. Mater. 2020, 32, 1904646. [Google Scholar] [CrossRef]
- Zhao, X.; Duan, G.; Li, A.; Chen, C.; Zhang, X. Integrating Microsystems with Metamaterials Towards Metadevices. Microsyst. Nanoeng. 2019, 5, 5. [Google Scholar] [CrossRef]
- Ji, C.; Xu, S.; Liang, Q.; Zhang, X.; Hong, X.; Wang, Y.; Li, X.; Jiang, L.; Wang, Y.; Ni, J.; et al. Observation of Magnetic Quadrupole Endowed Helical Dichroism in Artificial Propeller Meta-Molecules. Adv. Opt. Mater. 2024, 12, 2302795. [Google Scholar] [CrossRef]
- Tsai, H.-Y.; Chen, C.-C.; Chen, T.-A.; Tsai, D.P.; Tanaka, T.; Yen, T.-J. Realization of Negative Permeability in Vertical Double Split-Ring Resonators with Normal Incidence. ACS Photonics 2020, 7, 3298–3304. [Google Scholar] [CrossRef]
- Prakash, S.; Pitchappa, P.; Agrawal, P.; Jani, H.; Zhao, Y.; Kumar, A.; Thong, J.; Linke, J.; Ariando, A.; Singh, R.; et al. Electromechanically Reconfigurable Terahertz Stereo Metasurfaces. Adv. Mater. 2024, 36, 2402069. [Google Scholar] [CrossRef]
- Zhang, L.; Chen, X.Q.; Liu, S.; Zhang, Q.; Zhao, J.; Dai, J.Y.; Bai, G.D.; Wan, X.; Cheng, Q.; Castaldi, G.; et al. Space-Time-Coding Digital Metasurfaces. Nat. Commun. 2018, 9, 4334. [Google Scholar] [CrossRef]
- Chen, S.; Pan, T.; Wei, M.; Wang, B.-Z.; Lin, Y. Dynamic Modulation of Electromagnetically Induced Transparency Metamaterials Based on Mode Coupling with Stretchable Design. Eur. Phys. J. Spec. Top. 2025. [Google Scholar] [CrossRef]
- Li, F.; Pan, T.; Li, W.; Peng, Z.; Guo, D.; Jia, X.; Hu, T.; Wang, L.; Wang, W.; Gao, M.; et al. Flexible Intelligent Microwave Metasurface with Shape-Guided Adaptive Programming. Nat. Commun. 2025, 16, 3161. [Google Scholar] [CrossRef] [PubMed]
- Taghinejad, H.; Abdollahramezani, S.; Eftekhar, A.A.; Fan, T.; Hosseinnia, A.H.; Hemmatyar, O.; Dorche, A.E.; Gallmon, A.; Adibi, A. Ito-Based Microheaters for Reversible Multi-Stage Switching of Phase-Change Materials: Towards Miniaturized Beyond-Binary Reconfigurable Integrated Photonics. Opt. Express 2021, 29, 20449–20462. [Google Scholar] [CrossRef] [PubMed]
- Jeong, Y.-G.; Bahk, Y.-M.; Kim, D.-S. Dynamic Terahertz Plasmonics Enabled by Phase-Change Materials. Adv. Opt. Mater. 2020, 8, 1900548. [Google Scholar] [CrossRef]
- Zhou, S.; Wang, Z.; Nong, J.; Li, H.; Du, T.; Ma, H.; Li, S.; Deng, Y.; Zhao, F.; Zhang, Z.; et al. Optimized Wideband and Compact Multifunctional Photonic Device Based on Sb2s3 Phase Change Material. Optics Express 2024, 32, 8506–8519. [Google Scholar] [CrossRef]
- Naorem, R.; Dayal, G.; Ramakrishna, S.A.; Rajeswaran, B.; Umarji, A. Thermally Switchable Metamaterial Absorber with a VO2 Ground Plane. Opt. Commun. 2015, 346, 154–157. [Google Scholar] [CrossRef]
- Kim, H.; Charipar, N.; Breckenfeld, E.; Rosenberg, A.; Pique, A. Active Terahertz Metamaterials Based on the Phase Transition of VO2 Thin Films. Thin Solid Film. 2015, 596, 45–50. [Google Scholar] [CrossRef]
- Pitchappa, P.; Kumar, A.; Prakash, S.; Jani, H.; Venkatesan, T.; Singh, R. Chalcogenide Phase Change Material for Active Terahertz Photonics. Adv. Mater. 2019, 31, 1808157. [Google Scholar] [CrossRef]
- Sun, H.; Sun, T.; Song, Q.; Bian, L.; Yi, Z.; Zhang, J.; Hao, Z.; Tang, C.; Wu, P.; Zeng, Q. Temperature-Tunable Terahertz Metamaterial Device Based on VO2 Phase Transition Principle. Dalton Trans. 2024, 53, 17299–17307. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, Y.; Shi, Q.; Liang, S.; Huang, W.; Kou, W.; Yang, Z. Dynamic Photoinduced Controlling of the Large Phase Shift of Terahertz Waves via Vanadium Dioxide Coupling Nanostructures. ACS Photonics 2018, 5, 3040–3050. [Google Scholar] [CrossRef]
- Zhu, Y.; Vegesna, S.; Zhao, Y.; Kuryatkov, V.; Holtz, M.; Fan, Z.; Saed, M.; Bernussi, A.A. Tunable Dual-Band Terahertz Metamaterial Bandpass Filters. Opt. Lett. 2013, 38, 2382–2384. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Tan, Y.; Zhang, D.; Wen, Q.; Liao, Y.; Zhong, Z.; Wen, T. Plasmonic Nanocomposite for Active Dielectric Metasurface Terahertz Absorbers. IEEE Trans. Terahertz Sci. Technol. 2023, 13, 381–388. [Google Scholar] [CrossRef]
- Petzelt, J.; Bovtun, V.; Nuzhnyy, D.; Kempa, M.; Savinov, M.; Paściak, M.; Kamba, S.; Canu, G.; Buscaglia, V. Broadband Dielectric, Terahertz, and Infrared Spectroscopy of Batio3–Bazro3 Solid Solution: From Proper Ferroelectric over Diffuse and Relaxor Ferroelectrics and Dipolar Glass to Normal Dielectric. Phys. Status Solidi B 2021, 258, 2100259. [Google Scholar] [CrossRef]
- Singh, R.; Azad, A.K.; Jia, Q.X.; Taylor, A.J.; Chen, H.-T. Thermal Tunability in Terahertz Metamaterials Fabricated on Strontium Titanate Single-Crystal Substrates. Opt. Lett. 2011, 36, 1230–1232. [Google Scholar] [CrossRef]
- Yahiaoui, R.; Němec, H.; Kužel, P.; Kadlec, F.; Kadlec, C.; Mounaix, P. Tunable Thz Metamaterials Based on an Array of Paraelectric Srtio3 Rods. Appl. Phys. A 2011, 103, 689–692. [Google Scholar] [CrossRef]
- Chuwen, L.; Zhu, D.; Gao, J.; Li, B.; Gao, Z. Flexible and Tunable Terahertz All-Dielectric Metasurface Composed of Ceramic Spheres Embedded in Ferroelectric/Elastomer Composite. Opt. Express 2018, 26, 11633–11638. [Google Scholar]
- Bian, Y.; Wu, C.; Li, H.; Zhai, J. A Tunable Metamaterial Dependent on Electric Field at Terahertz with Barium Strontium Titanate Thin Film. Appl. Phys. Lett. 2014, 104, 042906. [Google Scholar] [CrossRef]
- Matthias, W.; Yamada, N. Phase-Change Materials for Rewriteable Data Storage. Nat. Mater. 2007, 6, 824–832. [Google Scholar]
- Kowerdziej, R.; Jaroszewicz, L.; Olifierczuk, M.; Parka, J. Experimental Study on Terahertz Metamaterial Embedded in Nematic Liquid Crystal. Appl. Phys. Lett. 2015, 106, 092905. [Google Scholar] [CrossRef]
- Bruno, P.; Paparo, D.; Rubano, A.; Andreone, A.; Conti, M.R.; Giove, D.; Vicuña-Hernández, V.; Koral, C.; Masullo, M.R.; Mettivier, G.; et al. Liquid Crystal-Based Geometric Phase-Enhanced Platform for Polarization and Wavefront Analysis Techniques with the Short-Terahertz Fel Oscillator Terra@Brixsino. Symmetry 2023, 15, 103. [Google Scholar]
- Chikhi, N.; Lisitskiy, M.; Papari, G.; Tkachenko, V.; Andreone, A. A Hybrid Tunable Thz Metadevice Using a High Birefringence Liquid Crystal. Sci. Rep. 2016, 6, 34536. [Google Scholar] [CrossRef] [PubMed]
- Gu, J.; Singh, R.; Tian, Z.; Cao, W.; Xing, Q.; He, M.; Zhang, J.W.; Han, J.; Chen, H.-T.; Zhang, W. Terahertz Superconductor Metamaterial. Appl. Phys. Lett. 2010, 97, 071102. [Google Scholar] [CrossRef]
- Chen, H.-T.; Yang, H.; Singh, R.; O’hara, J.F.; Azad, A.K.; Trugman, S.A.; Jia, Q.X.; Taylor, A.J. Tuning the Resonance in High-Temperature Superconducting Terahertz Metamaterials. Phys. Rev. Lett. 2010, 105, 247402. [Google Scholar] [CrossRef]
- Zhang, C.H.; Wu, J.B.; Jin, B.B.; Ji, Z.M.; Kang, L.; Xu, W.W.; Chen, J.; Tonouchi, M.; Wu, P.H. Low-Loss Terahertz Metamaterial from Superconducting Niobium Nitride Films. Opt. Express 2012, 20, 42–47. [Google Scholar] [CrossRef]
- Ke, Y.; Wang, S.; Liu, G.; Li, M.; White, T.J.; Long, Y. Vanadium Dioxide: The Multistimuli Responsive Material and Its Applications. Small 2018, 14, 1802025. [Google Scholar] [CrossRef]
- Liu, H.; Lu, J.; Xiao, R.W. Metamaterials Based on the Phase Transition of Vo2. Nanotechnology 2018, 29, 024002. [Google Scholar] [CrossRef]
- Shaltout, A.M.; Shalaev, V.M.; Brongersma, M.L. Spatiotemporal Light Control with Active Metasurfaces. Science 2019, 364, eaat3100. [Google Scholar] [CrossRef]
- Kim, C.-Y.; Slusar, T.; Cho, J.; Kim, H.-T. Mott Switching and Structural Transition in the Metal Phase of Vo2 Nanodomain. ACS Appl. Electron. Mater. 2021, 3, 605–610. [Google Scholar] [CrossRef]
- Kim, H.-T.; Chae, B.-G.; Youn, D.-H.; Maeng, S.-L.; Kim, G.; Kang, K.-Y.; Lim, Y.-S. Mechanism and Observation of Mott Transition in Vo2-Based Two- and Three-Terminal Devices. New J. Phys. 2004, 6, 52. [Google Scholar] [CrossRef]
- Ji, Y.; Pan, R.; Chen, H.; Zhang, H.; Zhao, R.; Yuan, Y.; Yang, H. Shear Strain Stabilized High-Temperature Metallic Monoclinic Vo2 Variants with Symmetry Permission. Appl. Phys. Lett. 2023, 122, 111603. [Google Scholar] [CrossRef]
- Lu, C.; Lu, Q.; Gao, M.; Lin, Y. Dynamic Manipulation of Thz Waves Enabled by Phase-Transition Vo2 Thin Film. Nanomaterials 2021, 11, 114. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Lee, J.H.; Zhu, Y.; Nazari, M.; Chen, C.; Wang, H.; Bernussi, A.; Holtz, M.; Fan, Z. Structural, Electrical, and Terahertz Transmission Properties of Vo2 Thin Films Grown on C-, R-, and M-Plane Sapphire Substrates. J. Appl. Phys. 2012, 111, 053533. [Google Scholar] [CrossRef]
- Cocker, T.L.; Titova, L.V.; Fourmaux, S.; Bandulet, H.-C.; Brassard, D.; Kieffer, J.-C.; El Khakani, M.A.; Hegmann, F.A. Terahertz Conductivity of the Metal-Insulator Transition in a Nanogranular VO2 Film. Appl. Phys. Lett. 2010, 97, 221905. [Google Scholar] [CrossRef]
- Cocker, T.L.; Titova, L.V.; Fourmaux, S.; Holloway, G.; Bandulet, H.-C.; Brassard, D.; Kieffer, J.-C.; El Khakani, M.A.; Hegmann, F.A. Phase Diagram of the Ultrafast Photoinduced Insulator-Metal Transition in Vanadium Dioxide. Phys. Rev. B 2012, 85, 155120. [Google Scholar] [CrossRef]
- Liang, W.; Li, G.; Zhou, Q.; Zhang, Z.; Zhang, S.; Ning, T.; Zhang, P.; Deng, Y.; Zhang, C.; Ge, C.; et al. Resonance Dependence of Electrically Reconfigurable VO2-Based Thz Metadevice for Memory Information Processing. Appl. Phys. Lett. 2023, 122, 071702. [Google Scholar] [CrossRef]
- Peng, S.; Lu, X.; Tang, L.; Chang, X.; Yan, J.; Shi, Q.; Chen, K.; Li, J.; Du, L.-H.; Huang, W. Thermal and Mechanical Thz Modulation of Flexible All-Dielectric Metamaterial. Opt. Express 2023, 31, 2644–2653. [Google Scholar] [CrossRef]
- Tan, T.C.; Srivastava, Y.K.; Ako, R.T.; Wang, W.; Bhaskaran, M.; Sriram, S.; Al-Naib, I.; Plum, E.; Singh, R. Active Control of Nanodielectric-Induced Thz Quasi-Bic in Flexible Metasurfaces: A Platform for Modulation and Sensing. Adv. Mater. 2021, 33, e2100836. [Google Scholar] [CrossRef]
- Peng, J.; Lin, X.; Yan, X.; Yan, X.; Hu, X.; Yao, H.; Liang, L.; Ma, G. Terahertz Biosensor Engineering Based on Quasi-Bic Metasurface with Ultrasensitive Detection. Nanomaterials 2024, 14, 799. [Google Scholar] [CrossRef]
- Jiao, R.; Wang, Q.; Liu, J.; Shu, F.; Pan, G.; Jing, X.; Hong, Z. High-Q Quasi-Bound States in the Continuum in Terahertz All-Silicon Metasurfaces. Micromachines 2023, 14, 1817. [Google Scholar] [CrossRef]
- Koshelev, K.; Favraud, G.; Bogdanov, A.; Kivshar, Y.; Fratalocchi, A. Nonradiating Photonics with Resonant Dielectric Nanostructures. Nanophotonics 2019, 8, 725–745. [Google Scholar] [CrossRef]
- Kužel, P.; Němec, H. Terahertz Spectroscopy of Nanomaterials: A Close Look at Charge—Carrier Transport. Adv. Opt. Mater. 2020, 8, 1900623. [Google Scholar] [CrossRef]
- Lu, C.; Gao, M.; Liu, J.; Lu, Y.; Wen, T.; Lin, Y. Separation of Terahertz and Dc Conductivity Transitions in Epitaxial Vanadium Dioxide Films. J. Appl. Phys. 2024, 135, 225301. [Google Scholar] [CrossRef]
- Choi, H.S.; Ahn, J.S.; Jung, J.H.; Noh, T.W.; Kim, D.H. Mid-Infrared Properties of a VO2 Film near the Metal-Insulator Transition. Phys. Rev. B 1996, 54, 4621–4628. [Google Scholar] [CrossRef] [PubMed]
- Qazilbash, M.M.; Tripathi, A.; Schafgans, A.A.; Kim, B.-J.; Kim, H.-T.; Cai, Z.; Holt, M.V.; Maser, J.M.; Keilmann, F.; Shpyrko, O.G.; et al. Nanoscale Imaging of the Electronic and Structural Transitions in Vanadium Dioxide. Phys. Rev. B 2011, 83, 165108. [Google Scholar] [CrossRef]
- Vidas, L.; Günther, C.M.; Miller, T.A.; Pfau, B.; Perez-Salinas, D.; Martínez, E.; Schneider, M.; Guehrs, E.; Gargiani, P.; Valvidares, M.; et al. Imaging Nanometer Phase Coexistence at Defects During the Insulator–Metal Phase Transformation in VO2 Thin Films by Resonant Soft X-Ray Holography. Nano Lett. 2018, 18, 3449–3453. [Google Scholar] [CrossRef]
- Stinson, H.T.; Sternbach, A.; Najera, O.; Jing, R.; Mcleod, A.S.; Slusar, T.V.; Mueller, A.; Anderegg, L.; Kim, H.T.; Rozenberg, M.; et al. Imaging the Nanoscale Phase Separation in Vanadium Dioxide Thin Films at Terahertz Frequencies. Nat. Commun. 2018, 9, 3604. [Google Scholar] [CrossRef]
- Qazilbash, M.M.; Brehm, M.; Chae, B.-G.; Ho, P.-C.; Andreev, G.O.; Kim, B.-J.; Yun, S.J.; Balatsky, A.V.; Maple, M.B.; Keilmann, F.; et al. Mott Transition in VO2 Revealed by Infrared Spectroscopy and Nano-Imaging. Science 2007, 318, 1750–1753. [Google Scholar] [CrossRef]
- Lu, C.; Liang, W. Reconfigurable Terahertz Metamaterials Based on the Refractive Index Change of Epitaxial Vanadium Dioxide Films across the Metal–Insulator Transition. Nanomaterials 2025, 15, 439. [Google Scholar] [CrossRef]
- Li, Y.; Ma, H.; Wang, Y.; Ding, J.; Qi, L.; Fu, Y.; Ning, R.; Rong, L.; Wang, D.; Zhang, X. Electrically Driven Active VO2/Mxene Metasurface for the Terahertz Modulation. Appl. Phys. Lett. 2024, 121, 241902. [Google Scholar]
- Li, Y.; Ma, H.; Shi, R.; Wu, Y.; Feng, S.; Fu, Y.; Wei, Y.; Zhao, X.; Dong, K.; Jiang, K.; et al. Wafer-Scale Transfer and Integration of Tungsten-Doped Vanadium Dioxide Films. ACS Nano 2025, 19, 6209–6220. [Google Scholar] [CrossRef]
- Yang, L.; Fan, F.; Chen, M.; Zhang, X.; Chang, S.-J. Active Terahertz Metamaterials Based on Liquid-Crystal Induced Transparency and Absorption. Opt. Commun. 2017, 382, 42–48. [Google Scholar] [CrossRef]
- Kowerdziej, R.; Olifierczuk, M.; Parka, J. Thermally Induced Tunability of a Terahertz Metamaterial by Using a Specially Designed Nematic Liquid Crystal Mixture. Opt. Express 2018, 26, 2443–2452. [Google Scholar] [CrossRef]
| Material | Phase Transition Mechanism | Metamaterial Structure | Triggering Condition | Permittivity Change in PCM | Published Year |
|---|---|---|---|---|---|
| BTO | Ferroelectric | Metal resonator–BTO film | Electric field (0–33 kV/cm) | 253 to 185 | 2014 [51] |
| STO | Ferroelectric | Metal resonator–STO bulk | Temperature (136 to −123 °C) | 219 to 829 | 2011 [48] |
| STO/AuNP/PDMS (all-dielectric metamaterial) | Continuous laser (2.5 W) | 10 to 12 * (composite) | 2023 [46] | ||
| Liquid Crystal (LC) | Adjusting molecular alignment | Metal resonator–LC | Electric field (0–7 kV/m) | 2.62 to 2.89 | 2017 [85] |
| Metal resonator–LC | Temperature (25 to 50 °C) | 3.6 to 2.9 | 2018 [86] | ||
| VO2 | MIT | ZrO2/VO2/PDMS (all-dielectric metamaterial) | Temperature (25 to 100 °C) | 5.3 to 10.2 * (composite) | 2023 [70] |
| Metal resonator–VO2 film | Heating circuits (0~0.7 A) | - | 2023 [69] | ||
| Metal resonator–VO2 film | Temperature (40 to 61 °C) | 80 to 1600 | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, C.; Liu, J.; Chen, S.; Guo, J. Exploiting the Modulation Effects of Epitaxial Vanadium Film in a Quasi-BIC-Based Terahertz Metamaterial. Materials 2025, 18, 2197. https://doi.org/10.3390/ma18102197
Lu C, Liu J, Chen S, Guo J. Exploiting the Modulation Effects of Epitaxial Vanadium Film in a Quasi-BIC-Based Terahertz Metamaterial. Materials. 2025; 18(10):2197. https://doi.org/10.3390/ma18102197
Chicago/Turabian StyleLu, Chang, Junxiao Liu, Sihong Chen, and Junxiong Guo. 2025. "Exploiting the Modulation Effects of Epitaxial Vanadium Film in a Quasi-BIC-Based Terahertz Metamaterial" Materials 18, no. 10: 2197. https://doi.org/10.3390/ma18102197
APA StyleLu, C., Liu, J., Chen, S., & Guo, J. (2025). Exploiting the Modulation Effects of Epitaxial Vanadium Film in a Quasi-BIC-Based Terahertz Metamaterial. Materials, 18(10), 2197. https://doi.org/10.3390/ma18102197






