Tension Strength of Multi-Fastener, Single-Lap Joints in Flax and Jute Composite Plates Using Bolts or Rivets
Abstract
1. Introduction
2. Materials and Methods
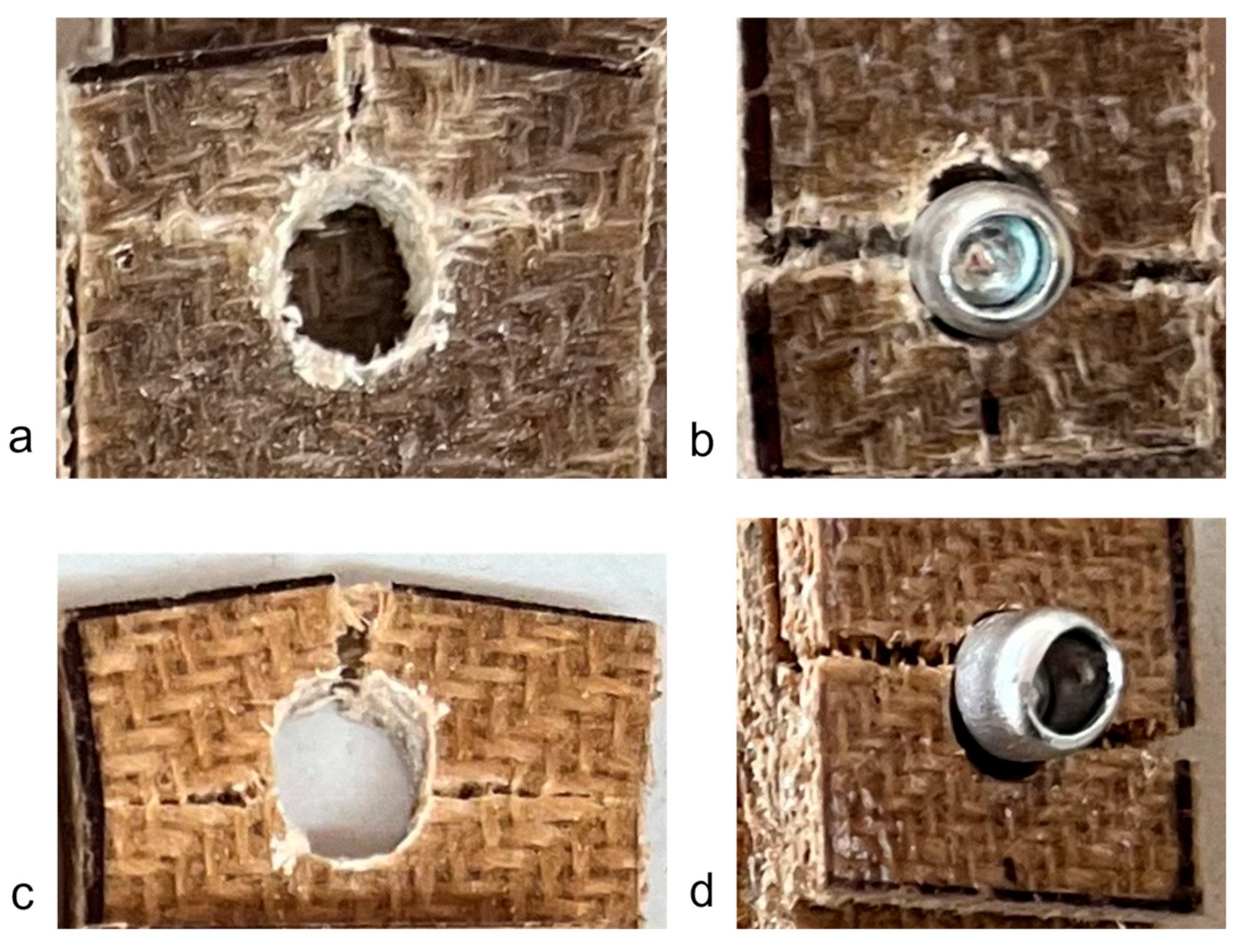
2.1. Design of Single-Lap Joints for the Experimental Program
2.2. Materials
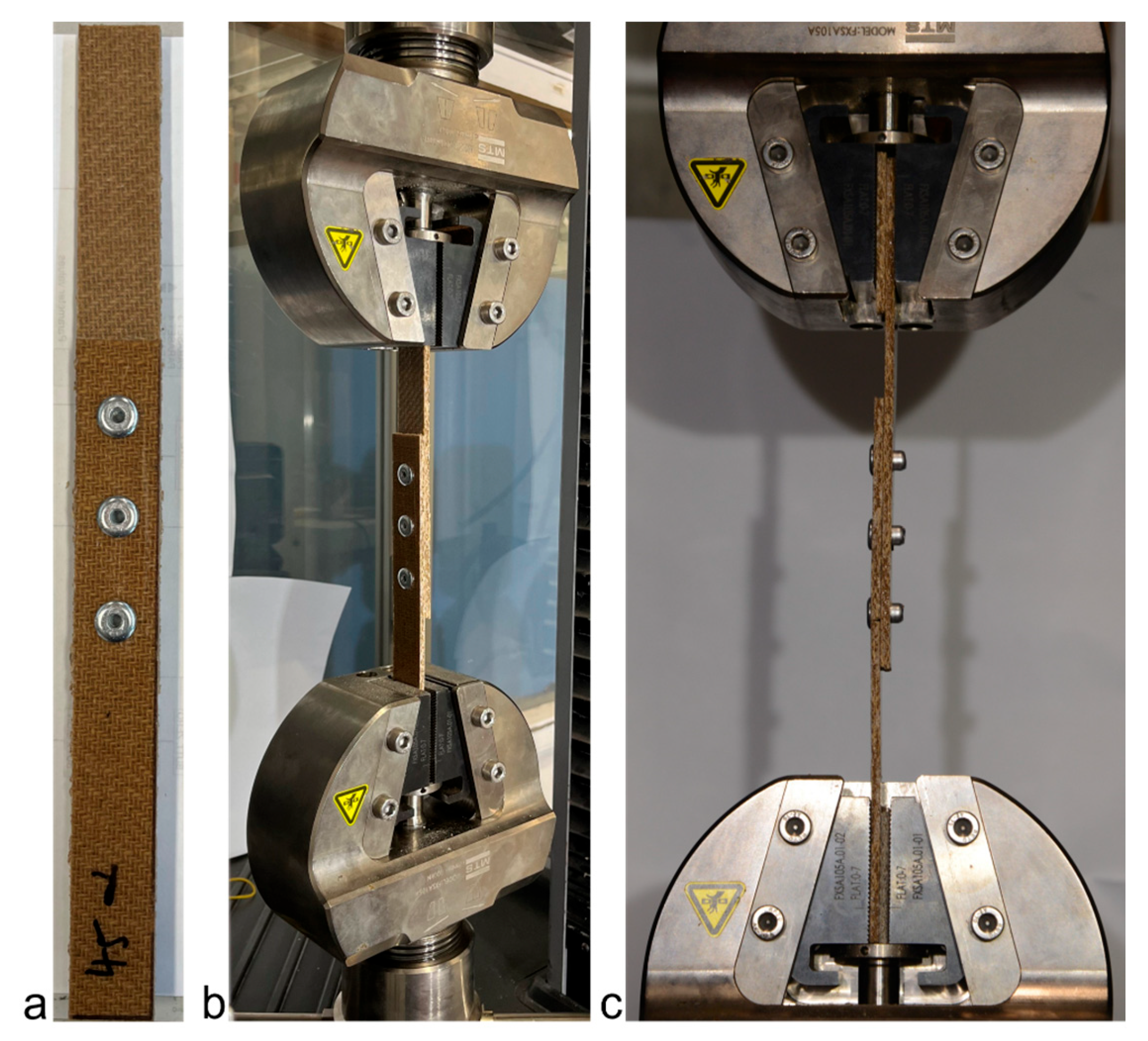
2.3. Experimental Setup
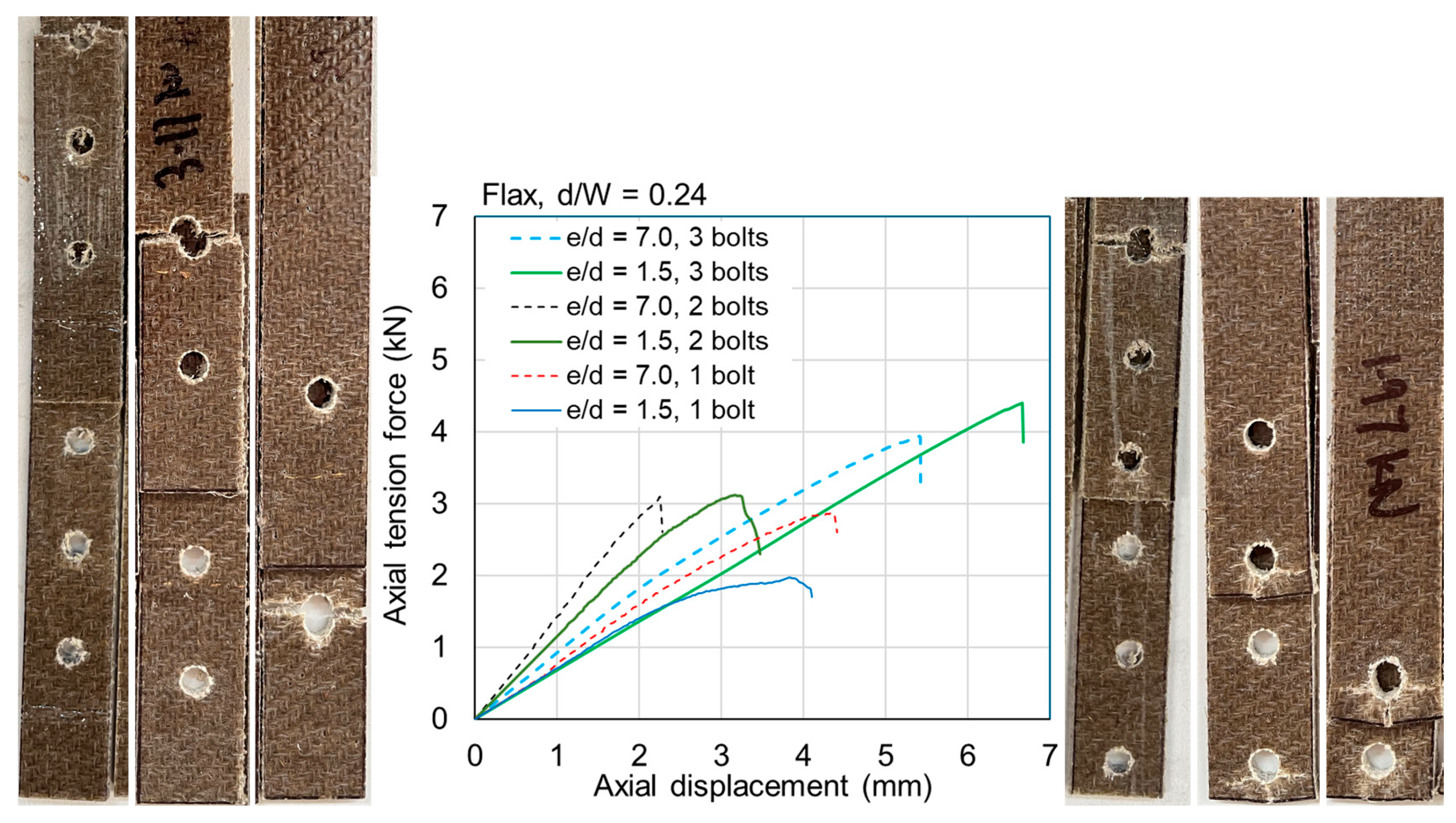
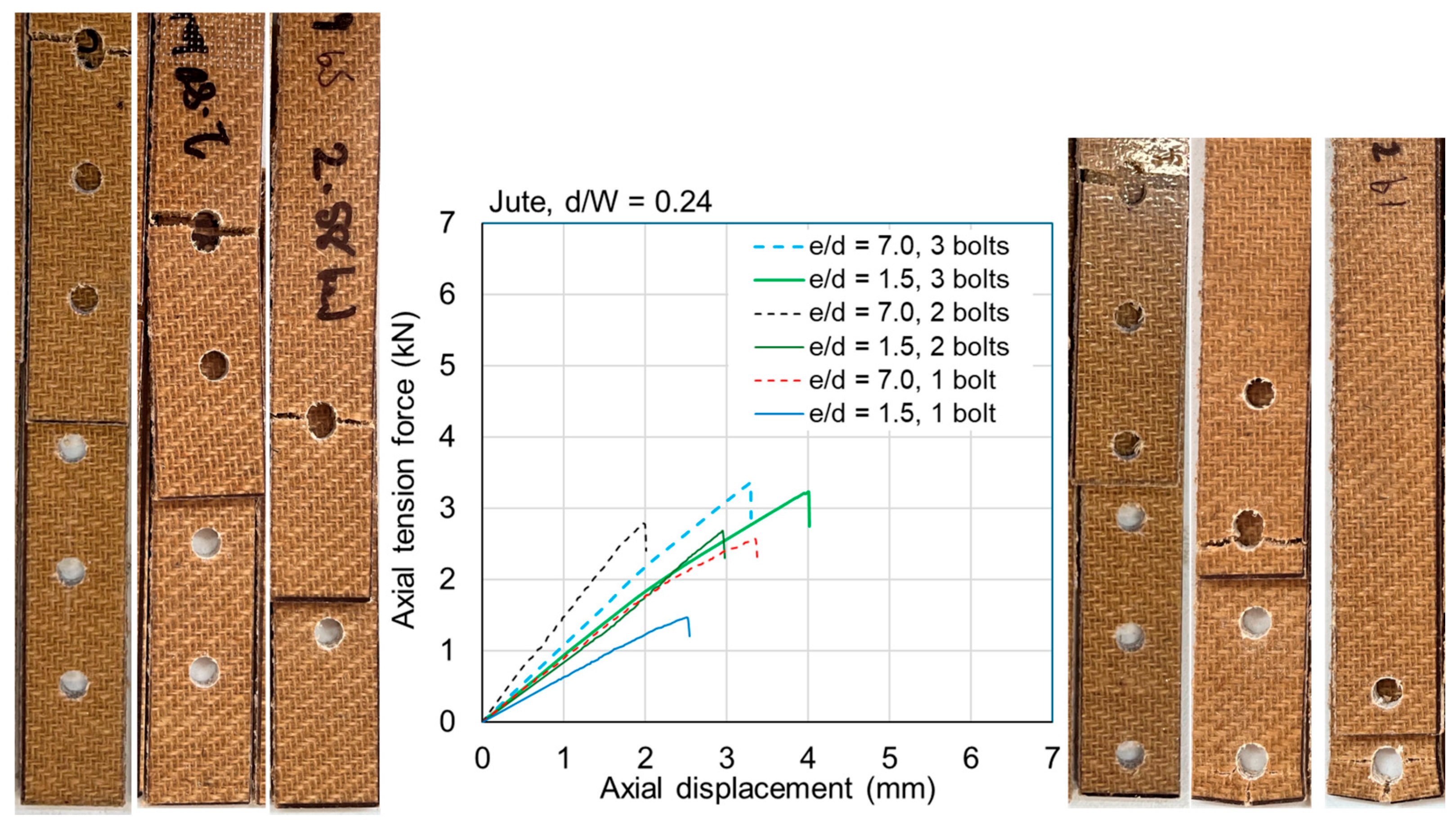
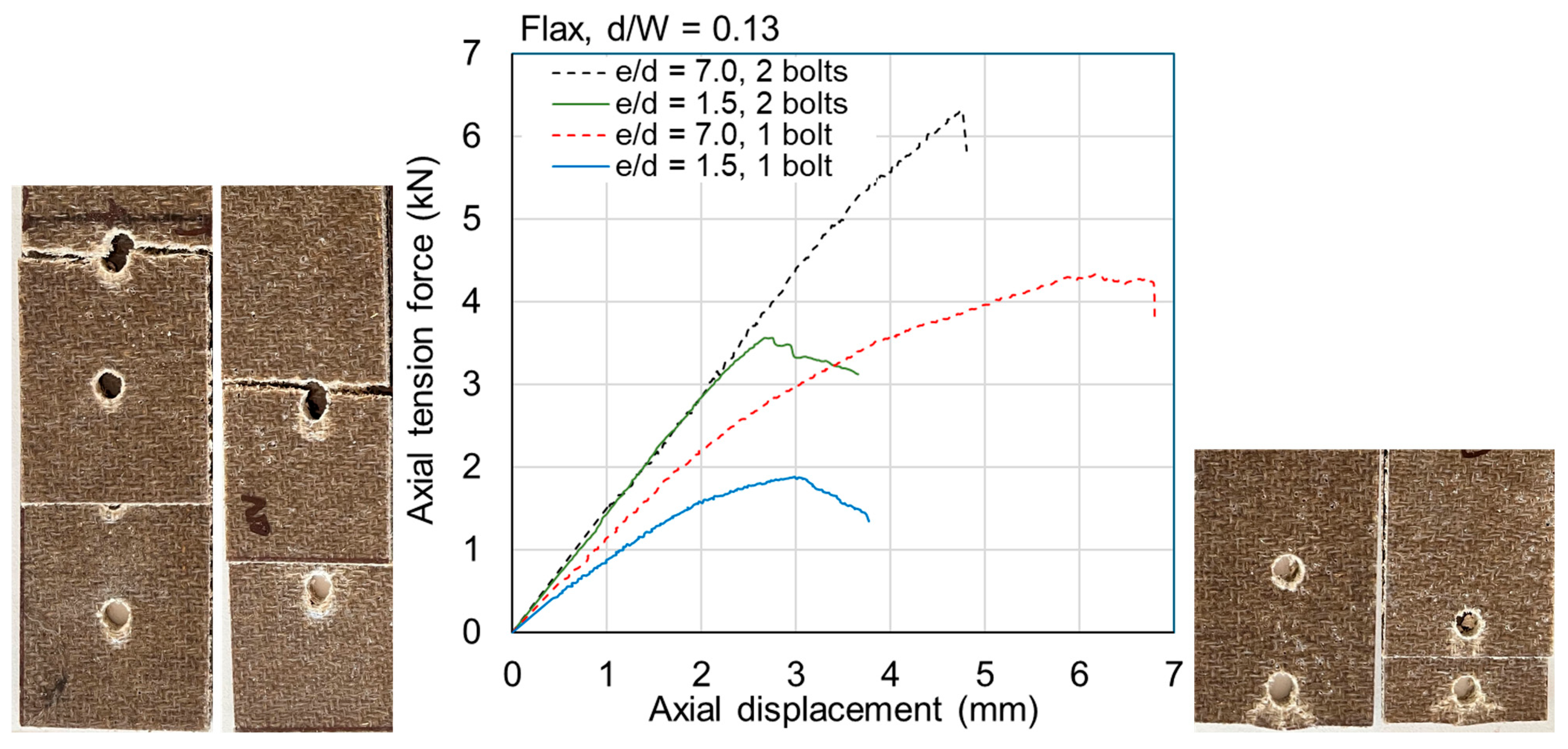
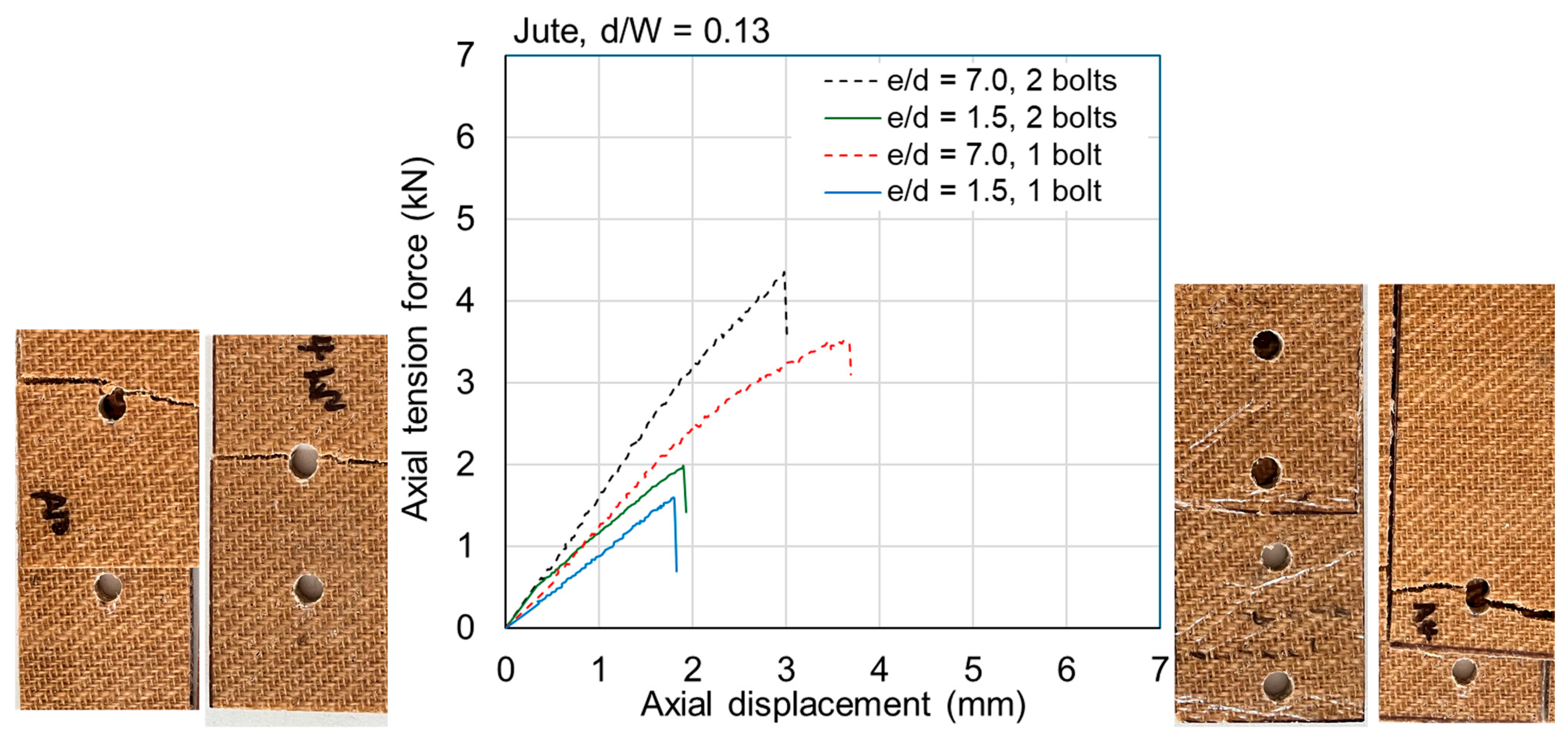
3. Results
3.1. Material Properties of the Flax and Jute Composites
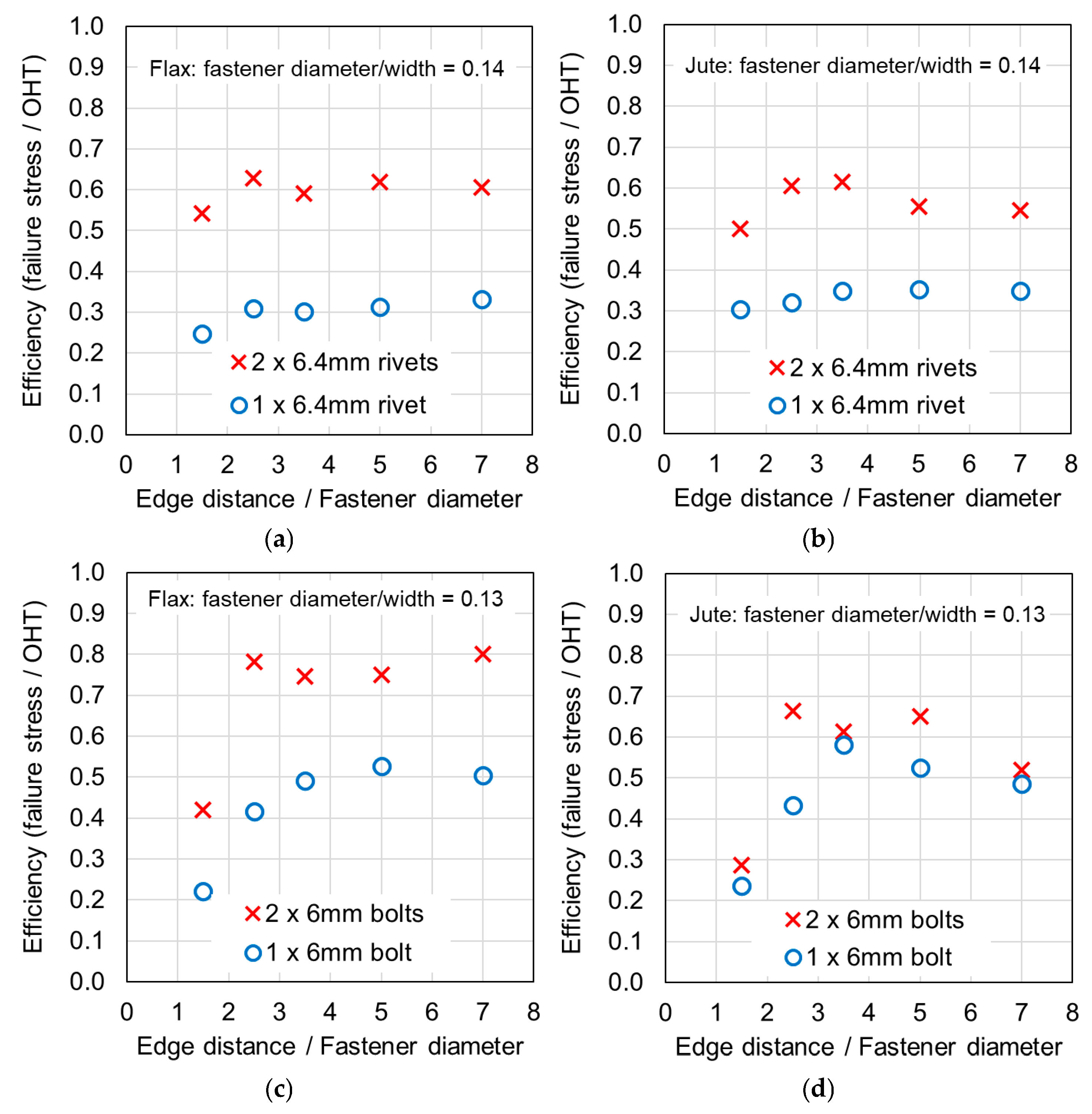
3.2. Efficiency of the Fastened Joints
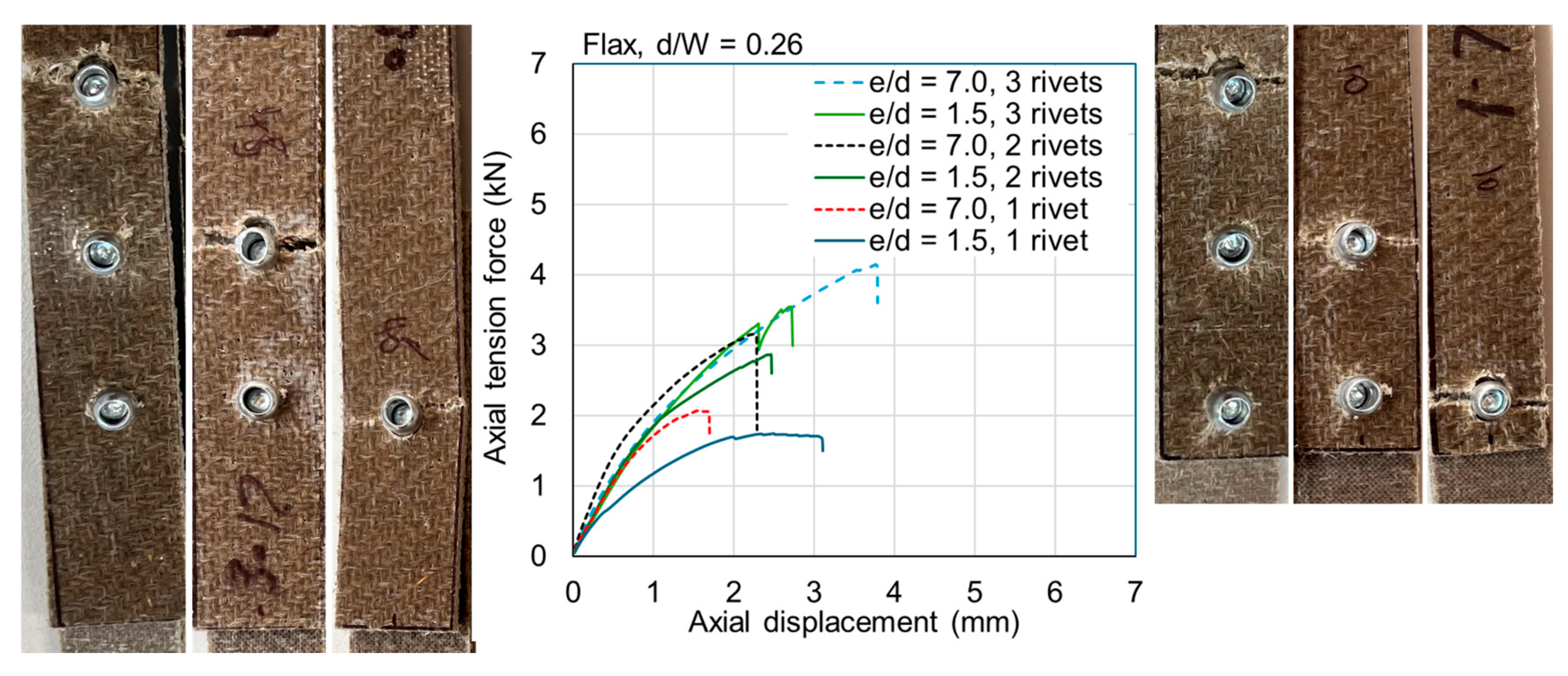
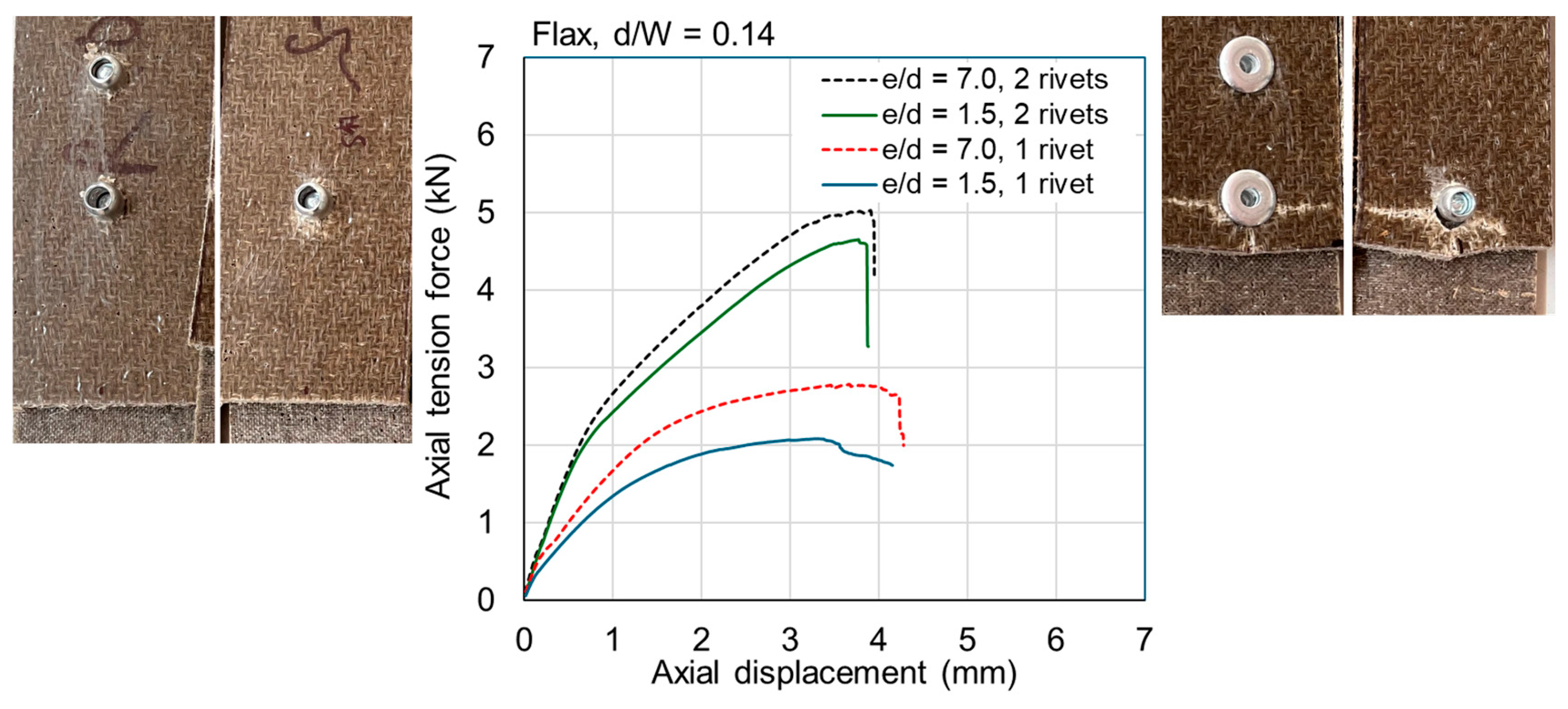
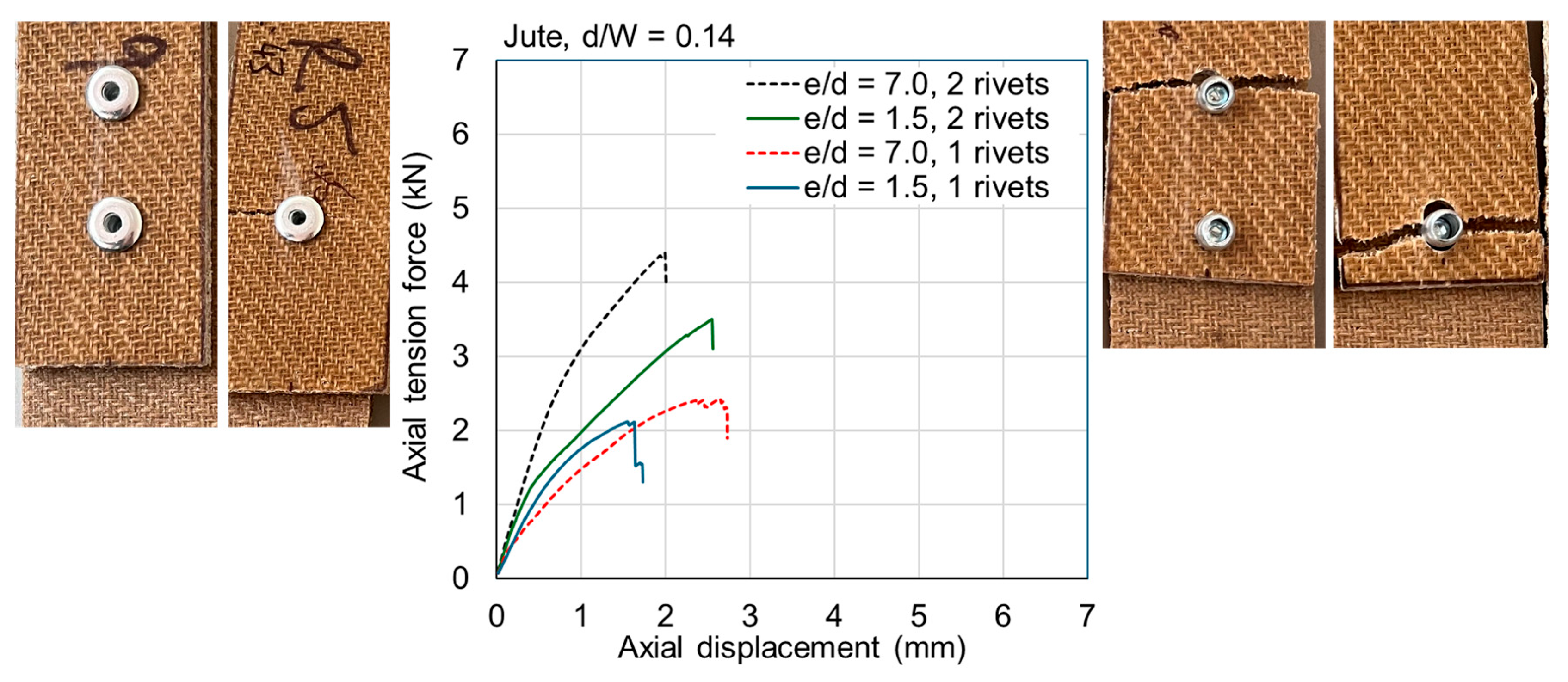
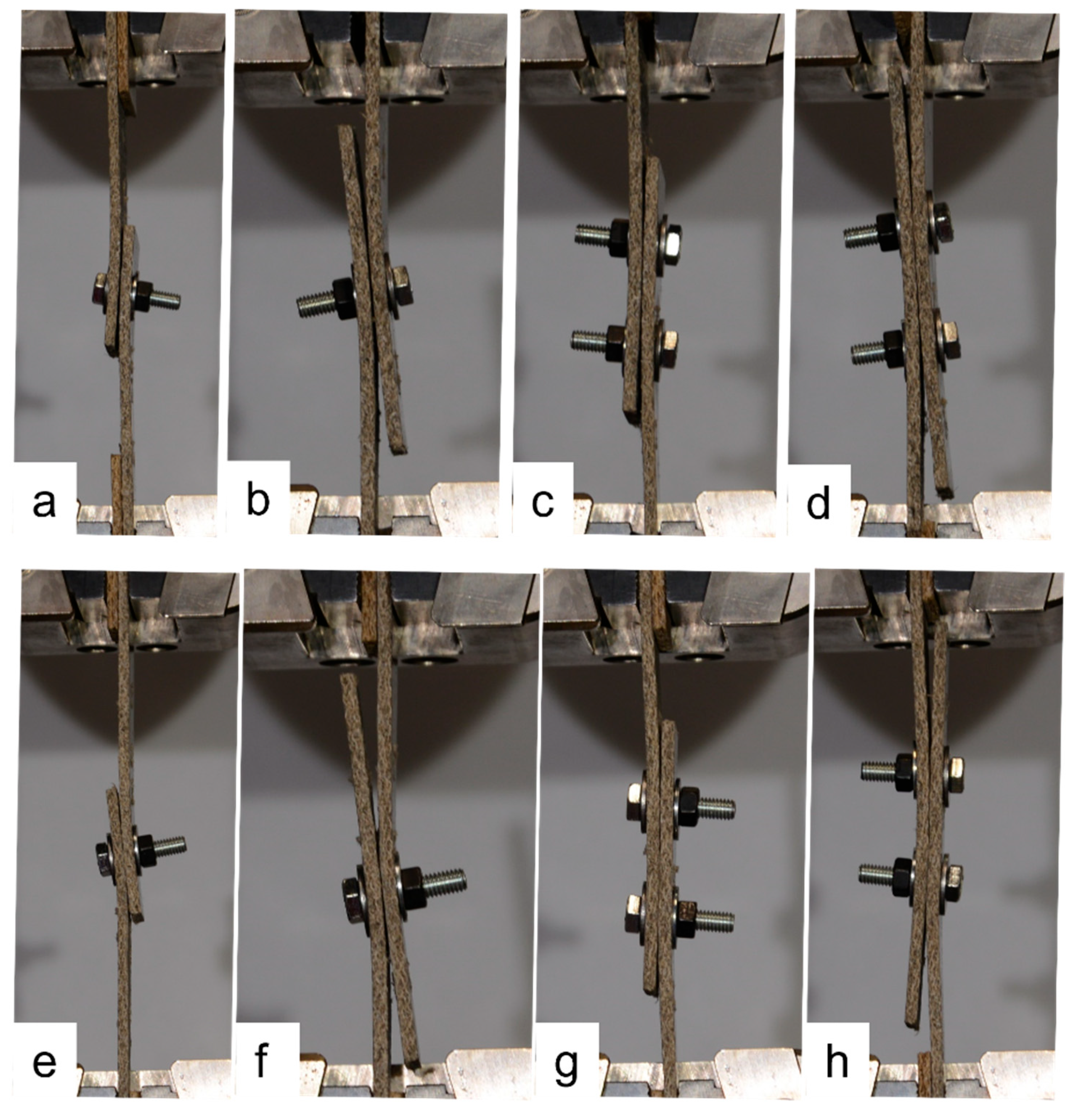
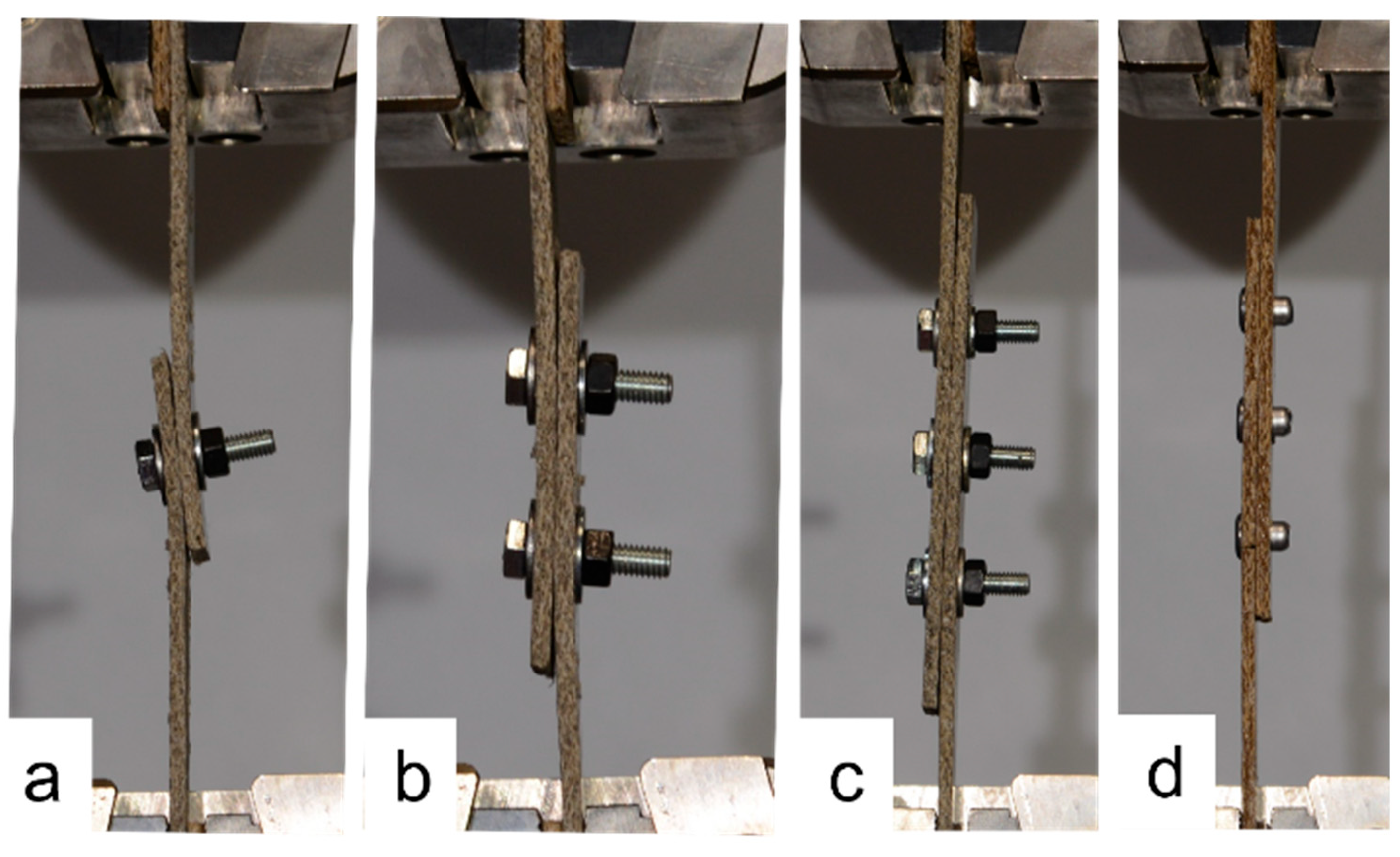
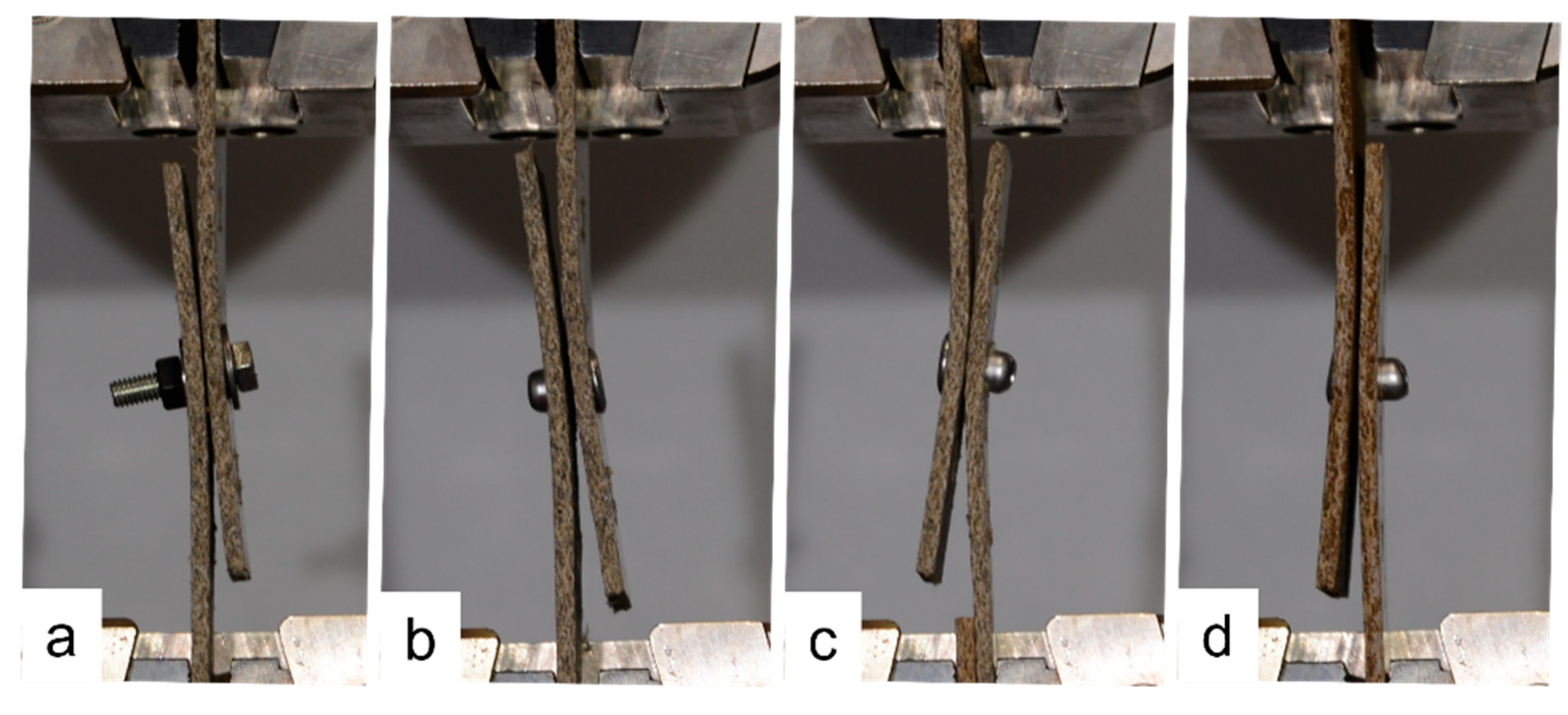
3.3. Ultimate Loads and Failure Modes
3.4. Tilting and Splaying
4. Discussion
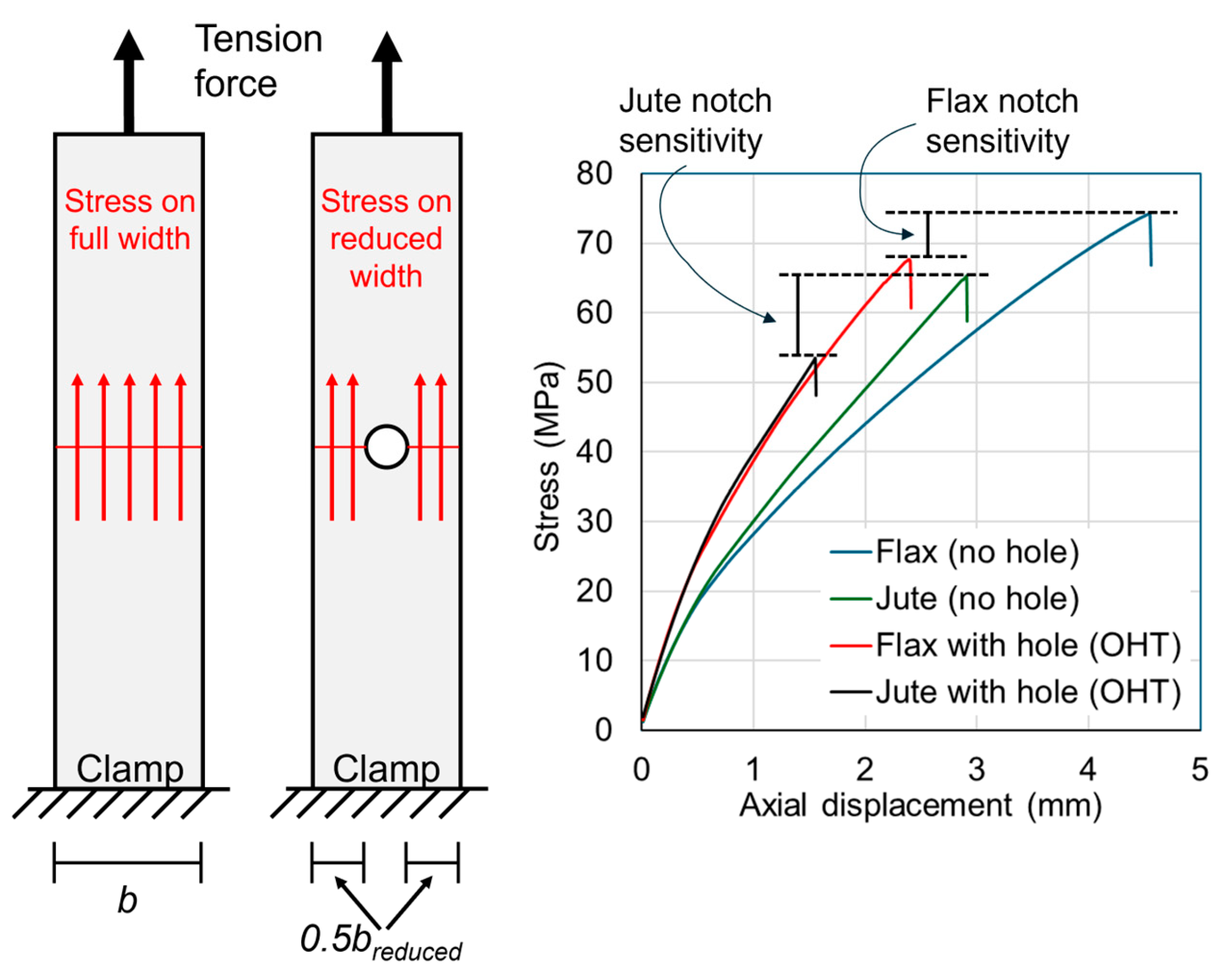
4.1. Notch Sensitivity
4.2. Efficiency and the Number of Fasteners
4.3. Effect of the Sample Width
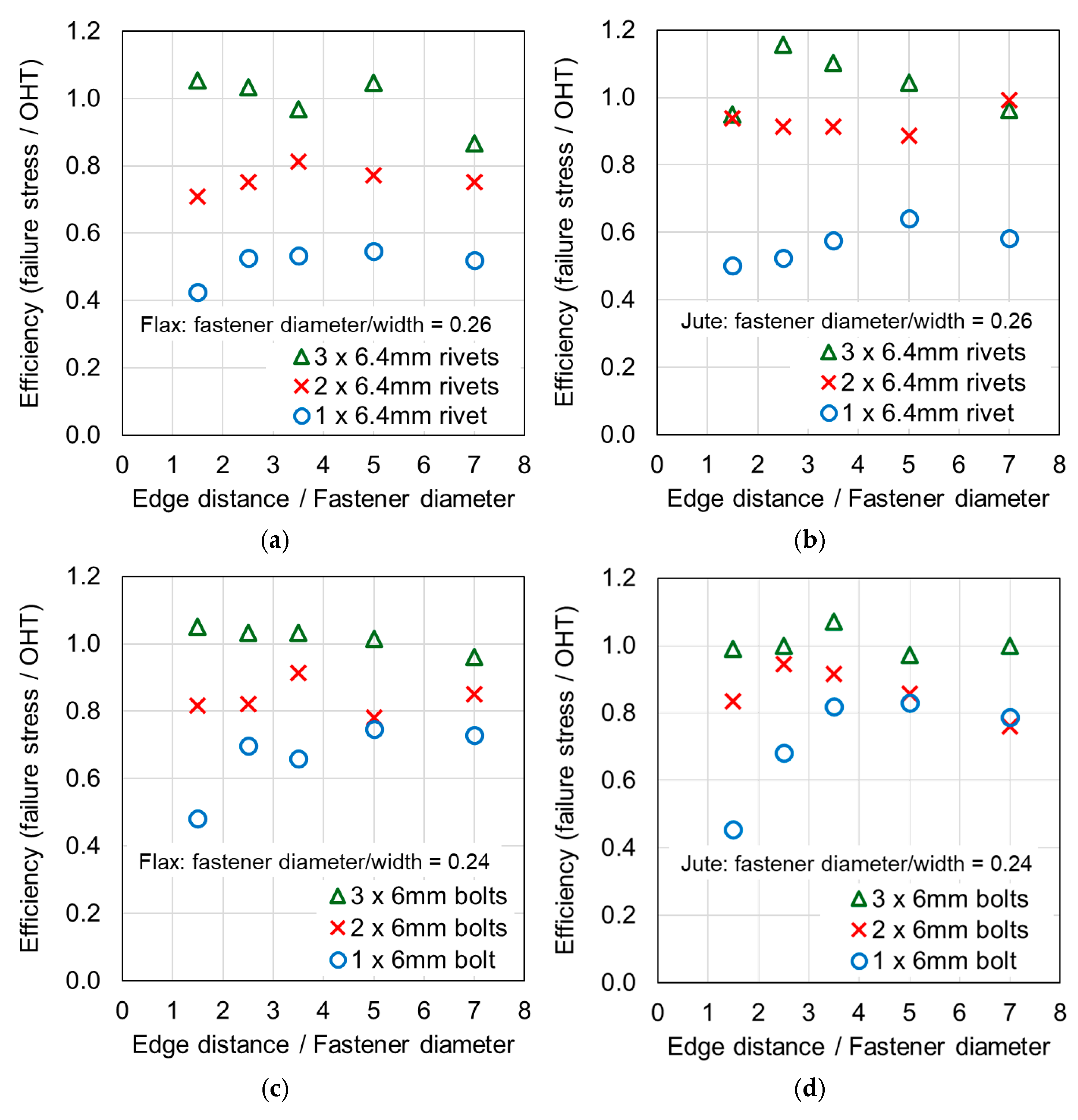
4.4. Effect of the Edge Distance
4.5. Effect of Tilting and Splaying
4.6. Comparisons of M6 and M4 Bolted Joints
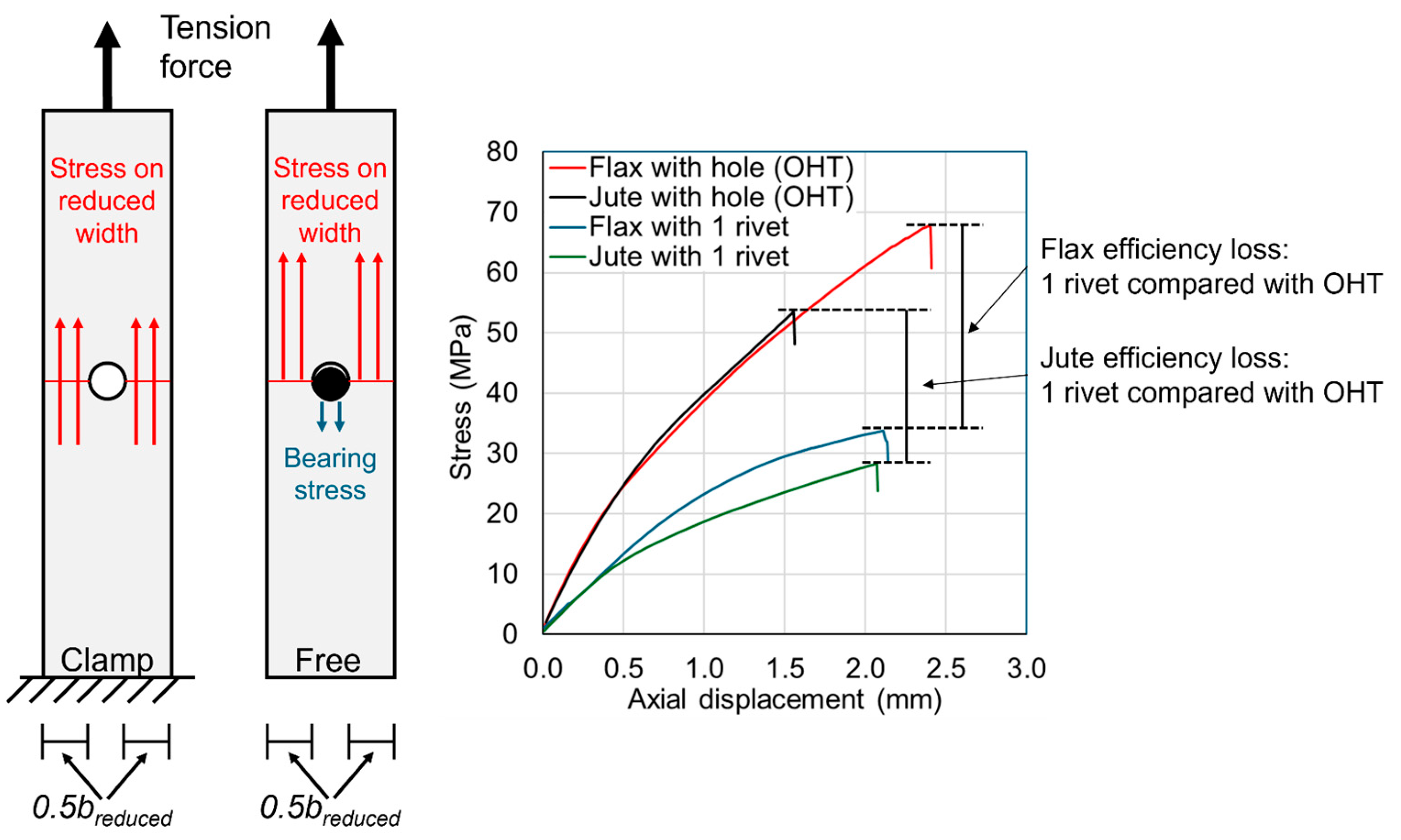
4.7. Comparisons of Bolted and Riveted Joints
4.8. Comparisons of the Present Study and Other Natural Fiber Composite Joint Studies
4.9. Comparisons of Joints of Natural and Synthetic Fiber Composites
5. Conclusions
- In single-fastener joints, the fastener should be located a distance of more than 2.5 times the fastener diameter from the free edge to reduce tear-out;
- In single-fastener joints, the fastener should be located a distance of less than seven times the fastener diameter from the free edge to reduce tilting and splaying;
- In single-fastener joints that follow Recommendations 1 and 2, bolts are more efficient fasteners than rivets;
- In single-fastener joints, joint efficiency increases with the increasing d/W ratio, and this ratio can be increased by either increasing the bolt diameter or decreasing the effective width (the width of the joined part that carries the bolt load);
- Multi-fastener joints are more efficient than single-fastener joints;
- Adding more fasteners (located along the line of action of the force), results in Recommendations 1 to 4 becoming less important, as the effects of edge distance, tilting and splaying, d/W ratio, and fastener type become less important to the joint efficiency;
- Adding more fasteners (located along the line of action of the force) improves joint efficiency until the OHT strength is reached, which acts as an upper bound to the joint strength;
- The OHT may be used as the design strength for multi-fastener joints with a sufficient number of fasteners (in the present study, three fasteners for a d/W ratio of 0.24).
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A

| Nominal | Nominal | Jute | Flax | Jute | Jute | Flax | Flax | Jute | Flax | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Fastener Type | Fastener Diameter | Number of Fasteners | Edge Distance /Diameter | Diameter /Width | Ultimate Force | Ultimate Force | Width | Ultimate Stress | Width | Ultimate Stress | Efficiency | Efficiency |
| (mm) | (e/d) | (d/W) | (kN) | (kN) | (mm) | (MPa) | (mm) | (MPa) | ||||
| Rivet | 6.4 | 1 | 1.5 | 0.14 | 2.1 | 2.1 | 45.5 | 15.8 | 45.5 | 15.5 | 0.30 | 0.25 |
| Rivet | 6.4 | 1 | 2.5 | 0.14 | 2.3 | 2.6 | 46.4 | 16.6 | 45.9 | 19.3 | 0.32 | 0.31 |
| Rivet | 6.4 | 1 | 3.5 | 0.14 | 2.4 | 2.5 | 45.5 | 18.1 | 45.0 | 18.9 | 0.35 | 0.30 |
| Rivet | 6.4 | 1 | 5 | 0.14 | 2.4 | 2.7 | 45.0 | 18.3 | 46.8 | 19.6 | 0.35 | 0.31 |
| Rivet | 6.4 | 1 | 7 | 0.14 | 2.4 | 2.8 | 45.5 | 18.1 | 45.5 | 20.7 | 0.35 | 0.33 |
| Rivet | 6.4 | 2 | 1.5 | 0.14 | 3.5 | 4.7 | 45.5 | 26.0 | 46.4 | 33.8 | 0.50 | 0.54 |
| Rivet | 6.4 | 2 | 2.5 | 0.14 | 4.3 | 5.2 | 46.4 | 31.5 | 45.0 | 39.3 | 0.61 | 0.63 |
| Rivet | 6.4 | 2 | 3.5 | 0.14 | 4.4 | 4.9 | 46.4 | 32.0 | 45.0 | 36.9 | 0.62 | 0.59 |
| Rivet | 6.4 | 2 | 5 | 0.14 | 4.0 | 5.1 | 46.8 | 28.8 | 45.0 | 38.6 | 0.56 | 0.62 |
| Rivet | 6.4 | 2 | 7 | 0.14 | 4.0 | 5.0 | 46.8 | 28.4 | 45.0 | 37.8 | 0.55 | 0.61 |
| Bolt | 6 | 1 | 1.5 | 0.13 | 1.6 | 1.9 | 44.1 | 12.3 | 45.9 | 13.8 | 0.24 | 0.22 |
| Bolt | 6 | 1 | 2.5 | 0.13 | 3.0 | 3.4 | 44.6 | 22.5 | 44.6 | 26.0 | 0.43 | 0.42 |
| Bolt | 6 | 1 | 3.5 | 0.13 | 3.9 | 4.3 | 44.1 | 30.1 | 46.8 | 30.7 | 0.58 | 0.49 |
| Bolt | 6 | 1 | 5 | 0.13 | 3.7 | 4.5 | 45.5 | 27.3 | 45.9 | 32.9 | 0.53 | 0.53 |
| Bolt | 6 | 1 | 7 | 0.13 | 3.5 | 4.3 | 46.8 | 25.2 | 46.4 | 31.5 | 0.49 | 0.50 |
| Bolt | 6 | 2 | 1.5 | 0.13 | 2.0 | 3.6 | 45.0 | 14.9 | 45.9 | 26.2 | 0.29 | 0.42 |
| Bolt | 6 | 2 | 2.5 | 0.13 | 4.8 | 6.3 | 46.8 | 34.5 | 44.1 | 48.8 | 0.66 | 0.78 |
| Bolt | 6 | 2 | 3.5 | 0.13 | 4.1 | 6.2 | 44.1 | 31.9 | 45.0 | 46.5 | 0.61 | 0.75 |
| Bolt | 6 | 2 | 5 | 0.13 | 4.4 | 6.3 | 44.1 | 33.7 | 45.5 | 46.8 | 0.65 | 0.75 |
| Bolt | 6 | 2 | 7 | 0.13 | 3.5 | 6.6 | 44.4 | 27.0 | 44.8 | 49.9 | 0.52 | 0.80 |
| Bolt | 4 | 1 | 1.5 | 0.09 | 1.2 | 1.3 | 46.4 | 8.2 | 45.9 | 9.0 | 0.16 | 0.14 |
| Bolt | 4 | 1 | 2.5 | 0.09 | 1.9 | 2.4 | 46.8 | 13.3 | 44.1 | 17.2 | 0.26 | 0.28 |
| Bolt | 4 | 1 | 3.5 | 0.09 | 2.2 | 2.8 | 44.1 | 16.0 | 45.0 | 19.8 | 0.31 | 0.32 |
| Bolt | 4 | 1 | 5 | 0.09 | 2.6 | 2.9 | 45.5 | 18.1 | 45.9 | 20.2 | 0.35 | 0.32 |
| Bolt | 4 | 1 | 7 | 0.09 | 2.3 | 2.8 | 45.0 | 16.5 | 45.9 | 19.6 | 0.32 | 0.31 |
| Bolt | 4 | 2 | 1.5 | 0.09 | 1.7 | 2.0 | 44.1 | 12.5 | 45.9 | 14.1 | 0.24 | 0.23 |
| Bolt | 4 | 2 | 2.5 | 0.09 | 3.5 | 4.3 | 44.6 | 25.1 | 46.4 | 29.4 | 0.48 | 0.47 |
| Bolt | 4 | 2 | 3.5 | 0.09 | 3.6 | 4.6 | 45.0 | 25.8 | 44.6 | 33.0 | 0.50 | 0.53 |
| Bolt | 4 | 2 | 5 | 0.09 | 4.0 | 4.8 | 44.6 | 28.5 | 45.5 | 33.9 | 0.55 | 0.54 |
| Bolt | 4 | 2 | 7 | 0.09 | 3.8 | 4.8 | 44.1 | 27.9 | 45.5 | 34.2 | 0.54 | 0.55 |
| Nominal | Nominal | Jute | Flax | Jute | Jute | Flax | Flax | Jute | Flax | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Fastener Type | Fastener Diameter | Number of Fasteners | Edge Distance /Diameter | Diameter /Width | Ultimate Force | Ultimate Force | Width | Ultimate Stress | Width | Ultimate Stress | Efficiency | Efficiency |
| (mm) | (e/d) | (d/W) | (kN) | (kN) | (mm) | (MPa) | (mm) | (MPa) | ||||
| Rivet | 6.4 | 1 | 1.5 | 0.26 | 1.7 | 1.7 | 25.5 | 26.1 | 25.8 | 26.6 | 0.50 | 0.43 |
| Rivet | 6.4 | 1 | 2.5 | 0.26 | 1.8 | 2.1 | 25.8 | 27.2 | 25.5 | 32.9 | 0.52 | 0.53 |
| Rivet | 6.4 | 1 | 3.5 | 0.26 | 2.0 | 2.2 | 25.8 | 29.9 | 25.5 | 33.3 | 0.58 | 0.53 |
| Rivet | 6.4 | 1 | 5 | 0.26 | 2.0 | 2.1 | 24.5 | 33.3 | 24.5 | 34.2 | 0.64 | 0.55 |
| Rivet | 6.4 | 1 | 7 | 0.26 | 2.0 | 2.1 | 26.0 | 30.2 | 25.3 | 32.5 | 0.58 | 0.52 |
| Rivet | 6.4 | 2 | 1.5 | 0.26 | 3.0 | 2.9 | 24.8 | 48.7 | 25.8 | 44.3 | 0.94 | 0.71 |
| Rivet | 6.4 | 2 | 2.5 | 0.26 | 3.0 | 2.9 | 25.0 | 47.4 | 24.5 | 46.9 | 0.91 | 0.75 |
| Rivet | 6.4 | 2 | 3.5 | 0.26 | 2.9 | 3.4 | 24.5 | 47.5 | 26.0 | 50.8 | 0.91 | 0.81 |
| Rivet | 6.4 | 2 | 5 | 0.26 | 2.9 | 3.2 | 25.0 | 46.0 | 25.8 | 48.2 | 0.89 | 0.77 |
| Rivet | 6.4 | 2 | 7 | 0.26 | 3.3 | 3.0 | 25.3 | 51.5 | 25.3 | 47.0 | 0.99 | 0.75 |
| Rivet | 6.4 | 3 | 1.5 | 0.26 | 2.8 | 4.1 | 25.3 | 43.9 | 25.0 | 65.8 | 0.95 | 1.05 |
| Rivet | 6.4 | 3 | 2.5 | 0.26 | 3.8 | 4.1 | 25.0 | 60.0 | 25.3 | 64.6 | 1.16 | 1.03 |
| Rivet | 6.4 | 3 | 3.5 | 0.26 | 3.6 | 4.0 | 25.0 | 57.2 | 26.0 | 60.5 | 1.10 | 0.97 |
| Rivet | 6.4 | 3 | 5 | 0.26 | 3.6 | 4.2 | 25.8 | 54.1 | 25.3 | 65.4 | 1.04 | 1.05 |
| Rivet | 6.4 | 3 | 7 | 0.26 | 3.3 | 3.6 | 26.0 | 50.0 | 25.8 | 54.2 | 0.96 | 0.87 |
| Bolt | 6 | 1 | 1.5 | 0.24 | 1.5 | 2.0 | 24.8 | 23.6 | 25.8 | 30.0 | 0.45 | 0.48 |
| Bolt | 6 | 1 | 2.5 | 0.24 | 2.4 | 2.7 | 26.0 | 35.4 | 24.5 | 43.6 | 0.68 | 0.70 |
| Bolt | 6 | 1 | 3.5 | 0.24 | 2.8 | 2.7 | 25.5 | 42.5 | 25.5 | 41.2 | 0.82 | 0.66 |
| Bolt | 6 | 1 | 5 | 0.24 | 2.8 | 3.0 | 25.8 | 43.1 | 25.5 | 46.6 | 0.83 | 0.75 |
| Bolt | 6 | 1 | 7 | 0.24 | 2.6 | 2.9 | 25.0 | 40.9 | 25.0 | 45.5 | 0.79 | 0.73 |
| Bolt | 6 | 2 | 1.5 | 0.24 | 2.7 | 3.1 | 24.8 | 43.3 | 24.5 | 51.0 | 0.83 | 0.82 |
| Bolt | 6 | 2 | 2.5 | 0.24 | 3.1 | 3.3 | 25.3 | 49.0 | 25.5 | 51.3 | 0.94 | 0.82 |
| Bolt | 6 | 2 | 3.5 | 0.24 | 3.2 | 3.5 | 26.0 | 47.6 | 24.5 | 57.1 | 0.92 | 0.91 |
| Bolt | 6 | 2 | 5 | 0.24 | 2.8 | 3.1 | 25.0 | 44.5 | 25.3 | 48.7 | 0.86 | 0.78 |
| Bolt | 6 | 2 | 7 | 0.24 | 2.5 | 3.2 | 25.0 | 39.4 | 24.5 | 53.1 | 0.76 | 0.85 |
| Bolt | 6 | 3 | 1.5 | 0.24 | 3.2 | 4.4 | 25.0 | 51.4 | 25.8 | 67.0 | 0.99 | 1.05 |
| Bolt | 6 | 3 | 2.5 | 0.24 | 3.4 | 4.2 | 25.5 | 51.8 | 25.5 | 64.5 | 1.00 | 1.03 |
| Bolt | 6 | 3 | 3.5 | 0.24 | 3.5 | 3.9 | 25.0 | 55.6 | 24.5 | 64.4 | 1.07 | 1.03 |
| Bolt | 6 | 3 | 5 | 0.24 | 3.3 | 3.9 | 25.8 | 50.4 | 24.8 | 63.4 | 0.97 | 1.02 |
| Bolt | 6 | 3 | 7 | 0.24 | 3.4 | 3.9 | 25.5 | 51.9 | 25.8 | 60.0 | 1.00 | 0.96 |
References
- Waszczak, J.P.; Cruse, T.A. Failure mode and strength predictions of anisotropic bolt bearing specimens. J. Compos. Mater. 1971, 5, 412–425. [Google Scholar] [CrossRef]
- Stockdale, J.H.; Matthews, F.L. The effect of clamping pressure on bolt bearing loads in glass fibre reinforced plastics. Composites 1976, 7, 34–39. [Google Scholar] [CrossRef]
- Duthinh, D. Connections of Fiber-Reinforced Polymer (FRP) Structural Members: A Review of the State of the Art; NIST Interagency/Internal Report (NISTIR); National Institute of Standards and Technology: Gaithersburg, MD, USA, 2000. Available online: https://tsapps.nist.gov/publication/get_pdf.cfm?pub_id=860284 (accessed on 5 May 2025).
- Gamdani, F.; Boukhili, R.; Vadean, A. Tensile strength of open-hole, pin-loaded and multi-bolted single-lap joints in woven composite plates. Mater. Des. 2015, 88, 702–712. [Google Scholar] [CrossRef]
- Gamdani, F.; Boukhili, R.; Vadean, A. Tensile behavior of hybrid multi-bolted/bonded joints in composite laminates. Int. J. Adhes. Adhes. 2019, 95, 102426. [Google Scholar] [CrossRef]
- Yun, J.H.; Choi, J.H.; Kweon, J.H. A study on the strength improvement of the multi bolted joint. Compos. Struct. 2014, 108, 409–416. [Google Scholar] [CrossRef]
- Feo, L.; Marra, G.; Mosallam, A.S. Stress analysis of multi-bolted joints for FRP pultruded composite structures. Compos. Struct. 2012, 94, 3769–3780. [Google Scholar] [CrossRef]
- Zhang, J.; Liu, F.; Zhao, L.; Fei, B. A novel characteristic curve for failure prediction of multi-bolt composite joints. Compos. Struct. 2014, 108, 129–136. [Google Scholar] [CrossRef]
- Summerscales, J.; Dissanayake, N.P.J.; Virk, A.S.; Hall, W. A review of bast fibers and their composites. Part 1—Fibers as reinforcements. Compos. Part A 2010, 41, 1329–1335. [Google Scholar] [CrossRef]
- Summerscales, J.; Dissanayake, N.P.J.; Virk, A.S.; Hall, W. A review of bast fibers and their composites. Part 2—Composites. Compos. Part A 2010, 41, 1336–1344. [Google Scholar] [CrossRef]
- Ku, H.; Wang, H.; Pattarachaiyakoop, N.; Trada, M. A review on the tensile properties of natural fiber reinforced polymer composites. Compos. Part B Eng. 2011, 42, 856–873. [Google Scholar] [CrossRef]
- Yan, L.B.; Chouw, N.; Jayaraman, K. Flax fibe and its composites—A review. Compos. Part B 2014, 56, 296–317. [Google Scholar] [CrossRef]
- Pickering, K.L.; Efendy, M.G.A.; Le, T.M. A review of recent developments in natural fiber composites and their mechanical performance. Compos. Part A 2016, 83, 98–112. [Google Scholar] [CrossRef]
- Faruk, O.; Bledzki, A.K.; Fink, H.-P.; Sain, M. Biocomposites reinforced with natural fibers: 2000–Prog. Polym. Sci. 2012, 37, 1552–1596. [Google Scholar]
- Dicker, M.P.M.; Duckworth, P.F.; Baker, A.B.; François, G.; Hazzard, M.K.; Weaver, P.M. Green composites: A review of material attributes and complementary applications. Compos. Part A Appl. Sci. Manuf. 2014, 56, 280–289. [Google Scholar] [CrossRef]
- Weclawski, B.T.; Fan, M.; Hui, D. Compressive behaviour of natural fiber composite. Compos. Part B 2014, 67, 183–191. [Google Scholar] [CrossRef]
- Costa, F.H.M.M.; D’Almeida, J.R.M. Effect of Water Absorption on the Mechanical Properties of Sisal and Jute Fiber Composites. Polym. Technol. Eng. 1999, 38, 1081–1094. [Google Scholar] [CrossRef]
- Hargitai, H.; Rácz, I.; Anandjiwala, R.D. Development of HEMP Fiber Reinforced Polypropylene Composites. J. Thermoplast. Compos. Mater. 2008, 21, 165–174. [Google Scholar] [CrossRef]
- Oksman, K. High quality flax fiber composites manufactured by the resin transfer moulding process. J. Reinf. Plast. Comp. 2001, 20, 621–627. [Google Scholar] [CrossRef]
- Da Silva, H.S.P.; Júnior, H.L.O.; Júnior, J.H.A.; Zattera, A.J.; Amico, S.C. Mechanical behavior and correlation between dynamic fragility and dynamic mechanical properties of curaua fiber composites. Polym. Compos. 2013, 35, 1078–1086. [Google Scholar] [CrossRef]
- Da Silva, L.V.; Júnior, J.H.A.; Angrizani, C.C.; Amico, S.C. Short beam strength of curaua, sisal, glass and hybrid composites. J. Reinf. Plast. Compos. 2012, 32, 197–206. [Google Scholar] [CrossRef]
- Bambach, M.R. Compression strength of natural fiber composite plates and sections of flax, jute and hemp. Thin Walled Struct. 2017, 119, 103–113. [Google Scholar] [CrossRef]
- Bambach, M.R. Geometric optimisation and compression design of natural fiber composite structural channel sections. Compos. Struct. 2018, 185, 549–560. [Google Scholar] [CrossRef]
- Bambach, M. Durability of natural fibre epoxy composite structural columns: High cycle compression fatigue and moisture ingress. Compos. Part C Open Access 2020, 2, 100013. [Google Scholar] [CrossRef]
- Shah, D.U.; Schubel, P.J.; Clifford, M.J. Can flax replace E-glass in structural composites? A small wind turbine blade case study. Compos. Part B Eng. 2013, 52, 172–181. [Google Scholar] [CrossRef]
- Yan, L.B.; Chouw, N. Crashworthiness characteristics of flax fiber reinforced epoxy tubes for energy absorption application. Mat. Des. 2013, 51, 629–640. [Google Scholar] [CrossRef]
- Yan, L.B.; Chouw, N. Behaviour and analytical modelling of natural flax fiber reinforced polymer tube encased coir fiber reinforced concrete composite column. J. Comp. Mater. 2013, 47, 2133–2148. [Google Scholar] [CrossRef]
- Mak, K.; Fam, A.; MacDougall, C. Flexural Behavior of Sandwich Panels with Bio-FRP Skins Made of Flax Fibers and Epoxidized Pine-Oil Resin. J. Compos. Constr. 2015, 19, 04015005. [Google Scholar] [CrossRef]
- Le Duigou, A.; Deux, J.-M.; Davies, P.; Baley, C. PLLA/Flax Mat/Balsa Bio-Sandwich Manufacture and Mechanical Properties. Appl. Compos. Mater. 2010, 18, 421–438. [Google Scholar] [CrossRef]
- Uddin, N.; Kalyankar, R. Manufacturing and Structural Feasibility of Natural Fiber Reinforced Polymeric Structural Insulated Panels for Panelized Construction. Int. J. Polym. Sci. 2011, 2011, 1–7. [Google Scholar] [CrossRef]
- Lansiaux, H.; Soulat, D.; Boussu, F.; Labanieh, A.R. Development and Multiscale Characterization of 3D Warp Interlock Flax Fabrics with Different Woven Architectures for Composite Applications. Fibers 2020, 8, 15. [Google Scholar] [CrossRef]
- Wahab, N.; Srinophakun, P.; Hussain, Q.; Chaimahawan, P. Performance of Concrete Confined with a Jute–Polyester Hybrid Fiber Reinforced Polymer Composite: A Novel Strengthening Technique. Fibers 2019, 7, 72. [Google Scholar] [CrossRef]
- Manaia, J.P.; Manaia, A.T.; Rodriges, L. Industrial Hemp Fibers: An Overview. Fibers 2019, 7, 106. [Google Scholar] [CrossRef]
- Choudhury, M.R.; Debnath, K. Experimental analysis of tensile and compressive failure load in single-lap bolted joint of green composites. Compos. Struct. 2019, 225, 111180. [Google Scholar] [CrossRef]
- Sajid, Z.; Karuppanan, S.; Shah, S.Z.H. Effect of Washer Size and Tightening Torque on Bearing Performance of Basalt Fiber Composite Bolted Joints. J. Nat. Fibers 2021, 19, 5910–5927. [Google Scholar] [CrossRef]
- Kaushik, D.; Singh, I. Comparative assessment of failure in single shear lap joints fabricated using various joining techniques. Eng. Fail. Anal. 2024, 162, 108332. [Google Scholar] [CrossRef]
- Malik, K.; Ahmad, F.; Gunister, E. Drilling Performance of Natural Fiber Reinforced Polymer Composites: A Review. J. Nat. Fibers 2022, 19, 4761–4779. [Google Scholar] [CrossRef]
- ASTM D5961; Standard Test Method for Bearing Response of Polymer Matrix Composite Laminates. ASTM International: West Conshohocken, PA, USA, 2023.
- ISO 527-4:2021; Part 4: Test Conditions for Isotropic and Orthotropic Fibre-Reinforced Plastic Composites. International Organization for Standardization: Geneva, Switzerland, 2021.
- O’Higgins, R.M.; McCarthy, M.A.; McCarthy, C.T. Comparison of open-hole tension characteristics of high strength glass and carbon fibre-reinforced composite materials. Compos. Sci. Technol. 2008, 68, 2770–2778. [Google Scholar] [CrossRef]
- Olmedo, A.; Santiuste, C.; Barbero, E. An analytical model for the secondary bending prediction in single-lap composite bolted-joints. Compos. Struct. 2014, 111, 354–361. [Google Scholar]
- Koppad, P.; Suryanarayana, R.S.; Reddy, N.; Sethuram, D. Experimental Studies on Mechanical and Failure Behaviour of Single Lap Joints of Woven Jute-Hemp Fabric Reinforced Polymeric Composite Laminates; SAE Technical Paper; No. 2024-01-1936; SAE International: Warrendale, PA, USA, 2024. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bambach, M.R. Tension Strength of Multi-Fastener, Single-Lap Joints in Flax and Jute Composite Plates Using Bolts or Rivets. Materials 2025, 18, 2180. https://doi.org/10.3390/ma18102180
Bambach MR. Tension Strength of Multi-Fastener, Single-Lap Joints in Flax and Jute Composite Plates Using Bolts or Rivets. Materials. 2025; 18(10):2180. https://doi.org/10.3390/ma18102180
Chicago/Turabian StyleBambach, Mike R. 2025. "Tension Strength of Multi-Fastener, Single-Lap Joints in Flax and Jute Composite Plates Using Bolts or Rivets" Materials 18, no. 10: 2180. https://doi.org/10.3390/ma18102180
APA StyleBambach, M. R. (2025). Tension Strength of Multi-Fastener, Single-Lap Joints in Flax and Jute Composite Plates Using Bolts or Rivets. Materials, 18(10), 2180. https://doi.org/10.3390/ma18102180





