Discussion on the Gradation and Interface Effects on the Dynamic Mechanical Behaviors of Hydraulic Concrete Based on Meso-Mechanical Simulation
Abstract
1. Introduction
2. Materials and Methods
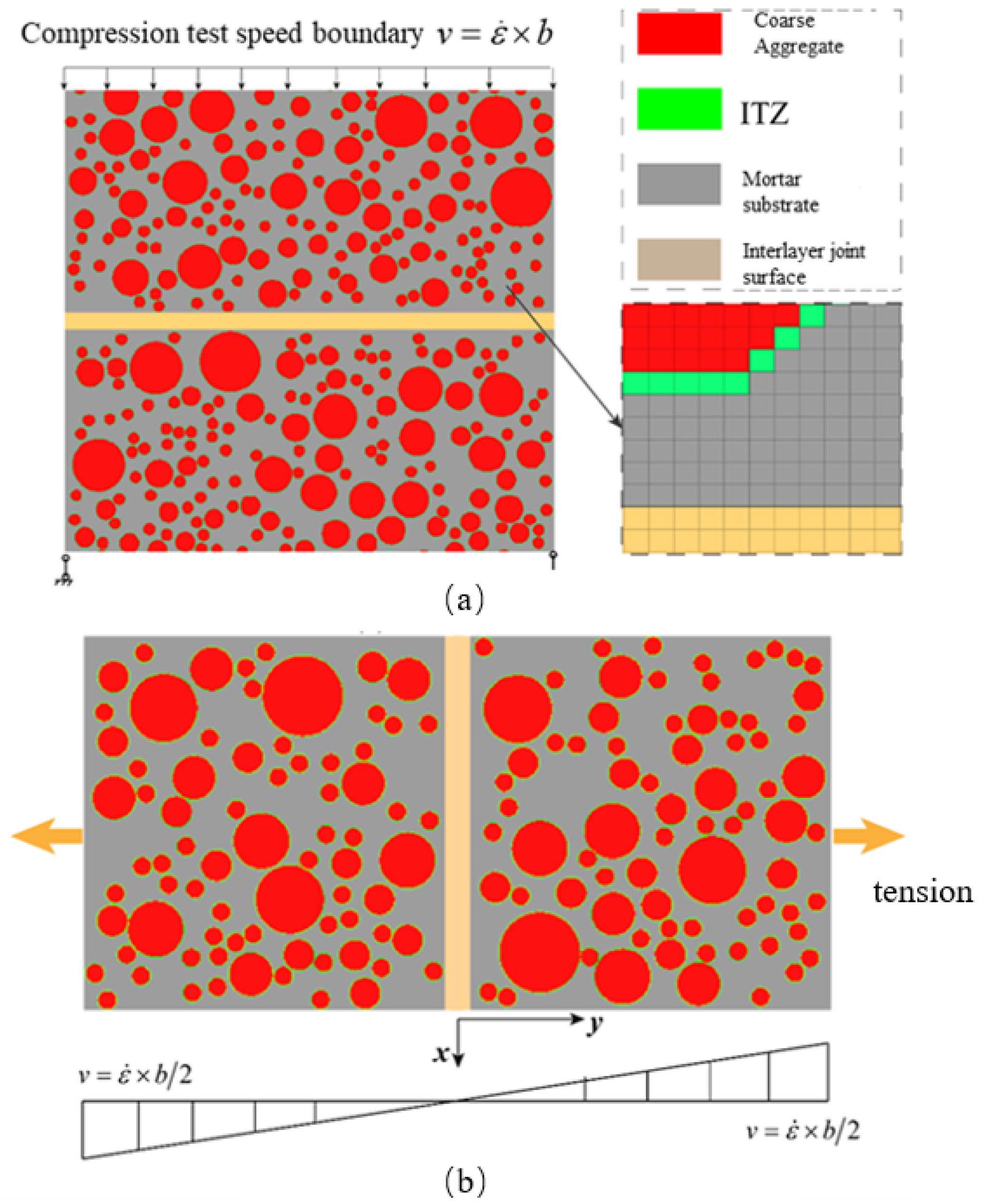
2.1. Simulation Method
2.2. Constitutive Model and Parameter Determination
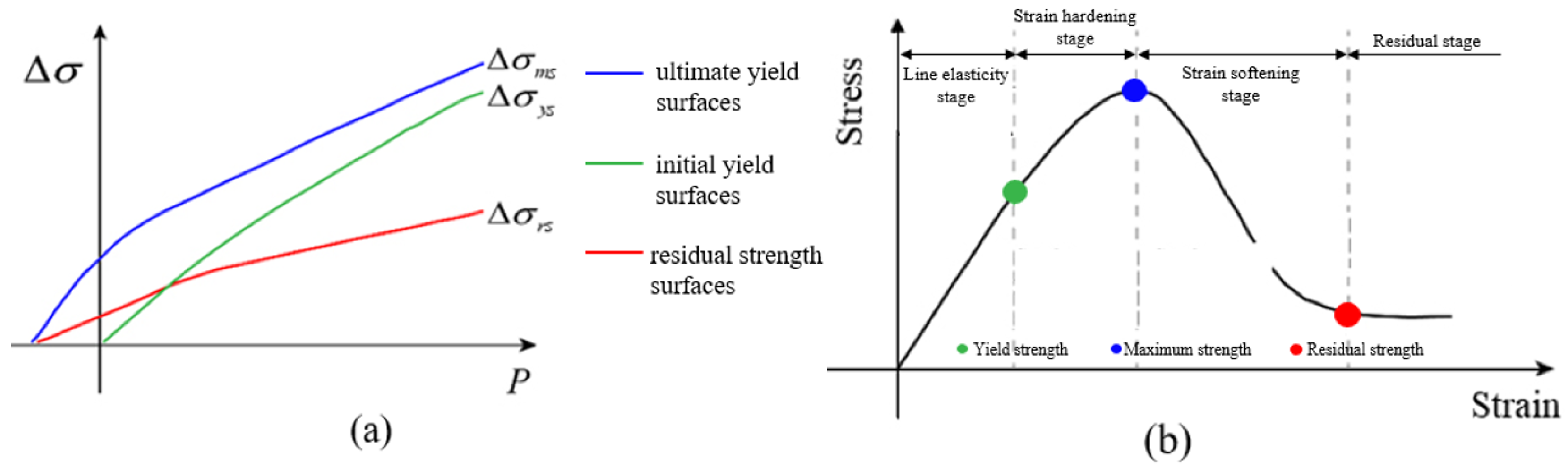
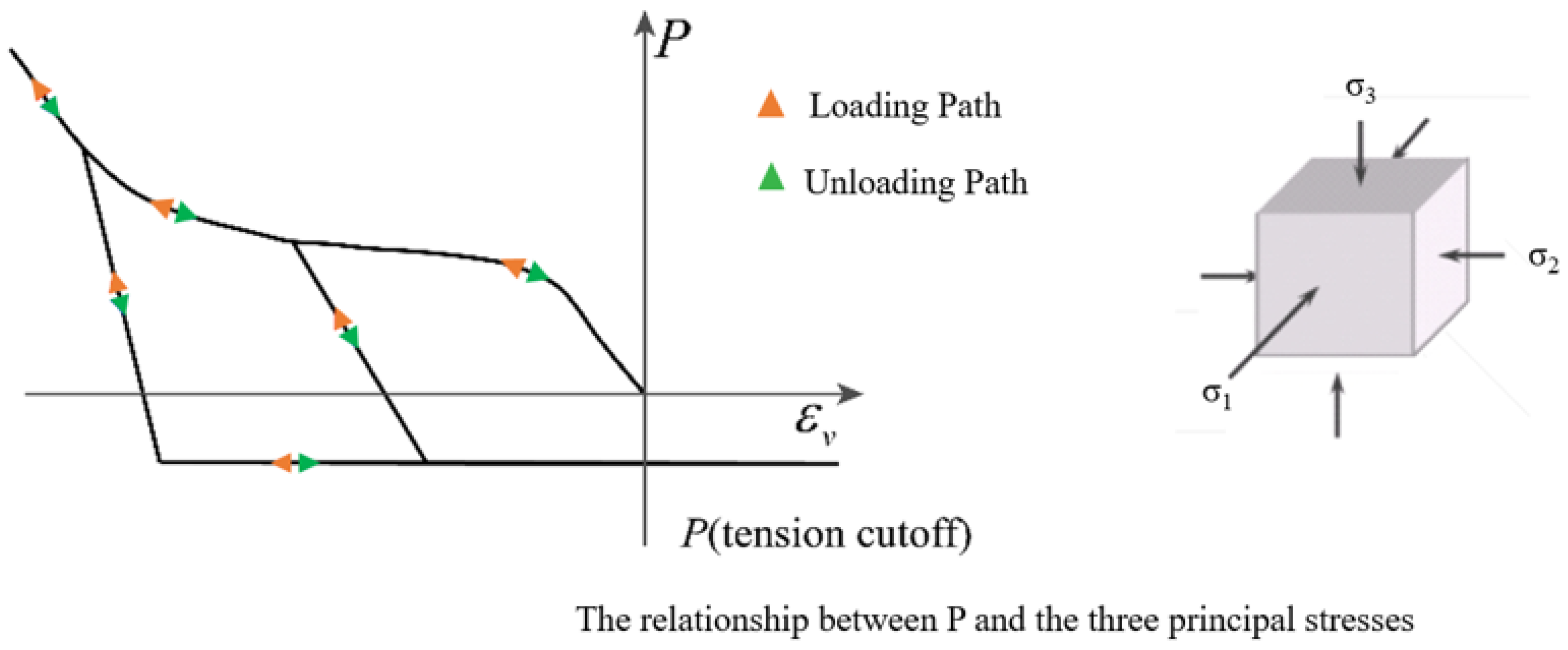
2.2.1. Concrete K&C Damage Model
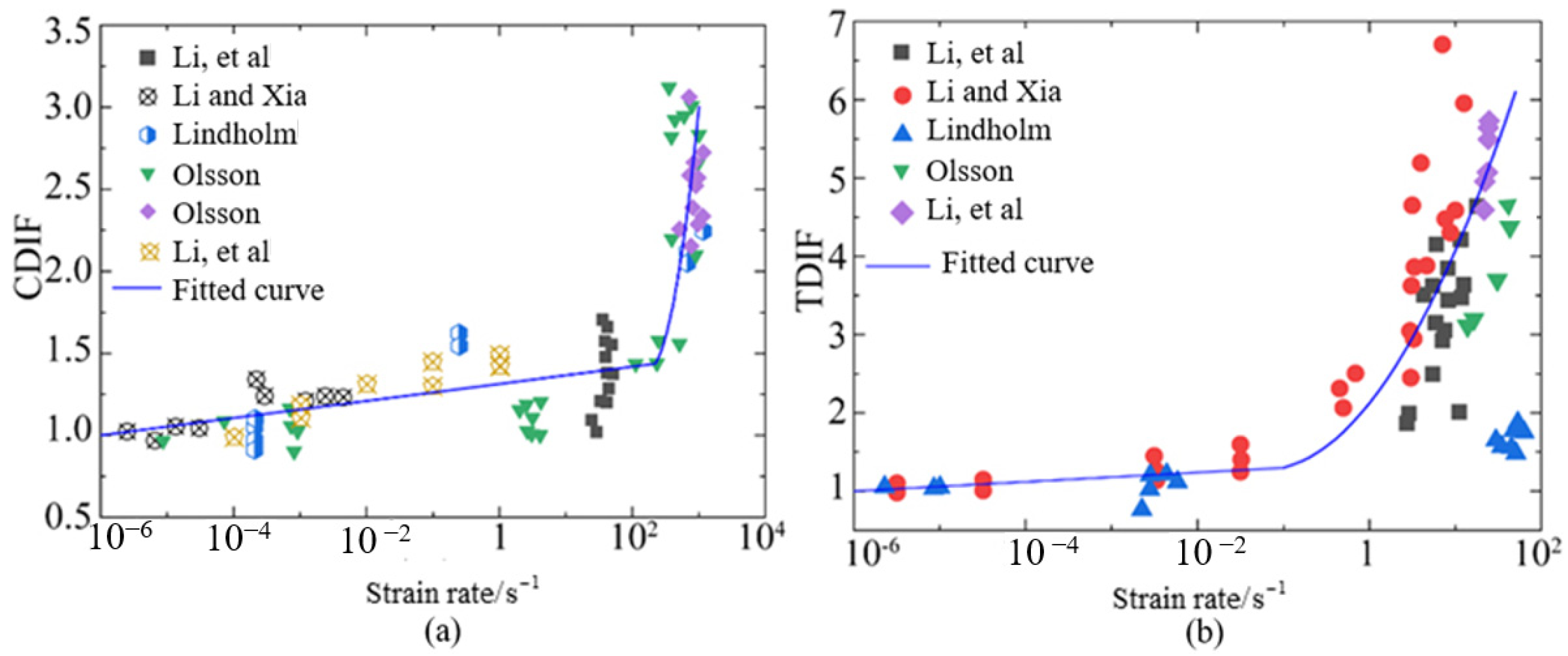
2.2.2. The Strain Rate Effect
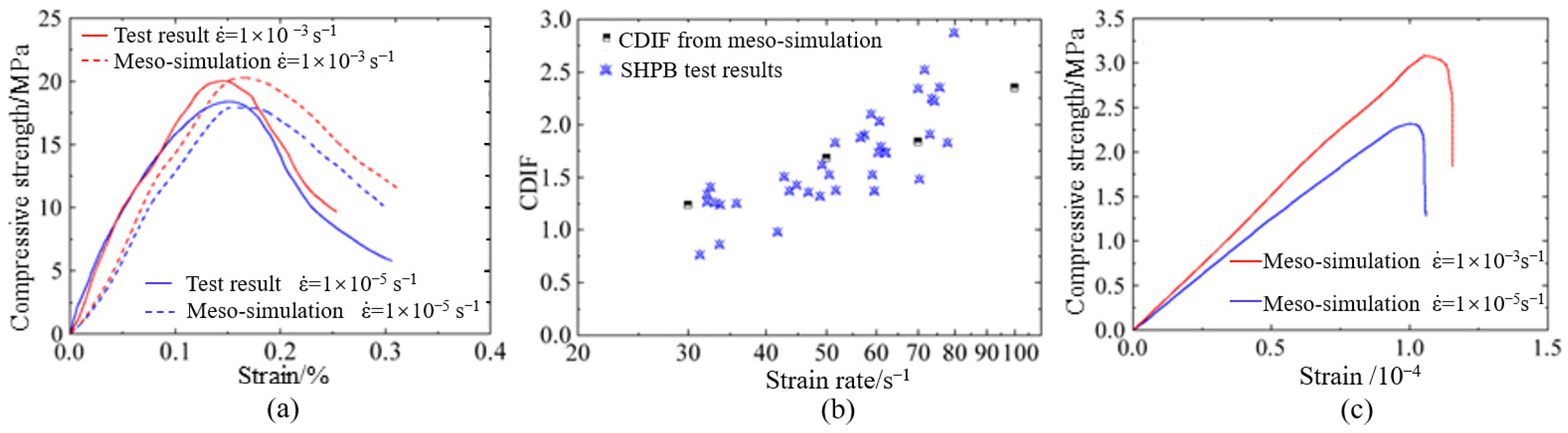
2.3. Verification of Simulation Model
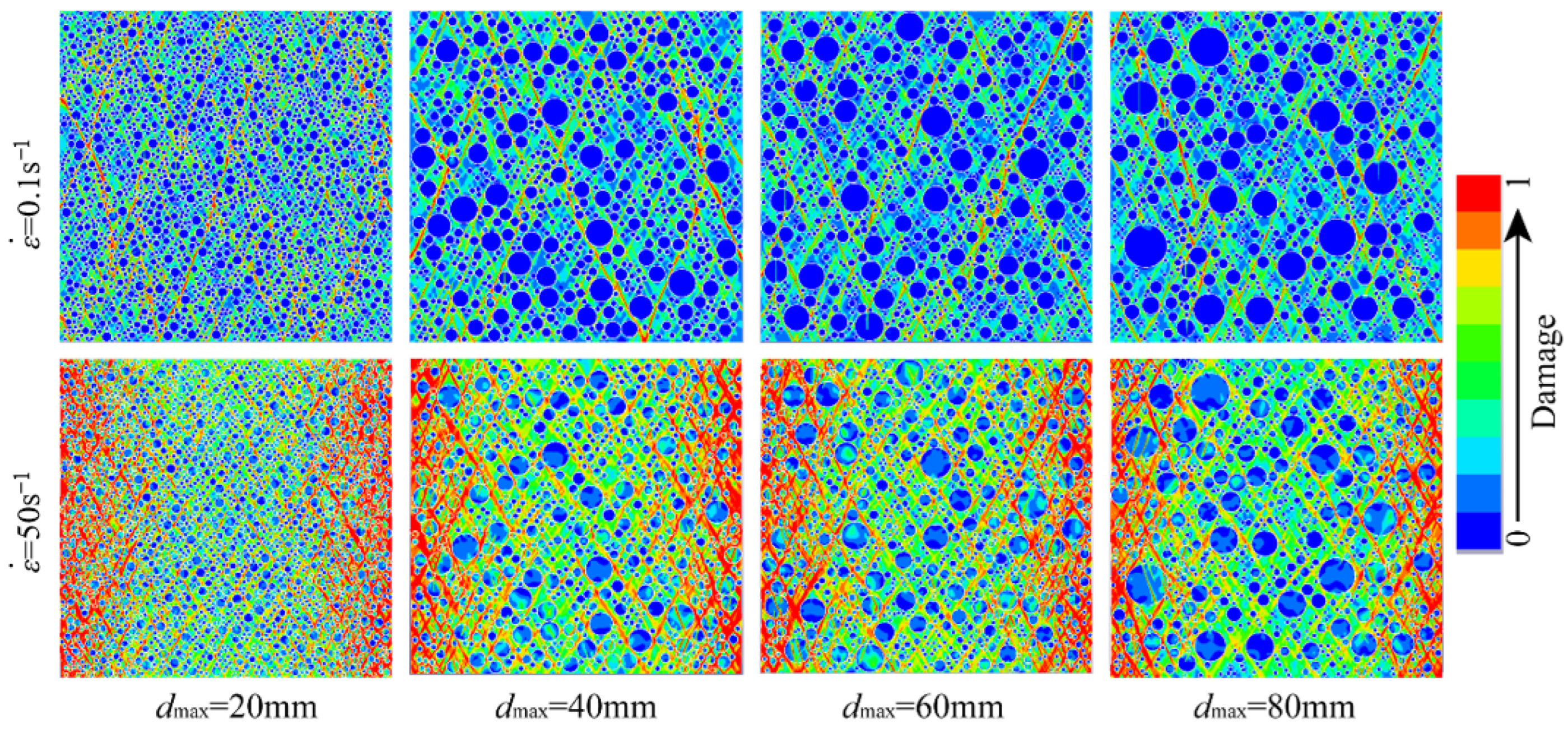
3. Aggregate Gradation Effect on the Dynamic Strength of Hydraulic Concrete
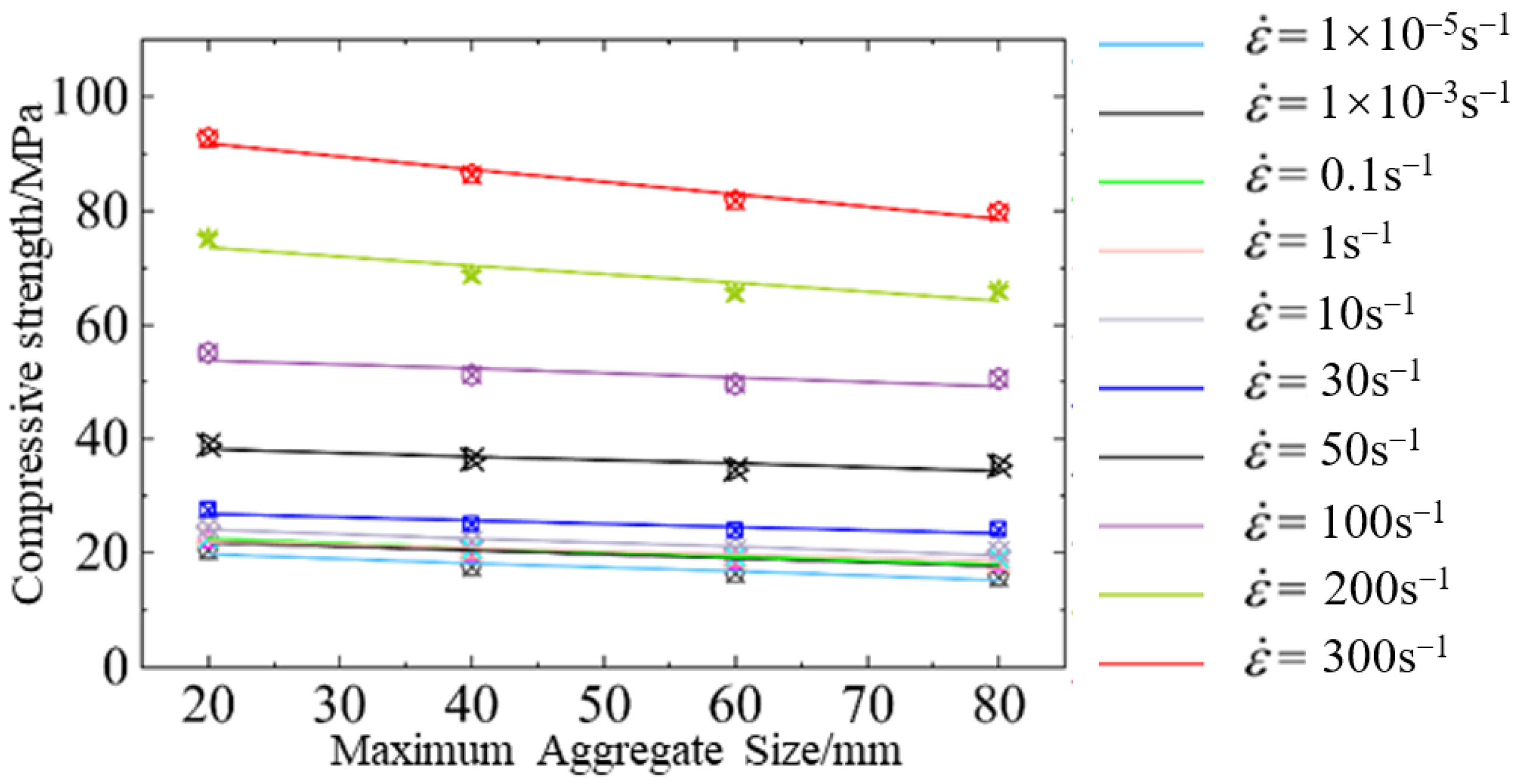
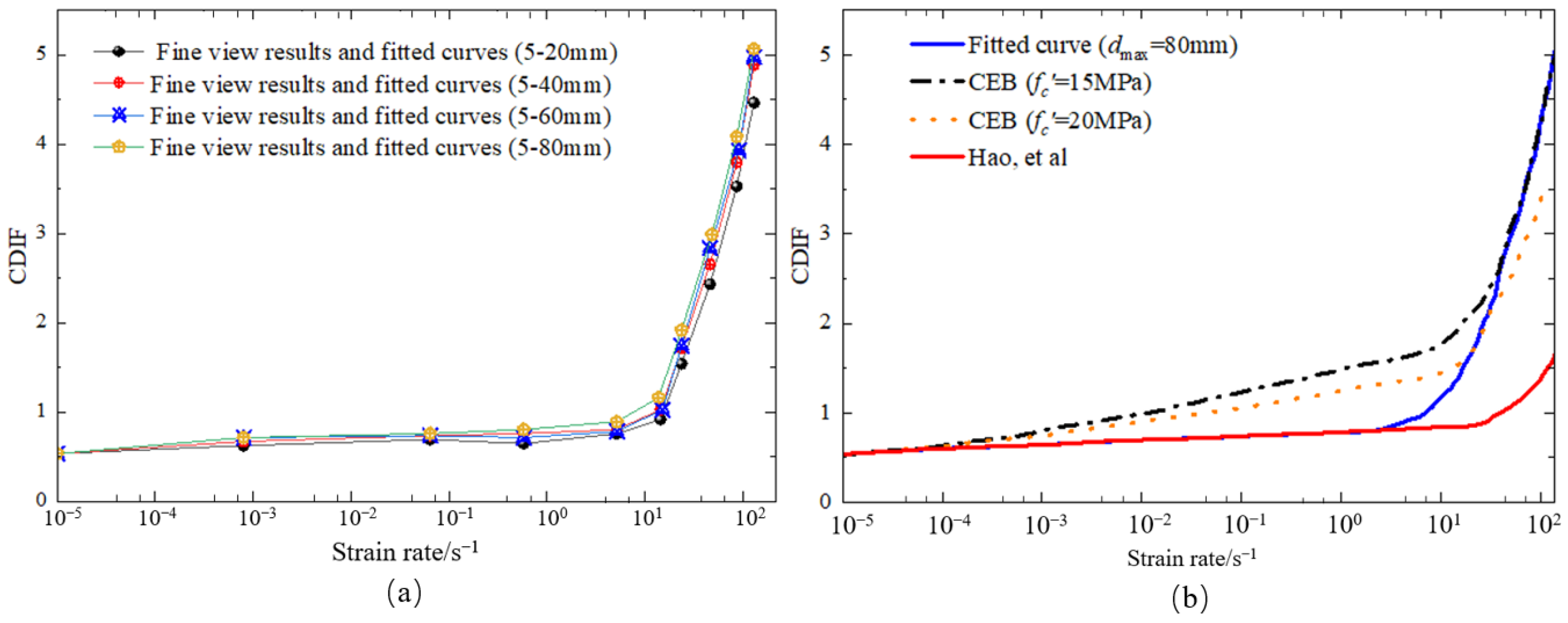
3.1. Aggregate Gradation Effect on the Dynamic Compressive Strength
3.2. Aggregate Gradation Effect on the Dynamic Tensile Strength
4. Interface Effect on the Dynamic Strength of Hydraulic Concrete
4.1. Interface Effect on the Dynamic Compressive Strength
4.2. Interface Effect on the Dynamic Tensile Strength
5. Conclusions
- (1)
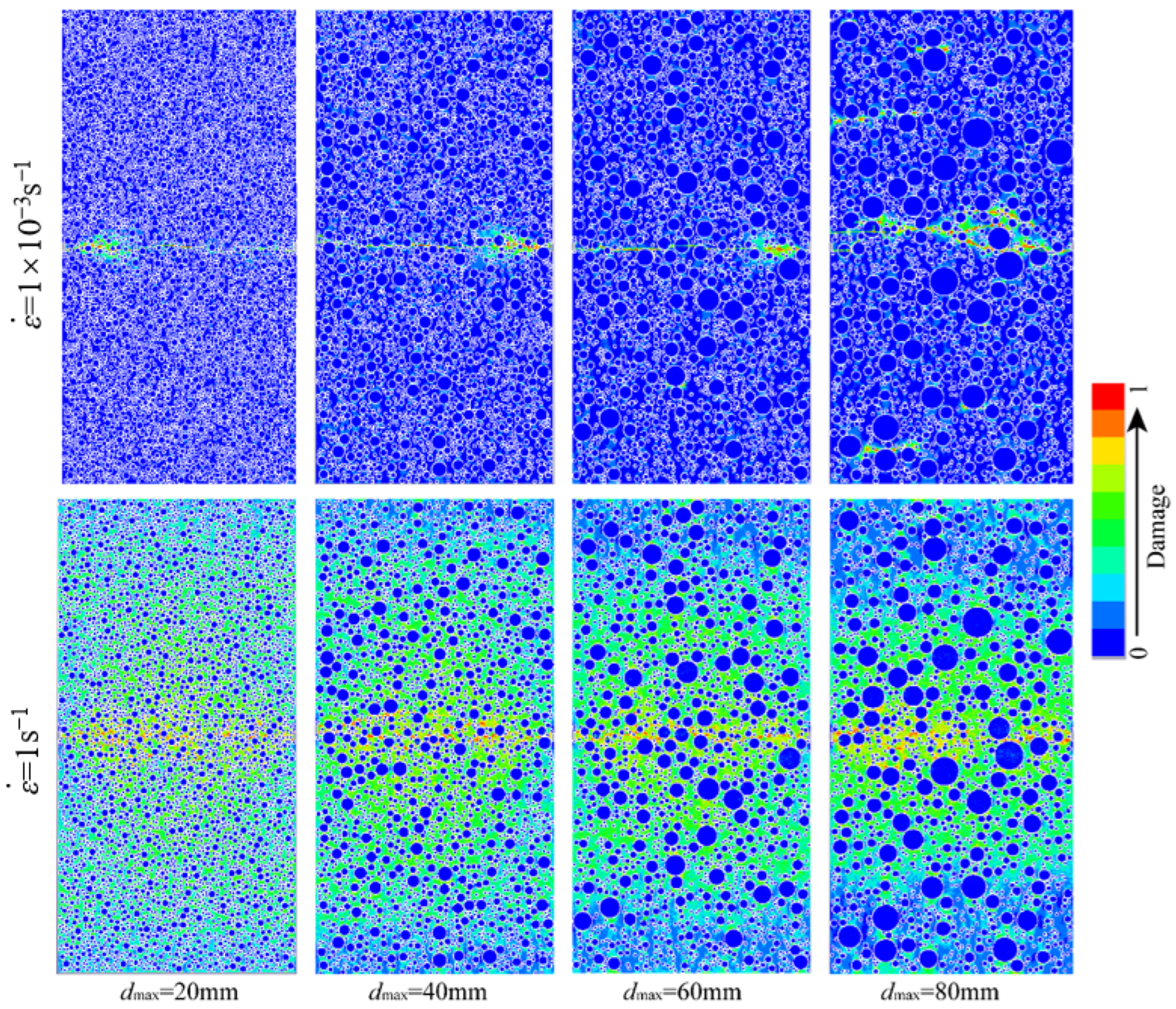
- The aggregate gradation usually had a significant influence on the failure patterns of hydraulic concrete under dynamic compressive and tensile loads, but the interface effect on the failure patterns was not obvious under dynamic loads. Moreover, as the loading rate increased, the stressing uniformity among different material components became better under both compressive and tensile loads. The stress distribution of low-gradation concrete was more uniform than that of high-gradation no matter what the loading rate was. This phenomenon was attributed to the excessive loading rate, which retarded the micro-cracks propagate along the weakest path and resulted in the more uniform stress distribution within the hydraulic concrete. Moreover, the damage expansion of concrete could be effectively restricted by the large aggregates due to the pronounced strength difference between coarse aggregates and cement matrix.
- (2)
- The dynamic compressive and tensile strengths of hydraulic concrete demonstrated a pronounced strain rate effect, which was independent of aggregate gradation. However, under the same loading conditions, the dynamic compressive and tensile strength progressively diminished with the enlargement of coarse aggregates, with this trend being particularly pronounced at higher loading rates. A rational reason for this observation lies in the fact that larger aggregates acted as barriers that interrupted the damage expansion and the crack propagation. Furthermore, empirical formulae were proposed in this study to describe the strain rate effect on compressive and tensile strength of hydraulic concrete considering the unique aggregate gradation. Thus, the effect of aggregate gradation on the design parameters of hydraulic concrete structures under dynamic loading conditions could be generalized as an increase in the design parameters for engineering applications.
- (3)
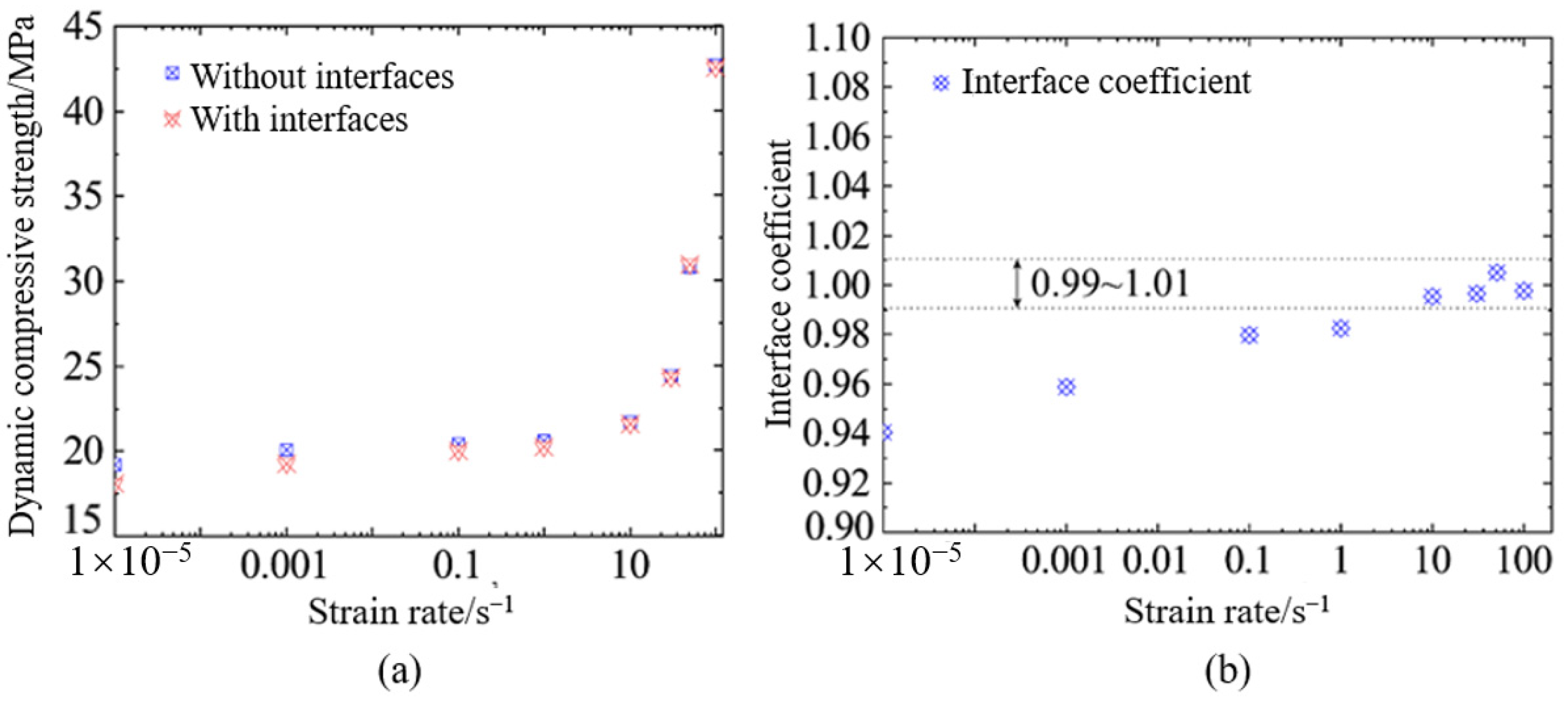
- The strength attenuation induced by weak-bonding interface was obvious at a low loading rate and decreased with the increasing loading rate. Moreover, it was obvious that the interface effect on dynamic tensile strength was more significant than that on dynamic compressive strength. When the interface coefficient was introduced to quantitatively assess the interface effect, it was obvious that the interface effect on dynamic compressive and tensile strength was strain rate-sensitive. However, the interface effect on the dynamic tensile strength of hydraulic concrete could gradually diminish at sufficiently high strain rates. Thus, in engineering applications, the interface effect on the design parameters of hydraulic concrete structures under dynamic loading conditions can be disregarded.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Rao, M.; Yang, H.; Lin, Y.; Li, J.; Shi, Y. Influence of maximum aggregate sizes on the performance of RCC. Constr. Build. Mater. 2016, 115, 42–47. [Google Scholar] [CrossRef]
- Hao, Y.F.; Hao, H.; Jiang, G.P.; Zhou, Y. Experimental confirmation of some factors influencing dynamic concrete compressive strengths in high-speed impact tests. Cem. Concr. Res. 2013, 52, 63–70. [Google Scholar] [CrossRef]
- Hao, Y.F.; Hao, H. Numerical Evaluation of the Influence of Aggregates on Concrete Compressive Strength at High Strain Rate. Int. J. Prot. Struct. 2011, 2, 177–206. [Google Scholar] [CrossRef]
- Wang, C.; Chen, W.; Hao, H.; Zhang, S.; Song, R.; Wang, X. Experimental investigations of dynamic compressive properties of roller compacted concrete (RCC). Constr. Build. Mater. 2018, 168, 671–682. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, W.; Wang, J.; Huang, R.; Lou, G.; Luo, S. Effects of coarse aggregate size on thickness and micro-properties of ITZ and the mechanical properties of concrete. Cem. Concr. Compos. 2024, 154, 105777. [Google Scholar] [CrossRef]
- Albarwary, I.H.M.; Aldoski, Z.N.S.; Askar, L.K. Effect of aggregate maximum size upon compressive strength of concrete. J. Duhok Univ. 2017, 20, 790–797. [Google Scholar] [CrossRef]
- Mkpaidem, N.; Ambrose, E.; Olutoge, F.; Afangideh, C. Effect of coarse aggregate size and gradation on workability and compressive strength of plain concrete. Appl. Sci. Environ. Manag. 2022, 26, 719–723. [Google Scholar] [CrossRef]
- Wang, X.-H.; Zhang, S.-R.; Wang, C.; Liu, F.-C.; Song, R.; Wei, P.-Y. Initial damage effect on dynamic compressive behaviors of roller compacted concrete (RCC) under impact loadings. Constr. Build. Mater. 2018, 186, 388–399. [Google Scholar] [CrossRef]
- Wang, X.-H.; Zhang, S.-R.; Wang, C.; Song, R.; Shang, C.; Cao, K.-L. Fragmentation-based dynamic size effect of layered roller compacted concrete (RCC) under impact loadings. Constr. Build. Mater. 2018, 192, 58–69. [Google Scholar] [CrossRef]
- Zhang, S.-R.; Wang, X.-H.; Wang, C.; Song, R.; Huo, H.-Y. Compressive behavior and constitutive model for roller compacted concrete under impact loading: Considering vertical stratification. Constr. Build. Mater. 2017, 151, 428–440. [Google Scholar] [CrossRef]
- He, Z.; Deng, H.; Fan, F.; Tan, J. Microstructure of four-graded roller compacted concrete. Constr. Build. Mater. 2018, 187, 25–37. [Google Scholar] [CrossRef]
- Lin, Y.Q.; Shi, Y.; Guo, D.M.; Li, J.Z.; Yang, H.Q. Study on Site Construction Technology of Four-Graded RCC. Adv. Mater. Res. 2011, 250–253, 2927–2930. [Google Scholar] [CrossRef]
- Jiang, J.-F.; Xiao, P.-C.; Li, B.-B. True-triaxial compressive behaviour of concrete under passive confinement. Constr. Build. Mater. 2017, 156, 584–598. [Google Scholar] [CrossRef]
- Chen, C.; Zhang, X.; Hao, H.; Cui, J. Discussion on the suitability of dynamic constitutive models for prediction of geopolymer concrete structural responses under blast and impact loading. Int. J. Impact Eng. 2022, 160, 104064. [Google Scholar] [CrossRef]
- Li, B.; Dai, S.; Zhan, Y.; Xu, J.; Guo, X.; Yang, Y.; Chen, Y. Strength criterion of recycled aggregate concrete under triaxial Compression: Model calibration. Constr. Build. Mater. 2022, 320, 126201. [Google Scholar] [CrossRef]
- Zhang, S.; Wei, P.; Wang, C.; Wang, G.; Lu, W.; Cao, K. Failure criteria calibration based on the triaxial compression behavior of roller compacted concrete (RCC). Mater. Struct. 2021, 54, 7. [Google Scholar] [CrossRef]
- Deng, R. Mechanical properties of roller compacted concrete. J. Southwest Jiaotong Univ. 2002, 1, 19–25. [Google Scholar]
- Gimenes, M.; Rodrigues, E.A.; Bitencourt, L.A.; Manzoli, O.L. 2D mesoscale modeling of compressive fracture in concrete using a mesh fragmentation technique. Int. J. Solids Struct. 2023, 260–261, 112031. [Google Scholar] [CrossRef]
- Grassl, P. 3D lattice meso-scale modelling of the effect of lateral compression on tensile fracture processes in concrete. Int. J. Solids Struct. 2023, 262–263, 112086. [Google Scholar] [CrossRef]
- Li, H.; Huang, Y.; Yang, Z.; Yu, K.; Li, Q. 3D meso-scale fracture modelling of concrete with random aggregates using a phase-field regularized cohesive zone model. Int. J. Solids Struct. 2022, 256, 111960. [Google Scholar] [CrossRef]
- Hao, Y.; Hao, H.; Li, Z. Influence of end friction confinement on impact tests of concrete material at high strain rate. Int. J. Impact Eng. 2013, 60, 82–106. [Google Scholar] [CrossRef]
- Hao, Y.; Hao, H.; Li, Z. Numerical analysis of lateral inertial confinement effects on impact test of concrete compressive material properties. Int. J. Prot. Struct. 2010, 1, 145–167. [Google Scholar] [CrossRef]
- Tian, R. Study on the Static, Dynamic Mechanical Properties of (Fully-Graded) Concrete Based on Mesomechanics. Master’s Thesis, Beijing University of Technology, Beijing, China, 2008. [Google Scholar]
- Zhou, Y.; Jin, H.; Wang, B. Modeling and mechanical influence of meso-scale concrete considering actual aggregate shapes. Constr. Build. Mater. 2019, 228, 116785. [Google Scholar] [CrossRef]
- Fang, Q.; Zhang, J.; Huang, Y.; Zhang, Y. Investigation into three-dimensional mesoscale modelling of fully-graded concrete. Eng. Mech. 2013, 30, 14–21+30. [Google Scholar]
- Jin, L.; Yang, W.; Yu, W.; Du, X. Influence of aggregate size on the dynamic tensile strength and size effect of concrete. J. Vib. Shock 2022, 39, 24–34. [Google Scholar]
- Niu, Y.; Wang, W.; Su, Y.; Jia, F.; Long, X. Plastic damage prediction of concrete under compression based on deep learning. Acta Mech. 2024, 235, 255–266. [Google Scholar] [CrossRef]
- Wang, C.; Qiu, Q.; Wang, X.; Zhang, S.; Wang, G.; Wei, P. Concrete Aggregate-Gradation Effect and Strength-Criterion Modification for Fully Graded Hydraulic Concrete. Materials 2024, 17, 3816. [Google Scholar] [CrossRef]
- Jia, J.-Y.; Gu, X.-L. Effects of coarse aggregate surface morphology on aggregate-mortar interface strength and mechanical properties of concrete. Constr. Build. Mater. 2021, 294, 123515. [Google Scholar] [CrossRef]
- Jin, L.; Yu, W.; Du, X.; Yang, W.-X. Meso-scale simulations of size effect on concrete dynamic splitting tensile strength: Influence of aggregate content and maximum aggregate size. Eng. Fract. Mech. 2020, 230, 106979. [Google Scholar] [CrossRef]
- Du, M.; Jin, L.; Li, D.; DU, X.L. Mesoscopic simulation study of the influence of aggregate size on mechanical properties and specimen size effect of concrete subjected to splitting tensile loading. Eng. Mech. 2017, 34, 54–63. (In Chinese) [Google Scholar]
- Liu, J.I.N.; Wangxian, Y.A.N.G.; Wenxuan, Y.U.; Xiuli, D.U. lnfluence of Maximum Aggregate Size on Dynamic Size Effect of Concrete Under Low Strain Rates: Meso-scale Simulations. Trans. Nanjing Univ. Aeronaut. Astronaut. 2020, 37, 27–39. [Google Scholar]
- Yu, W.-X. Meso-Scale Simulation in Dynamic Size Effect on Compressive and Tensile Failure of Concrete Materials. Master’s Thesis, School of Civil Engineering and Architecture, Beijing University of Technology, Beijing, China, 2019. (In Chinese). [Google Scholar]
- Jin, L.; Yu, W.; Du, X.; Yang, W. Dynamic size effect of concrete under tension: A numerical study. Int. J. Impact Eng. 2019, 132, 103318. [Google Scholar] [CrossRef]
- Jin, L.; Yu, W.; Du, X.; Yang, W.-X. Mesoscopic numerical simulation of dynamic size effect on the splitting-tensile strength of concrete. Eng. Fract. Mech. 2019, 209, 317–332. [Google Scholar] [CrossRef]
- Liu, J.; Wenxuan, Y.; Xiuli, D.U.; Zhang, S.; Dong, L.I. Meso-scale modelling of the size effect on dynamic compressive failure of concrete under different strain rates. Int. J. Impact Eng. 2019, 125, 1–12. [Google Scholar]
- Walraven, J.C. Fundamental analysis of aggregate interlock. J. Struct. Div. 1981, 107, 2245–2270. [Google Scholar] [CrossRef]
- Song, Z.; Lu, Y. Mesoscopic analysis of concrete under excessively high strain rate compression and implications on interpretation of test data. Int. J. Impact Eng. 2012, 46, 41–55. [Google Scholar] [CrossRef]
- Liu, G.; Hao, J. Simplified numerical method in stress analysis of RCC arch dam with layered structure. J. Tsinghua Univ. 1996, 36, 27–33. (In Chinese) [Google Scholar]
- Gu, C.S.; Song, J.X.; Fang, H.T. Analysis Model on Gradual Change Principle of Effect Zones of Layer Face for RCCD. Appl. Math. Mech. 2006, 27, 1335–1340. (In Chinese) [Google Scholar] [CrossRef]
- Peng, Y.J.; Li, B.K.; Liu, B. Numerical simulation of meso-level mechanical properties of roller compacted concrete. J. Hydraul. Eng. 2001, 6, 19–22. (In Chinese) [Google Scholar]
- Qu, Y.; Peng, Y.; Li, D. Numerical Simulation for Size Effect on Shear Strength of Roller Compacted Concrete Specimen. J. Water Resour. Archit. Eng. 2007, 05, 22–24. (In Chinese) [Google Scholar]
- Naderi, S.; Zhang, M. Meso-scale modelling of static and dynamic tensile fracture of concrete accounting for real-shape aggregates. Cem. Concr. Compos. 2021, 116, 103889. [Google Scholar] [CrossRef]
- Wu, Y.; Crawford, J.E. Crawford, Numerical modeling of concrete using a partially associative plasticity model. J. Engineering Mech. 2015, 141, 12. [Google Scholar]
- Hao, H.; Zhou, X. Concrete material model for high rate dynamic analysis. In Proceedings of the 7th International Conference on Shock and Impact Loads on Structures, Beijing, China, 17–19 October 2007; pp. 753–768. [Google Scholar]
- Li, X.; Lok, T.; Zhao, J. Dynamic characteristics of granite subjected to intermediate loading rate. Rock Mech. Rock Eng. 2005, 38, 21–39. [Google Scholar] [CrossRef]
- Li, Y.; Xia, C. Time-dependent tests on intact rocks in uniaxial compression. Int. J. Rock Mech. Min. Sci. 2000, 37, 467–475. [Google Scholar] [CrossRef]
- Lindholm, U.; Yeakley, L.; Nagy, A. The dynamic strength and fracture properties of dresser basalt. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1974, 11, 181–191. [Google Scholar] [CrossRef]
- Olsson, W. The compressive strength of tuff as a function of strain rate from 10-6 to 103/sec. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1991, 28, 115–118. [Google Scholar] [CrossRef]
- Li, H.B.; Zhao, J.; Li, J.R.; Liu, Y.Q.; Zhou, Q.C. Experimental studies on the strength of different rock types under dynamic compression. Int. J. Rock Mech. Min. Sci. 2004, 41, 01–06. [Google Scholar] [CrossRef]
- Wang, X.H.; Zhang, S.R.; Wang, C.; Song, R.; Shang, C.; Fang, X. Experimental investigation of the size effect of layered roller compacted concrete (RCC) under high-strain-rate loading. Constr. Build. Mater. 2018, 165, 45–57. [Google Scholar] [CrossRef]
- Peng, Y.; Li, B.; Qu, Y. Numerical Simulation of Shear Test of Specimen Roller Compacted Concrete on Me-so-Level. J. Univ. Hydraul. Electr. Eng. 2003, 25, 492–494+561. (In Chinese) [Google Scholar]
- Liu, P. Experimental Research and Numerical Analysis on Dynamic Mechanical Properties of Concrete. Master’s Thesis, School of Civil Engineering and Architecture, Dalian University, Dalian, China, 2011. (In Chinese). [Google Scholar]
- Lu, D.; Wang, G.; Du, X.; Wang, Y. A nonlinear dynamic uniaxial strength criterion that considers the ultimate dynamic strength of concrete. Int. J. Impact Eng. 2017, 103, 124–137. [Google Scholar] [CrossRef]
- Zhang, K.-H.; Wang, H.-B.; Tu, J.; Li, C.-L.; Zhong, H. Dynamic tensile test of fully-graded roller compacted concrete. J. China Inst. Water Resour. Hydropower Res. 2021, 19, 290–300. (In Chinese) [Google Scholar]
- Zhou, X.; Hao, H. Modelling of compressive behaviour of concrete-like materials at high strain rate. Int. J. Solids Struct. 2008, 45, 4648–4661. [Google Scholar] [CrossRef]
- Zhou, R.; Chen, H.-M.; Lu, Y. Mesoscale modelling of concrete under high strain rate tension with a rate-dependent cohesive interface approach. Int. J. Impact Eng. 2020, 139, 103500. [Google Scholar] [CrossRef]
- Erzar, B.; Forquin, P. Analysis and modelling of the cohesion strength of concrete at high strain-rates. Int. J. Solids Struct. 2014, 51, 2559–2574. [Google Scholar] [CrossRef]



| Basic Parameters | Strength Surface Parameters | Damage Parameters | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Mortar | ITZ | Aggr. | Mortar | ITZ | Aggr. | Mortar | ITZ | Aggr. | |||
| /kg·m−3 | 2000 | 1800 | 2650 | /MPa | 5.58 | 5.58 | 7.70 | 1.60 | 1.60 | 0.75 | |
| 0.18 | 0.20 | 0.25 | 0.625 | 0.625 | 0.513 | 1.35 | 1.35 | 3.21 | |||
| G/GPa | 10.63 | 8.65 | 20.65 | /×10−9 Pa−1 | 1.03 | 1.03 | 0.77 | 1.15 | 1.15 | 0.50 | |
| /MPa | 21 | 16 | 80 | /MPa | 1.60 | 1.60 | 79 | /mm | 10 | 10 | 1.35 |
| /MPa | 2.57 | 2.0 | 5.20 | 1.35 | 1.35 | 0.542 | 100 | 100 | 100 | ||
| 0.5 | /×10−9 Pa−1 | 1.15 | 1.15 | 0.15 | 2.60 | 2.60 | 2.60 | ||||
| /×10−3 | 8.7 | 8.7 | /Pa | 0 | 0 | 0 | 0.283 | 0.283 | 0.283 | ||
| 0.90 | 0.4417 | 0.4417 | 0.47 | 1.74 | 1.74 | 1.74 | |||||
| /×10−9 Pa−1 | 4.732 | 4.732 | 0.20 | ||||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, C.; Zhou, X.; Deng, Z.; Wang, X.; Zhang, S.; Wang, G.; Wei, P. Discussion on the Gradation and Interface Effects on the Dynamic Mechanical Behaviors of Hydraulic Concrete Based on Meso-Mechanical Simulation. Materials 2025, 18, 15. https://doi.org/10.3390/ma18010015
Wang C, Zhou X, Deng Z, Wang X, Zhang S, Wang G, Wei P. Discussion on the Gradation and Interface Effects on the Dynamic Mechanical Behaviors of Hydraulic Concrete Based on Meso-Mechanical Simulation. Materials. 2025; 18(1):15. https://doi.org/10.3390/ma18010015
Chicago/Turabian StyleWang, Chao, Xinyu Zhou, Zhaopeng Deng, Xiaohua Wang, Sherong Zhang, Gaohui Wang, and Peiyong Wei. 2025. "Discussion on the Gradation and Interface Effects on the Dynamic Mechanical Behaviors of Hydraulic Concrete Based on Meso-Mechanical Simulation" Materials 18, no. 1: 15. https://doi.org/10.3390/ma18010015
APA StyleWang, C., Zhou, X., Deng, Z., Wang, X., Zhang, S., Wang, G., & Wei, P. (2025). Discussion on the Gradation and Interface Effects on the Dynamic Mechanical Behaviors of Hydraulic Concrete Based on Meso-Mechanical Simulation. Materials, 18(1), 15. https://doi.org/10.3390/ma18010015








